Directionally Oriented Reinforcements of Warp-Knitted Fabrics for Composite Preforms
Abstract
:1. Introduction

2. Research Material
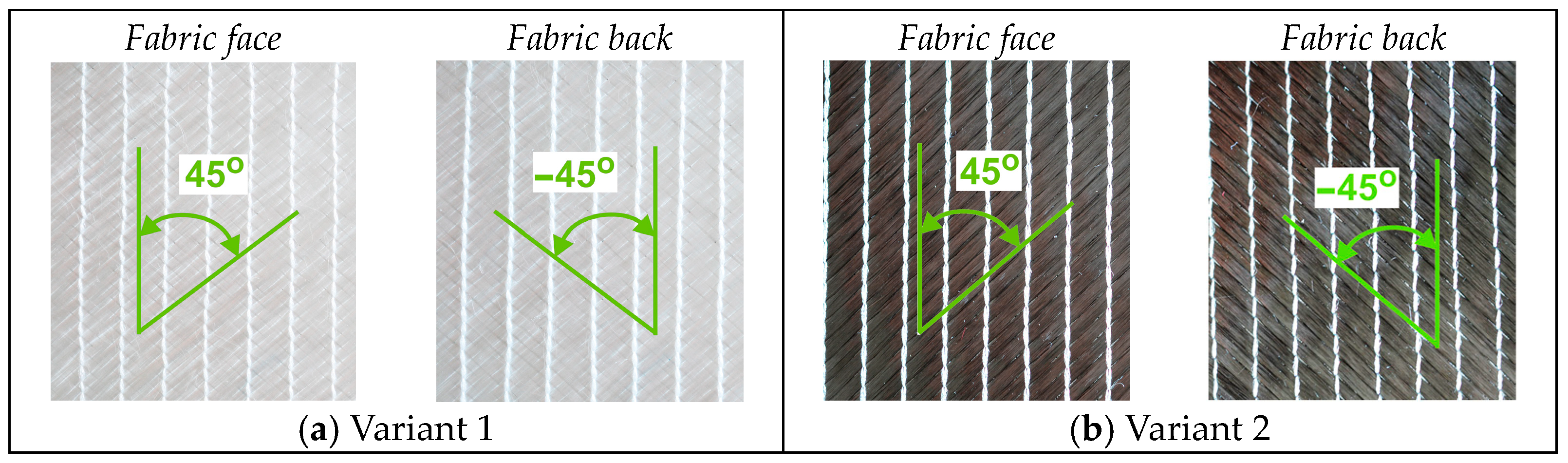
2.1. Fabric Variants under Study
2.2. Structural Modification of Biaxial DOS Fabrics
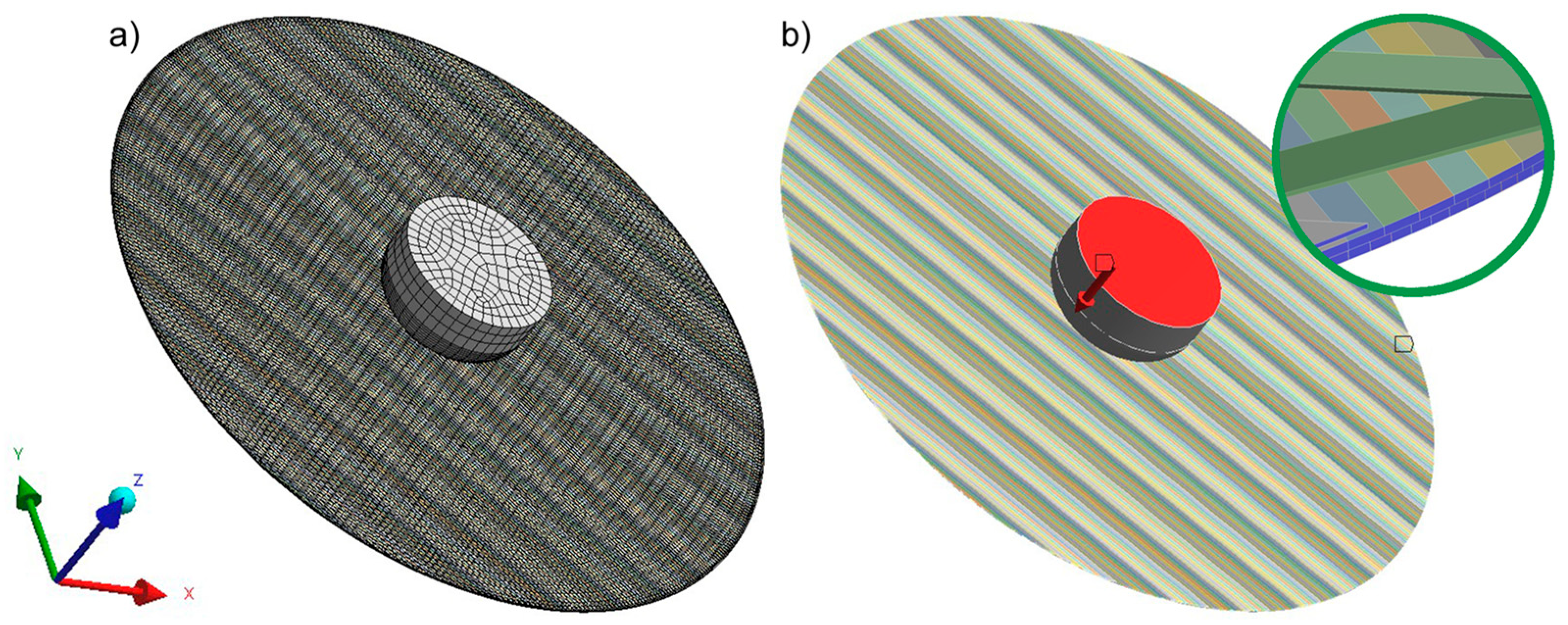
3. Numerical Modeling of Mechanical Properties of Multiaxial DOS Knitted Fabrics
4. Experimental Verification of the Mechanical Properties of Multiaxial DOS Knitted Fabrics
5. Conclusions
- Warp-knitted technical DOS fabrics are an increasingly popular group of textiles used for composite reinforcements. Depending on the intended application of the reinforcement, different mechanical characteristics of the textile system are required.
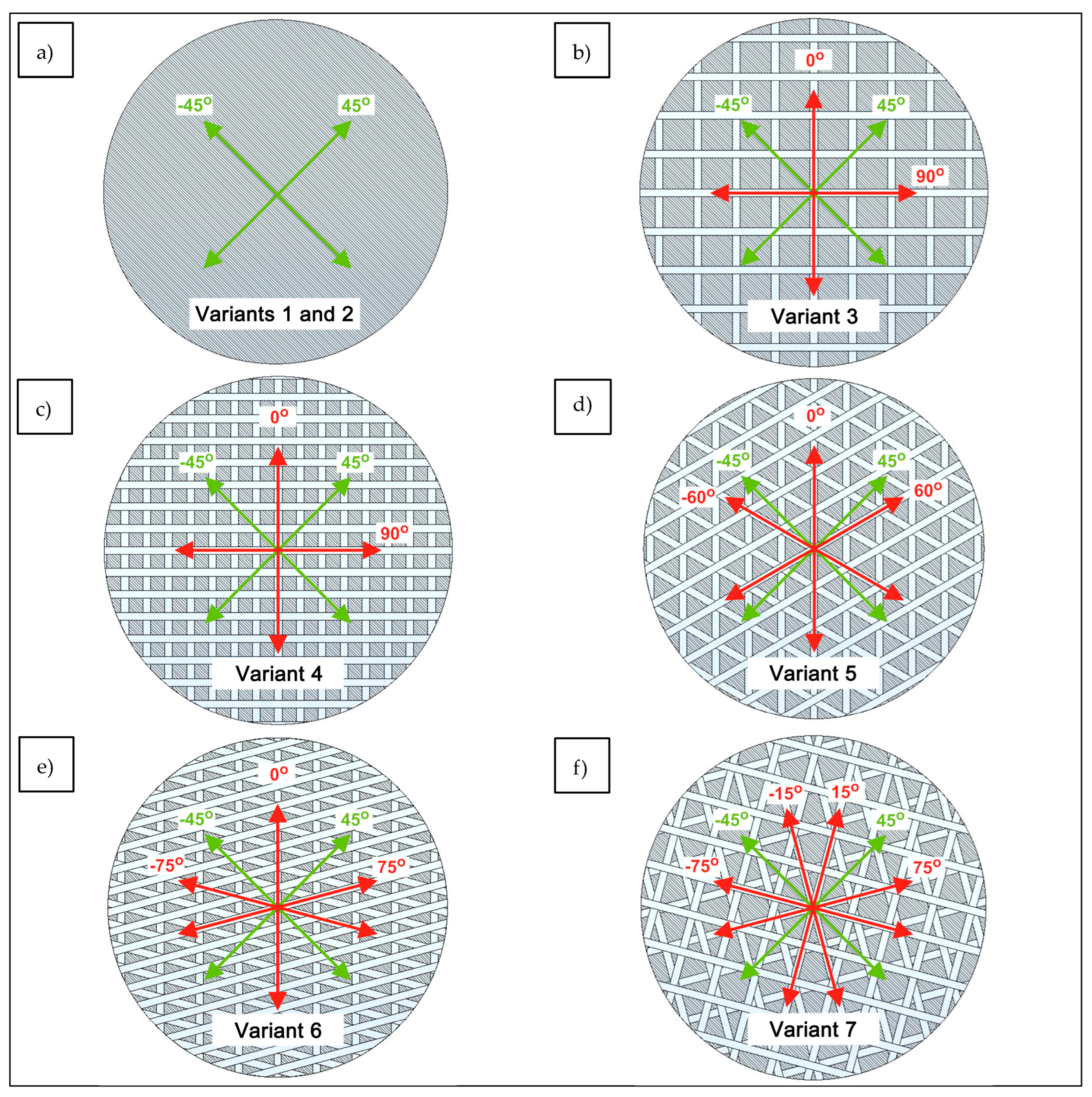
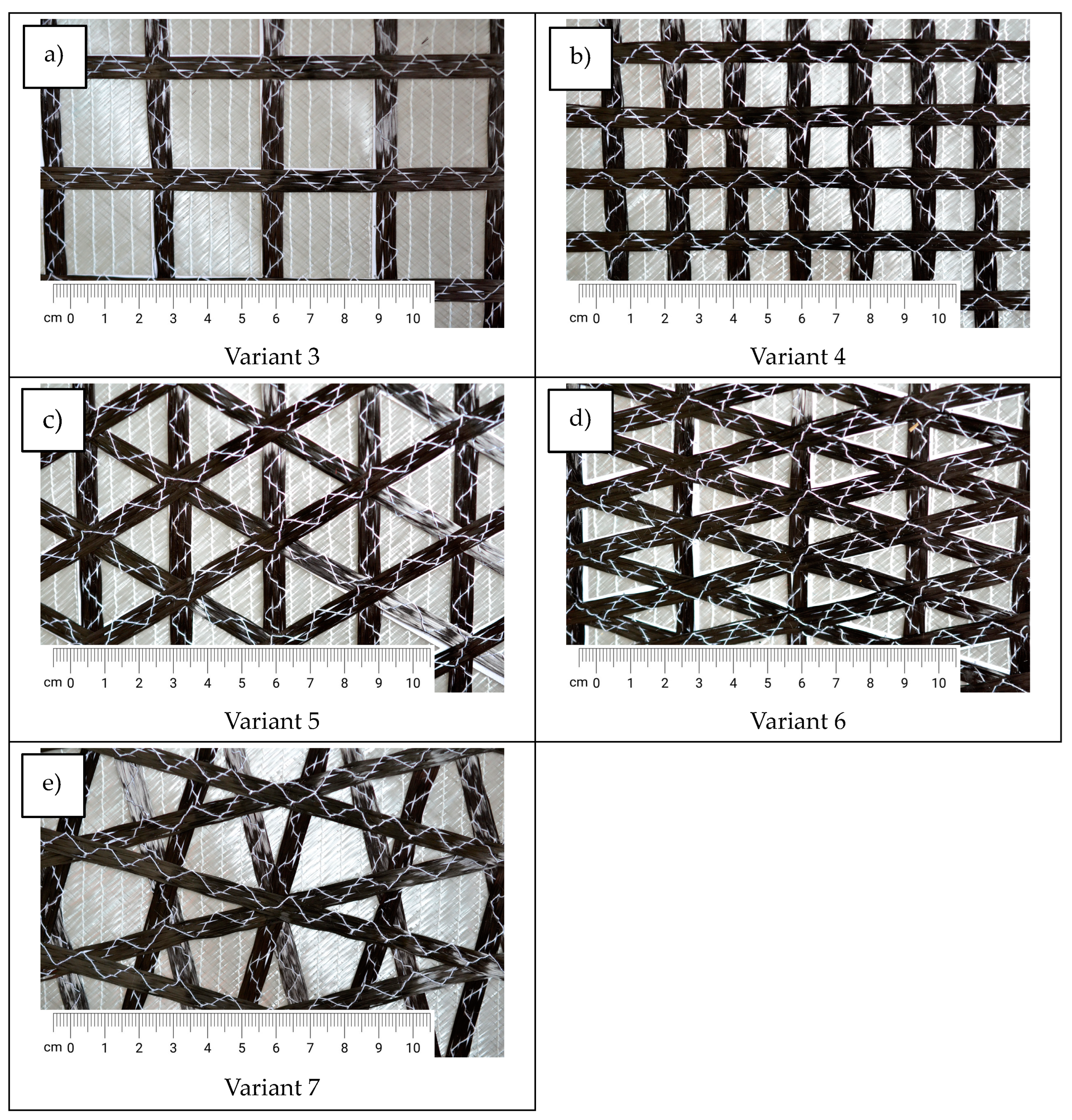
- This work presents the concept of the structural modification of knitted biaxial structures by reinforcing them with carbon tape. In total, five configurations of the reinforcement were designed, including four-axial structures with variable reinforcement densities, five-axial structures with axes of varying angles with respect to the wales in the fabric, and a six-axial structure with a fixed angle between the axes.
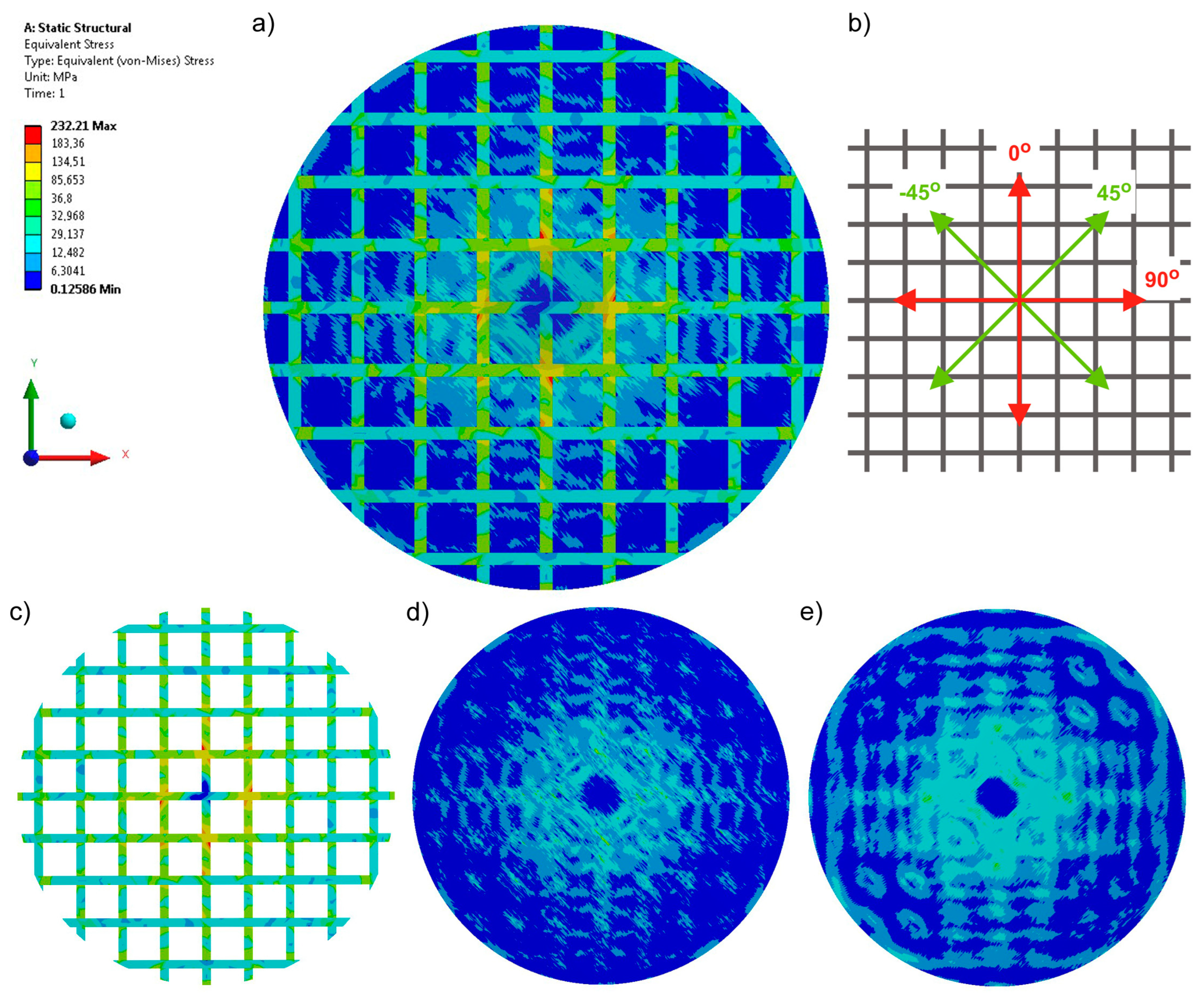
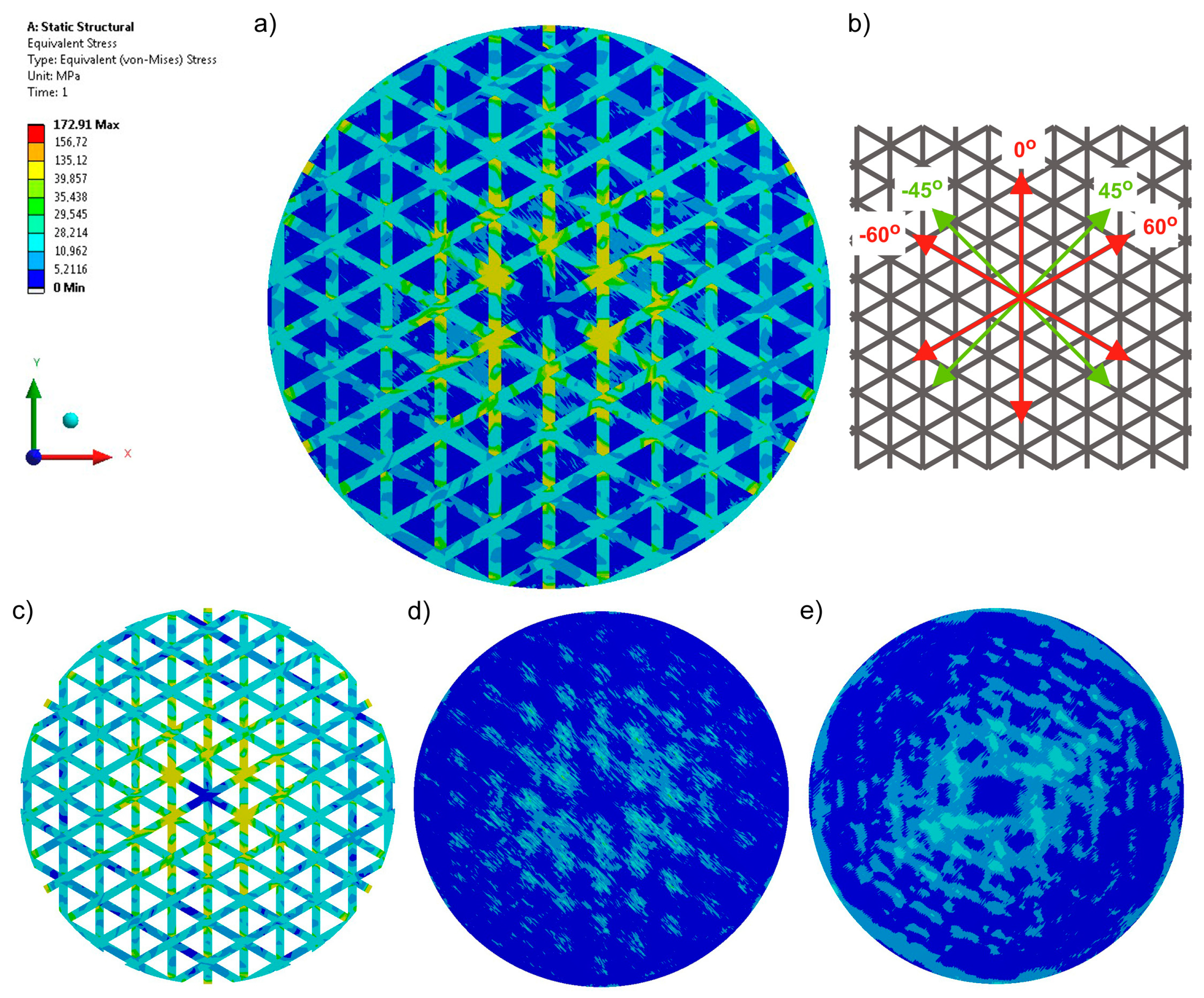
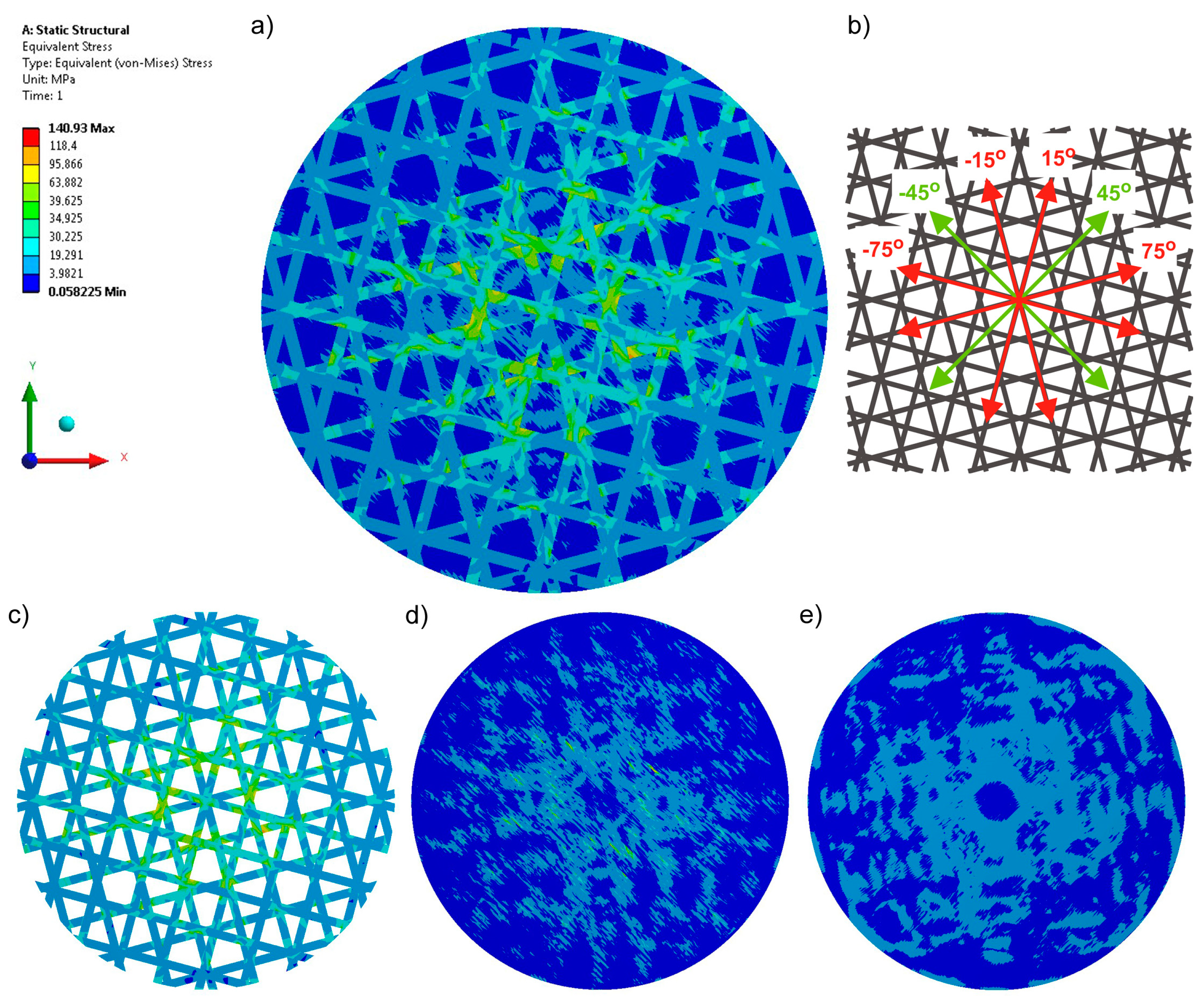
- The finite element simulation confirmed that introducing a reinforcement in the form of a tape made of carbon fibers changes the stress distribution of the modeled samples because part of the stress is taken on by the reinforcements.
- Concerning the initial variants of DOS warp-knitted fabrics with a biaxial structure made of glass yarns (Variant 1) and carbon yarns (Variant 2), Variant 2 exhibited 17% lower maximum equivalent stress values than Variant 1.
- Creating a four-axial structure by strengthening it with carbon tape in the 0° and 90° directions (Variant 3) reduced the maximum equivalent stress by approximately 75% in relation to Variant 1 and by 57% in relation to Variant 2.
- For Variant 5, a five-axis system with additional carbon tape reinforcement in the 0° and ±60° directions, the maximum equivalent stress was reduced by 72% compared to Variants 1 and 2 and by 25% compared to Variant 3.
- In the six-axis structure, the maximum equivalent stress was reduced by 77% for Variants 1 and 2, by 39% for Variant 3, and by 18% for Variant 5.
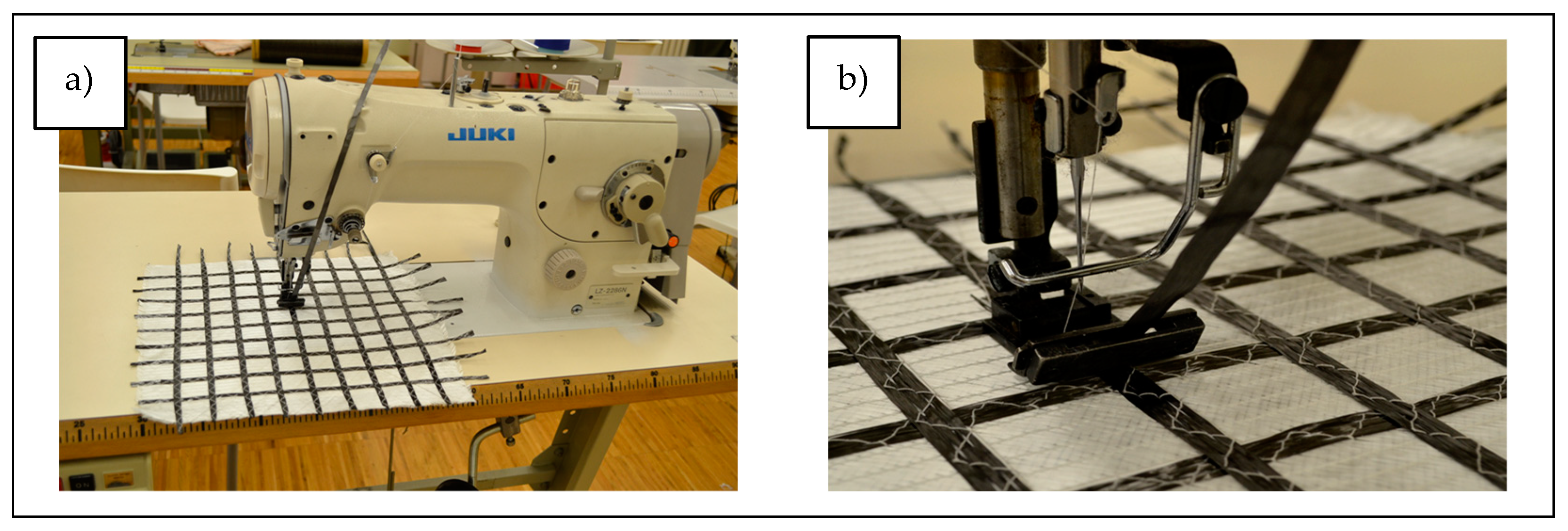
- To verify the model’s results, experimental research was carried out on seven DOS knitted fabrics. With the use of a lockstitch sewing machine, five knitted fabric variants were produced by sewing on a 6 mm wide carbon tape using a zig–zag stitch, according to the designed geometrical systems. The obtained knitted fabric variants were subjected to tests based on the original method of determining multiaxial stress distribution. Analysis of the obtained force distributions in the tested samples yielded the following results:
- For all samples, the distribution of the recorded maximum forces coincided with the direction of the reinforcement. The average recorded maximum forces caused by a load of 20 kg were as follows: in the two-axial structures, 121 N for Variant 1 and 129 N for Variant 2; in the four-axial structures, 87 N for Variant 3 and 75 N for Variant 4; in the five-axial structures, 78 N for Variant 5 and 79 N for Variant 6; and for the six-axial structure, the distribution of the recorded maximum forces on the sample’s surface was the most uniform, with an average value of 66 N. Therefore, the six-axial structure’s average maximum force was about 50% lower than in samples of Variants 1 and 2 and about 18% lower in relation to the remaining variants, i.e., Variants 3–6.
- The analysis of the qualitative distribution of forces on the surface of the tested samples showed that the greatest uniformity was obtained for Variants 3, 4, and 7, where the latter was considered the most favorable in terms of its mechanical parameters, having the lowest recorded force values obtained as a result of the applied loading force. The samples in the two-axial system, i.e., Variants 1 and 2, were isotropic, while Variants 5 and 6 in the three-axial system were anisotropic.
- In sum, the number of axes reinforcing the structure, their density, the angles between additional reinforcement and the wales, and the angles between individual axes have significant impacts on the mechanical properties of the samples.
- The research presented in this work is a continuation of theoretical considerations on the oriented multiaxial reinforcing of warp-knitted fabrics using various methods, including additive techniques, sewing, and embroidery, as well as welding, sticking, and laminating technologies, which can be applied in the design and production of flat and spatial composite preforms.
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Dobrzański, L. Materiały inżynierskie i projektowanie materiałowe: Podstawy nauki o materiałach i metałoznawstwo [Engineering Materials and Material Design: Basics of Materials Science and Metal Science], 2nd ed.; WNT: Warsaw, Poland, 2006; p. 1596. [Google Scholar]
- Iyer, C. Directionally-oriented inlay warp knits—Some aspects of production and application. Indian J. Fibre Text. Res. 1994, 19, 195–202. [Google Scholar]
- Hu, J. 3-D Fibrous Assemblies: Properties, Applications and Modelling of Three-Dimensional Textile Structures, 1st ed.; Woodhead Publishing: Cambridge, UK, 2008; p. 260. [Google Scholar]
- John, V.B. Introduction to Engineering Materials, 2nd ed.; Palgrave Macmillan: London, UK, 2003; p. 640. [Google Scholar]
- Ogale, V.; Alagirusamya, R. Textile preforms for advanced composites. Indian J. Fibre Text. Res. 2004, 29, 366–375. [Google Scholar]
- ElAgamy, N.; Laliberte, J. Historical development of geometrical modelling of textiles reinforcements for polymer composites: A review. J. Ind. Text. 2016, 45, 556–584. [Google Scholar] [CrossRef]
- Mayer, K. , The Karl Mayer Guide to Technical Textiles; Karl Mayer Textilmaschinenfabrik GmbH: Obertshausen, Germany, 2000; p. 36. [Google Scholar]
- Brochure|Multiaxial Non-Crimp Fabric (NCF) Reinforcements|May 2020. Available online: https://owenscorning.com/en-us/composites/product/multiaxial-non-crimp-fabrics (accessed on 15 July 2024).
- Spencer, D.J. Knitting Technology—A Comprehensive Handbook and Practical Guide, 3rd ed.; Woodhead Publishing Limited: Cambridge, UK, 2001; pp. 370–379. [Google Scholar]
- Du, G.-W.; Ko, F. Analysis of Multiaxial Warp-Knit Preforms for Composite Reinforcement. Compos. Sci. Technol. 1996, 56, 253–260. [Google Scholar] [CrossRef]
- Zănoagă, M.; Tanasă, F. Complex Textile Structures as Reinforcement for Advanced Composite Materials. In Proceedings of the International Conference of Scientific Paper AFASES 2014, Brasov, Romania, 22–24 May 2014. [Google Scholar]
- Bu, Y.; Feng, Y.; Liu, H. The Axial Warp-Knitted Fabric and its Reinforced Composite. In Proceedings of the International Conference on Textile Engineering and Materials, (ICTEM 2011), Tianjin, China, 23–25 September 2011. [Google Scholar]
- Ogin, S.L.; Potluri, P. Textile-reinforced composite materials. In Handbook of Technical Textiles—Technical Textile Applications, 2nd ed.; Horrocks, A.R., Anand, S.C., Eds.; Woodhead Publishing—Elsevier: Cambridge, UK, 2016; Volume 2, pp. 1–26. [Google Scholar]
- Goetzendorf-Grabowska, B.; Karaszewska, A.; Vlasenko, V.I.; Arabuli, A.T. Bending Stiffness of Knitted Fabrics—Comparison of Test Methods. Fibres Text. East. Eur. 2014, 22, 43–50. [Google Scholar]
- Sankaran, V.; Ruder, T.; Rittner, S.; Hufnagl, E.; Cherif, C. A multiaxial warp knitting based yarn path manipulation technology for the production of bionic-inspired multifunctional textile reinforcements in lightweight composites. J. Ind. Text. 2016, 45, 1188–1203. [Google Scholar] [CrossRef]
- Siemiński, P.; Budzik, G. Techniki przyrostowe. Druk 3D. Drukarki 3D [Additive Techniques. 3D Printing. 3D Printers], 1st ed.; OWPW: Warsaw, Poland, 2015; p. 186. [Google Scholar]
- Pieklak, K. Opracowanie technologii hybrydowych tekstyliów wieloosiowych wraz z analizą parametrów strukturalnych i mechanicznych [Developing the technology of multiaxial hybrid textiles with the analysis of structural and mechanical parameters]. In Proceedings of the XVII Konferencja Naukowa Wydziału Technologii Materiałowych i Wzornictwa Tekstyliów 2016 [XVII Scientific Conference of the FMT&TD 2016], Lodz, Poland, 18 March 2016. [Google Scholar]
- Pieklak, K. Hybrydowa technologia kompozytów przestrzennych wzmacnianych dzianinami dystansowymi 3D [Hybrid technology of spatial composites reinforced with 3D spacer fabrics]. In Proceedings of the XVIII Konferencja Naukowa Wydziału Technologii Materiałowych i Wzornictwa Tekstyliów 2017 [XVIII Scientific Conference of the FMT&TD 2017], Lodz, Poland, 10 March 2017. [Google Scholar]
- FramisItalia. Available online: https://www.framis.it (accessed on 28 March 2019).
- Embroidery Route to Preforms. Available online: https://www.insidecomposites.com (accessed on 28 March 2019).
- Gardiner, G. Tailored Fiber Placement: Besting Metal in Volume Production, Affordable Automated Production of Highly Optimized Preforms and Parts. Available online: https://www.compositesworld.com/articles/tailored-fiber-placement-besting-metal-in-volume-production (accessed on 22 January 2024).
- Baker, A.; Dutton, S.; Kelly, D. Composite Materials for Aircraft Structures, 2nd ed.; AIAA: Blacksburg, Virginia, 2004; p. 597. [Google Scholar]
- Techmasz—maszyny do szycia [Techmasz—Sewing Machines]. Available online: https://imaszynydoszycia.pl/pl/stopka-do-wszywania-cekinow-z-tasmy-i-tasiemek-janome-200332000.html (accessed on 18 January 2024).
- Gao, Z.; Ma, P.; Jiang, G.; Miao, X. Tensile properties and meso-scale mechanism of multi-axial warp-knitted fabrics of various structural designs. Indian J. Fibre Text. Res. 2014, 39, 122–129. [Google Scholar]
- Gao, X.; Li, D.; Wu, W.; Chen, S. Experimental investigation of the tensile and bending behavior of multi-axial warp-knitted fabric composites. Text. Res. J. 2018, 88, 333–344. [Google Scholar] [CrossRef]
- Dexter, H.B.; Hasko, G.H. Mechanical Properties and Damage Tolerance of Multiaxial Warp-Knit Composites. Compos. Sci. Technol. 1996, 56, 367–380. [Google Scholar] [CrossRef]
- Campbell, F.C. Manufacturing Technology for Aerospace Structural Materials, 1st ed.; Elsevier Ltd.: Oxford, UK, 2006; p. 540. [Google Scholar]
- Cherif, C. The Textile Process Chain and Classification of Textile Semi-finished Products. In Textile Materials for Lightweight Constructions: Technologies—Methods—Materials—Properties; Springer: Berlin Heidelberg, Germany, 2016; pp. 9–35. [Google Scholar]
- Rose, C. Designing for composites: Traditional and future views. In Green Composites—Polymer Composites and the Environment, 1st ed.; Baillie, C., Ed.; Woodhead Publishing Limited: Cambridge, UK, 2004; pp. 9–22. [Google Scholar]
- Stachowicz, K. Bionics—The implementation of practical goals inspired by nature. Wszechświat 2021, 122, 54–58. [Google Scholar]
- Dickinson, M.H. Bionics: Biological insight into mechanical design. Proc. Natl. Acad. Sci. USA 1999, 96, 14208–14209. [Google Scholar] [CrossRef] [PubMed]
- Teodorescu, M. Applied Biomimetics: A New Fresh Look of Textiles. J. Text. 2014, 2014, 154184. [Google Scholar] [CrossRef]
- Naderinejad, M.; Junge, K.; Hughes, J. Exploration of the Design of Spiderweb-Inspired Structures for Vibration-Driven Sensing. Biomimetics 2023, 8, 111. [Google Scholar] [CrossRef] [PubMed]
- Stegmaier, T.; Von Arnim, V.; Linke, M.; Milwich, M.; Sarsour, J.; Scherrieble, A.; Schneider, P.; Planck, H. Bionic developments based on textile materials for technical application. In Biologically Inspired Textiles; Abbot, A., Ellison, M., Eds.; Woodhead Publishing Limited: Cambridge, UK, 2008; pp. 193–211. [Google Scholar]
- Vassiliadis, S.; Prekas, P.; Katsoulis, A.; Vossou, K.; Gouvas, P.; Marmarali, A.; Blaga, M. Multiaxial Tensile Testing of Textile Fabrics. In Proceedings of the 47th IFKT Congress, Izmir, Turkey, 25–26 September 2014. [Google Scholar]
- Luo, Y.; Hu, H. Mechanical properties of PVC coated bi-axial warp knitted fabric with and without initial cracks under multi-axial tensile loads. Compos. Struct. 2009, 89, 536–542. [Google Scholar] [CrossRef]
- Frontczak-Wasiak, I.; Snycerski, M.; Stempień, Z.; Suszek, H. Measuring Method of Multidirectional Force Distribution in a Woven Fabric. Fibres Text. East. Eur. 2004, 12, 48–51. [Google Scholar]
- Bino, D.; Stanly Jones, B.; Ramachandran, M.; Sivapragash, M. Analysis of mechanical properties of glass and carbon fiber reinforced polymer material. Int. J. Appl. Eng. Res. 2015, 10, 10387–10391. [Google Scholar]
- High Strength Glass Fibers. Available online: https://www.agy.com/wp-content/uploads/2014/03/High_Strength_Glass_Fibers-Technical.pdf (accessed on 5 January 2024).

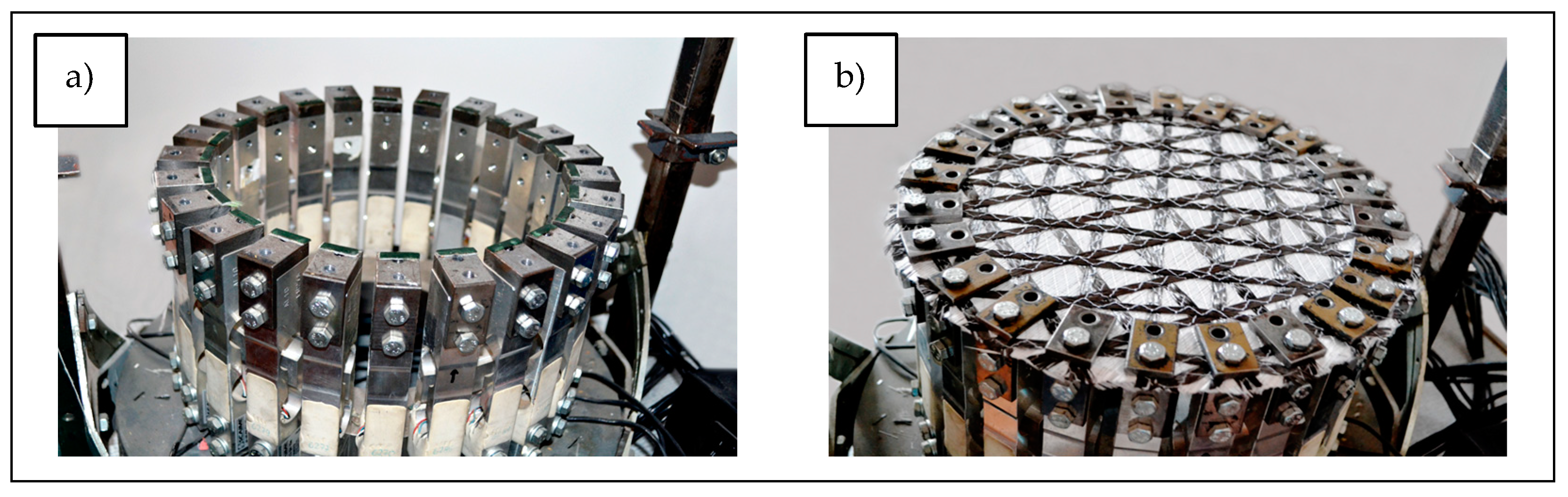
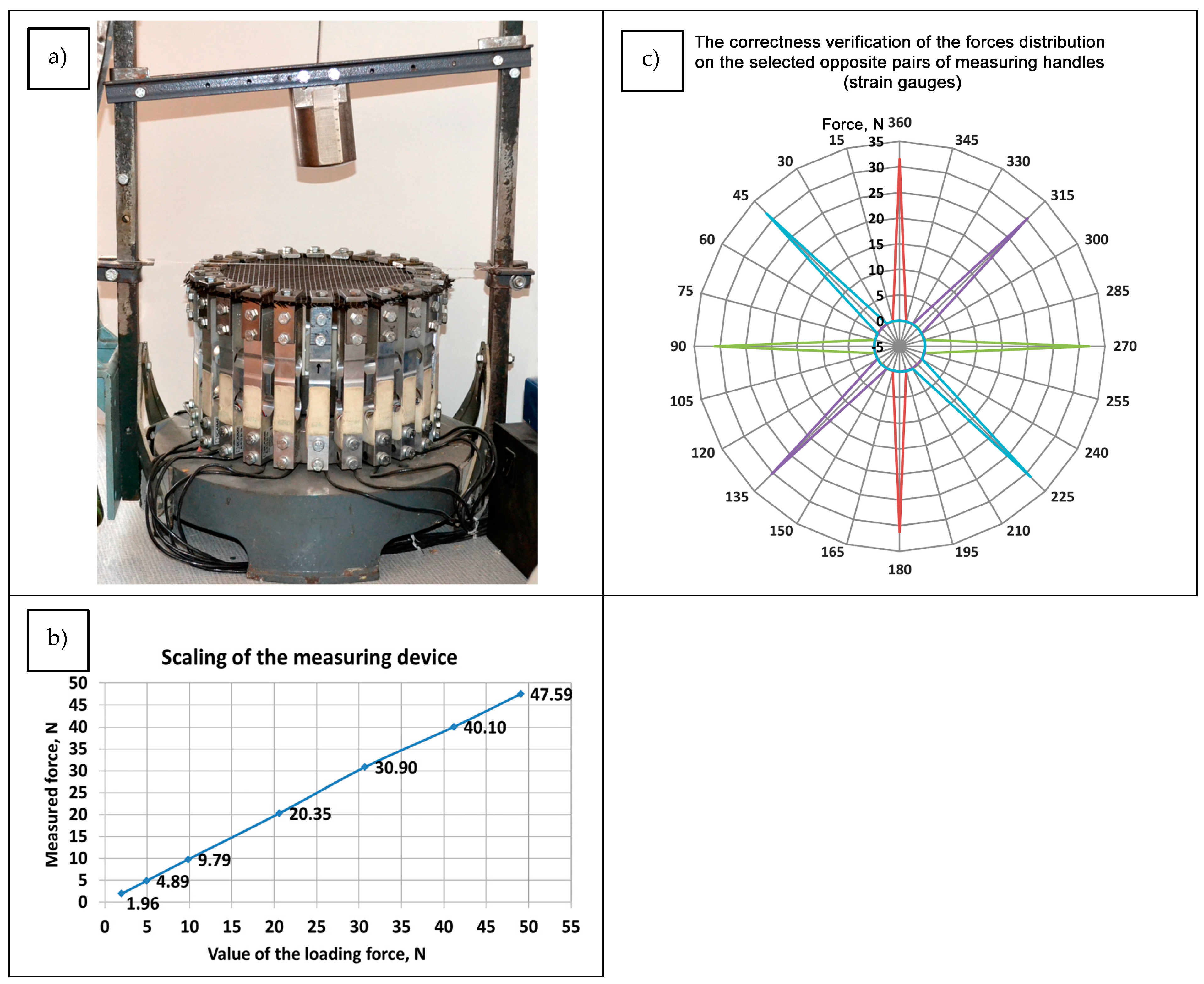
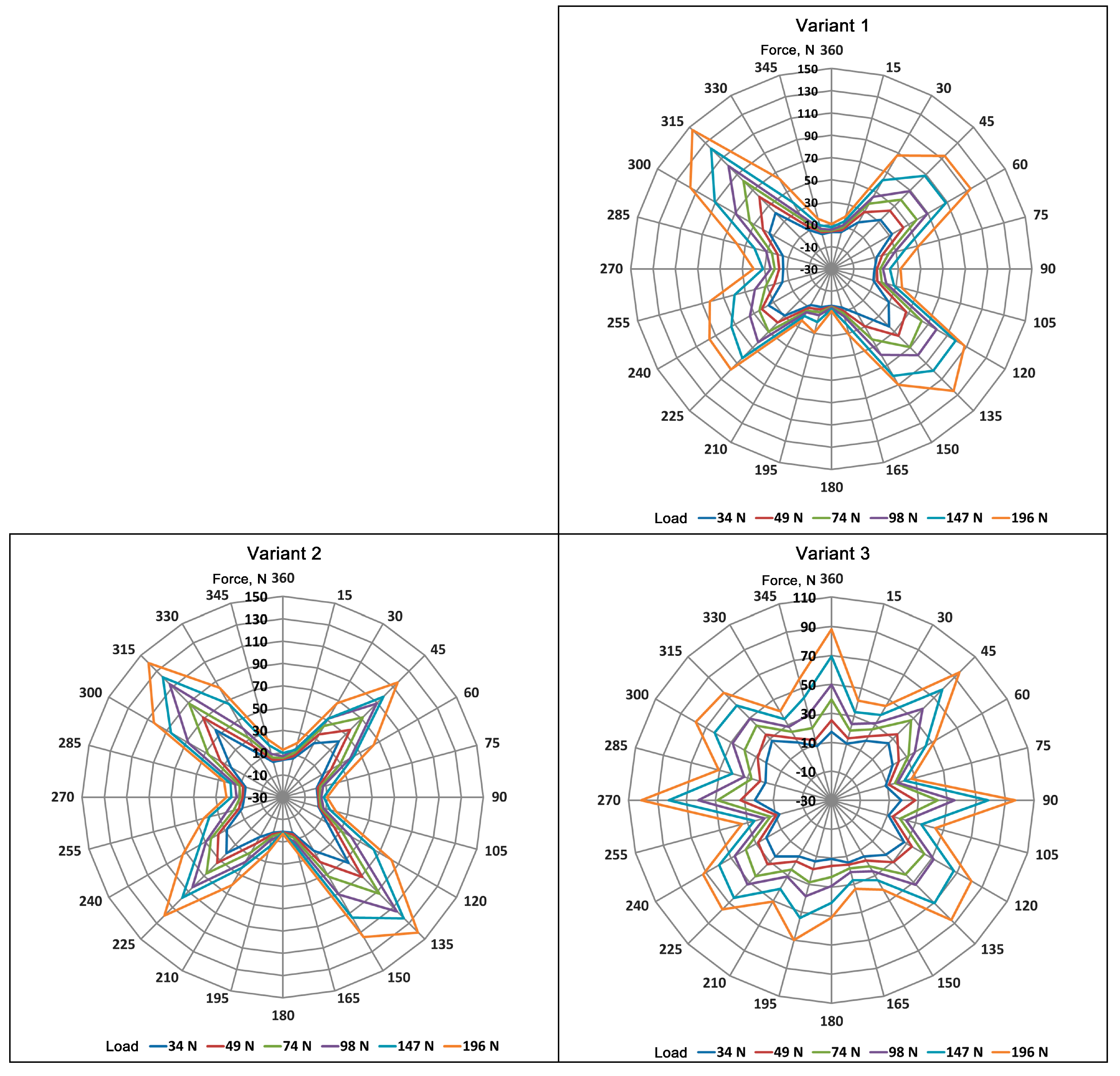
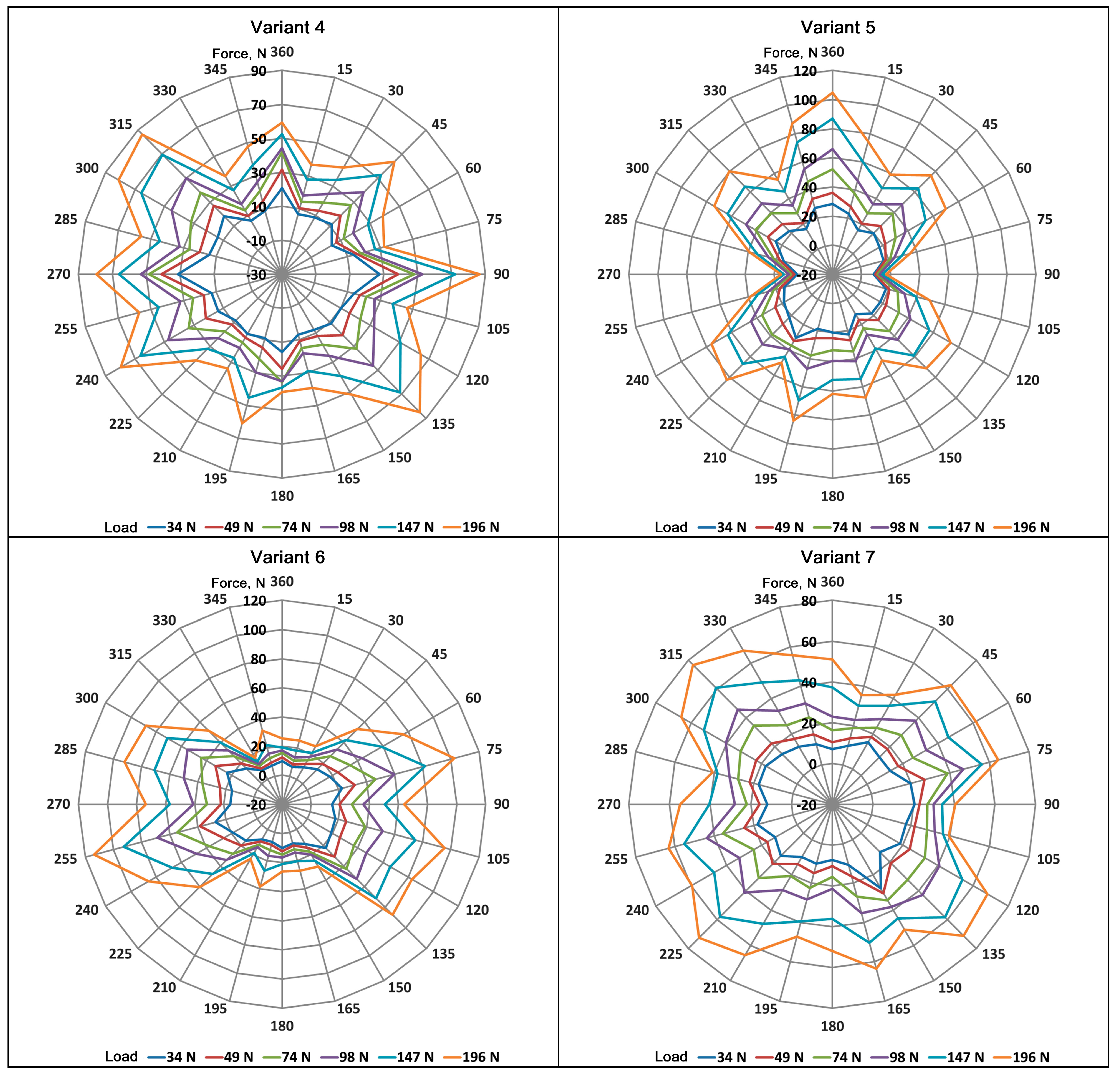
| Variant | 1 | 2 | 3 | 4 | 5 | 6 | 7 |
|---|---|---|---|---|---|---|---|
| Surface density (g/m2) | 400 | 400 | 490 | 552 | 553 | 606 | 556 |
| Thickness (mm) | 0.64 | 0.67 | 1.05 | 1.12 | 1.23 | 1.23 | 1.20 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pieklak, K. Directionally Oriented Reinforcements of Warp-Knitted Fabrics for Composite Preforms. Materials 2024, 17, 5221. https://doi.org/10.3390/ma17215221
Pieklak K. Directionally Oriented Reinforcements of Warp-Knitted Fabrics for Composite Preforms. Materials. 2024; 17(21):5221. https://doi.org/10.3390/ma17215221
Chicago/Turabian StylePieklak, Katarzyna. 2024. "Directionally Oriented Reinforcements of Warp-Knitted Fabrics for Composite Preforms" Materials 17, no. 21: 5221. https://doi.org/10.3390/ma17215221
APA StylePieklak, K. (2024). Directionally Oriented Reinforcements of Warp-Knitted Fabrics for Composite Preforms. Materials, 17(21), 5221. https://doi.org/10.3390/ma17215221






