Experimental Study on Thermal Decomposition Temperature and Thermal Expansion Coefficient of Typical Nonmetallic Materials in Aeroengine Components
Abstract
1. Introduction
2. Experimental Process
2.1. Object of Study
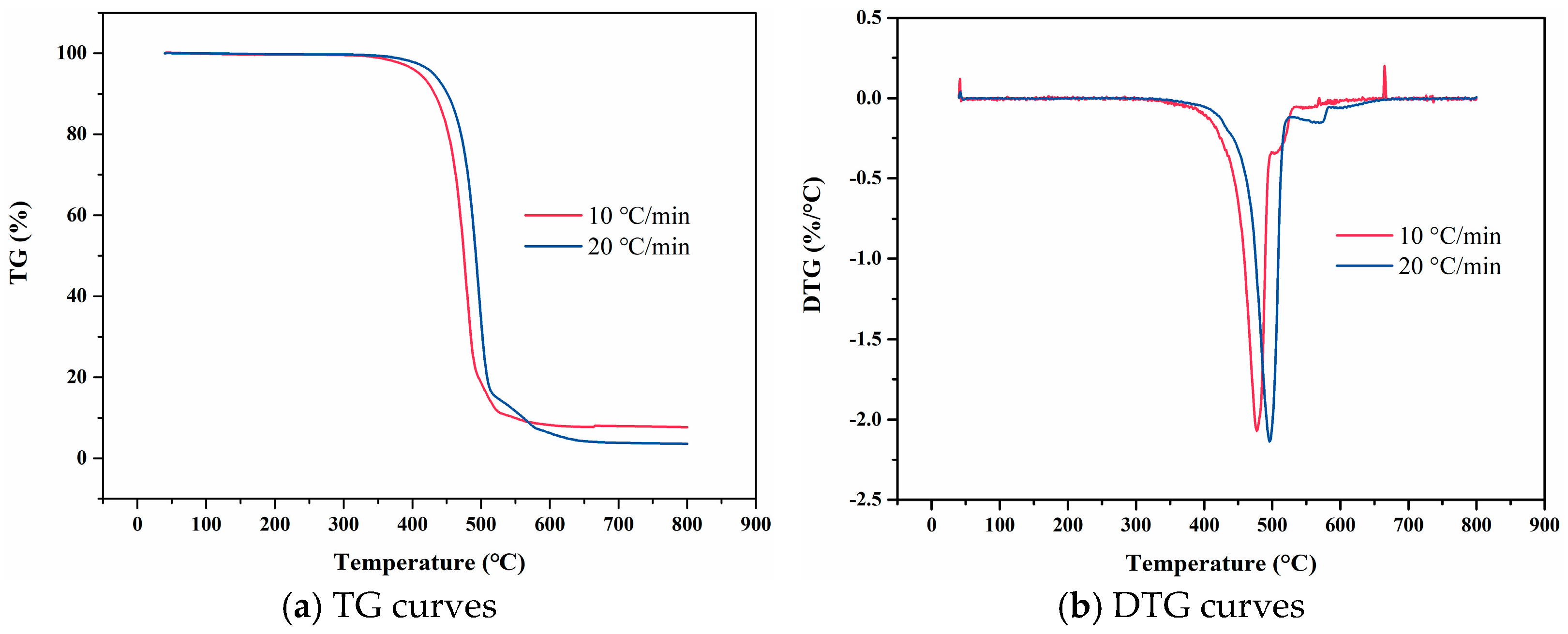
2.2. Thermal Decomposition Temperature
2.2.1. Basic Principle of Experiment
2.2.2. Experimental Steps
2.3. Linear Thermal Expansion Coefficient
2.3.1. Basic Principle of Experiment
2.3.2. Experimental Steps
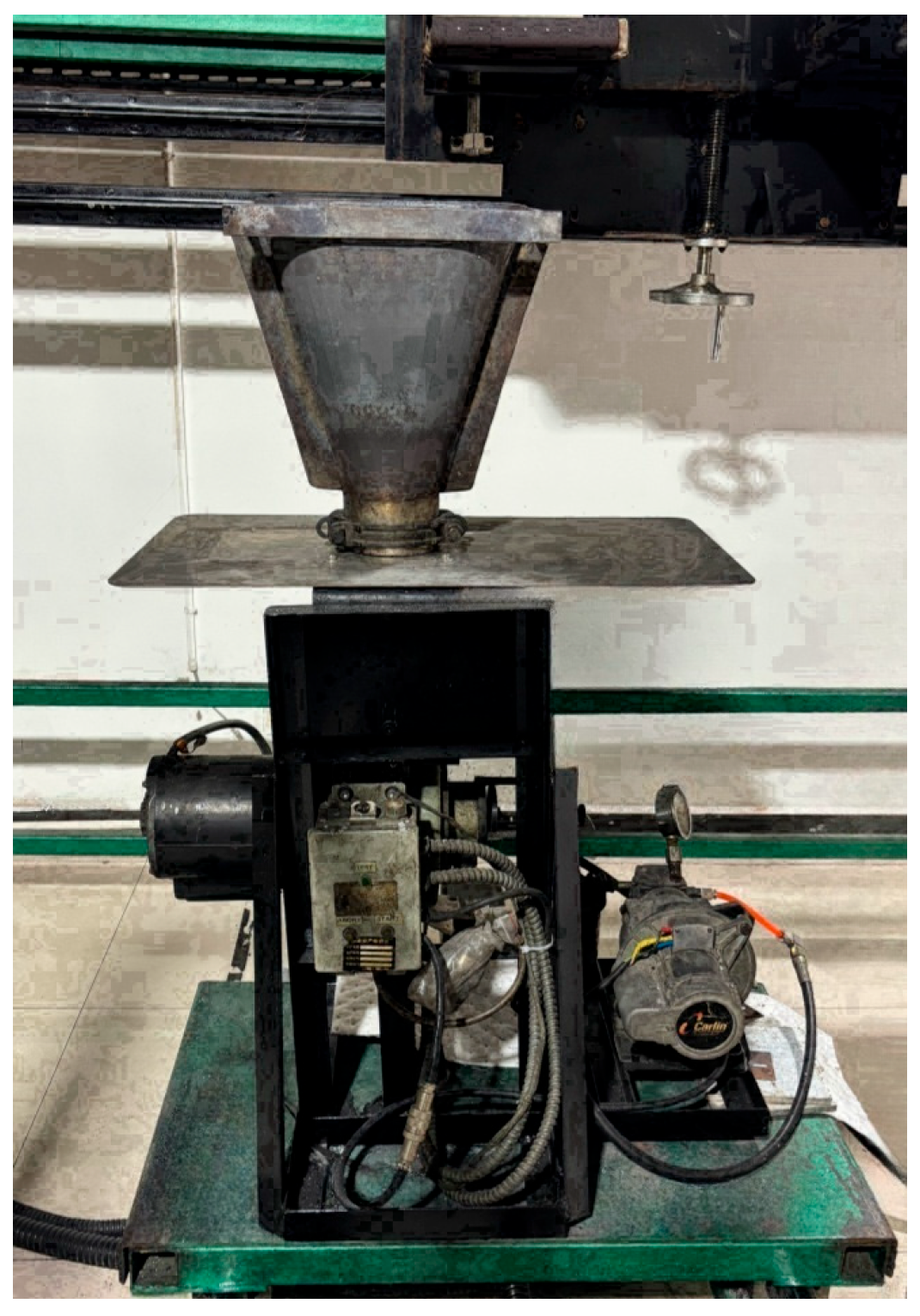
2.4. Material Fireproof Testing
2.4.1. Experimental Equipment
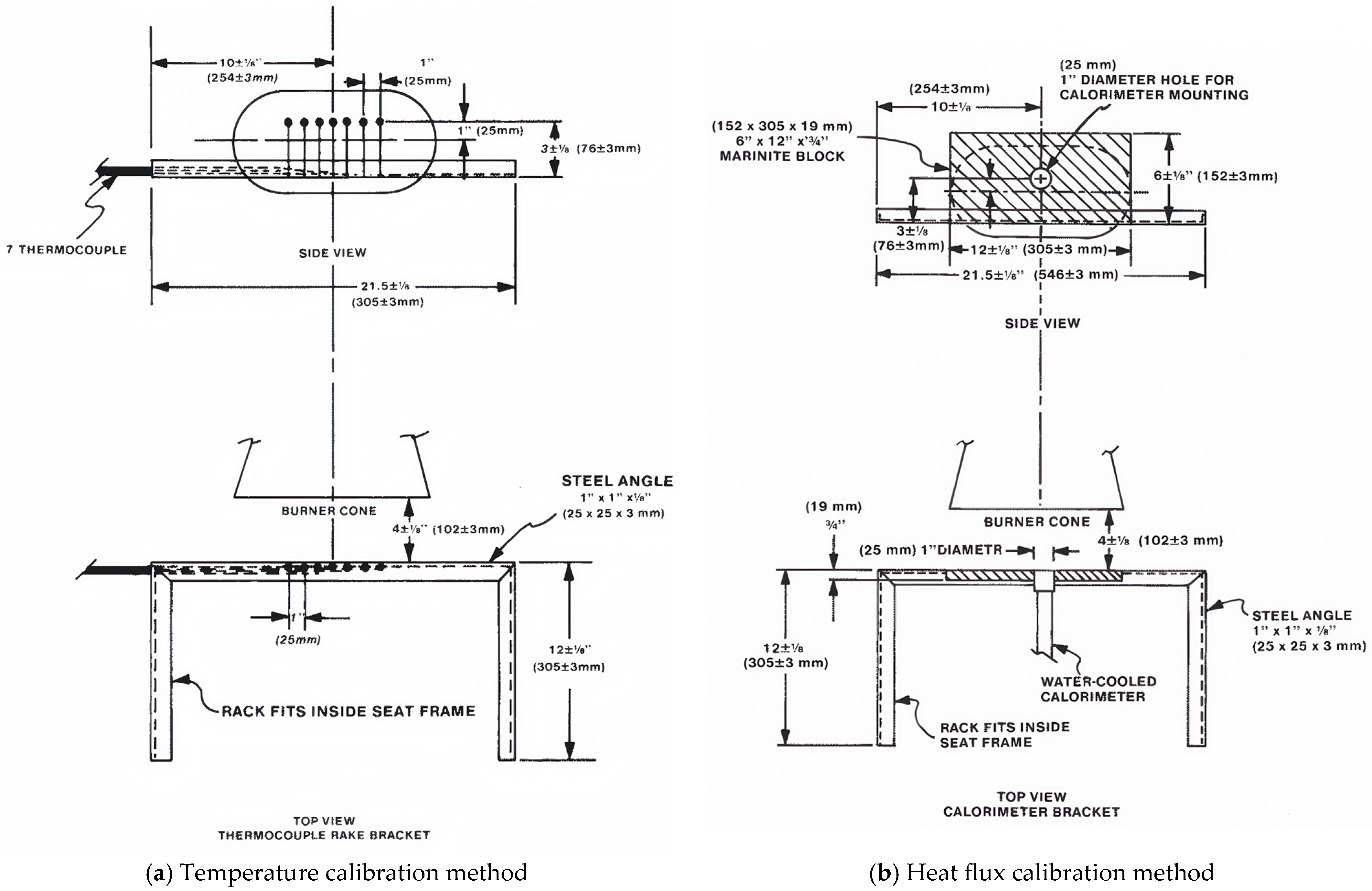
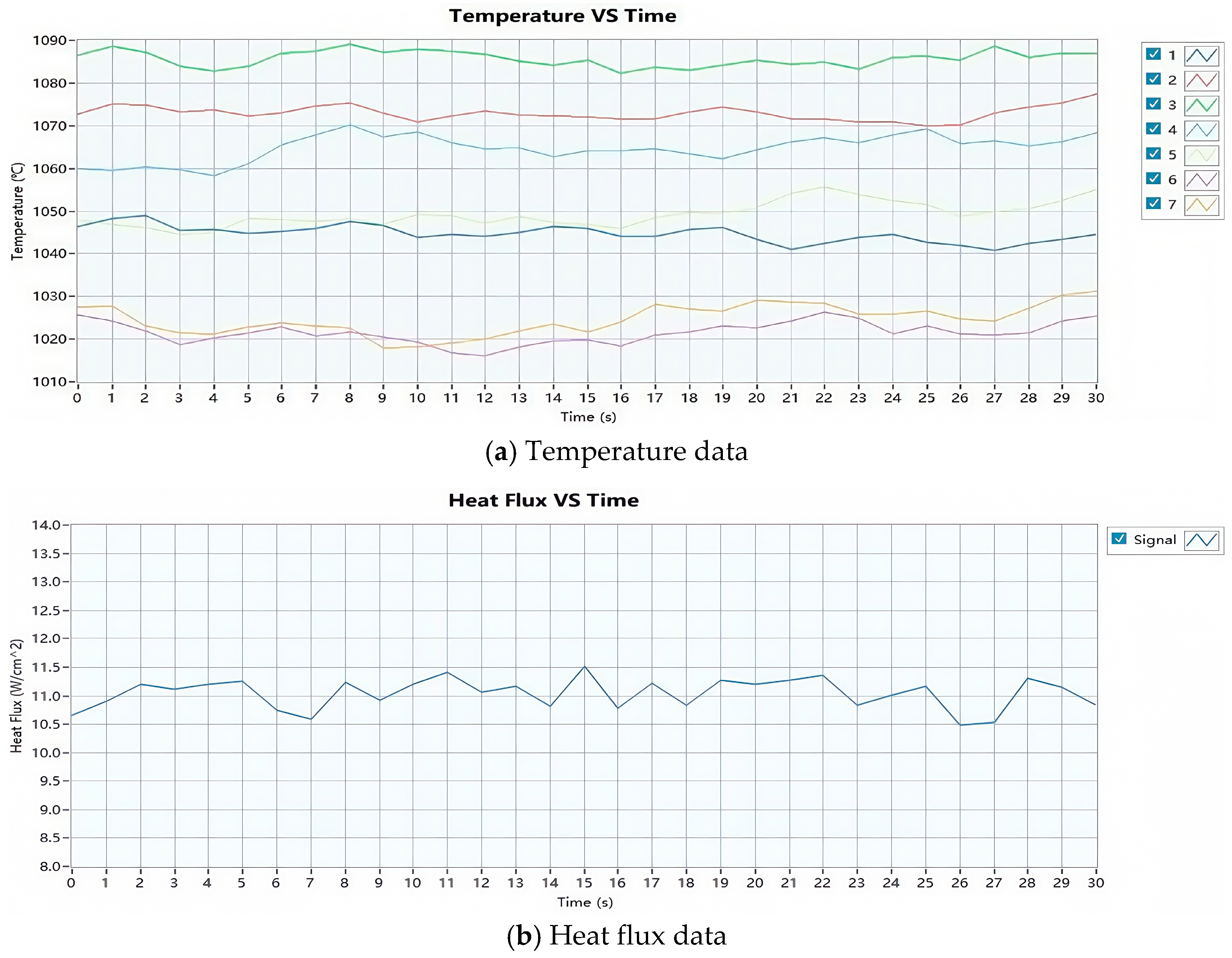
2.4.2. Flame Calibration
3. Uncertainty Analysis
3.1. Uncertainty Analysis of Mass Loss and Pyrolysis Temperature
3.1.1. TG
- m(T): the mass of the sample at temperature T;
- m0: initial mass.
- a: Weighing Accuracy;
- b: Weighing Precision;
- c: Repeatability;
- d: Blank Curve Reproducibility.
- e: Temperature Accuracy;
- f: Temperature Precision.
3.1.2. DTG
3.2. Uncertainty Analysis of the Coefficient of Linear Thermal Expansion
3.2.1. Mean Coefficient of Linear Thermal Expansion
3.2.2. Differential Coefficient of Linear Thermal Expansion
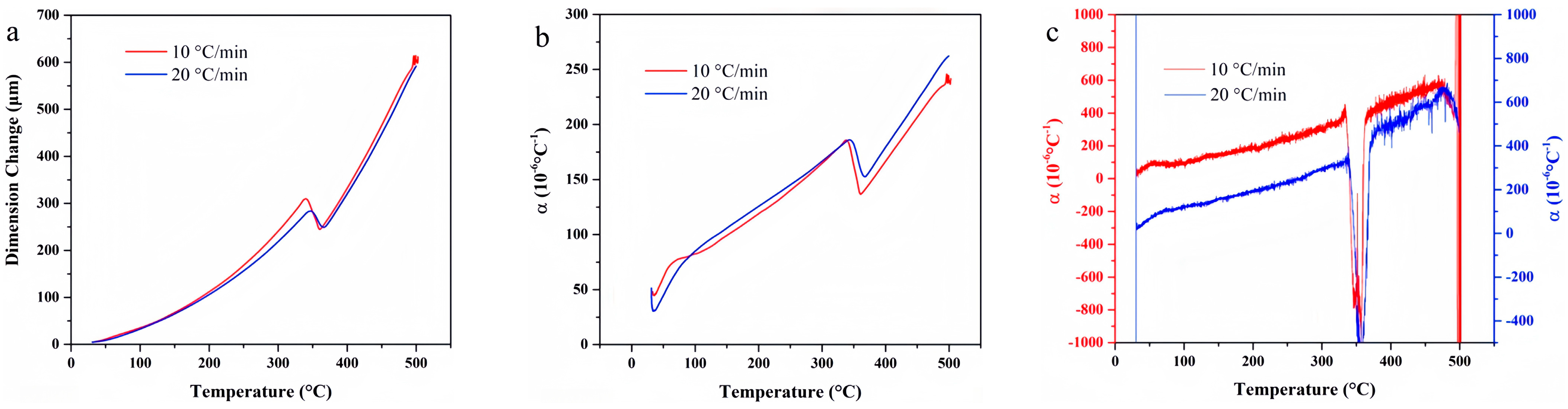
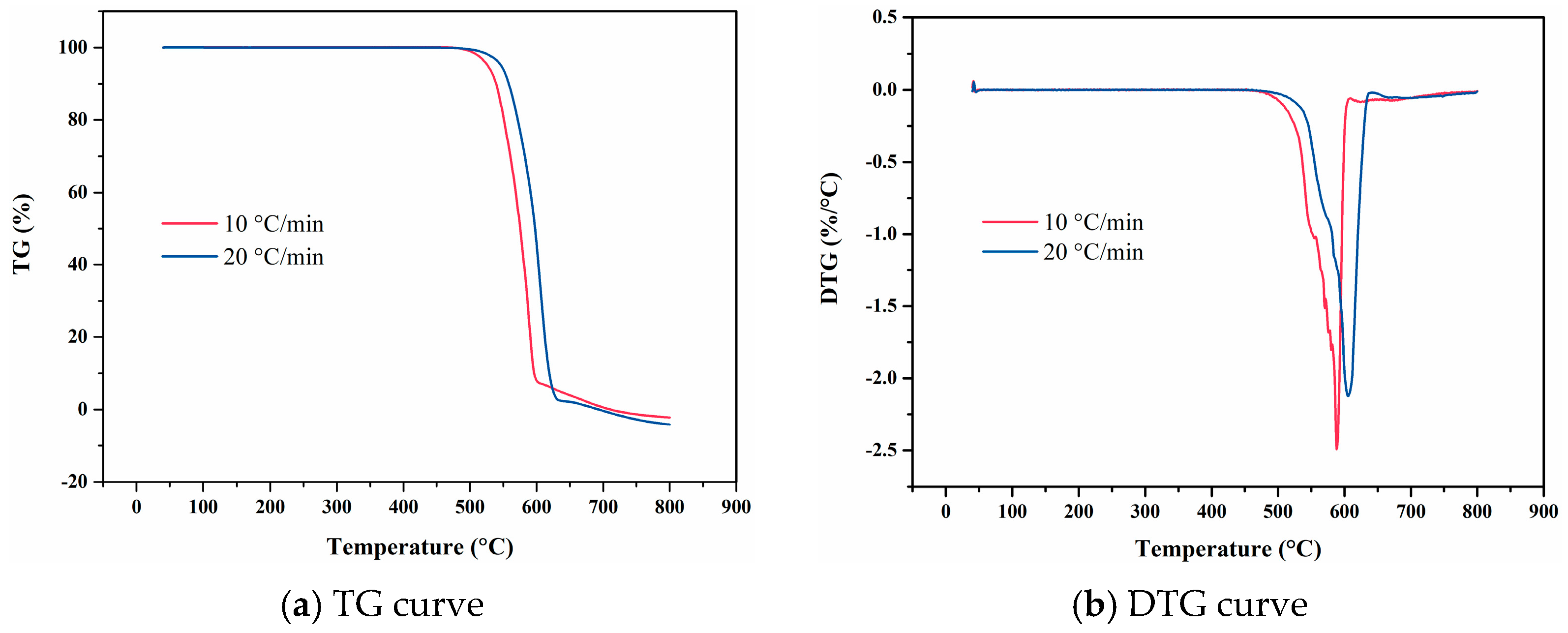
4. Experimental Results
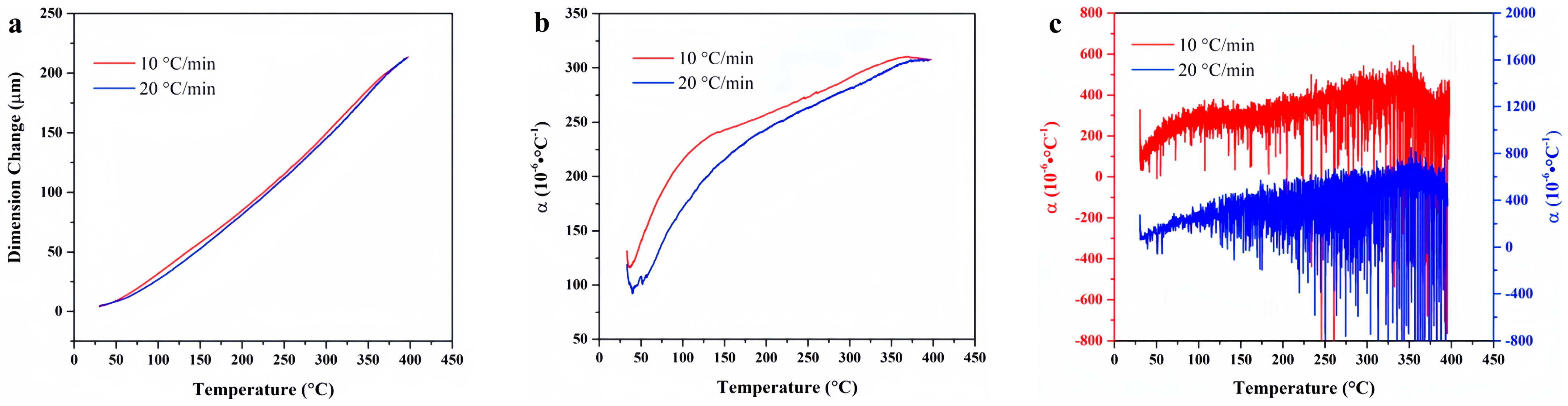
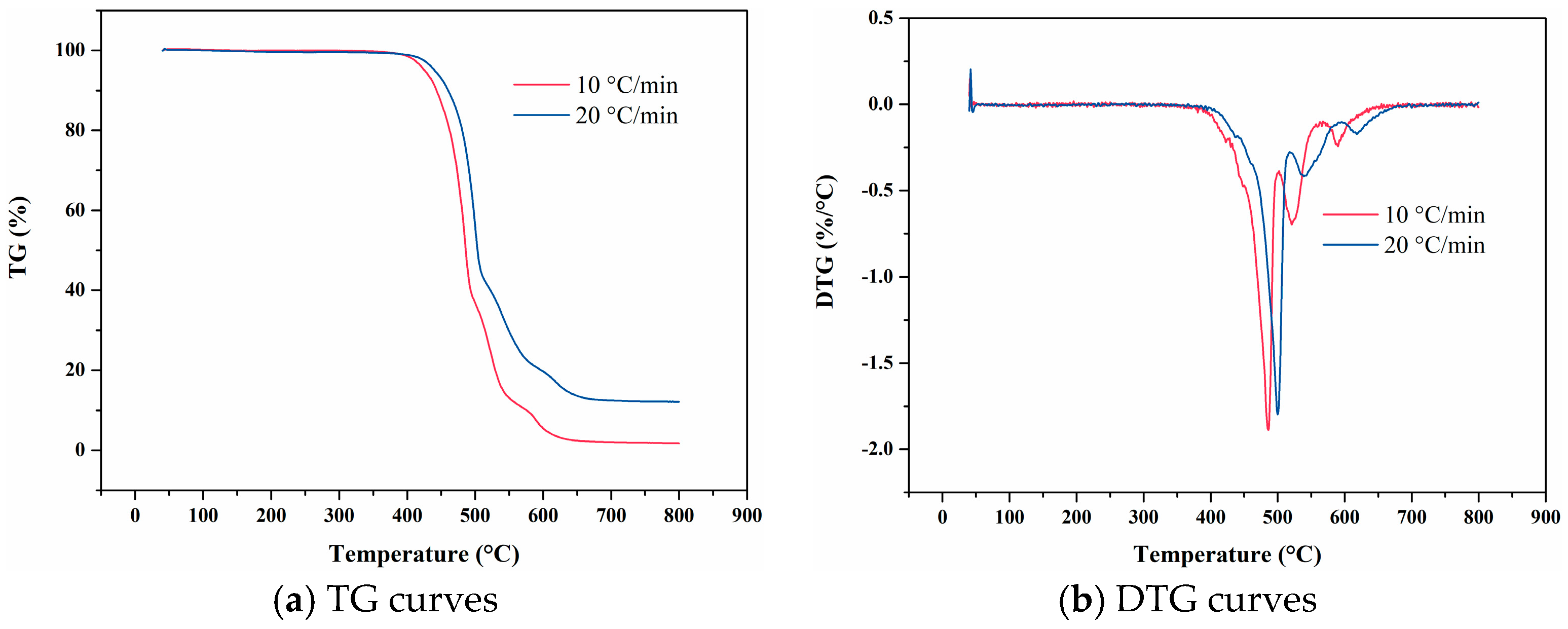
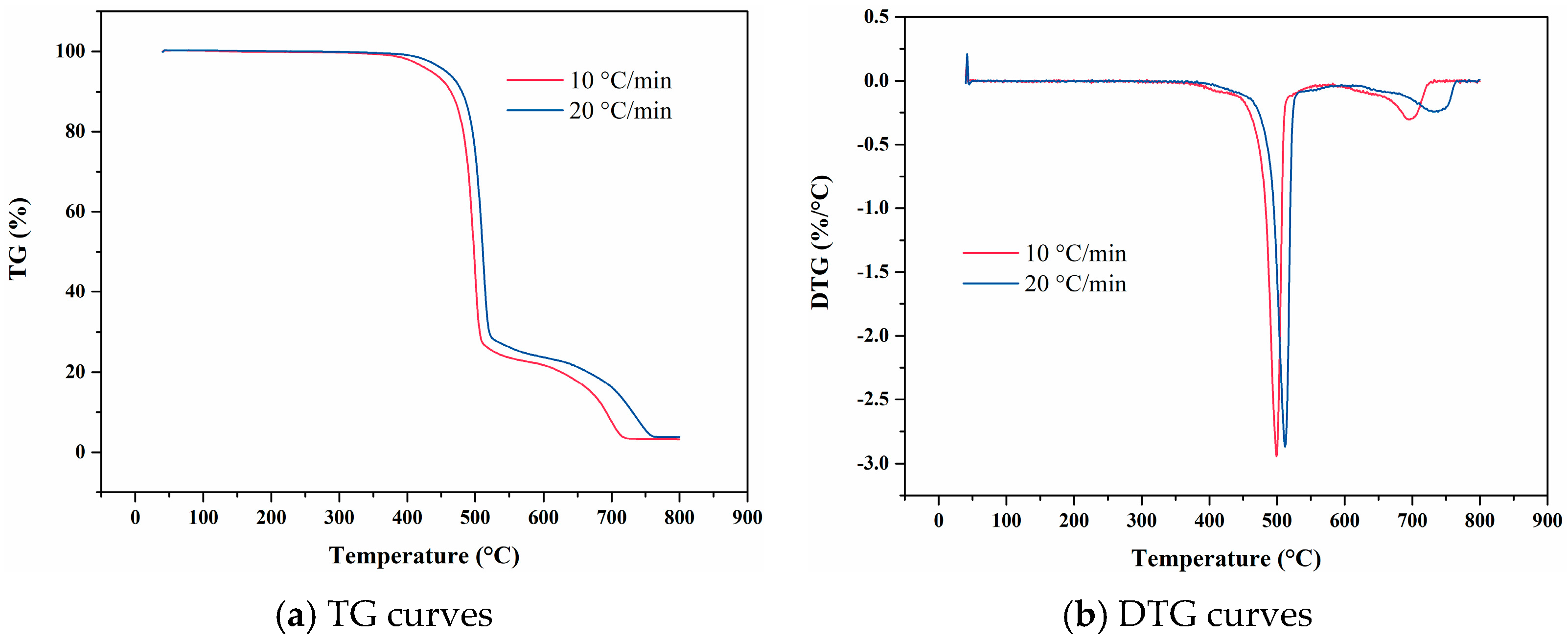
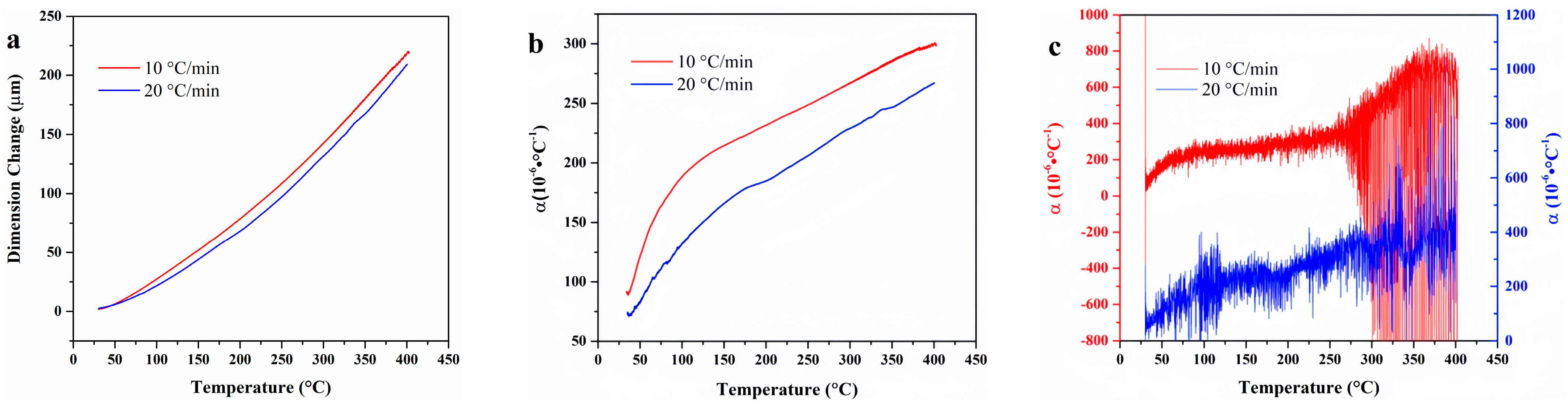
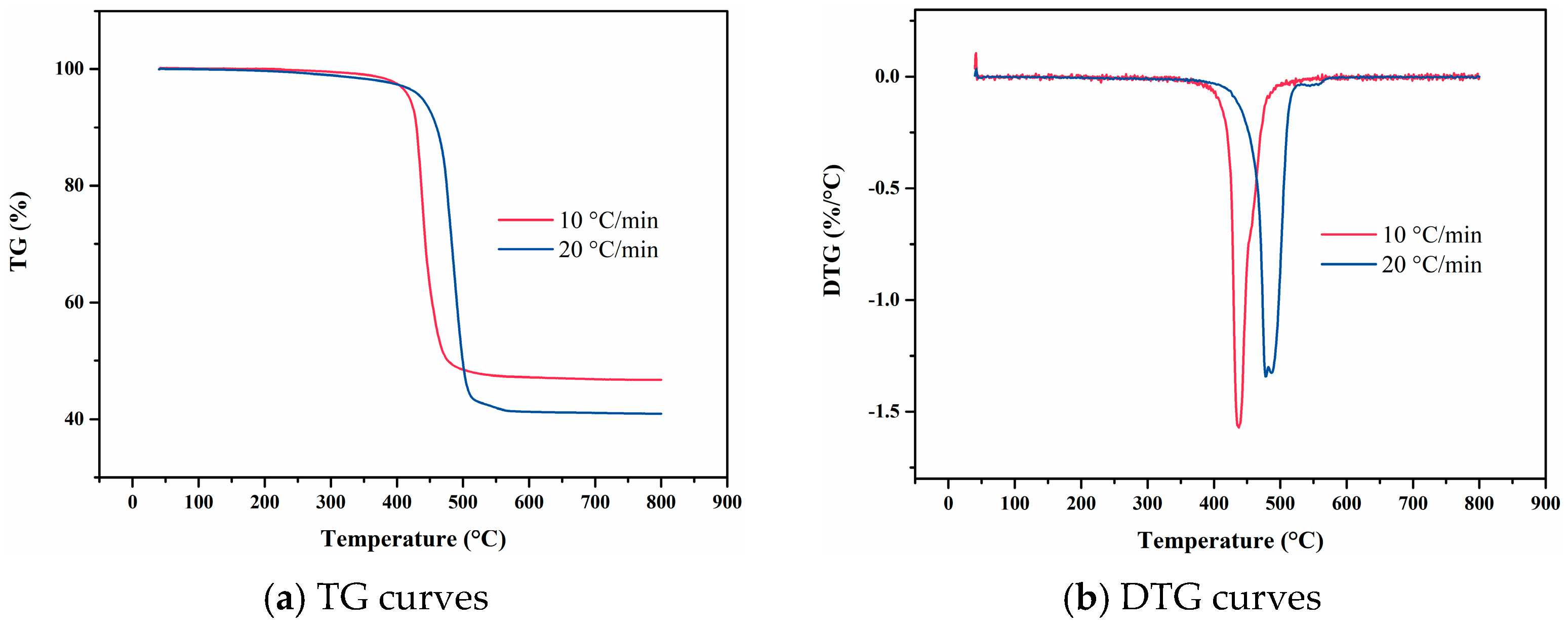
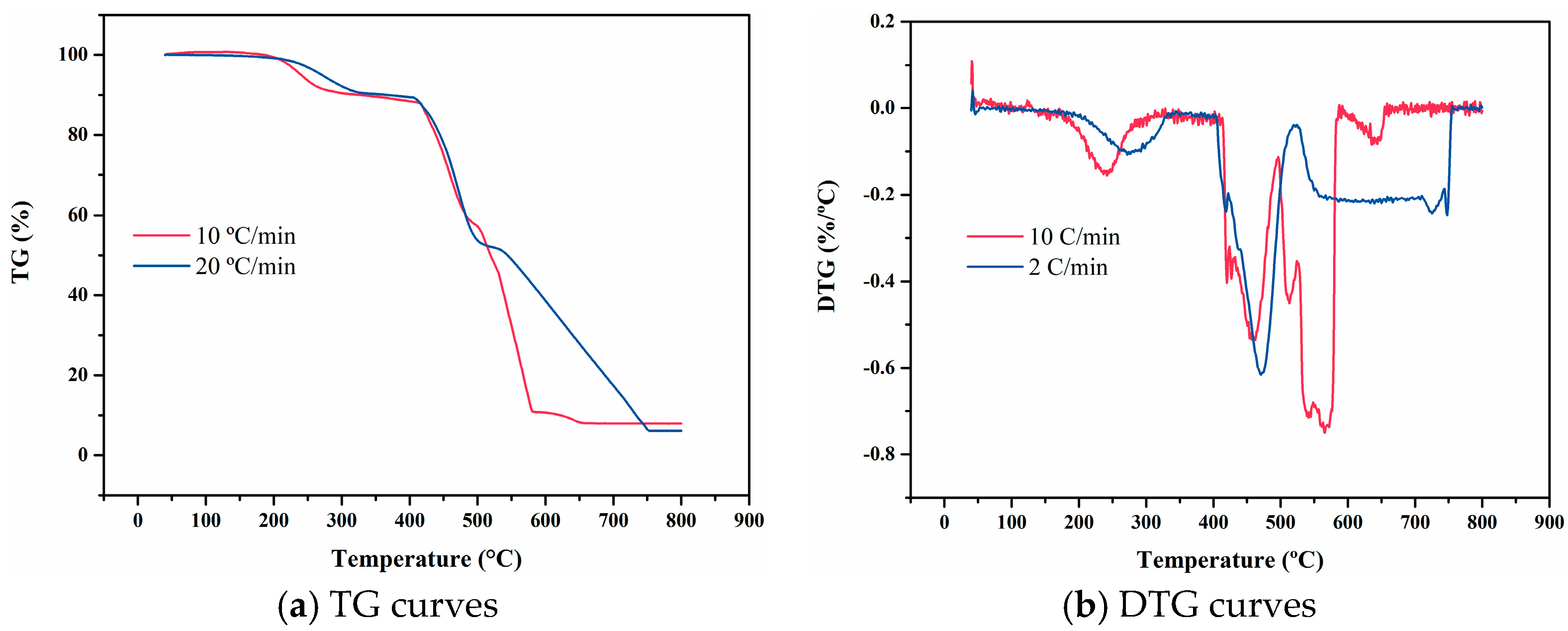
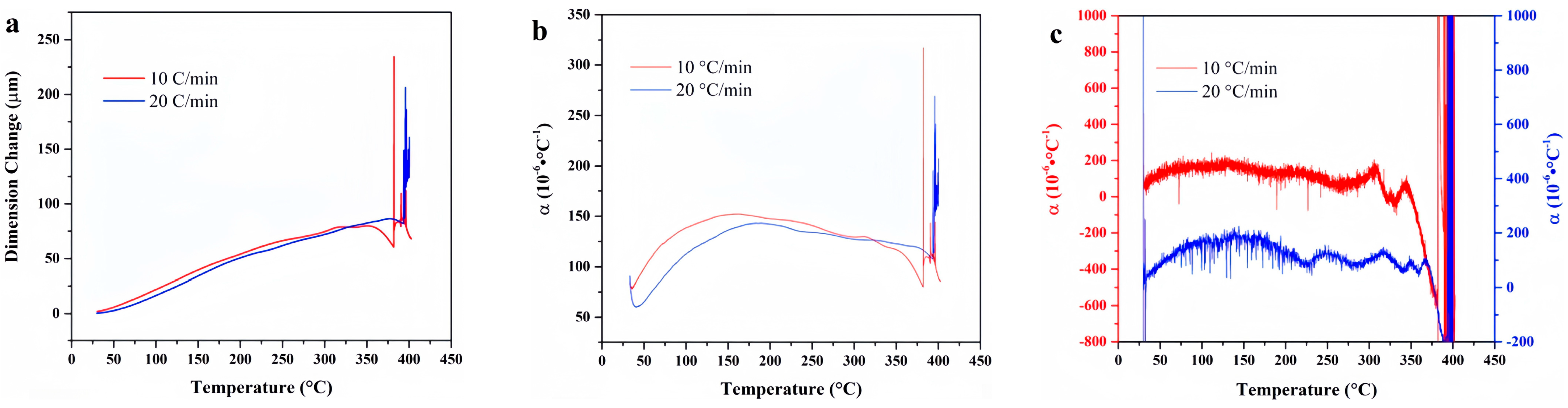
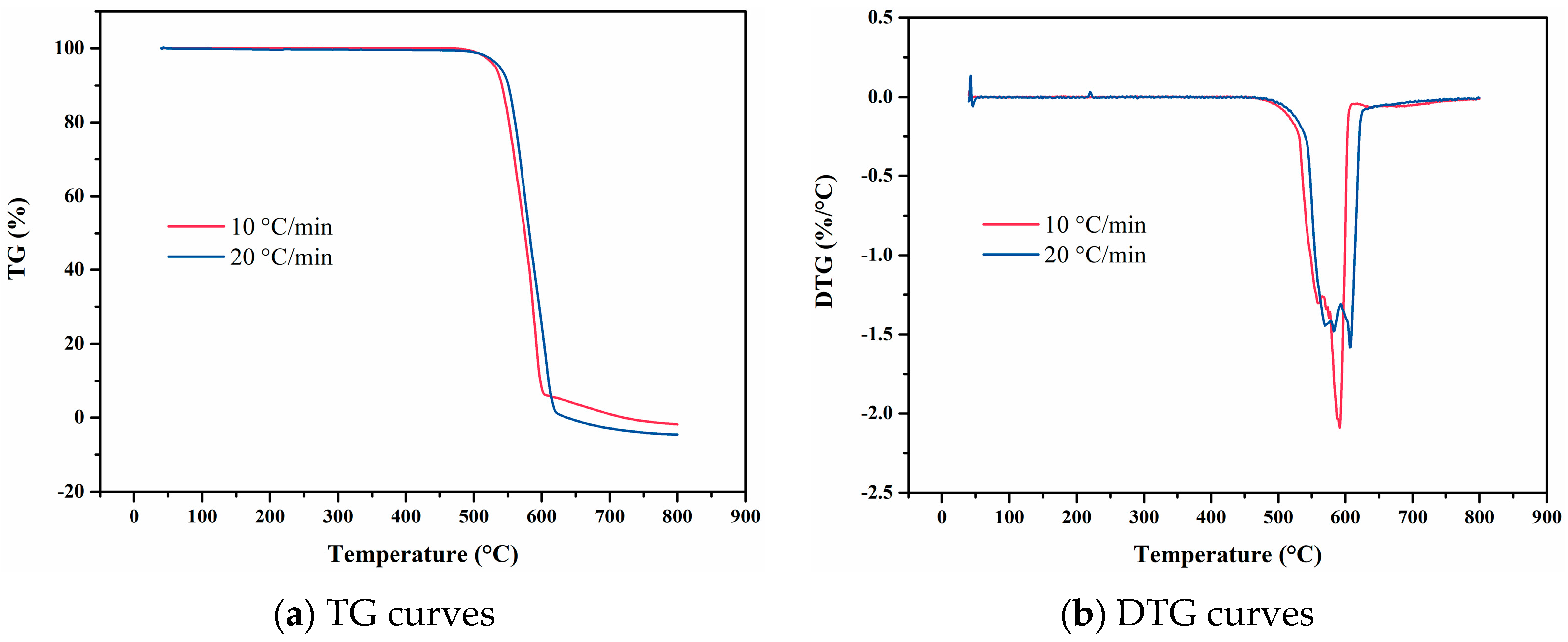
4.1. F117
4.2. FKM
4.3. FM-2D
4.4. FVMQ
4.5. NBR 5080
4.6. SFB-1
4.7. SFB-2
4.8. SZ7-3
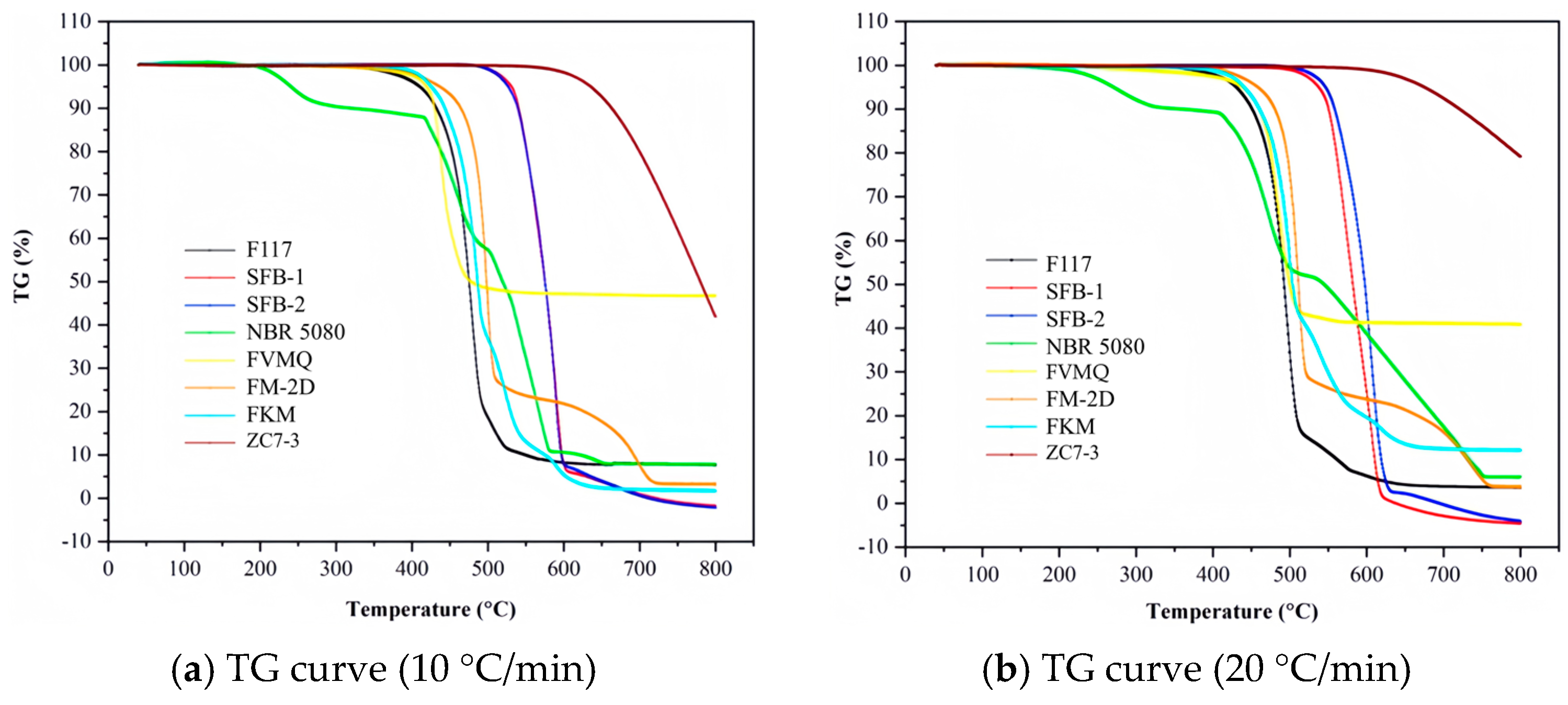
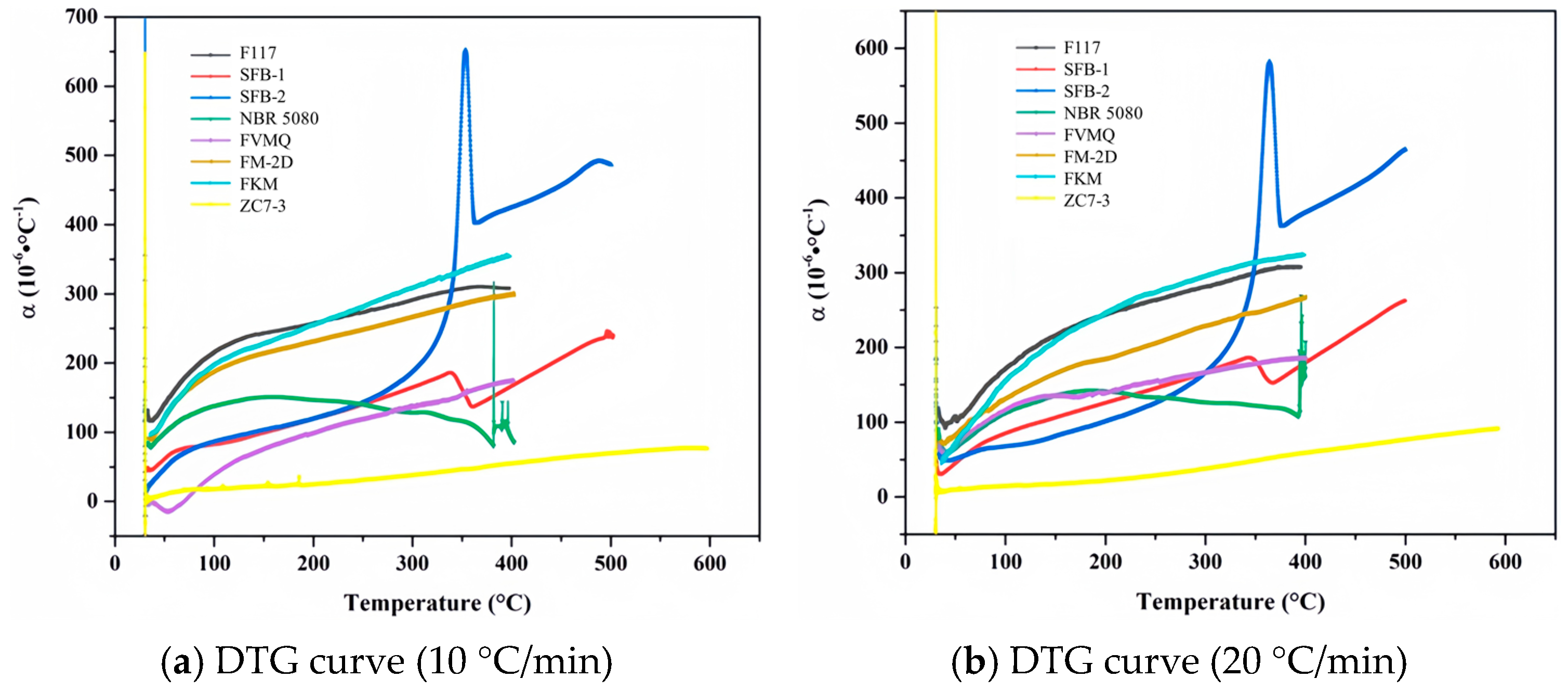
4.9. Data Comparison
5. Material Fire Test Verification
6. Conclusions
- (1)
- Experimental results revealed that ZC7-3 demonstrated the highest thermal decomposition temperature among the tested materials, underscoring its exceptional thermal stability. Polytetrafluoroethylene variants SFB-1 and SFB-2 also exhibited robust high-temperature resistance, with decomposition temperatures exceeding 500 °C. Fluorine-based elastomers, including F117, FKM, and FM-2D, displayed relatively high decomposition temperatures, indicating their potential for stability in elevated-temperature environments. In contrast, NBR 5080 exhibited the lowest thermal decomposition temperature, highlighting its limited thermal stability under extreme conditions.
- (2)
- ZC7-3 exhibited the lowest linear thermal expansion coefficient, establishing it as the material with the most superior dimensional stability. Polytetrafluoroethylene and FVMQ also demonstrated favorable thermal expansion properties, maintaining dimensional integrity even at elevated temperatures. While F117, FKM, and FM-2D exhibited higher thermal expansion coefficients, they remained dimensionally stable within a defined temperature range. Conversely, NBR 5080 displayed significant dimensional changes at high temperatures, rendering it unsuitable for applications requiring precise dimensional control.
- (3)
- Based on the combined assessment of thermal decomposition temperature and dimensional stability, ZC7-3, polytetrafluoroethylene, and FVMQ emerged as the most promising candidates for aero engine sealing applications. These materials not only exhibited exceptional high-temperature resistance but also maintained remarkable dimensional stability, thereby enhancing the operational safety and reliability of aero engines.
- (4)
- Fireproof performance tests were conducted on four representative non-metallic materials, referencing their thermal physical properties. The results indicated that ZC7-3 exhibited unparalleled fireproof performance, maintaining both dimensional stability and sealing integrity during 5-min and 15-min fire exposure tests. In contrast, FKM, NBR 5080, and polytetrafluoroethylene were deemed unsuitable for the static seal configuration employed in this study, as they failed to meet the required performance criteria under fire conditions.
- (5)
- To further validate the suitability of these materials for aero-engine applications, future studies should investigate their long-term performance under cyclic thermal and mechanical loading conditions. Additionally, the development of advanced composite materials, integrating the thermal stability of ZC7-3 with the flexibility of fluoropolymers, could offer a promising avenue for enhancing both performance and durability in extreme environments.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wu, W.; Li, X. Wear and thermal properties of carbon fiber reinforced silicone rubber/fluorine rubber composites. J. Rubber Res. 2019, 22, 119–126. [Google Scholar] [CrossRef]
- Wu, W.; Wang, Y. Vulcanization and thermal properties of silicone rubber/fluorine rubber blends. J. Macromol. Sci. Part B 2019, 58, 579–591. [Google Scholar] [CrossRef]
- Rahmani, S.; Hooshyari, K.; Jamalpour, S.; Tohidian, M.; Maroufkhani, M.; Vakili, H.; Nobakht, D.; Pourhossein, A. Thermal stability and thermomechanical properties of fluoropolymer nanocomposites. In Advanced Fluoropolymer Nanocomposites; Woodhead Publishing: Cambridge, UK, 2023; pp. 213–245. [Google Scholar]
- Pires, L.; Richaud, E.; Roland, S.; Beauvais, C.; Lemetayer, F.X. Thermal aging of a fluorosilicone rubber. In Constitutive Models for Rubber XII; CRC Press: Boca Raton, FL, USA, 2022; pp. 415–419. [Google Scholar]
- He, L.; Zhang, R.; Zhang, D.; Pi, H. Characteristics and kinetics of thermal degradation of fluoroether rubber. J. Macromol. Sci. Part B 2018, 57, 437–446. [Google Scholar] [CrossRef]
- Alfannakh, H.; Alnaim, N.; Ibrahim, S.S. Thermal stability and non-isothermal kinetic analysis of ethylene-propylene-diene rubber composite. Polymers 2023, 15, 1890. [Google Scholar] [CrossRef] [PubMed]
- Nayak, L.; Rahaman, M.; Khastgir, D.; Chaki, T.K. Thermal degradation kinetics of polyimide nanocomposites from different carbon nanofillers: Applicability of different theoretical models. J. Appl. Polym. Sci. 2018, 135, 45862. [Google Scholar] [CrossRef]
- Dong, Z.; Cai, F.; Jiang, Z.; Xu, W. Thermal property studies of in situ blended graphene/nature rubber nanocomposites. Int. J. Polym. Sci. 2020, 2020, 4694213. [Google Scholar] [CrossRef]
- Zhu, L.; Wang, X.; Wu, L.; Luo, Y.; Zhu, Y.; Lin, Q.; Shi, Z. Thermal decomposition properties of cross-linked polycarbonate derived from carbon dioxide, propylene oxide and pyromellitic dianhydride. Mater. Lett. 2014, 128, 396–399. [Google Scholar] [CrossRef]
- Zhang, W.; Zang, Y.; Lu, Y.; Lin, W.; Zhao, S.; Xiong, J.P. Thermal decomposition of brominated butyl rubber. Materials 2021, 14, 6767. [Google Scholar] [CrossRef] [PubMed]
- Erickson, K. Thermal decomposition mechanisms common to polyurethane, epoxy, poly (diallyl phthalate), polycarbonate and poly (phenylene sulfide). J. Therm. Anal. Calorim. 2007, 89, 427–440. [Google Scholar] [CrossRef]
- Prasad, A.V.; Singh, R.P. Thermal decomposition kinetics of a commercial fluoropolymer. Mater. Res. Bull. 1995, 30, 1407–1412. [Google Scholar] [CrossRef]
- Ichihara, S.; Endo, A.; Arii, T. Analysis of thermal decomposition behaviors with consecutive reactions by TG. Thermochim. Acta 2000, 360, 179–188. [Google Scholar] [CrossRef]
- Bettinger, H.F.; Peng, H. Thermolysis of fluorinated single-walled carbon nanotubes: Identification of gaseous decomposition products by matrix isolation infrared spectroscopy. J. Phys. Chem. B 2005, 109, 23218–23224. [Google Scholar] [CrossRef] [PubMed]
- Sokmen, K.F.; Cakhusr, A. Numerical and Experimental Investigation of the Effects of Thermal Expansion and Pressure Loss in Air Hoses made from Different Materials. Eur. J. Res. Dev. 2022, 2, 383–399. [Google Scholar] [CrossRef]
- Korolev, A.; Mishnev, M.; Ulrikh, D.V. Non-Linearity of Thermosetting Polymers’ and GRPs’ Thermal Expanding: Experimental Study and Modeling. Polymers 2022, 14, 4281. [Google Scholar] [CrossRef] [PubMed]
- Grange, N.; Manescau, B.; Chetehouna, K.; Gascoin, N.; Senave, S.; Reynaud, I. Experimental investigation of the fire behaviour of a carbon-PEKK composite used for aeronautical applications. J. Phys. Conf. Ser. 2018, 1107, 032023. [Google Scholar] [CrossRef]
- Czarnecka-Komorowska, D.; Krawiec, P.; Wargua, U.; Maozi, D.; Dziechciarz, A. The toxicological testing and thermal decomposition of drive and transport belts made of thermoplastic multilayer polymer materials. Polymers 2020, 12, 2232. [Google Scholar] [CrossRef] [PubMed]
- Qi, M.; Jia, X.; Wang, G.; Xu, Z.; Zhang, Y.; He, Q. Research on high temperature friction properties of PTFE/Fluorosilicone rubber/silicone rubber. Polym. Exp. 2020, 91, 106817. [Google Scholar] [CrossRef]
- Pinedo, B.; Hadfield, M.; Tzanakis, I.; Conte, M.; Anand, M. Thermal analysis and tribological investigation on TPU and NBR elastomers applied to sealing applications. Tribol. Int. 2018, 127, 24–36. [Google Scholar] [CrossRef]
- Federal Aviation Administration. 14 CFR Part 25: Airworthiness Standards: Transport Category Airplanes; U.S. Government Publishing Office: Washington, DC, USA, 2023.
- European Union Aviation Safety Agency. Certification Specifications for Large Aeroplanes (CS-25); EASA: Cologne, Germany, 2023.
- ARP 1179; Aerospace Fluid Power-Contamination Classification for Hydraulic Systems. SAE International: Warrendale, PA, USA, 2017.
- ISO 2685; Aircraft-Environmental Test Procedure for Airborne Equipment-Resistance to Fire in Designated Fire Zones. International Organization for Standardization: Geneva, Switzerland, 2020.
- RTCA, Inc. DO-160: Environmental Conditions and Test Procedures for Airborne Equipment; RTCA, Inc.: Washington, DC, USA, 2022. [Google Scholar]
- Q/6S1822-2002; Technical Specifications for Rubber Seals in Aviation. Aviation Industry Corporation of China: Beijing, China, 2002.
- AMS 7287; Rubber, Fluorocarbon Elastomer (FKM), High Temperature Resistant, 75–85 Hardness. SAE International: Warrendale, PA, USA, 2018.
- Q/6S 1590-2010; Test Methods for Rubber Seals in Aviation. Aviation Industry Corporation of China: Beijing, China, 2010.
- AMS-R-25988; Rubber, Fluorosilicone Elastomer, Oil-and-Fuel-Resistant, Sheets, Strips, Molded Parts, and Extruded Shapes. SAE International: Warrendale, PA, USA, 2015.
- GJB5037-2001; General Specifications for Rubber Seals in Aviation. General Armament Department of the People’s Liberation Army: Beijing, China, 2001.
- ASTM D4894; Standard Specification for Polytetrafluoroethylene (PTFE) Granular Molding and Ram Extrusion Materials. ASTM International: West Conshohocken, PA, USA, 2019.
- GB/T 20671; Polytetrafluoroethylene (PTFE) Molding and Extrusion Materials. China Standards Press: Beijing, China, 2017.
- Available online: https://www.mt.com/us/en/home/products/Laboratory_Analytics_Browse/TA_Family_Browse/ta-instruments/thermal-analysis-system-TGA-2.html (accessed on 22 February 2012).
- Available online: https://www.tainstruments.com/pdf/TMA.pdf (accessed on 22 February 2012).
- ISO/IEC Guide 98-1:2024; Guide to the Expression of Uncertainty in Measurement—Part 1: Introduction. International Organization for Standardization (ISO) & International Electrotechnical Commission (IEC): Geneva, Switzerland, 2024.
- Brown, M.E. Introduction to Thermal Analysis: Techniques and Applications; Chapman and Hall: London, UK, The Netherlands, 1988. [Google Scholar]
- Touloukian, Y.S.; Kirby, R.K.; Taylor, R.E.; Lee, T.Y.R. Thermal Expansion: Metallic Elements and Alloys. In Thermophysical Properties of Matter; IFI/Plenum: New York, NY, USA, 1975; Volume 12. [Google Scholar]





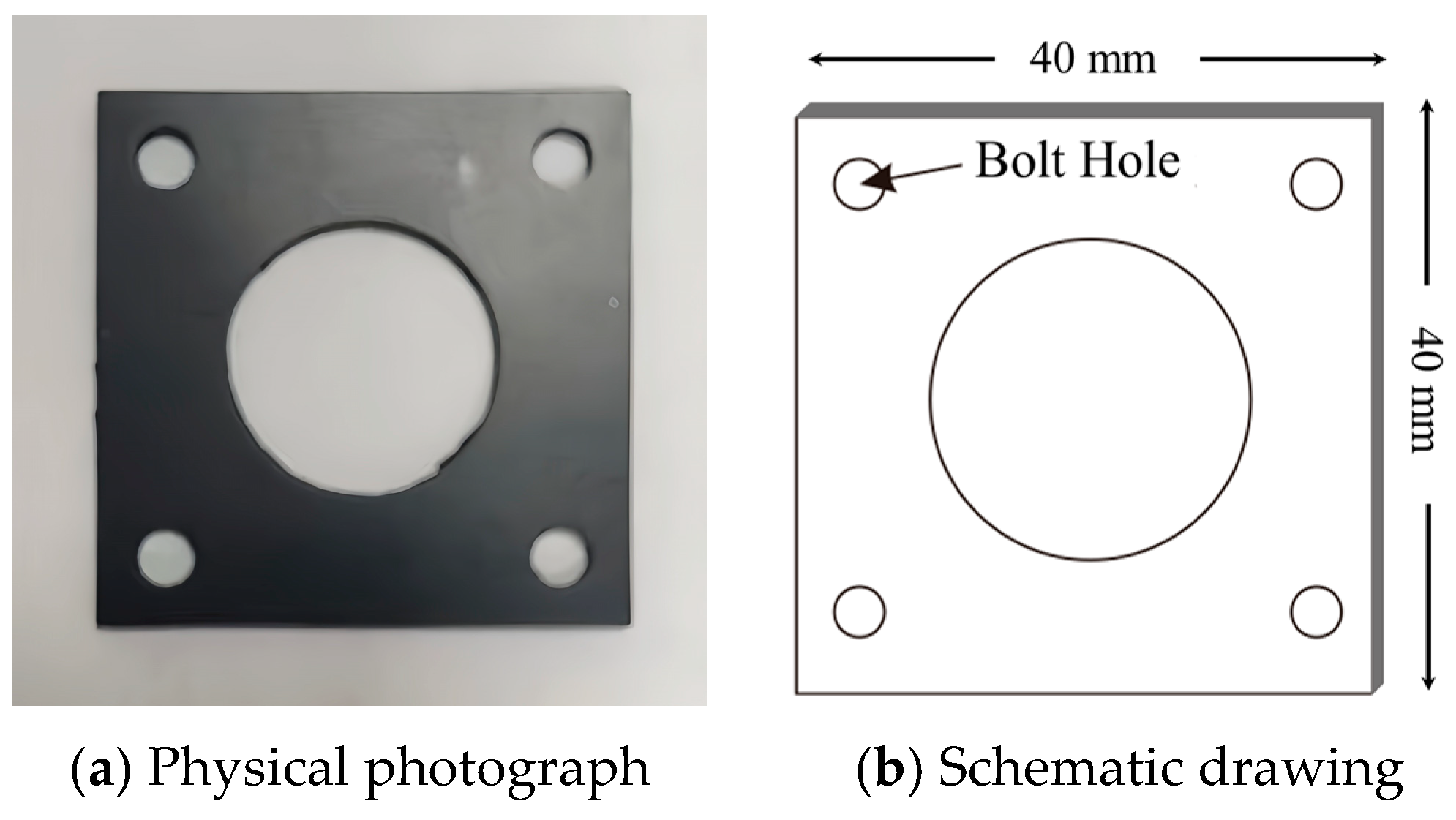
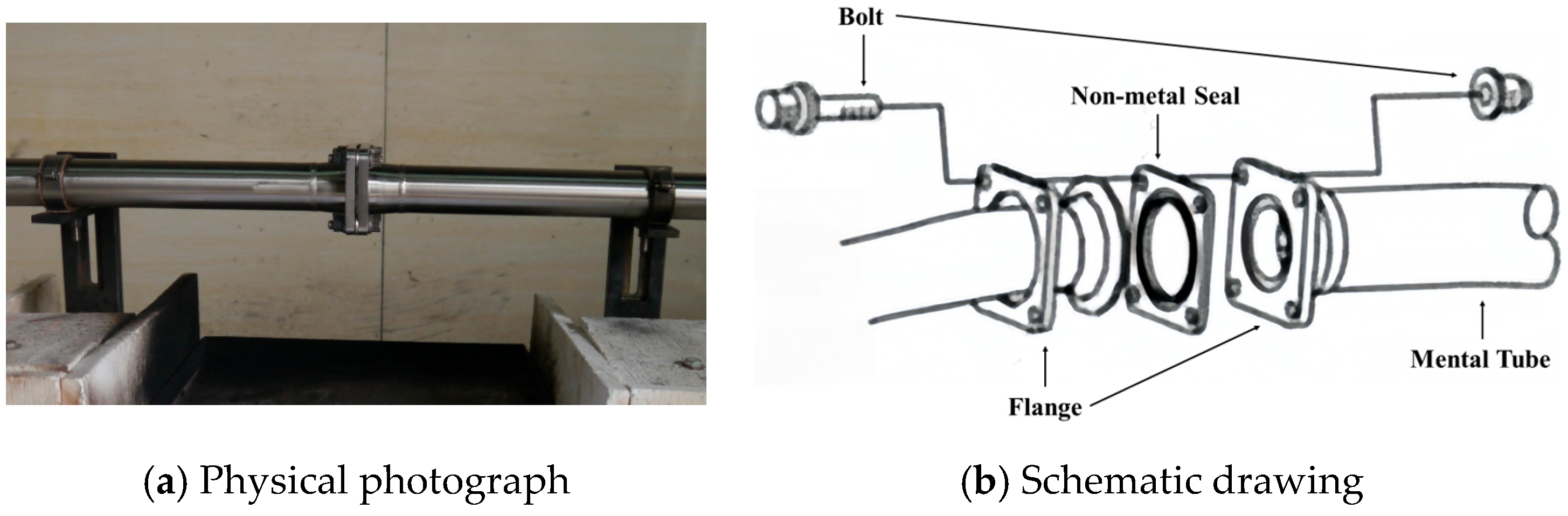
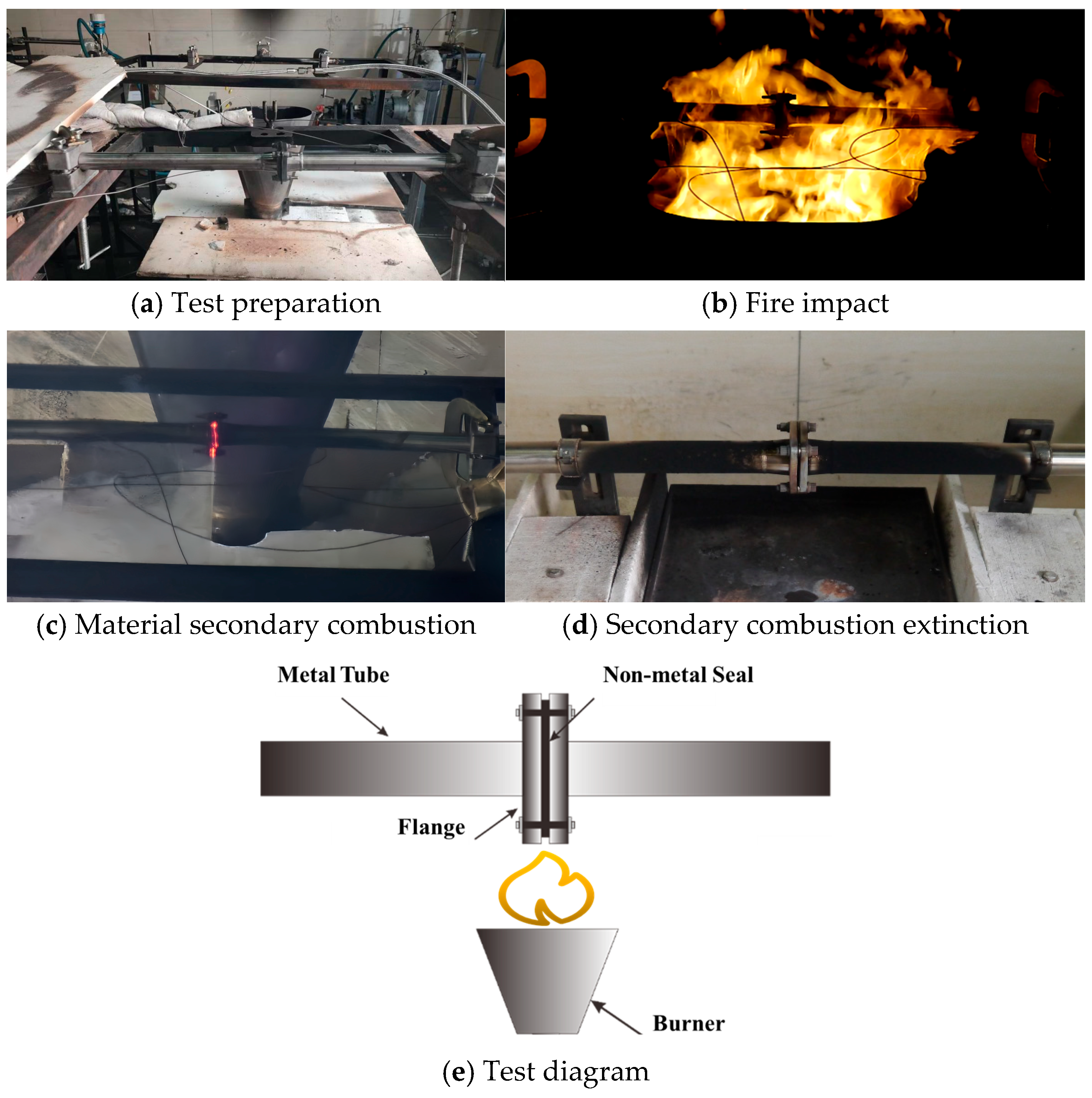
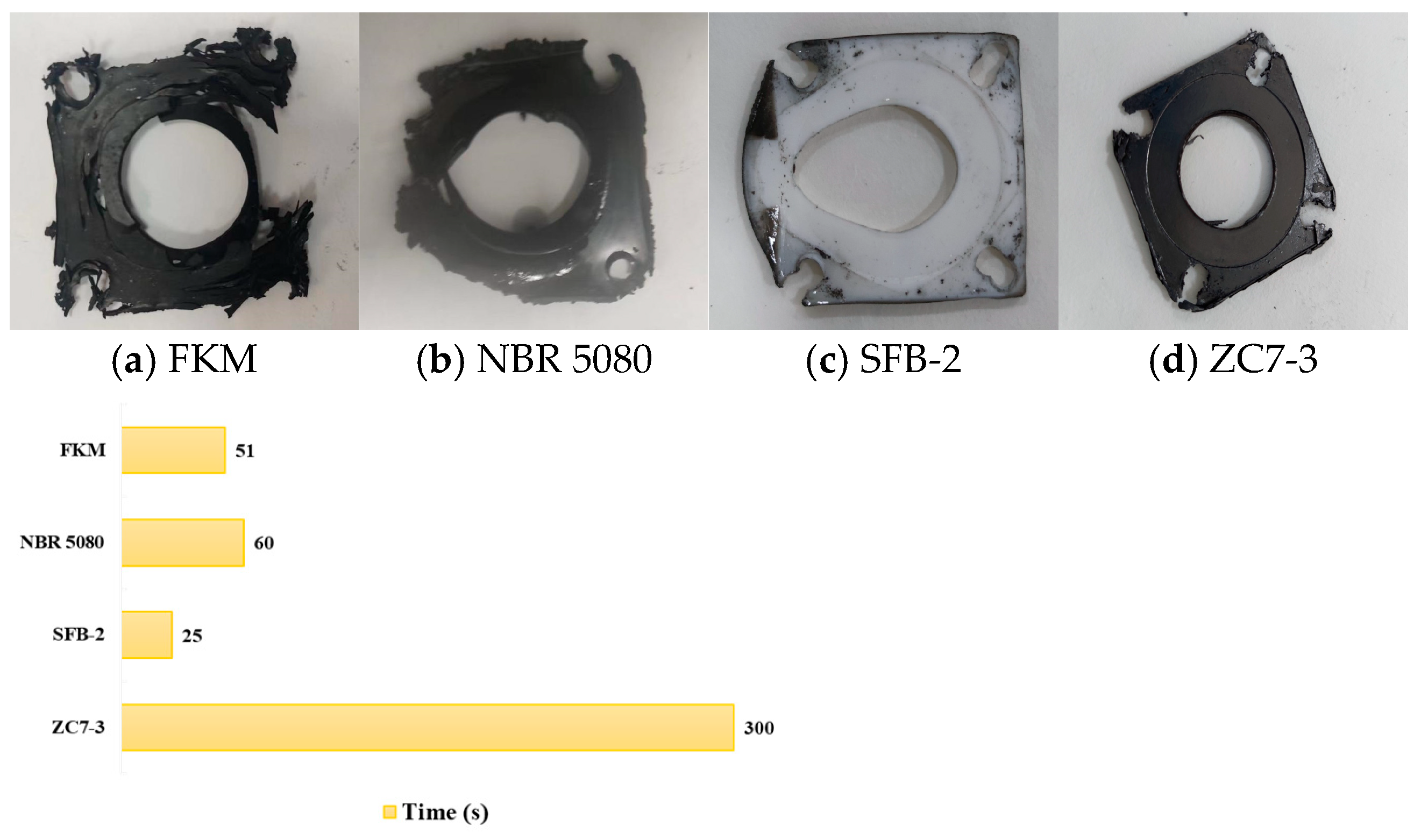

| No. | Type | Name | Tensile Strength (MPa) | Elongation at Break (%) | Compression Set (%) | Shore A (HA) | Standard |
|---|---|---|---|---|---|---|---|
| 1 | Fluororubber | F117 | 11 | 191 | (200 °C/24 h/25%) ≤ 22 | 64 | Q/6S1822-2002 [26] |
| 2 | Fluorocarbon rubber | FKM | ≥15 | ≥120 | (200 °C/24 h/25%) ≤ 35 | 70~80 | SAE AMS 7287 [27] |
| 3 | Fluoroelastomers | FM-2D | 9 | 110 | (250 °C/24 h/20%) ≤ 50 | 69 | Q/6S 1590-2010 [28] |
| 4 | Fluorosilicone rubber | FVMQ | ≥5.17 | ≥125 | (175 °C/22 h/25%) ≤ 35 | 50~80 | SAE AMS-R-25988 [29] |
| 5 | Nitrile butadiene rubber | NBR 5080 | 10~25 | 200~600 | (100 °C/24 h/25%) ≤ 40 | 50~90 | GJB5037-2001 [30] |
| No. | Type | Name | Tensile Strength (MPa) | Elongation at Break (%) | Compression Set (%) | Shore A (HA) | Standard |
|---|---|---|---|---|---|---|---|
| 1 | Polytetrafluoroethylene | SFB-1 | 20~35 | 200~400 | 5~15 | 50~65 | ASTM D4894/GB/T 20671 [31,32] |
| 2 | Polytetrafluoroethylene | SFB-2 | 25~40 | 250~500 | 5~10 | 55~70 | ASTM D4894/GB/T 20671 |
| Graphite | Volume Density (g/cm3) | Shore’s Hardness (Hs) | Break Resistant Strength (MPa) | Compression Resistance Strength (MPa) | Porosity (%) | Impact Resistance Strength (10−5 J/mm) | Elasticity Modulus (GPa) | Highest Make with Temperature (°C) |
|---|---|---|---|---|---|---|---|---|
| ZC7-3 | 1.90 | 100 | 100 | 250 | 0.5 | 4 | 15 | 500 |
| Parameter | Value |
|---|---|
| Temperature Data | |
| Temperature Range | Room temperature to 1100 °C |
| Temperature Accuracy | ±1 °C |
| Temperature Precision | ±0.6 °C |
| Heating Rate | 0.02~150 °C/min |
| Cooling Time | 20 min (1100~100 °C) |
| Helium Cooling Time | ≤11 min (1100~100 °C) |
| Balance Data | |
| Measurement Range | ≤5 g |
| Resolution | 0.1 μg |
| Weighing Accuracy | 0.005% |
| Weighing Precision | 0.0025% |
| Repeatability | <0.0009 mg |
| Typical Minimum Weighing Value | 0.17 mg |
| Blank Curve Reproducibility | >±10 μg |
| Parameter | Value |
|---|---|
| Temperature Range | −150~1000 °C |
| Temperature Precision | ±1 °C |
| Cooling Time | From 600 °C to 50 °C <10 min |
| Measurement Precision | ±0.1% |
| Sensitivity | 15 nm |
| Displacement Resolution | <0.5 nm |
| Dynamic Baseline Drift | <1 μm (100~500 °C) |
| Force Range | 0.001~2 N |
| Force Resolution | 0.001 N |
| Frequency Range | 0.01~2 Hz |
| No. | 1 | 2 | 3 | 4 | 5 | 6 | 7 |
|---|---|---|---|---|---|---|---|
| T (°C) | 1045 | 1073 | 1086 | 1065 | 1049 | 1022 | 1025 |
| Tave (°C) | 1052 | qave (W/cm2) | 11.0 | ||||
| Sample | (°C/min) | T1% (°C) | T5% (°C) | T10% (°C) | T50% (°C) | Te (°C) | Tm (°C) |
|---|---|---|---|---|---|---|---|
| F117 | 10 | 346 | 410 | 432 | 475 | 448 | 477 |
| 20 | 371 | 430 | 451 | 493 | 476 | 498 | |
| FKM | 10 | 391 | 425 | 443 | 486 | 449 | 485 |
| 20 | 395 | 441 | 460 | 504 | 474 | 500 | |
| FM-2D | 10 | 376 | 436 | 464 | 498 | 483 | 499 |
| 20 | 404 | 458 | 481 | 511 | 493 | 512 | |
| FVMQ | 10 | 355 | 419 | 430 | 477 | 425 | 437 |
| 20 | 295 | 438 | 460 | 500 | 457 | 479 | |
| NBR 5080 | 10 | 207 | 241 | 325 | 519 | —— | 241 |
| 20 | 209 | 271 | 367 | 544 | —— | 273 | |
| SFB-1 | 10 | 502 | 531 | 540 | 575 | 539 | 591 |
| 20 | 498 | 538 | 551 | 583 | 554 | 606 | |
| SFB-2 | 10 | 500 | 526 | 539 | 576 | 553 | 588 |
| 20 | 514 | 547 | 559 | 598 | 576 | 607 | |
| ZC7-3 | 10 | 582 | 635 | 663 | 779 | —— | —— |
| 20 | 599 | 675 | 721 | —— | —— | —— |
| Sample | (°C/min) | α100 (10−6 °C−1) | α150 (10−6 °C−1) | α200 (10−6 °C−1) | α250 (10−6 °C−1) | α300 (10−6 °C−1) | α350 (10−6 °C−1) |
|---|---|---|---|---|---|---|---|
| F117 | 10 | 215 | 243 | 257 | 273 | 291 | 307 |
| 20 | 171 | 216 | 243 | 263 | 280 | 299 | |
| FKM | 10 | 197 | 227 | 254 | 280 | 308 | 333 |
| 20 | 154 | 208 | 244 | 272 | 294 | 313 | |
| FM-2D | 10 | 188 | 214 | 231 | 249 | 267 | 286 |
| 20 | 131 | 166 | 184 | 206 | 229 | 247 | |
| FVMQ | 10 | 39 | 76 | 99 | 119 | 136 | 154 |
| 20 | 116 | 135 | 139 | 155 | 166 | 178 | |
| NBR 5080 | 10 | 139 | 152 | 147 | 140 | 129 | 117 |
| 20 | 112 | 136 | 140 | 132 | 127 | 123 | |
| SFB-1 | 10 | 82 | 99 | 119 | 140 | 165 | 163 |
| 20 | 85 | 106 | 125 | 145 | 167 | 182 | |
| SFB-2 | 10 | 86 | 103 | 119 | 143 | 188 | 584 |
| 20 | 67 | 82 | 101 | 125 | 167 | 319 | |
| ZC7-3 | 10 | 18 | 22 | 25 | 31 | 38 | 47 |
| 20 | 15 | 17 | 22 | 29 | 38 | 49 |
| Serial Number | Fluid Medium | Material Grade | Temperature (°C) | Pressure (MPa) | Flow Rate (L/h) | Fire Impact Time (min) |
|---|---|---|---|---|---|---|
| 1 | RP-3 | FKM | 120 | 5 | 130 | 5 |
| 2 | NBR 5080 | |||||
| 3 | SFB-2 | |||||
| 4 | ZC7-3 | |||||
| 5 | Mobil Jet™ Oil II | FKM | 120 | 0.5 | 60 | 15 |
| 6 | NBR 5080 | |||||
| 7 | SFB-2 | |||||
| 8 | ZC7-3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, B.; Wang, K.; Zeng, T.; Weng, W.; Xia, Z.; Su, Z.; Xie, F. Experimental Study on Thermal Decomposition Temperature and Thermal Expansion Coefficient of Typical Nonmetallic Materials in Aeroengine Components. Materials 2025, 18, 1250. https://doi.org/10.3390/ma18061250
Wu B, Wang K, Zeng T, Weng W, Xia Z, Su Z, Xie F. Experimental Study on Thermal Decomposition Temperature and Thermal Expansion Coefficient of Typical Nonmetallic Materials in Aeroengine Components. Materials. 2025; 18(6):1250. https://doi.org/10.3390/ma18061250
Chicago/Turabian StyleWu, Bin, Kai Wang, Tai Zeng, Wenguo Weng, Zuxi Xia, Zhengliang Su, and Fei Xie. 2025. "Experimental Study on Thermal Decomposition Temperature and Thermal Expansion Coefficient of Typical Nonmetallic Materials in Aeroengine Components" Materials 18, no. 6: 1250. https://doi.org/10.3390/ma18061250
APA StyleWu, B., Wang, K., Zeng, T., Weng, W., Xia, Z., Su, Z., & Xie, F. (2025). Experimental Study on Thermal Decomposition Temperature and Thermal Expansion Coefficient of Typical Nonmetallic Materials in Aeroengine Components. Materials, 18(6), 1250. https://doi.org/10.3390/ma18061250






