Performance Characterization of Recycled Carbon Fiber and Its Interfacial Bonding Properties with Cement Matrix
Abstract
1. Introduction
2. Experimental Materials and Methods
2.1. Materials
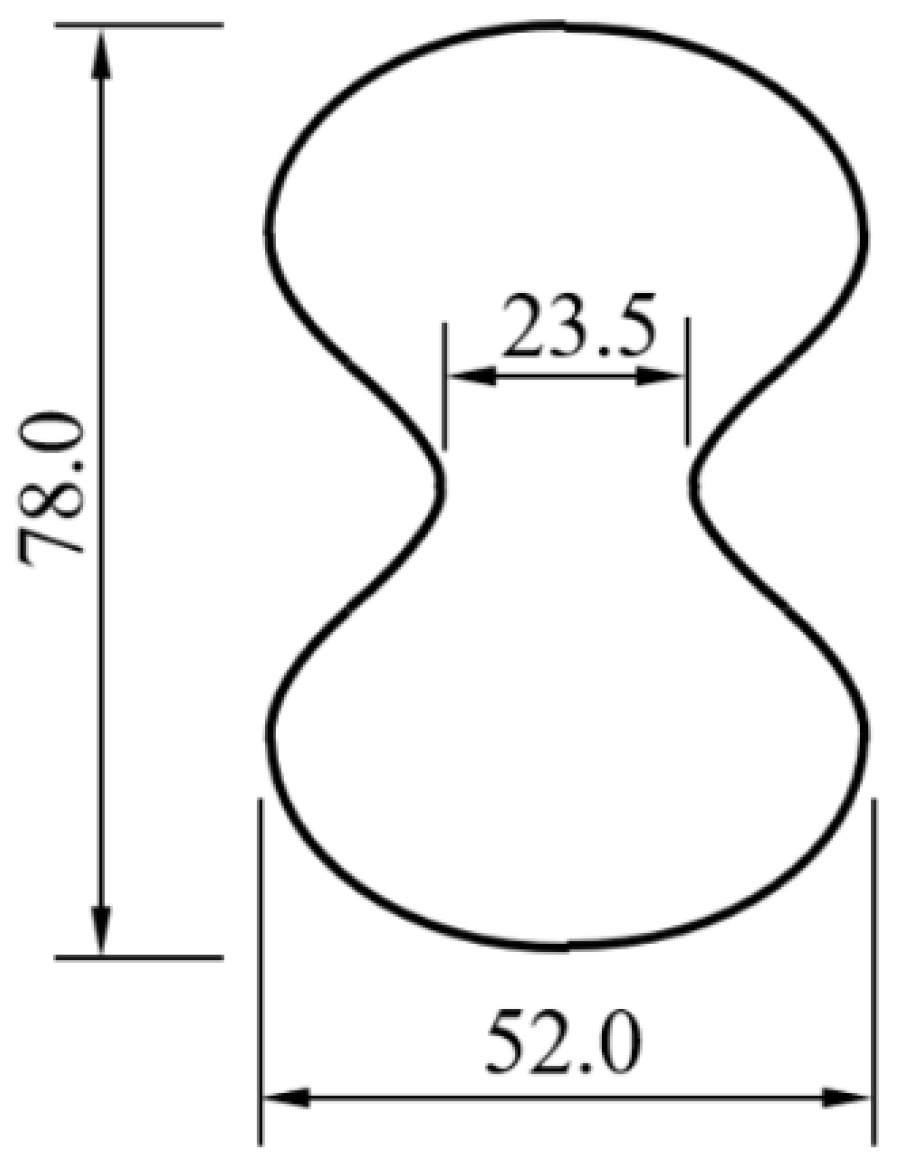
2.2. Tensile Performance Testing of RCF-Reinforced Cementitious Materials
2.3. Fiber Performance Characterization Tests
2.3.1. Fourier Transform Infrared Spectroscopy (FTIR) Test
2.3.2. Atomic Force Microscopy (AFM) Surface Roughness Testing
2.3.3. Scanning Electron Microscopy (SEM) Testing
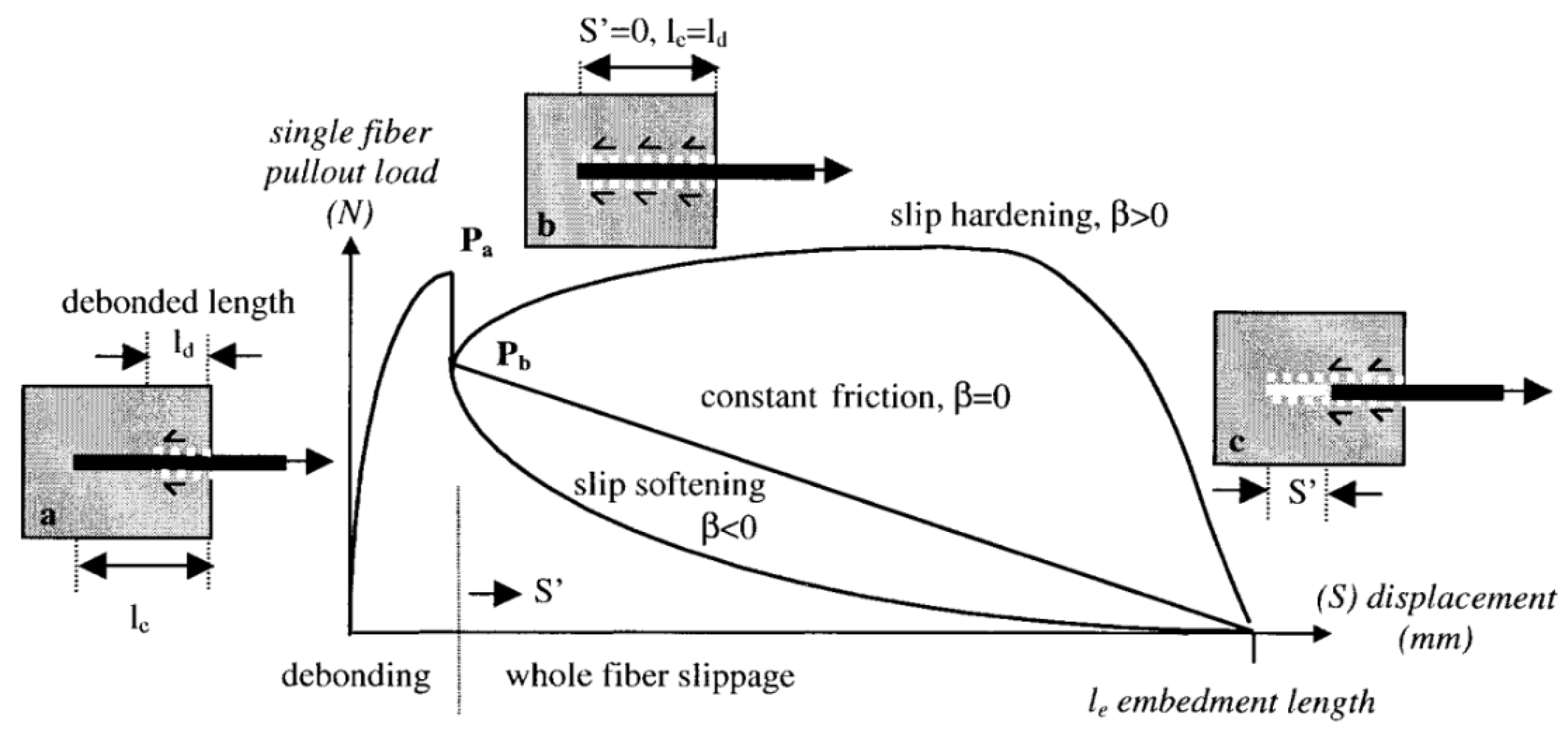
2.4. Single-Fiber Pull-Out Test
2.4.1. Selection of a Single Carbon Fiber
2.4.2. Mixing Ratio and Casting Process
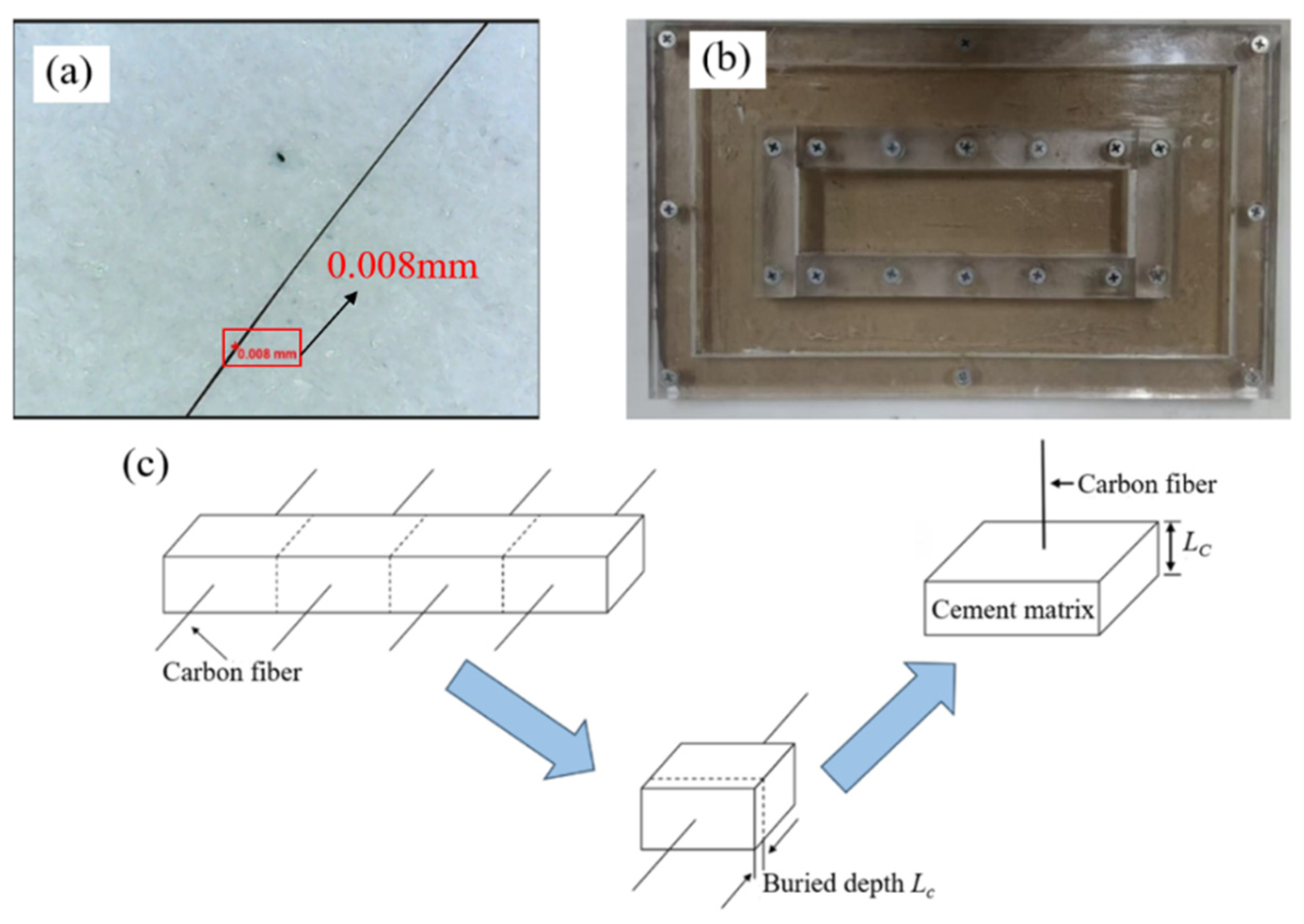
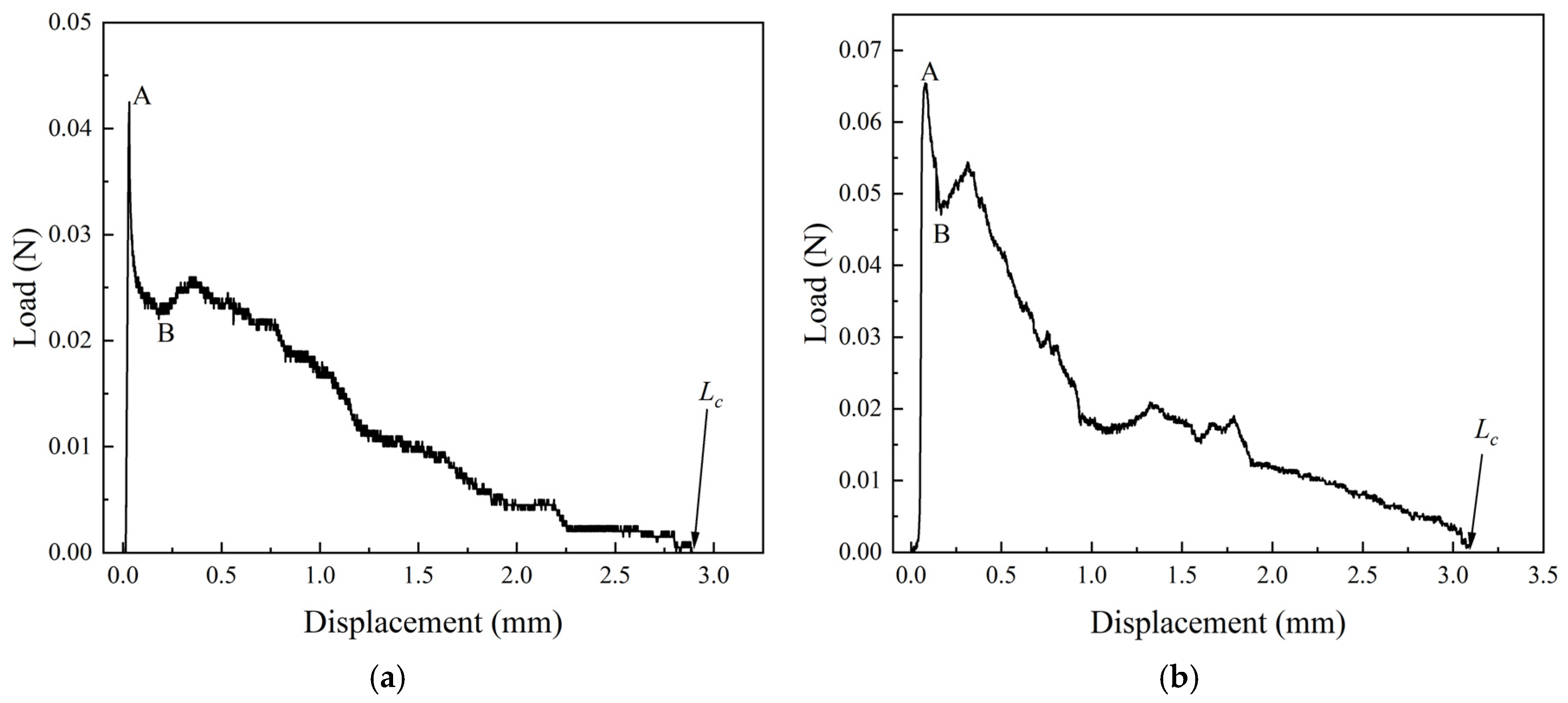
2.4.3. Determination of Critical Embedding Depth
2.4.4. Specimen Loading
3. Results and Discussion
3.1. Tensile Strength of Recycled Carbon Fiber-Reinforced Cementitious Materials
3.2. Fiber Characterization Tests
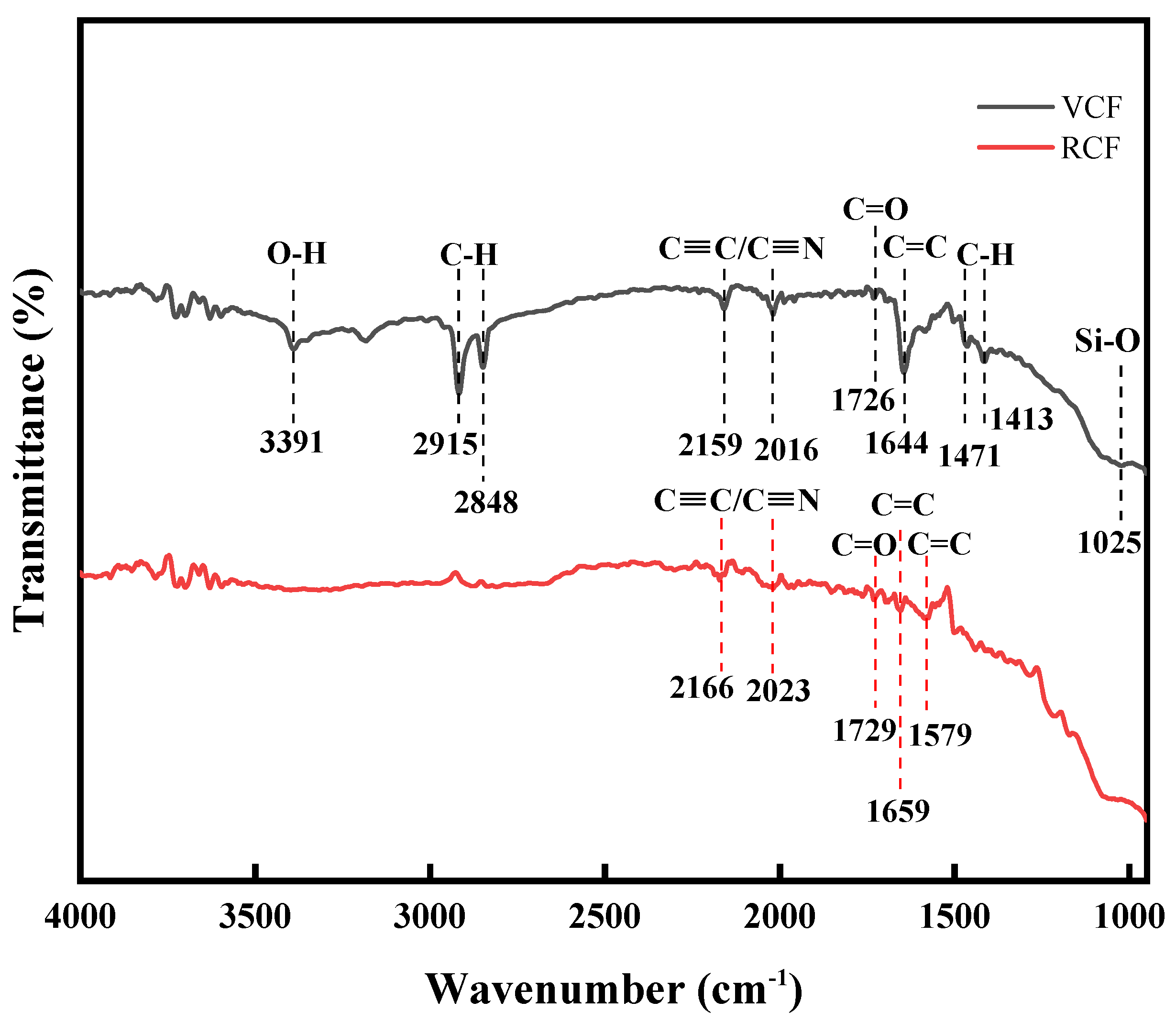
3.2.1. Surface Oxidation Functional Group
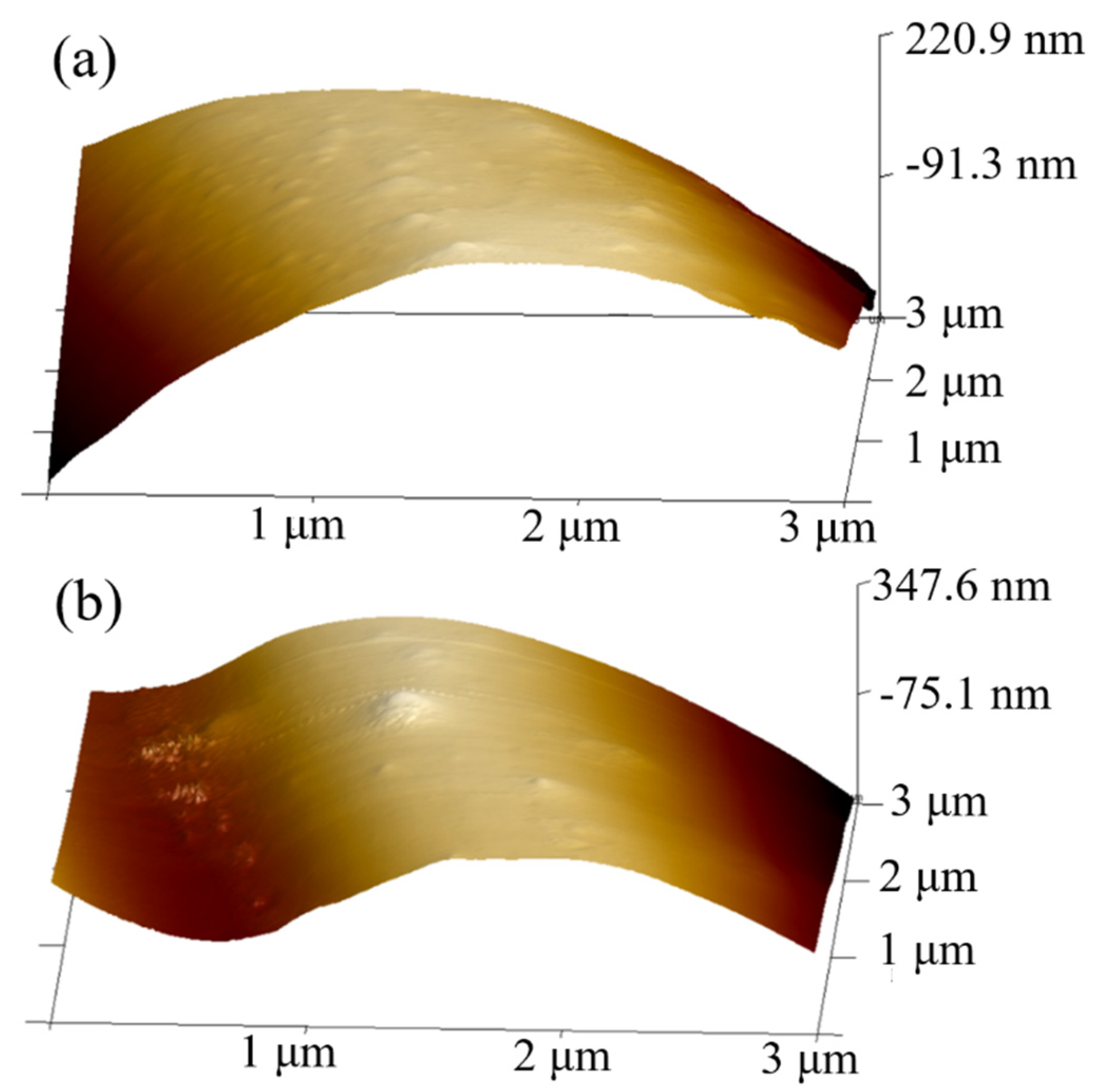
3.2.2. AFM Surface Roughness
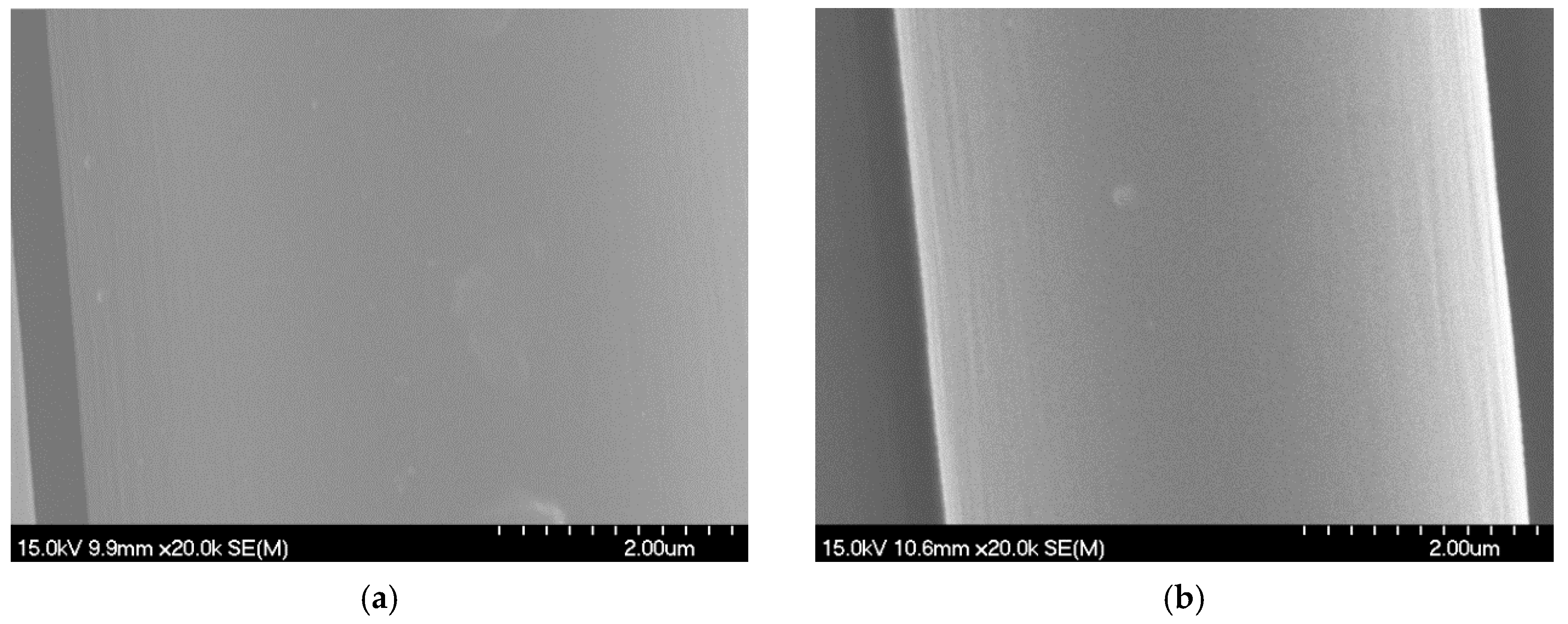
3.2.3. Microscopic Topography
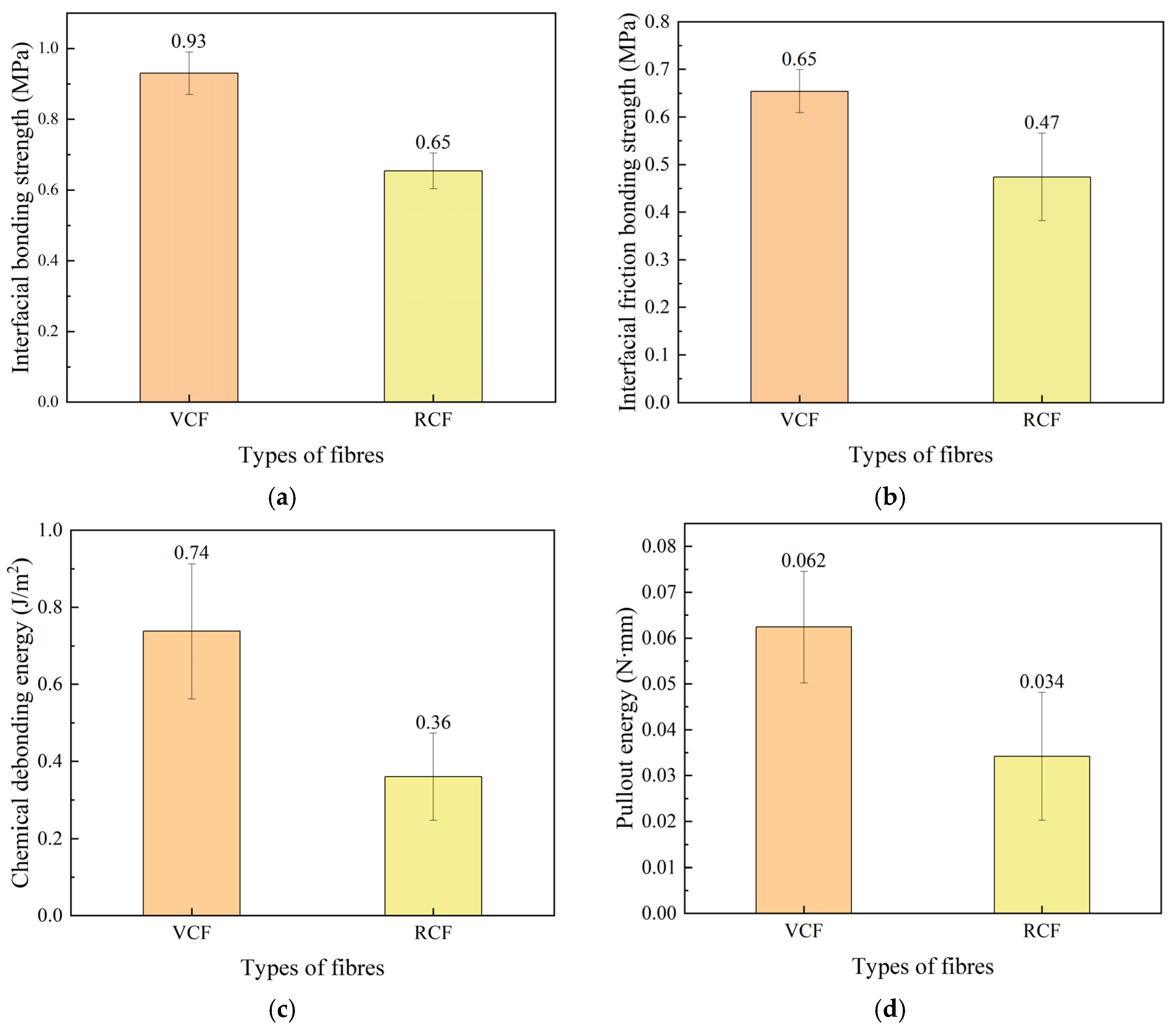
3.3. Interfacial Adhesion Properties
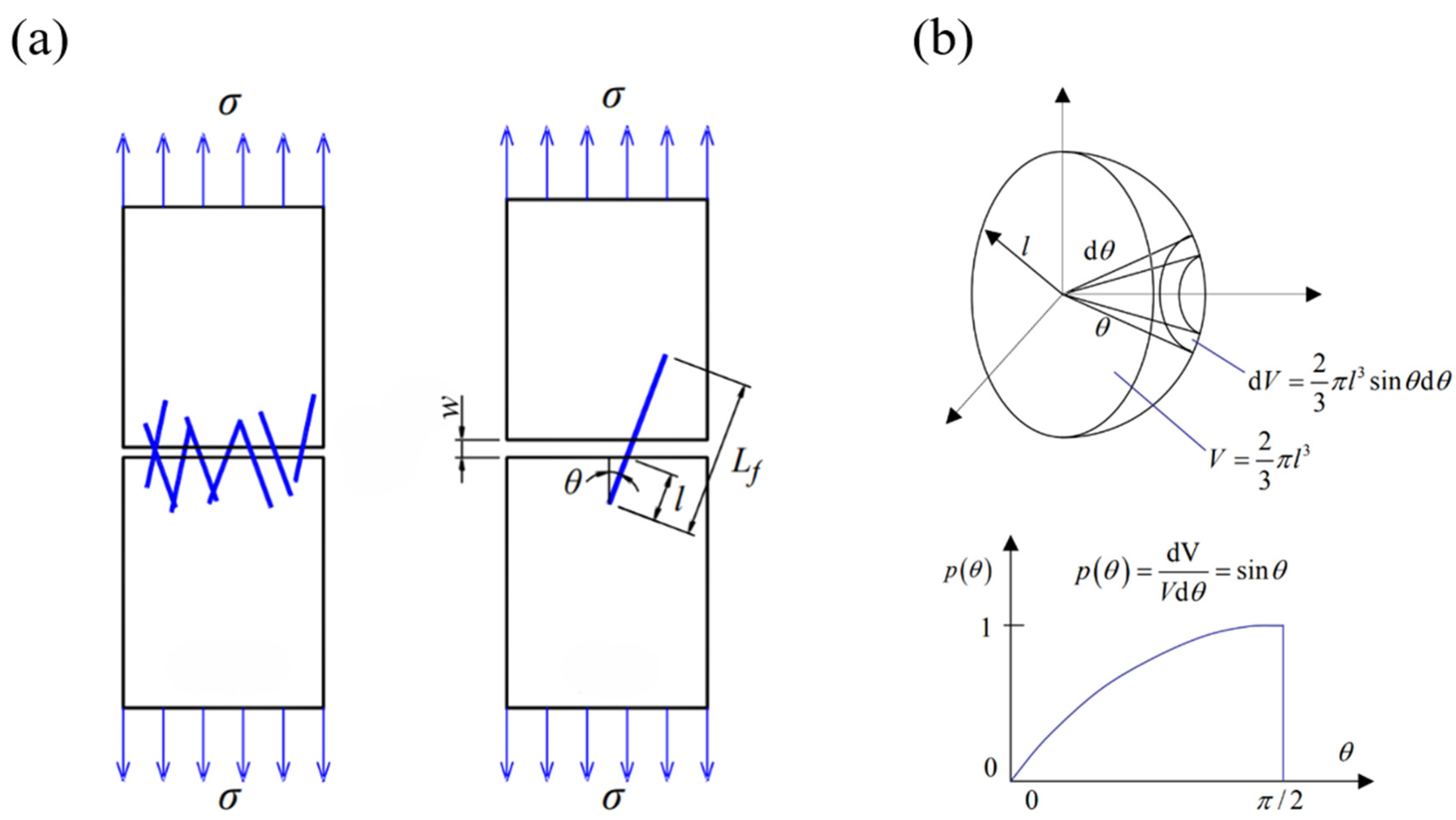
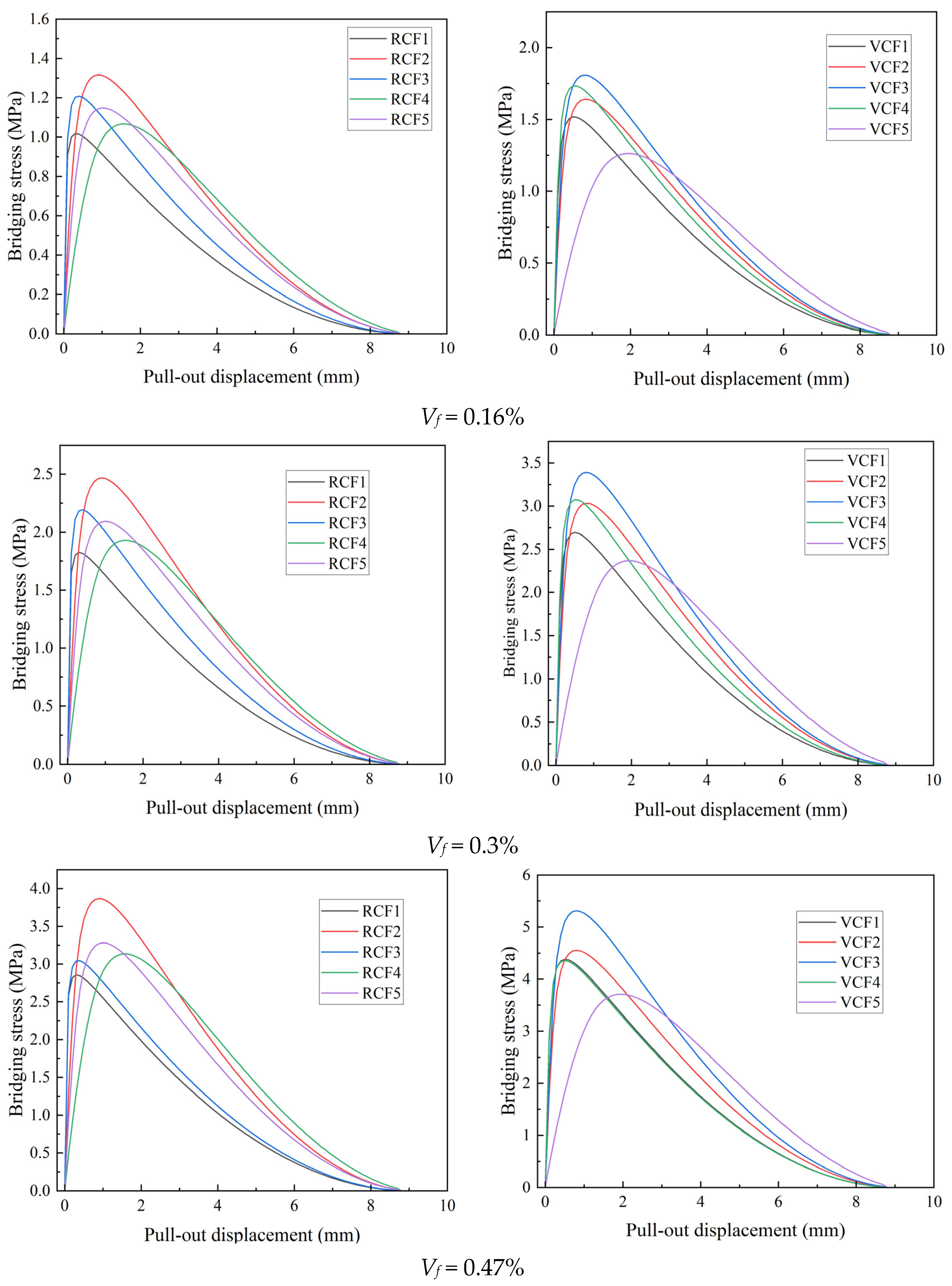
4. RCF Strengthens the Bridging Stress of Cementitious Materials
4.1. Establishment of a Single-Fiber Pull-Out Model
4.2. Calculation Result
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zheng, H.; Zhang, W.; Li, B.; Zhu, J.; Wang, C.; Song, G.; Wu, G.; Yang, X.; Huang, Y.; Ma, L. Recent advances of interphases in carbon fiber-reinforced polymer composites: A review. Compos. Part B-Eng. 2022, 233, 109639. [Google Scholar] [CrossRef]
- Sun, Z.; Luo, Y.; Chen, C.; Dong, Z.; Jiang, G.; Chen, F.; Ma, P. Mechanical enhancement of carbon fiber-reinforced polymers: From interfacial regulating strategies to advanced processing technologies. Prog. Mater. Sci. 2023, 142, 101221. [Google Scholar]
- Al-Lami, A.; Hilmer, P.; Sinapius, M. Eco-efficiency assessment of manufacturing carbon fiber reinforced polymers (CFRP) in aerospace industry. Aerosp. Sci. Technol. 2018, 79, 669–678. [Google Scholar]
- Wang, J.; Lai, S.; Li, F. Molding Process and Development of Large Carbon Fiber of Fan Blades with Composite Properties of Materials. In Proceedings of the International Conference on Environmental Science and Material Application (ESME 2012), Beijing, China, 13–14 October 2012; pp. 157–160. [Google Scholar]
- Song, Y.; Yang, L.; Guo, W.; Hua, L. A Survey on Key Technologies for Carbon Fiber-reinforced Plastics with Applications to Automobile Lightening. Mater. Rev. 2016, 30, 16–25, 50. [Google Scholar]
- Vijayan, D.S.; Sivasuriyan, A.; Devarajan, P.; Stefanska, A.; Wodzynski, L.; Koda, E. Carbon Fibre-Reinforced Polymer (CFRP) Composites in Civil Engineering Application—A Comprehensive Review. Buildings 2023, 13, 1509. [Google Scholar] [CrossRef]
- Akbar, A.; Kodur, V.; Liew, K. Microstructural changes and mechanical performance of cement composites reinforced with recycled carbon fibers. Cem. Concr. Compos. 2021, 121, 104069. [Google Scholar]
- Zhang, S.; Wang, Y.; Niu, D. Research Progress of the Application of Waste Fiber in Cement-based Materials. Mater. Rev. 2020, 34, 23042–23050. [Google Scholar]
- Lefeuvre, A.; Garnier, S.; Jacquemin, L.; Pillain, B.; Sonnemann, G. Anticipating in-use stocks of carbon fiber reinforced polymers and related waste flows generated by the commercial aeronautical sector until 2050. Resour. Conserv. Recycl. 2017, 125, 264–272. [Google Scholar]
- Akbar, A.; Liew, K.M. Assessing recycling potential of carbon fiber reinforced plastic waste in production of eco-efficient cement-based materials. J. Clean. Prod. 2020, 274, 123001. [Google Scholar]
- Song, Y.S.; Youn, J.R.; Gutowski, T.G. Life cycle energy analysis of fiber-reinforced composites. Compos. Part A Appl. Sci. Manuf. 2009, 40, 1257–1265. [Google Scholar]
- Meng, F.; Olivetti, E.A.; Zhao, Y.; Chang, J.C.; Pickering, S.J.; McKechnie, J. Comparing life cycle energy and global warming potential of carbon fiber composite recycling technologies and waste management options. ACS Sustain. Chem. Eng. 2018, 6, 9854–9865. [Google Scholar]
- Sun, H.; Guo, G.; Memon, S.A.; Xu, W.; Zhang, Q.; Zhu, J.-H.; Xing, F. Recycling of carbon fibers from carbon fiber reinforced polymer using electrochemical method. Compos. Part A Appl. Sci. Manuf. 2015, 78, 10–17. [Google Scholar]
- Jagadish, P.R.; Khalid, M.; Li, L.P.; Hajibeigy, M.T.; Amin, N.; Walvekar, R.; Chan, A. Cost effective thermoelectric composites from recycled carbon fibre: From waste to energy. J. Clean. Prod. 2018, 195, 1015–1025. [Google Scholar]
- Meng, F.; Cui, Y.; Pickering, S.; McKechnie, J. From aviation to aviation: Environmental and financial viability of closed-loop recycling of carbon fibre composite. Compos. Part B Eng. 2020, 200, 108362. [Google Scholar]
- Zhao, C.; Wang, Z.; Zhu, Z.; Guo, Q.; Wu, X.; Zhao, R. Research on different types of fiber reinforced concrete in recent years: An overview. Constr. Build. Mater. 2023, 365, 130075. [Google Scholar]
- Redon, C.; Li, V.C.; Wu, C.; Hoshiro, H.; Saito, T.; Ogawa, A. Measuring and modifying interface properties of PVA fibers in ECC matrix. J. Mater. Civ. Eng. 2001, 13, 399–406. [Google Scholar]
- Wu, J.; Xu, A.Q. Observation on Single Fiber Pull-Out Testing of Carbon Fiber Concrete with Scanning Electron Microscopy. Appl. Mech. Mater. 2015, 711, 434–437. [Google Scholar]
- Pi, Z.; Xiao, H.; Du, J.; Liu, M.; Li, H. Interfacial microstructure and bond strength of nano-SiO2-coated steel fibers in cement matrix. Cem. Concr. Compos. 2019, 103, 1–10. [Google Scholar]
- Si, W.; Cao, M.; Li, L. Establishment of fiber factor for rheological and mechanical performance of polyvinyl alcohol (PVA) fiber reinforced mortar. Constr. Build. Mater. 2020, 265, 120347. [Google Scholar]
- Liu, T.; Bai, R.; Chen, Z.; Li, Y.; Yang, Y. Tailoring of polyethylene fiber surface by coating silane coupling agent for strain hardening cementitious composite. Constr. Build. Mater. 2021, 278, 122263. [Google Scholar]
- Won, J.-P.; Lee, J.-H.; Lee, S.-J. Bonding behaviour of arch-type steel fibres in a cementitious composite. Compos. Struct. 2015, 133, 117–123. [Google Scholar]
- Zhang, Y.; Ju, J.W.; Xu, F.; Yan, Z.; Zhu, H. A novel micromechanical model of residual fracture energy of hooked-end steel fiber reinforced concrete exposed to high temperature. Constr. Build. Mater. 2021, 278, 122211. [Google Scholar]
- Wu, Z.; Shi, C.; He, W.; Wu, L. Effects of steel fiber content and shape on mechanical properties of ultra high performance concrete. Constr. Build. Mater. 2016, 103, 8–14. [Google Scholar]
- Lin, C.; Kanstad, T.; Jacobsen, S.; Ji, G. Bonding property between fiber and cementitious matrix: A critical review. Constr. Build. Mater. 2023, 378, 131169. [Google Scholar]
- Meng, J.; Zhong, J.; Xiao, H.; Ou, J. Enhancement of strength and ductility of cement-based composites by incorporating silica nanoparticle coated polyvinylalcohol fibers. AIP Adv. 2019, 9, 065108. [Google Scholar]
- He, S.; Qiu, J.; Li, J.; Yang, E.-H. Strain hardening ultra-high performance concrete (SHUHPC) incorporating CNF-coated polyethylene fibers. Cem. Concr. Res. 2017, 98, 50–60. [Google Scholar]
- Nguyen, H.; Carvelli, V.; Fujii, T.; Okubo, K. Cement mortar reinforced with reclaimed carbon fibres, CFRP waste or prepreg carbon waste. Constr. Build. Mater. 2016, 126, 321–331. [Google Scholar]
- Faneca, G.; Segura, I.; Torrents, J.M.; Aguado, A. Development of conductive cementitious materials using recycled carbon fibres. Cem. Concr. Compos. 2018, 92, 135–144. [Google Scholar]
- Yuan, H.; Zhang, S.; Lu, C.; He, S.; An, F. Improved interfacial adhesion in carbon fiber/polyether sulfone composites through an organic solvent-free polyamic acid sizing. Appl. Surf. Sci. 2013, 279, 279–284. [Google Scholar]
- Zheng, L.; Wang, Y.; Qin, J.; Wang, X.; Lu, R.; Qu, C.; Wang, C. Scalable manufacturing of carbon nanotubes on continuous carbon fibers surface from chemical vapor deposition. Vacuum 2018, 152, 84–90. [Google Scholar]
- Zhao, M.; Meng, L.; Ma, L.; Ma, L.; Yang, X.; Huang, Y.; Ryu, J.E.; Shankar, A.; Li, T.; Yan, C. Layer-by-layer grafting CNTs onto carbon fibers surface for enhancing the interfacial properties of epoxy resin composites. Compos. Sci. Technol. 2018, 154, 28–36. [Google Scholar] [CrossRef]
- Vazquez, M.; Marin, P.; Davies, H.A.; Olofinjana, A.O. Magnetic hardening of FeSiBCuNb ribbons and wires during the first stage of crystallization to a nanophase structure. Appl. Phys. Lett. 1994, 64, 3184–3186. [Google Scholar] [CrossRef]
- Zhou, A.; Yu, Z.; Wei, H.; Tam, L.-H.; Liu, T.; Zou, D. Understanding the Toughening Mechanism of Silane Coupling Agents in the Interfacial Bonding in Steel Fiber-Reinforced Cementitious Composites. ACS Appl. Mater. Interfaces 2020, 12, 44163–44171. [Google Scholar] [CrossRef]
- Homoro, O.; Michel, M.; Baranger, T.N. Pull-out response of glass yarn from ettringite matrix: Effect of pre-impregnation and embedded length. Compos. Sci. Technol. 2019, 170, 174–182. [Google Scholar] [CrossRef]
- Katz, A.; Li, V.C. Bond properties of micro-fibers in cementitious matrix. MRS Online Proc. Libr. (OPL) 1994, 370, 529. [Google Scholar] [CrossRef]
- Qiu, J.; Lim, X.-N.; Yang, E.-H. Fatigue-induced deterioration of the interface between micro-polyvinyl alcohol (PVA) fiber and cement matrix. Cem. Concr. Res. 2016, 90, 127–136. [Google Scholar] [CrossRef]
- Kang, N.; Yang, S.; Xiong, X.; Han, A.; Ren, R.; Wang, J. The Performance and Synthesis of Alkynyl−Functionalized Benzoxazine Containing Phthalide Side Groups and Cyano Groups with Different Molecular Weights. Polymers 2023, 15, 3478. [Google Scholar] [CrossRef] [PubMed]
- Islam, M.S.; Deng, Y.; Tong, L.; Faisal, S.N.; Roy, A.K.; Minett, A.I.; Gomes, V.G. Grafting carbon nanotubes directly onto carbon fibers for superior mechanical stability: Towards next generation aerospace composites and energy storage applications. Carbon 2016, 96, 701–710. [Google Scholar] [CrossRef]
- Wang, Z.; Bai, E.; Ren, B.; Liu, C.; Zhou, J. Mechanical properties of concrete modified by graphene oxide grafted carbon fiber reinforcement. Acta Mater. Compos. Sin. 2024, 41, 5504–5515. [Google Scholar]
- Pathak, A.K.; Borah, M.; Gupta, A.; Yokozeki, T.; Dhakate, S.R. Improved mechanical properties of carbon fiber/graphene oxide-epoxy hybrid composites. Compos. Sci. Technol. 2016, 135, 28–38. [Google Scholar] [CrossRef]
- Liu, S.; Luo, D.; He, M.; Qi, S. In situ crosslinking of silica aerogel with reactive carbon fiber for high mechanical property, thermal insulation and superhydrophobicity. Microporous Mesoporous Mater. 2024, 377, 113203. [Google Scholar] [CrossRef]
- Liu, J.; Ye, H.; Liu, Y.; Liu, Y.; Xu, X.; Chen, Z.; Wang, C.; Liang, B. Study on the properties of carbon fiber-reinforced polyurethane composite treated by electrochemical oxidation/silane coupling agent. New Chem. Mater. 2024, 52, 113–118, 123. [Google Scholar]
- Wang, Q.; Pu, L.; Jia, C.; Li, Z.; Li, J. Inhomogeneity of Interface Modification of Carbon Fiber/Epoxy Composites. Chin. J. Mater. Res. 2023, 37, 668–674. [Google Scholar]
- Zhang, Y.; Wu, Y.; Wei, W.; Chen, S.; Li, J.; Yang, Z.; Gao, G.; Wu, G. Multiscale enhancement of carbon/carbon composite performance by self-assembly of sulfonated graphene with silane-treated carbon fibers. Chem. Eng. J. 2024, 491, 152182. [Google Scholar] [CrossRef]
- Tiwari, S.; Bijwe, J. Surface treatment of carbon fibers—A review. Procedia Technol. 2014, 14, 505–512. [Google Scholar] [CrossRef]
- Osbeck, S.; Bradley, R.H.; Liu, C.; Idriss, H.; Ward, S. Effect of an ultraviolet/ozone treatment on the surface texture and functional groups on polyacrylonitrile carbon fibres. Carbon 2011, 49, 4322–4330. [Google Scholar] [CrossRef]
- Su, Y.-N.; Zhang, S.-C.; Zhang, X.-H.; Jing, D.-Q. Effect of surface functionalization on the surface and interfacial properties of thermoplastic-coated carbon fibers. Carbon 2022, 188, 547. [Google Scholar] [CrossRef]
- Li, H.; Liebscher, M.; Zhao, D.; Yin, B.; Du, Y.; Yang, J.; Kaliske, M.; Mechtcherine, V. A review of carbon fiber surface modification methods for tailor-made bond behavior with cementitious matrices. Prog. Mater. Sci. 2023, 132, 101040. [Google Scholar] [CrossRef]
- Zhong, Y.; Wang, T.; Yan, M.; Huang, X.; Zhou, X. A one-step hot pressing molding method of polyacrylonitrile carbon fibers: Influence on surface morphology, microstructure and mechanical property. J. Mater. Sci. 2022, 57, 2277–2291. [Google Scholar] [CrossRef]
- Kanda, T.; Li, V.C. Interface property and apparent strength of high-strength hydrophilic fiber in cement matrix. J. Mater. Civ. Eng. 1998, 10, 5–13. [Google Scholar] [CrossRef]
- Zhang, W.; Deng, X.; Sui, G.; Yang, X. Improving interfacial and mechanical properties of carbon nanotube-sized carbon fiber/epoxy composites. Carbon 2019, 145, 629–639. [Google Scholar]
- Akers, S.; Studinka, J.; Meier, P.; Dobb, M.; Johnson, D.; Hikasa, J. Long term durability of PVA reinforcing fibres in a cement matrix. Int. J. Cem. Compos. Lightweight Concr. 1989, 11, 79–91. [Google Scholar] [CrossRef]
- Qiu, J.; Yang, E.-H. A micromechanics-based fatigue dependent fiber-bridging constitutive model. Cem. Concr. Res. 2016, 90, 117–126. [Google Scholar] [CrossRef]
- Leung, C.K.; Ybanez, N. Pullout of inclined flexible fiber in cementitious composite. J. Eng. Mech. 1997, 123, 239–246. [Google Scholar] [CrossRef]
- Li, V.C.; Wang, Y.; Backer, S. Effect of inclining angle, bundling and surface treatment on synthetic fibre pull-out from a cement matrix. Composites 1990, 21, 132–140. [Google Scholar] [CrossRef]
- Wang, Y.; Backer, S.; Li, V.C. A statistical tensile model of fibre reinforced cementitious composites. Composites 1989, 20, 265–274. [Google Scholar] [CrossRef]
- Yang, E.-H.; Wang, S.; Yang, Y.; Li, V.C. Fiber-bridging constitutive law of engineered cementitious composites. J. Adv. Concr. Technol. 2008, 6, 181–193. [Google Scholar] [CrossRef]
- Lu, C.; Leung, C.K. A new model for the cracking process and tensile ductility of strain hardening cementitious composites (SHCC). Cem. Concr. Res. 2016, 79, 353–365. [Google Scholar]
- Du, M.; Li, Q. Meso-models for synthetic fiber reinforced concrete under uniaxial tension. J. Hydroelectr. Eng. 2005, 24, 21–25. [Google Scholar]
- Li, V.C.; Wang, Y.; Backer, S. A micromechanical model of tension-softening and bridging toughening of short random fiber reinforced brittle matrix composites. J. Mech. Phys. Solids 1991, 39, 607–625. [Google Scholar] [CrossRef]
- Ma, H.; Cai, J.; Lin, Z.; Qian, S.; Li, V.C. CaCO3 whisker modified Engineered Cementitious Composite with local ingredients. Constr. Build. Mater. 2017, 151, 1–8. [Google Scholar] [CrossRef]
- Yang, E.-H.; Yang, Y.; Li, V.C. Use of high volumes of fly ash to improve ECC mechanical properties and material greenness. ACI Mater. J. 2007, 104, 620. [Google Scholar]



| Heat Loss (%) | Specific Surface Area (m2/kg) | Initial Setting Time (min) | 28-Day Flexural Strength (MPa) | 28-Day Compressive Strength (MPa) |
|---|---|---|---|---|
| 3.62 | 354 | 216 | 8.6 | 52.6 |
| Length (mm) | Diameter (μm) | Carbon Content (%) | Density (g·cm−3) | Tensile Strength (MPa) | Tensile Modulus (GPa) | |
|---|---|---|---|---|---|---|
| VCF | 18 | 7 | 96 | 1.8 | 3450 | 230 |
| RCF | 18 | 7.28 | 92.8 | 1.79 | 2866 | 230 |
| Cement | Water | Sand | Carbon Fiber (vol.%) | Water Reducer (g) | Flowability (mm) | |
|---|---|---|---|---|---|---|
| CF0 | 1 | 0.5 | 1.5 | 0 | 0.5 | 250 |
| RCF0.16 | 1 | 0.5 | 1.5 | 0.16 | 0.5 | 232.5 |
| RCF0.30 | 1 | 0.5 | 1.5 | 030 | 0.5 | 214 |
| RCF0.47 | 1 | 0.5 | 1.5 | 0.47 | 0.5 | 203 |
| VCF0.16 | 1 | 0.5 | 1.5 | 0.16 | 0.5 | 222.5 |
| VCF0.30 | 1 | 0.5 | 1.5 | 0.30 | 0.5 | 209 |
| VCF0.47 | 1 | 0.5 | 1.5 | 0.47 | 0.5 | 190 |
| Specimen Number | |||||
|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | |
| RCF | 0.015 | 0.007 | 0.007 | 0.015 | 0.006 |
| VCF | 0.014 | 0.032 | 0.004 | 0.014 | 0.017 |
(MPa) | (MPa) | RCF Error Mean | (MPa) | VCF Error Mean | ||
|---|---|---|---|---|---|---|
| Vf = 0.16% | 1.15 | 0.98 | 17.3% | 1.59 | 1.35 | 17.7% |
| Vf = 0.3% | 2.10 | 1.83 | 14.8% | 2.91 | 2.52 | 15.5% |
| Vf = 0.47% | 3.24 | 2.86 | 13.3% | 4.46 | 3.95 | 12.9% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shi, T.; Li, K.; Shao, S.; Cai, X.; Wang, X.; Pan, C.; Fu, N.; Wang, H. Performance Characterization of Recycled Carbon Fiber and Its Interfacial Bonding Properties with Cement Matrix. Materials 2025, 18, 1532. https://doi.org/10.3390/ma18071532
Shi T, Li K, Shao S, Cai X, Wang X, Pan C, Fu N, Wang H. Performance Characterization of Recycled Carbon Fiber and Its Interfacial Bonding Properties with Cement Matrix. Materials. 2025; 18(7):1532. https://doi.org/10.3390/ma18071532
Chicago/Turabian StyleShi, Tao, Kunming Li, Sijie Shao, Xuanfeng Cai, Xinpeng Wang, Chonggen Pan, Ning Fu, and Haibo Wang. 2025. "Performance Characterization of Recycled Carbon Fiber and Its Interfacial Bonding Properties with Cement Matrix" Materials 18, no. 7: 1532. https://doi.org/10.3390/ma18071532
APA StyleShi, T., Li, K., Shao, S., Cai, X., Wang, X., Pan, C., Fu, N., & Wang, H. (2025). Performance Characterization of Recycled Carbon Fiber and Its Interfacial Bonding Properties with Cement Matrix. Materials, 18(7), 1532. https://doi.org/10.3390/ma18071532





