Controllable pH Manipulations in Micro/Nanofluidic Device Using Nanoscale Electrokinetics
Abstract
1. Introduction
2. Experimental Methods
2.1. Device Fabrication
2.2. Chemical Preparation
2.3. Measurements
2.4. Image Analysis
3. Results and Discussions
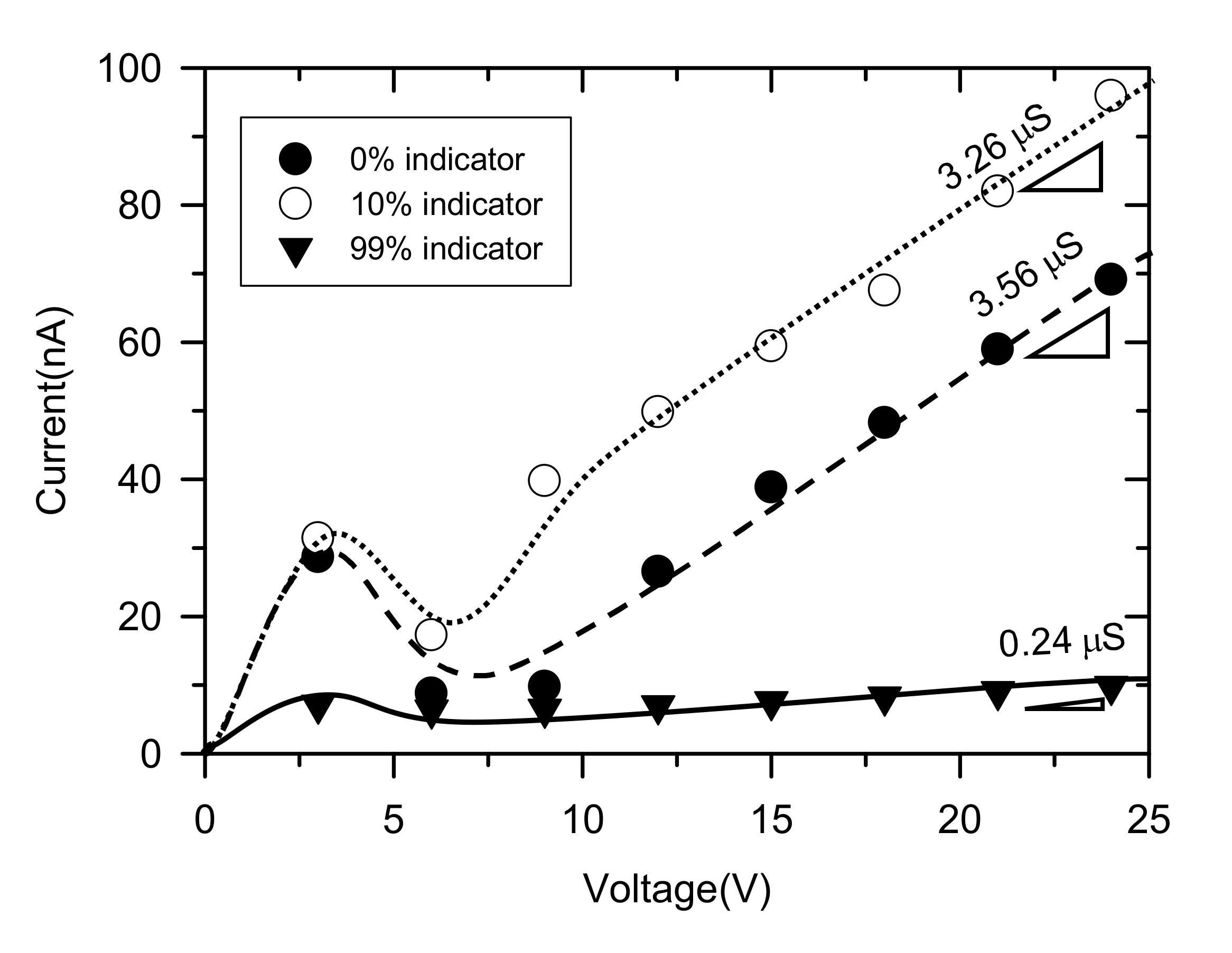
3.1. Effect of pH Indicator’s Concentration
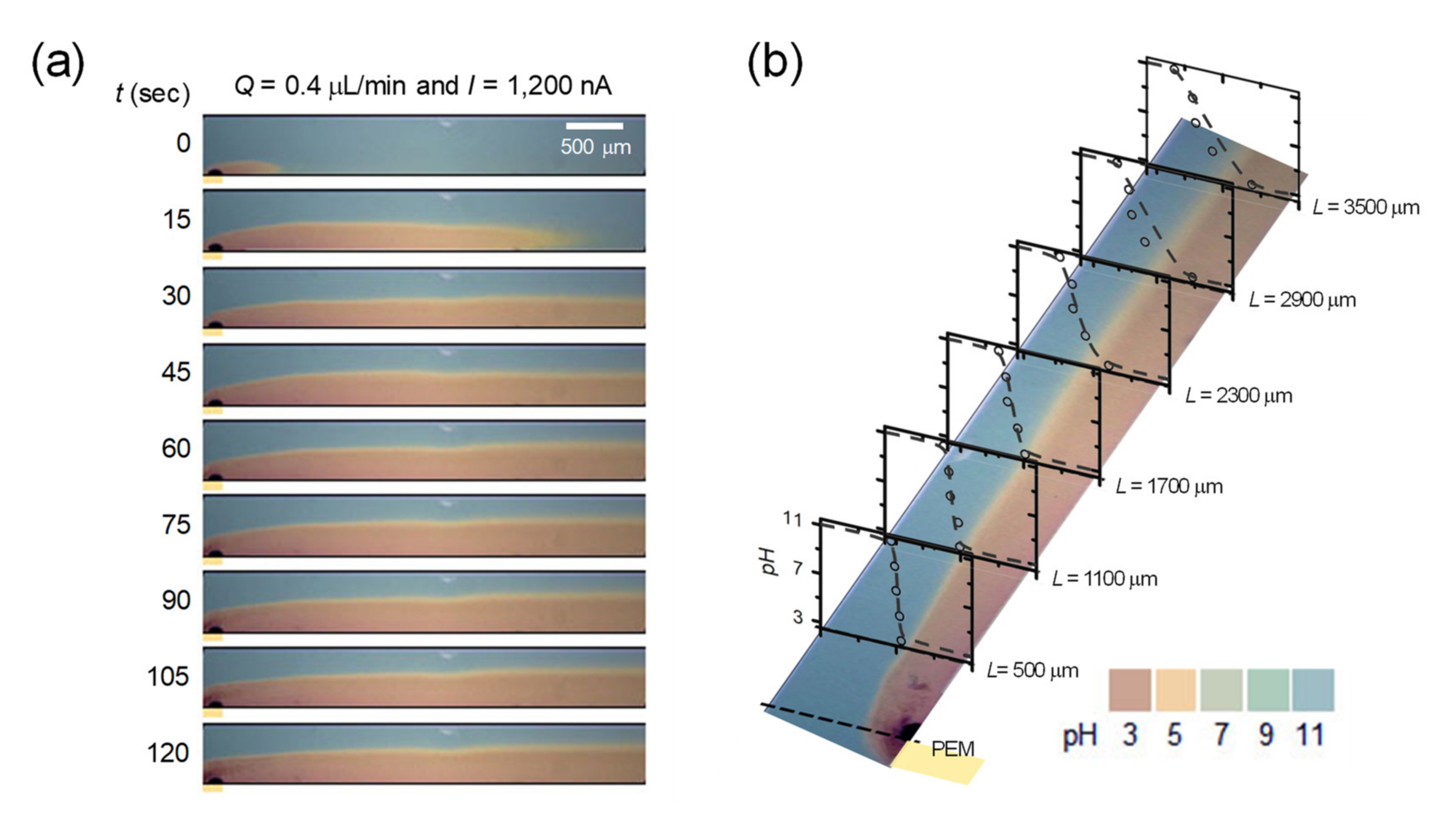
3.2. pH Gradient Generation by Proton Mass Transport
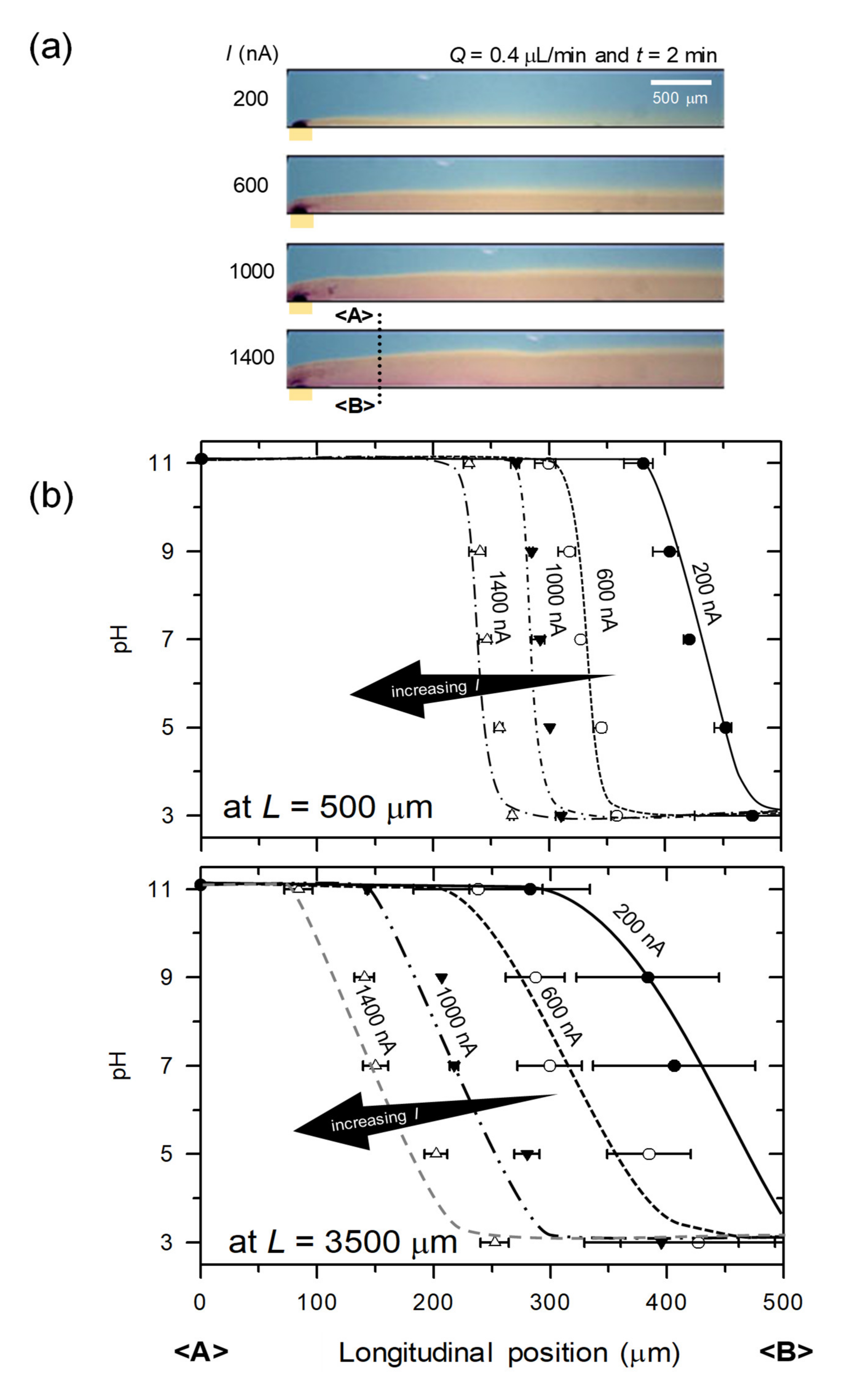
3.3. Tunable pH Gradient Generation by External Input Flow Rate
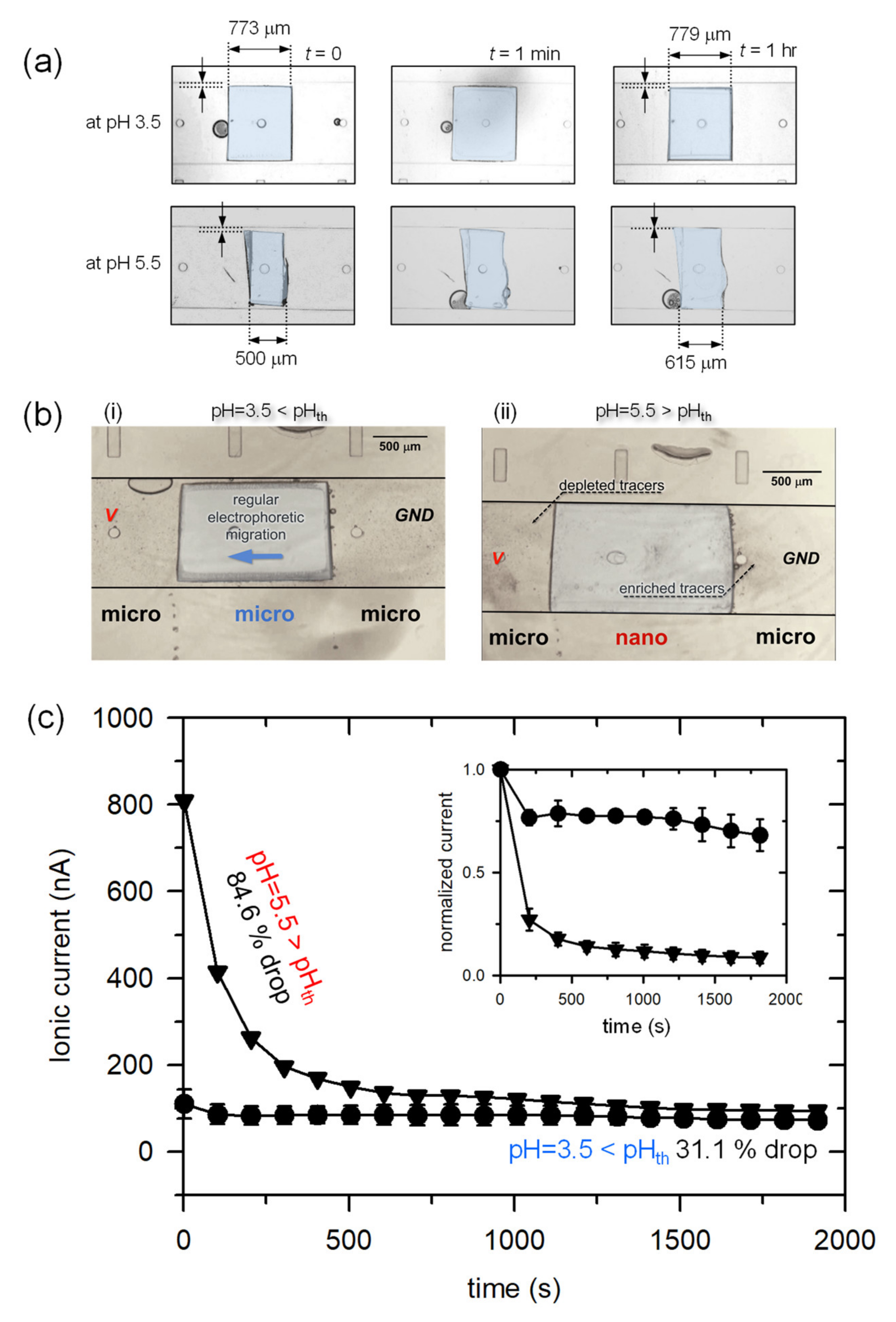
3.4. Nanofluidic Sensing of pH Stimulation
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Kim, S.J.; Ko, S.H.; Kang, K.H.; Han, J. Direct seawater desalination by ion concentration polarization. Nat. Nanotechnol. 2010, 5, 297–301. [Google Scholar] [CrossRef]
- Pu, Q.; Yun, J.; Temkin, H.; Liu, S. Ion-Enrichment and Ion-Depletion Effect of Nanochannel Structures. Nano Lett. 2004, 4, 1099–1103. [Google Scholar] [CrossRef]
- Probstein, R.F. Physicochemical Hydrodynamics: An Introduction; Wiley-Interscience: Hoboken, NJ, USA, 1994. [Google Scholar]
- Kim, S.J.; Wang, Y.-C.; Lee, J.H.; Jang, H.; Han, J. Concentration polarization and nonlinear electrokinetic flow near a nanofluidic channel. Phys. Rev. Lett. 2007, 99, 044501. [Google Scholar] [CrossRef] [PubMed]
- Rubinstein, I.; Zaltzman, B. Equilibrium Electroconvective Instability. Phys. Rev. Lett. 2015, 114, 114502. [Google Scholar] [CrossRef] [PubMed]
- Demekhin, E.A.; Nikitin, N.V.; Shelistov, V.S. Three-dimensional coherent structures of electrokinetic instability. Phys. Rev. E 2014, 90, 013031. [Google Scholar] [CrossRef] [PubMed]
- Druzgalski, C.L.; Andersen, M.B.; Mani, A. Direct numerical simulation of electroconvective instability and hydrodynamic chaos near an ion-selective surface. Phys. Fluids 2013, 25, 110804. [Google Scholar] [CrossRef]
- Yossifon, G.; Chang, H. Changing nanoslot ion flux with a dynamic nanocolloid ion-selective filter: Secondary overlimiting currents due to nanocolloid-nanoslot interaction. Phys. Rev. E 2010, 81, 066317. [Google Scholar] [CrossRef]
- Rubinstein, S.M.; Manukyan, G.; Staicu, A.; Rubinstein, I.; Zaltzman, B.; Lammertink, R.G.H.; Mugele, F.; Wessling, M. Direct observation of a nonequilibrium electro-osmotic instability. Phys. Rev. Lett. 2008, 101, 236101. [Google Scholar] [CrossRef]
- Zaltzman, B.; Rubinstein, I. Electro-osmotic slip and electroconvective instability. J. Fluid Mech. 2007, 579, 173. [Google Scholar] [CrossRef]
- Rubinstein, I.; Zaltzman, B. Electro-convective versus electroosmotic instability in concentration polarization. Adv. Colloid Interface Sci. 2007, 134, 190–200. [Google Scholar] [CrossRef]
- Dydek, E.V.; Zaltzman, B.; Rubinstein, I.; Deng, D.; Mani, A.; Bazant, M.Z. Overlimiting Current in a Microchannel. Phys. Rev. Lett. 2011, 107, 118301. [Google Scholar] [CrossRef]
- Sohn, S.; Cho, I.; Kwon, S.; Lee, H.; Kim, S.J. Surface Conduction in a Microchannel. Langmuir 2018, 34, 7916–7921. [Google Scholar] [CrossRef] [PubMed]
- Nam, S.; Cho, I.; Heo, J.; Lim, G.; Bazant, M.Z.; Moon, D.J.; Sung, G.Y.; Kim, S.J. Experimental Verification of Overlimiting Current by Surface Conduction and Electro-Osmotic Flow in Microchannels. Phys. Rev. Lett. 2015, 114, 114501. [Google Scholar] [CrossRef] [PubMed]
- Huh, K.; Yang, S.-Y.; Park, J.S.; Lee, J.A.; Lee, H.; Kim, S.J. Surface conduction and electroosmotic flow around charged dielectric pillar arrays in microchannels. Lab Chip 2020, 20, 675–686. [Google Scholar] [CrossRef] [PubMed]
- Lee, H.; Alizadeh, S.; Kim, T.J.; Park, S.-M.; Soh, H.T.; Mani, A.; Kim, S.J. Overlimiting current in non-uniform arrays of microchannels. arXiv 2019, arXiv:1910.09546. [Google Scholar]
- Kim, K.; Kim, W.; Lee, H.; Kim, S.J. Stabilization of ion concentration polarization layer using micro fin structure for high-throughput applications. Nanoscale 2017, 9, 3466–3475. [Google Scholar] [CrossRef] [PubMed]
- Alizadeh, S.; Bazant, M.Z.; Mani, A. Impact of network heterogeneity on electrokinetic transport in porous media. J. Colloid Interface Sci. 2019, 553, 451–464. [Google Scholar] [CrossRef]
- Alizadeh, S.; Mani, A. Multiscale Model for Electrokinetic Transport in Networks of Pores, Part II: Computational Algorithms and Applications. Langmuir 2017, 33, 6220–6231. [Google Scholar] [CrossRef]
- Alizadeh, S.; Mani, A. Multiscale Model for Electrokinetic Transport in Networks of Pores, Part I: Model Derivation. Langmuir 2017, 33, 6205–6219. [Google Scholar] [CrossRef]
- Kim, S.J.; Song, Y.-A.; Han, J. Nanofluidic concentration devices for biomolecules utilizing ion concentration polarization: Theory, fabrication, and applications. Chem. Soc. Rev. 2010, 39, 912–922. [Google Scholar] [CrossRef]
- Son, S.Y.; Lee, S.; Lee, H.; Kim, S.J. Engineered nanofluidic preconcentration devices by ion concentration polarization. BioChip J. 2016, 10, 251–261. [Google Scholar] [CrossRef]
- Huang, K.-D.; Yang, R.-J. A nanochannel-based concentrator utilizing the concentration polarization effect. Electrophoresis 2008, 29, 4862–4870. [Google Scholar] [CrossRef] [PubMed]
- Kwak, R.; Kim, S.J.; Han, J. Continuous-Flow Biomolecule and Cell Concentrator by Ion Concentration Polarization. Anal. Chem. 2011, 83, 7348–7355. [Google Scholar] [CrossRef] [PubMed]
- Kim, B.; Kwon, H.J.; Ko, S.H.; Lim, G.; Han, J. Partial desalination of hypersaline brine by lab-scale ion concentration polarization device. Desalination 2017, 412, 20–31. [Google Scholar] [CrossRef]
- Park, S.; Jung, Y.; Son, S.Y.; Cho, I.; Cho, Y.; Lee, H.; Kim, H.-Y.; Kim, S.J. Capillarity ion concentration polarization as spontaneous desalting mechanism. Nat. Commun. 2016, 7, 11223. [Google Scholar] [CrossRef] [PubMed]
- Knust, K.N.; Hlushkou, D.; Anand, R.K.; Tallarek, U.; Crooks, R.M. Electrochemically mediated seawater desalination. Angew. Chem. Int. Ed. 2013, 52, 8107–8110. [Google Scholar] [CrossRef] [PubMed]
- Kim, S.J.; Ko, S.H.; Kwak, R.; Posner, J.D.; Kang, K.H.; Han, J. Multi-vortical flow inducing electrokinetic instability in ion concentration polarization layer. Nanoscale 2012, 4, 7406. [Google Scholar] [CrossRef]
- Kim, P.; Kim, S.J.; Han, J.; Suh, K.Y. Stabilization of Ion Concentration Polarization Using a Heterogeneous Nanoporous Junction. Nano Lett. 2010, 10, 16–23. [Google Scholar] [CrossRef][Green Version]
- Green, Y.; Shloush, S.; Yossifon, G. Effect of geometry on concentration polarization in realistic heterogeneous permselective systems. Phys. Rev. E 2014, 89, 043015. [Google Scholar] [CrossRef]
- Green, Y.; Yossifon, G. Effects of three-dimensional geometric field focusing on concentration polarization in a heterogeneous permselective system. Phys. Rev. E 2014, 89, 013024. [Google Scholar] [CrossRef]
- Zeng, Z.; Yeh, L.-H.; Zhang, M.; Qian, S. Ion transport and selectivity in biomimetic nanopores with pH-tunable zwitterionic polyelectrolyte brushes. Nanoscale 2015, 7, 17020–17029. [Google Scholar] [CrossRef] [PubMed]
- Yeh, L.-H.; Zhang, M.; Qian, S. Ion Transport in a pH-Regulated Nanopore. Anal. Chem. 2013, 85, 7527–7534. [Google Scholar] [CrossRef] [PubMed]
- Yeh, L.-H.; Zhang, M.; Qian, S.; Hsu, J.-P. Regulating DNA translocation through functionalized soft nanopores. Nanoscale 2012, 4, 2685. [Google Scholar] [CrossRef] [PubMed]
- Yeh, L.-H.; Zhang, M.; Qian, S.; Hsu, J.-P.; Tseng, S. Ion Concentration Polarization in Polyelectrolyte-Modified Nanopores. J. Phys. Chem. C 2012, 116, 8672–8677. [Google Scholar] [CrossRef]
- Kim, W.; Oh, J.; Kwon, S.; Kim, K.; Kim, S.J. Quantifying the pH shift induced by selective anodic electrochemical reactions in the ion concentration polarization phenomenon. Lab Chip 2019, 19, 1359–1369. [Google Scholar] [CrossRef]
- Kim, W.; Park, S.; Kim, K.; Kim, S.J. Experimental verification of simultaneous desalting and molecular preconcentration by ion concentration polarization. Lab Chip 2017, 17, 3841–3850. [Google Scholar] [CrossRef]
- Cho, I.; Kim, W.; Lee, H. Non-Negligible Diffusio-Osmosis Inside an Ion Concentration Polarization Layer. Phys. Rev. Lett. 2016, 116, 254501. [Google Scholar] [CrossRef]
- Choi, J.; Lee, H.; Kim, S.J. Hierarchical micro/nanoporous ion-exchangeable sponge. Lab Chip 2019, 20, 505–513. [Google Scholar] [CrossRef]
- Cho, Y.; Yoon, J.; Lim, D.W.; Kim, J.; Lee, J.H.; Chung, S. Ion concentration polarization for pre-concentration of biological samples without pH change. Anal. 2016, 141, 6510–6514. [Google Scholar] [CrossRef]
- Chun, H. Inside Front Cover: Electropreconcentration-induced local pH change. Electrophoresis 2018, 39, 521–525. [Google Scholar] [CrossRef]
- Andersen, M.B.; Wang, K.M.; Schiffbauer, J.; Mani, A. Confinement effects on electroconvective instability. Electrophoresis 2016, 38, 702–711. [Google Scholar] [CrossRef] [PubMed]
- Storey, B.D.; Zaltzman, B.; Rubinstein, I. Bulk electroconvective instability at high Péclet numbers. Phys. Rev. E 2007, 76, 76. [Google Scholar] [CrossRef] [PubMed]
- Pundik, T.; Rubinstein, I.; Zaltzman, B. Bulk electroconvection in electrolyte. Phys. Rev. E 2005, 72, 061502. [Google Scholar] [CrossRef] [PubMed]
- Duffy, D.C.; McDonald, J.C.; Schueller, O.J.A.; Whitesides, G.M. Rapid Prototyping of Microfluidic Systems in Poly(dimethylsiloxane). Anal. Chem. 1998, 70, 4974–4984. [Google Scholar] [CrossRef]
- Cho, I.; Sung, G.Y.; Kim, S.J. Overlimiting current through ion concentration polarization layer: Hydrodynamic convection effects. Nanoscale 2014, 6, 4620. [Google Scholar] [CrossRef]
- Krol, J.; Wessling, M.; Strathmann, H. Concentration polarization with monopolar ion exchange membranes: Current–voltage curves and water dissociation. J. Membr. Sci. 1999, 162, 145–154. [Google Scholar] [CrossRef]
- Strathmann, H.; Krol, J.; Rapp, H.-J.; Eigenberger, G. Limiting current density and water dissociation in bipolar membranes. J. Membr. Sci. 1997, 125, 123–142. [Google Scholar] [CrossRef]
- Program to Change RGB Color Model to HSV Color Model. Available online: https://www.geeksforgeeks.org/program-change-rgb-color-model-hsv-color-model/ (accessed on 10 October 2019).
- Lee, H.; Kim, J.; Yang, J.; Seo, S.W.; Kim, S.J. Diffusiophoretic exclusion of colloidal particles for continuous water purification. Lab Chip 2018, 18, 1713–1724. [Google Scholar] [CrossRef]
- Lee, J.A.; Lee, D.; Park, S.; Lee, H.; Kim, S.J. Non-negligible Water-permeance through Nanoporous Ion Exchange Medium. Sci. Rep. 2018, 8, 12842. [Google Scholar] [CrossRef]
- Kim, S.J.; Li, L.D.; Han, J. Amplified Electrokinetic Response by Concentration Polarization near Nanofluidic Channel. Langmuir 2009, 25, 7759–7765. [Google Scholar] [CrossRef]
- Jin, X.; Joseph, S.; Gatimu, E.N.; Bohn, P.W.; Aluru, N.R. Induced Electrokinetic Transport in Micro−Nanofluidic Interconnect Devices. Langmuir 2007, 23, 13209–13222. [Google Scholar] [CrossRef] [PubMed]
- Zangle, T.; Mani, A.; Santiago, J.G. Theory and experiments of concentration polarization and ion focusing at microchannel and nanochannel interfaces. Chem. Soc. Rev. 2010, 39, 1014. [Google Scholar] [CrossRef] [PubMed]
- Kim, J.; Cho, I.; Lee, H.; Kim, S.J. Ion Concentration Polarization by Bifurcated Current Path. Sci. Rep. 2017, 7, 5091. [Google Scholar] [CrossRef] [PubMed]
- Lee, S.; Park, S.; Kim, W.; Moon, S.; Kim, H.-Y.; Lee, H.; Kim, S.J. Nanoelectrokinetic bufferchannel-less radial preconcentrator and online extractor by tunable ion depletion layer. Biomicrofluidics 2019, 13, 034113. [Google Scholar] [CrossRef]
- Ko, S.H.; Song, Y.-A.; Kim, S.J.; Kim, M.; Han, J.; Kang, K.H. Nanofluidic preconcentration device in a straight microchannel using ion concentration polarization. Lab Chip 2012, 12, 4472. [Google Scholar] [CrossRef]
- Kim, M.; Jia, M.; Kim, T. Ion concentration polarization in a single and open microchannel induced by a surface-patterned perm-selective film. Analyst 2013, 138, 1370. [Google Scholar] [CrossRef]
- Lee, D.; Lee, J.A.; Lee, H.; Kim, S.J. Spontaneous Selective Preconcentration Leveraged by Ion Exchange and Imbibition through Nanoporous Medium. Sci. Rep. 2019, 9, 2336. [Google Scholar] [CrossRef]




© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Park, J.S.; Oh, J.; Kim, S.J. Controllable pH Manipulations in Micro/Nanofluidic Device Using Nanoscale Electrokinetics. Micromachines 2020, 11, 400. https://doi.org/10.3390/mi11040400
Park JS, Oh J, Kim SJ. Controllable pH Manipulations in Micro/Nanofluidic Device Using Nanoscale Electrokinetics. Micromachines. 2020; 11(4):400. https://doi.org/10.3390/mi11040400
Chicago/Turabian StylePark, Jae Suk, Jeewhan Oh, and Sung Jae Kim. 2020. "Controllable pH Manipulations in Micro/Nanofluidic Device Using Nanoscale Electrokinetics" Micromachines 11, no. 4: 400. https://doi.org/10.3390/mi11040400
APA StylePark, J. S., Oh, J., & Kim, S. J. (2020). Controllable pH Manipulations in Micro/Nanofluidic Device Using Nanoscale Electrokinetics. Micromachines, 11(4), 400. https://doi.org/10.3390/mi11040400




