Amplitude-Phase Variation in a Graphene-Based Microstrip Line
Abstract
:1. Introduction
2. Materials and Methods
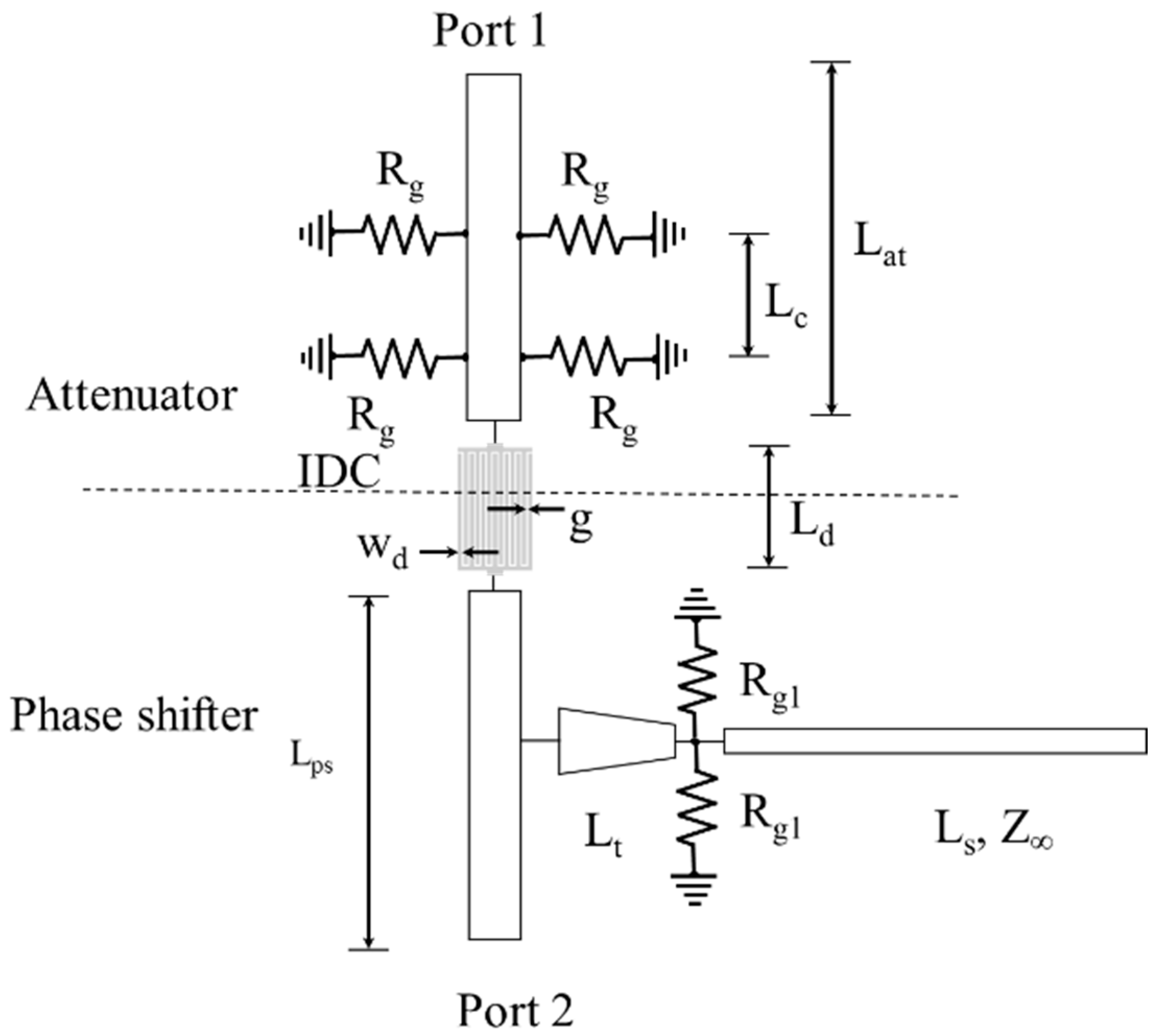
2.1. Design of the Amplitude Phase Variation Transmission Line
2.2. Finite Element Modelling
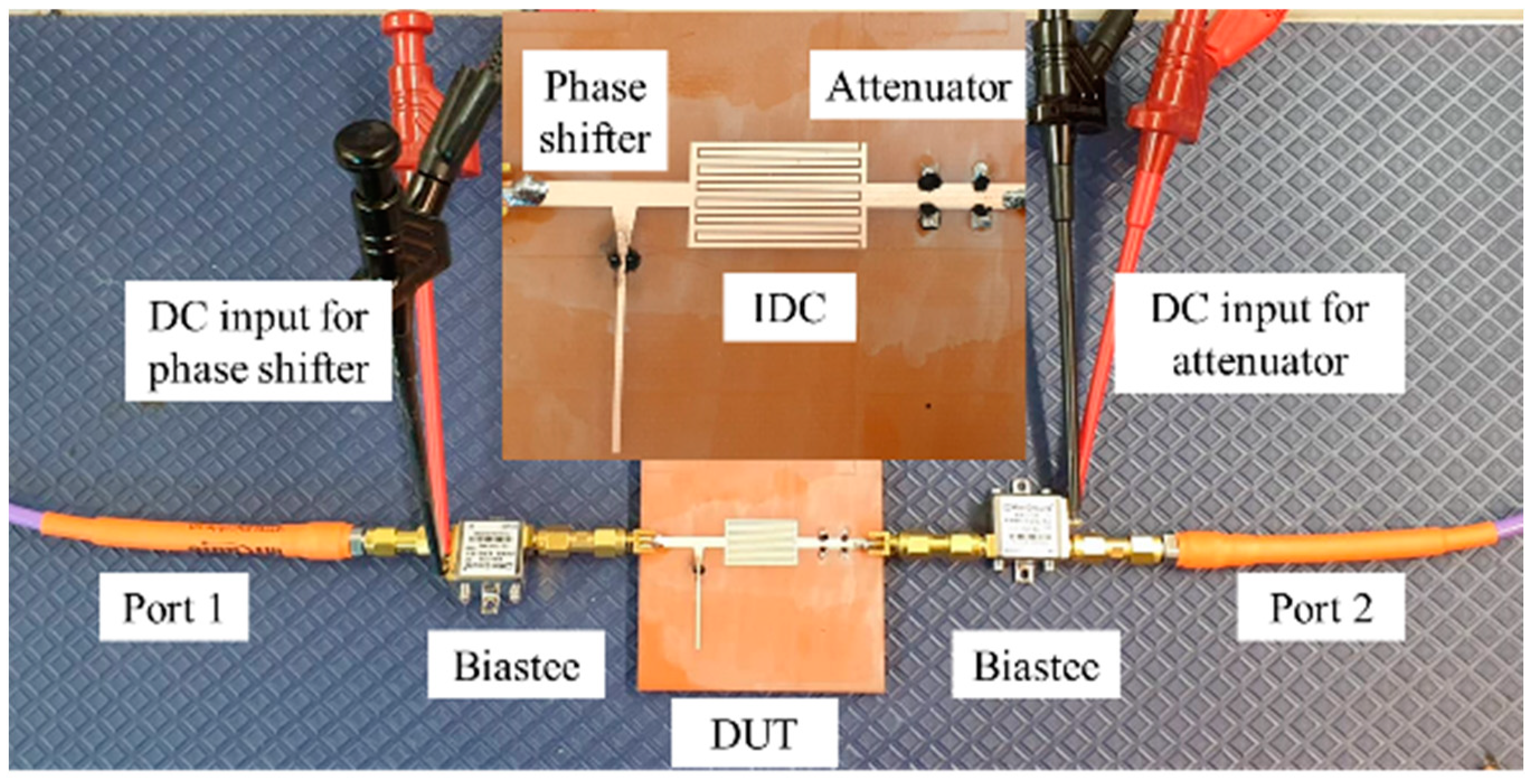
2.3. Fabrication of the Prototype and Scattering Parameter Measurements
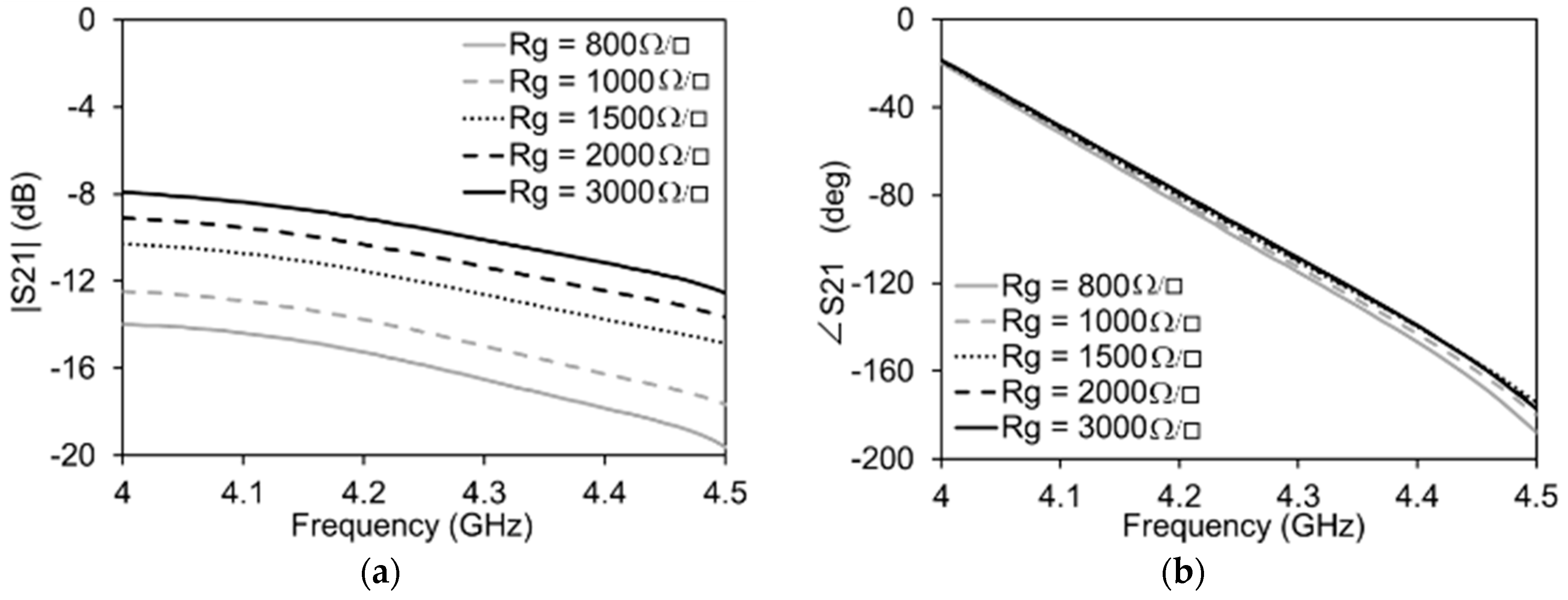
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Dragoman, M.; Neculoiu, D.; Dragoman, D.; Deligeorgis, G.; Konstantinidis, G.; Cismaru, A.; Coccetti, F.; Plana, R. Graphene for microwaves. IEEE Microw. Mag. 2010, 11, 81–86. [Google Scholar] [CrossRef]
- Quhe, R.; Ma, J.; Zeng, Z.; Tang, K.; Zheng, J.; Wang, Y.; Ni, Z.; Wang, L.; Gao, Z.; Shi, J.; et al. Tunable band gap in few-layer graphene by surface adsorption. Sci. Rep. 2013, 3, 1794. [Google Scholar] [CrossRef] [Green Version]
- Balci, O.; Polat, E.O.; Kakenov, N.; Kocabas, C. Graphene-enabled electrically switchable radar-absorbing surfaces. Nat. Commun. 2015, 6, 6628. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Dragoman, M.; Coccetti, F.; Plana, R.; Muller, A.A. Microwave switches based on graphene. J. Appl. Phys. 2009, 105, 054309. [Google Scholar] [CrossRef]
- Dragoman, M.; Aldrigo, M.; Dragoman, D. Perspectives on Atomic-Scale Switches for High-Frequency Applications Based on Nanomaterials. Nanomaterials 2021, 11, 625. [Google Scholar] [CrossRef]
- Sa’don SN, H.; Jamaluddin, M.H.; Kamarudin, M.R.; Ahmad, F.; Yamada, Y.; Kamardin, K.; Idris, I.H.; Seman, N. Characterisation of tunable graphene antenna. AEU-Int. J. Electron. Commun. 2020, 118, 153170. [Google Scholar] [CrossRef]
- Yasir, M.; Bistarelli, S.; Cataldo, A.; Bozzi, M.; Perregrini, L.; Bellucci, S. Voltage-Controlled and Input-Matched Tunable Microstrip Attenuators Based on Few-Layer Graphene. IEEE Trans. Microw. Theory Tech. 2019, 68, 701–710. [Google Scholar] [CrossRef]
- Yi, Y.; Zhang, A.-Q. A Tunable Graphene Filtering Attenuator Based on Effective Spoof Surface Plasmon Polariton Waveguide. IEEE Trans. Microw. Theory Tech. 2020, 68, 5169–5177. [Google Scholar] [CrossRef]
- Yasir, M.; Savi, P. Commercial graphene nanoplatelets-based tunable attenuator. Electron. Lett. 2020, 56, 184–187. [Google Scholar] [CrossRef]
- Yasir, M.; Bozzi, M.; Perregrini, L.; Bistarelli, S.; Cataldo, A.; Bellucci, S. Innovative tunable microstrip attenuators based on few-layer graphene flakes. In Proceedings of the 2016 16th mediterranean microwave symposium (MMS), Abu Dhabi, United Arab Emirates, 14 November 2016. [Google Scholar] [CrossRef]
- Yasir, M.; Savi, P. Dynamically Tunable Phase Shifter with Commercial Graphene Nanoplatelets. Micromachines 2020, 11, 600. [Google Scholar] [CrossRef] [PubMed]
- Correia, R.; Boaventura, A.; Carvalho, N.B. Quadrature Amplitude Backscatter Modulator for Passive Wireless Sensors in IoT Applications. IEEE Trans. Microw. Theory Tech. 2017, 65, 1103–1110. [Google Scholar] [CrossRef]
- Fan, C.-Y.; Wei, M.-D.; Gjurovski, P.; Saeed, M.; Hamed, A.; Negra, R.; Uzlu, B.; Wang, Z.; Neumaier, D. Concept for a 16-QAM RF Transmitter on Flexible Substrate using a Graphene Technology. In Proceedings of the 2019 26th IEEE International Conference on Electronics, Circuits and Systems (ICECS), Genoa, Italy, 27–29 November 2019. [Google Scholar] [CrossRef]
- Moon, S.-M.; Yun, S.; Yom, I.-B.; Lee, H.L. Phased Array Shaped-Beam Satellite Antenna with Boosted-Beam Control. IEEE Trans. Antennas Propag. 2019, 67, 7633–7636. [Google Scholar] [CrossRef]
- Yang, J.; Liu, X.; Tu, Y.; Li, W. Robust Adaptive Beamforming Algorithm for Sparse Subarray Antenna Array Based on Hierarchical Weighting. Micromachines 2022, 13, 859. [Google Scholar] [CrossRef]
- Norhakim, N.; Hawari, H.F.; Burhanudin, Z.A. Assessing the Figures of Merit of Graphene-Based Radio Frequency Electronics: A Review of GFET in RF Technology. IEEE Access 2022, 10, 17030–17042. [Google Scholar] [CrossRef]
- Medina-Rull, A.; Pasadas, F.; Marin, E.G.; Toral-Lopez, A.; Cuesta, J.; Godoy, A.; Jimenez, D.; Ruiz, F.G. A Graphene Field-Effect Transistor Based Analogue Phase Shifter for High-Frequency Applications. IEEE Access 2020, 8, 209055–209063. [Google Scholar] [CrossRef]
- Li, J.; Mao, X.; Gu, X.; Xie, S.; Geng, Z.; Chen, H. Phase Shift Induced by Gate-Controlled Quantum Capacitance in Graphene FET. IEEE Electron. Device Lett. 2021, 42, 601–604. [Google Scholar] [CrossRef]
- Ross, T.N.; Cormier, G.; Hettak, K.; Wight, J.S. High-Power X-Band GaN Switched-Filter Phase Shifter. In Proceedings of the IEEE MTT-S International Microwave Symposium, Tampa, FL, USA, 1–6 June 2014. [Google Scholar] [CrossRef]
- Ulusoy, A.; Schleicher, B.; Schumacher, H. A Tunable Differential All-Pass Filter for UWB True Time Delay and Phase Shift Applications. IEEE Microw. Wirel. Components Lett. 2011, 21, 462–464. [Google Scholar] [CrossRef]
- Woods, W.H.; Valdes-Garcia, A.; Ding, H.; Rascoe, J. CMOS millimeter wave phase shifter based on tunable transmission lines. In Proceedings of the IEEE 2013 Custom Integrated Circuits Conference, San Jose, CA, USA, 22–25 September 2013. [Google Scholar] [CrossRef]
- Tabesh, M.; Arbabian, A.; Niknejad, A. 60GHz low-loss compact phase shifters using a transformer-based hybrid in 65nm CMOS. In Proceedings of the 2011 IEEE Custom Integrated Circuits Conference (CICC), San Jose, CA, USA, 19–21 September 2011. [Google Scholar] [CrossRef]
- Krishnaswamy, H.; Valdes-Garcia, A.; Lai, J. A silicon-based, all-passive, 60 GHz, 4-element, phased-array beamformer featuring a differential, reflection-type phase shifter. In Proceedings of the 2010 IEEE International Symposium on Phased Array Systems and Technology, Waltham, MA, USA, 12–15 October 2010. [Google Scholar] [CrossRef]








| Vg (V) | Ig (mA) | Rg (Ω) | Vg1 (V) | Ig1 (mA) | Rg1 (Ω) | |
|---|---|---|---|---|---|---|
| Attenuator | 0 | - | 3100 | 0 | - | 3100 |
| 10 | 5 | 2000 | 0 | - | 3100 | |
| 20 | 12.5 | 1600 | 0 | - | 3100 | |
| 25 | 28 | 892 | 0 | - | 3100 | |
| Phase shifter | 0 | - | 3100 | 0 | - | 3100 |
| 0 | - | 3100 | 10 | 5 | 2000 | |
| 0 | - | 3100 | 12.5 | 8 | 1562 | |
| 0 | - | 3100 | 15 | 17 | 882 |
| Technology | Frequency (GHz) | Phase Shift (°) | ΔIL (dB) | FoM (°/dB) | Ref |
|---|---|---|---|---|---|
| GFET | 3 | 85 | 1.3 | 65.4 | [17] |
| GFET | 8 | 40 | - | - | [18] |
| GFET | 30 | 75 | - | - | [18] |
| GaN HEMT | 8–16 | 22.5 | 2 | 11.3 | [19] |
| SiGeHBT | 5 | 170 | - | - | [20] |
| 32-nm CMOS | 60 | 175 | 3.6 | 48.6 | [21] |
| 64-nm CMOS | 60 | 180 | 3.3 | 54.54 | [22] |
| 130-nm SiGe BiCMOS | 60 | 156 | 2.2 | 70.9 | [23] |
| Graphene loaded line | 4–4.5 | 23 | 0.42 | 54.7 | This work |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yasir, M.; Fatikow, S.; Haenssler, O.C. Amplitude-Phase Variation in a Graphene-Based Microstrip Line. Micromachines 2022, 13, 1039. https://doi.org/10.3390/mi13071039
Yasir M, Fatikow S, Haenssler OC. Amplitude-Phase Variation in a Graphene-Based Microstrip Line. Micromachines. 2022; 13(7):1039. https://doi.org/10.3390/mi13071039
Chicago/Turabian StyleYasir, Muhammad, Sergej Fatikow, and Olaf C. Haenssler. 2022. "Amplitude-Phase Variation in a Graphene-Based Microstrip Line" Micromachines 13, no. 7: 1039. https://doi.org/10.3390/mi13071039
APA StyleYasir, M., Fatikow, S., & Haenssler, O. C. (2022). Amplitude-Phase Variation in a Graphene-Based Microstrip Line. Micromachines, 13(7), 1039. https://doi.org/10.3390/mi13071039








