The Role of Interfacial Adhesion in Polymer Composites Engineered from Lignocellulosic Agricultural Waste
Abstract
:1. Introduction
2. Experimental
2.1. Materials
2.2. Sample Preparation
2.3. Characterization
2.4. Statistical Analysis
3. Results and Discussion
3.1. The Limiting Factors of Modulus
3.2. Performance at Failure
3.3. Reinforcing Effect of Filler
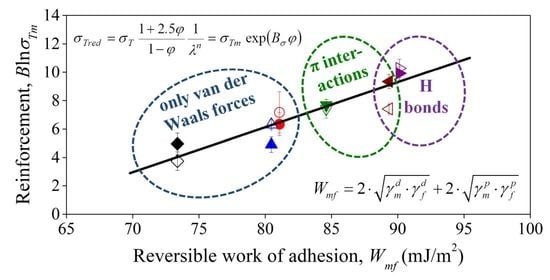
3.4. Effect of Adhesion and Structure on Reinforcement
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Siracusa, V.; Blanco, I. Bio-Polyethylene (Bio-PE), Bio-Polypropylene (Bio-PP) and Bio-Poly(ethylene terephthalate) (Bio-PET): Recent Developments in Bio-Based Polymers Analogous to Petroleum-Derived Ones for Packaging and Engineering Applications. Polymers 2020, 12, 1641. [Google Scholar] [CrossRef] [PubMed]
- Brito, G.F.; Agrawal, P.; Araújo, E.M.; de Mélo, T.J.A. Polylactide/biopolyethylene bioblends. Polímeros 2012, 22, 427–429. [Google Scholar] [CrossRef] [Green Version]
- Mancini, E.; Antonelli, M.G.; Zobel, P.B.; Sasso, M. Characterization and analytical parametrization of composite in cellulose fibre and PVA matrix. Compos. Part B Eng. 2019, 172, 496–505. [Google Scholar] [CrossRef]
- Grylewicz, A.; Spychaj, T.; Zdanowicz, M. Thermoplastic starch/wood biocomposites processed with deep eutectic solvents. Compos. Part A Appl. Sci. Manuf. 2019, 121, 517–524. [Google Scholar] [CrossRef]
- Olaiya, N.G.; Surya, I.; Oke, P.K.; Rizal, S.; Sadiku, E.R.; Ray, S.S.; Farayibi, P.K.; Hossain, M.S.; Khalil, H.P.S.A. Properties and characterization of a PLA-chitin-starch biodegradable polymer composite. Polymers 2019, 11, 1656. [Google Scholar] [CrossRef] [Green Version]
- Móczó, J.; Kun, D.; Fekete, E. Desiccant effect of starch in polylactic acid composites. Express Polym. Lett. 2018, 12, 1014–1024. [Google Scholar] [CrossRef]
- Singamneni, S.; Velu, R.; Behera, M.P.; Scott, S.; Brorens, P.; Harland, D.; Gerrard, J. Selective laser sintering responses of keratin-based bio-polymer composites. Mater. Des. 2019, 183, 108087. [Google Scholar] [CrossRef]
- Chen, J.; Guo, J.; Zhao, M.; Zhang, R.; Guan, F. Hydrogen bonding in chitosan/Antarctic krill protein composite system: Study on construction and enhancement mechanism. Int. J. Biol. Macromol. 2020, 142, 513–520. [Google Scholar] [CrossRef]
- Park, C.W.; Youe, W.J.; Namgung, H.W.; Han, S.Y.; Seo, P.N.; Chae, H.M.; Lee, S. Effect of lignocellulose nanofibril and polymeric methylene diphenyl diisocyanate addition on plasticized lignin/polycaprolactone composites. BioResources 2019, 13, 6802–6817. [Google Scholar]
- Romhányi, V.; Kun, D.; Pukánszky, B. Correlations among Miscibility, Structure, and Properties in Thermoplastic Polymer/Lignin Blends. ACS Sustain. Chem. Eng. 2018, 6, 14323–14331. [Google Scholar] [CrossRef] [Green Version]
- Bula, K.; Klapiszewski, Ł.; Jesionowski, T. Effect of processing conditions and functional silica/lignin content on the properties of bio-based composite thin sheet films. Polym. Test. 2019, 77, 105911. [Google Scholar] [CrossRef]
- Dias, O.A.T.; Sain, M.; Cesarino, I.; Leão, A.L. Development of high bio-content polypropylene composites with different industrial lignins. Polym. Adv. Technol. 2019, 30, 70–78. [Google Scholar] [CrossRef] [Green Version]
- Abdelwahab, M.A.; Misra, M.; Mohanty, A.K. Injection molded biocomposites from polypropylene and lignin: Effect of compatibilizers on interfacial adhesion and performance. Ind. Crop. Prod. 2019, 132, 497–510. [Google Scholar] [CrossRef]
- Muthuraj, R.; Hajee, M.; Horrocks, A.R.; Kandola, B.K. Biopolymer blends from hardwood lignin and bio-polyamides: Compatibility and miscibility. Int. J. Biol. Macromol. 2019, 132, 439–450. [Google Scholar] [CrossRef] [PubMed]
- Nagy, S.; Csiszár, E.; Kun, D.; Koczka, B. Cellulose nanocrystal/amino-aldehyde biocomposite films. Carbohydr. Polym. 2018, 194, 51–60. [Google Scholar] [CrossRef] [Green Version]
- De Andrade, M.R.; Nery, T.B.R.; e Santana, T.I.S.; Leal, I.L.; Rodrigues, L.A.P.; Reis, J.H.O.; Druzian, J.I.; Machado, B.A.S. Effect of cellulose nanocrystals from different lignocellulosic residues to chitosan/glycerol films. Polymers 2019, 11, 658. [Google Scholar] [CrossRef] [Green Version]
- Rigotti, D.; Checchetto, R.; Tarter, S.; Caretti, D.; Rizzuto, M.; Fambri, L.; Pegoretti, A. Polylactic acid-lauryl functionalized nanocellulose nanocomposites: Microstructural, thermo-mechanical and gas transport properties. Express Polym. Lett. 2019, 13, 858–876. [Google Scholar] [CrossRef]
- Montava-Jordà, S.; Torres-Giner, S.; Ferrandiz-Bou, S.; Quiles-Carrillo, L.; Montanes, N. Development of Sustainable and Cost-Competitive Injection-Molded Pieces of Partially Bio-Based Polyethylene Terephthalate through the Valorization of Cotton Textile Waste. Int. J. Mol. Sci. 2019, 20, 1378. [Google Scholar] [CrossRef] [Green Version]
- Panariello, L.; Coltelli, M.B.; Buchignani, M.; Lazzeri, A. Chitosan and nano-structured chitin for biobased anti-microbial treatments onto cellulose based materials. Eur. Polym. J. 2019, 113, 328–339. [Google Scholar] [CrossRef]
- Idumah, C.I.; Hassan, A.; Bourbigot, S. Synergistic effect of exfoliated graphene nanoplatelets and non-halogen flame retardants on flame retardancy and thermal properties of kenaf flour-PP nanocomposites. J. Therm. Anal. Calorim. 2018, 134, 1681–1703. [Google Scholar] [CrossRef]
- Kumar, A.; Tumu, V.R. Physicochemical properties of the electron beam irradiated bamboo powder and its bio-composites with PLA. Compos. Part B Eng. 2019, 175, 107098. [Google Scholar] [CrossRef]
- Panaitescu, D.M.; Vuluga, Z.; Sanporean, C.G.; Nicolae, C.A.; Gabor, A.R.; Trusca, R. High flow polypropylene/SEBS composites reinforced with differently treated hemp fibers for injection molded parts. Compos. Part B Eng. 2019, 174, 107062. [Google Scholar] [CrossRef]
- Várdai, R.; Lummerstorfer, T.; Pretschuh, C.; Jerabek, M.; Gahleitner, M.; Pukánszky, B.; Renner, K. Impact modification of PP/wood composites: A new approach using hybrid fibers. Express Polym. Lett. 2019, 13, 223–234. [Google Scholar] [CrossRef]
- Bazan, P.; Mierzwiński, D.; Bogucki, R.; Kuciel, S. Bio-Based Polyethylene Composites with Natural Fiber: Mechanical, Thermal, and Ageing Properties. Materials 2020, 13, 2595. [Google Scholar] [CrossRef] [PubMed]
- Muthuraj, R.; Lacoste, C.; Lacroix, P.; Bergeret, A. Sustainable thermal insulation biocomposites from rice husk, wheat husk, wood fibers and textile waste fibers: Elaboration and performances evaluation. Ind. Crop. Prod. 2019, 135, 238–245. [Google Scholar] [CrossRef] [Green Version]
- Dong, S.; Yuan, F.; Yang, L.; Chi, S.; Zhong, J.; Lei, J.; Bao, L.; Wang, J. Clean and sustainable biocomposites based on supramolecular interactions induced thermoplasticization of wheat straw powders. J. Clean. Prod. 2019, 233, 590–600. [Google Scholar] [CrossRef]
- Anggono, J.; Suwandi, S.; Rassy, A.; Hariyati, P.; Aria, W. Performance and evaluation of low cost sugarcane bagasse—Polypropylene biocomposites as candidate material for automotive parcel tray. Mater. Sci. Forum 2018, 923, 40–46. [Google Scholar] [CrossRef]
- Chen, B.; Cai, D.; Luo, Z.; Chen, C.; Zhang, C.; Qin, P.; Cao, H.; Tan, T. Corncob residual reinforced polyethylene composites considering the biorefinery process and the enhancement of performance. J. Clean. Prod. 2018, 198, 452–462. [Google Scholar] [CrossRef]
- Eschenhagen, A.; Raj, M.; Rodrigo, N.; Zamora, A.; Labonne, L.; Evon, P.; Welemane, H. Investigation of Miscanthus and Sunflower Stalk Fiber-Reinforced Composites for Insulation Applications. Adv. Civ. Eng. 2019, 2019, 9328087. [Google Scholar] [CrossRef]
- Kamireddy, S.R.; Schaefer, C.; Defrese, M.; Degenstein, J.; Ji, Y. Pretreatment and enzymatic hydrolysis of sunflower hulls for fermentable sugar production. Int. J. Agric. Biol. Eng. 2012, 5, 62–70. [Google Scholar]
- Seiler, G.J.; Gulya, T.J. Sunflower: Overview. In Reference Module in Food Science; Elsevier: Amsterdam, The Netherlands, 2016. [Google Scholar]
- Angellier-Coussy, H.; Guillard, V.; Gastaldi, E.; Peyron, S.; Gontard, N. Lignocellulosic Composite Materials; Kalia, S., Ed.; Springer Series on Polymer and Composite Materials; Springer: Cham, Switzerland, 2018. [Google Scholar]
- Grozdanov, A.; Avella, M.; Buzarovska, A.; Gentile, G.; Errico, M. Reuse of natural fiber reinforced eco-composites in polymer mortars. Polym. Eng. Sci. 2010, 50, 762–766. [Google Scholar] [CrossRef]
- Meyer, S.; Meyer, U.; Trumme, R.; Simeone, C.; Albers, S. Process for Producing a Bioplastics Product. W.O. Patent 186,743, 2 November 2017. [Google Scholar]
- Jacob, A. WPC industry focuses on performance and cost. Reinf. Plast. 2006, 50, 32–33. [Google Scholar] [CrossRef]
- Suddell, B.C.; Evans, W.J. Natural fiber composites in automotive applications. In Natural Fibers, Biopolymers, and Biocomposites; Mohanty, A.K., Misra, M., Drzal, L.T., Eds.; CRC Press: Boca Raton, FL, USA, 2005; pp. 231–259. [Google Scholar]
- Spear, M.J.; Eder, A.; Carus, M. 10-Wood polymer composites. In Wood Composites; Ansell, M.P., Ed.; Woodhead Publishing: Cambridge, UK, 2015; pp. 195–249. [Google Scholar]
- Kun, D.; Pukánszky, B. Polymer/lignin blends: Interactions, properties, applications. Eur. Polym. J. 2017, 93, 618–641. [Google Scholar] [CrossRef] [Green Version]
- Alam, M.A.; Sapuan, S.; Mansor, M. Design characteristics, codes and standards of natural fibre composites. In Advanced High Strength Natural Fibre Composites in Construction; Fan, M., Fu, F., Eds.; Elsevier: Amsterdam, The Netherlands, 2017; pp. 511–528. [Google Scholar]
- Friedrich, D. An analytic algorithm-based method to assess the long term structural performance of wood-polymer composites. J. Build. Eng. 2018, 20, 367–376. [Google Scholar] [CrossRef]
- Sui, G.; Fuqua, M.A.; Ulven, C.A.; Zhong, W.H. A plant fiber reinforced polymer composite prepared by a twin-screw extruder. Bioresource Technol. 2009, 100, 1246–1251. [Google Scholar] [CrossRef]
- Salasinska, K.; Ryszkowska, J. The effect of filler chemical constitution and morphological properties on the mechanical properties of natural fiber composites. Compos. Interface 2015, 22, 39–50. [Google Scholar] [CrossRef]
- Marhoon, I. Effect of sunflower husk particles added to polyurethane polymer matrix composite. Int. J. Sci. Technol. Res. 2017, 6, 174–176. [Google Scholar]
- Barczewski, M.; Matykiewicz, D.; Piasecki, A.; Szostak, M. Polyethylene green composites modified with post agricultural waste filler: Thermo-mechanical and damping properties. Compos. Interface 2018, 25, 287–299. [Google Scholar] [CrossRef]
- Maldas, D.; Kokta, V. Interfacial adhesion of lignocellulosic materials in polymer composites: An overview. Compos. Interface 1993, 1, 87–108. [Google Scholar] [CrossRef]
- Kárpáti, Z.; Kun, D.; Fekete, E.; Móczó, J. Structural biomaterials engineered from lignocellulosic agricultural waste. J. Appl. Polym. Sci. 2021, 138, 50617. [Google Scholar] [CrossRef]
- Owens, D.K.; Wendt, R.C. Estimation of the surface free energy of polymers. J. Appl. Polym. Sci. 1969, 13, 1741–1747. [Google Scholar] [CrossRef]
- Rabel, W. Einige Aspekte der Benetzungstheorie und ihre Anwendung auf die Untersuchung und Veränderung der Oberflächeneigenschaften von Polymeren. Farbe Lack 1971, 77, 997–1005. [Google Scholar]
- Kaelble, D.H.; Uy, K.C. A reinterpretation of organic liquid-polytetrafluoroethylene surface interactions. J. Adhes. 1970, 2, 50–60. [Google Scholar] [CrossRef]
- Kaelble, D.H. Dispersion-Polar Surface Tension Properties of Organic Solids. J. Adhes. 1970, 2, 66–81. [Google Scholar] [CrossRef]
- Lewis, T.B.; Nielsen, L.E. Dynamic mechanical properties of particulate-filled composites. J. Appl. Polym. Sci. 1970, 14, 1449–1471. [Google Scholar] [CrossRef]
- Móczó, J.; Pukánszky, B. Particulate Fillers in Thermoplastics. In Fillers for Polymer Applications; Polymers and Polymeric Composites: A Reference Series; Rothon, R., Ed.; Springer: Cham, Switzerland, 2017. [Google Scholar] [CrossRef] [Green Version]
- Donev, A.; Cisse, I.; Sachs, D.; Variano, E.A.; Stillinger, F.H.; Connelly, R.; Torquato, S.; Chaikin, P.M. Improving the Density of Jammed Disordered Packings Using Ellipsoids. Science 2004, 303, 990–993. [Google Scholar] [CrossRef] [Green Version]
- Kronbergs, E. Mechanical strength testing of stalk materials and compacting energy evaluation. Ind. Crop. Prod. 2000, 11, 211–216. [Google Scholar] [CrossRef]
- Young, R.A. Vegetable Fibers. In Encyclopedia of Polymer Science and Technology, 3rd ed.; Part 3; Mark, H.F., Ed.; Wiley: New York, NY, USA, 2004; Volume 12. [Google Scholar]
- Avérous, L.; Le Digabel, F. Properties of biocomposites based on lignocellulosic fillers. Carbohydr. Polym. 2006, 66, 480–493. [Google Scholar] [CrossRef]
- Dányádi, L.; Renner, K.; Szabó, Z.; Nagy, G.; Móczó, J.; Pukánszky, B. Wood flour filled PP composites: Adhesion, deformation, failure. Polym. Adv. Technol. 2006, 17, 967–974. [Google Scholar] [CrossRef]
- Anggono, J.; Farkas, Á.E.; Bartos, A.; Móczó, J.; Purwaningsih, H.; Pukánszky, B. Deformation and failure of sugarcane bagasse reinforced PP. Eur. Polym. J. 2019, 112, 153–160. [Google Scholar] [CrossRef] [Green Version]
- Dányádi, L.; Renner, K.; Móczó, J.; Pukánszky, B. Wood flour filled polypropylene composites: Interfacial adhesion and micromechanical deformations. Polym. Eng. Sci. 2007, 47, 1246–1255. [Google Scholar] [CrossRef]
- Renner, K.; Móczó, J.; Suba, P.; Pukánszky, B. Micromechanical deformations in PP/lignocellulosic filler composites: Effect of matrix properties. Compos. Sci. Technol. 2010, 70, 1141–1147. [Google Scholar] [CrossRef]
- Renner, K.; Móczó, J.; Pukánszky, B. Deformation and failure of PP composites reinforced with lignocellulosic fibers: Effect of inherent strength of the particles. Compos. Sci. Technol. 2009, 69, 1653–1659. [Google Scholar] [CrossRef]
- Faludi, G.; Dora, G.; Imre, B.; Renner, K.; Móczó, J.; Pukánszky, B. PLA/lignocellulosic fiber composites: Particle characteristics, interfacial adhesion, and failure mechanism. J. Appl. Polym. Sci. 2014, 131, 39902. [Google Scholar] [CrossRef] [Green Version]
- Pukánszky, B. Influence of interface interaction on the ultimate tensile properties of polymer composites. Composites 1990, 21, 255–262. [Google Scholar] [CrossRef]
- Nicolais, L.; Narkis, M. Stress-strain behavior of styrene-acrylonitrile/glass bead composites in the glassy region. Polym. Eng. Sci. 1971, 11, 194–199. [Google Scholar] [CrossRef]
- Turcsányi, B.; Pukánszky, B.; Tüdős, F. Effect on Interfacial Interaction on the Tensile Yield Stress of Polymer Composites. In Interfaces in Polymer, Ceramic, and Metal Matrix Composites; Ishida, H., Ed.; Elsevier: New York, NY, USA, 1988; pp. 467–477. [Google Scholar]
- Jang, L.W.; Lee, D.C. Polystyrene/bisphenol A polycarbonate molecular composite by in situ polymerization. I. Preparation and characterization. Polymer 2000, 41, 1749–1756. [Google Scholar] [CrossRef]
- Zhao, D.; Zhu, G.; Ding, Y.; Zheng, J. Construction of a Different Polymer Chain Structure to Study π-π Interaction between Polymer and Reduced Graphene Oxide. Polymers 2018, 10, 716. [Google Scholar] [CrossRef] [Green Version]
- Tardio, S.; Abel, M.-L.; Carr, R.H.; Watts, J.F. Polystyrene-silicon bonding through π electrons: A combined XPS and DFT study. Surf. Interface Anal. 2016, 48, 556–560. [Google Scholar] [CrossRef]
- Shen, B.; Zhai, W.; Chen, C.; Lu, D.; Wang, J.; Zheng, W. Melt Blending In situ Enhances the Interaction between Polystyrene and Graphene through π-π Stacking. ACS Appl. Mater. Interfaces 2011, 3, 3103–3109. [Google Scholar] [CrossRef]
- Müller, P.; Bere, J.; Fekete, E.; Móczó, J.; Nagy, B.; Kállay, M.; Gyarmati, B.; Pukánszky, B. Interactions, structure and properties in PLA/plasticized starch blends. Polymer 2016, 103, 9–18. [Google Scholar] [CrossRef]
- Kajtár, D.A.; Kenyó, C.; Renner, K.; Móczó, J.; Fekete, E.; Kröhnke, C.; Pukanszky, B. Interfacial interactions and reinforcement in thermoplastics/zeolite composites. Compos. Part B Eng. 2017, 114, 386–394. [Google Scholar] [CrossRef] [Green Version]
- Fowkes, F.M. Determination of interfacial tensions, contact angles, and dispersion forces in surfaces by assuming additivity of intermolecular interactions in surfaces. J. Phys. Chem. 1962, 66, 382. [Google Scholar] [CrossRef]
- Huntsberger, J.R. Adhesion of Plasticized Poly(vinyl Butyral) to Glass. J. Adhes. 1981, 13, 107–129. [Google Scholar] [CrossRef]
 ) PP; (
) PP; (  ) LDPE; (
) LDPE; (  ) HDPE; (
) HDPE; (  ) PS; (
) PS; (  ) PETG; (
) PETG; (  ) PLA; empty symbols: SP20; full symbols: SPF; Lewis–Nielsen model.
) PLA; empty symbols: SP20; full symbols: SPF; Lewis–Nielsen model.
 ) PP; (
) PP; (  ) LDPE; (
) LDPE; (  ) HDPE; (
) HDPE; (  ) PS; (
) PS; (  ) PETG; (
) PETG; (  ) PLA; empty symbols: SP20; full symbols: SPF; Lewis–Nielsen model.
) PLA; empty symbols: SP20; full symbols: SPF; Lewis–Nielsen model.
 ) PP; (
) PP; (  ) LDPE; (
) LDPE; (  ) HDPE; (
) HDPE; (  ) PS; (
) PS; (  ) PETG; (
) PETG; (  ) PLA; empty symbols: SP20; full symbols: SPF.
) PLA; empty symbols: SP20; full symbols: SPF.
 ) PP; (
) PP; (  ) LDPE; (
) LDPE; (  ) HDPE; (
) HDPE; (  ) PS; (
) PS; (  ) PETG; (
) PETG; (  ) PLA; empty symbols: SP20; full symbols: SPF.
) PLA; empty symbols: SP20; full symbols: SPF.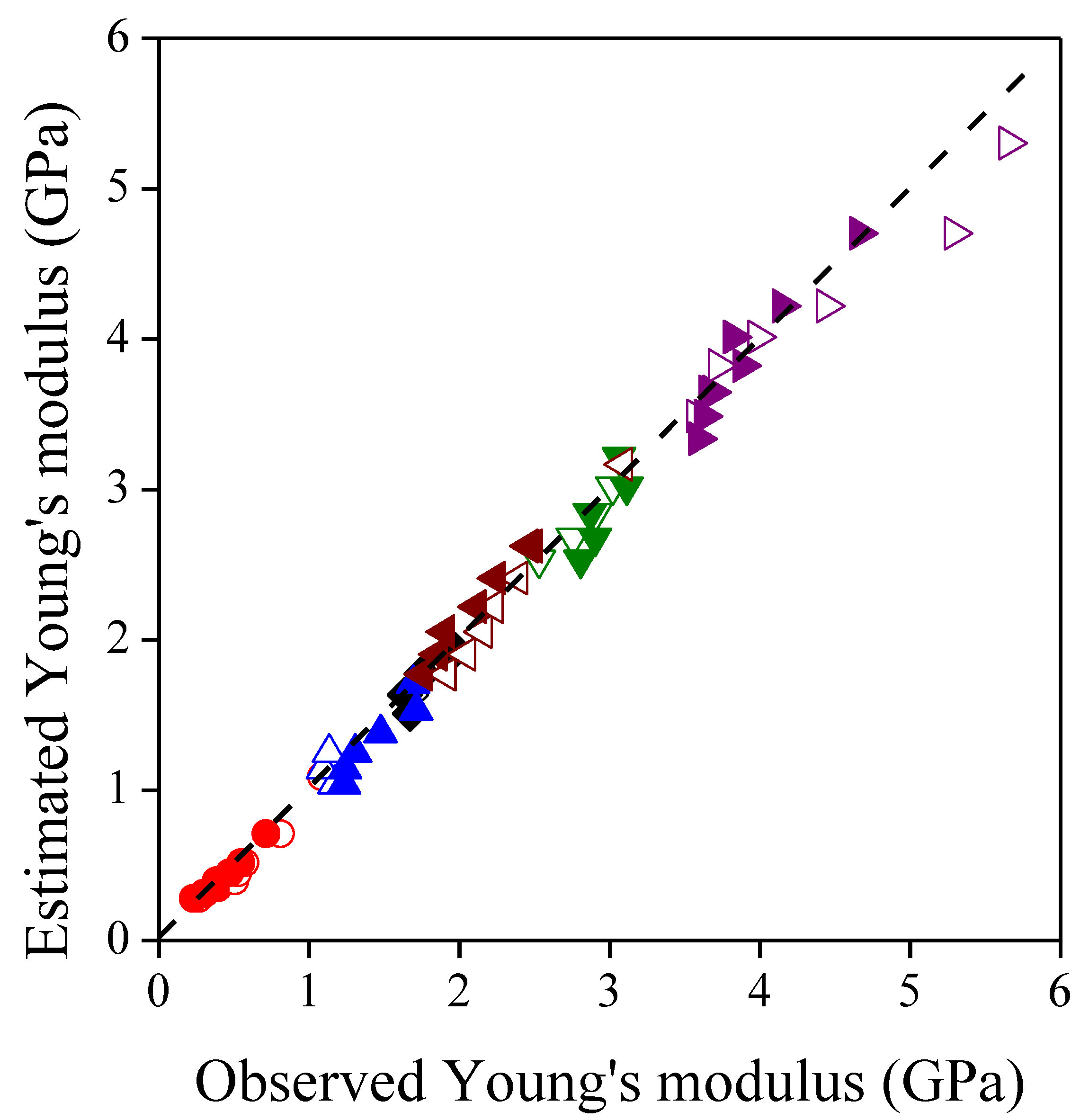

 ) PP; (
) PP; (  ) LDPE; (
) LDPE; (  ) HDPE; (
) HDPE; (  ) PS; (
) PS; (  ) PETG; (
) PETG; (  ) PLA; empty symbols: SP20; full symbols: SPF.
) PLA; empty symbols: SP20; full symbols: SPF.
 ) PP; (
) PP; (  ) LDPE; (
) LDPE; (  ) HDPE; (
) HDPE; (  ) PS; (
) PS; (  ) PETG; (
) PETG; (  ) PLA; empty symbols: SP20; full symbols: SPF.
) PLA; empty symbols: SP20; full symbols: SPF.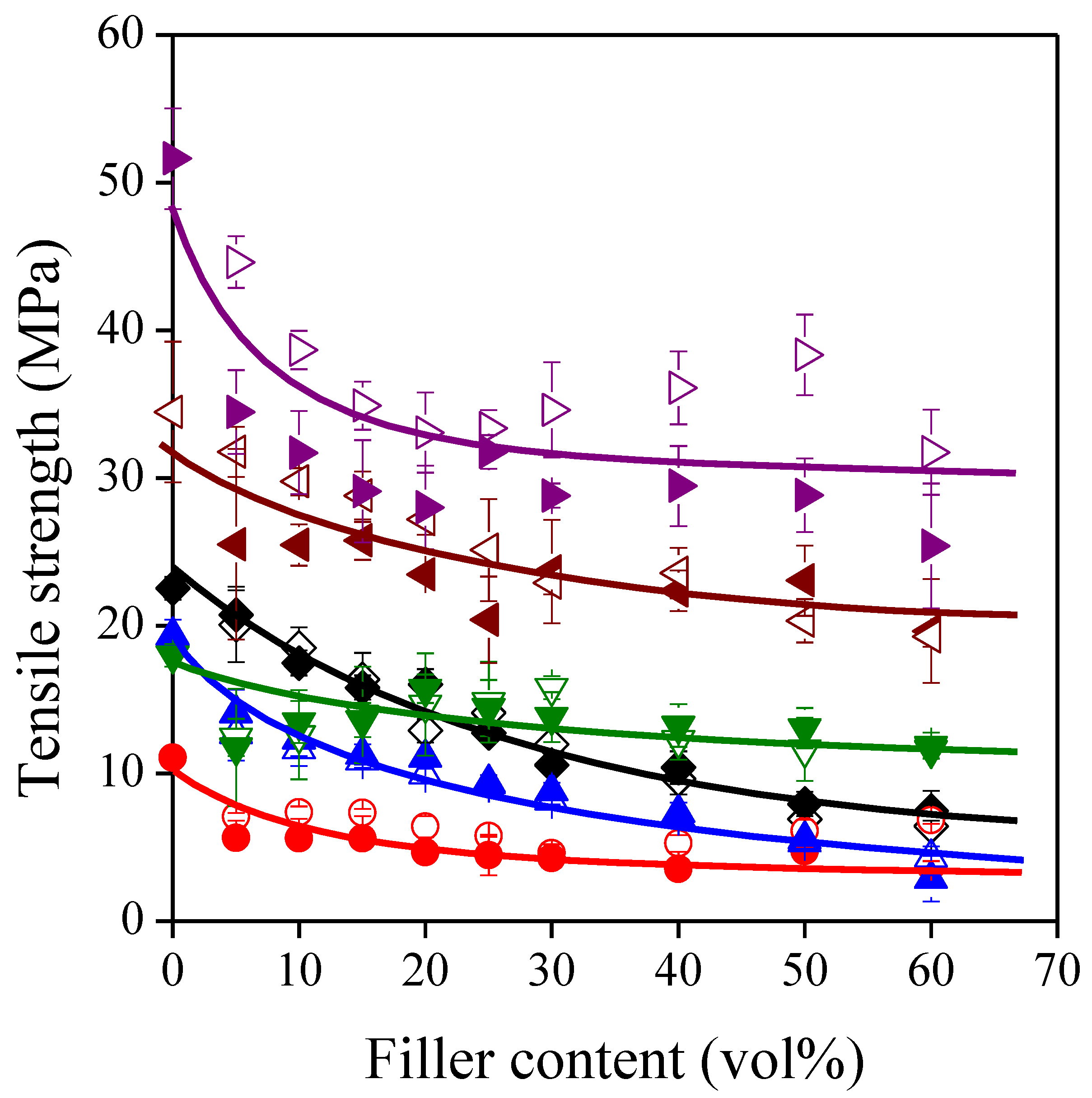
 ) PP; (
) PP; (  ) LDPE; (
) LDPE; (  ) HDPE; (
) HDPE; (  ) PS; (
) PS; (  ) PETG; (
) PETG; (  ) PLA; empty symbols: SP20; full symbols: SPF. One trend line represents the LDPE composites having the highest deformability, and the other one represents the much more rigid PLA composites.
) PLA; empty symbols: SP20; full symbols: SPF. One trend line represents the LDPE composites having the highest deformability, and the other one represents the much more rigid PLA composites.
 ) PP; (
) PP; (  ) LDPE; (
) LDPE; (  ) HDPE; (
) HDPE; (  ) PS; (
) PS; (  ) PETG; (
) PETG; (  ) PLA; empty symbols: SP20; full symbols: SPF. One trend line represents the LDPE composites having the highest deformability, and the other one represents the much more rigid PLA composites.
) PLA; empty symbols: SP20; full symbols: SPF. One trend line represents the LDPE composites having the highest deformability, and the other one represents the much more rigid PLA composites.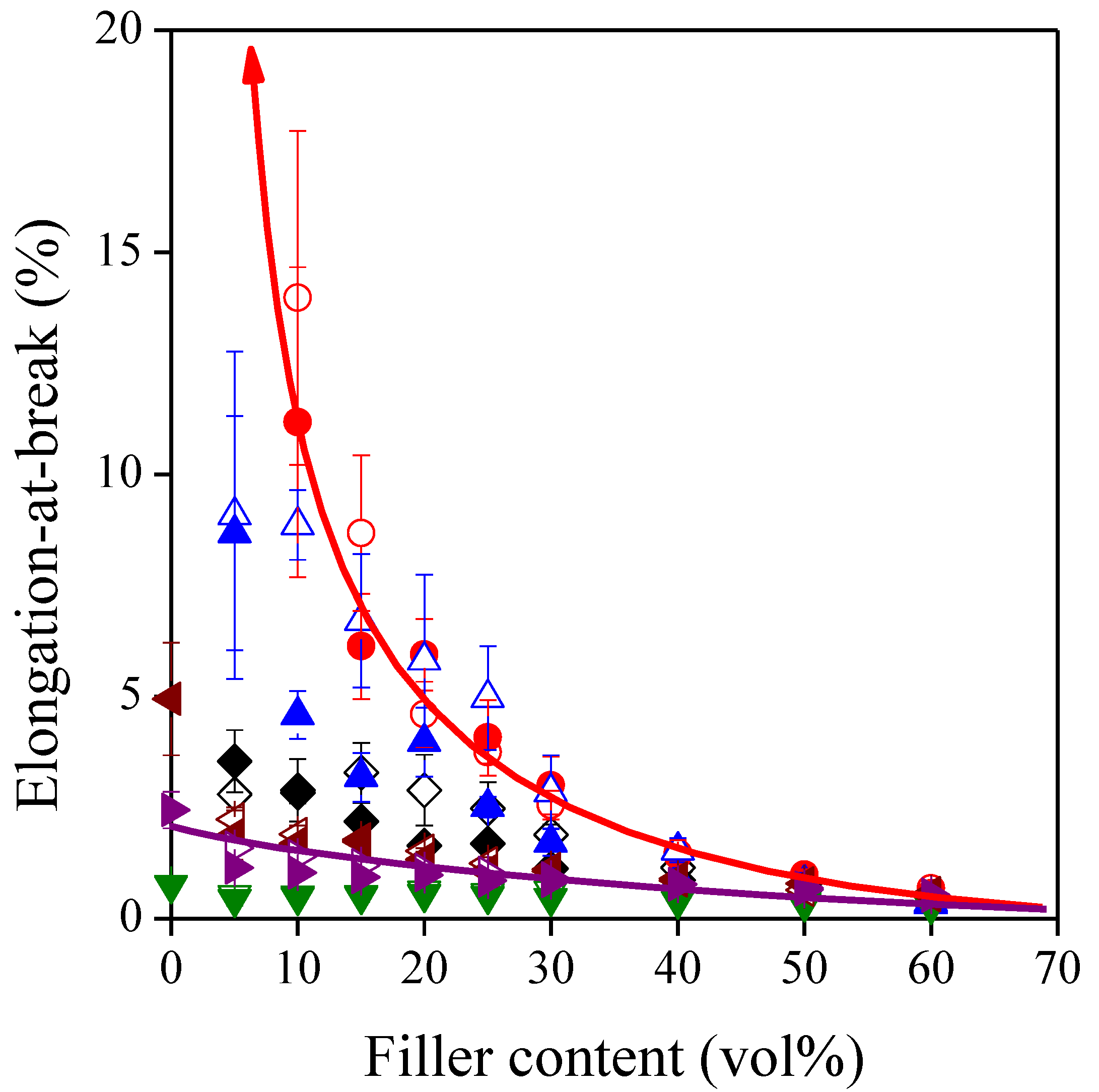
 ) PP; (
) PP; (  ) LDPE; (
) LDPE; (  ) HDPE; (
) HDPE; (  ) PS; (
) PS; (  ) PETG; (
) PETG; (  ) PLA; empty symbols: SP20; full symbols: SPF.
) PLA; empty symbols: SP20; full symbols: SPF.
 ) PP; (
) PP; (  ) LDPE; (
) LDPE; (  ) HDPE; (
) HDPE; (  ) PS; (
) PS; (  ) PETG; (
) PETG; (  ) PLA; empty symbols: SP20; full symbols: SPF.
) PLA; empty symbols: SP20; full symbols: SPF.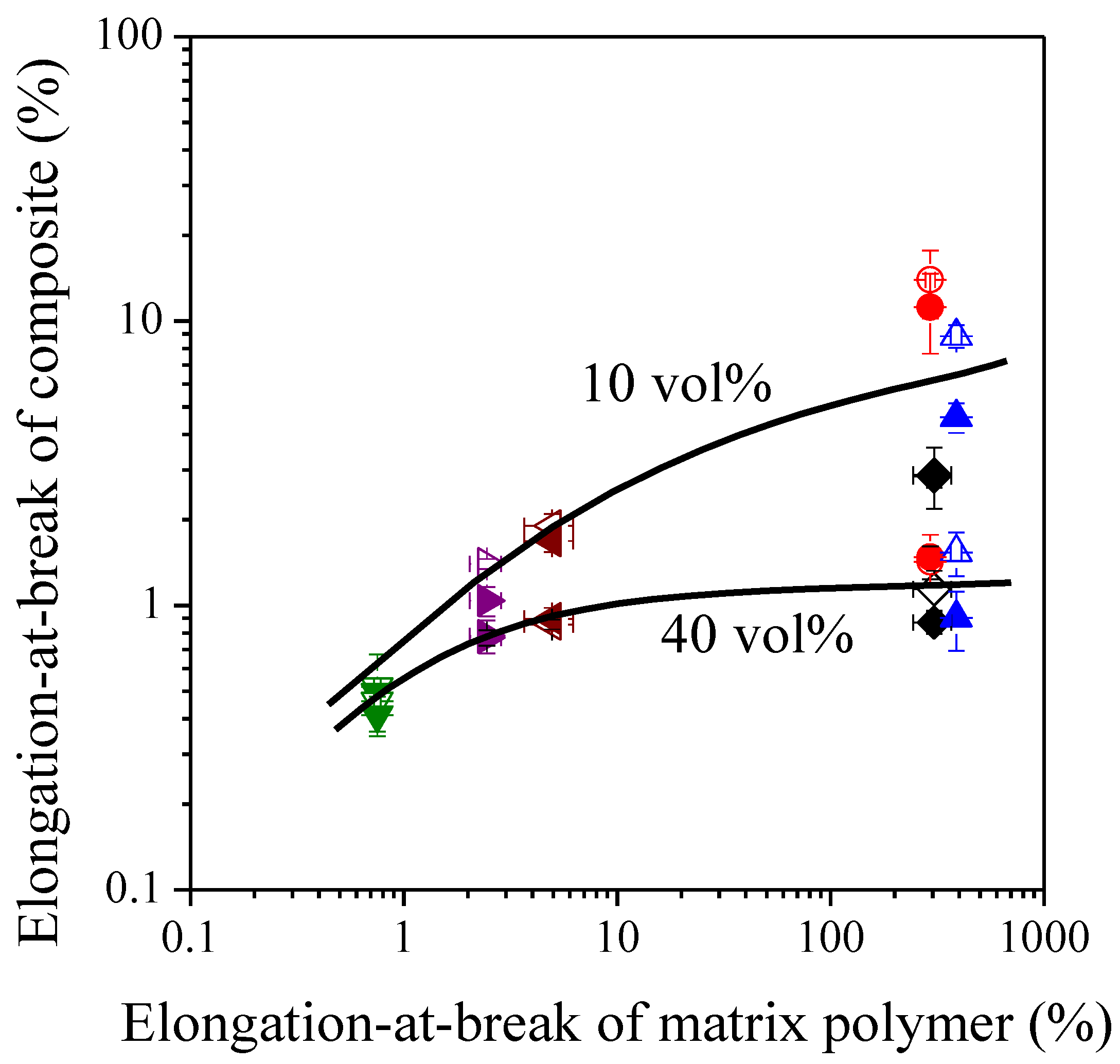
 )) PP/SP20; (
)) PP/SP20; (  )) PETG/SP20. Empty symbols represent data omitted from fitting.
)) PETG/SP20. Empty symbols represent data omitted from fitting.
 )) PP/SP20; (
)) PP/SP20; (  )) PETG/SP20. Empty symbols represent data omitted from fitting.
)) PETG/SP20. Empty symbols represent data omitted from fitting.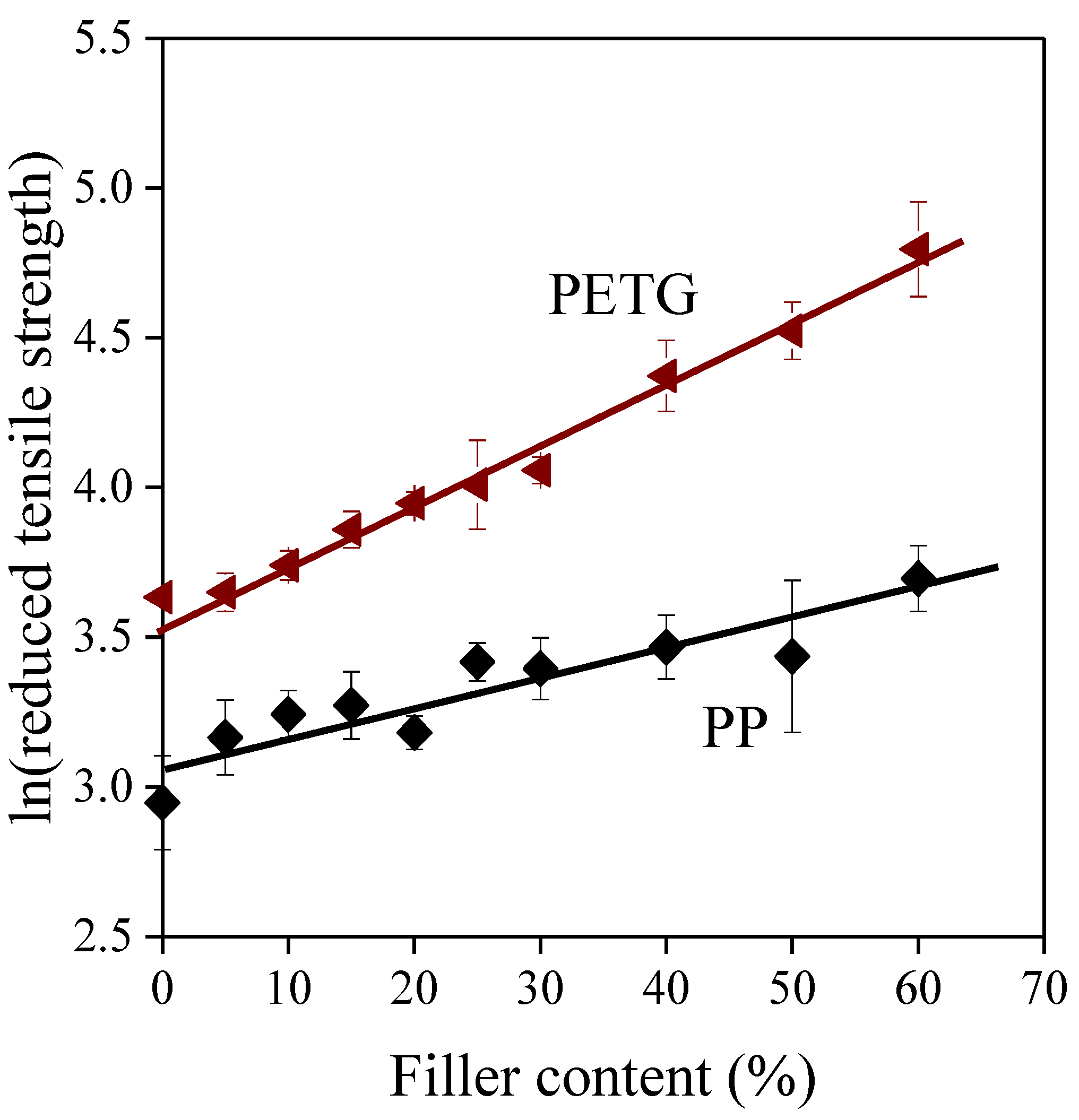
 ) PP; (
) PP; (  ) LDPE; (
) LDPE; (  ) HDPE; (
) HDPE; (  ) PS; (
) PS; (  ) PETG; (
) PETG; (  ) PLA; empty symbols: SP20; full symbols: SPF.
) PLA; empty symbols: SP20; full symbols: SPF.
 ) PP; (
) PP; (  ) LDPE; (
) LDPE; (  ) HDPE; (
) HDPE; (  ) PS; (
) PS; (  ) PETG; (
) PETG; (  ) PLA; empty symbols: SP20; full symbols: SPF.
) PLA; empty symbols: SP20; full symbols: SPF.
 ) PP; (
) PP; (  ) LDPE; (
) LDPE; (  ) HDPE; (
) HDPE; (  ) PS; (
) PS; (  ) PETG; (
) PETG; (  ) PLA; empty symbols: SP20; full symbols: SPF.
) PLA; empty symbols: SP20; full symbols: SPF.
 ) PP; (
) PP; (  ) LDPE; (
) LDPE; (  ) HDPE; (
) HDPE; (  ) PS; (
) PS; (  ) PETG; (
) PETG; (  ) PLA; empty symbols: SP20; full symbols: SPF.
) PLA; empty symbols: SP20; full symbols: SPF.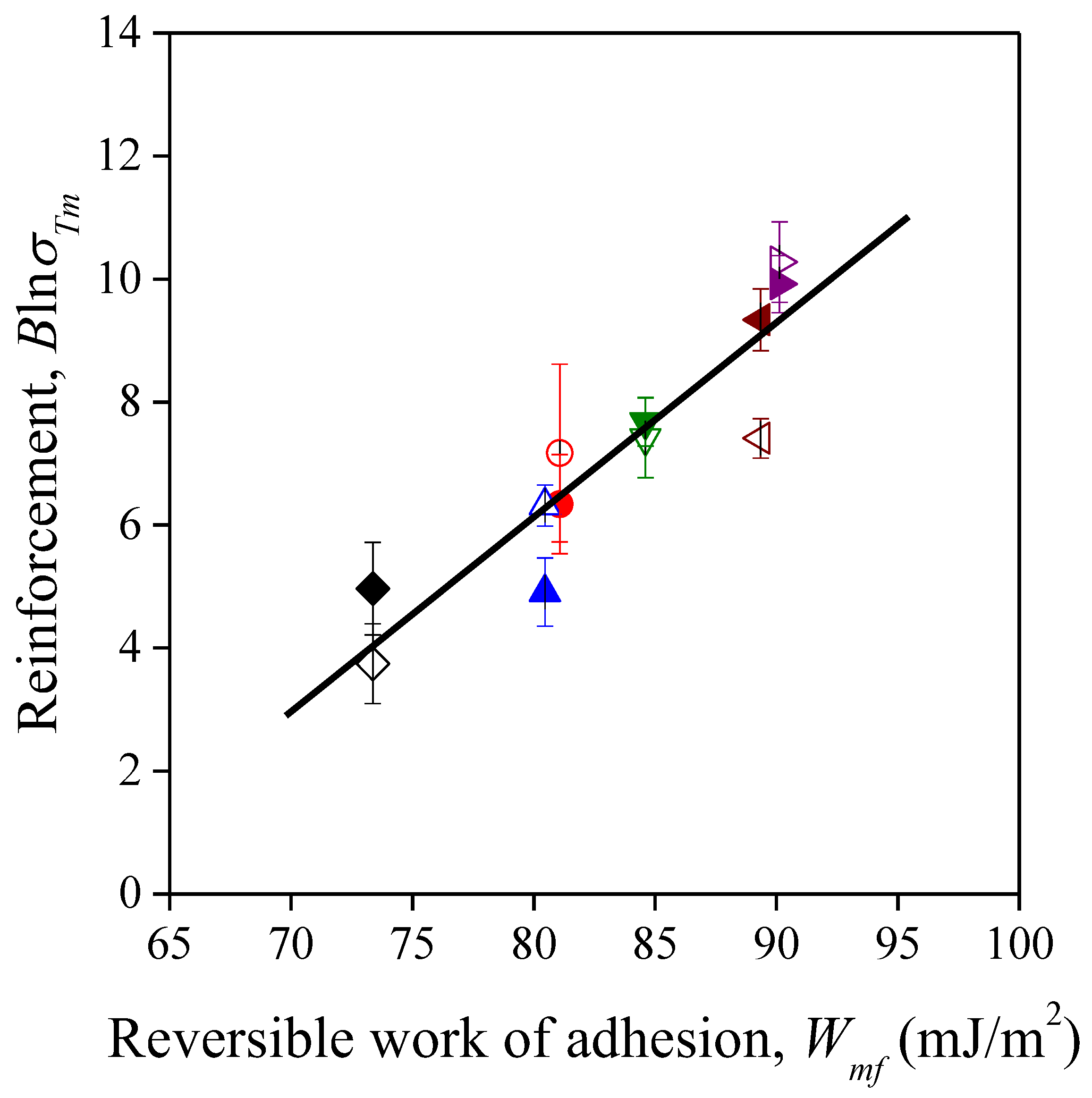
| Polymer | Trade Name | Producer | Density (g/cm3) | MFR a (g/10 min) | Temp. (°C) and Load (kg) of MFR |
|---|---|---|---|---|---|
| PP | H 649 FH | MOL Group (Budapest, Hungary) | 0.90 | 2.5 | 230, 2.16 |
| LDPE | FA-244-51 | 0.92 | 0.28 | 190, 2.16 | |
| HDPE | Tipelin 7100S | 0.95 | 0.25 | 190, 5.00 | |
| PS | Styron 686 E | Americas Styrenics (The Woodlands, TX, USA) | 1.05 | 2.5 | 200, 5.00 |
| PETG | Ecozen SE | SK Chemicals (Seongnam, Korea) | 1.27 | 10.9 | 250, 2.16 |
| PLA | Ingeo 4032 | NatureWorks (Minnetonka, MN, USA) | 1.24 | 3.9 | 190, 2.16 |
| Filler | Upper Composition Limit of Model Validity (vol% Filler Content) | Filler Modulus a (GPa) | R2 b | |||||
|---|---|---|---|---|---|---|---|---|
| PP | LDPE | HDPE | PS | PETG | PLA | |||
| SP20 | 0.15 | 0.50 | 0.15 | 0.20 | 0.40 | 0.50 | 7.63 ± 0.45 | 0.8859 |
| SPF | 0.20 | 0.40 | 0.30 | 0.25 | 0.30 | 0.40 | 7.45 ± 0.69 | 0.8519 |
| Matrix Polymer | Filler | True Tensile Strength of Matrix Polymer, σTm (MPa) | B | R2 a | BlnσTm |
|---|---|---|---|---|---|
| PP | SP20 | 91.19 ± 17.04 | 0.83 ± 0.14 | 0.8339 | 3.75 |
| SPF | 1.10 ± 0.16 | 0.8731 | 4.96 | ||
| LDPE | SP20 | 43.56 ± 4.08 | 1.90 ± 0.38 | 0.8299 | 7.17 |
| SPF | 1.68 ± 0.21 | 0.9274 | 6.34 | ||
| HDPE | SP20 | 94.20 ± 8.90 | 1.39 ± 0.07 | 0.9882 | 6.32 |
| SPF | 1.08 ± 0.12 | 0.9456 | 4.91 | ||
| PS | SP20 | 18.13 ± 0.78 | 2.56 ± 0.22 | 0.9558 | 7.42 |
| SPF | 2.65 ± 0.13 | 0.9847 | 7.68 | ||
| PETG | SP20 | 37.83 ± 2.54 | 2.04 ± 0.08 | 0.9895 | 7.41 |
| SPF | 2.57 ± 0.13 | 0.9834 | 9.34 | ||
| PLA | SP20 | 52.88 ± 3.40 | 2.59 ± 0.16 | 0.9748 | 10.28 |
| SPF | 2.50 ± 0.11 | 0.9877 | 9.92 |
| Matrix Polymer | Surface Tension (mJ/m2) | Reversible Work of Adhesion, Wmf (mJ/m2) | |
|---|---|---|---|
| Dispersion Component, γSd | Polar Component, γSp | ||
| PP | 39.0 | 0.2 | 73.4 |
| LDPE | 35.3 | 3.1 | 81.1 |
| HDPE | 37.2 | 0.6 | 80.5 |
| PS | 40.5 | 1.1 | 84.6 |
| PETG | 43.7 | 2.7 | 89.3 |
| PLA | 43.2 | 4.5 | 90.1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kun, D.; Kárpáti, Z.; Fekete, E.; Móczó, J. The Role of Interfacial Adhesion in Polymer Composites Engineered from Lignocellulosic Agricultural Waste. Polymers 2021, 13, 3099. https://doi.org/10.3390/polym13183099
Kun D, Kárpáti Z, Fekete E, Móczó J. The Role of Interfacial Adhesion in Polymer Composites Engineered from Lignocellulosic Agricultural Waste. Polymers. 2021; 13(18):3099. https://doi.org/10.3390/polym13183099
Chicago/Turabian StyleKun, Dávid, Zoltán Kárpáti, Erika Fekete, and János Móczó. 2021. "The Role of Interfacial Adhesion in Polymer Composites Engineered from Lignocellulosic Agricultural Waste" Polymers 13, no. 18: 3099. https://doi.org/10.3390/polym13183099
APA StyleKun, D., Kárpáti, Z., Fekete, E., & Móczó, J. (2021). The Role of Interfacial Adhesion in Polymer Composites Engineered from Lignocellulosic Agricultural Waste. Polymers, 13(18), 3099. https://doi.org/10.3390/polym13183099