Effect of Carbon Nanofibers on the Viscoelastic Response of Epoxy Resins
Abstract
:1. Introduction
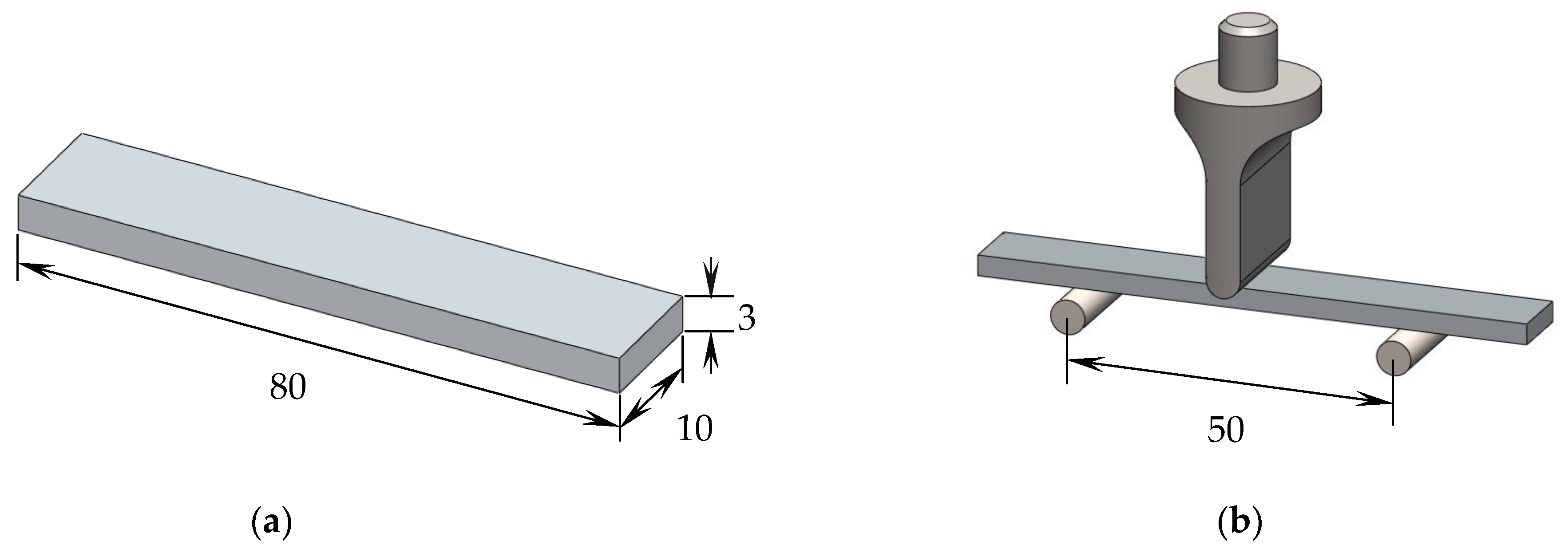
2. Materials and Experimental Procedure
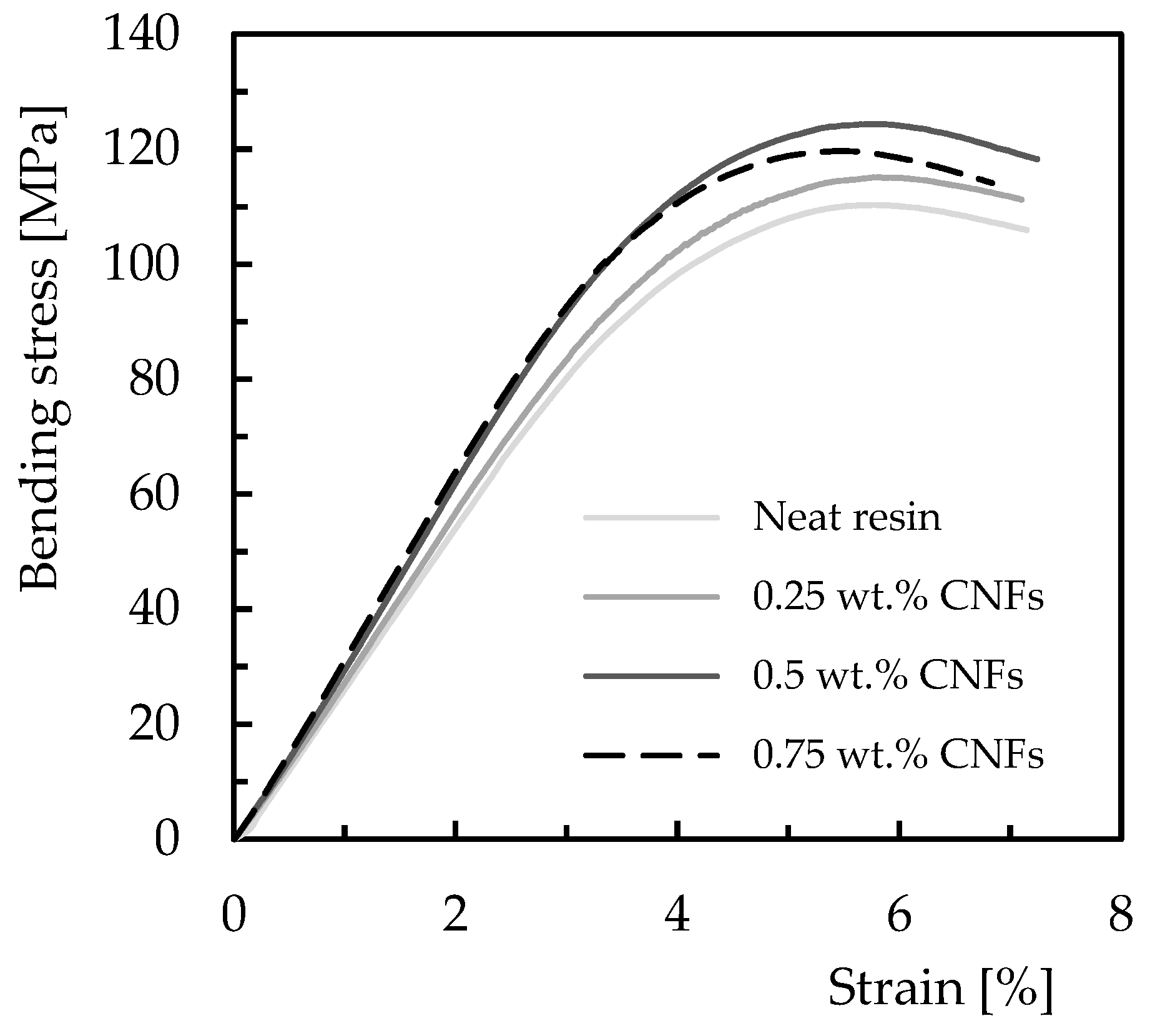
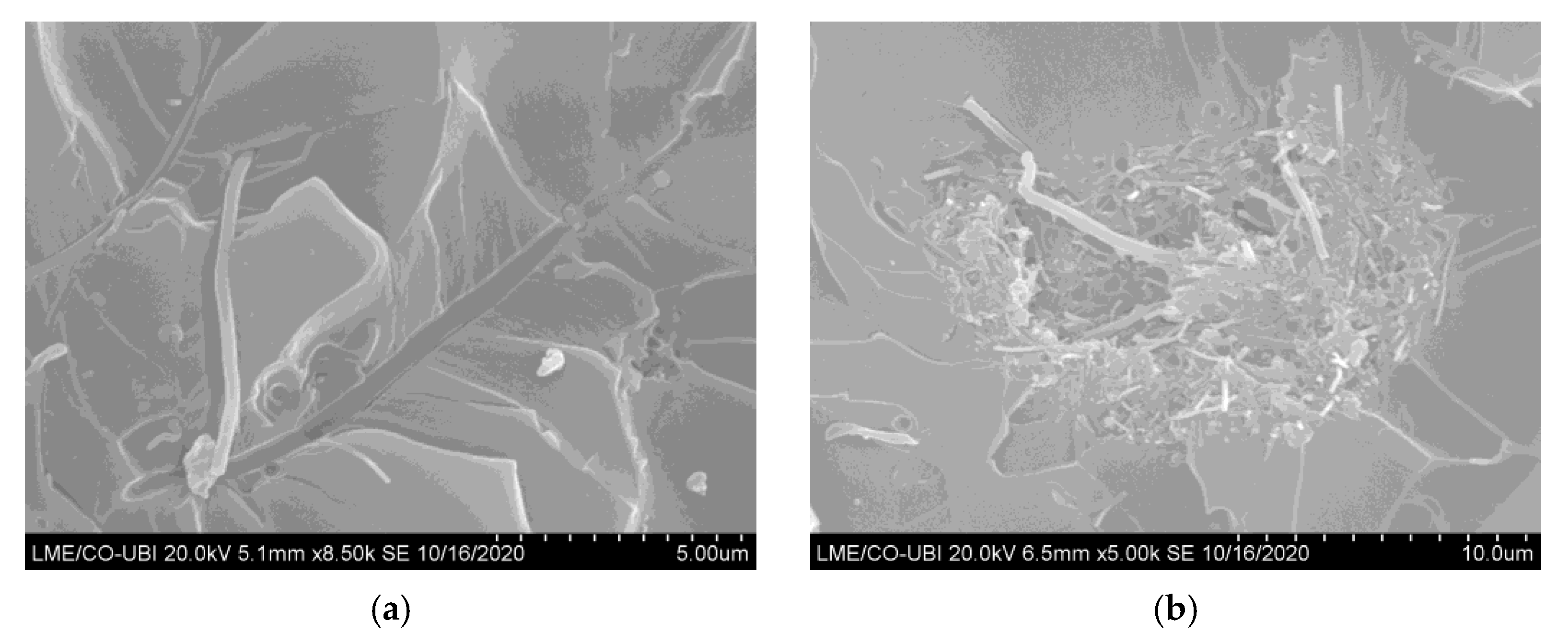
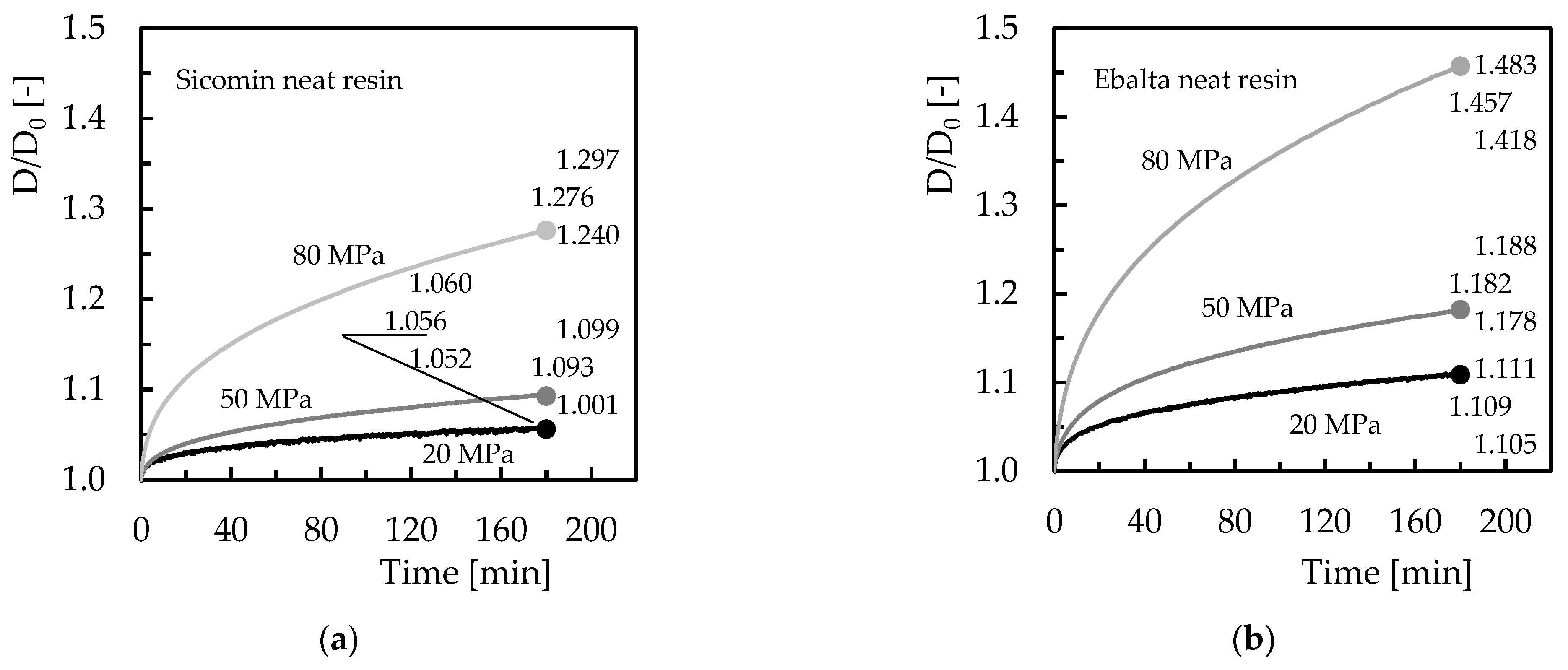
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Jin, F.-L.; Li, X.; Park, S.-J. Synthesis and Application of Epoxy Resins: A Review. J. Ind. Eng. Chem. 2015, 29, 1–11. [Google Scholar] [CrossRef]
- Higgins, C.; Cahill, J.; Jolanki, R.; Nixon, R. Epoxy Resins. In Kanerva’s Occupational Dermatology; Springer International Publishing: Cham, Switzerland, 2018; Volume 11, pp. 1–43. ISBN 9783319402215. [Google Scholar]
- Pina dos Santos, P.S.; Maceiras, A.; Valvez, S.; Reis, P.N.B. Mechanical Characterization of Different Epoxy Resins Enhanced with Carbon Nanofibers. Frat. Integrità Strutt. 2020, 15, 198–212. [Google Scholar] [CrossRef]
- Hsieh, T.H.; Kinloch, A.J.; Masania, K.; Taylor, A.C.; Sprenger, S. The Mechanisms and Mechanics of the Toughening of Epoxy Polymers Modified with Silica Nanoparticles. Polymer 2010, 51, 6284–6294. [Google Scholar] [CrossRef]
- Cho, J.; Joshi, M.S.; Sun, C.T. Effect of Inclusion Size on Mechanical Properties of Polymeric Composites with Micro and Nano Particles. Compos. Sci. Technol. 2006, 66, 1941–1952. [Google Scholar] [CrossRef]
- Bazrgari, D.; Moztarzadeh, F.; Sabbagh-Alvani, A.A.; Rasoulianboroujeni, M.; Tahriri, M.; Tayebi, L. Mechanical Properties and Tribological Performance of Epoxy/Al2O3 Nanocomposite. Ceram. Int. 2018, 44, 1220–1224. [Google Scholar] [CrossRef]
- Eskizeybek, V.; Ulus, H.; Kaybal, H.B.; Şahin, Ö.S.; Avcı, A. Static and Dynamic Mechanical Responses of CaCO3 Nanoparticle Modified Epoxy/Carbon Fiber Nanocomposites. Compos. Part B Eng. 2018, 140, 223–231. [Google Scholar] [CrossRef]
- Singh, S.K.; Singh, S.; Kohli, R.; Jain, A.; Kumar, A. Effect of TiO2 Dispersion on Mechanical Properties of Epoxy Polymer. AIP Conf. Proc. 2016, 1728, 020586. [Google Scholar] [CrossRef]
- Yadav, P.S.; Purohit, R.; Kothari, A. Study of Friction and Wear Behaviour of Epoxy/Nano SiO2 Based Polymer Matrix Composites-A Review. Mater. Today Proc. 2019, 18, 5530–5539. [Google Scholar] [CrossRef]
- Molina, J.; Szczucka-Lasota, B.; Węgrzyn, T.; Silva, A.P.; Maceiras, A. Manufacturing and Characterization of Epoxy Resin with Fe3O4 and SiO2 Particles. KnE Eng. 2020, 2020, 117–128. [Google Scholar] [CrossRef]
- Sun, T.; Fan, H.; Wang, Z.; Liu, X.; Wu, Z. Modified Nano Fe2O3-Epoxy Composite with Enhanced Mechanical Properties. Mater. Des. 2015, 87, 10–16. [Google Scholar] [CrossRef]
- Liu, S.; Chevali, V.S.; Xu, Z.; Hui, D.; Wang, H. A Review of Extending Performance of Epoxy Resins Using Carbon Nanomaterials. Compos. Part B Eng. 2018, 136, 197–214. [Google Scholar] [CrossRef]
- Kausar, A.; Rafique, I.; Muhammad, B. Review of Applications of Polymer/Carbon Nanotubes and Epoxy/CNT Composites. Polym.–Plast. Technol. Eng. 2016, 55, 1167–1191. [Google Scholar] [CrossRef]
- Zhang, B.; Zheng, X.; Li, H.; Lin, J. Analytica Chimica Acta Application of Carbon-Based Nanomaterials in Sample Preparation: A Review. Anal. Chim. Acta 2013, 784, 1–17. [Google Scholar] [CrossRef] [PubMed]
- Huang, J.; Liu, Y.; You, T. Carbon Nanofiber Based Electrochemical Biosensors: A Review. Anal. Methods 2010, 2, 202–211. [Google Scholar] [CrossRef]
- Feng, L.; Xie, N.; Zhong, J. Carbon Nanofibers and Their Composites: A Review of Synthesizing, Properties and Applications. Materials 2014, 7, 3919–3945. [Google Scholar] [CrossRef]
- Meek, N.; Penumadu, D.; Hosseinaei, O.; Harper, D.; Young, S.; Rials, T. Synthesis and Characterization of Lignin Carbon Fiber and Composites. Compos. Sci. Technol. 2016, 137, 60–68. [Google Scholar] [CrossRef]
- Lee, K.M.; Lee, S.E.; Lee, Y.S. Improved Mechanical and Electromagnetic Interference Shielding Properties of Epoxy Composites through the Introduction of Oxyfluorinated Multiwalled Carbon Nanotubes. J. Ind. Eng. Chem. 2017, 56, 435–442. [Google Scholar] [CrossRef]
- Santos, P.; Maceiras, A.; Reis, P.N.B. Influence of Manufacturing Parameters on the Mechanical Properties of Nano-Reinforced CFRP by Carbon Nanofibers. IOP Conf. Ser. Mater. Sci. Eng. 2021, 1126, 012012. [Google Scholar] [CrossRef]
- Shokrieh, M.M.; Esmkhani, M.; Vahedi, F.; Shahverdi, H.R. Improvement of Mechanical and Electrical Properties of Epoxy Resin with Carbon Nanofibers. Iran. Polym. J. 2013, 22, 721–727. [Google Scholar] [CrossRef]
- Sun, L.-H.; Ounaies, Z.; Gao, X.-L.; Whalen, C.A.; Yang, Z.-G. Preparation, Characterization, and Modeling of Carbon Nanofiber/Epoxy Nanocomposites. J. Nanomater. 2011, 2011, 1–8. [Google Scholar] [CrossRef] [Green Version]
- Chen, D.L.; Yang, P.F.; Lai, Y.S. A Review of Three-Dimensional Viscoelastic Models with an Application to Viscoelasticity Characterization Using Nanoindentation. Microelectron. Reliab. 2012, 52, 541–558. [Google Scholar] [CrossRef]
- Reis, P.N.B.; Silva, M.P.; Santos, P.; Parente, J.M.; Bezazi, A. Viscoelastic Behaviour of Composites with Epoxy Matrix Filled by Cork Powder. Compos. Struct. 2020, 234, 111669. [Google Scholar] [CrossRef]
- Farzaneh, A.; Rostami, A.; Nazockdast, H. Mono-Filler and Bi-Filler Composites Based on Thermoplastic Polyurethane, Carbon Fibers and Carbon Nanotubes with Improved Physicomechanical and Engineering Properties. Polym. Int. 2022, 71, 232–242. [Google Scholar] [CrossRef]
- Rana, S.; Alagirusamy, R.; Joshi, M. Mechanical Behavior of Carbon Nanofibre-Reinforced Epoxy Composites. J. Appl. Polym. Sci. 2010, 48, 2276–2283. [Google Scholar] [CrossRef]
- Oberdisse, J. Aggregation of Colloidal Nanoparticles in Polymer Matrices. Soft Matter 2006, 2, 29–36. [Google Scholar] [CrossRef] [PubMed]
- Padmanabhan, V.; Frischknecht, A.L.; Mackay, M.E. Effect of Chain Stiffness on Nanoparticle Segregation in Polymer/Nanoparticle Blends Near a Substrate. Macromol. Theory Simul. 2012, 21, 98–105. [Google Scholar] [CrossRef]
- Zare, Y. The Roles of Nanoparticles Accumulation and Interphase Properties in Properties of Polymer Particulate Nanocomposites by a Multi-Step Methodology. Compos. Part A Appl. Sci. Manuf. 2016, 91, 127–132. [Google Scholar] [CrossRef]
- Ma, X.; Zare, Y.; Rhee, K.Y. A Two-Step Methodology to Study the Influence of Aggregation/Agglomeration of Nanoparticles on Young’s Modulus of Polymer Nanocomposites. Nanoscale Res. Lett. 2017, 12, 621. [Google Scholar] [CrossRef] [PubMed]
- Shaffer, M.S.P.; Fan, X.; Windle, A.H. Dispersion and Packing of Carbon Nanotubes. Carbon 1998, 36, 1603–1612. [Google Scholar] [CrossRef]
- Fiedler, B.; Gojny, F.H.; Wichmann, M.H.G.; Nolte, M.C.M.; Schulte, K. Fundamental Aspects of Nano-Reinforced Composites. Compos. Sci. Technol. 2006, 66, 3115–3125. [Google Scholar] [CrossRef]
- Zhou, T.H.; Ruan, W.H.; Yang, J.L.; Rong, M.Z.; Zhang, M.Q.; Zhang, Z. A Novel Route for Improving Creep Resistance of Polymers Using Nanoparticles. Compos. Sci. Technol. 2007, 67, 2297–2302. [Google Scholar] [CrossRef]
- Lim, S.D.; Rhee, J.M.; Nah, C.; Lee, S.-H.; Lyu, M.-Y. Predicting the Long-Term Creep Behavior of Plastics Using the Short-Term Creep Test. Int. Polym. Process. 2004, 19, 313–319. [Google Scholar] [CrossRef]
- Zhang, Z.; Yang, J.-L.; Friedrich, K. Creep Resistant Polymeric Nanocomposites. Polymer 2004, 45, 3481–3485. [Google Scholar] [CrossRef]
- Yang, J.-L.; Zhang, Z.; Schlarb, A.K.; Friedrich, K. On the Characterization of Tensile Creep Resistance of Polyamide 66 Nanocomposites. Part I. Experimental Results and General Discussions. Polymer 2006, 47, 2791–2801. [Google Scholar] [CrossRef]
- Park, B.-D.; Balatinecz, J.J. Short Term Flexural Creep Behavior of Wood-Fiber/Polypropylene Composites. Polym. Compos. 1998, 19, 377–382. [Google Scholar] [CrossRef]
- Houshyar, S.; Shanks, R.A.; Hodzic, A. Tensile Creep Behaviour of Polypropylene Fibre Reinforced Polypropylene Composites. Polym. Test. 2005, 24, 257–264. [Google Scholar] [CrossRef]
- Wang, W.-H.; Huang, H.-B.; Du, H.-H.; Wang, H. Effects of Fiber Size on Short-Term Creep Behavior of Wood Fiber/HDPE Composites. Polym. Eng. Sci. 2015, 55, 693–700. [Google Scholar] [CrossRef]
- Jian, W.; Lau, D. Creep Performance of CNT-Based Nanocomposites: A Parametric Study. Carbon 2019, 153, 745–756. [Google Scholar] [CrossRef]
- Bouafif, H.; Koubaa, A.; Perré, P.; Cloutier, A. Creep Behaviour of HDPE/Wood Particle Composites. Int. J. Microstruct. Mater. Prop. 2013, 8, 225. [Google Scholar] [CrossRef]
- Vlasveld, D.P.N.; Bersee, H.E.N.; Picken, S.J. Creep and Physical Aging Behaviour of PA6 Nanocomposites. Polymer 2005, 46, 12539–12545. [Google Scholar] [CrossRef]
- Yang, J.; Zhang, Z.; Friedrich, K.; Schlarb, A.K. Creep Resistant Polymer Nanocomposites Reinforced with Multiwalled Carbon Nanotubes. Macromol. Rapid Commun. 2007, 28, 955–961. [Google Scholar] [CrossRef]
- Ferreira, J.A.; Costa, J.D.; Reis, P.N. Static and Fatigue Behaviour of Glass-Fibre-Reinforced Polypropylene Composites. Theor. Appl. Fract. Mech. 1999, 31, 67–74. [Google Scholar] [CrossRef]
- Reis, P.N.B.; Gorbatikh, L.; Ivens, J.; Lomov, S.V. Strain-Rate Sensitivity and Stress Relaxation of Hybrid Self-Reinforced Polypropylene Composites under Bending Loads. Compos. Struct. 2019, 209, 802–810. [Google Scholar] [CrossRef]
- Varghese, S.; Kuriakose, B.; Thomas, S. Stress Relaxation in Short Sisal-fiber-reinforced Natural Rubber Composites. J. Appl. Polym. Sci. 1994, 53, 1051–1060. [Google Scholar] [CrossRef]
- George, J.; Sreekala, M.S.; Thomas, S.; Bhagawan, S.S.; Neelakantan, N.R. Stress Relaxation Behavior of Short Pineapple Fiber Reinforced Polyethylene Composites. J. Reinf. Plast. Compos. 1998, 17, 651–672. [Google Scholar] [CrossRef]
- Sreekala, M.S.; Kumaran, M.G.; Joseph, R.; Thomas, S. Stress-Relaxation Behaviour in Composites Based on Short Oil-Palm Fibres and Phenol Formaldehyde Resin. Compos. Sci. Technol. 2001, 61, 1175–1188. [Google Scholar] [CrossRef]
- Scott, D.W.; Lai, J.S.; Zureick, A.H. Creep Behavior of Fiber-Reinforced Polymeric Composites: A Review of the Technical Literature. J. Reinf. Plast. Compos. 1995, 14, 588–617. [Google Scholar] [CrossRef]
- Xu, Y.; Wu, Q.; Lei, Y.; Yao, F. Creep Behavior of Bagasse Fiber Reinforced Polymer Composites. Bioresour. Technol. 2010, 101, 3280–3286. [Google Scholar] [CrossRef] [PubMed]
- Georgiopoulos, P.; Kontou, E.; Christopoulos, A. Short-Term Creep Behavior of a Biodegradable Polymer Reinforced with Wood-Fibers. Compos. Part B Eng. 2015, 80, 134–144. [Google Scholar] [CrossRef]
- Vidya Bhushan Gupta; Lahiri, J. Non Linear Viscoelastic Behavior of Polypropylene and Glass Reinforced Polypropylene in Creep. J. Compos. Mater. 1980, 14, 286–296. [Google Scholar] [CrossRef]
- Almeida, J.H.S.; Ornaghi, H.L.; Lorandi, N.P.; Bregolin, B.P.; Amico, S.C. Creep and Interfacial Behavior of Carbon Fiber Reinforced Epoxy Filament Wound Laminates. Polym. Compos. 2018, 39, E2199–E2206. [Google Scholar] [CrossRef]
- Reis, P.N.B.; Silva, M.P.; Santos, P.; Parente, J.M.; Valvez, S.; Bezazi, A. Mechanical Performance of an Optimized Cork Agglomerate Core-Glass Fibre Sandwich Panel. Compos. Struct. 2020, 245, 112375. [Google Scholar] [CrossRef]
- Vaidyanathan, T.K.; Vaidyanathan, J. Validity of Predictive Models of Stress Relaxation in Selected Dental Polymers. Dent. Mater. 2015, 31, 799–806. [Google Scholar] [CrossRef] [PubMed]
- Reis, P.N.B.; Neto, M.A.; Amaro, A.M. Effect of Hostile Solutions on Stress Relaxation of Carbon/Epoxy Composites. Polym. Degrad. Stab. 2019, 165, 60–67. [Google Scholar] [CrossRef]
- Reis, P.N.B.; Silva, M.P.; Santos, P. Stress Relaxation in Delaminated Carbon/Epoxy Composites. Fibers Polym. 2019, 20, 1284–1289. [Google Scholar] [CrossRef]


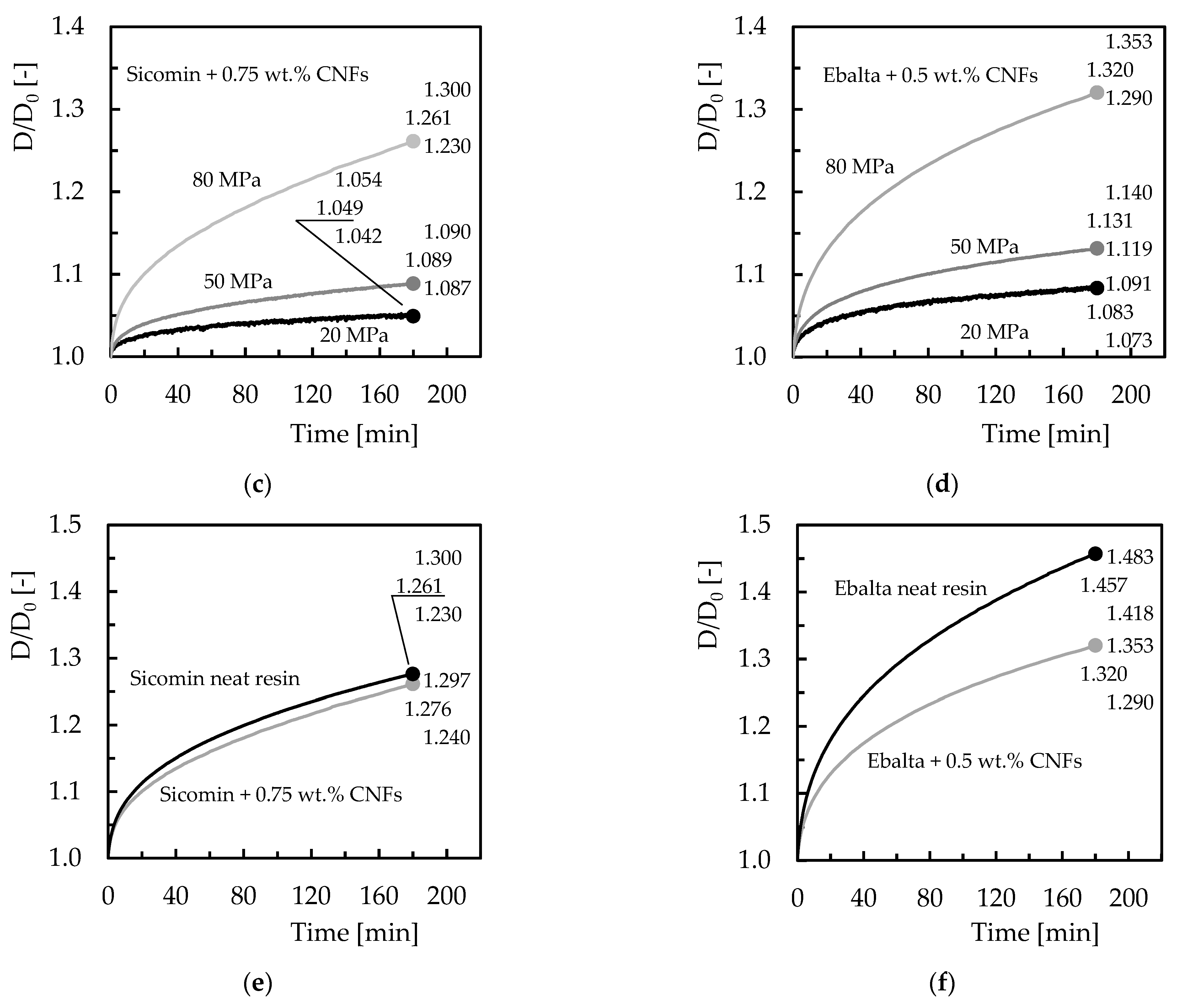
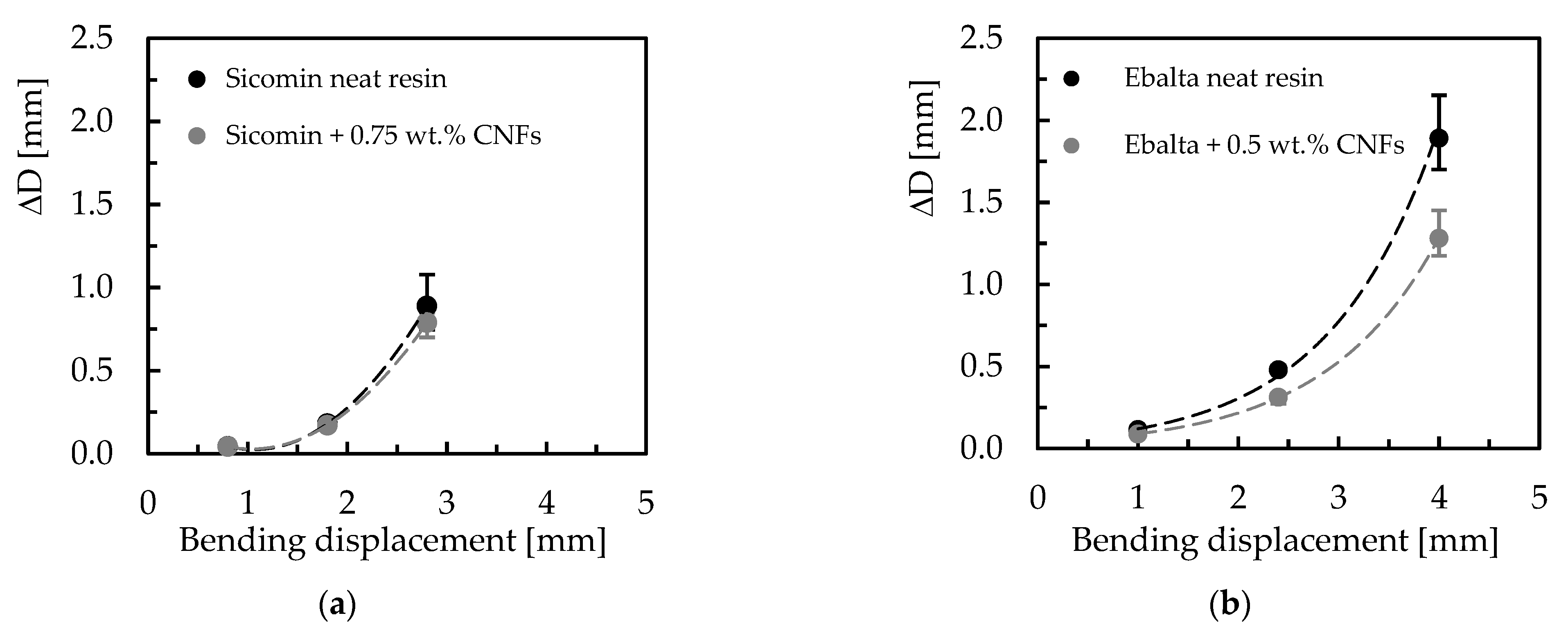
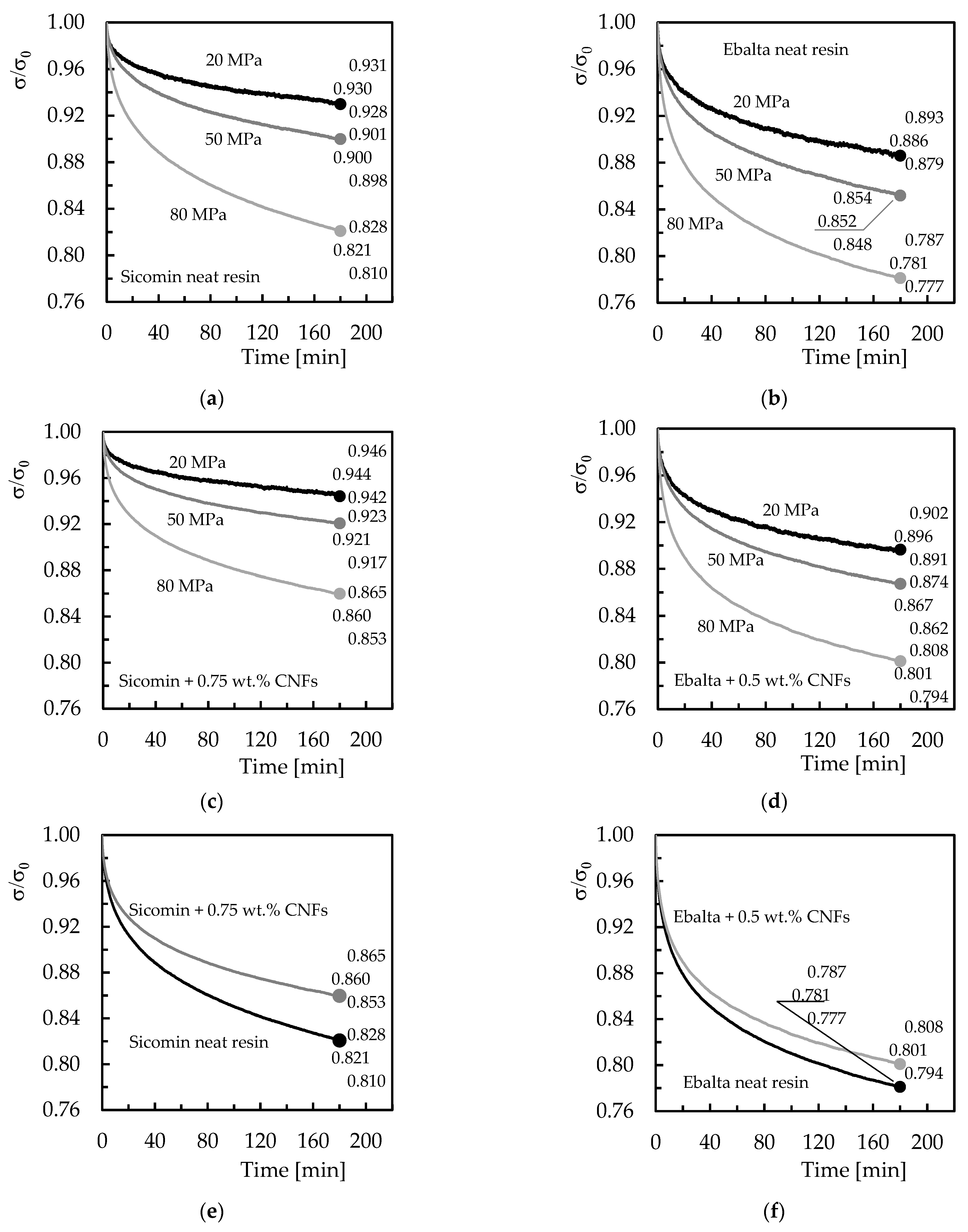


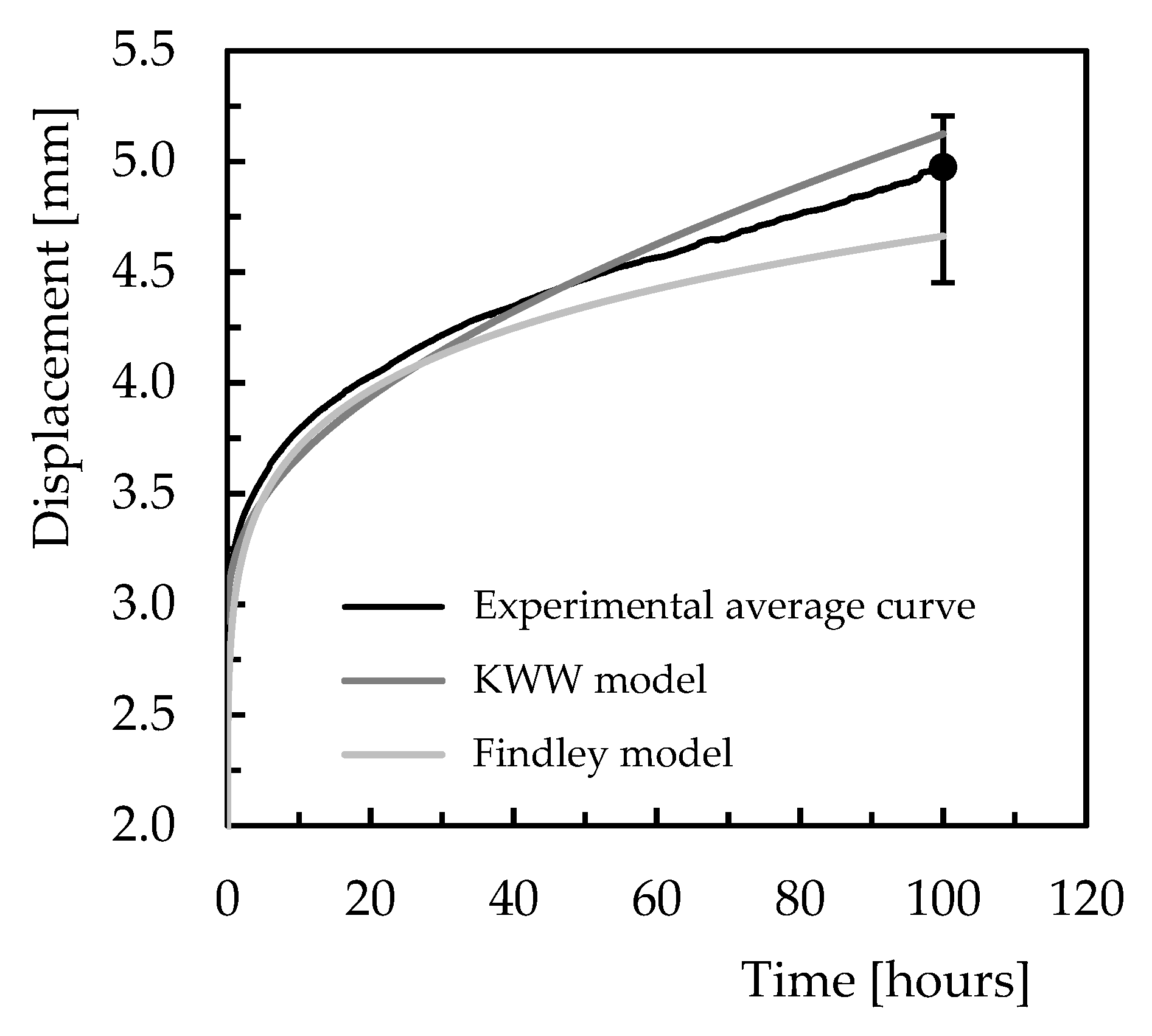



| Bending Stress [MPa] | β | τ | Displacement after 3 h [mm] | |||
|---|---|---|---|---|---|---|
| Experimental Value | KWW Value | Error [%] | ||||
| Sicomin resin | ||||||
| 20 | 0.892 | 0.333 | 1.37 × 106 | 0.936 | 0.939 | 0.319 |
| 50 | 2.03 | 0.392 | 8.94 × 104 | 2.21 | 2.22 | 0.253 |
| 80 | 3.40 | 0.431 | 5.12 × 103 | 4.29 | 4.31 | 0.409 |
| Sicomin resin + 0.75 wt.% CNFs | ||||||
| 20 | 0.765 | 0.318 | 1.50 × 106 | 0.807 | 0.809 | 0.216 |
| 50 | 1.81 | 0.416 | 5.44 × 104 | 1.98 | 1.99 | 0.437 |
| 80 | 2.86 | 0.417 | 4.86 × 103 | 3.64 | 3.68 | 0.949 |
| Ebalta resin | ||||||
| 20 | 1.06 | 0.362 | 8.72 × 104 | 1.18 | 1.18 | 0.36 |
| 50 | 2.67 | 0.407 | 1.27 × 104 | 3.16 | 3.19 | 0.954 |
| 80 | 4.02 | 0.430 | 1.53 × 103 | 5.83 | 5.99 | 2.75 |
| Ebalta resin + 0.5 wt.% CNFs | ||||||
| 20 | 1.07 | 0.335 | 2.89 × 105 | 1.16 | 1.16 | 0.444 |
| 50 | 2.37 | 0.385 | 3.38 × 104 | 2.68 | 2.71 | 0.983 |
| 80 | 3.51 | 0.409 | 6.90 × 103 | 4.34 | 4.39 | 1.29 |
| Bending Stress [MPa] | A | n | Displacement after 3 h [mm] | |||
|---|---|---|---|---|---|---|
| Experimental Value | Findley Value | Error [%] | ||||
| Sicomin resin | ||||||
| 20 | 0.406 | 0.454 | 0.017 | 0.936 | 0.935 | 0.035 |
| 50 | 0.979 | 0.873 | 0.037 | 2.21 | 2.21 | 0.253 |
| 80 | 0.780 | 1.913 | 0.063 | 4.29 | 4.22 | 1.67 |
| Sicomin resin + 0.75 wt.% CNFs | ||||||
| 20 | 0.368 | 0.367 | 0.019 | 0.807 | 0.806 | 0.135 |
| 50 | 0.822 | 0.847 | 0.033 | 1.98 | 1.97 | 0.600 |
| 80 | 0.792 | 1.477 | 0.069 | 3.64 | 3.60 | 1.27 |
| Ebalta resin | ||||||
| 20 | 0.414 | 0.563 | 0.032 | 1.18 | 1.17 | 0.418 |
| 50 | 0.796 | 1.500 | 0.048 | 3.16 | 3.13 | 0.829 |
| 80 | 0.932 | 2.042 | 0.091 | 5.83 | 5.71 | 2.087 |
| Ebalta resin + 0.5 wt.% CNFs | ||||||
| 20 | 0.427 | 0.576 | 2.49 × 10−2 | 1.16 | 1.15 | 0.203 |
| 50 | 0.726 | 1.410 | 3.45 × 10−2 | 2.68 | 2.67 | 0.536 |
| 80 | 0.827 | 2.053 | 5.63 × 10−2 | 4.34 | 4.29 | 1.049 |
| Material | β | τ | |||||||
|---|---|---|---|---|---|---|---|---|---|
| A | B | R | C | D | R | E | F | R | |
| Sicomin | |||||||||
| Neat resin | 0.042 | 0.018 | 0.997 | 1.64 × 10−3 | 0.304 | 0.993 | 9.01 × 106 | −0.093 | 0.999 |
| Resin + 0.75 wt.% CNFs | 0.035 | 0.069 | 0.999 | 1.64 × 10−3 | 0.301 | 0.869 | 8.74 × 106 | −0.096 | 0.996 |
| Ebalta | |||||||||
| Neat resin | 0.049 | 0.120 | 0.999 | 1.13 × 10−3 | 0.343 | 0.982 | 3.46 × 105 | −0.067 | 0.999 |
| Resin + 0.5 wt.% CNFs | 0.041 | 0.282 | 0.999 | 1.24 × 10−3 | 0.314 | 0.980 | 9.15 × 105 | −0.062 | 0.999 |
| Material | A | n | |||||||
|---|---|---|---|---|---|---|---|---|---|
| A | B | R | C | D | R | E | F | R | |
| Sicomin | |||||||||
| Neat resin | 6.24 × 10−3 | 0.410 | 0.643 | 0.024 | −0.136 | 0.971 | 7.77 × 10−4 | 3.26 × 10−5 | 0.997 |
| Resin + 0.75 wt.% CNFs | 7.06 × 10−3 | 0.308 | 0.834 | 0.018 | −0.028 | 0.997 | 8.35 × 10−4 | −1.43 × 10−3 | 0.969 |
| Ebalta | |||||||||
| Neat resin | 8.64 × 10−3 | 0.282 | 0.964 | 0.025 | 0.136 | 0.988 | 9.85 × 10−4 | 7.98 × 10−3 | 0.964 |
| Resin + 0.5 wt.% CNFs | 6.66 × 10−3 | 0.327 | 0.961 | 0.025 | 0.116 | 0.997 | 5.24 × 10−4 | 1.24 × 10−2 | 0.976 |
| Initial Bending Stress [MPa] | β | τ | Bending Stress after 3 h [MPa] | ||
|---|---|---|---|---|---|
| Experimental Value | KWW Value | Error [%] | |||
| Sicomin neat resin | |||||
| 20 | 0.321 | 6.36 × 105 | 18.62 | 18.63 | 0.012 |
| 50 | 0.388 | 5.56 × 104 | 45.07 | 44.90 | 0.378 |
| 80 | 0.394 | 1.15 × 104 | 66.24 | 65.88 | 0.554 |
| Sicomin resin + 0.75 wt.% CNFs | |||||
| 20 | 0.312 | 1.86 × 106 | 18.94 | 18.97 | 0.163 |
| 50 | 0.360 | 1.69 × 105 | 46.07 | 45.96 | 0.225 |
| 80 | 0.361 | 3.04 × 104 | 68.64 | 68.36 | 0.059 |
| Ebalta neat resin | |||||
| 20 | 0.338 | 8.57 × 104 | 17.73 | 17.68 | 0.299 |
| 50 | 0.376 | 2.12 × 104 | 42.59 | 42.33 | 0.622 |
| 80 | 0.349 | 8.60 × 103 | 62.47 | 61.69 | 1.255 |
| Ebalta resin + 0.5 wt.% CNFs | |||||
| 20 | 0.328 | 1.33 × 105 | 17.96 | 17.86 | 0.520 |
| 50 | 0.361 | 3.48 × 104 | 43.32 | 43.01 | 0.523 |
| 80 | 0.359 | 1.66 × 104 | 66.28 | 65.66 | 0.934 |
| Material | β | τ | ||||
|---|---|---|---|---|---|---|
| A | B | R | C | D | R | |
| Sicomin | ||||||
| Neat resin | 1.21 × 10−3 | 0.307 | 0.897 | 2.10 × 106 | −0.067 | 0.994 |
| Epoxy + 0.75 wt.% CNFs | 8.16 × 10−4 | 0.304 | 0.874 | 6.55 × 106 | −0.069 | 0.995 |
| Ebalta | ||||||
| Neat resin | 1.73 × 10−3 | 0.345 | 0.270 | 1.19 × 107 | −1.64 | 0.998 |
| Epoxy + 0.5 wt.% CNFs | 5.14 × 10−4 | 0.323 | 0.838 | 1.19 × 107 | −1.49 | 1.000 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Santos, P.; Silva, A.P.; Reis, P.N.B. Effect of Carbon Nanofibers on the Viscoelastic Response of Epoxy Resins. Polymers 2023, 15, 821. https://doi.org/10.3390/polym15040821
Santos P, Silva AP, Reis PNB. Effect of Carbon Nanofibers on the Viscoelastic Response of Epoxy Resins. Polymers. 2023; 15(4):821. https://doi.org/10.3390/polym15040821
Chicago/Turabian StyleSantos, Paulo, Abílio P. Silva, and Paulo N. B. Reis. 2023. "Effect of Carbon Nanofibers on the Viscoelastic Response of Epoxy Resins" Polymers 15, no. 4: 821. https://doi.org/10.3390/polym15040821
APA StyleSantos, P., Silva, A. P., & Reis, P. N. B. (2023). Effect of Carbon Nanofibers on the Viscoelastic Response of Epoxy Resins. Polymers, 15(4), 821. https://doi.org/10.3390/polym15040821








