Greenhouse Natural Ventilation Models: How Do We Develop with Chinese Greenhouses?
Abstract
1. Introduction
2. Current Models of Wind-Induced Ventilation
2.1. Theoretical Models
2.2. Application Scenarios
2.2.1. Mixing Ventilation
2.2.2. Displacement Ventilation
2.2.3. Cross-Ventilation
2.3. Factors Influencing Ventilation Rates
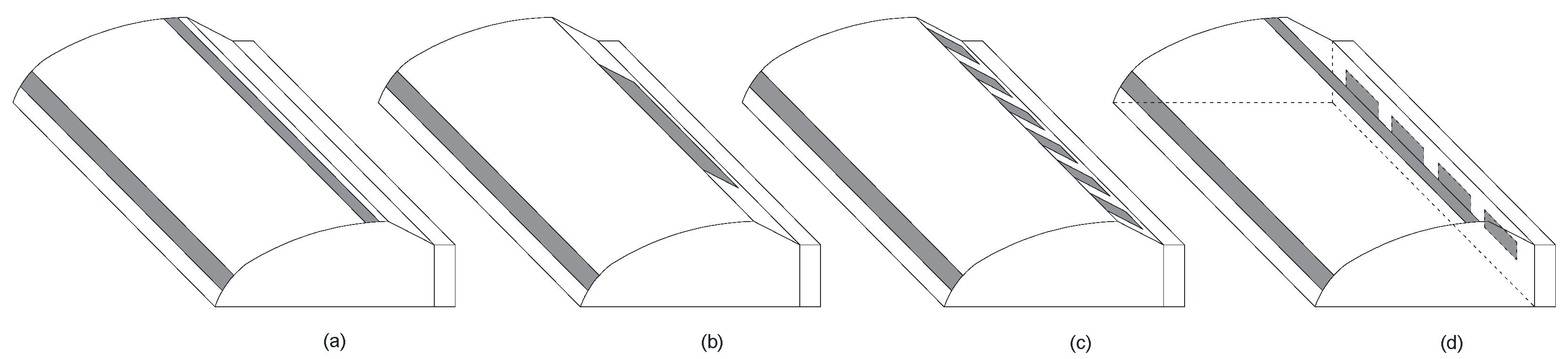
2.3.1. Vent Size, Shape, and Arrangement
2.3.2. Insect Screens
2.3.3. Plants and Their Orientation
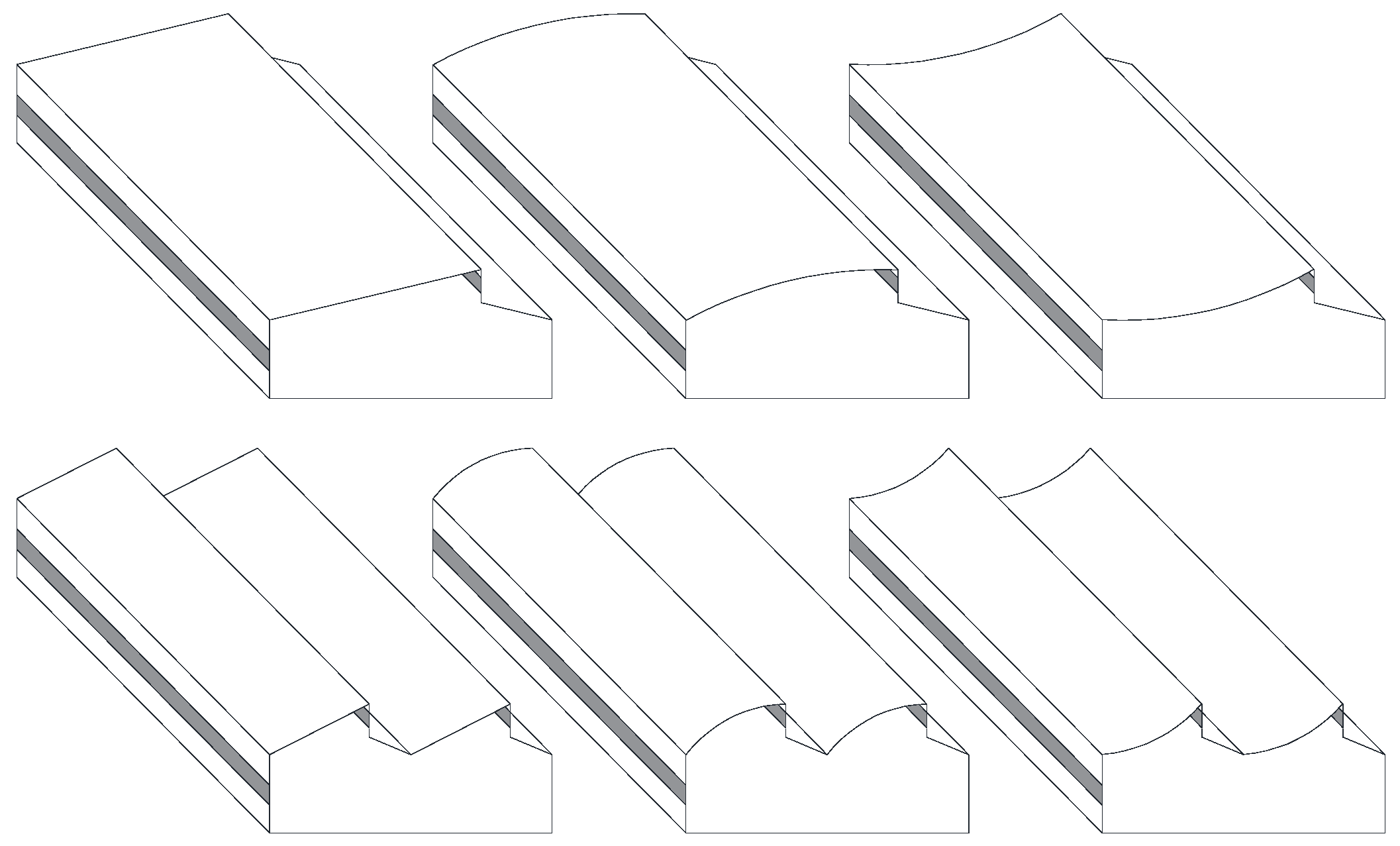
2.3.4. Roof Geometry and Slope
2.3.5. Wind Direction
3. Current Models of Buoyancy-Induced Ventilation
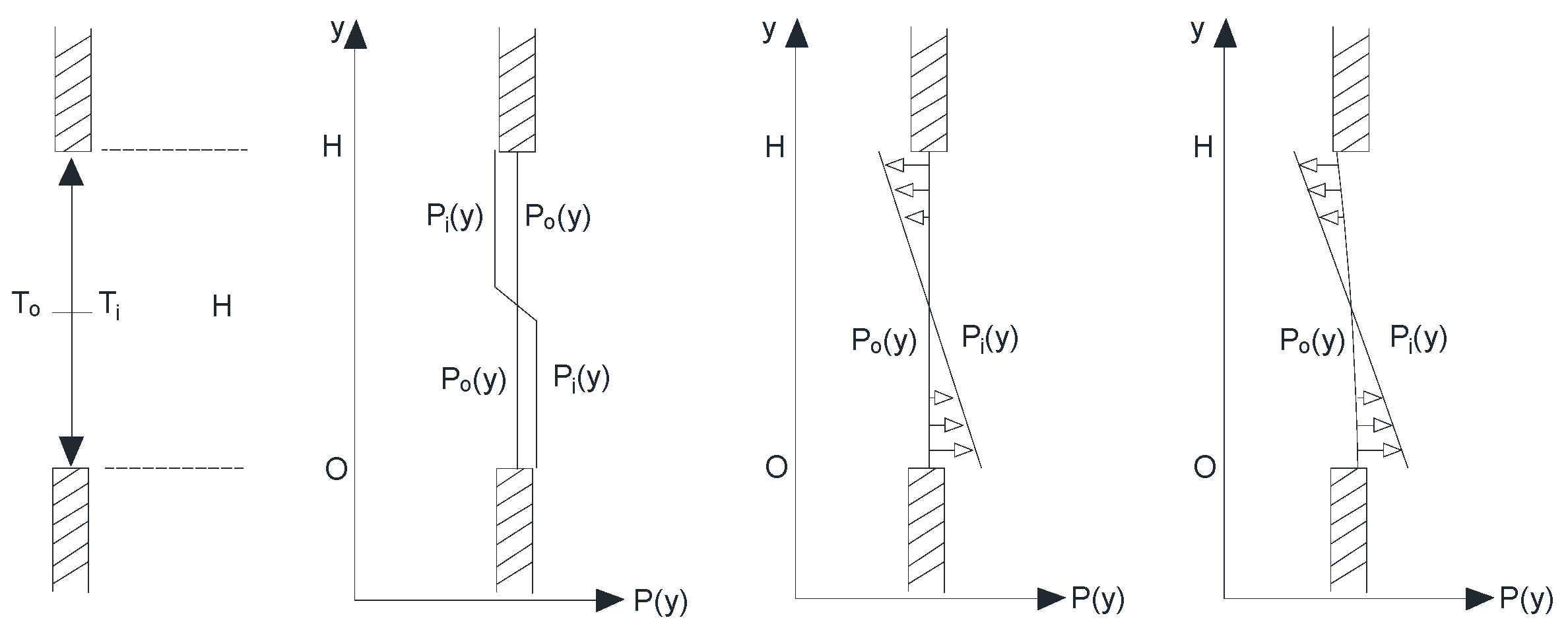
3.1. Theoretical Models
3.2. Application Scenarios
3.2.1. Vertical Openings
3.2.2. Horizontal Openings
3.3. Factors Influencing Ventilation Rates
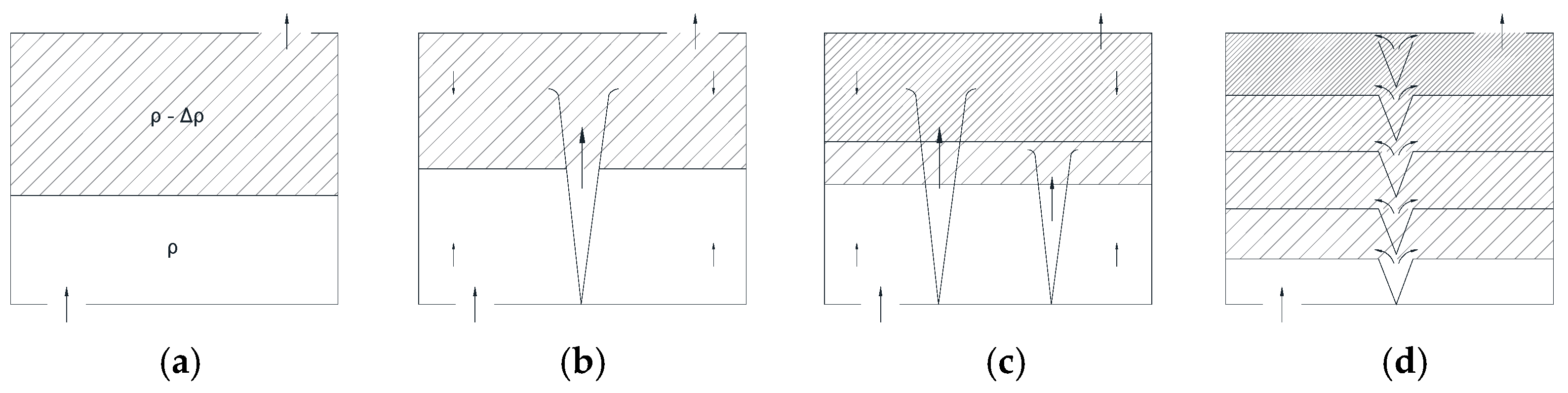
3.3.1. Buoyancy Source Strength and Distribution
3.3.2. Vent Size, Shape, and Arrangement
4. Current Natural Ventilation Models and Methods of Measurements
4.1. Theoretical Models
4.2. Methods of Measuring Ventilation Rates
4.2.1. Tracer Gas Technique
4.2.2. Pressure Difference Method
4.2.3. Energy Balance Simulation Method
4.2.4. Numerical Simulation Method
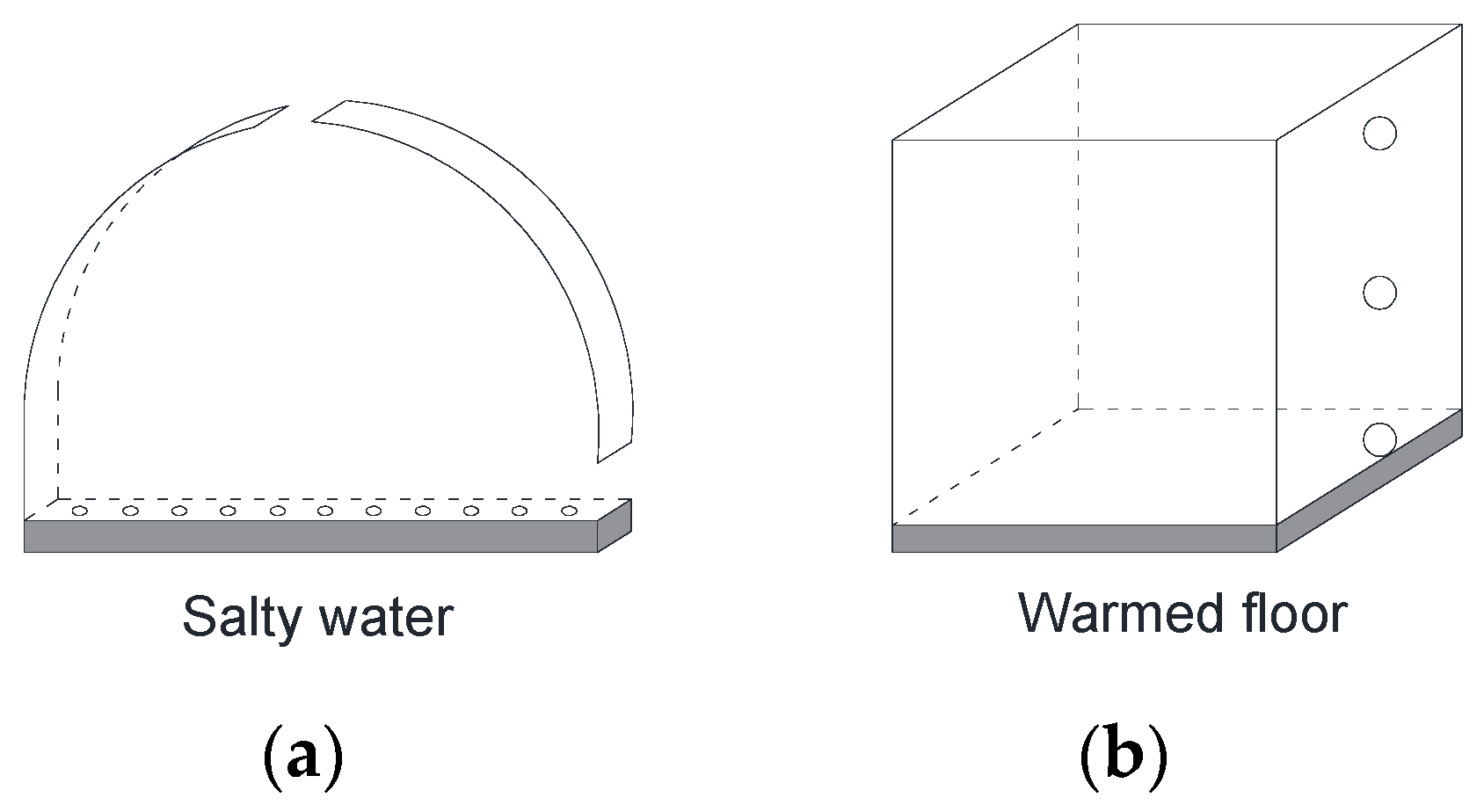
4.2.5. Emptying Fluid-Filling Box Method
4.3. Critical Wind Speed Determining Driving Forces of Natural Ventilation
| References | Ventilation Configuration | Opening Geometry | Driving Force | Critical Wind Speed (m/s) |
|---|---|---|---|---|
| Meneses and Raposo [184] | Displacement | Continuous | Buoyancy | 0.5–1.5 |
| Meneses and Raposo [184] | Displacement | Continuous | Wind | >1.5 |
| Boulard and Baille [17] | Mixing | Continuous | Combined | 0.5–2 |
| Boulard and Baille [17] | Mixing | Continuous | Buoyancy | 0–0.5 |
| Boulard, et al. [34] | Mixing | Continuous | Wind | >1.2 |
| Kittas, et al. [32] | Mixing | Continuous | Wind | >1.5 |
| Baptista, et al. [16] | Mixing | Discontinuous | Buoyancy | 0–1 |
5. Discussion
5.1. Further Research on Ventilation Models of Greenhouses with Horizontal Openings (CSG)
5.2. Further Research on Cross-Ventilation Models (CPG)
5.3. Influence of the Porosity and Height of Plants on Ventilation Models
5.4. More Variables Considered in Ventilation Models
5.5. Application of Ventilation Model in Greenhouse Climate Prediction
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Qi, F.; Ding, X.; Lu, S.; Yin, Y.; Lian, Q.; Wei, X.; Li, K. National Greenhouse Data System; Institute of Facility Agriculture, Planning and Design Institute, Ministry of Agriculture: Beijing, China, 2014.
- Mistriotis, A.; Arcidiacono, C.; Picuno, P.; Bot, G.P.A.; Scarascia Mugnozza, G. Computational analysis of ventilation in greenhouses at zero-and low-wind-speeds. Agric. For. Meteorol. 1997, 88, 121–135. [Google Scholar] [CrossRef]
- Linden, P.F.; Lane-Serff, G.F.; Smeed, D.A. Emptying filling boxes: The fluid mechanics of natural ventilation. J. Fluid Mech. 1990, 212, 309–335. [Google Scholar] [CrossRef]
- von Zabeltitz, C. Energy Conservation and Renewable Energies for Greenhouse Heating. CNRE Guideline, No. 2; REUR Technical Series No. 3; FAO: Rome, Italy, 1989. [Google Scholar]
- Boulard, T. Greenhouse natural ventilation modelling: A survey of the different approaches. In Proceedings of the International Symposium on Greenhouse Cooling 719, Almeria, Spain, 24–27 April 2006; pp. 29–30. [Google Scholar]
- Sethi, V.P.; Sharma, S.K. Survey of cooling technologies for worldwide agricultural greenhouse applications. Sol. Energy 2007, 81, 1447–1459. [Google Scholar] [CrossRef]
- Norton, T.; Sun, D.W.; Grant, J.; Fallon, R.; Dodd, V. Applications of computational fluid dynamics (CFD) in the modelling and design of ventilation systems in the agricultural industry: A review. Bioresour. Technol. 2007, 98, 2386–2414. [Google Scholar] [CrossRef] [PubMed]
- Bournet, P.E.; Boulard, T. Effect of ventilator configuration on the distributed climate of greenhouses: A review of experimental and CFD studies. Comput. Electron. Agric. 2010, 74, 195–217. [Google Scholar] [CrossRef]
- Khanal, R.; Lei, C. Solar chimney—A passive strategy for natural ventilation. Energy Build. 2011, 43, 1811–1819. [Google Scholar] [CrossRef]
- Sandberg, M.; Moshfegh, B. Ventilated-solar roof air flow and heat transfer investigation. Renew. Energy 1998, 15, 287–292. [Google Scholar] [CrossRef]
- Rong, L.; Bjerg, B.; Batzanas, T.; Zhang, G. Mechanisms of natural ventilation in livestock buildings: Perspectives on past achievements and future challenges. Biosyst. Eng. 2016, 151, 200–217. [Google Scholar] [CrossRef]
- Akrami, M.; Salah, A.H.; Javadi, A.A.; Fath, H.E.S.; Hassanein, M.J.; Farmani, R.; Dibaj, M.; Negm, A. Towards a sustainable greenhouse: Review of trends and emerging practices in analysing greenhouse ventilation requirements to sustain maximum agricultural yield. Sustainability 2020, 12, 2794. [Google Scholar] [CrossRef]
- Sakiyama, N.R.M.; Carlo, J.C.; Frick, J.; Garrecht, H. Perspectives of naturally ventilated buildings: A review. Renew. Sustain. Energy Rev. 2020, 130, 109933. [Google Scholar] [CrossRef]
- Wang, C.; Nan, B.; Wang, T.; Bai, Y.; Li, Y. Wind pressure acting on greenhouses: A review. Int. J. Agric. Biol. Eng. 2021, 14, 1–8. [Google Scholar] [CrossRef]
- Zhong, H.-Y.; Sun, Y.; Shang, J.; Qian, F.-P.; Zhao, F.-Y.; Kikumoto, H.; Jimenez-Bescos, C.; Liu, X. Single-sided natural ventilation in buildings: A critical literature review. Build. Environ. 2022, 212, 108797. [Google Scholar] [CrossRef]
- Baptista, F.J.; Bailey, B.J.; Randall, J.M.; Meneses, J.F. Greenhouse ventilation rate: Theory and measurement with tracer gas techniques. J. Agric. Eng. Res. 1999, 72, 363–374. [Google Scholar] [CrossRef]
- Boulard, T.; Baille, A. Modelling of air exchange rate in a greenhouse equipped with continuous roof vents. J. Agric. Eng. Res. 1995, 61, 37–47. [Google Scholar] [CrossRef]
- Kwon, K.S.; Lee, I.B.; Han, H.T.; Shin, C.Y.; Hwang, H.S.; Hong, S.W.; Bitog, J.P.; Seo, I.H.; Han, C.P. Analysing ventilation efficiency in a test chamber using age-of-air concept and CFD technology. Biosyst. Eng. 2011, 110, 421–433. [Google Scholar] [CrossRef]
- Bot, G.P.A. Greenhouse Climate: From Physical Processes to a Dynamic Model. Ph.D. Thesis, Wageningen University, Wageningen, The Netherlands, 1983. [Google Scholar]
- De Jong, T. Natural Ventilation of Large Multi-Span Greenhouses. Ph.D. Thesis, Wageningen University, Wageningen, The Netherlands, 1990. [Google Scholar]
- Fernandez, J.E.; Bailey, B.J. Predicting greenhouse ventilation rates. In Proceedings of the International Workshop on Greenhouse Crop Models 328, Saumane, France, 25–29 August 1991; pp. 107–114. [Google Scholar]
- Boulard, T.; Baille, A.; Draoui, B. Greenhouse Natural Ventilation Measurements and Modelling; International Workshop Agritech, Agriculture Research Organisation: Sophia Antipolis, France, 1993. [Google Scholar]
- Bruce, J.M. Natural convection through openings and its application to cattle building ventilation. J. Agric. Eng. Res. 1978, 23, 151–167. [Google Scholar] [CrossRef]
- Gong, B.; Ma, C.; Li, Z. Confirmation the Flow Coefficient of Greenhouse Ventilation by Computational Fluid Dynamics Technique. J. Agric. Mech. Res. 2010, 32, 11–15+74. [Google Scholar]
- Kozai, T.; Sase, S.; Nara, M. A modeling approach to greenhouse environmental control by ventilation. Acta Hort 1980, 106, 125–136. [Google Scholar] [CrossRef]
- Sase, S.; Takakura, T.; Nara, M. Wind tunnel testing on airflow and temperature distribution of a naturally ventilated greenhouse. In Proceedings of the III International Symposium on Energy in Protected Cultivation 148, Columbus, OH, USA, 21–26 August 1983; pp. 329–336. [Google Scholar]
- Fernandez, J.E.; Bailey, B.J. Measurement and prediction of greenhouse ventilation rates. Agric. For. Meteorol. 1992, 58, 229–245. [Google Scholar] [CrossRef]
- Bruce, J.M. Ventilation of a model livestock building by thermal buoyancy. Trans. ASAE 1982, 25, 1724–1726. [Google Scholar] [CrossRef]
- Van Der Maas, J. Air Flow through Large Openings in Buildings; International Energy Agency: Bern, Switzerland, 1992. [Google Scholar]
- Monteith, J.L.; Unsworth, M.H. Principles of Environmental Physics, 2nd ed.; Edward Arnold: London, UK, 1990. [Google Scholar]
- Gandemer, J.; Bietry, J. Cours dáérodynamique REEF, Vol. 2. In Sciences du Bâtiment; CSTB Nantes: Paris, France, 1989. [Google Scholar]
- Kittas, C.; Boulard, T.; Mermier, M.; Papadakis, G. Wind induced air exchange rates in a greenhouse tunnel with continuous side openings. J. Agric. Eng. Res. 1996, 65, 37–49. [Google Scholar] [CrossRef]
- Papadakis, G.; Mermier, M.; Meneses, J.F.; Boulard, T. Measurement and analysis of air exchange rates in a greenhouse with continuous roof and side openings. Agric. Eng. Res. 1996, 63, 219–227. [Google Scholar] [CrossRef]
- Boulard, T.; Meneses, J.F.; Mermier, M.; Papadakis, G. The mechanisms involved in the natural ventilation of greenhouses. Agric. For. Meteorol. 1996, 79, 61–77. [Google Scholar] [CrossRef]
- Hellickson, M.A.; Hinkle, C.N.; Jedele, D.G. Natural ventilation. In Ventilation of Agricultural Structures; ASAE: St. Joseph, MI, USA, 1983. [Google Scholar]
- Albright, L.D. Environment Control for Animals and Plants; American Society of Agricultural Engineers: St. Joseph, MI, USA, 1990. [Google Scholar]
- Nääs, I.A.; Moura, D.J.; Bucklin, R.A.; Fialho, F.B. An algorithm for determining opening effectiveness in natural ventilation by wind. Trans. ASAE 1998, 41, 767. [Google Scholar] [CrossRef]
- Kittas, C.; Boulard, T.; Papadakis, G. Natural ventilation of a greenhouse with ridge and side openings: Sensitivity to temperature and wind effects. Trans. ASAE 1997, 40, 415–425. [Google Scholar] [CrossRef]
- Zhang, J.S.; Janni, K.A.; Jacobson, L.D. Modeling natural ventilation induced by combined thermal buoyancy and wind. Trans. ASAE 1989, 32, 2165–2174. [Google Scholar] [CrossRef]
- Kittas, C.; Draoui, B.; Boulard, T. Quantification du taux d’aération d’une serre à ouvrant continu en toiture. Agric. For. Meteorol. 1995, 77, 95–111. [Google Scholar] [CrossRef]
- Boulard, T.; Draoui, B. Natural ventilation of a greenhouse with continuous roof vents: Measurements and data analysis. J. Agric. Eng. Res. 1995, 61, 27–35. [Google Scholar] [CrossRef]
- Miguel, A.A.F. Transport Phenomena through Porous Screens and Openings: From Theory to Greenhouse Practice; Wageningen University and Research: Wageningen, The Netherlands, 1998. [Google Scholar]
- Muñoz, P.; Montero, J.I.; Antón, A.; Giuffrida, F. Effect of insect-proof screens and roof openings on greenhouse ventilation. J. Agric. Eng. Res. 1999, 73, 171–178. [Google Scholar] [CrossRef]
- Hunt, G.R.; Linden, P.F. The fluid mechanics of natural ventilation—displacement ventilation by buoyancy-driven flows assisted by wind. Build. Environ. 1999, 34, 707–720. [Google Scholar] [CrossRef]
- Oca, J.; Montero, J.I.; Anton, A.; Crespo, D. A method for studying natural ventilation by thermal effects in a tunnel greenhouse using laboratory-scale models. J. Agric. Eng. Res. 1999, 72, 93–104. [Google Scholar] [CrossRef]
- Teitel, M.; Tanny, J. Natural ventilation of greenhouses: Experiments and model. Agric. For. Meteorol. 1999, 96, 59–70. [Google Scholar] [CrossRef]
- Hunt, G.R.; Linden, P.F. Steady-state flows in an enclosure ventilated by buoyancy forces assisted by wind. J. Fluid Mech. 2001, 426, 355–386. [Google Scholar] [CrossRef]
- Gladstone, C.; Woods, A.W. On buoyancy-driven natural ventilation of a room with a heated floor. J. Fluid Mech. 2001, 441, 293–314. [Google Scholar] [CrossRef]
- Parra, J.P.; Baeza, E.; Montero, J.I.; Bailey, B.J. Natural ventilation of parral greenhouses. Biosyst. Eng. 2004, 87, 355–366. [Google Scholar] [CrossRef]
- Si, H.; Miao, X. Opening model of natural vent ilation windows for East-China Multi-span Plast ic Greenhouse and studies on it. J. Zhejiang Univ. 2005, 31, 113–118. [Google Scholar]
- Liu, S.; He, Y.; Zhang, Y.; Miao, X. Prediction and analysis model of temperature and its application to a natural ventilation multi-span plastic greenhouse equipped with insect-proof screen. J. Zhejiang Univ. Sci. B 2005, 6, 523. [Google Scholar] [CrossRef]
- Katsoulas, N.; Bartzanas, T.; Boulard, T.; Mermier, M.; Kittas, C. Effect of vent openings and insect screens on greenhouse ventilation. Biosyst. Eng. 2006, 93, 427–436. [Google Scholar] [CrossRef]
- Teitel, M.; Ziskind, G.; Liran, O.; Dubovsky, V.; Letan, R. Effect of wind direction on greenhouse ventilation rate, airflow patterns and temperature distributions. Biosyst. Eng. 2008, 101, 351–369. [Google Scholar] [CrossRef]
- Wang, S.; Wang, X. Ventilation rate of various vents in plastic covered multi-span greenhouse. Trans. Chin. Soc. Agric. Eng. 2009, 25, 248–252. [Google Scholar]
- Baeza, E.J.; Pérez-Parra, J.J.; Montero, J.I.; Bailey, B.J.; López, J.C.; Gázquez, J.C. Analysis of the role of sidewall vents on buoyancy-driven natural ventilation in parral-type greenhouses with and without insect screens using computational fluid dynamics. Biosyst. Eng. 2009, 104, 86–96. [Google Scholar] [CrossRef]
- Mashonjowa, E.; Ronsse, F.; Milford, J.R.; Lemeur, R.; Pieters, J.G. Measurement and simulation of the ventilation rates in a naturally ventilated Azrom-type greenhouse in Zimbabwe. Appl. Eng. Agric. 2010, 26, 475–488. [Google Scholar] [CrossRef]
- Teitel, M.; Wenger, E. Air exchange and ventilation efficiencies of a monospan greenhouse with one inflow and one outflow through longitudinal side openings. Biosyst. Eng. 2014, 119, 98–107. [Google Scholar] [CrossRef]
- Fang, H.; Yang, Q.; Zhang, Y.; Cheng, R.; Zhang, F.; Lu, W. Simulation on Ventilation Flux of Solar Greenhouse Based on the Coupling between Stack and Wind Effects. Chin. J. Agrometeorol. 2016, 37, 531–537. [Google Scholar]
- Chu, C.R.; Lan, T.W.; Tasi, R.K.; Wu, T.R.; Yang, C.K. Wind-driven natural ventilation of greenhouses with vegetation. Biosyst. Eng. 2017, 164, 221–234. [Google Scholar] [CrossRef]
- Chu, C.R.; Lan, T.W. Effectiveness of ridge vent to wind-driven natural ventilation in monoslope multi-span greenhouses. Biosyst. Eng. 2019, 186, 279–292. [Google Scholar] [CrossRef]
- Villagran, E.A.; Romero, E.J.B.; Bojacá, C.R. Transient CFD analysis of the natural ventilation of three types of greenhouses used for agricultural production in a tropical mountain climate. Biosyst. Eng. 2019, 188, 288–304. [Google Scholar] [CrossRef]
- Li, N.; Xue, X.; Li, H. The risk assessment of wind disaster of greenhouse in Shandong province based on information dissemination theory. J. Meteorol. Environ. 2018, 34, 149–155. [Google Scholar]
- Yang, Z.; Zhang, B.; Xue, X.; Huang, C.; Zhu, K. The wind tunnel test of plastic greenhouse and its surface wind pressure patterns. Acta Ecol. Sin. 2012, 32, 7730–7737. [Google Scholar] [CrossRef][Green Version]
- Yang, Z.Q.; Li, Y.X.; Xue, X.P.; Huang, C.R.; Zhang, B. Wind loads on single-span plastic greenhouses and solar greenhouses. HortTechnology 2013, 23, 622–628. [Google Scholar] [CrossRef]
- Wang, J.; Ding, W.; Wu, Y. Wind tunnel test of wind pressure distribution on mutual insert multi-greenhouse roof. Trans. CSAE 2008, 24, 230–234. [Google Scholar]
- Zhang, Q.; Yu, H.; Zhang, Z.; Dong, L.; Zhang, Q.; Shao, C.; Yang, K.; Wang, X. Airflow simulation in solar greenhouse using CFD model. Trans. Chin. Soc. Agric. Eng. 2012, 28, 166–171. [Google Scholar]
- Su, W. CFD Numerical Simulation about Effect of Natural Ventilation on Microclimate in Greenhouse. Master’s Thesis, Nanjing University of Information Science & Technology, Nanjing, China, 2016. [Google Scholar]
- Tong, G.; Christopher, D.M.; Li, B. Numerical modelling of temperature variations in a Chinese solar greenhouse. Comput. Electron. Agric. 2009, 68, 129–139. [Google Scholar] [CrossRef]
- Tian, Y. Study on Natural Ventilation and Enhancement in Single-Slope Solar Greenhouse. Master’s Thesis, Xi’an University of Architecture and Technology, Xi’an, China, 2021. [Google Scholar]
- Zhao, R. Influence of Different Ventilation Conditions on Air Flow and Temperature and Humidity in Plastic Greenhouse. Master’s Thesis, Northwest A&F University, Xianyang, China, 2021. [Google Scholar]
- Nebbali, R.; Roy, J.C.; Boulard, T. Dynamic simulation of the distributed radiative and convective climate within a cropped greenhouse. Renew. Energy 2012, 43, 111–129. [Google Scholar] [CrossRef]
- Chu, C.R.; Chiu, Y.H.; Chen, Y.J.; Wang, Y.W.; Chou, C.P. Turbulence effects on the discharge coefficient and mean flow rate of wind-driven cross-ventilation. Build. Environ. 2009, 44, 2064–2072. [Google Scholar] [CrossRef]
- CIBSE. Natural Ventilation in Non-Domestic Buildings: Applications Manual AM10; Chartered Institution of Building Services Engineers (CIBSE): London, UK, 1997. [Google Scholar]
- Mumovic, D.; Santamouris, M. A Handbook of Sustainable Building Design and Engineering: An Integrated Approach to Energy, Health and Operational Performance; Routledge: London, UK, 2013. [Google Scholar]
- Chu, C.R.; Chiang, B.F. Wind-driven cross ventilation in long buildings. Build. Environ. 2014, 80, 150–158. [Google Scholar] [CrossRef]
- Bartzanas, T.; Katsoulas, N.; Kittas, C. Effect of vent configuration and insect screen on greenhouse microclimate. Int. J. Vent. 2005, 4, 193–202. [Google Scholar] [CrossRef]
- He, K.; Chen, D.; Sun, L.; Liu, Z. Effects of Wind Regime and Vent Configuration on Microclimate in Tunnel Greenhouses in Summer. Trans. Chin. Soc. Agric. Mach. 2017, 48, 311–318+339. [Google Scholar]
- Zhao, P. Simulation and Experimental Research on Indoor Environment of Solar Greenhouse under Natural Ventilation. Master’s Thesis, Shandong Jianzhu University, Jinan, China, 2021. [Google Scholar]
- Kittas, C.; Katsoulas, N.; Bartzanas, T.; Mermier, M.; Boulard, T. The impact of insect screens and ventilation openings on the greenhouse microclimate. Trans. ASABE 2008, 51, 2151–2165. [Google Scholar] [CrossRef]
- Majdoubi, H.; Fatnassi, H.; Boulard, T.; Senhaji, A.; Demrati, H.; Mouqallid, M.; Bouirden, L. Computational study of thermal performance of an unheated canarian-Type greenhouse: Influence of the opening configurations on airflow and climate patterns at the crop level. Acta Hortic. 2017, 1182, 87–94. [Google Scholar] [CrossRef]
- Luan, T. Numerieal Simulation and PIV Test Research on Natural Ventilation of Coupling Thermal Pressure and Wind Pressure. Master’s Thesis, Chang’an University, Xi’an, China, 2010. [Google Scholar]
- Shen, X.; Su, R.; Ntinas, G.K.; Zhang, G. Influence of sidewall openings on air change rate and airflow conditions inside and outside low-rise naturally ventilated buildings. Energy Build. 2016, 130, 453–464. [Google Scholar] [CrossRef]
- Fu, W.; You, S. The influence of intake size of strawberry solar greenhouse on the microclimate in natural ventilation: An experimental study. J. Hebei Univ. Technol. 2013, 42, 52–56. [Google Scholar]
- Bai, Z.; Xia, L.; Lv, X.; Zhang, M.; Tao, J. Simulations of greenhouse window settings with natural ventilation Based on CFD. Jiangsu Agric. Sci. 2016, 44, 379–382. [Google Scholar]
- Espinoza, K.; Lopez, A.; Valera, D.L.; Molina-Aiz, F.D.; Torres, J.A.; Pena, A. Effects of ventilator configuration on the flow pattern of a naturally-ventilated three-span Mediterranean greenhouse. Biosyst. Eng. 2017, 164, 13–30. [Google Scholar] [CrossRef]
- Miao, Z. Numerical Simulation and Optimization of Vent Configuration for Natural Ventilation of The Large Space Exhibition Greenhouse. Refrig. Air Cond. 2020, 34, 29–38. [Google Scholar]
- Yan, L. Effects of Different Natural Ventilation Methods on Solar Greenhouse Environment and Tomato Growth. Master’s Thesis, Northwest A&F University, Xianyang, China, 2020. [Google Scholar]
- Yang, Z.; Zou, Z.; Chen, S.; Wang, J. Distribution law of inside wind speed and relationship between wind speed inside and outside and ventilation area ratio in northwest type sunlight greenhouse. J. Northwest AF Univ. 2006, 34, 36–40. [Google Scholar]
- Yang, Z. Optimal Wind Speed and CFD Simulation in Sunlight Greenhouse. Ph.D. Thesis, Northwest A&F University, Xianyang, China, 2006. [Google Scholar]
- Miguel, A.F.; Van de Braak, N.J.; Bot, G.P.A. Analysis of the airflow characteristics of greenhouse screening materials. J. Agric. Eng. Res. 1997, 67, 105–112. [Google Scholar] [CrossRef]
- Teitel, M. The effect of insect-proof screens in roof openings on greenhouse microclimate. Agric. For. Meteorol. 2001, 110, 13–25. [Google Scholar] [CrossRef]
- Bailey, B.J.; Montero, J.I.; Parra, J.P.; Robertson, A.P.; Baeza, E.; Kamaruddin, R. Airflow resistance of greenhouse ventilators with and without insect screens. Biosyst. Eng. 2003, 86, 217–229. [Google Scholar] [CrossRef]
- Laws, E.M.; Livesey, J.L. Flow through screens. Annu. Rev. Fluid Mech. 1978, 10, 247–266. [Google Scholar] [CrossRef]
- Santolini, E.; Pulvirenti, B.; Benni, S.; Barbaresi, L.; Torreggiani, D.; Tassinari, P. Numerical study of wind-driven natural ventilation in a greenhouse with screens. Comput. Electron. Agric. 2018, 149, 41–53. [Google Scholar] [CrossRef]
- Wang, S.; Boulard, T.; Haxaire, R. Air speed profiles in a naturally ventilated greenhouse with a tomato crop. Agric. For. Meteorol. 1999, 96, 181–188. [Google Scholar] [CrossRef]
- Roy, J.C.; Boulard, T.; Kittas, C.; Wang, S. Convective and ventilation transfers in greenhouses, Part 1: The greenhouse considered as a perfectly stirred tank. Biosyst. Eng. 2002, 83, 1–20. [Google Scholar] [CrossRef]
- Boulard, T.; Wang, S. Experimental and numerical studies on the heterogeneity of crop transpiration in a plastic tunnel. Comput. Electron. Agric. 2002, 34, 173–190. [Google Scholar] [CrossRef]
- Majdoubi, H.; Boulard, T.; Fatnassi, H.; Bouirden, L. Airflow and microclimate patterns in a one-hectare Canary type greenhouse: An experimental and CFD assisted study. Agric. For. Meteorol. 2009, 149, 1050–1062. [Google Scholar] [CrossRef]
- Fatnassi, H.; Boulard, T.; Roy, J.C.; Suay, R.; Poncet, C. CFD coupled modeling of distributed plant activity and climate in greenhouse. Acta Hortic. 2017, 57–64. [Google Scholar] [CrossRef]
- Thom, A.S. Momentum absorption by vegetation. Q. J. R. Meteorol. Soc. 1971, 97, 414–428. [Google Scholar] [CrossRef]
- Haxaire, R. Caractérisation et Modélisation des écoulements d’air Dans une Serre. [Characterisation and Modelling of Air Flow in a Greenhouse]. Ph.D. Thesis, l’Université de Nice Sophia Antipolis, Nice, France, 1999. [Google Scholar]
- Green, S.R. Modeling turbulent air flow in a stand of widely spaced trees. PHOENICS J. Comput. Fluid Dyn. Its Appl. 1992, 5, 294–312. [Google Scholar]
- Bruse, M. Development of a Micro-Scale Model for the Calculation of Surface Temperature in Structured Terrain. Ph.D. Thesis, University of Bochum, Institute for Geography, Bochum, Germany, 1995. [Google Scholar]
- Sase, S.; Kacira, M.; Boulard, T.; Okushima, L. Wind tunnel measurement of aerodynamic properties of a tomato canopy. Trans. ASABE 2012, 55, 1921–1927. [Google Scholar] [CrossRef]
- Boulard, T.; Roy, J.C.; Pouillard, J.B.; Fatnassi, H.; Grisey, A. Modelling of micrometeorology, canopy transpiration and photosynthesis in a closed greenhouse using computational fluid dynamics. Biosyst. Eng. 2017, 158, 110–133. [Google Scholar] [CrossRef]
- Zheng, M.; Mao, H. CFD Analysis about Effect of Plant Height on Natural Ventilation in Greenhouse. J. Agric. Mech. Res. 2016, 38, 20–23+27. [Google Scholar]
- Chu, C.R.; Wang, Y.W. The loss factors of building openings for wind-driven ventilation. Build. Environ. 2010, 45, 2273–2279. [Google Scholar] [CrossRef]
- Chu, C.R.; Chiang, B.F. Wind-driven cross ventilation with internal obstacles. Energy Build. 2013, 67, 201–209. [Google Scholar] [CrossRef]
- Liang, Z. Study on the Effect of Applying the Cultivation Pattern of Overwintering Tomatoes from the East-West Ridge of Solar Greenhouse. Master’s Thesis, Shenyang Agricultrual University, Shenyang, China, 2018. [Google Scholar]
- Yang, Y. Tomato Yield Formation and Influencing Factors under East-West Cultivation Pattern in Slide Cover Type Solar Greenhouse. Master’s Thesis, Shenyang Agricultrual University, Shenyang, China, 2017. [Google Scholar]
- Zhiguo, L.; Zishuang, Y.; Liguo, Y.; Chuanyou, L.; Zongxu, L.; Xiaoming, L. Experimental study on tomato cultivation mode in solar greenhouse based on integration of agricultural machinery and agronomy. J. Chin. Agric. Mech. 2021, 42, 55–59. [Google Scholar]
- Song, W.; Li, C.; Sun, X.; Wang, P.; Zhao, S. Effects of ridge direction on growth and yield of tomato in solar greenhouse with diffuse film. Trans. Chin. Soc. Agric. Eng. 2017, 33, 242–248. [Google Scholar]
- Majdoubi, H.; Boulard, T.; Hanafi, A.; Bekkaoui, A.; Fatnassi, H.; Demrati, H.; Nya, M.B.; Bouirden, L. Natural Ventilation Performance of a Large Greenhouse Equipped with Insect Screens. Trans. ASABE 2007, 50, 641–650. [Google Scholar] [CrossRef]
- Perén, J.I.; Van Hooff, T.; Leite, B.C.C.; Blocken, B. CFD analysis of cross-ventilation of a generic isolated building with asymmetric opening positions: Impact of roof angle and opening location. Build. Environ. 2015, 85, 263–276. [Google Scholar] [CrossRef]
- Baeza, E.J. Optimización del Diseño de los Sistemas de Ventilación en Invernaderos Tipo Parral. Ph.D. Thesis, Universidad de Almería, Almería, Spain, 2007. [Google Scholar]
- Peren, J.I.; Van Hooff, T.; Ramponi, R.; Blocken, B.; Leite, B.C.C. Impact of roof geometry of an isolated leeward sawtooth roof building on cross-ventilation: Straight, concave, hybrid or convex? J. Wind. Eng. Ind. Aerodyn. 2015, 145, 102–114. [Google Scholar] [CrossRef]
- Perén, J.I.; van Hooff, T.; Leite, B.C.C.; Blocken, B. CFD simulation of wind-driven upward cross ventilation and its enhancement in long buildings: Impact of single-span versus double-span leeward sawtooth roof and opening ratio. Build. Environ. 2016, 96, 142–156. [Google Scholar] [CrossRef]
- Fatnassi, H.; Boulard, T.; Benamara, H.; Roy, J.C.; Suay, R.; Poncet, C. Increasing the height and multiplying the number of spans of greenhouse: How far can we go. Acta Hortic. 2017, 1170, 137–144. [Google Scholar] [CrossRef]
- Roy, J.C.; Boulard, T. CFD prediction of the natural ventilation in a tunnel-type greenhouse: Influence of wind direction and sensibility to turbulence models. Acta Hortic. 2004, 691, 457–464. [Google Scholar] [CrossRef]
- Pontikakos, C.; Ferentinos, K.P.; Tsiligiridis, T.A.; Sideridis, A.B. Natural ventilation efficiency in a twin-span greenhouse using 3D computational fluid dynamics. In Proceedings of the 3rd International Conference on Information and Communication Technologies in Agriculture, Volos, Greece, 20–23 September 2006. [Google Scholar]
- He, K.; Chen, D.; Liu, Z. Effects of vent configuration and wind regime on the microclimate in the tunnel greenhouse. In Proceedings of the 2017 6th International Conference on Agro-Geoinformatics, Fairfax, VA, USA, 7–10 August 2017; pp. 1–6. [Google Scholar]
- Chu, C.R.; Chiu, Y.H.; Tsai, Y.T.; Wu, S.L. Wind-driven natural ventilation for buildings with two openings on the same external wall. Energy Build. 2015, 108, 365–372. [Google Scholar] [CrossRef]
- Boulard, T.; Kittas, C.; Roy, J.C.; Wang, S. Convective and ventilation transfers in greenhouses, part 2: Determination of the distributed greenhouse climate. Biosyst. Eng. 2002, 83, 129–147. [Google Scholar] [CrossRef]
- Bailey, B.J. Constraints, limitations and achievements in greenhouse natural ventilation. Acta Hortic. 2000, 534, 21–30. [Google Scholar] [CrossRef]
- Tao, W. Heat Transfer; Higher Education Press: Beijing, China, 2019. [Google Scholar]
- Hou, C.; Ma, C. Study Applying Fluent in Greenhouse Ventilation. J. Agric. Mech. Res. 2007, 29, 5–9. [Google Scholar]
- Mezrhab, A.; Elfarh, L.; Naji, H.; Lemonnier, D. Computation of surface radiation and natural convection in a heated horticultural greenhouse. Appl. Energy 2010, 87, 894–900. [Google Scholar] [CrossRef]
- Foster, M.P.; Down, M.J. Ventilation of livestock buildings by natural convection. J. Agric. Eng. Res. 1987, 37, 1–13. [Google Scholar] [CrossRef]
- Handbook, A. Fundamentals, 1981; American Society of Heating, Refrigerating and Air-conditioning Engineers: Atlanta, GA, USA, 1981. [Google Scholar]
- IHVE. Guide; The Institution of Heating and Ventilating Engineers: London, UK, 1970; p. 64. [Google Scholar]
- Daly, B.B. Woods Practical Guide to Fan Engineering; Woods of Colchester Limited: Colchester, UK, 1978. [Google Scholar]
- Hellickson, M.A.; Walker, J.N. Ventilation of Agricultural Structures; American Society of Agricultural Engineers: St. Joseph, MI, USA, 1983. [Google Scholar]
- Sainsbury, D.; Sainsbury, P. Livestock Health and Housing; Baillière Tindall: London, UK, 1988. [Google Scholar]
- Liu, H.; Xu, Z. Determination of Hot Pressing Neutral Plane Position of High Building. China Saf. Sci. J. 2001, 11, 17–19. [Google Scholar]
- Kirkpatrick, A.T.; Hill, D.D. Mixed convection heat transfer in a passive solar building. Sol. Energy 1988, 40, 25–34. [Google Scholar] [CrossRef]
- Wise, N.H.; Hunt, G.R. Buoyancy-driven unbalanced exchange flow through a horizontal opening. J. Fluid Mech. 2020, 888, A22. [Google Scholar] [CrossRef]
- Wang, X.; Luo, J.; Li, X. CFD based study of heterogeneous microclimate in a typical chinese greenhouse in central China. J. Integr. Agric. 2013, 12, 914–923. [Google Scholar] [CrossRef]
- Zhao, Y.; Teitel, M.; Barak, M. SE—Structures and Environment: Vertical temperature and humidity gradients in a naturally ventilated greenhouse. J. Agric. Eng. Res. 2001, 78, 431–436. [Google Scholar] [CrossRef]
- Breuer, M.; Wessling, S.; Schmalzl, J.; Hansen, U. Effect of inertia in Rayleigh-Bénard convection. Phys. Rev. E 2004, 69, 026302. [Google Scholar] [CrossRef]
- Li, Y.; Sandberg, M.; Fuchs, L. Vertical temperature profiles in rooms ventilated by displacement: Full-scale measurement and nodal modelling. Indoor Air 1992, 2, 225–243. [Google Scholar] [CrossRef]
- Li, Y.; Delsante, A.E.; Symons, J.G.; Eng, B. Simulation tools for analysing natural ventilation of large enclosures with large openings. AIRAH J. 1997, 51, 21–28. [Google Scholar]
- Cooper, P.; Linden, P.F. Natural ventilation of an enclosure containing two buoyancy sources. J. Fluid Mech. 1996, 311, 153–176. [Google Scholar] [CrossRef]
- Linden, P.F.; Cooper, P. Multiple sources of buoyancy in a naturally ventilated enclosure. J. Fluid Mech. 1996, 311, 177–192. [Google Scholar] [CrossRef]
- Boulard, T.; Haxaire, R.; Lamrani, M.A.; Roy, J.C.; Jaffrin, A. Characterization and modelling of the air fluxes induced by natural ventilation in a greenhouse. J. Agric. Eng. Res. 1999, 74, 135–144. [Google Scholar] [CrossRef]
- Sherman, M.H. Superposition in infiltration modeling. Indoor Air 1992, 2, 101–114. [Google Scholar] [CrossRef]
- Walker, I.S.; Wilson, D.J. Evaluating models for superposition of wind and stack effect in air infiltration. Build. Environ. 1993, 28, 201–210. [Google Scholar] [CrossRef]
- Sherman, M.H.; Grimsrud, D.T. Infiltration-pressurization correlation: Simplified physical modeling. Am. Soc. Heat. Refrig. Air-Cond. Eng. 1980, 86, 778–807. [Google Scholar]
- Okada, M.; Takakura, T. Guide and Data for Greenhouse Air Conditioning 3. Heat loss due to air infiltration of heated greenhouse. J. Agric. Meteorol. 1973, 28, 223–230. [Google Scholar] [CrossRef]
- Li, Y.; Delsante, A. On natural ventilation of a building with two openings. In Proceedings of the Document-Air Infiltration Centre AIC Proc, Oslo, Norway, 28–30 September 1998; pp. 188–196. [Google Scholar]
- Li, Y.; Delsante, A. Natural ventilation induced by combined wind and thermal forces. Build. Environ. 2001, 36, 59–71. [Google Scholar] [CrossRef]
- Li, Y.; Delsante, A.; Chen, Z.; Sandberg, M.; Andersen, A.; Bjerre, M.; Heiselberg, P. Some examples of solution multiplicity in natural ventilation. Build. Environ. 2001, 36, 851–858. [Google Scholar] [CrossRef]
- Heiselberg, P.; Li, Y.; Andersen, A.; Bjerre, M.; Chen, Z. Experimental and CFD evidence of multiple solutions in a naturally ventilated building. Indoor Air 2004, 14, 43–54. [Google Scholar] [CrossRef]
- Andersen, A.; Bjerre, M.; Chen, Z.D.; Heiselberg, P.; Li, Y. Experimental Study of Wind-Opposed Buoyancy-Driven Natural Ventilation; Department of Building Technology and Structural Engineering, Aalborg University: Aalborg, Copenhagen, 2000. [Google Scholar]
- Hunt, G.R.; Linden, P.F. Displacement and mixing ventilation driven by opposing wind and buoyancy. J. Fluid Mech. 2005, 527, 27–55. [Google Scholar] [CrossRef]
- Whittle, R.M.; Lawrence, W.J.C. The climatology of glasshouses. II. Ventilation. J. Agric. Eng. Res. 1960, 5, 36–41. [Google Scholar]
- Nederhoff, E.M.; Van de Vooren, J.; Ten Cate, A.J.U. A practical tracer gas method to determine ventilation in greenhouses. J. Agric. Eng. Res. 1985, 31, 309–319. [Google Scholar] [CrossRef]
- Florentzou, F. Experiments in natural ventilation for passive cooling. In Proceedings of the 17th AIVC Conference, Gothenburg, Sweden, 17–20 September 1996; Volume1, pp. 121–134. [Google Scholar]
- Flourentzou, F.; Van der Maas, J.; Roulet, C.A. Natural ventilation for passive cooling: Measurement of discharge coefficients. Energy Build. 1998, 27, 283–292. [Google Scholar] [CrossRef]
- Liddament, M.W. Air Infiltration Calculation Techniques: An Applications Guide; Air Infiltration and Ventilation Centre: Berkshire, UK, 1986. [Google Scholar]
- Chalabi, Z.S.; Bailey, B.J. Simulation of the Energy Balance in a Greenhouse; Divisional Note; AFRC Institute of Engineering Research: Glasgow, UK, 1989. [Google Scholar]
- Wang, S. Measurement and Modelling of Natural Ventilation in a Large Venlo-Type Greenhouse. Ph.D. Thesis, Faculté Universitaire des Sciences Agronomiques de Gembloux, Gembloux, Belgium, 1998. [Google Scholar]
- Ducarme, D.; Vandaele, L.; Wouters, P. Single sided ventilation: A comparison of the measured air change rates with tracer gas and with the heat balance approach. In Proceedings of the 15. AIVC Conference on the Role of Ventilation, Buxton, UK, 27–30 September 1994; pp. 26–35. [Google Scholar]
- Demrati, H.; Boulard, T.; Bekkaoui, A.; Bouirden, L. SE—Structures and Environment: Natural ventilation and microclimatic performance of a large-scale banana greenhouse. J. Agric. Eng. Res. 2001, 80, 261–271. [Google Scholar] [CrossRef]
- Kittas, C.; Giaglaras, P.; Papadakis, G. Heat and mass transfer processes in the greenhouse ecosystem. In Proceedings of the AgEng Oslo 98, International Conference on Agricultural Engineering, Oslo, Norway, 24–27 August 1998; Norges Landbrukshoegskole: Oslo, Norway, 1998. [Google Scholar]
- Baille, M.; Laury, J.C.; Baille, A.; Sappe, G. Influence du matériau de couverture sur les échanges énergétiques d’une serre: Étude comparative verre normal-verre à faible émissivité. I. Influence sur les déperditions thermiques. Agronomie 1983, 3, 197–202. [Google Scholar] [CrossRef]
- Choab, N.; Allouhi, A.; El Maakoul, A.; Kousksou, T.; Saadeddine, S.; Jamil, A. Review on greenhouse microclimate and application: Design parameters, thermal modeling and simulation, climate controlling technologies. Sol. Energy 2019, 191, 109–137. [Google Scholar] [CrossRef]
- Piscia, D.; Montero, J.I.; Bailey, B.; Muñoz, P.; Oliva, A. A new optimisation methodology used to study the effect of cover properties on night-time greenhouse climate. Biosyst. Eng. 2013, 116, 130–143. [Google Scholar] [CrossRef]
- Zhang, T. Numerical Simulation and Experimental Research on Microclimate Environment in Greenhouse-Take Greenhouses of Shandong Shouguang as Examples. Master’s Thesis, Xi’an University of Architecture & Technology, Xi’an, China, 2006. [Google Scholar]
- Cheng, X.; Mao, H.; Wu, D.; Li, B. Numerical Simulation of Thermal Profiles in Spatial and Temporal Field for Natural Ventilated Glasshouse. Trans. Chin. Soc. Agric. Mach. 2009, 40, 179–183. [Google Scholar]
- Fatnassi, H.; Boulard, T.; Bouirden, L. Simulation of climatic conditions in full-scale greenhouse fitted with insect-proof screens. Agric. For. Meteorol. 2003, 118, 97–111. [Google Scholar] [CrossRef]
- Jones, P.J.; Whittle, G.E. Computational fluid dynamics for building air flow prediction—current status and capabilities. Build. Environ. 1992, 27, 321–338. [Google Scholar] [CrossRef]
- Roy, J.C.; Boulard, T. CFD predictions of natural ventilation and climate in a tunnel-type greenhouse using a transpiration active crop model. Acta Hortic. 2004, 205–212. [Google Scholar] [CrossRef]
- Lee, I.B.; Short, T.H. Two-dimensional numerical simulation of natural ventilation in a multi-span greenhouse. Trans. ASAE 2000, 43, 757. [Google Scholar]
- Piscia, D.; Muñoz, P.; Panadès, C.; Montero, J.I. A method of coupling CFD and energy balance simulations to study humidity control in unheated greenhouses. Comput. Electron. Agric. 2015, 115, 129–141. [Google Scholar] [CrossRef]
- Zhai, Z.; Chen, Q.; Haves, P.; Klems, J.H. On approaches to couple energy simulation and computational fluid dynamics programs. Build. Environ. 2002, 37, 857–864. [Google Scholar] [CrossRef]
- Zhai, Z.; Chen, Q.Y. Solution characters of iterative coupling between energy simulation and CFD programs. Energy Build. 2003, 35, 493–505. [Google Scholar] [CrossRef]
- Zhai, Z.J.; Chen, Q.Y. Performance of coupled building energy and CFD simulations. Energy Build. 2005, 37, 333–344. [Google Scholar] [CrossRef]
- Zhang, R.; Lam, K.P.; Yao, S.-C.; Zhang, Y. Coupled EnergyPlus and computational fluid dynamics simulation for natural ventilation. Build. Environ. 2013, 68, 100–113. [Google Scholar] [CrossRef]
- Baines, W.D.; Turner, J.S. Turbulent buoyant convection from a source in a confined region. J. Fluid Mech. 1969, 37, 51–80. [Google Scholar] [CrossRef]
- Worster, M.G.; Huppert, H.E. Time-dependent density profiles in a filling box. J. Fluid Mech. 1983, 132, 457–466. [Google Scholar] [CrossRef]
- Hunt, G.R.; Linden, P.F. Natural ventilation by the combined effects of buoyancy and wind. In Proceedings of the ROOMVENT 96 the 5th Annual Conference on Air Distribution in Rooms, Yokohama, Japan, 17–19 July 1996; pp. 239–246. [Google Scholar]
- Li, Y. Buoyancy-driven natural ventilation in a thermally stratified one-zone building. Build. Environ. 2000, 35, 207–214. [Google Scholar] [CrossRef]
- Andersen, K.T. Theoretical considerations on natural ventilation by thermal buoyancy. ASHRAE Trans. 1995, 101, 1–15. [Google Scholar]
- Meneses, J.F.; Raposo, J.R. Ventilação natural de instalações agrícolas: Teoria e métodos de cálculo (Natural ventilation of animal housings: Theory and methods of calculation). An. Inst. Super. Agron. 1987, 42, 249. [Google Scholar]
- Kurabuchi, T.; Ohba, M.; Endo, T.; Akamine, Y.; Nakayama, F. Local dynamic similarity model of cross-ventilation part 1-theoretical framework. Int. J. Vent. 2004, 2, 371–382. [Google Scholar] [CrossRef]
- Kurabuchi, T.; Ohba, M.; Goto, T.; Akamine, Y.; Endo, T.; Kamata, M. Local dynamic similarity concept as applied to evaluation of discharge coefficients of cross-ventilated buildings—part 1 basic idea and underlying wind tunnel tests; part 2 applicability of local dynamic similarity concept; part 3 simplified method for estimating dynamic pressure tangential to openings of cross-ventilated buildings. Int. J. Vent. 2005, 4, 285–300. [Google Scholar]
- Ohba, M.; Kurabuchi, T.; Tomoyuki, E.; Akamine, Y.; Kamata, M.; Kurahashi, A. Local dynamic similarity model of cross-ventilation Part 2-Application of local dynamic similarity model. Int. J. Vent. 2004, 2, 383–394. [Google Scholar] [CrossRef]
- Boulard, T.; Feuilloley, P.; Kittas, C. Natural ventilation performance of six greenhouse and tunnel types. J. Agric. Eng. Res. 1997, 67, 249–266. [Google Scholar] [CrossRef]
- Critten, D.L.; Bailey, B.J. A review of greenhouse engineering developments during the 1990s. Agric. For. Meteorol. 2002, 112, 1–22. [Google Scholar] [CrossRef]
- Levine, B.D.; Stray Gundersen, J.; Mehta, R.D. Effect of altitude on football performance. Scand. J. Med. Sci. Sports 2008, 18, 76–84. [Google Scholar] [CrossRef] [PubMed]
- Buck, A.L. New equations for computing vapor pressure and enhancement factor. J. Appl. Meteorol. Climatol. 1981, 20, 1527–1532. [Google Scholar] [CrossRef]
- Liu, W.; Wang, J.; Li, Y.; Zhu, Z.; Qie, D.; Ding, L. Natural convection heat transfer at reduced pressures. Exp. Heat Transf. 2019, 32, 14–24. [Google Scholar] [CrossRef]
- Marty, C. Surface Radiation, Cloud Forcing and Greenhouse Effect in the Alps; ETH Zurich: Zurich, Switzerland, 2000. [Google Scholar]
- Saidi, M.; Abardeh, R.H. Air pressure dependence of natural-convection heat transfer. In Proceedings of the World Congress on Engineering, London, UK, 30 June–2 July 2010; pp. 1–5. [Google Scholar]
- Pandey, D. Solar Greenhouse as an Energy Alternative Solution for Growing Vegetable in High Altitude Region: A Case of Baragaon, Mustang. J. Inst. Eng. 2019, 15, 234–244. [Google Scholar] [CrossRef]
- Candy, S.; Moore, G.; Freere, P. Design and modeling of a greenhouse for a remote region in Nepal. Procedia Eng. 2012, 49, 152–160. [Google Scholar] [CrossRef][Green Version]
- Fuller, R.J.; Aye, L.; Zahnd, A.; Thakuri, S. Thermal evaluation of a greenhouse in a remote high altitude area of Nepal. Int. Energy J. 2009, 10, 71–80. [Google Scholar]
- Zeng, S.; Hu, H.; Xu, L.; Li, G. Nonlinear adaptive PID control for greenhouse environment based on RBF network. Sensors 2012, 12, 5328–5348. [Google Scholar] [CrossRef]
- Guo, Y.; Zhao, H.; Zhang, S.; Wang, Y.; Chow, D. Modeling and optimization of environment in agricultural greenhouses for improving cleaner and sustainable crop production. J. Clean. Prod. 2021, 285, 124843. [Google Scholar] [CrossRef]
- Iddio, E.; Wang, L.; Thomas, Y.; McMorrow, G.; Denzer, A. Energy efficient operation and modeling for greenhouses: A literature review. Renew. Sustain. Energy Rev. 2020, 117, 109480. [Google Scholar] [CrossRef]
- Katzin, D.; van Henten, E.J.; van Mourik, S. Process-based greenhouse climate models: Genealogy, current status, and future directions. Agric. Syst. 2022, 198, 103388. [Google Scholar] [CrossRef]
- Zhang, S.; Guo, Y.; Zhao, H.; Wang, Y.; Chow, D.; Fang, Y. Methodologies of control strategies for improving energy efficiency in agricultural greenhouses. J. Clean. Prod. 2020, 274, 122695. [Google Scholar] [CrossRef]
- Fatnassi, H.; Boulard, T.; Bouirden, L. Development, validation and use of a dynamic model for simulate the climate conditions in a large scale greenhouse equipped with insect-proof nets. Comput. Electron. Agric. 2013, 98, 54–61. [Google Scholar] [CrossRef]
- Wang, S.; Boulard, T. Predicting the microclimate in a naturally ventilated plastic house in a Mediterranean climate. J. Agric. Eng. Res. 2000, 75, 27–38. [Google Scholar] [CrossRef]
- De Halleux, D.; Deltour, J.; Nijskens, J.; Nisen, A.; Coutisse, S. Dynamic simulation of heat fluxes and temperatures in horticultural and low emissivity glass-covered greenhouses. Greenh. Constr. Cover. Mater. 1984, 170, 91–96. [Google Scholar] [CrossRef]
- Deltour, J.; De Halleux, D.; Nijskens, J.; Coutisse, S.; Nisen, A. Dynamic modelling of heat and mass transfer in greenhouses. In Proceedings of the Symposium Greenhouse Climate and its Control, Wageningen, The Netherlands, 19–24 May 1985; International Society for Horticultural Science (ISHS): Leuven, Belgium, 1985; pp. 119–126. [Google Scholar]
- Nijskens, J.; Deltour, J.; Halleux, D.D. Sensitivity Study of a Greenhouse Climate Dynamic Model; Bulletin des Recherches Agronomiques de Gembloux: Gembloux, Belguim, 1991. [Google Scholar]
- Pieters, J.G.; Deltour, J.M. Performances of greenhouses with the presence of condensation on cladding materials. J. Agric. Eng. Res. 1997, 68, 125–137. [Google Scholar] [CrossRef]
- Pieters, J.G.; Deltour, J.M.; Debruyckere, M.J. Condensation and dynamic heat transfer in greenhouses, Part I: Theoretical model. Int. Agric. Eng. J. 1996, 5, 119–133. [Google Scholar]
- Pieters, J.G.; Deltour, J.M.; Debruyckere, M.J. Condensation and dynamic heat transfer in greenhouses, Part II: Results for a standard glasshouse. Int. Agric. Eng. J. 1996, 5, 135–148. [Google Scholar]
- Zhu, S.; Deltour, J.; Wang, S. Modeling the thermal characteristics of greenhouse pond systems. Aquac. Eng. 1998, 18, 201–217. [Google Scholar] [CrossRef]
- Singh, M.C.; Singh, J.P.; Singh, K.G. Development of a microclimate model for prediction of temperatures inside a naturally ventilated greenhouse under cucumber crop in soilless media. Comput. Electron. Agric. 2018, 154, 227–238. [Google Scholar] [CrossRef]
- Mashonjowa, E.; Ronsse, F.; Milford, J.R.; Pieters, J.G. Modelling the thermal performance of a naturally ventilated greenhouse in Zimbabwe using a dynamic greenhouse climate model. Sol. Energy 2013, 91, 381–393. [Google Scholar] [CrossRef]
- Bartzanas, T.; Boulard, T.; Kittas, C. Effect of vent arrangement on windward ventilation of a tunnel greenhouse. Biosyst. Eng. 2004, 88, 479–490. [Google Scholar] [CrossRef]
- Park, J.; Rhee, G.H. Comparison of volume flow rate and volume-averaged local mean age of air for evaluating ventilation performance in natural ventilation. J. Mech. Sci. Technol. 2017, 31, 5801–5812. [Google Scholar] [CrossRef]
- Li, X.; Li, D.; Yang, X.; Yang, J. Total air age: An extension of the air age concept. Build. Environ. 2003, 38, 1263–1269. [Google Scholar] [CrossRef]
- Park, S.; Lee, I.-B.; Hong, S. New development of a straightforward method of estimating age-of-air using CFD. Acta Hortic. 2014, 1037, 963–969. [Google Scholar] [CrossRef]
- Zhou, J.; Hua, Y.; Xiao, Y.; Ye, C.; Yang, W. Analysis of Ventilation Efficiency and Effective Ventilation Flow Rate for Wind-driven Single-sided Ventilation Buildings. Aerosol Air Qual. Res. 2021, 21, 200383. [Google Scholar] [CrossRef]
- Sandberg, M. What is ventilation efficiency? Build. Environ. 1981, 16, 123–135. [Google Scholar] [CrossRef]





| References | Greenhouse | Continuous Roof Vents | Continuous Side Vents | Ventilation Type | Wind | Wind Effect Coefficient | Discharge Coefficient | Opening Effectiveness | Effective Area of Vents | Continuous Buoyancy Source | Combination Method |
|---|---|---|---|---|---|---|---|---|---|---|---|
| Bruce [23] | Cattle | Yes | Yes | Mixing and Displacement | No | - | 0.6 | - | - | Yes | Energy balance |
| Bot [19] | Multispan | No | - | Mixing | Yes | 0.6–1.6 | 0.64 + 0.001α | - | - | Yes | ϕ = √(ϕw2 + ϕb2) |
| Zhang, et al. [39] | Swine finishing | Yes | Yes | Displacement | Yes | - | 0.605 | - | - | Yes | Volume balance |
| Albright [36] | - | - | - | - | Yes | 0.35 | - | 0.5–0.6; 0.25–0.35 | - | - | - |
| De Jong [20] | Quasi infinite | No | Mixing and Displacement | Yes | 0.09 | 0.74 | - | - | Yes | ϕ = √(ϕw2 + ϕb2) | |
| Linden, et al. [3] | Enclosure | - | - | Displacement | No | - | - | - | Yes | Yes | - |
| Fernandez and Bailey [27] | Multispan | No | - | Mixing | Yes | 0.17 | - | - | - | Yes | Energy balance |
| Kittas, et al. [40] | Multispan | Yes | - | Mixing | Yes | 0.27 | - | - | - | Yes | ϕ = ϕw + ϕb |
| Boulard and Draoui [41] | 2-span | Yes | - | Mixing | Yes | 0.21 | - | - | Yes | Yes | ϕ(ζ) |
| Boulard and Baille [17] | 2-span | Yes | - | Mixing | 2–4; 0–2 | 0.07–0.1 | 0.43; 0.45; 0.64 | - | - | Yes | ϕ = ϕw + ϕb |
| Boulard, et al. [34] | 2-span | Yes | - | Mixing | 2–3 | 0.2; 0.26; 0.29 | - | - | - | - | ϕ = ϕw + ϕb |
| Papadakis, et al. [33] | 2-span | Yes | Yes | Mixing and Displacement | 0.1–7.6 | 0.246, 0.142 (global roof and side); 0.21 (global) | - | - | - | Yes | ϕ = √(ϕw2 + ϕb2) |
| Kittas, et al. [32] | Multispan | Yes | - | Mixing | Yes | 0.2 (global) | - | - | - | Yes | ϕ = ϕw + ϕb |
| Kittas, et al. [38] | 2-span | Yes | Yes | Displacement | 0–9.5 | 0.07 | 0.74 | - | Yes | - | ϕ = √(ϕw2 + ϕb2) |
| Miguel [42] | 2-span | No | - | Mixing | 1.27–5.5 | 1.13–1.52 | 0.61 | - | - | Yes | - |
| Nääs, et al. [37] | Poultry | - | Yes | Mixing | Yes | - | - | 0.25–0.6 | - | - | - |
| Muñoz, et al. [43] | 3-span | Yes | - | Mixing | 1–4 | 0.32–0.48; 0.061–0.089 (screened) | ξ = 1.75 + 0.7exp(−L/(32.5Hsinζ)) | - | - | - | - |
| Baptista, et al. [16] | Multispan | No | - | Mixing | 0.1–11 | 0.1; 0.09 | 0.64; 0.65 | 0.2 | - | Yes | ϕ = ϕw + ϕb; ϕ = √(ϕw2 + ϕb2) |
| Hunt and Linden [44] | Enclosure | Yes | Yes | Displacement | Yes | - | 0.6 | - | Yes | Transient | ϕ = √(ϕw2 + ϕb2) |
| Oca, et al. [45] | Tunnel | Yes | Yes | Displacement | No | - | 0.75 | - | - | Yes | Energy balance |
| Teitel and Tanny [46] | 4-span | Yes | Yes | Mixing | 0–4.2 | 0.11 (global) | 0.7 | - | - | Yes | Energy balance |
| Hunt and Linden [47] | Enclosure | Yes | Yes | Displacement | Yes | - | 0.6 | - | Yes | Yes | ϕ = √(ϕw2 + ϕb2) |
| Gladstone and Woods [48] | Enclosure | Holes | Holes | Displacement | No | - | 0.5 | - | Yes | Yes | Energy balance |
| Parra, et al. [49] | Multispan | Yes | Yes | Displacement | 0–11 | 0.0017 | 0.656 | - | Yes | Yes | ϕ = ϕw + ϕb |
| Si and Miao [50] | 3-span | Yes | Yes | Displacement | Yes | 0.8 (windward); −0.4, −0.5 (leeward) | 0.33 | - | - | Yes | ϕ = ϕw + ϕb |
| Liu, et al. [51] | 3-span | Yes (screened) | Yes (screened) | Displacement | 2 | 0.038 | 0.127 | - | Yes | Yes | Energy balance |
| Katsoulas, et al. [52] | Multispan | Yes (screened) | Yes (screened) | Displacement | 2.2 | 0.07 | 0.363 | - | Yes | Yes | ϕ = √(ϕw2 + ϕb2) |
| Teitel, et al. [53] | Multispan | Yes (screened) | Yes (screened) | Displacement | 4.9 | 0.075 | 0.253 | - | - | Yes | CFD simulation |
| Wang and Wang [54] | Multispan | Yes (screened) | - | Mixing | 1–5 | 0.178 (window); 0.318 (rolling-up) | - | - | - | Yes | - |
| Wang and Wang [54] | Multispan | Yes (screened) | - | Mixing | 0 | - | 0.667; 0.863 | - | - | Yes | - |
| Baeza, et al. [55] | 3-, 5-, 7-, 10-, 15-, 20-span | Yes (screened) | Yes (screened) | Mixing and Displacement | 0 | - | 0.65; 0.055 | - | - | Yes | - |
| Mashonjowa, et al. [56] | Multispan | Yes | Yes | Displacement | 0.35–3.4 | 0.029 | 0.414 | - | Yes | Yes | ϕ = √(ϕw2 + ϕb2) |
| Teitel and Wenger [57] | Single span | - | Yes | Cross | 1–7 | 0.6 (windward); −0.35 (leeward) | - | - | - | - | - |
| Fang, et al. [58] | Chinese solar | Yes | - | Mixing | Yes | 0.04; 0.05; 0.07 | 0.78; 0.60; 0.44 | - | - | Yes | ϕ = ϕw + ϕb |
| Chu, et al. [59] | Single; 2-span; 3-span | - | Yes | Cross | 10 | 0.00623 | 0.66 | - | Yes | - | - |
| Chu and Lan [60] | Single; 3-span | Yes | Yes | Displacement | 7.2 | 1.15–1.28 (difference) | 0.66 | - | Yes | - | - |
| Villagran, et al. [61] | Multispan | No | No | Displacement | 0.31–1.47 | - | - | - | - | - | CFD simulation |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, J.; Zhao, S.; Dai, A.; Wang, P.; Liu, Z.; Liang, B.; Ding, T. Greenhouse Natural Ventilation Models: How Do We Develop with Chinese Greenhouses? Agronomy 2022, 12, 1995. https://doi.org/10.3390/agronomy12091995
Zhang J, Zhao S, Dai A, Wang P, Liu Z, Liang B, Ding T. Greenhouse Natural Ventilation Models: How Do We Develop with Chinese Greenhouses? Agronomy. 2022; 12(9):1995. https://doi.org/10.3390/agronomy12091995
Chicago/Turabian StyleZhang, Jingfu, Shumei Zhao, Anguo Dai, Pingzhi Wang, Zhiwei Liu, Bohua Liang, and Tao Ding. 2022. "Greenhouse Natural Ventilation Models: How Do We Develop with Chinese Greenhouses?" Agronomy 12, no. 9: 1995. https://doi.org/10.3390/agronomy12091995
APA StyleZhang, J., Zhao, S., Dai, A., Wang, P., Liu, Z., Liang, B., & Ding, T. (2022). Greenhouse Natural Ventilation Models: How Do We Develop with Chinese Greenhouses? Agronomy, 12(9), 1995. https://doi.org/10.3390/agronomy12091995