A Qualitative Study of the Ionospheric Weak Response to Super Geomagnetic Storms
Abstract
:1. Introduction
2. Data and Methodology
2.1. Data
2.2. Algorithms: SWM and MMM
3. Results and Analysis
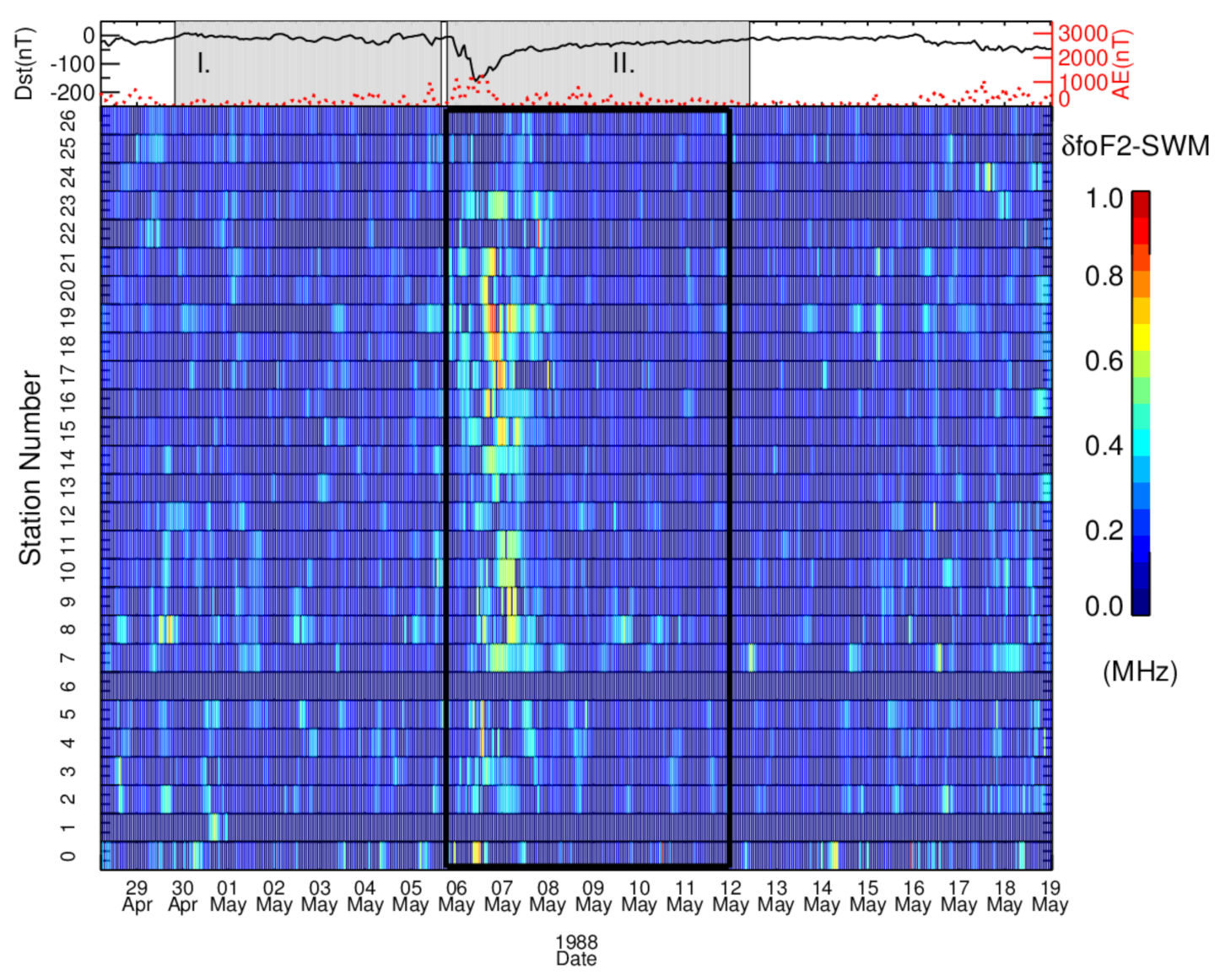
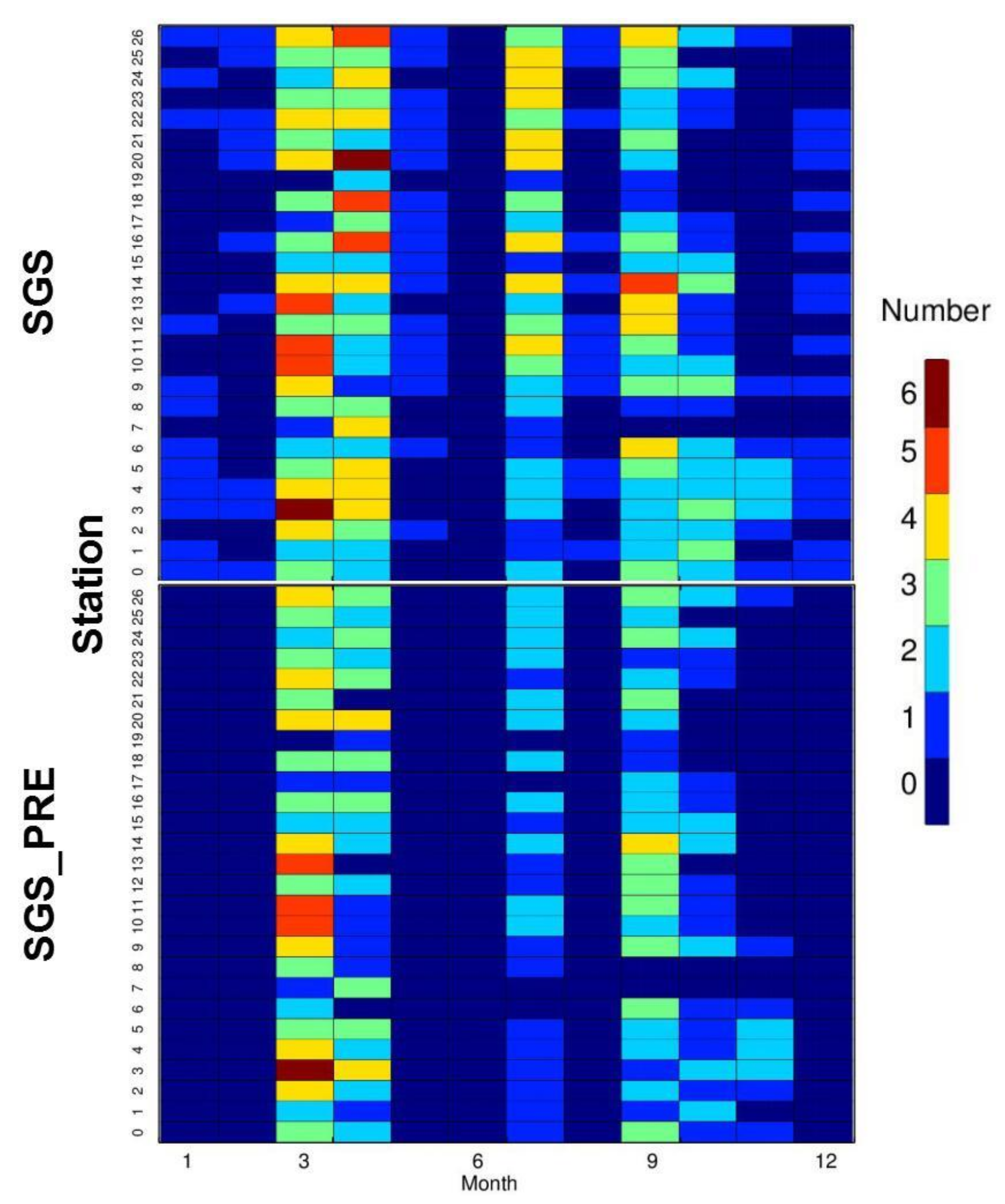
3.1. Single Weak Event
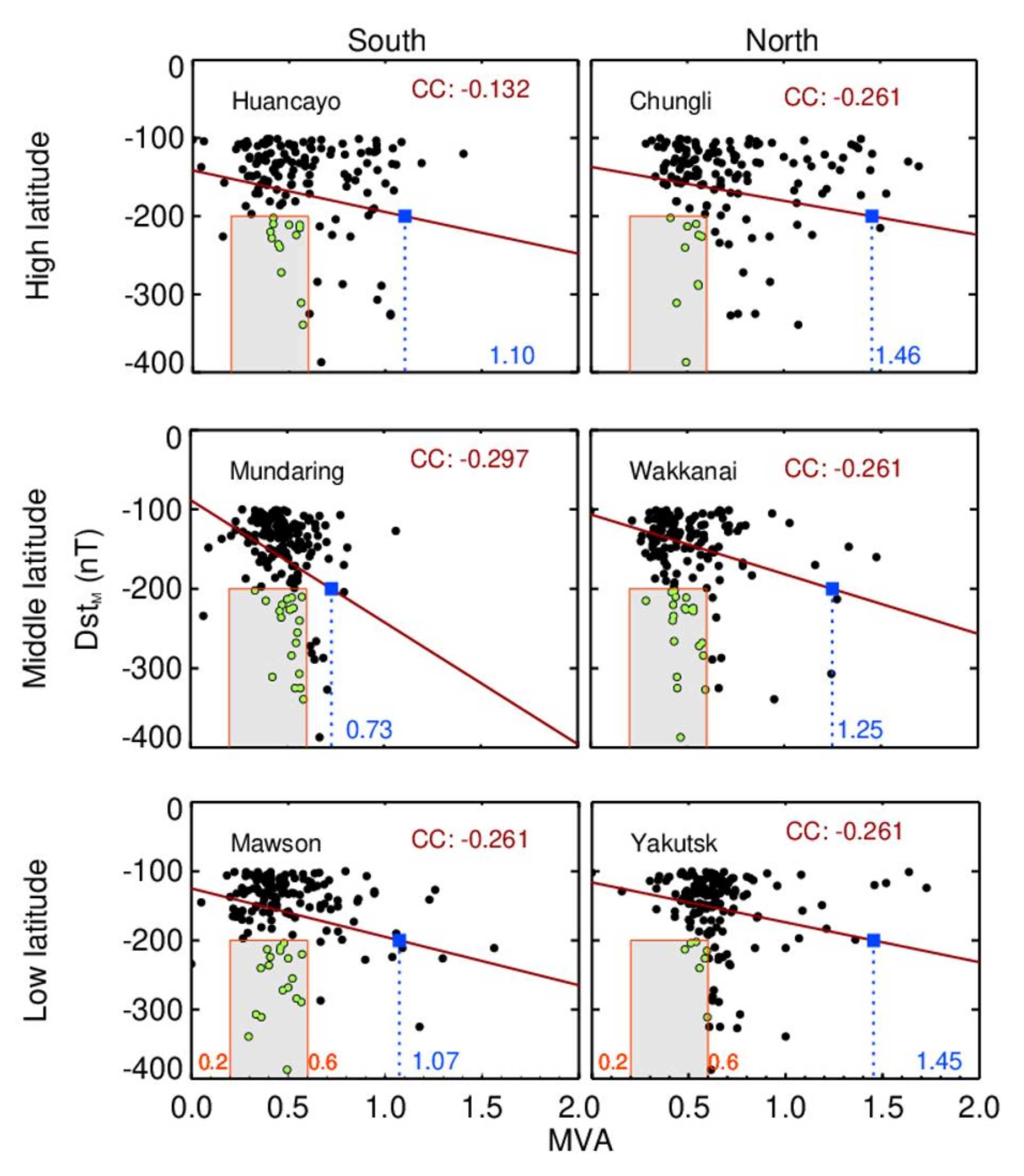
3.2. Regional Results and Analysis
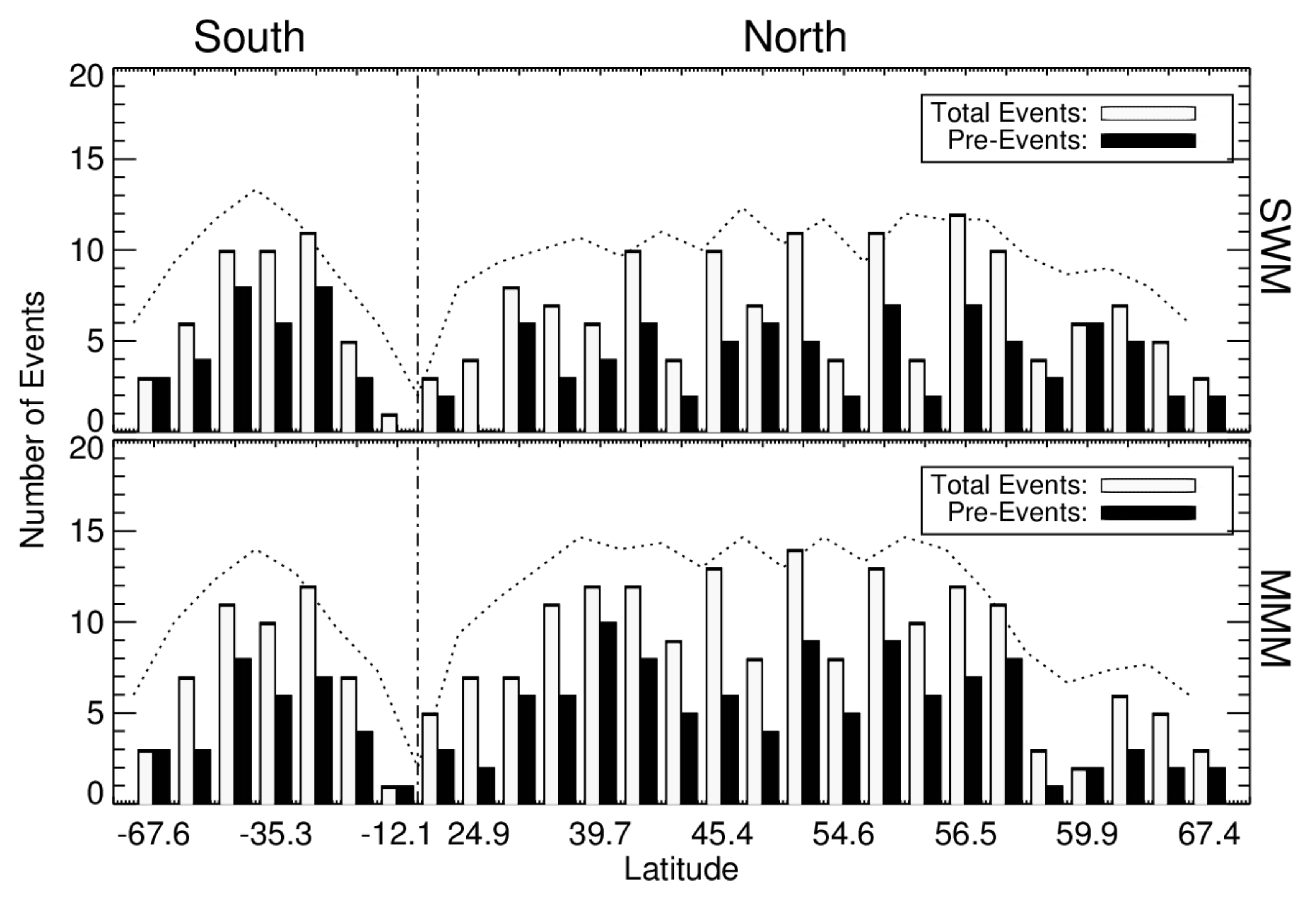
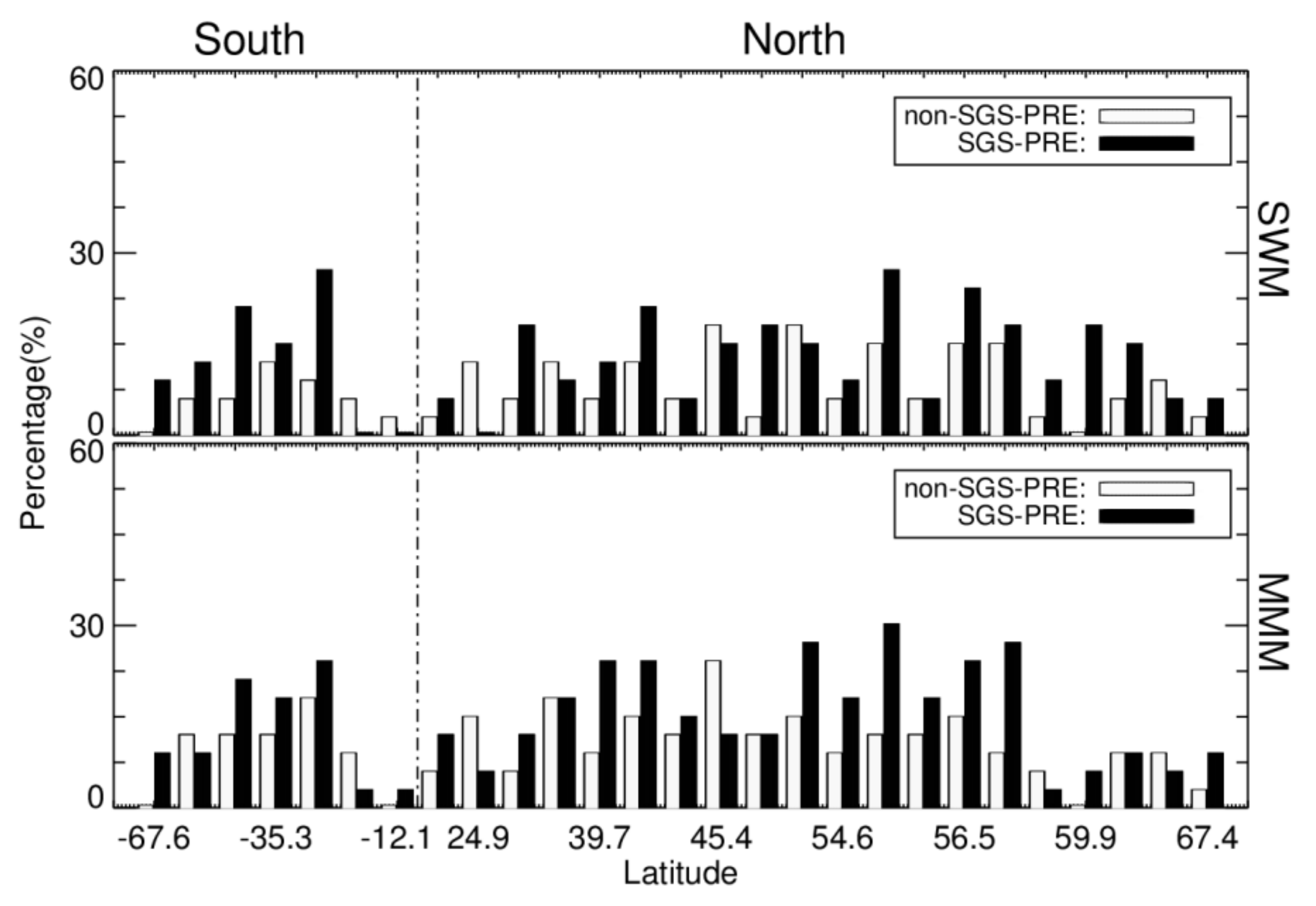
3.3. Global Results and Analysis
4. Conclusions and Summary
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Fuller-Rowell, T.J.; Codrescu, M.V.; Rishbeth, H.; Moffett, R.J.; Quegan, S. On the seasonal response of the thermosphere and ionosphere to geomagnetic storms. J. Geophys. Res. Space Phys. 1996, 101, 2343–2353. [Google Scholar] [CrossRef]
- Fuller-Rowell, T.M.; Codrescu, M.V.; Roble, R.G.; Richmond, A.D. How does the thermosphere and ionosphere react to a geomagnetic storm? Magn. Storms Geophys. Monogr. Ser. 1997, 98, 203–225. [Google Scholar]
- Prölss, G.W. Magnetic storm associated perturbations of the upper atmosphere. Magn. Storms 1997, 98, 227–241. [Google Scholar]
- Ridley, A.; Deng, Y.; Toth, G. The global ionosphere–thermosphere model. J. Atmos. Sol. Terr. Phys. 2006, 68, 839–864. [Google Scholar] [CrossRef]
- Wang, W.; Lei, J.; Burns, A.G.; Solomon, S.C.; Wiltberger, M.; Xu, J.; Zhang, Y.; Paxton, L.J.; Coster, A. Ionospheric response to the initial phase of geomagnetic storms: Common features. J. Geophys. Res. Space Phys. 2010, 115, A7. [Google Scholar] [CrossRef]
- Mendillo, M. Storms in the ionosphere: Patterns and processes for total electron content. Rev. Geophys. 2006, 44. [Google Scholar] [CrossRef]
- Wang, J.-S.; Chen, Z.; Huang, C.-M. A method to identify aperiodic disturbances in the ionosphere. Ann. Geophys. 2014, 32, 563–569. [Google Scholar] [CrossRef] [Green Version]
- Mednikova, N.B. Mid-Latitude Ionospheric Disturbances. In Physics of Solar Corpuscular Fluxes and Their Impact on the Upper Atmosphere of the Earth; Academic Press of USSR: Moscow, Russia, 1957; p. 183. (In Russian) [Google Scholar]
- Radicella, S.; Zhang, M.-L.; Romanelli, L.; Figliola, A. A critical analysis of monthly medians and variability of ionospheric parameters. Adv. Space Res. 1995, 15, 45–50. [Google Scholar] [CrossRef]
- Kouris, S.; Fotiadis, D. Ionospheric variability: A comparative statistical study. Adv. Space Res. 2002, 29, 977–985. [Google Scholar] [CrossRef]
- Mikhailov, A.V.; Depueva, A.K.; Leschinskaya, T.Y. Morphology of quiet time F2-layer disturbances: High to lower latitudes. Int. J. Geomagn. Aeron. 2004, 5, GI1006. [Google Scholar] [CrossRef]
- Jakowski, N.; Stankov, S.; Schlueter, S.; Klaehn, D. On developing a new ionospheric perturbation index for space weather operations. Adv. Space Res. 2006, 38, 2596–2600. [Google Scholar] [CrossRef]
- Gulyaeva, T.L.; Stanislawska, I. Derivation of a planetary ionospheric storm index. Ann. Geophys. 2008, 26, 2645–2648. [Google Scholar] [CrossRef] [Green Version]
- Depueva, A.K.; Mikhailov, A.V.; Depuev, V.K. Quiet timeF2–layer disturbances at geomagnetic equator. Int. J. Geomagn. Aeron. 2005, 5, GI3001. [Google Scholar] [CrossRef] [Green Version]
- Mikhailov, A.V.; Depueva, A.H.; Depuev, V.H. Daytime F2-layer negative storm effect: What is the difference between storm-induced and Q-disturbance events? Ann. Geophys. 2007, 25, 1531–1541. [Google Scholar] [CrossRef] [Green Version]
- Chen, Z.; Jin, M.; Deng, Y.; Wang, J.-S.; Huang, H.; Deng, X.; Huang, C.M. Improvement of a deep learning algorithm for total electron content maps: Image completion. J. Geophys. Res. Space Phys. 2019, 124, 790–800. [Google Scholar] [CrossRef] [Green Version]
- Tang, R.; Zeng, F.; Chen, Z.; Wang, J.-S.; Huang, C.-M.; Wu, Z. The comparison of predicting storm-time ionospheric TEC by three methods: ARIMA, LSTM, and Seq2Seq. Atmosphere 2020, 11, 316. [Google Scholar] [CrossRef] [Green Version]
- Chen, Z.; Wang, J.; Huang, C.M.; Huang, L. A new pair of indices to describe the relationship between ionospheric disturbances and geomagnetic activity. J. Geophys. Res. Space Phys. 2014, 119. [Google Scholar] [CrossRef]
- Chen, Z.; Wang, J.-S.; Deng, X.; Deng, Y.; Huang, C.M.; Li, H.M.; Wu, Z.X. Study on the relationship between the residual 27 day quasiperiodicity and ionospheric Q disturbances. J. Geophys. Res. Space Phys. 2017, 122, 2542–2550. [Google Scholar] [CrossRef]
- Chen, Z.; Wang, J.-S.; Deng, Y.; Huang, C.-M. Extraction of the geomagnetic activity effect from TEC data: A comparison between the spectral whitening method and 28 day running median. J. Geophys. Res. Space Phys. 2017, 122, 3632–3639. [Google Scholar] [CrossRef]
- Blanc, M.; Richmond, A. The ionospheric disturbance dynamo. J. Geophys. Res. Space Phys. 1980, 85, 1669–1686. [Google Scholar] [CrossRef]
- Foster, J.C.; Maurice, J.P.S.; Abreu, V.J. Joule heating at high latitudes. J. Geophys. Res. Space Phys. 1983, 88, 4885–4897. [Google Scholar] [CrossRef]
- Rishbeth, H.; Fuller-Rowell, T.J.; Rodger, A.S. F-layer storms and thermospheric composition. Phys. Scr. 1987, 36, 327–336. [Google Scholar] [CrossRef]
- Gonzalez, W.D.; Joselyn, J.A.; Kamide, Y.; Kroehl, H.W.; Rostoker, G.; Tsurutani, B.T.; Vasyliunas, V.M. What is a geomagnetic storm? J. Geophys. Res. Space Phys. 1994, 99, 5771–5792. [Google Scholar] [CrossRef]
- Millward, G.H.; Rishbeth, H.; Fuller-Rowell, T.J.; Aylward, A.D.; Quegan, S.; Moffett, R.J. Ionospheric F2 layer seasonal and semiannual variations. J. Geophys. Res. Space Phys. 1996, 101, 5149–5156. [Google Scholar] [CrossRef]
- Rishbeth, H.; Mendillo, M. Patterns of F2-layer variability. J. Atmos. Sol. Terr. Phys. 2001, 63, 1661–1680. [Google Scholar] [CrossRef]
- Richmond, A.; Peymirat, C.; Roble, R.G. Long-lasting disturbances in the equatorial ionospheric electric field simulated with a coupled magnetosphere-ionosphere-thermosphere model. J. Geophys. Res. Space Phys. 2003, 108. [Google Scholar] [CrossRef]
- Yue, X.; Wang, W.; Lei, J.; Burns, A.; Zhang, Y.; Wan, W.; Liu, L.; Hu, L.; Zhao, B.; Schreiner, W.S. Long-lasting negative ionospheric storm effects in low and middle latitudes during the recovery phase of the 17 March 2013 geomagnetic storm. J. Geophys. Res. Space Phys. 2016, 121, 9234–9249. [Google Scholar] [CrossRef] [Green Version]
- Lei, J.; Thayer, J.P.; Burns, A.G.; Lu, G.; Deng, Y. Wind and temperature effects on thermosphere mass density response to the November 2004 geomagnetic storm. J. Geophys. Res. Space Phys. 2010, 115. [Google Scholar] [CrossRef]
- Rishbeth, H. How the thermospheric circulation affects the ionospheric F2-layer. J. Atmos. Sol. Terr. Phys. 1998, 60, 1385–1402. [Google Scholar] [CrossRef]


| Number | Station | Time Coverage | Lat (° N) | Long (° E) |
|---|---|---|---|---|
| 0 | Mawson | 1958–2000 | −67.6 | 62.9 |
| 1 | Port Stanley | 1957–1990 | −51.7 | −57.8 |
| 2 | Hobart | 1950–2007 | −42.9 | 147.3 |
| 3 | Canberra | 1950–2007 | −35.3 | 149.0 |
| 4 | Mundaring | 1959–2007 | −32.0 | 116.2 |
| 5 | Townsville | 1951–2007 | −19.6 | 146.9 |
| 6 | Huancayo | 1957–1990 | −12.1 | −75.2 |
| 7 | Maui | 1957–1990 | +20.8 | −156.3 |
| 8 | Chungli | 1959–1990 | +24.9 | 121.4 |
| 9 | Yamagawa | 1957–1990 | +31.2 | 130.6 |
| 10 | Kokubunji | 1957–1990 | +35.7 | 139.5 |
| 11 | Akita | 1957–1990 | +39.7 | 140.1 |
| 12 | Boulder | 1958–1990 | +40.0 | −105.3 |
| 13 | Alma Ata | 1957–1990 | +43.2 | 76.9 |
| 14 | Wakkanai | 1957–1990 | +45.4 | 141.7 |
| 15 | Dourbes | 1957–1990 | +50.1 | 4.6 |
| 16 | Irkutsk | 1957–1992 | +52.5 | 104.0 |
| 17 | Juliusruh–Ruegen | 1957–2012 | +54.6 | 13.4 |
| 18 | Moscow | 1957–1995 | +55.5 | 37.3 |
| 19 | Gorky | 1958–1990 | +56.1 | 44.3 |
| 20 | Tomsk | 1957–1994 | +56.5 | 84.9 |
| 21 | Sverdlovsk | 1957–1995 | +56.4 | 58.6 |
| 22 | Churchill | 1957–1996 | +58.8 | −94.2 |
| 23 | Uppsala | 1957–1990 | +59.8 | 17.6 |
| 24 | Yakutsk | 1957–1991 | +62.0 | 129.6 |
| 25 | Lycksele | 1957–1990 | +64.7 | 18.8 |
| 26 | Sodankyla | 1957–1990 | +67.4 | 26.6 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, H.; Chen, Z.; Xie, L.; Li, F. A Qualitative Study of the Ionospheric Weak Response to Super Geomagnetic Storms. Atmosphere 2020, 11, 635. https://doi.org/10.3390/atmos11060635
Li H, Chen Z, Xie L, Li F. A Qualitative Study of the Ionospheric Weak Response to Super Geomagnetic Storms. Atmosphere. 2020; 11(6):635. https://doi.org/10.3390/atmos11060635
Chicago/Turabian StyleLi, Haimeng, Zhou Chen, Lianqi Xie, and Fan Li. 2020. "A Qualitative Study of the Ionospheric Weak Response to Super Geomagnetic Storms" Atmosphere 11, no. 6: 635. https://doi.org/10.3390/atmos11060635
APA StyleLi, H., Chen, Z., Xie, L., & Li, F. (2020). A Qualitative Study of the Ionospheric Weak Response to Super Geomagnetic Storms. Atmosphere, 11(6), 635. https://doi.org/10.3390/atmos11060635




