Numerical Modeling of Flow Over a Rectangular Broad-Crested Weir with a Sloped Upstream Face
Abstract
:1. Introduction
- 0 < H0/L ≤ 0.1 for long-crested weirs;
- 0.1 < H0/L ≤ 0.4 for broad-crested weirs;
- 0.4 < H0/L ≤ 1.5 for short-crested weirs; and
- 1.5 < H0/L for sharp-crested weirs,
2. Method
2.1. Governing Equations and Turbulence Modeling
2.2. Free-Surface Modeling
2.3. Numerical Schemes
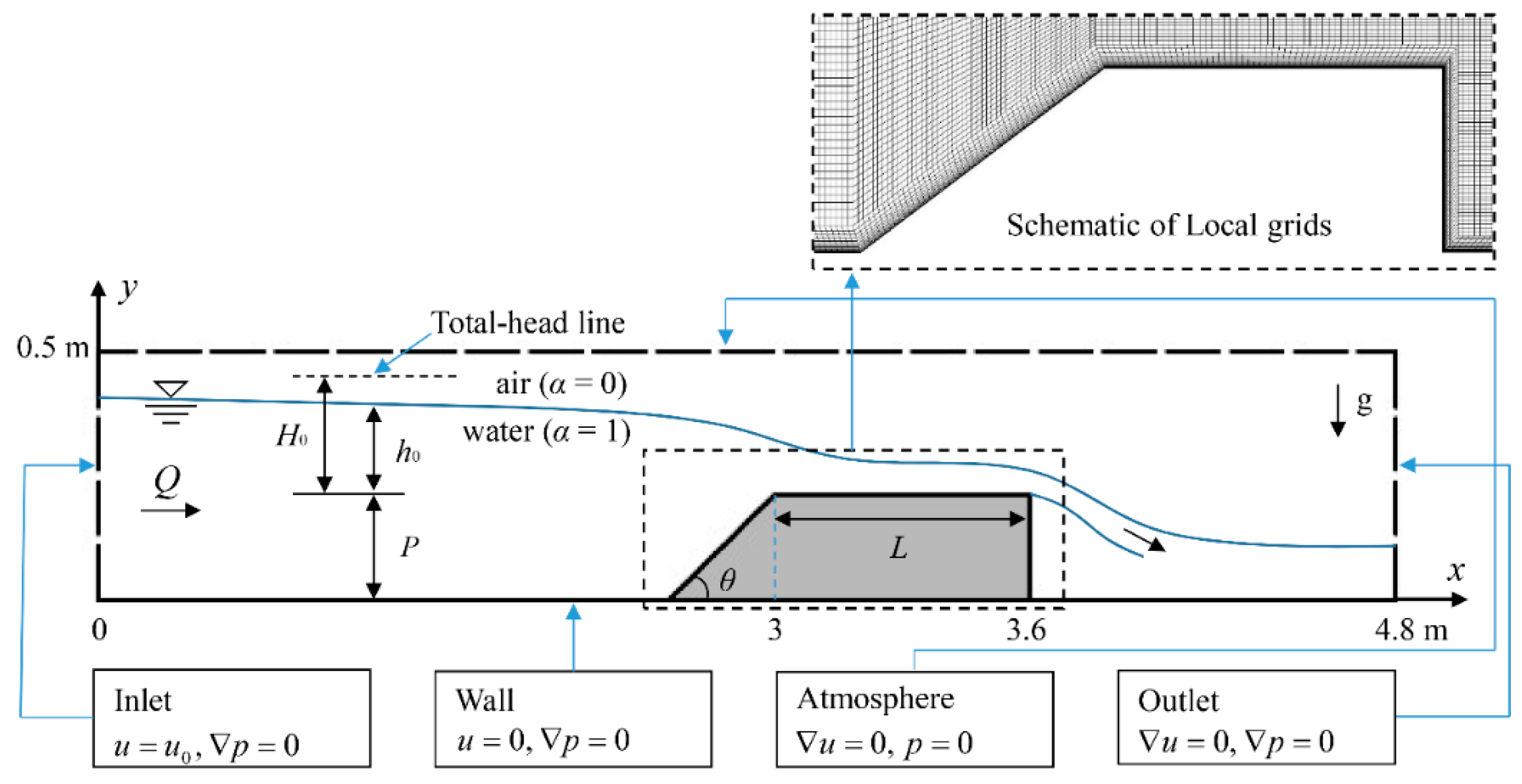
2.4. Broad-Crested Weir Parameters and Simulation Domain
2.5. Boundary Conditions and Mesh
3. Results
3.1. Discretization Error Analysis
3.2. Free-Surface Profiles
3.3. Discharge Coefficient
- For the standard k-ε model and the SST k-w model, the maximum relative errors are 3.957% and 3.439%, respectively, which is an excellent indication.
- For 45° < θ ≤ 90°, the upstream angle increases Cd significantly. However, when θ is less than 45°, the effect of the upstream angle on Cd is no longer obvious. In other words, an upstream slope of less than 45° is more expensive in construction terms and does not improve Cd. Note that this value of θ is approximately 60° (1V:0.5H), as recommended by Noori and Juma [39].
3.4. Computed Streamline Patterns
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| b | width of weir (in the direction perpendicular to the flow direction); |
| Cd | discharge coefficient; |
| e | error between two adjacent meshes; |
| Fs | safety factor; |
| gravity force; | |
| f | value of indicator variable; |
| g | gravitational acceleration; |
| GCI | grid convergence index; |
| H0 | upstream total head; |
| h0 | upstream overflow piezometric head; |
| L | width of weir crest in the flow direction; |
| MAPE | mean absolute percent error; |
| P | weir height; |
| time-averaged pressure; | |
| p | local order of accuracy; |
| Q | flow discharge rate; |
| q | unit discharge; |
| Re | Reynolds number; |
| RE | relative error; |
| R2 | determination coefficient. |
| r | mesh refinement ratio; |
| RMSE | root mean square error; |
| T | total simulation time. |
| t | time; |
| time-averaged velocity vector; | |
| W | Weber number; |
| y+ | dimensionless wall distance; |
| α | volume fraction; |
| θ | upstream face angle; |
| λ | generic fluid property; |
| μ | viscosity of water; |
| ξ | relative crest length; |
| ρ | density; |
| σ | surface tension of water. |
Appendix
References
- Felder, S.; Islam, N. Hydraulic Performance of an Embankment Weir with Rough Crest. J. Hydraul. Eng. 2017, 143, 04016086. [Google Scholar] [CrossRef]
- Hager, W.H.; Schwalt, M. Broad-crested weir. J. Irrig. Drain. Eng. 1994, 120, 13–26. [Google Scholar] [CrossRef]
- Tracy, H.J. Discharge Characteristics of Broad-Crested Weirs; US Department of the Interior, Geological Survey: Washington, DC, USA, 1957.
- Isaacs, L.T. Effects of Laminar Boundary Layer on a Model Broad-Crested Weir; University of Queensland: Brisbane, Australia, 1981. [Google Scholar]
- Horton, R.E.; Murphy, E.C. Weir Experiments, Coefficients, and Formulas; US Government Printing Office: Washington, DC, USA, 1906.
- Kindsvater, C.E. Discharge Characteristics of Embankment-Shaped Weirs; US Government Printing Office: Washington, DC, USA, 1964.
- Fritz, H.M.; Hager, W.H. Hydraulics of embankment weirs. J. Hydraul. Eng. 1998, 124, 963–971. [Google Scholar] [CrossRef]
- Sargison, J.E.; Percy, A. Hydraulics of broad-crested weirs with varying side slopes. J. Irrig. Drain. Eng. 2009, 135, 115–118. [Google Scholar] [CrossRef]
- Azimi, A.H.; Rajaratnam, N.; Zhu, D.Z. Discharge Characteristics of Weirs of Finite Crest Length with Upstream and Downstream Ramps. J. Irrig. Drain. Eng. 2013, 139, 75–83. [Google Scholar] [CrossRef]
- Madadi, M.R.; Dalir, A.H.; Farsadizadeh, D. Control of undular weir flow by changing of weir geometry. Flow Meas. Instrum. 2013, 34, 160–167. [Google Scholar] [CrossRef]
- Hakim, S.S.; Azimi, A.H. Hydraulics of Submerged Triangular Weirs and Weirs of Finite-Crest Length with Upstream and Downstream Ramps. J. Irrig. Drain. Eng. 2017, 143, 06017008. [Google Scholar] [CrossRef]
- Sarker, M.A.; Rhodes, D.G. Calculation of free-surface profile over a rectangular broad-crested weir. Flow Meas. Instrum. 2004, 15, 215–219. [Google Scholar] [CrossRef]
- Kirkgoz, M.S.; Akoz, M.S.; Oner, A.A. Experimental and theoretical analyses of two-dimensional flows upstream of broad-crested weirs. Can. J. Civ. Eng. 2008, 35, 975–986. [Google Scholar] [CrossRef]
- Haun, S.; Olsen, N.R.B.; Feurich, R. Numerical Modeling of Flow Over Trapezoidal Broad-Crested Weir. Eng. Appl. Comput. Fluid Mech. 2011, 5, 397–405. [Google Scholar] [CrossRef] [Green Version]
- Akoz, M.S.; Gumus, V.; Kirkgoz, M.S. Numerical Simulation of Flow over a Semicylinder Weir. J. Irrig. Drain. Eng. 2014, 140, 04014016. [Google Scholar] [CrossRef]
- Lobosco, R.; Schulz, H.; Simões, A. Analysis of two phase flows on stepped spillways. In Hydrodynamics-Optimizing Methods and Tools; InTech: London, UK, 2011. [Google Scholar]
- Sweeney, B.P. Converged Stepped Spillway Models in OpenFOAM. Master’s Thesis, Kansas State University, Manhattan, KS, USA, 2014. [Google Scholar]
- Duguay, J.M.; Lacey, R.W.J.; Gaucher, J. A case study of a pool and weir fishway modeled with OpenFOAM and FLOW-3D. Ecol. Eng. 2017, 103, 31–42. [Google Scholar] [CrossRef]
- Kızılaslan, M.A.; Demirel, E. Numerical Investigation of the Recirculation Zone Length Upstream of the Round-Nosed Broad Crested Weir. In Proceedings of the International Conference on Urban Design, Transportation, Architectural and Environmental Engineering, Istanbul, Turkey, 8–10 September 2017. [Google Scholar]
- Toro, J.P.; Bombardelli, F.A.; Paik, J. Detached Eddy Simulation of the Nonaerated Skimming Flow over a Stepped Spillway. J. Hydraul. Eng. 2017, 143, 04017032. [Google Scholar] [CrossRef]
- Fuentes-Pérez, J.F.; Silva, A.T.; Tuhtan, J.A.; García-Vega, A.; Carbonell-Baeza, R.; Musall, M.; Kruusmaa, M. 3D modelling of non-uniform and turbulent flow in vertical slot fishways. Environ. Model. Softw. 2018, 99, 156–169. [Google Scholar] [CrossRef]
- Launder, B.E.; Sharma, B.I. Application of the energy-dissipation model of turbulence to the calculation of flow near a spinning disc. Lett. Heat Mass Transf. 1974, 1, 131–137. [Google Scholar] [CrossRef]
- Wilcox, D.C. Reassessment of the scale-determining equation for advanced turbulence models. AIAA J. 1988, 26, 1299–1310. [Google Scholar] [CrossRef]
- Menter, F.; Esch, T. Elements of industrial heat transfer predictions. In Proceedings of the 16th Brazilian Congress of Mechanical Engineering (COBEM), Uberlândia, Brazil, 26–30 November 2001. [Google Scholar]
- Menter, F.R.; Kuntz, M.; Langtry, R. Ten years of industrial experience with the SST turbulence model. Turbul. Heat Mass Transf. 2003, 4, 625–632. [Google Scholar]
- Bayon, A.; Toro, J.P.; Bombardelli, F.A.; Matos, J.; López-Jiménez, P.A. Influence of VOF technique, turbulence model and discretization scheme on the numerical simulation of the non-aerated, skimming flow in stepped spillways. J. Hydro-Environ. Res. 2018, 19, 137–149. [Google Scholar] [CrossRef]
- Beg, M.N.A.; Carvalho, R.F.; Tait, S.; Brevis, W.; Rubinato, M.; Schellart, A.; Leandro, J. A comparative study of manhole hydraulics using stereoscopic PIV and different RANS models. Water Sci. Technol. 2018. [Google Scholar] [CrossRef] [PubMed]
- Hirt, C.W.; Nichols, B.D. Volume of fluid (VOF) method for the dynamics of free boundaries. J. Comput. Phys. 1981, 39, 201–225. [Google Scholar] [CrossRef]
- Bayon, A.; Valero, D. Performance assessment of OpenFOAM and FLOW-3D in the numerical modeling of a low Reynolds number hydraulic jump. Environ. Model. Softw. 2016, 80, 322–335. [Google Scholar] [CrossRef]
- Bayon-Barrachina, A. Numerical analysis of hydraulic jumps using OpenFOAM. J. Hydroinform. 2015, 17, 662–678. [Google Scholar] [CrossRef] [Green Version]
- OpenFOAM User Guide; OpenFOAM Foundation: London, UK, 2011; Available online: https://www.openfoam.com/documentation/user-guide/ (accessed on 14 November 2018).
- Zhou, Z.; Hsu, T.J.; Cox, D.; Liu, X. Large-eddy simulation of wave-breaking induced turbulent coherent structures and suspended sediment transport on a barred beach. J. Geophys. Res. 2017, 122, 207–235. [Google Scholar] [CrossRef]
- Goodarzi, E.; Farhoudi, J.; Shokri, N. Flow Characteristics of Rectangular Broad-Crested Weirs with Sloped Upstream Face. J. Hydrol. Hydromech. 2012, 60, 87–100. [Google Scholar] [CrossRef]
- Moukalled, F.; Mangani, L.; Darwish, M. Boundary Conditions in OpenFOAM® and uFVM; Springer International Publishing: Berlin/Heidelberg, Germany, 2016; pp. 745–759. [Google Scholar]
- Jak, E.; Hayes, P.C. Procedure of Estimation and Reporting of Uncertainty Due to Discretization in CFD Applications. J. Fluids Eng. 2008, 130, 078001. [Google Scholar]
- Elsayed, K.; Lacor, C. CFD modeling and multi-objective optimization of cyclone geometry using desirability function, artificial neural networks and genetic algorithms. Appl. Math. Model. 2013, 37, 5680–5704. [Google Scholar] [CrossRef]
- Chen, Y.; Fu, Z.; Chen, Q.; Cui, Z. Discharge Coefficient of Rectangular Short-Crested Weir with Varying Slope Coefficients. Water 2018, 10, 204. [Google Scholar] [CrossRef]
- Madadi, M.R.; Dalir, A.H.; Farsadizadeh, D. Investigation of flow characteristics above trapezoidal broad-crested weirs. Flow Meas. Instrum. 2014, 38, 139–148. [Google Scholar] [CrossRef]
- Noori, B.M.A.; Juma, I.A.K. Performance Improvement of Broad Crested Weirs. AL Rafdain Eng. J. 2009, 17, 95–107. [Google Scholar]
- Zachoval, Z.; Roušar, L. Flow structure in front of the broad-crested weir. In Proceedings of the Experimental Fluid Mechanics 2014, Český Krumlov, Czech Republic, 18–21 November 2014; p. 02117. [Google Scholar]
- Hargreaves, D.; Morvan, H.; Wright, N. Validation of the volume of fluid method for free surface calculation: The broad-crested weir. Eng. Appl. Comput. Fluid Mech. 2007, 1, 136–146. [Google Scholar] [CrossRef]
- Zachoval, Z.; Mistrová, I.; Roušar, L.; Šulc, J.; Zubík, P. Zone of flow separation at the upstream edge of a rectangular broad-crested weir/Oblast odtržení proudu na návodní hraně pravoúhlého přelivu se širokou korunou. J. Hydrol. Hydromech. 2012, 60, 288–298. [Google Scholar] [CrossRef]



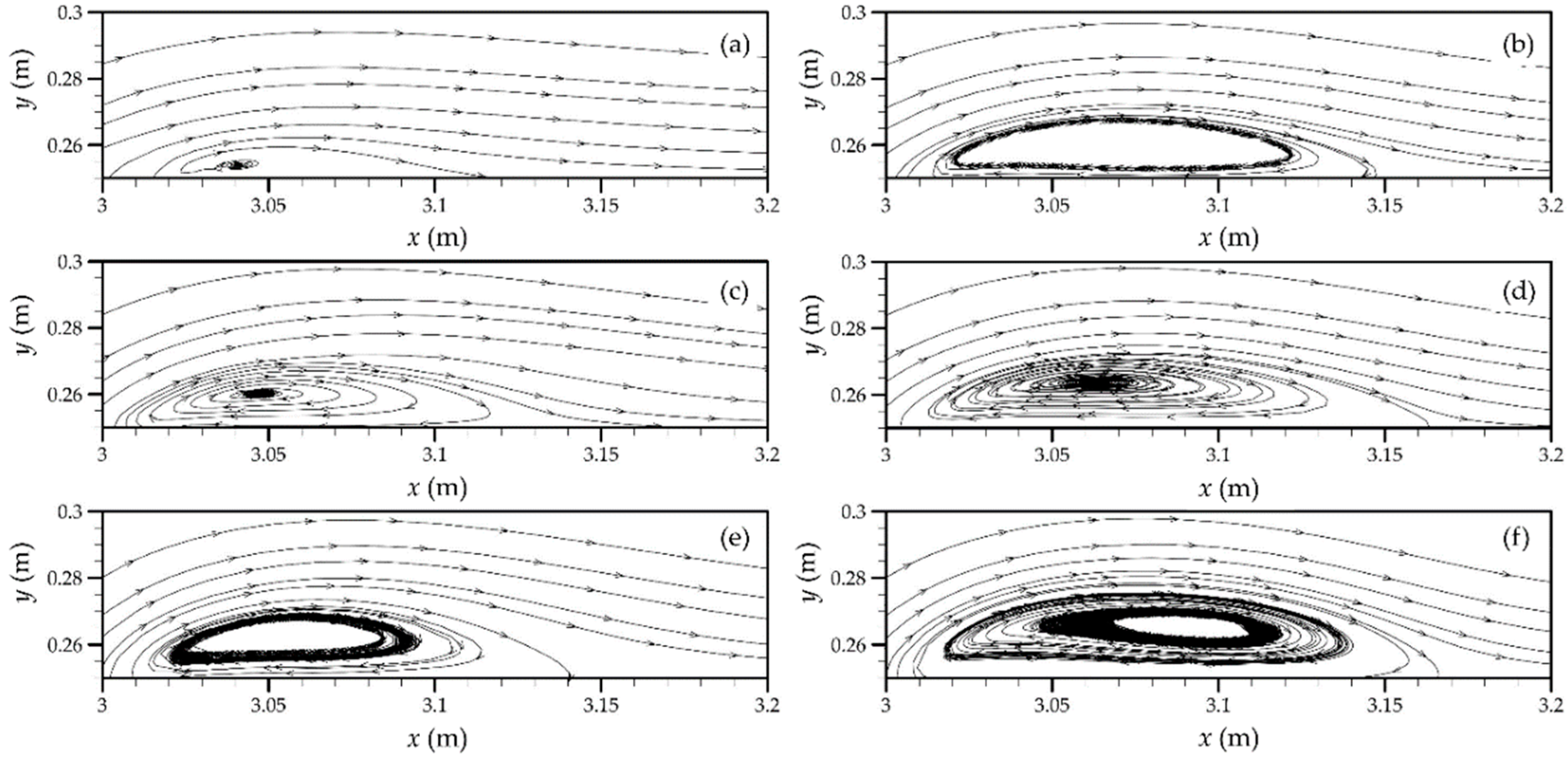
| Angles | Turbulence Model | Section | h1 (m) | pave | GCI12 (%) | GCI23 (%) | GCI32/rpGCI21 | ||
|---|---|---|---|---|---|---|---|---|---|
| f1 | f2 | f3 | |||||||
| θ = 30° | Standard k-e | A-A | 0.16288 | 0.16175 | 0.15969 | 2.138 | 0.025 | 0.023 | 0.8861 |
| B-B | 0.12100 | 0.12074 | 0.12014 | 2.138 | 0.008 | 0.018 | 1.0022 | ||
| C-C | 0.08010 | 0.08016 | 0.08022 | 2.138 | 0.003 | 0.003 | 0.9993 | ||
| SST k-w | A-A | 0.15784 | 0.15837 | 0.15464 | 8.240 | 0.193 | 1.352 | 0.9967 | |
| B-B | 0.11756 | 0.11843 | 0.11990 | 8.240 | 0.425 | 0.712 | 0.9927 | ||
| C-C | 0.08077 | 0.08107 | 0.08188 | 8.240 | 0.213 | 0.573 | 0.9963 | ||
| θ = 45° | Standard k-e | A-A | 0.16718 | 0.16772 | 0.16534 | 12.234 | 0.088 | 0.388 | 0.9968 |
| B-B | 0.12012 | 0.12008 | 0.12145 | 12.234 | 0.009 | 0.312 | 1.0003 | ||
| C-C | 0.08238 | 0.08224 | 0.08208 | 12.234 | 0.047 | 0.053 | 1.0017 | ||
| SST k-w | A-A | 0.16831 | 0.16832 | 0.15086 | 28.472 | 0.000 | 0.243 | 0.9999 | |
| B-B | 0.11759 | 0.11753 | 0.11987 | 28.472 | 0.001 | 0.047 | 1.0005 | ||
| C-C | 0.08170 | 0.07994 | 0.08409 | 28.472 | 0.050 | 0.122 | 1.0220 | ||
| θ = 90° | Standard k-e | A-A | 0.42985 | 0.42951 | 0.43008 | 3.861 | 0.137 | 0.231 | 1.0008 |
| B-B | 0.11402 | 0.11423 | 0.11447 | 3.861 | 0.320 | 0.365 | 0.9982 | ||
| C-C | 0.07816 | 0.07793 | 0.07854 | 3.861 | 0.511 | 1.360 | 1.0160 | ||
| SST k-w | A-A | 0.43169 | 0.43149 | 0.43092 | 6.326 | 0.041 | 0.116 | 1.0005 | |
| B-B | 0.11059 | 0.11033 | 0.11137 | 6.326 | 0.206 | 0.824 | 1.0024 | ||
| C-C | 0.07740 | 0.07709 | 0.07748 | 6.326 | 0.350 | 0.442 | 1.0040 | ||
| Model | θ = 10° | θ = 45° | θ = 90° | |||
|---|---|---|---|---|---|---|
| RMSE | MAPE | RMSE | MAPE | RMSE | MAPE | |
| Standard k-e | 0.35 | 0.50 | 0.67 | 1.48 | 0.58 | 0.67 |
| SST k-w | 0.25 | 0.55 | 0.66 | 1.41 | 0.44 | 0.76 |
| θ | Exp | Cdk-e | Cdk-w | REk-e (%) | REk-w (%) |
|---|---|---|---|---|---|
| 10° | 0.412 | 0.406 | 0.402 | 1.458 | 2.394 |
| 15° | 0.404 | 0.389 | 0.390 | 3.656 | 3.439 |
| 22.5° | 0.396 | 0.380 | 0.386 | 3.957 | 2.327 |
| 30° | 0.385 | 0.378 | 0.380 | 1.656 | 1.357 |
| 45° | 0.383 | 0.369 | 0.370 | 3.451 | 3.261 |
| 60° | 0.368 | 0.367 | 0.355 | 0.256 | 3.458 |
| 75° | 0.355 | 0.353 | 0.353 | 0.440 | 0.440 |
| 90° | 0.347 | 0.345 | 0.344 | 0.532 | 0.990 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiang, L.; Diao, M.; Sun, H.; Ren, Y. Numerical Modeling of Flow Over a Rectangular Broad-Crested Weir with a Sloped Upstream Face. Water 2018, 10, 1663. https://doi.org/10.3390/w10111663
Jiang L, Diao M, Sun H, Ren Y. Numerical Modeling of Flow Over a Rectangular Broad-Crested Weir with a Sloped Upstream Face. Water. 2018; 10(11):1663. https://doi.org/10.3390/w10111663
Chicago/Turabian StyleJiang, Lei, Mingjun Diao, Haomiao Sun, and Yu Ren. 2018. "Numerical Modeling of Flow Over a Rectangular Broad-Crested Weir with a Sloped Upstream Face" Water 10, no. 11: 1663. https://doi.org/10.3390/w10111663
APA StyleJiang, L., Diao, M., Sun, H., & Ren, Y. (2018). Numerical Modeling of Flow Over a Rectangular Broad-Crested Weir with a Sloped Upstream Face. Water, 10(11), 1663. https://doi.org/10.3390/w10111663




