Viscosity Approximation Methods for a General Variational Inequality System and Fixed Point Problems in Banach Spaces
Abstract
:1. Introduction
2. Preliminaries
3. Main Results
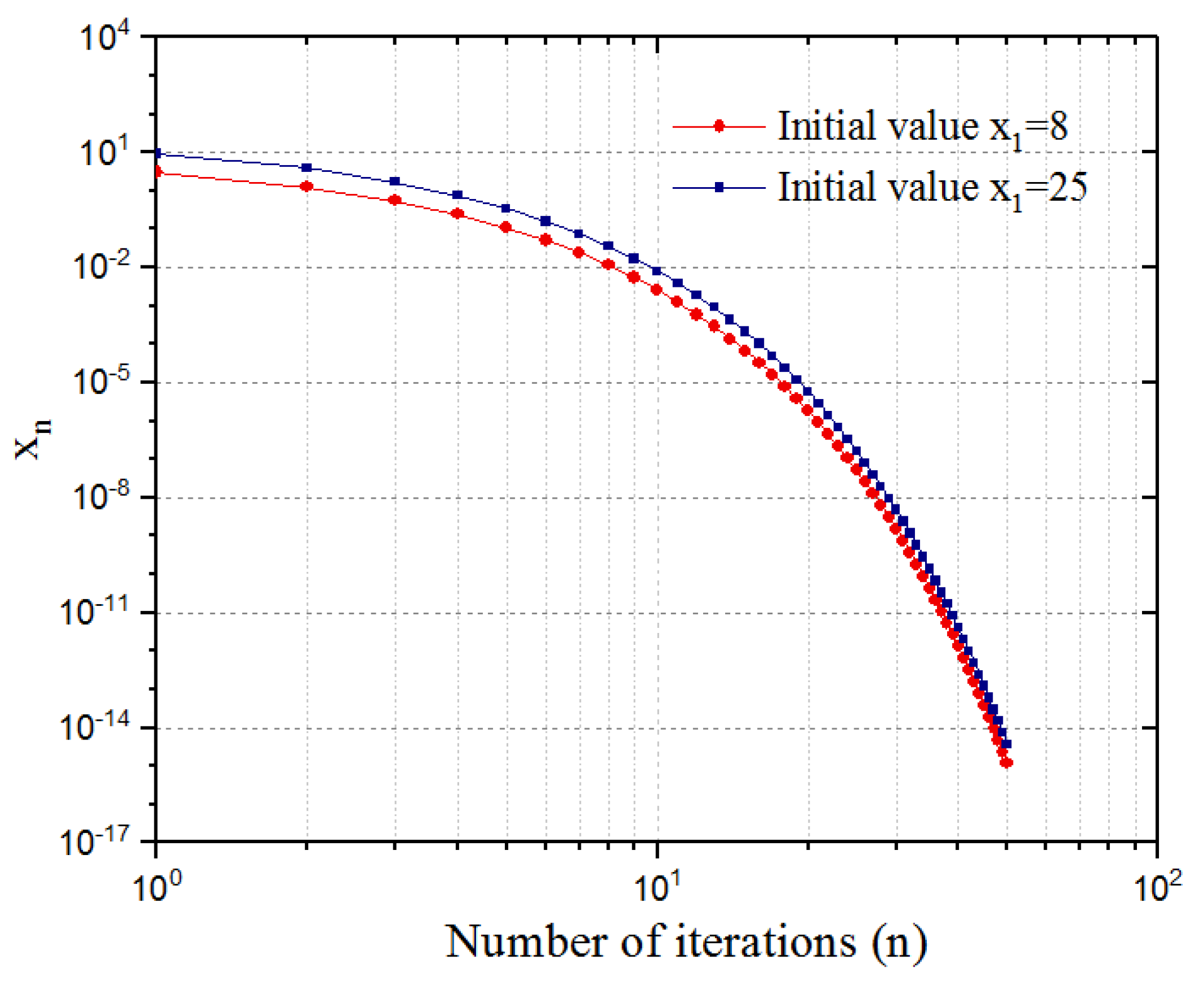
4. Numerical Examples
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Moudafi, A. Viscosity approximation methods for fixed-points problems. J. Math. Anal. Appl. 2000, 241, 46–55. [Google Scholar] [CrossRef] [Green Version]
- Yao, Y.; Maruster, S. Strong convergence of an iterative algorithm for variational inequalities in Banach spaces. Math. Comput. Modell. 2011, 54, 325–329. [Google Scholar] [CrossRef]
- Ceng, L.C.; Yao, J.C.; Muglia, L. An extragradient-like approximation method for variational inequality problems and fixed point problems. Appl. Math. Comput. 2007, 190, 205–215. [Google Scholar] [CrossRef]
- Xie, S.; Imani, M.; Dougherty, E.R.; Braga-Neto, U.M. Nonstationary Linear Discriminant Analysis. In Proceedings of the 51st Asilomar Conference on Signals, Systems, and Computers, Pacific Grove, CA, USA, 29 October–1 November 2017; pp. 161–165. [Google Scholar]
- Imnang, S. Viscosity iterative method for a new general system of variational inequalities in Banach spaces. J. Inequalities Appl. 2013, 2013, 249. [Google Scholar] [CrossRef] [Green Version]
- Qin, X.; Cho, S.Y.; Kang, S.M. Convergence of an iterative algorithm for systems of variational inequalities and nonexpansive mappings with applications. J. Comput. Appl. Math. 2009, 233, 231–240. [Google Scholar] [CrossRef] [Green Version]
- Sen, M.D.L.; Muglia, L. Fixed and best proximity points of cyclic jointly accretive and contractive self-mappings. J. Appl. Math. 2012, 2012, 419–429. [Google Scholar]
- Pan, C.; Wang, Y. Generalized viscosity implicit iterative process for asymptotically non-expansive mappings in Banach spaces. Mathematics 2019, 7, 379. [Google Scholar] [CrossRef] [Green Version]
- Iiduka, H.; Takahashi, W.; Toyoda, M. Approximation of solutions of variational inequalities for monotone mappings. Pan. Math. J. 2004, 14, 49–61. [Google Scholar]
- Yao, Y.; Noor, M.A.; Noor, K.I.; Liou, Y.C.; Yaqoob, H. Modified extragradient methods for a system of variational inequalities in Banach spaces. Acta Appl. Math. 2010, 110, 1211–1224. [Google Scholar] [CrossRef]
- Ceng, L.C.; Petrusel, A.; Yao, J.C.; Yao, Y. Systems of variational inequalities with hierarchical variational inequality constraints for Lipschitzian pseudocontractions. Fixed Point Theory 2019, 20, 113–134. [Google Scholar] [CrossRef] [Green Version]
- Katchang, P.; Kumam, P. Convergence of iterative algorithm for finding common solution of fixed points and general system of variational inequalities for two accretive operators. Positivity 2012, 15, 281–295. [Google Scholar] [CrossRef]
- Cai, G.; Shehu, Y.; Iyiola, O.S. Iterative algorithms for solving variational inequalities and fixed point problems for asymptotically nonexpansive mappings in Banach spaces. Numer. Algorithms 2016, 73, 869–906. [Google Scholar] [CrossRef]
- Cai, G.; Shehu, Y.; Iyiola, O.S. Strong convergence theorems for fixed point problems for strict pseudo-contractions and variational inequalities for inverse-strongly accretive mappings in uniformly smooth Banach spaces. J. Fixed Point Theory Appl. 2019, 21, 41. [Google Scholar] [CrossRef]
- Ceng, L.C.; Petrusel, A.; Yao, J.C.; Yao, Y. Hybrid viscosity extragradient method for systems of variational inequalities, fixed points of nonexpansive mappings, zero points of accretive operators in Banach spaces. Fixed Point Theory 2018, 19, 487–502. [Google Scholar] [CrossRef]
- Sunthrayuth, P.; Kumam, P. Viscosity approximation methods base on generalized contraction mappings for a countable family of strict pseudo-contractions, a general system of variational inequalities and a generalized mixed equilibrium problem in Banach spaces. Math. Comput. Model. 2013, 58, 1814–1828. [Google Scholar] [CrossRef]
- Luo, H.; Wang, Y. Iterative approximation for the common solutions of a infinite variational inequality system for inverse-strongly accretive mappings. J. Math. Comput. Sci. 2012, 6, 1660–1670. [Google Scholar]
- Reich, S. Asymptotic behavior of contractions in Banach spaces. J. Math. Anal. Appl. 1973, 44, 57–70. [Google Scholar] [CrossRef] [Green Version]
- Liu, L.S. Iterative processes with errors for nonlinear strongly accretive mappings in Banach spaces. J. Math. Anal. Appl. 1995, 194, 114–125. [Google Scholar] [CrossRef] [Green Version]
- Yao, Y.; Shahzad, N.; Liou, Y.C. Modified semi-implicit midpoint rule for nonexpansive mappings. Fixed Point Theory Appl. 2015, 2015, 166. [Google Scholar] [CrossRef] [Green Version]
- Lim, T.C.; Xu, H.K. Fixed point theorems for asymptotically nonexpansive mappings. Nonlinear Anal. 1994, 22, 1345–1355. [Google Scholar] [CrossRef]
- Xu, H.K. Viscosity approximation methods for nonexpansive mappings. J. Math. Anal. Appl. 2004, 298, 279–291. [Google Scholar] [CrossRef] [Green Version]
- Kamimura, S.; Takahashi, W. Strong convergence of a proximal-type algorithm in a Banach space. SIAM J. Optim. 2002, 13, 938–945. [Google Scholar] [CrossRef]

© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Pan, C. Viscosity Approximation Methods for a General Variational Inequality System and Fixed Point Problems in Banach Spaces. Symmetry 2020, 12, 36. https://doi.org/10.3390/sym12010036
Wang Y, Pan C. Viscosity Approximation Methods for a General Variational Inequality System and Fixed Point Problems in Banach Spaces. Symmetry. 2020; 12(1):36. https://doi.org/10.3390/sym12010036
Chicago/Turabian StyleWang, Yuanheng, and Chanjuan Pan. 2020. "Viscosity Approximation Methods for a General Variational Inequality System and Fixed Point Problems in Banach Spaces" Symmetry 12, no. 1: 36. https://doi.org/10.3390/sym12010036
APA StyleWang, Y., & Pan, C. (2020). Viscosity Approximation Methods for a General Variational Inequality System and Fixed Point Problems in Banach Spaces. Symmetry, 12(1), 36. https://doi.org/10.3390/sym12010036




