Dynamic Asymmetries Do Not Match Spatiotemporal Step Asymmetries during Split-Belt Walking
Abstract
:1. Introduction
2. Materials and Methods
2.1. Participants
2.2. Instruments and Methods
2.3. Experimental Protocol
2.4. Experimental Session
2.5. Tagging Walking Patterns and Lower Limbs
2.6. Tagging the Test Phases
- (1)
- Habituation: 3 min tied-walking at a velocity increasing from 0.2 m s−1 (h-0202) to 1.2 m s−1 (h-1212). These changes occurred in 0.2 m s−1 increments, with each tested velocity lasting about 30 s. At the end of this phase, rest for about 1–2 min was allowed.
- (2)
- Baseline: 30 s tied-walking at 0.4 m s−1 (b-0404).
- (3)
- Adaptation: 15 min split-walking; at the end of the baseline, the belt’s velocity under the dominant lower limb was increased to 1.2 m s−1 (0412).
- (4)
- For analysis, the adaptation phase was further divided into two phases:
- (a)
- initial adaptation: including the 7th to 12th strides (i-0412).
- (b)
- final adaptation: including the last six strides (f-0412).
- (5)
- Post-adaptation: Return to tied-walking at 0.4 m s−1 for 5 min (0404post).
- (a)
- initial post-adaptation: the 7th to 12th strides (i-0404post).
- (b)
- final post-adaptation: the last six strides (f-0404post).
2.7. Tagging Spatiotemporal Walking Variables
- Step: the ensemble of kinematic and dynamic events taking place between two subsequent foot–ground contacts.
- SL: the sagittal distance between the markers put on the lateral malleolus of the posterior and anterior feet at the ground strike of the anterior foot.
- Side of the step: the side of the posterior foot during a double stance.
- Single stance time (SST): for each lower limb, the time interval during which a vertical ground reaction ≥ 30 N was recorded under the limb.
- Double stance time (DST): the time interval during which a vertical ground reaction ≥ 30 N was recorded under both lower limbs.
- Side of the double stance time (pDST): the side of the posterior foot.
2.8. Correction of Drift in Force Signals
2.9. Computing the Velocity of the Body’s Centre of Mass
2.10. Test Sequencing
2.11. Data Analysis
2.12. Statistical Analysis
2.13. Computations
2.14. Ethical Approval
3. Results
3.1. Dynamic and Spatiotemporal Observations
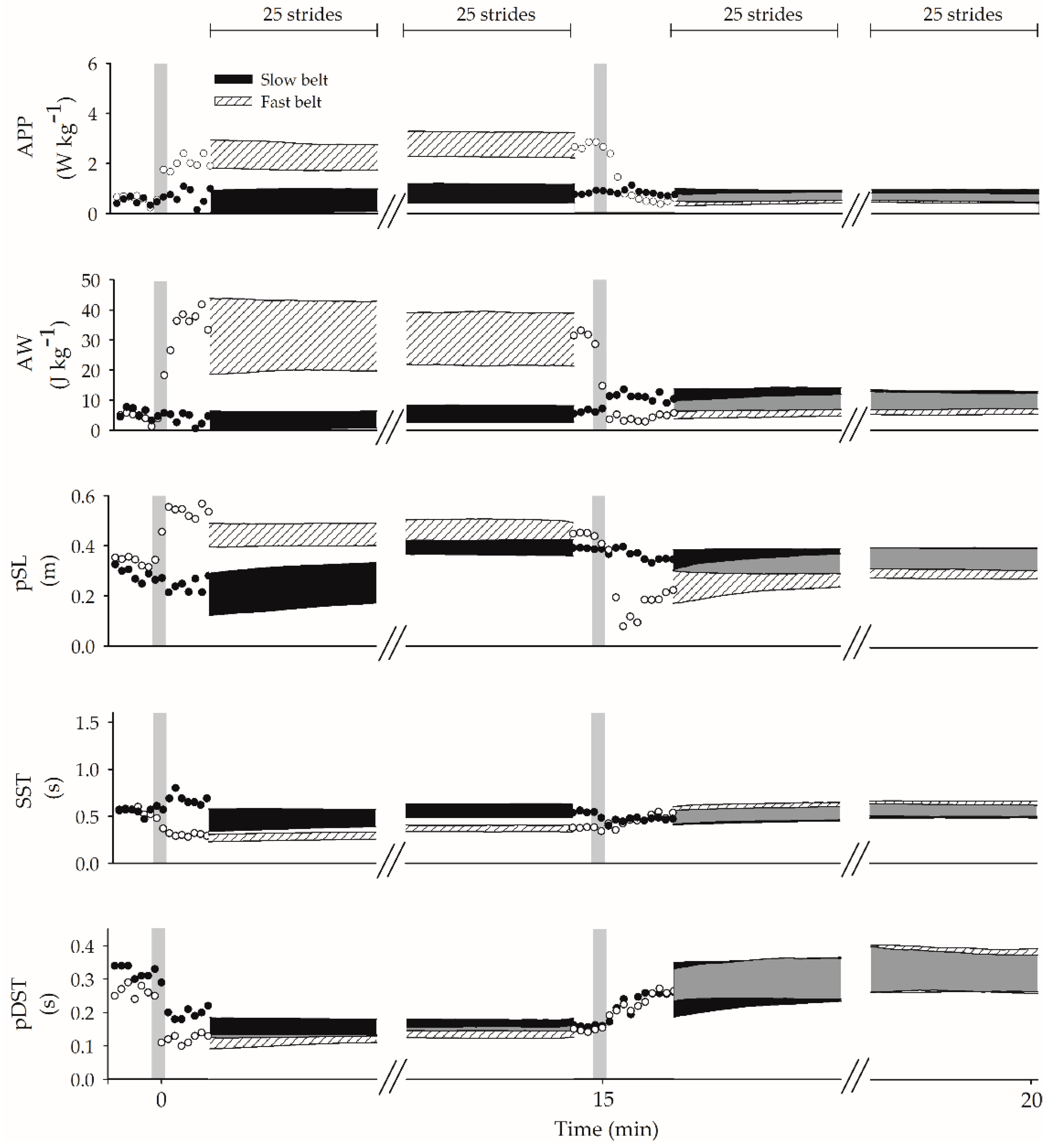
3.2. Graphic Description of Dynamic Changes
3.2.1. APP
3.2.2. AW
3.3. Graphic Description of Spatiotemporal Changes
3.3.1. pSL
3.3.2. SST
3.3.3. pDST
3.4. Graphic Summary of the Time Course of Walking Parameters
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Dietz, V.; Zijlstra, W.; Duysens, J. Human neuronal interlimb coordination during split-belt locomotion. Exp. Brain Res. 1994, 101, 513–520. [Google Scholar] [CrossRef] [Green Version]
- Reisman, D.S.; McLean, H.; Bastian, A.J. Split-belt treadmill training poststroke: A case study. J. Neurol. Phys. Ther. 2010, 34, 202–207. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Reisman, D.S.; McLean, H.; Keller, J.; Danks, K.A.; Bastian, A.J. Repeated split-belt treadmill training improves poststroke step length asymmetry. Neurorehabil. Neural Repair 2013, 27, 460–468. [Google Scholar] [CrossRef] [Green Version]
- Reisman, D.S.; Wityk, R.; Silver, K.; Bastian, A.J. Split-belt treadmill adaptation transfers to overground walking in persons poststroke. Neurorehabil. Neural Repair 2009, 23, 735–744. [Google Scholar] [CrossRef] [Green Version]
- Kline, P.; Murray, A.; Mille, M.; Fields, T.; Christiansen, C. Error-augmentation gait training to improve gait symmetry in patients with non-traumatic lower limb amputation: A proof of concept study. Prosthet. Orthot. Int. 2019, 43, 426–433. [Google Scholar] [CrossRef] [PubMed]
- Lauzière, S.; Mièville, C.; Betschart, M.; Duclos, C.; Aissaoui, R.; Nadeau, S. A more symmetrical gait after split-belt treadmill walking increases the effort in paretic plantar flexors in people post-stroke. J. Rehabil. Med. 2016, 48, 576–582. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Tesio, L.; Malloggi, C.; Malfitano, C.; Coccetta, C.A.; Catino, L.; Rota, V. Limping on split-belt treadmills implies opposite kinematic and dynamic lower limb asymmetries. Int. J. Rehabil. Res. 2018, 41, 304–315. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Sutherland, D.H. An electromyographic study of the plantar flexors of the ankle in normal walking on the level. J. Bone Jt. Surg. 1966, 48, 66–71. [Google Scholar] [CrossRef]
- Meinders, M.; Gitter, A.; Czerniecki, J.M. The role of ankle plantar flexor muscle work during walking. Scand. J. Rehabil. Med. 1998, 30, 39–46. [Google Scholar] [CrossRef]
- Kepple, T.M.; Siegel, K.L.; Stanhope, S.J. Relative contributions of the lower extremity joint moments to forward progression and support during gait. Gait Posture 1997, 6, 1–8. [Google Scholar] [CrossRef]
- Zelik, K.E.; Adamczyk, P.G. A unified perspective on ankle push-off in human walking. J. Exp. Biol. 2016, 219, 3676–3683. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Vervoort, D.; Rob Den Otter, A.; Buurke, T.J.W.; Vuillerme, N.; Hortobágyi, T.; Lamoth, C.J.C. Effects of aging and task prioritization on split-belt gait adaptation. Front. Aging Neurosci. 2019, 11, 1–12. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hinton, D.C.; Conradsson, D.; Bouyer, L.; Paquette, C. Does dual task placement and duration affect split-belt treadmill adaptation? Gait Posture 2020, 75, 115–120. [Google Scholar] [CrossRef] [PubMed]
- Selgrade, B.P.; Thajchayapong, M.; Lee, G.E.; Toney, M.E.; Chang, Y.H. Changes in mechanical work during neural adaptation to asymmetric locomotion. J. Exp. Biol. 2017, 220, 2993–3000. [Google Scholar] [CrossRef] [Green Version]
- Reisman, D.S.; Wityk, R.; Silver, K.; Bastian, A.J. Locomotor adaptation on a split-belt treadmill can improve walking symmetry post-stroke. Brain 2007, 130, 1861–1872. [Google Scholar] [CrossRef] [Green Version]
- Reisman, D.S.; Block, H.J.; Bastian, A.J. Interlimb coordination during locomotion: What can be adapted and stored? J. Neurophysiol. 2005, 94, 2403–2415. [Google Scholar] [CrossRef] [Green Version]
- Martin, T.A.; Keating, J.G.; Goodkin, H.P.; Bastian, A.J.; Thach, W.T. Throwing while looking through prisms II. Specificity and storage of multiple gaze-throw calibrations. Brain 1996, 119, 1199–1211. [Google Scholar] [CrossRef] [Green Version]
- Kline, P.W.; Murray, A.M.; Miller, M.J.; So, N.; Fields, T.; Christiansen, C.L. Step length symmetry adaptation to split-belt treadmill walking after acquired non-traumatic transtibial amputation. Gait Posture 2020, 80, 162–167. [Google Scholar] [CrossRef]
- Helm, E.E.; Reisman, D.S. The split-belt walking paradigm: Exploring motor learning and spatiotemporal asymmetry poststroke. Phys. Med. Rehabil. Clin. N. Am. 2015, 26, 703–713. [Google Scholar] [CrossRef] [Green Version]
- Patterson, K.K.; Gage, W.H.; Brooks, D.; Black, S.E.; McIlroy, W.E. Evaluation of gait symmetry after stroke: A comparison of current methods and recommendations for standardization. Gait Posture 2010, 31, 241–246. [Google Scholar] [CrossRef] [PubMed]
- Rudroff, T.; Proessl, F. Effects of muscle function and limb loading asymmetries on gait and balance in people with multiple sclerosis. Front. Physiol. 2018, 15, 531. [Google Scholar] [CrossRef]
- Tesio, L.; Civaschi, P.; Tessari, L. Motion of the center of gravity of the body in clinical evaluation of gait. Am. J. Phys. Med. 1985, 64, 57–70. [Google Scholar]
- Cavagna, G.A.; Tesio, L.; Fuchimoto, T.; Heglund, N.C. Ergometric evaluation of pathological gait. J. Appl. Physiol. 1983, 55, 607–613. [Google Scholar] [CrossRef] [PubMed]
- Tesio, L.; Lanzi, D.; Detrembleur, C. The 3-D motion of the centre of gravity of the human body during level walking. II. Lower limb amputees. Clin. Biomech. 1998, 13, 83–90. [Google Scholar] [CrossRef]
- Rota, V.; Benedetti, M.G.; Okita, Y.; Manfrini, M.; Tesio, L. Knee rotationplasty: Motion of the body centre of mass during walking. Int. J. Rehabil. Res. 2016, 39, 346–353. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Roemmich, R.T.; Stegemöller, E.L.; Hass, C.J. Lower extremity sagittal joint moment production during split-belt treadmill walking. J. Biomech. 2012, 45, 2817–2821. [Google Scholar] [CrossRef] [Green Version]
- Tesio, L.; Rota, V.; Malloggi, C.; Brugliera, L.; Catino, L. Crouch gait can be an effective form of forced-use/no constraint exercise for the paretic lower limb in stroke. Int. J. Rehabil. Res. 2017, 40, 254–267. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Elias, L.J.; Bryden, M.P.; Bulman-Fleming, M.B. Footedness is a better predictor than is handedness of emotional lateralization. Neuropsychologia 1998, 36, 37–43. [Google Scholar] [CrossRef]
- Tesio, L.; Rota, V. Gait analysis on split-belt force treadmills: Validation of an instrument. Am. J. Phys. Med. Rehabil. 2008, 87, 515–526. [Google Scholar] [CrossRef]
- Davis, R.B.; Õunpuu, S.; Tyburski, D.; Gage, J.R. A gait analysis data collection and reduction technique. Hum. Mov. Sci. 1991, 10, 575–587. [Google Scholar] [CrossRef]
- Ogawa, T.; Kawashima, N.; Ogata, T.; Nakazawa, K. Predictive control of ankle stiffness at heel contact is a key element of locomotor adaptation during split-belt treadmill walking in humans. J. Neurophysiol. 2014, 111, 722–732. [Google Scholar] [CrossRef] [Green Version]
- Finley, J.M.; Bastian, A.J.; Gottschall, J.S. Learning to be economical: The energy cost of walking tracks motor adaptation. J. Physiol. 2013, 591, 1081–1095. [Google Scholar] [CrossRef]
- Lauzière, S.; Mièville, C.; Betschart, M.; Duclos, C.; Aissaoui, R.; Nadeau, S. Plantarflexion moment is a contributor to step length after-effect following walking on a split-belt treadmill in individuals with stroke and healthy individuals. J. Rehabil. Med. 2014, 46, 849–857. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Roper, J.A.; Roemmich, R.T.; Tillman, M.D.; Terza, M.J.; Hass, C.J. Split-belt treadmill walking alters lower extremity frontal plane mechanics. J. Appl. Biomech. 2017, 33, 256–260. [Google Scholar] [CrossRef] [PubMed]
- Tesio, L.; Rota, V. The motion of the body center of mass during walking: A review oriented to clinical applications. Front. Neurol. 2019, 10. [Google Scholar] [CrossRef] [PubMed]
- Bocian, M.; Brownjohn, J.M.W.; Racic, V.; Hester, D.; Quattrone, A.; Monnickendam, R. A framework for experimental determination of localised vertical pedestrian forces on full-scale structures using wireless attitude and heading reference systems. J. Sound Vib. 2016, 376, 217–243. [Google Scholar] [CrossRef] [Green Version]
- Hurkmans, H.L.P.; Bussmann, J.B.J.; Benda, E.; Verhaar, J.A.N.; Stam, H.J. Accuracy and repeatability of the pedar mobile system in long-term vertical force measurements. Gait Posture 2006, 23, 118–125. [Google Scholar] [CrossRef]
- Tesio, L.; Scarano, S.; Cerina, V.; Malloggi, C.; Catino, L. Velocity of the body center of mass during walking on split-belt treadmill. Am. J. Phys. Med. Rehabil. 2021, 100, 620–624. [Google Scholar] [CrossRef]
- Tesio, L.; Malloggi, C.; Portinaro, N.M.; Catino, L.; Lovecchio, N.; Rota, V. Gait analysis on force treadmill in children: Comparison with results from ground-based force platforms. Int. J. Rehabil. Res. 2017, 40, 315–324. [Google Scholar] [CrossRef] [Green Version]
- Cohen, J. Eta-squared and partial eta-squared in fixed factor Anova designs. Educ. Psychol. Meas. 1973, 33, 107–112. [Google Scholar] [CrossRef]
- Tesio, L.; Roi, G.S.; Möller, F. Pathological gaits: Inefficiency is not a rule. Clin. Biomech. 1991, 6, 47–50. [Google Scholar] [CrossRef]
- Milot, M.-H.; Nadeau, S.; Gravel, D. Muscular utilization of the plantarflexors, hip flexors and extensors in persons with hemiparesis walking at self-selected and maximal speeds. J. Electromyogr. Kinesiol. 2007, 17, 184–193. [Google Scholar] [CrossRef]
- Werner, C.; Lindquist, A.R.; Bardeleben, A.; Hesse, S. The influence of treadmill inclination on the gait of ambulatory hemiparetic subjects. Neurorehabil. Neural Repair 2007, 21, 76–80. [Google Scholar] [CrossRef]
- Betschart, M.; McFayden, B.J.; Nadeau, S. Lower limb joint moments on the fast belt contribute to a reduction of step length asymmetry over ground after split-belt treadmill training in stroke: A pilot study. Physiother. Theory Pract. 2020, 36, 989–999. [Google Scholar] [CrossRef]
- Ryan, H.P.; Husted, C.; Lewek, M.D. Improving spatiotemporal gait asymmetry has limited functional benefit for individuals poststroke. J. Neurol. Phys. Ther. 2020, 44, 197–204. [Google Scholar] [CrossRef]
- Patton, J.L.; Stoykov, M.E.; Kovic, M.; Mussa-Ivaldi, F.A. Evaluation of robotic training forces that either enhance or reduce error in chronic hemiparetic stroke survivors. Exp. Brain Res. 2006, 168, 368–383. [Google Scholar] [CrossRef]
- Torres-Oviedo, G.; Bastian, A.J. Natural error patterns enable transfer of motor learning to novel contexts. J. Neurophysiol. 2012, 107, 346–356. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Sánchez, N.; Park, S.; Finley, J.M. Evidence of energetic optimization during adaptation differs for metabolic, mechanical, and perceptual estimates of energetic cost. Sci. Rep. 2017, 7, 7682. [Google Scholar] [CrossRef] [Green Version]
- Redding, G.M.; Wallace, B. Adaptive eye-hand coordination: Implications of prism adaptation for perceptual-motor organization. Adv. Psychol. 1992, 85, 105–127. [Google Scholar]
- Rossetti, Y.; Koga, K.; Mano, T. Prismatic displacement of vision induces transient changes in the timing of eye-hand coordination. Percept. Psychophys. 1993, 54, 355–364. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Rossetti, Y.; Rode, G.; Pisella, L.; Farné, A.; Li, L.; Boisson, D.; Perenin, R.S. Prism adaptation to a rightward optical deviation rehabilitates left hemispatial neglect. Lett. Nat. 1998, 395, 8–11. [Google Scholar] [CrossRef]
- Redding, G.M.; Rossetti, Y.; Wallace, B. Applications of prism adaptation: A tutorial in theory and method. Neurosci. Biobehav. Rev. 2005, 29, 431–444. [Google Scholar] [CrossRef]
- Kleynen, M.; Braun, S.M.; Bleijlevens, M.H.; Lexis, M.A.; Rasquin, S.M.; Halfens, J.; Wilson, M.R.; Beurskens, A.J.; Masters, R.S.W. Using a delphi technique to seek consensus regarding definitions, descriptions and classification of terms related to implicit and explicit forms of motor learning. PLoS ONE 2014, 9, e100227. [Google Scholar] [CrossRef] [Green Version]
- French, M.A.; Morton, S.M.; Reisman, D.S. Use of explicit processes during a visually guided locomotor learning task predicts 24-h retention after stroke. J. Neurophysiol. 2021, 125, 211–222. [Google Scholar] [CrossRef]
- Lacquaniti, F.; Ivanenko, Y.P.; Zago, M. Patterned control of human locomotion. J. Physiol. 2012, 590, 2189–2199. [Google Scholar] [CrossRef]
- Grillner, S.; El Manira, A. Current principles of motor control, with special reference to vertebrate locomotion. Physiol. Rev. 2020, 100, 271–320. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hsiao, H.Y.; Knarr, B.A.; Higginson, J.S.; Binder-Macleod, S.A. The relative contribution of ankle moment and trailing limb angle to propulsive force during gait. Hum. Mov. Sci. 2015, 39, 212–221. [Google Scholar] [CrossRef] [Green Version]
- Wagner, D.R. ACSM’s Resource Manual for Guidelines for Exercise Testing and Prescription, 7th ed.; Kluwer, W., Ed.; 2014; Available online: https://shop.lww.com/ (accessed on 1 May 2021).
- Sánchez, N.; Simha, S.N.; Donelan, J.M.; James, M.; Finley, J.M. Taking advantage of external mechanical work to reduce metabolic cost: The mechanics and energetics of split-belt treadmill walking. J. Physiol. 2019, 597, 4053–4068. [Google Scholar] [CrossRef] [PubMed]
- Roper, J.A.; Stegemöller, E.L.; Tillman, M.D.; Hass, C.J. Oxygen consumption, oxygen cost, heart rate, and perceived effort during split-belt treadmill walking in young healthy adults. Eur. J. Appl. Physiol. 2013, 113, 729–734. [Google Scholar] [CrossRef] [PubMed]
- Sombric, C.J.; Torres-Oviedo, G. Augmenting propulsion demands during split-belt walking increases locomotor adaptation of asymmetric step lengths. J. Neuroeng. Rehabil. 2020, 17. [Google Scholar] [CrossRef] [PubMed]
- Stenum, J.; Choi, J.T. Step time asymmetry but not step length asymmetry is adapted to optimize energy cost of split-belt treadmill walking. J. Physiol. 2020, 598, 4063–4078. [Google Scholar] [CrossRef] [PubMed]
| Habituation | Baseline | Adaptation | Post-Adaptation | |
|---|---|---|---|---|
| h-0202 → h-0404 → h-0606 → h-0808 → h-1010 → h-1212 | Rest | b-0404 | 0412 | 0404post |
| 3 min | 2 min | 30 s | 15 min | 5 min |
| APP (W kg−1) | AW (J kg−1) | pSL (m) | SST (s) | pDST (s) | ||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Fast | Slow | Fast | Slow | Fast | Slow | Fast | Slow | Fast | Slow | |||||||||||
| Mean | SD | Mean | SD | Mean | SD | Mean | SD | Mean | SD | Mean | SD | Mean | SD | Mean | SD | Mean | SD | Mean | SD | |
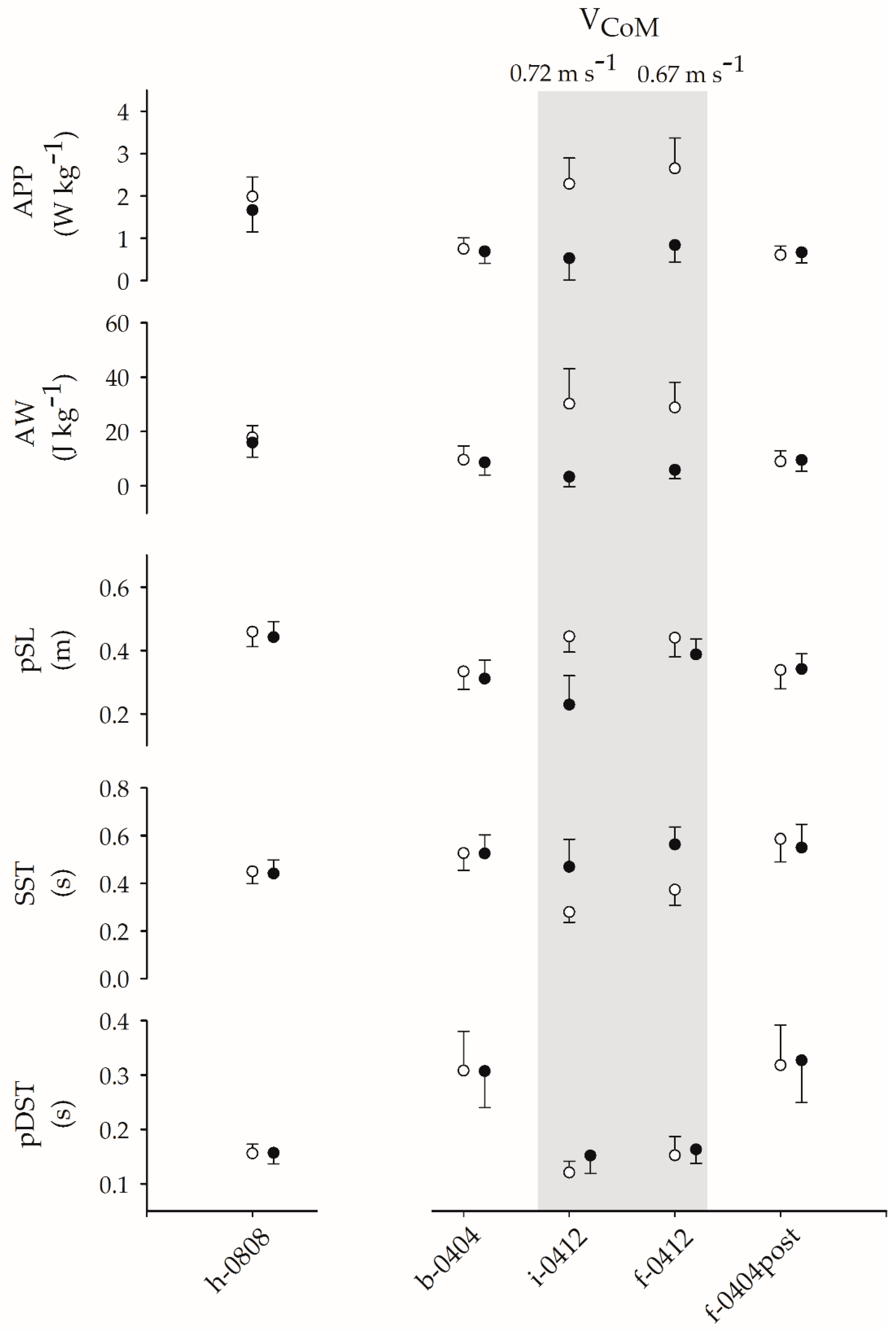
| h-0808 | 1.98 | 0.46 | 1.66 | 0.51 | 17.82 | 4.39 | 15.9 | 5.31 | 0.46 | 0.05 | 0.44 | 0.05 | 0.45 | 0.05 | 0.44 | 0.06 | 0.16 | 0.02 | 0.16 | 0.02 |
| b-0404 | 0.75 | 0.26 | 0.69 | 0.28 | 9.61 | 5.09 | 8.61 | 4.62 | 0.33 | 0.06 | 0.31 | 0.06 | 0.53 | 0.07 | 0.53 | 0.08 | 0.31 | 0.07 | 0.31 | 0.07 |
| i-0412 | 2.28 | 0.61 | 0.52 | 0.51 | 30.18 | 12.90 | 3.32 | 3.62 | 0.44 | 0.05 | 0.23 | 0.09 | 0.28 | 0.04 | 0.47 | 0.12 | 0.12 | 0.02 | 0.15 | 0.03 |
| f-0412 | 2.65 | 0.72 | 0.84 | 0.40 | 28.81 | 9.32 | 5.85 | 3.21 | 0.44 | 0.06 | 0.39 | 0.05 | 0.37 | 0.07 | 0.56 | 0.07 | 0.15 | 0.03 | 0.16 | 0.03 |
| i-0404post | 0.49 | 0.38 | 0.70 | 0.35 | 6.04 | 5.84 | 10.19 | 5.29 | 0.35 | 0.04 | 0.22 | 0.09 | 0.51 | 0.13 | 0.46 | 0.09 | 0.26 | 0.12 | 0.27 | 0.06 |
| f-0404post | 0.60 | 0.21 | 0.66 | 0.25 | 8.97 | 3.97 | 9.51 | 4.12 | 0.34 | 0.06 | 0.34 | 0.05 | 0.59 | 0.10 | 0.55 | 0.10 | 0.32 | 0.07 | 0.33 | 0.08 |
| APP (n = 346; n-Out = 14) | AW (n = 349; n-Out = 11) | pSL (n = 344; n-Out = 16) | SST (n = 343; n-Out = 17) | pDST (n = 344; n-Out = 16) | |
|---|---|---|---|---|---|
| Baseline phase values, mean (95% C.I.) | 0.07 (−0.22 ÷ 0.33) | 0.06 (−0.27 ÷ 0.35) | 0.03 (−0.05 ÷ 0.11) | 0.01 (−0.07 ÷ 0.07) | 0.00 (−0.10 ÷ 0.10) |
| Repeated ANOVA model | |||||
| R2 | 0.89 | 0.91 | 0.94 | 0.90 | 0.76 |
| Bonferroni-corrected p-value = 0.01 | |||||
| Model | 0.00 * | 0.00 * | 0.00 * | 0.00 * | 0.00 * |
| Test modality | 0.00 * | 0.00 * | 0.00 * | 0.00 * | 0.00 * |
| η2 | |||||
| Model | 0.89 | 0.91 | 0.94 | 0.90 | 0.76 |
| Test modality | 0.85 | 0.89 | 0.92 | 0.87 | 0.50 |
| Tukey’s post hoc test | |||||
| b-0404 vs. i-0412 | 0.00# | 0.00# | 0.00# | 0.00# | 0.00# |
| b-0404 vs. f-0412 | 0.00 # | 0.00 # | 0.77 | 0.00 # | 0.13 |
| b-0404 vs. i-0404post | 0.00 # | 0.00 # | 0.00 # | 0.02 # | 0.00 # |
| b-0404 vs. f-0404post | 0.25 | 0.45 | 0.33 | 0.71 | 0.99 |
| i-0412 vs. f-0412 | 0.00 # | 0.00 # | 0.00 # | 0.02 # | 0.00 # |
| i-0412 vs. i-0404post | 0.00 # | 0.00 # | 0.00 # | 0.00 # | 0.00 # |
| i-0412 vs. f-0404post | 0.00 # | 0.00 # | 0.00 # | 0.00 # | 0.00 # |
| f-0412 vs. i-0404post | 0.00 # | 0.00 # | 0.00 # | 0.00 # | 0.00 # |
| f-0412 vs. f-0404post | 0.00 # | 0.00 # | 0.02 # | 0.00 # | 0.35 |
| i-0404post vs. f-0404post | 0.03 # | 0.00 # | 0.00 # | 0.31 | 0.00 # |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Scarano, S.; Tesio, L.; Rota, V.; Cerina, V.; Catino, L.; Malloggi, C. Dynamic Asymmetries Do Not Match Spatiotemporal Step Asymmetries during Split-Belt Walking. Symmetry 2021, 13, 1089. https://doi.org/10.3390/sym13061089
Scarano S, Tesio L, Rota V, Cerina V, Catino L, Malloggi C. Dynamic Asymmetries Do Not Match Spatiotemporal Step Asymmetries during Split-Belt Walking. Symmetry. 2021; 13(6):1089. https://doi.org/10.3390/sym13061089
Chicago/Turabian StyleScarano, Stefano, Luigi Tesio, Viviana Rota, Valeria Cerina, Luigi Catino, and Chiara Malloggi. 2021. "Dynamic Asymmetries Do Not Match Spatiotemporal Step Asymmetries during Split-Belt Walking" Symmetry 13, no. 6: 1089. https://doi.org/10.3390/sym13061089
APA StyleScarano, S., Tesio, L., Rota, V., Cerina, V., Catino, L., & Malloggi, C. (2021). Dynamic Asymmetries Do Not Match Spatiotemporal Step Asymmetries during Split-Belt Walking. Symmetry, 13(6), 1089. https://doi.org/10.3390/sym13061089






