A Review on Some Linear Positive Operators Defined on Triangles
Abstract
:1. Introduction
2. Bernstein Type Operator
2.1. Bernstein Operator on Triangle with All Straight Sides
Product and Boolean Sum Operators
- (i)
- (ii)
- (iii)
2.2. Bernstein Operator on Triangle with One Curved Side
- (i)
- on
- (ii)
- onand
- (iii)
- (iv)
Product and Boolean Sum Operators
- (i)
- onand
- (ii)
- on
3. Cheney–Sharma Operator of the Second Kind
3.1. Cheney–Sharma Operator on Triangle with All Straight Sides
3.2. Cheney–Sharma Operator on Triangle with One Curved Side
- (i)
- on
- (ii)
- on
- (i)
- (ii)
- where
Product and Boolean Sum Operators
- (i)
- (ii)
4. Cheney–Sharma Operator of the First Kind
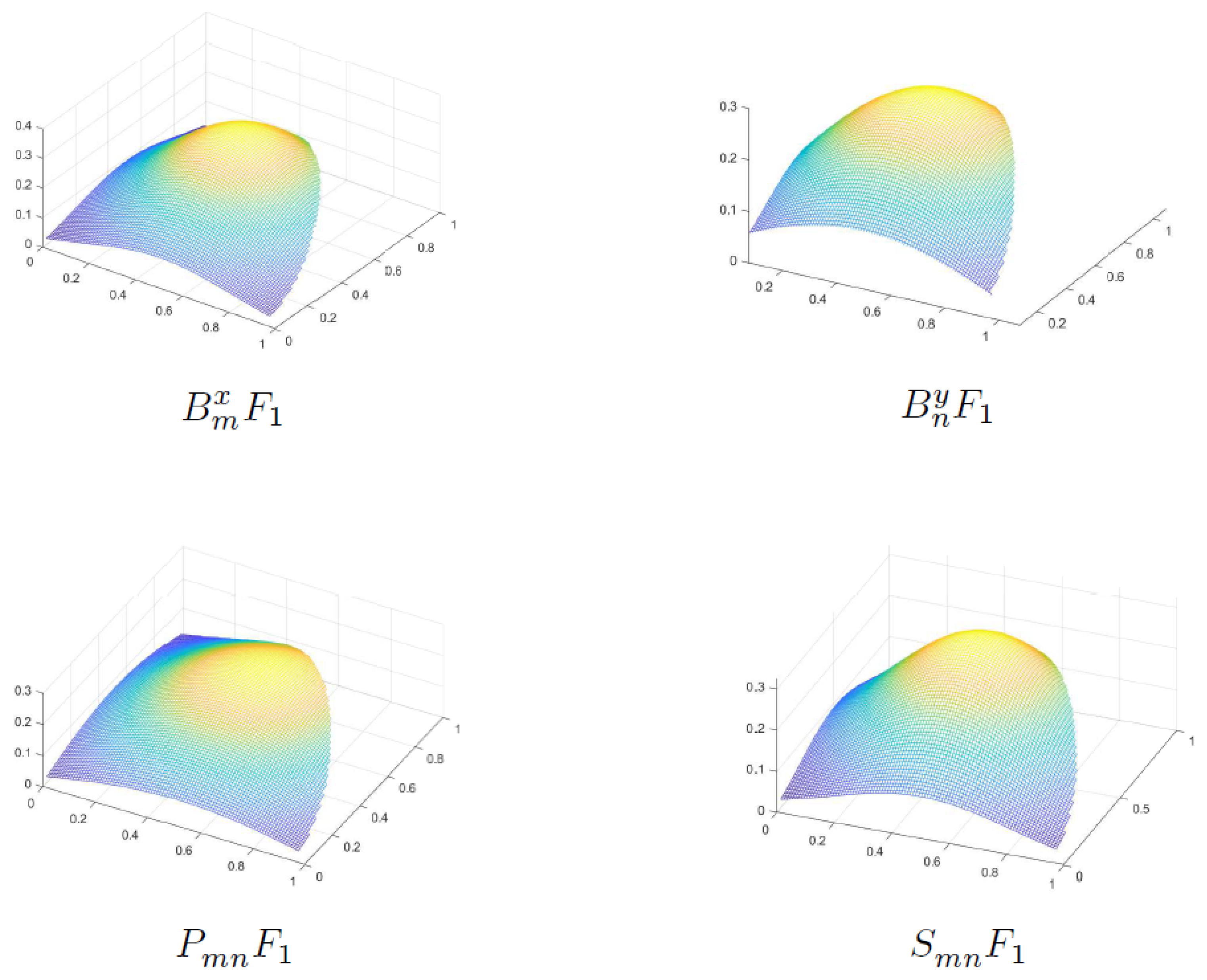
5. Numerical Examples
6. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Barnhill, R.E.; Birkhoff, G.; Gordon, W.J. Smooth interpolation in triangles. J. Approx. Theory 1973, 8, 114–128. [Google Scholar] [CrossRef]
- Barnhill, R.E.; Gregory, J.A. Polynomial interpolation to boundary data on triangles. Math. Comp. 1975, 29, 726–735. [Google Scholar] [CrossRef]
- Blaga, P.; Coman, G. Bernstein-type operators on triangle. Rev. Anal. Numer. Theor. Approx. 2009, 37, 9–21. [Google Scholar]
- Böhmer, K.; Coman, G. Blending interpolation schemes on triangle with error bounds. Lect. Notes Math. 1977, 571, 14–37. [Google Scholar]
- Cătinaş, T.; Coman, G. Some interpolation operators on a simplex domain. Stud. Univ. Babeş–Bolyai Math. 2007, 52, 25–34. [Google Scholar]
- Coman, G.; Blaga, P. Interpolation operators with applications. Sci. Math. Jpn. 2008, 68, 383–416. [Google Scholar]
- Coman, G.; Blaga, P. Interpolation operators with applications. Sci. Math. Jpn. 2009, 69, 111–152. [Google Scholar]
- Costabile, F.A.; Dell’Accio, F. Expansions over a simplex of real functions by means of Bernoulli polynomials. Numer. Algorithms 2001, 28, 63–86. [Google Scholar] [CrossRef]
- Costabile, F.A.; Dell’Accio, F. Lidstone approximation on the triangle. Appl. Numer. Math. 2005, 52, 339–361. [Google Scholar] [CrossRef]
- Nielson, G.M.; Thomas, D.H.; Wixom, J.A. Interpolation in triangles. Bull. Austral. Math. Soc. 1979, 20, 115–130. [Google Scholar] [CrossRef]
- Barnhill, R.E.; Gregory, J.A. Compatible smooth interpolation in triangles. J. Approx. Theory 1975, 15, 214–225. [Google Scholar] [CrossRef]
- Bernardi, C. Optimal finite-element interpolation on curved domains. SIAM J. Numer. Anal. 1989, 26, 1212–1240. [Google Scholar] [CrossRef]
- Blaga, P.; Cătinaş, T.; Coman, G. Bernstein-type operators on a square with one and two curved sides. Stud. Univ. Babeş-Bolyai Math. 2010, 55, 51–67. [Google Scholar]
- Blaga, P.; Cătinaş, T.; Coman, G. Bernstein-type operators on triangle with all curved sides. Appl. Math. Comput. 2011, 218, 3072–3082. [Google Scholar] [CrossRef]
- Blaga, P.; Cătinaş, T.; Coman, G. Bernstein-type operators on triangle with one curved side. Mediterr. J. Math. 2012, 9, 843–855. [Google Scholar] [CrossRef]
- Cătinaş, T. Some classes of surfaces generated by Nielson and Marshall type operators on the triangle with one curved side. Stud. Univ. Babes-Bolyai Math. 2016, 61, 305–314. [Google Scholar]
- Cătinaş, T. Extension of some particular interpolation operators to a triangle with one curved side. Appl. Math. Comput. 2017, 315, 286–297. [Google Scholar] [CrossRef]
- Cătinaş, T. Extension of Some Cheney-Sharma Type Operators to a Triangle With One Curved Side. Miskolc Math. 2020, 21, 101–111. [Google Scholar] [CrossRef]
- Cătinaş, T. Cheney-Sharma Operator on Triangle with Straight Sides. Babeş-Bolyai University, Cluj-Napoca, Romania. 2022; Unpublished work. [Google Scholar]
- Cătinaş, T.; Blaga, P.; Coman, G. Surfaces generation by blending interpolation on a triangle with one curved side. Results Math. 2013, 64, 343–355. [Google Scholar] [CrossRef]
- Coman, G.; Cătinaş, T. Interpolation operators on a tetrahedron with three curved sides. Calcolo 2010, 47, 113–128. [Google Scholar] [CrossRef]
- Coman, G.; Cătinaş, T. Interpolation operators on a triangle with one curved side. BIT Numer. Math. 2010, 50, 243–267. [Google Scholar] [CrossRef]
- Marshall, J.A.; Mitchell, A.R. An exact boundary tehnique for improved accuracy in the finite element method. J. Inst. Maths. Applics. 1973, 12, 355–362. [Google Scholar] [CrossRef]
- Mitchell, A.R.; McLeod, R. Curved elements in the finite element method. Conf. Numer. Sol. Diff. Eq. Lect. NotesIn Math. 1974, 363, 89–104. [Google Scholar]
- Barnhill, R.E. Blending function interpolation: A survey and some new results. In Numerishe Methoden der Approximationstheorie; Collatz, L., Ed.; Birkhauser-Verlag: Basel, Switzerland, 1976; Volume 30, pp. 43–89. [Google Scholar]
- Barnhill, R.E. Representation and approximation of surfaces. In Mathematical Software III; Rice, J.R., Ed.; Academic Press: New York, NY, USA, 1977; pp. 68–119. [Google Scholar]
- Barnhill, R.E.; Gregory, J.A. Sard kernels theorems on triangular domains with applications to finite element error bounds. Numer. Math. 1976, 25, 215–229. [Google Scholar] [CrossRef]
- Özger, F.; Aljimi, E.; Temizer, M. Rate of Weighted Statistical Convergence for Generalized Blending-Type Bernstein-Kantorovich Operators. Mathematics 2022, 10, 2027. [Google Scholar] [CrossRef]
- Ciarlet, P.G. The Finite Element Method for Elliptic Problems; SIAM: Philadelphia, PA, USA, 2002. [Google Scholar]
- Gordon, W.J.; Hall, C. Transfinite element methods: Blending-function interpolation over arbitrary curved element domains. Numer. Math. 1973, 21, 109–129. [Google Scholar] [CrossRef]
- Gordon, W.J.; Wixom, J.A. Pseudo-harmonic interpolation on convex domains. SIAM J. Numer. Anal. 1974, 11, 909–933. [Google Scholar] [CrossRef]
- Marshall, J.A.; Mitchell, A.R. Blending interpolants in the finite element method. Inter. J. Numer. Meth. Eng. 1978, 12, 77–83. [Google Scholar] [CrossRef]
- Dell’Accio, F.; Di Tommaso, F.; Nouisser, O.; Zerroudi, B. Increasing the approximation order of the triangular Shepard method. Appl. Numer. Math. 2018, 126, 78–91. [Google Scholar] [CrossRef]
- Dell’Accio, F.; Di Tommaso, F.; Nouisser, O.; Zerroudi, B. Fast and accurate scattered Hermite interpolation by triangular Shepard operators. J. Comput. Appl. Math. 2021, 382, 113092. [Google Scholar] [CrossRef]
- Di Tommaso, F.; Zerroudi, B. On Some Numerical Integration Formulas on the d-Dimensional Simplex. Mediterr. J. Math. 2020, 17, 142. [Google Scholar] [CrossRef]
- Cheney, E.W.; Sharma, A. On a generalization of Bernstein polynomials. Riv. Mat. Univ. Parma 1964, 5, 77–84. [Google Scholar]
- Stancu, D.D.; Cişmaşiu, C. On an approximating linear positive operator of Cheney-Sharma. Rev. Anal. Numer. Theor. Approx. 1997, 26, 221–227. [Google Scholar]
- Agratini, O. Approximation by Linear Operators; Cluj University Press: Cluj, Romania, 2000. [Google Scholar]
- Sard, A. Linear Approximation; American Mathematical Society: Providence, RI, USA, 1963. [Google Scholar]
- Renka, R.J.; Cline, A.K. A triangle-based C1 interpolation method. Rocky Mt. J. Math. 1984, 14, 223–237. [Google Scholar] [CrossRef]



| Max Error | ||
|---|---|---|
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cătinaş, T. A Review on Some Linear Positive Operators Defined on Triangles. Symmetry 2022, 14, 1880. https://doi.org/10.3390/sym14091880
Cătinaş T. A Review on Some Linear Positive Operators Defined on Triangles. Symmetry. 2022; 14(9):1880. https://doi.org/10.3390/sym14091880
Chicago/Turabian StyleCătinaş, Teodora. 2022. "A Review on Some Linear Positive Operators Defined on Triangles" Symmetry 14, no. 9: 1880. https://doi.org/10.3390/sym14091880
APA StyleCătinaş, T. (2022). A Review on Some Linear Positive Operators Defined on Triangles. Symmetry, 14(9), 1880. https://doi.org/10.3390/sym14091880







