The Formulae and Symmetry Property of Bernstein Type Polynomials Related to Special Numbers and Functions
Abstract
1. Introduction
2. Special Polynomials in Terms of Bernstein Polynomials
3. Recurrence Relation and Derivative Formulae for and
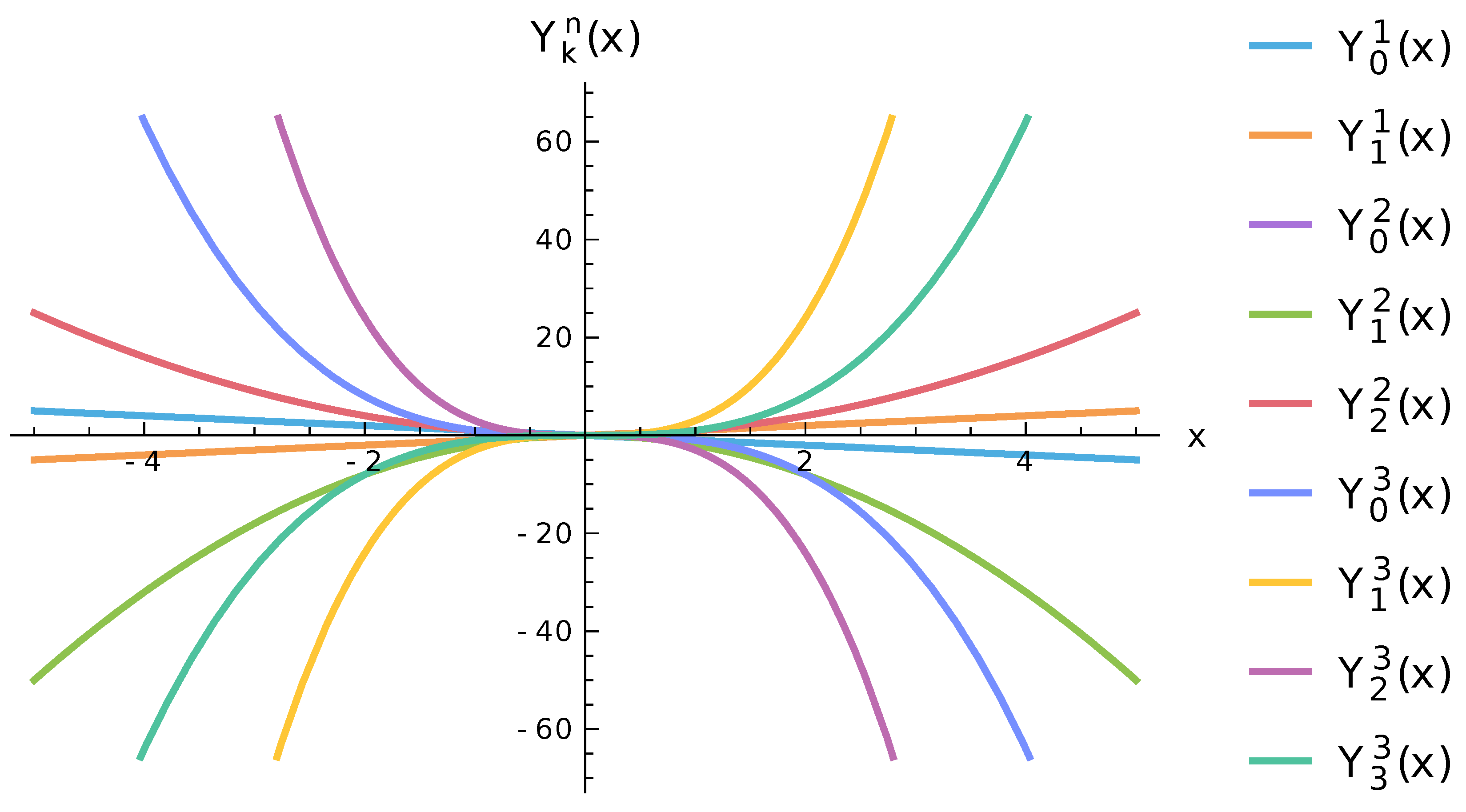
Relations Involving the Symmetry Property among , , and
4. Combinatorial Finite Sums Formulae
5. Relations among the Integral of the Polynomials and Other Special Numbers
6. Bezier Type Curves
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Comtet, L. Advanced Combinatorics; D. Reidel Publication Company: Dordrecht, The Netherlands; Boston, MA, USA, 1974. [Google Scholar]
- Srivastava, H.M. Zeta and q-Zeta Functions and Associated Series and Integrals; Elsevier: Amsterdam, The Netherlands, 2012. [Google Scholar]
- Simsek, Y. Explicit formulas for p-adic Integral: Approach to p-adic distributions and some families of special numbers and polynomials. Montes Taurus J. Pure Appl. Math. 2019, 1, 1–76. [Google Scholar]
- Milovanović, M.; Oarcea, A.; Nikolić, S.; Djordjević, A.; Spasić, M. An Approach to Networking a New Type of Artificial Orthogonal Glands within Orthogonal Endocrine Neural Networks. Appl. Sci. 2022, 12, 5372. [Google Scholar] [CrossRef]
- Rainville, E.D. Special Functions; The Macmillan Company: New York, NY, USA, 1960. [Google Scholar]
- Acikgoz, M.; Araci, S. On the generating function for Bernstein polynomials. Am. Inst. Phys. Conf. Proc. 2010, CP1281, 1141–1144. [Google Scholar]
- Bayad, A.; Chikhi, J. Reduction and duality of the generalized Hurwitz-Lerch zetas. Fixed Point Theory Appl. 2013, 82, 82. [Google Scholar] [CrossRef]
- Bernstein, S.N. Demonstration of a theorem of Weierstrass based on the calculus of probabilities. Comm. Kharkov Math. Soc. 1912, 13, 1–2. [Google Scholar]
- Bustamante, J. Bernstein Operators and Their Properties; Birkhauser Springer International Publishing: Berlin/Heidelberg, Germany, 2017. [Google Scholar]
- Kim, T.; Choi, J.; Kim, Y.H.; Ryoo, C.S. On the Fermionic p-adic integral representation of Bernstein polynomials associated with Euler numbers and polynomials. J. Inequal. Appl. 2010, 2010, 864247. [Google Scholar] [CrossRef][Green Version]
- Kucukoglu, I.; Simsek, B.; Simsek, Y. Multidimensional Bernstein polynomials and Bezier curves: Analysis of machine learning algorithm for facial expression recognition based on curvature. Appl. Math. Comput. 2019, 344–345, 150–162. [Google Scholar] [CrossRef]
- Lorentz, G.G. Bernstein Polynomials; Chelsea Publishing Company: New York, NY, USA, 1986. [Google Scholar]
- Simsek, Y. Functional equations from generating functions: A novel approach to deriving identities for the Bernstein basis functions. Fixed Point Theory Appl. 2013, 2013, 80. [Google Scholar] [CrossRef]
- Simsek, Y. Generating functions for the Bernstein type polynomials: A new approach to deriving identities and applications for the polynomials. Hacet. J. Math. Stat. 2014, 43, 1–14. [Google Scholar]
- Simsek, Y. Construction method for generating functions of special numbers and polynomials arising from analysis of new operators. Math. Methods Appl. Sci. 2018, 41, 6934–6954. [Google Scholar] [CrossRef]
- Fermo, L.; Mezzanotte, D.; Occorsio, D. On the numerical solution of Volterra integral equations on equispaced nodes. Electron. Trans. Numer. Anal. 2023, 59, 9–23. [Google Scholar] [CrossRef]
- Micchelli, C. The saturation class and iterates of the Bernstein polynomials. J. Approx. Theory 1973, 8, 1–18. [Google Scholar] [CrossRef]
- Occorsio, D.; Russo, M.G.; Themistoclakis, W. Some numerical applications of generalized Bernstein operators. Constr. Math. Anal. 2021, 4, 186–214. [Google Scholar] [CrossRef]
- Simsek, B. Formulas derived from moment generating functions and Bernstein polynomials. Appl. Anal. Discret. Math. 2019, 13, 839–848. [Google Scholar] [CrossRef]
- Simsek, B. A note on characteristic function for Bernstein polynomials involving special numbers and polynomials. Filomat 2020, 34, 543–549. [Google Scholar] [CrossRef]
- Simsek, B.; Simsek, B. The computation of expected values and moments of special polynomials via characteristic and generating functions. AIP Conf. Proc. 2017, 1863, 543–549. [Google Scholar]
- Simsek, Y. Combinatorial sums and binomial identities associated with the Beta-type polynomials. Hacet. J. Math. Stat. 2018, 47, 1144–1155. [Google Scholar]
- Venturino, E.; Anit¸a, S.; Mezzanotte, D.; Occorsio, D. A high order numerical scheme for a nonlinear nonlocal reaction–diffusion model arising in population theory. J. Comput. Appl. Math. 2024, 451, 116082. [Google Scholar] [CrossRef]
- Berry, M.V.; Dennis, M.R. Natural superoscillations in monochromatic waves in D dimension. J. Phys. A 2009, 42, 022003. [Google Scholar] [CrossRef]
- Colombo, F.; Krausshar, R.S.; Sabadini, I.; Simsek, Y. On the generating functions and special functions associated with superoscillations. Discrete Appl. Math. 2023, 340, 215–227. [Google Scholar] [CrossRef]
- Kim, D.S.; Kim, T. Daehee Numbers and Polynomials. Appl. Math. Sci. 2013, 1167, 5969–5976. [Google Scholar] [CrossRef]
- Wolfram Research Inc. Mathematica Online (Wolfram Cloud); Wolfram Research Inc.: Champaign, IL, USA, 2021; Available online: https://www.wolframcloud.com (accessed on 1 July 2024).
- Simsek, Y. Analysis of the Bernstein basis functions: An approach to combinatorial sums involving binomial coefficients and Catalan numbers. Math. Meth. Appl. Sci. 2015, 38, 3007–3021. [Google Scholar] [CrossRef]
- Doha, E.; Bhrawy, A.; Saker, M. On the Derivatives of Bernstein Polynomials: An Application for the Solution of High Even-Order Differential Equations. Bound. Value Probl. 2011, 2011, 829543. [Google Scholar] [CrossRef]
- Riordan, J. Introduction to Combinatorial Analysis; Princeton University Press: Princeton, NJ, USA, 1958. [Google Scholar]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yilmaz Ceylan, A.; Simsek, B. The Formulae and Symmetry Property of Bernstein Type Polynomials Related to Special Numbers and Functions. Symmetry 2024, 16, 1159. https://doi.org/10.3390/sym16091159
Yilmaz Ceylan A, Simsek B. The Formulae and Symmetry Property of Bernstein Type Polynomials Related to Special Numbers and Functions. Symmetry. 2024; 16(9):1159. https://doi.org/10.3390/sym16091159
Chicago/Turabian StyleYilmaz Ceylan, Ayse, and Buket Simsek. 2024. "The Formulae and Symmetry Property of Bernstein Type Polynomials Related to Special Numbers and Functions" Symmetry 16, no. 9: 1159. https://doi.org/10.3390/sym16091159
APA StyleYilmaz Ceylan, A., & Simsek, B. (2024). The Formulae and Symmetry Property of Bernstein Type Polynomials Related to Special Numbers and Functions. Symmetry, 16(9), 1159. https://doi.org/10.3390/sym16091159





