Computational Modeling and Constructal Design Theory Applied to the Geometric Optimization of Thin Steel Plates with Stiffeners Subjected to Uniform Transverse Load
Abstract
:1. Introduction
2. Theory of Plates
Theory of Stiffened Plates
3. Computational Modeling
4. Constructal Design Method
5. Results and Discussion
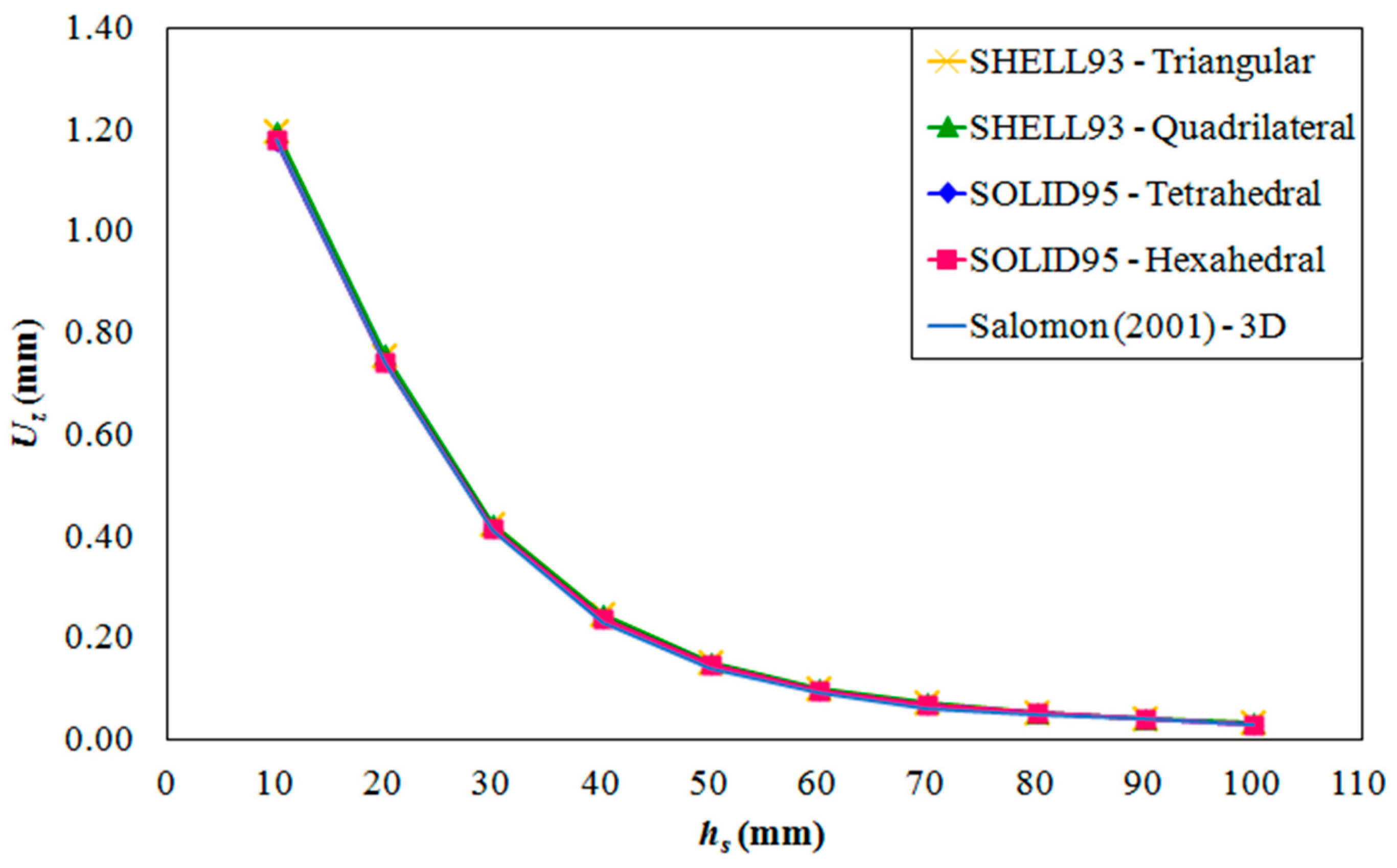
5.1. Mesh Convergence Test and Verification of Computational Models
5.1.1. Rectangular Plate without Stiffeners (Reference Plate)
5.1.2. Square Plate with a Central Stiffener
5.1.3. Square Plate with Orthogonal Stiffeners
5.2. Case Study
6. Further Considerations
- Despite keeping the same steel volume, same dimensions (except thickness), same load and same support conditions, in a general way, all proposed stiffened plates presented lower central deflection when compared with non-stiffened reference plate. Those apparently obvious results demonstrate how the proposed method is in the right direction toward the correct prediction of physical effects. Furthermore, they show how, for a fixed amount of weight, stiffened plates are more efficient. Adequate stiffening is therefore necessary. Welding techniques are also required.
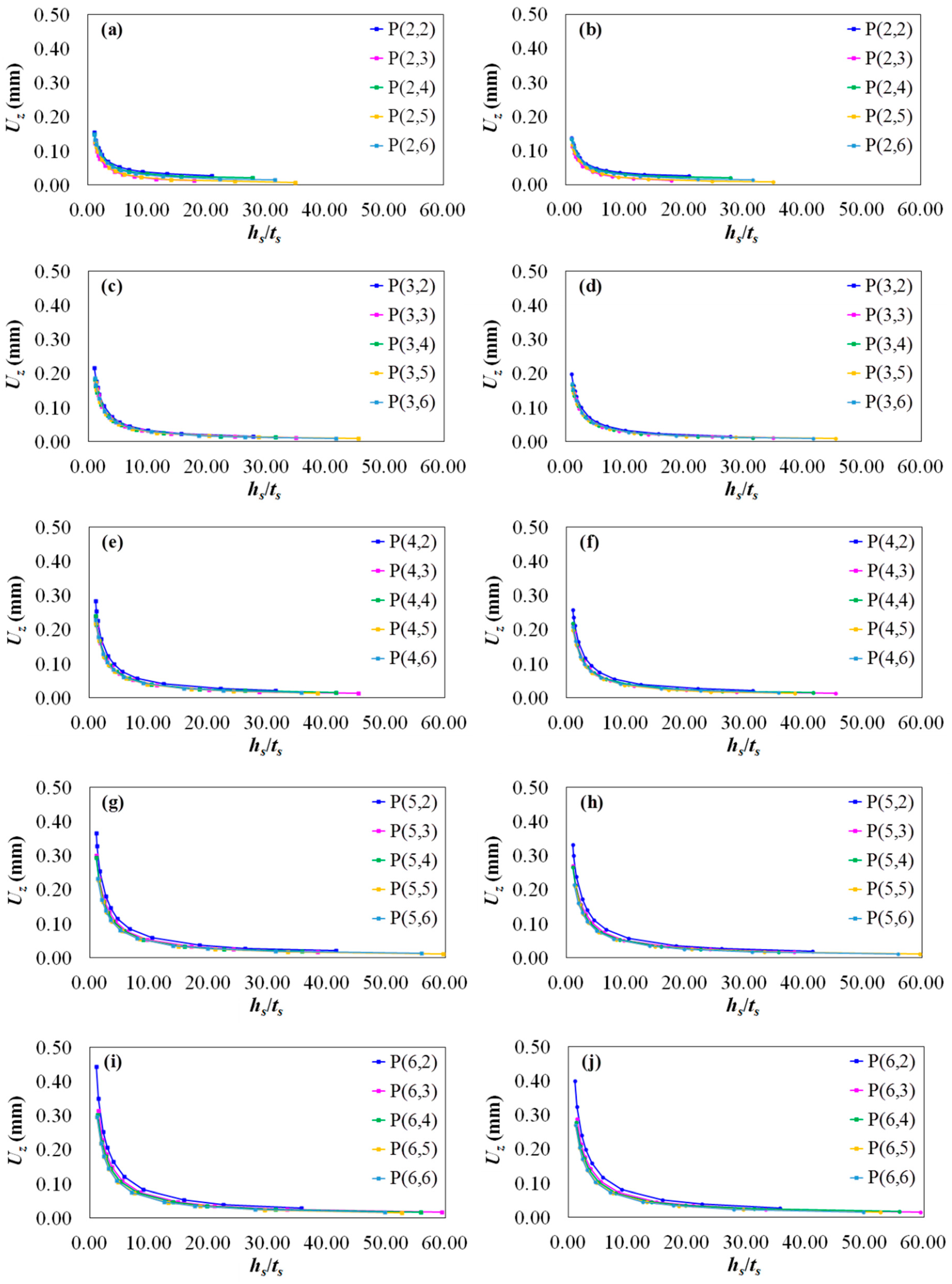
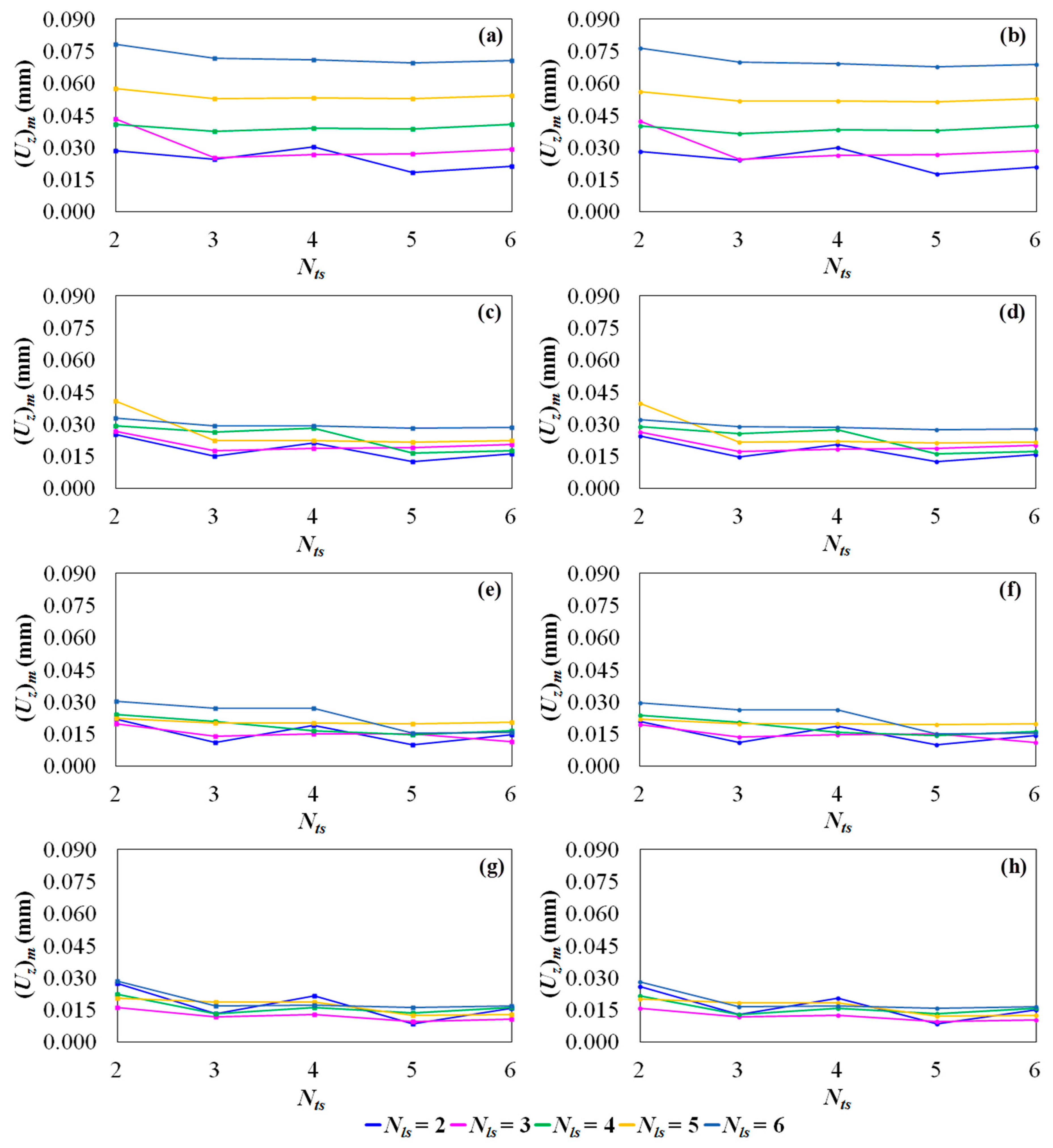
- The number of transverse stiffeners (Nts), number of longitudinal stiffeners (Nls), and ratio between height and thickness of stiffeners (hs/ts) have a deep influence on plates’ stiffness and hence in its deflections. These results also permit us to give additional trust to the method, confirming an obvious physical reality regarding the fact that more stiffeners influence the response of the plate.
- For stiffened plates fabricated with the same amount of steel volume, the increase in the number of stiffeners does not necessarily imply a reduction of its central deflection, highlighting the importance of performing studies involving geometric evaluation in order to efficiently use the material when projecting and constructing these kind of structures.
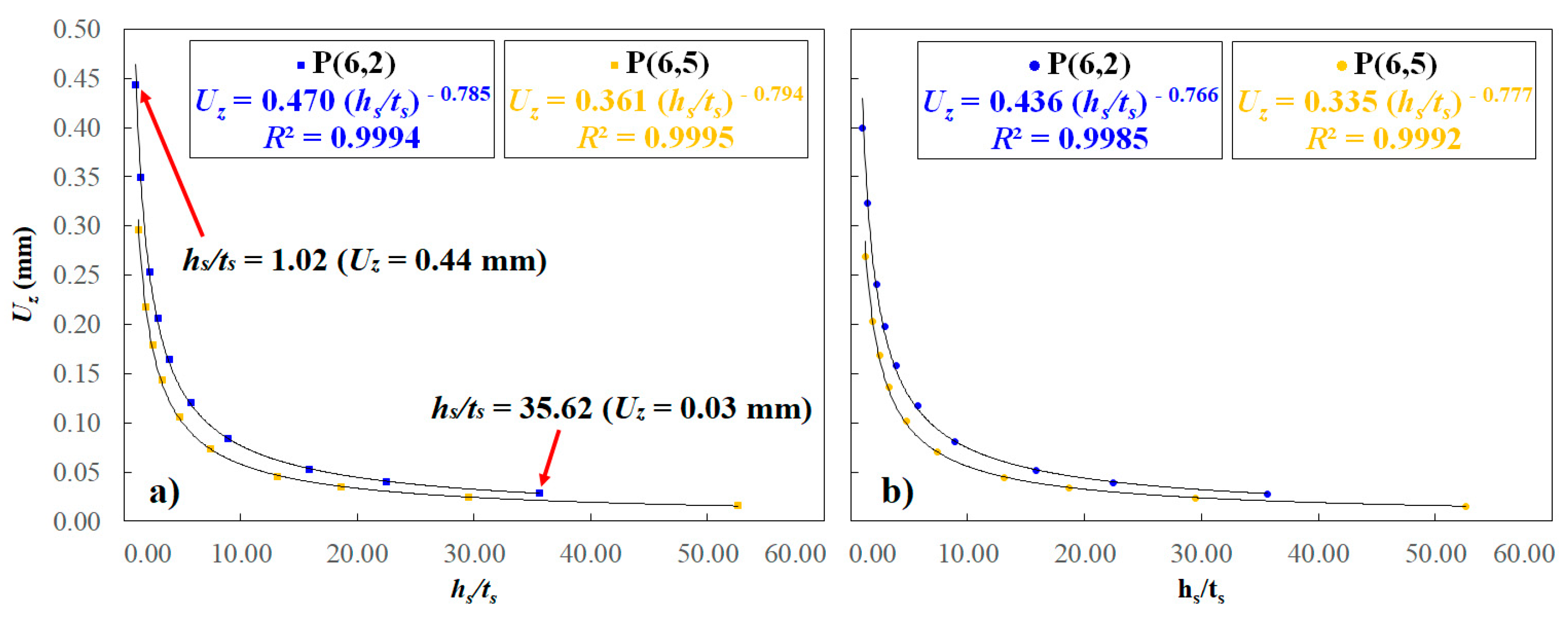
- In a general way, the central deflection values for the stiffened plates tend to stabilize as the ratio hs/ts increases. This asymptotic trend indicates that for values of hs/ts ≥ 20 there is no significant reduction of central transverse displacement. It is an important finding because over-incrementing hs/ts can be harmful to the structure as it increases the reinforcements’ slenderness and, consequently, intensifies the mechanical element’s propensity to instability problems (local buckling).
- For each combination of Nls and Nts a power curve was fitted to mathematically describe the relation between the central displacement and the ratio hs/ts. The coefficients of determination R² presented values from 92% up to 99.99%, evidencing the great accuracy of the performed curve fitting. The equations derived from these curves are highly useful in determining the central displacements of the plates for values of hs/ts within the simulated range and even to extrapolate these results for different values of hs/ts.
- The global optimized stiffened plate, i.e., the best performance among all analyzed geometric configurations, was the one with optimized φo = 0.5, four-times optimized (hs/ts)oooo = 35.03, three times optimized (Nts)ooo = 5 and twice optimized (Nls)oo = 2, which presented a four-times minimized deflection of (Uz)mmmm = 0.0086 mm for the simulation performed using SHELL93 elements and (Uz)mmmm = 0.0084 mm for the simulation with SOLID95 elements. This geometric configuration reached a reduction of 98.77% in the transverse central displacement, if compared with the reference plate.
- Concerning the excellent convergence between the obtained results for both numerical models (SHELL93 and SOLID95), one can indicate the employment of shell finite element for stiffened plate simulations, since it has accuracy and needs a somewhat lower amount of processing time.
- Through the application of the Constructal Design Method, recommendations were obtained about the best geometric configurations of stiffened plates with the aim of minimizing the central out-of-plane displacement of these structures. In addition, it was also possible to draw conclusions which can serve as a support for researches related to this topic, about the mechanical behavior of structures composed of plates and stiffeners.
- The present study specifically considered the mechanical behavior related to the transverse displacements of plates. To do so, an ideal structure (with no imperfections) having a linear (geometric and material) behavior was considered. This simple approach was adopted, aiming to show the applicability of the CDM in this kind of engineering problem. Therefore, in future works a stress analysis, as well as a Geometrically and Materially Nonlinear Analysis with Imperfections Included (GMNIA), can be performed. Moreover, other geometric parameters can be varied, other types of stiffeners can be investigated, and other types of metallic alloys can be tested.
7. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A
| P(Nls,Nts) | hs/ts range | SHELL93 | SOLID95 | ||||
|---|---|---|---|---|---|---|---|
| C1 | C2 | R2 | C1 | C2 | R2 | ||
| P(2,2) | 1.05 ≤ hs/ts ≤ 66.07 | 0.663 | −0.519 | 0.9854 | 0.650 | −0.518 | 0.9840 |
| P(2,3) | 1.18 ≤ hs/ts ≤ 56.66 | 0.666 | −0.542 | 0.9831 | 0.653 | −0.541 | 0.9818 |
| P(2,4) | 1.04 ≤ hs/ts ≤ 49.60 | 0.643 | −0.511 | 0.9792 | 0.631 | −0.511 | 0.9777 |
| P(2,5) | 1.23 ≤ hs/ts ≤ 44.11 | 0.672 | −0.524 | 0.9790 | 0.661 | −0.524 | 0.9778 |
| P(2,6) | 1.11 ≤ hs/ts ≤ 39.71 | 0.657 | −0.499 | 0.9750 | 0.647 | −0.498 | 0.9736 |
| P(3,2) | 1.03 ≤ hs/ts ≤ 49.56 | 0.767 | −0.448 | 0.9664 | 0.749 | −0.445 | 0.9630 |
| P(3,3) | 1.23 ≤ hs/ts ≤ 44.09 | 0.769 | −0.471 | 0.9704 | 0.752 | −0.469 | 0.9680 |
| P(3,4) | 1.11 ≤ hs/ts ≤ 39.71 | 0.736 | −0.454 | 0.9671 | 0.721 | −0.452 | 0.9646 |
| P(3,5) | 1.01 ≤ hs/ts ≤ 36.12 | 0.710 | −0.440 | 0.9631 | 0.695 | −0.438 | 0.9602 |
| P(3,6) | 1.35 ≤ hs/ts ≤ 33.12 | 0.749 | −0.457 | 0.9703 | 0.736 | −0.456 | 0.9684 |
| P(4,2) | 1.10 ≤ hs/ts ≤ 39.66 | 0.839 | −0.384 | 0.9522 | 0.820 | −0.381 | 0.9474 |
| P(4,3) | 1.01 ≤ hs/ts ≤ 36.08 | 0.790 | −0.386 | 0.9512 | 0.770 | −0.383 | 0.9463 |
| P(4,4) | 1.35 ≤ hs/ts ≤ 33.10 | 0.820 | −0.410 | 0.9623 | 0.804 | −0.408 | 0.9595 |
| P(4,5) | 1.25 ≤ hs/ts ≤ 30.58 | 0.789 | −0.401 | 0.9600 | 0.773 | −0.399 | 0.9569 |
| P(4,6) | 1.26 ≤ hs/ts ≤ 28.41 | 0.765 | −0.388 | 0.9578 | 0.750 | −0.386 | 0.9546 |
| P(5,2) | 1.34 ≤ hs/ts ≤ 33.05 | 0.904 | −0.341 | 0.9474 | 0.884 | −0.337 | 0.9417 |
| P(5,3) | 1.24 ≤ hs/ts ≤ 30.54 | 0.857 | −0.347 | 0.9484 | 0.836 | −0.343 | 0.9430 |
| P(5,4) | 1.16 ≤ hs/ts ≤ 28.38 | 0.824 | −0.342 | 0.9480 | 0.805 | −0.339 | 0.9427 |
| P(5,5) | 1.08 ≤ hs/ts ≤ 26.51 | 0.796 | −0.337 | 0.9469 | 0.777 | −0.334 | 0.9413 |
| P(5,6) | 1.02 ≤ hs/ts ≤ 24.87 | 0.774 | −0.329 | 0.9455 | 0.756 | −0.326 | 0.9395 |
| P(6,2) | 1.15 ≤ hs/ts ≤ 28.33 | 0.892 | −0.277 | 0.9314 | 0.869 | −0.272 | 0.9217 |
| P(6,3) | 1.07 ≤ hs/ts ≤ 26.47 | 0.853 | −0.286 | 0.9341 | 0.829 | −0.280 | 0.9248 |
| P(6,4) | 1.01 ≤ hs/ts ≤ 24.84 | 0.825 | −0.285 | 0.9348 | 0.803 | −0.281 | 0.9255 |
| P(6,5) | 1.49 ≤ hs/ts ≤ 23.40 | 0.864 | −0.320 | 0.9558 | 0.845 | −0.317 | 0.9519 |
| P(6,6) | 1.41 ≤ hs/ts ≤ 22.12 | 0.840 | −0.315 | 0.9553 | 0.823 | −0.313 | 0.9516 |
| P(Nls,Nts) | hs/ts Range | SHELL93 | SOLID95 | ||||
|---|---|---|---|---|---|---|---|
| C1 | C2 | R2 | C1 | C2 | R2 | ||
| P(2,2) | 1.35 ≤ hs/ts ≤ 59.28 | 0.400 | −0.660 | 0.9987 | 0.390 | −0.658 | 0.9986 |
| P(2,3) | 1.16 ≤ hs/ts ≤ 50.86 | 0.393 | −0.706 | 0.9985 | 0.379 | −0.701 | 0.9981 |
| P(2,4) | 1.02 ≤ hs/ts ≤ 44.53 | 0.389 | −0.669 | 0.9982 | 0.376 | −0.665 | 0.9977 |
| P(2,5) | 1.42 ≤ hs/ts ≤ 88.21 | 0.410 | −0.701 | 0.9989 | 0.398 | −0.698 | 0.9987 |
| P(2,6) | 1.28 ≤ hs/ts ≤ 79.41 | 0.413 | −0.681 | 0.9986 | 0.402 | −0.678 | 0.9983 |
| P(3,2) | 1.01 ≤ hs/ts ≤ 44.48 | 0.550 | −0.657 | 0.9956 | 0.528 | −0.649 | 0.9942 |
| P(3,3) | 1.41 ≤ hs/ts ≤ 88.18 | 0.534 | −0.682 | 0.9983 | 0.514 | −0.677 | 0.9978 |
| P(3,4) | 1.28 ≤ hs/ts ≤ 79.41 | 0.516 | −0.672 | 0.9977 | 0.498 | −0.667 | 0.9971 |
| P(3,5) | 1.17 ≤ hs/ts ≤ 72.23 | 0.507 | −0.673 | 0.9965 | 0.487 | −0.667 | 0.9956 |
| P(3,6) | 1.07 ≤ hs/ts ≤ 66.24 | 0.501 | −0.663 | 0.9954 | 0.482 | −0.657 | 0.9944 |
| P(4,2) | 1.27 ≤ hs/ts ≤ 79.31 | 0.701 | −0.637 | 0.9955 | 0.675 | −0.632 | 0.9943 |
| P(4,3) | 1.16 ≤ hs/ts ≤ 72.17 | 0.641 | −0.647 | 0.9942 | 0.612 | −0.640 | 0.9929 |
| P(4,4) | 1.07 ≤ hs/ts ≤ 66.21 | 0.606 | −0.635 | 0.9936 | 0.581 | −0.628 | 0.9921 |
| P(4,5) | 1.29 ≤ hs/ts ≤ 61.15 | 0.614 | −0.653 | 0.9942 | 0.589 | −0.648 | 0.9931 |
| P(4,6) | 1.20 ≤ hs/ts ≤ 56.82 | 0.597 | −0.642 | 0.9933 | 0.574 | −0.637 | 0.9920 |
| P(5,2) | 1.06 ≤ hs/ts ≤ 66.10 | 0.791 | −0.597 | 0.9882 | 0.753 | −0.588 | 0.9854 |
| P(5,3) | 1.28 ≤ hs/ts ≤ 61.08 | 0.760 | −0.623 | 0.9909 | 0.725 | −0.616 | 0.9891 |
| P(5,4) | 1.20 ≤ hs/ts ≤ 56.77 | 0.715 | −0.618 | 0.9903 | 0.683 | −0.610 | 0.9884 |
| P(5,5) | 1.12 ≤ hs/ts ≤ 53.02 | 0.682 | −0.615 | 0.9889 | 0.649 | −0.607 | 0.9868 |
| P(5,6) | 1.06 ≤ hs/ts ≤ 49.74 | 0.657 | −0.607 | 0.9878 | 0.627 | −0.599 | 0.9855 |
| P(6,2) | 1.18 ≤ hs/ts ≤ 56.66 | 0.903 | −0.572 | 0.9839 | 0.861 | −0.563 | 0.9805 |
| P(6,3) | 1.11 ≤ hs/ts ≤ 52.94 | 0.823 | −0.580 | 0.9839 | 0.780 | −0.570 | 0.9805 |
| P(6,4) | 1.05 ≤ hs/ts ≤ 49.68 | 0.772 | −0.576 | 0.9836 | 0.734 | −0.567 | 0.9804 |
| P(6,5) | 1.32 ≤ hs/ts ≤ 46.80 | 0.785 | −0.601 | 0.9871 | 0.748 | −0.593 | 0.9849 |
| P(6,6) | 1.25 ≤ hs/ts ≤ 44.23 | 0.753 | −0.594 | 0.9863 | 0.719 | −0.587 | 0.9840 |
| P(Nls,Nts) | hs/ts Range | SHELL93 | SOLID95 | ||||
|---|---|---|---|---|---|---|---|
| C1 | C2 | R2 | C1 | C2 | R2 | ||
| P(2,2) | 1.04 ≤ hs/ts ≤ 31.42 | 0.255 | −0.688 | 0.9989 | 0.244 | −0.681 | 0.9991 |
| P(2,3) | 1.04 ≤ hs/ts ≤ 42.75 | 0.245 | −0.753 | 0.9995 | 0.234 | −0.744 | 0.9993 |
| P(2,4) | 1.07 ≤ hs/ts ≤ 37.44 | 0.247 | −0.695 | 0.9989 | 0.236 | −0.687 | 0.9990 |
| P(2,5) | 1.37 ≤ hs/ts ≤ 59.41 | 0.259 | −0.754 | 0.9992 | 0.250 | −0.748 | 0.9992 |
| P(2,6) | 1.23 ≤ hs/ts ≤ 53.49 | 0.264 | −0.719 | 0.9989 | 0.255 | −0.714 | 0.9989 |
| P(3,2) | 1.06 ≤ hs/ts ≤ 37.38 | 0.378 | −0.734 | 0.9992 | 0.360 | −0.724 | 0.9986 |
| P(3,3) | 1.36 ≤ hs/ts ≤ 59.38 | 0.352 | −0.745 | 0.9993 | 0.336 | −0.736 | 0.9992 |
| P(3,4) | 1.23 ≤ hs/ts ≤ 53.49 | 0.344 | −0.738 | 0.9993 | 0.328 | −0.730 | 0.9990 |
| P(3,5) | 1.13 ≤ hs/ts ≤ 48.67 | 0.341 | −0.745 | 0.9991 | 0.325 | −0.735 | 0.9986 |
| P(3,6) | 1.04 ≤ hs/ts ≤ 44.64 | 0.341 | −0.738 | 0.9988 | 0.325 | −0.729 | 0.9982 |
| P(4,2) | 1.22 ≤ hs/ts ≤ 53.39 | 0.503 | −0.718 | 0.9989 | 0.480 | −0.710 | 0.9984 |
| P(4,3) | 1.12 ≤ hs/ts ≤ 48.60 | 0.455 | −0.732 | 0.9986 | 0.430 | −0.721 | 0.9978 |
| P(4,4) | 1.03 ≤ hs/ts ≤ 44.60 | 0.430 | −0.717 | 0.9984 | 0.407 | −0.707 | 0.9976 |
| P(4,5) | 1.49 ≤ hs/ts ≤ 91.73 | 0.428 | −0.732 | 0.9991 | 0.408 | −0.725 | 0.9990 |
| P(4,6) | 1.39 ≤ hs/ts ≤ 85.23 | 0.419 | −0.720 | 0.9991 | 0.401 | −0.714 | 0.9989 |
| P(5,2) | 1.02 ≤ hs/ts ≤ 44.50 | 0.614 | −0.704 | 0.9967 | 0.579 | −0.691 | 0.9950 |
| P(5,3) | 1.47 ≤ hs/ts ≤ 91.62 | 0.569 | −0.723 | 0.9990 | 0.539 | −0.714 | 0.9986 |
| P(5,4) | 1.38 ≤ hs/ts ≤ 85.15 | 0.533 | −0.718 | 0.9989 | 0.506 | −0.709 | 0.9984 |
| P(5,5) | 1.29 ≤ hs/ts ≤ 79.53 | 0.511 | −0.721 | 0.9986 | 0.483 | −0.712 | 0.9980 |
| P(5,6) | 1.22 ≤ hs/ts ≤ 74.61 | 0.496 | −0.717 | 0.9983 | 0.469 | −0.707 | 0.9976 |
| P(6,2) | 1.36 ≤ hs/ts ≤ 84.99 | 0.751 | −0.700 | 0.9979 | 0.712 | −0.691 | 0.9971 |
| P(6,3) | 1.28 ≤ hs/ts ≤ 79.41 | 0.667 | −0.708 | 0.9977 | 0.627 | −0.697 | 0.9967 |
| P(6,4) | 1.21 ≤ hs/ts ≤ 74.52 | 0.618 | −0.702 | 0.9976 | 0.583 | −0.692 | 0.9966 |
| P(6,5) | 1.14 ≤ hs/ts ≤ 70.20 | 0.586 | −0.705 | 0.9970 | 0.550 | −0.693 | 0.9958 |
| P(6,6) | 1.09 ≤ hs/ts ≤ 66.35 | 0.562 | −0.699 | 0.9967 | 0.529 | −0.688 | 0.9954 |
| P(Nls,Nts) | hs/ts Range | SHELL93 | SOLID95 | ||||
|---|---|---|---|---|---|---|---|
| C1 | C2 | R2 | C1 | C2 | R2 | ||
| P(2,2) | 1.07 ≤ hs/ts ≤ 29.55 | 0.184 | −0.662 | 0.9952 | 0.172 | −0.653 | 0.9956 |
| P(2,3) | 1.20 ≤ hs/ts ≤ 35.96 | 0.175 | −0.784 | 0.9994 | 0.165 | −0.772 | 0.9995 |
| P(2,4) | 1.06 ≤ hs/ts ≤ 31.50 | 0.176 | −0.672 | 0.9962 | 0.165 | −0.660 | 0.9966 |
| P(2,5) | 1.09 ≤ hs/ts ≤ 44.40 | 0.183 | −0.781 | 0.9994 | 0.174 | −0.771 | 0.9995 |
| P(2,6) | 1.15 ≤ hs/ts ≤ 39.98 | 0.187 | −0.714 | 0.9979 | 0.178 | −0.706 | 0.9981 |
| P(3,2) | 1.04 ≤ hs/ts ≤ 31.44 | 0.275 | −0.771 | 0.9997 | 0.260 | −0.756 | 0.9995 |
| P(3,3) | 1.09 ≤ hs/ts ≤ 44.37 | 0.250 | −0.775 | 0.9996 | 0.235 | −0.760 | 0.9994 |
| P(3,4) | 1.15 ≤ hs/ts ≤ 39.98 | 0.247 | −0.771 | 0.9995 | 0.233 | −0.760 | 0.9994 |
| P(3,5) | 1.06 ≤ hs/ts ≤ 36.39 | 0.246 | −0.781 | 0.9996 | 0.232 | −0.767 | 0.9993 |
| P(3,6) | 1.38 ≤ hs/ts ≤ 59.52 | 0.248 | −0.770 | 0.9991 | 0.237 | −0.762 | 0.9991 |
| P(4,2) | 1.14 ≤ hs/ts ≤ 39.88 | 0.371 | −0.751 | 0.9995 | 0.351 | −0.739 | 0.9992 |
| P(4,3) | 1.04 ≤ hs/ts ≤ 36.32 | 0.334 | −0.775 | 0.9994 | 0.312 | −0.759 | 0.9989 |
| P(4,4) | 1.38 ≤ hs/ts ≤ 59.47 | 0.314 | −0.742 | 0.9987 | 0.298 | −0.733 | 0.9988 |
| P(4,5) | 1.28 ≤ hs/ts ≤ 54.95 | 0.313 | −0.773 | 0.9995 | 0.295 | −0.762 | 0.9993 |
| P(4,6) | 1.19 ≤ hs/ts ≤ 51.07 | 0.307 | −0.754 | 0.9993 | 0.290 | −0.744 | 0.9992 |
| P(5,2) | 1.35 ≤ hs/ts ≤ 59.33 | 0.482 | −0.762 | 0.9993 | 0.456 | −0.751 | 0.9991 |
| P(5,3) | 1.26 ≤ hs/ts ≤ 54.85 | 0.425 | −0.769 | 0.9994 | 0.398 | −0.755 | 0.9990 |
| P(5,4) | 1.18 ≤ hs/ts ≤ 51.00 | 0.397 | −0.764 | 0.9993 | 0.373 | −0.751 | 0.9989 |
| P(5,5) | 1.11 ≤ hs/ts ≤ 47.65 | 0.381 | −0.767 | 0.9992 | 0.355 | −0.753 | 0.9986 |
| P(5,6) | 1.05 ≤ hs/ts ≤ 44.72 | 0.371 | −0.763 | 0.9990 | 0.347 | −0.749 | 0.9983 |
| P(6,2) | 1.16 ≤ hs/ts ≤ 50.86 | 0.585 | −0.754 | 0.9989 | 0.549 | −0.741 | 0.9981 |
| P(6,3) | 1.10 ≤ hs/ts ≤ 47.55 | 0.512 | −0.761 | 0.9988 | 0.475 | −0.744 | 0.9978 |
| P(6,4) | 1.04 ≤ hs/ts ≤ 44.64 | 0.472 | −0.752 | 0.9987 | 0.439 | −0.737 | 0.9978 |
| P(6,5) | 1.53 ≤ hs/ts ≤ 93.60 | 0.455 | −0.759 | 0.9992 | 0.427 | −0.748 | 0.9991 |
| P(6,6) | 1.45 ≤ hs/ts ≤ 88.46 | 0.437 | −0.751 | 0.9992 | 0.411 | −0.741 | 0.9990 |
| P(Nls,Nts) | hs/ts Range | SHELL93 | SOLID95 | ||||
|---|---|---|---|---|---|---|---|
| C1 | C2 | R2 | C1 | C2 | R2 | ||
| P(2,2) | 1.06 ≤ hs/ts ≤ 20.84 | 0.150 | −0.592 | 0.9921 | 0.134 | −0.578 | 0.9917 |
| P(2,3) | 1.16 ≤ hs/ts ≤ 17.91 | 0.138 | −0.823 | 0.9998 | 0.129 | −0.804 | 0.9999 |
| P(2,4) | 1.02 ≤ hs/ts ≤ 27.79 | 0.140 | −0.608 | 0.9890 | 0.127 | −0.591 | 0.9891 |
| P(2,5) | 1.18 ≤ hs/ts ≤ 35.03 | 0.143 | −0.807 | 0.9994 | 0.135 | −0.793 | 0.9996 |
| P(2,6) | 1.07 ≤ hs/ts ≤ 31.55 | 0.148 | −0.683 | 0.9949 | 0.137 | −0.670 | 0.9955 |
| P(3,2) | 1.01 ≤ hs/ts ≤ 27.72 | 0.218 | −0.800 | 0.9996 | 0.204 | −0.781 | 0.9996 |
| P(3,3) | 1.17 ≤ hs/ts ≤ 35.00 | 0.196 | −0.802 | 0.9995 | 0.182 | −0.783 | 0.9997 |
| P(3,4) | 1.07 ≤ hs/ts ≤ 31.55 | 0.192 | −0.797 | 0.9997 | 0.179 | −0.778 | 0.9997 |
| P(3,5) | 1.13 ≤ hs/ts ≤ 45.48 | 0.191 | −0.797 | 0.9995 | 0.178 | −0.781 | 0.9995 |
| P(3,6) | 1.04 ≤ hs/ts ≤ 41.73 | 0.194 | −0.796 | 0.9996 | 0.181 | −0.780 | 0.9995 |
| P(4,2) | 1.05 ≤ hs/ts ≤ 31.45 | 0.293 | −0.764 | 0.9994 | 0.273 | −0.746 | 0.9995 |
| P(4,3) | 1.12 ≤ hs/ts ≤ 45.40 | 0.261 | −0.796 | 0.9995 | 0.242 | −0.778 | 0.9995 |
| P(4,4) | 1.03 ≤ hs/ts ≤ 41.69 | 0.245 | −0.751 | 0.9988 | 0.227 | −0.735 | 0.9990 |
| P(4,5) | 1.13 ≤ hs/ts ≤ 38.53 | 0.245 | −0.801 | 0.9997 | 0.228 | −0.784 | 0.9995 |
| P(4,6) | 1.05 ≤ hs/ts ≤ 35.82 | 0.240 | −0.766 | 0.9995 | 0.223 | −0.751 | 0.9994 |
| P(5,2) | 1.01 ≤ hs/ts ≤ 41.55 | 0.380 | −0.789 | 0.9996 | 0.351 | −0.770 | 0.9991 |
| P(5,3) | 1.11 ≤ hs/ts ≤ 38.43 | 0.335 | −0.799 | 0.9996 | 0.309 | −0.779 | 0.9992 |
| P(5,4) | 1.04 ≤ hs/ts ≤ 35.75 | 0.313 | −0.793 | 0.9996 | 0.289 | −0.774 | 0.9991 |
| P(5,5) | 1.39 ≤ hs/ts ≤ 59.57 | 0.301 | −0.793 | 0.9993 | 0.280 | −0.779 | 0.9993 |
| P(5,6) | 1.31 ≤ hs/ts ≤ 55.90 | 0.295 | −0.791 | 0.9994 | 0.276 | −0.777 | 0.9993 |
| P(6,2) | 1.02 ≤ hs/ts ≤ 35.62 | 0.470 | −0.785 | 0.9994 | 0.436 | −0.766 | 0.9985 |
| P(6,3) | 1.37 ≤ hs/ts ≤ 59.43 | 0.412 | −0.794 | 0.9994 | 0.383 | −0.778 | 0.9993 |
| P(6,4) | 1.30 ≤ hs/ts ≤ 55.80 | 0.378 | −0.780 | 0.9993 | 0.352 | −0.766 | 0.9992 |
| P(6,5) | 1.23 ≤ hs/ts ≤ 52.58 | 0.361 | −0.794 | 0.9995 | 0.335 | −0.777 | 0.9992 |
| P(6,6) | 1.17 ≤ hs/ts ≤ 49.72 | 0.348 | −0.782 | 0.9995 | 0.322 | −0.767 | 0.9992 |
References
- Timoshenko, S.; Gere, J. Theory of Elastic Stability; McGraw-Hill: New York, NY, USA, 1961. [Google Scholar]
- Yasuhisa, Y.; Yu, T.; Masaki, M.; Tetsuo, O. Design of Ship Hull Structures: A Practical Guide for Engineers; Springer: Berlin, Germany, 2009. [Google Scholar]
- Mandal, N.R. Ship Construction and Welding; Springer: Singapore, 2017. [Google Scholar]
- Tan, J.; Zhan, M.; Liu, S. Guideline for Forming Stiffened Panels by Using the Electromagnetic Forces. Metals 2016, 6, 267. [Google Scholar] [CrossRef] [Green Version]
- Tan, J.; Zhan, M.; Gao, P.; Li, H. Electromagnetic Forming Rules of a Stiffened Panel with Grid Ribs. Metals 2017, 7, 559. [Google Scholar] [CrossRef] [Green Version]
- Rossow, M.P.; Ibrahimkhail, A.K. A Constraint Method Analysis of Stiffened Plates. Comput. Struct. 1978, 8, 51–60. [Google Scholar] [CrossRef]
- Bedair, O. Analysis of stiffened plates under lateral loading using sequential quadratic programming (SQP). Comput. Struct. 1997, 6, 63–80. [Google Scholar] [CrossRef]
- Tanaka, M.; Bercin, A.N. Static bending analysis of stiffened plates using the boundary element method. Eng. Anal. Bound. Elem. 1997, 21, 147–154. [Google Scholar] [CrossRef]
- Sapountzakis, E.; Katsikadelis, J. Analysis of Plates Reinforced with Beams. Comput. Mech. 2000, 26, 66–74. [Google Scholar] [CrossRef]
- Salomon, A. An Evaluation of Finite Element Models of Stiffened Plates. Master’s Thesis, Massachusetts Institute of Technology, Cambridge, MA, USA, 2001. [Google Scholar]
- Hasan, M. Optimum design of stiffened square plates for longitudinal and square ribs. ALKEJ J. 2007, 3, 13–30. [Google Scholar]
- Silva, H.B.S. Análise Numérica da Influência da Excentricidade na Ligação Placa-viga em Pavimentos Usuais de Edifícios. Master’s Thesis, Universidade de São Paulo, São Carlos, Brazil, 10 January 2010. [Google Scholar]
- De Queiroz, J.; Cunha, M.L.; Pavlovic, A.; Rocha, L.A.O.; Dos Santos, E.D.; Troina, G.S.; Isoldi, L.A. Geometric Evaluation of Stiffened Steel Plates Subjected to Transverse Loading for Naval and Offshore Applications. J. Mar. Sci. Eng. 2019, 7. [Google Scholar] [CrossRef] [Green Version]
- Kallassy, A.; Marcelin, J.L. Optimization of stiffened plates by genetic search. Struct. Optim. 1997, 13, 134–141. [Google Scholar] [CrossRef]
- Cunha, M.L.; Estrada, E.D.S.D.; da Silva Troina, S.D.; dos Santos, E.D.; Rocha, L.A.O.; Isoldi, L.A. Verification of a genetic algorithm for the optimization of stiffened plates through the constructal design method. Res. Eng. Struct. Mater. 2019, 5, 437–446. [Google Scholar]
- Putra, G.L.; Kitamura, M.; Takezawa, A. Structural optimization of stiffener layout for stiffened plate using hybrid GA. Int. J. Nav. Arch. Ocean Eng. 2019, 11, 809–818. [Google Scholar] [CrossRef]
- Lee, J.-C.; Shin, S.-C.; Kim, S.-Y. An optimal design of wind turbine and ship structure based on neuro-response surface method. Int. J. Nav. Arch. Ocean Eng. 2015, 7, 750–769. [Google Scholar] [CrossRef] [Green Version]
- Anyfantis, K.N. Evaluating the influence of geometric distortions to the buckling capacity of stiffened panels. Thin Wall. Struct. 2019, 140, 450–465. [Google Scholar] [CrossRef]
- Szilard, R. Theories and Applications of Plate Analysis: Classical Numerical and Engineering Methods; John Wiley & Sons: Hoboken, NJ, USA, 2004. [Google Scholar]
- Salmon, C.G.; Johnson, J.E. Steel Structures: Design and Behavior, Emphasizing Load and Resistance Factor Design; HarperCollins College Publishers: New York, NY, USA, 1996. [Google Scholar]
- Bernuzzi, C.; Cordova, B. Structural Steel Design to Eurocode 3 and AISC Specifications; John Wiley & Sons, Ltd: Oxford, UK, 2016. [Google Scholar]
- Yamaguchi, E. Basic Theory of Plates and Elastic Stability. Structural Engineering Handbook; CRC Press LLC: Boca Raton, FL, USA, 1999. [Google Scholar]
- Timoshenko, S.; Woinowsky-Krieger, S. Theory of Plates and Shells, 2nd ed.; McGraw-Hill: New York, NY, USA, 1959. [Google Scholar]
- Ventsel, E.; Krauthammer, T. Thin Plates and Shells: Theory, Analysis and Applications; CRC Press: New York, NY, USA, 2001. [Google Scholar]
- Ugural, A.C. Plates and Shells: Theory and Analysis; CRC Press: New York, NY, USA, 2018. [Google Scholar]
- Schäfer, M. Computational Engineering—Introduction to Numerical Methods; Springer: Berlin, Germany, 2006. [Google Scholar]
- Burnett, D. Finite Element Analysis—From Concepts to Applications; Addison–Wesley: Boston, MA, USA, 1989. [Google Scholar]
- Gallagher, R. Finite Element Analysis: Fundamentals; Prentice-Hall: Englewood Cliffs, NJ, USA, 1975. [Google Scholar]
- Zienkiewicz, C.; Taylor, R. The Finite Element Method; McGraw-Hill: London, UK, 1989. [Google Scholar]
- Bathe, K.J. Finite Element Procedures; Prentice-Hall: Upper Saddle River, NJ, USA, 1996. [Google Scholar]
- ANSYS Inc. ANSYS User’s Manual: Analysis Systems. 2009. Available online: http://research.me.udel.edu/~lwang/teaching/MEx81/ansys56manual.pdf (accessed on 31 January 2020).
- Bejan, A. Shape and Structure, from Engineering to Nature; Cambridge University Press: Cambridge, MA, USA, 2000. [Google Scholar]
- Bejan, A.; Lorente, S. Design with Constructal Theory; Wiley: Hoboken, NJ, USA, 2008. [Google Scholar]
- Hazarika, S.A.; Deshmukhya, T.; Bhanja, D.; Nath, S. A novel optimum constructal fork-shaped fin array design for simultaneous heat and mass transfer application in a space-constrained situation. Int. J. Therm. Sci. 2020, 150, 106225. [Google Scholar] [CrossRef]
- Ganjehkaviri, A.; Mohd Jaafar, M.N. Multi-objective particle swarm optimization of flat plate solar collector using constructal theory. Energy 2020, 194, 116846. [Google Scholar] [CrossRef]
- Li, B.; Yin, X.; Tang, W.; Zhang, J. Optimization design of grooved evaporator wick structures in vapor chamber heat spreaders. Appl. Therm. Eng. 2020, 166, 114657. [Google Scholar] [CrossRef]
- Lee, J. Maximal Heat Transfer Density in Heat Exchangers of Wet Flue Gas Desulfurization Equipment. Heat Transfer Eng. 2020, 41. [Google Scholar] [CrossRef]
- Ariyo, D.O.; Bello-Ochende, T. Constructal design of subcooled microchannel heat exchangers. Int. J. Heat Mass Trans. 2020, 146, 118835. [Google Scholar] [CrossRef]
- Brum, R.S.; Ramalho, J.V.A.; Rodrigues, M.K.; Rocha, L.A.O.; Isoldi, L.A.; dos Santos, E.D. Design evaluation of Earth-Air Heat Exchangers with multiple ducts. Renew. Energy 2019, 135, 1371–1385. [Google Scholar] [CrossRef]
- Wu, Z.; Feng, H.; Chen, L.; Xie, Z.; Cai, C.; Xia, S. Optimal design of dual-pressure turbine in OTEC system based on constructal theory. Energ. Convers. Manag. 2019, 201, 112179. [Google Scholar] [CrossRef]
- Lugarini, A.; Franco, A.T.; Errera, M.R. Flow distribution uniformity in a comb-like microchannel network. Microfluid. Nanofluid. 2019, 23, 44. [Google Scholar] [CrossRef]
- Cai, C.; Feng, H.; Chen, L.; Wu, Z.; Xie, Z. Constructal design of a shell-and-tube evaporator with ammonia-water working fluid. Int. J. Heat Mass Trans. 2019, 135, 541–547. [Google Scholar] [CrossRef]
- Salimpour, M.R.; Darabi, J.; Mahjoub, S. Design and Optimization of Internal Longitudinal Fins of a Tube Using Constructal Theory. J. Eng. Thermophys. 2019, 28, 239–254. [Google Scholar] [CrossRef]
- Gomes, M.; das, N.; Lorenzini, G.; Rocha, L.A.O.; dos Santos, E.D.; Isoldi, L.A. Constructal Design Applied to the Geometric Evaluation of an Oscillating Water Column Wave Energy Converter Considering Different Real Scale Wave Periods. J. Eng. Thermophys. 2018, 27, 173–190. [Google Scholar] [CrossRef]
- Liu, X.; Feng, H.; Chen, L. Constructal Design of a Converter Steelmaking Procedure Based on Multi-objective Optimization. Arab. J. Sci. Eng. 2018, 43, 5003. [Google Scholar] [CrossRef]
- Hermany, L.; Lorenzini, G.; Klein, R.J.; Zinani, F.F.; dos Santos, E.D.; Isoldi, L.A.; Rocha, L.A.O. Constructal design applied to elliptic tubes in convective heat transfer cross-flow of viscoplastic fluids. Int. J. Heat Mass Trans. 2018, 116, 1054–1063. [Google Scholar] [CrossRef]
- Solé, A.; Falcoz, Q.; Cabeza, L.F.; Neveu, P. Geometry optimization of a heat storage system for concentrated solar power plants (CSP). Renew. Energy 2018, 123, 227–235. [Google Scholar] [CrossRef]
- Martins, J.C.; Goulart, M.M.; Gomes, M.; das, N.; Souza, J.A.; Rocha, L.A.O.; Isoldia, L.A.; dos Santos, E.D. Geometric evaluation of the main operational principle of an overtopping wave energy converter by means of Constructal Design. Renew. Energy 2018, 118, 727–741. [Google Scholar] [CrossRef]
- Craig, S.; Grinham, J. Breathing walls: The design of porous materials for heat exchange and decentralized ventilation. Energy Build. 2017, 149, 246–259. [Google Scholar] [CrossRef]
- Cetkin, E. Constructal Microdevice Manifold Design With Uniform Flow Rate Distribution by Consideration of the Tree-Branching Rule of Leonardo da Vinci and Hess–Murray Rule. J. Heat Transf. 2017, 139, 082401. [Google Scholar] [CrossRef]
- Barros, G.M.; Lorenzini, G.; Isoldi, L.A.; Rocha, L.A.O.; dos Santos, E.D. Influence of mixed convection laminar flows on the geometrical evaluation of a triangular arrangement of circular cylinders. Int. J. Heat Mass Trans. 2017, 114, 1188–1200. [Google Scholar] [CrossRef]
- Feng, H.; Chen, L.; Liu, X.; Xie, Z. Constructal design for an iron and steel production process based on the objectives of steel yield and useful energy. Int. J. Heat Mass Trans. 2017, 111, 1192–1205. [Google Scholar] [CrossRef]
- Vieira, R.S.; Petry, A.P.; Rocha, L.A.O.; Isoldi, L.A.; dos Santos, E.D. Numerical evaluation of a solar chimney geometry for different ground temperatures by means of constructal design. Renew. Energy 2017, 109, 222–234. [Google Scholar] [CrossRef]
- Bejan, A.; Lorente, S.; Lee, J. Unifying constructal theory of tree roots, canopies and forests. J. Theor. Biol. 2008, 254, 529–540. [Google Scholar] [CrossRef]
- Lorente, S.; Lee, J.; Bejan, A. The “flow of stresses” concept: the analogy between mechanical strength and heat convection. Int. J. Heat Mass Trans. 2010, 53, 2963–2968. [Google Scholar] [CrossRef]
- Isoldi, L.A.; Real, M.V.; Correia, A.L.G.; Vaz, J.; Dos Santos, E.D.; Rocha, L.A.O. Flow of Stresses: Constructal Design of Perforated Plates Subjected to Tension or Buckling. In Constructal Law and the Unifying Principle of Design—Understanding Complex Systems, 1st ed.; Rocha, L.A.O., Lorente, S., Bejan, A., Eds.; Springer: New York, NY, USA, 2013; pp. 195–217. [Google Scholar]
- Isoldi, L.A.; Real, M.V.; Vaz, J.; Correia, A.L.G.; Dos Santos, E.D.; Rocha, L.A.O. Numerical Analysis and Geometric Optimization of Perforated Thin Plates Subjected to Tension or Buckling. Mar. Syst. Ocean Tech. 2013, 8, 99–107. [Google Scholar] [CrossRef]
- Rocha, L.A.O.; Isoldi, L.A.; Real, M.V.; Dos Santos, E.D.; Correia, A.L.G.; Biserni, C.; Lorenzini, G. Constructal design applied to the elastic buckling of thin plates with holes. Cent. Eur. J. Eng. 2013, 3, 475–483. [Google Scholar] [CrossRef]
- Helbig, D.; Real, M.V.; Dos Santos, E.D.; Isoldi, L.A.; Rocha, L.A.O. Computational modeling and constructal design method applied to the mechanical behavior improvement of thin perforated steel plates subject to buckling. J. Eng. Therm. 2016, 25, 197–215. [Google Scholar] [CrossRef]
- Lorenzini, G.; Helbig, D.; Da Silva, C.C.C.; Real, M.V.; Dos Santos, E.D.; Isoldi, L.A.; Rocha, L.A.O. Numerical Evaluation of the Effect of Type and Shape of Perforations on the Buckling of Thin Steel Plates by means of the Constructal Design Method. Int. J. Heat Technol. 2016, 34, S9–S20. [Google Scholar] [CrossRef] [Green Version]
- Helbig, D.; Da Silva, C.C.C.; Real, M.V.; Dos Santos, E.D.; Isoldi, L.A.; Rocha, L.A.O. Study About Buckling Phenomenon in Perforated Thin Steel Plates Employing Computational Modeling and Constructal Design Method. Lat. Am. J. Solids Struct. 2016, 13, 1912–1936. [Google Scholar] [CrossRef]
- Helbig, D.; Cunha, M.L.; Da Silva, C.C.C.; Dos Santos, E.D.; Iturrioz, I.; Real, M.V.; Isoldi, L.A.; Rocha, L.A.O. Numerical study of the elasto-plastic buckling in perforated thin steel plates using the constructal design method. Res. Eng. Struct. Mat. 2018, 4, 169–187. [Google Scholar] [CrossRef]
- Da Silva, C.C.C.; Helbig, D.; Cunha, M.L.; Dos Santos, E.D.; Rocha, L.A.O.; Real, M.V.; Isoldi, L.A. Numerical Buckling Analysis of Thin Steel Plates with Centered Hexagonal Perforation Through Constructal Design Method. J. Braz. Soc. Mech. Sci. Eng. 2019, 41, 309. [Google Scholar] [CrossRef]
- Lima, J.P.S.; Rocha, L.A.O.; Santos, E.D.; Real, M.V.; Isoldi, L.A. Constructal design and numerical modeling applied to stiffened steel plates submitted to elasto-plastic buckling. Proc. Roman. Acad. Ser. A 2018, 19, 195–200. [Google Scholar]
- Lima, J.P.S.; Cunha, M.L.; dos Santos, E.D.; Rocha, L.A.O.; Real, M.V.; Isoldi, L.A. Constructal Design for the ultimate buckling stress improvement of stiffened plates submitted to uniaxial compressive load. Eng. Struct. 2020, 203, 109883. [Google Scholar] [CrossRef]
- Cunha, M.L.; Troina, G.S.; Rocha, L.A.O.; Dos Santos, E.D.; Isoldi, L.A. Computational modeling and Constructal Design method applied to the geometric optimization of stiffened steel plates subjected to uniform transverse load. Res. Eng. Struct. Mater. 2018, 4, 139–149. [Google Scholar] [CrossRef]
- Amaral, R.R.; Troina, G.S.; Nogueira, C.M.; Cunha, M.L.; Rocha, L.A.O.; Santos, E.D.; Isoldi, L.A. Computational modeling and constructal design method applied to the geometric evaluation of stiffened thin steel plates considering symmetry boundary condition. Res. Eng. Struct. Mater. 2019, 5, 393–402. [Google Scholar] [CrossRef]
- Pinto, V.T.; Cunha, M.L.; Troina, G.S.; Martins, K.L.; dos Santos, E.D.; Isoldi, L.A.; Rocha, L.A.O. Constructal design applied to geometrical evaluation of rectangular plates with inclined stiffeners subjected to uniform transverse load. Res. Eng. Struct. Mater. 2019, 5, 379–392. [Google Scholar]
- Mardanpour, P.; Izadpanahi, E.; Rastkar, S.; Lorente, S.; Bejan, A. Constructal design of aircraft: flow of stresses and aeroelastic stability. AIAA J. 2019. [Google Scholar] [CrossRef]
- Izadpanahi, E.; Moshtaghzadeh, M.; Radnezhad, H.R.; Mardanpour, P. Constructal approach to design of wing cross-section for better flow of stresses. AIAA J. 2020. [Google Scholar] [CrossRef]
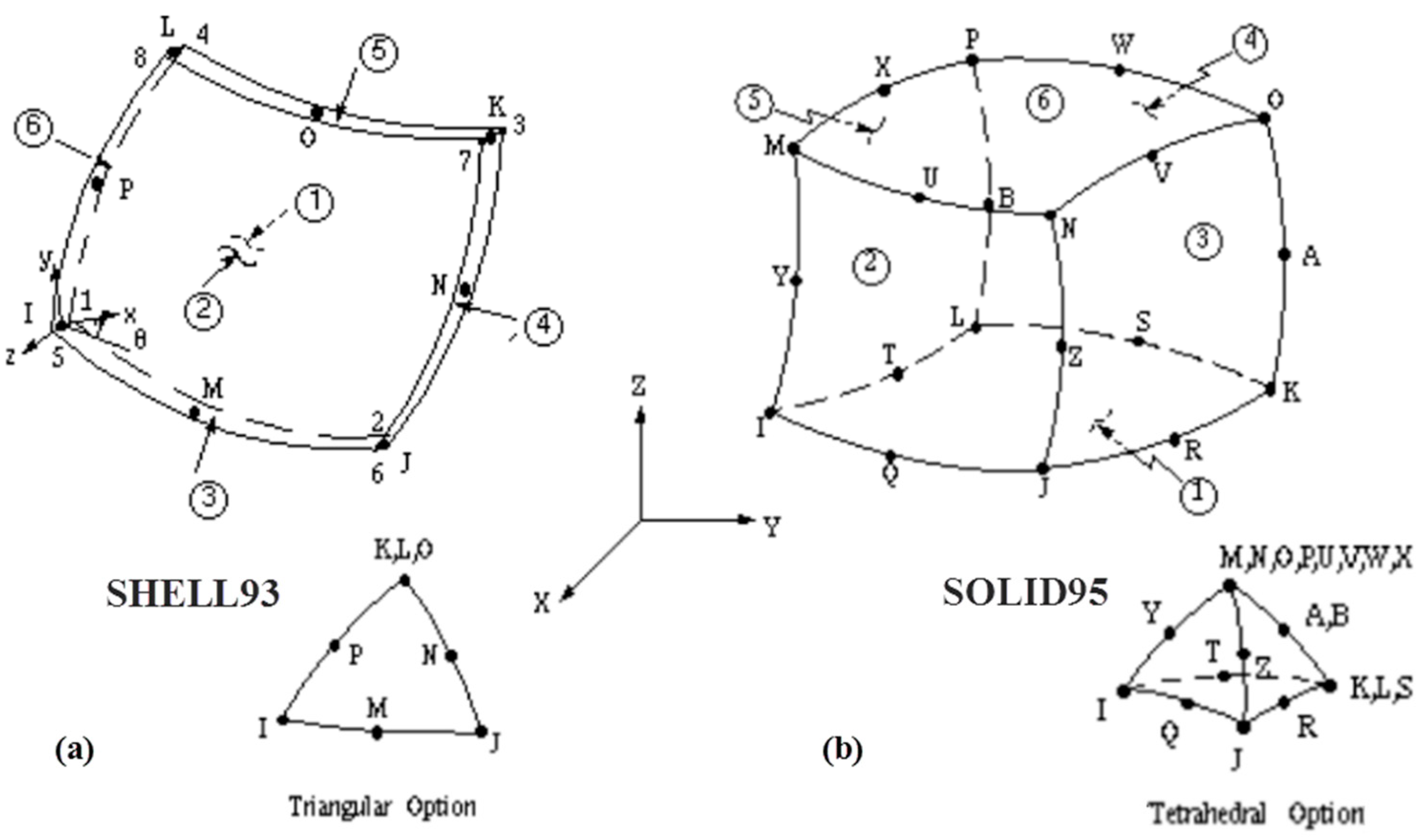

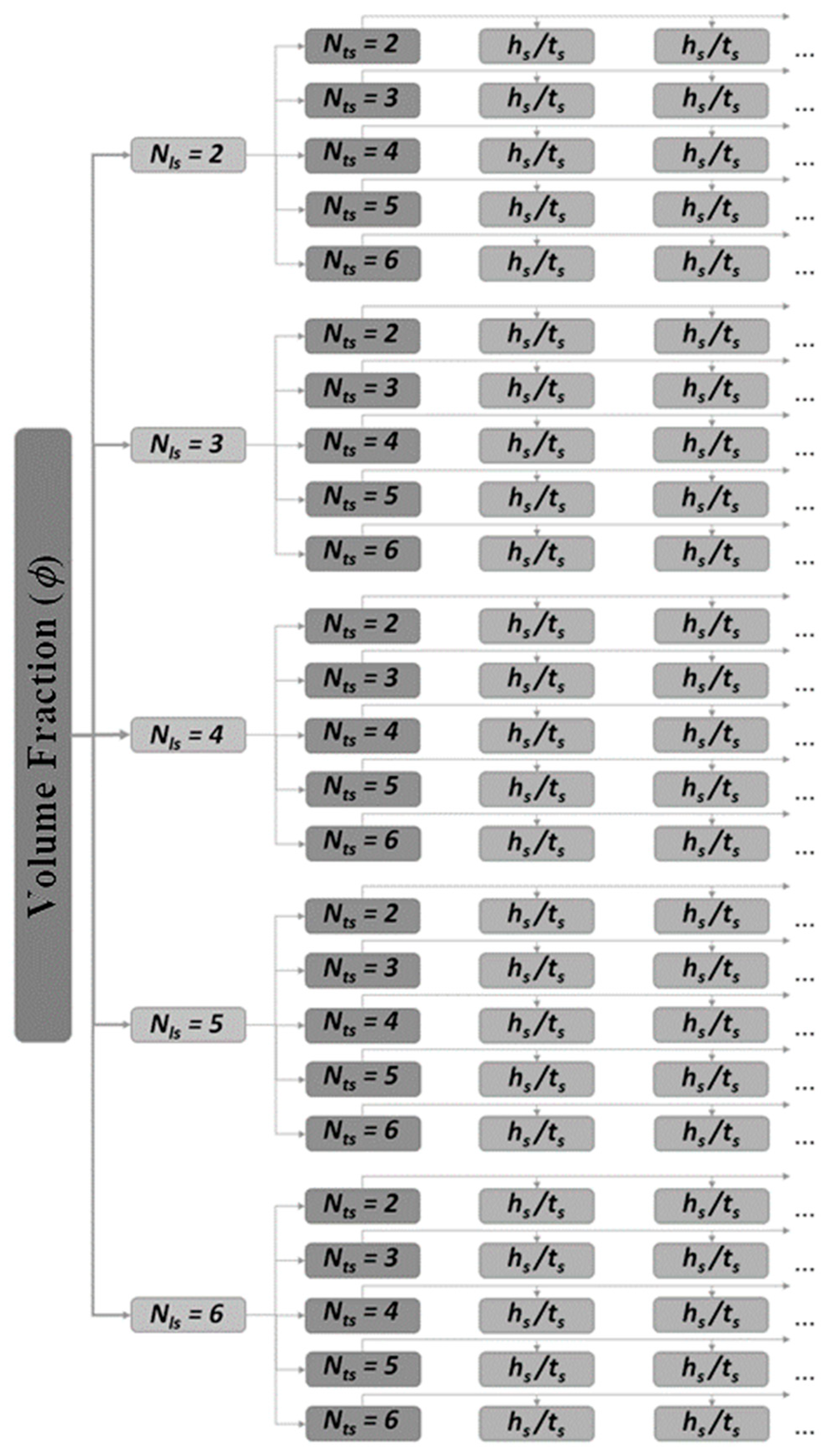


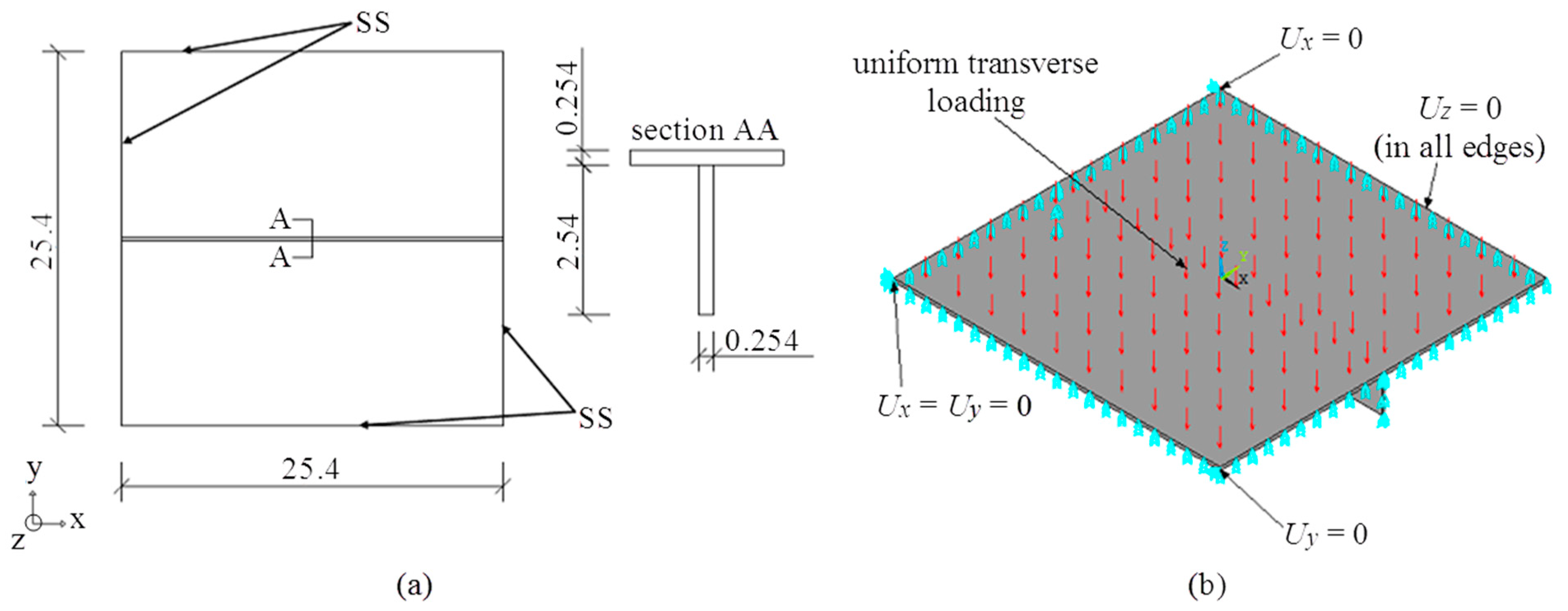

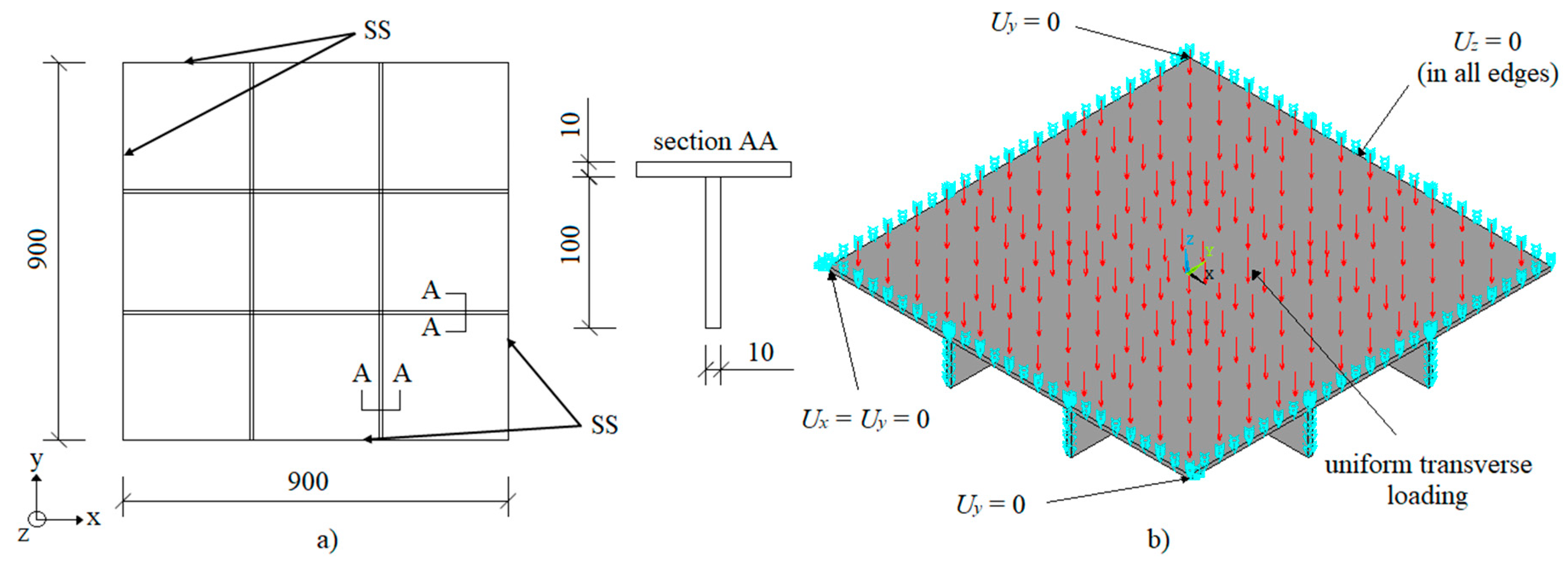




| Reference | Type | Year | Scope | Methodology | Relationship |
|---|---|---|---|---|---|
| [3] | Book | 2017 | MP | Theoretical | Exemplify how stiffened plates can be obtained by welding process |
| [4] | Paper | 2016 | MP | Experimental and Numerical | Exemplify how stiffened plates can be obtained by electromagnetic forming |
| [5] | Paper | 2017 | MP | Experimental and Numerical | Exemplify how stiffened plates can be obtained by electromagnetic forming |
| [6] | Paper | 1978 | MB | Numerical | Verification of the computational models |
| [7] | Paper | 1997 | MB | Numerical | Exemplify an analysis procedure employed for stiffened plates |
| [8] | Paper | 1997 | MB | Numerical | Verification of the computational models |
| [9] | Paper | 2000 | MB | Numerical | Exemplify an analysis procedure employed for stiffened plates |
| [10] | Master’s Thesis | 2001 | MB | Numerical | Verification of the computational models |
| [11] | Paper | 2007 | MB | Numerical | Exemplify an analysis procedure employed for stiffened plates |
| [12] | Master’s Thesis | 2010 | MB | Numerical | Verification of the computational models |
| [13] | Paper | 2019 | GO | Numerical | Preliminary study associating CDM, FEM and ES for stiffened plates |
| [14] | Paper | 1997 | GO | Numerical | Exemplify the geometric optimization of stiffened plates by means GA |
| [15] | Paper | 2019 | GO | Numerical | Exemplify the geometric optimization of stiffened plates by means GA |
| [16] | Paper | 2019 | GO | Numerical | Exemplify the geometric optimization of stiffened plates by means GA |
| [17] | Paper | 2015 | GO | Numerical | Exemplify the geometric optimization of stiffened plates by means RSM |
| [18] | Paper | 2019 | GO | Numerical | Exemplify the geometric optimization of stiffened plates by means RSM |
| Reference | Year | Area | Description |
|---|---|---|---|
| [34] | 2020 | FM and HT | Design and analysis of an array of constructal fork-shaped fins (with two and three branches) adhered to a circular tube and operating under fully wet conditions, aiming the maximization of the net heat transfer rate. |
| [35] | 2020 | HT | Design and thermo-economic assessment of a flat plate solar collector has been studied with the multi-objective of improving its thermal efficiency and total annual cost. |
| [36] | 2020 | FM and HT | Optimization method applied for designing the layout of grooved evaporator wick structures in vapor chamber heat spreaders, reaching a capillary pressure improvement and temperature gradient homogeneity. |
| [37] | 2020 | FM and HT | Overall net heat transfer maximization for a cooler and reheater in the wet flue gas desulfurization equipment of coal-firing thermal power plant was explored, by means the geometric optimization of its flow architectures. |
| [38] | 2020 | FM and HT | Geometric optimization and flow parameters modeling for subcooled flow boiling (two-phase flow) were performed, aiming to minimize the thermal resistance of the microchannel heat exchanger. |
| [39] | 2019 | HT | Optimization of the geometrical configurations to assemble the ducts of an earth-air heat exchanger with the aim of improving its thermal performance. |
| [40] | 2019 | FM | Optimal design of a dual-pressure turbine in an ocean thermal energy conversion system is obtained, being the total power output of the turbine chosen as the optimization objective. |
| [41] | 2019 | FM | New geometries for a comb-like network (single manifold duct ramified to several branches, all subject to a pressure reservoir) were obtained with perform significantly better than those with constant diameter and spacing. |
| [42] | 2019 | FM and HT | Design of a shell-and-tube evaporator with ammonia-water working fluid is evaluated, adopting a complex function (composed by heat transfer rate and total pumping power) as optimization objective. |
| [43] | 2019 | FM and HT | Geometrical optimization of internal longitudinal fins of a tube (extended inward from the pipe perimeter to a prescribed radius) is carried out ensuring maximum heat transfer and thermal efficiency. |
| [44] | 2018 | FM | Study of the geometry influence on the performance of an oscillating water column wave energy converter subject to several real scale waves with different periods, aiming the maximization of its hydrodynamic power. |
| [45] | 2018 | FM and HT | Optimization of a converter steelmaking procedure is performed by a complex function considering molten steel yield and useful energy as performance parameters. |
| [46] | 2018 | FM and HT | Determination of geometries that maximize the heat transfer and minimize pressure drop for viscoplastic fluids in cross flow around elliptical section tubes. |
| [47] | 2018 | FM and HT | Geometry optimization of a phase change material heat storage system is developed with the purpose to find the optimum shape factor for its elemental volume. |
| [48] | 2018 | FM | A geometric evaluation of an overtopping wave energy converter in real scale and submitted to incident regular waves was developed, being the goal to promote the maximization of the device available power. |
| [49] | 2017 | FM and HT | Study demonstrating how to design pores in building materials so that incoming fresh air can be efficiently tempered with low-grade heat while conduction losses are kept to a minimum. |
| [50] | 2017 | FM | As the design of a microdevice manifold should be tapered for uniform flow rate distribution, it is inferred that not only pressure drop but also velocity distribution in the microdevice play an integral role in the flow uniformity. |
| [51] | 2017 | FM and HT | Geometrical evaluation of a triangular arrangement of circular cylinders subjected to convective flows, having the multi-objective of maximizing the Nusselt number and minimizing the drag coefficient. |
| [52] | 2017 | FM and HT | An iron and steel production whole process is considered, being adopted a complex function composed of steel yield, useful energy, and maximum temperature difference as the optimization objective. |
| [53] | 2017 | FM and HT | Evaluation about the influence of geometric parameters of a solar chimney power plant, with the purpose of maximizing its available power. |
| φ | Nls | (Nts)o | ts (mm) | hs (mm) | (hs/ts)oo | (Uz)mm (mm) SHELL93 | (Uz)mm (mm) SOLID95 |
|---|---|---|---|---|---|---|---|
| 0.1 | 2 | 3 | 3.18 | 180.19 | 56.66 | 0.0652 | 0.0639 |
| 0.1 | 3 | 3 | 3.18 | 140.21 | 44.09 | 0.1115 | 0.1094 |
| 0.1 | 4 | 3 | 3.18 | 114.75 | 36.08 | 0.1679 | 0.1649 |
| 0.1 | 5 | 3 | 3.18 | 97.11 | 30.54 | 0.2310 | 0.2269 |
| 0.1 | 6 | 5 | 3.18 | 74.41 | 23.40 | 0.2902 | 0.2852 |
| 0.2 | 2 | 5 | 3.18 | 280.52 | 88.21 | 0.0180 | 0.0177 |
| 0.2 | 3 | 3 | 3.18 | 280.42 | 88.18 | 0.0251 | 0.0245 |
| 0.2 | 4 | 3 | 3.18 | 229.50 | 72.17 | 0.0373 | 0.0365 |
| 0.2 | 5 | 5 | 3.18 | 168.61 | 53.02 | 0.0528 | 0.0515 |
| 0.2 | 6 | 5 | 3.18 | 148.82 | 46.80 | 0.0696 | 0.0679 |
| 0.3 | 2 | 5 | 4.75 | 282.20 | 59.41 | 0.0126 | 0.0123 |
| 0.3 | 3 | 3 | 4.75 | 282.04 | 59.38 | 0.0174 | 0.0171 |
| 0.3 | 4 | 5 | 3.18 | 291.70 | 91.73 | 0.0163 | 0.0159 |
| 0.3 | 5 | 5 | 3.18 | 252.91 | 79.53 | 0.0216 | 0.0211 |
| 0.3 | 6 | 5 | 3.18 | 223.23 | 70.20 | 0.0279 | 0.0272 |
| 0.4 | 2 | 5 | 6.35 | 281.95 | 44.40 | 0.0100 | 0.0098 |
| 0.4 | 3 | 6 | 4.75 | 282.72 | 59.52 | 0.0113 | 0.0111 |
| 0.4 | 4 | 5 | 4.75 | 261.02 | 54.95 | 0.0146 | 0.0143 |
| 0.4 | 5 | 5 | 4.75 | 226.35 | 47.65 | 0.0197 | 0.0192 |
| 0.4 | 6 | 5 | 3.18 | 297.64 | 93.60 | 0.0153 | 0.0149 |
| 0.5 | 2 | 5 | 8.00 | 280.27 | 35.03 | 0.0086 | 0.0084 |
| 0.5 | 3 | 5 | 6.35 | 288.83 | 45.48 | 0.0096 | 0.0094 |
| 0.5 | 4 | 3 | 6.35 | 288.33 | 45.41 | 0.0132 | 0.0129 |
| 0.5 | 5 | 5 | 4.75 | 282.94 | 59.57 | 0.0124 | 0.0121 |
| 0.5 | 6 | 5 | 4.75 | 249.77 | 52.58 | 0.0160 | 0.0156 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Troina, G.; Cunha, M.; Pinto, V.; Rocha, L.; dos Santos, E.; Fragassa, C.; Isoldi, L. Computational Modeling and Constructal Design Theory Applied to the Geometric Optimization of Thin Steel Plates with Stiffeners Subjected to Uniform Transverse Load. Metals 2020, 10, 220. https://doi.org/10.3390/met10020220
Troina G, Cunha M, Pinto V, Rocha L, dos Santos E, Fragassa C, Isoldi L. Computational Modeling and Constructal Design Theory Applied to the Geometric Optimization of Thin Steel Plates with Stiffeners Subjected to Uniform Transverse Load. Metals. 2020; 10(2):220. https://doi.org/10.3390/met10020220
Chicago/Turabian StyleTroina, Grégori, Marcelo Cunha, Vinícius Pinto, Luiz Rocha, Elizaldo dos Santos, Cristiano Fragassa, and Liércio Isoldi. 2020. "Computational Modeling and Constructal Design Theory Applied to the Geometric Optimization of Thin Steel Plates with Stiffeners Subjected to Uniform Transverse Load" Metals 10, no. 2: 220. https://doi.org/10.3390/met10020220







