Poisson’s Ratio of Selected Metallic Materials in the Elastic–Plastic Region
Abstract
:1. Introduction
2. Materials and Methods
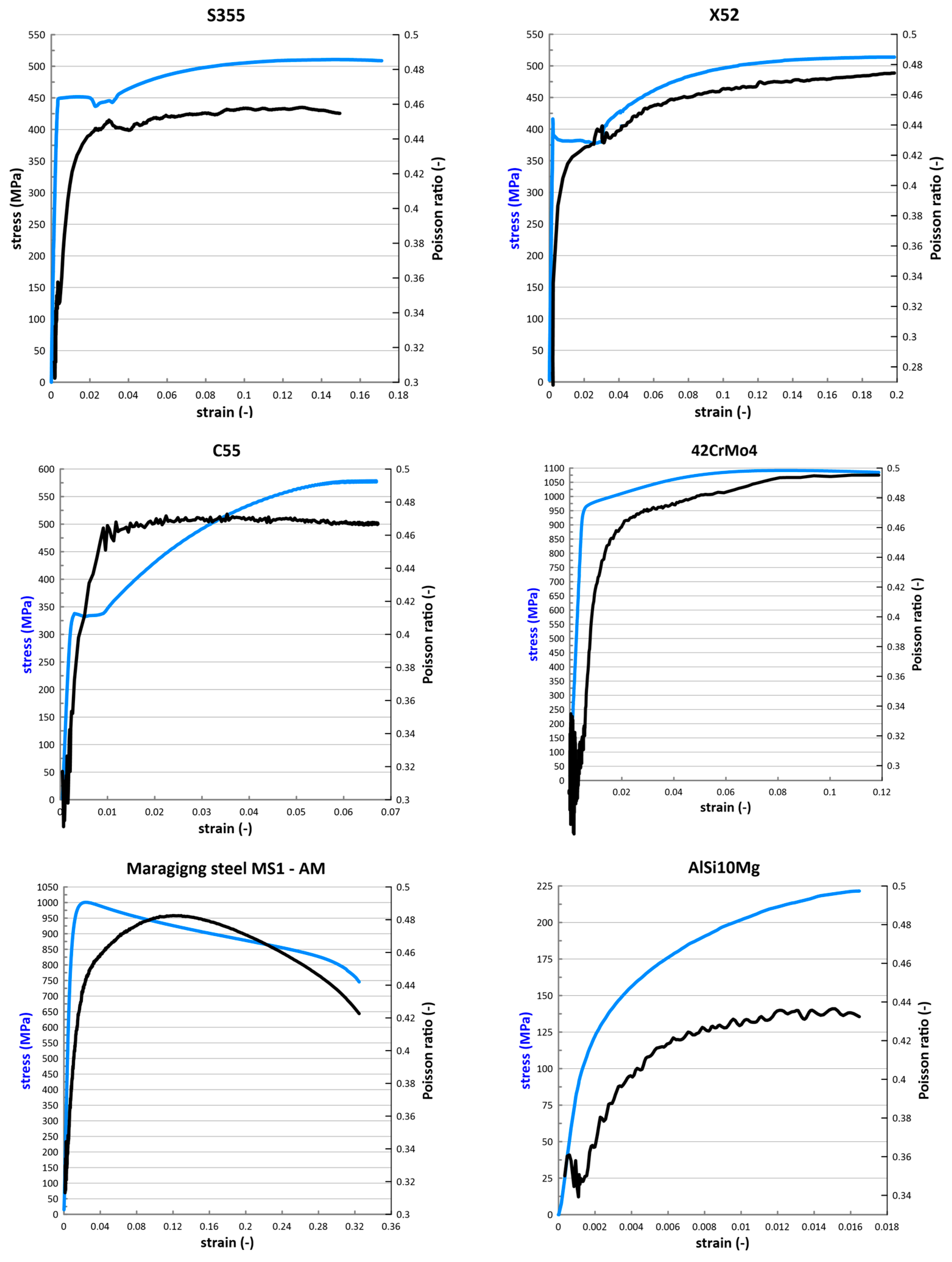
3. Results
4. Discussion
5. Conclusions
- -
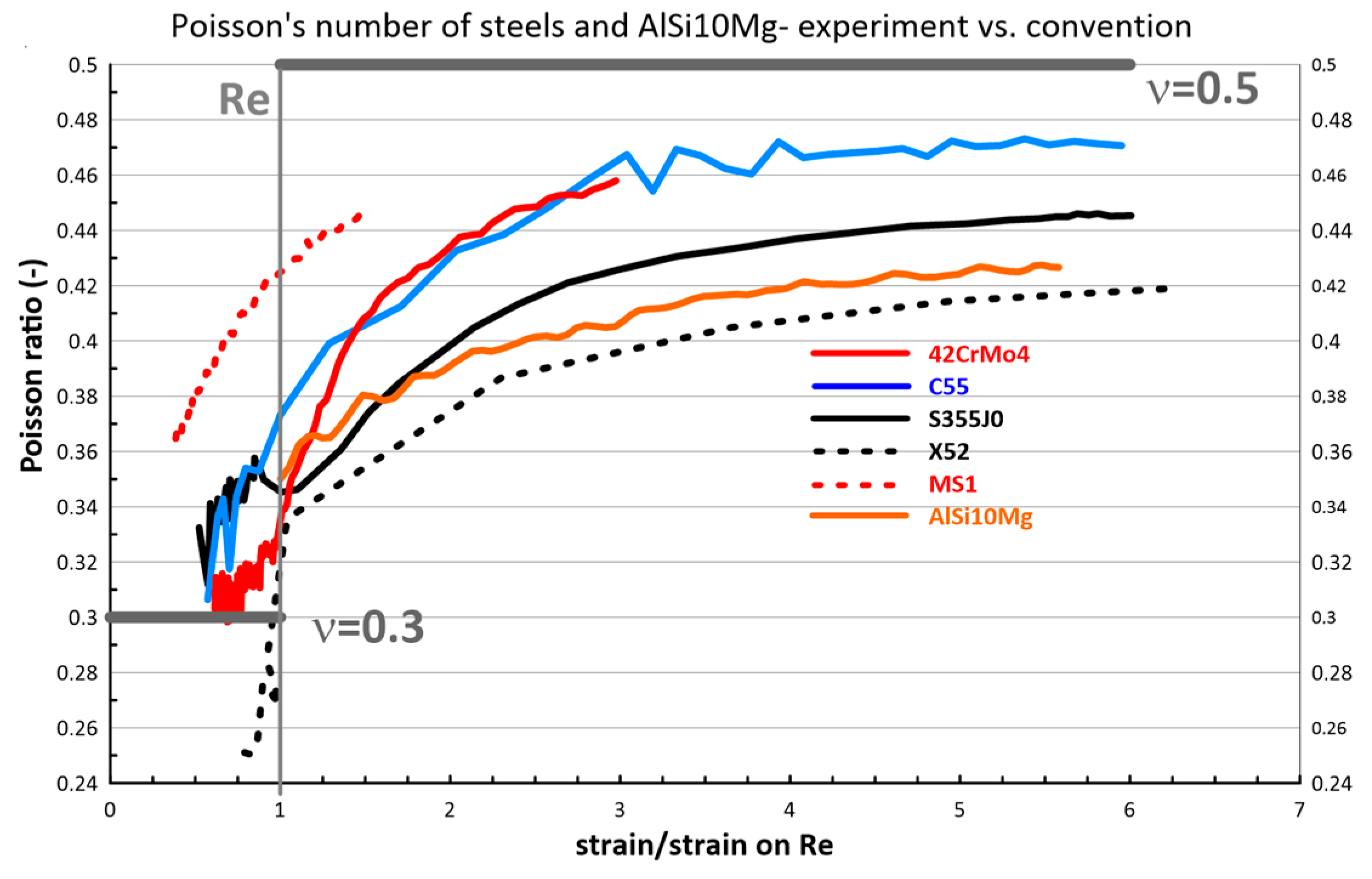
- In the group of steels and aluminum alloys studied, the Poisson ratio can be considered stable up to about 0.75 Re; above this limit, its begins significantly increasing.
- -
- The increase in Poisson’s ratio is at least partially saturated in character, i.e., the gradient of change is significantly reduced in the region above 5% of the total strain, and, from about 8% of the strain, its value becomes significantly saturated.
- -
- A Poisson’s ratio value of 0.5, representing the condition of constant volume, is reached only by the 42crmo4 and C55 steels; for the other materials studied. this value did not exceed 0.46 (for AlSi10Mg 0.44).
- -
- After the initiation of the localization of plastic deformation (formation of the neck or wall buckling of the annular cross-section in the case of MS1), it is not possible to correctly evaluate the magnitude of the Poisson ratio due to the local change in the state of stress.
- -
- The evolution of Poisson’s ratio from 0.3 in the elastic region to 0.46 in the plastic region has the greatest influence on the simulation results in the region of high gradient of this change—that is, in the region of the yield stress; in the steady-state creep region, the influence of Poisson’s ratio changes decreases significantly.
- -
- The influence of the evolution of Poisson’s ratio has to be considered in stress–strain simulations for steels that are especially in the region (0.75 ÷ 1.2) Re because of the significant influence on stress values, but specifically because of the deformations that occur in this region with a significant gradient of change; an example of this in engineering applications is represented by a location of sharp notches leading to the need for life assessment in the region of high-cycle fatigue.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| FEM | Finite element method |
| FBG | Fiber Bragg grating sensors |
| ESPI | Electronic speckle-pattern interferometry |
| DIC | Digital image correlation method |
Nomenclature
| Re | Yield stress |
| ν | Poisson’s ratio |
| σ | Normal stress |
| E | Young’s modulus in tension |
| G | Modulus in shear |
References
- Greaves, G. Poisson’s Ratio over Two Centuries: Challenging Hypotheses. Notes Rec. R. Soc. Lond. 2013, 67, 37–58. [Google Scholar] [CrossRef] [PubMed]
- Poisson, S.D. Note sur l’Extension des Fils et des Plaques elastiques. Annls Chim. Phys. 1827, 36, 384–387. [Google Scholar]
- Cagniard de la Tour, C. Notice sur les Travaux Scientifiques de M. Cagniard-Latour; Bachelier: Paris, France, 1851. [Google Scholar]
- Cauchy, A.L. Recherches sur l’e’quilibre et le mouvement inte´rieur des corps solides ou fluides, élastiques ou non-élastiques’. In Sur les E´quations qui Expriment les Conditions d’e´Quilibre, ou Les Lois du Mouvement Inte´Rieur d’un Corps Solide e´Lastique ou non e´Lastique; Gauthier-Villars: Paris, France, 1828; Volume 8, pp. 227–277. [Google Scholar]
- Köster, W.; Franz, H. Poisson’s ratio for metals and alloys. Metall. Rev. 1961, 6, 1–55. [Google Scholar] [CrossRef]
- Kirchhoff, G.R. Uber das Verhältinis der Quercontraction zur Längen-dilatation bei Stäben von federafrten Stahl. Poggendorfs Annln 1859, 108, 369–392. [Google Scholar] [CrossRef]
- Grüneisen, E. Interferenzapparat zur Messung der Querkontraktion eines Stabes bei Belastung. Z. InstrumKunde 1908, 28, 89–100. [Google Scholar]
- Motra, H.B.; Hildebrand, J.; Dimmig-Osburg, A. Assessment of strain measurement techniques to characterise mechanical properties of structural steel. Eng. Sci. Technol. Int. J. 2014, 17, 260–269. [Google Scholar] [CrossRef]
- Chmelko, V.; Harakaľ, M.; Žlábek, P.; Margetin, M.; Ďurka, R. Simulation of Stress Concentrations in Notches. Metals 2021, 12, 43. [Google Scholar] [CrossRef]
- Williams, J.G.; Gamonpilas, C. Using the simple compression test to determine Young’s modulus, Poisson’s ratio and the Coulomb friction coefficient. Int. J. Solids Struct. 2008, 16, 4448–4459. [Google Scholar] [CrossRef]
- Chang, J.-Y.; Yu, G.-P.; Huang, J.-H. Determination of Young’s modulus and Poisson’s ratio of thin films by combining sin2ψ X-ray diffraction and laser curvature methods. Thin Solid Film. 2009, 517, 6759–6766. [Google Scholar] [CrossRef]
- Baughman, R.; Shacklette, J.; Zakhidov, A.; Stafström, S. Negative Poisson’s ratios as a common feature of cubic metals. Nature 1998, 392, 362–365. [Google Scholar] [CrossRef]
- Kohlhauser, C.; Hellmich, C. Determination of Poisson’s ratios in isotropic, transversely isotropic, and orthotropic materials by means of combined ultrasonic-mechanical testing of normal stiffnesses: Application to metals and wood. Eur. J. Mech. A Solids 2012, 33, 82–98. [Google Scholar] [CrossRef]
- ICózar, R.; Arbeláez-Toro, J.J.; Maimí, P.; Otero, F.; González, E.V.; Turon, A.; Camanho, P.P. A novel methodology to measure the transverse Poisson’s ratio in the elastic and plastic regions for composite materials. Compos. Part B Eng. 2023, 272, 111098. [Google Scholar] [CrossRef]
- Lührs, L.; Soyarslan, C.; Markmann, J.; Bargmann, S.; Weissmüller, J. Elastic and plastic Poisson’s ratios of nanoporous gold. Scr. Mater. 2016, 110, 65–69. [Google Scholar] [CrossRef]
- Koščo, T.; Ďurka, R. Digital image correlation for accurate strain measurement on sharp notched specimens. Procedia Struct. Integr. 2022, 42, 1600–1606. [Google Scholar] [CrossRef]
- Huber, N.; Viswanath, R.N.; Mameka, N.; Markmann, J.; Weimüller, J. Scaling laws of nanoporous metals under uniaxial compression. Acta Mater. 2014, 67, 252–265. [Google Scholar] [CrossRef]
- Gu, X.J.; McDermott, A.G.; Poon, J.S.; Shiflet, G.J. Critical Poisson’s ratio for plasticity in Fe–Mo–C–B–Ln bulk amorphous steel. Appl. Phys. Lett. 2006, 88, 211905. [Google Scholar] [CrossRef]
- Mohammadi, M.; Dryden, J.R. Influence of the spatial variation of Poisson’s ratio upon the elastic field in nonhomogeneous axisymmetric bodies. Int. J. Solids Struct. 2009, 46, 788–795. [Google Scholar] [CrossRef]
- Chen, D.H.; Nisitani, H. Effect of poisson’s ratio on elastic-plastic stress under plane deformation. Eng. Anal. Bound. Elem. 1997, 20, 17–24. [Google Scholar] [CrossRef]
- Gu, T.; Jia, L.-J.; Chen, B.; Min Xia, M.; Guo, H.; He, M.-C. Unified full-range plasticity till fracture of meta steel and structural steels. Eng. Fract. Mech. 2021, 253, 107869. [Google Scholar] [CrossRef]
- Chmelko, V.; Biro, D. Safety of pressure pipe operation with corrosive defect. Procedia Struct. Integr. 2019, 17, 520–525. [Google Scholar] [CrossRef]
- Chmelko, V.; Garan, M.; Berta, I. Calculation of burst pressure of pipeline with local defect. Procedia Struct. Integr. 2020, 26, 417–421. [Google Scholar] [CrossRef]
- Garan, M.; Chmelko, V.; Šulko, M.; Musil, M. Fatigue failure of a pressing machine. Appl. Sci. 2021, 11, 398. [Google Scholar] [CrossRef]
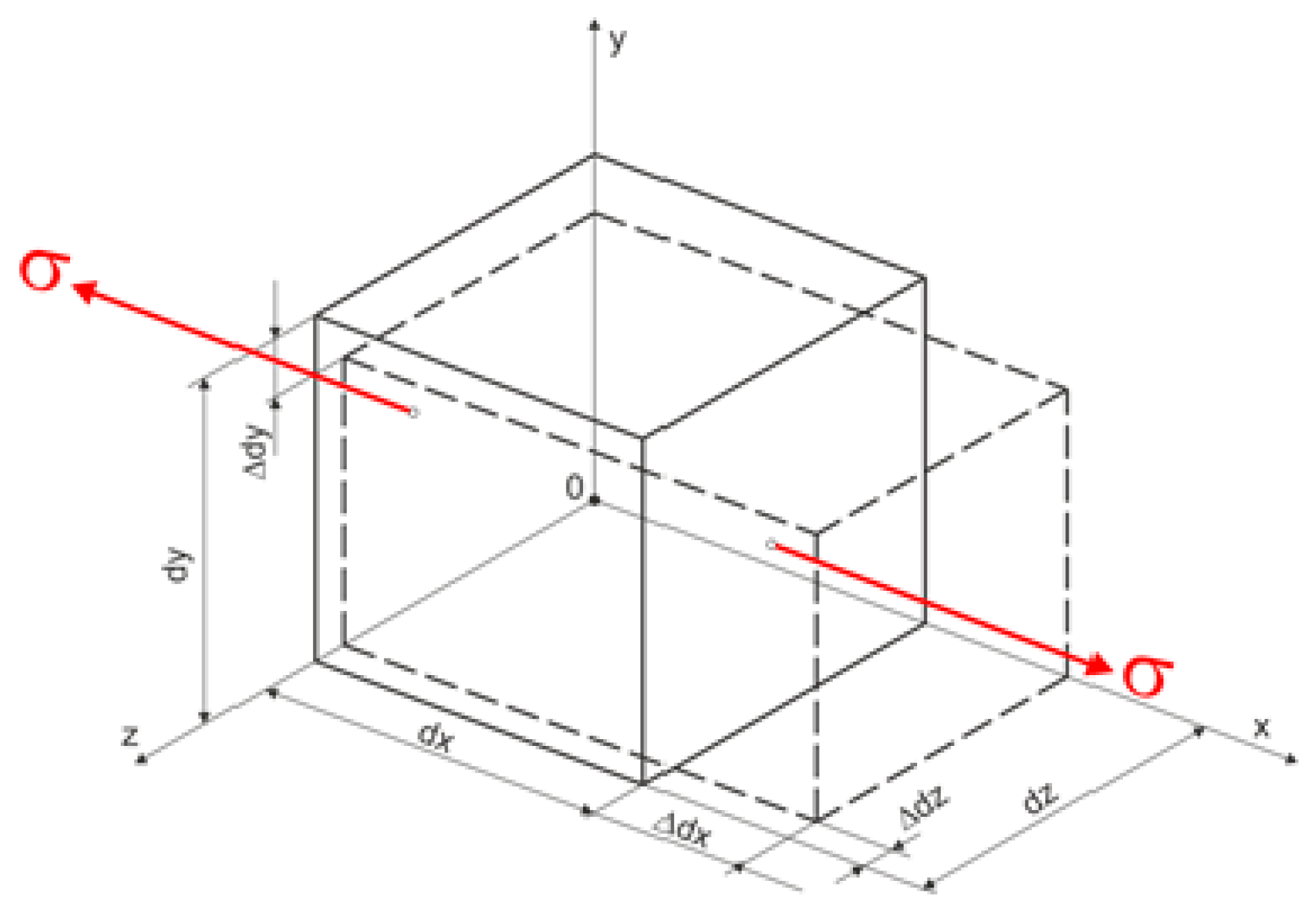
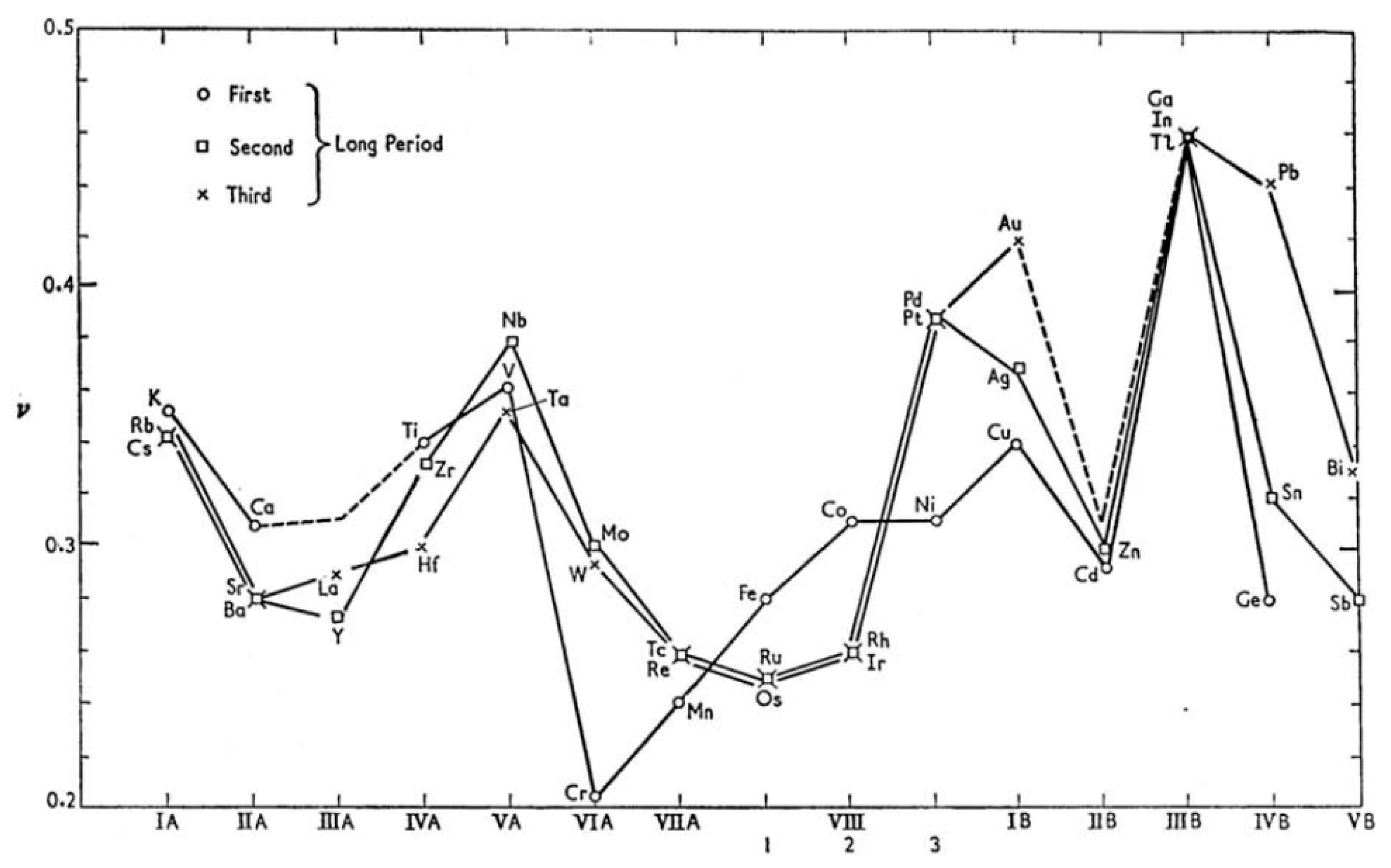




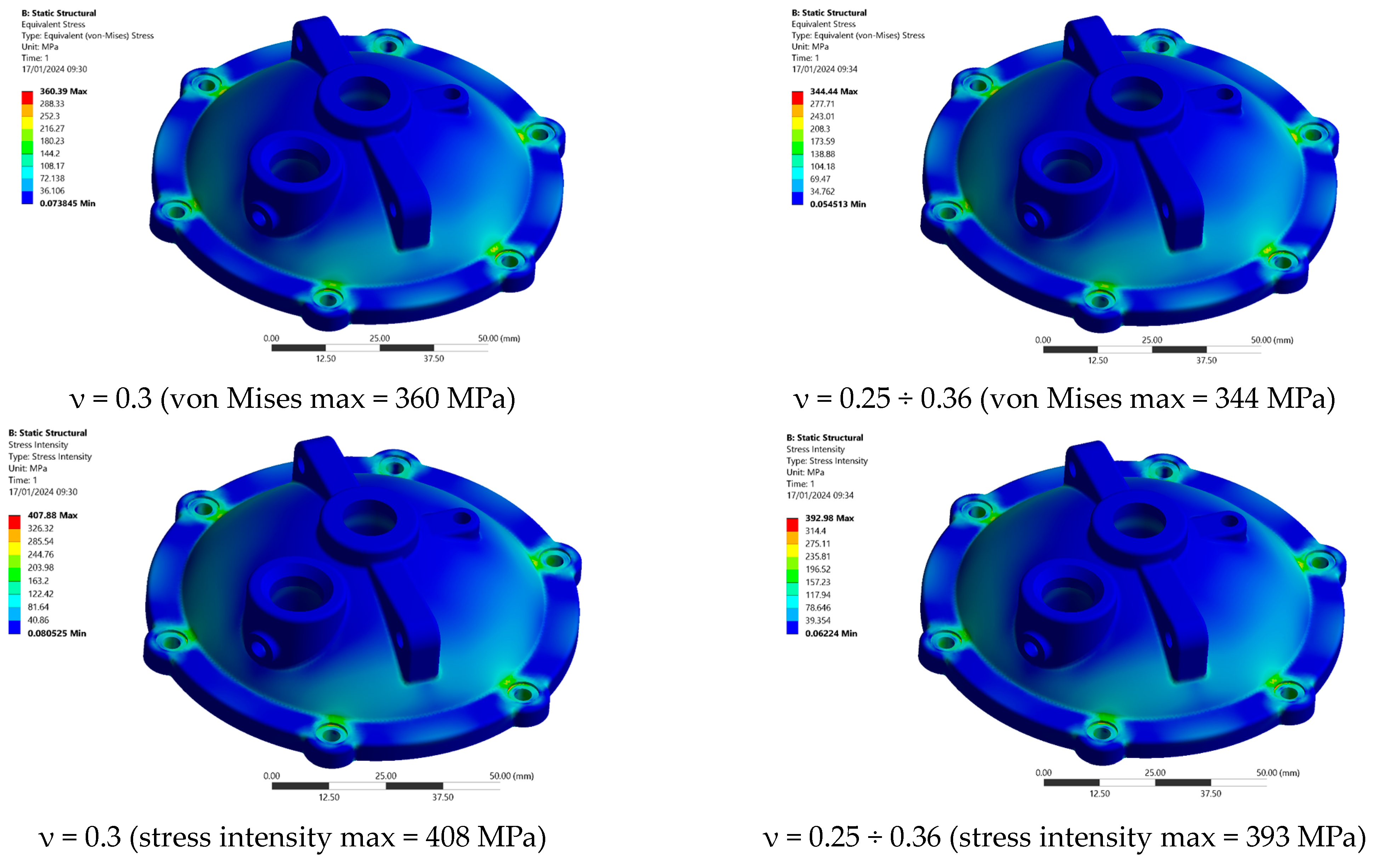

| Material | Chemical Composition (%) | Yield Stress (MPa) | Ultimate Strength (MPa) | Poisson Ratio Average (0 ÷ 0.75) Re |
|---|---|---|---|---|
| S355 plate | C 0.16, Mn 0.53, Si 0.026, Cu 0.035 Al 0.03, Cr 0.028 | 450 | 520 | 0.285 |
| X52 | C 0.15, Mn 0.6, Si 0.32, Cr 0.055, Cu 0.04 | 410 | 550 | 0.265 |
| C55 | C 0.54, Mn 0.64, Si 0.25 | 337 | 578 | 0.305 |
| 42CrMo4 | C 0.41, Mn 0.63, Cr 1.1, Mo 0.25 | 960 | 1090 | 0.293 |
| MS1 | Ni 18, C 8.9, Mo 5.1, Ti 0.61, Al 0.1 | 990 | 1000 | 0.338 |
| AlSi10Mg | Si 10.2, Mg 0.35, Fe 0.112, Mn 0.05, Cu 0.017 | 110 | 221 | 0.35 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chmelko, V.; Koščo, T.; Šulko, M.; Margetin, M.; Škriniarová, J. Poisson’s Ratio of Selected Metallic Materials in the Elastic–Plastic Region. Metals 2024, 14, 433. https://doi.org/10.3390/met14040433
Chmelko V, Koščo T, Šulko M, Margetin M, Škriniarová J. Poisson’s Ratio of Selected Metallic Materials in the Elastic–Plastic Region. Metals. 2024; 14(4):433. https://doi.org/10.3390/met14040433
Chicago/Turabian StyleChmelko, Vladimír, Tomáš Koščo, Miroslav Šulko, Matúš Margetin, and Jaroslava Škriniarová. 2024. "Poisson’s Ratio of Selected Metallic Materials in the Elastic–Plastic Region" Metals 14, no. 4: 433. https://doi.org/10.3390/met14040433
APA StyleChmelko, V., Koščo, T., Šulko, M., Margetin, M., & Škriniarová, J. (2024). Poisson’s Ratio of Selected Metallic Materials in the Elastic–Plastic Region. Metals, 14(4), 433. https://doi.org/10.3390/met14040433









