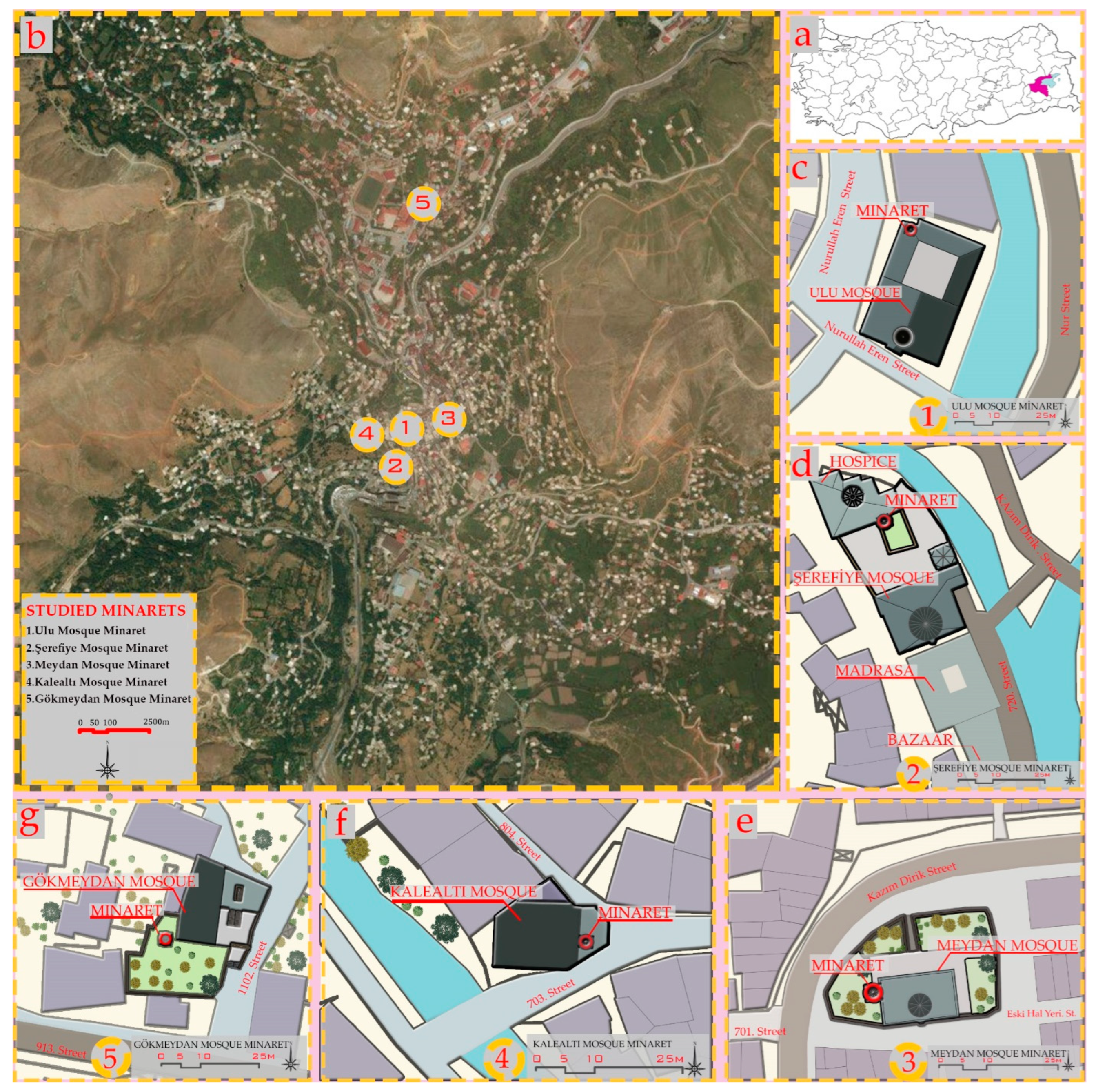
3.2. The Determination of the Bitlis Stone Properties Used in Minarets
It has been considered as a single type of the material, which is locally called Bitlis Stone, and is used in all the minarets considered in the study. Modulus of elasticity (E), Poisson ratio, and the weight per unit volume (γ) values for Bitlis stone was taken as a single value for all minarets. While determining these values, the unit volume and the specific weight values were taken directly from the study by Işık et al. (2020) [
50]. For the other two properties, the results were obtained by using the nondestructive test method. The propagation changes of the ultrasonic pulse velocity (UPV) wave are analyzed and applied without causing any deterioration in the material in the UPV method. This method, which enables the investigation of material homogeneity, can be considered an important method in the evaluation of concrete or natural stone structures [
51]. In this method, an idea of the strength of the specimen is obtained based on the propagation speed of ultrasonic sound waves at certain frequencies in the specimen. Sound waves give an idea of cracks in the sample. An ultrasonic pulse is applied to one side of the sample with an ultrasonic pulse velocity tester, and pressure waves (P waves) are generated and recorded from the other side of the specimen. The ultrasonic pulse velocity tester measures the time taken by the pulse to proceed through the specimen. UPV equipment consists of a receiver, a transmitter, and a digital display [
52]. The propagation times of the waves read from the device display were divided by the size of the specimen, and the propagation rates were determined for each sample. UPV test was applied to cube specimens of 15 cm × 15 cm × 15 cm. The results obtained for the specimens are shown in
Table 1.
According to the fracture test performed on cube specimens of 15 × 15 × 15 cm dimensions taken from natural stones known as Bitlis ignimbrites and used as building stones and the elastic property examinations obtained separately in two directions, the following results were obtained:
Specimen 1 has the highest fracture load (179.5 kN). The weight per unit volume was calculated as 14.2 kN/m3, average modulus of elasticity 4.34 × 106 (kN/m2), average Poisson ratio 0.21, and average shear modulus 1.79 × 106 (kN/m2);
The fracture load of specimen 2 was obtained as 163.8 kN. The weight per unit volume was calculated as 14.12 kN/m3, average modulus of elasticity 4.75 × 106 (kN/m2), average Poisson ratio 0.21, and average shear modulus 1.96 × 106 (kN/ m2);
The fracture load of specimen 3 was obtained as 146.4 kN. The weight per unit volume was calculated as 14.02 kN/m3, average modulus of elasticity 3.92 × 106 (kN/m2), average Poisson ratio 0.22, and average shear modulus 1.60 × 106 (kN/m2);
The fracture load of specimen 4 was determined as 153.2 kN. The weight per unit volume was calculated as 14.51 kN/m3, average modulus of elasticity 3.03 × 106 (kN/m2), average Poisson ratio 0.23, and average shear modulus 1.23 × 106 (kN/m2);
The fracture load of specimen 5 was obtained as 153.2 kN. The weight per unit volume was calculated as 14.22 kN/m3, average elastic modulus 4.04 × 106 (kN/m2), average Poisson ratio 0.24, and average shear modulus 1.63 × 106 (kN/m2);
The fracture load of specimen 6 was obtained as 153.2 kN. The unit volume weight was calculated as 14.32 kN/m3, average modulus of elasticity 3.97 × 106 (kN/m2), average Poisson ratio 0.20, and average shear modulus 1.65 × 106 (kN/m2).
Ignimbrites may be of different compositions due to their formation and may change over short distances when an evaluation is made about the specimens in general. The weight per unit volume of the six specimens examined is very close to each other and does not show great compositional differences. Porosity values are also in the range of 25%–26%, and it is understood that they do not have a significant difference in terms of their formation. However, strength properties show significant changes. Measurements of the specimens taken from both directions showed that there were different strength values in both directions. Although the specimens have similar physical properties, the main reason why they have different values in strength properties is considered to be meteorological conditions. Particularly, the small amount of cracking in the direction where the specimen is exposed to the natural environment may cause a decrease in material strength. However, in general terms, it has sufficient strength conditions as a building block. Using the average values of these results, the material properties that are the basis for structural analysis are shown in
Table 2.
Modelling of masonry walls is extremely important in the evaluation and design of historical and modern masonry structures. Masonry walls can be modelled using three different modelling techniques such as detailed micro-modelling, simplified micro-modelling, and macro-modelling. These models can be seen in
Figure 5.
In detailed micro-modelling, the mechanical properties of the masonry unit and the mortar forming the masonry wall are taken separately. In this approach, it is assumed that cracks will occur at the interfaces between the masonry unit and the mortar. In simplified micro-modelling, mass densification was made at each connection point consisting of a mortar and two masonry unit–mortar interfaces, and it was accepted that cracks that could occur in masonry could occur at the mean interface line, assuming the average interface. Findings differ slightly from detailed micro-modelling, as the Poisson ratio of the mortar is not taken into account here. However, this difference is so small that it can be neglected [
53,
54,
55].
The macro modeling technique is one of the masonry structural modeling techniques and is widely used. While performing this type of modeling, analyses are carried out without making any distinction between the binding material (mortar, etc.) used in the building and the structural elements. In this modelling, the masonry unit and the properties of the mortar are homogenized and considered as a masonry composite material. The mechanical properties of this model are the values obtained as a result of the homogenization process. Macro modelling is more convenient in practice because it requires less memory and time. However, with macro modelling, stress distributions in masonry units and mortar can be obtained accurately [
55,
56,
57,
58] In this respect, structural masonry elements are considered composites, and an equivalent material model is used for all minarets models. Structural analyses were carried out for the Five Minarets in Bitlis using this macro-modeling technique. The mortar and Bitlis stone used in the minarets were considered as a single material. The sign criterion for the stress components of the elements used in the finite element model of the structure is shown in
Figure 6, in accordance with the assumptions stipulated by the software [
59] in which numerical modeling is made.
As stated in
Figure 6, S11 is vertical stress in (x) direction, S22 is vertical stress in (y) direction, S33 is vertical stress in (z) direction, and S12 = S21 constitute shear stresses in the x-y plane. With TBEC-2018, which was updated in 2018 and entered into force on 1 January 2019, the biggest change was the use of site-specific design spectra. Turkey Earthquake Maps Interactive Web Application has been developed to calculate design spectra and site-specific earthquake parameters. With the help of this application, horizontal and vertical design spectra can be obtained as well as earthquake parameters belonging to any desired geographical location. By using the coordinate values obtained for each minaret, design spectra and earthquake parameters were obtained with the help of this application. While obtaining these values, the design ground motion level DD-2 was chosen as the earthquake ground motion level. From the ground survey reports received from the relevant institutions, the ZB soil class was taken into account for all five minarets as the local soil class. As it can be seen from
Table 3, the design spectra were obtained close to each other since the seismic parameters for the minarets are close to each other. The horizontal and vertical spectra obtained for the Ulu Mosque Minaret are shown in
Figure 7 as an example. In these curves, the horizontal axis represents period values, while the vertical axes represent the horizontal and vertical elastic design spectral accelerations, respectively.
The earthquake parameters obtained for each minaret and used in structural analysis with the help of the Interactive Web Earthquake application are shown in
Table 3.
While the dimensions of Five Minarets are shown in
Figure 8, the three-dimensional models obtained from the software program are shown in
Figure 9.
In the structural analysis of the minarets, the finite element method, where the cross-section and material properties can be easily defined, was used. In finite element analysis, the geometry of the structure or structural elements is determined by a finite number of nodal points. The general structural properties of the minarets, the number of nodes, and the number of shell elements modelled in the software are shown in
Table 4.
Modal analysis is a dynamic analysis method that enables the determination of free vibration periods, frequency values, mass participation rates, and mode shapes of the structure. In order to determine the dynamic properties of the minarets, primarily modal analyzes were carried out. In TBEC-2018, it was requested to be determined according to the rule that the mass participation rates in the X and Y directions should not be less than 95%. In this case, modal analyses were carried out by considering the first 34 modes for the Ulu Mosque minaret; the first 24 modes for Gökmeydan mosque minaret; the first 44 modes for the Meydan Mosque minaret; the first 31 modes for the Kalealtı mosque minaret; and the first 43 modes for the Şerefiye Mosque minaret. The values related to the mass participation rates, natural vibration periods, and effective modes obtained by considering the first five modes of Five Minarets as a result of the modal analysis are shown in
Table 5. Torsion in all minarets occurred in the fifth mode.
The dead-load, live-load, and earthquake-load are taken into account for stress calculations. The software program according to material properties made dead load calculations. Horizontal and vertical elastic design spectra obtained from Turkey Earthquake Hazard Maps Interactive Web Applications were used as design spectra. For the earthquake load, load definition was made in three directions as EQx, EQy, and EQz. Structural analyses were performed for different load combinations by using these values. The load combinations envisaged in the TBEC-2018, which is currently used in Turkey, have been taken into account. Load combinations have been selected in accordance with the definition under the title of Combining Earthquake Effect with other Effects in TBEC-2018. Load combinations are defined by the constant load effect, live load effect, earthquake effects defined in perpendicular directions, and the vertical earthquake effect together with the load coefficients. The stress diagrams for S11 (vertical stress in the x-direction) obtained from different load combinations are shown in
Figure 10.
The maximum stress diagrams for the vertical stress in the y-direction (S22) obtained from different load combinations are shown in
Figure 11.
The diagrams of shear stress in the x-y directions (S12) for five minarets under different load combinations are shown in
Figure 12.
The comparison of the maximum tensile stresses for Five Minarets according to the results of the structural analysis is given in
Table 6.
The comparison of the maximum compressive stresses according to the analysis results for Five Minarets is given in
Table 7.
The comparison of the maximum shear stress values obtained from the structural analyzes for Five Minarets is given in
Table 8.
According to the analysis results of Five Minarets, the maximum displacement of connection elements in both negative and positive Ux (U
1) directions is given in
Table 9.
According to the analysis results of Five Minarets, the maximum displacement of connection elements in both negative and positive Uy (U
2) directions is given in
Table 10.
According to the analysis results of Five Minarets, the maximum displacement of connection elements in both negative and positive Uz (U
3) directions is given in
Table 11.
The highest period value was obtained for the Gökmeydan mosque minaret, which is the highest minaret, while the lowest period value was obtained for the Kalealtı mosque minaret. The variation of height directly affected the period values. The highest tensile stress values were obtained for the minaret of the Şerefiye Mosque, while the lowest values were obtained for the minaret of the Gökmeydan Mosque. The differences in the structural dimensions affected the tensile stresses. The highest values in terms of compressive stresses were obtained for the minaret of the Şerefiye Mosque, while the lowest values were obtained for the minaret of the Kalealtı Mosque. The highest shear stresses occurred in the minaret of the Şerefiye mosque, while the lowest shear stresses occurred in the minaret of the Kalealtı mosque. The largest displacements were obtained for the Gökmeydan mosque minaret, which is the highest minaret, while the smallest displacement values were obtained for the Kalealtı mosque minaret, which is the lowest one. All values obtained are considerably smaller than the minimum compression, tensile, and shear stress values in TS EN 1467 [
60], which is used for natural stones and raw blocks in Turkey and includes natural stone properties. Accordingly, the minimum safe compressive stress is 34 MPa [
60]. Therefore, the values found in all of the minarets were obtained below this value. In addition, the minimum tensile strength in bending for blasted stones can be taken as 8 MPa [
61] For stone walls built in masonry, the safe shear stress (τ
s) can be calculated by Equation (1).
Here, σ indicates the compressive strength of the material. The compressive strength value, which was calculated as 34 MPa above, was substituted in Equation (1), and the safe shear stress value was calculated as 17 MPa. The maximum compressive, shear, and tensile stresses obtained from the analyses show thag these stresses can be safe to be carried by the structure. This result is in accordance with the fact that the structure survived in the process.