Development of a New Response Spectrum Analysis Approach for Determining Elastic Shear Demands on Shear-Dominated Steel Building Frames
Abstract
:1. Introduction
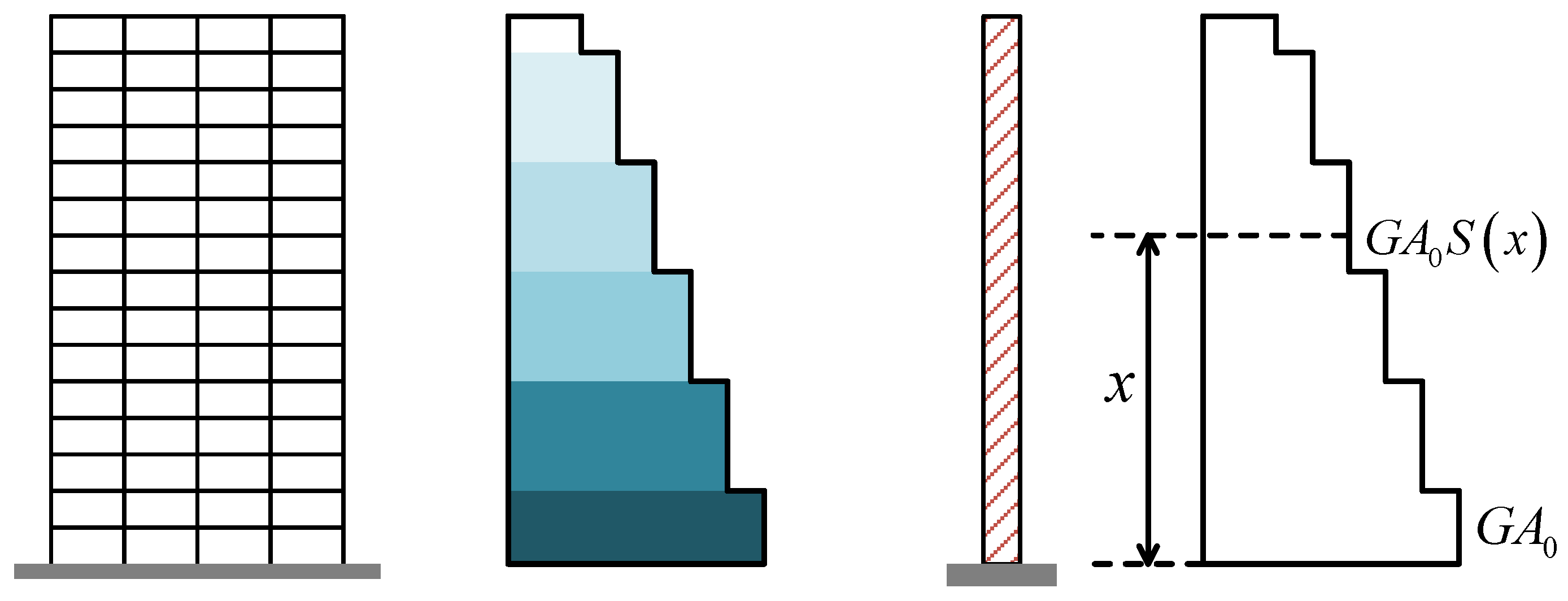
2. A Simplified Model for Shear Building
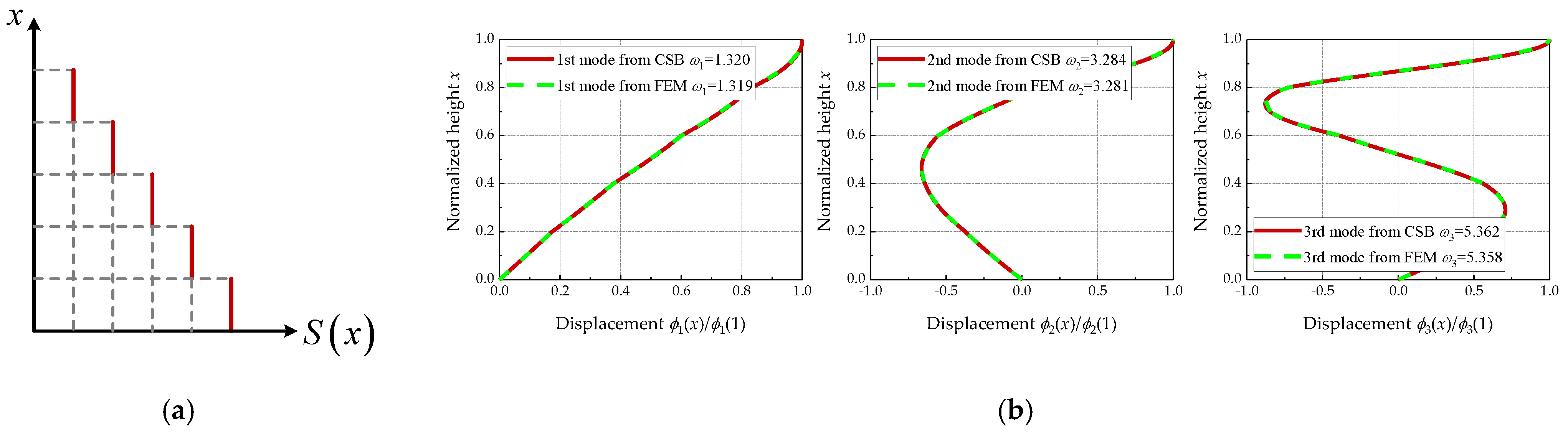
2.1. Continuous Shear Beam with Stepped Stiffness
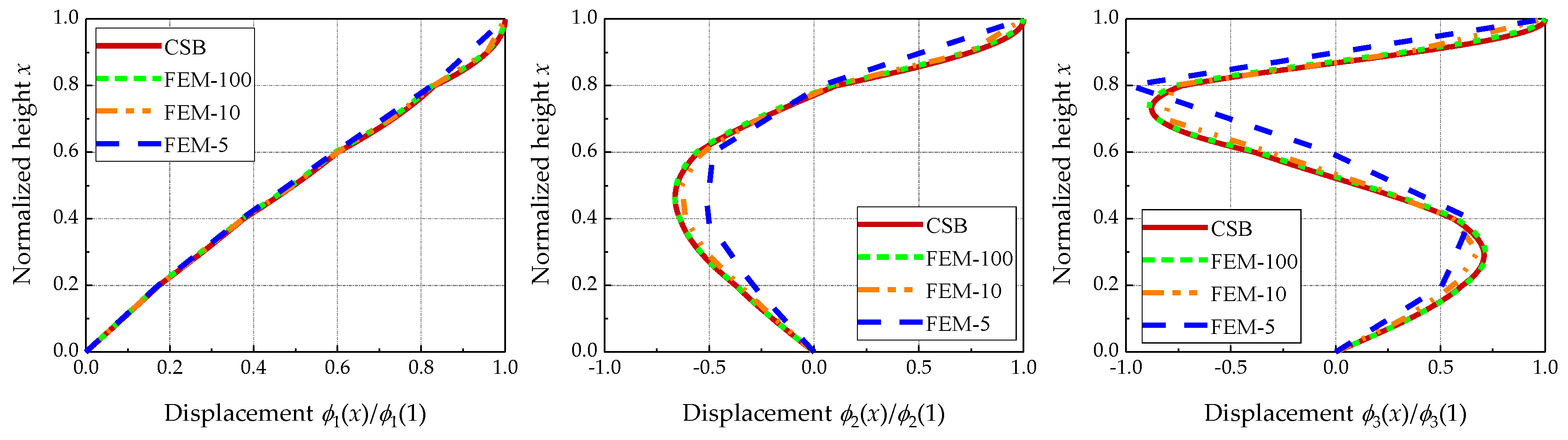
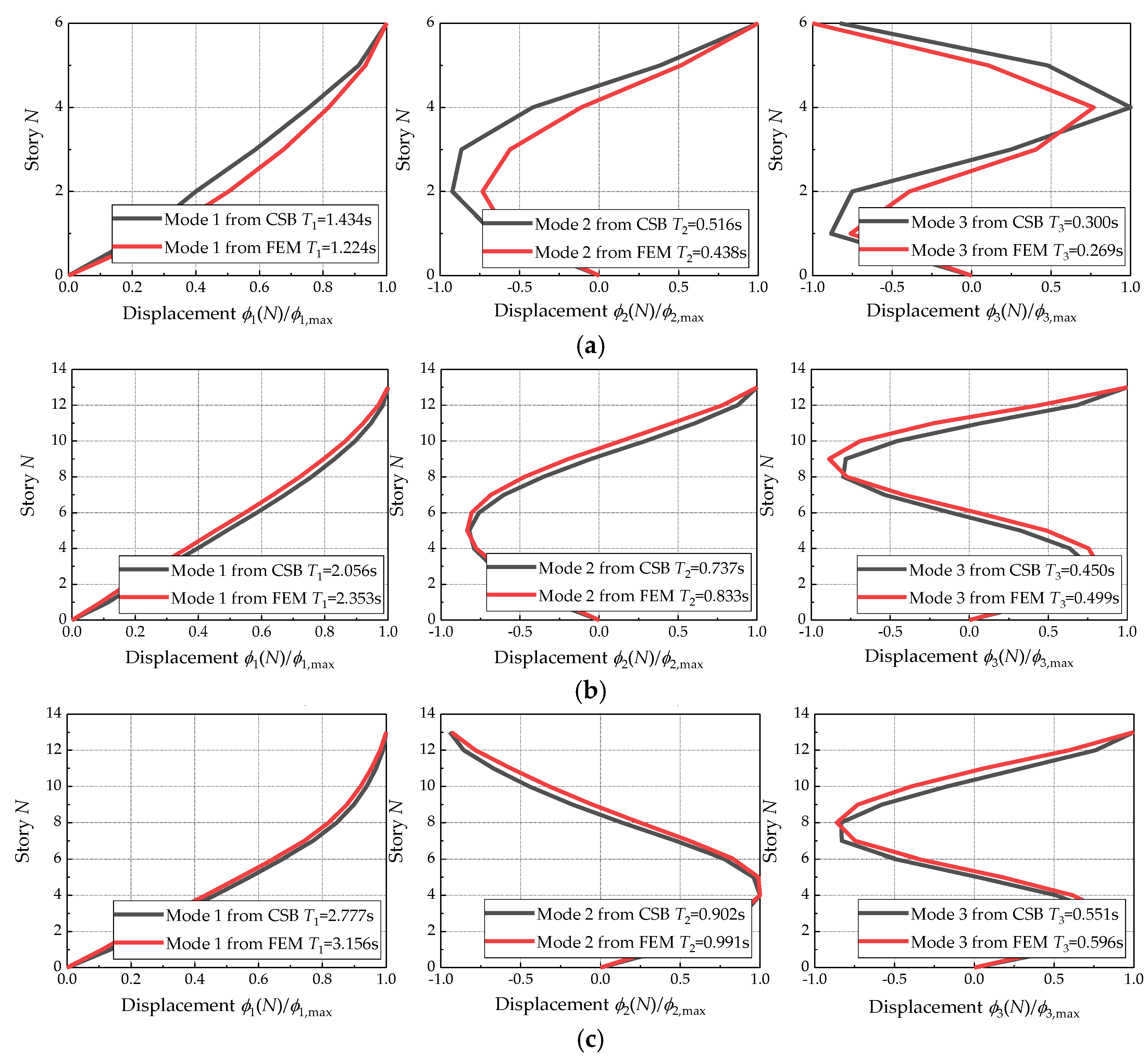
2.2. Validation of the Approach
3. Modified SRSS Method for Improved Response Spectrum Analysis
3.1. Response Spectrum Analysis with CSRSS
3.2. The MSRSS Method
4. Validation of the IRSA Method
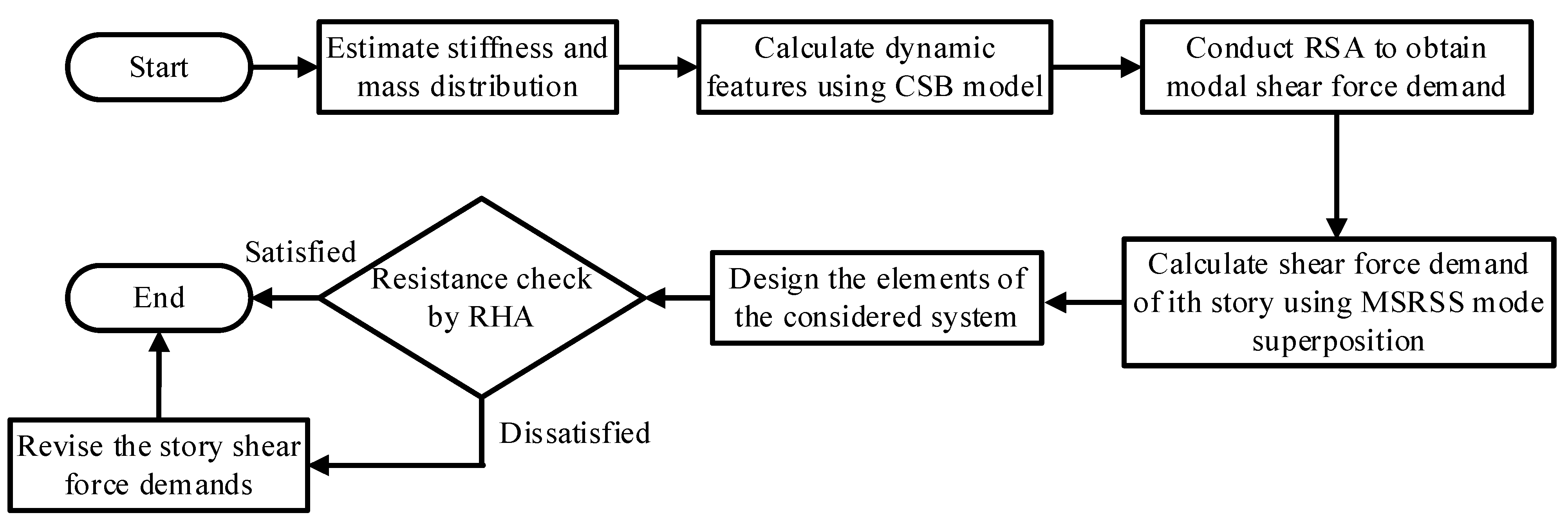
4.1. Design Procedure of IRSA
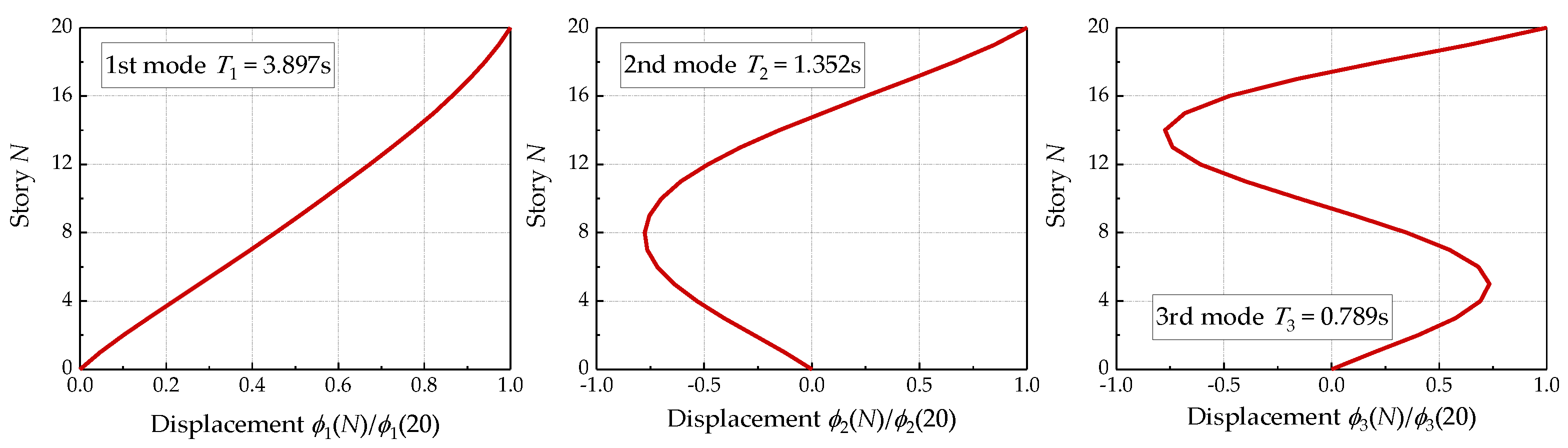
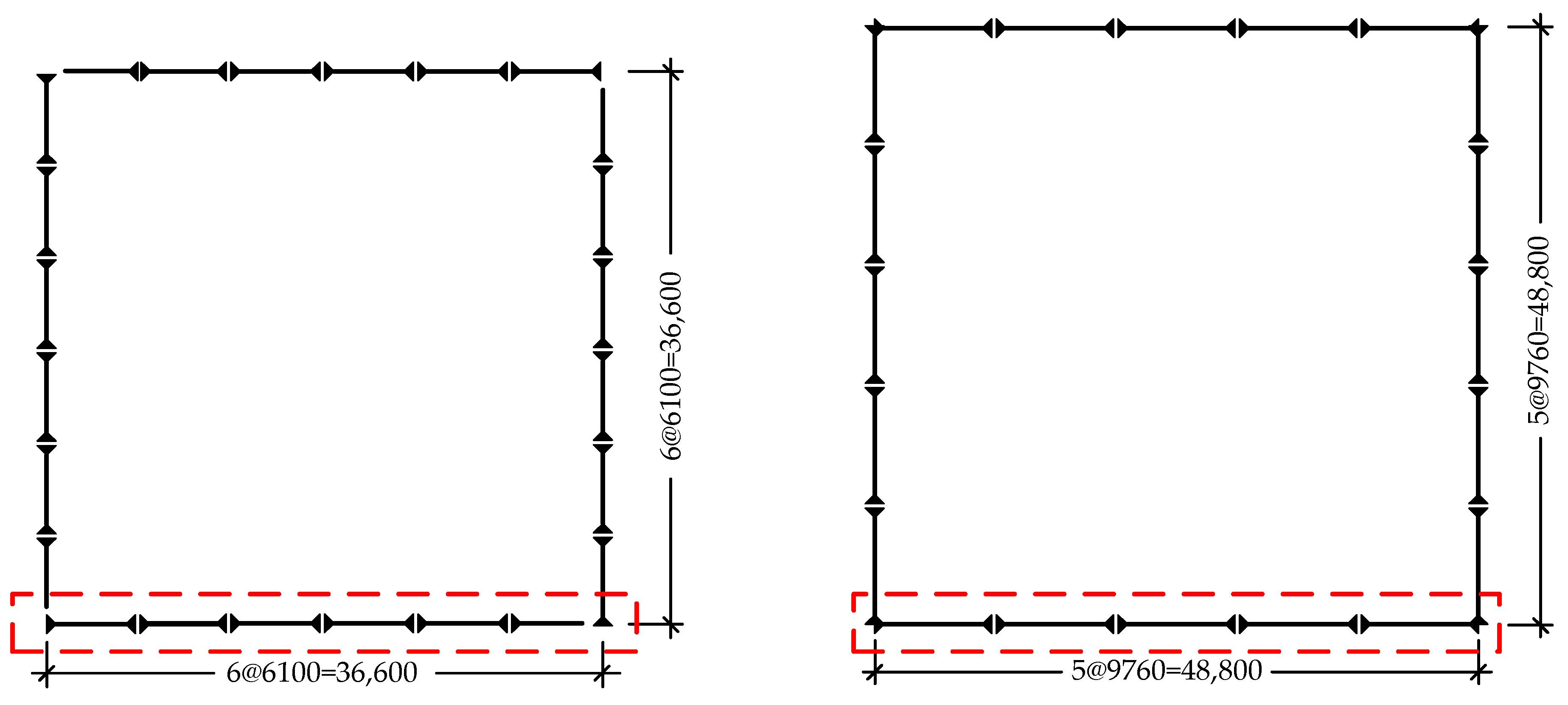
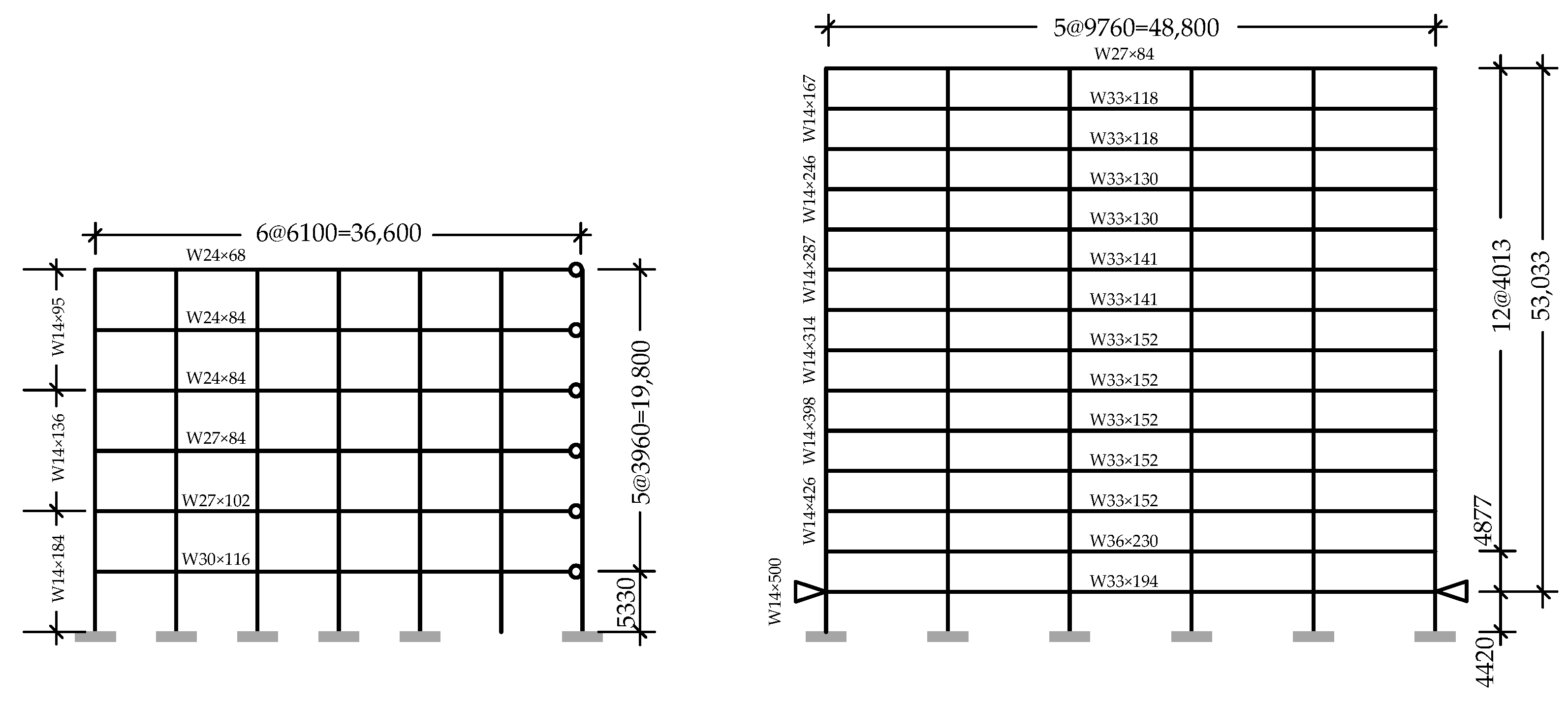
4.2. Demonstration Buildings
4.3. Computer Modeling and Seismic Excitations
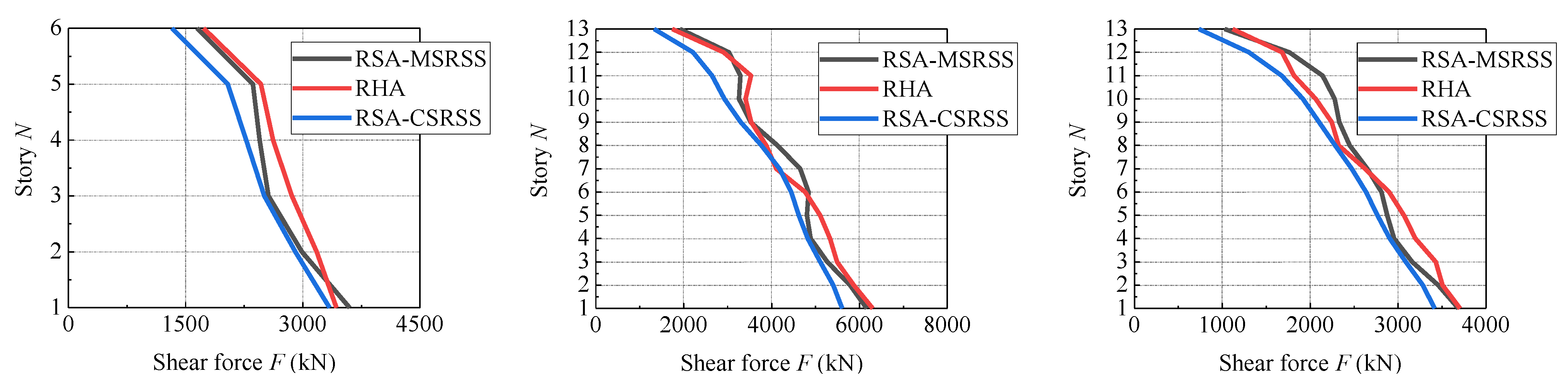
4.4. Discussions of Analysis Results
5. Concluding Remarks
- The continuous shear beam model with a stepped stiffness could achieve a precise estimation of the dynamic parameters. Following the proposed procedure, the modal periods and modal shapes can be easily and accurately captured without conducting an eigenvalue analysis using numerical software.
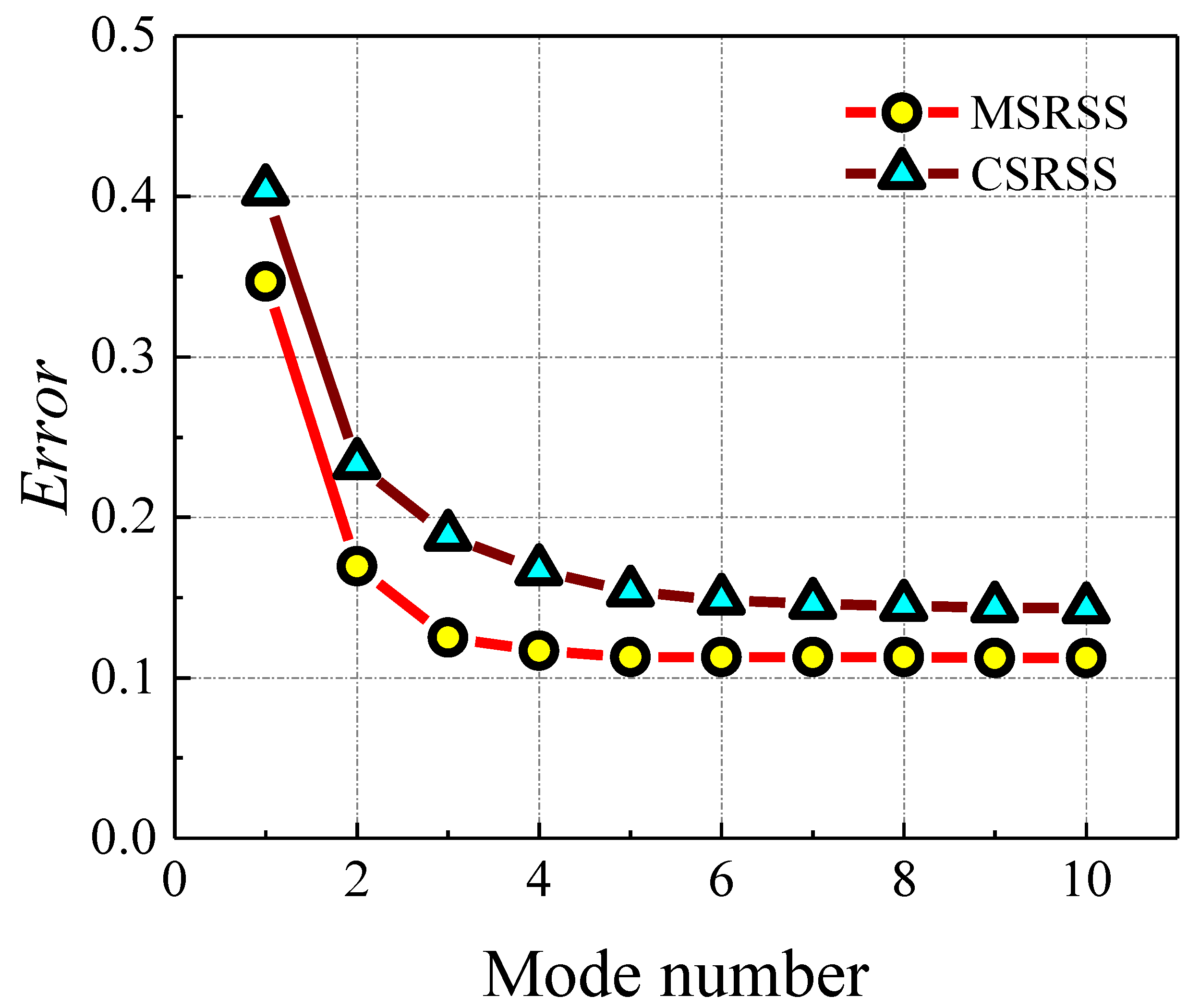
- The conventional response spectrum analysis method, which uses limited modes in the SRSS method will underestimate the shear demands of a steel building frame. Moreover, the underestimation of the shear demands on the top of the considered system is more significant.
- It was found that adjusting the weighting coefficient for each modal shear demand could improve the adequacy of the RSA method in determining the elastic shear force in the steel building frames. The optimal weighting coefficient set derived from the genetic algorithm validates the fact that adding a weighting coefficient that is greater than 1.0 to high modal shear items could result in the better estimation of shear force demands. Moreover, using the first three modal shear demands to conduct mode superposition can achieve a satisfactory estimation, and so, more modes are not necessary.
- The proposed IRSA are performed on two demonstration building frames. Results validate the superiority of the proposed method and the adequacy of the proposed weighting coefficients.
- This study is conducted mainly on a regular building frame model in two dimensions. The cross-correlation between the modal responses is minor and negligible in this study. Thus, the application scope is limited. Much more irregular scenarios should be included to explore the method to improve the adequacy of conventional RSA. Moreover, since the cross-correlation between the modal responses of a structure in three dimensions is significant, the relevant research based on CQC is also promising.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Chopra, A.K. Dynamics of Structures: Theory and Applications to Earthquake Engineering, 4th ed.; Prentice Hall: Upper Saddle River, NJ, USA, 2012; pp. 562–595. [Google Scholar]
- ASCE. Minimum Design Loads for Buildings and Other Structures; American Society of Civil Engineers: Reston, VA, USA; Structural Engineering Institute: Weston, FL, USA, 2016. [Google Scholar]
- Rosenblueth, E. A Basis for Aseismic Design. Ph.D. Thesis, University of Illinois, Urbana, IL, USA, 1951. [Google Scholar]
- GB 50011-2010; Code for Seismic Design of Buildings. Ministry of Housing and Urban-Rural Development of the People’s Republic of China (MHURD-PRC): Beijing, China, 2010. (In Chinese)
- Newmark, N.M.; Rosenblueth, E. Fundamentals of Earthquake Engineering, 1st ed.; Prentice Hall: Englewood Cliffs, NJ, USA, 1971. [Google Scholar]
- Khy, K.; Chintanapakdee, C.; Warnitchai, P.; Wijeyewickrema, A.C. Modified Response Spectrum Analysis to Compute Shear Force in Tall RC Shear Wall Buildings. Eng. Struct. 2019, 180, 295–309. [Google Scholar] [CrossRef]
- Sullivan, T.J.; Priestley, M.J.N.; Calvi, G.M. Estimating the Higher-Mode Response of Ductile Structures. J. Earthq. Eng. 2008, 12, 456–472. [Google Scholar] [CrossRef]
- Pennucci, D.; Sullivan, T.J.; Calvi, G.M. Inelastic Higher-Mode Response in Reinforced Concrete Wall Structures. Earthq. Spec. 2015, 31, 1493–1514. [Google Scholar] [CrossRef]
- Hu, S.; Wang, W. Self-Centering Energy-Absorbing Rocking Core System with Friction Spring Damper: Experiments, Modeling and Design. Eng. Struct. 2020, 225, 111338. [Google Scholar] [CrossRef]
- Ke, K.; Chen, Y.; Zhou, X.; Yam, M.C.H. Experimental and Numerical Study of a Brace-Type Hybrid Damper with Steel Slit Plates Enhanced by Friction Mechanism. Thin Wall Struct. 2023, 182, 110249. [Google Scholar] [CrossRef]
- Timoshenko, S.P. On the correction for shear of the differential equation for transverse vibrations of prismatic bars. Philos. Mag. 1921, 41, 744–746. [Google Scholar] [CrossRef] [Green Version]
- Andrés, A.R.; Miranda, E. Assessment of Effects of Reductions of Lateral Stiffness along Height on Buildings Modeled as Elastic Cantilever Shear Beams. J. Earth. Eng. 2016, 22, 553–568. [Google Scholar]
- Ohtori, Y.; Christenson, R.E.; Spencer, B.F. Benchmark Control Problems for Seismically Excited Nonlinear Buildings. J. Struct. Eng. 2005, 130, 366–385. [Google Scholar] [CrossRef] [Green Version]
- Mazzoni, S.; McKenna, F.; Scott, M.H.; Fenves, G.L. OpenSees Command Language Manual. Pac. Earthq. Eng. Res. (PEER) Cent. 2006, 264, 137–158. [Google Scholar]
- Somerville, P.; Smith, N.; Punyamurthula, S.; Sun, J. Development of Ground Motion Time Histories for Phase 2 of the FEMA/SAC Steel Project; SAC Background Document; Report submitted to the Report SAC/DB-97/04; SAC Joint Venture: Sacramento, CA, USA, 1997. [Google Scholar]
- ICBO. Uniform Building Code; International Conference of Building Official: Wittie, CA, USA, 1973. [Google Scholar]
- Kunnath, S.K.; Nghiem, Q.; El-Tawil, S. Modeling and Response Prediction in Performance-Based Seismic Evaluation: Case Studies of Instrumented Steel Moment-Frame Buildings. Earthq. Spec. 2004, 20, 883–915. [Google Scholar] [CrossRef]
- FEMA-695; Quantification of Building Seismic Performance Factors. Federal Emergency Management Agency: Washington, DC, USA, 2009.




| Weighting Coefficients | Value |
|---|---|
| A1 | 1.000 |
| A2 | 1.276 |
| A3 | 1.663 |
| Mode Superposition | Error (%) | ||
|---|---|---|---|
| 6F | 13F | 13F-W | |
| MSRSS | 8.38 | 11.49 | 11.93 |
| CSRSS | 9.68 | 13.86 | 15.05 |
| Rate of improvement | 13.4 | 17.1 | 20.7 |
| 6F | 13F | 13F-W | ||||
|---|---|---|---|---|---|---|
| ELF | CSRSS | ELF | CSRSS | ELF | CSRSS | |
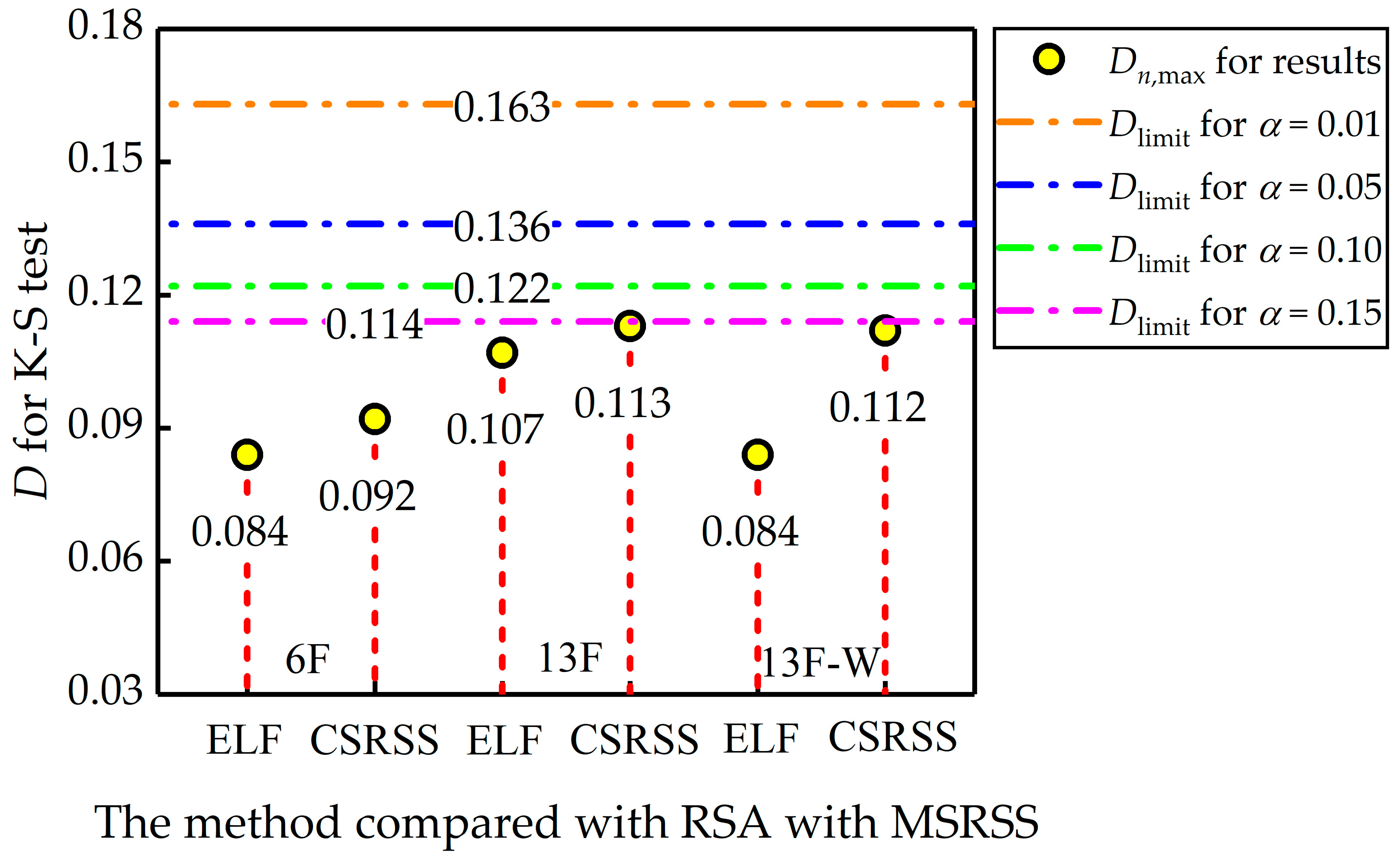
| Mean | 0.464 | 0.066 | 0.447 | 0.083 | 0.403 | 0.094 |
| SD | 0.300 | 0.200 | 0.307 | 0.243 | 0.292 | 0.254 |
| Dlimit-0.01 | 0.163 | |||||
| Dlimit-0.15 | 0.114 | |||||
| D | 0.084 | 0.092 | 0.107 | 0.113 | 0.084 | 0.112 |
| H0 | A | A | A | A | A | A |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, J.; Wang, W. Development of a New Response Spectrum Analysis Approach for Determining Elastic Shear Demands on Shear-Dominated Steel Building Frames. Buildings 2023, 13, 258. https://doi.org/10.3390/buildings13010258
Li J, Wang W. Development of a New Response Spectrum Analysis Approach for Determining Elastic Shear Demands on Shear-Dominated Steel Building Frames. Buildings. 2023; 13(1):258. https://doi.org/10.3390/buildings13010258
Chicago/Turabian StyleLi, Junlin, and Wei Wang. 2023. "Development of a New Response Spectrum Analysis Approach for Determining Elastic Shear Demands on Shear-Dominated Steel Building Frames" Buildings 13, no. 1: 258. https://doi.org/10.3390/buildings13010258
APA StyleLi, J., & Wang, W. (2023). Development of a New Response Spectrum Analysis Approach for Determining Elastic Shear Demands on Shear-Dominated Steel Building Frames. Buildings, 13(1), 258. https://doi.org/10.3390/buildings13010258







