Determining the Thermal Resistance of Enclosed Reflective Airspace
Abstract
:1. Introduction
2. Objectives
- (a)
- A full building assembly containing enclosed airspace as shown in Figure 1a. The assembly consists of enclosed airspace of 50.8 mm thick and 406.4 long. As shown in Figure 1a, the enclosed airspace is bounded by two wood studs, each 38.1 mm wide and 50.8 mm thick; an EPS layer of 25.4 mm thick; and a gypsum board of 12.7 mm thick.
- (b)
- Enclosed airspace only as shown in Figure 1b. It is important to point out that the purpose of considering this case to test the validity of isothermal assumption on the airspace R-values is that most (if not all, to the best of our knowledge) airspace R-values in the literature (e.g., see [10,11,23,24,25,26,27,28,33,34]) are based on the isothermal conditions of both the hot and cold surfaces.
3. Model Description, Simulation Parameters and Boundary Conditions
4. Methodology for Testing Isothermal Boundary Conditions Assumption
5. Results and Discussion
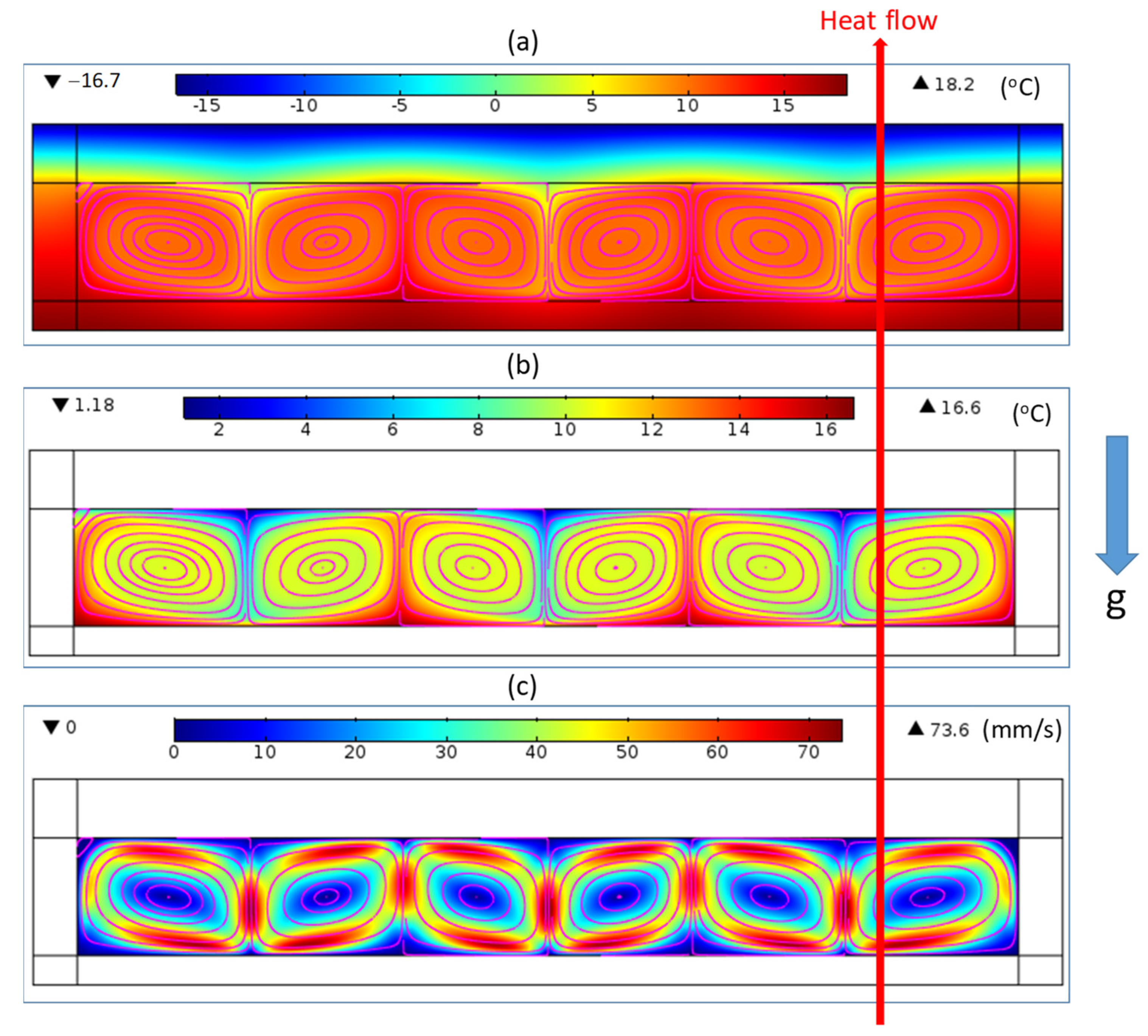
5.1. Effect of Radiative Heat Exchange at Airspace Edges
- No radiation takes place at the two ends of airspace surfaces (i.e., E3 = 0.0). This case is called “without end (or edge) effect”, which represents the case of net radiative transport between two large/infinite parallel surfaces that is currently being used in ISO 6946 [23] and ASHRAE [24,25]. The surfaces of the two ends of the airspace are usually the surfaces of the framing (e.g., furring or spacers) that bound the airspace. Note that the case of without end effect would represent the situation in which low-e material is present on the surfaces of the framing/spacers facing the airspace. It is important to point out that the main reason for addressing this case is to explore the impact on R-value due to installing low-e foil or coating on the surfaces of the framing/spacers that face the airspace and are parallel to the heat-flow direction, which is of interest to reflective insulation manufacturers, building authorities and designers.
- Radiation takes place at the two ends of the airspace surfaces at E3 = 0.9 [24]. This case is called “with end effect”.
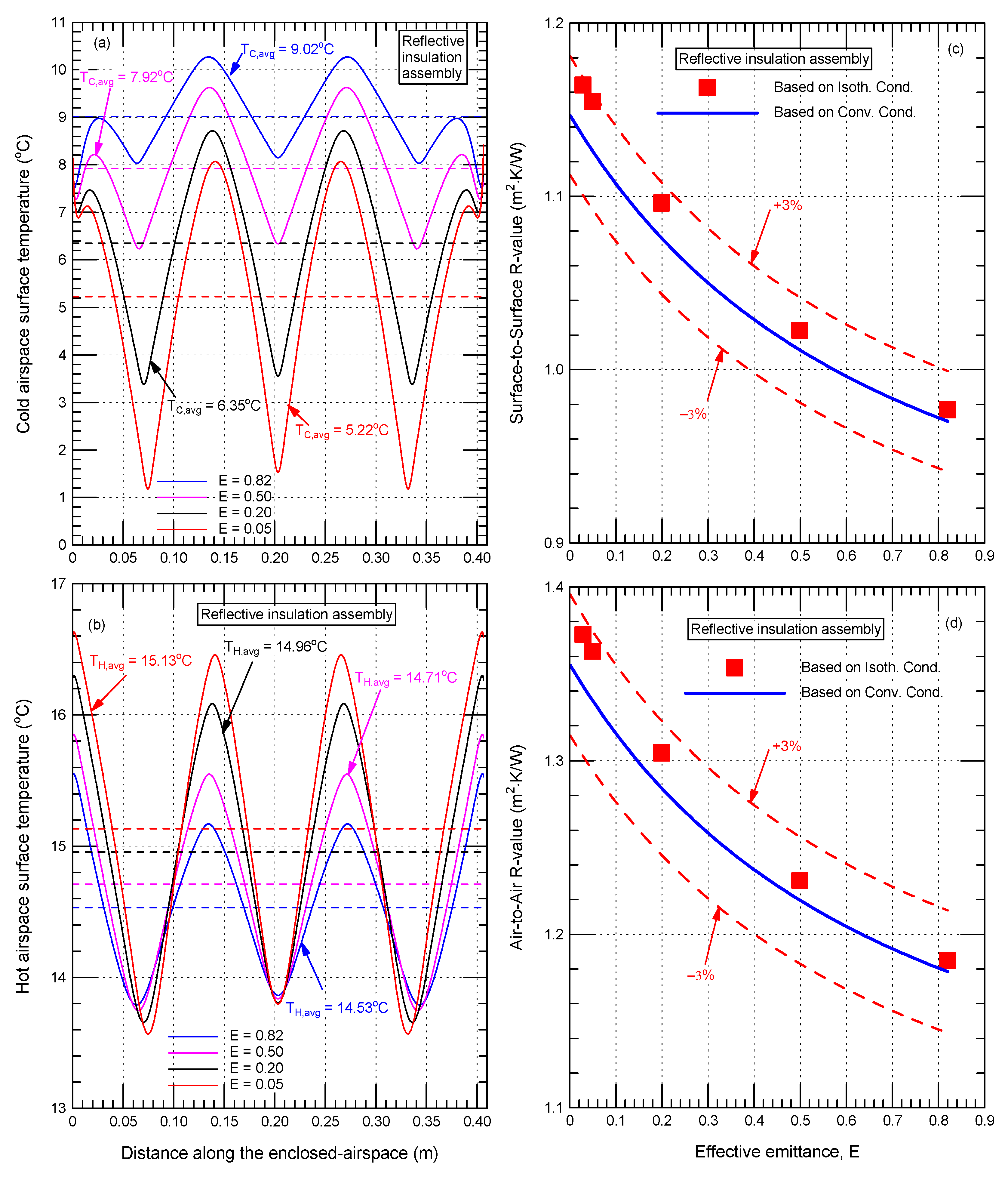
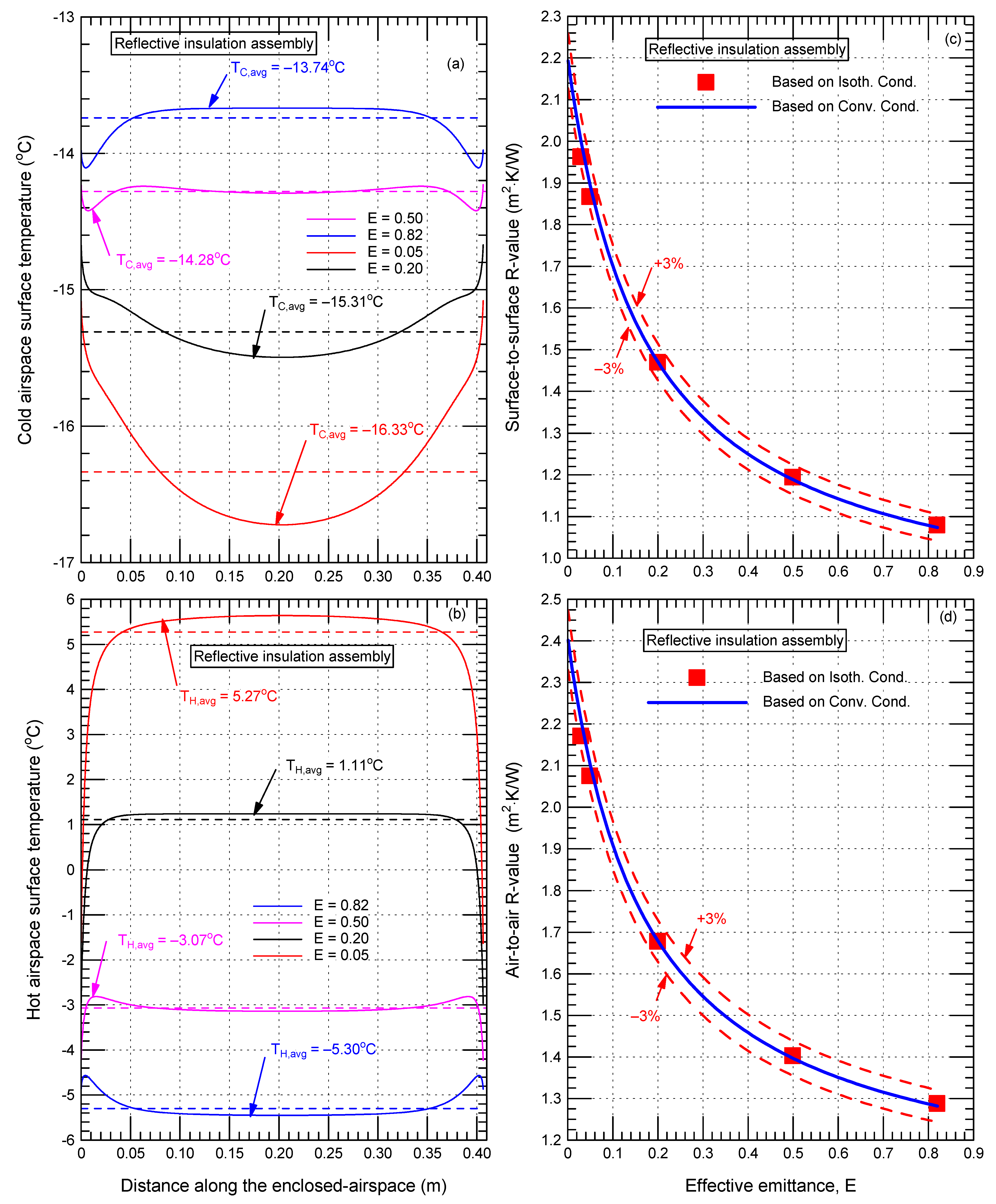
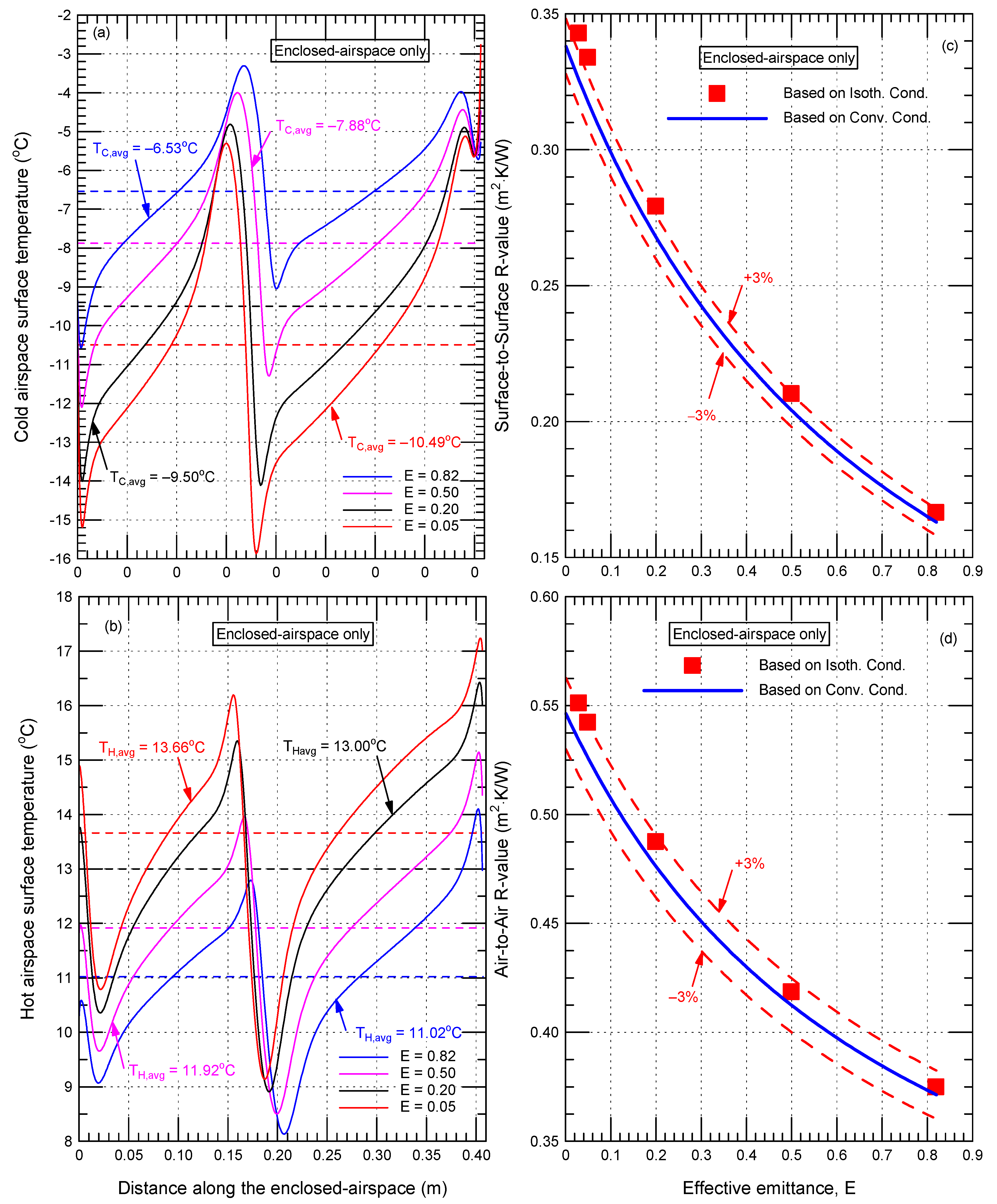
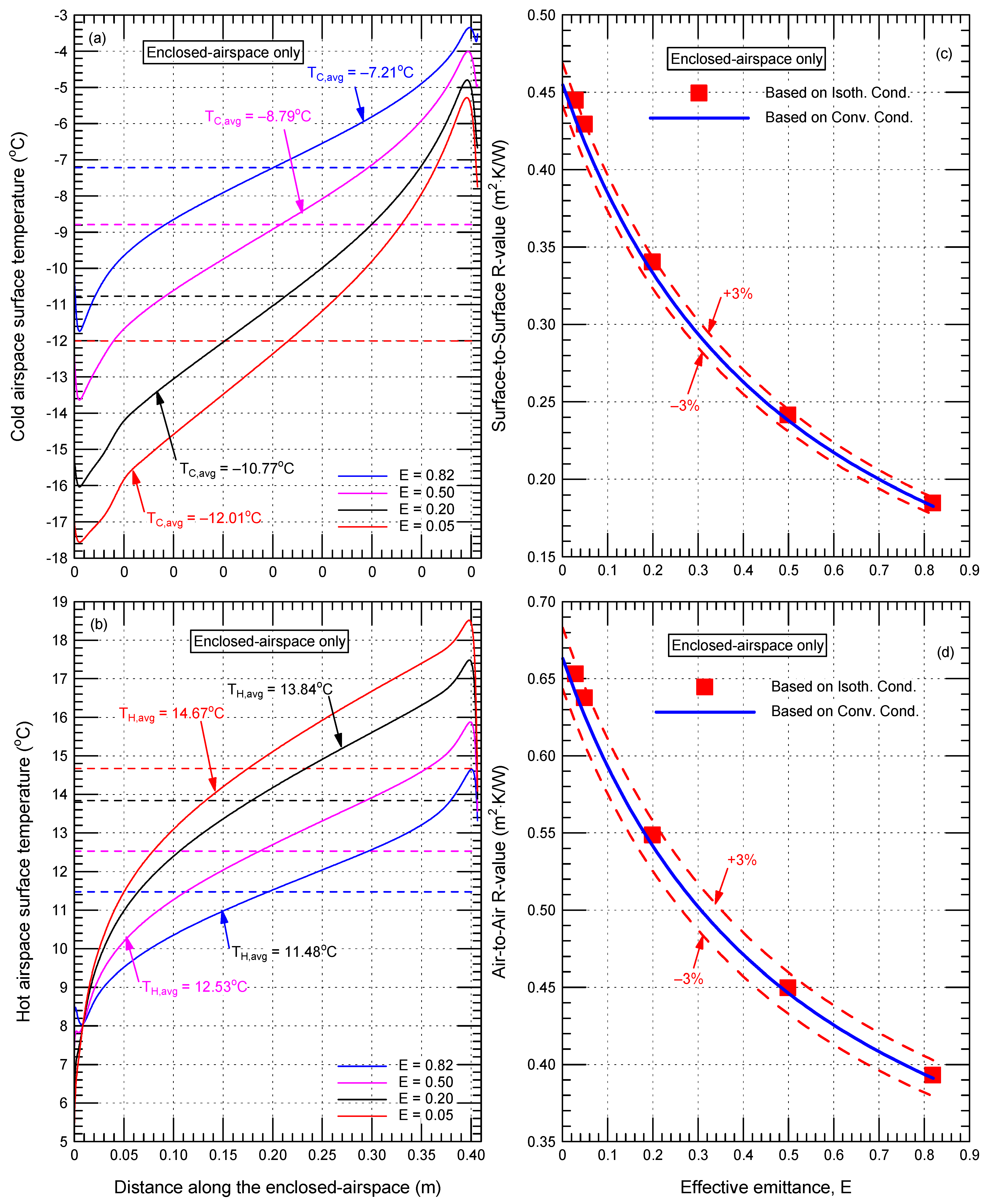
5.2. Test the Assumption of Isothermal Boundary Conditions
5.2.1. Upward Heat Flow for θ = 0°
5.2.2. Downward Heat Flow for θ = 0°
5.2.3. Horizontal Heat Flow for θ = 90°
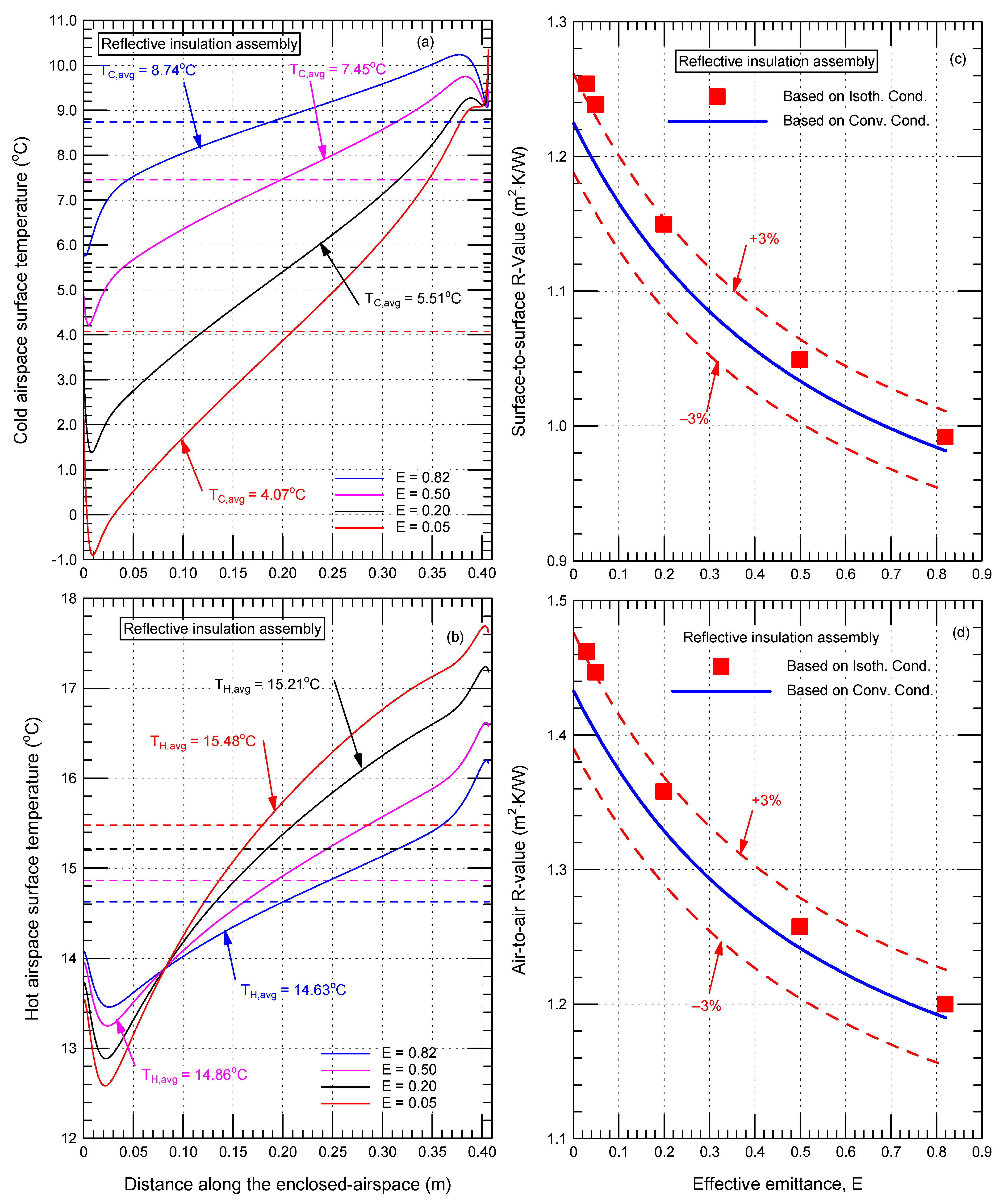
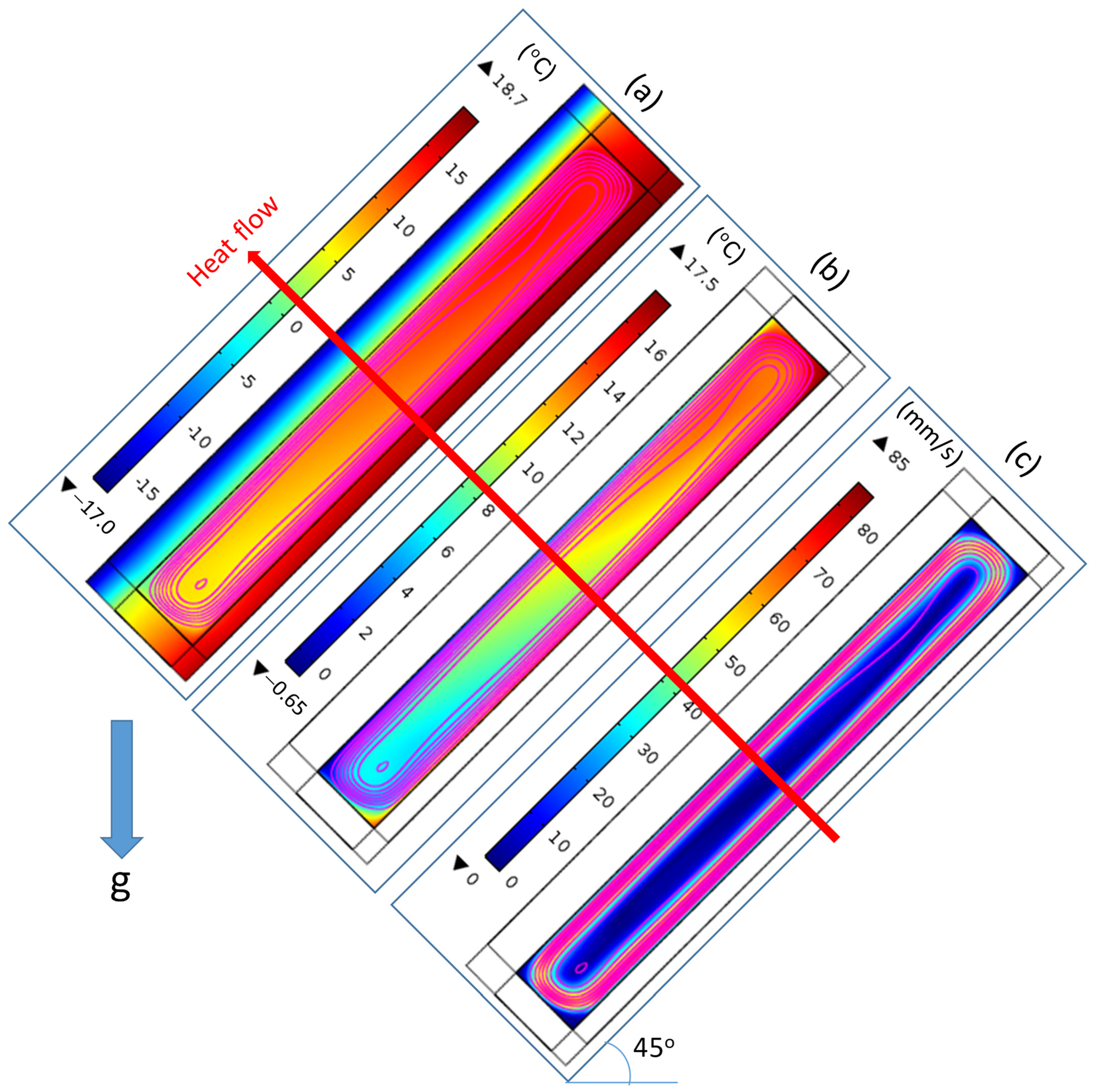
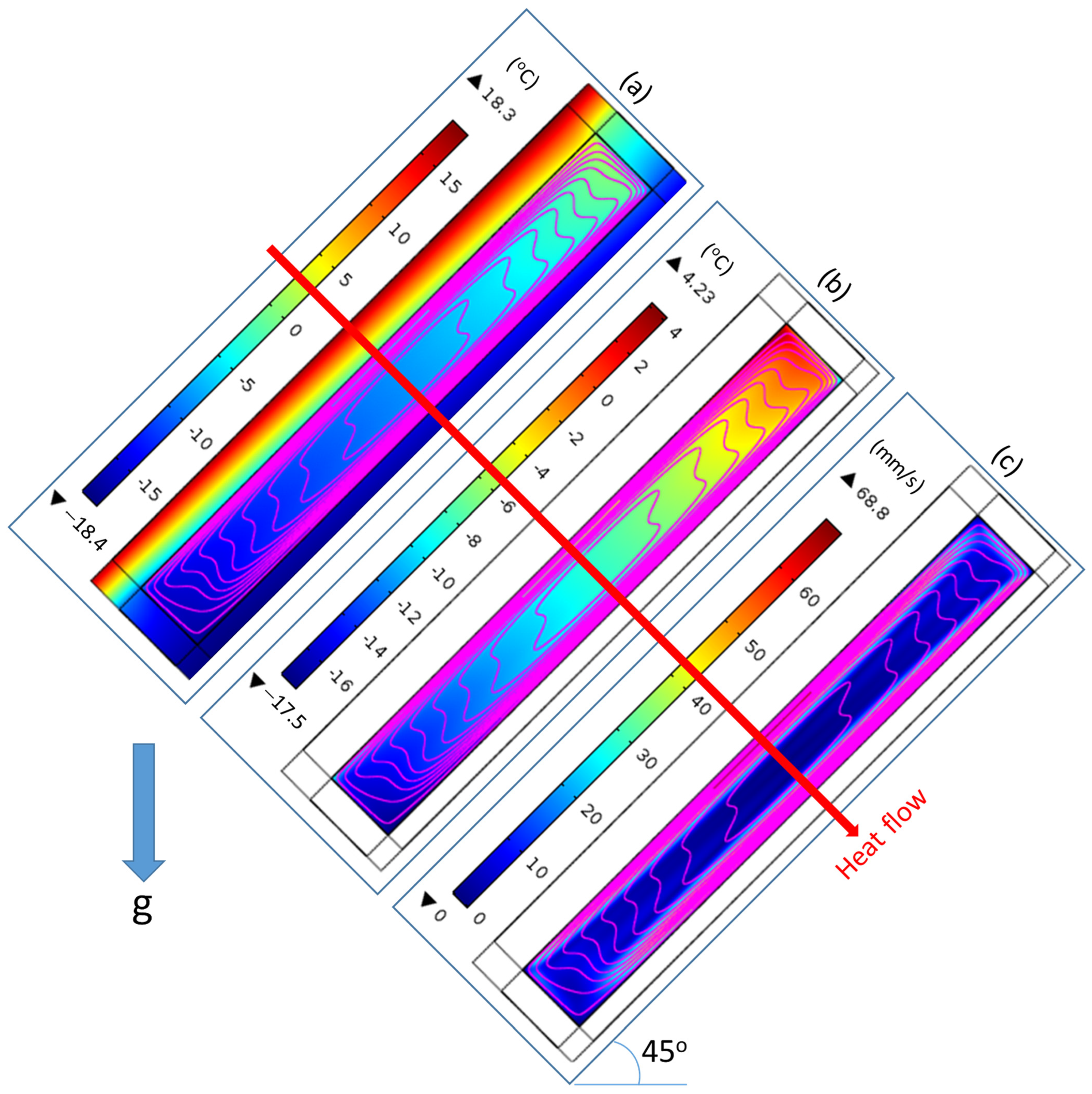
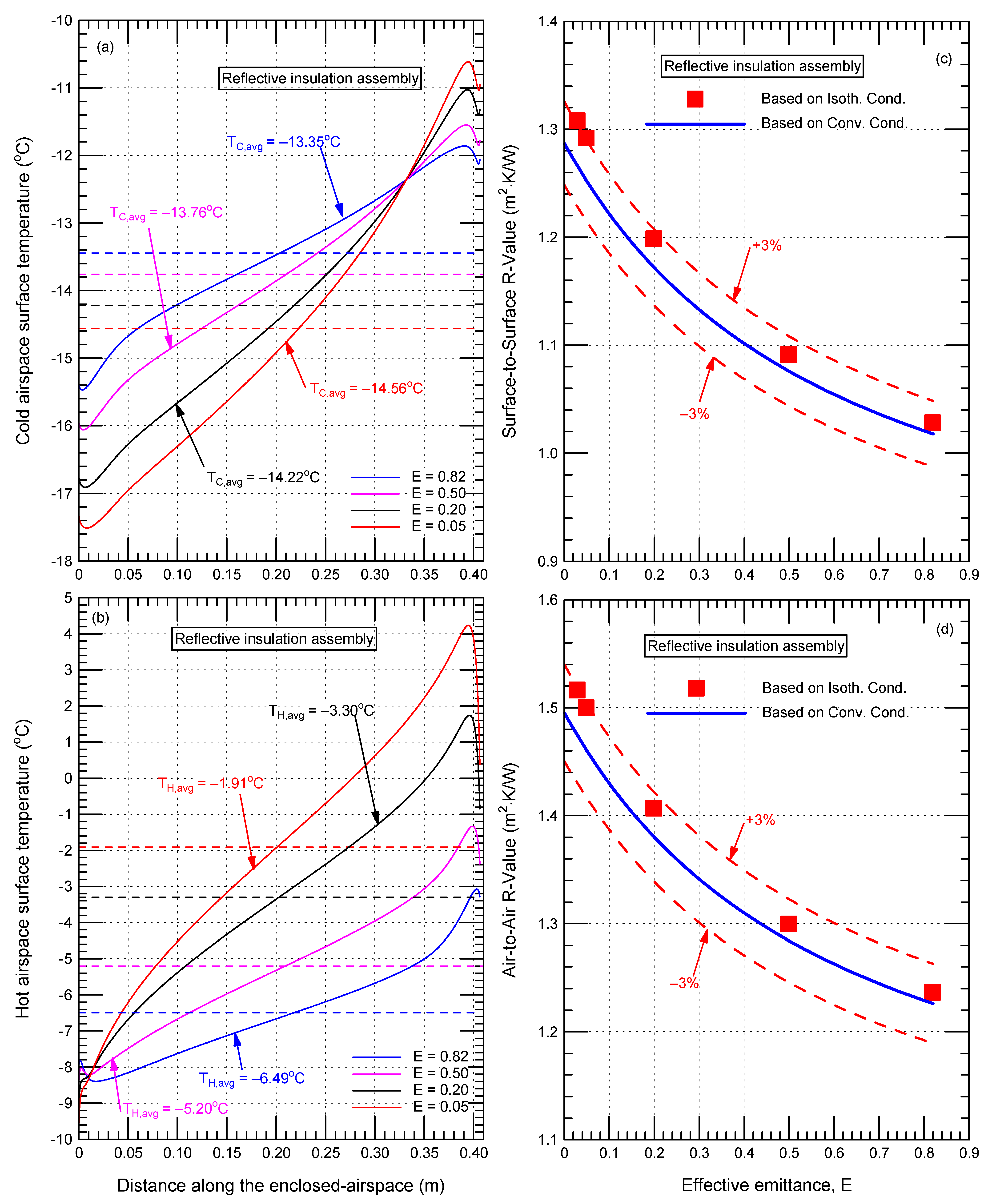
5.2.4. Upward and Downward Heat Flow and for Sloped Orientation for θ = 45°
5.3. Effect of Airspace Aspect Ratio on R-Value
6. Conclusions
- The percentage overestimates of the assembly surface-to-surface R-value increase as the heat-flow direction rotates from upward to downward heat flow. The overestimates of the assembly surface-to-surface R-value are below ~2.0% for heat flow from upward to horizontal directions. The percentage estimates increase significantly as heat flow approaches the downward direction, where radiation is dominant.
- The neglect of radiative exchange for heat-flow directions of 45° upward, horizontal, and 45° down impacts the reflective airspace R-values by less than 3% and can be neglected.
- The neglect of radiative exchange with airspace ends impacts the reflective airspace R-values by more than 10%. Consideration should be given to inclusion of radiative exchanges with the airspace ends (edges) for downward heat flow.
- The use of isothermal airspace surfaces results that are greater than the R-values obtained with convective boundary conditions by less than 3% for effective emittance range from 0 to 0.82 for upward heat flow, 45° up and horizontal. Additionally, the impact of the isothermal assumption for heat flow 45° down and down is negligible for the assemblies modeled.
- The isothermal assumption is valid for the type assemblies that were modeled in this research.
- The R-values obtained using numerical modeling increase as the aspect ratio increases.
- The R-values obtained using numerical modeling are lower than the one-dimensional results for aspect ratios less than seven.
- The R-values obtained using numerical modeling are greater than the one-dimensional R-values for aspect ratios greater than seven.
- The R-value results exceeds the one-dimensional values by as much as 40% as the aspect ratio approaches 27.
- The impact of the airspace aspect ratio should not be neglected when the thermal resistances of enclosed airspaces are evaluated.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Pratt, A.W. Heat Transmission in Buildings; John Wiley and Sons: New York, NY, USA, 1981; pp. 66–78. [Google Scholar]
- Wilkes, G.B. Reflective Insulation; John Wiley and Sons: New York, NY, USA, 1950; Chapter 6. [Google Scholar]
- Nash, G.D.; Comrie, J.; Broughton, H.F. The Thermal Insulation of Buildings-Design Data and How to Use; Her Majesty’s Stationary Office: London, UK, 1955. [Google Scholar]
- Hollingsworth, M., Jr. Thermal Testing of Reflective Insulation; ASTM STP 922; American Society for Testing and Materials: West Conshohocken, PA, USA, 1987; pp. 506–517. [Google Scholar]
- Lee, S.W.; Lim, C.; Salleh, E.B. Reflective thermal insulation systems in buildings: A review on radiant barrier and reflective insulation. Renew. Sustain. Energy Rev. 2016, 65, 643–661. [Google Scholar] [CrossRef]
- Tenpierik, M.J.; Hasselaar, E. Reflective multi-foil insulations for buildings: A review. J. Energy Build. 2013, 56, 233–343. [Google Scholar] [CrossRef]
- Fairey, P. The Measured Side-by-Side Performance of Attic Radiant Barrier Systems in Hot and Humid Climates; Thermal Conductivity 19; Plenum Press: New York, NY, USA, 1988; pp. 481–510. [Google Scholar]
- Craven, C.; Garber-Slaght, R. Product Test: Reflective Insulation in Cold Climates; Technical Report TR 2011-01; Cold Climate Research Center: Fairbanks, AK, USA, 2011. [Google Scholar]
- Goss, W.P.; Miller, R.G. Literature Review of Measurements and Predictions of Reflective Building Insulation Systems Performance: 1900–1986. ASHRAE Trans. 1989, 95, 651–664. [Google Scholar]
- Robinson, H.E.; Powlitch, F.J.; Dill, R.S. The Thermal Insulation Value of Airspaces; Housing Research Paper 32; Housing and Home Finance Agency: Washington, DC, USA; U.S. National Bureau of Standards: Gaithersburg, MD, USA, 1954. [Google Scholar]
- Robinson, H.E.; Powell, F.J. The Thermal Insulation Value of Air Spaces; Housing Research Paper 32 (HRP 32); National Bureau of Standards: Gaithersburg, MD, USA, 1956. [Google Scholar]
- Desjarlais, A.O.; Tye, R.P. Research and Development Data to Define the Thermal Performance of Reflective Materials Used to Conserve Energy in Building Applications; ORNL/Sub/88-SA835/1; U.S. Department of Energy Office of Scientific and Technical Information: Oak Ridge, TN, USA, 1990. [Google Scholar]
- Reflective Insulation Manufacturers Association International (RIMA-I). Reflective Insulation, Radiant Barriers and Radiation Control Coatings; RIMA-I: Olathe, KS, USA, 2002. [Google Scholar]
- Vrachopoulos, M.G.; Koukou, M.K.; Stavlas, D.G.; Stamatopoulos, V.N.; Gonidis, A.F.; Kravvaritis, E.D. Testing reflective insulation for improvement of buildings energy efficiency. Open Eng. 2012, 2, 83–90. [Google Scholar] [CrossRef]
- Escudero, C.; Martin, K.; Erkoreka, A.; Floresb, I.; Sala, J.M. Experimental thermal characterization of radiant barriers for building insulation. J. Energy Build. 2013, 59, 62–72. [Google Scholar] [CrossRef]
- Medina, M.A. On the performance of radiant barriers in combination with different attic insulation levels. J. Energy Build. 2000, 33, 31–40. [Google Scholar] [CrossRef]
- Miranville, F.; Fakra, A.H.; Guichard, S.; Boyer, H.; Praene, J.-P.; Bigot, D. Evaluation of the thermal resistance of a roof-mounted multi-reflective radiant barrier for tropical and humid conditions: Experimental study from field measurements. J. Energy Build. 2012, 48, 79–90. [Google Scholar] [CrossRef] [Green Version]
- D’Orazio, M.; Di Perna, C.; Di Giuseppe, E.; Morodo, M. Thermal performance of an insulated roof with reflective insulation: Field tests under hot climate conditions. J. Build. Phys. 2013, 36, 229–246. [Google Scholar] [CrossRef]
- Asadi, S.; Hassan, M.M. Evaluation of the thermal performance of a roof-mounted radiant barrier in residential buildings: Experimental study. J. Build. Phys. 2014, 38, 66–80. [Google Scholar] [CrossRef]
- Ficker, T. Numerical study of heat losses of building walls containing reflective foils. Indoor Build Environ. 2022, 31, 1932–1948. [Google Scholar] [CrossRef]
- Teh, K.S.; Yarbrough, D.W.; Lim, C.L.; Salleh, E. Field Evaluation of reflective insulation in southeast Asia. Open Eng. 2017, 7, 352–362. [Google Scholar] [CrossRef]
- IEA. Annex XII, International Energy Agency, Energy Conservation in Buildings and Community Systems Programme, Annex XII, Windows and Fenestration, Step 2, Thermal and Solar Properties of Windows, Expert Guide; IEA: Paris, France, 1987. [Google Scholar]
- ISO 6946:2017; Building Components and Building Elements-Thermal Resistance and Thermal Transmittance-Calculation Methods-Annex D-Thermal Resistance of Airspaces. International Organization for Standardization: Geneva, Switzerland, 2017.
- ASHRAE. Design Heat Transmission Coefficients. In ASHRAE Handbook of Fundamentals; ASHRAE: Peachtree Corners, GA, USA, 1972; Chapter 20. [Google Scholar]
- ASHRAE. Heat, Air, and Moisture Control in Building Assemblies-Material Properties. In ASHRAE Handbook of Fundamentals; ASHRAE: Peachtree Corners, GA, USA, 2009; Chapter 26. [Google Scholar]
- ASHRAE. Heat, Air, and Moisture Control in Building Assemblies-Material Properties. In ASHRAE Handbook of Fundamentals; ASHRAE: Peachtree Corners, GA, USA, 2017; Chapter 26, Table 3. [Google Scholar]
- Saber, H.H.; Alshehri, S.A.; Yarbrough, D.W. Innovative evaluation, optimization and design tool for assessing enclosed reflective airspace performance. In Proceedings of the 2022 Buildings XV International Conference on Thermal Performance of the Exterior Envelopes of Whole Buildings, Clearwater Beach, FL, USA, 5–8 December 2022. [Google Scholar]
- Saber, H.H. Overview of Thermal Performance of Air Cavities and Reflective Insulations. In Thermal Insulation and Radiation Control Technologies for Buildings; Kośny, J., Yarbrough, D.W., Eds.; ASIN: B0B3HN6W4J; Springer: Berlin/Heidelberg, Germany, 2022; Chapter 3; pp. 55–82. [Google Scholar]
- ASTM C1224; Standard Specification for Reflective Insulation for Building Applications. ASTM-International: West Conshohocken, PA, USA, 2021; Volume 04.06, pp. 706–710.
- ASTM C518; Standard Test Method for Steady-State Heat Flux Measurements and Thermal Transmission Properties by Means of the Heat Flow Meter Apparatus. ASTM-International: West Conshohocken, PA, USA, 2021; Volume 04.06, pp. 163–178.
- ASTM C1363; Standard Test Method for the Thermal Performance of Building Assemblies by Means of a Hot Box Apparatus. ASTM-International: West Conshohocken, PA, USA, 2021; Volume 04.06, pp. 792–836.
- Seigel, R.; Howell, J.R. Thermal Radiation Heat Transfer; McGraw-Hill Book Company: New York, NY, USA, 1972; pp. 240–241. [Google Scholar]
- Saber, H.H.; Yarbrough, D.W. Advanced modeling of enclosed-airspaces to determine thermal resistance for building applications. Energies 2021, 14, 7772. [Google Scholar] [CrossRef]
- Saber, H.H.; Yarbrough, D.W. Advancements in the evaluation of reflective insulation assemblies. Constr. Specif. 2022, 75, 20–27. [Google Scholar]
- Cook, J.C.; Yarbrough, D.W.; Wilkes, K.E. Contamination of reflective foils in horizontal applications and the effect on thermal performance. ASHRAE Trans. 1989, 95, 677–681. [Google Scholar]
- Bird, R.B.; Stewart, W.E.; Lightfoot, E.N. Transport Phenomena; John Wiley & Sons, Inc.: New York, NY, USA, 1960; pp. 446–447. [Google Scholar]





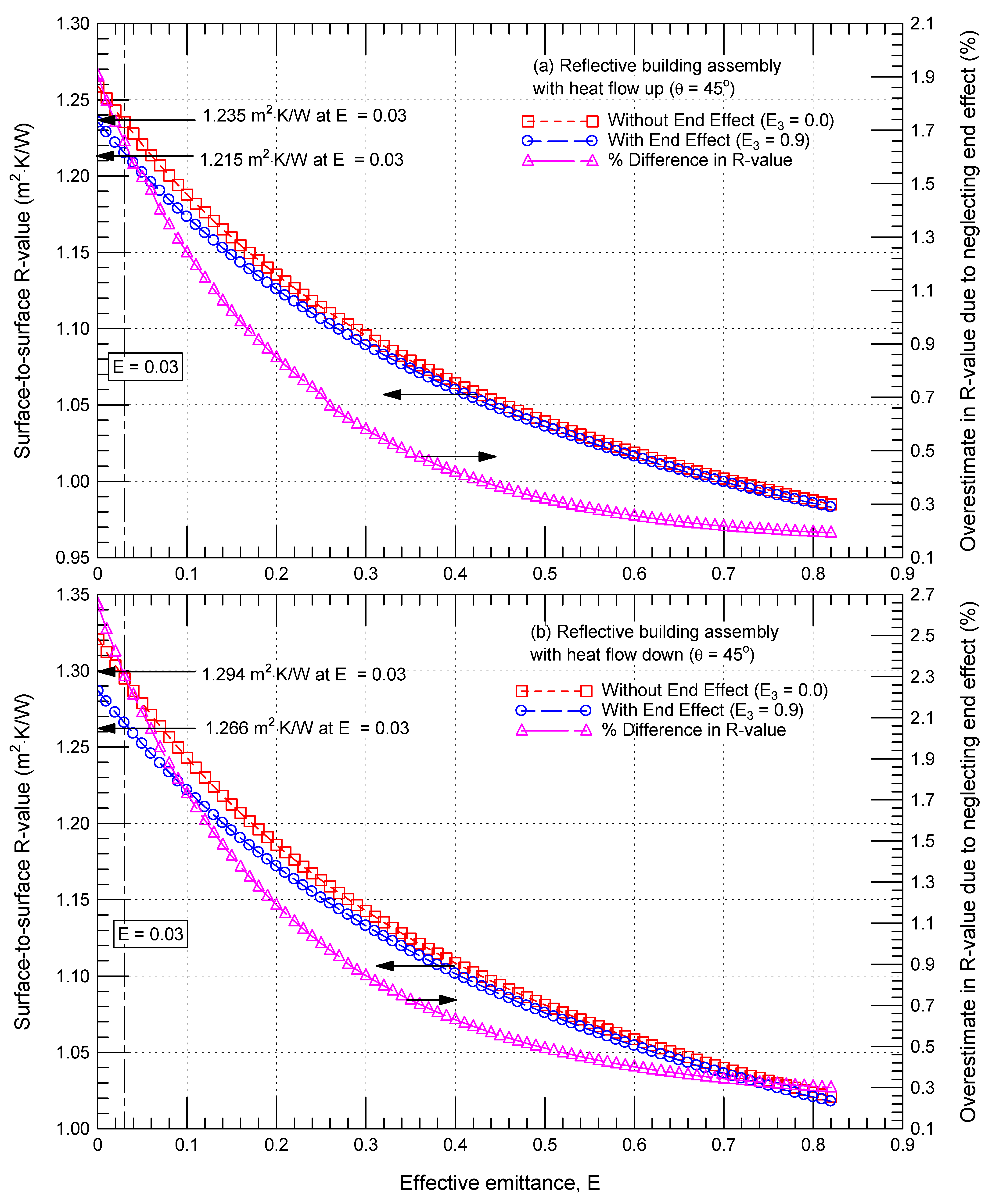








| Heat-Flow Direction | Associated Figure | Max. % Overestimate (E→0) |
|---|---|---|
| Up | Figure 6a | 2.0 |
| 45° Up | Figure 5a | 1.9 |
| Horizontal | Figure 4 | 1.9 |
| 45° Down | Figure 5b | 2.7 |
| Down | Figure 6b | 13.3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Saber, H.H.; Yarbrough, D.W. Determining the Thermal Resistance of Enclosed Reflective Airspace. Buildings 2023, 13, 662. https://doi.org/10.3390/buildings13030662
Saber HH, Yarbrough DW. Determining the Thermal Resistance of Enclosed Reflective Airspace. Buildings. 2023; 13(3):662. https://doi.org/10.3390/buildings13030662
Chicago/Turabian StyleSaber, Hamed H., and David W. Yarbrough. 2023. "Determining the Thermal Resistance of Enclosed Reflective Airspace" Buildings 13, no. 3: 662. https://doi.org/10.3390/buildings13030662
APA StyleSaber, H. H., & Yarbrough, D. W. (2023). Determining the Thermal Resistance of Enclosed Reflective Airspace. Buildings, 13(3), 662. https://doi.org/10.3390/buildings13030662






