Elastic Buckling Behavior of Functionally Graded Material Thin Skew Plates with Circular Openings
Abstract
:1. Introduction
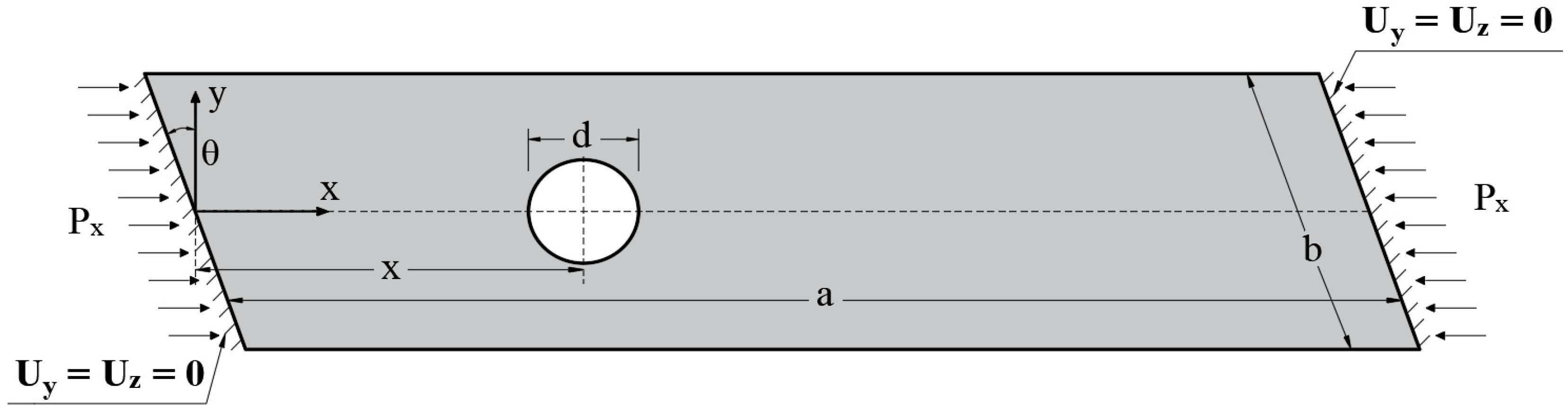
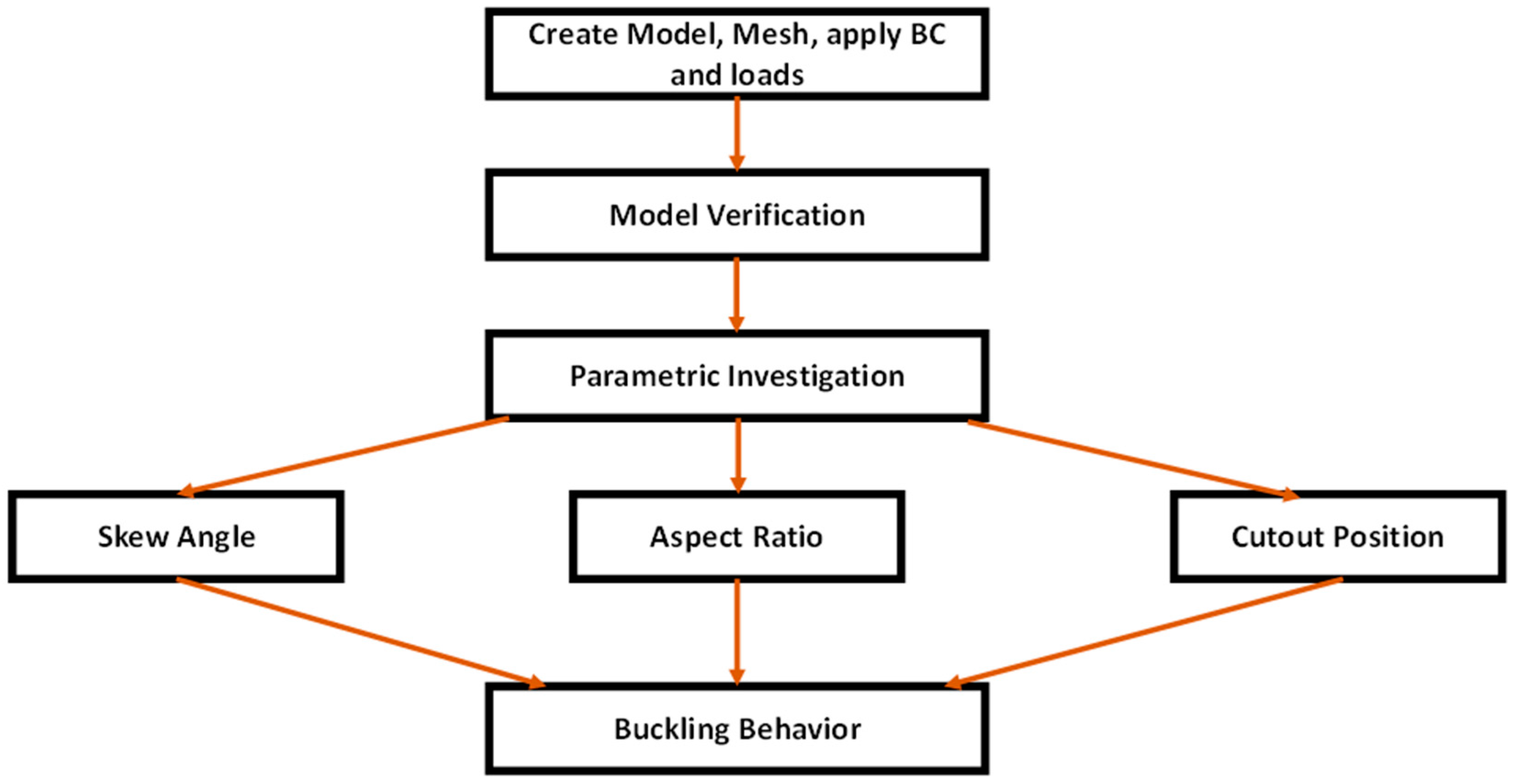
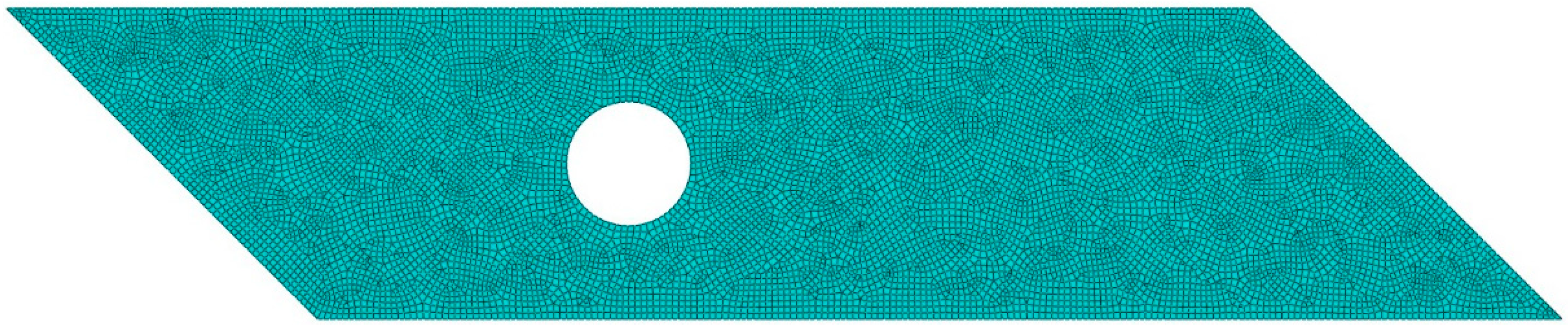
2. Materials and Methods
3. Results and Discussion
3.1. Model Verification
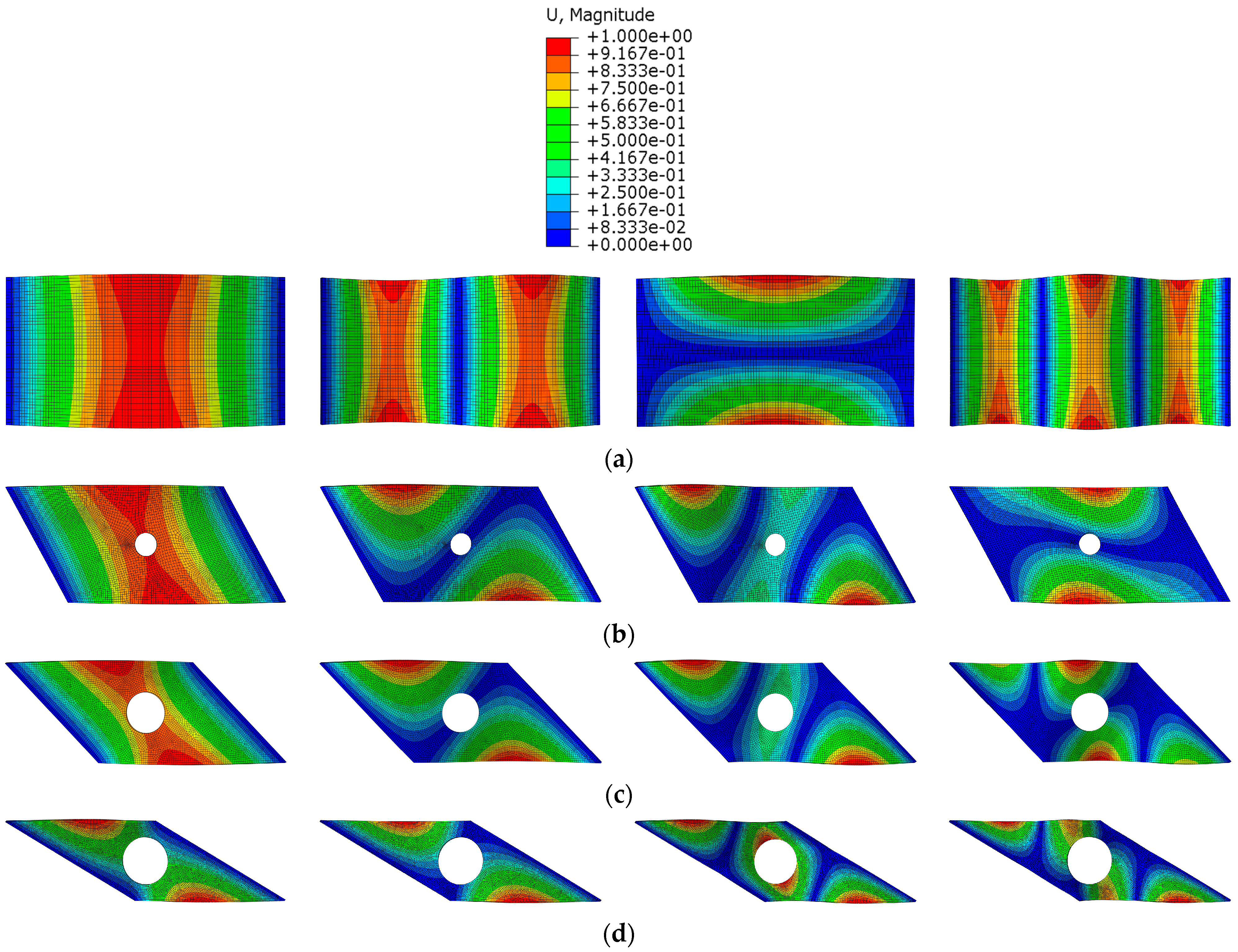
3.2. Parametric Investigation
4. Conclusions
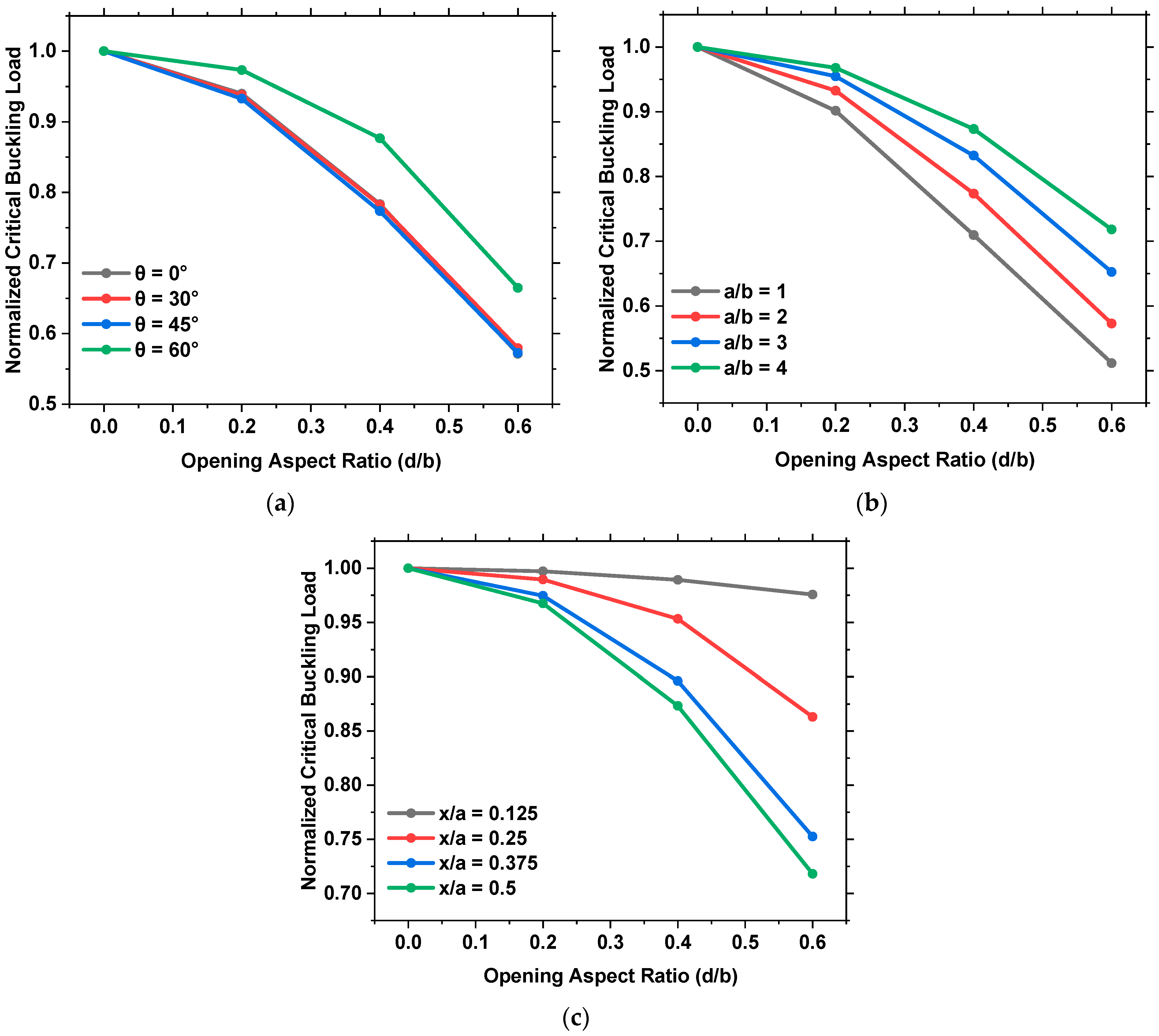
- The presence of a cutout and the increase in its size decreases the critical buckling load of the plate.
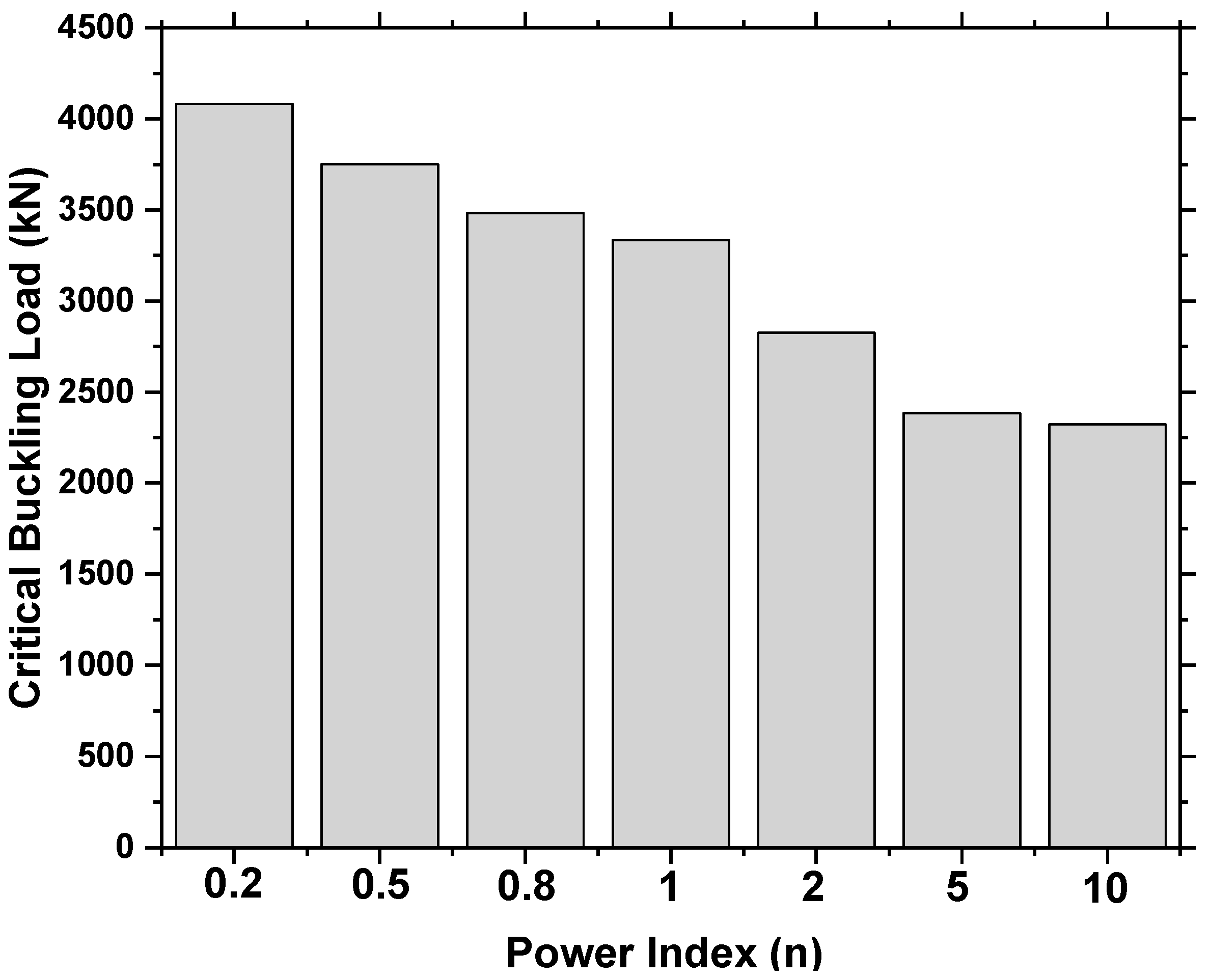
- The power index inversely affects the modulus of elasticity of the FGM, and in turn the critical buckling load of the FGM plate. An increase in the power index reduces the critical bucking load. For instance, the critical buckling decreased by 1759 kN when the power index increased from 0.2 to 10.
- Thin plates with skew angle of 60° obtained higher critical buckling loads by 206%, 134%, and 65%, compared to plates with skew angles of 0°, 30°, and 45°, respectively.
- An inversely proportional relationship between the critical buckling load and the aspect ratios of the plate is present.
- The position of the opening also affects the buckling load, as an opening closer to the plate’s edge showed a higher buckling load than that close to the centre of the plate.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Saleh, B.; Jiang, J.; Fathi, R.; Al-Hababi, T.; Xu, Q.; Wang, L.; Song, D.; Ma, A. 30 Years of functionally graded materials: An overview of manufacturing methods, Applications and Future Challenges. Compos. Part B Eng. 2020, 201, 108376. [Google Scholar] [CrossRef]
- Saffari, P.R.; Sirimontree, S.; Thongchom, C.; Jearsiripongkul, T.; Saffari, P.R.; Keawsawasvong, S. Effect of uniform and nonuniform temperature distributions on sound transmission loss of double-walled porous functionally graded magneto-electro-elastic sandwich plates with subsonic external flow. Int. J. Thermofluids 2023, 17, 100311. [Google Scholar] [CrossRef]
- Elishakoff, I.; Eisenberger, M.; Delmas, A. Buckling and Vibration of Functionally Graded Material Columns Sharing Duncan’s Mode Shape, and New Cases. Structures 2016, 5, 170–174. [Google Scholar] [CrossRef]
- Zhang, X.; Wang, Y.; Foster, A.; Su, M. Elastic local buckling behavior of beetle elytron plate. Thin-Walled Struct. 2021, 165, 107922. [Google Scholar] [CrossRef]
- Fieber, A.; Gardner, L.; Macorini, L. Formulae for determining elastic local buckling half-wavelengths of structural steel cross-sections. J. Constr. Steel Res. 2019, 159, 493–506. [Google Scholar] [CrossRef]
- Peng, C.; Tran, P.; Mouritz, A.P. Compression and buckling analysis of 3D printed carbon fibre-reinforced polymer cellular composite structures. Compos. Struct. 2022, 300, 116167. [Google Scholar] [CrossRef]
- Khoram-Nejad, E.S.; Moradi, S.; Shishehsaz, M. Effect of crack characteristics on the vibration behavior of post-buckled functionally graded plates. Structures 2023, 50, 181–199. [Google Scholar] [CrossRef]
- Moita, J.S.; Araújo, A.L.; Correia, V.F.; Soares, C.M.M. Mechanical and thermal buckling of functionally graded axisymmetric shells. Compos. Struct. 2021, 261, 113318. [Google Scholar] [CrossRef]
- Hosseini, S.M.H.; Arvin, H. Thermo-rotational buckling and post-buckling analyses of rotating functionally graded microbeams. Int. J. Mech. Mater. Des. 2021, 17, 55–72. [Google Scholar] [CrossRef]
- Zenkour, A.M.; Aljadani, M.H. Buckling analysis of actuated functionally graded piezoelectric plates via a quasi-3D refined theory. Mech. Mater. 2020, 151, 103632. [Google Scholar] [CrossRef]
- Kumar, V.; Singh, S.; Saran, V.; Harsha, S. Effect of elastic foundation and porosity on buckling response of linearly varying functionally graded material plate. Structures 2023, 55, 1186–1203. [Google Scholar] [CrossRef]
- Khalil, A.E.-H.; Etman, E.; Atta, A.; Essam, M. Nonlinear behavior of RC beams strengthened with strain hardening cementitious composites subjected to monotonic and cyclic loads. Alex. Eng. J. 2016, 55, 1483–1496. [Google Scholar] [CrossRef]
- AlHamaydeh, M.; Elkafrawy, M.; Banu, S. Seismic Performance and Cost Analysis of UHPC Tall Buildings in UAE with Ductile Coupled Shear Walls. Materials 2022, 15, 2888. [Google Scholar] [CrossRef]
- Khalil, A.; Elkafrawy, M.; Abuzaid, W.; Hawileh, R.; AlHamaydeh, M. Flexural Performance of RC Beams Strengthened with Pre-Stressed Iron-Based Shape Memory Alloy (Fe-SMA) Bars: Numerical Study. Buildings 2022, 12, 2228. [Google Scholar] [CrossRef]
- Sitli, Y.; Mhada, K.; Bourihane, O.; Rhanim, H. Buckling and post-buckling analysis of a functionally graded material (FGM) plate by the Asymptotic Numerical Method. Structures 2021, 31, 1031–1040. [Google Scholar] [CrossRef]
- Dhuria, M.; Grover, N.; Goyal, K. Influence of porosity distribution on static and buckling responses of porous functionally graded plates. Structures 2021, 34, 1458–1474. [Google Scholar] [CrossRef]
- Van Vinh, P.; Van Chinh, N.; Tounsi, A. Static bending and buckling analysis of bi-directional functionally graded porous plates using an improved first-order shear deformation theory and FEM. Eur. J. Mech. A/Solids 2022, 96, 104743. [Google Scholar] [CrossRef]
- Jamali, M.; Shojaee, T.; Mohammadi, B.; Kolahchi, R. Cut out effect on nonlinear post-buckling behavior of FG-CNTRC micro plate subjected to magnetic field via FSDT. Adv. Nano Res. 2019, 7, 405–417. [Google Scholar] [CrossRef]
- Le Thanh, C.; Nguyen, T.N.; Vu, T.H.; Khatir, S.; Wahab, M.A. A geometrically nonlinear size-dependent hypothesis for porous functionally graded micro-plate. Eng. Comput. 2022, 38, 449–460. [Google Scholar] [CrossRef]
- Prabowo, A.R.; Ridwan, R.; Muttaqie, T. On the Resistance to Buckling Loads of Idealized Hull Structures: FE Analysis on Designed-Stiffened Plates. Designs 2022, 6, 46. [Google Scholar] [CrossRef]
- Alashkar, A.; Elkafrawy, M.; Hawileh, R.; AlHamaydeh, M. Buckling Analysis of Functionally Graded Materials (FGM) Thin Plates with Various Circular Cutout Arrangements. J. Compos. Sci. 2022, 6, 277. [Google Scholar] [CrossRef]
- Elkafrawy, M.; Alashkar, A.; Hawileh, R.; AlHamaydeh, M. FEA Investigation of Elastic Buckling for Functionally Graded Material (FGM) Thin Plates with Different Hole Shapes under Uniaxial Loading. Buildings 2022, 12, 802. [Google Scholar] [CrossRef]
- Kiani, Y.; Żur, K.K. Free vibrations of graphene platelet reinforced composite skew plates resting on point supports. Thin-Walled Struct. 2022, 176, 109363. [Google Scholar] [CrossRef]
- Kiani, Y.; Mirzaei, M. Rectangular and skew shear buckling of FG-CNT reinforced composite skew plates using Ritz method. Aerosp. Sci. Technol. 2018, 77, 388–398. [Google Scholar] [CrossRef]
- Zhu, J.; Fang, Z.; Liu, X.; Zhang, J.; Kiani, Y. Vibration characteristics of skew sandwich plates with functionally graded metal foam core. Structures 2023, 55, 370–378. [Google Scholar] [CrossRef]
- Civalek, O.; Jalaei, M.H. Buckling of carbon nanotube (CNT)-reinforced composite skew plates by the discrete singular convolution method. Acta Mech. 2020, 231, 2565–2587. [Google Scholar] [CrossRef]
- Duan, Y.; Zhang, B.; Zhang, X.; Zhang, L.; Shen, H. Accurate mechanical buckling analysis of couple stress-based skew thick microplates. Aerosp. Sci. Technol. 2023, 132, 108056. [Google Scholar] [CrossRef]
- Singh, J.; Prasad, R.B. Vibration and Buckling Analysis of Skew Sandwich Plate using Radial Basis Collocation Method. Mech. Adv. Compos. Struct. 2019, 10, 383–392. [Google Scholar] [CrossRef]
- Tahmasebi Nejad, A.; Shanmugam, N.E. Elastic buckling of uniaxially loaded skew plates containing openings. Thin-Walled Struct. 2011, 49, 1208–1216. [Google Scholar] [CrossRef]
- Yuan, Y.; Zhao, K.; Sahmani, S.; Safaei, B. Size-dependent shear buckling response of FGM skew nanoplates modeled via different homogenization schemes. Appl. Math. Mech. 2020, 41, 587–604. [Google Scholar] [CrossRef]
- Shahrestani, M.G.; Azhari, M.; Foroughi, H. Elastic and inelastic buckling of square and skew FGM plates with cutout resting on elastic foundation using isoparametric spline finite strip method. Acta Mech. 2018, 229, 2079–2096. [Google Scholar] [CrossRef]
- Systems, D. Abaqus: Finite Element Analysis for Mechanical Engineering and Civil Engineering. [Online]. Available online: https://www.3ds.com/products-services/simulia/products/abaqus/.
- Ali, E.Y.; Bayleyegn, Y.S. Analytical and numerical buckling analysis of rectangular functionally-graded plates under uniaxial compression. In Proceedings of the Structural Stability Research Council Annual Stability Conference 2019, SSRC 2019, St. Louis, MO, USA, 2–5 April 2019; Volume 2, pp. 534–547. [Google Scholar]
- Shariat, B.A.S.; Javaheri, R.; Eslami, M.R. Buckling of imperfect functionally graded plates under in-plane compressive loading. Thin-Walled Struct. 2005, 43, 1020–1036. [Google Scholar] [CrossRef]
- Chi, S.-H.; Chung, Y.-L. Mechanical behavior of functionally graded material plates under transverse load—Part I: Analysis. Int. J. Solids Struct. 2006, 43, 3657–3674. [Google Scholar] [CrossRef]


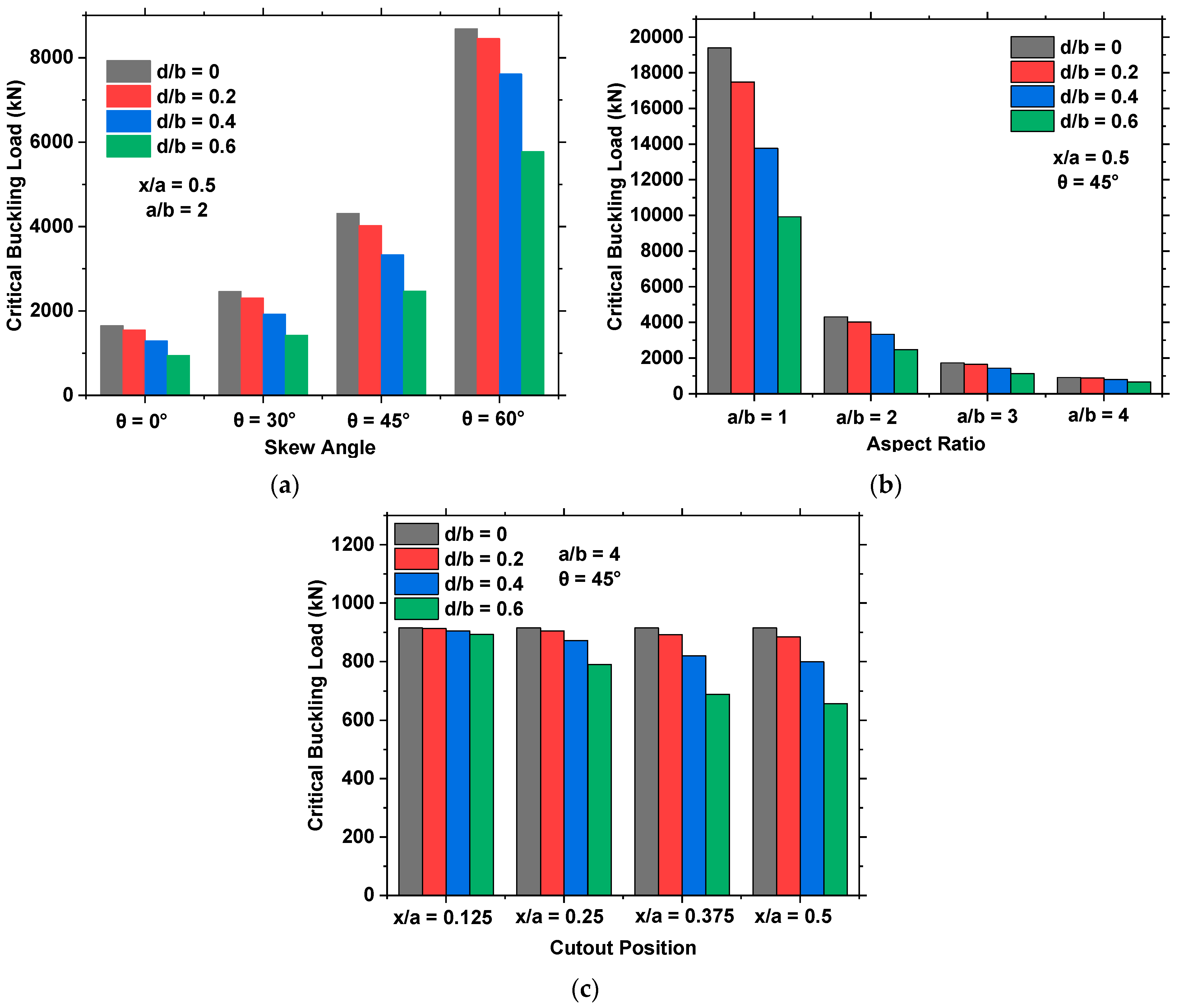


| Aspect Ratio (a/b) | Opening Position (x/a) | Opening Size (d/b) | Skew Angle (θ°) | Critical Buckling Load (kN) | |
|---|---|---|---|---|---|
| Control | 2 | 0 | 0 | 0 | 1652 |
| 30 | 2461 | ||||
| 45 | 4311 | ||||
| 60 | 8683 | ||||
| Small Opening | 2 | 0.5 | 0.2 | 0 | 1552 |
| 30 | 2308 | ||||
| 45 | 4021 | ||||
| 60 | 8450 | ||||
| Medium Opening | 2 | 0.5 | 0.4 | 0 | 1294 |
| 30 | 1926 | ||||
| 45 | 3335 | ||||
| 60 | 7613 | ||||
| Large Opening | 2 | 0.5 | 0.6 | 0 | 944 |
| 30 | 1426 | ||||
| 45 | 2469 | ||||
| 60 | 5772 |
| Skew Angle (θ°) | Opening Position (x/a) | Opening Size (d/b) | Aspect Ratio (a/b) | Critical Buckling Load (kN) | |
|---|---|---|---|---|---|
| Control | 45 | 0 | 0 | 1 | 19,392 |
| 2 | 4311 | ||||
| 3 | 1728 | ||||
| 4 | 915 | ||||
| Small Opening | 45 | 0.5 | 0.2 | 1 | 17,482 |
| 2 | 4021 | ||||
| 3 | 1650 | ||||
| 4 | 885 | ||||
| Medium Opening | 45 | 0.5 | 0.4 | 1 | 13,758 |
| 2 | 3335 | ||||
| 3 | 1438 | ||||
| 4 | 799 | ||||
| Large Opening | 45 | 0.5 | 0.6 | 1 | 9922 |
| 2 | 2469 | ||||
| 3 | 1128 | ||||
| 4 | 657 |
| Skew Angle (θ°) | Aspect Ratio (a/b) | Opening Size (d/b) | Opening Position (x/a) | Critical Buckling Load (kN) | |
|---|---|---|---|---|---|
| Control | 45 | 4 | 0 | 0 | 915 |
| Small Opening | 45 | 4 | 0.2 | 0.125 | 913 |
| 0.250 | 905 | ||||
| 0.375 | 892 | ||||
| 0.500 | 885 | ||||
| Medium Opening | 45 | 4 | 0.4 | 0.125 | 905 |
| 0.250 | 872 | ||||
| 0.375 | 820 | ||||
| 0.500 | 799 | ||||
| Large Opening | 45 | 4 | 0.6 | 0.125 | 893 |
| 0.250 | 790 | ||||
| 0.375 | 689 | ||||
| 0.500 | 657 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alashkar, A.; Elkafrawy, M.; Hawileh, R.; AlHamaydeh, M. Elastic Buckling Behavior of Functionally Graded Material Thin Skew Plates with Circular Openings. Buildings 2024, 14, 572. https://doi.org/10.3390/buildings14030572
Alashkar A, Elkafrawy M, Hawileh R, AlHamaydeh M. Elastic Buckling Behavior of Functionally Graded Material Thin Skew Plates with Circular Openings. Buildings. 2024; 14(3):572. https://doi.org/10.3390/buildings14030572
Chicago/Turabian StyleAlashkar, Adnan, Mohamed Elkafrawy, Rami Hawileh, and Mohammad AlHamaydeh. 2024. "Elastic Buckling Behavior of Functionally Graded Material Thin Skew Plates with Circular Openings" Buildings 14, no. 3: 572. https://doi.org/10.3390/buildings14030572
APA StyleAlashkar, A., Elkafrawy, M., Hawileh, R., & AlHamaydeh, M. (2024). Elastic Buckling Behavior of Functionally Graded Material Thin Skew Plates with Circular Openings. Buildings, 14(3), 572. https://doi.org/10.3390/buildings14030572










