Study on the Damping Efficiency of a Structure with Additional Viscous Dampers Based on the Shaking Table Test

Abstract

This study specifically focuses on the damping efficiency of a damped structure with additional viscous dampers. A two-layer steel frame structure with eight sets of viscous dampers is used to conduct a series of seismic simulation shaking table tests, including a non-damped structure without dampers and two damped structures with dampers placed at 1/2 and 1/6 of the beam span, respectively. By conducting these tests, the energy dissipation, force, and displacement of the damper, as well as the parameters of the structure such as floor displacement and acceleration, are obtained. The main damping efficiency indicators of the damped structure are calculated, including the additional damping ratio, inter-story displacement utilization rate, as well as the reduction rate of the vertex displacement and the base shear relative to the non-damped structure. The study shows that the viscous dampers exhibit full hysteresis loops and a strong energy dissipation capacity in the structure. The seismic response of the vertex displacement and base shear in the damped structure is significantly smaller than that in the non-damped structure. Under different seismic levels, including frequent earthquakes, occasional earthquakes, and rare earthquakes, the damping effect of the dampers placed at 1/2 of the beam span is significantly better than that placed at 1/6 of the beam span. For example, the additional damping ratio for the X-direction artificial wave REN is 19% and 11%, 20% and 13%, and 13% and 11%, respectively. The patterns for inter-story displacement utilization ratio, reduction rate of the vertex displacement, and reduction rate of the base shear are similar. The research findings strongly indicate that the damped structure with additional viscous dampers exhibits excellent damping efficiency. In future damping design, designers need to fully consider the placement of viscous dampers within the beam span.

1. Introduction

Energy dissipation structures are designed to reduce the impact of seismic or other external forces on a building by using a series of energy dissipation systems. These systems can absorb and dissipate energy, effectively reducing the vibration response of the structure and preventing or minimizing structural damage [1,2]. There are four main types of energy dissipation control: active, semi-active, passive, and intelligent [3,4]. Passive control devices are further classified into velocity-dependent dampers, displacement-dependent dampers, and hybrid dampers based on their relationship with velocity and displacement. They can also be categorized as rate-dependent or rate-independent dampers based on their dependence on velocity [5,6]. Viscous dampers [7,8,9] are the most commonly used velocity-based dissipative devices that utilize the damping characteristics of viscous materials to reduce structural vibration response. They generate a damping force when subjected to external forces, which can absorb and dissipate energy. Compared to other types of dampers, viscous dampers have several advantages, including a higher additional damping ratio, stable and reliable damping characteristics within the frequency response range, and relatively simple structures resulting in low installation and maintenance costs. As a result, viscous dampers have been widely applied in practical engineering. There are various installation methods for viscous dampers in damped structures, including supported and cantilever wall types. The supported type includes single diagonal support, V-shaped support, and inverted V-shaped support. However, from the perspective of functional use in building structures, designers and construction units prefer viscous dampers arranged in a cantilever wall type as they do not obstruct doors and windows and they minimize the impact on the building’s functionality.

To optimize the implementation of viscous dampers in damped structures, numerous researchers have conducted thorough studies on the damping efficacy of various types of damped structures. Hwang et al. [10] proposed a new calculation formula to accurately predict the additional damping ratio of mid-high-rise buildings. Xie et al. [11] studied the effectiveness and optimization design of non-linear viscous dampers in inelastic structures. Gherbi et al. [12] minimized the design time by estimating the damping coefficient of non-linear fluid viscous dampers. The calculation methods for the additional damping ratio have been a hot topic of research. There are non-dimensional parameter methods based on non-linear viscous dampers [13], simplified calculation methods based on structural characteristics, excitation frequency, and damper parameters [14], as well as non-classical modal damping ratio calculation methods based on the known state-space representation of the dynamic system [15]. The seismic performance of structures with additional viscous dampers has been significantly improved [16]; therefore, viscous dampers have also been applied in steel structures [17], super-tall building structures [18], and even in structural retrofitting [19,20].

As is well known, seismic excitation is essentially a random vibration. Tubaldi et al. [21,22] investigated the probabilistic response assessment and non-stationary stochastic response of structures. Zhang et al. [23] proposed a structural analysis method based on Lyapunov differential equations. Su et al. [24] explored the optimal design of non-linear viscous dampers for large-scale structures under non-stationary seismic excitation, employing a stochastic approach. Rodolfo et al. [25] conducted a probabilistic evaluation of high-rise buildings’ dynamic response using transfer functions. Experimental research on the damped structures can be conducted using pseudo-static tests [26]. Alternatively, a more direct approach is to utilize a seismic simulation shaking table for dynamic testing [27,28]. Nevertheless, there have been only a few shaking table tests that have specifically focused on the damped structure with additional viscous dampers and the non-damped structure without any additional viscous dampers.

Scholars have also conducted extensive research on the damping efficiency of optimal control and the most effective placement of dampers in damped structures. Singh et al. [29] suggested a gradient optimization technique, utilizing inter-story displacement, base shear, or floor acceleration as performance indicators. Aguirre et al. [30] evaluated and compared the optimal distribution of spatial dampers for linear and non-linear systems. Parcianello et al. [31] studied the placement of dampers aiming to reduce the displacement and acceleration of frame structures under seismic action. Whittle et al. [32] investigated the impact of five distinct configurations of viscous dampers on the seismic performance of structures. In order to achieve relatively uniform parameters for each damper size in the structure, Lopez [33] proposed a simplified algorithm based on sequential search. Takewaki [34,35], Lin et al. [36], and Aydin et al. [37] conducted research on the optimal placement of dampers considering the minimum transfer function, critical excitation, damping coefficients, and seismic rehabilitation. However, no studies have been found on the damping efficiency of damped structures when dampers are placed at different positions within the same beam span.

In the field of engineering, it is common to reveal the laws of things through the in-depth study of specific phenomena. This process usually begins with observing the phenomena, followed by establishing the corresponding theoretical models through logical reasoning and theoretical analysis. Numerical simulation methods, such as finite element analysis, are then used to verify the related laws, and appropriate experiments are designed for further validation. Finally, the results are modified based on feedback from actual engineering applications to achieve higher applicability accuracy. The main objective of this experiment is to compare and analyze the experimental results of the structure with and without the attached viscous damper. The vibration frequency and velocity under actual working conditions are simulated, and the response of the damper is measured to evaluate its damping effect. This study examines the damping efficiency of structures equipped with viscous dampers and investigates how damper placement affects the damping efficiency across beam spans. The analysis focuses on key damping efficiency indicators, including the additional damping ratio, reduction in the vertex displacement, reduction in the base shear, and inter-story displacement utilization rate. The findings of this research will serve as valuable guidance and reference for design professionals when incorporating viscous dampers into future damped structure designs.

2. Shaking Table Test Design

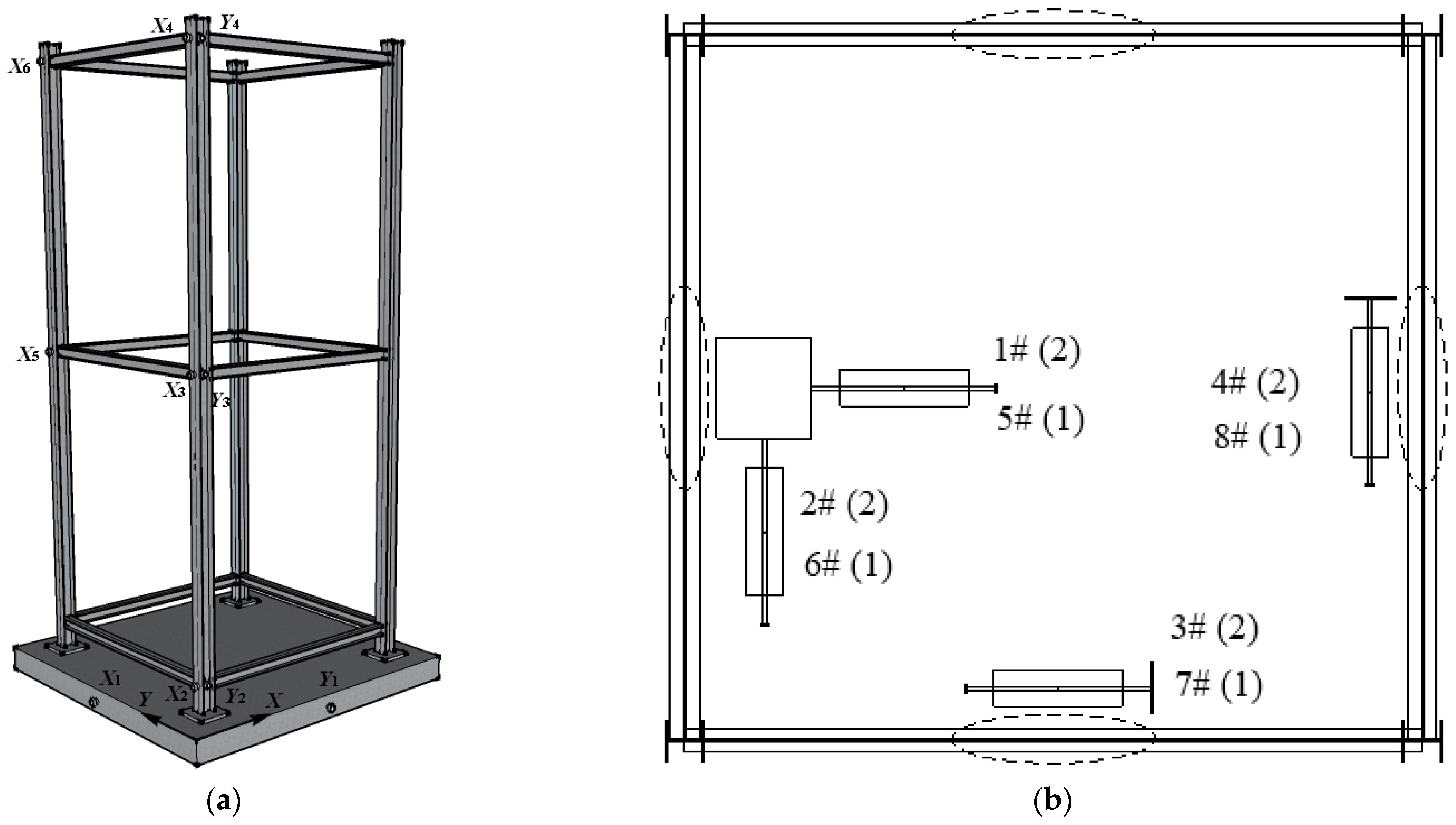
2.1. Test Equipment

The viscous damper is a type of damper that is dependent on velocity. When used in building construction projects, its frequency typically falls between 1 and 3 Hz, and the velocity range is usually between 100 mm/s and 600 mm/s. Therefore, traditional quasi-static tests or static tests are not suitable for evaluating the performance of the structure. This experiment utilized a dynamic loading system based on the seismic simulation shaking table platform at the Engineering Seismic Research Institute of Kunming University of Science and Technology. The damping effect on structures was evaluated through the loading of earthquake waves onto structural systems. The fundamental parameters of the shaking table are presented in

Table 1. Basic parameters of the shaking table.

Projects	Basic Parameters
Tabletop dimensions	4 m × 4 m
Maximum load capacity	30 tons
Vibration direction	Bi-directional three degrees of freedom (X and Y translational motion, and Z rotational motion)
Vibration waveforms	Various types of waves, including regular waves, random waves, and simulated seismic waves.
The maximum acceleration	±1.0 g (Payload of 20 tons), ±0.8 g (Payload of 30 tons)
Maximum velocity	800 mm/s
Maximum displacement	±125 mm
Frequency range	0.1 Hz~100 Hz

.

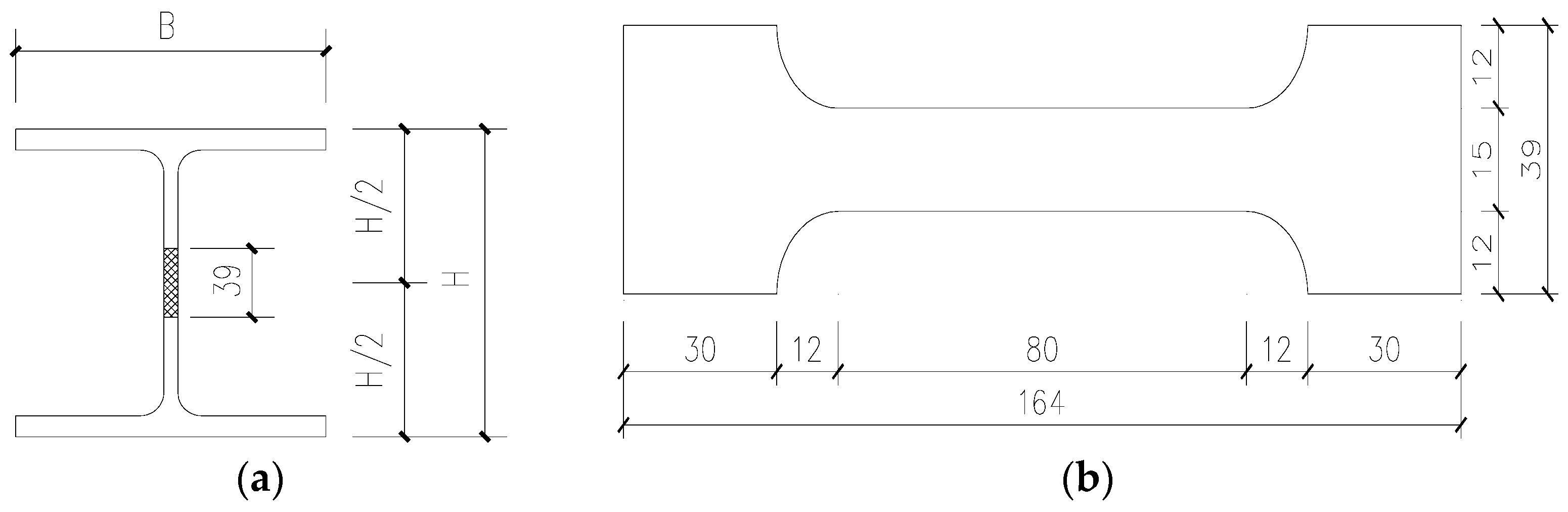
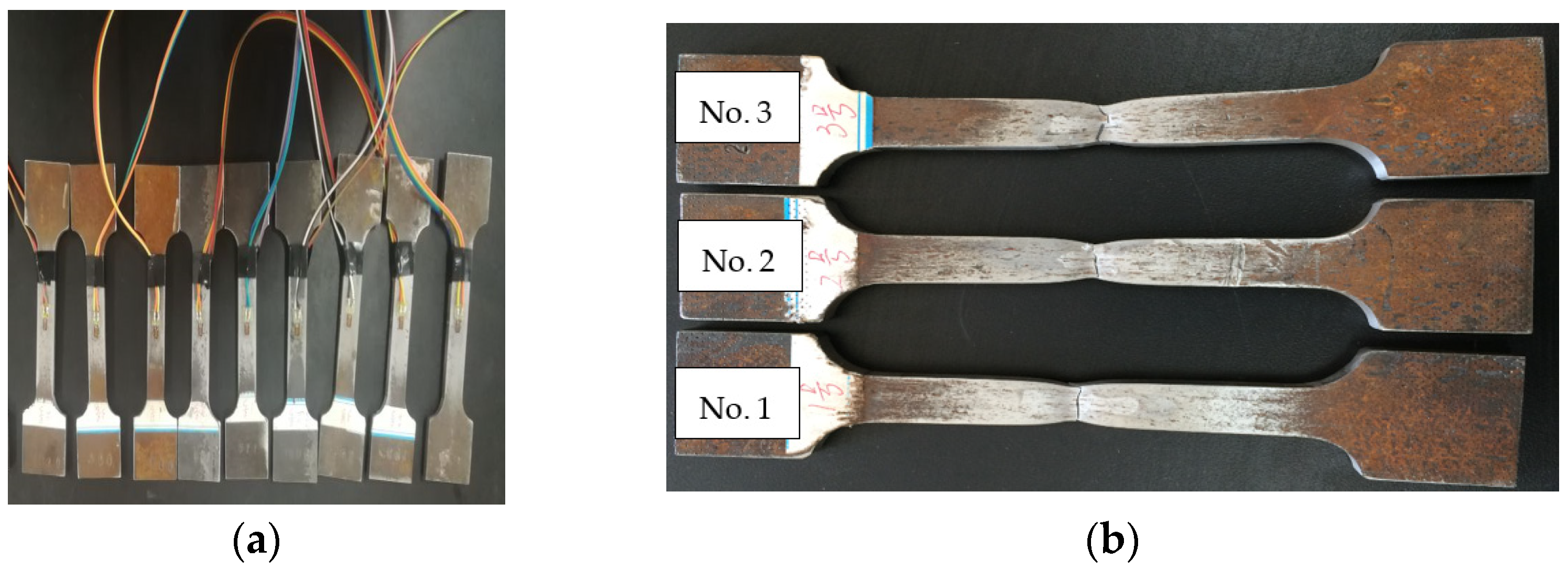
2.2. Selection of Materials and Their Material Property Testing

The steel used was conventional Q235 H-section steel commonly found on the market. The H-section steel consisted of different cross-section sizes from the same manufacturer and batch. The three main types were HW100 × 100, HW125 × 125, and HW175 × 175, with actual dimensions shown in

Table 2. Actual dimensions of various types of H-section steel profiles.

Types	Width b/mm	Height h/mm	The Thickness of the Web Plate/mm	The Thickness of the Flange/mm
HW100 × 100	100	100	5.3	7.0
HW125 × 125	125	125	6.0	8.0
HW175 × 175	175	175	6.4	9.3

. According to the standard of “Metallic materials-Tensile testing-Part 1: Method of test at room temperature” [38], samples were taken from the web position of the H-section steel as shown in Figure 1a. Then, the specimens were prepared as shown in Figure 1b for tensile testing, and the values of Young’s modulus, yield strength, tensile strength, and elongation at fracture of the specimens were measured, as presented in

Table 3. Test results of specimen material properties.

Types	Test Specimen Number	Measured Yield Strength fy/MPa	Measured Tensile Strength fu/MPa	fu/fy ≥ 1.25	fy/235 ≤ 1.30	Young’s Modulus E/GPa	Elongation at Fracture δ/%
HW 100 × 100	No.1	263	376	1.43	1.12	195	31
	No.2	264	378	1.43	1.12	193	27
	No.3	256	375	1.47	1.09	190	31
	Average	261	376	1.44	1.11	193	30
HW 125 × 125	No.1	262	429	1.64	1.11	202	28
	No.2	261	430	1.65	1.11	203	27
	No.3	261	425	1.63	1.11	204	27
	Average	261	428	1.64	1.11	203	27
HW 175 × 175	No.1	260	424	1.63	1.10	204	27
	No.2	258	419	1.62	1.10	212	26
	No.3	262	427	1.63	1.12	209	28
	Average	260	423	1.63	1.11	208	27

.

The specimens were tested for tensile strength using an electronic universal testing machine with a capacity of 100 kN, as illustrated in Figure 2.

From Table 3, it can be observed that the material test results for the three types of H-section steel specimens in the experiment meet all the specified criteria.

2.3. Experimental Model Fabrication

In order to achieve the purpose of this experiment, a two-story single-span steel frame structure system model was constructed. The height of each floor was 3.6 m, and the span in both the X- and Y-directions was 3.0 m. The cross-section dimensions of the four columns were all HW175 × 175, and the column bases were connected by welding. The cross-section dimensions of the beams in the strong axis direction of the columns (X-direction) were HW100 × 100, and the cross-section dimensions of the beams in the weak axis direction of the columns (Y-direction) were HW125 × 125. The beams and columns were connected by “T” type keys with bolt connections. The damper was connected to the beam through bolts, with the upper and lower columns of the intermediate column. The upper and lower columns of the intermediate column were made of steel plates with a width of 400 mm and a thickness of 10 mm. The out-of-plane stiffness was satisfied by structural measures. In order to connect the lower column of the intermediate column, a ground beam layer was specifically designed in the model. The elevation from the apex of the ground beam to the base of the column measured 0.40 m, as depicted in Figure 3. The connection configuration between the beam–column joints and the damper installation method are clearly demonstrated. The shaking table test models are presented in Figure 4; specifically, Figure 4a illustrates the undamped structural model without dampers, while Figure 4b portrays the damping structural model equipped with dampers. The model weight was determined by the combination of the self-weight of components and additional counterweights. According to the requirement of 3.0 kN/m², the counterweights were uniformly arranged on the floor slabs of the model. The final total weight of the model was 6.7 tons.

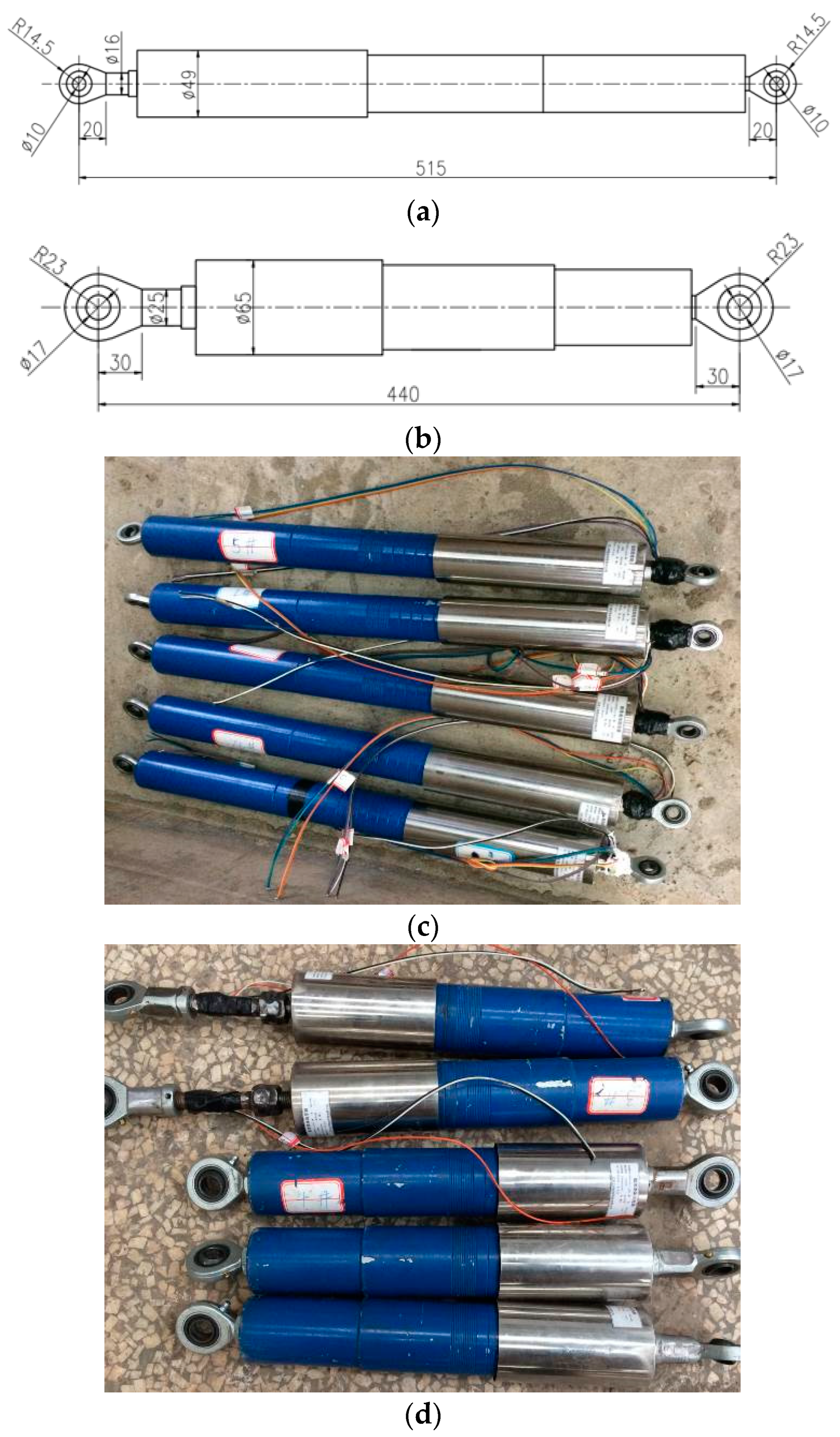
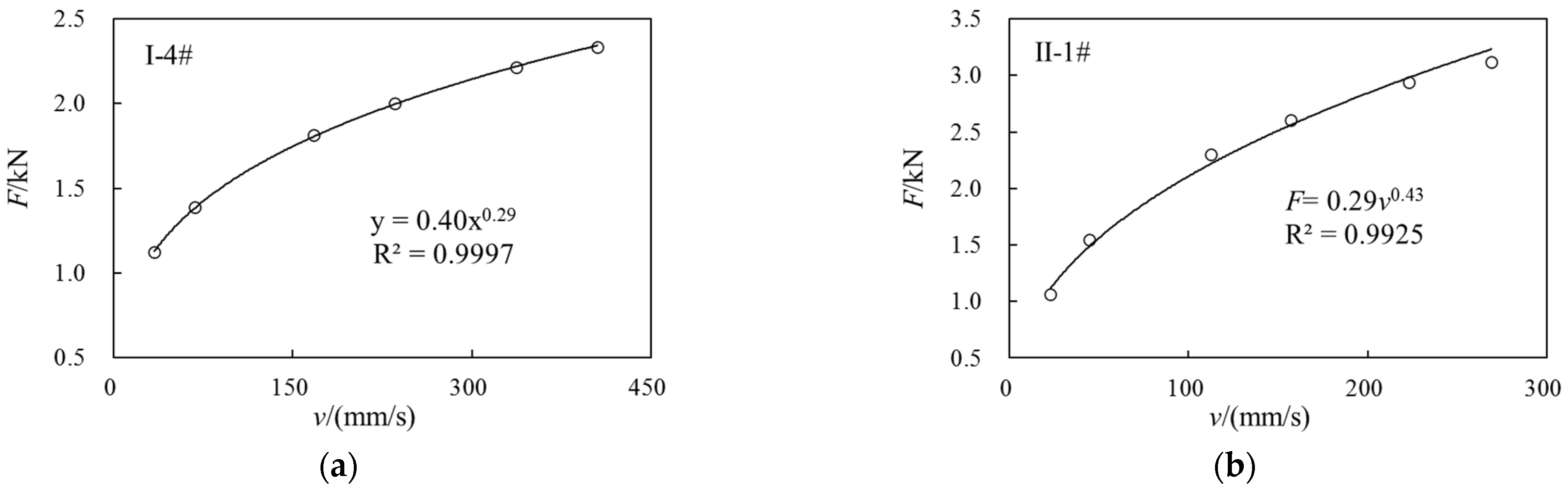
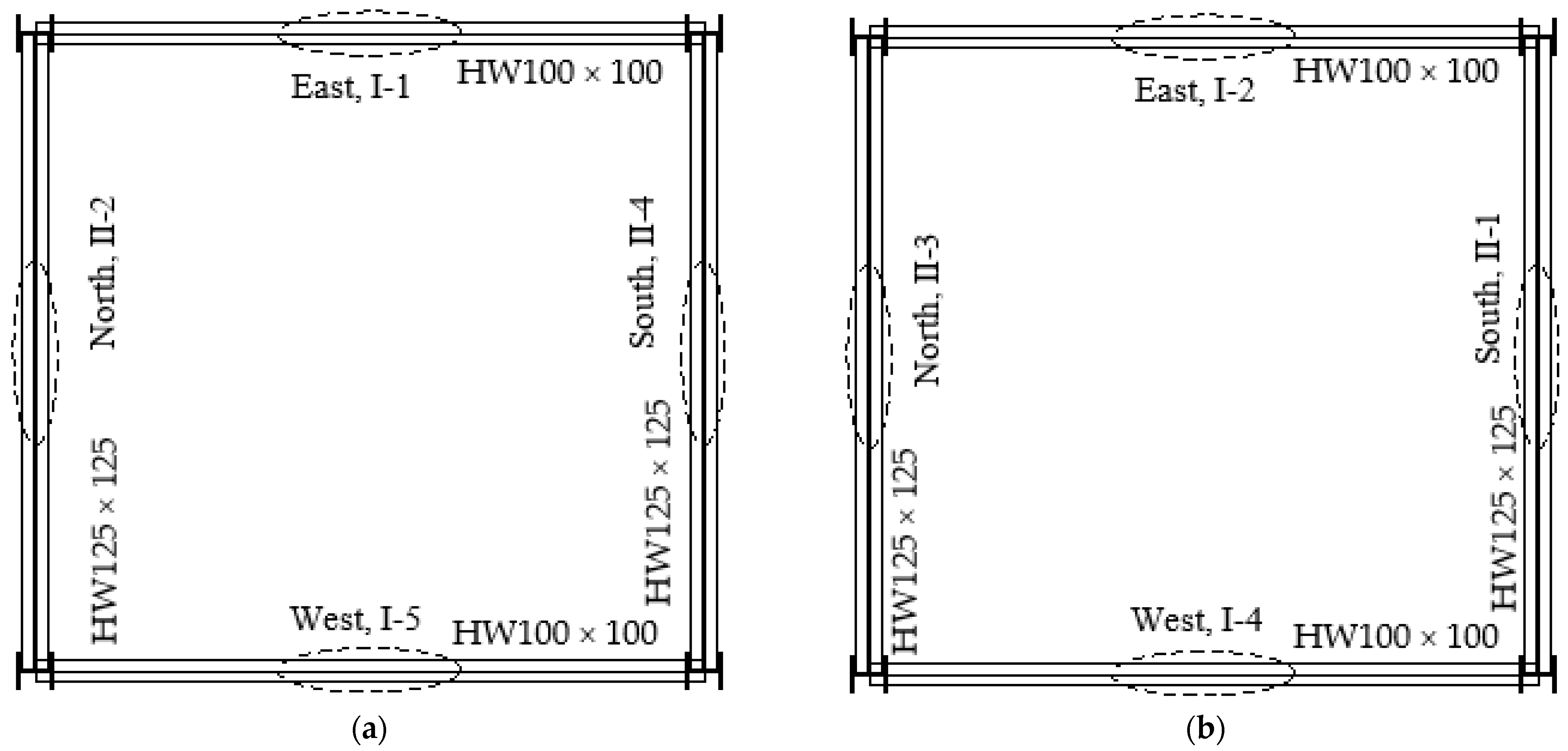
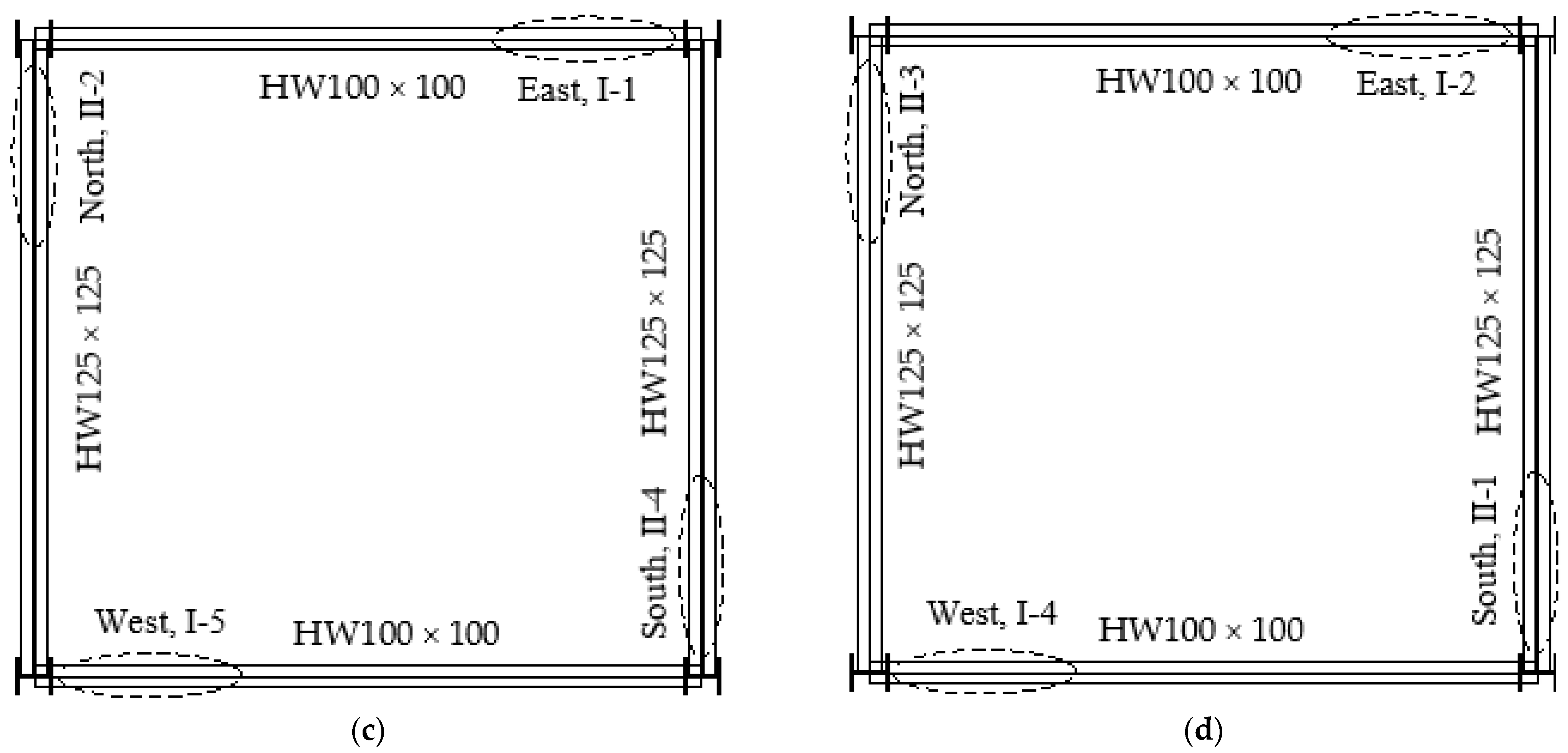
2.4. Damping Device Design Parameters and Arrangement Positions

The experiment employed eight sets of dampers, with four sets of Type I dampers and four sets of Type II dampers. Five sets of each type of damper were prepared. The design parameters of the dampers mainly included the damping coefficient and damping index. Typically, the total damping coefficient required for a seismic structure is derived based on three parameters: the energy dissipation of the damper, the strain energy of the structure, and the additional damping ratio of the structure. Subsequently, the damping coefficient for each damper is obtained by evenly distributing the total damping coefficient among the dampers. The damping index is usually selected between 0.25 and 0.50 based on practical engineering experience [39]. The final design parameters of the dampers for this experiment are shown in

Table 4. Damping device design parameters.

Types	Damping Coefficient C/kN·(s/mm)α	Damping Index α	Design Stroke/mm	Design Load/kN	Quantity/Set	Quantity in Reserve/Set
I	0.4	0.3	±30	2.25	4	1
II	0.3	0.45	±20	3.33	4	1

. The dimensional and physical appearance of the dampers are illustrated in Figure 5.

In accordance with the stipulations of “Technical specification for seismic energy dissipation of buildings” [40], the mechanical performance test of dampers was conducted. The following were the specific steps: (1) Using the sine excitation method, the loading system of the testing machine was controlled by the input displacement u = u₀sin(ωt), where u₀ is the designed displacement of the damper and ω = 2πf₁ is the circular frequency corresponding to the structural fundamental frequency f₁. (2) Frequency f1 was applied to the damper, with displacement amplitudes of 0.1u₀, 0.2u₀, 0.5u₀, 0.7u₀, 1.0u₀, and 1.2u₀, respectively. Five continuous cycles were completed and the force–displacement hysteresis loops of the damper were plotted. (3) The damping coefficient and damping index corresponding to the third cycle under each operating condition were obtained through regression fitting, and they were taken as the measurement values. Figure 6 and Figure 7 show one representative damper for each of the two types, namely I-4 and II-1. The measured parameters of all dampers are shown in

Table 5. Damper measured parameters.

Types	Damping Coefficient C	Types	Damping Coefficient C
I-1	0.52 kN/(mm/s)0.28	II-1	0.29 kN/(mm/s)0.43
I-2	0.20 kN/(mm/s)0.38	II-2	0.21 kN/(mm/s)0.49
I-3	0.28 kN/(mm/s)0.34	II-3	0.37 kN/(mm/s)0.40
I-4	0.40 kN/(mm/s)0.29	II-4	0.27 kN/(mm/s)0.44
I-5	0.36 kN/(mm/s)0.30	II-5	0.31 kN/(mm/s)0.42

.

In the experimental model, the dampers in the east–west direction are Type I, with the second floor designated as I-2 and I-4, and the first floor as I-1 and I-5. The dampers in the north–south direction are Type II, with the second floor designated as II-1 and II-3, and the first floor as II-4 and II-2. Figure 8 shows the arrangement of dampers at the 1/2 position within the beam span. We conducted experimental tests on the damped structures corresponding to the damper arrangement at both the 1/2 position and the 1/6 position within the beam span.

2.5. Sensor Placement

The experiment mainly considered two aspects: the input of seismic excitation and the output of a structural response. In this experiment, control was achieved by inputting iteratively converged displacements into the seismic simulation shaking table, while data collection was performed using acceleration sensors and displacement sensors for the output. The acceleration sensors were used to collect the acceleration values of the table and the floors. On the shaking table surface, one was arranged in the X-direction and another was arranged in the Y-direction. At the position of the ground beam layer, one was arranged in the X-direction and another was arranged in the Y-direction. In the first and second layers of the structure, there were two arrangements in the X-direction and one arrangement in the Y-direction. Therefore, a total of ten acceleration sensors were arranged according to the positions shown in Figure 9a. The primary purpose of arranging displacement sensors was to collect the displacement of the dampers and obtain the displacement time-history curves of the dampers under seismic action, and then plot the hysteresis loops together with the force time-history curves of the dampers. Secondly, the floor displacement of the structure was measured. Two displacement sensors were installed on each floor to measure the inter-story displacements, with one installed in the X-direction and the other in the Y-direction. The top pin of the sensor was placed on the outer surface of the cantilever square steel column. Displacement sensors for measuring damper displacement were installed on both the southern and western floors. Therefore, a total of eight displacement sensors were arranged, as shown in Figure 9b.

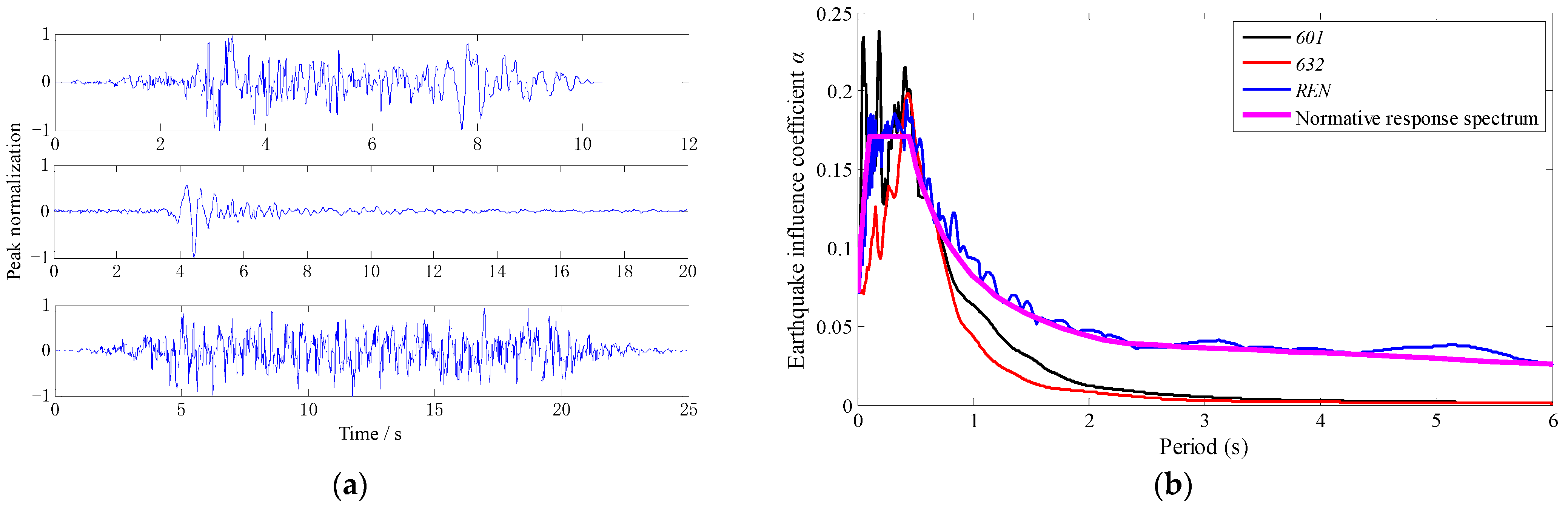
2.6. Experimental Loading Conditions

The experiment considered three seismic levels: frequent earthquakes, occasional earthquakes, and rare earthquakes. The corresponding peak ground accelerations were 0.07 g, 0.20 g, and 0.40 g, respectively. According to the “Code for Seismic Design of Buildings” [41], two natural waves and one artificial wave were selected to input into the seismic simulation shaking table system. The seismic wave records were standardized to peak values, as depicted in Figure 10a. To adjust for various seismic levels, these records were amplified using a scaling factor. However, the time axis of the records remained unaltered. The information derived from the natural wave records is presented in

Table 6. Basic information of natural seismic waves.

Numbered	Earthquake Name	Occurrence Time	Recording Station	PGA (m/s2)
601	Imperial Valley-07	15 October 1979	USGS STATION 412	3.20
632	Northridge-05	17 January 1994	SYLMAR-COUNTY HOSPITAL PARKING LOT	0.90

. The time-history curves of the seismic waves and their response spectrum curves under the frequent earthquake level are shown in Figure 10b.

There were a total of 22 loading conditions in the experimental test, mainly considering three seismic waves, the X- and Y-axes of the structure, including the levels of frequent earthquakes, occasional earthquakes, and rare earthquakes, as well as white noise conditions, as shown in

Table 7. Experimental loading conditions. W1, W2, W3, and W4 represent the first, second, third, and fourth instances of white noise, respectively. The peak ground accelerations loaded during the tests are considered to be relatively small at 0.07 g.

Operating Condition Numbers	Operating Condition Name	Seismic Levels	Seismic Waves	Direction	PGA/g
1	W1	the first white noise		XY	0.07
2	FX-601	Frequent earthquake	601	X	0.07
3	FY-601		601	Y	0.07
4	FX-632		632	X	0.07
5	FY-632		632	Y	0.07
6	FX-REN		REN	X	0.07
7	FY-REN		REN	Y	0.07
8	W2	the second white noise		XY	0.07
9	OX-601	Occasional earthquake	601	X	0.20
10	OY-601		601	Y	0.20
11	OX-632		632	X	0.20
12	OY-632		632	Y	0.20
13	OX-REN		REN	X	0.20
14	OY-REN		REN	Y	0.20
15	W3	the third white noise		XY	0.07
16	RX-601	Rare earthquake	601	X	0.40
17	RY-601		601	Y	0.40
18	RX-632		632	X	0.40
19	RY-632		632	Y	0.40
20	RX-REN		REN	X	0.40
21	RY-REN		REN	Y	0.40
22	W4	the fourth white noise		XY	0.07

. The experiments were divided into three groups: the non-damped structure without additional dampers, the damped structure with additional dampers placed at the 1/2 position within the beam span, and the damped structure with dampers placed at the 1/6 position within the beam span. All three groups of shaking table tests were loaded according to the conditions in Table 7. The three sets of experiments conducted white noise testing under the first working condition and obtained consistent structural frequency results of 2.0 Hz in the X-direction and 1.5 Hz in the Y-direction. This indicates that the stiffness along the strong axis (X-direction) of the H-shaped steel column section was larger, while the stiffness along the weak axis (Y-direction) was smaller. Furthermore, the structural frequency remained unchanged after the fourth white noise test.

3. Test Results Analysis

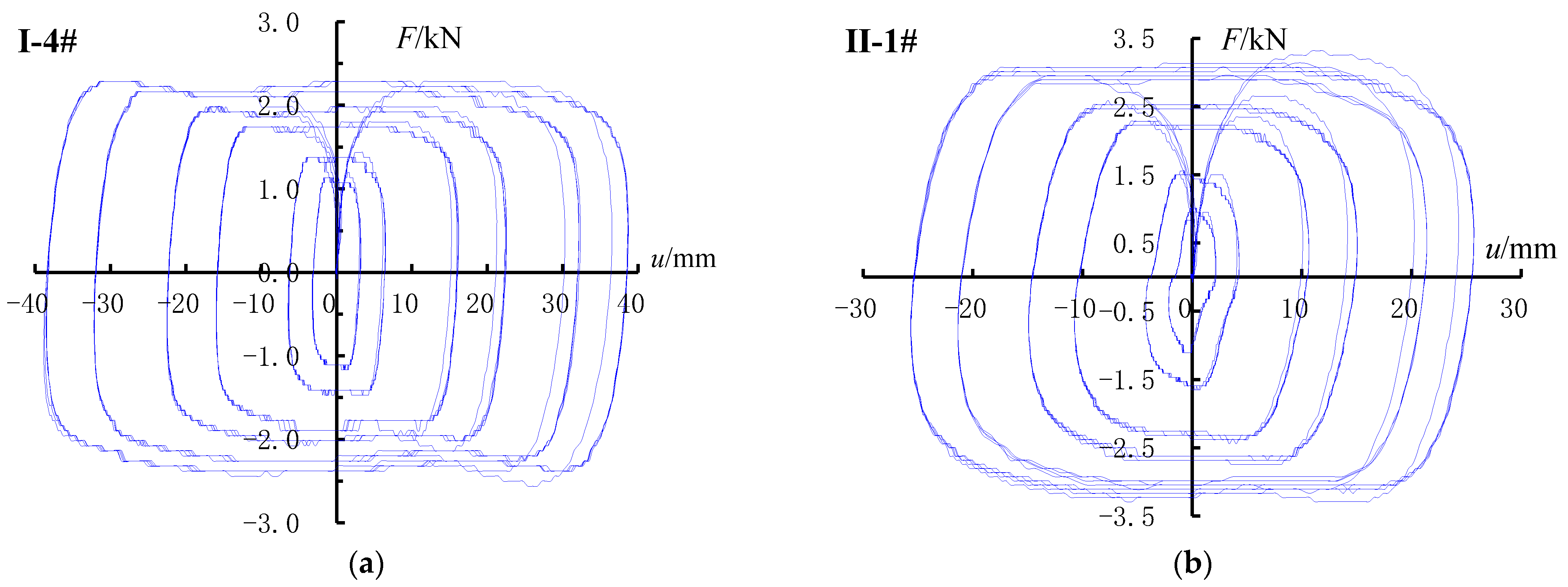
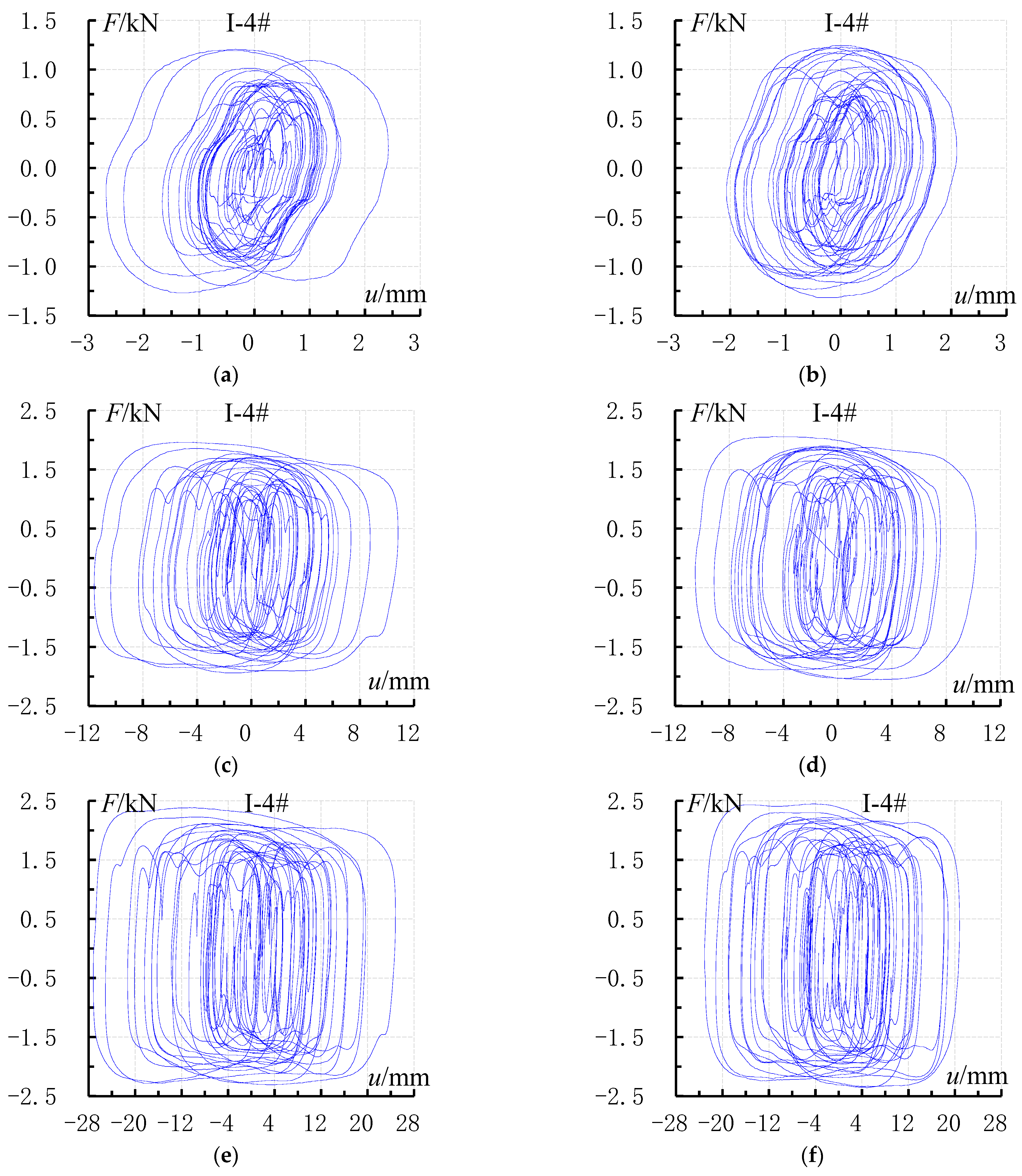
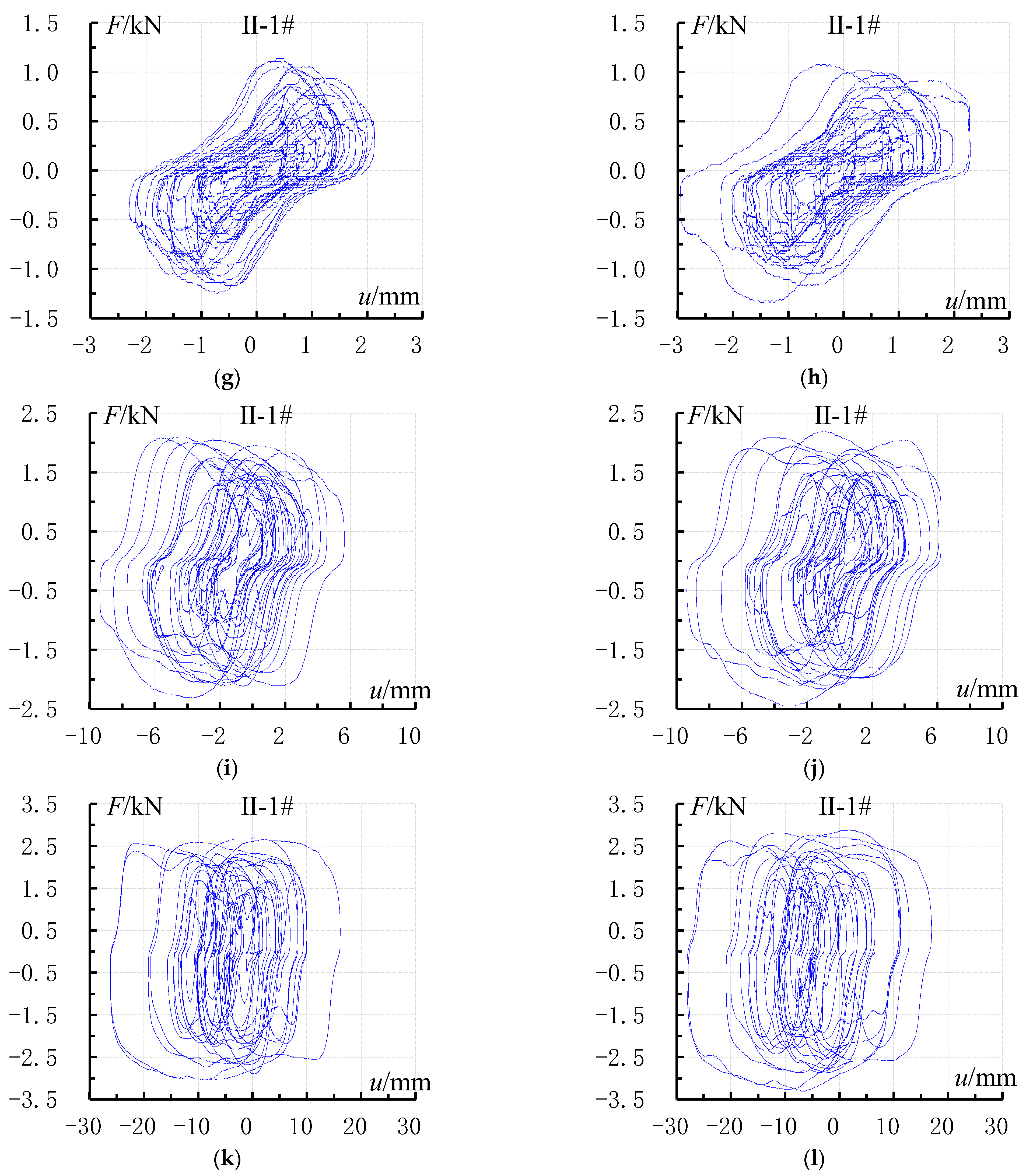
3.1. Hysteresis Loops of the Dampers

To facilitate the comparative study of the dampers’ energy dissipation effect at various locations, their hysteresis energy dissipation loops were tested and plotted under frequent, occasional, and rare earthquakes. The hysteresis loops of damper No. I-4 were plotted in the X-direction, and the hysteresis loops of damper No. II-1 was plotted in the Y-direction, as shown in Figure 11.

When the damper works under seismic excitation, the degree of fullness of the hysteresis loops and the size of the area reflected in the force and displacement are important indicators to measure the energy dissipation effect of the damper. By studying and comparing the hysteresis loops of the I-4 damper arranged in the X-direction and the II-1 damper arranged in the Y-direction in Figure 11, the following conclusions can be drawn:

(1)

The hysteresis loops of the dampers are uniformly full at different seismic levels, indicating strong energy dissipation capacity. With the increase in seismic level, the energy dissipation capacity of the dampers increases rapidly.

(2)

The area of the hysteresis loops when the damper is arranged at the 1/2 position within the beam span, i.e., the energy dissipation effect of the damper, is significantly larger than that when the damper is arranged at the 1/6 position within the beam span.

(3)

The force and displacement of the I-4 and II-1 dampers under rare earthquakes are in good agreement with the design parameters in Table 4.

3.2. Additional Damping Ratio

The additional damping ratio is a crucial parameter for evaluating the damping efficiency of a structural system. Viscous dampers are a prime example of velocity-dependent dampers. In accordance with the prevailing standards in China [40,41], the effective additional damping ratio provided by dampers to the structural system can be calculated using the following formula.

$${\xi }_{\mathrm{d}}=\frac{{\displaystyle \sum _{j=1}^n{W}_{\mathrm{c}j}}}{4\mathsf{\pi }{W}_{\mathrm{s}}}$$

(1)

where ${\xi }_{\mathrm{d}}$ represents the additional effective damping ratio provided by dampers to the structure; $W_{\mathrm{c}j}$ is the energy dissipated by the jth damper in the structure during one cycle of reciprocating motion with an inter-story displacement $\Delta u_j$; n is the number of dampers; $W_{\mathrm{s}}$ is the total strain energy of the damped structure under the expected displacement.

The structural model’s frequency remained constant throughout the initial phase, frequent earthquakes, occasional earthquakes, and rare earthquakes. This suggests that the structure was still in an elastic or near-elastic state. Consequently, the shaking table experiment results can be calculated separately for additional damping ratios in the X- and Y-directions for three seismic waves under frequent, occasional, and rare earthquake conditions using Equation (1), as illustrated in Figure 12.

From Figure 12a, it can be observed that the stiffness of the structure in the X-direction is higher, and its natural frequency is also higher, with a value of 2.0 Hz. The following points can be derived:

(1)

The additional damping ratio varies significantly with different locations of damper arrangement.

(2)

Regardless of whether the earthquake is frequent, occasional, or rare, the additional damping ratio of the damper arrangement at the 1/2 position within the beam span is significantly greater than that at the 1/6 position within the beam span. For example, for the artificial seismic wave REN, the additional damping ratios are 19% and 11%, 20% and 13%, and 13% and 11% at different seismic levels and different arrangement positions, respectively.

(3)

The overall trend is that the additional damping ratio decreases with increasing seismic level, especially when the damper is arranged at the 1/2 position of the beam span. For instance, the additional damping ratios of the natural seismic wave 601 under frequent, occasional, and rare earthquake conditions are 21%, 17%, and 13%, respectively.

From Figure 12b, it can be observed that the stiffness of the structure in the Y-direction is relatively low, and the natural frequency is also low, with a value of 1.5 Hz. The difference in additional damping ratio with different damper arrangement positions is not as significant as in the X-direction for different seismic levels.

In conclusion, it can be inferred that the additional damping ratio provided by the damper to the structure is closely related to the lateral stiffness of the structure under different arrangement positions and seismic levels. The larger the lateral stiffness, the greater the difference and regularity in the additional damping ratio, as in the X-direction. Conversely, the smaller the lateral stiffness, the smaller the difference and less obvious the regularity in the additional damping ratio, as in the Y-direction.

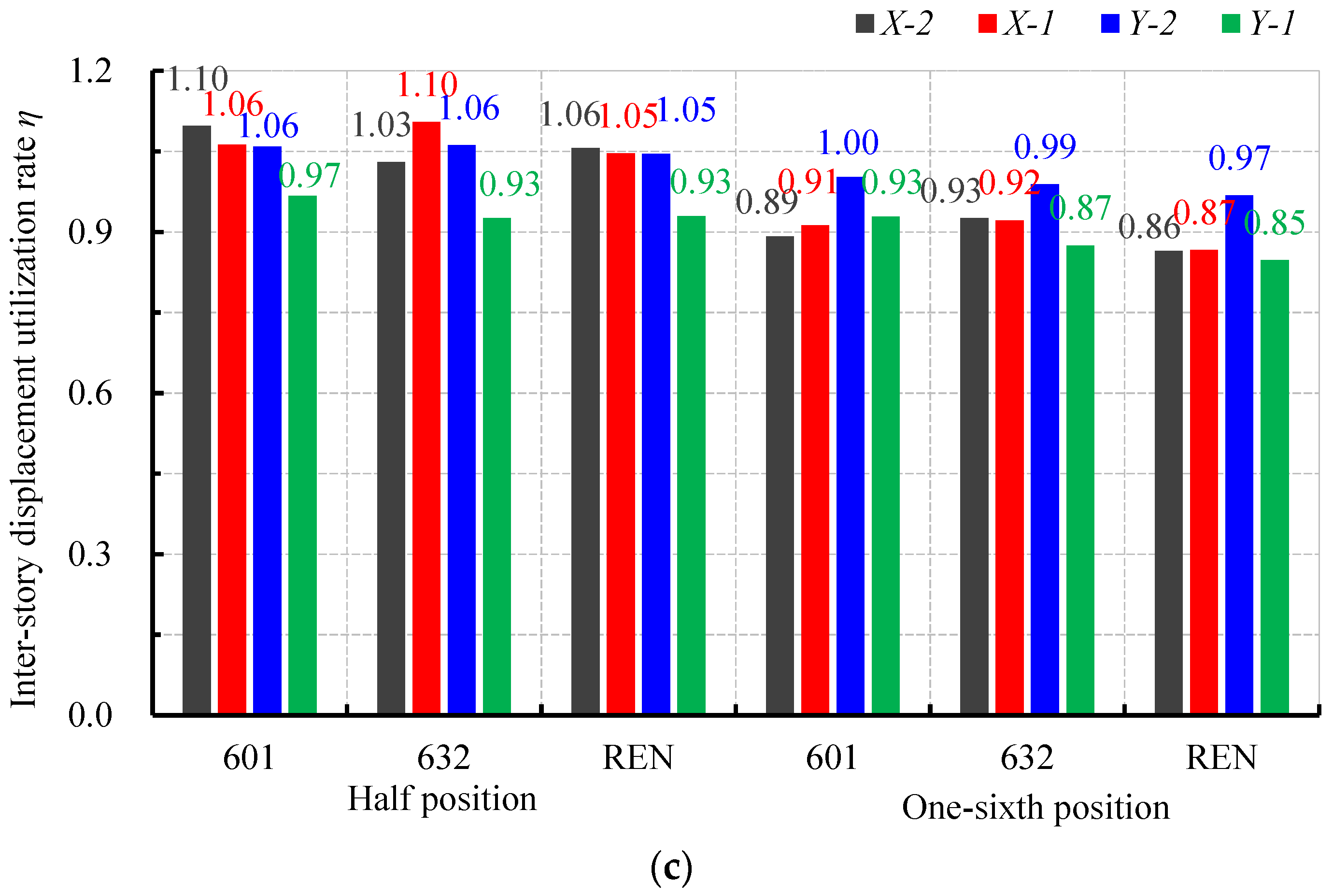
3.3. Inter-Story Displacement Utilization Rate

In damped structures, the displacement of a damper under seismic excitation is an important indicator of their energy dissipation, which can be clearly observed from the hysteresis loops of dampers. By testing the displacement of floors and the corresponding damper displacement under seismic action, the ratio between the two can be calculated under frequent earthquakes, occasional earthquakes, and rare earthquakes. The definition of the inter-story displacement utilization rate is as follows: the ratio between the displacement of a damper and the floor displacement of the floor where the damper is located in a damped structure. The calculation expression is as follows:

$$\eta =\frac{u_{\mathrm{d}}}{u}\times 100\%$$

(2)

where $\eta$ represents the inter-story displacement utilization rate; u represents the displacement of the floor where the damping device is located; $u_{\mathrm{d}}$ represents the displacement of the damper.

The experiment investigated the inter-story displacement utilization rate under different arrangements of dampers for frequent, occasional, and rare earthquakes. This newly proposed evaluation index, which can effectively measure the energy dissipation effect of dampers, can be used to assess the damping efficiency of damped structure. The inter-story displacement utilization rate obtained by calculation according to Equation (2) is shown in Figure 13.

From Figure 13, the following can be observed:

(1)

There is a significant difference in the inter-story displacement utilization rate for different damper placement positions, regardless of whether it is subjected to frequent, occasional, or rare earthquakes. The inter-story displacement utilization rate is significantly higher when the dampers are placed at the 1/2 position within the beam span compared to the 1/6 position within the beam span.

(2)

With the increase in seismic levels, the inter-story displacement utilization rate also noticeably increases. For the 1/2 position, the inter-story displacement utilization rate ranges from 0.74 to 0.90 for frequent earthquakes, from 0.87 to 1.04 for occasional earthquakes, and from 0.93 to 1.10 for rare earthquakes.

(3)

It can also be observed from the figure that the inter-story displacement utilization rate of the second floor is greater than that of the first floor.

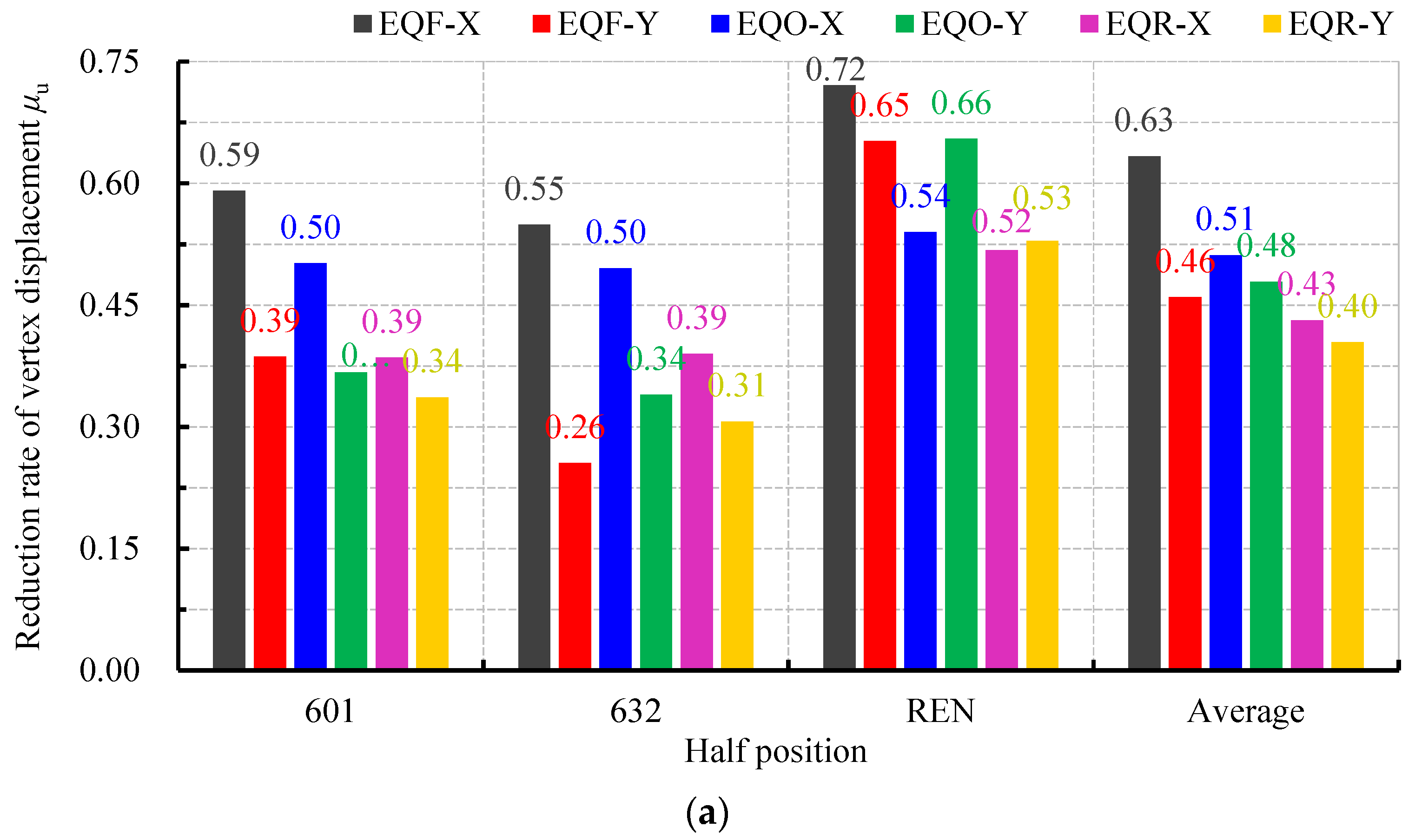
3.4. Reduction Rate of Vertex Displacement

In comparison to non-damped structures, damped structures with additional viscous dampers exhibited a reduced displacement response for each floor. This led to a significant decrease in the vertex displacement of the structure, thereby enhancing its seismic performance. Consequently, the reduction rate of the vertex displacement served as an important indicator to measure the damping efficiency of the structure. This is calculated by comparing the vertex displacement of a damped structure to that of a non-damped structure and is expressed as a ratio. The calculation formula for this ratio is as follows:

$${\mu }_{\mathrm{u}}=\frac{u_0-u}{u_0}\times 100\%$$

(3)

where ${\mu }_{\mathrm{u}}$ represents the reduction rate of the vertex displacement, $u_0$ represents the vertex displacement in the non-damped structure, and $u$ represents the vertex displacement in the damped structure.

The reduction rate of the vertex displacement and the averages of three seismic waves in both the X- and Y-directions for structures under frequent, occasional, and rare earthquakes were calculated using Equation (3), as illustrated in Figure 14. Figure 14c presents the average vertex displacements of three seismic waves corresponding to the structure’s X- and Y-directions with dampers arranged at the 1/2 and 1/6 positions within the beam span, respectively. When these values were compared with the average vertex displacement of non-damped structures, the comparison results revealed a distinct contrast.

As can be seen from Figure 14, the following is evident:

(1)

The reduction rate of the vertex displacement in the damped structure is significant. When the dampers are arranged at the 1/2 position within the beam span, the reduction rates of the vertex displacement for the three waves range from 0.26 to 0.72, while when the dampers are arranged at the 1/6 position within the beam span, the reduction rates of the vertex displacement for the three waves range from 0.17 to 0.64. The average reduction rates of the vertex displacement for the three seismic waves range from 0.40 to 0.63 and 0.35 to 0.48, respectively.

(2)

The reduction rate of the vertex displacement under the action of the artificial wave REN is significantly higher than that of the other two natural waves, 601 and 632.

(3)

From Figure 14c, it can be seen that the vertex displacement of the damped structure is significantly smaller than that of the non-damped structure. For example, under rare earthquakes, the vertex displacement in the X-direction for the damped and non-damped structures is 50-plus mm and 92 mm, respectively, while in the Y-direction, it is 50-plus mm and 84 mm, respectively. This indicates that the damped structure with additional viscous dampers has excellent damping efficiency. Moreover, the vertex displacement when the dampers are arranged at the 1/2 position within the beam span is smaller than that when the dampers are arranged at the 1/6 position, indicating that the closer the damper is to the middle of the beam span, the better the damping efficiency of the structure.

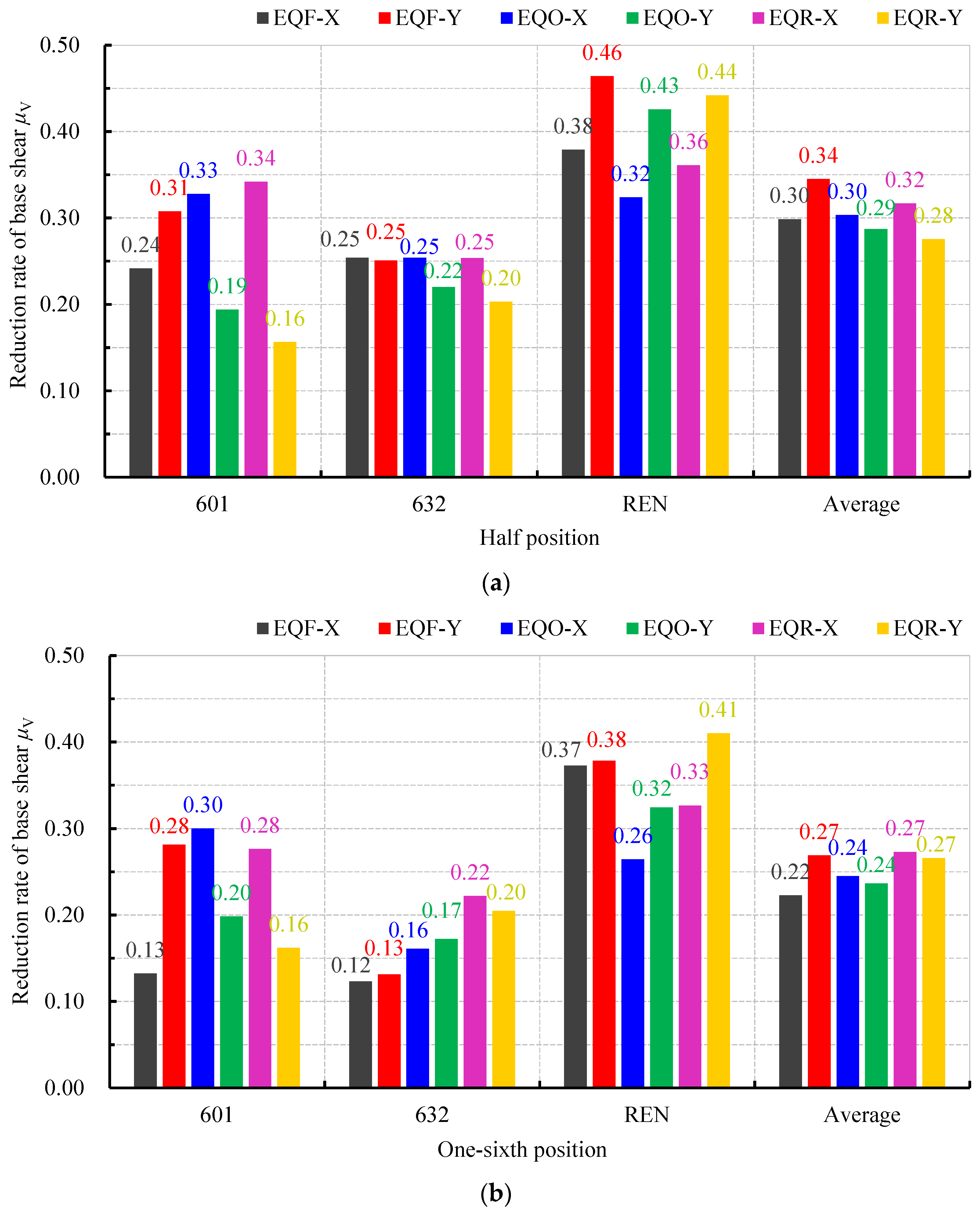
3.5. Reduction Rate of Base Shear

The reduction rate of the base shear is a crucial indicator for assessing the damping efficiency of a structure. When a damped structure is equipped with additional viscous dampers, its response to seismic excitation decreases, leading to a significant reduction in the base shear of the structure and improving its seismic performance. The reduction rate of the base shear is calculated by dividing the reduction in the base shear of the damped structure by that of the non-damped structure. The formula for calculating this rate is as follows:

$${\mu }_{\mathrm{V}}=\frac{V_0-V}{V_0}\times 100\%$$

(4)

In the equation, ${\mu }_{\mathrm{V}}$ represents the base shear reduction rate, $V_0$ represents the base shear of the non-damped structure, and V represents the base shear of the damped structure.

The reduction rate of the base shear and the averages of three seismic waves corresponding to the structure’s X- and Y-directions under frequent earthquakes, occasional earthquakes, and rare earthquakes were calculated, according to Equation (4), as shown in Figure 15. Figure 15c presents the average base shear of the three seismic waves corresponding to the structure’s X- and Y-directions when dampers were arranged at the 1/2 and 1/6 positions within the beam span, respectively. When these values were compared with the average base shear of non-damped structures, the comparison results revealed a distinct contrast.

The following conclusions can be drawn from Figure 15:

(1)

The reduction rate of the base shear in the damped structure is significant. When dampers are arranged at the 1/2 position within the beam span, the reduction rates of the base shear for the three waves range from 0.16 to 0.46. When dampers are arranged at the 1/6 position within the beam span, the reduction rates of the base shear for the three waves range from 0.12 to 0.41. The average reduction rates of the base shear for the three waves are between 0.28 and 0.34, and between 0.22 and 0.27, respectively.

(2)

The reduction rate of the base shear under the artificial wave REN is significantly higher than that of the other two natural waves, 601 and 632.

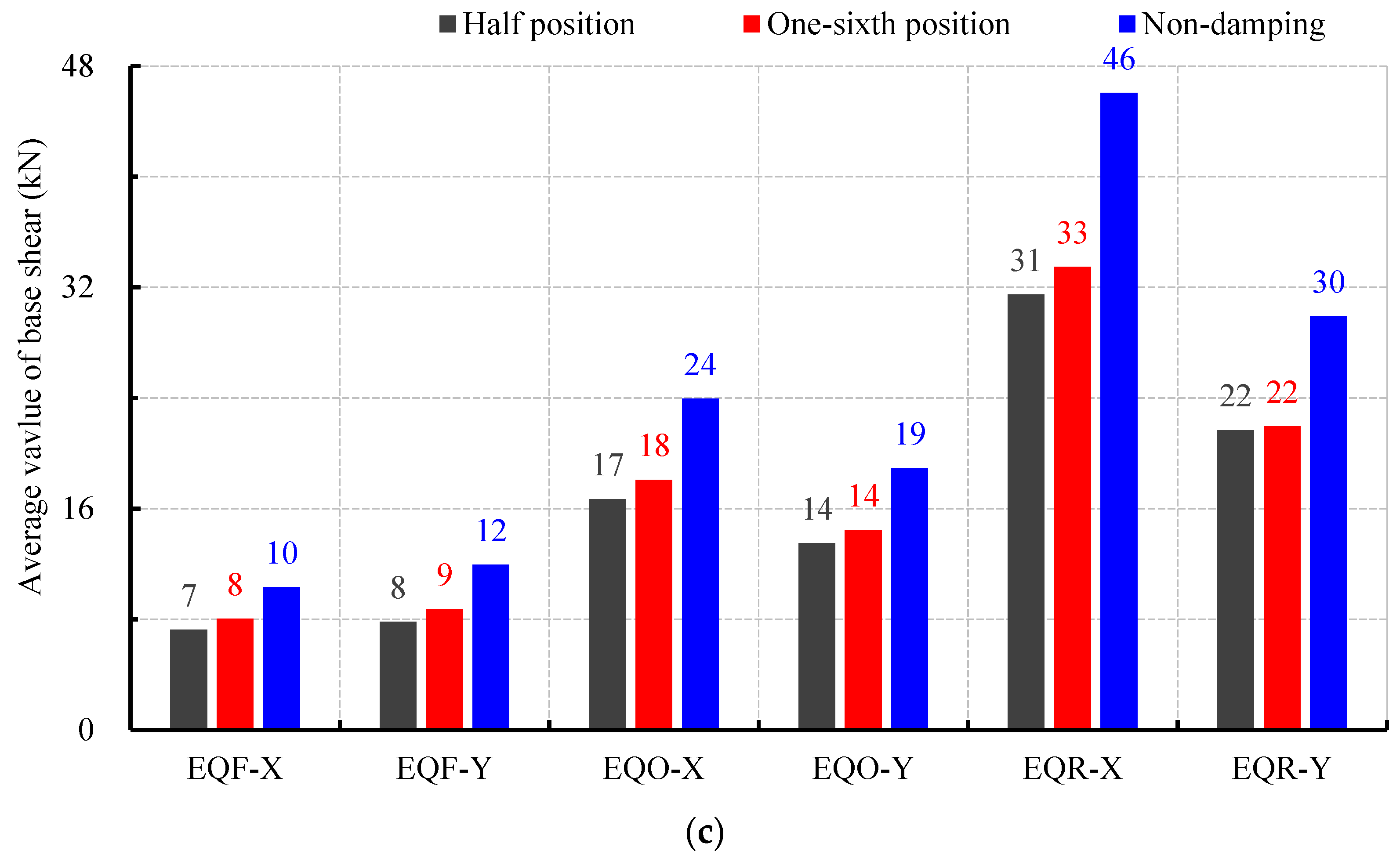
(3)

From Figure 15c, it can be observed that the base shear of the damped structure is significantly smaller than that of the non-damped structure. For example, under rare earthquake conditions, the base shear in the X-direction for the damped and non-damped structures are 30-plus kN and 46 kN, respectively, and in the Y-direction, they are 20-plus kN and 30 kN, respectively. This indicates that the damped structure with additional viscous dampers has excellent damping efficiency. Moreover, the base shear when dampers are arranged at the 1/2 span position is smaller than that when dampers are arranged at the 1/6 span position, indicating that the closer the damper is to the middle of the beam span, the better the damping efficiency of the structure.

From Figure 14 and Figure 15, it can be observed that the reduction rate of the base shear in the damped structure is significantly smaller than the reduction rate of the vertex displacement. This is an important principle that designers need to pay attention to when conducting the damping design.

4. Conclusions

This study utilized a two-story steel structure for shaking table testing, examining the response of both non-damped and damped structures to seismic excitation. Additionally, the impact of varying the position of viscous dampers on the damping efficiency of damped structures was explored. The key findings are summarized below.

(1)

The response of damped structures and non-damped structures under different seismic levels is significantly different. The vertex displacement was reduced from 17–18 mm to 7–11 mm for frequent earthquakes, from 47 mm to 23–28 mm for occasional earthquakes, and from 84–92 mm to 50–57 mm for rare earthquakes. The base shear was reduced from 10–12 kN to 7–9 kN for frequent earthquakes, from 19–24 kN to 14–18 kN for occasional earthquakes, and from 30–46 kN to 22–31 kN for rare earthquakes. This fully demonstrated that the damped structures with additional viscous dampers had good damping effects.

(2)

From the hysteresis loops of the damper, it can be seen that the viscous damper added to the structure effectively dissipated seismic energy. This further significantly reduced the seismic response of the structure. Compared with the non-damped structures, the average reduction rates of the vertex displacement and base shear for the three waves of damped structures were 0.35–0.63 and 0.22–0.34, respectively. This fully demonstrated that the damped structures using viscous dampers exhibited excellent damping efficiency.

(3)

The arrangement position of dampers within the beam span significantly affected the damping efficiency of the damped structure. Overall, the damping effect was significantly better when the dampers were placed at the 1/2 position of the beam span compared to the 1/6 position. The hysteresis loops representing the energy dissipation of the dampers illustrated this phenomenon. As for the additional damping ratio of the structures, taking the artificial wave REN in the X-direction as an example, they were 19% and 11% for frequent earthquakes, 20% and 13% for occasional earthquakes, and 13% and 11% for rare earthquakes under the two placement positions, respectively. The inter-story displacement utilization rate was 0.74–0.90 and 0.48–0.69, 0.87–1.04 and 0.73–0.95, and 0.93–1.10 and 0.85–1.0 for the two positions under frequent, occasional, and rare earthquakes, respectively. The average reduction rate of the vertex displacement for the three waves was 0.40–0.63 and 0.35–0.48 for the two placement positions, respectively. The average reduction rate of the base shear for the three waves was 0.28–0.34 and 0.22–0.27 for the two placement positions, respectively.

(4)

Introducing a novel evaluation metric known as the inter-story displacement utilization rate, which is primarily utilized to gauge the displacement utilization of dampers in damped structures based on the floor’s displacement where the damper is located. This study revealed that the closer the damper was positioned to the center of the beam span, the higher the inter-story displacement utilization rate. Furthermore, as seismic levels increased, so did the inter-story displacement utilization rate. When a centrally placed damper experienced significant seismic activity, the inter-story displacement utilization rate approached 1.0, indicating that the damper’s displacement can reach a level comparable to that of the floor.