Geometric Design Methodology for Deployable Self-Locking Semicylindrical Structures
Abstract
:1. Introduction
2. Outline of a Double-Layer Semicylindrical Grid Structure with Ideal Joints
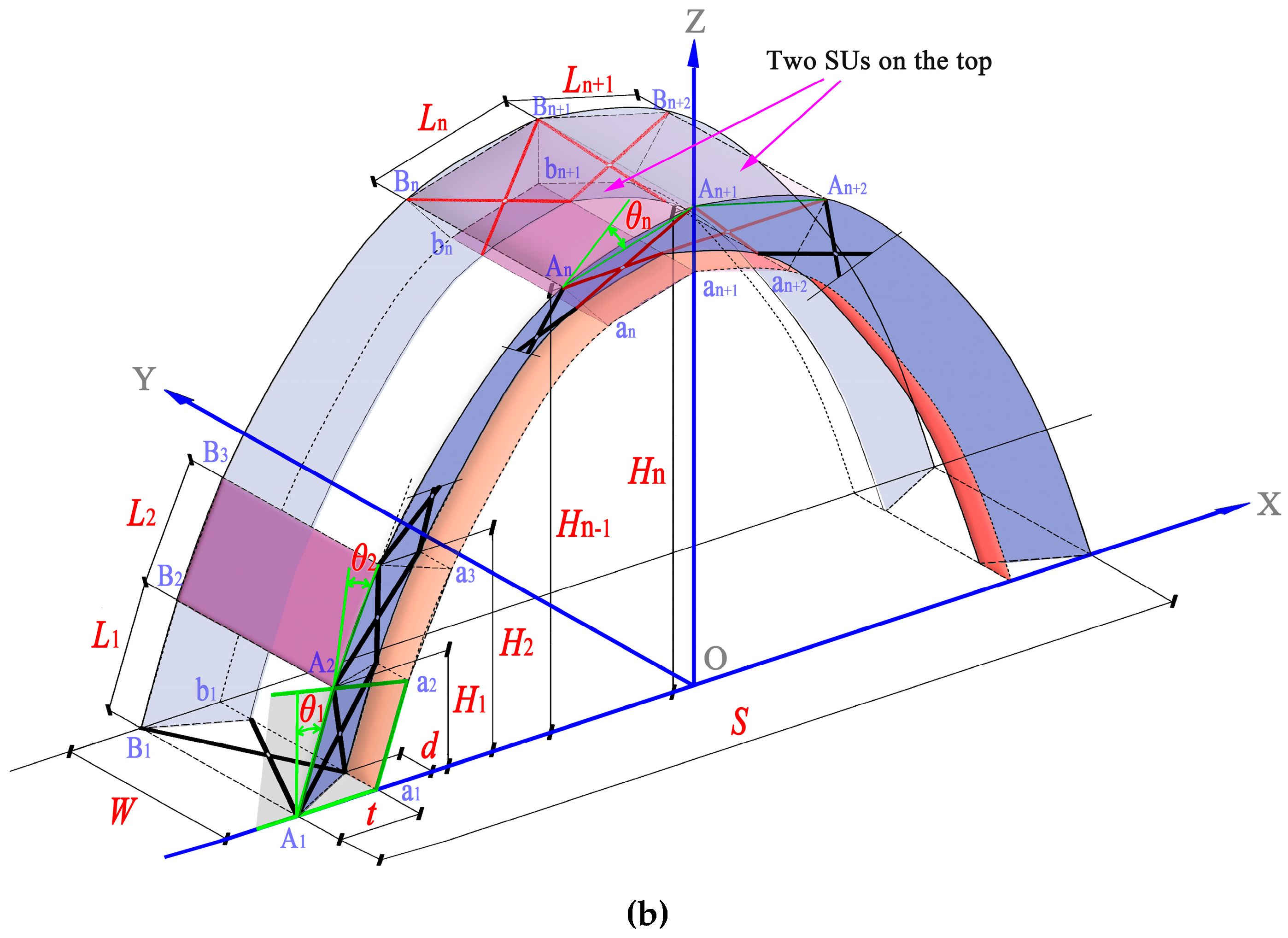
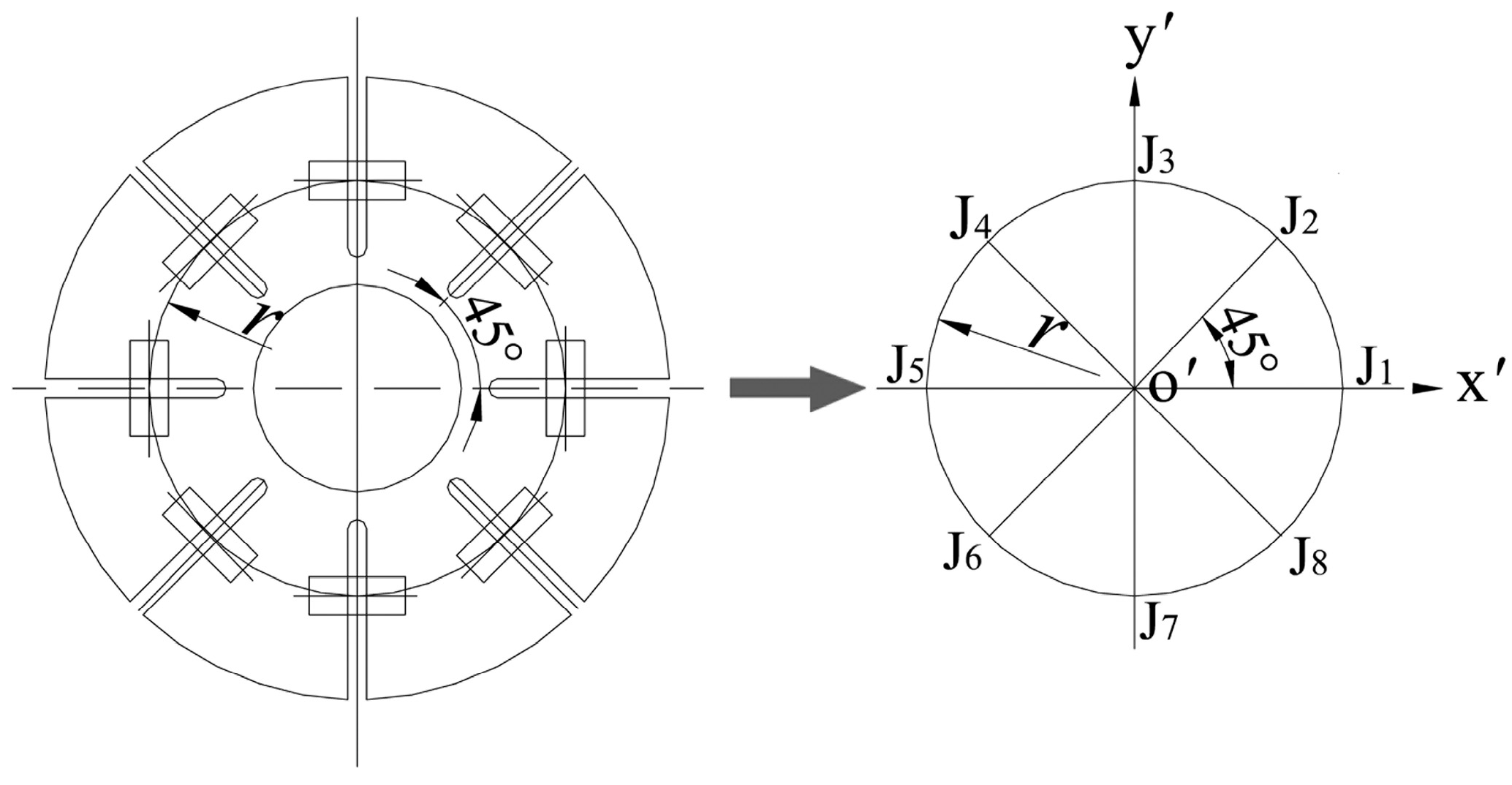
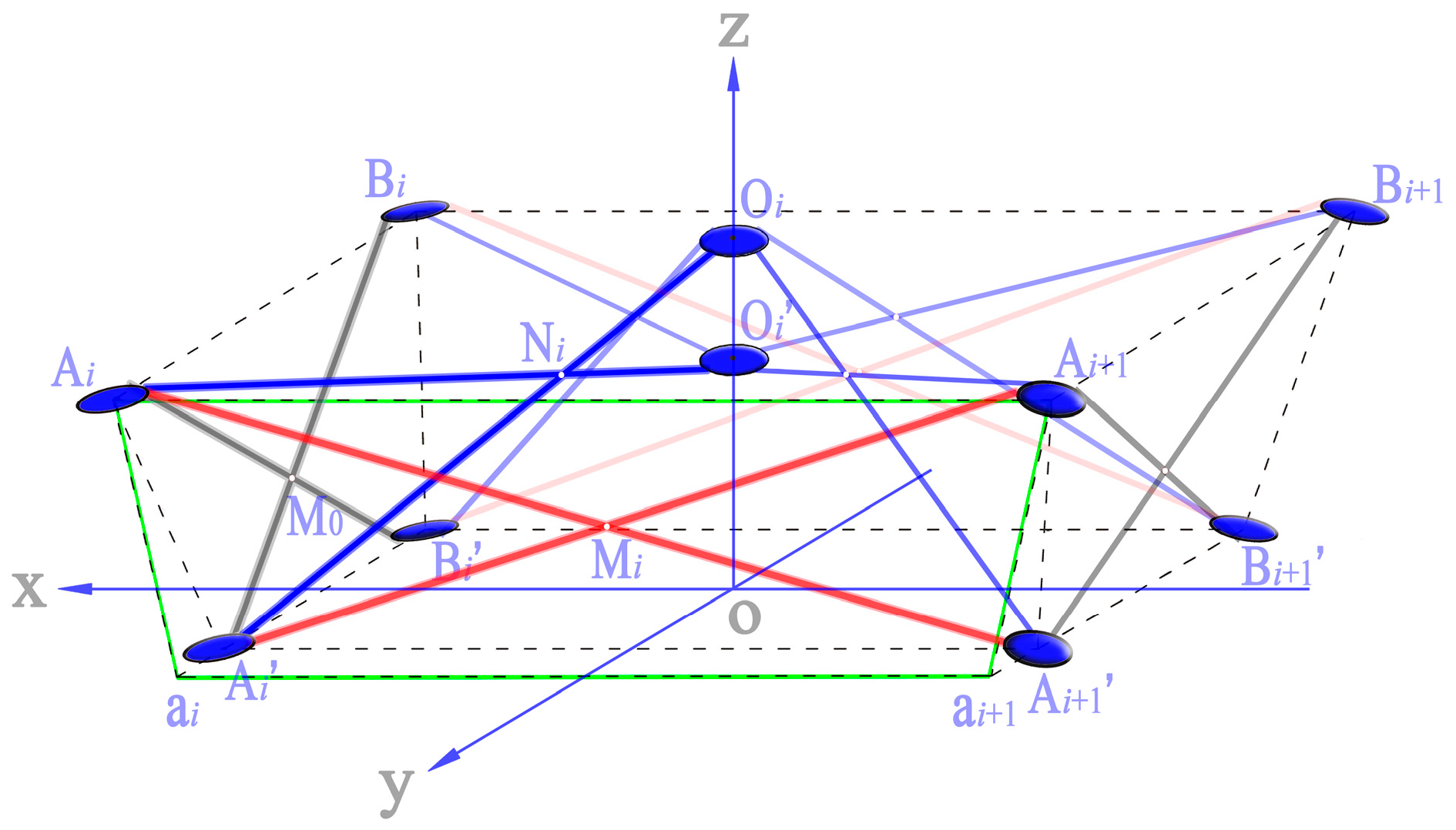
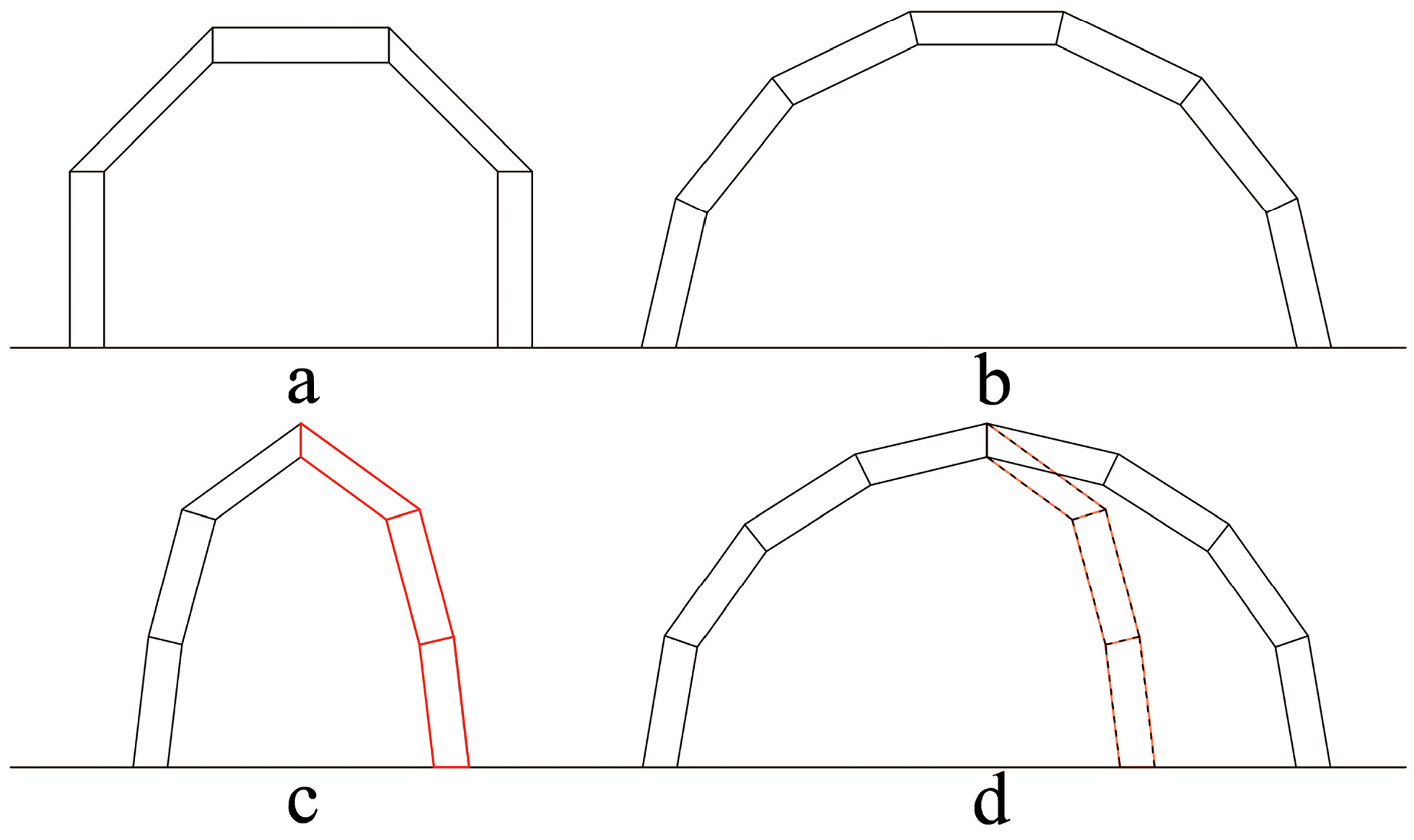
2.1. Construction of the Semicylindrical Grid Structure Configuration
2.2. Selection of Geometric Parameters
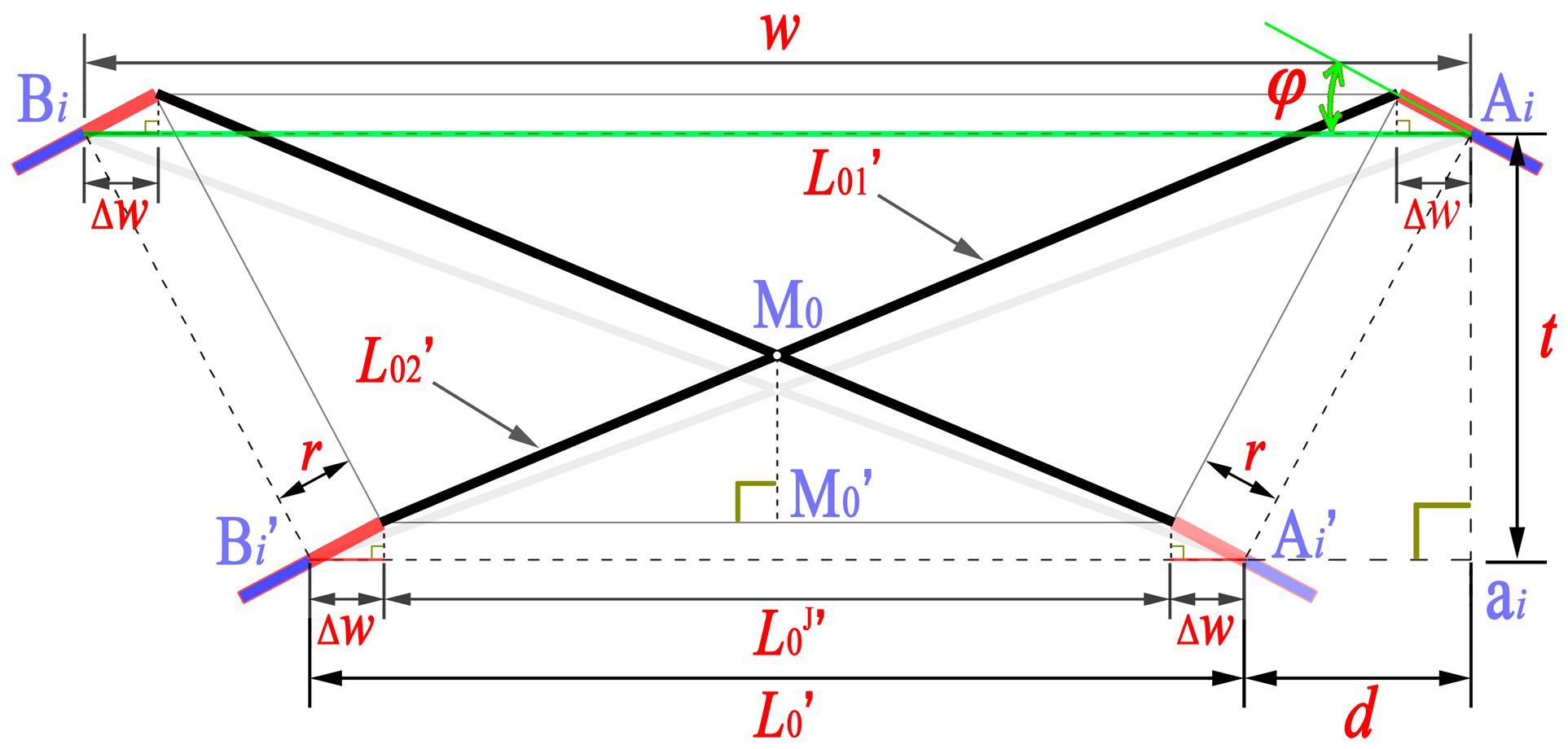
2.3. Mathematical Descriptions of the Structural Profile
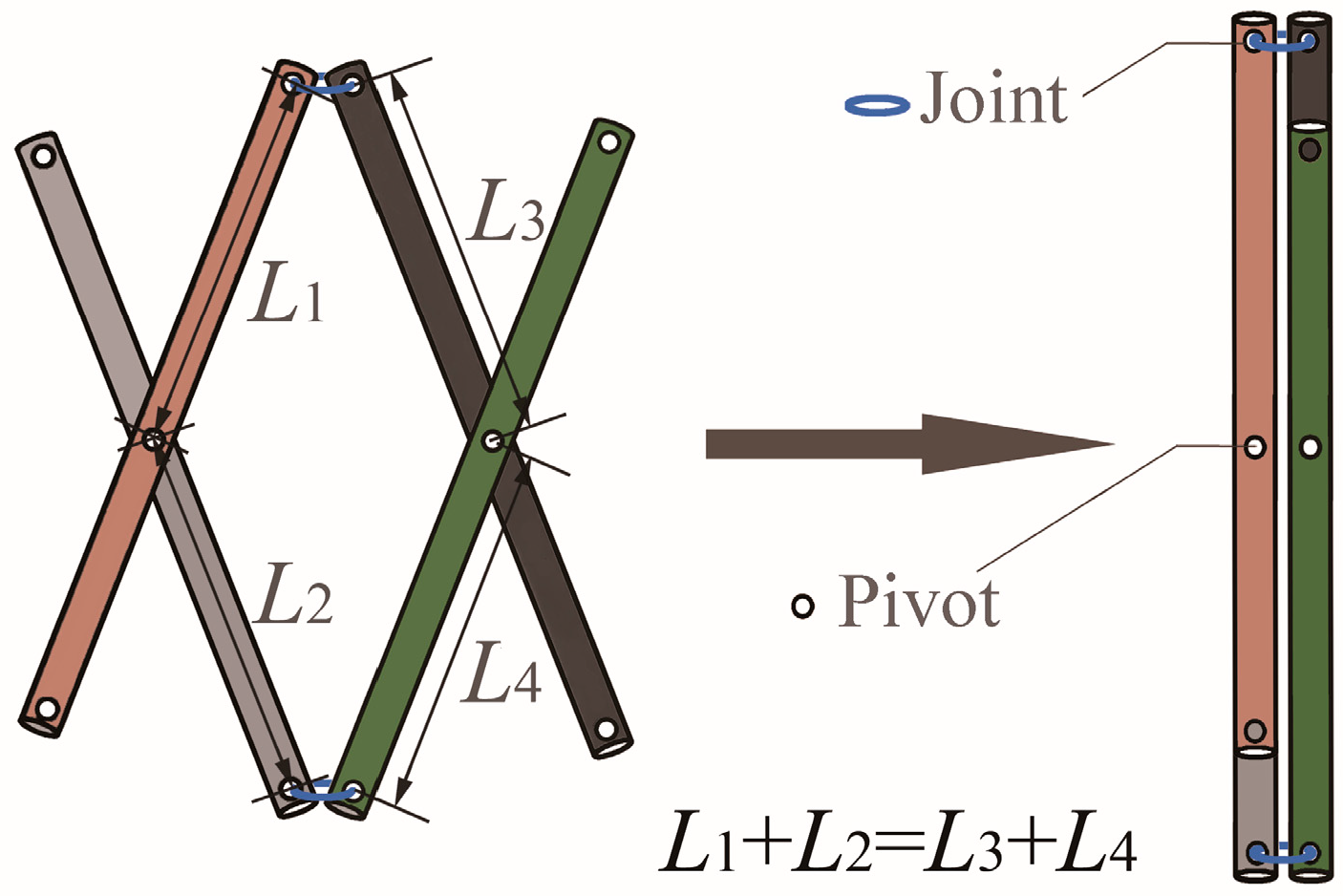
2.4. Geometric Constraints on the Member Lengths of the SU
2.5. Geometric Constraint Equations Describing the Structural Profile
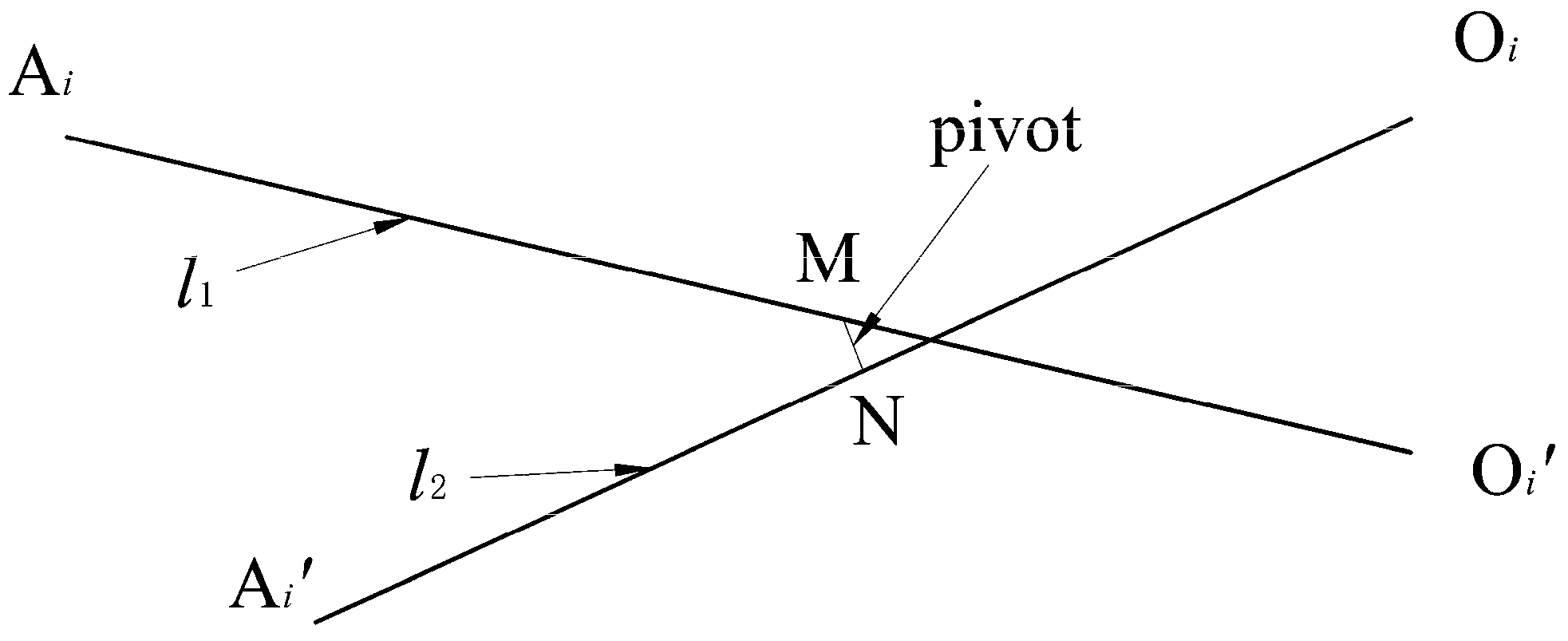
3. Pivot Endpoint Positions on the SLEs
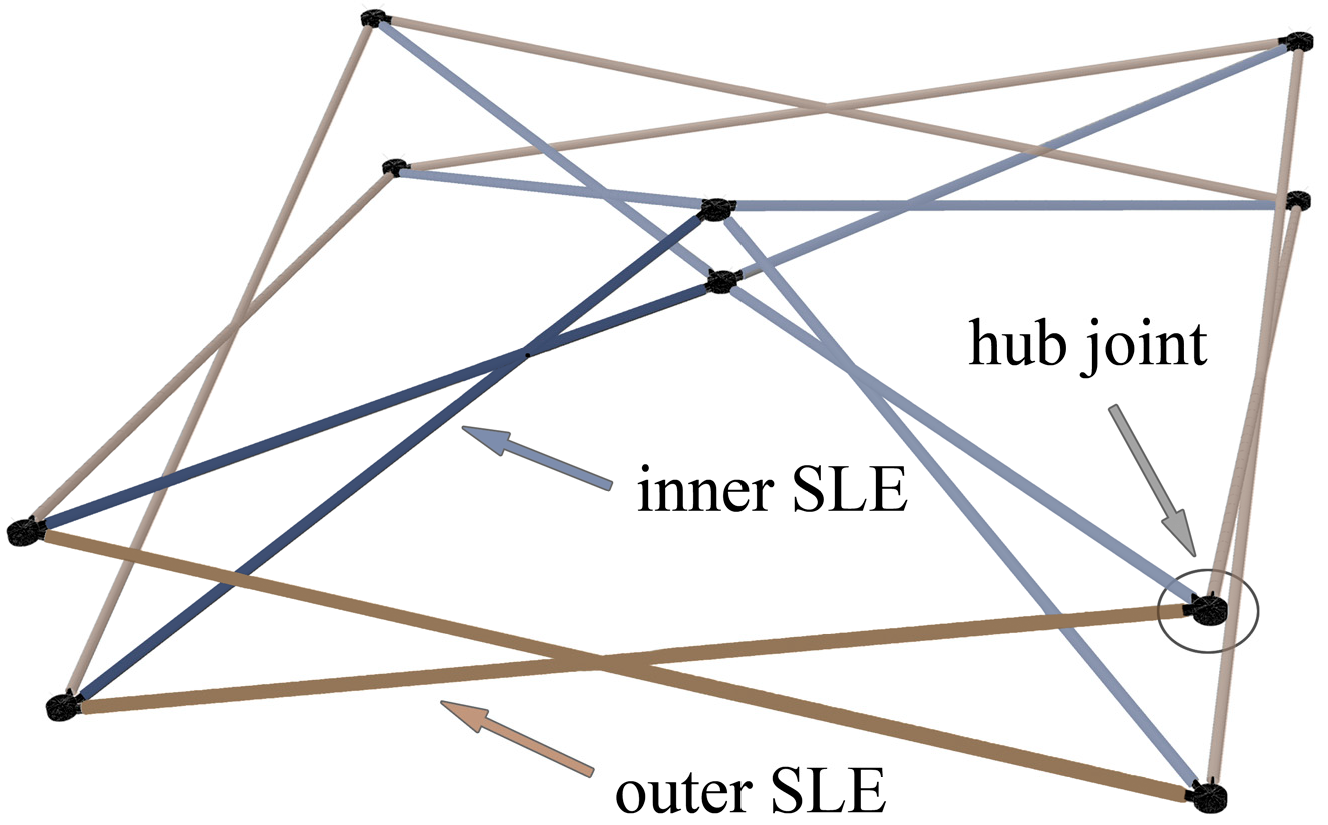
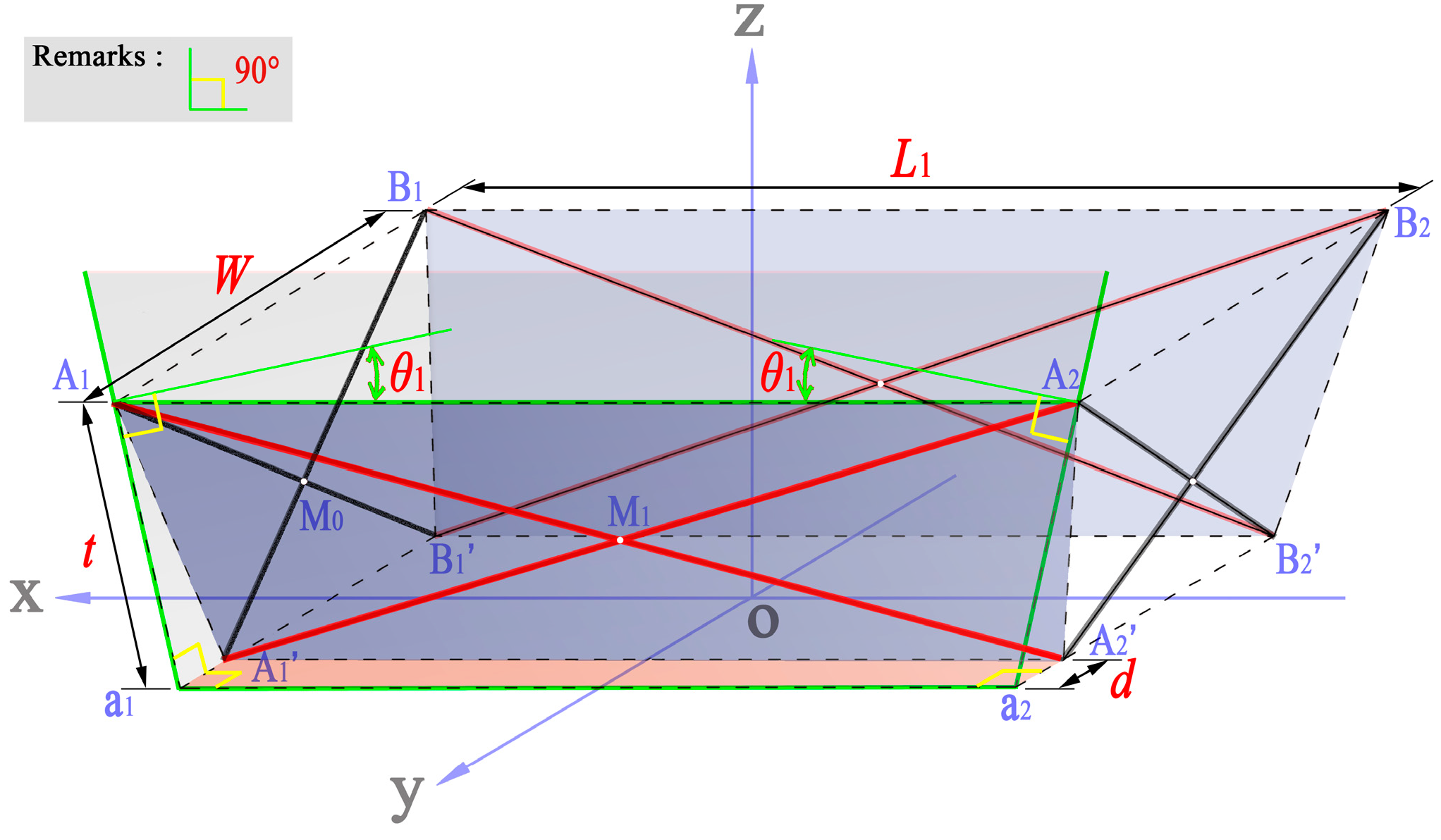
3.1. Outer SLEs of the SU
3.2. Inner SLEs of the SU
4. Geometric Design Methodology Considering Joint Sizes
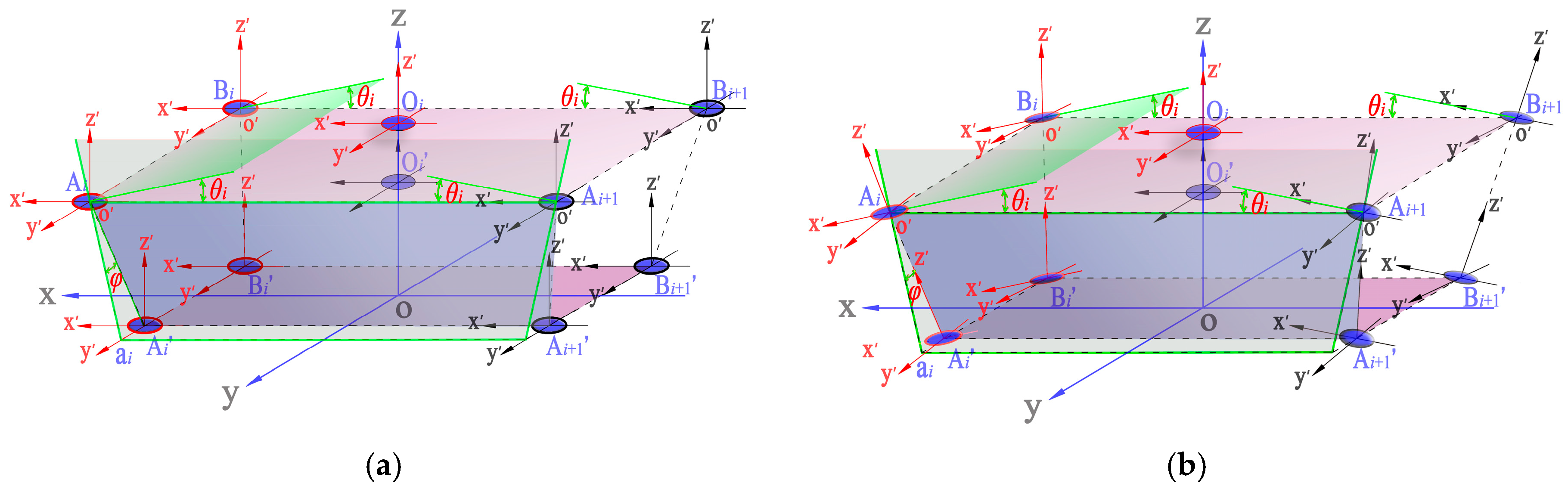
4.1. Spatial Configuration of Sus Considering Joint Dimensions
- The original relative positions between the joint coordinate systems and the unit coordinate system are illustrated in Figure 13a. The ideal joint positions are replaced by the circle centers. The three axes of the coordinate system of one hub joint are all parallel to the corresponding axes of the SU.
- Rotate the joints at points Ai, Ai’, Bi’, and Bi about their y’-axes through an angle θi, and rotate the others in the peripheral SLEs through an angle -θi.
- Rotate these joints again about their x’-axes. The joints at the points Ai, Ai’, Ai+1’, and Ai+1 are rotated through an angle -φ, and the others in the peripheral SLEs are rotated through an angle φ. Accordingly, based on the model construction process, the new unit configuration is obtained (Figure 13b) and (Figure 9).
4.2. Outer SLEs Considering Joint Sizes
4.3. Inner SLEs Considering Joint Sizes
5. Design Examples and Physical Grid Structure
5.1. Design Examples of General Grid Structures
5.2. Design Example of One Self-Locking Grid Structure
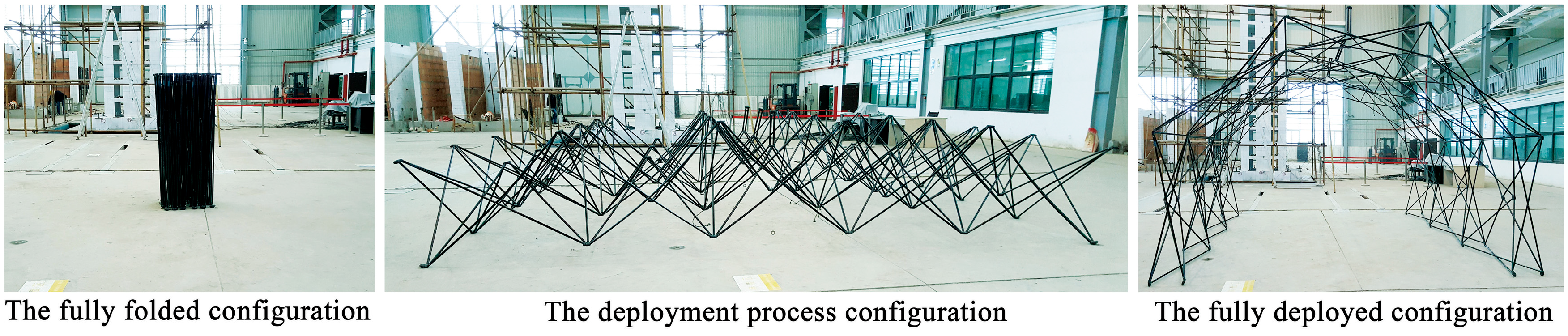
5.3. Physical Self-Locking Grid Structure
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Piñero, E.P. Three Dimensional Reticular Structure. U.S. Patent 3185164, 15 November 1961. [Google Scholar]
- Escrig, F.; Perez Valcarcel, J.; Sanchez, J. Deployable cover on a swimming pool in Seville. J. Int. Assoc. Shell Sp. 1996, 37, 39–70. [Google Scholar]
- Akgün, Y.; Gantes, C.J.; Sobek, W.; Korkmaz, K.; Kalochairetis, K. A novel adaptive spatial scissor-hinge structural mechanism for convertible roofs. Eng. Struct. 2011, 33, 1365–1376. [Google Scholar] [CrossRef]
- Buhl, T.; Jensen, F.V.; Pellegrino, S. Shape optimization of cover plates for retractable roof structures. Comput. Struct. 2004, 82, 1227–1236. [Google Scholar] [CrossRef]
- Wu-Jun, C.; Yao-Zhi, L.; Gong-Yi, F.; Jing-Hai, G.; Shi-Lin, D. A study on space masts based on octahedral truss family. Int. J. Space Struct. 2001, 16, 75–82. [Google Scholar] [CrossRef]
- Shi, Z.; Li, T.; Tang, Y.; Li, M.; Zhou, X.; Qian, J. Design and analysis of scissor-like hoop truss deployable antenna mechanism with arbitrary curvature support ribs. Acta Astronaut. 2024, 219, 24–40. [Google Scholar] [CrossRef]
- Roovers, K.; De Temmerman, N. Deployable scissor grids consisting of translational units. Int. J. Solids Struct. 2017, 121, 45–61. [Google Scholar] [CrossRef]
- Roovers, K.; De Temmerman, N. Geometric design of deployable scissor grids consisting of generalized polar units. J. Int. Assoc. Shell Sp. 2017, 58, 227–238. [Google Scholar] [CrossRef]
- Dinevari, N.F.; Shahbazi, Y.; Maden, F. Geometric and analytical design of angulated scissor structures. Mech. Mach. Theory 2021, 164, 104402. [Google Scholar] [CrossRef]
- García-Mora, C.J.; Sánchez-Sánchez, J. Geometric strategies to design a bistable deployable structure with straight scissors using stiff and flexible rods. Int. J. Solids Struct. 2022, 238, 111381. [Google Scholar] [CrossRef]
- Langbecker, T.; Albermani, F. Kinematic and non-linear analysis of foldable barrel vaults. Eng. Struct. 2001, 23, 158–171. [Google Scholar] [CrossRef]
- Mira, L.A.; Thrall, A.P.; De Temmerman, N. Deployable scissor arch for transitional shelters. Autom. Constr. 2014, 43, 123–131. [Google Scholar] [CrossRef]
- Kaveh, A.; Davaran, A. Analysis of pantograph foldable structures. Comput. Struct. 1996, 59, 131–140. [Google Scholar] [CrossRef]
- Pellegrino, S.; Calladine, C.R. Matrix analysis of statically and kinematically indeterminate frameworks. Int. J. Solids Struct. 1986, 22, 409–428. [Google Scholar] [CrossRef]
- Liao, Y.; Kiper, G.; Krishnan, S. Mobility analysis of tripod scissor structures using screw theory. Mech. Mach. Theory 2024, 191, 105468. [Google Scholar] [CrossRef]
- Arnouts, L.I.; De Temmerman, N.; Massart, T.J.; Berke, P. Geometric design of triangulated bistable scissor structures taking into account finite hub size. Int. J. Solids Struct. 2020, 206, 84–100. [Google Scholar] [CrossRef]
- Liao, Y.; Krishnan, S. Geometric design and kinematics of spatial deployable structures using tripod-scissor units. Structures 2022, 38, 323–339. [Google Scholar] [CrossRef]
- Zhang, R.; Guo, X.; Liu, Y.; Leng, J. Theoretical analysis and experiments of a space deployable truss structure. Compos. Struct. 2014, 112, 226–230. [Google Scholar] [CrossRef]
- Zhao, W.; Li, N.; Liu, L.; Leng, J.; Liu, Y. Mechanical behaviors and applications of shape memory polymer and its composites. Appl. Phys. Rev. 2023, 10, 011306. [Google Scholar] [CrossRef]
- Moy, J.J.; Tan, C.S.; Mohammad, S.; Abidin, A.R.Z. State-of-art review on deployable scissor structure in construction. Structures 2022, 42, 160–180. [Google Scholar] [CrossRef]
- Giménez Azorín, R.; Santana, M.V.B.; Berke, P.Z. Computational investigation of bistable and non-bistable deployable scissor structures. Structures 2024, 61, 106063. [Google Scholar] [CrossRef]
- Santana, M.V.B.; Arnouts, L.I.W.; Massart, T.J.; Gonçalves, P.B.; Berke, P.Z. Corotational 3D joint finite element tailored for the simulation of bistable deployable structures. Eng. Struct. 2021, 227, 111387. [Google Scholar] [CrossRef]
- Gantes, C.J. Deployable Structures: Design and Analysis; WIT Press: Southampton, UK, 2001. [Google Scholar]
- García-Mora, C.J.; Sánchez-Sánchez, J. The convergence surface method for the design of deployable scissor structures. Autom. Const. 2021, 122, 103488. [Google Scholar] [CrossRef]
- Zeigler, T.R. Collapsible Self-Supporting Structures. U.S. Patent 3968808, 13 July 1976. [Google Scholar]
- Zhao, Z.; Hu, W.; Yu, L. Experimental and numerical studies on the deployment process of self-locking cuboid foldable structural units. Adv. Struct. Eng. 2020, 23, 3496–3508. [Google Scholar] [CrossRef]
- Freire-Tellado, M.J.; Muñoz-Vidal, M.; Pérez-Valcárcel, J. Design of diagonalised square-base bistable modules. Arab. J. Sci. Eng. 2024, 49, 5949–5971. [Google Scholar] [CrossRef]
- Santana, M.V.B.; Hjiaj, M.; Berke, P.Z. Modal analysis of a bistable deployable module with a refined joint model. Eng. Struct. 2022, 269, 114798. [Google Scholar] [CrossRef]
- Pérez-Valcárcel, J.; Muñoz-Vidal, M.; Suárez-Riestra, F.; López-César, I.R.; Freire-Tellado, M.J. Deployable cylindrical vaults with reciprocal linkages for emergency buildings. Structures 2021, 33, 4461–4474. [Google Scholar] [CrossRef]
- Pérez-Valcárcel, J.; Muñoz-Vidal, M.; López-César, I.R.; Freire-Tellado, M.J.; Suárez-Riestra, F. Analysis of deployable cylindrical space bar structures of reciprocal linkages with frustoconical ends. Designs 2024, 8, 46. [Google Scholar] [CrossRef]
- Zhao, Z.; Yu, L.; Hu, W. Self-locking mechanism of foldable grid structures and capability evaluation of their structural units. Structures 2020, 27, 583–594. [Google Scholar] [CrossRef]
- Gantes, C.J.; Connor, J.J.; Logcher, R.D.; Rosenfeld, Y. Structural analysis and design of deployable structures. Comput. Struct. 1989, 32, 661–669. [Google Scholar] [CrossRef]
- Mira, L.A.; Coelho, R.F.; Thrall, A.P.; De Temmerman, N. Parametric evaluation of deployable scissor arches. Eng. Struct. 2015, 99, 479–491. [Google Scholar] [CrossRef]
- Escrig, F.; Valcarcel, J.P. Geometry of expandable space structures. Int. J. Space Struct. 1993, 8, 71–84. [Google Scholar] [CrossRef]
- Wujun, C.; Gongyi, F.; Jinghai, G.; Yanli, H.; Shilin, D. A new design conception for large span deployable flat grid structures. Int. J. Space Struct. 2002, 17, 293–299. [Google Scholar] [CrossRef]
- Kim, T.H.; Suh, J.E.; Han, J.H. Deployable truss structure with flat-form storability using scissor-like elements. Mech. Mach. Theory 2021, 159, 104252. [Google Scholar] [CrossRef]
- Gantes, C.J.; Konitopoulou, E. Geometric design of arbitrarily curved bi-stable deployable arches with discrete joint size. Int. J. Solids Struct. 2004, 41, 5517–5540. [Google Scholar] [CrossRef]







| Parameter | Unit | Shape Type | |||
|---|---|---|---|---|---|
| a | b | c | d | ||
| n | 3 | 4 | 3 | 4 | |
| S/2 | mm | 2020.00 | 3010.00 | 1465.90 | 3000.00 |
| W | 1540.64 | 1271.07 | 1110.26 | 1110.26 | |
| t | 300.00 | 300.00 | 300.00 | 300.00 | |
| d | 0.00 | 0.00 | 0.00 | 0.00 | |
| H1 | 1540.64 | 1305.99 | 1138.03 | 1145.00 | |
| H2 | 2790.32 | 2353.31 | 2218.49 | 2122.05 | |
| H3 | 2790.32 | 2934.53 | 3000.00 | 2732.45 | |
| H4 | / | 2934.53 | / | 3000.00 | |
| φ | rad | 0.0000 | 0.0000 | 0.0000 | 0.0000 |
| θ1 | 0.0000 | 0.2244 | 0.1171 | 0.1662 | |
| θ2 | 0.7854 | 0.2244 | 0.0259 | 0.2843 | |
| θ3 | 0.0000 | 0.2244 | 0.6424 | 0.1063 | |
| θ4 | / | 0.2244 | / | 0.2287 | |
| L’ | mm | 1569.58 | 1305.99 | 1150.09 | 1150.09 |
| L1 | 1540.64 | 1339.58 | 1145.88 | 1160.99 | |
| L2 | 1767.31 | 1339.58 | 1118.07 | 1197.59 | |
| L3 | 1540.64 | 1339.58 | 1304.46 | 1142.55 | |
| L4 | / | 1339.58 | / | 1180.34 | |
| Structural Configuration | Outer SLEs | Inner SLEs | ||||||
|---|---|---|---|---|---|---|---|---|
| n | 3 | L0J’ | mm | 1491.64 | LA1M | mm | 739.99 | |
| S/2 | mm | 2020.00 | L’ | 1521.52 | LMO1′ | 303.15 | ||
| W | 1540.64 | L01’ | 760.76 | LA1′N | 781.53 | |||
| t | 300.00 | L02’ | 760.76 | LNO1 | 321.35 | |||
| d | 0.00 | L1J | 1495.50 | LA2M | 818.42 | |||
| r | 24.50 | L1J’ | 1487.80 | LMO2′ | 301.02 | |||
| H1 | 1544.37 | L11’ | 762.71 | LA2′N | 703.09 | |||
| H2 | 2790.32 | L12’ | 758.79 | LNO2 | 317.84 | |||
| H3 | 2790.32 | L2J | 1710.21 | LA3M | 743.34 | |||
| θ1 | rad | 0.0128 | L2J’ | 1301.00 | LMO3′ | 303.16 | ||
| θ2 | 0.7505 | L21’ | 864.15 | LA3′N | 778.17 | |||
| θ3 | 0.0441 | L22’ | 657.37 | LNO3 | 321.43 | |||
| L’ | mm | 1521.51 | L3J | 1504.93 | ||||
| L1 | 1544.49 | L3J’ | 1478.47 | |||||
| L2 | 1746.05 | L31’ | 767.50 | |||||
| L3 | 1553.88 | L32’ | 754.01 | |||||
| Rotation Matrix and Position Vector | First Unit | Second Unit | Third Unit | |
|---|---|---|---|---|
| / | ||||
| mm | (−17.32, −17.32, 0.00)T | (−17.32, −17.32, 0.00)T | (−17.32, −17.32, 0.00)T | |
| (772.25, 770.32, 299.97)T | (873.02, 770.32, 219.40)T | (776.94, 770.32, 299.70)T | ||
| (754.92, 753.00, 300.20)T | (860.35, 753.00, 231.22)T | (759.63, 753.00, 300.47)T | ||
| (−17.32, −17.32, 0.00)T | (−17.32, −17.32, 0.00)T | (−17.32, −17.32, 0.00)T | ||
| (768.40, 770.32, 0.00)T | (668.42, 770.32, 0.00)T | (763.71, 770.32, 0.00)T | ||
| (751.07, 753.00, 0.22)T | (655.75, 753.00, 11.82)T | (746.40, 753.00, 0.76)T | ||
| (17.32, 17.32, 370.00)T | (17.32, 17.32, 317.60)T | (17.32, 17.32, 370.00)T | ||
| (17.32, 17.32, 246.71)T | (17.32, 17.32, 196.22)T | (17.32, 17.32, 246.32)T | ||
| M | (231.68, 231.12, 262.25)T | (244.01, 215.15, 205.63)T | (232.36, 230.44, 262.01)T | |
| N | (231.12, 231.68, 262.27)T | (216.08, 246.36, 222.40)T | (230.44, 232.37, 262.07)T | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, Z.; Yu, L. Geometric Design Methodology for Deployable Self-Locking Semicylindrical Structures. Buildings 2024, 14, 1690. https://doi.org/10.3390/buildings14061690
Zhao Z, Yu L. Geometric Design Methodology for Deployable Self-Locking Semicylindrical Structures. Buildings. 2024; 14(6):1690. https://doi.org/10.3390/buildings14061690
Chicago/Turabian StyleZhao, Zhanwei, and Lei Yu. 2024. "Geometric Design Methodology for Deployable Self-Locking Semicylindrical Structures" Buildings 14, no. 6: 1690. https://doi.org/10.3390/buildings14061690





