Effect of Bidirectional Hysteretic Dampers on the Seismic Performance of Skewed Multi-Span Highway Bridges
Abstract
:1. Introduction
2. Prototype Bridge Description
Modeling Details for the Case Studies
3. Hysteretic Damper Design
3.1. Probabilistic Seismic Hazard Analysis (PSHA)

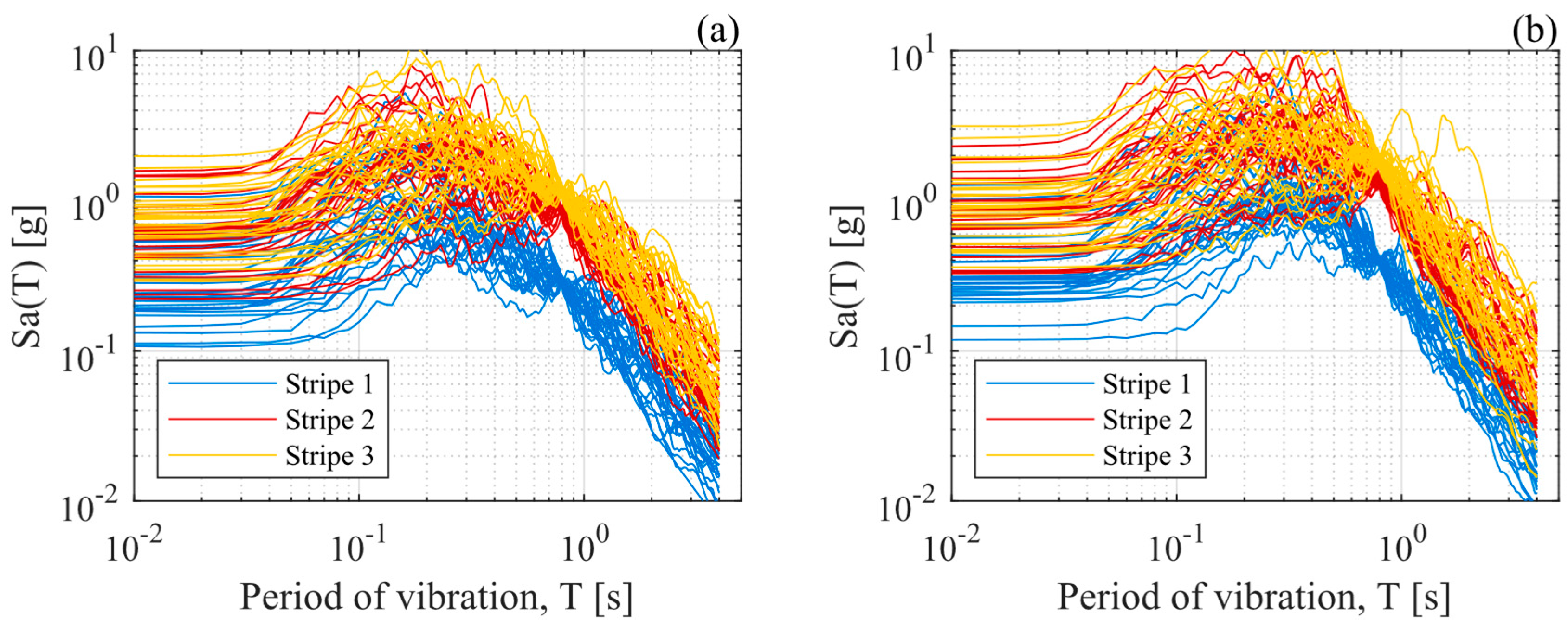
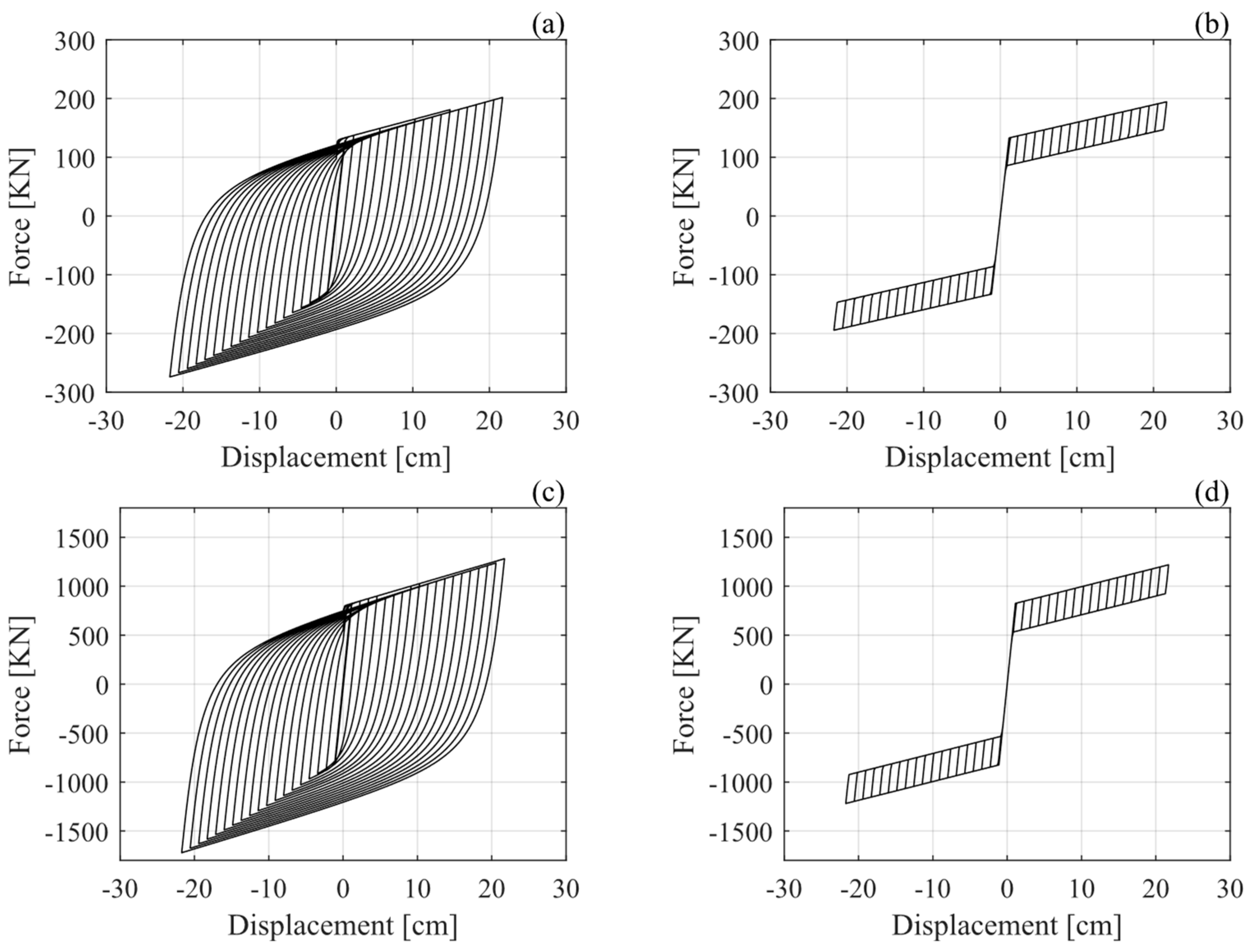
3.2. Damper Design Variables
3.3. Damper Design Results
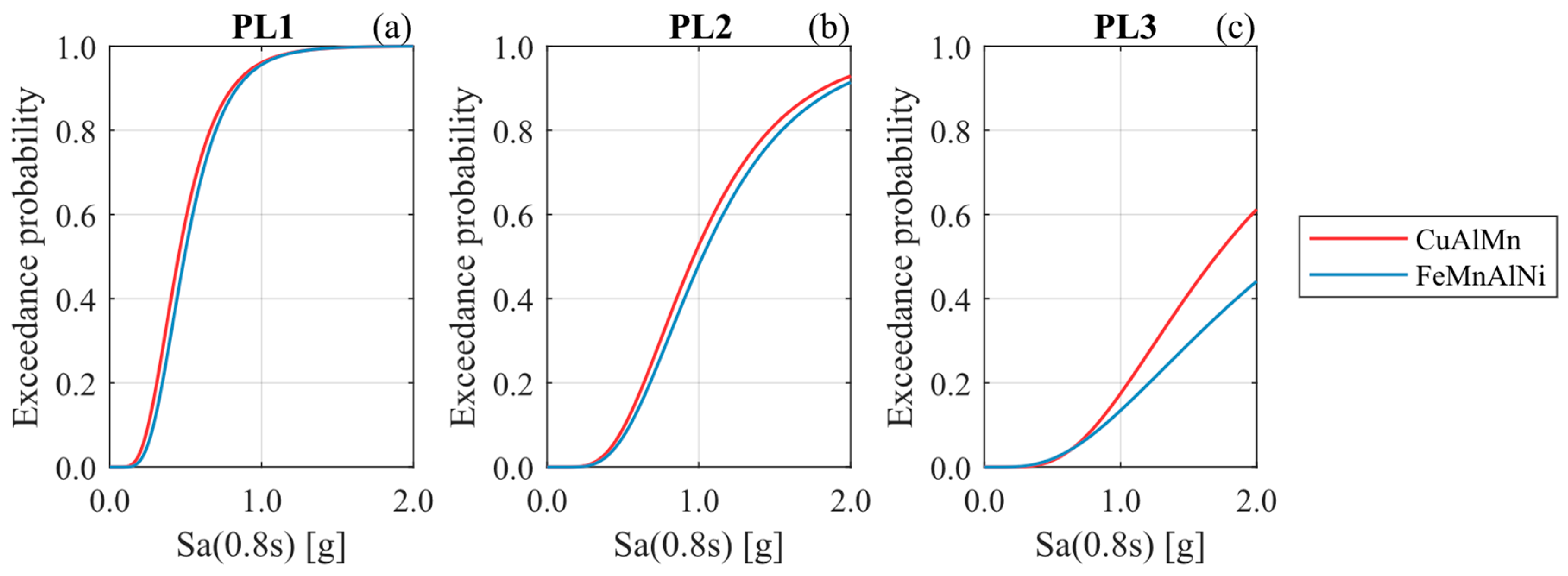
3.4. Design Verification
Multiple Stripe Analysis and Probability of Damage
4. Effect of Hysteretic Dampers on Seismic Performance
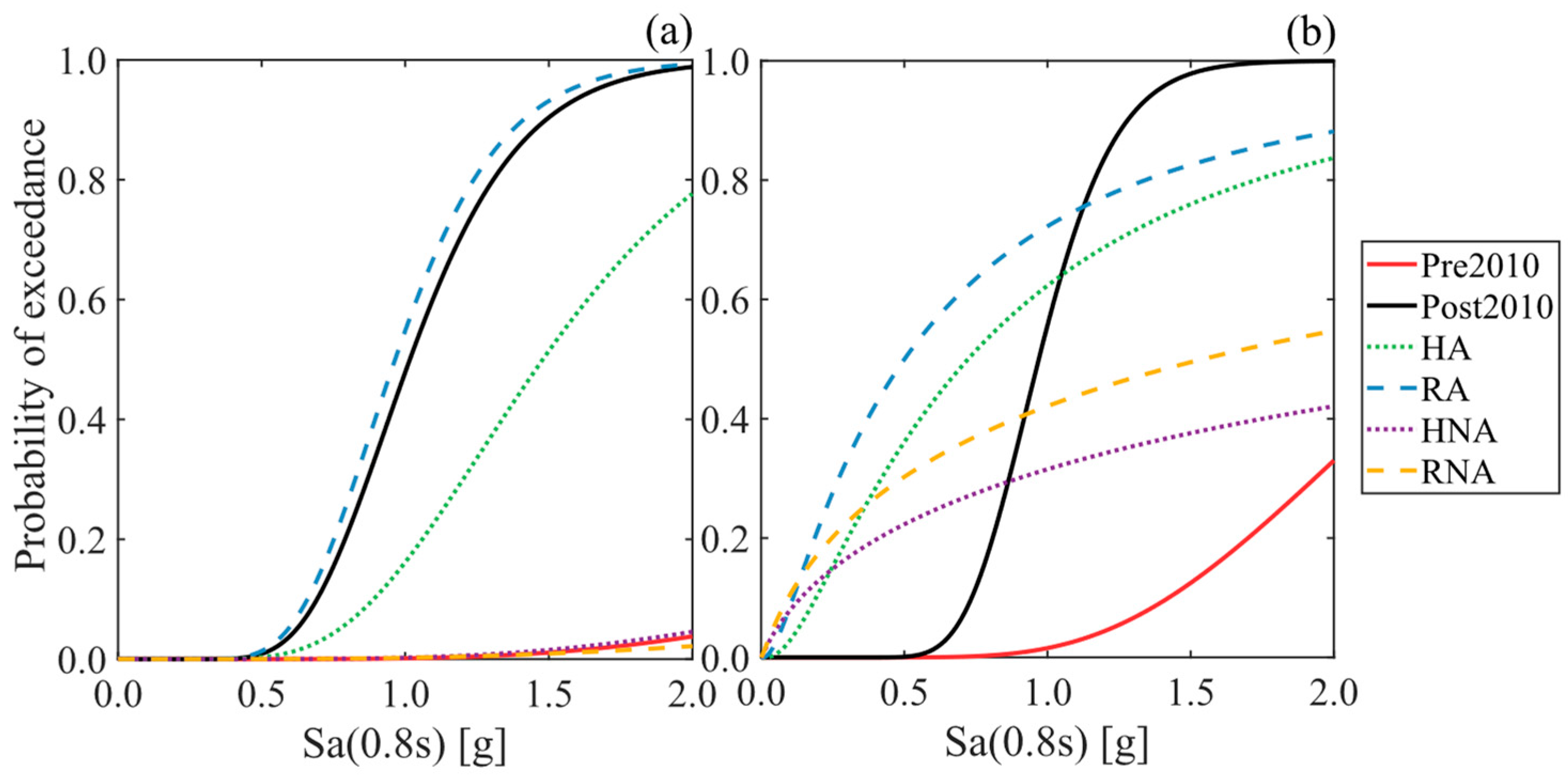
4.1. Influence of Dampers’ Direction
4.2. Influence of Dampers on the Substructure Response
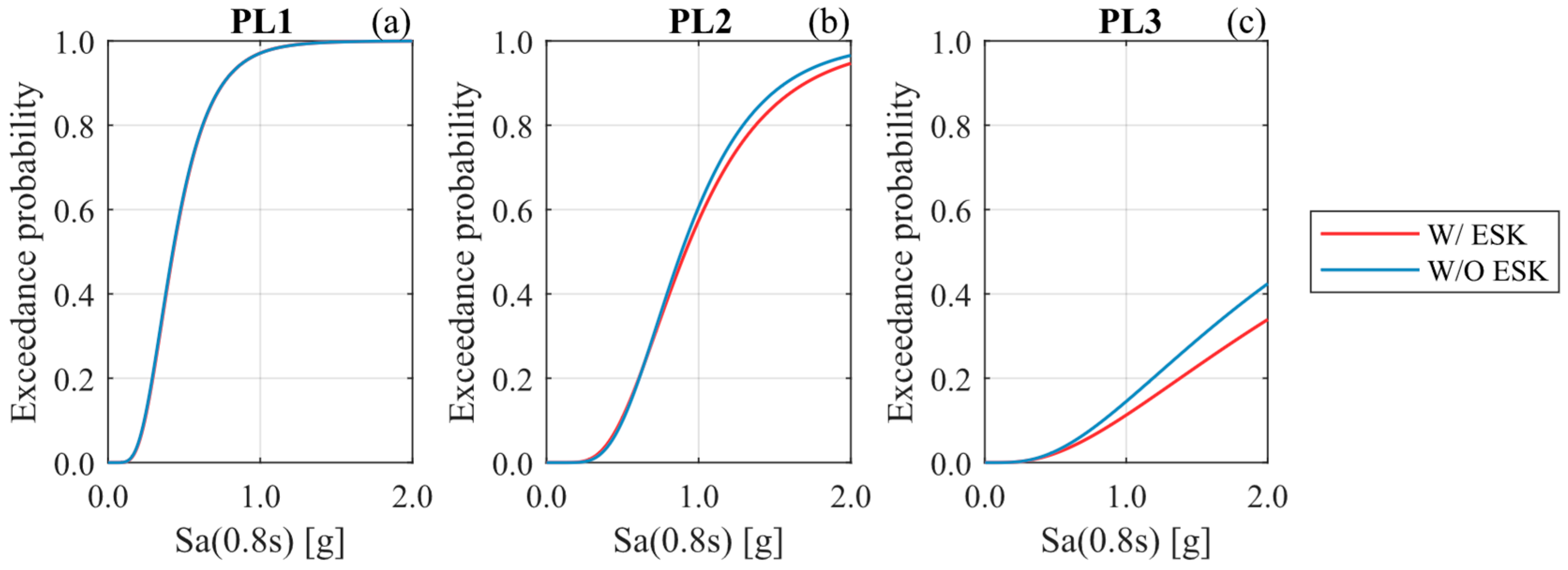
4.3. Influence of Including External Shear Keys with Hysteretic Dampers
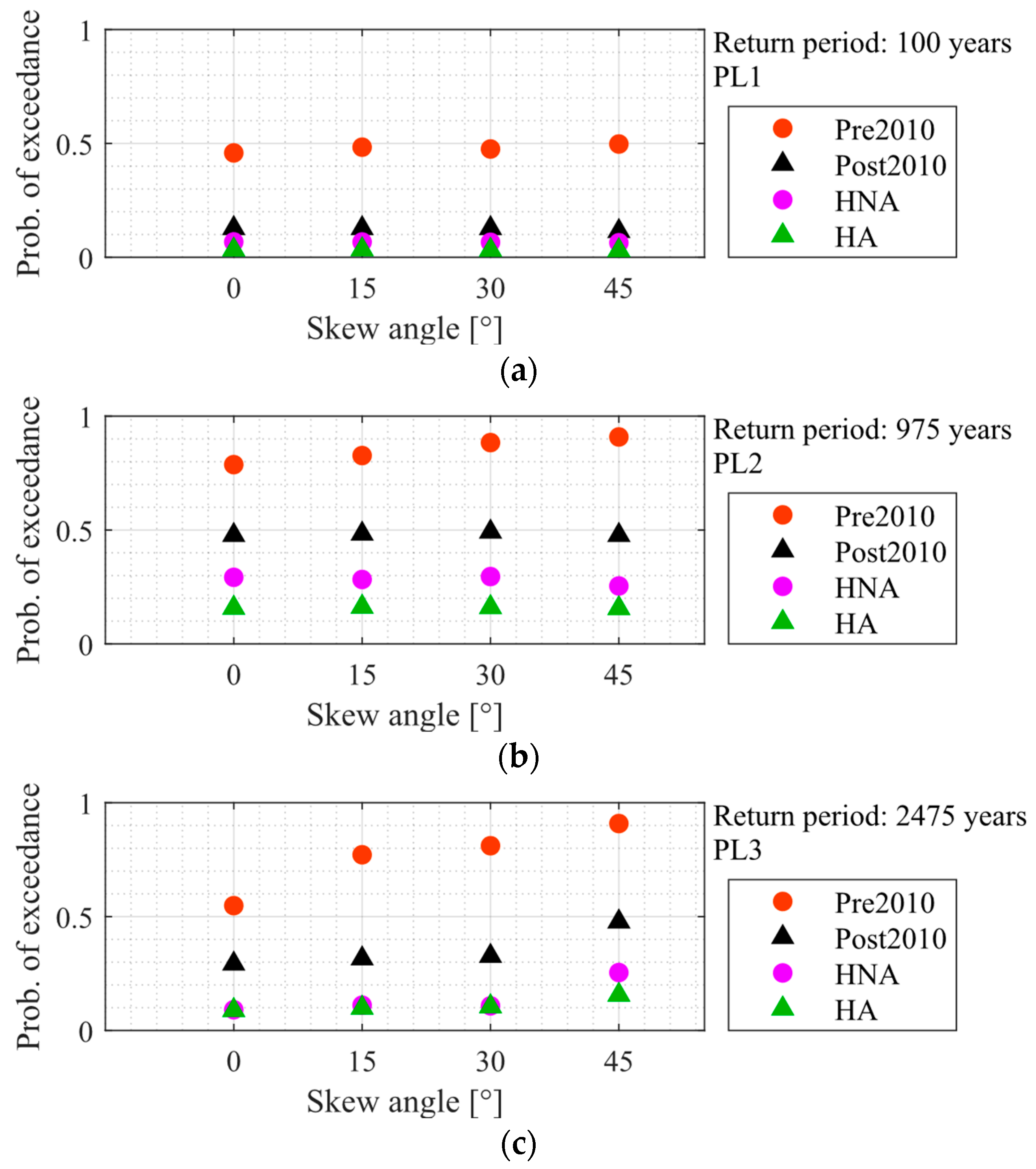
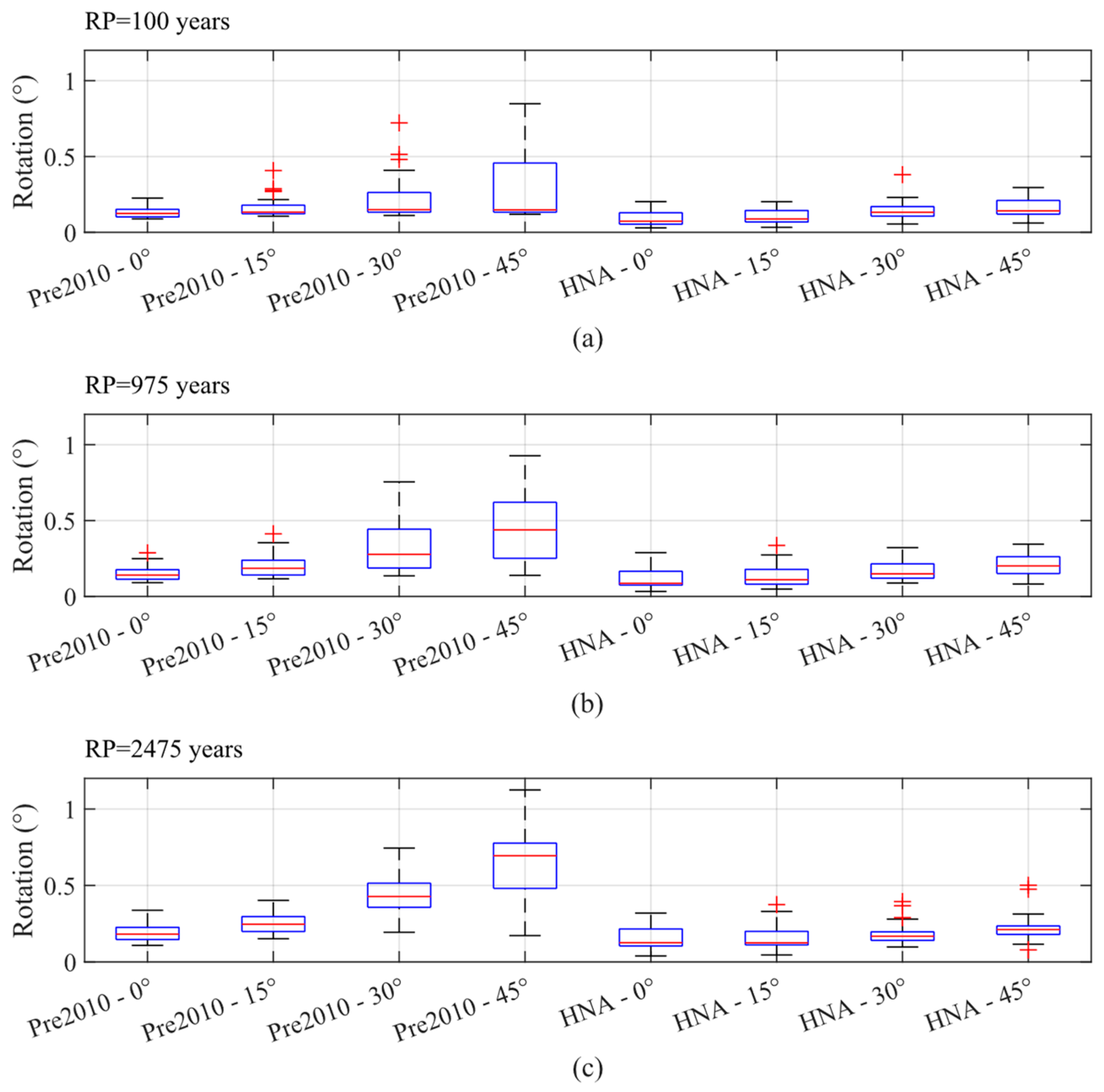
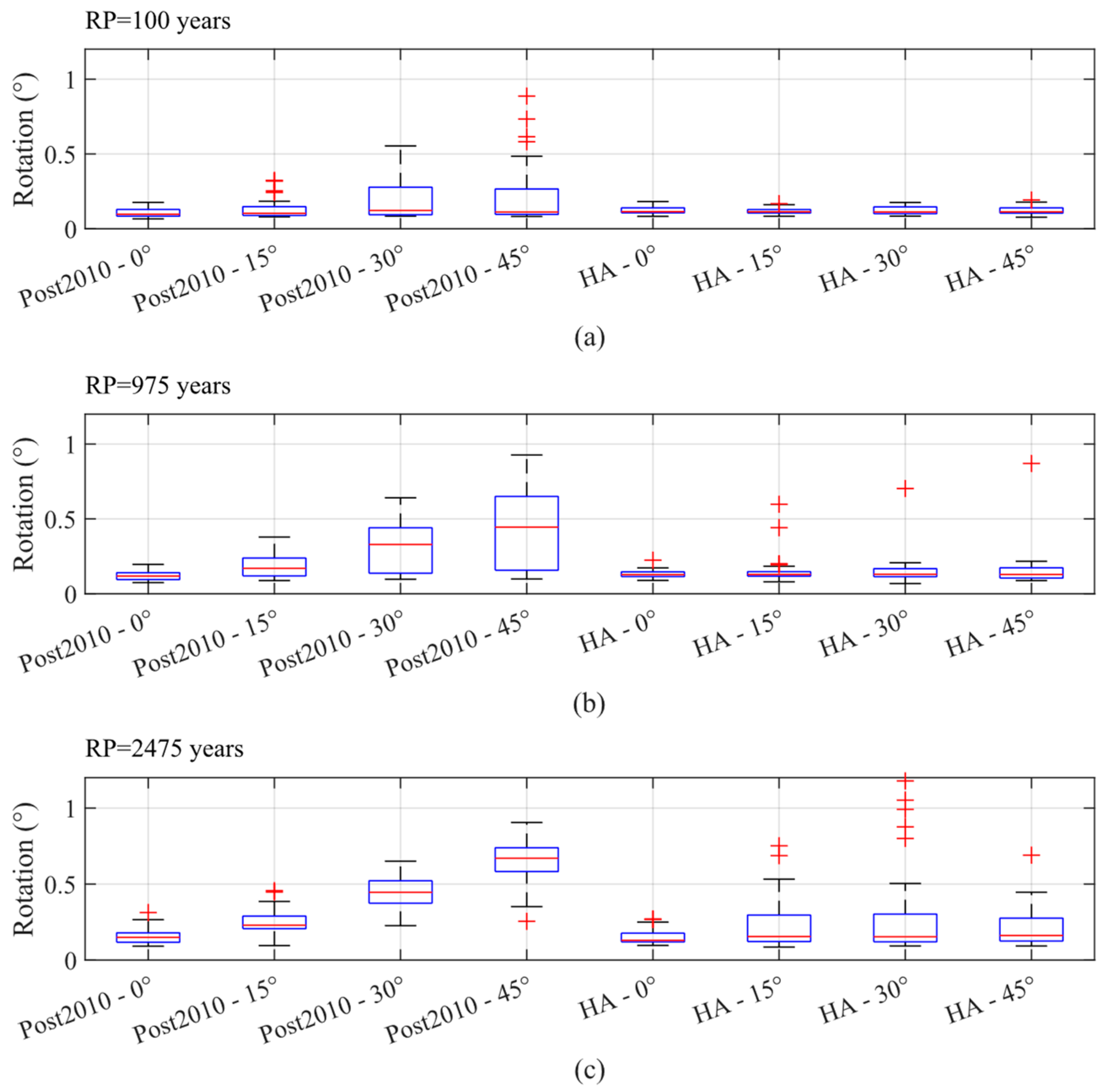
4.4. Influence of Skew Angle
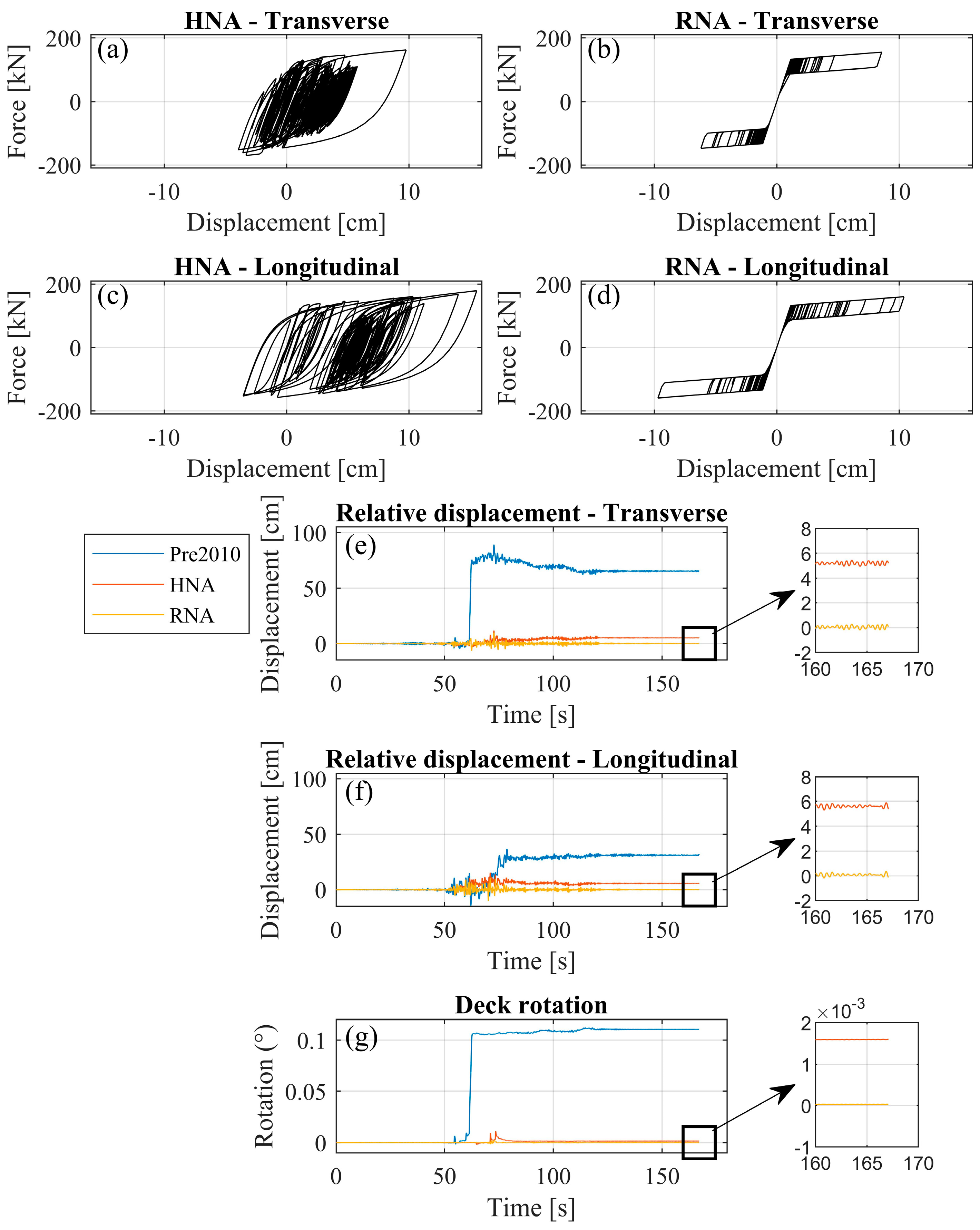
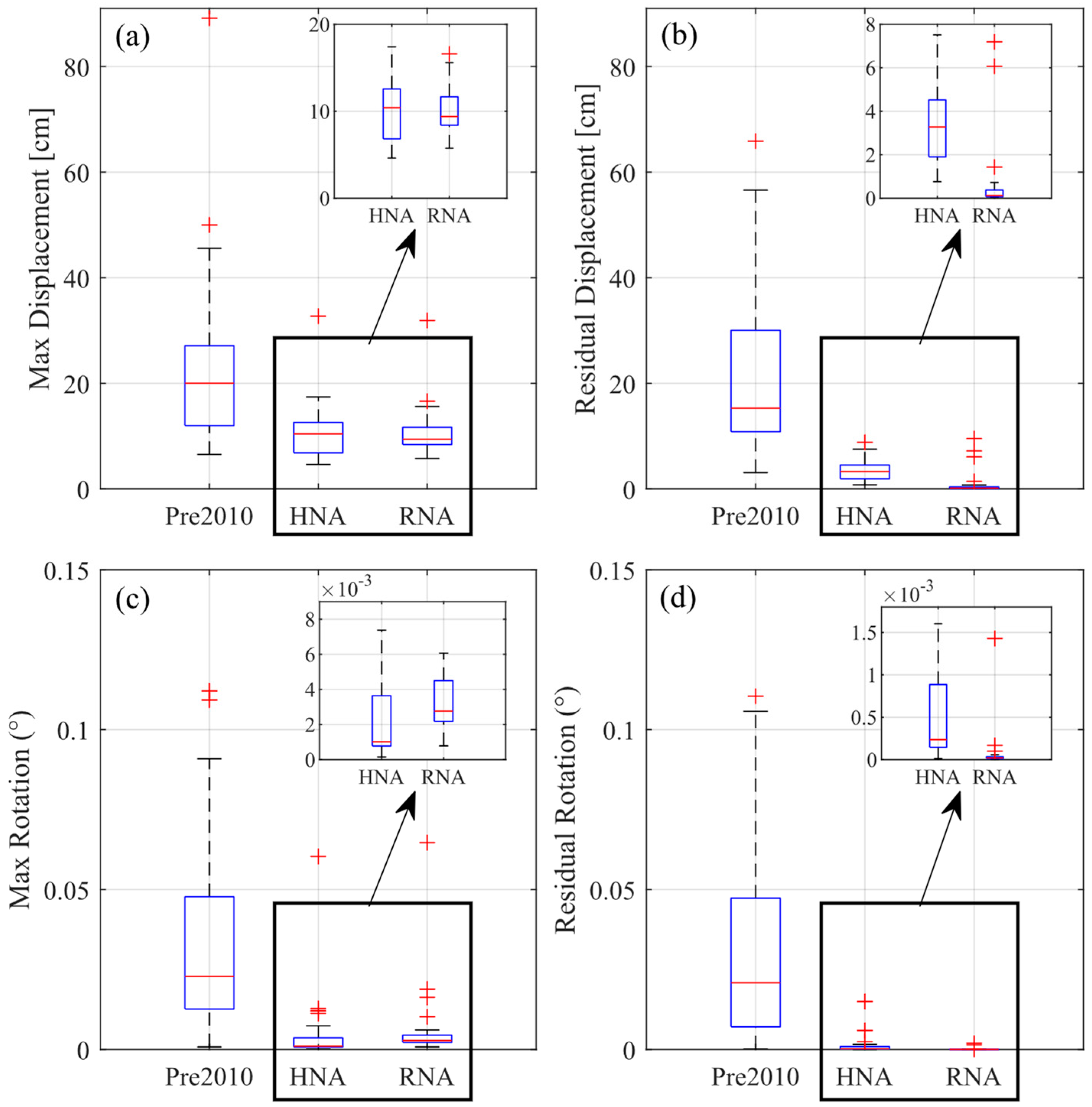
4.5. Influence of Recentering Dampers
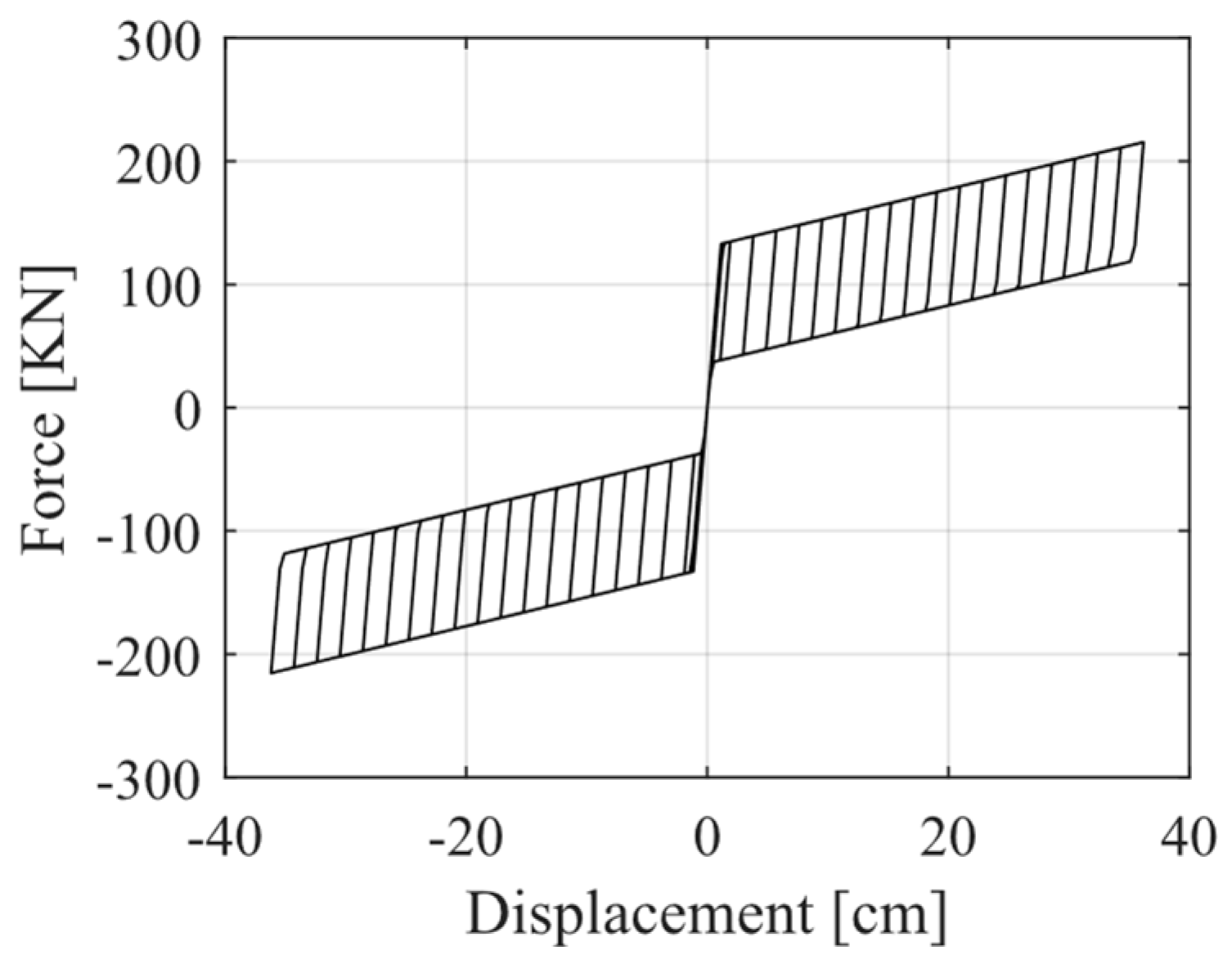
4.6. Influence of the Hysteretic Curve of Recentering Dampers
5. Summary and Conclusions
- Bidirectional hysteretic dampers with and without recentering capabilities are an effective measure to improve the seismic performance of skewed multi-span highway bridges compared to Chilean bridges built prior to 2010 (i.e., pre-2010) and repaired after the 2010 Maule earthquake (i.e., post-2010). In this context, bidirectional dampers were able to reduce the probability of damage by at least 20% compared to the pre-2010 and post-2010 cases. The improvement in seismic performance was even noticeable at lower levels of ground-motion intensity (PL1), something that traditional seismic bars and reinforced concrete shear keys in the post-2010 case failed to achieve since shear keys only activate at high transverse displacements.
- This study provides new evidence that unidirectional hysteretic dampers, which are placed only in the transverse direction, as suggested by other researchers [17], are insufficient for achieving desired performance levels. The probability of damage increased by at least a factor of 2.0 when unidirectional dampers were considered instead of bidirectional dampers. This finding underscores the necessity for bidirectional dampers, which are essential for achieving operational performance levels in skewed bridges.
- From a capacity design perspective, the results showed that hysteretic dampers can effectively reduce the seismic demands transmitted to the substructure in certain cases while they can increase them in others. Hence, it is essential to conduct capacity design checks on the substructure to avoid excessive demand and potential damage. The damper design parameters need to be adjusted in cases where large forces are transferred to the bents and foundations. This step ensures that the benefits of dampers do not lead to unintended structural issues. Moreover, this study suggests that including external RC shear keys, despite their slight impact on seismic performance when used with dampers, is crucial to prevent span unseating in the case of damper failure.
- For bridges without dampers, this study confirms that rotational demands and damage probability significantly increase with the skew angle, especially for PL3. Conversely, it is worth highlighting that the skew angle has a minimal influence on the rotational response and seismic fragility of bridges equipped with bidirectional hysteretic dampers and anchored elastomeric bearings. This negligible effect of the skew angle on damage probability is a significant finding, as using hysteretic dampers might allow for isolating the skew angle from bridge fragility considerations. This observation presents an additional advantage of employing bidirectional energy-dissipation devices in skewed bridges.
- Bidirectional hysteretic dampers, particularly those with recentering capabilities, are recommended for improving seismic performance. They effectively reduce both displacements and rotations, minimizing the probability of damage. Dampers should be installed in both transverse and longitudinal directions to ensure comprehensive seismic performance improvements. The superior performance of recentering dampers in reducing residual responses is particularly important for achieving fully operational performance levels, where stringent residual displacement limits may be imposed, making recentering dampers preferable.
- Recentering dampers with different materials or hysteretic curves slightly affect the fragility at PL2 and PL3 since there is no significant increase in energy dissipation. Further analysis and experimental research must be conducted regarding residual displacements and the practical application of this type of device.
- This study provides valuable insights that can be directly used in engineering applications, particularly in the design and retrofitting of skewed highway bridges to enhance their seismic performance. While the performance-based design methodology used in this study can be implemented globally, the specific performance objectives are tailored for the case of Chile. For different regions, performance objectives can be referenced from various standards, such as NCHRP-949 [52] and the new AASHTO Guidelines for Performance-Based Seismic Design of Highway Bridges [89] for the United States and CSA-S6 [53] for Canada.
- A large amount of experimental and numerical research is still warranted to attain more reliable damage states of various bridge components such as shear keys, laminated elastomeric bearings, RC columns, abutments, and bidirectional hysteretic dampers. Furthermore, conducting a sensitivity analysis on the mechanical properties of the dampers would be highly beneficial for understanding the robustness and extent of the findings presented in this study.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Jennings, P. Engineering Features of the San Fernando Earthquake of February 9, 1971; Report 71-02; Earthquake Engineering Research Laboratory: Pasadena, CA, USA, 1971. [Google Scholar]
- Wakefield, R.R.; Nazmy, A.S.; Billington, D.P. Analysis of seismic failure in skew RC bridge. J. Struct. Eng. 1991, 117, 972–986. [Google Scholar] [CrossRef]
- Mitchell, D.; Bruneau, M.; Williams, M.; Anderson, D.; Saatcioglu, M.; Sexsmith, R. Performance of bridges in the 1994 Northridge earthquake. Can. J. Civ. Eng. 1995, 22, 415–427. [Google Scholar] [CrossRef]
- Han, Q.; Du, X.; Liu, J.; Li, Z.; Li, L.; Zhao, J. Seismic damage of highway bridges during the 2008 Wenchuan earthquake. Earthq. Eng. Eng. Vib. 2009, 8, 263–273. [Google Scholar] [CrossRef]
- Kawashima, K.; Takahashi, Y.; Ge, H.; Wu, Z.; Zhang, J. Reconnaissance report on damage of bridges in 2008 Wenchuan, China, earthquake. J. Earthq. Eng. 2009, 13, 965–996. [Google Scholar] [CrossRef]
- Buckle, I.; Hube, M.; Chen, G.; Yen, W.; Arias, J. Structural performance of bridges in the offshore Maule earthquake of 27 February 2010. Earthq. Spectra 2012, 28 (Suppl. S1), 533–552. [Google Scholar] [CrossRef]
- Kawashima, K.; Unjoh, S.; Hoshikuma, J.; Kosa, K. Damage of bridges due to the 2010 Maule, Chile, earthquake. J. Earthq. Eng. 2011, 15, 1036–1068. [Google Scholar] [CrossRef]
- Wilches, J.; Santa Maria, H.; Leon, R.; Riddell, R.; Hube, M.; Arrate, C. Evolution of seismic design codes of highway bridges in Chile. Earthq. Spectra 2021, 37, 2174–2204. [Google Scholar] [CrossRef]
- Dimitrakopoulos, E.G. Seismic response analysis of skew bridges with pounding deck-abutment joints. Eng. Struct. 2011, 33, 813–826. [Google Scholar] [CrossRef]
- Deepu, S.P.; Prajapat, K.; Ray-Chaudhuri, S. Seismic vulnerability of skew bridges under bi-directional ground motions. Eng. Struct. 2014, 71, 150–160. [Google Scholar] [CrossRef]
- Kun, C.; Jiang, L.; Chouw, N. Influence of pounding and skew angle on seismic response of bridges. Eng. Struct. 2017, 148, 890–906. [Google Scholar] [CrossRef]
- Wu, S.; Buckle, I.G.; Itani, A.M.; Istrati, D. Experimental Studies on Seismic Response of Skew Bridges with Seat-Type Abutments. I: Shake Table Experiments. J. Bridge Eng. 2019, 24, 04019097. [Google Scholar] [CrossRef]
- Ministerio de Obras Públicas (MOP). Manual de Carreteras, Volumen 3; MOP: Santiago, Chile, 2020; Available online: https://mc.mop.gob.cl/ (accessed on 22 October 2020).
- Wilches, J.; Santa María, H.; Riddell, R.; Arrate, C. Effects of changes in seismic design criteria in the transverse and vertical response of Chilean highway bridges. Eng. Struct. 2019, 191, 370–385. [Google Scholar] [CrossRef]
- Aldea, S.; Bazaez, R.; Astroza, R.; Hernandez, F. Seismic fragility assessment of Chilean skewed highway bridges. Eng. Struct. 2021, 249, 113300. [Google Scholar] [CrossRef]
- American Association of State Highway and Transportation Officials (AASHTO). AASHTO: Standard Specification for Highway Bridges, 16th ed.; AASHTO: Washington, DC, USA, 1996. [Google Scholar]
- Xiang, N.; Li, J. Seismic Performance of Highway Bridges with Different Transverse Unseating-Prevention Devices. J. Bridge Eng. 2016, 21, 04016045. [Google Scholar] [CrossRef]
- Xiang, N.; Alam, M.S. Comparative Seismic Fragility Assessment of an Existing Isolated Continuous Bridge Retrofitted with Different Energy Dissipation Devices. J. Bridge Eng. 2019, 24, 04019070. [Google Scholar] [CrossRef]
- Xiang, N.; Alam, M.S.; Li, J. Shake table studies of a highway bridge model by allowing the sliding of laminated-rubber bearings with and without restraining devices. Eng. Struct. 2018, 171, 583–601. [Google Scholar] [CrossRef]
- El-Bahey, S.; Bruneau, M. Buckling restrained braces as structural fuses for the seismic retrofit of reinforced concrete bridge bents. Eng. Struct. 2011, 33, 1052–1061. [Google Scholar] [CrossRef]
- Bazaez, R.; Dusicka, P. Repair of seismically damaged RC bridge bent with ductile steel bracing. Steel Compos. Struct. 2018, 26, 745–757. [Google Scholar] [CrossRef]
- Bazaez, R.; Dusicka, P. Performance assessment of multi-column RC bridge bents seismically retrofitted with buckling-restrained braces. Bull. Earthq. Eng. 2018, 16, 2135–2160. [Google Scholar] [CrossRef]
- Bazaez, R.; Dusicka, P. Cyclic behavior of reinforced concrete bridge bent retrofitted with buckling restrained braces. Eng. Struct. 2016, 119, 34–48. [Google Scholar] [CrossRef]
- Wang, Y.; Ibarra, L.; Pantelides, C. Collapse capacity of reinforced concrete skewed bridges retrofitted with buckling-restrained braces. Eng. Struct. 2019, 184, 99–114. [Google Scholar] [CrossRef]
- Wang, Y.; Ibarra, L.; Pantelides, C. Seismic Retrofit of a Three-Span RC Bridge with Buckling-Restrained Braces. J. Bridge Eng. 2016, 21, 04016073. [Google Scholar] [CrossRef]
- Mahjoubi, S.; Maleki, S. Pipe dampers as passive devices for seismic control of isolated bridges. Struct. Control. Health Monit. 2017, 24, e1869. [Google Scholar] [CrossRef]
- Celik, O.C.; Bruneau, M. Skewed Slab-on-Girder Steel Bridge Superstructures with Bidirectional-Ductile End Diaphragms. J. Bridge Eng. 2011, 16, 207–218. [Google Scholar] [CrossRef]
- Deng, K.; Pan, P.; Wang, C. Development of crawler steel damper for bridges. J. Constr. Steel Res. 2013, 85, 140–150. [Google Scholar] [CrossRef]
- Omori, T.; Ando, K.; Okano, M.; Xu, X.; Tanaka, Y.; Ohnuma, I.; Kainuma, R.; Ishida, K. Superelastic effect in polycrystalline ferrous alloys. Science 2011, 333, 68–71. [Google Scholar] [CrossRef] [PubMed]
- Shrestha, K.; Araki, Y.; Nagae, T.; Koetaka, Y.; Suzuki, Y.; Omori, T.; Sutou, Y.; Kainuma, R.; Ishida, K. Feasibility of Cu-Al-Mn superelastic alloy bars as reinforcement elements in concrete beams. Smart Mater. Struct. 2013, 22, 025025. [Google Scholar] [CrossRef]
- Billah, A.; Alam, M. Seismic fragility assessment of concrete bridge pier reinforced with superelastic shape memory alloy. Earthq. Spectra 2015, 31, 1515–1541. [Google Scholar] [CrossRef]
- Mazzoni, S.; McKenna, F.; Scott, M.; Fenves, G. Open System for Earthquake Engineering Simulation (OpenSees); Pacific Earthquake Engineering Research Center: Oakland, CA, USA, 2006. [Google Scholar]
- Jalayer, F.; Cornell, C. Alternative nonlinear demand estimation methods for probability-based seismic assessments. Earthq. Eng. Struct. Dyn. 2009, 38, 951–972. [Google Scholar] [CrossRef]
- American Society of Civil Engineers. ASCE STANDARD ASCE/SEI 7-16 Minimum Design Loads and Associated Criteria for Buildings and Other Structures; ASCE: Reston, VA, USA, 2016. [Google Scholar]
- Xiang, N.; Goto, Y.; Alam, M.S.; Li, J. Effect of bonding or unbonding on seismic behavior of bridge elastomeric bearings: Lessons learned from past earthquakes in China and Japan and inspirations for future design. Adv. Bridge Eng. 2021, 2, 14. [Google Scholar] [CrossRef]
- Kaviani, P.; Zareian, F.; Taciroglu, E. Seismic behavior of reinforced concrete bridges with skew-angled seat-type abutments. Eng. Struct. 2012, 45, 137–150. [Google Scholar] [CrossRef]
- Gazetas, G. Foundation Vibrations. In Foundation Engineering Handbook; Springer: Berlin/Heidelberg, Germany, 1991. [Google Scholar] [CrossRef]
- Muthukumar, S. A Contact Element Approach with Hysteresis Damping for the Analysis and Design of Pounding in Bridges. Ph.D. Dissertation, Georgia Institute of Technology, Atlanta, GA, USA, 2003. [Google Scholar]
- Guo, A.; Shen, Y.; Bai, J.; Li, H. Application of the endurance time method to the seismic analysis and evaluation of highway bridges considering pounding effects. Eng. Struct. 2017, 131, 220–230. [Google Scholar] [CrossRef]
- Rezaei, H.; Moayyedi, S.A.; Jankowski, R. Probabilistic seismic assessment of RC box-girder highway bridges with unequal-height piers subjected to earthquake-induced pounding. Bull. Earthq. Eng. 2020, 18, 1547–1578. [Google Scholar] [CrossRef]
- California Department of Transportation. Seismic Design Criteria, Version 2.0. CALTRANS 2019. Available online: https://dot.ca.gov/programs/engineering-services/manuals/seismic-design-criteria (accessed on 22 June 2020).
- Shamsabadi, A.; Rollins, K.; Kapuskar, M. Nonlinear soil–abutment–bridge structure interaction for seismic performance-based design. J. Geotech. Geoenviron. Eng. 2007, 133, 707–720. [Google Scholar] [CrossRef]
- Rubilar, F. Modelo No Lineal Para Predecir la Respuesta Sísmica de Pasos Superiores. Master’s Thesis, Pontificia Universidad Católica de Chile, Santiago, Chile, 2015. [Google Scholar]
- Megally, S.; Silva, P.; Seible, F. Seismic Response of Sacrificial Shear Keys in Bridge Abutments; UCSD/SSRP-2001/23; University of California: San Diego, CA, USA, 2002. [Google Scholar]
- Goel, R.; Chopra, A. Role of shear keys in seismic behavior of bridges crossing fault-rupture zones. J. Bridge Eng. 2008, 13, 398–408. [Google Scholar] [CrossRef]
- Martínez, A.; Hube, M.A.; Rollins, K.M. Analytical fragility curves for non-skewed highway bridges in Chile. Eng. Struct. 2017, 141, 530–542. [Google Scholar] [CrossRef]
- Steelman, J.; Fahnestock, L.; Filipov, E.; LaFave, J.; Hajjar, J.; Foutch, D. Shear and friction response of nonseismic laminated elastomeric bridge bearings subject to seismic demands. J. Bridge Eng. 2013, 18, 612–623. [Google Scholar] [CrossRef]
- Birrell, M.; Astroza, R.; Restrepo, J.I.; Loftizadeh, K.; Carreño, R.; Bazáez, R.; Hernández, F. Bayesian inference for calibration and validation of uniaxial reinforcing steel models. Eng. Struct. 2021, 243, 112386. [Google Scholar] [CrossRef]
- Pinto, F.J.; Toledo, J.; Birrell, M.; Bazáez, R.; Hernández, F.; Astroza, R. Uncertainty Quantification in Constitutive Models of Highway Bridge Components: Seismic Bars and Elastomeric Bearings. Materials 2023, 16, 1792. [Google Scholar] [CrossRef] [PubMed]
- Porter, K.A. An Overview of PEER’s Performance-Based Earthquake Engineering Methodology. In Proceedings of the 9th International Conference on Applications of Statistics and Probability in Civil Engineering, San Francisco, CA, USA, 6–9 July 2003; Volume 273. [Google Scholar]
- Deierlein, G.G.; Krawinkler, H.; Cornell, C.A. A framework for performance-based earthquake engineering. In Proceedings of the 7th Pacific Conference on Earthquake Engineering, Christchurch, New Zealand, 13–15 February 2003. [Google Scholar]
- The National Academies Press. Proposed AASHTO Guidelines for Performance-Based Seismic Bridge Design; National Academies of Sciences, Engineering, and Medicine: Washington, DC, USA, 2020. [Google Scholar] [CrossRef]
- CSA S6-19; Canadian Highway Bridge Design Code. Canadian Standards Association: Toronto, ON, Canada, 2019.
- Collins, K.R.; Stojadinovic, B. Limit States for Performance-Based Design. In Proceedings of the 12th World Conference on Earthquake Engineering, Auckland, New Zealand, 30 January–4 February 2000. [Google Scholar]
- Bertero, R.D.; Bertero, V.V. Performance-based seismic engineering: The need for a reliable conceptual comprehensive approach. Earthq. Eng. Struct. Dyn. 2002, 31, 627–652. [Google Scholar] [CrossRef]
- Pang, W.; Rosowsky, D.V.; Pei, S.; van de Lindt, J.W. Simplified Direct Displacement Design of Six-Story Woodframe Building and Pretest Seismic Performance Assessment. J. Struct. Eng. 2010, 136, 813–825. [Google Scholar] [CrossRef]
- Bolvardi, V.; Pei, S.; van de Lindt, J.W.; Dolan, J.D. Direct displacement design of tall cross laminated timber platform buildings with inter-story isolation. Eng. Struct. 2018, 167, 740–749. [Google Scholar] [CrossRef]
- Wu, S. Unseating mechanism of a skew bridge with seat-type abutments and a Simplified Method for estimating its support length requirement. Eng. Struct. 2019, 191, 194–205. [Google Scholar] [CrossRef]
- Yang, C.S.W.; Werner, S.D.; DesRoches, R. Seismic fragility analysis of skewed bridges in the central southeastern United States. Eng. Struct. 2015, 83, 116–128. [Google Scholar] [CrossRef]
- Esteva, L. Criterios para la construcción de espectros para diseño sísmico. In Proceedings of the 3er Simposio Panamericano de Estructuras, Caracas, Venezuela, 2–8 July 1967. [Google Scholar]
- Cornell, C. Engineering seismic risk analysis. Bull. Seismol. Soc. Am. 1968, 58, 1583–1606. [Google Scholar] [CrossRef]
- Hedayati Dezfuli, F.; Alam, M.S. Effect of different steel-reinforced elastomeric isolators on the seismic fragility of a highway bridge. Struct. Control Health Monit. 2017, 24, e1866. [Google Scholar] [CrossRef]
- Pagani, M.; Monelli, D.; Weatherill, G.; Danciu, L.; Crowley, H.; Silva, V.; Henshaw, P.; Butler, L.; Nastasi, M.; Panzeri, L.; et al. Openquake engine: An open hazard (and risk) software for the global earthquake model. Seismol. Res. Lett. 2014, 85, 692–702. [Google Scholar] [CrossRef]
- Hayes, G.; Moore, G.; Portner, D.; Hearne, M.; Flamme, H.; Furtney, M.; Smoczyk, G.M. Slab2, a comprehensive subduction zone geometry model. Science 2018, 362, 58–61. [Google Scholar] [CrossRef] [PubMed]
- Poulos, A.; Monsalve, M.; Zamora, N.; de la Llera, J. An updated recurrence model for chilean subduction seismicity and statistical validation of its poisson nature. Bull. Seismol. Soc. Am. 2019, 109, 66–74. [Google Scholar] [CrossRef]
- GEM Foundation. South American Risk Assessment. SARA Project. 2015. Available online: https://www.globalquakemodel.org/proj/sara (accessed on 22 October 2020).
- Idini, B.; Rojas, F.; Ruiz, S.; Pastén, C. Ground motion prediction equations for the Chilean subduction zone. Bull. Earthq. Eng. 2017, 15, 1853–1880. [Google Scholar] [CrossRef]
- Montalva, G.; Bastías, N.; Rodriguez-Marek, A. Ground-motion prediction equation for the Chilean subduction zone. Bull. Seismol. Soc. Am. 2017, 107, 901–911. [Google Scholar] [CrossRef]
- Bommer, J.; Scherbaum, F.; Bungum, H.; Cotton, F.; Sabetta, F.; Abrahamson, N. On the use of logic trees for ground-motion prediction equations in seismic-hazard analysis. Bull. Seismol. Soc. Am. 2005, 95, 377–389. [Google Scholar] [CrossRef]
- Akkar, S.; Sandıkkaya, M.; Bommer, J. Empirical ground-motion models for point- and extended-source crustal earthquake scenarios in Europe and the Middle East. Bull. Earthq. Eng. 2014, 12, 359–387. [Google Scholar] [CrossRef]
- Bindi, D.; Massa, M.; Luzi, L.; Ameri, G.; Pacor, F.; Puglia, R.; Augliera, P. Pan-European ground-motion prediction equations for the average horizontal component of PGA, PGV, and 5%-damped PSA at spectral periods up to 3.0 s using the RESORCE dataset. Bull. Earthq. Eng. 2014, 12, 391–430. [Google Scholar] [CrossRef]
- Boore, D.; Stewart, J.; Seyhan, E.; Atkinson, G. NGA-West2 equations for predicting PGA, PGV, nd 5% damped PGA for shallow crustal earthquakes. Earthq. Spectra 2014, 30, 1057–1085. [Google Scholar] [CrossRef]
- Bayat, M.; Daneshjoo, F.; Nisticò, N. The effect of different intensity measures and earthquake directions on the seismic assessment of skewed highway bridges. Earthq. Eng. Eng. Vib. 2017, 16, 165–179. [Google Scholar] [CrossRef]
- Lin, T.; Baker, J. Conditional Spectra. In Encyclopedia of Earthquake Engineering; Springer: Berlin/Heidelberg, Germany, 2015. [Google Scholar] [CrossRef]
- Baker, J.; Lee, C. An Improved Algorithm for Selecting Ground Motions to Match a Conditional Spectrum. J. Earthq. Eng. 2018, 22, 708–723. [Google Scholar] [CrossRef]
- CSN Red Nacional de Acelerógrafos; Centro Sismológico Nacional, Universidad de Chile: Santiago, Chile; Available online: http://www.csn.uchile.cl/red-sismologica-nacional/red-acelerografos/ (accessed on 22 October 2020).
- RENADIC Red de Cobertura Nacional de Acelerógrafos; Departamento de Ingeniería Civil, Facultad de Ciencias Físicas y Matemáticas, Universidad de Chile: Santiago, Chile; Available online: https://www.renadic.cl/ (accessed on 22 October 2020).
- Castro, S.; Benavente, R.; Crempien, J.G.F.; Candia, G.; de la Llera, J.C. A Consistently Processed Strong-Motion Database for Chilean Earthquakes. Seismol. Res. Lett. 2022, 93, 2700–2718. [Google Scholar] [CrossRef]
- Dávalos, H.; Miranda, E. Evaluation of the scaling factor bias influence on the probability of collapse using SA(T1) as the intensity measure. Earthq. Spectra 2019, 35, 679–702. [Google Scholar] [CrossRef]
- Dávalos, H.; Miranda, E. Evaluation of bias on the probability of collapse from amplitude scaling using spectral-shape-matched records. Earthq. Eng. Struct. Dyn. 2019, 48, 970–986. [Google Scholar] [CrossRef]
- Wang, Y.; Ibarra, L.; Pantelides, C. Effect of incidence angle on the seismic performance of skewed bridges retrofitted with buckling-restrained braces. Eng. Struct. 2020, 211, 110411. [Google Scholar] [CrossRef]
- Xiang, N.; Alam, M.; Li, J. Yielding steel dampers as restraining devices to control seismic sliding of laminated rubber bearings for highway bridges: Analytical and experimental study. J. Bridge Eng. 2019, 24, 04019103. [Google Scholar] [CrossRef]
- Camara, A.; Cristantielli, R.; Astiz, M.A.; Málaga-Chuquitaype, C. Design of hysteretic dampers with optimal ductility for the transverse seismic control of cable-stayed bridges. Earthq. Eng. Struct. Dyn. 2017, 46, 1811–1833. [Google Scholar] [CrossRef]
- Nielson, B. Analytical Fragility Curves for Highway Bridges in Moderate Seismic Zones; Georgia Institute of Technology: Atlanta, GA, USA, 2005. [Google Scholar]
- Zhou, L.; Shahria Alam, M.; Song, A.; Ye, A. Probability-based residual displacement estimation of unbonded laminated rubber bearing supported highway bridges retrofitted with Transverse Steel Damper. Eng. Struct. 2022, 272, 115053. [Google Scholar] [CrossRef]
- Huo, Y.; Zhang, J. Effects of Pounding and Skewness on Seismic Responses of Typical Multispan Highway Bridges Using the Fragility Function Method. J. Bridge Eng. 2013, 18, 499–515. [Google Scholar] [CrossRef]
- Luo, J.; Fahnestock, L.A.; LaFave, J.M. Seismic Performance Assessment of Quasi-Isolated Highway Bridges with Seat-Type Abutments. J. Earthq. Eng. 2019, 25, 2285–2324. [Google Scholar] [CrossRef]
- Noori, H.R.; Memarpour, M.M.; Yakhchalian, M.; Soltanieh, S. Effects of ground motion directionality on seismic behavior of skewed bridges considering SSI. Soil Dyn. Earthq. Eng. 2019, 127, 105820. [Google Scholar] [CrossRef]
- AASHTO. Guidelines for Performance-Based Seismic Design of Highway Bridges; AASHTO: Washington, DC, USA, 2023. [Google Scholar]



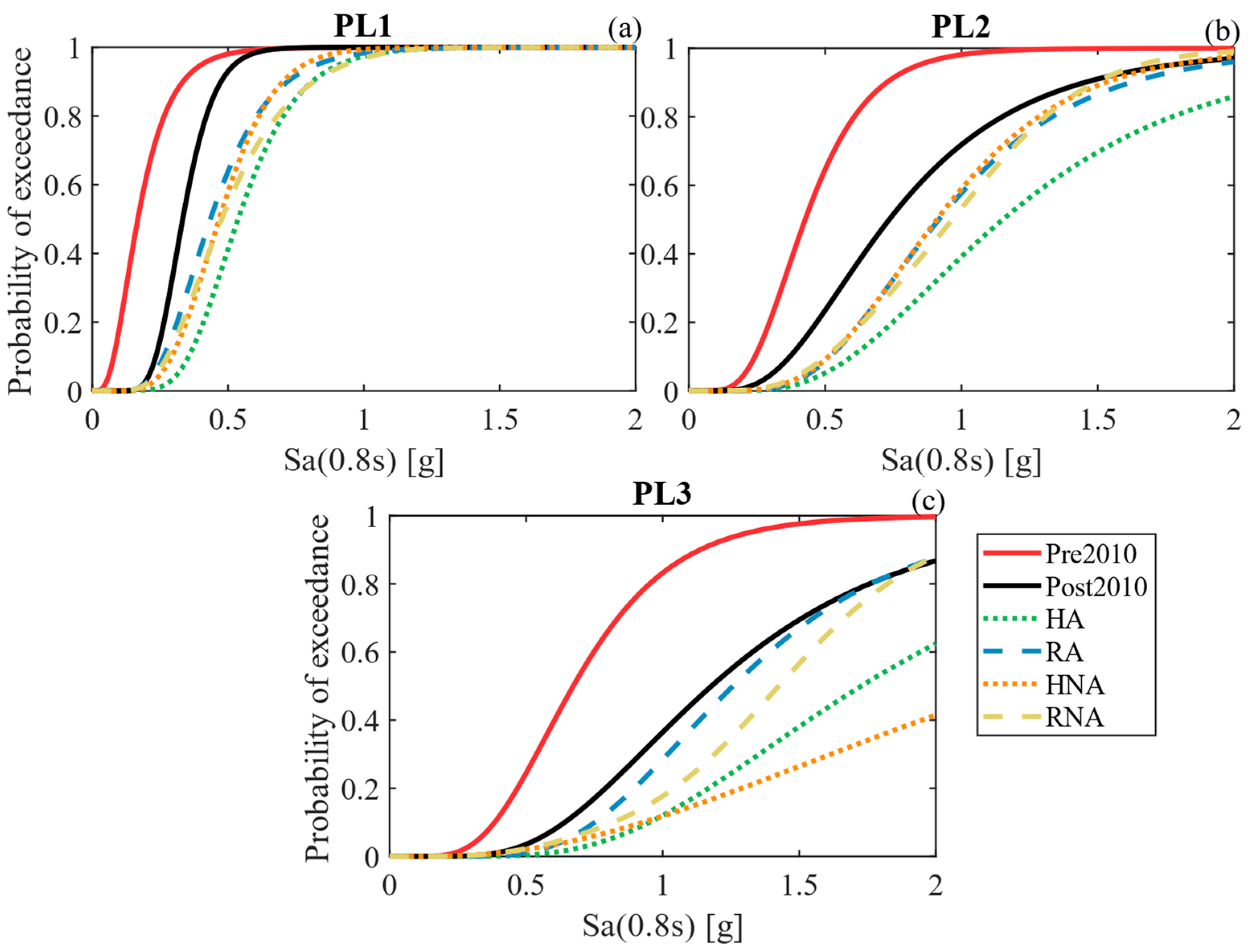

| Bridge Case | Hysteretic Dampers | Seismic Bars | Internal RC Shear Keys | Anchorage (Bonding) of Elastomeric Bearings |
|---|---|---|---|---|
| Pre-2010 | No | No | No | No |
| Post-2010 | Partially (seismic bars) | Yes | Yes | Yes |
| HA | Hysteretic damper without recentering capabilities | No | No | Yes |
| RA | Hysteretic damper with recentering capabilities | No | No | Yes |
| HNA | Hysteretic damper without recentering capabilities | No | No | No |
| RNA | Hysteretic damper with recentering capabilities | No | No | No |
| Hazard Level: Ground-Motion Return Period (Probability of Exceedance in 50 Years) | Performance Level (PL) | Maximum Probability of Exceeding the Respective PL (Allowable Probability of Damage) |
|---|---|---|
| 100 years (40%) | PL1: Fully operational | 30% |
| 975 years (5%) | PL2: Operational | 30% |
| 2475 years (2%) | PL3: Extensive damage | 20% |
| Performance Level (PL) | Engineering Demand Parameter (EDP) | EDP Threshold Value |
|---|---|---|
| PL1: Fully Operational | Elastomeric bearing shear strain | 100% [62] |
| PL2: Operational | Elastomeric bearing shear strain Superstructure-to-substructure residual displacement | 250% [62] The minimum value between the 50% of the seismic design displacement of the dampers [53] and 5 [cm] [46] |
| PL3: Extensive damage | Superstructure-to-substructure maximum displacement Superstructure-to-substructure residual displacement | The minimum value between the damper ultimate displacement [53] and the unseating displacement 15.2 [cm] [52] |
| Stripe (Return Period) | Soft Rock [g] | Stiff Soil [g] |
|---|---|---|
| Stripe 1 (100 years) | 0.24 | 0.34 |
| Stripe 2 (975 years) | 0.71 | 1.11 |
| Stripe 3 (2475 years) | 0.97 | 1.53 |
| Site Conditions | Soft Rock | Stiff Soil | ||
|---|---|---|---|---|
| Ground-motion return period [years] | 100 | 975 | 100 | 975 |
| [kN] | 532 | 457 | 2274 | 3301 |
| [kN/m] | 46,333 | 45,696 | 169,054 | 296,568 |
| EDP | Fully Operational (PL1) | Operational (PL2) | Extensive Damage (PL3) | |||
|---|---|---|---|---|---|---|
| Median | Dispersion | Median | Dispersion | Median | Dispersion | |
| Superstructure-to-substructure maximum displacement [cm] | - | - | - | - | 20 | 0.46 |
| Elastomeric bearing maximum shear strain [%] | 100 | 0.25 | 250 | 0.46 | - | - |
| Superstructure-to-substructure residual displacement [cm] | - | - | 5 | 0.46 | 15.24 | 0.46 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Aldea, S.; Bazáez, R.; Heresi, P.; Astroza, R. Effect of Bidirectional Hysteretic Dampers on the Seismic Performance of Skewed Multi-Span Highway Bridges. Buildings 2024, 14, 1778. https://doi.org/10.3390/buildings14061778
Aldea S, Bazáez R, Heresi P, Astroza R. Effect of Bidirectional Hysteretic Dampers on the Seismic Performance of Skewed Multi-Span Highway Bridges. Buildings. 2024; 14(6):1778. https://doi.org/10.3390/buildings14061778
Chicago/Turabian StyleAldea, Sofía, Ramiro Bazáez, Pablo Heresi, and Rodrigo Astroza. 2024. "Effect of Bidirectional Hysteretic Dampers on the Seismic Performance of Skewed Multi-Span Highway Bridges" Buildings 14, no. 6: 1778. https://doi.org/10.3390/buildings14061778
APA StyleAldea, S., Bazáez, R., Heresi, P., & Astroza, R. (2024). Effect of Bidirectional Hysteretic Dampers on the Seismic Performance of Skewed Multi-Span Highway Bridges. Buildings, 14(6), 1778. https://doi.org/10.3390/buildings14061778







