Theoretical Analysis of Energy Distribution Characteristics in Deeply Buried Circular Tunnels with a Revealed Cave
Abstract
1. Introduction
2. Materials and Methodology
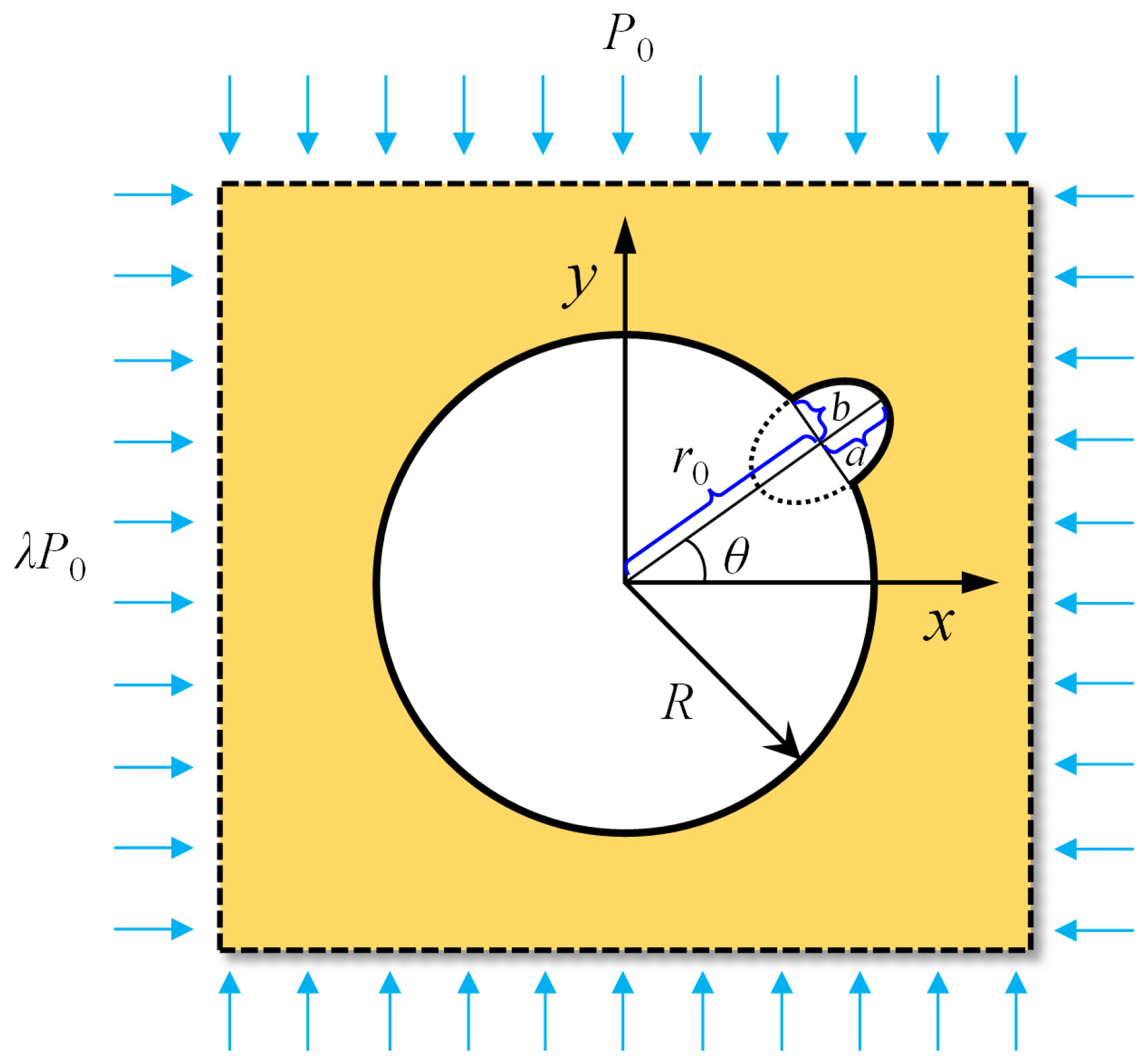
2.1. Statement of the Problem
- (1)
- The surrounding ground is assumed to be an elastic, homogeneous, and isotropic medium.
- (2)
- The excavation is assumed to be instantaneous, and the tunnel is not yet supported.
- (3)
- Compressive stress is considered positive.
2.2. Conformal Transformation
- (1)
- In z plane, m + 1 sample points zi (xi, yi) are picked with equal angles on the tunnel contour starting from the x-axis and following the counterclockwise direction. Similarly, m + 1 corresponding sample points ξi (1, θi) are extracted on the unit circle in ξ plane. The constant coefficients ak and bk in the first iteration are calculated aswith
- (2)
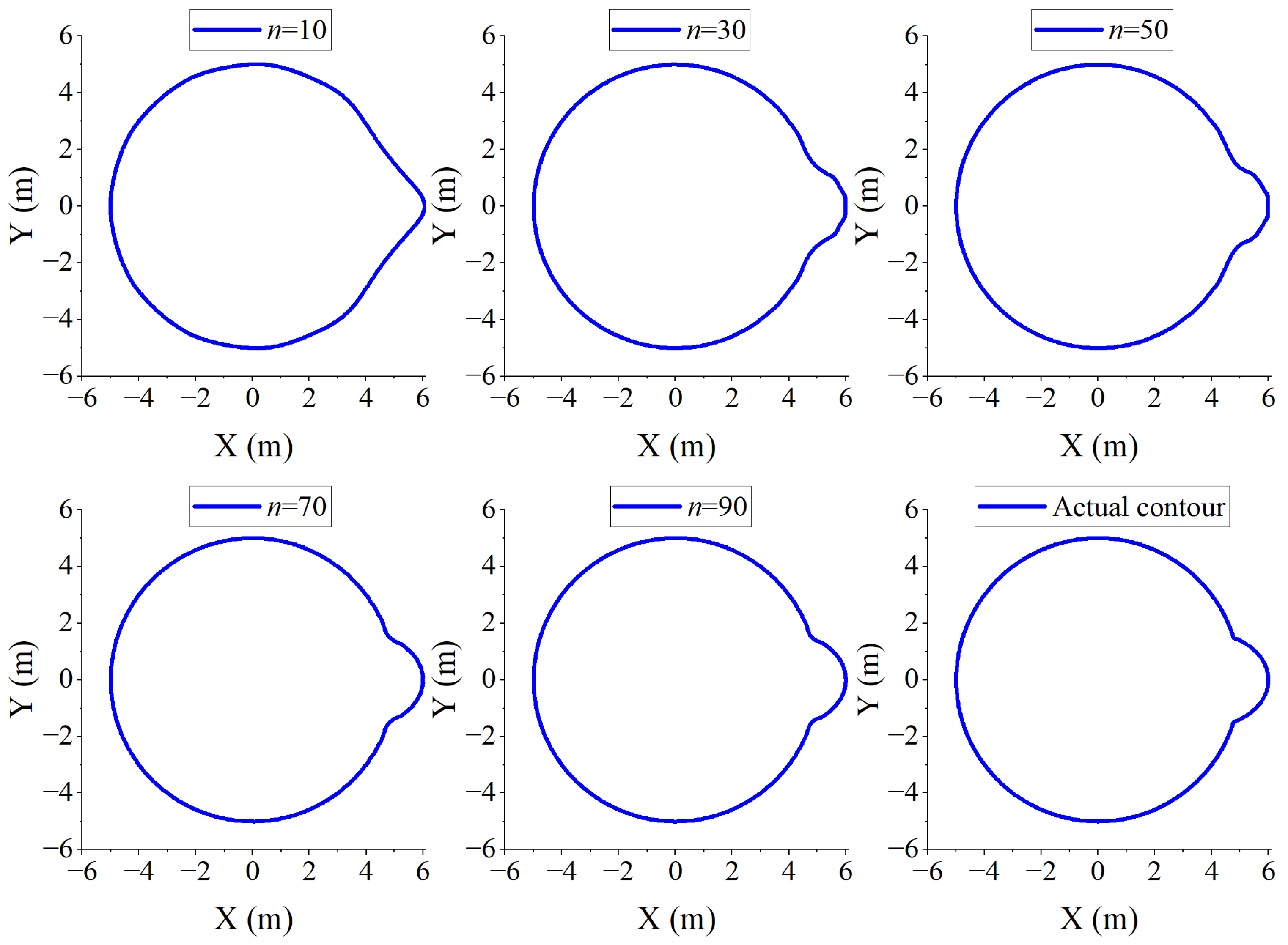
- A new set of points B1 = {(x0, y0), …, (xm, ym)} representing the mapping profile and the first mapping function is obtained after the first iteration. The accuracy of the mapping function is calculated by Equation (8). Keep performing Equation (4) until the mapping accuracy is achieved.
2.3. Complex-Variable Method
2.3.1. Analytical Expressions of Stress and Displacement Fields
2.3.2. Expressions of Complex Functions for Boundary Conditions
2.3.3. Cauchy Integral Formula
2.4. ElasticEnergy-Storage Theory
2.5. Analytical Solution for Deeply Buried Circular Tunnel with a Revealed Cave
2.5.1. Solution of the Conformal Transformation Function
2.5.2. Solution Procedure for Determining the Analytic Functions
3. Results and Discussion
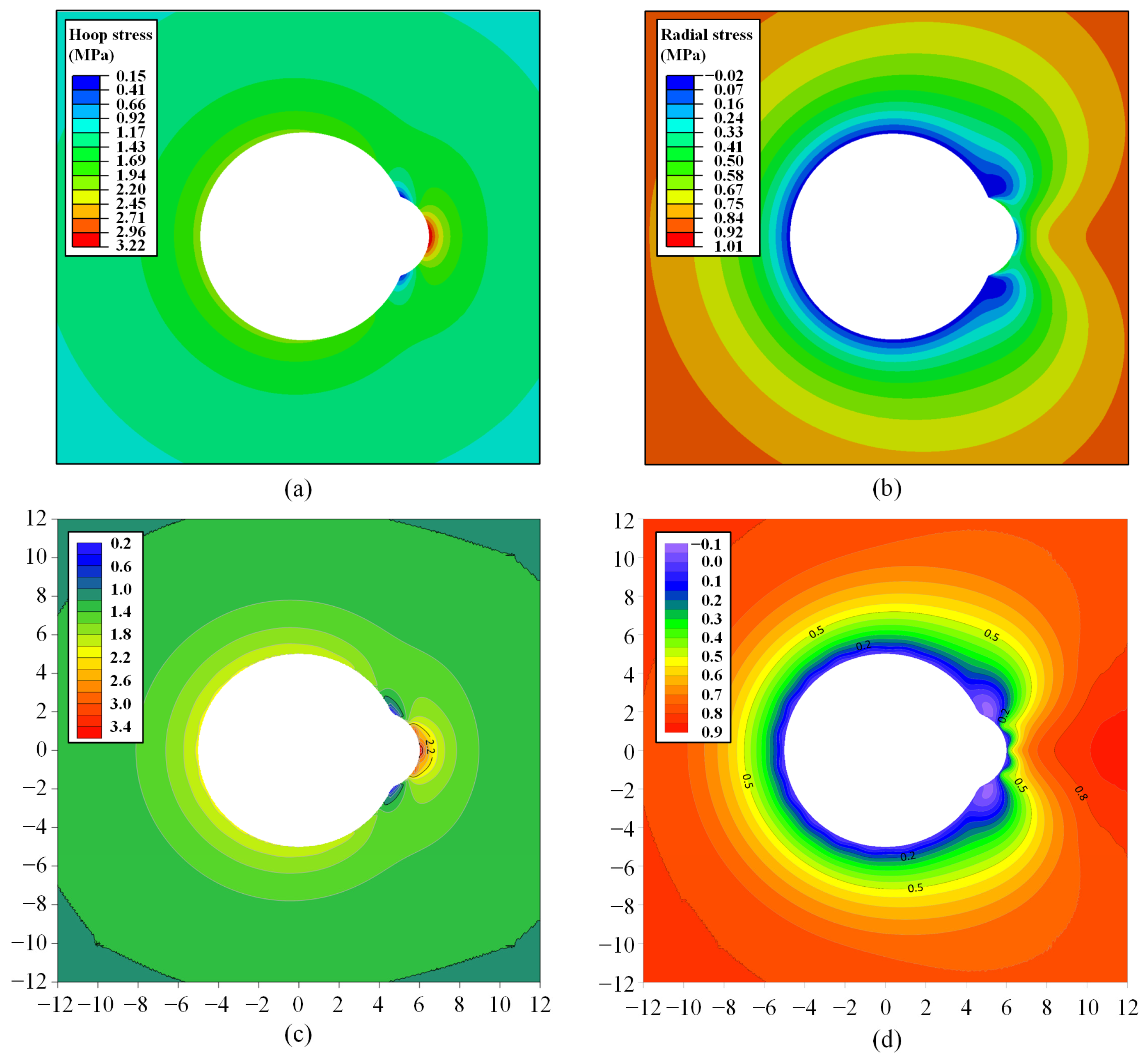
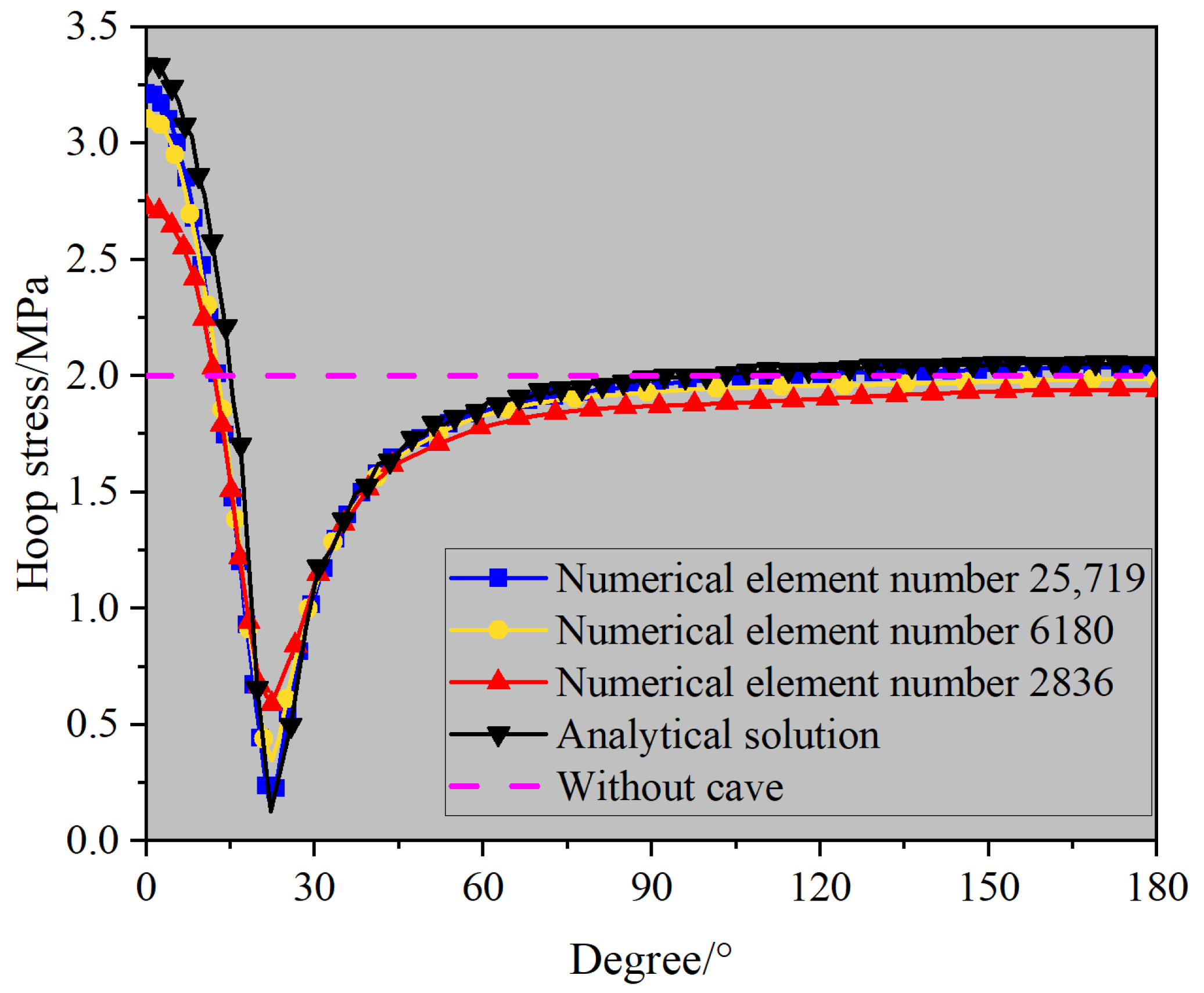
3.1. Numerical Validation and Stress Analysis
3.2. Parametric Analysis
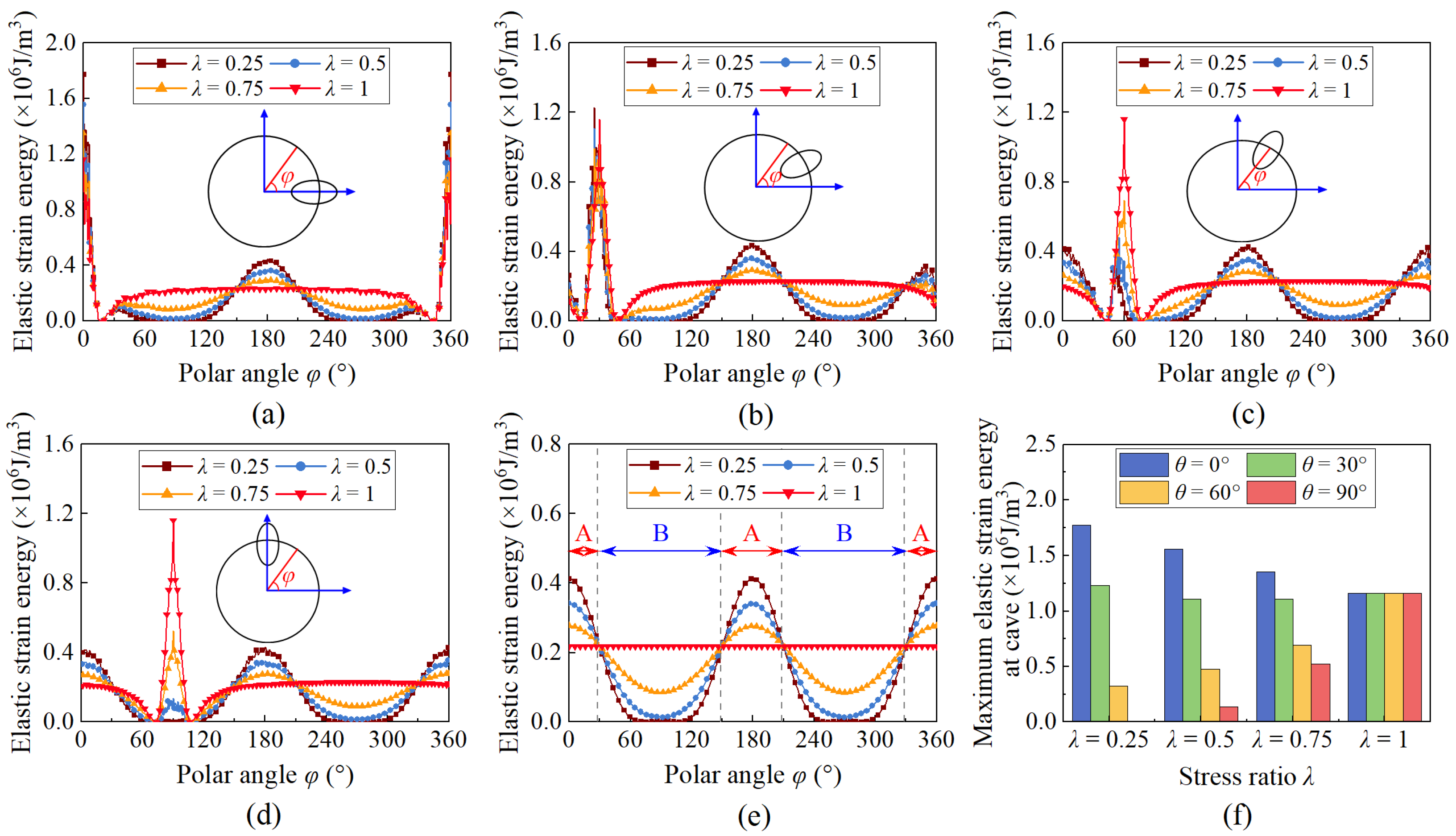
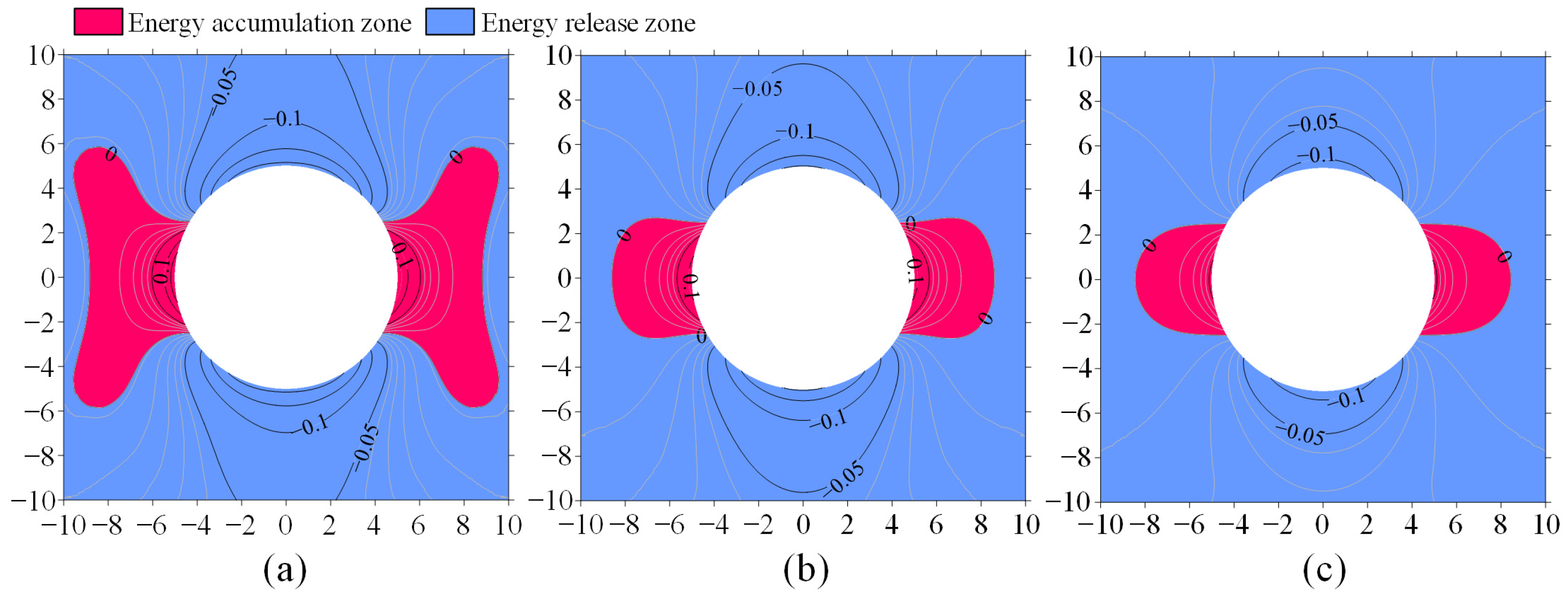
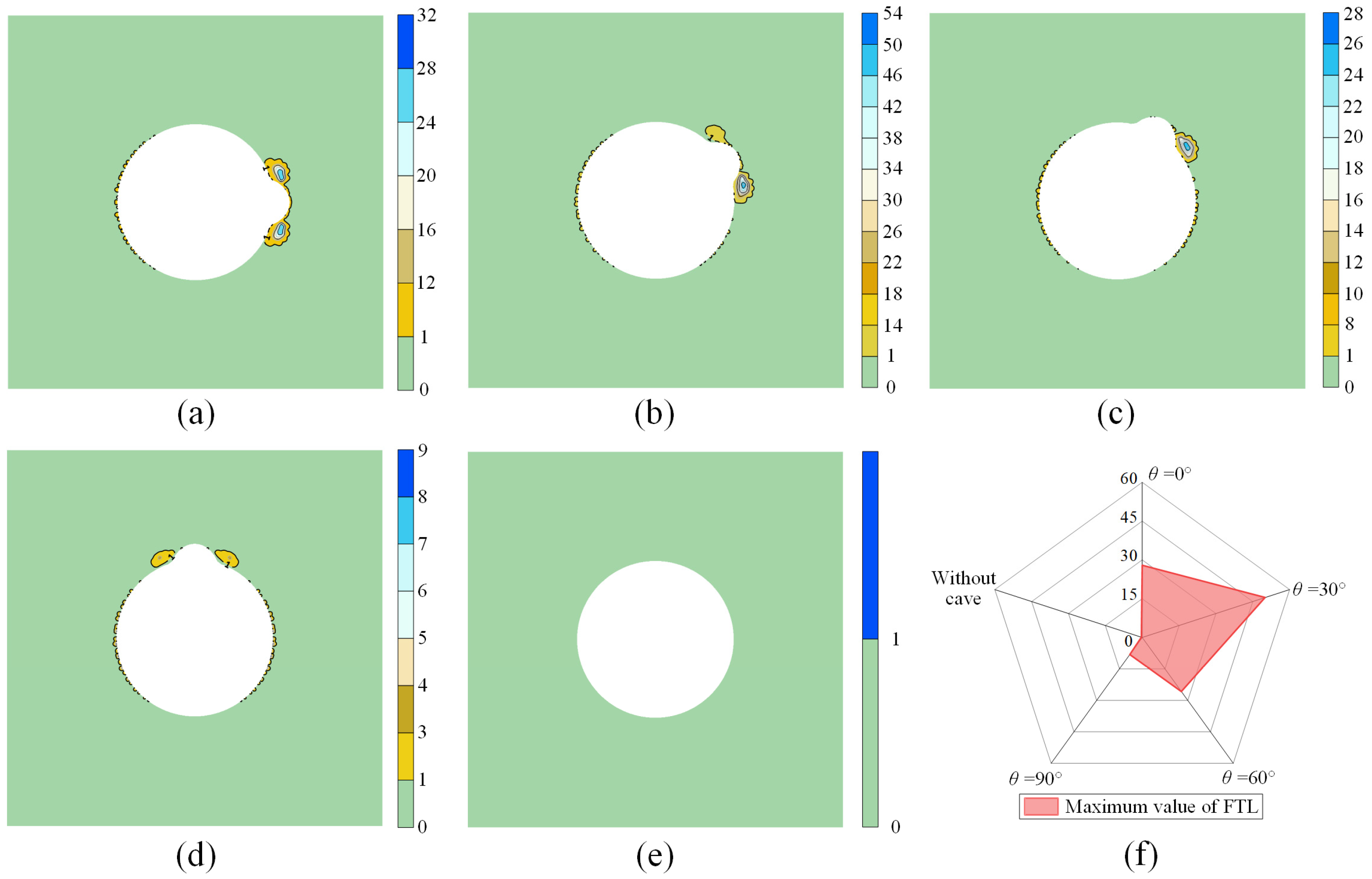
3.2.1. Effect of Cave Orientation on Energy Distribution Characteristics
3.2.2. Effect of Cave Shape b/a on Energy Distribution along the Hole
3.2.3. Effect of the Cave Protrusion on Strain Energy along the Hole
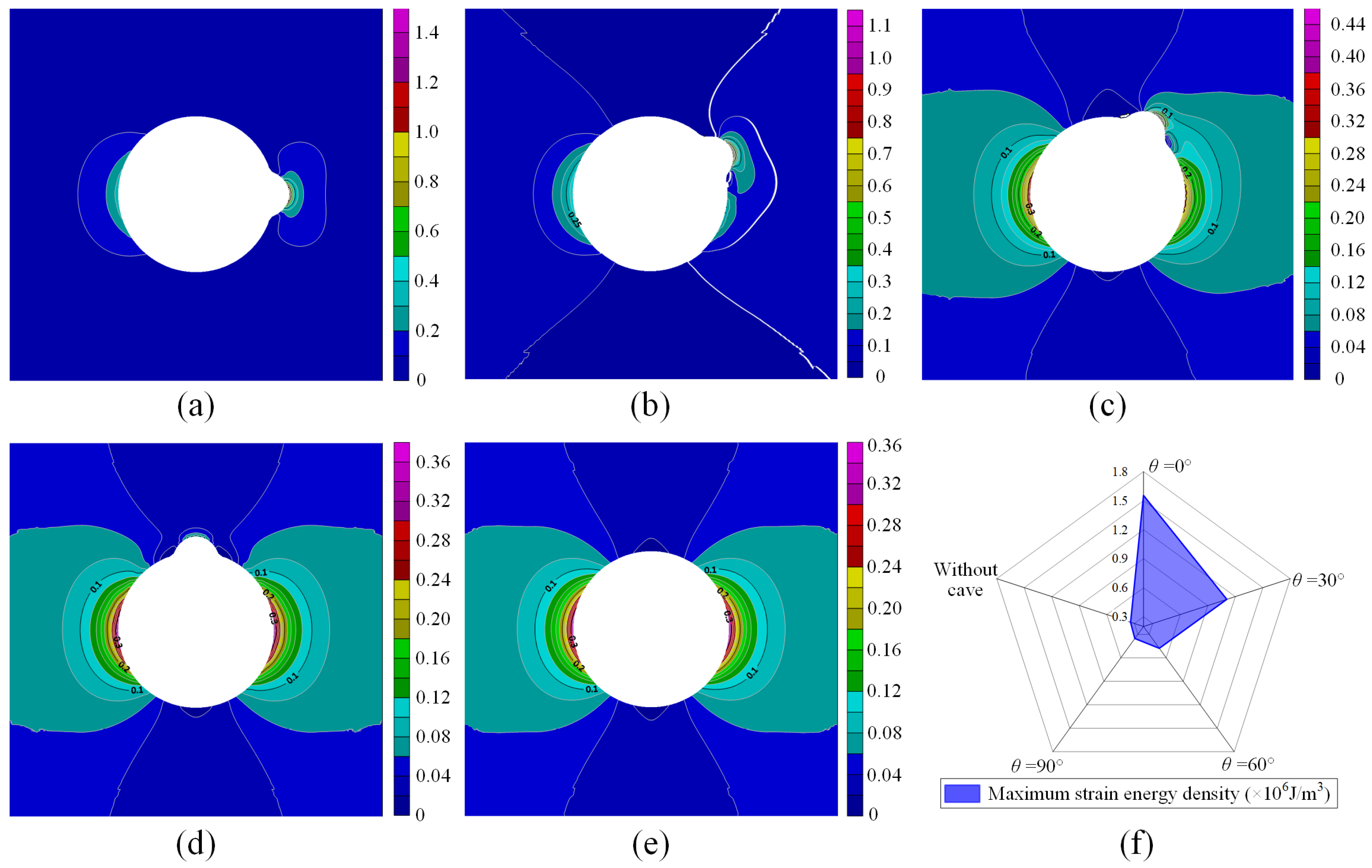
3.2.4. Effect of Cave on the Stability of the Surrounding Rock
3.3. Limitations and Future Prospects
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Li, C.C.; Zhao, T.; Zhang, Y.; Wan, W. A study on the energy sources and the role of the surrounding rock mass in strain burst. Int. J. Rock Mech. Min. Sci. 2022, 154, 105114. [Google Scholar] [CrossRef]
- Ma, C.S.; Chen, W.Z.; Tan, X.J.; Tian, H.M.; Yang, J.P.; Yu, J.X. Novel rockburst criterion based on the TBM tunnel construction of the Neelum–Jhelum (NJ) hydroelectric project in Pakistan. Tunn. Undergr. Space Technol. 2018, 81, 391–402. [Google Scholar] [CrossRef]
- Xue, T.; Zhang, Q.; Duan, K.; Wang, P.; Lin, H.; Fan, Q.; Liu, L. Geo-mechanical model test on the water inrush induced by zonal disintegration of deep tunnel under hydro-mechanical coupling. Int. J. Rock Mech. Min. Sci. 2022, 160, 105278. [Google Scholar] [CrossRef]
- Pei, Q.; Wang, X.; He, L.; Liu, L.; Tian, Y.; Wu, C. Estimation Method for an In Situ Stress Field along a Super-Long and Deep-Buried Tunnel and Its Application. Buildings 2023, 13, 1924. [Google Scholar] [CrossRef]
- Zhang, R.; Liu, Y.; Hou, S. Evaluation of rockburst risk in deep tunnels considering structural planes based on energy dissipation rate criterion and numerical simulation. Tunn. Undergr. Space Technol. 2023, 137, 105128. [Google Scholar] [CrossRef]
- Ortlepp, W.D. The behaviour of tunnels at great depth under large static and dynamic pressures. Tunn. Undergr. Space Technol. 2001, 16, 41–48. [Google Scholar] [CrossRef]
- Feng, X.-T.; Yang, C.-X.; Kong, R.; Zhao, J.; Zhou, Y.; Yao, Z.; Hu, L. Excavation-induced deep hard rock fracturing: Methodology and applications. J. Rock Mech. Geotech. Eng. 2022, 14, 1–34. [Google Scholar] [CrossRef]
- He, M.; Ding, M.; Yuan, Z.; Zhao, J.; Luo, B.; Ma, X. Numerical simulation of rock bursts triggered by blasting disturbance for deep-buried tunnels in jointed rock masses. Comput. Geotech. 2023, 161, 105609. [Google Scholar] [CrossRef]
- Kaiser, P.K.; Tang, C.A. Numerical Simulation of Damage Accumulation and Seismic Energy Release During Brittle Rock Failure—Part II: Rib Pillar Collapse. Int. J. Rock Mech. Min. Sci. 1998, 35, 123–134. [Google Scholar] [CrossRef]
- Lu, J.; Yin, G.; Gao, H.; Li, X.; Zhang, D.; Deng, B.; Wu, M.; Li, M. True Triaxial Experimental Study of Disturbed Compound Dynamic Disaster in Deep Underground Coal Mine. Rock Mech. Rock Eng. 2020, 53, 2347–2364. [Google Scholar] [CrossRef]
- Wu, B.; Sun, W.; Meng, G. A Calculation Method for Reliability Index of a Deep–Bedded Karst Tunnel Construction with Cavity Located Ahead of Tunnel Working Face. Buildings 2024, 14, 1349. [Google Scholar] [CrossRef]
- Fan, H.; Zhang, Y.; He, S.; Wang, K.; Wang, X.; Wang, H. Hazards and treatment of karst tunneling in Qinling-Daba mountainous area: Overview and lessons learnt from Yichang–Wanzhou railway system. Environ. Earth Sci. 2018, 77, 679. [Google Scholar] [CrossRef]
- Dong, X.; Karrech, A.; Basarir, H.; Elchalakani, M.; Qi, C. Analytical solution of energy redistribution in rectangular openings upon in-situ rock mass alteration. Int. J. Rock Mech. Min. Sci. 2018, 106, 74–83. [Google Scholar] [CrossRef]
- Zhao, Z.; Li, C.; Chen, S.; Zhang, M.; Ma, Q. Theoretical analysis of the surrounding rock stress field and energy field in shallow rectangular chambers during excavation. Comput. Geotech. 2024, 165, 105937. [Google Scholar] [CrossRef]
- Walsh, J.B. Energy changes due to mining. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1977, 14, 25–33. [Google Scholar] [CrossRef]
- Kramarenko, V.I.; Revuzhenko, A.F. Flow of energy in a deformed medium. Sov. Min. Sci. 1988, 24, 536–540. [Google Scholar] [CrossRef]
- Lindin, G.L.; Lobanova, T.V. Energy sources of rockbursts. J. Min. Sci. 2013, 49, 36–43. [Google Scholar] [CrossRef]
- Ge, D.; Jiang, F.; Wang, C.; Chen, Y.; Dong, C.; Zhu, S.; Wang, Z.; Han, F. Sliding Impact Mechanism of Square Roadway Based on Complex Function Theory. Shock Vib. 2021, 2021, 6655694. [Google Scholar] [CrossRef]
- Xin, X.-K.; Meng, Q.-B.; Pu, H.; Wu, J.-Y. Theoretical analysis and numerical simulation analysis of energy distribution characteristics of surrounding rocks of roadways. Tunn. Undergr. Space Technol. 2024, 147, 105747. [Google Scholar] [CrossRef]
- Zheng, K.; Shi, C.; Lou, Y.; Jia, C.; Lei, M.; Yang, Y. A computational method for tunnel energy evolution in strain-softening rock mass during excavation unloading based on triaxial stress paths. Comput. Geotech. 2024, 169, 106212. [Google Scholar] [CrossRef]
- Muskhelishvili, N.I. Some Basic Problems of the Mathematical Theory of Elasticity; Noordhoff: Groningen, The Netherlands, 1953; Volume 15. [Google Scholar]
- Fan, Y.; Lu, W.B.; Yan, P.; Chen, M.; Zhang, Y.Z. Transient characters of energy changes induced by blasting excavation of deep-buried tunnels. Tunn. Undergr. Space Technol. 2015, 49, 9–17. [Google Scholar] [CrossRef]
- Xie, H.; Li, L.; Peng, R.; Ju, Y. Energy analysis and criteria for structural failure of rocks. J. Rock Mech. Geotech. Eng. 2009, 1, 11–20. [Google Scholar] [CrossRef]
- An, X.; Hu, Z.; Su, Y.; Cao, S.; Tao, L.; Zhang, Y. Initial support distance of a non-circular tunnel based on convergence constraint method and integral failure criteria of rock. J. Cent. South Univ. 2022, 29, 3732–3744. [Google Scholar] [CrossRef]
- Liu, S.; Liu, S.; Lu, S.; Ma, F.; Pei, G. Seismic Behaviour of Shallow Tunnelling Method Tunnels Accounting for Primary Lining Effects. Buildings 2023, 13, 20. [Google Scholar] [CrossRef]
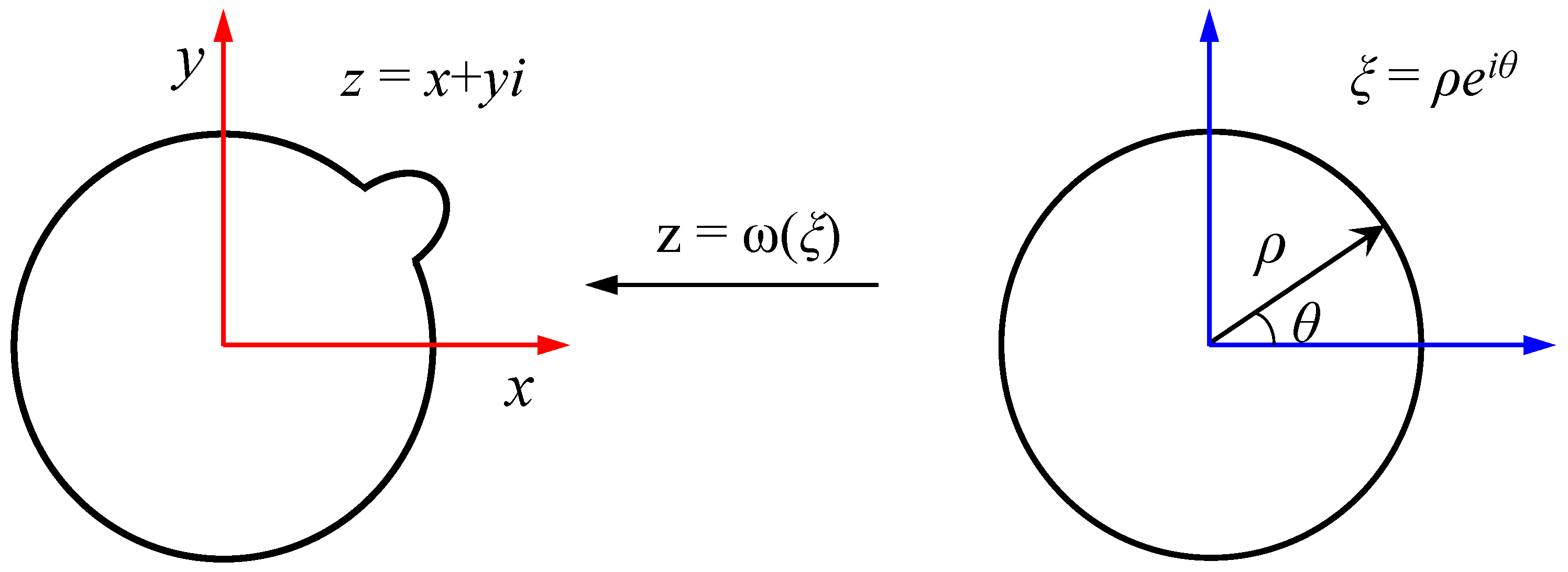





| Reference | Year | Method | Tunnel Shape |
|---|---|---|---|
| Walsh [15] | 1977 | Integral formulation | Arbitrary |
| Karamarenko and Revuzhenko [16] | 1988 | Energy flow | Circular |
| Lindin and Lobanova [17] | 2013 | Energy flow | Circular |
| Dong et al. [13] | 2018 | Complex-variable method | Rectangular |
| Ge et al. [18] | 2021 | Complex-variable method | Rectangular |
| Xin et al. [19] | 2024 | Elastic–plastic mechanics | Circular |
| Zhao et al. [14] | 2024 | Complex-variable method | Rectangular |
| Zheng et al. [20] | 2024 | Elastic–plastic mechanics | Circular |
| Surrounding Rock Parameters | Cave Parameters | Far-Field Stress Conditions | |||||
|---|---|---|---|---|---|---|---|
| Tunnel Radius (m) | Modulus of Elasticity (GPa) | Poisson’s Ratio | a (m) | b (m) | r0 (m) | Horizontal Stress (MPa) | Vertical Stress (MPa) |
| 5 | 13.3 | 0.3 | 2 | 1.6 | 4 | 20 | 40 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, D.; Wang, Y.; Huang, J. Theoretical Analysis of Energy Distribution Characteristics in Deeply Buried Circular Tunnels with a Revealed Cave. Buildings 2024, 14, 2343. https://doi.org/10.3390/buildings14082343
Xu D, Wang Y, Huang J. Theoretical Analysis of Energy Distribution Characteristics in Deeply Buried Circular Tunnels with a Revealed Cave. Buildings. 2024; 14(8):2343. https://doi.org/10.3390/buildings14082343
Chicago/Turabian StyleXu, Deming, Yuan Wang, and Jingqi Huang. 2024. "Theoretical Analysis of Energy Distribution Characteristics in Deeply Buried Circular Tunnels with a Revealed Cave" Buildings 14, no. 8: 2343. https://doi.org/10.3390/buildings14082343
APA StyleXu, D., Wang, Y., & Huang, J. (2024). Theoretical Analysis of Energy Distribution Characteristics in Deeply Buried Circular Tunnels with a Revealed Cave. Buildings, 14(8), 2343. https://doi.org/10.3390/buildings14082343







