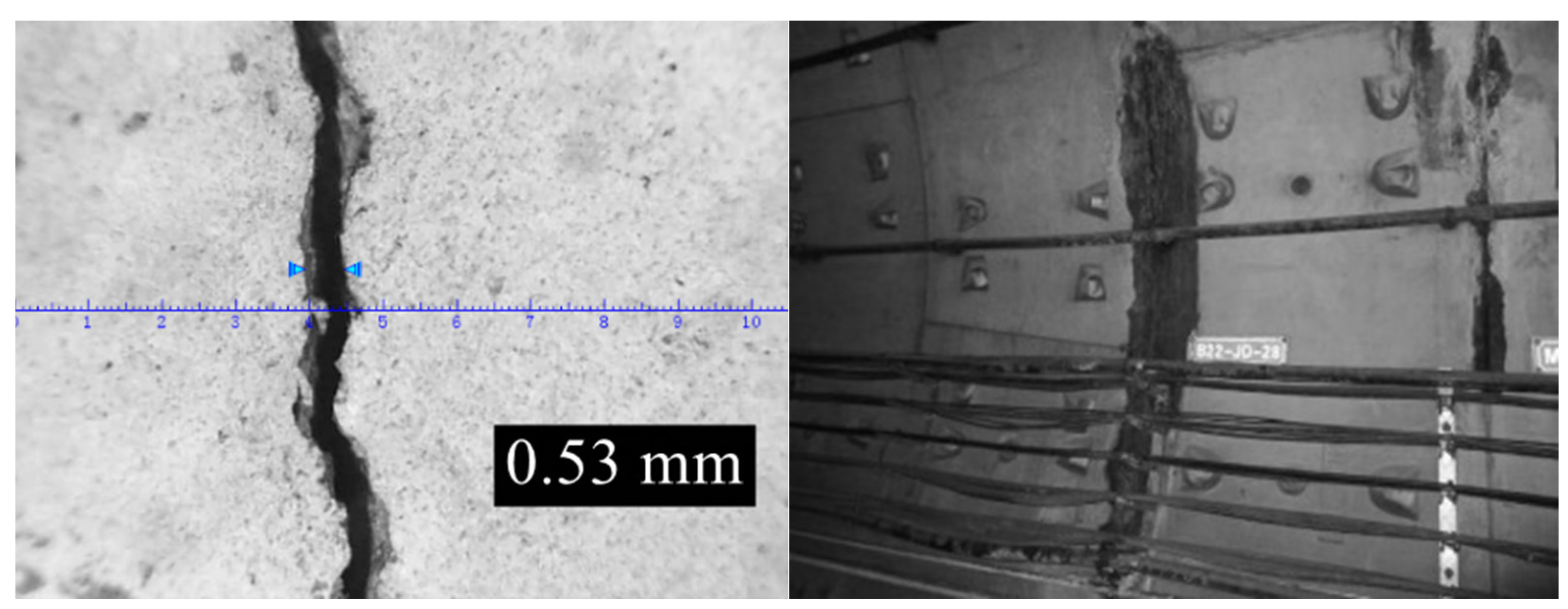
1. Introduction
In recent years, China’s rapid urbanization has led to a continuous expansion of operational urban rail transit networks. By December 2023, 55 cities across 31 provinces (including autonomous regions and municipalities directly under the central government) and the Xinjiang Production and Construction Corps had opened and operated 306 urban rail transit lines, spanning a total of 10,165.7 km. Concurrently, vibrations generated during train operations have attracted significant attention and become a focal point of research for scholars both domestically and internationally.
Scholars both domestically and internationally have conducted extensive research on vibrations generated during train operations using various methods. For instance, in numerical simulations, Lombaert et al. [
1] utilized the Monte Carlo method to analyze the impact of random irregularities on the prediction of vibration responses. Kouroussis et al. [
2] examined the effects of track irregularities, local track damage, and uneven wheel wear on environmental vibrations. Yang et al. [
3] employed centrifuge testing and numerical simulations to study the dynamic response patterns of tunnel structures, discussing the influences of boundary conditions and soil parameters in numerical simulations. Yaseri et al. [
4] analyzed the dynamic responses of tunnel structures and subway surfaces by establishing a three-dimensional proportional boundary coupling model. Real et al. [
5] developed both two-dimensional and three-dimensional finite element models to predict the dynamic responses of tunnel structures under train vibration loads, demonstrating the reliability of the three-dimensional model through the comparison and analysis of field measurement data. Di et al. [
6] investigated the dynamic response of elastic semi-space in porous double-track subway tunnels. Lai et al. [
7] studied the characteristics and propagation laws of tunnel vibration responses under vehicle and subway train loading. Clot et al. [
8] researched the differences in dynamic responses between double-deck circular tunnels and simple tunnels, establishing a 2.5D semi-analytical model to predict ground vibrations. Song et al. [
9] proposed a theoretical analysis model for deeply buried tunnels in unsaturated soil, considering the coupling of soil, groundwater, and air, and analyzed the dynamic responses of tunnel vaults under vertical and harmonic loads. Huang et al. [
10] utilized a two-dimensional soil–water coupled dynamic finite element analysis method to study the long-term settlement of subway tunnels in saturated clay induced by train vibrations. Yuan et al. [
11] derived a semi-analytical solution to calculate ground vibrations induced by subway train vibrations and used it to investigate the effects of hydraulic boundary conditions and groundwater level fluctuations on ground vibrations. Pan et al. [
12] established a finite element model of a train tunnel influenced by Nanjing Metro Line 10, analyzing the dynamic responses of the tunnel surroundings under vibration loads for single-hole and double-track subway tunnels. Faizan et al. [
13] developed a two-dimensional finite element model of railway–soil coupling to perform computational analyses on four different soil types and compared them with field measurements from the Istanbul–Ankara high-speed railway section. Further, Kirtel [
14] established a nonlinear two-dimensional finite element model, fully considering the effects of local plastic deformation of soil-on-soil vibration-coupled dynamic responses, and predicted the mitigation effects of thin-wall isolation measures on structural vibrations.
In addition to traditional theoretical analyses and analytical–numerical models, field experiments are also a direct and reliable method for studying train vibration responses. Zheng and Yan [
15] measured vibrations caused by high-speed trains on dock roofs, flat ground, and slopes to study the influence of train speed and gradients on vibrations. Nelson et al. [
16] conducted force density level tests in a tunnel similar to the profile of the Lighthouse Hill Tunnel to investigate the impact of subway operations on the University of Washington. Verbraken [
17] provided extensive field test data within a section, analyzing in detail the effects of different hammer strike positions, equivalent line source lengths, and excitation intervals on force density levels and the line transmission rate. Zhai et al. [
18] conducted field tests on ground vibrations of the Beijing–Shanghai railway, analyzing the temporal and frequency-domain characteristics of ground vibration accelerations. Li and Yuan [
19] studied the ground displacement response patterns of double-track closed tunnels through field monitoring. Ma et al. [
20] conducted field monitoring on Xi’an Metro Lines 6 and 2, studying the effects of train-induced vibrations on ancient buildings above double-track subway tunnels. Zhang et al. [
21] recorded acceleration results for six different train speeds on traditional railway tracks, showing that vibration propagation along the longitudinal direction resembles a sinusoidal waveform and decays exponentially with propagation distance. Qu et al. [
22] conducted field measurements on the Shenzhen double-track subway, showing that ground vibrations in the 50–63 Hz frequency band are enhanced when a nearby train is about to pass the test section. When a distant train passes the test section and a nearby train has just left, acceleration decreases by 12 dB in the 10–16 Hz frequency range. He et al. [
23] measured the operation of bullet trains and analyzed them from the perspective of bridge–train interactions and ground–train interactions. Sanayei et al. [
24] conducted in situ tests on full-size buildings and compared the predicted results of impedance models with actual response results, showing good agreement between predicted and actual responses. Yang et al. [
25] reported on-site vibration measurements at the Tianjin West Elevated Bridge Railway Station, where data were primarily collected from trains passing through the station. Vibration measurements were recorded in different directions in the waiting hall to obtain an accelerated time response. Ma et al. [
26] conducted real-time monitoring tests on the subway tunnels over one year. Each test lasted 24 h, recording all vibration responses of passing trains. Cao et al. [
27] conducted vibration tests on a seven-story residential building built on an elevated subway station section, measuring acceleration time processes at different locations and comparing corresponding spectra. The study investigated the impact of track position on vibration and assessed vibration maintainability through two different indicators. Cao et al. [
28] studied vibrations in a three-story subway station section where trains pass through elevated bridges, measuring acceleration times on three levels of tracks and floors. Amplitudes and the frequency content of vibrations at different locations were compared using respective spectra and root mean square (RMS) spectra of one-third octave frequency equations. The study examined the impact of track position on floor vibrations and assessed building vibration levels using two indicators.
Furthermore, many scholars abroad have conducted model testing and research in the field of tunnel train dynamic response. Jiang et al. [
29] employed actual engineering design methods to construct a model measuring 5 m in length, 15 m in width, and 6 m in height. Using a sequential loading system consisting of eight high-performance hydraulic actuators, they simulated the load of a running train with a maximum speed of 360 km/h. Al Shaer et al. [
30] proposed a simplified scaled experiment based on three sleepers to study the dynamic behavior and settlement of ballast tracks. Yang. et al. [
31] investigated the influence of different tunnel cross-sectional shapes on train dynamic response through model experiments, highlighting the significant impact of rectangular cross-sections on dynamic response, with the cross-sectional shape mainly affecting areas near the tunnel. Yang. et al. [
32] conducted physical model tests on the dynamic characteristics of shield tunnels and surrounding soil, revealing a significant amplification of tunnel and soil responses with increasing train speed. Mousavi-Rahimi et al. [
33] developed a 1 g scale physical model to study the effects of floor frequencies related to local bending modes, primary soil frequencies in vertical translation and foundation geometry on building vibration levels. The experimental results under impulse pulse loads in the frequency range of 0–156 Hz showed minimal vibration levels transmitted to the floor in the lining structure. Yang et al. [
34] tested three different tunnel lining models and explored the dynamic characteristics of tunnel linings through a series of physical model experiments. Yi et al. [
35] established a 1:10 scale model based on Guangzhou Metro Line 9 to study the cumulative deformation of tunnel linings under long-term dynamic loads from trains, revealing the impact of long-term dynamic loads on tunnel cumulative deformation. Bao et al. [
36] proposed a multi-scale modeling and simulation method for shield tunnel tests and validated it through vibration platform tests, demonstrating consistency in macroscopic responses between the two methods. Huang et al. [
37] primarily analyzed the acceleration, dynamic coefficient, and dynamic stress of foundation soil and tunnels in dynamic model tests with different train speeds at a geometric ratio of 1:4. They discussed the relationship with train speed, indicating that as train speed increases, the response of the tunnel bottom and foundation soil to vibration slightly increases, while the pressure of the foundation soil decreases exponentially with distance.
Real-time monitoring systems play a critical role in assessing structural health and ensuring the safety of tunnel infrastructures. Previous studies have implemented various monitoring technologies to capture real-time data on structural responses. Strauss et al. [
38] provided a comprehensive review of testing methods and monitoring strategies for tunnel assessment, categorizing various technologies and techniques. They concluded that a systematic approach to monitoring, including both non-destructive and semi-destructive techniques, is essential for evaluating structural performance and ensuring tunnel safety. Jiang et al. [
39] employed various non-destructive testing methods to detect defects in tunnel linings and concluded that early detection and retrofitting are crucial for maintaining tunnel integrity and extending service life. Despite these advancements, there is a notable gap in the literature regarding the specific impact of cracks on the dynamic response of tunnel structures. Most existing studies focus on general vibration analyses without addressing the effects of structural defects.
This study uses a segment of a metro tunnel as an example. It measures the internal dynamic response of cracked and intact tunnel sections, capturing the average peak acceleration and vibration levels. Additionally, a Fourier transform and one-third octave band analysis are applied to time-domain data to extract the full-frequency vibration characteristics of the tunnel, both with and without cracks. This approach provides essential field measurement data to support theoretical calculations and numerical simulations.
4. Analysis of Test Results
The train operating in the test section is a type B train, with each car measuring 22 m in length. The operational speed of the subway train ranges from 50 to 60 km/h, resulting in an effective vibration duration of 8 to 10 s. The UIC60 type rail is used, with a cast-in-place concrete track bed. By statistically analyzing the peak acceleration and vibration levels at each measurement point, a more reliable average peak value and vibration level can be obtained. Additionally, Fourier transformation and one-third octave band analysis are employed to investigate the influence of tunnel cracks on train vibrations in the frequency domain.
4.1. Time-Domain Analysis of Train Vibration
The average peak acceleration and vibration level for the left and right rails, track bed, and tunnel wall are shown in
Table 2. The vibrations induced by the rails, track bed, and tunnel wall are considered as underground vibrations, classified as engineering-grade vibrations, which do not require the consideration of human sensitivity to vibration levels. Therefore, the vibration level calculation weighting for these components is ignored.
From the acceleration time–history curve, it can be observed that the vibrations generated by the train at a distance trigger the sensor data collection. When the train passes through the measurement point, the acceleration curve experiences significant fluctuations, lasting for 8–10 s. The measurement section is a straight track segment of the subway train, and the tunnel structure is symmetrical, resulting in almost identical left and right acceleration responses. This symmetry also leads to the Z-axis acceleration of the rails being over three times higher than the Y-axis acceleration. Regarding vibration propagation, the Z-axis average acceleration response of the rails is the highest, reaching up to 29.39 m/s2. However, as the vibration propagates to the track bed, the Z-axis acceleration significantly decreases to 0.76 m/s2, representing a difference of approximately 40 s between the two. The connection between the track bed and the tunnel wall is more integral, and the materials are similar, resulting in less noticeable vibration attenuation compared to the rails. The Z-axis acceleration decreases from 0.76 m/s2 to 0.11 m/s2, representing a difference of about 7 s.
Similar to the transmission pattern of acceleration, the vibration levels of the
Z-axis and
Y-axis decrease by approximately 10%. However, the vibration level decreases significantly as the acceleration is transmitted from the rails to the bed, dropping from 143.69 dB to 73.59 dB, a decrease of up to 20%. Due to the integral nature of the tunnel structure, the vibration level decreases by only approximately 7% as the acceleration is transmitted from the track bed to the tunnel wall. Representative acceleration time–history curves for the
Z-axis and
Y-axis of the rails, track bed, and tunnel wall are shown in
Figure 9 and
Figure 10.
Table 3 shows the average acceleration and vibration levels for the rails, track bed, and tunnel wall at the cracked section. Regarding attenuation patterns, the acceleration and vibration levels at the cracked section are consistent with those at the intact section, with the hierarchy being rail > track bed > tunnel wall and the magnitudes at each part remaining unchanged. In terms of response increments at each part, different degrees of increase were observed: vertical acceleration of the rails increased by approximately 7%, horizontal acceleration of the rails increased by about 10%, acceleration of the track bed increased by around 14%, and acceleration of the tunnel wall increased by about 70%. Similarly, the vertical and horizontal vibration levels of the rails increased by 14%, the vibration level of the track bed increased by 20%, and the vibration level of the tunnel wall increased by 35%. As the measurement points approach the crack, the average acceleration and vibration levels progressively increase, indicating that the presence of the crack intensifies the dynamic response of various parts of the tunnel. This was reflected in the time–history curve as an increase in peak acceleration and effective vibration values.
Figure 11 shows representative measured acceleration data for the rails, track bed, and tunnel wall, along with their corresponding time–history curves.
4.2. Full-Frequency Analysis of Train Vibration
Applying the Fourier transform to the acceleration time–history curves for each measurement point provides the spectral data of train-induced vibrations. This reveals the frequency characteristics of vibrations in various tunnel structures during subway train operations. It can be observed that as the train-induced vibration propagates from the rails to the track bed and then to the tunnel wall, the high-frequency components continuously attenuate.
The main frequency range for the vertical vibration of the rails is between 200 (Hz) and 1400 (Hz), with a peak at 570 (Hz). The spectral distribution of the lateral vibration of the rails is similar to that of the vertical vibration, but the overall peak is about 40% lower. Both vertical and lateral vibrations are mainly characterized by medium-frequency to high-frequency components.
The spectral plot for the track bed shows a distribution between 200 Hz and 1400 Hz, with minimal peaks between 600 Hz and 1400 Hz and between 600 Hz and 1400 Hz. The main frequency range is concentrated within 400 Hz, with a peak at 70 Hz, indicating that low-frequency to medium-frequency vibrations are predominant.
For the tunnel wall, the main frequency range is between 200 Hz and 400 Hz, with a peak at 300 Hz. Due to the integral nature of the tunnel structure, its frequency distribution is similar to the track bed, with predominantly low-frequency to medium-frequency vibrations. The peak values are consistent with those of the track bed. Representative spectral data for the acceleration of the rails, track bed, and tunnel wall are shown in
Figure 12.
4.3. Frequency-Domain Analysis of Crack Cross-Section
The spectral analysis of the measurement point data at the cracked section revealed that the presence of cracks has minimal impact on the overall spectral distribution of vibrations, with the data trends remaining consistent, as shown in
Figure 13. For the rails, the presence of cracks significantly enhances the mid-frequency range (400 Hz–800 Hz) in both the Z- and Y-directions, with an amplitude increase of approximately 70%, while the low- and high-frequency ranges remain largely unchanged. For the track bed, the spectral curve at the cracked measurement points shows a significant increase in the low-frequency to mid-frequency range (200 Hz–600 Hz), with the peak value increasing by about 100%, while other frequency ranges remain unchanged. For the tunnel wall, the presence of cracks causes a substantial increase in the low-frequency range (200 Hz–400 Hz), with an increase of about 300%.
Considering the spectral changes corresponding to the cracked measurement points, it can be observed that the presence of cracks causes varying degrees of increase at different frequencies for the tunnel structures. The tunnel wall, being directly affected by the cracks, shows the most significant increase. However, due to the initially low vibration response of the tunnel wall, the degradation does not result in a magnitude change. The degree of increase gradually decreases with the increasing distance and connectivity between the measurement point and the crack. The sensitivity to cracks varies among the tunnel structures: the rails are mainly affected in the mid-frequency range, while the track bed and tunnel wall are mainly affected in the low-frequency range. Since the cracks alter the tunnel structure and strength without changing the excitation conditions of the train vibration source, the spectral trend remains consistent. The changes in internal structure and strength lead to a noticeable enhancement of the resonance effect, with the peak of the response spectrum indicating the resonance frequency of the structure. This explains why the enhanced segments of the spectra are primarily in the main frequency range.
Resonance occurs when the frequency of external vibrations matches the natural frequency of the tunnel structure, leading to amplified vibrations. This amplification can result in higher stress and strain on the tunnel components, potentially accelerating wear and tear and leading to structural damage over time. Additionally, resonance can compromise the structural integrity of the tunnel by causing excessive vibrations that exceed the design limits. This can lead to cracks, joint failures, and other forms of structural degradation.
4.4. One-Third Octave Analysis
The time-domain data were analyzed using the one-third octave band spectrum analysis, displaying the cracked and non-cracked sections overlapping. The range of 0–1000 Hz was selected and displayed on a logarithmic scale. As shown in
Figure 14, the spectral trends of the cracked sections generally match those of the non-cracked sections, both showing a slow increase in the low-frequency range and a rapid increase in the high-frequency range. The presence of cracks effectively increases the vibration levels within the main frequency range of each component. For the vertical response of the rails at a center frequency of 500 Hz, the vibration level increases by 2.83 dB, with an amplitude increase of 2.2%. For the lateral response of the rails at a center frequency of 400 Hz, the vibration level increases by 0.6 dB. For the track bed response at a center frequency of 100 Hz, the vibration level increases by 7.87 dB, with an amplitude increase of 8.8%. For the tunnel wall response at a center frequency of 315 Hz, the vibration level increases by 10.74 dB, with an amplitude increase of 12.4%.
From the above analysis, it is evident that the center frequencies of each component closely match their main vibration frequencies. Compared to the normal sections, the vibration levels at the center frequencies of the cracked sections are slightly increased. The sensitivity of the responses to structural degradation follows the order tunnel wall > rails > track bed. Additionally, the peak frequencies of the vibration levels in each component are consistent with those obtained from the Fourier spectrum analysis.
The spectral curves of different positions within the same section are overlapped, as shown in
Figure 15, for both the cracked and non-cracked sections. The trends in vibration level development for cracked and non-cracked sections are generally consistent. Regarding the magnitude of vibration levels, the order is vertical rail > lateral rail > track bed > tunnel wall. This aligns with the results obtained from the time-domain analysis of acceleration for each component. For specific vibration level magnitudes at each location, refer to
Table 4.
According to DB11/T838-2019 “Subway Noise and Vibration Control Specification” [
42], for a standard B-type train operating at a speed of 20 m/s, the maximum vertical vibration level for the tunnel wall is 84 (dB). In this measurement, the vibration level in the intact section was −1.3 dB below the standard, while the cracked section exceeded the standard by 13.5 (dB).
Table 5 provides appropriate vibration reduction measures. The table shows that, in the absence of cracks, the existing low-level to medium-level vibration reduction measures inside the tunnel are sufficient to meet the required standards. However, in the presence of cracks, excessive vibration indicates that these cracks amplify the tunnel’s dynamic response under the train’s vibration load, which severely impacts the train’s normal operation. Therefore, it is essential to promptly repair the cracks and implement specialized vibration reduction measures in future operations.
4.5. Track Displacement
When the train passes the measurement point, both vertical and lateral displacements of the rail are observed. The preloaded displacement sensor used in this measurement indicates that as the train approaches the measurement point, the displacement amplitude gradually increases, and as the train moves away, the displacement gradually returns to zero. The maximum vertical displacement of the rail can reach 1.1 (mm), and the maximum lateral displacement can reach 0.51 (mm), as shown in
Figure 16.
In the section with cracks, the displacement response is similar to that of the intact section, occurring between 15 (s) and 25 (s). However, the amplitude increases significantly, with the vertical displacement amplitude of the rail increasing by 112% and the lateral displacement amplitude increasing by 53%, as shown in
Figure 17.
4.6. Experimental Error Analysis
This study identified several sources of error that could potentially affect the vibration measurements of subway tunnel structures. Firstly, the accuracy of the sensors and the performance of the data acquisition system are the primary sources of error. The accuracy, linearity, and drift characteristics of the acceleration and displacement sensors, as well as the resolution and sampling frequency of the data acquisition system and the signal-to-noise ratio, could all impact the measurement results. Secondly, environmental conditions are significant factors. External environmental vibrations (such as other trains passing by or construction work) and variations in temperature and humidity may affect sensor performance and data stability. Additionally, the installation position and fixation method of the sensors (such as the use of adhesives or mechanical fixtures) might introduce errors. To evaluate the repeatability and reproducibility of the experiment, multiple repeated measurements were conducted, and possible errors were compensated for during the Fourier transform and one-third octave band analysis. Statistical indicators such as standard deviation and confidence intervals were used to assess the reliability of the experimental data, and the results were compared with existing literature to discuss differences and possible sources of error. Finally, an analysis was conducted to determine whether these errors would substantially affect the conclusions, and any corrective or compensatory measures taken during the experiment were detailed to enhance the persuasiveness of the conclusions.
Through a comparison with existing literature, validation of the research methods, and a practical application of the results, this study thoroughly explored the impact of cracks on the vibration response of tunnel structures, thereby verifying and expanding upon existing theories. Firstly, the cracks significantly increased the vibration levels of the tunnel walls, sleepers, and rails compared to the existing studies, which is in line with the findings of the dynamic response of concrete structures with cracks as mentioned in a study by Xu et al. [
43]. This comparison not only confirms the critical role of cracks in underground structures but also further validates the use of vibration measurements as a key tool for assessing structural integrity.
In addition to these factors, the mass and speed of the train are critical in determining the characteristics of the sound intensity–frequency graph. Heavier trains tend to generate greater vibration energy, which is reflected in the higher sound intensity levels observed in the frequency domain. This is particularly noticeable in the low-frequency to mid-frequency ranges, where the impact of mass is most significant. Higher train speeds, on the other hand, tend to shift the vibration spectrum towards higher frequencies, amplifying the sound intensity in the high-frequency range. This relationship between train mass, speed, and frequency response has been well documented in studies such as those by Zhang et al. [
44] and Gupta et al. [
45], where increased mass and speed were shown to significantly influence the overall vibration characteristics. Incorporating these parameters into the analysis allowed for a more comprehensive understanding of the impact of cracks on tunnel vibrations and provided a deeper insight into the complex interplay between these variables.
In terms of research methods, a Fourier transform and one-third octave band analysis were employed, both of which are widely used in vibration analysis. However, by comparing RMS values and vibration levels across different frequency ranges, a deeper understanding was achieved regarding how cracks affect various components of tunnel structures. This methodological improvement enabled a more precise identification of the impact of cracks on tunnel vibrations and provided a new perspective for exploring the complex relationship between cracks and vibration responses, thereby supplementing and expanding upon existing research. Additionally, the results of this study have significant practical implications. The impact of cracks on the vibration response of tunnel walls was found to be the greatest, a finding that aligns with the results of Wu et al. [
46] on the vibration characteristics of tunnel structures in the presence of cracks. This suggests that special attention should be paid to the condition of tunnel walls during inspection and maintenance. This not only provides practical guidance for the maintenance and management of tunnels but also offers concrete support for relevant theories through experimental data. Through these methodological innovations and validation in practical applications, this study’s results not only enhance the scientific rigor of existing theories but also point to new directions for future research, demonstrating important academic and practical significance.
5. Conclusions
Vibration tests were conducted on a section of the subway, comparing normal and cracked segments. The dynamic response transmission within the tunnel and the amplification effects of cracks on the dynamic response of various components were analyzed using a time-domain analysis, a frequency-domain analysis, acceleration vibration levels, and a one-third octave band analysis. The specific conclusions are as follows:
(1) During train operations, various structures within the tunnel experience vibrations of different magnitudes. The vertical response of the rails, which are in direct contact with the train, is the most significant, with an average peak value of 29.39 (m/s2). This vibration decreases as it propagates to the track bed and tunnel walls. Due to the integral nature of the tunnel structure, the magnitudes of acceleration and vibration levels for the track bed and tunnel walls are generally consistent.
(2) The presence of cracks significantly affects the tunnel’s dynamic response. Acceleration peak values increase at locations with cracks compared to intact sections, with a noticeable increase in average acceleration and vibration levels. The magnitude of this increase decreases as the distance from the cracks and connections increases, with the tunnel walls experiencing the highest increases, followed by the track bed and then the rails.
(3) Fourier transformation of the measured data reveals that the vibration induced by train operations attenuates as it propagates through the tunnel, consistent with the conclusions drawn from the time-domain analysis. Vibration spectra at different measurement points show variations. The rail spectrum is primarily concentrated in the mid-to-high frequency range (200 Hz–1400 Hz), while the spectra for the track bed and tunnel walls are concentrated in the low-to-medium frequency range (50 Hz–400 Hz).
(4) The analysis of the spectra at crack locations shows that the cracks enhance the peak values within the main frequency range of each structure’s spectrum without altering the overall spectrum distribution. The amplitude of the main frequency increases by 70% in the rail spectrum, 100% in the track bed spectrum, and 300% in the tunnel wall spectrum, indicating that cracks have a greater impact on the tunnel wall, followed by the track bed and then the rails.
(5) The analysis using the one-third octave method reveals that the tunnel’s dynamic response follows a characteristic pattern of slow growth in low frequencies and rapid growth in high frequencies. Due to cracks, there is an average increase of 2% in peak vibration levels for the rails, 2.3% for the track bed, and 6% for the lining. The peak frequency of the vibration levels matches those obtained from the Fourier spectrum conversion. Compared to noise control regulations, the impact of cracks on tunnel dynamic response is significant, and existing measures cannot meet the vibration tolerance requirements, necessitating prompt crack repairs and special vibration reduction measures.
(6) The measured results indicate that vertical rail displacement is greater than lateral displacement, consistent with the theory of adhesion and slip. When the train passes the measurement points, significant vibration occurs, gradually decreasing as the train moves away, with rail displacement gradually returning to its normal value. Under normal conditions, vertical rail displacement is 1.1 mm, and lateral displacement is 0.51 mm. The presence of cracks increases vertical displacement by 112% and lateral displacement by 53%, without altering the distribution of displacement responses.
(7) Advanced technologies such as Ground Penetrating Radar (GPR), fiber optic sensors, and acoustic emission monitoring are used to detect and monitor cracks in tunnel structures more effectively. These methods are highly sensitive and accurate, allowing for rapid detection and timely maintenance interventions.
(8) Incorporating advanced computational techniques such as machine learning (ML) [
47], artificial intelligence (AI) [
48], and artificial neural network (ANN) [
49] algorithms can significantly enhance our analysis of the vibration–frequency data for various tunnel components, both with and without cracks. By training these algorithms with the extracted data, we can estimate the magnitude of vibration based on the induced frequency under specific conditions. This approach facilitates optimal design and rapid mechanical analysis, providing valuable insights for maintaining and improving tunnel infrastructure.