Development of a Sinusoidal Corrugated Dual-Axial Flexure Mechanism for Planar Nanopositioning
Abstract
:1. Introduction
2. The Sinusoidal Corrugated Flexure Linkage
2.1. Stiffness Modeling of the Sinusoidal Corrugated Flexure Linkage
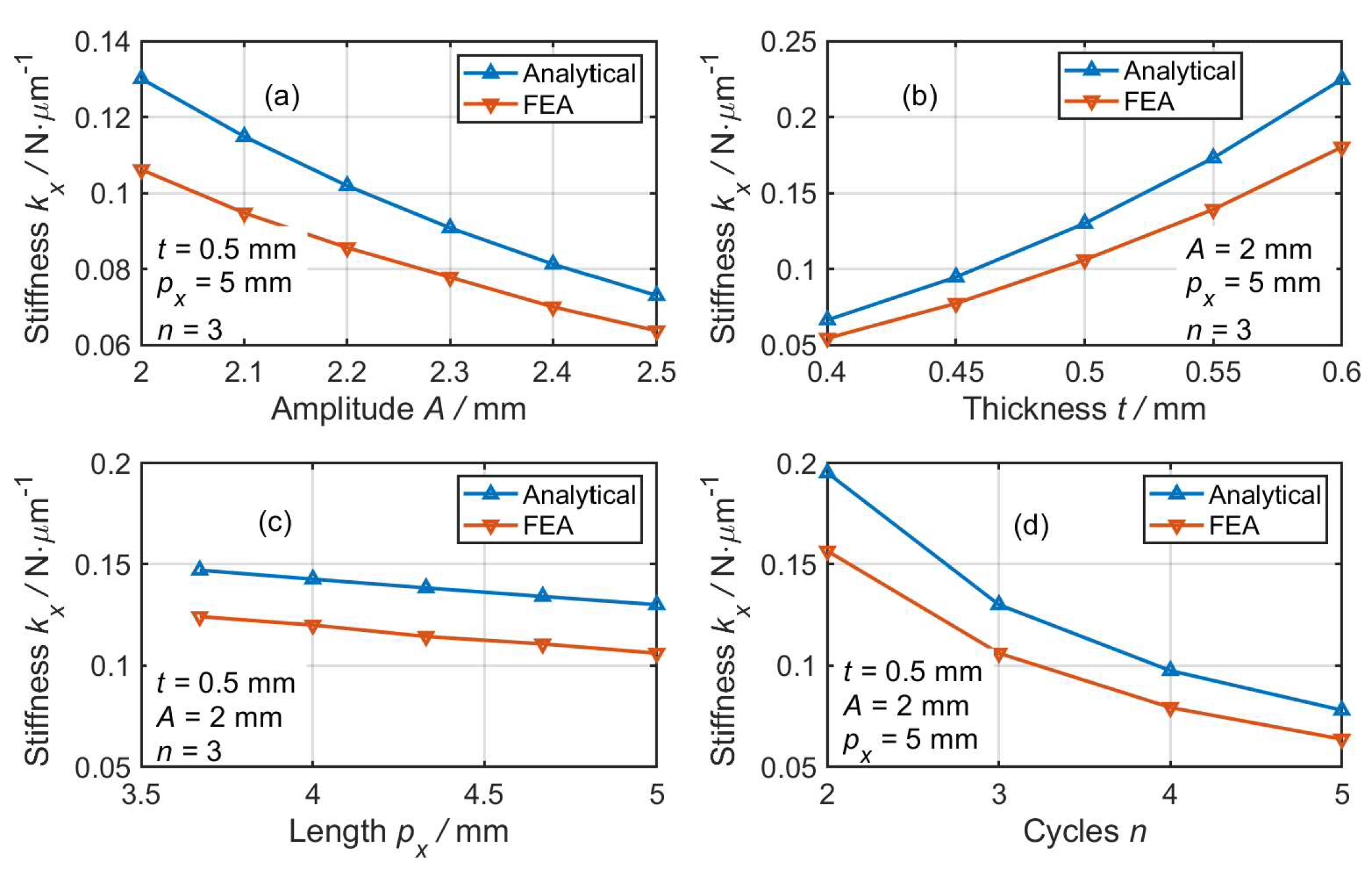
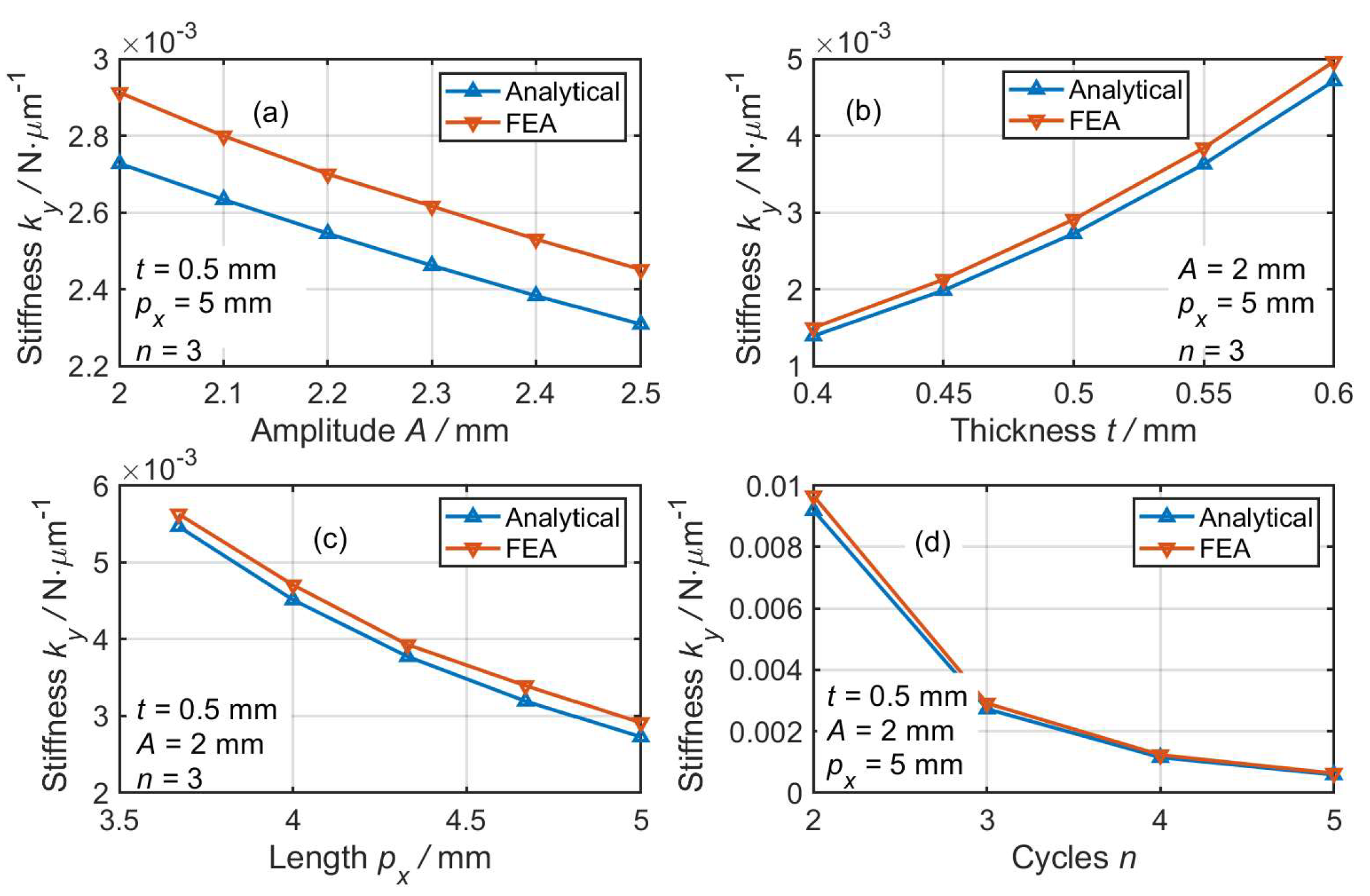
2.2. FEA-Based Stiffness Verification
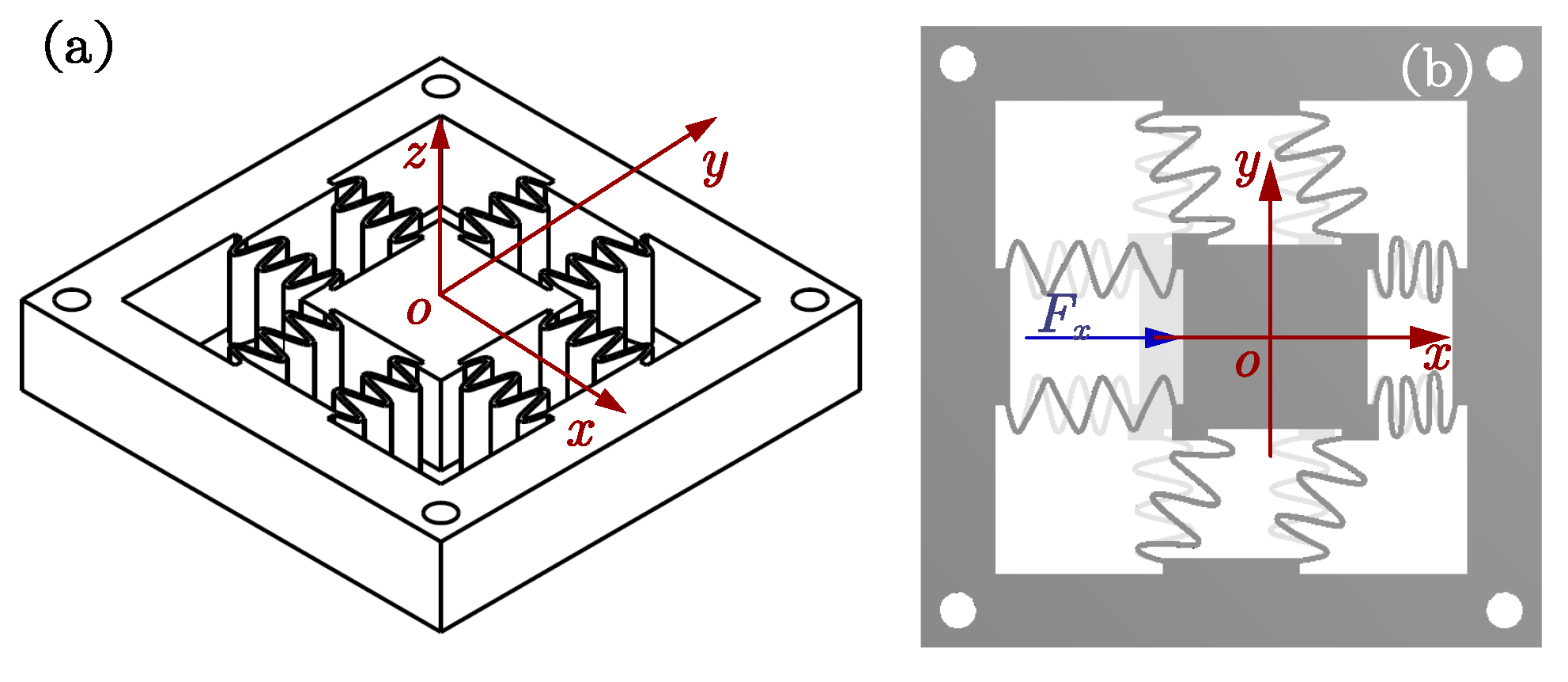
3. The Sinusoidal Corrugated Dual-Axial Mechanism
3.1. Modeling of the Dual-Axial Mechanism
3.1.1. Stiffness Modeling
3.1.2. Dynamics Modeling
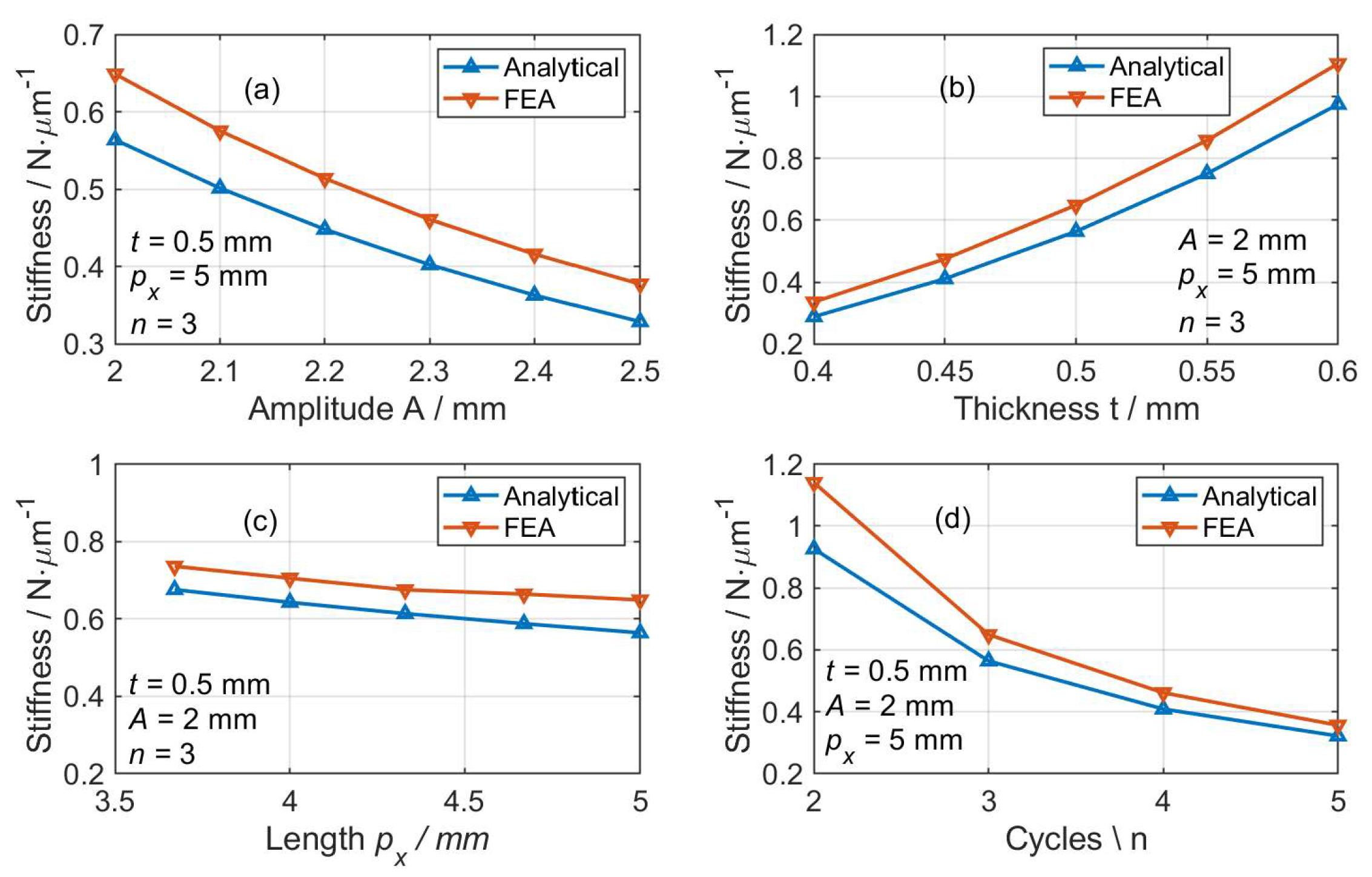
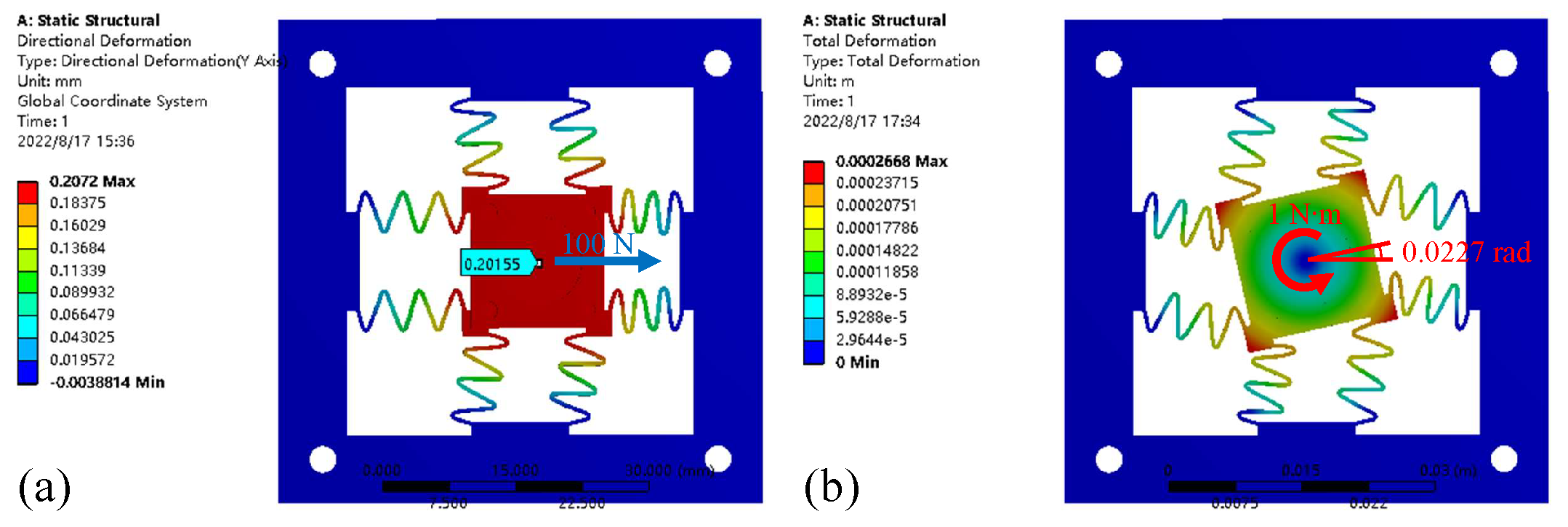
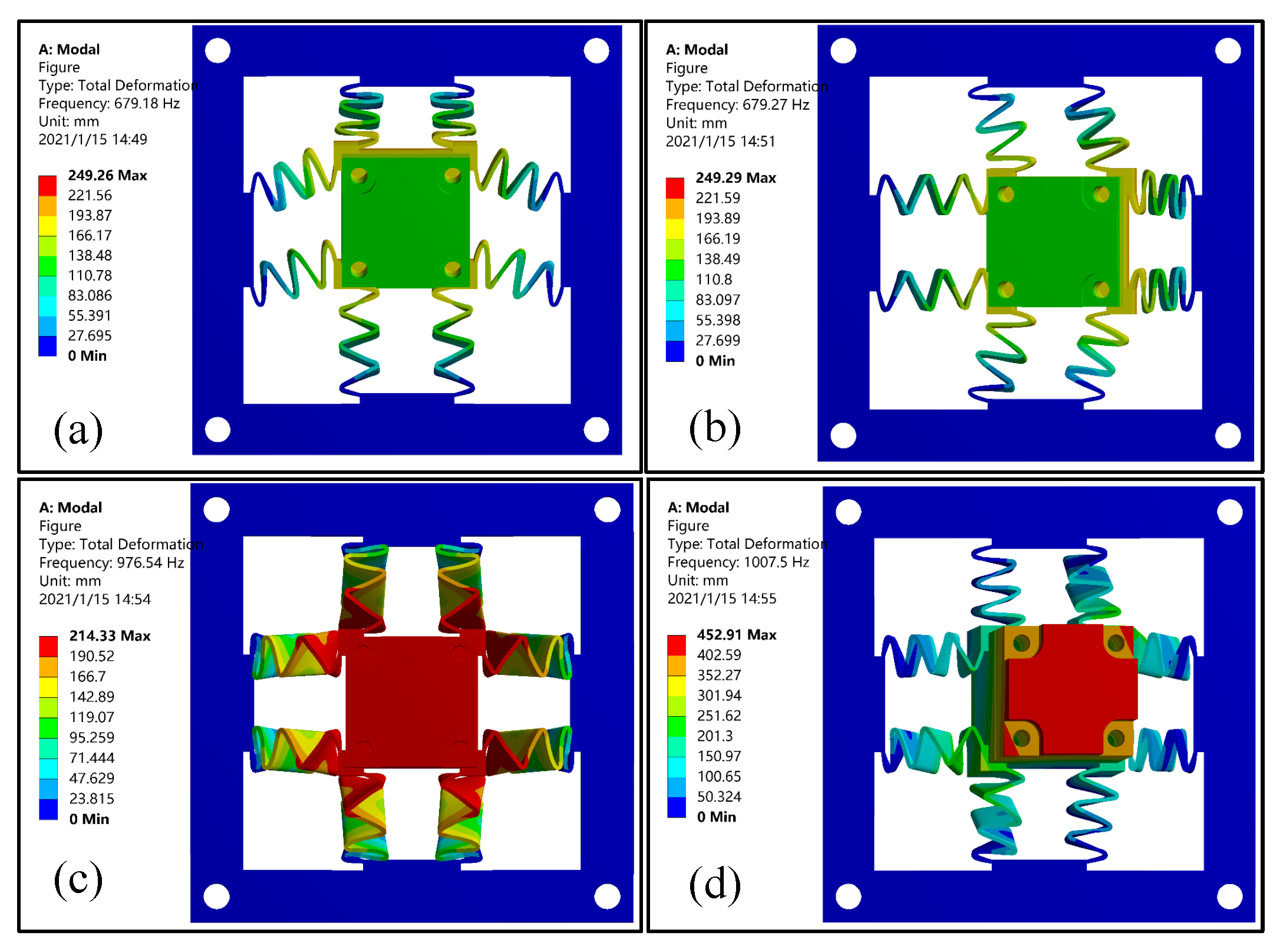
3.2. FEA Verification of the Mechanism
4. Structure Parameter Determination for a Planar Nanopositioning Stage
5. Experimental Results and Discussion
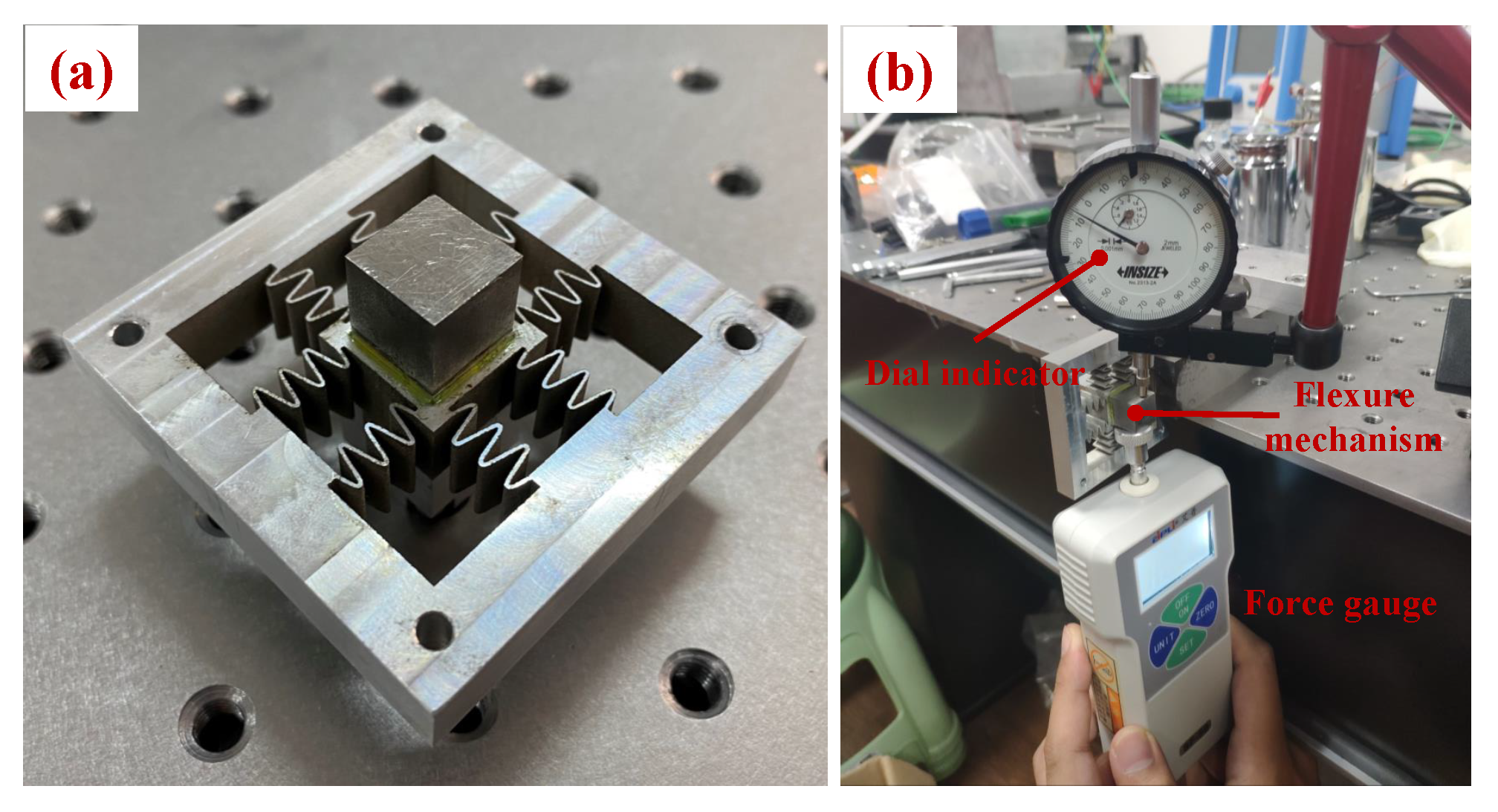
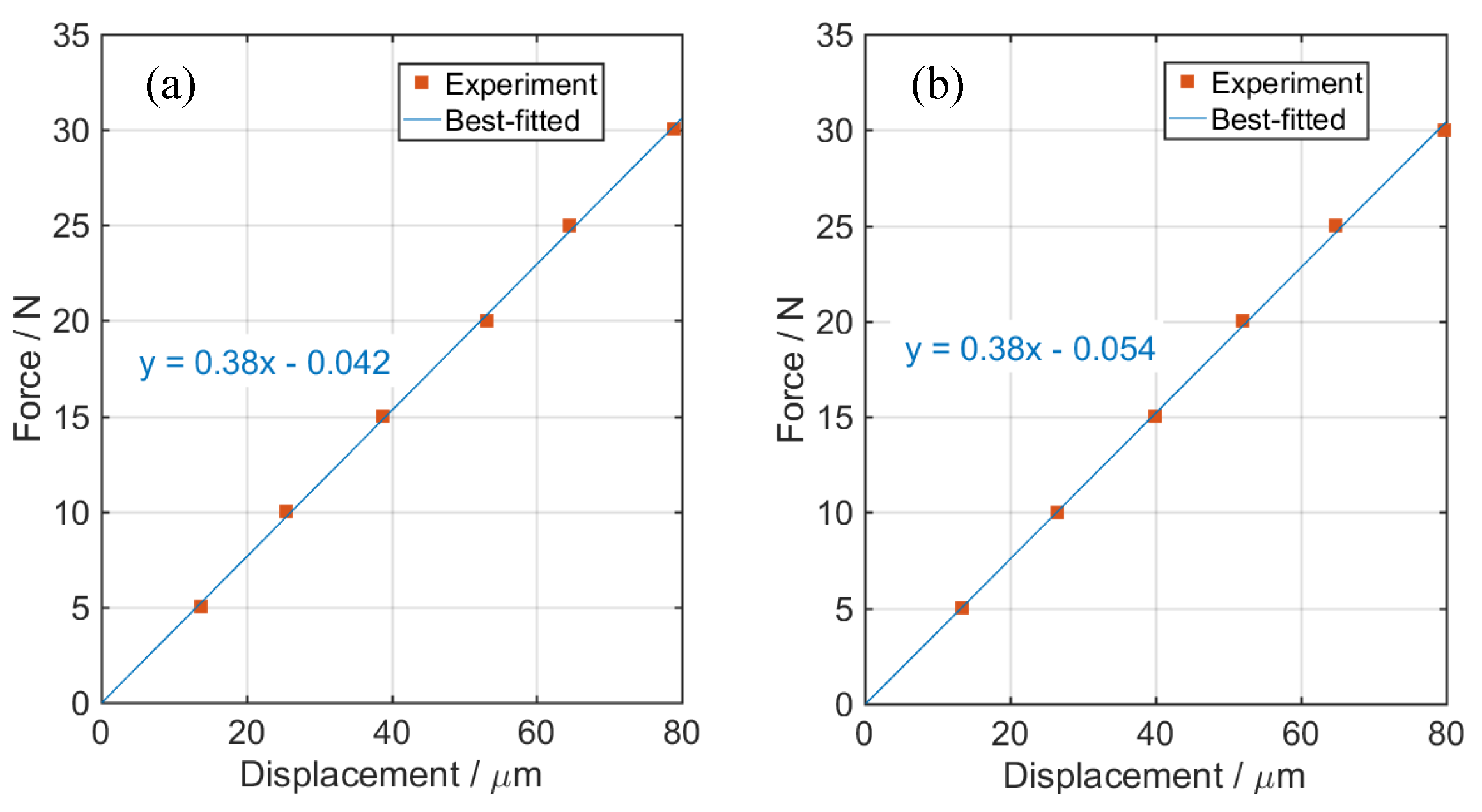
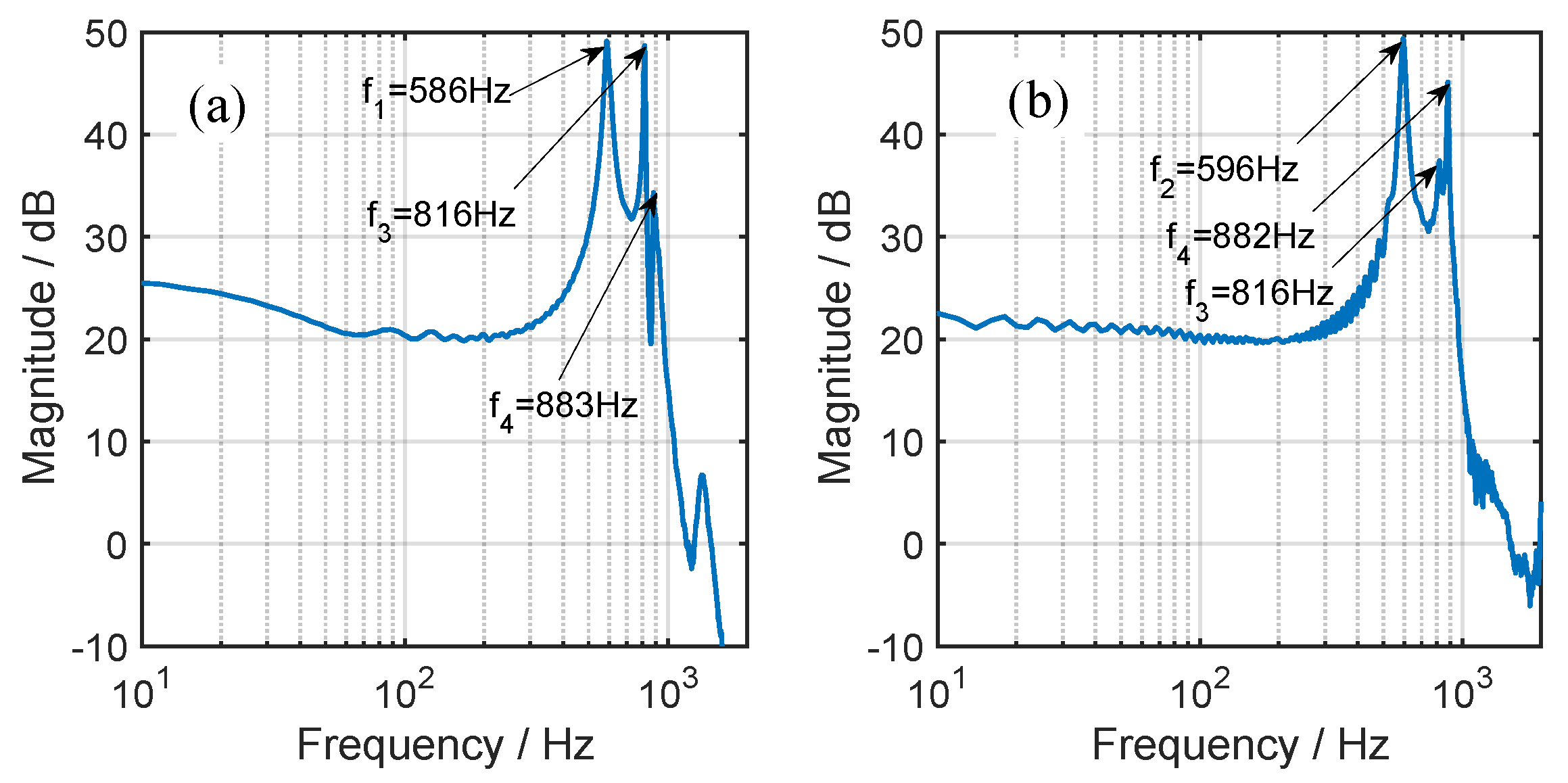
5.1. Stiffness-Testing the Mechanism
5.2. Performance Testing for the Planar Stage
5.2.1. Open-Loop Performance
5.2.2. Closed-Loop Performance
5.3. Results Comparison and Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
Appendix A.1. The Compliance Equations for the Sinusoidal Corrugated Flexure Unit
Appendix A.2. The Compliance Transformation Matrix
Appendix A.3. The Characteristic Matrix of the Designed Stage
References
- Yong, Y.K.; Moheimani, S.O.R.; Kenton, B.J.; Leang, K.K. Invited Review Article: High-speed flexure-guided nanopositioning: Mechanical design and control issues. Rev. Sci. Instruments 2012, 83, 121101. [Google Scholar] [CrossRef] [PubMed]
- Ortlepp, I.; Fröhlich, T.; Füßl, R.; Reger, J.; Schäffel, C.; Sinzinger, S.; Strehle, S.; Theska, R.; Zentner, L.; Zöllner, J.P.; et al. Tip-and Laser-based 3D Nanofabrication in Extended Macroscopic Working Areas. Nanomanuf. Metrol. 2021, 4, 132–148. [Google Scholar] [CrossRef]
- Matsukuma, H.; Adachi, K.; Sugawara, T.; Shimizu, Y.; Gao, W.; Niwa, E.; Sasaki, Y. Closed-Loop Control of an XYZ Micro-Stage and Designing of Mechanical Structure for Reduction in Motion Errors. Nanomanuf. Metrol. 2021, 4, 53–66. [Google Scholar] [CrossRef]
- Zhu, W.L.; Zhu, Z.; Guo, P.; Ju, B.F. A novel hybrid actuation mechanism based XY nanopositioning stage with totally decoupled kinematics. Mech. Syst. Signal Process. 2018, 99, 747–759. [Google Scholar] [CrossRef]
- Wang, F.; Zhao, X.; Huo, Z.; Shi, B.; Liang, C.; Tian, Y.; Zhang, D. A 2-DOF nano-positioning scanner with novel compound decoupling-guiding mechanism. Mech. Mach. Theory 2021, 155, 104066. [Google Scholar] [CrossRef]
- Zhu, Z.; Tong, Z.; To, S.; Jiang, X. Tuned diamond turning of micro-structured surfaces on brittle materials for the improvement of machining efficiency. CIRP Ann. 2019, 68, 559–562. [Google Scholar] [CrossRef]
- Polit, S.; Dong, J. Development of a high-bandwidth XY nanopositioning stage for high-rate micro-/nanomanufacturing. IEEE/ASME Trans. Mechatronics 2010, 16, 724–733. [Google Scholar] [CrossRef]
- Tian, Y.; Ma, Y.; Wang, F.; Lu, K.; Zhang, D. A novel XYZ micro/nano positioner with an amplifier based on L-shape levers and half-bridge structure. Sens. Actuators A Phys. 2020, 302, 111777. [Google Scholar] [CrossRef]
- Zhu, Z.; To, S.; Ehmann, K.F.; Zhou, X. Design, analysis, and realization of a novel piezoelectrically actuated rotary spatial vibration system for micro-/nanomachining. IEEE/ASME Trans. Mechatronics 2017, 22, 1227–1237. [Google Scholar] [CrossRef]
- Howell, L.L. Compliant mechanisms. In 21st Century Kinematics; Springer: Berlin/Heidelberg, Germany, 2013; pp. 189–216. [Google Scholar]
- Wang, N.; Zhang, Z.; Zhang, X.; Cui, C. Optimization of a 2-DOF micro-positioning stage using corrugated flexure units. Mech. Mach. Theory 2018, 121, 683–696. [Google Scholar] [CrossRef]
- Xi, S.; Lai, L.J. Paired double parallelogram flexure mechanism clamped by corrugated beam for underconstraint elimination. Rev. Sci. Instruments 2020, 91, 086102. [Google Scholar] [CrossRef]
- Li, C.; Wang, N.; Yue, F.; Zhang, X. Optimization of Translational Flexure Joints Using Corrugated Units Under Stress Constraints. J. Mech. Robot. 2021, 13, 061006. [Google Scholar] [CrossRef]
- Wang, N.; Liang, X.; Zhang, X. Stiffness Analysis of Corrugated Flexure Beam Used in Compliant Mechanisms. Chin. J. Mech. Eng. 2015, 28, 776–784. [Google Scholar] [CrossRef]
- Wang, N.; Zhang, Z.; Yue, F.; Zhang, X. Design and analysis of translational joints using corrugated flexural beams with conic curve segments. Mech. Mach. Theory 2019, 132, 223–235. [Google Scholar] [CrossRef]
- Wu, J.; Zhang, Y.; Cai, S.; Cui, J. Modeling and analysis of conical-shaped notch flexure hinges based on NURBS. Mech. Mach. Theory 2018, 128, 560–568. [Google Scholar] [CrossRef]
- Lobontiu, N.; Cullin, M. In-plane elastic response of two-segment circular-axis symmetric notch flexure hinges: The right circular design. Precis. Eng. 2013, 37, 542–555. [Google Scholar] [CrossRef]
- Li, Y.; Xu, Q. Design and analysis of a totally decoupled flexure-based XY parallel micromanipulator. IEEE Trans. Robot. 2009, 25, 645–657. [Google Scholar]
- Zhu, Z.; Zhou, X.; Liu, Z.; Wang, R.; Zhu, L. Development of a piezoelectrically actuated two-degree-of-freedom fast tool servo with decoupled motions for micro-/nanomachining. Precis. Eng. 2014, 38, 809–820. [Google Scholar] [CrossRef]
- Zhu, Z.; Chen, L.; To, S. A novel direct drive electromagnetic XY nanopositioning stage. CIRP Ann. 2021, 70, 415–418. [Google Scholar] [CrossRef]
- Khawwaf, J.; Zheng, J.; Chai, R.; Lu, R.; Man, Z. Adaptive Microtracking Control for an Underwater IPMC Actuator Using New Hyperplane-Based Sliding Mode. IEEE/ASME Trans. Mechatronics 2019, 24, 2108–2117. [Google Scholar] [CrossRef]
- Yi, J.; Chang, S.; Shen, Y. Disturbance-observer-based hysteresis compensation for piezoelectric actuators. IEEE/ASME Trans. Mechatronics 2009, 14, 456–464. [Google Scholar]
- Zhu, Z.; Chen, L.; Huang, P.; Schönemann, L.; Riemer, O.; Yao, J.; To, S.; Zhu, W.L. Design and control of a piezoelectrically actuated fast tool servo for diamond turning of microstructured surfaces. IEEE Trans. Ind. Electron. 2020, 67, 6688–6697. [Google Scholar] [CrossRef]
- Ito, S.; Troppmair, S.; Lindner, B.; Cigarini, F.; Schitter, G. Long-range fast nanopositioner using nonlinearities of hybrid reluctance actuator for energy efficiency. IEEE Trans. Ind. Electron. 2018, 66, 3051–3059. [Google Scholar] [CrossRef]
- Wang, F.; Huo, Z.; Liang, C.; Shi, B.; Tian, Y.; Zhao, X.; Zhang, D. A Novel Actuator-Internal Micro/Nano Positioning Stage with an Arch-Shape Bridge-Type Amplifier. IEEE Trans. Ind. Electron. 2019, 66, 9161–9172. [Google Scholar] [CrossRef]
- Du, Y.; Li, T.; Jiang, Y.; Wang, H. Design and analysis of a 2-degree-of-freedom flexure-based micro-motion stage. Adv. Mech. Eng. 2016, 8, 168781401663830. [Google Scholar] [CrossRef] [Green Version]

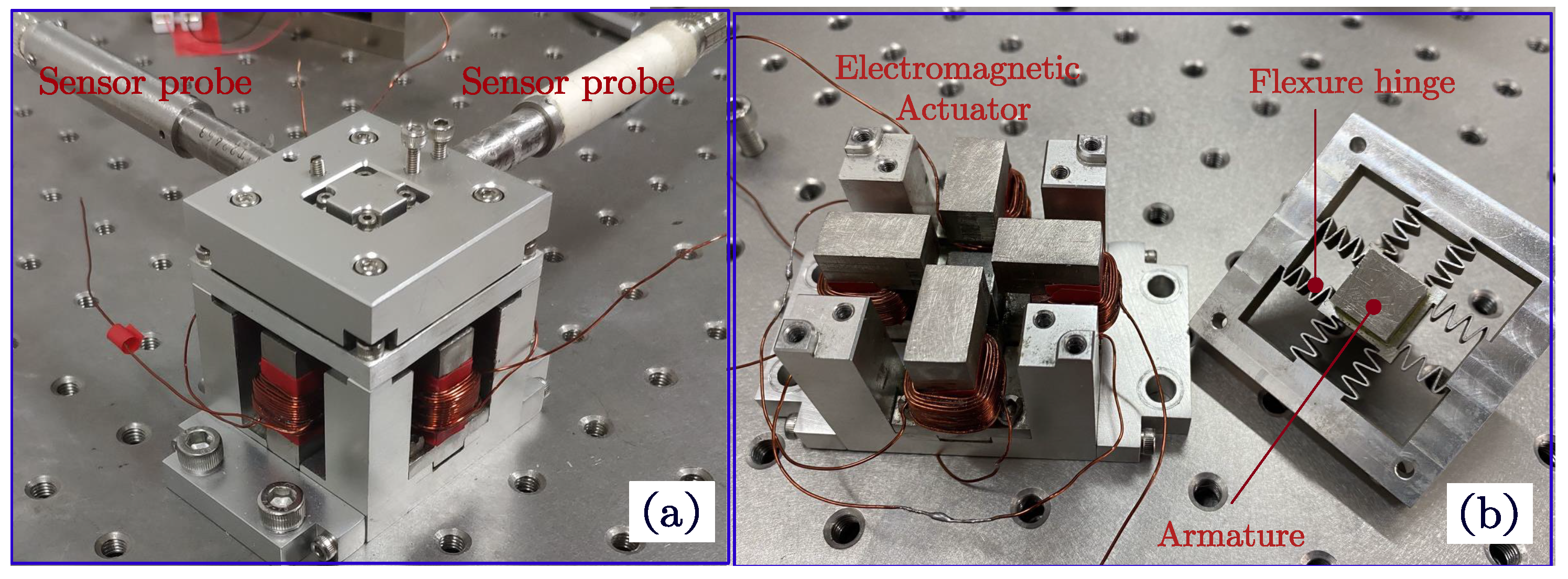



| (N/m) | (Hz) | |
|---|---|---|
| Analytical | 0.408 | 599 |
| FEA | 0.496 | 679 |
| Experiment | 0.38 | 586 |
| Error | 7.4% | 2.2% |
| Reference | Dimension (mm) | Workspace (m) | Bandwidth (Hz) | Coupling Ratio (%) |
|---|---|---|---|---|
| [4] | 165 × 145 | 31.5 × 31.5 | 570 | 0.7/0.9 |
| [5] | 142 × 142 | 40.2 × 42.9 | 483 | 0.58/0.56 |
| [11] | - | 1800 × 1820 | 72 | - |
| [25] | 160 × 160 | 55.4 × 53.2 | 253 | 0.42/0.45 |
| [26] | 190 × 190 | 19.5 × 19 | 2k | 0.62/0.99 |
| This work | 54.4 × 54.4 | 133.5 × 132.6 | 586 | 2.47/0.95 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Niu, Y.; Chen, X.; Chen, L.; Zhu, Z.; Huang, P. Development of a Sinusoidal Corrugated Dual-Axial Flexure Mechanism for Planar Nanopositioning. Actuators 2022, 11, 276. https://doi.org/10.3390/act11100276
Niu Y, Chen X, Chen L, Zhu Z, Huang P. Development of a Sinusoidal Corrugated Dual-Axial Flexure Mechanism for Planar Nanopositioning. Actuators. 2022; 11(10):276. https://doi.org/10.3390/act11100276
Chicago/Turabian StyleNiu, Yuhan, Xingyou Chen, Li Chen, Zhiwei Zhu, and Peng Huang. 2022. "Development of a Sinusoidal Corrugated Dual-Axial Flexure Mechanism for Planar Nanopositioning" Actuators 11, no. 10: 276. https://doi.org/10.3390/act11100276
APA StyleNiu, Y., Chen, X., Chen, L., Zhu, Z., & Huang, P. (2022). Development of a Sinusoidal Corrugated Dual-Axial Flexure Mechanism for Planar Nanopositioning. Actuators, 11(10), 276. https://doi.org/10.3390/act11100276







