Experimental Analysis of Electrohydrodynamic Jet Actuation Modes Based on the Phase Doppler Technique
Abstract
1. Introduction
2. Materials and Methods
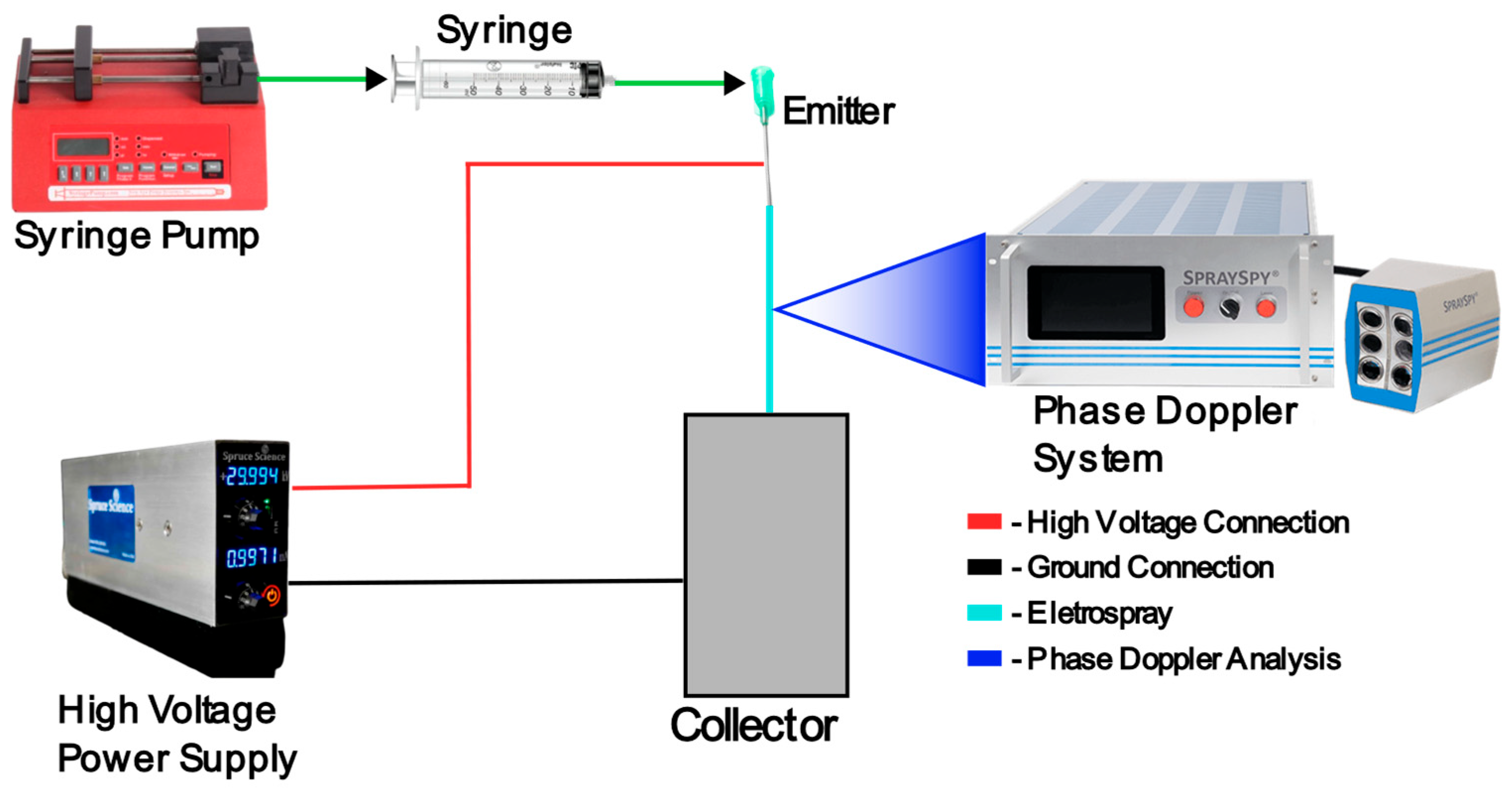
2.1. Electrospray Setup
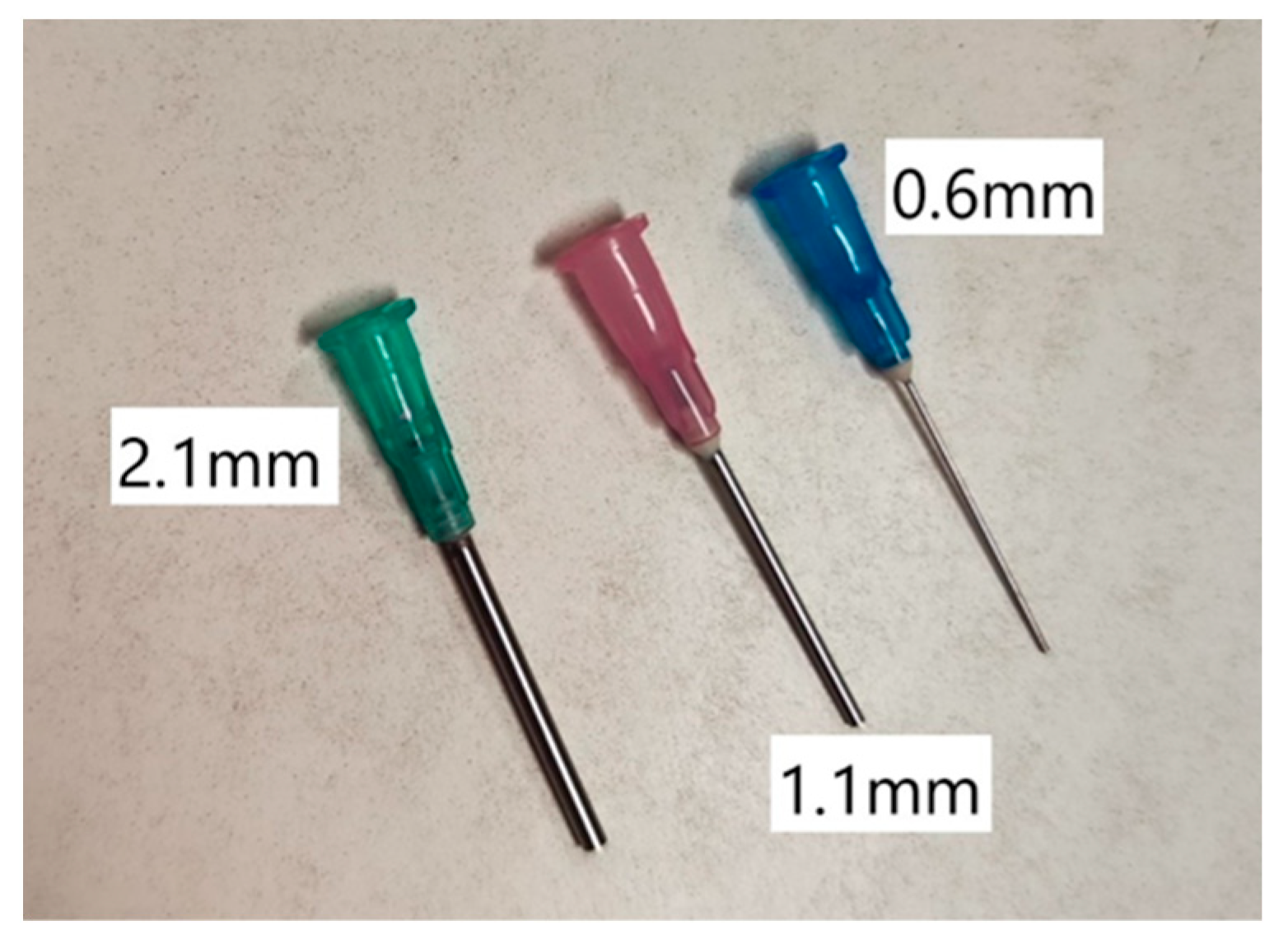
2.2. Emitter and Collector
2.3. Supply Systems
2.4. Phase Doppler System
2.5. High-Speed Camera
3. Results
3.1. Flow Rate
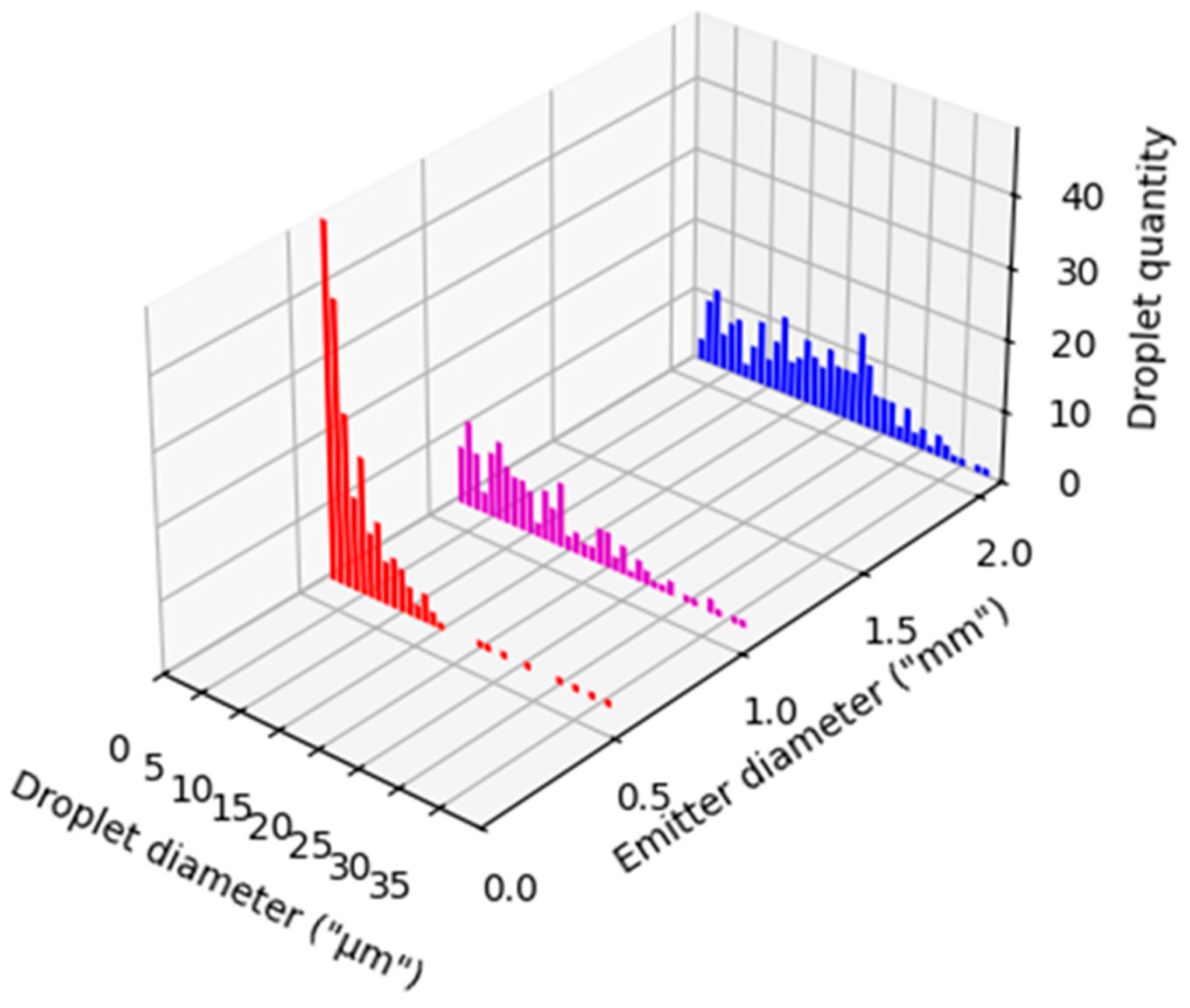
3.2. Emitter Diameter
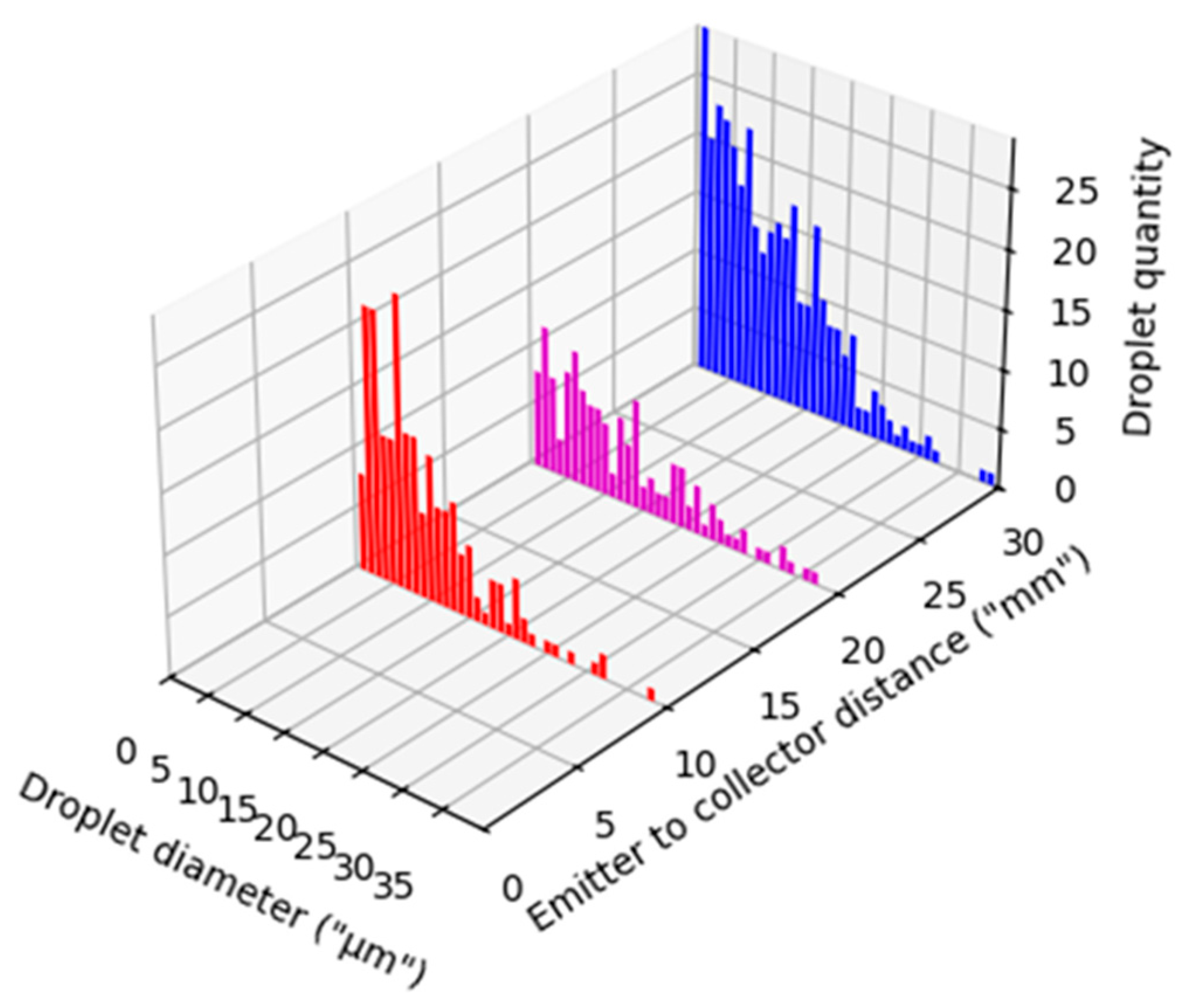
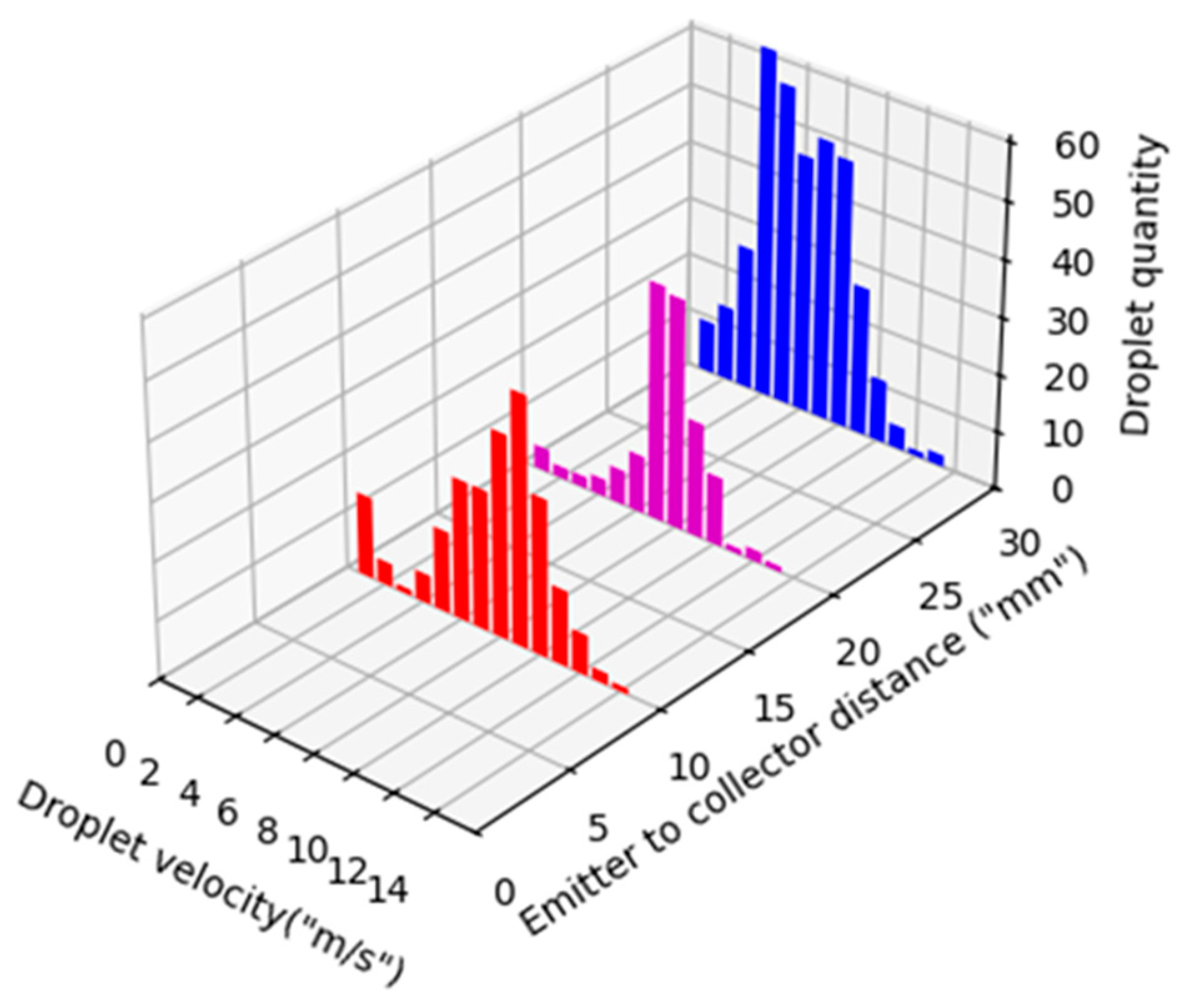
3.3. Emitter to Collector Distance
3.4. Taylor Cone Angle
3.5. Experimental Parameter Study
3.6. Results Comparison
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| PDPA | Phase Doppler Particle Analyzer |
References
- Taylor, G. Disintegration of water drops in an electric field. Proc. R. Soc. Lond. A Math. Phys. Sci. 1964, 280, 383–397. [Google Scholar] [CrossRef]
- Cloupeau, M.; Prunet-Foch, B. Electrohydrodynamic spraying functioning modes: A critical review. J. Aerosol Sci. 1994, 25, 1021–1036. [Google Scholar] [CrossRef]
- Kim, J.Y.; Lee, S.J.; Hong, J.G. Spray Mode and Monodisperse Droplet Properties of an Electrospray. ACS Omega 2022, 7, 28667–28674. [Google Scholar] [CrossRef]
- Zeleny, J. Electrical discharges from pointed conductors. Phys. Rev. B 1920, 16, 102–125. [Google Scholar] [CrossRef]
- Zeleny, J. Instability of electrified liquid surfaces. Phys. Rev. B 1917, 10, 1. [Google Scholar] [CrossRef]
- Melcher, J.R.; Taylor, G.I. Electrohydrodynamics: A Review of the Role of Interfacial Shear Stresses. Annu. Rev. Fluid Mech. 1969, 1, 111–146. [Google Scholar] [CrossRef]
- Hayati, I.; Bailey, A.I.; Tadros, T.H.F. Investigations into the Mechanisms of Electrohydrodynamic Spraying of Liquids I. Effect of Electric Field and the Environment on Pendant Drops and Factors Affecting the Formation of Stable Jets and Atomization. J. Colloid Interface Sci. 1987, 117, 205–221. [Google Scholar] [CrossRef]
- Cloupeau, M.; Prunet-Foch, B. Electrostatic spraying of liquids in cone-jet mode. J. Electrost. 1989, 22, 135–159. [Google Scholar] [CrossRef]
- De La Mora, J.F. The fluid dynamics of Taylor Cones. Annu. Rev. Fluid Mech. 2007, 39, 217–243. [Google Scholar] [CrossRef]
- De La Mora, J.F. The effect of charge emission from electrified liquid cones. J. Fluid Mech. 1992, 243, 561–574. [Google Scholar] [CrossRef]
- De La Mora, J.F.; Loscertales, I.G. The current emitted by highly conducting Taylor cones. J. Fluid Mech. 1994, 260, 155–184. [Google Scholar] [CrossRef]
- Chen, D.R.; Pui, D.Y.H. Experimental investigation of scaling laws for electrospraying: Dielectric constant effect. Aerosol Sci. Technol. 1997, 27, 367–380. [Google Scholar] [CrossRef]
- Gañán-Calvo, A.M. On the general scaling theory for electrospraying. J. Fluid Mech. 2004, 507, 203–212. [Google Scholar] [CrossRef]
- Gañán-Calvo, A.M. The Nature and Scaling Laws of the Surface Charge in Electrohydrodynamic Spraying. In Proceedings of the American Physical Society, Division of Fluid Dynamics Meeting, Philadelphia, PA, USA, 22–24 November 1998; Available online: https://www.researchgate.net/publication/241405133 (accessed on 24 January 2025).
- Gañán-Calvo, A.M.; Lasheras, J.C.; Vila, J.D.; Barrero, A. The electrostatic spray emitted from an electrified conical meniscus. J. Aerosol Sci. 1994, 25, 1121–1142. [Google Scholar] [CrossRef]
- Gañán-Calvo, A.M.; López-Herrera, J.M.; Herrada, M.A.; Ramos, A.; Montanero, J.M. Review on the physics of electrospray: From electrokinetics to the operating conditions of single and coaxial Taylor cone-jets, and AC electrospray. J. Aerosol Sci. 2018, 125, 32–56. [Google Scholar] [CrossRef]
- Cândido, S.; Páscoa, J.C. Data-driven surrogate modelling of multistage Taylor cone–Jet dynamics. Phys. Fluids 2024, 36, 052102. [Google Scholar] [CrossRef]
- Wang, F.; Elbadawi, M.; Tsilova, S.L.; Gaisford, S.; Basit, A.W.; Parhizkar, M. Machine learning predicts electrospray particle size. Mater. Des. 2022, 219, 110735. [Google Scholar] [CrossRef]
- Cândido, S.; Páscoa, J.C. Dynamics of three-dimensional electrohydrodynamic instabilities on Taylor cone jets using a numerical approach. Phys. Fluids 2023, 35, 052110. [Google Scholar] [CrossRef]
- Chiu, Y.-H.; Austin, B.L.; Dressler, R.A.; Levandier, D.; Murray, P.T.; Lozano, P.; Martinez-Sanchez, M. Mass spectrometric analysis of colloid thruster ion emission from selected propellants. J. Propuls. Power 2005, 21, 416–423. [Google Scholar] [CrossRef]
- Fenn, J.B.; Mann, M.; Meng, C.K.; Wong, S.F.; Whitehouse, C.M. Electrospray Ionization for Mass Spectrometry of Large Biomolecules. Science 1989, 246, 64–71. [Google Scholar] [CrossRef]
- Gamero-Castanõ, M.; Magnani, M. The minimum flow rate of electrosprays in the cone-jet mode. J. Fluid Mech. 2019, 876, 553–572. [Google Scholar] [CrossRef]
- Thuppul, A.; Collins, A.L.; Wright, P.L.; Uchizono, N.M.; Wirz, R.E. Mass flux and current density distributions of electrospray plumes. J. Appl. Phys. 2021, 130, 103301. [Google Scholar] [CrossRef]
- Chiu, Y.H.; Dressler, R.A. Ionic liquids for space propulsion. In ACS Symposium Series; ACS Publications: Washington, DC, USA, 2007; pp. 138–160. [Google Scholar] [CrossRef]
- Lenguito, G.; Gomez, A. Pressure-driven operation of microfabricated multiplexed electrosprays of ionic liquid solutions for space propulsion applications. J. Microelectromechanical Syst. 2014, 23, 689–698. [Google Scholar] [CrossRef]
- Morais, A.Í.S.; Vieira, E.G.; Afewerki, S.; Sousa, R.B.; Honorio, L.M.C.; Cambrussi, A.N.C.O.; Santos, J.A.; Bezerra, R.D.S.; Furtini, J.A.O.; Silva-Filho, E.C.; et al. Fabrication of polymeric microparticles by electrospray: The impact of experimental parameters. J. Funct. Biomater. 2020, 11, 4. [Google Scholar] [CrossRef] [PubMed]
- Jaworek, A. Micro- and nanoparticle production by electrospraying. Powder Technol. 2007, 176, 18–35. [Google Scholar] [CrossRef]
- Guo, L.; Duan, Y.; Huang, Y.A.; Yin, Z. Experimental study of the influence of ink properties and process parameters on ejection volume in electrohydrodynamic jet printing. Micromachines 2018, 9, 522. [Google Scholar] [CrossRef]
- Yu, M.; Ahn, K.H.; Lee, S.J. Design optimization of ink in electrohydrodynamic jet printing: Effect of viscoelasticity on the formation of Taylor cone jet. Mater. Des. 2016, 89, 109–115. [Google Scholar] [CrossRef]
- Grace, J.M.; Marijnissen, J.C.M. A review of liquid atomization by electrical means. J. Aerosol Sci. 1994, 25, 1005–1019. [Google Scholar] [CrossRef]
- Miao, P.; Balachandran, W.; Member, S.; Xiao, P.; Miao, P.; Balachandran, W. Formation of Ceramic Thin Films Using Electrospray in Cone-Jet Mode. IEEE Trans. Ind. Appl. 2002, 38, 50–56. [Google Scholar] [CrossRef]
- Pendar, M.R.; Rodrigues, F.; Páscoa, J.C.; Lima, R. Review of coating and curing processes: Evaluation in automotive industry. Phys. Fluids 2022, 34, 101301. [Google Scholar] [CrossRef]
- Maktabi, S.; Chiarot, P.R. Electrohydrodynamic printing of organic polymeric resistors on flat and uneven surfaces. J. Appl. Phys. 2016, 120, 084903. [Google Scholar] [CrossRef]
- Richardson, S.M.; Hoyland, J.A.; Mobasheri, R.; Csaki, C.; Shakibaei, M.; Mobasheri, A. Mesenchymal stem cells in regenerative medicine: Opportunities and challenges for articular cartilage and intervertebral disc tissue engineering. J. Cell. Physiol. 2009, 222, 23–32. [Google Scholar] [CrossRef] [PubMed]
- Guastaferro, M.; Baldino, L.; Cardea, S.; Reverchon, E. Supercritical-CO2-Assisted Electrospray for the Production of the PLA-Antibiotic-Sustained Drug-Delivery System. Processes 2023, 11, 2957. [Google Scholar] [CrossRef]
- Vu, H.-D.; Vu, T.-H.; Mai, N.L.; Pande, D.C.; Dao, D.V.; Rehm, B.H.; Nguyen, N.-T.; Grant, G.D.; Tran, C.-D.; Zhu, Y.; et al. In-flight electro-neutralisation electrospray for pulmonary drug delivery. Nano Today 2024, 55, 102217. [Google Scholar] [CrossRef]
- Marsh, B.M.; Iyer, K.; Cooks, R.G. Reaction Acceleration in Electrospray Droplets: Size, Distance, and Surfactant Effects. J. Am. Soc. Mass Spectrom. 2019, 30, 2022–2030. [Google Scholar] [CrossRef]
- Rovelli, G.; Jacobs, M.I.; Willis, M.D.; Rapf, R.J.; Prophet, A.M.; Wilson, K.R. A critical analysis of electrospray techniques for the determination of accelerated rates and mechanisms of chemical reactions in droplets. Chem. Sci. 2020, 11, 13026–13043. [Google Scholar] [CrossRef]
- Gomez, A.; Tang, K. Charge and fission of droplets in electrostatic sprays. Phys. Fluids 1994, 6, 404–414. [Google Scholar] [CrossRef]
- Tang, K.; Gomez, A. On the structure of an electrostatic spray of monodisperse droplets. Phys. Fluids 1994, 6, 2317–2332. [Google Scholar] [CrossRef]
- Olumee, Z.; Callahan, J.H.; Vertes, A. Droplet dynamics changes in electrostatic sprays of methanol—Water mixtures. J. Phys. Chem. A 1998, 102, 9154–9160. [Google Scholar] [CrossRef]
- Rosell-Llompart, J.; Grifoll, J.; Loscertales, I.G. Electrosprays in the cone-jet mode: From Taylor cone formation to spray development. J. Aerosol Sci. 2018, 125, 2–31. [Google Scholar] [CrossRef]
- Sultan, F.; Allaf-Akbari, E.; Ashgriz, N. Effects of a Coflowing Air on the Characteristics of an Electrospray. Aerosol Sci. Eng. 2020, 4, 210–218. [Google Scholar] [CrossRef]
- Gafian-Calvo, A.M.; Davila, J.; Barrero, A. Current and droplet size in the electrospraying of liquids. Scaling Laws. J. Aerosol Sci. 1997, 28, 249–275. [Google Scholar] [CrossRef]
- López-Herrera, J.M.; Barrero, A.; López, A.; Loscertales, I.G.; Márquez, M. Coaxial jets generated from electrified Taylor cones. Scaling laws. J. Aerosol Sci. 2003, 34, 535–552. [Google Scholar] [CrossRef]
- Ku, B.K.; Kim, S.S. Electrospray Characteristics of Highly Viscous Liquids. J. Aerosol Sci. 2002, 33, 1361–1378. [Google Scholar] [CrossRef]
- Tang, K.; Gomez, A. Monodisperse Electrosprays of Low Electric Conductivity Liquids in the Cone-Jet Mode. J. Colloid Interface Sci. 1996, 184, 500–511. [Google Scholar] [CrossRef] [PubMed]
- Huh, H.; Wirz, R.E. Simulation of electrospray emission processes for low to moderate conductivity liquids. Phys. Fluids 2022, 34, 112017. [Google Scholar] [CrossRef]
- Haynes, W.M. CRC Handbook of Chemistry and Physics; CRC Press: Boca Raton, FL, USA, 2016. [Google Scholar] [CrossRef]
- Deng, H.; Sun, Y.; Liu, X.; Kang, X. Investigations and analysis of current modes and emission properties of emitters with different tip shapes for ionic liquid electrospray thrusters. Acta Astronaut. 2024, 214, 95–109. [Google Scholar] [CrossRef]
- Pumps, N.E. ‘Just Infusion’TM NE-1000 Series of Programmable Syringe Pumps. Available online: www.SyringePump.com (accessed on 24 January 2025).
- Spruce Science. Lab Mate High Voltage Power Supply Operating Manual; Spruce Science: Sunnyvale, CA, USA.
- Sijs, R.; Kooij, S.; Holterman, H.J.; Van De Zande, J.; Bonn, D. Drop size measurement techniques for sprays: Comparison of image analysis, phase Doppler particle analysis, and laser diffraction. AIP Adv. 2021, 11, 015315. [Google Scholar] [CrossRef]
- AOM-Systems. SpraySpy ® LabLine Spray & Droplet Analysis for Research & Development. Available online: https://www.aom-systems.com/en/wp-content/uploads/2023/03/SpraySpy_LabLine_Product_info_2023.pdf (accessed on 24 January 2025).
- ORCA-R2 Digital CCD Camera. Available online: https://spectraservices.com/mm5/graphics/00000001/C10600-10B.pdf (accessed on 24 January 2025).
- Stark, J.P.W.; Alexander, M.S.; Smith, K.L. Electrospray pulsation: A diagnostic to understand cone-jet stability and minimum flow. J. Appl. Phys. 2014, 115, 044905. [Google Scholar] [CrossRef]
- Marginean, I.; Kelly, R.T.; Page, J.S.; Tang, K.; Smith, R.D. Electrospray characteristic curves: In pursuit of improved performance in the nanoflow regime. Anal. Chem. 2007, 79, 8030–8036. [Google Scholar] [CrossRef]
- Ponce-Torres, A.; Rebollo-Munõz, N.; Herrada, M.A.; Ganãn-Calvo, A.M.; Montanero, J.M. The steady cone-jet mode of electrospraying close to the minimum volume stability limit. J. Fluid Mech. 2018, 857, 142–172. [Google Scholar] [CrossRef]
- Huh, H.; Wirz, R.E. Simulation of Electrospray Emission Processes for Highly Conductive Liquids. arXiv 2021, arXiv:2111.10383. [Google Scholar]
- Rosell-Llompart, J.; De, J.F.; Mora, L.A. Generation of monodisperse droplets 0.3 to 4/tm in diameter from electrified cone-jets of highly conducting and viscous liquids. J. Aerosol Sci. 1994, 25, 1093–1119. [Google Scholar] [CrossRef]
- Jordan, J.; Miller, Z.; Harper, C.; Hanozin, E.; Williams, E. Lighting Up at High Potential: Effects of Voltage and Emitter Size in Nanoelectrospray Ionization. J. Am. Soc. Mass Spectrom. 2023, 34, 1186–1195. [Google Scholar] [CrossRef]
- Subbotin, A.V.; Semenov, A.N. Electrohydrodynamics of stationary cone-jet streaming. Proc. R. Soc. A Math. Phys. Eng. Sci. 2015, 471, 20150290. [Google Scholar] [CrossRef]
- Ryan, C.N.; Smith, K.L.; Alexander, M.S.; Stark, J.P.W. Effect of emitter geometry on flow rate sensitivity to voltage in cone jet mode electrospray. J. Phys. D Appl. Phys. 2009, 42, 155504. [Google Scholar] [CrossRef]
- Shui, W.; Yu, Y.; Xu, X.; Huang, Z.; Xu, G.; Yang, P. Micro-electrospray with stainless steel emitters. Rapid. Commun. Mass Spectrom. 2003, 17, 1541–1547. [Google Scholar] [CrossRef]







| Parameters | Values | Voltage |
|---|---|---|
| Flow Rate | Q = 2.5 mL/h | 4.80 kV |
| Q = 5 mL/h | 4.57 kV | |
| Q = 7.5 mL/h | 4.53 kV | |
| Q = 10 mL/h | 4.47 kV | |
| Emitter Diameter | d = 0.6 mm | 3.93 kV |
| d = 1.1 mm | 4.53 kV | |
| d = 2.1 mm | 5.01 kV | |
| Emitter to Collector Distance | L = 10 mm | 3.59 kV |
| L = 20 mm | 4.53 kV | |
| L = 30 mm | 5.64 kV |
| Parameter | Values | Droplet Size | Droplet Velocity |
|---|---|---|---|
| Flow rate | Q = 2.5 mL/h | 8.8 µm | 11.3 m/s |
| Q = 5 mL/h | 8.8 µm | 9.0 m/s | |
| Q = 7.5 mL/h | 9.2 µm | 8.0 m/s | |
| Q = 10 mL/h | 8.0 µm | 7.8 m/s | |
| Emitter diameter | d = 0.6 mm | 3.7 µm | 9.0 m/s |
| d = 1.1 mm | 9.2 µm | 8.0 m/s | |
| d = 2.1 mm | 16.7 µm | 7.5 m/s | |
| Emitter to collector distance | L = 10 mm | 7.3 µm | 8.6 m/s |
| L = 20 mm | 9.2 µm | 8.0 m/s | |
| L = 30 mm | 9.6 µm | 6.2 m/s |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nunes, G.; Moreira, M.; Rodrigues, F.; Páscoa, J. Experimental Analysis of Electrohydrodynamic Jet Actuation Modes Based on the Phase Doppler Technique. Actuators 2025, 14, 141. https://doi.org/10.3390/act14030141
Nunes G, Moreira M, Rodrigues F, Páscoa J. Experimental Analysis of Electrohydrodynamic Jet Actuation Modes Based on the Phase Doppler Technique. Actuators. 2025; 14(3):141. https://doi.org/10.3390/act14030141
Chicago/Turabian StyleNunes, Gustavo, Miguel Moreira, Frederico Rodrigues, and José Páscoa. 2025. "Experimental Analysis of Electrohydrodynamic Jet Actuation Modes Based on the Phase Doppler Technique" Actuators 14, no. 3: 141. https://doi.org/10.3390/act14030141
APA StyleNunes, G., Moreira, M., Rodrigues, F., & Páscoa, J. (2025). Experimental Analysis of Electrohydrodynamic Jet Actuation Modes Based on the Phase Doppler Technique. Actuators, 14(3), 141. https://doi.org/10.3390/act14030141







