Incorporating Intracellular Processes in Virus Dynamics Models
Abstract
:1. Introduction
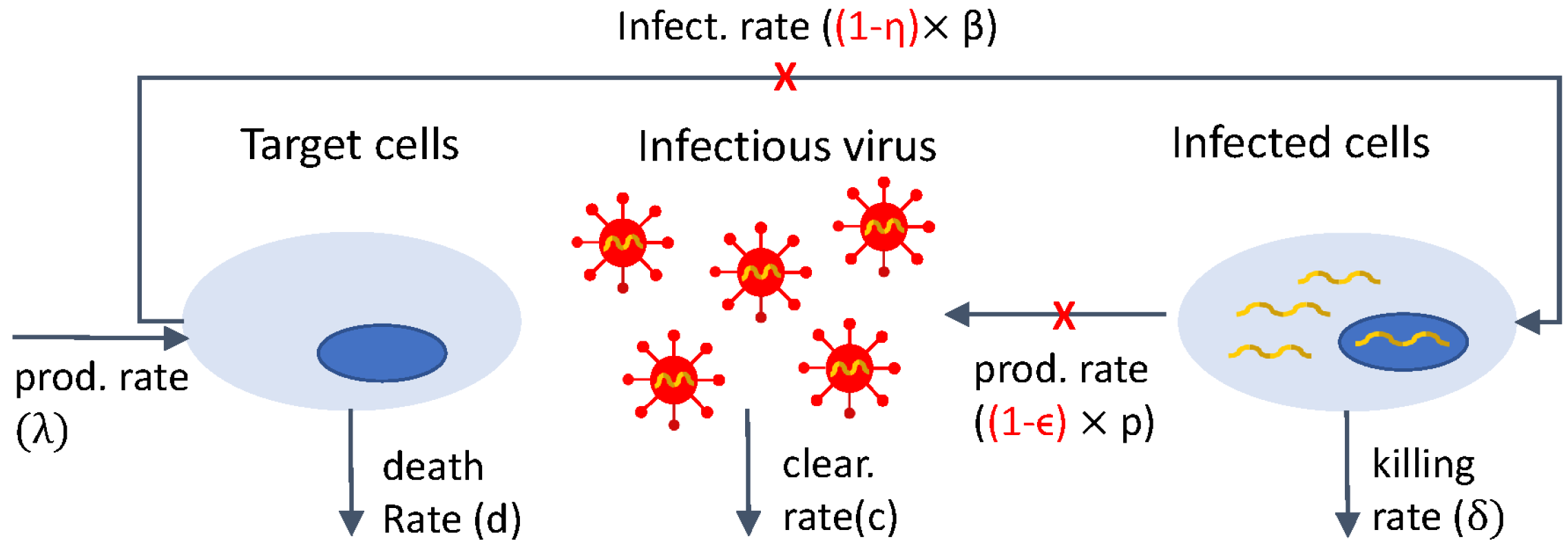
2. Modeling Viral Dynamics: The Standard Model
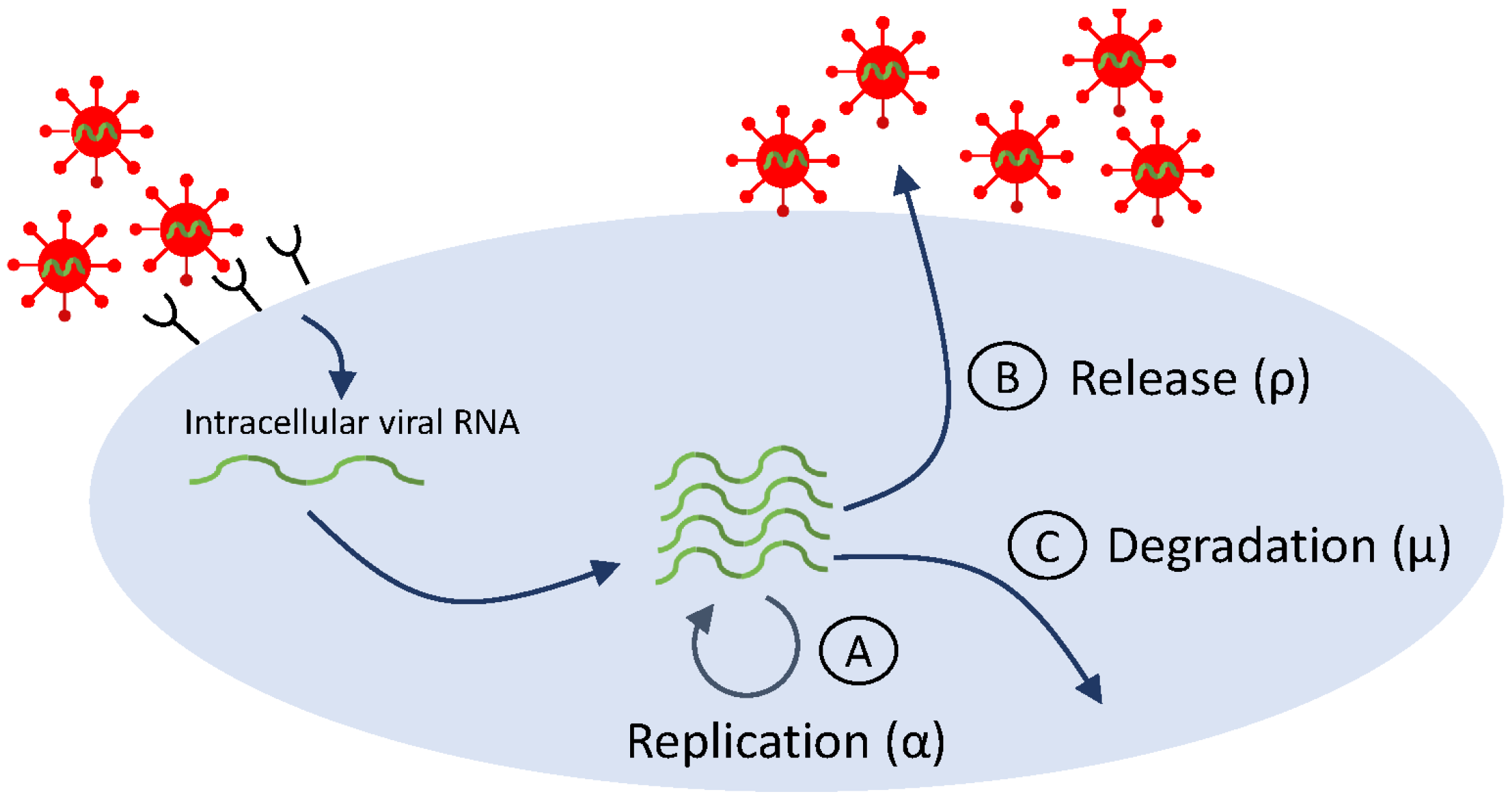
3. Modeling Viral Eclipse Phase
4. Modeling Cellular Coinfection
5. Modeling Viral Life Cycle: A Few Stories
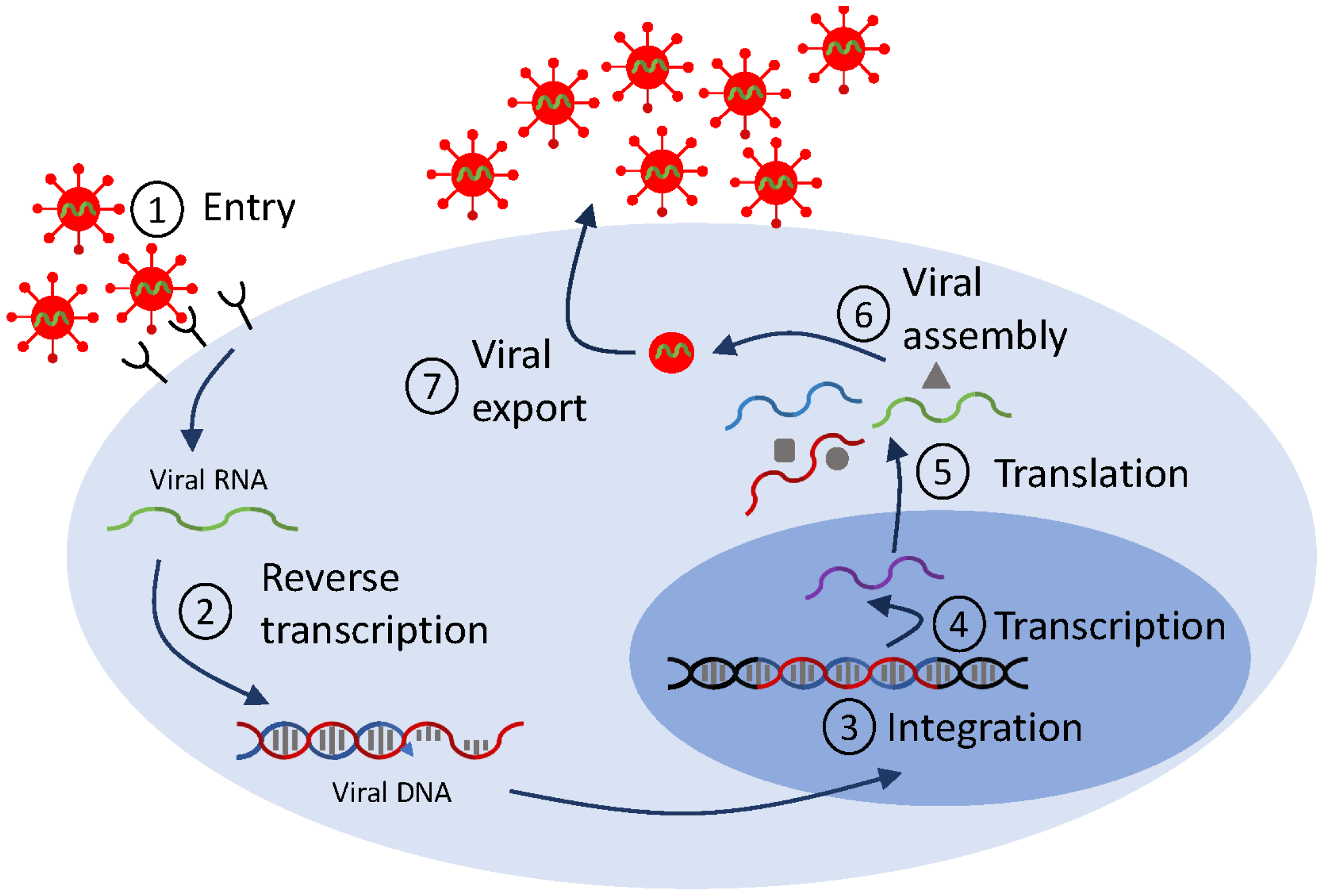
5.1. Intracellular Modeling of HIV Infection and Therapy
5.2. Intracellular Modeling of HCV Infection and Therapy
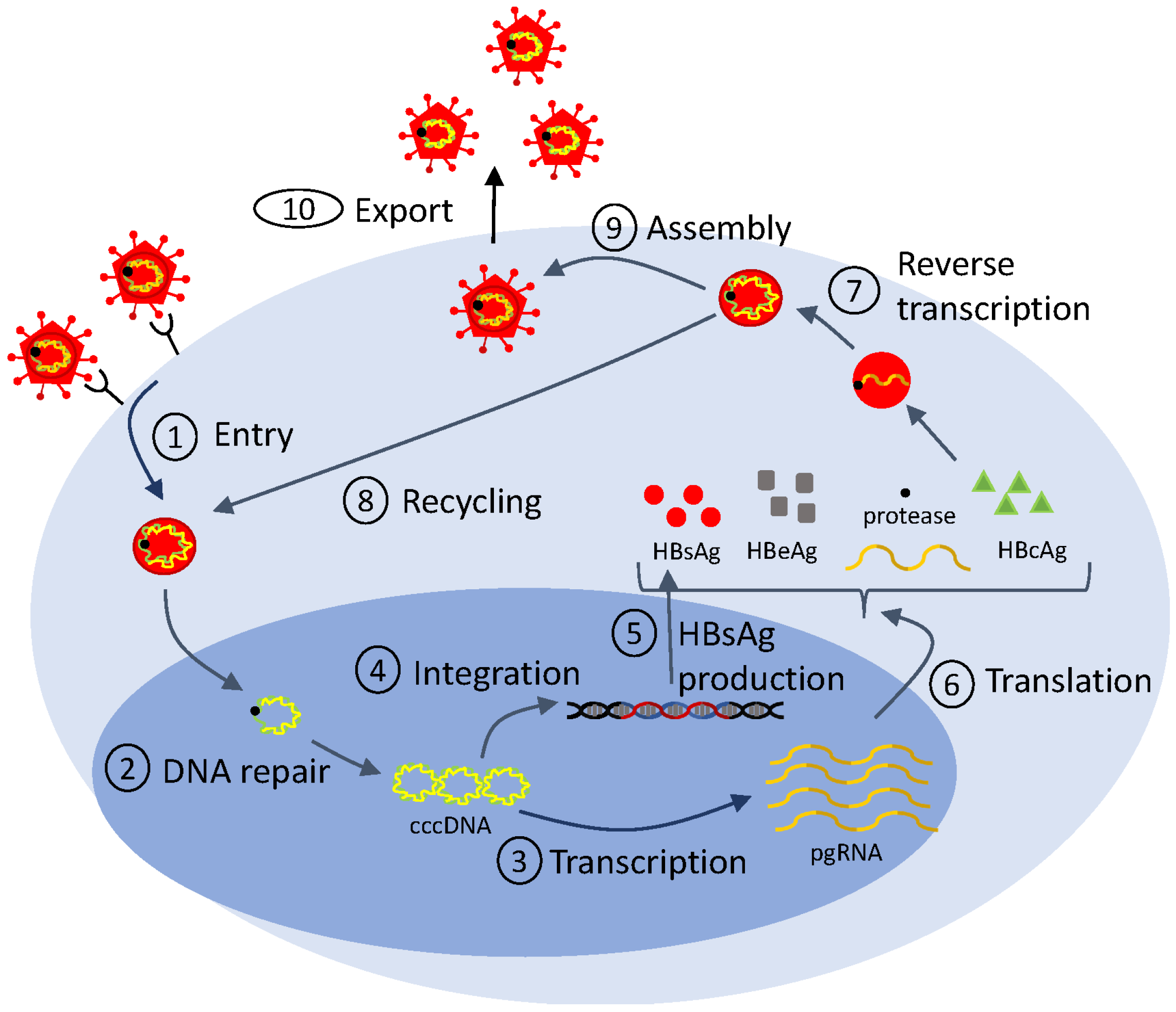
5.3. Intracellular Modeling of HBV Infection and Therapy
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| HIV | human immunodeficiency virus |
| HBV | hepatitis B virus |
| HCV | hepatitis C virus |
| HDV | hepatitis D virus |
| basic reproduction number | |
| MOI | multiplicity of infection |
| HBsAg | hepatitis B s-antigen |
| HBeAg | hepatitis B e-antigen |
| cccDNA | closed covalent circular DNA |
| ART | Antiretroviral therapy |
| PI | Protease inhibitors |
| RT | Reverse transcriptase inhibitors |
| DAA | Direct acting antivirals |
| INF | Interferon |
| MOA | mode of action |
| ODE | ordinary differential equations |
| DDE | delay differential equations |
| PDE | partial differential equations |
References
- Nowak, M.A.; Anderson, R.M.; McLean, A.R.; Wolfs, T.F.W.; Goudsmit, J.; May, R.M. Antigenic Diversity Thresholds and the Development of AIDS. Science 1991, 254, 963–969. [Google Scholar] [CrossRef] [PubMed]
- Nowak, M.A.; May, R.M. Mathematical biology of HIV infections: Antigenic variation and diversity threshold. Math. Biosci. 1991, 106, 1–21. [Google Scholar] [CrossRef] [PubMed]
- de Boer, R.J.; Boerlijst, M.C. Diversity and virulence thresholds in AIDS. Proc. Natl. Acad. Sci. USA 1994, 91, 544–548. [Google Scholar] [CrossRef] [PubMed]
- Essunger, P.; Perelson, A. Modeling HIV Infection of CD4+ T-cell Subpopulations. J. Theor. Biol. 1994, 170, 367–391. [Google Scholar] [CrossRef] [PubMed]
- Perelson, A.S.; Neumann, A.U.; Markowitz, M.; Leonard, J.M.; Ho, D.D. HIV-1 dynamics in vivo: Virion clearance rate, infected cell life-span, and viral generation time. Science 1996, 271, 1582–1586. [Google Scholar] [CrossRef] [PubMed]
- Ciupe, M.; Bivort, B.; Bortz, D.; Nelson, P. Estimating kinetic parameters from HIV primary infection data through the eyes of three different mathematical models. Math. Biosci. 2006, 200, 1–27. [Google Scholar] [CrossRef] [PubMed]
- Rong, L.; Gilchrist, M.A.; Feng, Z.; Perelson, A.S. Modeling within-host HIV-1 dynamics and the evolution of drug resistance: Trade-offs between viral enzyme function and drug susceptibility. J. Theor. Biol. 2007, 247, 804–818. [Google Scholar] [CrossRef] [PubMed]
- Perelson, A.S.; Nelson, P.W. Mathematical analysis of HIV-1 dynamics in vivo. SIAM Rev. 1999, 41, 3–44. [Google Scholar] [CrossRef]
- Hill, A.L. Mathematical models of HIV latency. In HIV-1 Latency; Current Topics in Microbiology and Immunology; Springer International Publishing: Berlin/Heidelberg, Germany, 2018; Volume 417, pp. 131–156. [Google Scholar] [CrossRef]
- Conway, J.M.; Ribeiro, R.M. Modeling the immune response to HIV infection. Curr. Opin. Syst. Biol. 2018, 12, 61–69. [Google Scholar] [CrossRef]
- Hernandez-Vargas, E.A.; Middleton, R.H. Modeling the three stages in HIV infection. J. Theor. Biol. 2013, 320, 33–40. [Google Scholar] [CrossRef] [PubMed]
- Conway, J.M.; Perelson, A.S. Post-treatment control of HIV infection. Proc. Natl. Acad. Sci. USA 2015, 112, 5467–5472. [Google Scholar] [CrossRef] [PubMed]
- Ren, X.; Tian, Y.; Liu, L.; Liu, X. A reaction–diffusion within-host HIV model with cell-to-cell transmission. J. Math. Biol. 2018, 76, 1831–1872. [Google Scholar] [CrossRef]
- Gao, Y.; Wang, J. Threshold dynamics of a delayed nonlocal reaction-diffusion HIV infection model with both cell-free and cell-to-cell transmissions. J. Math. Anal. Appl. 2020, 488, 124047. [Google Scholar] [CrossRef]
- Wu, P.; Zhao, H. Mathematical analysis of an age-structured HIV/AIDS epidemic model with HAART and spatial diffusion. Nonlinear Anal. Real World Appl. 2021, 60, 103289. [Google Scholar] [CrossRef]
- Günerhan, H.; Dutta, H.; Dokuyucu, M.A.; Adel, W. Analysis of a fractional HIV model with Caputo and constant proportional Caputo operators. Chaos Solitons Fractals 2020, 139, 110053. [Google Scholar] [CrossRef]
- Kongson, J.; Thaiprayoon, C.; Sudsutad, W. Analysis of a fractional model for HIV CD4+ T-cells with treatment under generalized Caputo fractional derivative. AIMS Math. 2021, 6, 7285–7304. [Google Scholar] [CrossRef]
- Neumann, A.U.; Lam, N.P.; Dahari, H.; Gretch, D.R.; Wiley, T.E.; Layden, T.J.; Perelson, A.S. Hepatitis C viral dynamics in vivo and the antiviral efficacy of interferon-α therapy. Science 1998, 282, 103–107. [Google Scholar] [CrossRef] [PubMed]
- Dahari, H.; Shudo, E.; Ribeiro, R.M.; Perelson, A.S. Mathematical modeling of HCV infection and treatment. Hepat. C Methods Protoc. 2009, 510, 439–453. [Google Scholar] [CrossRef] [PubMed]
- Conway, J.M.; Perelson, A.S. A hepatitis C virus infection model with time-varying drug effectiveness: Solution and analysis. PLoS Comput. Biol. 2014, 10, e1003769. [Google Scholar] [CrossRef]
- Sadki, M.; Danane, J.; Allali, K. Hepatitis C virus fractional-order model: Mathematical analysis. Model. Earth Syst. Environ. 2023, 9, 1695–1707. [Google Scholar] [CrossRef] [PubMed]
- Ciupe, S.M.; Ribeiro, R.M.; Nelson, P.W.; Perelson, A.S. Modeling the mechanisms of acute hepatitis B virus infection. J. Theor. Biol. 2007, 247, 23–35. [Google Scholar] [CrossRef] [PubMed]
- Lewin, S.R.; Ribeiro, R.M.; Walters, T.; Lau, G.K.; Bowden, S.; Locarnini, S.; Perelson, A.S. Analysis of hepatitis B viral load decline under potent therapy: Complex decay profiles observed. Hepatology 2001, 34, 1012–1020. [Google Scholar] [CrossRef] [PubMed]
- Dahari, H.; Shudo, E.; Ribeiro, R.M.; Perelson, A.S. Modeling complex decay profiles of hepatitis B virus during antiviral therapy. Hepatology 2009, 49, 32–38. [Google Scholar] [CrossRef] [PubMed]
- Murray, J.M.; Purcell, R.H.; Wieland, S.F. The half-life of hepatitis B virions. Hepatology 2006, 44, 1117–1121. [Google Scholar] [CrossRef] [PubMed]
- Ciupe, S.M.; Ribeiro, R.M.; Nelson, P.W.; Dusheiko, G.; Perelson, A.S. The role of cells refractory to productive infection in acute hepatitis B viral dynamics. Proc. Natl. Acad. Sci. USA 2007, 104, 5050–5055. [Google Scholar] [CrossRef] [PubMed]
- Wang, K.; Wang, W. Propagation of HBV with spatial dependence. Math. Biosci. 2007, 210, 78–95. [Google Scholar] [CrossRef]
- Wang, J.; Wu, X.; Kuniya, T. Analysis of a diffusive HBV model with logistic proliferation and non-cytopathic antiviral mechanisms. Commun. Nonlinear Sci. Numer. Simul. 2022, 106, 106110. [Google Scholar] [CrossRef]
- Baccam, P.; Beauchemin, C.; Macken, C.A.; Hayden, F.G.; Perelson, A.S. Kinetics of influenza A virus infection in humans. J. Virol. 2006, 80, 7590–7599. [Google Scholar] [CrossRef]
- Smith, A.M.; Perelson, A.S. Influenza A virus infection kinetics: Quantitative data and models. Wiley Interdiscip. Rev. Syst. Biol. Med. 2011, 3, 429–445. [Google Scholar] [CrossRef]
- Liao, L.E.; Carruthers, J.; Smither, S.J.; Weller, S.A.; Williamson, D.; Laws, T.R.; García-Dorival, I.; Hiscox, J.; Holder, B.P.; Beauchemin, C.A.A.; et al. Quantification of Ebola virus replication kinetics in vitro. PLoS Comput. Biol. 2020, 16, e1008375. [Google Scholar] [CrossRef]
- Goyal, A.; Reeves, D.B.; Cardozo-Ojeda, E.F.; Schiffer, J.T.; Mayer, B.T. Viral load and contact heterogeneity predict SARS-CoV-2 transmission and super-spreading events. eLife 2021, 10, e63537. [Google Scholar] [CrossRef]
- Heitzman-Breen, N.; Ciupe, S.M. Modeling within-host and aerosol dynamics of SARS-CoV-2: The relationship with infectiousness. PLoS Comput. Biol. 2022, 18, e1009997. [Google Scholar] [CrossRef] [PubMed]
- Ciupe, S.M.; Tuncer, N. Identifiability of parameters in mathematical models of SARS-CoV-2 infections in humans. Sci. Rep. 2022, 12, 14637. [Google Scholar] [CrossRef] [PubMed]
- Ke, R.; Zitzmann, C.; Ho, D.D.; Ribeiro, R.M.; Perelson, A.S. In vivo kinetics of SARS-CoV-2 infection and its relationship with a person’s infectiousness. Proc. Natl. Acad. Sci. USA 2021, 118, e2111477118. [Google Scholar] [CrossRef] [PubMed]
- Wang, S.; Pan, Y.; Wang, Q.; Miao, H.; Brown, A.N.; Rong, L. Modeling the viral dynamics of SARS-CoV-2 infection. Math. Biosci. 2020, 328, 108438. [Google Scholar] [CrossRef] [PubMed]
- Hernandez-Vargas, E.A.; Velasco-Hernandez, J.X. In-host mathematical modelling of COVID-19 in humans. Annu. Rev. Control 2020, 50, 448–456. [Google Scholar] [CrossRef] [PubMed]
- Wu, P.; Wang, X.; Feng, Z. Spatial and temporal dynamics of SARS-CoV-2: Modeling, analysis and simulation. Appl. Math. Model. 2023, 113, 220–240. [Google Scholar] [CrossRef] [PubMed]
- Guedj, J.; Dahari, H.; Rong, L.; Sansone, N.D.; Nettles, R.E.; Cotler, S.J.; Layden, T.J.; Uprichard, S.L.; Perelson, A.S. Modeling shows that the NS5A inhibitor daclatasvir has two modes of action and yields a shorter estimate of the hepatitis C virus half-life. Proc. Natl. Acad. Sci. USA 2013, 110, 3991–3996. [Google Scholar] [CrossRef]
- Lynch, D.L.; Pavlova, A.; Fan, Z.; Gumbart, J.C. Understanding virus structure and dynamics through molecular simulations. J. Chem. Theory Comput. 2023, 19, 3025–3036. [Google Scholar] [CrossRef] [PubMed]
- Durmuş, S.; Çakır, T.; Özgür, A.; Guthke, R. A review on computational systems biology of pathogen–host interactions. Front. Microbiol. 2015, 6, 128759. [Google Scholar] [CrossRef] [PubMed]
- Ciupe, S.M.; Heffernan, J.M. In-host modeling. Infect. Dis. Model. 2017, 2, 188–202. [Google Scholar] [CrossRef] [PubMed]
- Heffernan, J.M.; Smith, R.J.; Wahl, L.M. Perspectives on the basic reproductive ratio. J. R. Soc. Interface 2005, 2, 281–293. [Google Scholar] [CrossRef] [PubMed]
- Lloyd, A.L. The dependence of viral parameter estimates on the assumed viral life cycle: Limitations of studies of viral load data. Proc. R. Soc. Lond. Ser. B Biol. Sci. 2001, 268, 847–854. [Google Scholar] [CrossRef]
- Rong, L.; Perelson, A.S. Mathematical analysis of multiscale models for hepatitis C virus dynamics under therapy with direct-acting antiviral agents. Math. Biosci. 2013, 245, 22–30. [Google Scholar] [CrossRef] [PubMed]
- Cardozo, E.F.; Ji, D.; Lau, G.; Schinazi, R.F.; Chen, G.; Ribeiro, R.M.; Perelson, A.S. Disentangling the lifespans of hepatitis C virus-infected cells and intracellular vRNA replication-complexes during direct-acting anti-viral therapy. J. Viral Hepat. 2019, 27, 261–269. [Google Scholar] [CrossRef]
- Pawelek, K.A.; Huynh, G.T.; Quinlivan, M.; Cullinane, A.; Rong, L.; Perelson, A.S. Modeling within-host dynamics of influenza virus infection including immune responses. PLoS Comput. Biol. 2012, 8, e1002588. [Google Scholar] [CrossRef] [PubMed]
- Ben-Shachar, R.; Koelle, K. Minimal within-host dengue models highlight the specific roles of the immune response in primary and secondary dengue infections. J. R. Soc. Interface 2015, 12, 20140886. [Google Scholar] [CrossRef] [PubMed]
- Nikin-Beers, R.; Ciupe, S.M. The role of antibody in enhancing dengue virus infection. Math. Biosci. 2015, 263, 83–92. [Google Scholar] [CrossRef] [PubMed]
- Nikin-Beers, R.; Ciupe, S.M. Modelling original antigenic sin in dengue viral infection. Math. Med. Biol. 2018, 35, 257–272. [Google Scholar] [CrossRef] [PubMed]
- Clapham, H.E.; Tricou, V.; Van Vinh Chau, N.; Simmons, C.P.; Ferguson, N.M. Within-host viral dynamics of dengue serotype 1 infection. J. R. Soc. Interface 2014, 11, 20140094. [Google Scholar] [CrossRef]
- Best, K.; Guedj, J.; Madelain, V.; de Lamballerie, X.; Lim, S.Y.; Osuna, C.E.; Whitney, J.B.; Perelson, A.S. Zika plasma viral dynamics in nonhuman primates provides insights into early infection and antiviral strategies. Proc. Natl. Acad. Sci. USA 2017, 114, 8847–8852. [Google Scholar] [CrossRef] [PubMed]
- Guo, E.; Dobrovolny, H.M. Mathematical Modeling of Oncolytic Virus Therapy Reveals Role of the Immune Response. Viruses 2023, 15, 1812. [Google Scholar] [CrossRef]
- Guedj, J.; Rong, L.; Dahari, H.; Perelson, A.S. A perspective on modelling hepatitis C virus infection. J. Viral Hepat. 2010, 17, 825–833. [Google Scholar] [CrossRef] [PubMed]
- Perelson, A.S.; Ribeiro, R.M. Modeling the within-host dynamics of HIV infection. BMC Biol. 2013, 11, 96. [Google Scholar] [CrossRef] [PubMed]
- Ho, D.D.; Neumann, A.U.; Perelson, A.S.; Chen, W.; Leonard, J.M.; Markowitz, M. Rapid turnover of plasma virions and CD4 lymphocytes in HIV-1 infection. Nature 1995, 373, 123–126. [Google Scholar] [CrossRef] [PubMed]
- Stafford, M.A.; Corey, L.; Cao, Y.; Daar, E.S.; Ho, D.D.; Perelson, A.S. Modeling plasma virus concentration during primary HIV infection. J. Theor. Biol. 2000, 203, 285–301. [Google Scholar] [CrossRef] [PubMed]
- Ribeiro, R.M.; Qin, L.; Chavez, L.L.; Li, D.; Self, S.G.; Perelson, A.S. Estimation of the Initial Viral Growth Rate and Basic Reproductive Number during Acute HIV-1 Infection. J. Virol. 2010, 84, 6096–6102. [Google Scholar] [CrossRef] [PubMed]
- Lord, J.S.; Bonsall, M.B. The evolutionary dynamics of viruses: Virion release strategies, time delays and fitness minima. Virus Evol. 2021, 7, veab039. [Google Scholar] [CrossRef] [PubMed]
- Herz, A.V.; Bonhoeffer, S.; Anderson, R.M.; May, R.M.; Nowak, M.A. Viral dynamics in vivo: Limitations on estimates of intracellular delay and virus decay. Proc. Natl. Acad. Sci. USA 1996, 93, 7247–7251. [Google Scholar] [CrossRef]
- Nelson, P.W.; Mittler, J.E.; Perelson, A.S. Effect of Drug Efficacy and the Eclipse Phase of the Viral Life Cycle on Estimates of HIV Viral Dynamic Parameters. JAIDS J. Acquir. Immune Defic. Syndr. 2001, 26, 405–412. [Google Scholar] [CrossRef] [PubMed]
- Nelson, P.W.; Perelson, A.S. Mathematical analysis of delay differential equation models of HIV-1 infection. Math. Biosci. 2002, 179, 73–94. [Google Scholar] [CrossRef] [PubMed]
- Müller, T.; Dürr, R.; Isken, B.; Schulze-Horsel, J.; Reichl, U.; Kienle, A. Distributed modeling of human influenza A virus-host cell interactions during vaccine production. Biotechnol. Bioeng. 2013, 110, 2252–2266. [Google Scholar] [CrossRef] [PubMed]
- Song, X.; Neumann, A.U. Global stability and periodic solution of the viral dynamics. J. Math. Anal. Appl. 2007, 329, 281–297. [Google Scholar] [CrossRef]
- Li, D.; Ma, W. Asymptotic properties of a HIV-1 infection model with time delay. J. Math. Anal. Appl. 2007, 335, 683–691. [Google Scholar] [CrossRef]
- Huang, G.; Ma, W.; Takeuchi, Y. Global properties for virus dynamics model with Beddington–DeAngelis functional response. Appl. Math. Lett. 2009, 22, 1690–1693. [Google Scholar] [CrossRef]
- Huang, G.; Ma, W.; Takeuchi, Y. Global analysis for delay virus dynamics model with Beddington–DeAngelis functional response. Appl. Math. Lett. 2011, 24, 1199–1203. [Google Scholar] [CrossRef]
- Miao, H.; Teng, Z.; Abdurahman, X. Stability and Hopf bifurcation for a five-dimensional virus infection model with Beddington–DeAngelis incidence and three delays. J. Biol. Dyn. 2017, 12, 146–170. [Google Scholar] [CrossRef] [PubMed]
- Xiang, H.; Feng, L.X.; Huo, H.F. Stability of the virus dynamics model with Beddington–DeAngelis functional response and delays. Appl. Math. Model. 2013, 37, 5414–5423. [Google Scholar] [CrossRef]
- Li, X.; Fu, S. Global stability of a virus dynamics model with intracellular delay and CTL immune response. Math. Methods Appl. Sci. 2014, 38, 420–430. [Google Scholar] [CrossRef]
- Yang, Y.; Xu, Y. Global stability of a diffusive and delayed virus dynamics model with Beddington–DeAngelis incidence function and CTL immune response. Comput. Math. Appl. 2016, 71, 922–930. [Google Scholar] [CrossRef]
- Kang, C.; Miao, H.; Chen, X.; Xu, J.; Huang, D. Global stability of a diffusive and delayed virus dynamics model with Crowley-Martin incidence function and CTL immune response. Adv. Differ. Equ. 2017, 2017, 324. [Google Scholar] [CrossRef]
- Wang, Y.; Lu, M.; Jiang, D. Viral dynamics of a latent HIV infection model with Beddington-DeAngelis incidence function, B-cell immune response and multiple delays. Math. Biosci. Eng. 2021, 18, 274–299. [Google Scholar] [CrossRef]
- Dixit, N.M.; Perelson, A.S. Complex patterns of viral load decay under antiretroviral therapy: Influence of pharmacokinetics and intracellular delay. J. Theor. Biol. 2004, 226, 95–109. [Google Scholar] [CrossRef]
- Dixit, N.M.; Perelson, A.S. HIV dynamics with multiple infections of target cells. Proc. Natl. Acad. Sci. USA 2005, 102, 8198–8203. [Google Scholar] [CrossRef] [PubMed]
- Coffin, J.M. HIV Population Dynamics in Vivo: Implications for Genetic Variation, Pathogenesis, and Therapy. Science 1995, 267, 483–489. [Google Scholar] [CrossRef] [PubMed]
- Eastman, P.S.; Mittler, J.; Kelso, R.; Gee, C.; Boyer, E.; Kolberg, J.; Urdea, M.; Leonard, J.M.; Norbeck, D.W.; Mo, H.; et al. Genotypic Changes in Human Immunodeficiency Virus Type 1 Associated with Loss of Suppression of Plasma Viral RNA Levels in Subjects Treated with Ritonavir (Norvir) monotherapy. J. Virol. 1998, 72, 5154–5164. [Google Scholar] [CrossRef] [PubMed]
- Mittler, J.E.; Sulzer, B.; Neumann, A.U.; Perelson, A.S. Influence of delayed viral production on viral dynamics in HIV-1 infected patients. Math. Biosci. 1998, 152, 143–163. [Google Scholar] [CrossRef] [PubMed]
- Kakizoe, Y.; Nakaoka, S.; Beauchemin, C.A.A.; Morita, S.; Mori, H.; Igarashi, T.; Aihara, K.; Miura, T.; Iwami, S. A method to determine the duration of the eclipse phase for in vitro infection with a highly pathogenic SHIV strain. Sci. Rep. 2015, 5, 10371. [Google Scholar] [CrossRef] [PubMed]
- Patel, K.; Kirkpatrick, C.M.; Nieforth, K.A.; Chanda, S.; Zhang, Q.; McClure, M.; Fry, J.; Symons, J.A.; Blatt, L.M.; Beigelman, L.; et al. Respiratory syncytial virus-A dynamics and the effects of lumicitabine, a nucleoside viral replication inhibitor, in experimentally infected humans. J. Antimicrob. Chemother. 2018, 74, 442–452. [Google Scholar] [CrossRef] [PubMed]
- Xie, X.T.; Yitbarek, A.; Khan, S.U.; Sharif, S.; Poljak, Z.; Greer, A.L. A within-host mathematical model of H9N2 avian influenza infection and type-I interferon response pathways in chickens. J. Theor. Biol. 2020, 499, 110320. [Google Scholar] [CrossRef] [PubMed]
- Shartouny, J.R.; Lee, C.Y.; Delima, G.K.; Lowen, A.C. Beneficial effects of cellular coinfection resolve inefficiency in influenza A virus transcription. PLoS Pathog. 2022, 18, e1010865. [Google Scholar] [CrossRef] [PubMed]
- Mavilia, M.G.; Wu, G.Y. HBV-HCV coinfection: Viral interactions, management, and viral reactivation. J. Clin. Transl. Hepatol. 2018, 6, 296. [Google Scholar] [CrossRef] [PubMed]
- Maya, S.; Hershkovich, L.; Cardozo-Ojeda, E.F.; Shirvani-Dastgerdi, E.; Srinivas, J.; Shektman, L.; Uprichard, S.L.; Berneshawi, A.R.; Cafiero, T.R.; Dahari, H.; et al. Hepatitis delta virus RNA decline post inoculation in human NTCP transgenic mice is biphasic. bioRxiv 2023. [Google Scholar] [CrossRef] [PubMed]
- Negro, F. Hepatitis D virus coinfection and superinfection. Cold Spring Harb. Perspect. Med. 2014, 4, a021550. [Google Scholar] [CrossRef] [PubMed]
- Qesmi, R.; ElSaadany, S.; Heffernan, J.M.; Wu, J. A hepatitis B and C virus model with age since infection that exhibits backward bifurcation. SIAM J. Appl. Math. 2011, 71, 1509–1530. [Google Scholar] [CrossRef]
- Goyal, A.; Murray, J.M. Dynamics of in vivo hepatitis D virus infection. J. Theor. Biol. 2016, 398, 9–19. [Google Scholar] [CrossRef] [PubMed]
- Mhlanga, A.; Zakh, R.; Churkin, A.; Reinharz, V.; Glenn, J.S.; Etzion, O.; Cotler, S.J.; Yurdaydin, C.; Barash, D.; Dahari, H. Modeling the Interplay between HDV and HBV in Chronic HDV/HBV Patients. Mathematics 2022, 10, 3917. [Google Scholar] [CrossRef] [PubMed]
- Sausen, D.G.; Shechter, O.; Bietsch, W.; Shi, Z.; Miller, S.M.; Gallo, E.S.; Dahari, H.; Borenstein, R. Hepatitis B and Hepatitis D Viruses: A Comprehensive update with an immunological focus. Int. J. Mol. Sci. 2022, 23, 15973. [Google Scholar] [CrossRef] [PubMed]
- Packer, A.; Forde, J.; Hews, S.; Kuang, Y. Mathematical models of the interrelated dynamics of hepatitis D and B. Math. Biosci. 2014, 247, 38–46. [Google Scholar] [CrossRef] [PubMed]
- Guedj, J.; Rotman, Y.; Cotler, S.J.; Koh, C.; Schmid, P.; Albrecht, J.; Haynes-Williams, V.; Liang, T.J.; Hoofnagle, J.H.; Heller, T.; et al. Understanding early serum hepatitis D virus and hepatitis B surface antigen kinetics during pegylated interferon-alpha therapy via mathematical modeling. Hepatology 2014, 60, 1902–1910. [Google Scholar] [CrossRef] [PubMed]
- Shekhtman, L.; Cotler, S.J.; Hershkovich, L.; Uprichard, S.L.; Bazinet, M.; Pantea, V.; Cebotarescu, V.; Cojuhari, L.; Jimbei, P.; Krawczyk, A.; et al. Modelling hepatitis D virus RNA and HBsAg dynamics during nucleic acid polymer monotherapy suggest rapid turnover of HBsAg. Sci. Rep. 2020, 10, 7837. [Google Scholar] [CrossRef] [PubMed]
- Koh, C.; Canini, L.; Dahari, H.; Zhao, X.; Uprichard, S.L.; Haynes-Williams, V.; Winters, M.A.; Subramanya, G.; Cooper, S.L.; Pinto, P.; et al. Oral prenylation inhibition with lonafarnib in chronic hepatitis D infection: A proof-of-concept randomised, double-blind, placebo-controlled phase 2A trial. Lancet Infect. Dis. 2015, 15, 1167–1174. [Google Scholar] [CrossRef] [PubMed]
- Jung, A.; Maier, R.; Vartanian, J.P.; Bocharov, G.; Jung, V.; Fischer, U.; Meese, E.; Wain-Hobson, S.; Meyerhans, A. Multiply infected spleen cells in HIV patients. Nature 2002, 418, 144. [Google Scholar] [CrossRef] [PubMed]
- Onafuwa-Nuga, A.; Telesnitsky, A. The Remarkable Frequency of Human Immunodeficiency Virus Type 1 Genetic Recombination. Microbiol. Mol. Biol. Rev. 2009, 73, 451–480. [Google Scholar] [CrossRef] [PubMed]
- Marshall, N.; Priyamvada, L.; Ende, Z.; Steel, J.; Lowen, A.C. Influenza Virus Reassortment Occurs with High Frequency in the Absence of Segment Mismatch. PLoS Pathog. 2013, 9, e1003421. [Google Scholar] [CrossRef] [PubMed]
- Brooke, C.B.; Ince, W.L.; Wei, J.; Bennink, J.R.; Yewdell, J.W. Influenza A virus nucleoprotein selectively decreases neuraminidase gene-segment packaging while enhancing viral fitness and transmissibility. Proc. Natl. Acad. Sci. USA 2014, 111, 16854–16859. [Google Scholar] [CrossRef] [PubMed]
- Fukuyama, S.; Katsura, H.; Zhao, D.; Ozawa, M.; Ando, T.; Shoemaker, J.E.; Ishikawa, I.; Yamada, S.; Neumann, G.; Watanabe, S.; et al. Multi-spectral fluorescent reporter influenza viruses (Color-flu) as powerful tools for in vivo studies. Nat. Commun. 2015, 6, 6600. [Google Scholar] [CrossRef] [PubMed]
- Neher, R.A.; Leitner, T. Recombination rate and selection strength in HIV intra-patient evolution. PLoS Comput. Biol. 2010, 6, e1000660. [Google Scholar] [CrossRef] [PubMed]
- Batorsky, R.; Kearney, M.F.; Palmer, S.E.; Maldarelli, F.; Rouzine, I.M.; Coffin, J.M. Estimate of effective recombination rate and average selection coefficient for HIV in chronic infection. Proc. Natl. Acad. Sci. USA 2011, 108, 5661–5666. [Google Scholar] [CrossRef] [PubMed]
- Martin, B.E.; Harris, J.D.; Sun, J.; Koelle, K.; Brooke, C.B. Cellular co-infection can modulate the efficiency of influenza A virus production and shape the interferon response. PLoS Pathog. 2020, 16, e1008974. [Google Scholar] [CrossRef]
- Saeki, K.; Sasaki, A. Cell-to-cell transmission promotes the emergence of double-drug resistance. Virus Evol. 2023, 9, vead017. [Google Scholar] [CrossRef] [PubMed]
- Althaus, C.L.; Boer, R.J.D. Impaired immune evasion in HIV through intracellular delays and multiple infection of cells. Proc. R. Soc. B Biol. Sci. 2012, 279, 3003–3010. [Google Scholar] [CrossRef] [PubMed]
- Wodarz, D.; Levy, D.N. Multiple HIV-1 infection of cells and the evolutionary dynamics of cytotoxic T lymphocyte escape mutants. Evolution 2009, 63, 2326–2339. [Google Scholar] [CrossRef] [PubMed]
- Wodarz, D.; Levy, D.N. Effect of different modes of viral spread on the dynamics of multiply infected cells in human immunodeficiency virus infection. J. R. Soc. Interface 2010, 8, 289–300. [Google Scholar] [CrossRef] [PubMed]
- Wodarz, D.; Levy, D.N. Effect of multiple infection of cells on the evolutionary dynamics of HIV in vivo: Implications for host adaptation mechanisms. Exp. Biol. Med. 2011, 236, 926–937. [Google Scholar] [CrossRef] [PubMed]
- Phan, D.; Wodarz, D. Modeling multiple infection of cells by viruses: Challenges and insights. Math. Biosci. 2015, 264, 21–28. [Google Scholar] [CrossRef] [PubMed]
- Koelle, K.; Farrell, A.P.; Brooke, C.B.; Ke, R. Within-host infectious disease models accommodating cellular coinfection, with an application to influenza. Virus Evol. 2019, 5, vez018. [Google Scholar] [CrossRef] [PubMed]
- Roberts, M. A pocket guide to host-parasite models. Parasitol. Today 1995, 11, 172–177. [Google Scholar] [CrossRef] [PubMed]
- Zhu, H.; Allman, B.E.; Koelle, K. Fitness estimation for viral variants in the context of cellular coinfection. Viruses 2021, 13, 1216. [Google Scholar] [CrossRef] [PubMed]
- Allman, B.; Koelle, K.; Weissman, D. Heterogeneity in viral populations increases the rate of deleterious mutation accumulation. Genetics 2022, 222, iyac127. [Google Scholar] [CrossRef] [PubMed]
- Mainou, E.; Ribeiro, R.M.; Conway, J. Investigating alternative models of acute HIV infection. bioRXiv 2024. [Google Scholar] [CrossRef]
- Suryawanshi, G.W.; Hoffmann, A. A multi-scale mathematical modeling framework to investigate anti-viral therapeutic opportunities in targeting HIV-1 accessory proteins. J. Theor. Biol. 2015, 386, 89–104. [Google Scholar] [CrossRef] [PubMed]
- Shcherbatova, O.; Grebennikov, D.; Sazonov, I.; Meyerhans, A.; Bocharov, G. Modeling of the HIV-1 life cycle in productively infected cells to predict novel therapeutic targets. Pathogens 2020, 9, 255. [Google Scholar] [CrossRef] [PubMed]
- Food, U.; Administration, D. Drug Approval Package for Isentress (raltegravir) 400 mg Tablets. 2008. Available online: https://www.tga.gov.au/resources/artg/140238 (accessed on 12 April 2024).
- Gilmore, J.B.; Kelleher, A.D.; Cooper, D.A.; Murray, J.M. Explaining the determinants of first phase HIV decay dynamics through the effects of stage-dependent drug action. PLoS Comput. Biol. 2013, 9, e1002971. [Google Scholar] [CrossRef] [PubMed]
- Policicchio, B.B.; Cardozo-Ojeda, E.F.; Xu, C.; Ma, D.; He, T.; Raehtz, K.D.; Sivanandham, R.; Kleinman, A.J.; Perelson, A.S.; Apetrei, C.; et al. CD8+ T cells control SIV infection using both cytolytic effects and non-cytolytic suppression of virus production. Nat. Commun. 2023, 14, 6657. [Google Scholar] [CrossRef]
- Klatt, N.R.; Shudo, E.; Ortiz, A.M.; Engram, J.C.; Paiardini, M.; Lawson, B.; Miller, M.D.; Else, J.; Pandrea, I.; Estes, J.D.; et al. CD8+ lymphocytes control viral replication in SIVmac239-infected rhesus macaques without decreasing the lifespan of productively infected cells. PLoS Pathog. 2010, 6, e1000747. [Google Scholar] [CrossRef] [PubMed]
- Wong, J.K.; Strain, M.C.; Porrata, R.; Reay, E.; Sankaran-Walters, S.; Ignacio, C.C.; Russell, T.; Pillai, S.K.; Looney, D.J.; Dandekar, S. In vivo CD8+ T-cell suppression of SIV viremia is not mediated by CTL clearance of productively infected cells. PLoS Pathog. 2010, 6, e1000748. [Google Scholar] [CrossRef] [PubMed]
- Hosseini, I.; Mac Gabhann, F. APOBEC3G-augmented stem cell therapy to modulate HIV replication: A computational study. PLoS ONE 2013, 8, e63984. [Google Scholar] [CrossRef]
- Hosseini, I.; Mac Gabhann, F. Multi-scale modeling of HIV infection in vitro and APOBEC3G-based anti-retroviral therapy. PLoS Comput. Biol. 2012, 8, e1002371. [Google Scholar] [CrossRef]
- Shcherbatova, O.; Grebennikov, D.; Sazonov, I.; Meyerhans, A.; Bocharov, G. Quantifying the antiviral effect of APOBEC3 on HIV-1 infection in humanized mouse model. J. Theor. Biol. 2020, 498, 110295. [Google Scholar] [CrossRef] [PubMed]
- Althaus, C.L.; Joos, B.; Perelson, A.S.; Günthard, H.F. Quantifying the turnover of transcriptional subclasses of HIV-1-infected cells. PLoS Comput. Biol. 2014, 10, e1003871. [Google Scholar] [CrossRef] [PubMed]
- Althaus, C.L.; Boer, R.J.D. Implications of CTL-mediated killing of HIV-infected cells during the non-productive stage of infection. PLoS ONE 2011, 6, e16468. [Google Scholar] [CrossRef] [PubMed]
- Murillo, L.N.; Murillo, M.S.; Perelson, A.S. Towards multiscale modeling of influenza infection. J. Theor. Biol. 2013, 332, 267–290. [Google Scholar] [CrossRef] [PubMed]
- Conway, J.M.; Konrad, B.P.; Coombs, D. Stochastic analysis of pre- and postexposure prophylaxis against HIV infection. SIAM J. Appl. Math. 2013, 73, 904–928. [Google Scholar] [CrossRef]
- Konrad, B.P.; Taylor, D.; Conway, J.M.; Ogilvie, G.S.; Coombs, D. On the duration of the period between exposure to HIV and detectable infection. Epidemics 2017, 20, 73–83. [Google Scholar] [CrossRef] [PubMed]
- Zitzmann, C.; Kaderali, L. Mathematical analysis of viral replication dynamics and antiviral treatment strategies: From basic models to age-based multi-scale modeling. Front. Microbiol. 2018, 9, 1546. [Google Scholar] [CrossRef]
- Browne, C.J.; Pan, X.; Shu, H.; Wang, X.S. Resonance of periodic combination antiviral therapy and intracellular delays in virus model. Bull. Math. Biol. 2020, 82, 29. [Google Scholar] [CrossRef] [PubMed]
- Dahari, H.; Ribeiro, R.M.; Rice, C.M.; Perelson, A.S. Mathematical modeling of subgenomic hepatitis C virus replication in huh-7 cells. J. Virol. 2007, 81, 750–760. [Google Scholar] [CrossRef] [PubMed]
- Dahari, H.; Sainz, B.; Perelson, A.S.; Uprichard, S.L. Modeling subgenomic hepatitis C virus RNA kinetics during treatment with alpha interferon. J. Virol. 2009, 83, 6383–6390. [Google Scholar] [CrossRef] [PubMed]
- Hoofnagle, J.H. A step forward in therapy for hepatitis C. N. Engl. J. Med. 2009, 360, 1899–1901. [Google Scholar] [CrossRef] [PubMed]
- Fusco, D.N.; Chung, R.T. Novel therapies for hepatitis C: Insights from the structure of the virus. Annu. Rev. Med. 2012, 63, 373–387. [Google Scholar] [CrossRef] [PubMed]
- Collins, L.F.; Chan, A.; Zheng, J.; Chow, S.C.; Wilder, J.M.; Muir, A.J.; Naggie, S. Direct-acting antivirals improve access to care and cure for patients with HIV and chronic HCV infection. Open Forum Infect. Dis. 2018, 5, ofx264. [Google Scholar] [CrossRef] [PubMed]
- Reinharz, V.; Ishida, Y.; Tsuge, M.; Durso-Cain, K.; Chung, T.L.; Tateno, C.; Perelson, A.S.; Uprichard, S.L.; Chayama, K.; Dahari, H. Understanding hepatitis B virus dynamics and the antiviral effect of interferon alpha treatment in humanized chimeric mice. J. Virol. 2021, 95, e0049220. [Google Scholar] [CrossRef] [PubMed]
- Goyal, A.; Chauhan, R. The dynamics of integration, viral suppression and cell-cell transmission in the development of occult Hepatitis B virus infection. J. Theor. Biol. 2018, 455, 269–280. [Google Scholar] [CrossRef] [PubMed]
- Gonçalves, A.; Lemenuel-Diot, A.; Cosson, V.; Jin, Y.; Feng, S.; Bo, Q.; Guedj, J. What drives the dynamics of HBV RNA during treatment? J. Viral Hepat. 2020, 28, 383–392. [Google Scholar] [CrossRef] [PubMed]
- Kadelka, S.; Dahari, H.; Ciupe, S.M. Understanding the antiviral effects of RNAi-based therapy in HBeAg-positive chronic hepatitis B infection. Sci. Rep. 2021, 11, 200. [Google Scholar] [CrossRef] [PubMed]
- Ciupe, S.M.; Dahari, H.; Ploss, A. Mathematical Models of Early Hepatitis B Virus Dynamics in Humanized Mice. Bull. Math. Biol. 2024, 86, 53. [Google Scholar] [CrossRef] [PubMed]
- Murray, J.M.; Goyal, A. In silico single cell dynamics of hepatitis B virus infection and clearance. J. Theor. Biol. 2015, 366, 91–102. [Google Scholar] [CrossRef] [PubMed]
- Ciupe, S.M.; Hews, S. Mathematical models of e-antigen mediated immune tolerance and activation following prenatal HBV infection. PLoS ONE 2012, 7, e39591. [Google Scholar] [CrossRef] [PubMed]
- Kitagawa, K.; Kim, K.S.; Iwamoto, M.; Hayashi, S.; Park, H.; Nishiyama, T.; Nakamura, N.; Fujita, Y.; Nakaoka, S.; Aihara, K.; et al. Multiscale modeling of HBV infection integrating intra-and intercellular viral propagation to analyze extracellular viral markers. PLoS Comput. Biol. 2024, 20, e1011238. [Google Scholar] [CrossRef]
- Carracedo Rodriguez, A.; Chung, M.; Ciupe, S.M. Understanding the complex patterns observed during hepatitis B virus therapy. Viruses 2017, 9, 117. [Google Scholar] [CrossRef] [PubMed]
- Lau, G.K.; Piratvisuth, T.; Luo, K.X.; Marcellin, P.; Thongsawat, S.; Cooksley, G.; Gane, E.; Fried, M.W.; Chow, W.C.; Paik, S.W.; et al. Peginterferon Alfa-2a, lamivudine, and the combination for HBeAg-positive chronic hepatitis B. N. Engl. J. Med. 2005, 352, 2682–2695. [Google Scholar] [CrossRef] [PubMed]
- Fanning, G.C.; Zoulim, F.; Hou, J.; Bertoletti, A. Therapeutic strategies for hepatitis B virus infection: Towards a cure. Nat. Rev. Drug Discov. 2019, 18, 827–844. [Google Scholar] [CrossRef] [PubMed]
- Hershkovich, L.; Shekhtman, L.; Bazinet, M.; Pantea, V.; Placinta, G.; Moscalu, I.; Cotler, S.J.; Vaillant, A.; Dahari, H. Rapid monophasic HBsAg decline during NAP-based therapy predicts functional cure. Hepatology 2021, 74, 514A–515A. [Google Scholar] [CrossRef] [PubMed]
- Wentworth, M.T.; Smith, R.C.; Banks, H.T. Parameter selection and verification techniques based on global sensitivity analysis illustrated for an HIV model. SIAM/ASA J. Uncertain. Quantif. 2016, 4, 266–297. [Google Scholar] [CrossRef]
- Georgescu, P.; Hsieh, Y.H. Global stability for a virus dynamics model with nonlinear incidence of infection and removal. SIAM J. Appl. Math. 2007, 67, 337–353. [Google Scholar] [CrossRef]
- Leber, A.; Abedi, V.; Hontecillas, R.; Viladomiu, M.; Hoops, S.; Ciupe, S.; Caughman, J.; Andrew, T.; Bassaganya-Riera, J. Bistability analyses of CD4+ T follicular helper and regulatory cells during Helicobacter pylori infection. J. Theor. Biol. 2016, 398, 74–84. [Google Scholar] [CrossRef] [PubMed]
- Heitzman-Breen, N.; Liyanage, Y.R.; Duggal, N.; Tuncer, N.; Ciupe, S.M. The effect of model structure and data availability on Usutu virus dynamics at three biological scales. R. Soc. Open Sci. 2024, 11, 231146. [Google Scholar] [CrossRef] [PubMed]
- Nemeth, L.; Tuncer, N.; Martcheva, M. Structural and Practical Identifiability Analysis of a Multiscale Immuno-Epidemiological Model. In Computational and Mathematical Population Dynamics; World Scientific: Singapore, 2023; pp. 169–201. [Google Scholar] [CrossRef]
- Chung, M.; Binois, M.; Gramacy, R.B.; Bardsley, J.M.; Moquin, D.J.; Smith, A.P.; Smith, A.M. Parameter and uncertainty estimation for dynamical systems using surrogate stochastic processes. SIAM J. Sci. Comput. 2019, 41, A2212–A2238. [Google Scholar] [CrossRef] [PubMed]
- Korobeinikov, A.; Archibasov, A.; Sobolev, V. Order reduction for an RNA virus evolution model. Math. Biosci. Eng. 2015, 12, 1007–1016. [Google Scholar] [CrossRef] [PubMed]
- Snowden, T.J.; van der Graaf, P.H.; Tindall, M.J. Methods of model reduction for large-scale biological systems: A survey of current methods and trends. Bull. Math. Biol. 2017, 79, 1449–1486. [Google Scholar] [CrossRef] [PubMed]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ciupe, S.M.; Conway, J.M. Incorporating Intracellular Processes in Virus Dynamics Models. Microorganisms 2024, 12, 900. https://doi.org/10.3390/microorganisms12050900
Ciupe SM, Conway JM. Incorporating Intracellular Processes in Virus Dynamics Models. Microorganisms. 2024; 12(5):900. https://doi.org/10.3390/microorganisms12050900
Chicago/Turabian StyleCiupe, Stanca M., and Jessica M. Conway. 2024. "Incorporating Intracellular Processes in Virus Dynamics Models" Microorganisms 12, no. 5: 900. https://doi.org/10.3390/microorganisms12050900
APA StyleCiupe, S. M., & Conway, J. M. (2024). Incorporating Intracellular Processes in Virus Dynamics Models. Microorganisms, 12(5), 900. https://doi.org/10.3390/microorganisms12050900







