Next Article in Journal
Physiological Response of Corynebacterium glutamicum to Indole
Previous Article in Journal
Burkholderia gladioli CGB10: A Novel Strain Biocontrolling the Sugarcane Smut Disease
Previous Article in Special Issue
Prophages and Past Prophage-Host Interactions Revealed by CRISPR Spacer Content in a Fish Pathogen
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Microorganisms
Volume 8
Issue 12
10.3390/microorganisms8121944
microorganisms-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles thumb_up
...
Endorse textsms
...
Comment
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

The Missing Tailed Phages: Prediction of Small Capsid Candidates

by
Antoni Luque
1,2,3,*,
Sean Benler
4,
Diana Y. Lee
1,2,
Colin Brown
1,5 and
Simon White
6
1
Viral Information Institute, San Diego State University, San Diego, CA 92182, USA
2
Computational Science Research Center, San Diego State University, San Diego, CA 92182, USA
3
Department of Mathematics and Statistics, San Diego State University, San Diego, CA 92182, USA
4
National Center for Biotechnology Information (NCBI), Bethesda, MD 20894, USA
5
Department of Physics, San Diego State University, San Diego, CA 92182, USA
6
Department of Molecular and Cell Biology, University of Connecticut, Storrs, CT 06269, USA
*
Author to whom correspondence should be addressed.
Microorganisms 2020, 8(12), 1944; https://doi.org/10.3390/microorganisms8121944
Submission received: 29 October 2020 / Revised: 4 December 2020 / Accepted: 5 December 2020 / Published: 8 December 2020
(This article belongs to the Special Issue Understanding Phage Particles)
Browse Figures
Review Reports Versions Notes

Abstract

:
Tailed phages are the most abundant and diverse group of viruses on the planet. Yet, the smallest tailed phages display relatively complex capsids and large genomes compared to other viruses. The lack of tailed phages forming the common icosahedral capsid architectures T = 1 and T = 3 is puzzling. Here, we extracted geometrical features from high-resolution tailed phage capsid reconstructions and built a statistical model based on physical principles to predict the capsid diameter and genome length of the missing small-tailed phage capsids. We applied the model to 3348 isolated tailed phage genomes and 1496 gut metagenome-assembled tailed phage genomes. Four isolated tailed phages were predicted to form T = 3 icosahedral capsids, and twenty-one metagenome-assembled tailed phages were predicted to form T < 3 capsids. The smallest capsid predicted was a T = 4/3 ≈ 1.33 architecture. No tailed phages were predicted to form the smallest icosahedral architecture, T = 1. We discuss the feasibility of the missing T = 1 tailed phage capsids and the implications of isolating and characterizing small-tailed phages for viral evolution and phage therapy.

1. Introduction

Tailed phages are viruses that infect bacteria and are the most abundant biological entity on Earth [1]. They are responsible for the regulation of biogeochemical processes at a planetary scale [2,3], the control of microbial populations [4,5], and the mobility of genes across hosts and ecosystems [6,7]. The broad functionality of tailed phages is facilitated by their vast reservoir of genes, resulting in a large range of genome lengths, from 10 to 500 kilobase pairs (kbp) [8,9]. Tailed phages can accommodate these genomes because the proteins building their protective capsids display a high degree of plasticity, allowing for a broad range of different size capsids, from about 30 to 160 nm in diameter [10]. The majority of tailed phages, about 80–90%, form capsids with icosahedral symmetry [11], while the remaining 10–20% form elongated capsids with icosahedral caps [12,13]. The formation of icosahedral capsids is not unique to tailed phages—it is the most common viral capsid architecture in the virosphere [14]. However, despite the abundance and diversity of tailed phages, even the smallest tailed phages form far more complex capsids and store far larger genomes than most icosahedral viral families [15]. Furthermore, the major capsid proteins of tailed phages adopt the HK97-fold, which is also found in the building blocks of bacterial and archeal enzymatic cellular nanocompartments called encapsulins [16,17]. Intriguingly, encapsulins can form the smallest icosahedral protein shells that are absent among tailed phages (Figure 1).
Icosahedral capsids are characterized by the triangulation number T, which determines the number of quasi-equivalent proteins (complexity) and the total number of proteins forming the capsid [18,19]. As illustrated in Figure 2, icosahedral capsids can organize their proteins in four different lattices, accommodating different stoichiometries of major and minor capsid proteins [19]. The generalized T-number is Ti(h,k) = αi T0(h,k), where h and k are the steps in the hexagonal sublattice joining two consecutive vertices in the icosahedral capsid. T0 is the classic T-number associated with the hexagonal lattice and is given by the equation T0(h,k) = h2 + hk + k2. The subindex i takes the values h, t, s, and r, associated, respectively, with the hexagonal, trihexagonal, snub hexagonal, and rhombitrihexagonal icosahedral lattices. The number of major capsid proteins in a capsid is 60T0(h,k). The hexagonal lattice case only contains major capsid proteins, that is, Th(h,k) = T0(h,k), with αh = 1. If the size of the major capsid protein is conserved across lattices, the other three lattices must include minor capsid proteins occupying the secondary polygons (triangles and squares). This increases the surface of the capsid by a factor αt = 4/3 ≈ 1.33 (trihexagonal), αs = 7/3 ≈ 2.33 (snub hexagonal), and αr = 4/3 + 2/ 3 ≈ 2.49 (rhombitrihexagonal). When combining all lattices, the first four elements of the generalized T-number are T = 1, 1.33, 2.33, and 2.49, containing 60 major capsid proteins each. The fifth element of the series is T = 3 and contains 180 major capsid proteins. Tailed phages have been observed to form capsids adopting the hexagonal and trihexagonal lattices [19,20], but no tailed phages have been observed to form T ≤ 3 capsids (Figure 1). The smallest characterized tailed phage structure corresponds to the Bacillus phage phi29, which adopts an elongated structure with icosahedral T = 3 caps and a Q = 5 body [12,21,22], and Streptococcus phage C1, which adopts a T = 4 icosahedral capsid [10,23].
There are several interrelated scientific observations that make the lack of small icosahedral capsids striking among tailed phages. First, T = 1 and T = 3 icosahedral capsid architectures are optimal thermodynamic configurations for protein shells and are the most kinetically favorable icosahedral capsids [13,24,25,26,27]—they are the most common architectures imaged among viruses [15]. Second, tailed phages belong to the Duplodnaviria viral realm, which also includes archaeal and eukaryotic viruses [28,29]. These viruses are characterized by building their capsids with a major capsid protein adopting the highly conserved HK97-fold, and packing their genome in the form of double-stranded DNA at quasi-crystalline densities (Figure 1). The structural elements of this realm appear to have emerged prior to the Last Universal Cellular Ancestor [30]. Modern tailed phages forming T = 4 and larger capsid shells are expected to have evolved from precursor capsids that used T = 1 and T = 3 architectures. Third, encapsulins organize the HK97-fold in T = 1, T = 3, and T = 4 architectures, showing that the HK97-fold is capable of forming small icosahedral capsids [16,17] (Figure 1). Finally, non-tailed icosahedral phages in the Microviridae family adopt T = 1 capsids, storing a small genome capable of executing a lytic replication strategy analogous to tailed phages [31,32]. This suggests that tailed phages should be able to store the instructions for their lytic lifestyle in T = 1 capsids.
This body of evidence indicates that T = 1 and T = 3 should exist among tailed phages. Our working hypothesis is that these small-tailed phages have not been isolated because they are relatively low in abundance in most environments sampled. Larger tailed phage capsids capable of storing longer genomes may have been favored by incorporating genes that can alter the host’s physiology, protect the bacterial host against other phages, and overcome the host’s resistance mechanisms [6,33,34,35,36]. Here, we applied modeling and bioinformatics to narrow the search for small-tailed phages, overcoming the current limitations of sampling. As a proof of concept, we analyzed the capsid architecture and genome size of twenty-three tailed phages that have had high-resolution structures of their capsid determined. We used an allometric model based on conserved structural characteristics of tailed phages and trained the model to infer the genome size and capsid diameter size of tailed phages as a function of the T-number icosahedral architecture. The predictions of the model were compared with more than 3348 isolated phage genomes and 1496 gut metagenome-assembled tailed phage genomes. Four isolated tailed phages and twenty-one metagenome-assembled tailed phages were predicted to form, respectively, T = 3 and T < 3 icosahedral capsids’ architectures, currently missing among tailed phages.

2. Materials and Methods

Structural features of tailed phage capsids: High-resolution tailed phage structures were obtained from twenty-three cryo-EM maps [23,37,38,39,40,41,42,43,44,45,46,47,48,49,50,51,52,53,54,55,56,57,58]. UCSF Chimera software was used to visualize the structures and measure the geometrical properties of the capsids [59]. The icosahedral tool was fitted manually to the internal and external surface of the capsids by adjusting the radius and sphericity parameters. Error measurements were assigned by comparing the outputs at one discrete step below and above the final measurement. The direct measurements were the internal radius, sphericity, surface, and volume, as well as external radius, sphericity, surface, and volume. The shell thickness was estimated by subtracting the internal radius from the external radius. The number of major capsid proteins was calculated using the icosahedral T-number associated with each capsid [10,18,19]. The internal and external major capsid protein surfaces were obtained by dividing the fraction of internal and external surfaces associated with major capsid proteins with respect to the total number of major capsid proteins. The genome density was estimated by dividing the genome size by the internal volume. The Electron Microscopy Database ID (EMDB) and measurements obtained for each tailed phage are provided in Supplementary Data File S1. The correlation with the capsid diameter of all variables measured was evaluated as a function of the interior and external capsid diameters using the non-parametric Spearman coefficient correlation and a two-tailed test. The normality of the variables that were not correlated with capsid diameter was assessed using the Shapiro–Wilk test.
Statistical model: Allometric models were used to relate the genome length (G) and the capsid diameter (D) as a function of the capsid architecture, T-number. To reduce the bias associated with the oversampling of some T architectures, such as T = 7 capsids, the model was built using the average genome lengths and capsid diameters for each T-number. The models were, respectively, G ( T ) = a G T b G and D ( T ) = a D T b D . Each model had two parameters: the pre-factor a i and the allometric exponent b i . The subindex i took the values G for the genome length model and D for the capsid diameter model. The variables were logged on base 10 to obtain the best fit using a linear regression least-squares approach for the parameters log ( a i ) and b i . The residuals were analyzed to evaluate the accuracy of the standard errors and confidence intervals extracted from the linear regression analysis, which relied on the standard assumptions of linearity, normality, homoscedasticity, and low leverage [60,61]. The linear statistical analysis and predictions were performed using the lm and predict functions in the statistical computing language R [62].
Three-dimensional (3D) icosahedral capsid models: Small capsid 3D model architectures with T-number T ≤ 4 were generated for hexagonal (h), trihexagonal (t), snub hexagonal (s), and rhombitrihexagonal (r) icosahedral lattices. These Archimedean lattices minimize the structural information necessary for building icosahedral capsids and are the basis for the generalized theory of icosahedral capsids [19]. The 3D geometrical models were generated computationally using the new hkcage tool in ChimeraX, developed by co-authors C. Brown and A. Luque and available in https://github.com/luquelab/hkcage.git. This new tool can display any element of the infinite series of icosahedral capsids for the four fundamental lattices and their associated dual Laves lattices.
Predicted capsid architectures among tailed phage isolates: The genomes of isolated tailed phages were obtained from the NCBI Reference Sequence Database [63]. The genome information was filtered by the viral realm (Duplodnaviria) and host (bacteria). The database was accessed in October 2020 and downloaded in table format. For 95% of the tailed phages, the NCBI id and genome length were extracted using a Unix shell script. The remaining 5% of tailed phages had information that was not standardized with the NCBI format and was extracted manually. The final file analyzed included the NCBI identifiers and genome lengths (Supplementary Data File S2). The probability density distribution of the genome lengths was obtained using Gaussian kernels with the default bandwidth for the density function in the statistical computing language R [62]. The predicted icosahedral architecture for each tailed phage was obtained by identifying the nearest average genome length obtained in the statistical T-number model described above. A frequency analysis of the predicted T-numbers was obtained. The tailed phages associated with architectures T ≤ 4 were filtered and analyzed in depth. The phage genomes associated to larger capsids were not analyzed in detail.
Predicted structures of metagenome-assembled tailed phages: The genomes of uncultured human gut tailed phages were obtained from 5742 whole-community human gut metagenomes studied by Benler et al. in Reference [64] and available in the NCBI repository: https://ftp.ncbi.nih.gov/pub/yutinn/benler_2020/gut_phages/. Only ‘circular’ contigs with relatively long direct repeats (50–200 bp) at their termini were searched for open reading frames (ORFs). The contigs were also required to include a known phage marker profile, that is, the terminase large subunit, major capsid protein, or portal protein. The contig identifier and genome length of the selected gut metagenome-assembled tailed phages are provided in Supplementary Data File S3. The analysis of the distribution of complete circular genomes and predicted T-number architectures was conducted following the same protocol described above for isolated tailed phage genomes. The tailed phage genomes associated with predicted architectures T ≤ 3 were annotated using the same procedure described in Reference [64]. The phage genomes associated to larger capsids were not analyzed in detail.

3. Results

3.1. Structural Properties of Characterized Tailed Phage Capsids

The range of structural properties obtained from the twenty-three capsids studied is summarized in Table 1. The T-number ranged from 4 to 27, containing 240 to 1620 major capsid proteins, although 5 of those major capsid proteins were replaced by portal proteins in the virion. The external capsid surface was relatively angular, with sphericity ranging from 0.14 to 0.55. The interior capsid surfaces were more spherical, with sphericity ranging from 0.25 to 0.75. The capsid sphericity was negatively correlated with capsid diameter (rho = −0.68, p-value < 0.001). The largest capsid had a diameter three times bigger than the smallest capsid (143 versus 49 nm). The capsid thickness ranged from 3 to 8 nm and was positively correlated with capsid diameter (rho = 0.66, p-value = 0.0010). The largest interior capsid volume was about 25 times larger than the smallest one (3.36 × 104 to 8.22 × 105 nm3 range). The largest genome (280 kbp) was about 16 times larger than the smallest one (17 kbp). The average genome packing density within the capsid was approximately 0.5 bp/nm3, ranging from 0.34 to 0.62 bp/nm3. The values for the interior and exterior capsid surface areas spanned 8.5-fold (5.08 × 103–4.32 × 104 nm2) and 8-fold (6.55 × 103–5.19 × 104 nm2) respectively, with the exterior capsid surface area being 15% to 43% larger than the interior area. The average surface area for each major capsid protein in the interior and exterior parts of the capsid was approximately 23 nm2 (19 to 26 nm2) and 30 nm2 (24 to 35 nm2) per major capsid protein, respectively. The genome density (rho = −0.16, p-value = 0.47) and major capsid exterior surface area (rho = 0.38, p-value = 0.082) were the only variables that did not display a significant correlation with capsid size (see Supplementary Table S1).

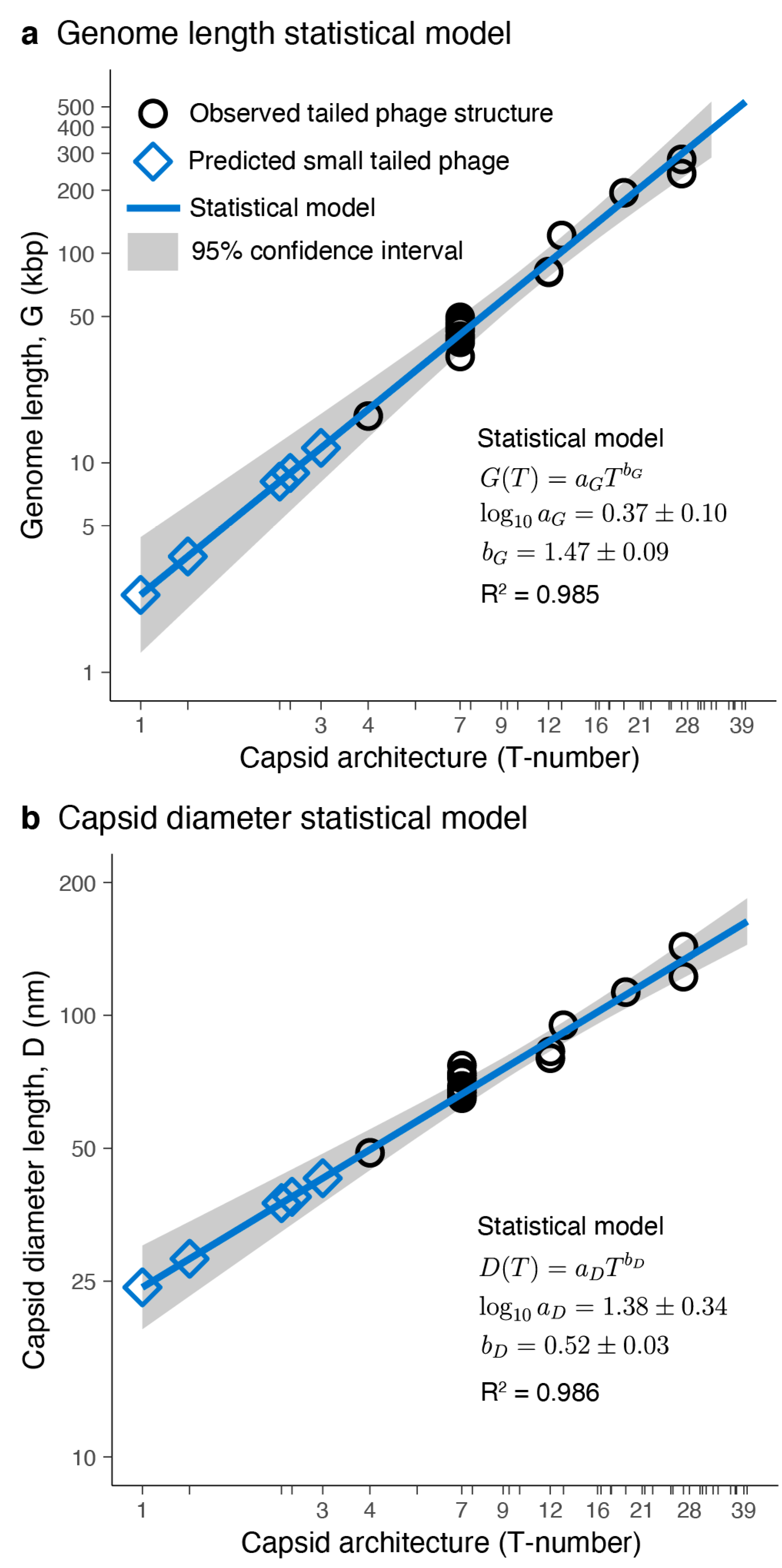
3.2. Tailed Phage Capsids: Statistical Models and Predictions

The genome lengths and capsid diameters obtained from the high-resolution phage structures were used to build a statistical model relating the icosahedral capsid architecture with these two accessible quantities. The allometric model for the average genome length, G, as a function of the architecture index, T, explained 98.5% (R2 = 0.985, n = 7) of the variance (Figure 3a). The pre-factor was log a G = 0.37 ± 0.10 (S.E.) with a G in kbp units. The allometric exponent was b G = 1.47 ± 0.09 . The coefficient of variation (CV = S.E./mean) for the intercept was 27%, significantly larger than the CV associated with the power exponent, 6.1%. Similarly, the allometric model for the average capsid diameter, D, as a function of the architecture index, T, explained 98.6% (R2 = 0.986, n = 7) of the variance (Figure 3b). The pre-factor was log a D = 1.38 ± 0.34 (S.E.) with a D in nm units and coefficient of variation (CV) of 25%. The allometric exponent was b D = 0.52 ± 0.03 with a CV of 5.8%. The qualitative diagnostic of the residuals was similar for both models, consistent with the standard assumptions associated with the statistics of the linear regression analysis (Supplementary Figures S1 and S2). The residuals were scattered around zero with a near-normal distribution and a standardized range on the order of ±1 with relatively homoscedastic variance and relatively low leverage.
The allometric exponents obtained in the statistical models were compared with theoretical scaling relationships. The capsid structural analysis revealed that the genome density was constant. This implied that the genome length (G) was proportional to the capsid volume (V). The volume of a quasi-spherical capsid depends on the third power of the diameter (D), leading to the scaling G ~ D3 between the genome length and capsid diameter. The T-number, by definition, is proportional to the capsid surface, and the units are implicitly related to the major capsid protein surface [19,65]. The exposed surface of the major capsid protein obtained in the structural analysis was constant. Since the capsid surface of a quasi-spherical shell depends on the square of the diameter, this leads to the scaling T ~ D2 relating to the T-number and the capsid diameter. The scaling relationships derived led to the allometric relationships G ~ T3/2 and D ~ T1/2. The theoretical exponent for the genome length versus capsid architecture relationship was b G t h = 3 / 2 = 1.5 , which was within the empirical range obtained in the statistical model, b G = 1.47 ± 0.09 (Figure 3a). The theoretical exponent for the capsid diameter versus capsid architecture yielded b D t h = 1 / 2 = 0.5 , which was also within the empirical range obtained in the statistical model b D = 0.52 ± 0.03 (Figure 3b). The agreement between the statistical model and the theoretical allometric exponents provides confidence in using the statistical model to predict the properties of capsids for T-number architectures outside the range used to train the statistical model.
The average genome length predicted for small-tailed phage architectures was 2.34 kbp (T = 1), 3.57 kbp (T ≈ 1.33), 8.14 kbp (T ≈ 2.33), 8.94 kbp (T ≈ 2.49), 11.78 kbp (T = 3), and 17.98 kbp (T = 4). The capsid architectures and 95% confidence intervals for the genomes are shown in Figure 4. The average capsid diameter predicted for these architectures was 24.20 nm (T = 1), 28.09 nm (T ≈ 1.33), 37.54 nm (T ≈ 2.33), 38.81 nm (T ≈ 2.49), 42.76 nm (T = 3), and 49.63 nm (T = 4). The 95% confidence intervals are also displayed in Figure 4. The confidence intervals for the predicted genome lengths and capsid diameters of these small capsids were relatively large and overlapped. This was a consequence of the relatively large coefficient of variation of the intercepts in statistical models due to the limited number of different T-number capsid architectures used to generate the models (n = 7). The 95% confidence interval ranges for the genome lengths and capsid diameters associated with each T-number (T < 40) are provided, respectively, in Supplementary Data Files S4 and S5.

3.3. Putative Small-Tailed Phage Candidates

3.3.1. Predictions from Isolated Tailed Phages

The genome lengths of 3348 isolated tailed phages were investigated. The distribution of genome lengths was multimodal (Figure 5a). The most dominant peak was at 42.0 kbp. The second dominant peak was among the largest genomes with a length of 158.9 kbp. The shortest genomes displayed a minor peak at 18.3 kbp. The minimum genome length was 11.6 kbp, while the maximum was 497.5 kbp. The median genome length was 50.3 kbp, while the mean was 72.7 kbp. The associated icosahedral structures predicted ranged from T = 3 to T = 39 (Figure 5b). The median architecture was at T ≈ 7.46, and the mean architecture value was T ≈ 9.95. The three genome length peaks were associated with predicted T ~ 4-like structures (18.3 kbp peak), T ~ 7-like structures (42.0 kbp peak), and T ~ 16–19-like structures (158.9 kbp peak). Among the smallest tailed phages, seventy-seven were predicted to form T = 4 architectures, and six were initially predicted to form T = 3 architectures. The identifiers for the isolated tailed phages predicted to form T ≤ 4 capsid architectures are provided in Supplementary Data File S6. The T-number predictions for the full set of isolated tailed phages are provided in Supplementary Data File S7.

3.3.2. Predictions from Metagenome-Assembled Tailed Phages

The genome lengths of 1496 metagenome-assembled tailed phages obtained from gut metagenomes were investigated. The distribution of genome lengths was also multimodal (Figure 5c). The most dominant peak was at 42.9 kbp, similar to the dominant peak among isolated genomes. The second dominant peak was at 98.2 kbp, which was absent in the isolated genomes. The third dominant peak was among the largest genomes with a length of 160.8 kbp, similar to the isolated genomes. The shortest genomes displayed a minor peak at 12.6 kbp, which was slightly shorter in length compared to the analogous peak among isolated genomes. The minimum genome length was 4.5 kbp, while the maximum was 294.5 kbp. The median genome length was 44.8 kbp, while the mean was 55.8 kbp. The associated icosahedral structures predicted ranged from T ≈ 1.33 to T = 27 (Figure 5d). The median architecture was at T ≈ 7.46, and the mean architecture value was T ≈ 8.34. The four genome length peaks were associated with predicted T ~ 3-like structures (12.6 kbp peak), T ~ 7-like structures (42.9 kbp peak), T ~ 12–13-like structures (98.2 kbp peak), and T ~ 16–19-like structures (160.8 kbp peak). Among the smallest tailed phages, two were predicted to form T ≈ 1.33 architectures, nine were predicted to form T ≈ 2.33 architectures, ten were predicted to form T ≈ 2.49 architectures, forty-three were predicted to form T = 3 architectures, and forty-one were predicted to form T = 4 architectures. The identifiers for the metagenome-assembled tailed phages predicted to form T ≤ 4 capsid architectures are provided in Supplementary Data File S8. The T-number predictions for the full set of metagenome-assembled tailed phages are provided in Supplementary Data File S9.

3.3.3. Small-Tailed Phage Capsid Candidates

Six entries in the isolated tailed phage database were predicted to form T = 3 capsid architectures. This was the smallest architecture predicted for this group. However, only four out of those six were kept in the pool of predicted T = 3 phage capsids after further scrutiny. Enterobacteria phage P4 (NC_001609 and genome length 11.6 kbp) was discarded because it is a satellite phage that relies on the infection and genes of phage P2 to produce particles, forming a larger capsid than predicted, T = 4 [66]. Enterobacteria phage BF23 was discarded because the entry identified in NCBI (NC_042564) is a partial genome encompassing only the tRNA gene region (gene length 14.5 kbp). The complete genome was not deposited, but the phage is T5-like, encoding a 100–120 kbp genome. The remaining four were complete phage genomes and infected hosts from four different phyla (Figure 6a). Salmonella phage astrithr (11.6 kbp, NC_48862) was a Podoviridae infecting Salmonella enterica in the phylum Proteobacteria. Rhodococcus phage RRH1 (14.3 kbp, NC_016651) was a Siphoviridae infecting Rhodococcus rhodochrous in the phylum Firmicutes. The two more distant phages were Lactococcus phage bIL311 (14.5 kbp, NC_002670), a Siphoviridae infecting Lactococcus lactic in the phylum Actinobacteria, and Mycoplasma virus P1 (11.7 kbp, NC_002515), a Podoviridae infecting Mycoplasma pulmonis in the phylum Tenericutes.
Twenty-one metagenome-assembled tailed phages analyzed were predicted to form T < 3 capsid architectures. No candidates, however, were predicted to form the smallest icosahedral architecture, T = 1 (Figure 6b). The 3D capsid models and labels of the contigs associated with T ≈ 1.33 (two contigs), T ≈ 2.33 (nine contigs), and T ≈ 2.49 (ten contigs) are displayed in Figure 6b. The genome architectures were diverse (Supplementary Figure S3). The genomes associated with T ≈ 1.33 did not encode major capsid proteins. The smallest genome containing a major capsid protein was OLNE01004159.1, which had a genome length of 7.4 kbp and a predicted architecture of T ≈ 2.33 (Figure 6c).

4. Discussion

Our hypothesis was that tailed phages adopting small icosahedral structures do exist, but their low abundance in the environment has precluded isolating them to be characterized in high-resolution capsid reconstruction studies. The modeling and bioinformatic approach introduced here supports this hypothesis. The allometric models trained using tailed phage with high-resolution structures predicted capsids among isolated and metagenome-assembled tailed phages that were smaller than the icosahedral tailed phages characterized to date. Among isolated tailed phages, we predicted four T = 3 icosahedral capsids. Among metagenome-assembled tailed phages, the study predicted twenty-one potential T < 3 architectures, including two T = 4/3 ≈ 1.33 icosahedral capsids.
The agreement between the theoretical and statistical scaling exponents for the genome length and the T-number rigorously quantified the expected relationships for tailed phages. Tailed phages pack their genome at a similar density, ~0.5 bp/nm3. This leads to a genome length scaling with the volume of the capsid. Since the T-number is proportional to the surface of the capsid and the exposed surface of the capsid protein is conserved, this led to a scaling of 3/2 (statistically, 1.47 ± 0.09 ) relating the genome length and the capsid architecture expressed in terms of the T-number. The genome length can be determined experimentally through molecular biology methods while the T-number can be obtained from high-resolution microscopy methods. The derived relationship between the genome length and T-number thus provided a direct approach to estimate the capsid architectures from the abundant molecular biology data. It also reduced the uncertainty of incorporating intermediate relationships, for example, with the capsid volume. The application of such relationship to isolated and assembled tailed phage genomes provided a method to predict the existence of missing small-tailed phage capsids.
The four isolated phages predicted to form T = 3 capsid architectures infected hosts from four distantly related phyla, suggesting that small-tailed phage capsids might be prevalent across bacterial hosts. The two isolated phages with the smallest genomes adopting T = 3 capsid architectures were Podoviridae: Salmonella phage astrithr (11.6 kbp, NC_48862) and Mycoplasma virus P1 (11.7 kbp, NC_002515). Their genome lengths were very similar to the predicted average genome length for T = 3 tailed phage capsids, 11.8 kbp. The other two phages were Siphoviridae and they had genomes ~ 3 kbp longer: Rhodococcus phage RRH1 (14.3 kbp, NC_016651) and Lactococcus phage bIL311 (14.5 kbp, NC_002670). Their genome lengths were well within the confidence limit predicted for T = 3 tailed phage capsids, 8.1–17.1 kbp. But their genome lengths also overlapped with the lower limit predicted for T = 4 tailed phage capsids, in the 13.2–24.5 kbp range. It thus cannot be excluded that these two phages adopt T = 4 instead of T = 3 capsids.
Among the gut metagenome-assembled tailed phages, the putative phages OGQL01007720.1 (4.5 kbp) and OMEC01003054.1 (5.4 kbp) were predicted to adopt T = 4/3 ≈ 1.33 icosahedral capsids. The trihexagonal lattice associated with T = 4/3 ≈ 1.33 has been observed among higher T-numbers for tailed phages [19,20]. However, no major capsid protein or portal protein was annotated in those two genomes as well as in five other genomes predicted to adopt larger T-numbers (Supplementary Figure S3). The absence of a major capsid protein, however, does not imply that the assembled genomes in our study were incomplete. All seven of these phages encoded at least one phage hallmark gene (portal protein or a large terminase subunit) and possessed terminal repeats at their contig termini, which are indicative of a closed genome assembly. One possibility explaining the lack of an identifiable major capsid protein is that the correct open reading frame (ORF) was not predicted [67]. Another interpretation is that there could be overlapping open reading frames, although this is unusual among tailed phages [67]. Alternatively, these contigs could be associated to satellite phages, which often lack the major capsid protein and other structural genes. In this case, it cannot be ruled out that the capsid structure adopted would be bigger than the predicted architecture. This is analogous to Enterobacteria phage P4 (NC_001609, genome length 11.6 kbp), which is a satellite phage of P2. Phage P4 does not encode a major capsid protein and forms a T = 4 capsid [66], which is larger than the predicted T = 3 capsid based on its genome length.
Nine metagenome-assembled tailed phages that displayed genomes from 7.3 to 8.5 kbp and were predicted to adopt a T = 7/3 ≈ 2.33 icosahedral capsid (Figure 6b). This group of diverse phages encoded major capsid proteins (Supplementary Figure S3), providing confidence to this prediction. Nonetheless, the capsid predicted adopted a snub hexagonal lattice, which has not been observed among larger tailed phages [19]. The same applies to the next group of ten tailed phages, which were predicted to adopt the T = 4/3 + 2/ 3 ≈ 2.49 capsid architecture. This capsid is associated with the rhombitrihexagonal lattice, which has not been observed among tailed phages either. If these predictions are confirmed, these would be the first viruses known to adopt regular snub hexagonal and rhombitrihexagonal lattices [19]. Alternatively, these two groups of phages could form elongated T = 1 capsids or icosahedral T = 3 capsids because the genome length of these phages is close to the lower 95% confidence interval predicted for T = 3 capsids, 8.1 kbp (Figure 4). No tailed phages were predicted to adopt the smallest icosahedral capsid, T = 1.
Additional studies will be necessary to determine if such small-tailed phage capsids exist. However, the bioinformatic identification of capsid architectures among uncultured phages adds to the trend of computational-based methods directing discoveries in phage biology. The assembly of co-occurring contigs in metagenomic samples recently led to the bioinformatic discovery of crAssphage (cross-assembly phage) [68]. CrAssphage is the most common group of phages in humans, but it had not been isolated previously [69]. The bioinformatic discovery fueled a search for this virus, and a crAssphage candidate, ΦCrAss001, was finally isolated after expanding the initial taxonomy of crAssphage [70,71,72]. The genome size of this candidate is 102 kbp. Our model predicts that it should adopt a T = 12 or T = 13 capsid architecture. This genome length and capsid architecture coincide with the frequency peak observed in the distribution of assembled genomes at ~98.2 kbp (Figure 5c). This peak is absent in the distribution of isolated genomes (Figure 5a). This result indicates that the architecture of crAssphage-like viruses has been under-sampled among isolated phages. The peaks associated with crAssphage-like genome lengths as well as small genome size phages are the only regions that differ significantly between the isolated and assembled genomes (Figure 5). This suggests that, besides these exceptional cases, the structural analysis of the current pool of isolated phages is probably a good proxy to account for the diversity of tailed phage architectures in the biosphere.
The allometric models developed in this study apply to tailed phages and other viruses in the Duplodnaviria realm because they share the same building block (HK97-fold major capsid protein) and genome packing strategy (dsDNA packed at high densities). The scaling exponents or geometric pre-factors differ for viruses that build their capsids with different capsid protein folds or pack their genome in other chemical and physical configurations [36]. Therefore, it is not strictly possible to validate the genome length and capsid size predictions for T = 1 and T = 3 capsids using viruses that do not belong to the Duplodnaviria realm. However, divergences between the different allometric models should reduce for small capsids because, in these capsids, the surface-to-volume ratio is higher. Viruses that pack their genome at lower densities than tailed phages tend to accumulate genome layers near the interior wall of the capsid and leave a void in the interior [73,74]. As capsids become smaller, the volume near the interior capsid wall, which contains the genome, will become larger than the central volume without the genome. Therefore, the model predictions could be estimated qualitatively with existing T = 1 viruses in realms other than Duplodnaviria. The model would provide an upper limit in the expected amount of genome stored, while the discrepancies in the capsid size should be associated—in a first approximation—to differences in the typical amino acid sequence length of the associated capsid proteins.
All isolated viruses that have been characterized to form T = 1 capsids—excluding satellite viruses and viral-like particles—store their genome as single-stranded DNA (ssDNA), and they belong to the Microviridae, Parvoviridae, and Circoviridae viral families in the Monodnaviria realm [29,75]. Viruses in these families all form capsids using the single-jellyroll fold [14]. However, the capsid proteins differ in size, and the viruses in each family infect different organisms and adopt different capsid sizes and genome lengths [76]. Microviridae viruses infect bacteria, display genomes lengths of 4.4 to 6.1 kb (kilobases), and capsid diameters of 30 nm, which include decoration capsid proteins and a single jellyroll capsid protein of 427 amino acids for the reference virus phiX174 (PDB 2BPA). Parvoviridae viruses infect vertebrates and insects, display genome lengths of 4 to 6 kb, and capsid diameters of 18–26 nm, with a single jellyroll capsid protein of 519 amino acids for the reference virus adeno-associated virus (PDB 1LP3). Circoviridae viruses infect birds and pigs, display genome lengths of 1.8 to 2.0 kb, and capsid diameters of 17 nm, with a single jellyroll capsid protein of 226 amino acids for the reference virus porcine circovirus 2 (PDB 3R0R). The model in our study predicted that tailed phages adopting T = 1 capsids would display a genome length in the range of 1.2–4.4 kbp and a capsid diameter in the range of 19.5–30.1 nm (Figure 4). Since tailed phages store dsDNA, the predicted range must be doubled for ssDNA viruses, that is, 2.4–8.8 kb. This represents an upper limit range because tailed phages store their genome at high densities throughout the entire interior capsid volume. In other words, 8.8 kb would be the maximum genome length for ssDNA viruses (with 95% confidence). The genome lengths of Microviridae and Parvoviridae viruses fall within the mid-range predictions of our model. The capsid diameter of Microviridae viruses is in the upper range, probably due to the presence of the decoration proteins. The capsid diameter of Parvoviridae viruses is in the mid-range of the capsid diameters predicted by the model. The case of Circoviridae viruses falls on the lower limit of the predicted genome lengths and capsid diameters. This is probably due to the fact that the capsid protein of tailed phages is typically ~300–350 amino acids—much larger than for Circoviridae viruses. In any case, the qualitative comparison between the predictions of the T = 1 capsids of tailed phages and the observed T = 1 viruses is consistent.
The comparison with Microviridae viruses is particularly appealing because these viruses infect bacteria and follow an analogous life cycle as tailed phages: translocating the genome through the host cell upon infection, assembling an empty procapsid, packing the genome to form the mature capsid, and lysing the host to release the new mature virions [31,32]. The absence of a tail in Microviridae viruses suggest that tailed phages in the Podoviridae family might be more likely to form T = 1 capsid architectures or small phage capsids. This is consistent with the fact that the smallest isolated tailed phages predicted to form T = 3 capsids were Podoviridae.
It has been observed that the genome length of viruses, bacteria, and archaea are smaller at higher temperatures [77,78,79]. This suggests that T ≤ 3 architectures would be more likely to be present in hot environments, such as hydrothermal vents, which can reach temperatures of ~100 °C or higher. Warm-blooded animals may also be a good source of small-tailed phage capsids. The temperature is lower than in hydrothermal vents but higher than in most other environments. Due to medical and economic reasons, metagenomes from warm-animal sources are more accessible and abundant, increasing the odds of finding small-tailed phage capsids. This is consistent with our finding of small capsids predicted among human gut tailed phages.
The discovery and study of small-tailed phage capsids would help interrogate the origin of the ancient Duplodnaviria realm and the origin of the last universal common ancestor for phages [30]. The assumption is that the smallest icosahedral capsids were precursors of tailed phage capsids. Therefore, the molecular information and divergence of small-tailed phages could provide insightful clues on how viruses emerged on Earth. These small-tailed phages could also help uncover a potential evolutionary relationship between tailed phages and cellular compartments like encapsulins [14,16,17].
The discovery and characterization of small-tailed phages would also have important biomedical implications. Their shorter genomes would contain fewer genes. It is expected that most of the genes would be involved in structural functions. The conserved folds of these proteins, such as the major capsid protein and the portal, would help narrow the functions of genes in these small genomes. This would facilitate the annotation and functional characterization of these phages, circumventing one of the main issues of phage therapy nowadays. Even in scenarios where phages have been used successfully as therapeutics, the number of unknown gene functions encoded in those phage genomes was significant and may be involved in increasing bacterial virulence [80,81]. Small-tailed phages would make the application of phage therapy more predictable and easier to regulate.

5. Conclusions

Small-tailed phages with icosahedral architectures T ≤ 3 have not yet been observed by high-resolution imaging techniques. Here, we proposed that these structures exist in the environment but are challenging to sample due to low abundances. The modeling and bioinformatic approach applied here predicted small icosahedral capsids among isolated and metagenome-assembled tailed phages. The smallest capsid predicted was a trihexagonal T = 4/3 ≈ 1.33. No candidate was found to form the smallest icosahedral capsid T = 1, but we proposed that Podoviridae in high-temperature environments, like warm-blooded animals and hydrothermal vents, would be potential candidates for the missing T = 1 tailed phage capsids. The discovery of these small-tailed phages would be transformative for the study of viral evolution as well as biomedical and biotechnological applications.

Supplementary Materials

The following are available online at https://www.mdpi.com/2076-2607/8/12/1944/s1, Figure S1: Residual diagnostics for the genome length model, Figure S2: Residual diagnostics for the capsid diameter model, Figure S3: Annotation of small metagenome-assembled tailed phage genomes. Table S1: Correlation analysis for the capsid structural properties as a function of the capsid diameter. Data File S1: Geometrical measurements of high-resolution tailed phage capsids. Data File S2: NCBI identifiers and genome length for associated isolated genomes. Data File S3: Contig identifiers and genome lengths of gut metagenome-assembled tailed phage genomes. Data File S4: genome length range predicted for T-number architectures (T < 40). Data File S5: capsid diameter range predicted for T-number architectures (T < 40). Data File S6: Associated isolated tailed phages predicted to form T ≤ 3 capsid architectures. Data File S7: T-number architectures predicted for isolated phages. Data File S8: Associated gut metagenome-assembled tailed phages predicted to form T ≤ 3 capsid architectures. Data File S9: T-number architectures predicted for metagenome-assembled tailed phages.

Author Contributions

Conceptualization, A.L. and S.W.; methodology, A.L., D.Y.L. and S.B.; software, A.L., C.B. and S.B.; validation, A.L., S.B. and S.W.; formal analysis, A.L.; investigation, A.L.; resources, A.L. and S.B.; data curation, A.L.; writing—original draft preparation, A.L.; writing—review and editing, S.W., S.B., D.Y.L. and C.B.; visualization, A.L. and C.B. All authors have read and agreed to the published version of the manuscript.

Funding

A.L. acknowledges that this research was funded by the National Science Foundation Award 1951678 in the Division of Mathematical Sciences. S.B. is supported by the Intramural Research Program of the National Institutes of Health (National Library of Medicine).

Acknowledgments

Molecular graphics and analyses were performed with UCSF Chimera, developed by the Resource for Biocomputing, Visualization, and Informatics at the University of California, San Francisco, with support from NIH P41-GM103311.

Conflicts of Interest

The authors declare no conflict of interest. The funders had no role in the design of the study; in the collection, analyses, or interpretation of data; in the writing of the manuscript, or in the decision to publish the results.

References

  1. Cobián Güemes, A.G.; Youle, M.; Cantú, V.A.; Felts, B.; Nulton, J.; Rohwer, F. Viruses as winners in the game of life. Annu. Rev. Virol. 2016, 3, 197–214. [Google Scholar] [CrossRef] [PubMed]
  2. Wommack, K.E.; Colwell, R.R. Virioplankton: Viruses in aquatic ecosystems. Microbiol. Mol. Biol. Rev. 2000, 64, 69–114. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  3. Danovaro, R.; Corinaldesi, C.; Dell’Anno, A.; Fuhrman, J.A.; Middelburg, J.J.; Noble, R.T.; Suttle, C.A. Marine viruses and global climate change. FEMS Microbiol. Rev. 2011, 35, 993–1034. [Google Scholar] [CrossRef] [PubMed]
  4. Knowles, B.; Silveira, C.B.; Bailey, B.A.; Barott, K.; Cantu, V.A.; Cobián-Güemes, A.G.; Coutinho, F.H.; Dinsdale, E.A.; Felts, B.; Furby, K.A.; et al. Lytic to temperate switching of viral communities. Nature 2016, 531, 466–470. [Google Scholar] [CrossRef]
  5. Luque, A.; Silveira, C. Quantification of lysogeny caused by phage coinfections in microbial communities from biophysical principles. mSystems 2020, 5, e00353-20. [Google Scholar] [CrossRef]
  6. Silveira, C.B.; Coutinho, F.H.; Cavalcanti, G.S.; Benler, S.; Doane, M.P.; Dinsdale, E.A.; Edwards, R.A.; Francini-Filho, R.B.; Thompson, C.C.; Luque, A.; et al. Genomic and ecological attributes of marine bacteriophages encoding bacterial virulence genes. BMC Genomics 2020, 21, 126. [Google Scholar] [CrossRef]
  7. Paez-Espino, D.; Eloe-Fadrosh, E.A.; Pavlopoulos, G.A.; Thomas, A.D.; Huntemann, M.; Mikhailova, N.; Rubin, E.; Ivanova, N.N.; Kyrpides, N.C. Uncovering Earth’s virome. Nature 2016, 5636, 425–430. [Google Scholar] [CrossRef]
  8. Hua, J.; Huet, A.; Lopez, C.A.; Toropova, K.; Pope, W.H.; Duda, R.L.; Hendrix, R.W.; Conway, J.F. Capsids and genomes of jumbo-sized bacteriophages reveal the evolutionary reach of the HK97 fold. MBio 2017, 8. [Google Scholar] [CrossRef] [Green Version]
  9. Briani, F.; Dehò, G.; Forti, F.; Ghisotti, D. The plasmid status of satellite bacteriophage P4. Plasmid 2001, 45, 1–17. [Google Scholar] [CrossRef]
  10. Suhanovsky, M.M.; Teschke, C.M. Nature’s favorite building block: Deciphering folding and capsid assembly of proteins with the HK97-fold. Virology 2015, 479–480, 487–497. [Google Scholar] [CrossRef] [Green Version]
  11. Ackermann, H.W. 5500 Phages examined in the electron microscope. Arch. Virol. 2007, 152, 227–243. [Google Scholar] [CrossRef] [PubMed]
  12. Luque, A.; Reguera, D. The structure of elongated viral capsids. Biophys. J. 2010, 98, 2993–3003. [Google Scholar] [CrossRef] [Green Version]
  13. Luque, A.; Zandi, R.; Reguera, D. Optimal architectures of elongated viruses. Proc. Natl. Acad. Sci. USA 2010, 107, 5323–5328. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  14. Krupovic, M.; Koonin, E. V Multiple origins of viral capsid proteins from cellular ancestors. Proc. Natl. Acad. Sci. USA 2017, 114, E2401–E2410. [Google Scholar] [CrossRef] [Green Version]
  15. Ho, P.T.; Montiel-Garcia, D.J.; Wong, J.J.; Carrillo-Tripp, M.; Brooks III, C.L.; Johnson, J.E.; Reddy, V.S. VIPERdb: A Tool for Virus Research. Annu. Rev. Virol. 2018, 5, 477–488. [Google Scholar] [CrossRef] [PubMed]
  16. Sutter, M.; Boehringer, D.; Gutmann, S.; Günther, S.; Prangishvili, D.; Loessner, M.J.; Stetter, K.O.; Weber-Ban, E.; Ban, N. Structural basis of enzyme encapsulation into a bacterial nanocompartment. Nat. Struct. Mol. Biol. 2008, 15, 939–947. [Google Scholar] [CrossRef] [PubMed]
  17. Giessen, T.W.; Orlando, B.J.; Verdegaal, A.A.; Chambers, M.G.; Gardener, J.; Bell, D.C.; Birrane, G.; Liao, M.; Silver, P.A. Large protein organelles form a new iron sequestration system with high storage capacity. Elife 2019, 8, e46070. [Google Scholar] [CrossRef] [PubMed]
  18. Caspar, D.L.; Klug, A. Physical principles in the construction of regular viruses. Cold Spring Harb. Symp. Quant. Biol. 1962, 27, 1–24. [Google Scholar] [CrossRef]
  19. Twarock, R.; Luque, A. Structural puzzles in virology solved with an overarching icosahedral design principle. Nat. Commun. 2019, 10, 4414. [Google Scholar] [CrossRef] [Green Version]
  20. Podgorski, J.; Calabrese, J.; Alexandrescu, L.; Jacobs-Sera, D.; Pope, W.; Hatfull, G.; White, S. Structures of three actinobacteriophage capsids: Roles of symmetry and accessory proteins. Viruses 2020, 12, 294. [Google Scholar] [CrossRef] [Green Version]
  21. Tao, Y.; Olson, N.H.; Xu, W.; Anderson, D.L.; Rossmann, M.G.; Baker, T.S. Assembly of a tailed bacterial virus and its genome release studied in three dimensions. Cell 1998, 95, 431–437. [Google Scholar] [CrossRef] [Green Version]
  22. Choi, K.H.; Morais, M.C.; Anderson, D.L.; Rossmann, M.G. Determinants of bacteriophage phi29 head morphology. Structure 2006, 14, 1723–1727. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  23. Aksyuk, A.; Bowman, V.D.; Kaufmann, B.; Fields, C.; Klose, T.; Holdaway, H.; Fischetti, V.; Rossmann, M.G. Structural investigations of a Podoviridae streptococcus phage C1, implications for the mechanism of viral entry. Proc. Natl. Acad. Sci. USA 2012, 109, 14001–14006. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  24. Zandi, R.; Reguera, D.; Bruinsma, R.F.; Gelbart, W.M.; Rudnick, J. Origin of icosahedral symmetry in viruses. Proc. Natl. Acad. Sci. USA 2004, 101, 15556–15560. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  25. Luque, A.; Reguera, D.; Morozov, A.; Rudnick, J.; Bruinsma, R. Physics of shell assembly: Line tension, hole implosion, and closure catastrophe. J. Chem. Phys. 2012, 136, 184507. [Google Scholar] [CrossRef] [PubMed]
  26. Hagan, M.F. Modeling viral capsid assembly. Adv. Chem. Phys. 2014, 155, 1. [Google Scholar] [CrossRef] [Green Version]
  27. Aznar, M.; Reguera, D. Physical ingredients controlling stability and structural selection of empty viral capsids. J. Phys. Chem. B 2016, 120, 6147–6159. [Google Scholar] [CrossRef]
  28. Rice, G.; Tang, L.; Stedman, K.; Roberto, F.; Spuhler, J.; Gillitzer, E.; Johnson, J.E.; Douglas, T.; Young, M. The structure of a thermophilic archaeal virus shows a double-stranded DNA viral capsid type that spans all domains of life. Proc. Natl. Acad. Sci. USA 2004, 101, 7716–7720. [Google Scholar] [CrossRef] [Green Version]
  29. Koonin, E.V.; Dolja, V.V.; Krupovic, M.; Varsani, A.; Wolf, Y.I.; Yutin, N.; Zerbini, F.M.; Kuhn, J.H. Global organization and proposed megataxonomy of the virus world. Microbiol. Mol. Biol. Rev. 2020, 84. [Google Scholar] [CrossRef]
  30. Krupovic, M.; Dolja, V.V.; Koonin, E.V. The LUCA and its complex virome. Nat. Rev. Microbiol. 2020, 18, 661–670. [Google Scholar] [CrossRef]
  31. Doore, S.M.; Fane, B.A. The microviridae: Diversity, assembly, and experimental evolution. Virology 2016, 491, 45–55. [Google Scholar] [CrossRef]
  32. Creasy, A.; Rosario, K.; Leigh, B.A.; Dishaw, L.J.; Breitbart, M. Unprecedented diversity of ssDNA phages from the family Microviridae detected within the gut of a protochordate model organism (Ciona robusta). Viruses 2018, 10, 404. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  33. Mavrich, T.N.; Hatfull, G.F. Evolution of Superinfection Immunity in Cluster A Mycobacteriophages. Am. Soc. Microbiol. 2019, 10. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  34. Wiegand, T.; Karambelkar, S.; Bondy-Denomy, J.; Wiedenheft, B. Structures and Strategies of Anti-CRISPR-Mediated Immune Suppression. Annu. Rev. Microbiol. 2020, 74, E5122–E5128. [Google Scholar] [CrossRef] [PubMed]
  35. Breitbart, M.; Thompson, L.R.; Suttle, C.A.; Sullivan, M.B. Exploring the vast diversity of marine viruses. Oceanography 2007, 20, 135–139. [Google Scholar] [CrossRef]
  36. Edwards, K.F.; Steward, G.F.; Schvarcz, C.A. Making sense of virus size and the tradeoffs shaping viral fitness. Ecol. Lett. 2020. [Google Scholar] [CrossRef]
  37. Liu, X.; Zhang, Q.; Murata, K.; Baker, M.L.; Sullivan, M.B.; Fu, C.; Dougherty, M.T.; Schmid, M.F.; Osburne, M.S.; Chisholm, S.W.; et al. Structural changes in a marine podovirus associated with release of its genome into Prochlorococcus. Nat. Struct. Mol. Biol. 2010, 17, 830–836. [Google Scholar] [CrossRef] [Green Version]
  38. Bebeacua, C.; Lai, L.; Vegge, C.S.; Brøndsted, L.; van Heel, M.; Veesler, D.; Cambillau, C. Visualizing a complete Siphoviridae member by single-particle electron microscopy: The structure of lactococcal phage TP901-1. J. Virol. 2013, 87, 1061–1068. [Google Scholar] [CrossRef] [Green Version]
  39. Parent, K.N.; Tang, J.; Cardone, G.; Gilcrease, E.B.; Janssen, M.E.; Olson, N.H.; Casjens, S.R.; Baker, T.S. Three-dimensional reconstructions of the bacteriophage CUS-3 virion reveal a conserved coat protein I-domain but a distinct tailspike receptor-binding domain. Virology 2014, 464, 55–66. [Google Scholar] [CrossRef] [Green Version]
  40. Spilman, M.S.; Dearborn, A.D.; Chang, J.R.; Damle, P.K.; Christie, G.E.; Dokland, T. A conformational switch involved in maturation of Staphylococcus aureus bacteriophage 80α capsids. J. Mol. Biol. 2011, 405, 863–876. [Google Scholar] [CrossRef] [Green Version]
  41. Effantin, G.; Figueroa-Bossi, N.; Schoehn, G.; Bossi, L.; Conway, J.F. The tripartite capsid gene of Salmonella phage Gifsy-2 yields a capsid assembly pathway engaging features from HK97 and λ. Virology 2010, 402, 355–365. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  42. Shen, P.S.; Domek, M.J.; Sanz-García, E.; Makaju, A.; Taylor, R.M.; Hoggan, R.; Culumber, M.D.; Oberg, C.J.; Breakwell, D.P.; Prince, J.T. Sequence and structural characterization of great salt lake bacteriophage CW02, a member of the T7-like supergroup. J. Virol. 2012, 86, 7907–7917. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  43. Dai, W.; Hodes, A.; Hui, W.H.; Gingery, M.; Miller, J.F.; Zhou, Z.H. Three-dimensional structure of tropism-switching Bordetella bacteriophage. Proc. Natl. Acad. Sci. USA 2010, 107, 4347–4352. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  44. White, H.E.; Sherman, M.B.; Brasilès, S.; Jacquet, E.; Seavers, P.; Tavares, P.; Orlova, E. V Capsid Structure and Its Stability at the Late Stages of Bacteriophage SPP1 Assembly. J. Virol. 2012, 86, 6768–6777. [Google Scholar] [CrossRef] [Green Version]
  45. Grose, J.H.; Belnap, D.M.; Jensen, J.D.; Mathis, A.D.; Prince, J.T.; Merrill, B.D.; Burnett, S.H.; Breakwell, D.P. The Genomes, Proteomes, and Structures of Three Novel Phages That Infect the Bacillus cereus Group and Carry Putative Virulence Factors. J. Virol. 2014, 88, 11846–11860. [Google Scholar] [CrossRef] [Green Version]
  46. Lander, G.C.; Baudoux, A.; Azam, F.; Potter, C.S.; Carragher, B.; Johnson, J.E. Article Capsomer Dynamics and Stabilization in the T = 12 Marine Bacteriophage SIO-2 and Its Procapsid Studied by CryoEM. Struct. Des. 2012, 20, 498–503. [Google Scholar] [CrossRef] [Green Version]
  47. Vernhes, E.; Renouard, M.; Gilquin, B.; Cuniasse, P.; Durand, D.; England, P.; Hoos, S.; Huet, A.; Conway, J.F.; Glukhov, A. High affinity anchoring of the decoration protein pb10 onto the bacteriophage T5 capsid. Sci. Rep. 2017, 7, 41662. [Google Scholar] [CrossRef]
  48. Lander, G.C.; Tang, L.; Casjens, S.R.; Gilcrease, E.B.; Prevelige, P.; Poliakov, A.; Potter, C.S.; Carragher, B.; Johnson, J.E. The structure of an infectious P22 virion shows the signal for headful DNA packaging. Science 2006, 312, 1791–1795. [Google Scholar] [CrossRef] [Green Version]
  49. Stroupe, M.E.; Brewer, T.E.; Sousa, D.R.; Jones, K.M. The structure of Sinorhizobium meliloti phage ΦM12, which has a novel T= 19l triangulation number and is the founder of a new group of T4-superfamily phages. Virology 2014, 450, 205–212. [Google Scholar] [CrossRef] [Green Version]
  50. Effantin, G.; Hamasaki, R.; Kawasaki, T.; Bacia, M.; Moriscot, C.; Weissenhorn, W.; Yamada, T.; Schoehn, G. Cryo-electron microscopy three-dimensional structure of the jumbo phage ΦRSL1 infecting the phytopathogen Ralstonia solanacearum. Structure 2013, 21, 298–305. [Google Scholar] [CrossRef] [Green Version]
  51. Fokine, A.; Kostyuchenko, V.; Efimov, A.V.; Kurochkina, L.P.; Sykilinda, N.N.; Robben, J.; Volckaert, G.; Hoenger, A.; Chipman, P.R.; Battisti, A.J.; et al. A three-dimensional cryo-electron microscopy structure of the bacteriophage φKZ head. J. Mol. Biol. 2005, 352, 117–124. [Google Scholar] [CrossRef] [PubMed]
  52. Pietilä, M.; Laurinmäki, P.; Russell, D.A.; Ching-Chung, K.; Jacobs-Sera, D.; Hendrix, R.W.; Bamford, D.H.; Butcher, S.J. Structure of the archaeal head-tailed virus HSTV-1 completes the HK97 fold story. Proc. Natl. Acad. Sci. USA 2013, 110, 10604–10609. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  53. Guo, F.; Liu, Z.; Fang, P.-A.; Zhang, Q.; Wright, E.T.; Wu, W.; Zhang, C.; Vago, F.; Ren, Y.; Jakana, J. Capsid expansion mechanism of bacteriophage T7 revealed by multistate atomic models derived from cryo-EM reconstructions. Proc. Natl. Acad. Sci. USA 2014, 111, E4606–E4614. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  54. Parent, K.N.; Gilcrease, E.B.; Casjens, S.R.; Baker, T.S. Structural evolution of the P22-like phages: Comparison of Sf6 and P22 procapsid and virion architectures. Virology 2012, 427, 177–188. [Google Scholar] [CrossRef] [Green Version]
  55. Baker, M.L.; Hryc, C.F.; Zhang, Q.; Wu, W.; Jakana, J.; Haase-Pettingell, C.; Afonine, P.V.; Adams, P.D.; King, J.; Jiang, W.; et al. Validated near-atomic resolution structure of bacteriophage epsilon15 derived from cryo-EM and modeling. Proc. Natl. Acad. Sci. USA 2013, 110, 12301–12306. [Google Scholar] [CrossRef] [Green Version]
  56. Gipson, P.; Baker, M.L.; Raytcheva, D.; Haase-Pettingell, C.; Piret, J.; King, J.A.; Chiu, W. Protruding knob-like proteins violate local symmetries in an icosahedral marine virus. Nat. Commun. 2014, 5, 4278. [Google Scholar] [CrossRef] [Green Version]
  57. Leiman, P.G.; Battisti, A.J.; Bowman, V.D.; Stummeyer, K.; Mühlenhoff, M.; Gerardy-Schahn, R.; Scholl, D.; Molineux, I.J. The structures of bacteriophages K1E and K1-5 explain processive degradation of polysaccharide capsules and evolution of new host specificities. J. Mol. Biol. 2007, 371, 836–849. [Google Scholar] [CrossRef]
  58. Lander, G.C.; Evilevitch, A.; Jeembaeva, M.; Potter, C.S.; Carragher, B.; Johnson, J.E. Bacteriophage lambda stabilization by auxiliary protein gpD: Timing, location, and mechanism of attachment determined by cryo-EM. Structure 2008, 16, 1399–1406. [Google Scholar] [CrossRef] [Green Version]
  59. Pettersen, E.F.; Goddard, T.D.; Huang, C.C.; Couch, G.S.; Greenblatt, D.M.; Meng, E.C.; Ferrin, T.E. UCSF Chimera—A visualization system for exploratory research and analysis. J. Comput. Chem. 2004, 25, 1605–1612. [Google Scholar] [CrossRef] [Green Version]
  60. Kutner, M.H.; Neter, J.; Nachtsheim, C.J.; Li, W. Applied Linear Statistical Models, 5th ed.; McGraw-Hill Education: New York, NY, USA, 2004. [Google Scholar]
  61. James, G.; Witten, D.; Hastie, T.; Tibshirani, R. An Introduction to Statistical Learning, 7th ed.; Springer: New York, NY, USA, 2017. [Google Scholar]
  62. R Core Team. R: A Language and Environment for Statistical Computing; R Foundation for Statistical Computing: Vienna, Austria, 2018. [Google Scholar]
  63. O’Leary, N.A.; Wright, M.W.; Brister, J.R.; Ciufo, S.; Haddad, D.; McVeigh, R.; Rajput, B.; Robbertse, B.; Smith-White, B.; Ako-Adjei, D. Reference sequence (RefSeq) database at NCBI: Current status, taxonomic expansion, and functional annotation. Nucleic Acids Res. 2016, 44, D733–D745. [Google Scholar] [CrossRef] [Green Version]
  64. Benler, S.; Yutin, N.; Antipov, D.; Raykov, M.; Shmakov, S.A.; Gussow, A.B.; Pevzner, P.A.; Koonin, E. V Thousands of previously unknown phages discovered in whole-community human gut metagenomes. bioRxiv 2020. [Google Scholar] [CrossRef]
  65. Aznar, M.; Luque, A.; Reguera, D. Relevance of capsid structure in the buckling and maturation of spherical viruses. Phys. Biol. 2012, 9, 036003. [Google Scholar] [CrossRef] [PubMed]
  66. Dearborn, A.D.; Laurinmaki, P.; Chandramouli, P.; Rodenburg, C.M.; Wang, S.; Butcher, S.J.; Dokland, T. Structure and size determination of bacteriophage P2 and P4 procapsids: Function of size responsiveness mutations. J. Struct. Biol. 2012, 178, 215–224. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  67. McNair, K.; Zhou, C.; Dinsdale, E.A.; Souza, B.; Edwards, R.A. PHANOTATE: A novel approach to gene identification in phage genomes. Bioinformatics 2019, 35, 4537–4542. [Google Scholar] [CrossRef] [Green Version]
  68. Dutilh, B.E.; Cassman, N.; McNair, K.; Sanchez, S.E.; Silva, G.G.Z.; Boling, L.; Barr, J.J.; Speth, D.R.; Seguritan, V.; Aziz, R.K. A highly abundant bacteriophage discovered in the unknown sequences of human faecal metagenomes. Nat. Commun. 2014, 5, 1–11. [Google Scholar] [CrossRef] [Green Version]
  69. Edwards, R.A.; Vega, A.A.; Norman, H.M.; Ohaeri, M.; Levi, K.; Dinsdale, E.A.; Cinek, O.; Aziz, R.K.; McNair, K.; Barr, J.J. Global phylogeography and ancient evolution of the widespread human gut virus crAssphage. Nat. Microbiol. 2019, 4, 1727–1736. [Google Scholar] [CrossRef] [Green Version]
  70. Shkoporov, A.N.; Khokhlova, E.V.; Fitzgerald, C.B.; Stockdale, S.R.; Draper, L.A.; Ross, R.P.; Hill, C. ΦCrAss001 represents the most abundant bacteriophage family in the human gut and infects Bacteroides intestinalis. Nat. Commun. 2018, 9, 1–8. [Google Scholar] [CrossRef] [Green Version]
  71. Guerin, E.; Shkoporov, A.; Stockdale, S.R.; Clooney, A.G.; Ryan, F.J.; Sutton, T.D.S.; Draper, L.A.; Gonzalez-Tortuero, E.; Ross, R.P.; Hill, C. Biology and taxonomy of crAss-like bacteriophages, the most abundant virus in the human gut. Cell Host Microbe 2018, 24, 653–664. [Google Scholar] [CrossRef] [Green Version]
  72. Yutin, N.; Makarova, K.S.; Gussow, A.B.; Krupovic, M.; Segall, A.; Edwards, R.A.; Koonin, E. V Discovery of an expansive bacteriophage family that includes the most abundant viruses from the human gut. Nat. Microbiol. 2018, 3, 38–46. [Google Scholar] [CrossRef]
  73. Sivanandam, V.; Mathews, D.; Garmann, R.; Erdemci-Tandogan, G.; Zandi, R.; Rao, A.L.N. Functional analysis of the N-terminal basic motif of a eukaryotic satellite RNA virus capsid protein in replication and packaging. Sci. Rep. 2016, 6, 26328. [Google Scholar] [CrossRef] [Green Version]
  74. Beren, C.; Cui, Y.; Chakravarty, A.; Yang, X.; Rao, A.L.N.; Knobler, C.M.; Zhou, Z.H.; Gelbart, W.M. Genome organization and interaction with capsid protein in a multipartite RNA virus. Proc. Natl. Acad. Sci. USA 2020, 117, 10673–10680. [Google Scholar] [CrossRef] [PubMed]
  75. Carrillo-Tripp, M.; Shepherd, C.M.; Borelli, I.A.; Venkataraman, S.; Lander, G.; Natarajan, P.; Johnson, J.E.; Brooks III, C.L.; Reddy, V.S. VIPERdb2: An enhanced and web API enabled relational database for structural virology. Nucleic Acids Res. 2008, 37, D436–D442. [Google Scholar] [CrossRef]
  76. Hulo, C.; De Castro, E.; Masson, P.; Bougueleret, L.; Bairoch, A.; Xenarios, I.; Le Mercier, P. ViralZone: A knowledge resource to understand virus diversity. Nucleic Acids Res. 2011, 39, D576–D582. [Google Scholar] [CrossRef] [Green Version]
  77. Daufresne, M.; Lengfellner, K.; Som-mer, U. Global warming benefits the small in aquatic ecosystems, 12788-12793. Proc. Natl. Acad. Sci. USA 2009, 106, 21. [Google Scholar] [CrossRef] [Green Version]
  78. Morán, X.A.G.; López-Urrutia, Á.; Calvo-Díaz, A.; Li, W.K.W. Increasing importance of small phytoplankton in a warmer ocean. Glob. Chang. Biol. 2010, 16, 1137–1144. [Google Scholar] [CrossRef]
  79. Nifong, R.L.; Gillooly, J.F. Temperature effects on virion volume and genome length in dsDNA viruses. Biol. Lett. 2016, 12, 20160023. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  80. Schooley, R.T.; Biswas, B.; Gill, J.J.; Hernandez-Morales, A.; Lancaster, J.; Lessor, L.; Barr, J.J.; Reed, S.L.; Rohwer, F.; Benler, S. Development and use of personalized bacteriophage-based therapeutic cocktails to treat a patient with a disseminated resistant Acinetobacter baumannii infection. Antimicrob. Agents Chemother. 2017, 61. [Google Scholar] [CrossRef] [Green Version]
  81. Hatfull, G.F. Actinobacteriophages: Genomics, Dynamics, and Applications. Annu. Rev. Virol. 2020, 7, 37–61. [Google Scholar] [CrossRef]
Microorganisms 08 01944 g001 550
Figure 1. HK97-fold protein compartments. The left side of the figure focuses on encapsulins, nanocompartments responsible for chemical storage and biochemical reactions in bacteria and archaea. The right side of the figure focuses on viruses and gene transfer agents. The viruses belong to the realm of Duplodnaviria. Tailed phages in the phylum Uroviricota and class Cauviricetes are the most diverse and abundant representatives of this group.
Figure 1. HK97-fold protein compartments. The left side of the figure focuses on encapsulins, nanocompartments responsible for chemical storage and biochemical reactions in bacteria and archaea. The right side of the figure focuses on viruses and gene transfer agents. The viruses belong to the realm of Duplodnaviria. Tailed phages in the phylum Uroviricota and class Cauviricetes are the most diverse and abundant representatives of this group.
Microorganisms 08 01944 g001
Microorganisms 08 01944 g002 550
Figure 2. Icosahedral lattices for T(2,1) = 7 capsids. The label on the top displays the name of the lattice. The blue arrows and blue dots display the h and k steps in the hexagonal lattice, h = 2 and k = 1 in this case. The generalized Th, Tt, Ts, and Tr numbers are obtained from the classic T-number multiplied by the lattice factor associated with the minor polygons (triangles and squares) of each lattice: h (hexagonal), t (trihexagonal), s (snub hexagonal), and r (rhombitrihexagonal).
Figure 2. Icosahedral lattices for T(2,1) = 7 capsids. The label on the top displays the name of the lattice. The blue arrows and blue dots display the h and k steps in the hexagonal lattice, h = 2 and k = 1 in this case. The generalized Th, Tt, Ts, and Tr numbers are obtained from the classic T-number multiplied by the lattice factor associated with the minor polygons (triangles and squares) of each lattice: h (hexagonal), t (trihexagonal), s (snub hexagonal), and r (rhombitrihexagonal).
Microorganisms 08 01944 g002
Microorganisms 08 01944 g003 550
Figure 3. Tailed phage statistical models. Genome length (a) and capsid diameter (b) plotted as a function of capsid architecture for the studied tailed phage structures (black circles) and the predicted small-tailed phage structures (blue diamonds). (a,b) The solid blue lines and grey areas correspond, respectively, to the mean values and 95% confidence interval predicted from the statistical model. The mean values and standard errors of the fitted parameters, as well as the coefficient of determination (R2), are displayed.
Figure 3. Tailed phage statistical models. Genome length (a) and capsid diameter (b) plotted as a function of capsid architecture for the studied tailed phage structures (black circles) and the predicted small-tailed phage structures (blue diamonds). (a,b) The solid blue lines and grey areas correspond, respectively, to the mean values and 95% confidence interval predicted from the statistical model. The mean values and standard errors of the fitted parameters, as well as the coefficient of determination (R2), are displayed.
Microorganisms 08 01944 g003
Microorganisms 08 01944 g004 550
Figure 4. Predicted small-tailed phage capsids. The sequence of three-dimensional (3D) capsid icosahedral models generated with the new hkcage tool in ChimeraX and available in https://github.com/luquelab/hkcage.git. The information below each structure corresponds to the T-number architecture, (h,k) steps, lattice (h: hexagonal, t: trihexagonal, s: snub hexagonal, and r: rhombitrihexagonal), the average predicted genome length (95% confidence interval), and the average predicted capsid diameter (95% confidence interval).
Figure 4. Predicted small-tailed phage capsids. The sequence of three-dimensional (3D) capsid icosahedral models generated with the new hkcage tool in ChimeraX and available in https://github.com/luquelab/hkcage.git. The information below each structure corresponds to the T-number architecture, (h,k) steps, lattice (h: hexagonal, t: trihexagonal, s: snub hexagonal, and r: rhombitrihexagonal), the average predicted genome length (95% confidence interval), and the average predicted capsid diameter (95% confidence interval).
Microorganisms 08 01944 g004
Microorganisms 08 01944 g005 550
Figure 5. Predicted architectures among tailed phage isolates. (a) Genome length distribution for tailed phage isolates obtained from the NCBI Reference Sequence Database. (b) Frequency (percentage) of predicted T-number architectures from the genome lengths of isolated tailed phages. The grey area highlights the absence of T < 3 architectures. (c,d) Genome length distribution and predicted T-number architectures for putative gut tailed phages. (a–d) The arrows indicate the significant peaks of the genome length distribution and the associated T-number architectures. (b,d) The parenthesis indicates the number of predicted T ≤ 4 phage capsid architectures.
Figure 5. Predicted architectures among tailed phage isolates. (a) Genome length distribution for tailed phage isolates obtained from the NCBI Reference Sequence Database. (b) Frequency (percentage) of predicted T-number architectures from the genome lengths of isolated tailed phages. The grey area highlights the absence of T < 3 architectures. (c,d) Genome length distribution and predicted T-number architectures for putative gut tailed phages. (a–d) The arrows indicate the significant peaks of the genome length distribution and the associated T-number architectures. (b,d) The parenthesis indicates the number of predicted T ≤ 4 phage capsid architectures.
Microorganisms 08 01944 g005
Microorganisms 08 01944 g006 550
Figure 6. Predicted small-tailed phages. (a) List of isolated tailed phages predicted to adopt a T = 3 capsid architecture. The panel displays the phage name, genome length, host, and hosts’ phylogenetic tree. (b) List of putative gut tailed phage genomes predicted to adopt T ≤ 3 capsid architectures. The list displays the contig name and genome length in parenthesis. (c) Genome map of the smallest genome encoding a major capsid protein (MCP). The scale is 1 kbp. The genes are grouped by function: packaging (red), replication (orange), structural (green), and hypothetical (yellow).
Figure 6. Predicted small-tailed phages. (a) List of isolated tailed phages predicted to adopt a T = 3 capsid architecture. The panel displays the phage name, genome length, host, and hosts’ phylogenetic tree. (b) List of putative gut tailed phage genomes predicted to adopt T ≤ 3 capsid architectures. The list displays the contig name and genome length in parenthesis. (c) Genome map of the smallest genome encoding a major capsid protein (MCP). The scale is 1 kbp. The genes are grouped by function: packaging (red), replication (orange), structural (green), and hypothetical (yellow).
Microorganisms 08 01944 g006
Table
Table 1. Summary of measured structural properties. * The sphere factor ranged from 0 (polyhedral) to 1 (spherical). † Maximum (icosahedral) diameter determined from the vertex (5-fold) to vertex (5-fold).
Table 1. Summary of measured structural properties. * The sphere factor ranged from 0 (polyhedral) to 1 (spherical). † Maximum (icosahedral) diameter determined from the vertex (5-fold) to vertex (5-fold).
PropertyRangePropertyRange
Capsids analyzed23Genome size17–280 kbp
T-number4–27Genome density0.34–0.62 bp/nm3
Interior sphericity *0.25–0.75Interior surface5.08 × 103–4.32 × 104 nm2
Exterior sphericity *0.14–0.55MCP interior area19–26 nm2
Capsid diameter †49–143 nmExterior surface6.55 × 103–5.19 × 104 nm2
Capsid thickness3–8 nmMCP exterior area24–35 nm2
Interior volume3.36 × 104–8.22 × 105 nm3MCP ratio (%)15–43%
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Share and Cite

MDPI and ACS Style

Luque, A.; Benler, S.; Lee, D.Y.; Brown, C.; White, S. The Missing Tailed Phages: Prediction of Small Capsid Candidates. Microorganisms 2020, 8, 1944. https://doi.org/10.3390/microorganisms8121944

AMA Style

Luque A, Benler S, Lee DY, Brown C, White S. The Missing Tailed Phages: Prediction of Small Capsid Candidates. Microorganisms. 2020; 8(12):1944. https://doi.org/10.3390/microorganisms8121944

Chicago/Turabian Style

Luque, Antoni, Sean Benler, Diana Y. Lee, Colin Brown, and Simon White. 2020. "The Missing Tailed Phages: Prediction of Small Capsid Candidates" Microorganisms 8, no. 12: 1944. https://doi.org/10.3390/microorganisms8121944

APA Style

Luque, A., Benler, S., Lee, D. Y., Brown, C., & White, S. (2020). The Missing Tailed Phages: Prediction of Small Capsid Candidates. Microorganisms, 8(12), 1944. https://doi.org/10.3390/microorganisms8121944

Note that from the first issue of 2016, this journal uses article numbers instead of page numbers. See further details here.

Article Metrics

Back to TopTop