Hybrid Resonant Converter with Three Half-Bridge Legs for Wide Voltage Operation
Abstract
:1. Introduction
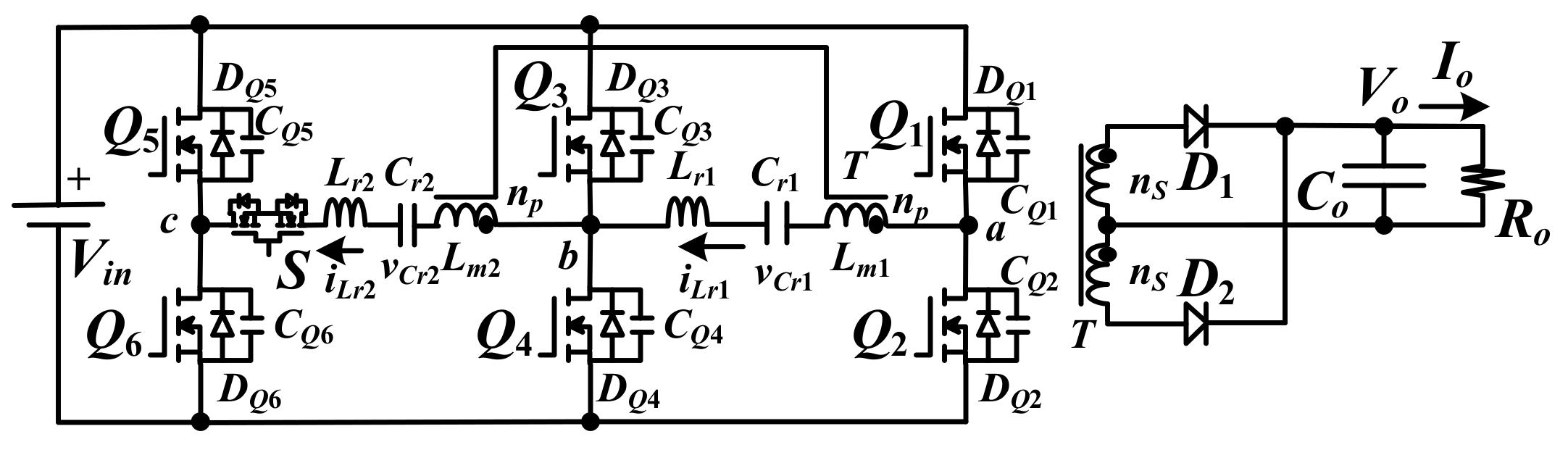
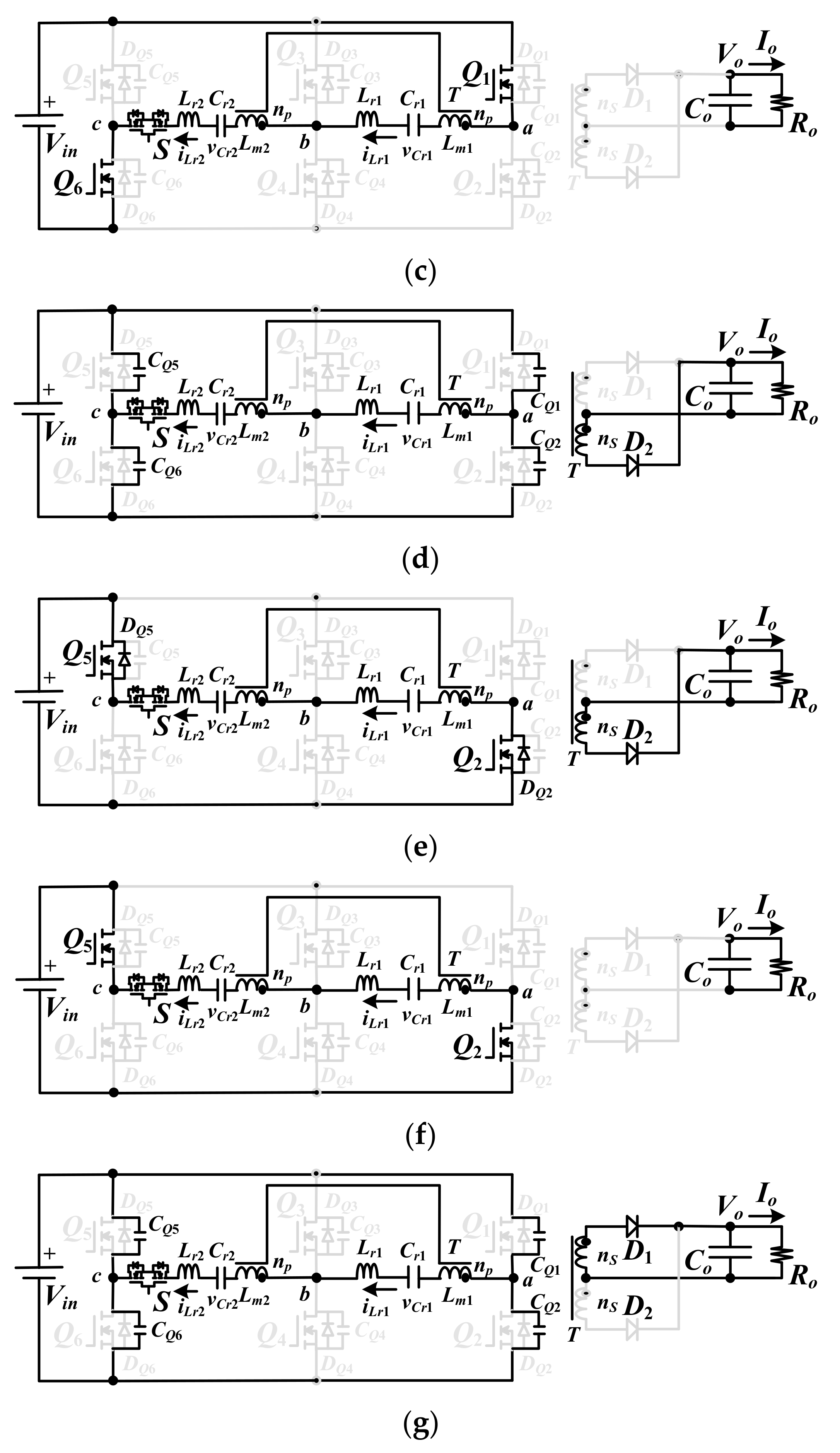
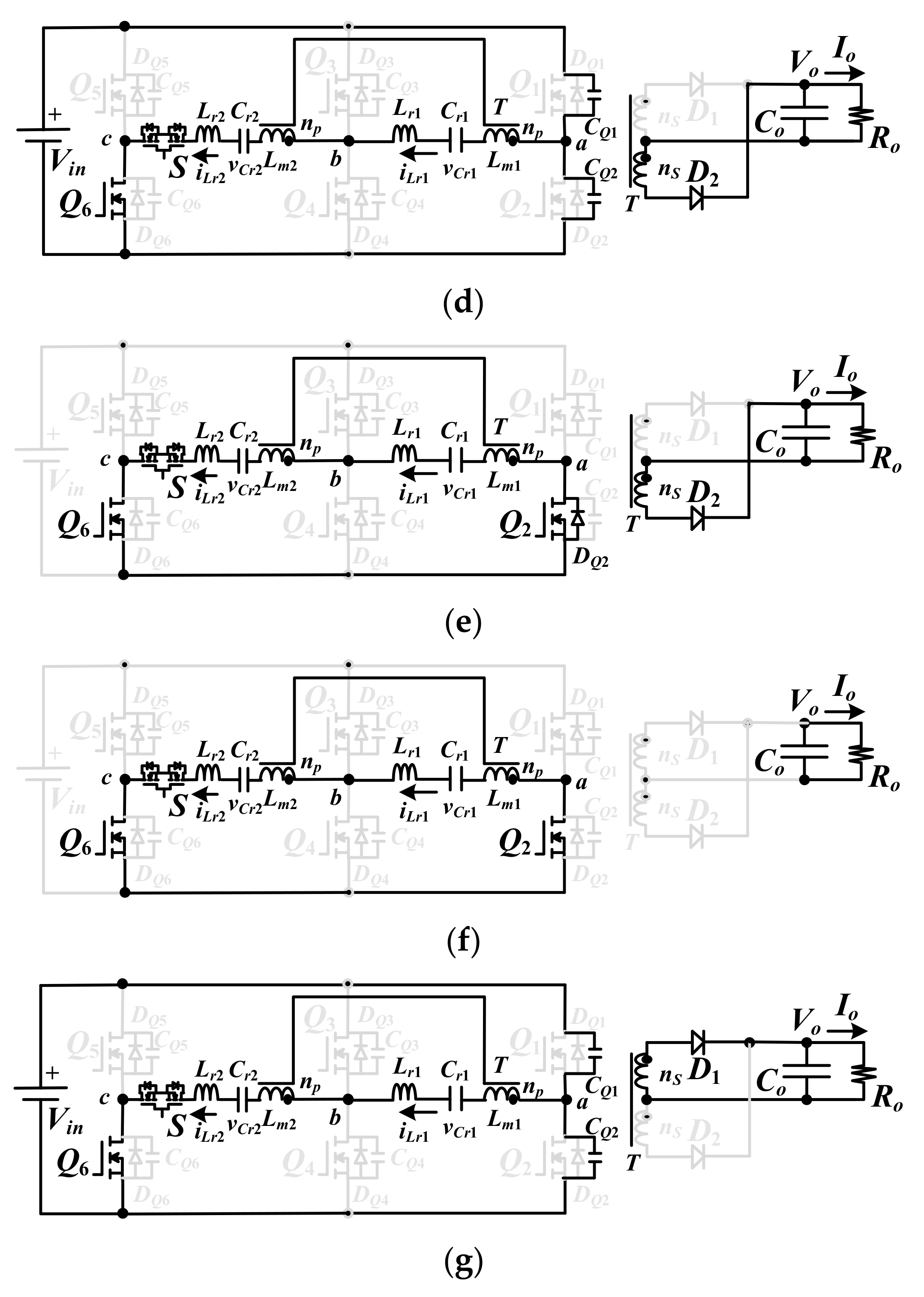
2. Circuit Structure and Principle of Operation
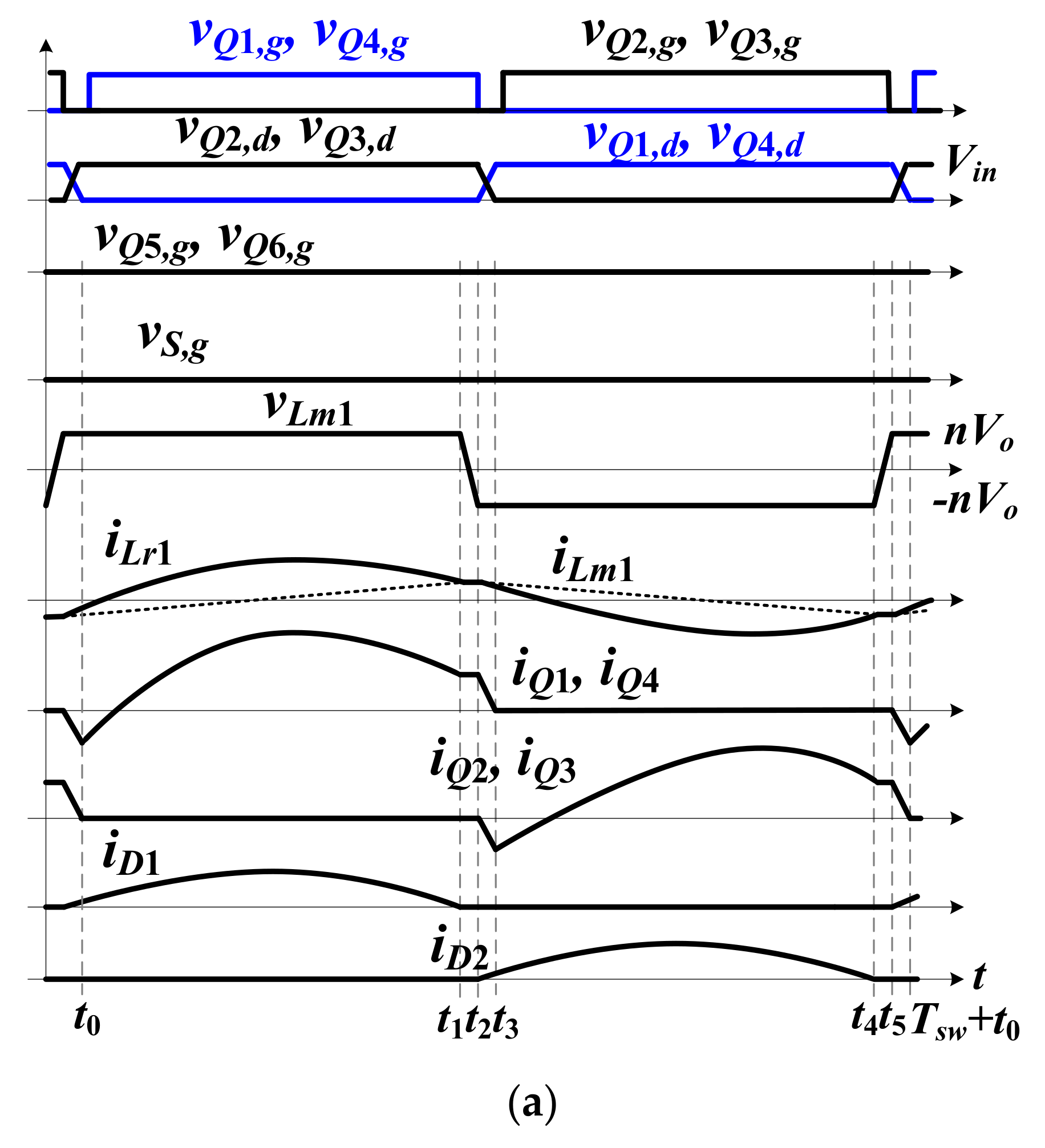
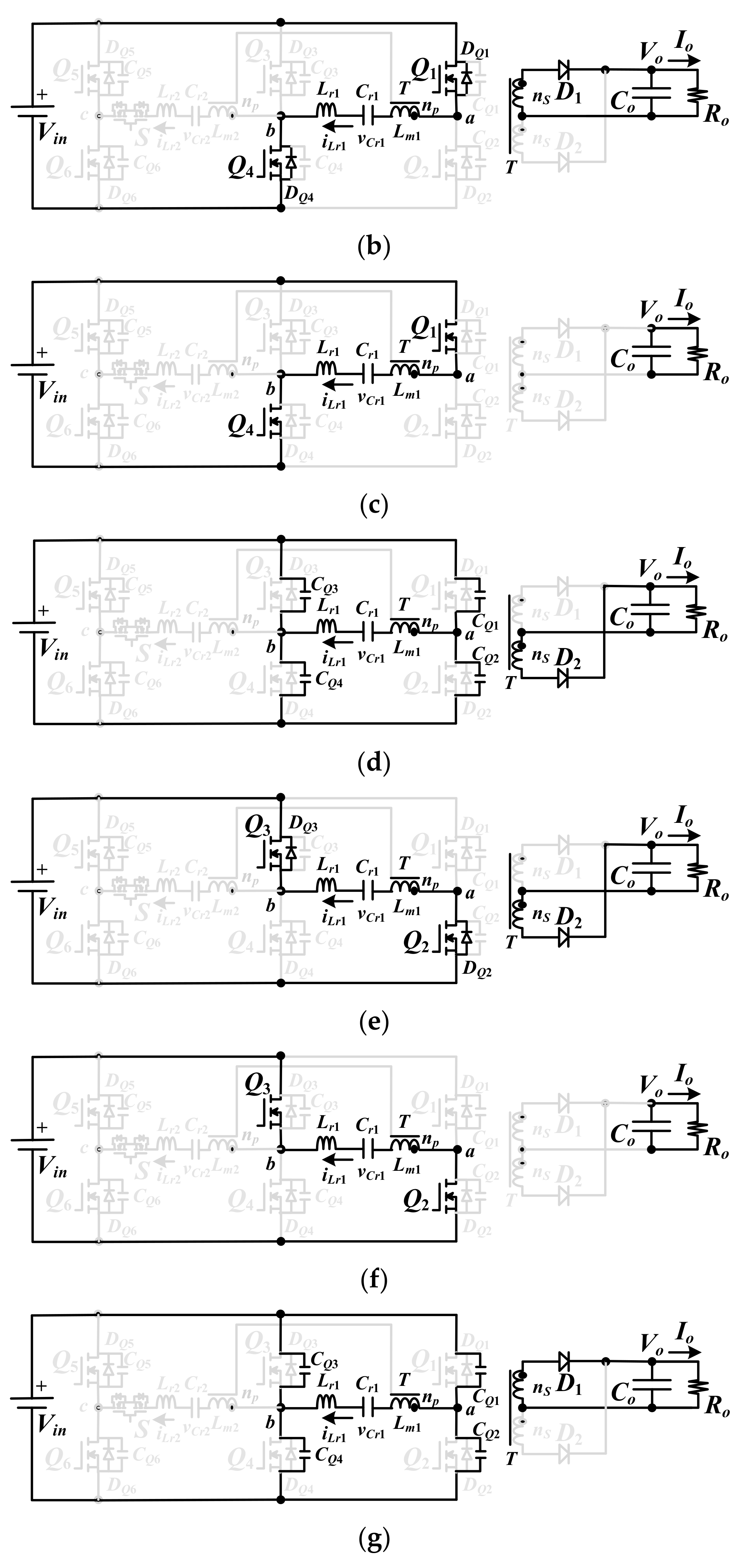
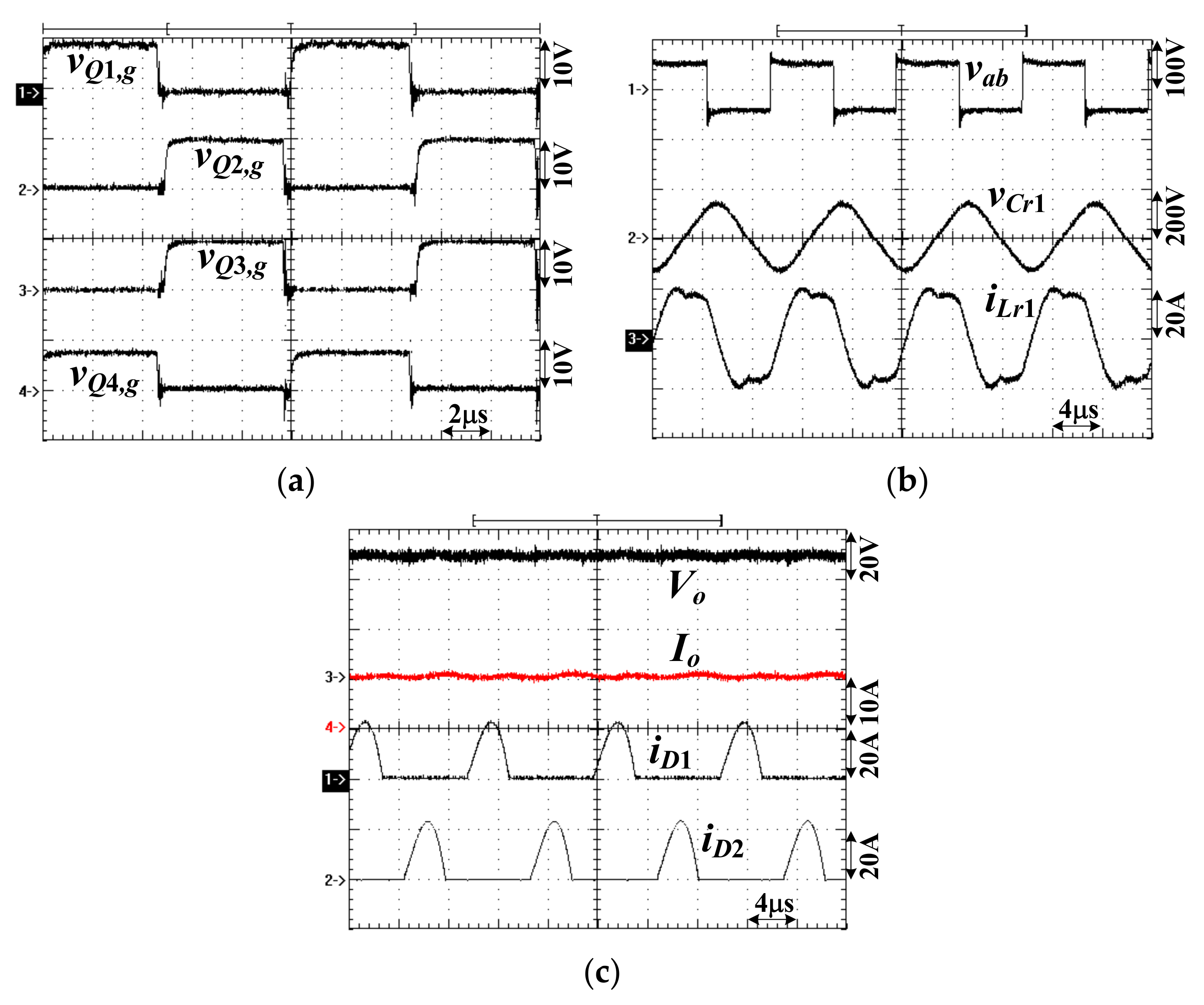
2.1. Low Input Voltage Range (S, Q5, and Q6 off, Vin,L = Vin,max/8–Vin,max/4)
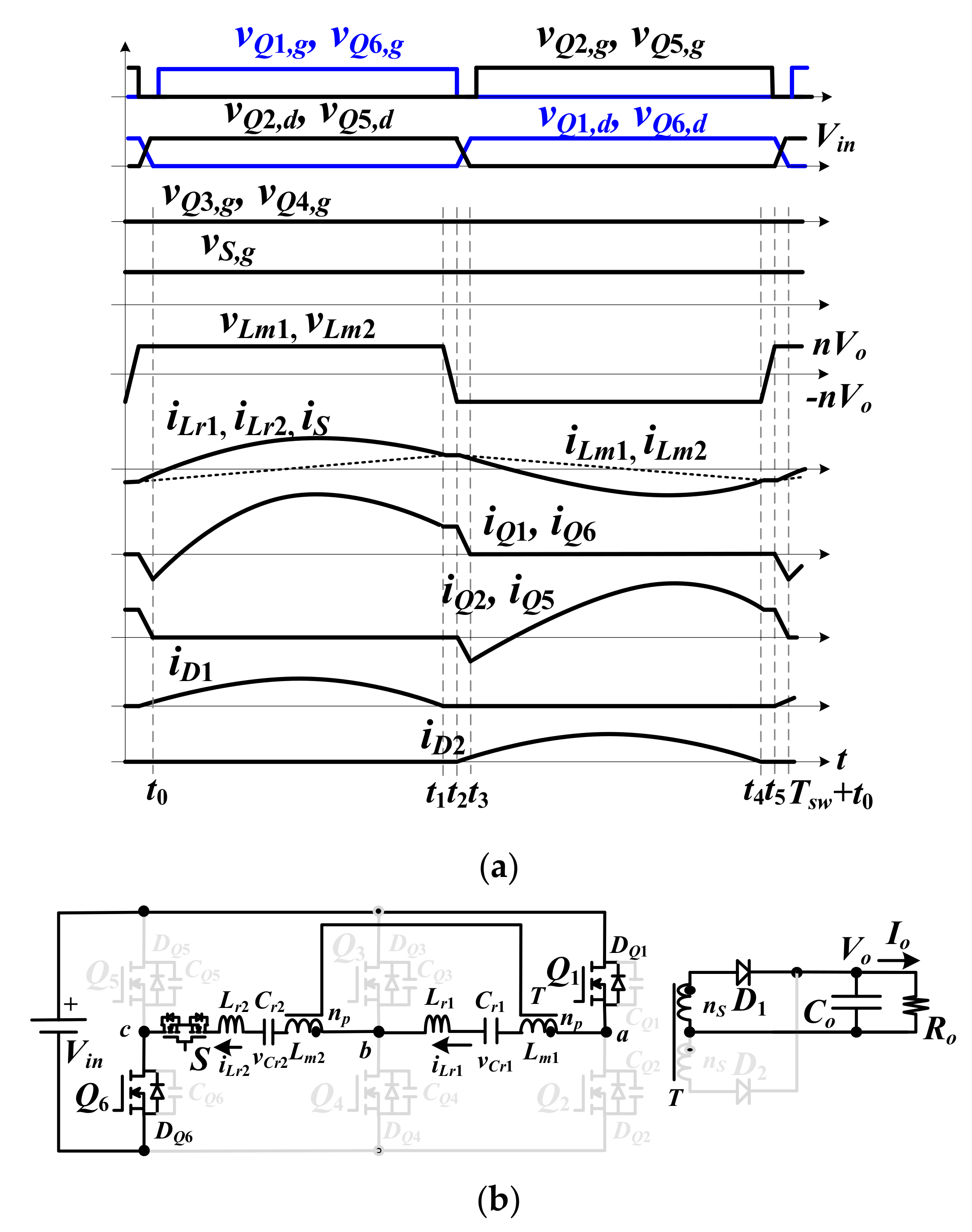
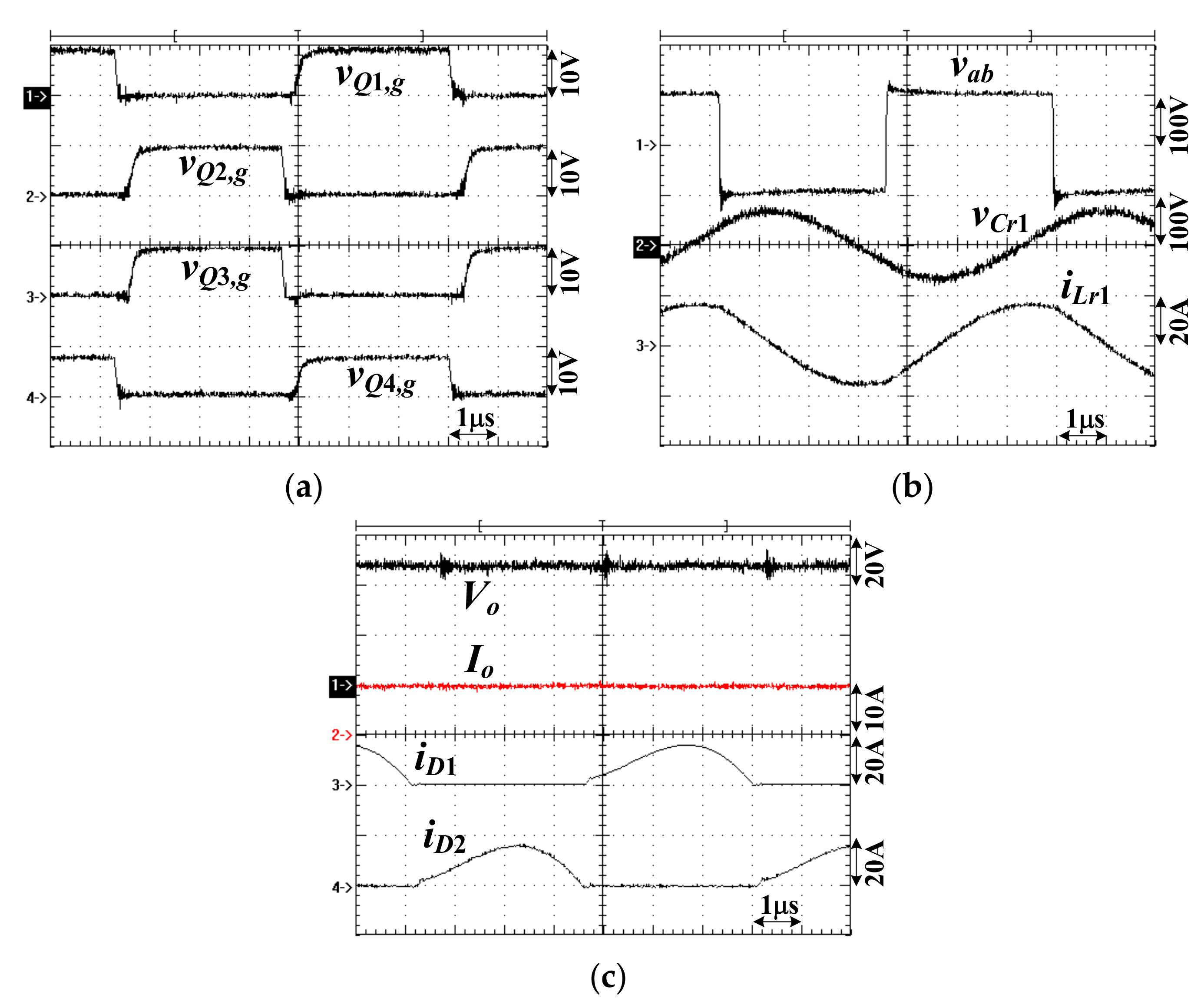
2.2. Medium Input Voltage Range (Q3 and Q4 off, Vin,M = Vin,max/4–Vin,max/2)
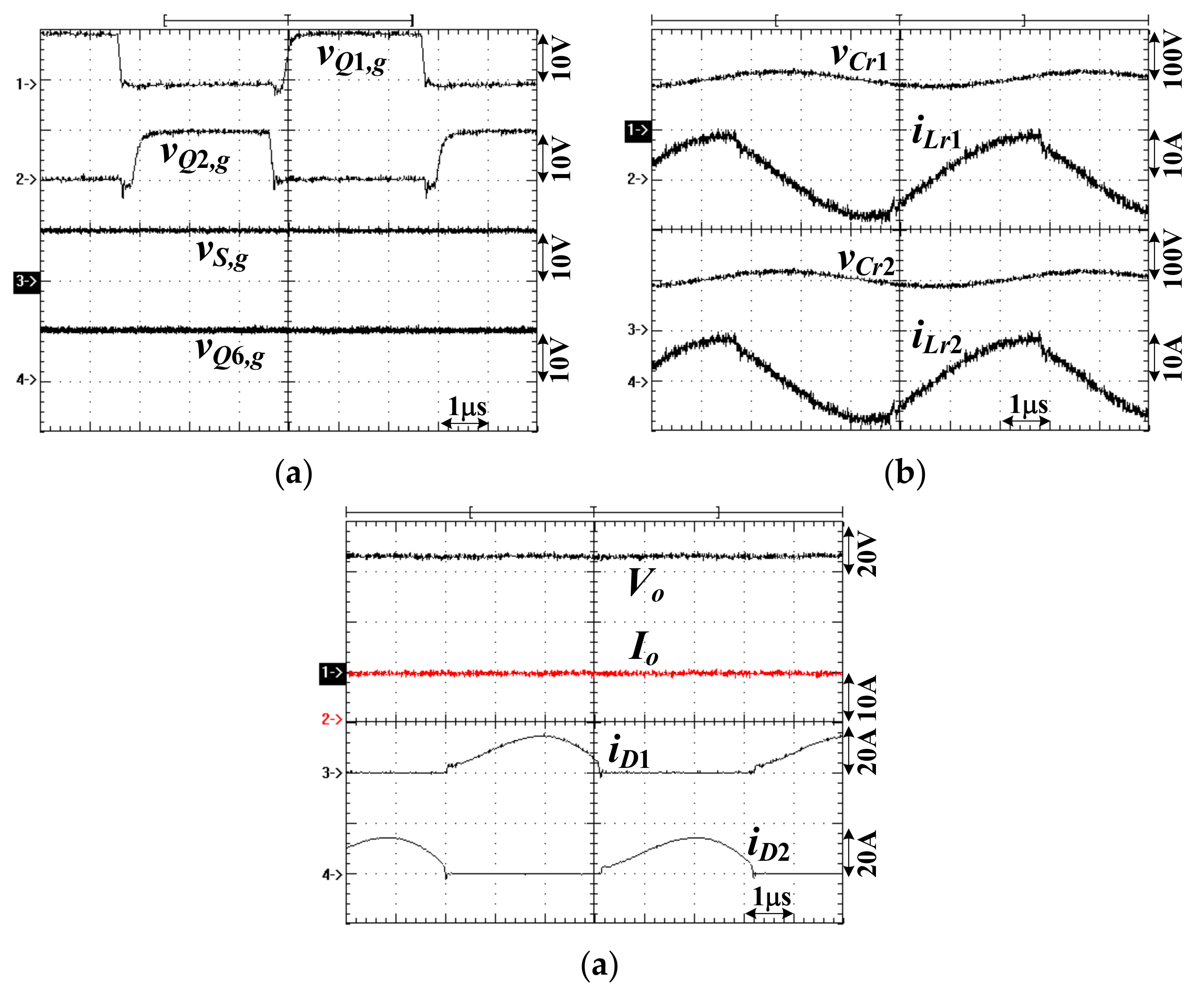
2.3. High Input Voltage Range (Q3–Q4 off, Vin,H = Vin,max/2–Vin,max)
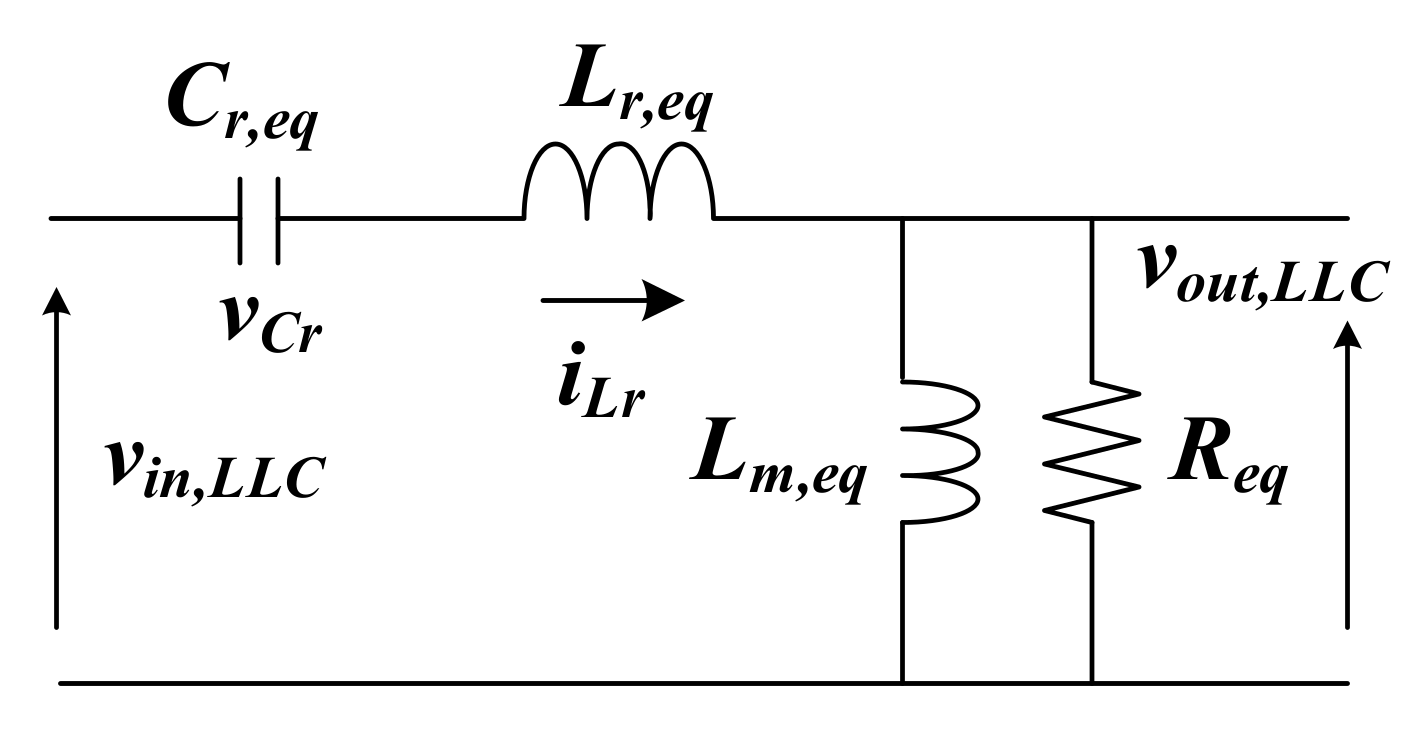
3. Circuit Characteristics
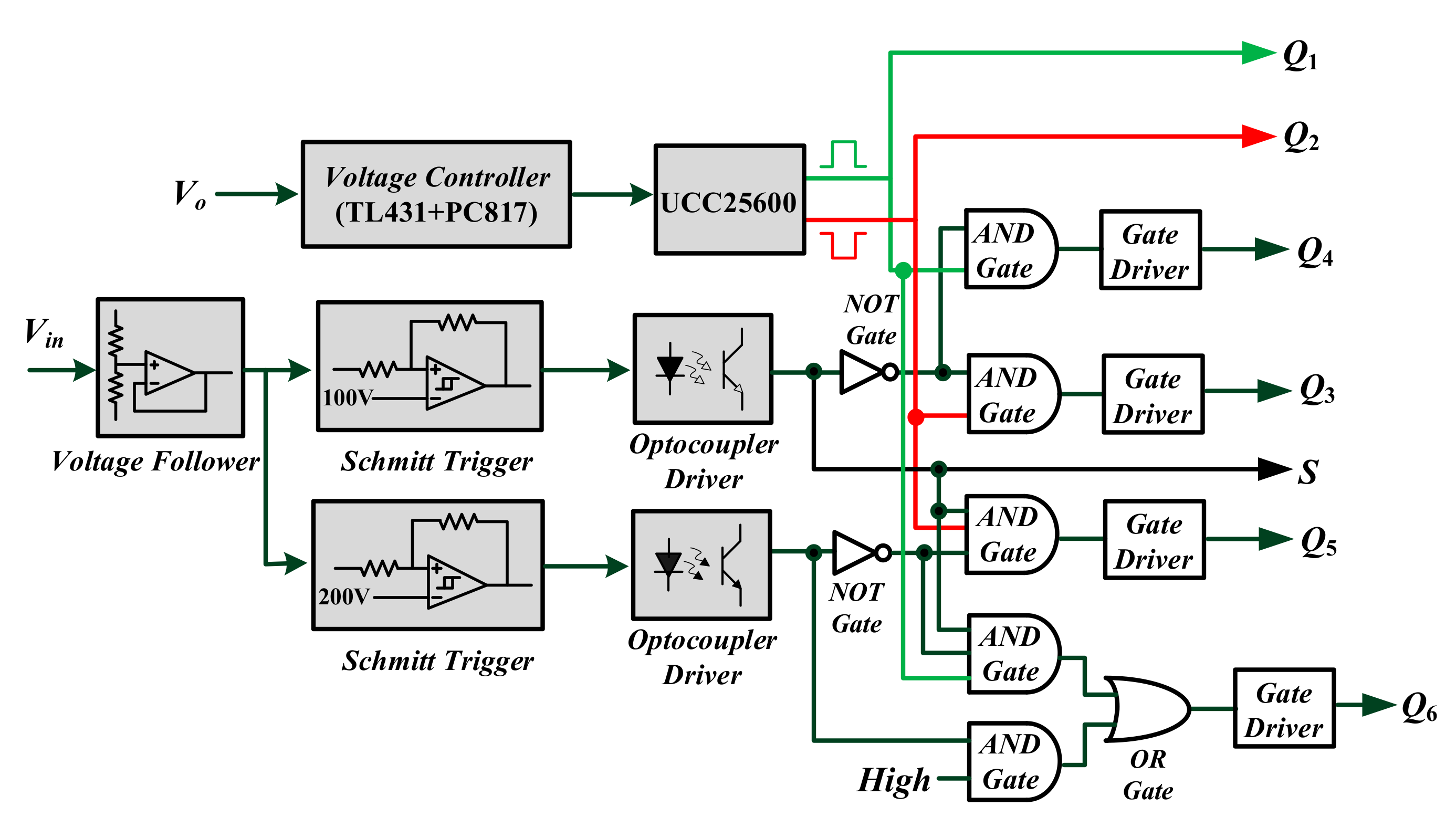
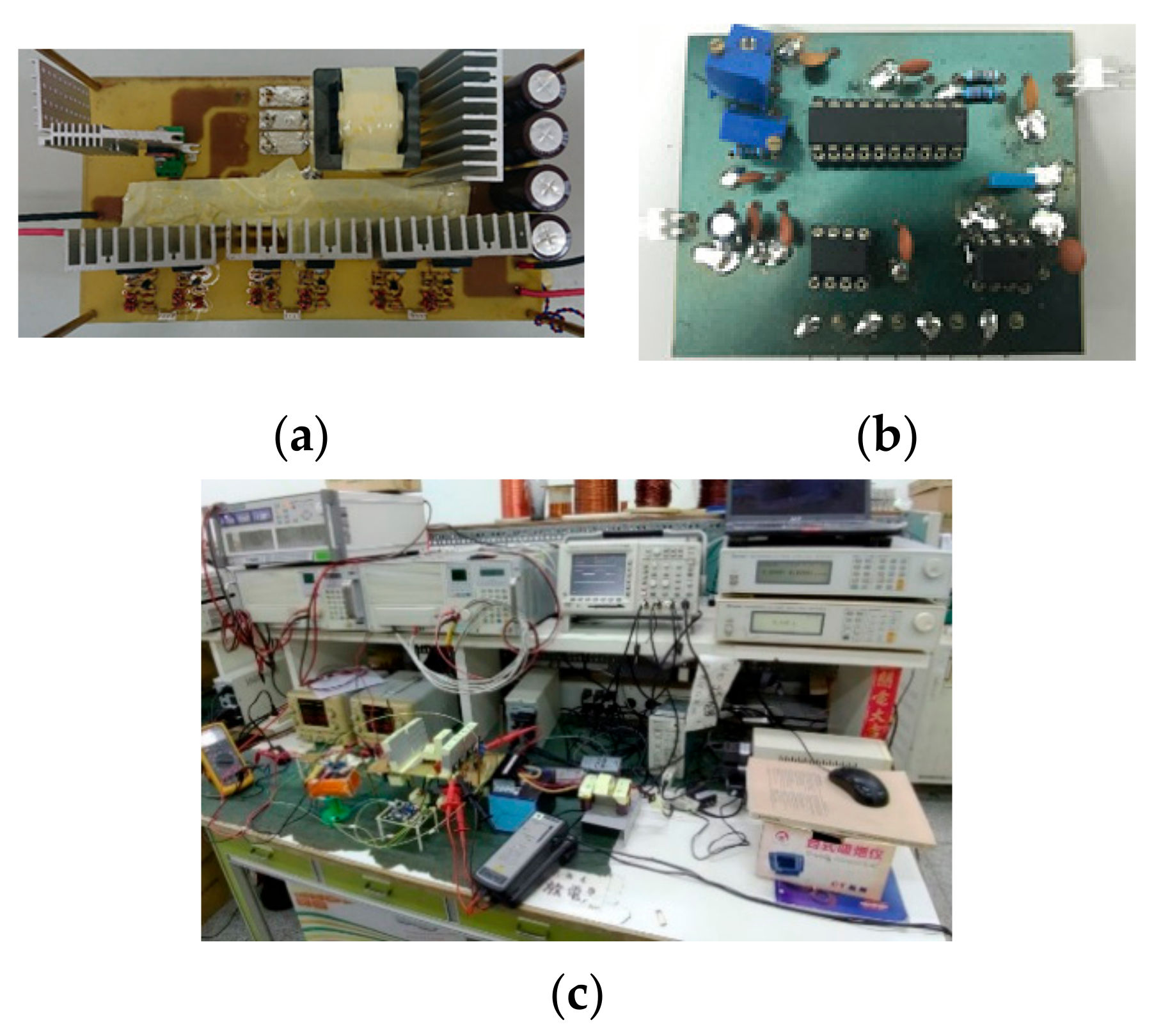
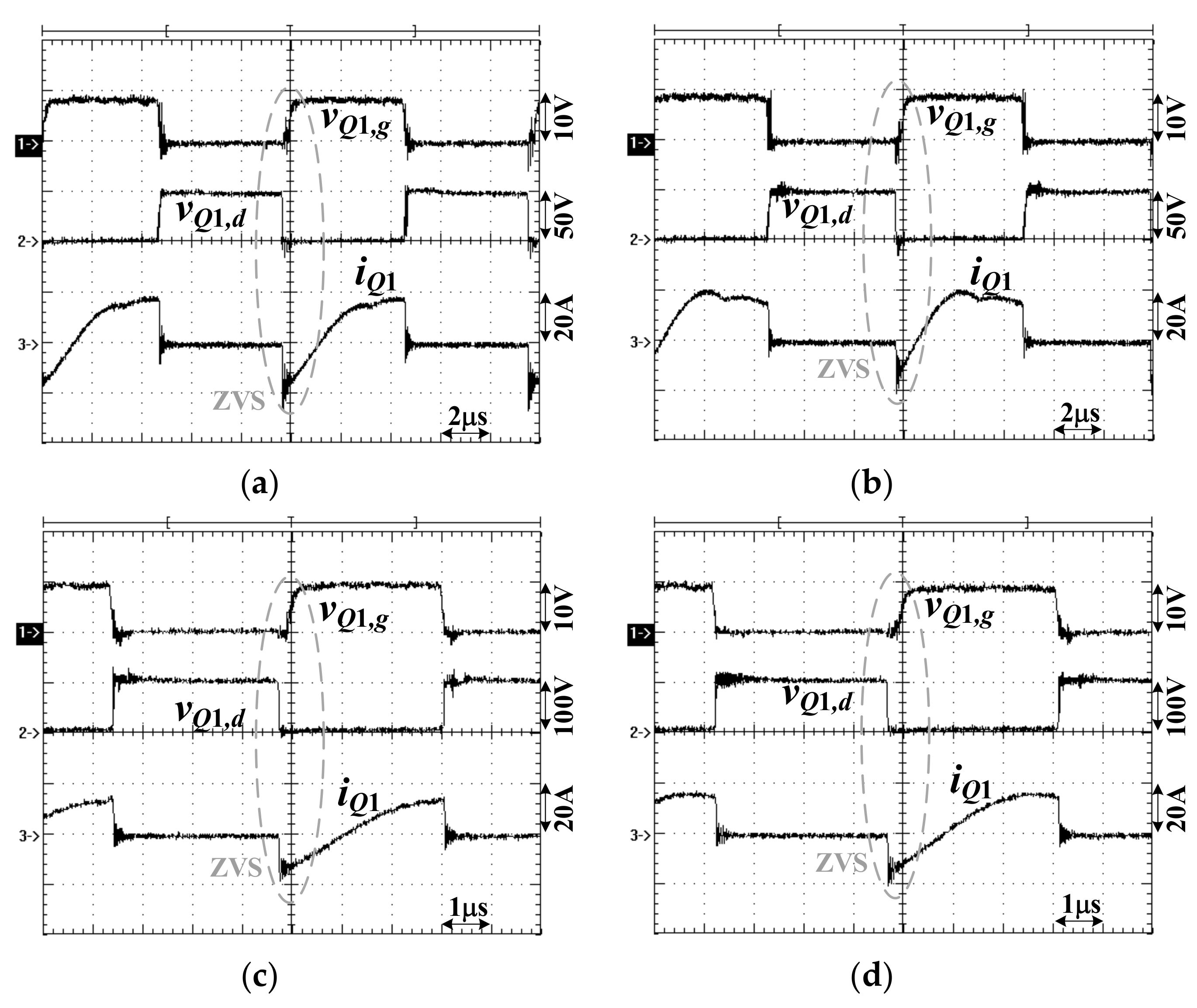
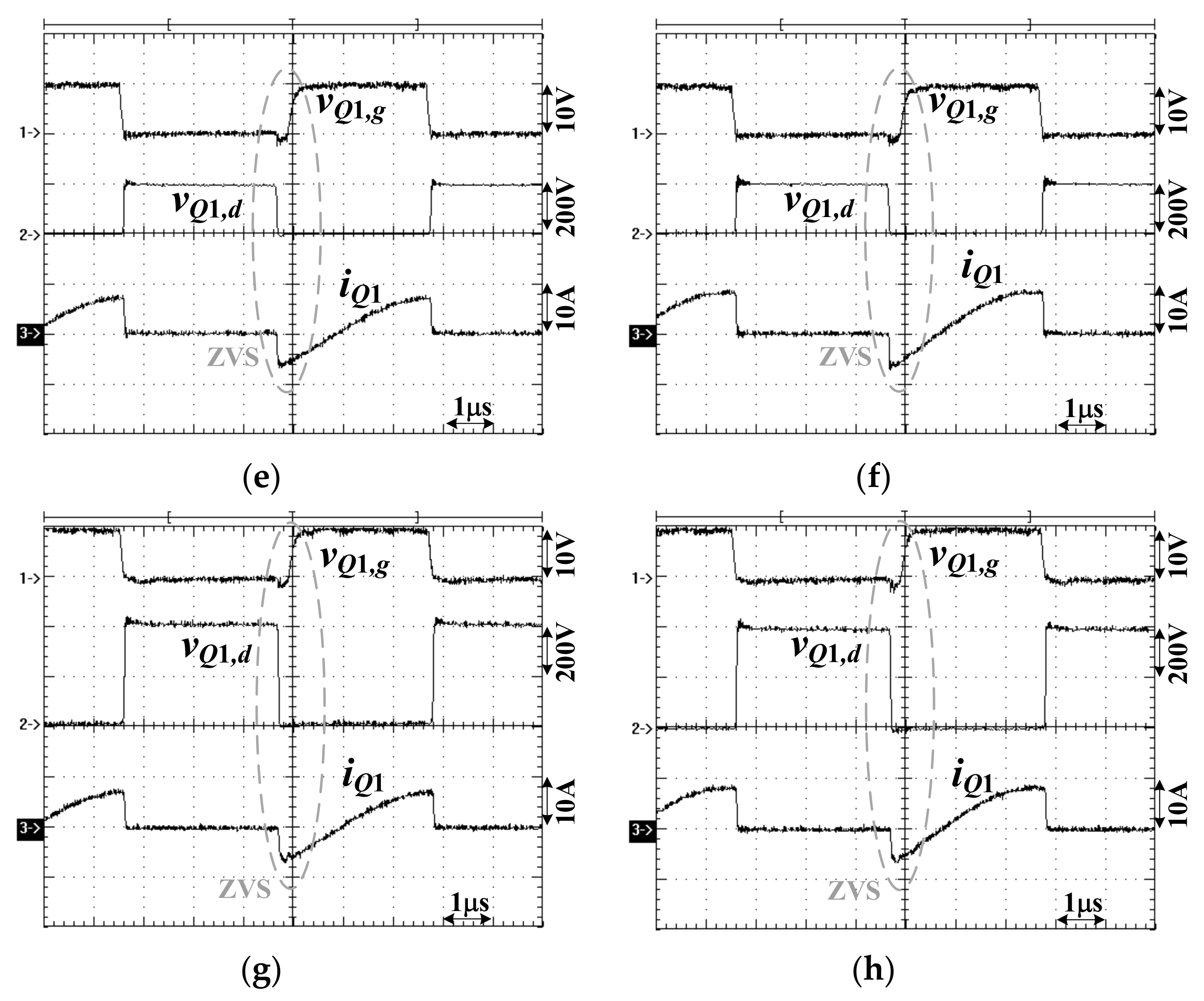
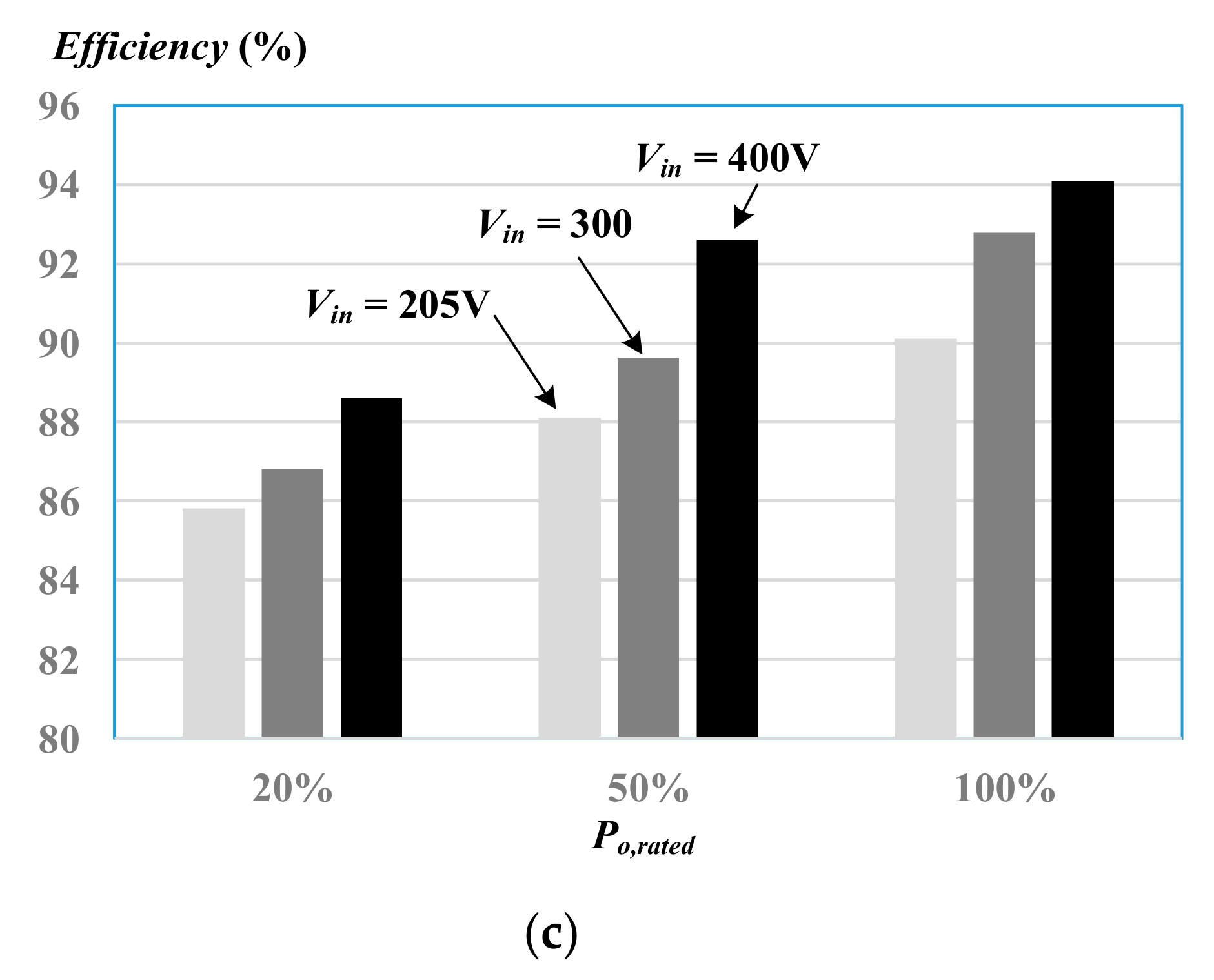
4. Design Procedures and Test Results
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Liu, P.J.; Hsu, Y.C.; Hsu, S.R. Drain-voltage balance and phase-shifted PWM control schemes for high-efficiency parallel-string dimmable LED drivers. IEEE Trans. Ind. Electron. 2018, 65, 6168–6176. [Google Scholar] [CrossRef]
- Safaee, A.; Jain, P.; Bakhshai, A. A ZVS pulsewidth modulation full-bridge converter with a low-RMS-current resonant auxiliary circuit. IEEE Trans. Power Electron. 2016, 31, 4031–4047. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, S.; Guan, Y.; Liu, X.; Xu, D. Single-stage QR ac-dc converter based on buck-boost and flyback circuits. IET Power Electron. 2017, 10, 103–111. [Google Scholar] [CrossRef]
- Xie, X.; Li, J.; Peng, K.; Zhao, C.; Lu, Q. Study on the single-stage forward-flyback PFC converter with QR control. IEEE Trans. Power Electron. 2016, 31, 430–442. [Google Scholar] [CrossRef]
- Lin, B.R.; Chiang, H.K.; Chen, C.C. Analysis and implementation of a zvs-pwm converter with series-connected transformers. IEEE Trans. Circuits Syst. II 2007, 54, 917–921. [Google Scholar] [CrossRef]
- Lin, B.R.; Lin, Y. Parallel current-fed resonant converter with balance current sharing and no input ripple current. IET Power Electron. 2019, 12, 212–219. [Google Scholar] [CrossRef]
- Haga, H.; Kurokawa, F. Modulation method of a full-bridge three-level LLC resonant converter for battery charger of electric vehicles. IEEE Trans. Power Electron. 2017, 32, 2498–2507. [Google Scholar] [CrossRef]
- Zhang, Y.; Fu, C.; Sumner, M.; Wang, P. A wide input-voltage range quasi-Z-source boost DC–DC converter with high-voltage gain for fuel cell vehicles. IEEE Trans. Ind. Electron. 2018, 65, 5201–5212. [Google Scholar] [CrossRef] [Green Version]
- Yao, Z.; Xu, J. A three-phase DC-DC converter for low and wide input-voltage range application. In Proceedings of the 2016 IEEE Transportation Electrification Conference and Expo, Asia-Pacific (ITEC Asia-Pacific), Busan, Korea, 1–4 June 2016; pp. 208–213. [Google Scholar]
- Li, W.; Zong, S.; Liu, F.; Yang, H.; He, X.; Wu, B. Secondary-side phase-shift-controlled ZVS DC/DC converter with wide voltage gain for high input voltage applications. IEEE Trans. Power Electron. 2013, 28, 5128–5139. [Google Scholar] [CrossRef]
- Lu, J.; Kumar, A.; Afridi, K.K. Step-down impedance control network resonant DC-DC converter utilizing an enhanced phase-shift control for wide-input-range operation. IEEE Trans. Ind. Appl. 2018, 54, 4523–4536. [Google Scholar] [CrossRef]
- Wu, H.; Wan, C.; Sun, K.; Xing, Y. A high step-down multiple output converter with wide input voltage range based on quasi two-stage architecture and dual-output LLC resonant converter. IEEE Trans. Power Electron. 2015, 30, 1793–1796. [Google Scholar] [CrossRef]
- Hu, H.; Fang, X.; Chen, F.; Shen, Z.J.; Batarseh, I. A modified high-efficiency LLC converter with two transformers for wide input-voltage range applications. IEEE Trans. Power Electron. 2012, 28, 1946–1960. [Google Scholar] [CrossRef]
- Shang, C.; Liu, L.; Liu, M.; Men, S. A highly-efficient two-stage DC-DC converter with wide input voltage. In Proceedings of the 2015 IEEE International Telecommunications Energy Conference (INTELEC), Osaka, Japan, 18–22 October 2015; pp. 1–6. [Google Scholar]
- Zhou, G.; Ruan, X.; Wang, X. Input voltage feed-forward control strategy for cascaded DC/DC converters with wide input voltage range. In Proceedings of the 2016 IEEE 8th International Power Electronics and Motion Control Conference (IPEMC-ECCE Asia), Hefei, China, 22–26 May 2016; pp. 603–608. [Google Scholar]
- Jeong, Y.; Kim, J.K.; Lee, J.B.; Moon, G.W. An asymmetric half-bridge resonant converter having a reduced conduction loss for DC/DC power applications with a wide range of low input voltage. IEEE Trans. Power Electron. 2017, 32, 7795–7804. [Google Scholar] [CrossRef]
- Wang, P.; Zhou, L.; Zhang, Y.; Li, J.; Sumner, M. Input-parallel output-series DC-DC boost converter with a wide input voltage range, for fuel cell vehicles. IEEE Trans. Veh. Technol. 2017, 66, 7771–7781. [Google Scholar] [CrossRef]
- Kim, B.; Kim, S.; Huh, D.Y.; Choi, J.H.; Kim, M. Hybrid resonant half-bridge DC/DC converter with wide input voltage range. In Proceedings of the 2018 IEEE Applied Power Electronics Conference and Exposition (APEC), San Antonio, TX, USA, 4–8 March 2018; pp. 1876–1881. [Google Scholar]
- Singh, A.K.; Das, P.; Panda, S.K. Analysis and design of SQR-based high-voltage LLC resonant dc-dc converter. IEEE Trans. Power Electron. 2017, 32, 4466–4481. [Google Scholar] [CrossRef]
- Sun, W.; Xing, Y.; Wu, H.; Ding, J. Modified high-efficiency LLC converters with two split resonant branches for wide input-voltage range applications. IEEE Trans. Power Electron. 2018, 33, 7867–7870. [Google Scholar] [CrossRef]
- Jovanović, M.M.; Irving, B.T. On the fly topology-morphing control efficiency optimization method for LLC resonant converters operating in wide input and/or output-voltage range. IEEE Trans. Power Electron. 2016, 31, 2596–2608. [Google Scholar] [CrossRef]
- Steigerwald, R.L. A comparison of half-bridge resonant converter topologies. IEEE Trans. Power Electron. 1988, 3, 174–182. [Google Scholar] [CrossRef]








| Items | Symbol | Parameter |
|---|---|---|
| Input voltage | Vin | 50–400 V |
| Output voltage | Vo | 48 V |
| Rated load current | Io | 10 A |
| Series resonant frequency | fr | 150 kHz |
| Output capacitor | Co | 1080 μF (1080 μF/100 V) |
| Resonant capacitors | Cr1, Cr2 | 273 nF |
| Power MOSFETs | Q1–Q6, S | Infineon-6R070P6 |
| Resonant inductors | Lr1, Lr2 | 4.13 μH |
| Rectifier diodes | D1, D2 | PS30M100SFP |
| Primary and secondary turns of T | np, ns | 8 turns, 4 turns |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lin, B.-R.; Zhuang, Y.-S. Hybrid Resonant Converter with Three Half-Bridge Legs for Wide Voltage Operation. Appl. Sci. 2020, 10, 310. https://doi.org/10.3390/app10010310
Lin B-R, Zhuang Y-S. Hybrid Resonant Converter with Three Half-Bridge Legs for Wide Voltage Operation. Applied Sciences. 2020; 10(1):310. https://doi.org/10.3390/app10010310
Chicago/Turabian StyleLin, Bor-Ren, and Yong-Sheng Zhuang. 2020. "Hybrid Resonant Converter with Three Half-Bridge Legs for Wide Voltage Operation" Applied Sciences 10, no. 1: 310. https://doi.org/10.3390/app10010310
APA StyleLin, B.-R., & Zhuang, Y.-S. (2020). Hybrid Resonant Converter with Three Half-Bridge Legs for Wide Voltage Operation. Applied Sciences, 10(1), 310. https://doi.org/10.3390/app10010310





