Concrete Silos: Failures, Design Issues and Repair/Strengthening Methods
Abstract
:1. Introduction
2. Failure of Reinforced Concrete Silos
2.1. Material-Related Factors
2.2. Material Variability and Loading/Unloading Factors
2.3. Materials for Reinforcing Concrete
- The design and material selection process should take into account the climate-induced thermal effects (such as solar radiation and air temperature), which impact temperature distribution and silo operating conditions.
- The engineers should define the uniform temperature component in line with the ambient air temperature (operating temperature).
- Solar gain-based stepped temperature component should be estimated at 15 °C unless otherwise stated.
- The differences between the inside fillings and the ambient air are considered as the difference between the inner and the outer surfaces, after accommodating the impact of insulation.
- The temperature-related estimates and assumptions should be based on hot summer days (to accurately determine the effect of solar gains), and an empty silo.
Impact of Silo Materials on Quality of Stored Grains and Reverse Effects
2.4. Reverse Effects of the Stored Bulk Solids—Corrosion, Thermal Gradient, and Differentials
2.5. Material Design Factors and the Failure of Reinforced Concrete Structures
Impact of Bulk Grain Abrasion on the Hopper Wall Materials
2.6. Failure Due to Foundation/Soil Factors
Soil Effects
3. Design Issues in Reinforced Concrete Silos
3.1. Design Factors
3.1.1. Silo Design Codes
3.1.2. Silo Design Geometry
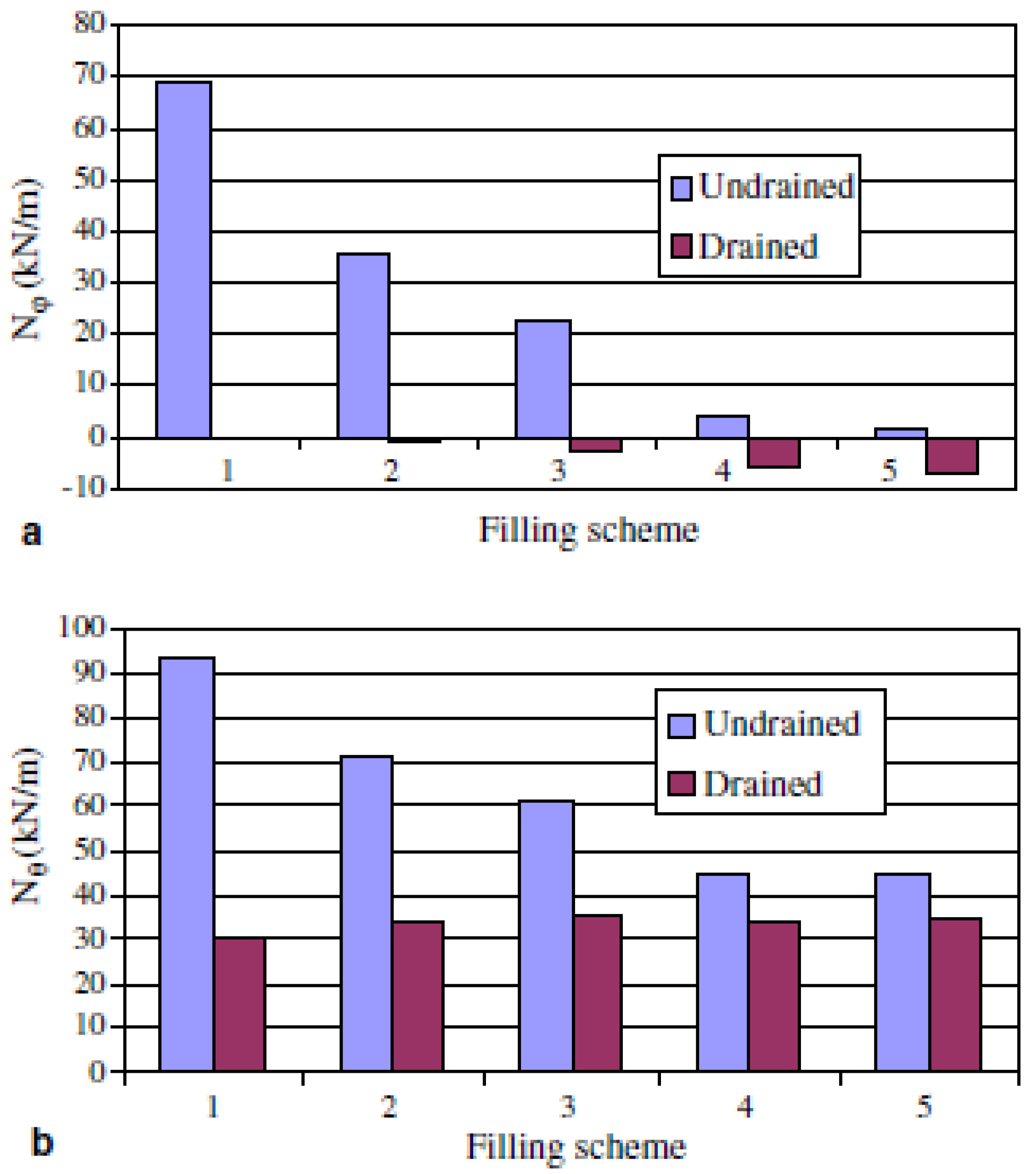
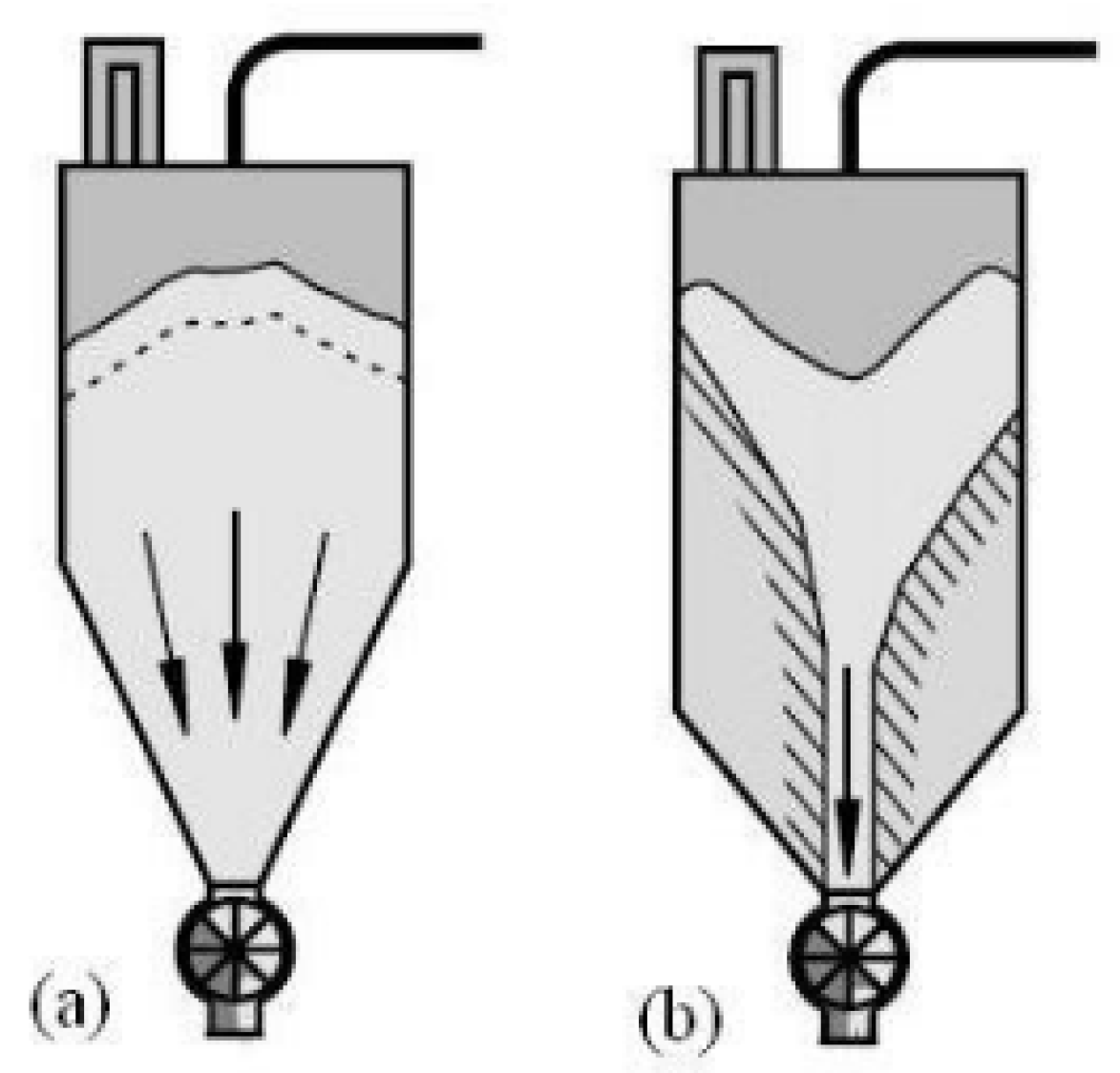
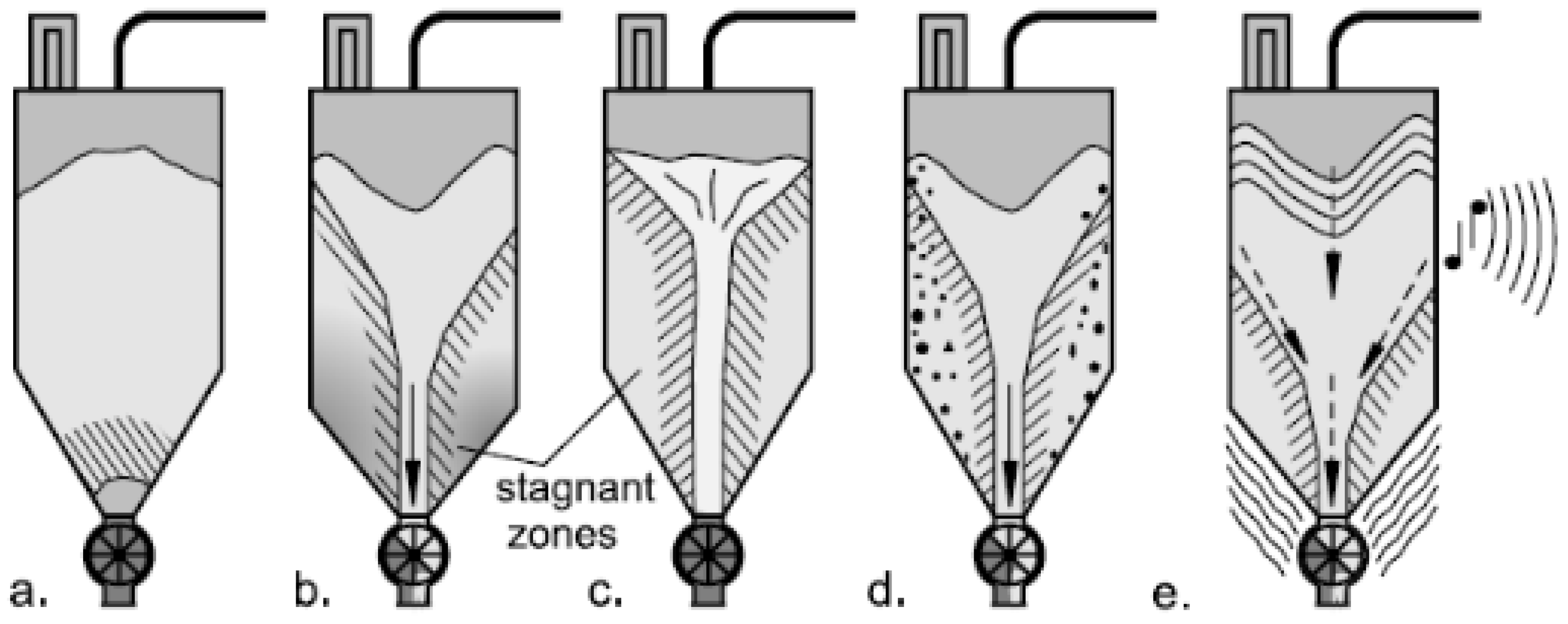
3.1.3. Mass Flow and Funnel Flow Design Issues
3.1.4. Seismic Activity and Other Natural Phenomenon
4. Repair and Strengthening Methods for Reinforced Concrete Silos
4.1. Repair of RC Silos
Equation of Silo Quaking
4.2. Strengthening of RC Silos
4.2.1. Distributed Tuned Mass Dampers (D-TMDs) and Distributed Tuned Vibration Absorbers Vertically (D-MTVAs)
4.2.2. External Pre-Stressing for Repair/Strengthening
4.2.3. RC Concrete Repair Using FRP Materials
4.3. Durability Improvement and Corrosion Protection
5. Conclusions
Funding
Conflicts of Interest
References
- Carson, J.; Craig, D. Silo design codes: Their limits and inconsistencies. Procedia Eng. 2015, 102, 647–656. [Google Scholar] [CrossRef] [Green Version]
- Maj, M. Some Causes of Reinforced Concrete Silos Failure. Procedia Eng. 2017, 172, 685–691. [Google Scholar] [CrossRef]
- Elghazouli, A.Y.; Rotter, J.M. Long-term performance and assessment of circular reinforced concrete silos. Constr. Build. Mater. 1996, 10, 117–122. [Google Scholar] [CrossRef]
- Grigoriadis, K. Use of laser interferometry for measuring concrete substrate roughness in patch repairs. Autom. Constr. 2016, 64, 27–35. [Google Scholar] [CrossRef] [Green Version]
- Razl, I. Flexible Polymer-Cement Repair Materials and Their Applications. Repair Rehab Polym. Cem. 2012, 98–104. [Google Scholar]
- Foster, A.; Atkins, C.; Buckley, L. Preserving reinforced concrete. WIT Trans. Built Environ. 2007, 95, 363–371. [Google Scholar]
- Carson, J. Silo Failures: Case Histories and Lessons Learnt. Handb. Powder. Technol. 2003, 1–15. [Google Scholar]
- Revenet, J. Silo Problems. Silo. Bins Bunkers 1981, 1, 667–679. [Google Scholar]
- Xie, Y. Structural Behavior of Grain Bin Steel Silo; University of Windsor: Windsor, ON, Canada, 2015. [Google Scholar]
- Lapko, A.; Gnatowski, M.; Prusiel, J.A. Analysis of some effects caused by interaction between bulk solid and r.c. silo wall structure. Powder Technol. 2003, 133, 44–53. [Google Scholar] [CrossRef]
- Carson, J.W.; Holmes, T. Silo Failures: Why Do They Happen? Task, Q. 2003, 7, 499–512. [Google Scholar]
- Yuan, Y.C.; Yin, T.; Rong, M.Z.; Zhang, M.Q. Self healing in polymers and polymer composites. Concepts, realization and outlook: A review. Express Polym. Lett. 2008, 2, 238–250. [Google Scholar] [CrossRef]
- Galao, O.; Bañón, L.; Baeza, F.J.; Carmona, J.; Garcés, P. Highly Conductive Carbon Fiber Reinforced Concrete for Icing Prevention and Curing. Materials (Basel) 2016, 9, 281. [Google Scholar] [CrossRef] [Green Version]
- Luckachan, G.E.; Pillai, C.K.S. Biodegradable Polymers—A Review on Recent Trends and Emerging Perspectives. J. Polym. Environ. 2011, 19, 637–676. [Google Scholar] [CrossRef]
- Bagherpour, S. Fibre Reinforced Polyester Composites (Composition Perfect). Polyester 2012, 135–166. [Google Scholar] [CrossRef] [Green Version]
- Khanam, N.; Khalil, A.; Reddy, R.; Ramachandra, G.; Salima, N. Tensile, Flexural and Chemical Resistance Properties of Sisal Fibre Reinforced Polymer Composites: Effect of Fibre Surface Treatment. J. Polym. Environ. 2011, 19, 115–119. [Google Scholar] [CrossRef]
- Farzadnia, N.; Hessam, S.; Asadi, A.; Hosseini, S. Mechanical and microstructural properties of cement pastes with rice husk ash coated with carbon nanofibers using a natural polymer binder. Constr. Build. Mater. 2018, 175, 691–704. [Google Scholar] [CrossRef]
- Archila, H.; Kaminski, S.; Trujillo, D.; Zea Escamilla, E.; Harries, K.A. Bamboo reinforced concrete: A critical review. Mater. Struct. Constr. 2018, 51, 1–18. [Google Scholar] [CrossRef]
- Saba, N.; Tahir, P.M.; Jawaid, M. A review on potentiality of nano filler/natural fiber filled polymer hybrid composites. Polymers (Basel) 2014, 6, 2247–2273. [Google Scholar] [CrossRef]
- Deng, L.; Eichhorn, S.J.; Kao, C.-C.; Young, R.J. The Effective Young’s Modulus of Carbon Nanotubes in Composites. ACS Appl. Mater. Interfaces. 2011, 3, 433–440. [Google Scholar] [CrossRef]
- Parra, C.; Valcuende, M.; Gómez, F. Splitting tensile strength and modulus of elasticity of self-compacting concrete. Constr. Build. Mater. 2011, 25, 201–207. [Google Scholar] [CrossRef]
- Nielsen, J. From silo phenomena to load models. In Proceedings of the International Conference on Structures and Granular Solids, The Royal Society of Edinburgh; Taylor & Francis: Edinburgh, EDN, UK, 2008; pp. 49–57. [Google Scholar]
- Rotter, J.M. Background Discussion Document for EN 1991-4. Available online: https://www.eurocodes.fi/wp-content/uploads/1991/1991-4/Background_to_prEN_1991-4_version_15_2002-04.pdf (accessed on 12 February 2020).
- Fank, M.Z.; Nascimento, J.W.B.; Cardoso, D.L.; Meira, A.S.; Willrich, F.L. Vertical Pressures and Compressive Friction Force in a Large Silo. Eng. Agrícola Jaboticabal. 2018, 38, 498–503. [Google Scholar] [CrossRef]
- Aydin, F. Experimental Investigation of Thermal Expansion and Concrete Strength Effects on FRP Bars Behavior Embedded in Concrete Experimental investigation of thermal expansion and concrete strength effects on FRP bars behavior embedded in concrete. Constr. Build. Mater. 2018, 163, 1–8. [Google Scholar] [CrossRef]
- Sadler, J.E.; Johnston, F.T.; Mahmoud, M.H. Designing Silo Walls for Flow Patterns. ACI Struct. J. 1995, 219–228. [Google Scholar]
- Jayachandran, L.E.; Nitin, B.; Srinivasa, P. Simulation of the stress regime during grain filling in bamboo reinforced concrete silo. J. Stored Prod. Res. 2019, 83, 123–129. [Google Scholar] [CrossRef]
- Poursaee, A. Corrosion sensing for assessing and monitoring civil infrastructures. Sens. Technol. Civl Infrastruct. 2014, 357–382. [Google Scholar]
- Rodrigo, A.C.; José, P.L.N.; Marcilene, V.; José, W.B.; Jefferson, H.G. Fiber-reinforced concrete for the flat bottom of silos. Rev. Bras. Eng. Agríc. Ambient. 2020, 24, 274–279. [Google Scholar]
- Topçuoğlu, K.; Ünal, H.B. The use of ferrocement in the construction of squat grain silos. Comput. Concr. 2016, 18, 53–68. [Google Scholar] [CrossRef]
- Eldho, C.A.; Jones, S. Performance of Concrete Patch Repairs: From a Durability Point of View. In Proceedings of the 5th International Conference on Durability of Concrete Structures, Shenzhen, Guangdong, China, 30 June–1 July 2016; pp. 66–72. [Google Scholar]
- Law, D.W.; Holden, L.; Silcock, D. The assessment of crack development in concrete using a terrestrial laser scanner (TLS). Aust. J. Civ. Eng. 2015, 13, 22–31. [Google Scholar]
- Banville, M.H. Assessment and repair of concrete structures. Interface 2008, 26–34. [Google Scholar]
- Mujinya, B.B.; Mees, F.; Erens, H.; Dumon, M.; Baert, G.; Boeckx, P.; Ngongo, M.; Van Ranst, E. Clay composition and properties in termite mounds of the Lubumbashi area, D.R. Congo. Geoderma 2013, 192, 304–315. [Google Scholar] [CrossRef]
- Omobowale, M.; Mijinyawa, Y.; Armstrong, P.; Igbeka, J.; Maghirang, E. Performance evaluation of termite-mound clay, concrete and steel silos for the storage of maize grains in the humid tropics. J. Stored Prod. Postharvest Res. 2015, 6, 56–65. [Google Scholar]
- Bywalski, C.; Kamiński, M. A case study of the collapse of the over-chamber reinforced concrete ceiling of a meal silo. Eng. Struct. 2019, 192, 103–112. [Google Scholar] [CrossRef]
- Ooi, J.Y.; Chen, J.F.; Rotter, J.M. Measurement of solids flow patterns in a gypsum silo. Powder Technol. 1998, 99, 272–284. [Google Scholar] [CrossRef]
- Rotter, J.M.; Goodey, R.J.; Brown, C.J. Towards design rules for rectangular silo filling pressures. Eng. Struct. 2019, 198, 109547. [Google Scholar] [CrossRef]
- Abdel-Fattah, M.T.; Moore, I.D.; Abdel-Fattah, T.T. Behaviour of elevated concrete silos filled with saturated solids. Can. J. Civ. Eng. 2006, 33, 227–239. [Google Scholar] [CrossRef]
- Grengg, C.; Florian, M.; Neven, U.; Gìnther, K.; Sabine, K.; Dietzel, M. Advances in concrete materials for sewer systems affected by microbial induced concrete corrosion: A review. Water Res. 2018, 134, 41–352. [Google Scholar] [CrossRef]
- Guan, F.; Zhai, X.; Duan, J.; Zhang, M.; Hou, B. Influence of sulfate-reducing bacteria on the corrosion behavior of high strength steel eq70 under cathodic polarization. PLoS ONE 2016, 11. [Google Scholar] [CrossRef]
- De Belie, N.; Sonck, B.; Braam, C.R.; Lenehan, J.J.; Svennerstedt, B.; Richardson, M. Durability of Building Materials and Components in the Agricultural Environment, Part II: Metal Structures. J. Agric. Eng. Res. 2000, 75, 333–347. [Google Scholar] [CrossRef]
- Nateghi, F.; Yakhchalian, M. Seismic Behavior of Reinforced Concrete Silos Considering Granular Material-Structure Interaction. Procedia Eng. 2011, 14, 3050–3058. [Google Scholar] [CrossRef] [Green Version]
- Matiaskova, L.; Bilcik, J.; Soltesz, J. Failure analysis of reinforced concrete walls of cylindrical silos under elevated temperatures. Eng. Fail. Anal. 2020, 109, 104281. [Google Scholar] [CrossRef]
- Ichim, A.; Teodoriu, C.; Falcone, G. Estimation of cement thermal properties through the three-phase model with application to geothermal wells. Energies 2018, 11, 2839. [Google Scholar] [CrossRef] [Green Version]
- Rowe, R.E.; Guest, J.E. An investigation into the cause of cracking in a reinforced concrete silo containing cement. Mag. Concr. Res. 1960, 12, 40–43. [Google Scholar] [CrossRef]
- Sagarnaga, J.C. Concrete Silo Failures Due to Design Errors. Forensic Eng. 2018. [Google Scholar] [CrossRef]
- Dutta, A.B. Study of Types of Failures in Silos. Glob. Res. Anal. Int. 2013, 2, 41–43. [Google Scholar]
- Horabik, J.; Molenda, M. Properties of Grain for Silo Strength Calculation. Grain. Prop. Silo. Strength Calc. 2002, 195–217. [Google Scholar]
- Sun, S.; Zhao, J.; Zhang, C. Calculation of Silo Wall Pressure considering the Intermediate Stress Effect. Adv. Civ. Eng. 2018, 2018, 1–11. [Google Scholar] [CrossRef]
- Shedd, C.K.; Foster, W.A. “Silo construction,”. Bulletin 2017, 16, 94–148. [Google Scholar]
- Bozozuk, M. Tower Silo Foundations. Canadian Building Digests. 1976. Available online: http://web.mit.edu/parmstr/Public/NRCan/CanBldgDigests/cbd177_e.html. (accessed on 17 May 2020).
- Martins, C.J.; Figueiredo, O.A.; Martins, L.A.; Peixoto, R.A.F. Silo foundation optimization through reinforcement concrete shell structure. Comput. Aided Optim. Des. Eng. 2009, XI, 233–243. [Google Scholar]
- Yuksel, S.B. Design Formulas for the Groups of Six Cylindrical Silos Due to Interstice Loadings. Adv. Struct. Eng. 2011, 14, 265–280. [Google Scholar] [CrossRef]
- TSE-6989. Rules for Design, Construction and Use of Reinforced Concrete Silos; Turkish Standards Institution (TSE): Ankara, Turkey, 1989. [Google Scholar]
- Chen, C. Study on Failure Plane of Bulk Solid in Squat Silos. Open Civ. Eng. J. 2012, 6, 33–37. [Google Scholar]
- Ooi, J.Y.; Pham, L.; Rotter, J.M. Systematic and random features of measured pressures on full-scale silo walls. Eng. Struct. 1990, 12, 74–87. [Google Scholar] [CrossRef]
- Linek, J.; Rösch, N. The right approach how to design the conditioning system of a sugar silo. Sugar Ind. 2017, 142, 476–480. [Google Scholar] [CrossRef]
- Ansari, A.; Armaghan, K.; Kulkarni, S.S. Design and Optimization of RCC Silo. Int. J. Res. Appl. Sci. Eng. Technol. 2016, 4, 458–466. [Google Scholar]
- Chithra, S.; Indupriya, G. Contributions of Different Standards and Codes for the Design of Silo: A Review. Asian Rev. Civ. Eng. 2016, 6, 27–33. [Google Scholar]
- Lapko, A.; Prusiel, J.A. Structural analysis of RC circular grouped silos under patch actions. Granul. Matter 2004, 6, 185–190. [Google Scholar] [CrossRef]
- ACI Committee 313. Practice for Design and Construction of Concrete Silos and Stacking Tubes for Storing Granular Materials (ACI 313-97); American Concrete Institute Standard: Detroit, MI, USA, 2004; pp. 1–17. [Google Scholar]
- Yuksel, S.B.; Arslan, M.H. Design Force Estimation Using Artificial Neural Network for Groups of Four Cylindrical Silos. Adv. Struct. Eng. 2010, 13, 681–693. [Google Scholar] [CrossRef]
- Yuksel, S.B.; Arikan, S. A New Set of Design Aids for Groups of Four Cylindrical Silos due to Interstice and Internal Loadings. Struct. Des. Tall Spec. Build. 2009, 169, 149–169. [Google Scholar] [CrossRef]
- Mehos, G.; Eggleston, M.; Grenier, S.; Malanga, C.; Shrestha, G.; Trautman, T. Designing Hoppers, Bins, and Silos for Reliable Flow. AIChE. 2018. Available online: https://www.aiche.org/resources/publications/cep/2018/april/designing-hoppers-bins-and-silos-reliable-flow. (accessed on 5 May 2020).
- Watson, G.R.; Rotter, J.M. A Finite Element Kinematic Analysis of Planar Granular Solids Flow. Chem. Eng. Sci. 1996, 51, 3967–3978. [Google Scholar] [CrossRef]
- Lobato, J.C.M.; Mesquita, A.L.A.; Mesquita, A.L.A. Conical hopper design for mass flow—Case of study for red mud powder. In Proceedings of the 15th Brazilian Congress of Thermal Sciences and Engineering, Belém, PA, Brazil, 10–13 November 2014; pp. 1–9. [Google Scholar]
- Ding, S.; Li, H.; Ooi, J.Y.; Rotter, J.M. Particuology Prediction of flow patterns during silo discharges using a finite element approach and its preliminary experimental verification. Particuology 2015, 18, 42–49. [Google Scholar] [CrossRef]
- Boucheham, N.; Galet, L.; Patry, S.; Zidoune, M.N. Physicochemical and hydration properties of different cereal and legume gluten-free powders. Food Sci. Nutr. 2019, 7, 3081–3092. [Google Scholar] [CrossRef] [Green Version]
- Ituen, E.E.; Mittal, J.; Adeoti, J.S. Water absorption in cereal grains and its effect on their rupture stress. J. Food Process Eng. 2007, 8, 147–158. [Google Scholar] [CrossRef]
- Mali, M.Z.; Patil, S.J.; Talikoti, R.S. Effect of Earthquake and Wind on Silo. Int. J. Civ. Struct. Eng. Res. 2015, 3, 376–383. [Google Scholar]
- Togarsi, R.S. Seismic Response of Reinforced Concrete Frames. Int. J. Res. Eng. Technol. 2015, 4, 174–178. [Google Scholar]
- Carson, J.; Jenkyn, R. How to prevent silo failure with routine inspections and proper repairs. Powder Bulk Eng. 1990, 4, 18–24. [Google Scholar]
- Alrazooqi, M.; De Silva, R. Mobile and wireless services and technologies for m-government solution proposal for Dubai government. WSEAS Trans. Inf. Sci. Appl. 2010, 7, 1037–1047. [Google Scholar]
- Tu, P. Dynamic Response of Silo Supporting Structure under Pulsating Loads. Ph.D. Thesis, Curtin University, Perth, Australia, September 2017. [Google Scholar]
- Maraveas, C. Durability Issues and Corrosion of Structural Materials and Systems in Farm Environment. Appl. Sci. 2020, 10, 990. [Google Scholar] [CrossRef] [Green Version]
- Elias, S.; Matsagar, V.; Datta, T.K. Effectiveness of distributed tuned mass dampers for multi-mode control of chimney under earthquakes. Eng. Struct. 2016, 124, 1–16. [Google Scholar] [CrossRef]
- Nordin, H. Strengthening Structures with Externally Prestressed Tendons. Master’s Thesis, Luleå University of Technology, Luleå, Sweden, 2004. [Google Scholar]
- Kaur, H.; Singh, J. A Review on External Prestressing in Concrete. Int. Res. J. Eng. Technol. 2017, 4, 1801–1805. [Google Scholar]
- Di Gioacchino, F.; Quinta da Fonseca, J. Plastic Strain Mapping with Sub-micron Resolution Using Digital Image Correlation. Exp. Mech. 2013, 53, 743–754. [Google Scholar] [CrossRef]
- Paul, S.; Sivaprasad, S.; Dhar, S.; Tarafder, S. Key issues in cyclic plastic deformation: Experimentation. Mech. Mater. 2011, 43, 705–720. [Google Scholar] [CrossRef]
- Prota, A.; Parretti, R.; Nanni, A. Upgrade of RC Silos Using Near Surface Mounted FRP Composites. Adv. Struct. Eng. 2003, 7, 170–183. [Google Scholar]
- de Lorenzis, L.; Micelli, F.; la Tegola, A. Fiber-Reinforced Polymer for Structural Strengthening: Post-Tensioning of Steel Silos. Struct. Eng. Int. 2003, 2, 124–127. [Google Scholar] [CrossRef]
- Cleland, J.H. Corrosion in crop storage silos. Eng. Fail. Anal. 1994, I, 17–28. [Google Scholar] [CrossRef]
- Söylev, T.A.; Richardson, M.G. Corrosion inhibitors for steel in concrete: State-of-the-art report. Constr. Build. Mater. 2008, 22, 609–622. [Google Scholar] [CrossRef]
- Assaad Abdelmseeh, V.; Jofriet, J.; Hayward, G. Sulphate and sulphide corrosion in livestock buildings, Part II: Reinforcing steel corrosion. Biosyst. Eng. 2008, 99, 382–389. [Google Scholar] [CrossRef]
- Monteiro, F.C.B.; Trautwein, L.M.; Almeida, L.C. The importance of the European standard EN 1504, on the protection and repair of concrete structures. J. Build. Pathol. Rehabil. 2017, 2, 1–12. [Google Scholar] [CrossRef]




| Type of Concrete Reinforcement | Thermal Expansion Coefficient (1/°C) (Longitudinal) | Thermal Expansion Coefficient (1/°C) (Transverse) | Values in the Literature (1/°C) (Longitudinal) | Values in the Literature (1/°C) (Transverse) |
|---|---|---|---|---|
| Steel | 11.3 × 10−6 | 11.3 × 10−6 | 11.7 × 10−6 | 11.7 × 10−6 |
| GFRP | 4.43 × 10−6 | 22.5 × 10−6 | 6–10 × 10−6 | 21–23 × 10−6 |
| CFRP | 1.05 × 10−6 | 93 × 10−6 | (−9)–(0) × 10−6 | 74–104 × 10−6 |
| AFRP | −5.2 × 10−6 | 51 × 10−6 | (−6) × 10−6 | 60–80 × 10−6 |
| BFRP | 1.92 × 10−6 | 17.1 × 10−6 | 4 × 10−6 | 18–26 × 10−6 |
| Consistency Test (Slump Test) | ||||
| 0 | 3 | 10.33 a | 0.47 | 6.00 |
| 3.0 | 3 | 9.50 a | 0.41 | |
| 4.5 | 3 | 7.16 b | 0.24 | |
| 6.0 | 3 | 6.33 b | 0.47 | |
| fc medium—28 days (MPa) | ||||
| Axial Compression Test on Cylindrical Specimens | ||||
| 0 | 4 | 5.45 c | 0.12 | 6.39 |
| 3.0 | 4 | 14.86 a | 0.73 | |
| 4.5 | 4 | 12.94 b | 0.65 | |
| 6.0 | 4 | 2.69 d | 0.14 | |
| Tensile Test in Bending on Prismatic Specimens | ||||
| 0 | 4 | 5.78 b | 0.00 | 10.55 |
| 3.0 | 4 | 8.30 a | 1.11 | |
| 4.5 | 4 | 8.15 a | 0.42 | |
| 6.0 | 4 | 5.33 b | 0.00 | |
| Physical Properties | Chemical Properties | ||
|---|---|---|---|
| Specific Weight | 2.98 gr/cm3 | Ignition Loss | 1.20% |
| Specific Surface (Blaine) | 3135 cm2/gr | SiO2 | 19.80% |
| Volume Expansion | 3 mm | Al2O3 | 5.71% |
| Initial Set | 160 min. | Fe2O3 | 3.14% |
| Final Set | 235 min. | CaO | 63.23% |
| 2-day Compressive Strength | 25.0 MPa | MgO | 2.43% |
| 7-day Compressive Strength | 34.4 MPa | SO3 | 2.85% |
| 28-day Compressive Strength | 49.1 MPa | Cl | 0.006% |
| Type of Storage Silo | Storage Period (Months) | Moisture Content (%) | Maize Quality Parameters (%) | ||||
|---|---|---|---|---|---|---|---|
| Protein Content | Oil Content | Crude Fiber | Starch Content | Ash Content | |||
| 0 | 11.2(0.1) | 10.6(0.1) | 5.1(0.1) | 0.2(0.0) | 60.6(0.1) | 1.06(0.03) | |
| 2 | 11.8(0.1) | 10.3(0.1) | 4.8(0.2) | 0.2(0.1) | 60.5(0.5) | 1.01(0.03) | |
| Termite Mound clay | 4 | 11.7(0.2) | 9.9(0.1) | 4.7(0.4) | 0.2(0.0) | 60.1(0.6) | 0.91(0.05) |
| 6 | 13.4(0.1) | 9.5(0.4) | 4.5(0.1) | 0.3(0.1) | 59.3(0.2) | 0.94(0.04) | |
| 8 | 16.0(0.2) | 8.3(0.2) | 4.6(0.4) | 0.5(0.2) | 59.1(0.7) | 0.96(0.04) | |
| 0 | 11.2(0.1) | 10.6(0.1) | 5.1(0.1) | 0.2(0.0) | 60.6(0.1) | 1.06(0.03) | |
| 2 | 11.4(0.1) | 9.9(0.1) | 4.8(0.2) | 0.2(0.1) | 60.3(0.2) | 1.00(0.06) | |
| Reinforced concrete | 4 | 11.8(0.2) | 10.0(0.2) | 4.7(0.2) | 0.2(0.1) | 59.7(0.6) | 0.95(0.02) |
| 6 | 13.3(0.3) | 9.1(0.5) | 4.6(0.5) | 0.2(0.1) | 59.3(0.7) | 0.99(0.02) | |
| 8 | 15.1(0.3) | 10.0(0.3) | 4.7(0.5) | 0.4(0.1) | 58.2(0.7) | 0.99(0.04) | |
| 0 | 11.2(0.1) | 10.6(0.1) | 5.1(0.1) | 0.2(0.0) | 60.6(0.1) | 1.06(0.03) | |
| 2 | 11.3(0.2) | 10.2(0.2) | 5.0(0.2) | 0.3(0.1) | 59.8(0.4) | 1.02(0.04) | |
| Galvanized steel | 4 | 11.0(0.3) | 10.2(0.3) | 4.6(0.2) | 0.2(0.1) | 60.4(0.2) | 0.94(0.03) |
| 6 | 11.8(0.3) | 9.6(0.3) | 4.5(0.4) | 0.3(0.0) | 59.9(0.5) | 0.96(0.03) | |
| 8 | 12.7(0.4) | 9.9(0.4) | 4.7(0.2) | 0.3(0.1) | 59.0(0.9) | 0.97(0.07) | |
| Grain | Moisture | Angle of | Pressure Ratio | ||
|---|---|---|---|---|---|
| Content | Internal Friction | Measured | Calculated kf. | ||
| w.b. (%) | ϕ (0) | Ks | Eurocode 1 | Jaky | |
| 10 | 27.8 ± 0.4 | 0.45 ± 0.02 | 0.59 | 0.48 | |
| 12.5 | 28.5 ± 0.5 | 0.47 ± 0.03 | 0.56 | 0.47 | |
| Barley | 15 | 31.2 ± 0.3 | 0.43 ± 0.02 | 0.50 | 0.43 |
| 17.5 | 30.6 ± 1.0 | 0.45 ± 0.03 | 0.54 | 0.43 | |
| 20 | 33.2 ± 0.5 | 0.39 ± 0.03 | 0.51 | 0.40 | |
| 10 | 26.7 ± 0.6 | 0.48 ± 0.04 | 0.60 | 0.49 | |
| 12.5 | 31.7 ± 0.5 | 0.40 ± 0.03 | 0.52 | 0.42 | |
| Corm | 15 | 32.0 ± 1.4 | 0.36 ± 0.05 | 0.51 | 0.41 |
| 17.5 | 33.4 ± 0.8 | 0.34 ± 0.03 | 0.50 | 0.40 | |
| 20 | 33.6 ± 1.5 | 0.30 ± 0.05 | 0.51 | 0.39 | |
| 10 | 22.1 ± 1.1 | 0.49 ± 0.03 | 0.67 | 0.57 | |
| 12.5 | 22.4 ± 0.9 | 0.44 ± 0.04 | 0.68 | 0.56 | |
| Oat | 15 | 24.0 ± 0.5 | 0.45 ± 0.03 | 0.64 | 0.54 |
| 17.5 | 23.9 ± 1.0 | 0.40 ± 0.03 | 0.66 | 0.54 | |
| 20 | 26.4 ± 1.7 | 0.41 + 0.06 | 0.63 | 0.50 | |
| 10 | 25.7 ±0.3 | 0.44 ±0.02 | 0.62 | 0.51 | |
| 12.5 | 26.2 ± 0.4 | 0.38 ± 0.01 | 0.61 | 0.50 | |
| Wheat | 15 | 27.0± 0.5 | 0.34 ± 0.02 | 0.60 | 0.49 |
| 17.5 | 33.0 ± 1.0 | 0.31 ± 0.02 | 0.50 | 0.40 | |
| 20 | 35.5 ± 0.5 | 0.35 ± 0.0l | 0.46 | 0.37 | |
| Back- | Optimum | Training | Test | Training | R2 | ||
|---|---|---|---|---|---|---|---|
| Propagation | Number | ANN | Error | Error | Iteration | Time | |
| Methods | of HN | Structure | (%) | (%) | Number | (Second) | (%) |
| BFG | 6 | 3:6:1 | 0.38 | 1.80 | 2813 | 49.17 | 98.37 |
| CGB | 6 | 3:6:1 | 3.21 | 3.20 | 1148 | 7.97 | 98.45 |
| CGF | 6 | 3:6:1 | 0.41 | 3.12 | 839 | 5.91 | 98.38 |
| CGP | 6 | 3:6:1 | 0.44 | 3.19 | 658 | 4.61 | 98.19 |
| GDA | 4 | 3:4:1 | 3.59 | 5.27 | 5000 | 11.96 | 96.55 |
| GDM | 6 | 3:6:1 | 6.55 | 8.27 | 5000 | 12.05 | 92.68 |
| GDX | 4 | 3:4:1 | 2.34 | 5.76 | 5000 | 11.61 | 95.78 |
| LM | 4 | 3:4:1 | 0.27 | 1.28 | 3900 | 19.08 | 99.65 |
| OSS | 4 | 3:4:1 | 0.63 | 1.11 | 5000 | 43.02 | 99.87 |
| RP | 4 | 3:4:1 | 2.69 | 3.61 | 5000 | 13.22 | 98.22 |
| SCG | 4 | 3:4:1 | 0.25 | 0.58 | 5000 | 23.87 | 99.94 |
| Type of | Unit Weight | Angle | Effective Angle | Lateral | Max. Flow | |||
|---|---|---|---|---|---|---|---|---|
| Bulk Solid | of | of Internal | Pressure | Pressure | ||||
| γ | Repose | Friction | Ratio | Multiplier | ||||
| φr | φi | K or λ | Co | |||||
| γl | γu | φr | φil | φiu | Kl, λl | Ku, λu | ||
| Lower | Upper | Lower | Upper | Lower | Upper | |||
| kN/m3 | kN/m3 | Degrees | Degrees | Degrees | ||||
| Alumina | 10.0 | 12.0 | 27 | 25 | 40 | 0.42 | 0.53 | 1.40 |
| Barley | 7.0 | 8.5 | 20 | 26 | 33 | 0.50 | 0.63 | 1.35 |
| Cement | 13.0 | 16.0 | 28 | 40 | 50 | 0.45 | 0.58 | 1.40 |
| Cement clinker | 15.0 | 18.0 | 33 | 42 | 52 | 0.41 | 0.52 | 1.40 |
| Flour (wheat) | 6.5 | 7.0 | 40 | 23 | 30 | 0.36 | 0.46 | 1.45 |
| Fly ash | 8.0 | 14.0 | 22 | 30 | 35 | 0.41 | 0.52 | 1.45 |
| Iron ore pellets | 19.0 | 22.0 | 35 | 35 | 45 | 0.35 | 0.45 | 1.40 |
| Lime, hydrated | 6.0 | 8.0 | 40 | 35 | 45 | 0.35 | 0.45 | 1.40 |
| Limestone powder | 11.0 | 13.0 | 30 | 40 | 60 | 0.35 | 0.45 | 1.45 |
| Maize | 7.0 | 8.5 | 30 | 28 | 33 | 0.45 | 0.58 | 1.40 |
| Phosphate rock | 16.0 | 19.0 | 27 | 35 | 55 | 0.35 | 0.45 | 1.40 |
| Sand: coarse dry | 14.0 | 17.0 | 30 | 30 | 40 | 0.41 | 0.52 | 1.40 |
| Sand: quartz | 15.0 | 17.0 | 30 | 35 | 40 | 0.35 | 0.45 | 1.40 |
| Slag: granular, dry | 10.5 | 12.0 | 40 | 35 | 38 | 0.36 | 0.47 | 1.40 |
| Soya beans | 7.0 | 8.0 | 23 | 25 | 32 | 0.47 | 0.60 | 1.30 |
| Sugar | 8.0 | 9.5 | 29 | 33 | 38 | 0.45 | 0.58 | 1.40 |
| Wheat | 7.5 | 9.0 | 20 | 26 | 32 | 0.50 | 0.63 | 1.30 |
| Type of Damage on RC | Rehabilitation Method | Ref |
|---|---|---|
| Corrosion of RC | Reinforcement of concrete with alternative materials and corrosion inhibitors such as tolytriazole or sodium silicate | [76] |
| Exposure of steel reinforcement though abrasion | Surface furnishing, liners and sacrificial plates | [47] |
| Obstructions in the mass flow of the bulk solids | Addition of inserts and modification of design codes | [48] |
© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Maraveas, C. Concrete Silos: Failures, Design Issues and Repair/Strengthening Methods. Appl. Sci. 2020, 10, 3938. https://doi.org/10.3390/app10113938
Maraveas C. Concrete Silos: Failures, Design Issues and Repair/Strengthening Methods. Applied Sciences. 2020; 10(11):3938. https://doi.org/10.3390/app10113938
Chicago/Turabian StyleMaraveas, Chrysanthos. 2020. "Concrete Silos: Failures, Design Issues and Repair/Strengthening Methods" Applied Sciences 10, no. 11: 3938. https://doi.org/10.3390/app10113938
APA StyleMaraveas, C. (2020). Concrete Silos: Failures, Design Issues and Repair/Strengthening Methods. Applied Sciences, 10(11), 3938. https://doi.org/10.3390/app10113938





