Tunable Terahertz Metamaterial Using an Electric Split-Ring Resonator with Polarization-Sensitive Characteristic
Abstract
:1. Introduction
2. Materials and Methods
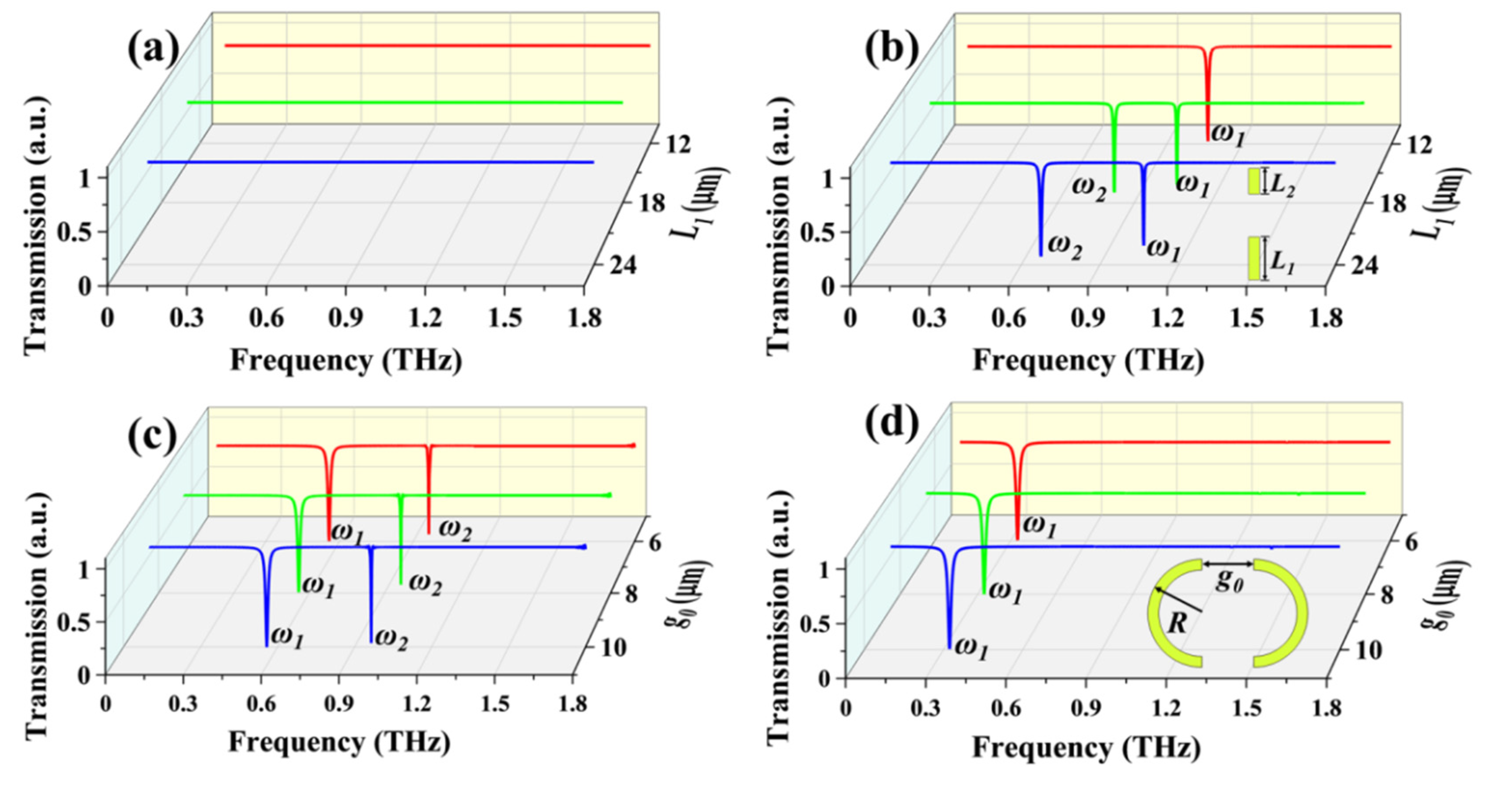
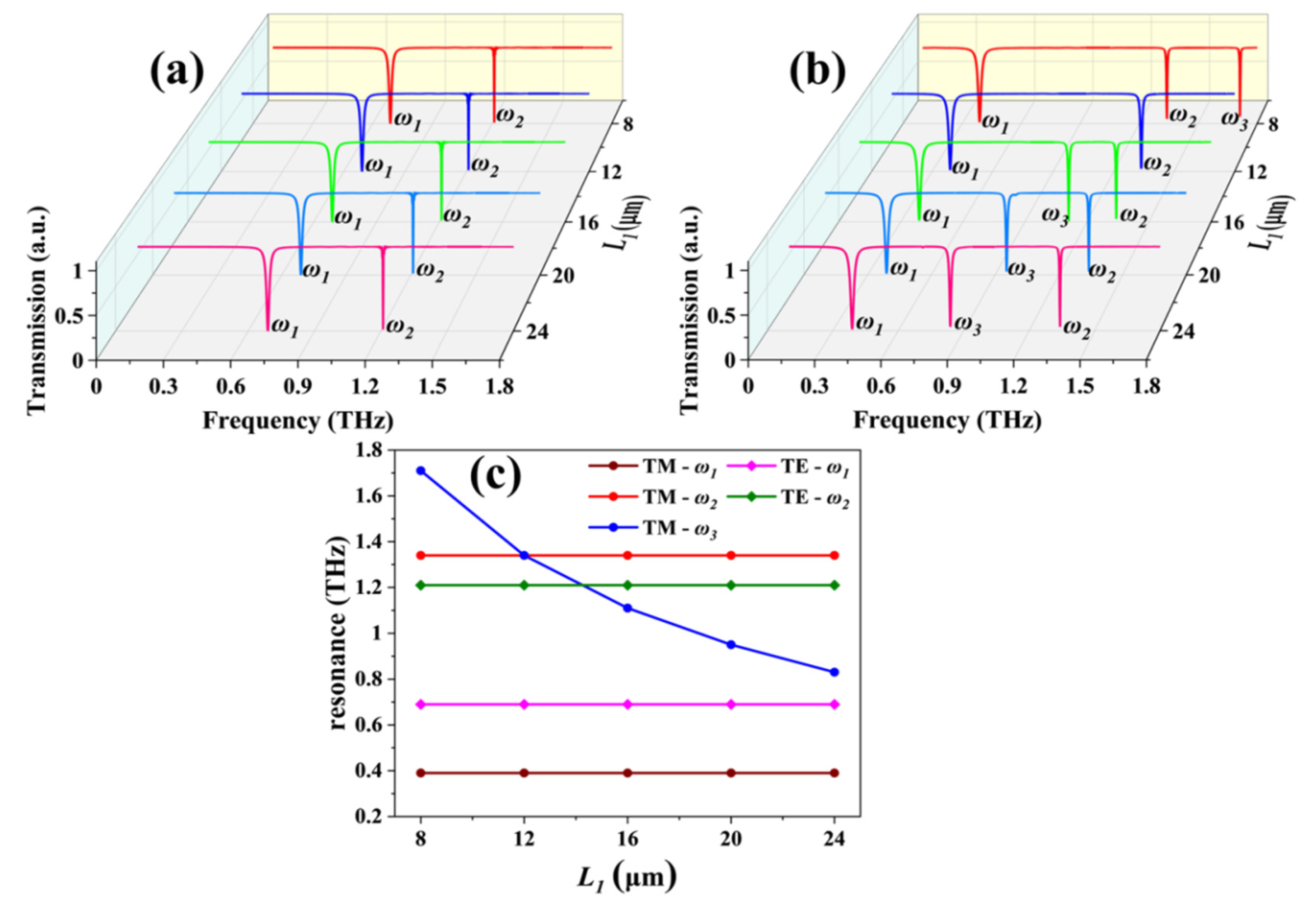
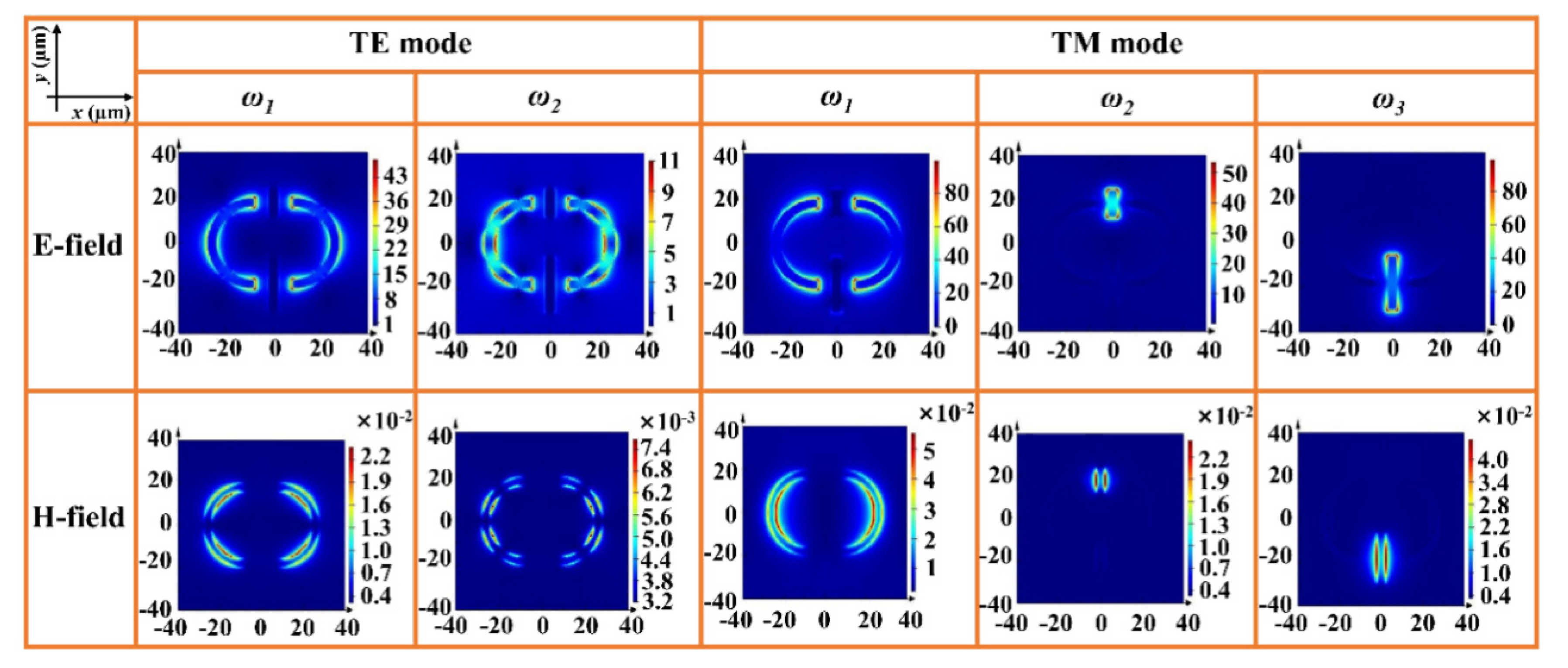
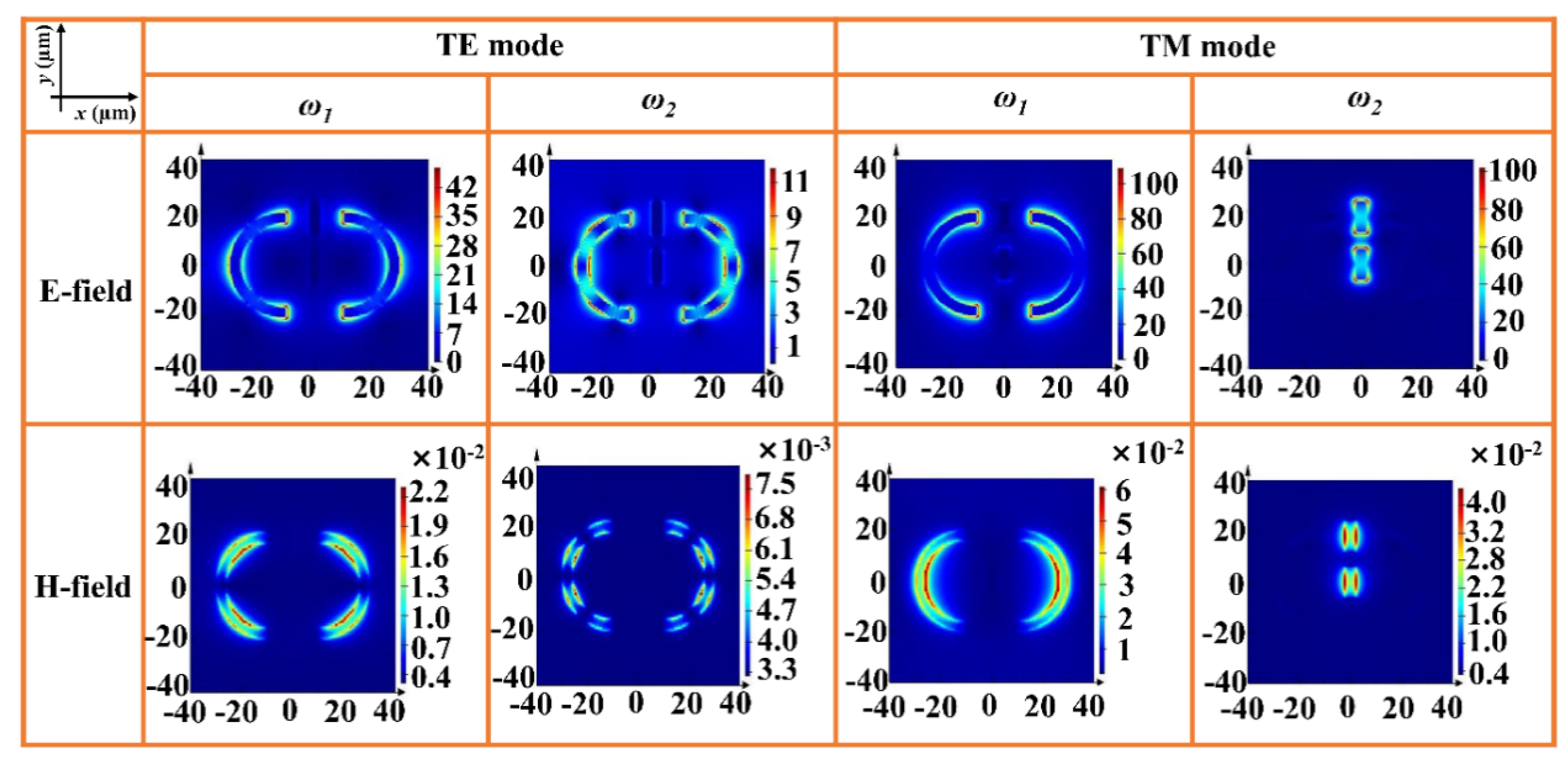
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Booske, J.H.; Dobbs, R.J.; Joye, C.D.; Kory, C.L.; Neil, G.R.; Park, G.-S.; Park, J.; Temkin, R.J. Vacuum Electronic High Power Terahertz Sources. IEEE Trans. Terahertz Sci. Technol. 2011, 1, 54–75. [Google Scholar] [CrossRef]
- Shi, W.; Ding, Y.J.; Fernelius, N.; Vodopyanov, K.L. An efficient tunable and coherent 0.18–5.27 terahertz source based on GaSe crystal. Opt. Lett. 2002, 27, 1454–1456. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Libon, I.H.; Hecker, N.E.; Koch, M.; Baumgartner, S.; Hempel, M.; Feldmann, J.; Dawson, P. An optically controllable terahertz filter. Appl. Phys. Lett. 2000, 76, 2821–2823. [Google Scholar] [CrossRef]
- Wu, X.; Quan, B.; Pan, X.; Xu, X.; Lu, X.; Gu, C.-Z.; Wang, L. Alkanethiol-functionalized terahertz metamaterial as label-free, highly-sensitive and specificbiosensor. Biosens. Bioelectron. 2013, 42, 626–631. [Google Scholar] [CrossRef] [PubMed]
- Ahi, K. Mathematical Modeling of THz Point Spread Function and Simulation of THz Imaging Systems. IEEE Trans. Terahertz Sci. Technol. 2017, 7, 747–754. [Google Scholar] [CrossRef]
- Petrov, N.; Kulya, M.; Tsypkin, A.N.; Bespalov, V.G.; Gorodetsky, A. Application of Terahertz Pulse Time-Domain Holography for Phase Imaging. IEEE Trans. Terahertz Sci. Technol. 2016, 6, 464–472. [Google Scholar] [CrossRef] [Green Version]
- Van Der Weide, D.; Murakowski, J.; Keilmann, F. Gas-absorption spectroscopy with electronic terahertz techniques. IEEE Trans. Microw. Theory Tech. 2000, 48, 740–743. [Google Scholar] [CrossRef]
- Yan, X.; Yang, M.; Zhang, Z.; Liang, L.; Wei, D.; Wang, M.; Zhang, Z.; Wang, T.; Liu, L.; Xie, J.; et al. The terahertz electromagnetically induced transparency-like metamaterials for sensitive biosensors in the detection of cancer cells. Biosens. Bioelectron. 2019, 126, 485–492. [Google Scholar] [CrossRef]
- Lannebère, S.; Campione, S.; Aradian, A.; Albani, M.; Capolino, F. Artificial magnetism at terahertz frequencies from three-dimensional lattices of TiO_2 microspheres accounting for spatial dispersion and magnetoelectric coupling. J. Opt. Soc. Am. B 2014, 31, 1078. [Google Scholar] [CrossRef] [Green Version]
- Fan, Y.; Zhang, F.; Zhao, Q.; Wei, Z.; Li, H. Tunable terahertz coherent perfect absorption in a monolayer graphene. Opt. Lett. 2014, 39, 6269–6272. [Google Scholar] [CrossRef] [Green Version]
- Ou, H.; Lu, F.; Xu, Z.; Lin, Y.-S. Terahertz Metamaterial with Multiple Resonances for Biosensing Application. Nanomaterials 2020, 10, 1038. [Google Scholar] [CrossRef] [PubMed]
- Zheng, D.; Hu, X.; Lin, Y.-S.; Chen, C.-H. Tunable multi-resonance of terahertz metamaterial using split-disk resonators. AIP Adv. 2020, 10, 025108. [Google Scholar] [CrossRef] [Green Version]
- Lu, F.; Ou, H.; Liao, Y.; Zhu, F.; Lin, Y.-S. Actively switchable terahertz metamaterial. Results Phys. 2019, 15, 102756. [Google Scholar] [CrossRef]
- Xu, Z.; Lin, Z.; Cheng, S.; Lin, Y.-S. Reconfigurable and tunable terahertz wrench-shape metamaterial performing programmable characteristic. Opt. Lett. 2019, 44, 3944–3947. [Google Scholar] [CrossRef] [PubMed]
- Xu, Z.; Lin, Y.-S. A Stretchable Terahertz Parabolic-Shaped Metamaterial. Adv. Opt. Mater. 2019, 7, 1900379. [Google Scholar] [CrossRef]
- Lin, Y.-S.; Liao, S.; Liu, X.; Tong, Y.; Xu, Z.; Xu, R.; Yao, D.; Yu, Y. Tunable terahertz metamaterial by using three-dimensional double split-ring resonators. Opt. Laser Technol. 2019, 112, 215–221. [Google Scholar] [CrossRef]
- Liu, P.; Liang, Z.; Lin, Z.; Xu, Z.; Xu, R.; Yao, D.; Lin, Y.-S. Actively tunable terahertz chain-link metamaterial with bidirectional polarization-dependent characteristic. Sci. Rep. 2019, 9, 9917. [Google Scholar] [CrossRef]
- Xu, R.; Liu, X.; Lin, Y.-S. Tunable ultra-narrowband terahertz perfect absorber by using metal-insulator-metal microstructures. Results Phys. 2019, 13, 102176. [Google Scholar] [CrossRef]
- Luo, J.; Lin, Y.-S. High-efficiency of infrared absorption by using composited metamaterial nanotubes. Appl. Phys. Lett. 2019, 114, 051601. [Google Scholar] [CrossRef]
- Zhan, F.; Lin, Y.S. Tunable Multi-Resonance Using Complementary Circular Metamaterial. Opt. Lett. 2020, 45, 3633–3636. [Google Scholar] [CrossRef]
- Lin, Y.-S.; Yan, K.; Yao, D.; Yu, Y. Investigation of electromagnetic response of terahertz metamaterial by using split-disk resonator. Opt. Laser Technol. 2019, 111, 509–514. [Google Scholar] [CrossRef]
- Lin, Y.-S.; Dai, J.; Zeng, Z.; Yang, B.-R. Metasurface Color Filters Using Aluminum and Lithium Niobate Configurations. Nanoscale Res. Lett. 2020, 15, 77. [Google Scholar] [CrossRef] [PubMed]
- Xu, R.; Lin, Y.-S. Characterizations of reconfigurable infrared metamaterial absorbers. Opt. Lett. 2018, 43, 4783–4786. [Google Scholar] [CrossRef] [PubMed]
- Gómez-Castaño, M.; Zheng, H.; Garcia-Pomar, J.L.; Vallée, R.A.L.; Mihi, A.; Ravaine, S. Tunable index metamaterials made by bottom-up approaches. Nanoscale Adv. 2019, 1, 1070–1076. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Weis, P.; Garcia-Pomar, J.L.; Rahm, M. Towards loss compensated and lasing terahertz metamaterials based on optically pumped graphene. Opt. Express 2014, 22, 8473–8489. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Pendry, J.; Holden, A.; Robbins, D.; Stewart, W. Magnetism from conductors and enhanced nonlinear phenomena. IEEE Trans. Microw. Theory Tech. 1999, 47, 2075–2084. [Google Scholar] [CrossRef] [Green Version]
- Ma, F.; Lin, Y.-S.; Zhang, X.; Lee, V.C. Tunable multiband terahertz metamaterials using a reconfigurable electric split-ring resonator array. Light. Sci. Appl. 2014, 3, e171. [Google Scholar] [CrossRef] [Green Version]
- Lan, F.; Yang, Z.; Qi, L.; Gao, X.; Shi, Z. Terahertz dual-resonance bandpass filter using bilayer reformative complementary metamaterial structures. Opt. Lett. 2014, 39, 1709–1712. [Google Scholar] [CrossRef]
- Yu, Y.; Lin, Y.-S. Multi-functional terahertz metamaterial using symmetrical and asymmetrical electric split-ring resonator. Results Phys. 2019, 13, 102321. [Google Scholar] [CrossRef]
- Lin, Z.; Xu, Z.; Liu, P.; Liang, Z.; Lin, Y.-S. Polarization-sensitive terahertz resonator using asymmetrical F-shaped metamaterial. Opt. Laser Technol. 2020, 121, 105826. [Google Scholar] [CrossRef]
- Kishor, K.; Baitha, M.N.; Sinha, R.K.; Lahiri, B. Tunable negative refractive index metamaterial from V-shaped SRR structure: Fabrication and characterization. J. Opt. Soc. Am. B 2014, 31, 1410. [Google Scholar] [CrossRef]
- Cao, W.; Zhang, B.; Liu, A.; Yu, T.; Guo, D.; Wei, Y. Broadband High-Gain Periodic Endfire Antenna by Using I-Shaped Resonator (ISR) Structures. IEEE Antennas Wirel. Propag. Lett. 2012, 11, 1470–1473. [Google Scholar] [CrossRef]
- Lin, Y.-S.; Huang, C.-Y.; Lee, V.C. Reconfiguration of Resonance Characteristics for Terahertz U-Shape Metamaterial Using MEMS Mechanism. IEEE J. Sel. Top. Quantum Electron. 2014, 21, 93–99. [Google Scholar] [CrossRef]
- Zhang, Z.; Zhang, X.; Liang, Z.; Cheng, S.; Liu, P.; Lin, Y.-S. Reconfigurable double C-shape metamaterial (DCM) for a terahertz resonator. OSA Contin. 2019, 2, 3026–3036. [Google Scholar] [CrossRef]
- Ma, F.; Qian, Y.; Lin, Y.-S.; Liu, H.; Zhang, X.; Liu, Z.; Tsai, J.M.; Lee, V.C. Polarization-sensitive microelectromechanical systems based tunable terahertz metamaterials using three dimensional electric split-ring resonator arrays. Appl. Phys. Lett. 2013, 102, 161912. [Google Scholar] [CrossRef]
- Mo, Y.; Zhong, J.; Lin, Y.-S. Tunable chevron-shaped infrared metamaterial. Mater. Lett. 2020, 263, 127291. [Google Scholar] [CrossRef]
- Ou, H.; Lu, F.; Liao, Y.; Zhu, F.; Lin, Y.-S. Tunable terahertz metamaterial for high-efficiency switch application. Results Phys. 2020, 16, 102897. [Google Scholar] [CrossRef]
- Cheng, S.; Xu, Z.; Yao, D.; Zhang, X.; Zhang, Z.; Lin, Y.-S. Electromagnetically induced transparency in terahertz complementary spiral-shape metamaterials. OSA Contin. 2019, 2, 2137–2144. [Google Scholar] [CrossRef]
- Hu, X.; Zheng, D.; Lin, Y.-S. Actively tunable terahertz metamaterial with single-band and dual-band switching characteristic. Appl. Phys. A 2020, 126, 110. [Google Scholar] [CrossRef]




© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, T.; Lin, Y.-S. Tunable Terahertz Metamaterial Using an Electric Split-Ring Resonator with Polarization-Sensitive Characteristic. Appl. Sci. 2020, 10, 4660. https://doi.org/10.3390/app10134660
Xu T, Lin Y-S. Tunable Terahertz Metamaterial Using an Electric Split-Ring Resonator with Polarization-Sensitive Characteristic. Applied Sciences. 2020; 10(13):4660. https://doi.org/10.3390/app10134660
Chicago/Turabian StyleXu, Tao, and Yu-Sheng Lin. 2020. "Tunable Terahertz Metamaterial Using an Electric Split-Ring Resonator with Polarization-Sensitive Characteristic" Applied Sciences 10, no. 13: 4660. https://doi.org/10.3390/app10134660
APA StyleXu, T., & Lin, Y.-S. (2020). Tunable Terahertz Metamaterial Using an Electric Split-Ring Resonator with Polarization-Sensitive Characteristic. Applied Sciences, 10(13), 4660. https://doi.org/10.3390/app10134660




