1. Introduction
The aircraft turbine engine consists of three fundamental segments – a compressor, a combustion segment, and a turbine [
1]. The connection between the compressor disc and the turbine disc is provided by a rotating shaft. Part of the engine performance loss originates between the rotating shaft and the stator case, because the secondary air flows here and does not follow the primary path [
2]. A labyrinth seal is a way of suppressing the airflow in cavities between the shaft and the case [
3,
4]. Current turbomachinery (not only aircraft engines) is facing problems of reducing the airflows outside the primary air flow path, but simultaneously there are problems with the necessity of turbine blade cooling [
4,
5] and bearings control [
6] requiring fresh airflow. The labyrinth seal is advantageous due to its relatively simple manufacture procedure and the fact that its use does not disrupt the balancing of the shaft. A relevant geometric parameter of the labyrinth seal is its radial clearance (RC), i.e., the radial length of the gap between the labyrinth seal teeth and the opposite wall. Due to the contactless disposition, the radial clearance cannot be zero and it is evident that it will increase during operation life of the engine. The aim of this article is, based on the computational fluid dynamics (CFD) [
4], to clarify and describe the influence of the radial clearance on the thermodynamic parameters of the labyrinth seal.
The physical principle of the turbomachinery labyrinth seal operation is energy loss in cavities between the teeth in the seal; the loss practically restrains the parasitic flow between the rotating shaft and the fixed case. Because the labyrinth seal is contactless, the air flows and thus a vortex is developed between the teeth. Modern labyrinth seals are characterized by pressure and mass flow drop, named sealing effect. Initial results of research can be found in the steam turbine’s design. However, the important difference between a steam turbine and a turbine engine consists of very different angular velocities of the shaft. In steam turbines, the velocity is approximately 500 rad/s and, in turbine engines, it is approximately 4100 rad/s [
1,
7,
8]. Literature sources addressing the topic are rather rare [
9,
10,
11]. In the presented article, the influence of different settings of the radial clearance and different locations of the teeth are examined, developing the previous research presented in [
12]. The number of teeth was out of interest. The typical number of teeth [
3,
5,
13] used was equal to four, because it corresponds to the standard conditions in labyrinth seals.
2. Labyrinth Seal Geometry
The labyrinth seal geometry consists of 5 geometric values. All geometric values were represented by parameters that are typical for the turbine engine labyrinth seals [
3]:
Shaft radius 2013 88 mm
Thickness of the teeth–0.57 mm
Height of the flow channel–2.54 mm
Length of the seal cavity–2.15 mm
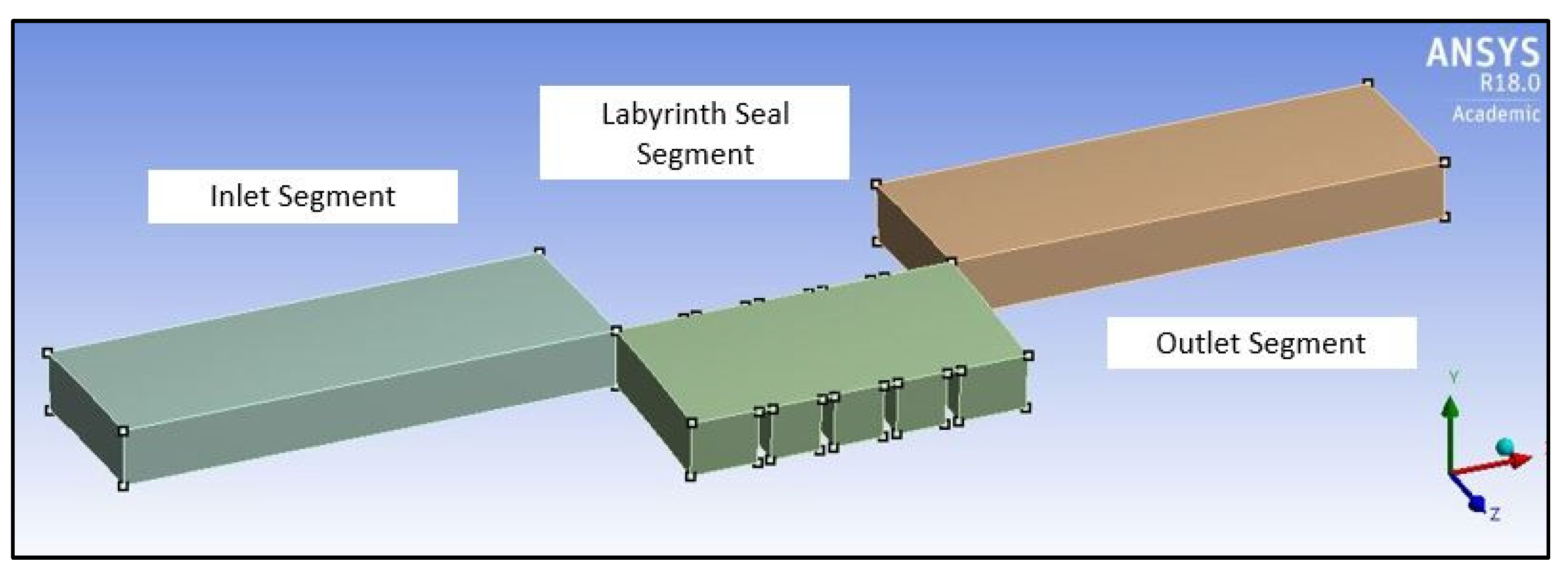
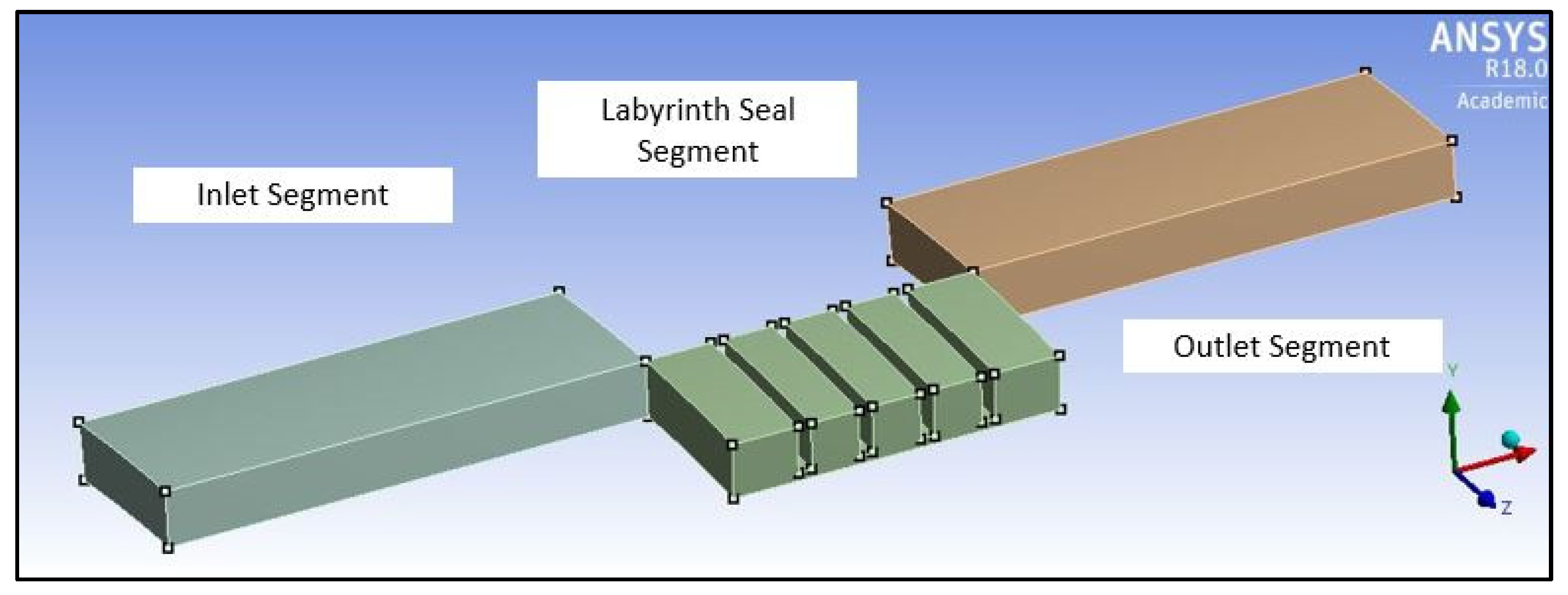
All teeth have a rectangular cross-section. Two variants of the locations of the teeth are studied–with the first inlet location being on the rotor (i.e., teeth are rotating) and the second one being on the stator (i.e., teeth are non-rotating). The CFD geometry consists of 3 segments. The first inlet segment is a non-rotating conical segment where the inlet boundary conditions are defined; second segment is a rotating labyrinth seal conical segment where the labyrinth seal geometry is modeled; and third segment is a non-rotating conical segment where the outlet boundary conditions are defined. The inlet and the outlet segments are inserted to stabilize and monitor the air flow in the channel. All segments consist of 5° conical elements.
In
Figure 1 and
Figure 2, the considered setup is presented. In both (rotor and stator teeth) variants, the labyrinth teeth have a rectangular cross-section. The air is flowing from the left to the right. Four different
RC are applied for both variants. Dimensionless corrected radial clearance
RCCORR is used as a geometric parameter
where
RCF is the radial clearance between the shaft and the case without considering the presence of the teeth (e.g., 0.2 mm). All variants of
RCCORR are listed in
Table 1. The significance of the font colors is described in Chapter 0.
3. Simulation Mesh and Calculation
3.1. Simulation Mesh
All simulation meshes were prepared in the ANSYS Meshing software, the mapping mesh was applied. Based on the experience in [
13], the tetrahedral- and hexahedral-mapped mesh with influences in all walls was selected as a suitable variant. The mesh in the labyrinth seal segment has 2.4 million of elements in the inlet and in the outlet, segments have 0.5 million of elements. Twenty-five rows of elements were applied in the critical flow path in the location of the radial clearance. The calculation mesh example is in
Figure 3.
3.2. Boundary Conditions
The boundary conditions were set to the constant pressure ratio
πR that is defined by (2):
where
pTIN [Pa] is the inlet total pressure and
pSOUT [Pa] is the outlet static pressure.
The angular velocity ratio
ωC is defined by (3):
where
ωA [rad/s] is the current angular velocity and
ωF [rad/s] is the reference angular velocity, as it is in [
1] (e.g., 30e03 RPM). The list of the boundary conditions is in
Table 2:
The calculations were performed in the ANSYS CFX Software. The fluid was set as the air ideal gas with the ratio of specific heats k = 1.4. Based on [
8,
11,
14,
15], the standard two-equation k-ε turbulent model [
4] was set. The time step was set the same as in [
12]. In the rotor labyrinth teeth scheme (
Figure 1), the labyrinth seal segment was rotated and, in the opposite side “counter rotation wall” condition, (i.e., the same rotating speed at the opposite side) was defined in the stator wall. In the stator labyrinth teeth setup (
Figure 2), only the shaft wall without the teeth was rotated. Between segments is defined the interface wall. The total pressure inlet and static pressure outlet were defined, corresponding to
πR listed in
Table 2. On the sides, the periodical conditions were defined. The rotor boundary conditions are presented in
Figure 4 and the stator boundary conditions are presented in
Figure 5.
3.3. Calculation and Convergence
All calculations were finished after 1000 iterations, where the residuals were converged to the value lower than 1E-03. The residuals, turbulent, and mass flow were monitored. The turbulent parameters show a trend similar to the residuals (
Figure 6), i.e., less than 1E-03 (
Figure 7). The mass flow value convergence trend (
Figure 8) shows that at the inlet (pink curve) and at the outlet (green curve), the residuals are similar (the negative values here as the gas flows are out of the domain). It is possible to say that the calculation was converged well.
4. Calculation Discussion
The aim is to obtain deeper understanding of the thermodynamics in a turbine engine labyrinth seal at different angular velocities ωC, different radial clearances RCCORR, and different locations of the teeth. The total temperature, static pressure, total enthalpy, flow coefficient, kinetic energy, and density were chosen as evaluation parameters.
Based on the following Formulas (4)–(10), all average values of thermodynamic parameters were plotted.
Total temperature
where
TT [K] is the calculated total temperature and
TTF [K] is the reference total temperature.
Static pressure
where
pS [Pa] is the calculated static pressure and
pSF [Pa] is the reference static pressure.
Total enthalpy
where
hT [kJ/kg] is the total enthalpy and
hTF [kJ/kg] is the reference total enthalpy
Flow coefficient
where
Q [kg/s] is the calculated mass flow and
QC [kg/s] is the critical mass flow, defined by (8)
where
k is the Poisson constant [-],
r is the Gas constant [J/kg/K],
T0 [K] is the ambient total temperature, and
ρ0 is the ambient density [kg/m
3]. Ambient conditions are values near the teeth.
Kinetic energy
where
EkF is [kJ] is the reference kinetic energy.
Density
where
ρF [kg/m
3] is the reference density.
A list of reference conditions (subscripted with
F) is in
Table 3:
The above stated thermodynamic parameters are plotted in the following charts. The angular velocity
ωC is plotted in the x–axis in all charts. The calculated data contains both the rotor teeth and stator teeth variants. The RC
CORR values for the various teeth locations are listed in
Table 1. The total temperatures and the statics pressure fields for RC
CORR = 0.15 are presented in
Appendix A. The same characteristics for RC
CORR = 0.04 are presented in
Appendix B. The font colors listed in
Table 1 correspond to the colors used in the charts. The total temperature (based on (4)) trendlines are given in
Figure 9. Here, the trendlines with the identical radial clearances have similar development. The differences (of approximately 0.03) can be seen at high angular velocities at minimal radial clearance. With a small radial clearance, the teeth positions (stator or rotor) do not show any influence; the trendlines are similar.
It is possible to say that the dominant factor of the total temperature increase is the radial clearance value. In
Figure 10, the static pressure (based on (5)) trendlines are presented. It is visible that the static pressure is of nearly constant value. Similar results appear for different angular velocities, where only a small difference (approx. 0.05) at radial clearance 0.15 at maximal angular velocity is registered. With small radial clearance, the static pressure trendlines are moved to higher value (from 1.35 to 1.63). The main geometric parameter of the labyrinth seal to control the static pressure development is the radial clearance.
The total enthalpy trendlines (based on (6)) are given in
Figure 11. They correspond to the temperature trendline results. The main factor influencing the enthalpy is the radial clearance. The total enthalpy difference (approx. 0.05) at the highest angular velocity is similar to the total temperature difference. The flow coefficient trendlines (based on (7)) are given in
Figure 12. The kinetic energy trendlines (based on (9)) are given in
Figure 13. The density trendlines (based on (10)) are given in
Figure 14. The main consequences of radial clearance in the rotor and stator teeth location are shown. If the radial clearance is 0.04, the absolute value of the flow coefficient is higher than if the clearance is 0.15. The sealing effect of the labyrinth seal is better with a low radial clearance (0.04) and teeth on the rotor.
5. Conclusions
Typical thermodynamic parameters of a turbine engine labyrinth seal were simulated using the ANSYS CFX computational software. A constant number of four teeth was selected. The airflow in the labyrinth seal was modelled using the standard two-equations k-ε turbulence model. A study of the thermodynamic parameters was performed for four-geometry settings: two different teeth locations with two different radial clearances and for different angular velocities using the constant pressure ratio π
R = 2.467. Based on the convergence charts (
Figure 6,
Figure 7,
Figure 8), it was found that the numerical calculation method converged well and it is applicable to labyrinth seal computations. The results of the computations show that the total temperature (
Figure 9) and total enthalpy (
Figure 11) increase with increasing angular velocity. The next thermodynamic parameters, static pressure (
Figure 10) and flow coefficient (
Figure 12), correspond to expectations [
3,
11], i.e., static pressure decreases and total temperature increases. Similar results (i.e., kinetic energy increases and density decreases) were observed for the kinetic energy (
Figure 12) and density (
Figure 13). The labyrinth sealing effect (i.e., mass flow decrease) is not influenced by the angular velocity. The radial clearance has a dominant influence on the sealing effect; the location of teeth (i.e., the teeth either on the rotor or the stator) is of secondary importance. The phenomenon mentioned in all angular velocities can be seen, because in all plots the trendlines with the same radial clearance (RC
CORR = 0.04–orange and purple lines; RC
CORR = 0.15–blue and green lines) have a similar trend.
The study presented other thermodynamic materials for better design of aircraft turbine engines. Based on the labyrinth seal improvements, better engine design could be possible, improving so the performance parameters (i.e., turbine temperature, compressor pressure ratio, thrust or power, etc.).
Author Contributions
Writing—original draft, M.C.; Writing—review & editing, Z.P. and T.V. Software: M.C. and Z.P., Methodology M.C. and T.V. All authors have read and agreed to the published version of the manuscript.
Funding
This research received no external funding
Acknowledgments
Authors acknowledge the support of the ESIF, EU Operational Programme Research, Development and Education, and of the Center of Advanced Aerospace Technology (CZ.02.1.01/0.0/0.0/16_019/0000826), Faculty of Mechanical Engineering, Czech Technical University in Prague.
Conflicts of Interest
The authors declare no conflict of interest.
Appendix A. RCCORR = 0.15, Total Temperature and Static Pressure Fields
Table A1.
Total Temperature Fields.
Table A1.
Total Temperature Fields.
| ωC = 1e+00 | |
|---|
| Stator Teeth | Total Temperature | ![Applsci 10 06830 i001]() |
| Rotor Teeth | ![Applsci 10 06830 i002]() |
Table A2.
Static pressure fields.
Table A2.
Static pressure fields.
| ωC = 1e+00 | |
|---|
| Stator Teeth | Static Pressure | ![Applsci 10 06830 i003]() |
| Rotor Teeth | ![Applsci 10 06830 i004]() |
Table A3.
Total temperature fields.
Table A3.
Total temperature fields.
| ωC = 2e-02 | |
|---|
| Stator Teeth | Total Temperature | ![Applsci 10 06830 i005]() |
| Rotor Teeth | ![Applsci 10 06830 i006]() |
Table A4.
Static pressure fields.
Table A4.
Static pressure fields.
| ωC = 2e-02 | |
|---|
| Stator Teeth | Static Pressure | ![Applsci 10 06830 i007]() |
| Rotor Teeth | ![Applsci 10 06830 i008]() |
Appendix B. RCCORR = 0.04, Total Temperature and Static Pressure Fields
Table A5.
Total temperature fields.
Table A5.
Total temperature fields.
| ωC = 1e+00 | |
|---|
| Stator Teeth | Total Temperature | ![Applsci 10 06830 i009]() |
| Rotor Teeth | ![Applsci 10 06830 i010]() |
Table A6.
Static pressure fields.
Table A6.
Static pressure fields.
| ωC = 1e+00 | |
|---|
| Stator Teeth | Static Pressure | ![Applsci 10 06830 i011]() |
| Rotor Teeth | ![Applsci 10 06830 i012]() |
Table A7.
Total temperature fields.
Table A7.
Total temperature fields.
| ωC = 2e-02 | |
|---|
| Stator Teeth | Total Temperature | ![Applsci 10 06830 i013]() |
| Rotor Teeth | ![Applsci 10 06830 i014]() |
Table A8.
Static pressure fields.
Table A8.
Static pressure fields.
| ωC = 2e-02 | |
|---|
| Stator Teeth | Static Pressure | ![Applsci 10 06830 i015]() |
| Rotor Teeth | ![Applsci 10 06830 i016]() |
References
- Kerrebrock, J.L. The Turbojet. In Aircraft Engines and Gas Turbines; Massachusetts Institute of Technology: Cambridge, MA, USA, 1992; pp. 36–43. [Google Scholar]
- Eser, D.; Kazakia, J.Y. Air flow in cavities of labyrinth seals. Int. J. Eng. Sci. 1995, 33, 2309–2326. [Google Scholar] [CrossRef]
- Sultanian, B.K. Labyrinth Seals. Internal Flow Systems Modeling; Cambridge University Press: Cambridge, UK, 2018; pp. 237–256. [Google Scholar]
- Han, J.-C.; Dutta, S.; Ekkad, S. Numerical Modeling. Gas Turbine, Heat Transfer, and Cooling Technology; CRC Press: Boca Raton, FL, USA, 2013; pp. 771–830. [Google Scholar]
- Moustapha, H.; Zelesky, M.F.; Baines, N.C.; Japikse, D. Turbine Durability and Cooling. Axial and Radial Turbines; Concept NREC: White River Junction, VT, USA, 2003; pp. 145–190. [Google Scholar]
- Soares, C. Cooling and Load Bearing Systems. In Gas Turbines: A Handbook of Air, Land and Sea Applications; Butterworth Heinemann: Waltham, MA, USA, 2015; pp. 255–278. [Google Scholar]
- Meherwan, P. Boyce, Non-contacting Seal. In Gas Turbine Engineering Handbook; Butterworth Heinemann: Waltham, MA, USA, 2012; pp. 579–585. [Google Scholar]
- Kurzke, J.; Halliwell, I. Secondary Air System. In Propulsion and Power; Springer Internationally Publishing: Cham, Switzerland, 2018; pp. 687–696. [Google Scholar]
- Ilieva, G.; Pirovsky, C. Labyrinth seals with application to turbomachinery. Mater. Sci. Eng. Technol. 2019, 50, 479–491. [Google Scholar] [CrossRef]
- Furst, J. Numerical simulation of flows through Labyrinth seals. Appl. Mech. Mater. 2015, 826, 16–22. [Google Scholar] [CrossRef]
- Subramanian, S.; Sekhar, A.V.; Prasad, B. Assessment of Analytical Predictions for Radial Growth of Rotating Labyrinth Seals. Int. J. Turbo Jet Engines 2018, 35, 265–279. [Google Scholar] [CrossRef]
- Čížek, M.; Pátek, Z. On CFD Investigation of Radial Clearance of Labyrinth Seals of a Turbine Engine. Acta Polytech. 2020, 60, 38–48. [Google Scholar] [CrossRef]
- Čížek, M.; Vampola, T. Labyrinth Seal of Aircraft Turbine Engine Air Flow Calculation at High Viskosity. Acta Mech. Slovaca 2019, 23, 6–12. [Google Scholar] [CrossRef]
- Selvaraji, M.; Sam, P.J.; Nirmal, N. Optimization of Labyrinth Seal for Screw Compressor. In Proceedings of the ASME/JSME 2007 Thermal Engineering Summer Heat Transfer Conference, Vancouver, BC, Canada, 8–12 July 2007; pp. 969–975. [Google Scholar] [CrossRef]
- ANSYS Help Viewer 18.0. 2016. Available online: https://ansyshelp.ansys.com/account/secured?returnurl=/Views/Secured/corp/v201/en/wb2_help/wb2_help.html%23wb2_help (accessed on 25 September 2020).
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).