Multimode Guided Wave Detection for Various Composite Damage Types
Abstract
:1. Introduction
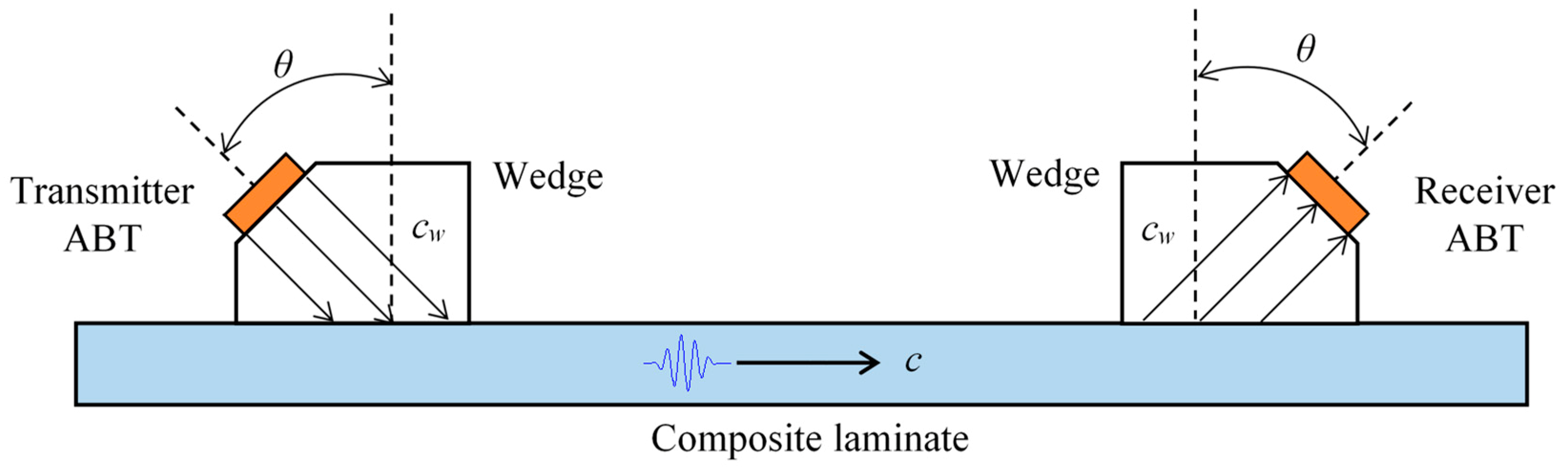
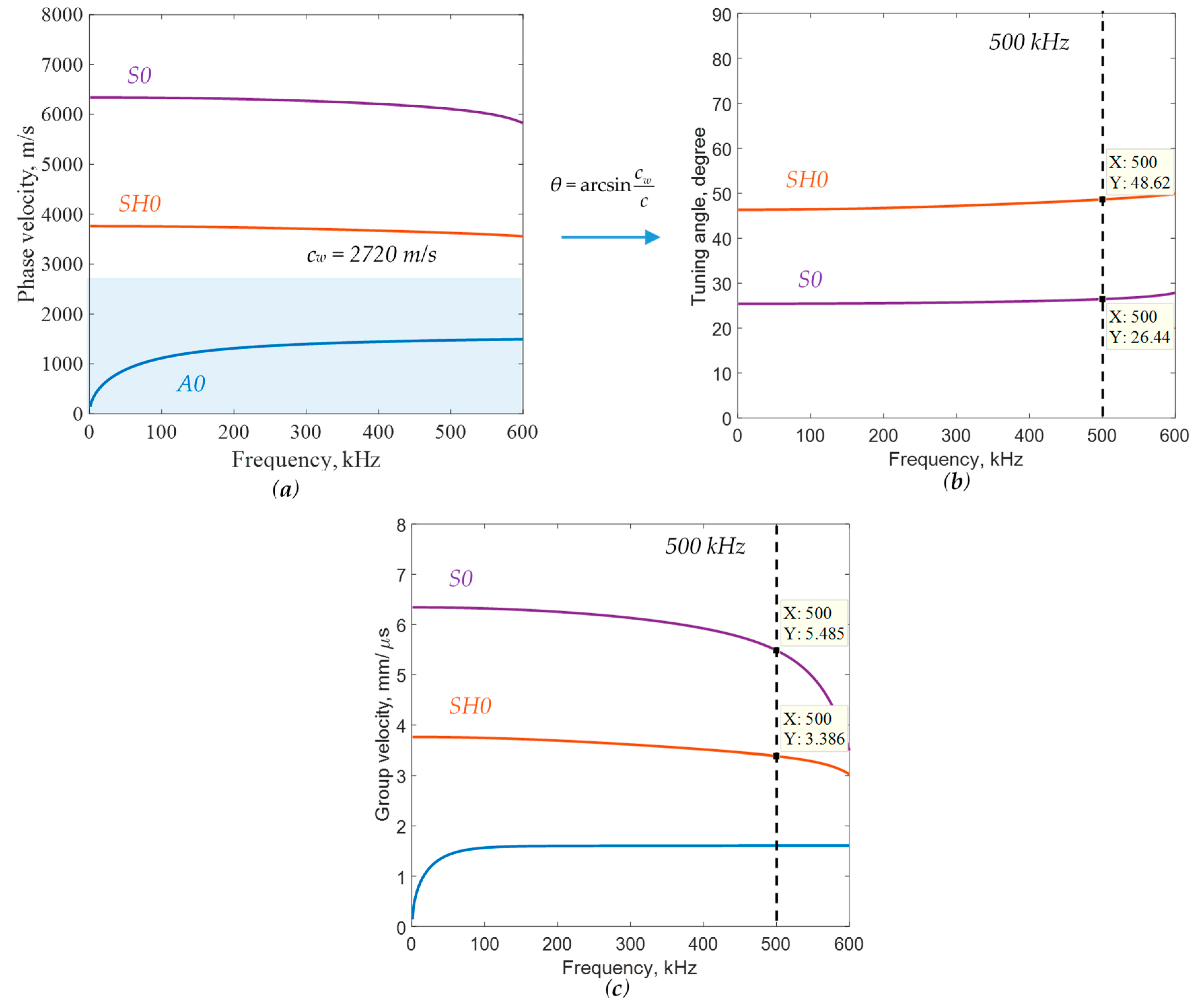
2. The Theory of Angle Beam Transducers for Single-Mode Excitation
3. Detection of Various Damage Types Using ABT–ABT on a 2-mm Quasi-Isotropic Composite Plate
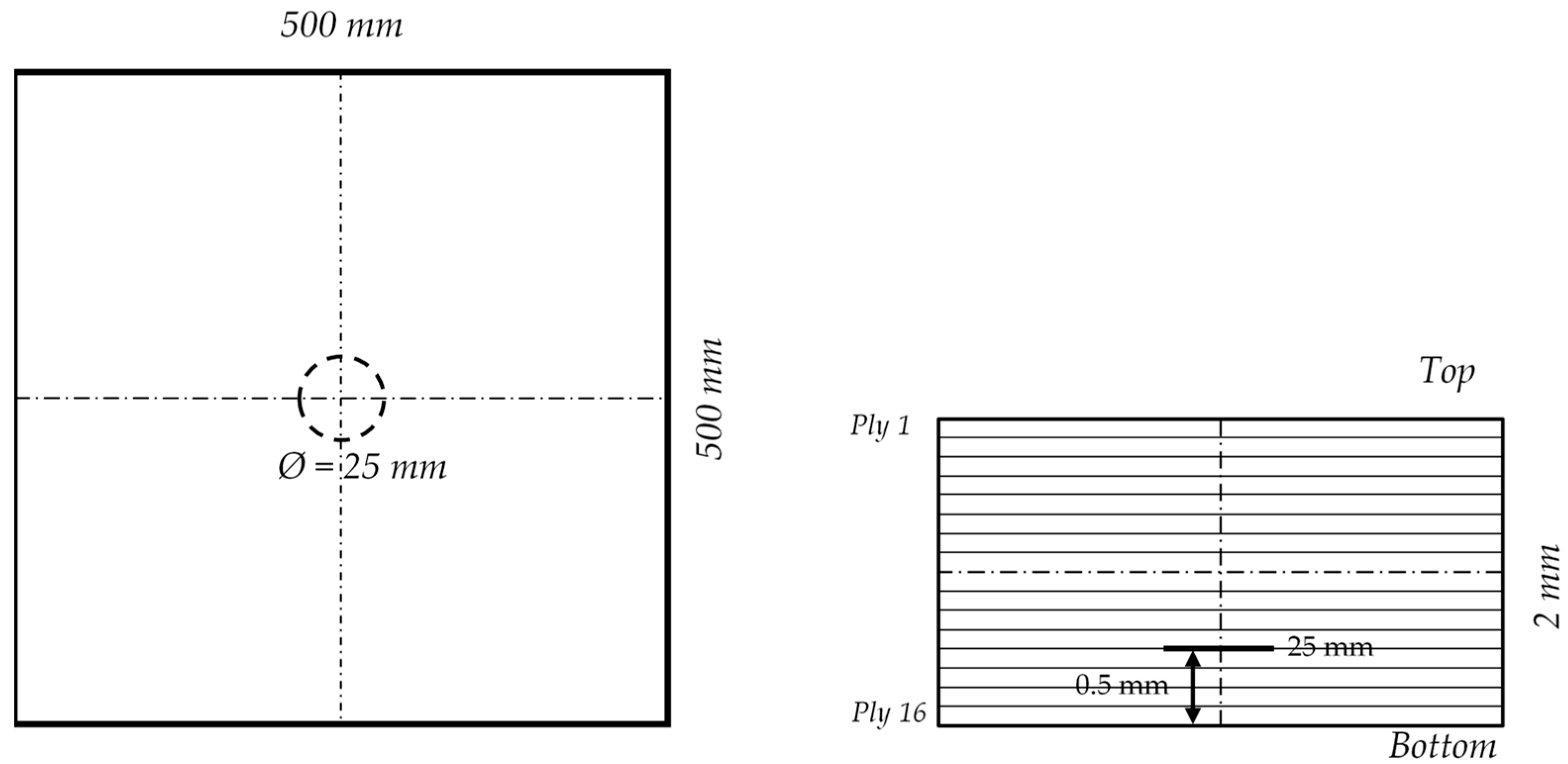
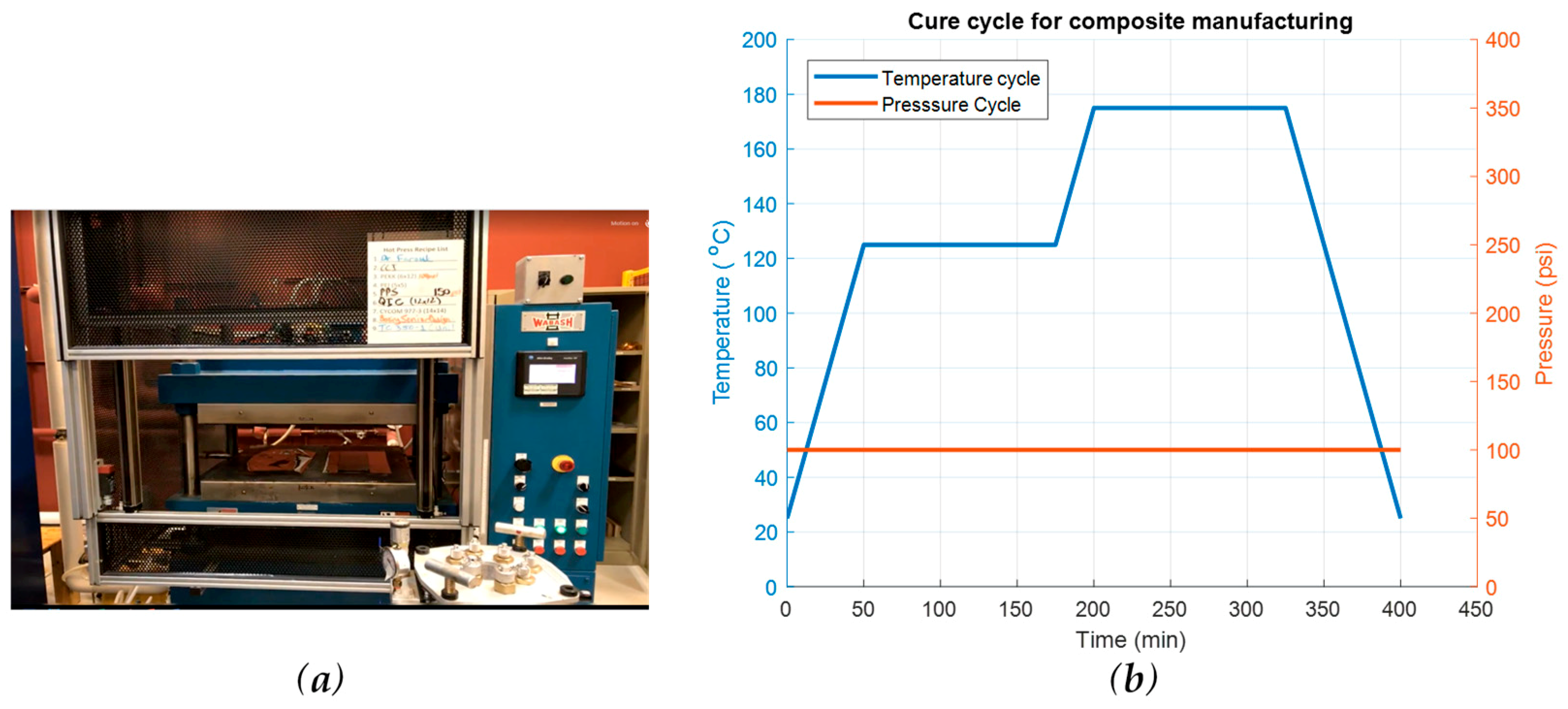
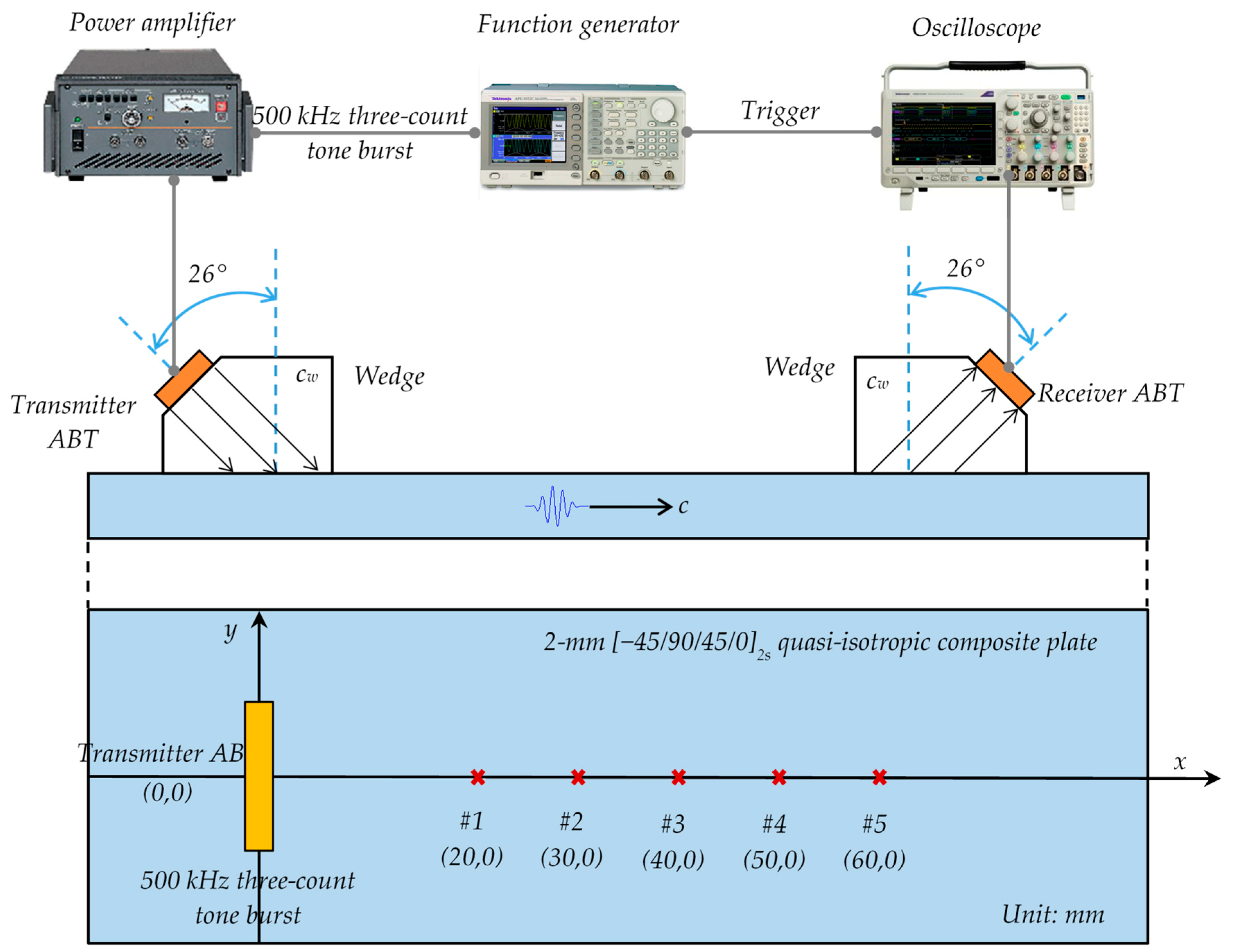
3.1. Composite Specimen
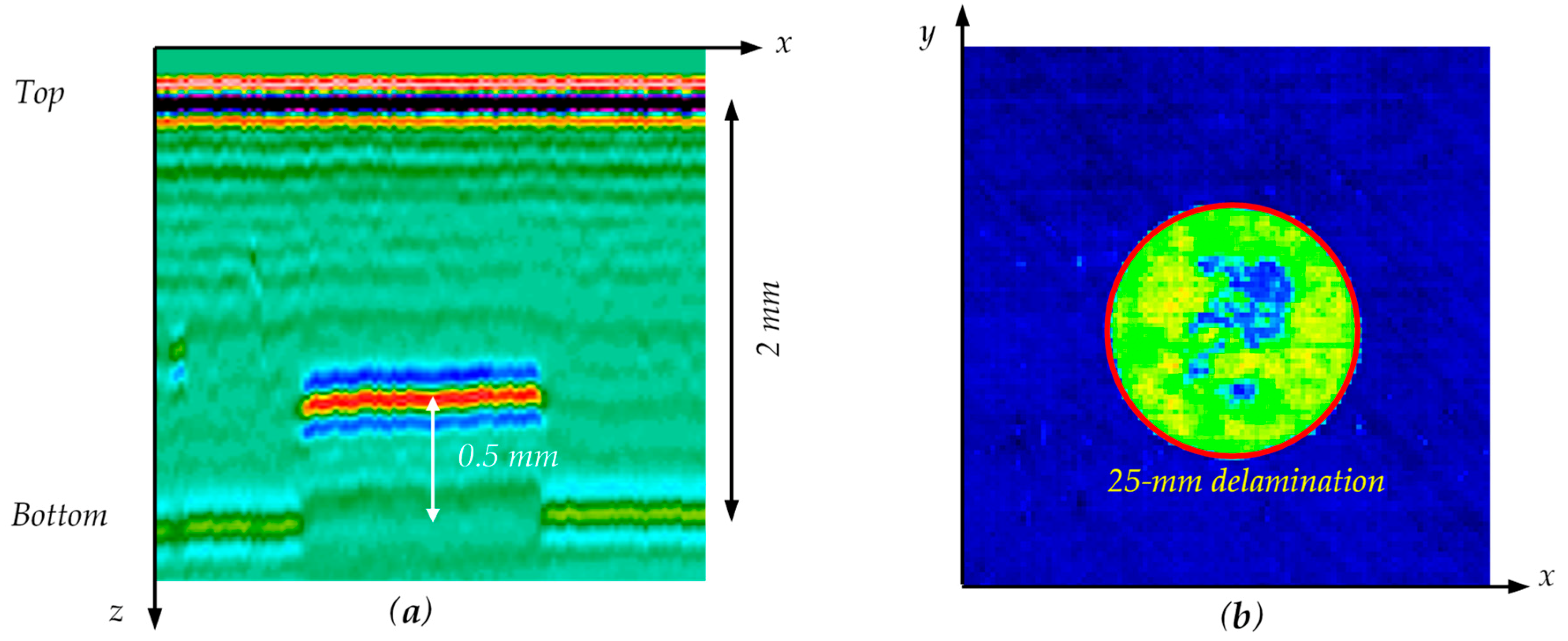
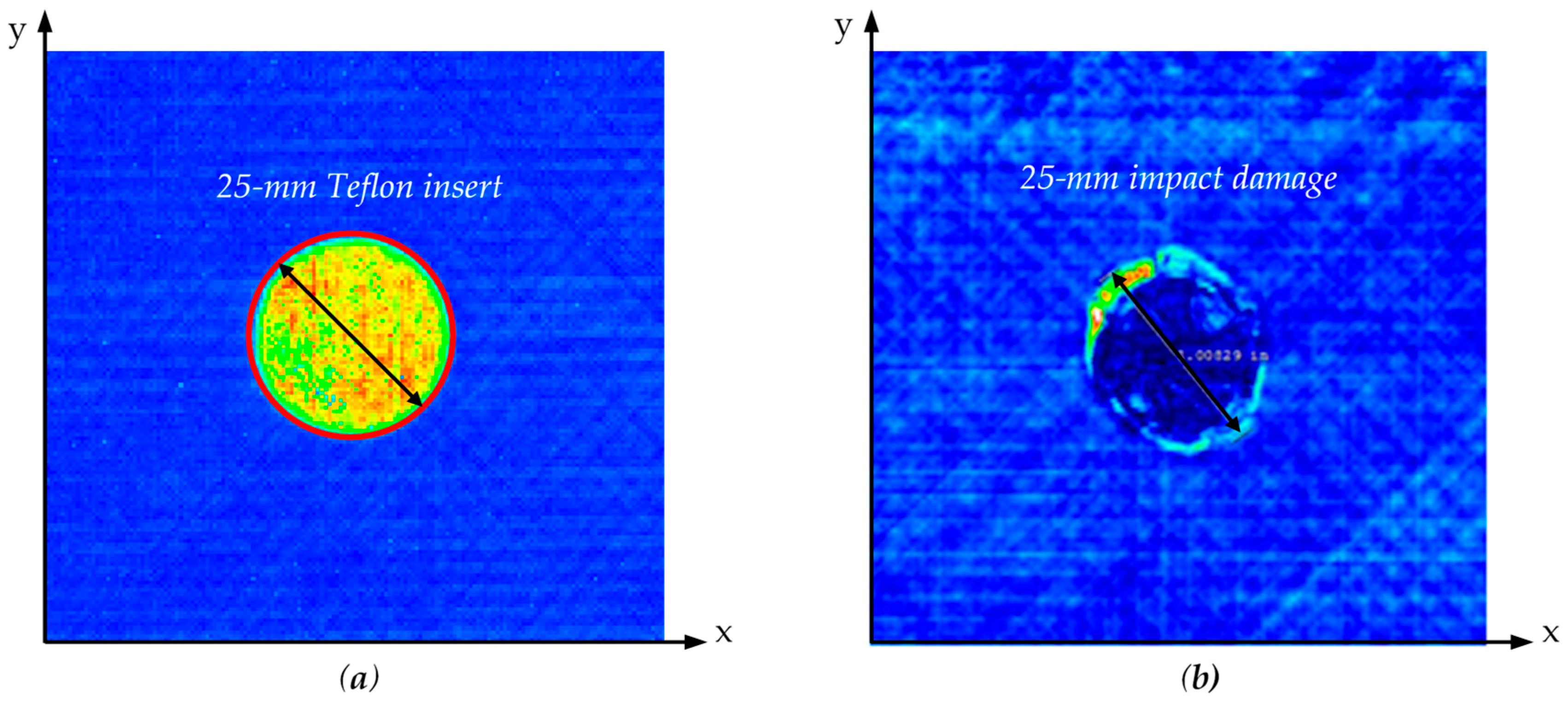
3.1.1. Simulated Delamination
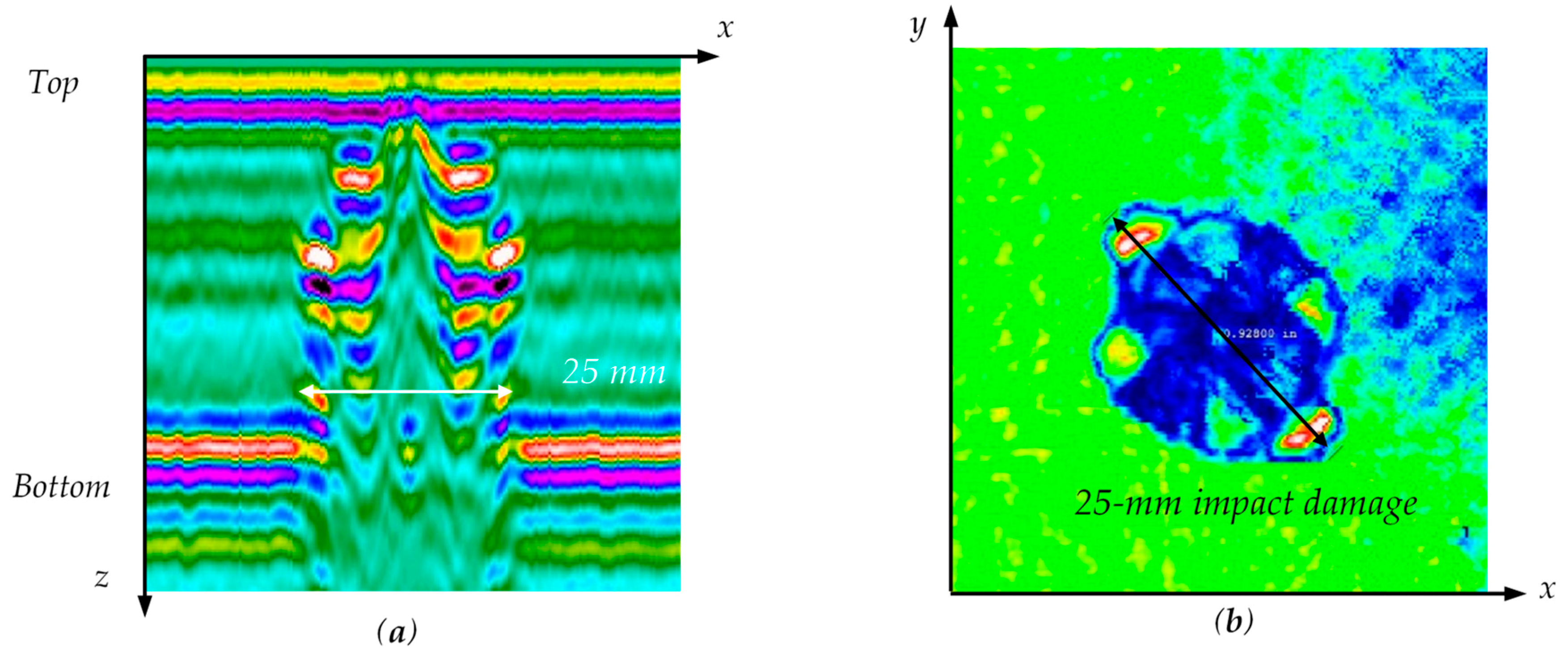
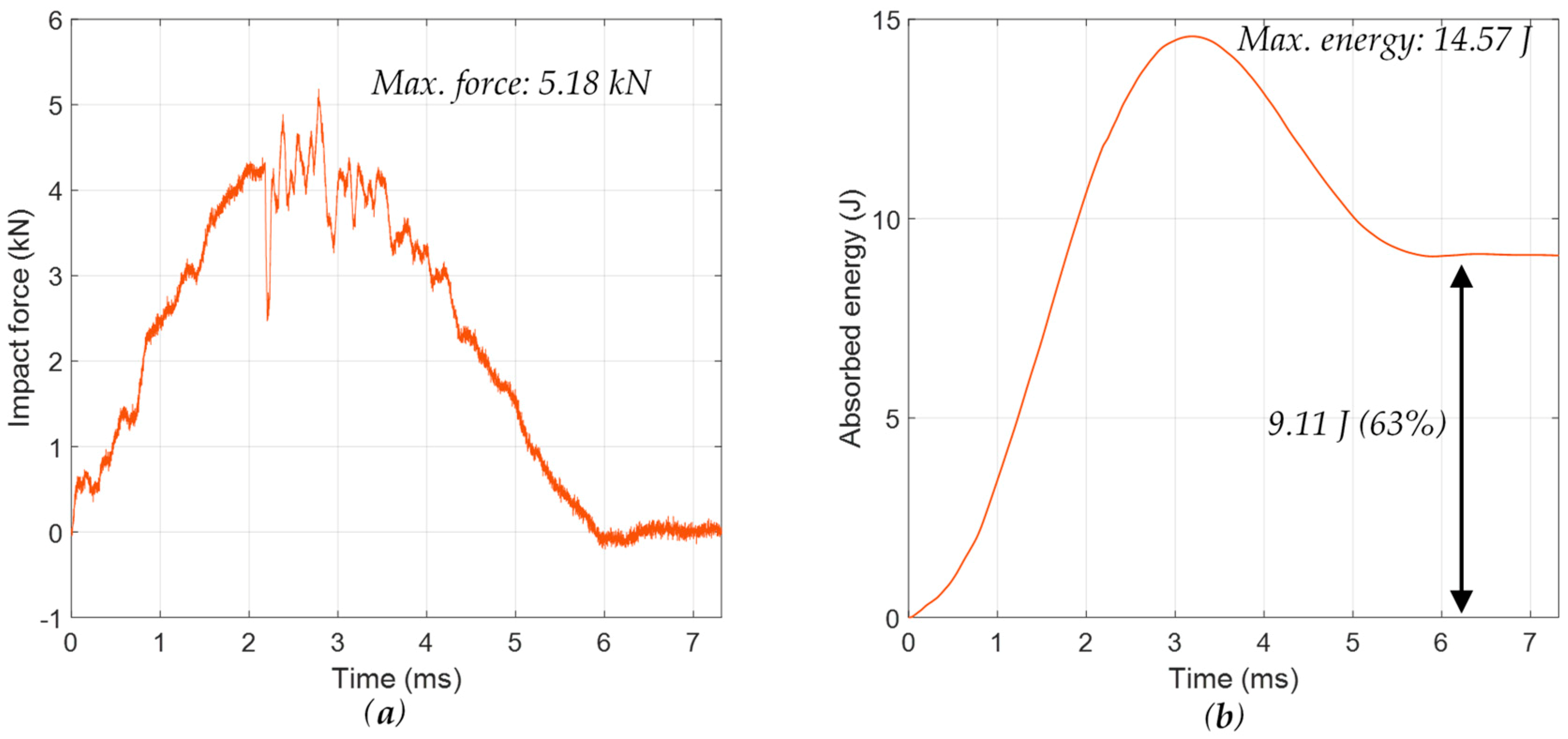
3.1.2. Impact Damage Due to Low-Velocity Impact
3.2. Pure Mode Generation Using ABT–ABT
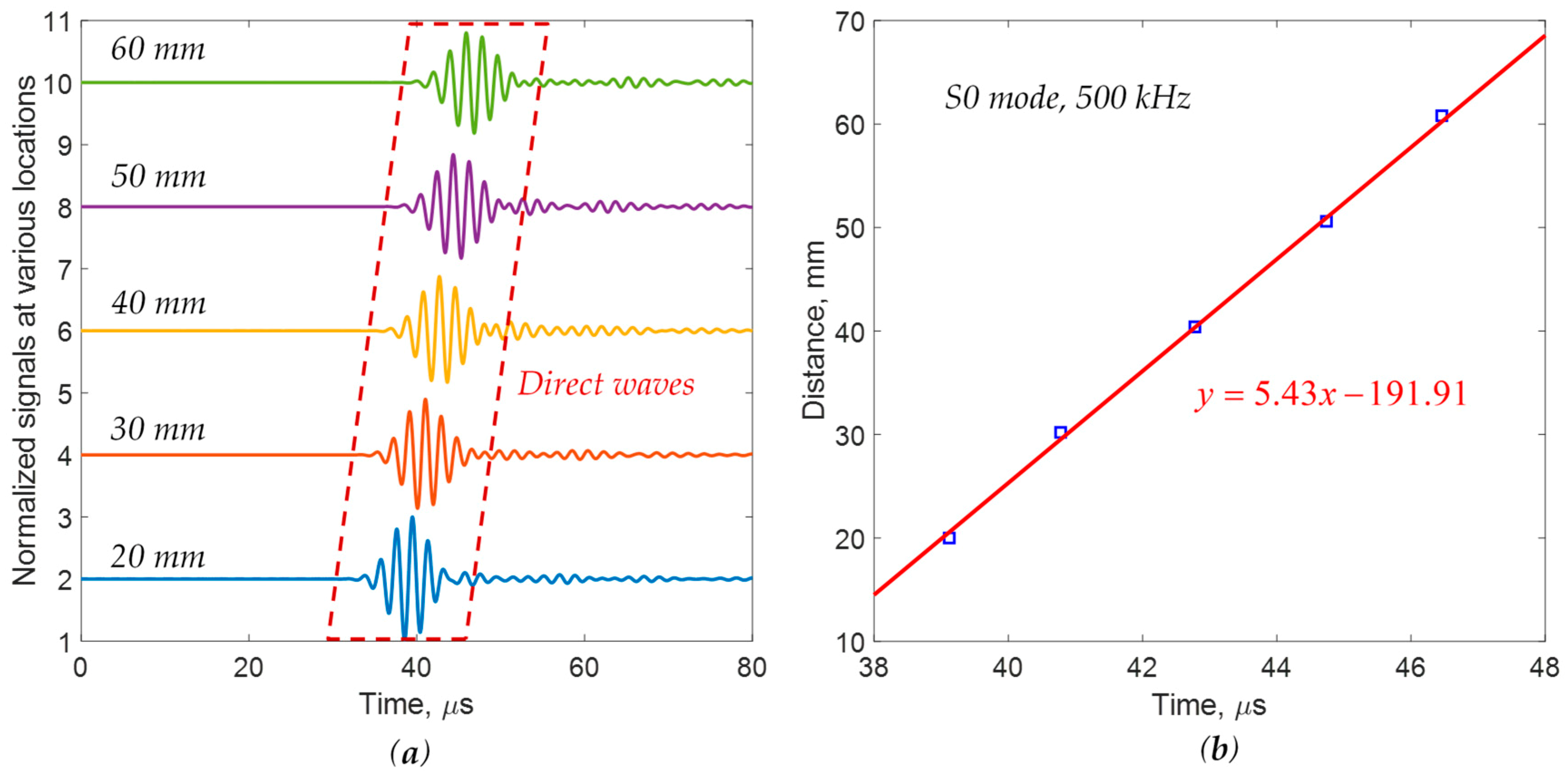
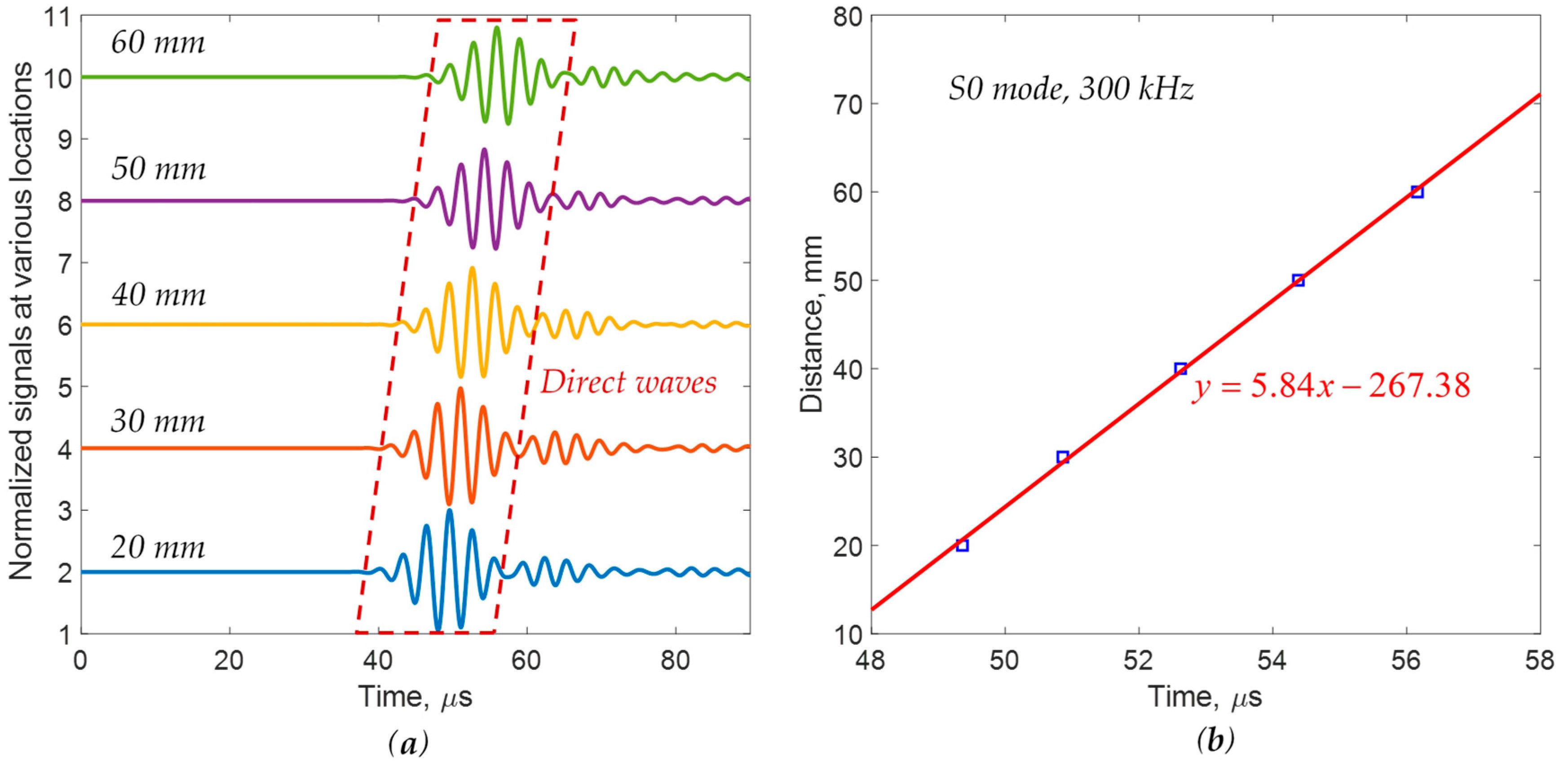
3.2.1. Pure S0 Mode Generation
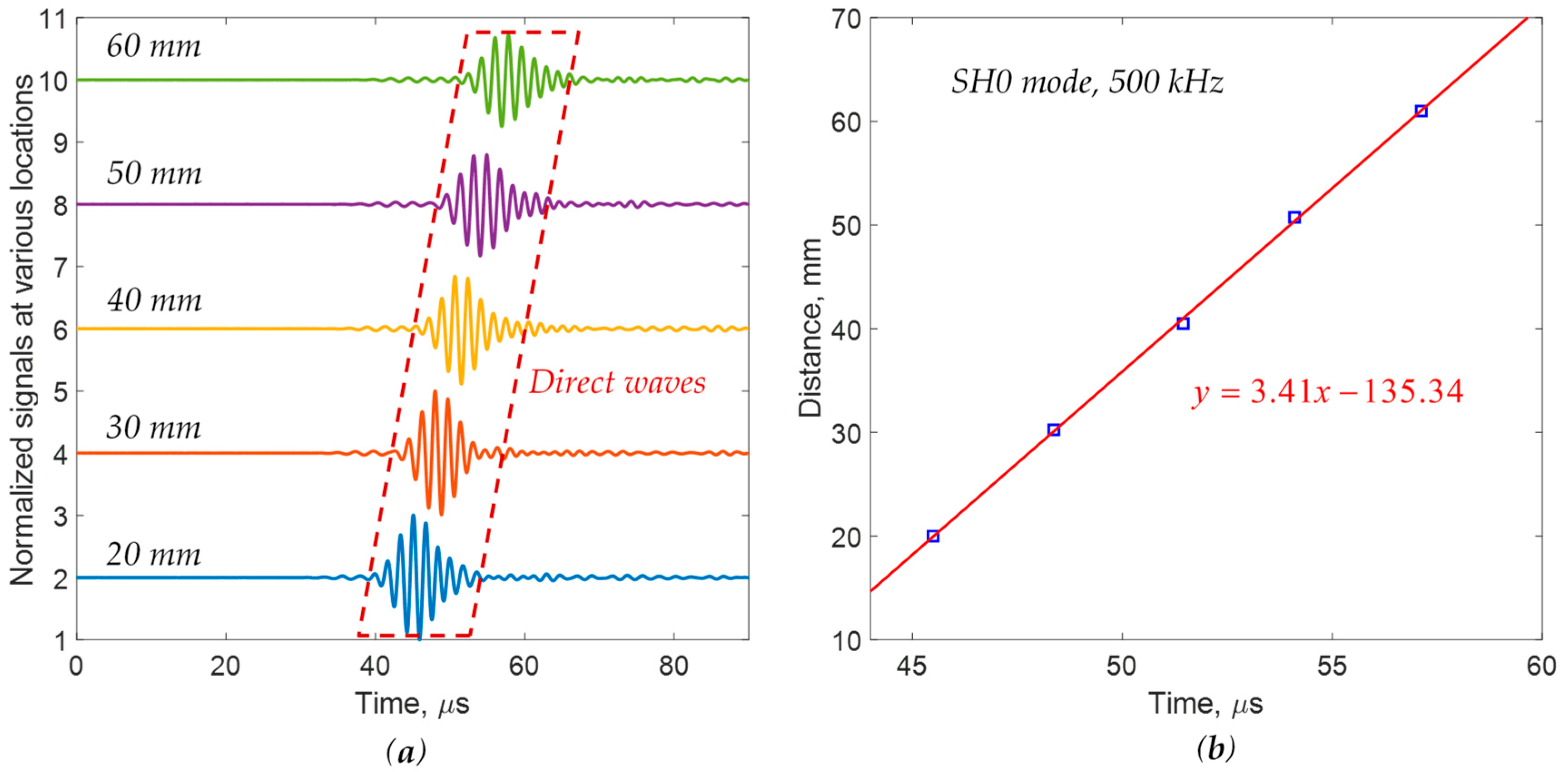
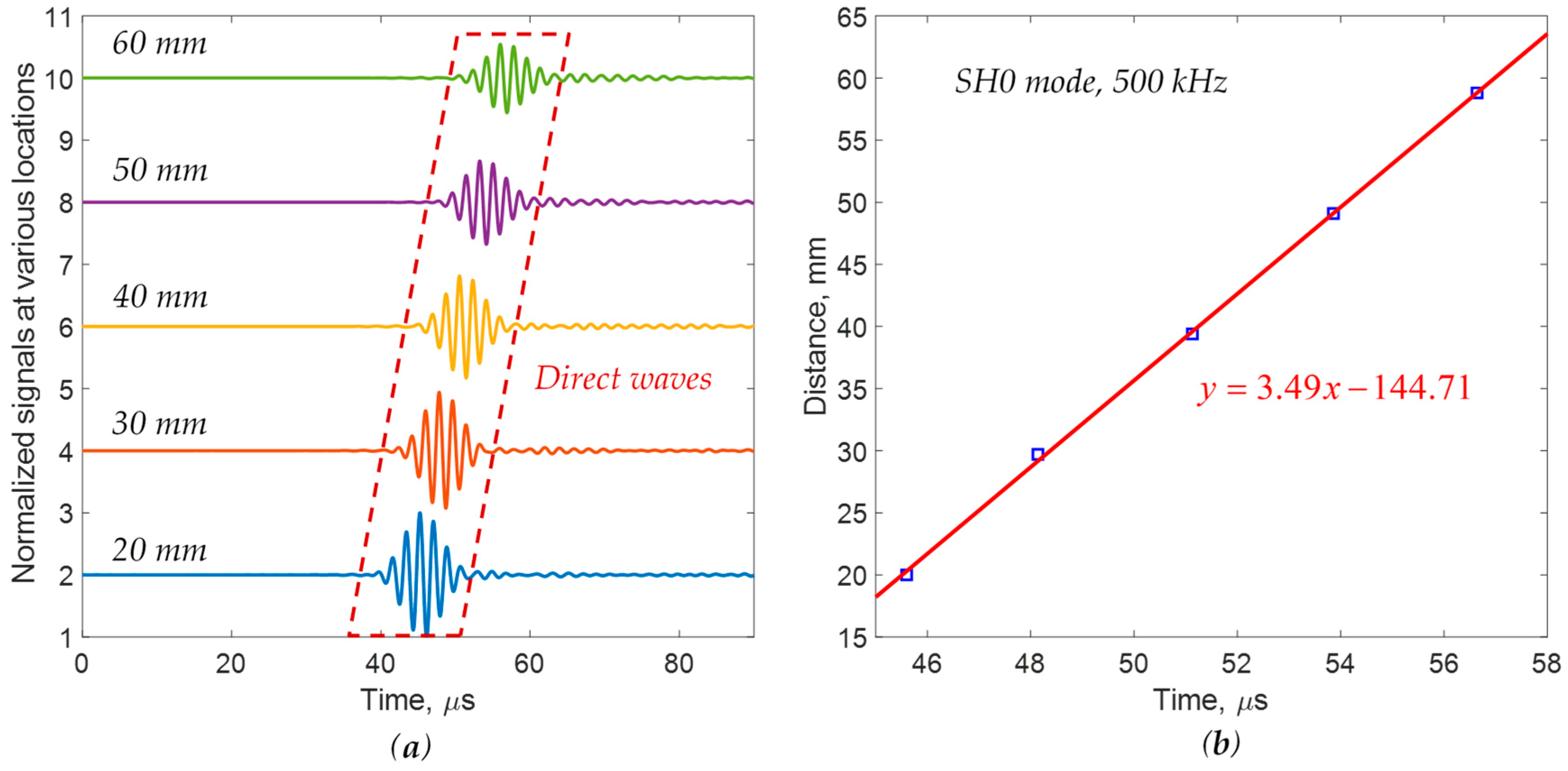
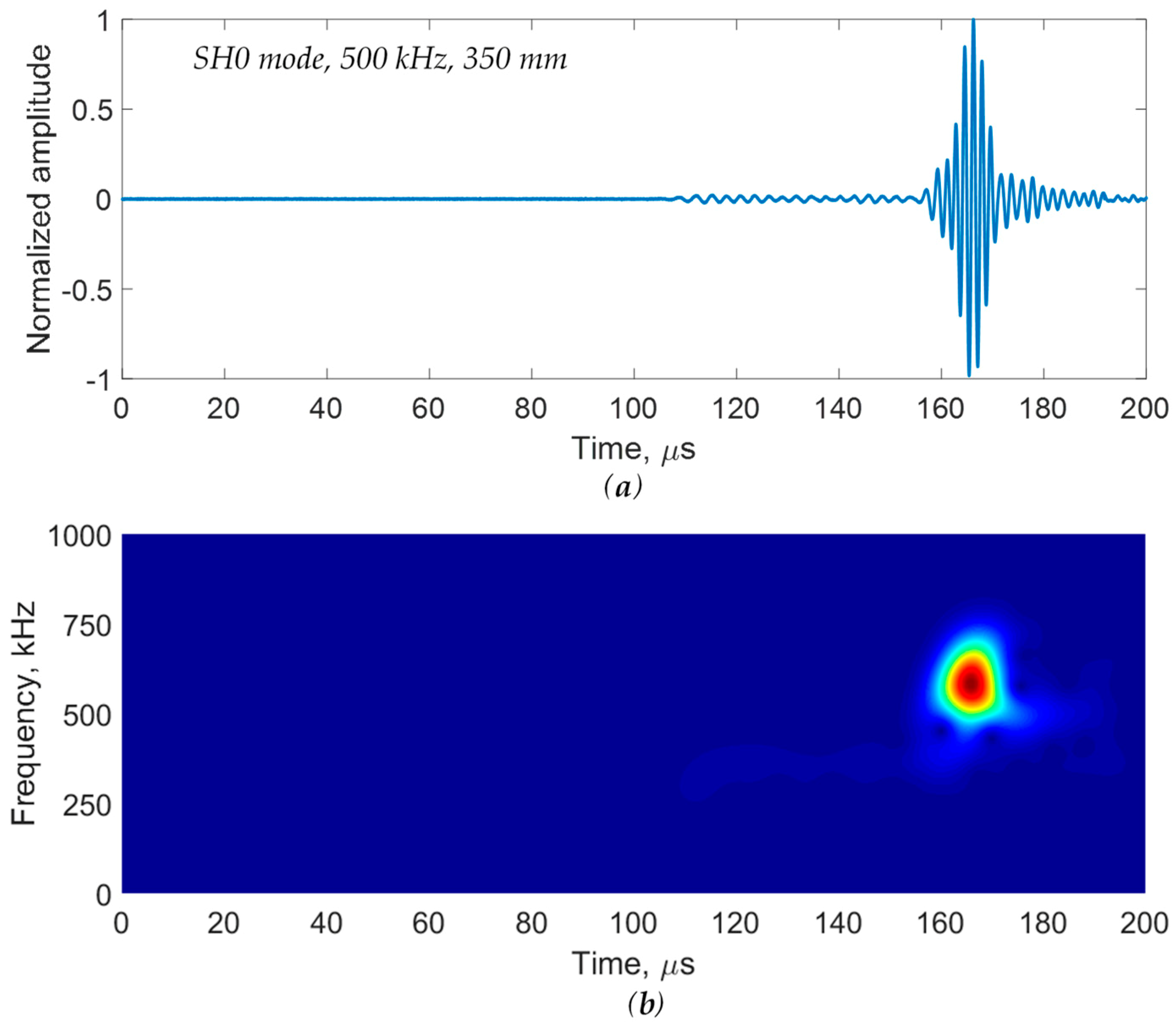
3.2.2. Pure SH0 Mode Generation
3.3. Damage Detection Using Multiple Pure-Mode Guided Waves
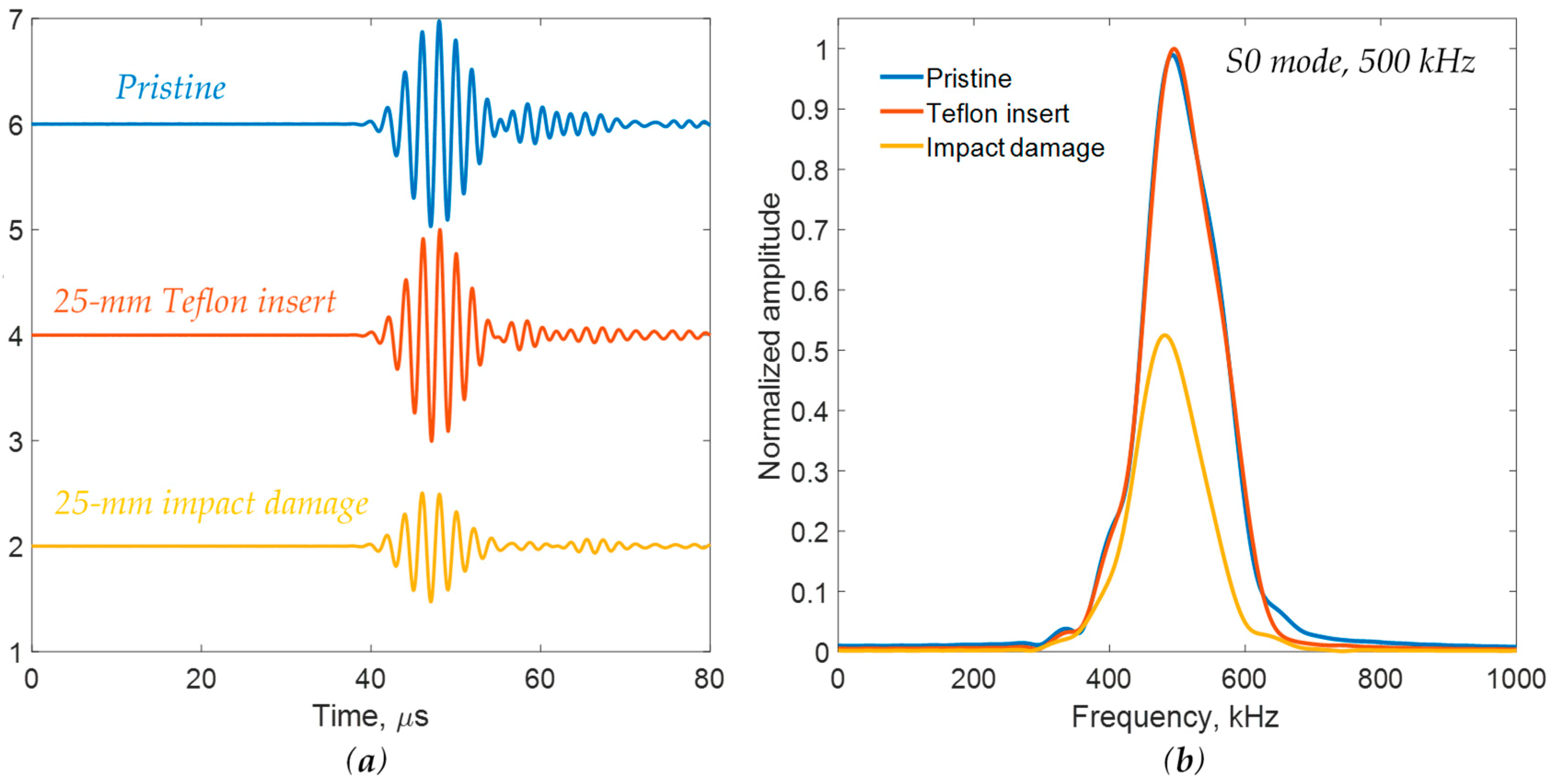
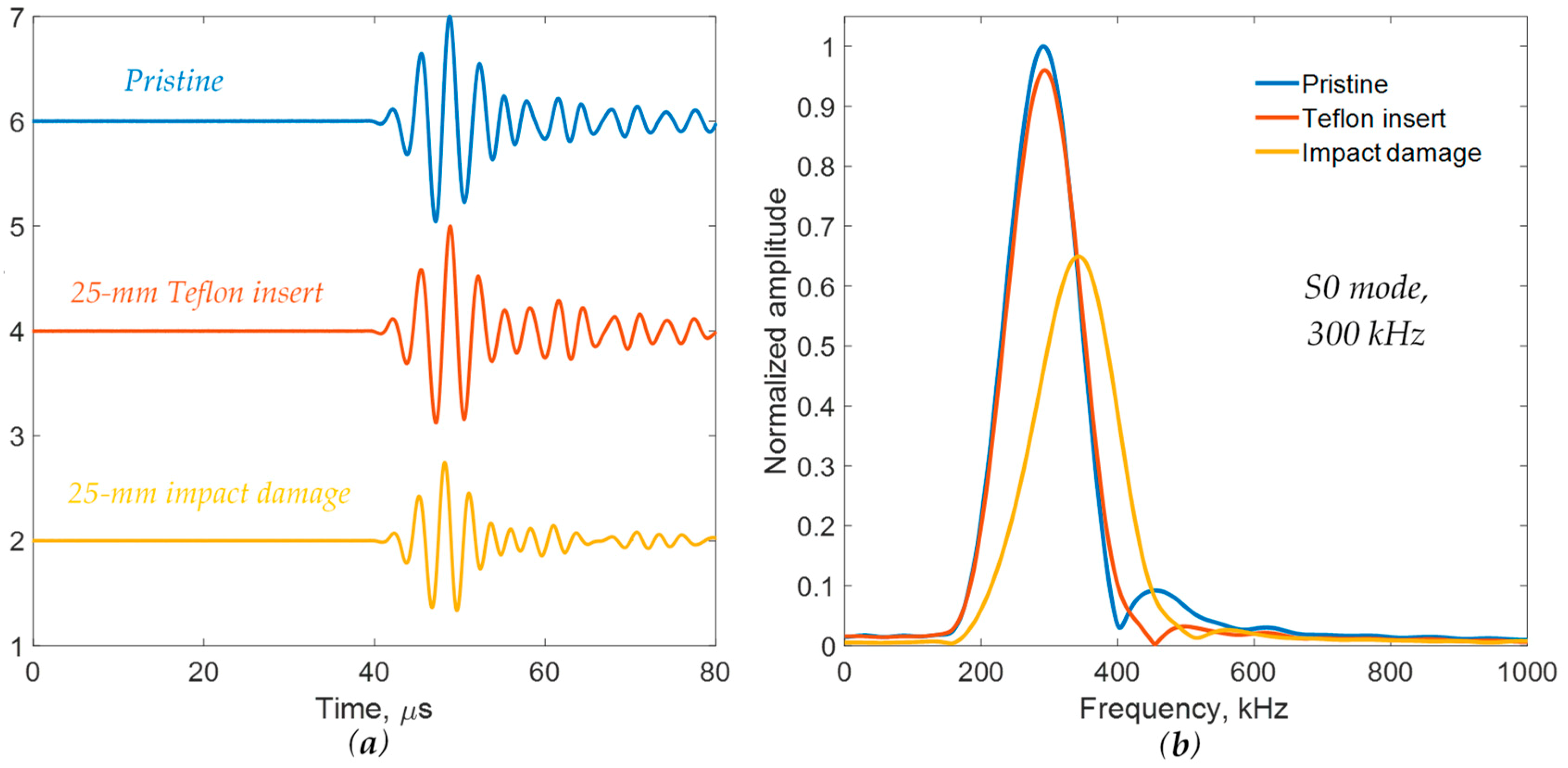
3.3.1. Damage Detection Using Pure S0 Mode
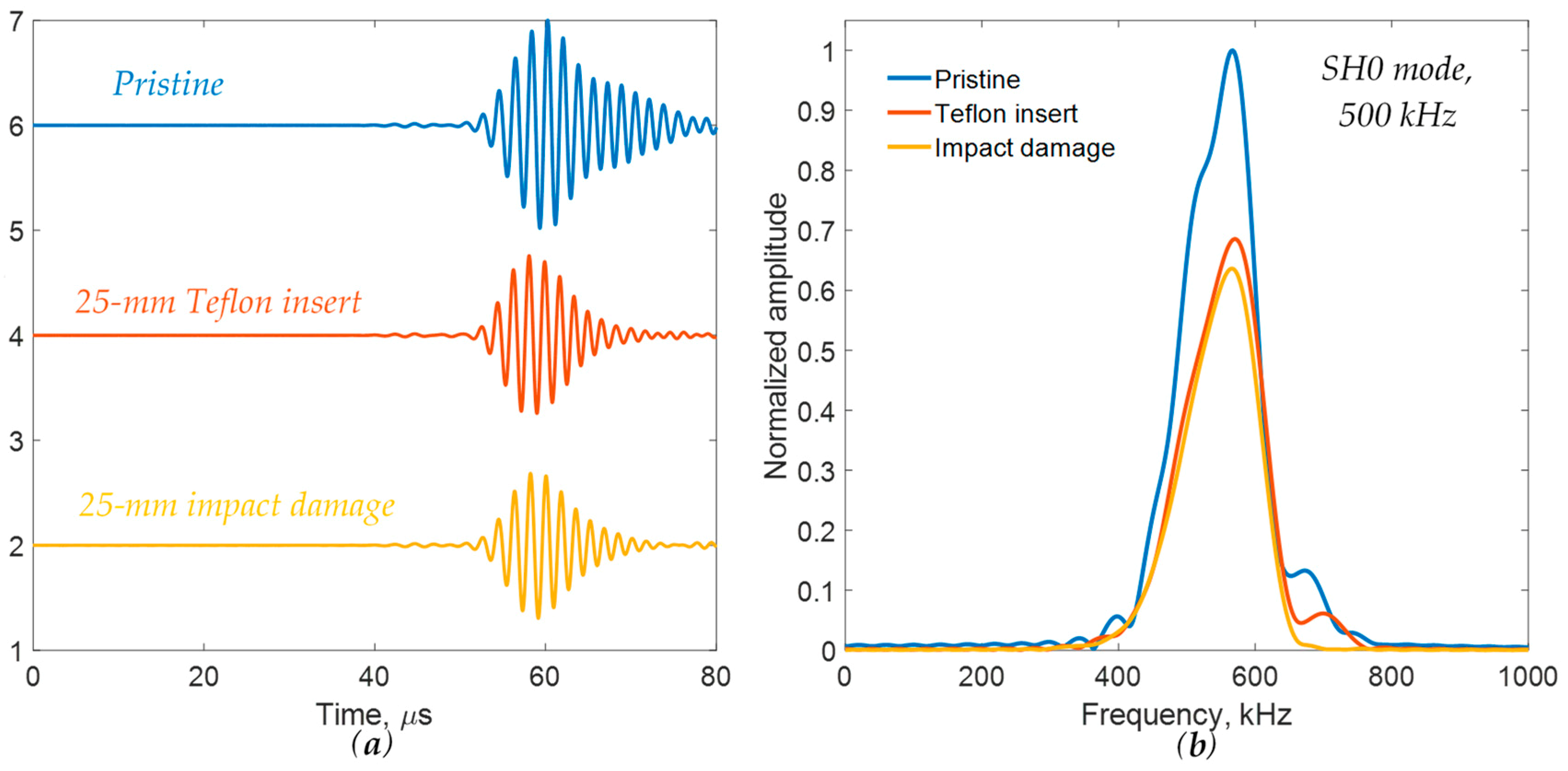
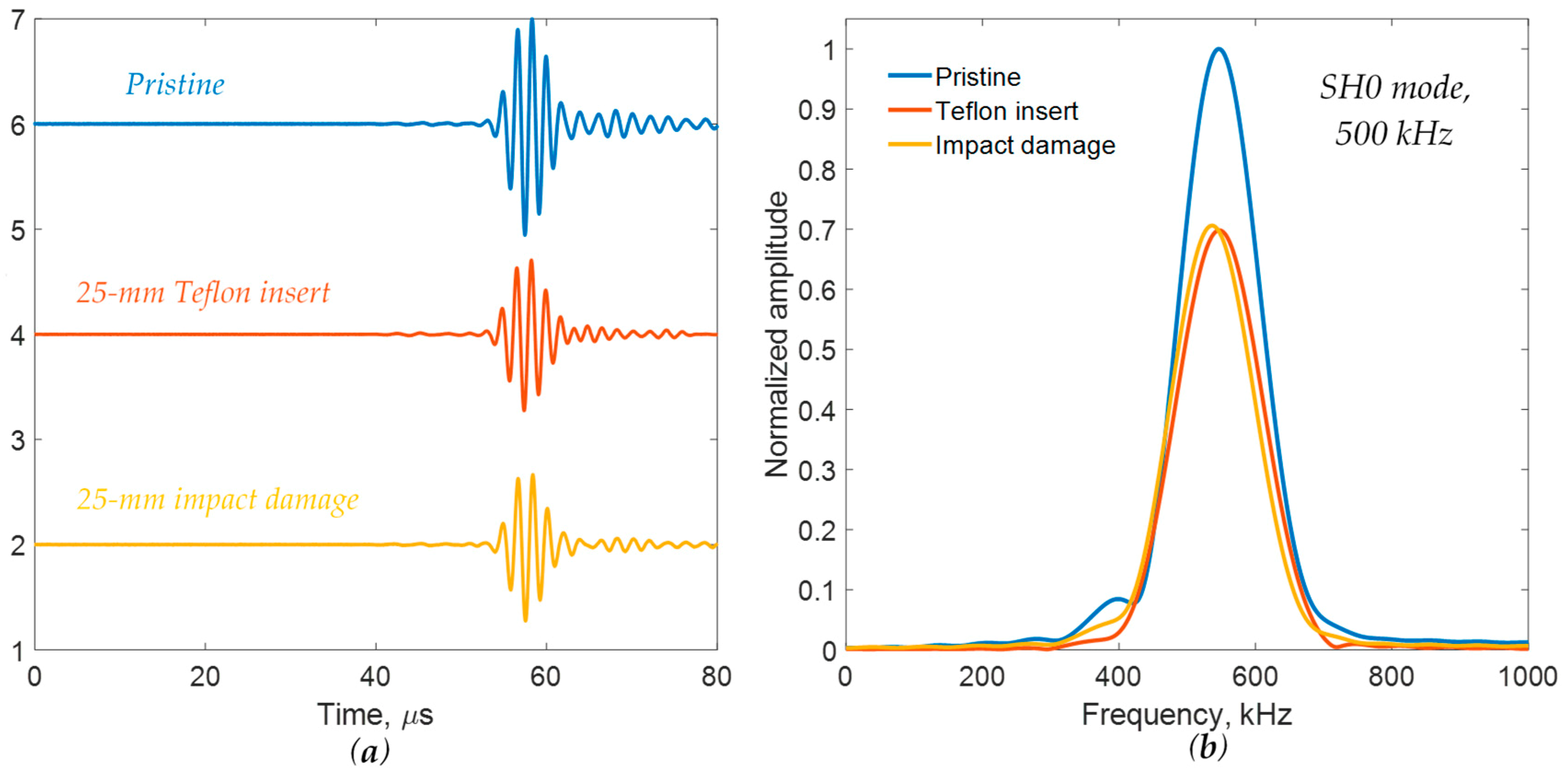
3.3.2. Damage Detection Using Pure SH0 Mode
4. Detection of Various Damage Types Using ABT–ABT on a 3-mm Quasi-Isotropic Composite Plate
4.1. Composite Specimen
4.2. Pure Mode Generation
4.2.1. Pure S0 Mode Generation
4.2.2. Pure SH0 Mode Generation
4.3. Multimode Guided Wave Detection
4.3.1. Damage Detection Using Pure S0 Mode
4.3.2. Damage Detection Using Pure SH0 Mode
5. Summary, Conclusions, and Future Work
5.1. Summary
5.2. Conclusions
5.3. Future Work
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Giurgiutiu, V. Structural Health Monitoring of Aerospace Composites; Elsevier Academic Press: Cambridge, MA, USA, 2016. [Google Scholar]
- Mei, H.; Migot, A.; Haider, M.F.; Joseph, R.; Bhuiyan, M.Y.; Giurgiutiu, V. Vibration-Based In-Situ Detection and Quantification of Delamination in Composite Plates. Sensors 2019, 19, 1734. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Flores, M.; Mollenhauer, D.; Runatunga, V.; Beberniss, T.; Rapking, D.; Pankow, M. High-speed 3D digital image correlation of low-velocity impacts on composite plates. Compos. Part B Eng. 2017, 131, 153–164. [Google Scholar] [CrossRef]
- Yuan, S.; Ren, Y.; Qiu, L.; Mei, H. A multi-response-based wireless impact monitoring network for aircraft composite structures. IEEE Trans. Ind. Electron. 2016, 63, 7712–7722. [Google Scholar] [CrossRef]
- Mei, H.; Giurgiutiu, V. Guided wave excitation and propagation in damped composite plates. Struct. Health Monit. 2019, 18, 690–714. [Google Scholar] [CrossRef]
- Wu, J.; Xu, C.; Qi, B.; Hernandez, F. Detection of impact damage on PVA-ECC beam using infrared thermography. Appl. Sci. 2018, 8, 839. [Google Scholar] [CrossRef] [Green Version]
- James, R.; Faisal Haider, M.; Giurgiutiu, V.; Lilienthal, D. A Simulative and Experimental Approach Toward Eddy Current Nondestructive Evaluation of Manufacturing Flaws and Operational Damage in CFRP Composites. J. Nondestruct. Eval. Diagn. Progn. Eng. Syst. 2020, 3. [Google Scholar] [CrossRef]
- Wallentine, S.M.; Uchic, M.D. A study on ground truth data for impact damaged polymer matrix composites. AIP Conf. Proc. 2018, 1949, 120002. [Google Scholar] [CrossRef]
- Nikbakht, M.; Yousefi, J.; Hosseini-Toudeshky, H.; Minak, G. Delamination evaluation of composite laminates with different interface fiber orientations using acoustic emission features and micro visualization. Compos. Part B Eng. 2017, 113, 185–196. [Google Scholar] [CrossRef]
- Fiborek, P.; Malinowski, P.H.; Kudela, P.; Wandowski, T.; Ostachowicz, W.M. Time-domain spectral element method for modelling of the electromechanical impedance of disbonded composites. J. Intell. Mater. Syst. Struct. 2018, 29, 3214–3221. [Google Scholar] [CrossRef]
- Mei, H.; Haider, M.F.; Joseph, R.; Migot, A.; Giurgiutiu, V. Recent advances in piezoelectric wafer active sensors for structural health monitoring applications. Sensors 2019, 19, 383. [Google Scholar] [CrossRef] [Green Version]
- Tian, Z.; Yu, L.; Leckey, C. Delamination detection and quantification on laminated composite structures with Lamb waves and wavenumber analysis. J. Intell. Mater. Syst. Struct. 2015, 26, 1723–1738. [Google Scholar] [CrossRef]
- Park, B.; An, Y.K.; Sohn, H. Visualization of hidden delamination and debonding in composites through noncontact laser ultrasonic scanning. Compos. Sci. Technol. 2014, 100, 10–18. [Google Scholar] [CrossRef]
- Sikdar, S.; Fiborek, P.; Kudela, P.; Banerjee, S.; Ostachowicz, W. Effects of debonding on Lamb wave propagation in a bonded composite structure under variable temperature conditions. Smart Mater. Struct. 2018, 28, 015021. [Google Scholar] [CrossRef]
- Girolamo, D.; Chang, H.Y.; Yuan, F.G. Impact damage visualization in a honeycomb composite panel through laser inspection using zero-lag cross-correlation imaging condition. Ultrasonics 2018, 87, 152–165. [Google Scholar] [CrossRef] [PubMed]
- Rogge, M.D.; Leckey, C.A. Characterization of impact damage in composite laminates using guided wavefield imaging and local wavenumber domain analysis. Ultrasonics 2013, 53, 1217–1226. [Google Scholar] [CrossRef]
- Kudela, P.; Radzienski, M.; Ostachowicz, W. Impact induced damage assessment by means of Lamb wave image processing. Mech. Syst. Signal Process. 2018, 102, 23–36. [Google Scholar] [CrossRef]
- Sikdar, S.; Kudela, P.; Radzieński, M.; Kundu, A.; Ostachowicz, W. Online detection of barely visible low-speed impact damage in 3D-core sandwich composite structure. Compos. Struct. 2018, 185, 646–655. [Google Scholar] [CrossRef] [Green Version]
- Xu, B.; Giurgiutiu, V. Single mode tuning effects on Lamb wave time reversal with piezoelectric wafer active sensors for structural health monitoring. J. Nondestruct. Eval. 2007, 26, 123–134. [Google Scholar] [CrossRef] [Green Version]
- Wang, L.; Yuan, F.G. Group velocity and characteristic wave curves of Lamb waves in composites: Modeling and experiments. Compos. Sci. Technol. 2007, 67, 1370–1384. [Google Scholar] [CrossRef]
- Quaegebeur, N.; Micheau, P.; Masson, P.; Belanger, H. Structural health monitoring of bonded composite joints using piezoceramics. In Proceedings of the Smart Materials, Structures & NDT in Aerospace Conference, Montreal, QC, Canada, 2–4 November 2011. [Google Scholar]
- Rafik, H.; Boudjema, B.; Ali, B.B.; Choaib, A. Damage evaluation in composite laminate by the use of ultrasonic lamb wave. In Proceedings of the la 3 Conférence Internationale sur le Soudage, le CND et l’Industrie des Matériaux et Alliages (IC-WNDT-MI’12), CSC, Oran, Algeria, 26–28 November 2012. [Google Scholar]
- Toyama, N.; Takatsubo, J. Lamb wave method for quick inspection of impact-induced delamination in composite laminates. Compos. Sci. Technol. 2004, 64, 1293–1300. [Google Scholar] [CrossRef]
- Ribichini, R.; Cegla, F.; Nagy, P.B.; Cawley, P. Study and comparison of different EMAT configurations for SH wave inspection. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2011, 58, 2571–2581. [Google Scholar] [CrossRef] [PubMed]
- Su, Z.; Yang, C.; Pan, N.; Ye, L.; Zhou, L.M. Assessment of delamination in composite beams using shear horizontal (SH) wave mode. Compos. Sci. Technol. 2007, 67, 244–251. [Google Scholar] [CrossRef]
- Chen, M.; Huan, Q.; Su, Z.; Li, F. A tunable bidirectional SH wave transducer based on antiparallel thickness-shear (d15) piezoelectric strips. Ultrasonics 2019, 98, 35–50. [Google Scholar] [CrossRef] [PubMed]
- Kamal, A.; Giurgiutiu, V. Shear horizontal wave excitation and reception with shear horizontal piezoelectric wafer active sensor (SH-PWAS). Smart Mater. Struct. 2014, 23, 085019. [Google Scholar] [CrossRef] [Green Version]
- Castaings, M.; Hosten, B. Lamb and SH waves generated and detected by air-coupled ultrasonic transducers in composite material plates. NDT E Int. 2001, 34, 249–258. [Google Scholar] [CrossRef]
- Sun, Z.; Rocha, B.; Wu, K.T.; Mrad, N. A methodological review of piezoelectric based acoustic wave generation and detection techniques for structural health monitoring. Int. J. Aerosp. Eng. 2013, 2013, 928627. [Google Scholar] [CrossRef]
- Sun, X.C.; Hallett, S.R. Barely visible impact damage in scaled composite laminates: Experiments and numerical simulations. Int. J. Impact Eng. 2017, 109, 178–195. [Google Scholar] [CrossRef] [Green Version]
- Barazanchy, D.; Roth, W.; Giurgiutiu, V. A non-destructive material characterization framework for retrieving a stiffness matrix using bulk waves. Compos. Struct. 2018, 185, 27–37. [Google Scholar] [CrossRef]
- Hudson, T.B.; Hou, T.H.; Grimsley, B.W.; Yuan, F.G. Imaging of local porosity/voids using a fully non-contact air-coupled transducer and laser Doppler vibrometer system. Struct. Health Monit. 2017, 16, 164–173. [Google Scholar] [CrossRef]
- Chakrapani, S.K.; Barnard, D.; Dayal, V. Detection of in-plane fiber waviness in composite laminates using guided Lamb modes. AIP Conf. Proc. 2014, 1581, 1134–1140. [Google Scholar]
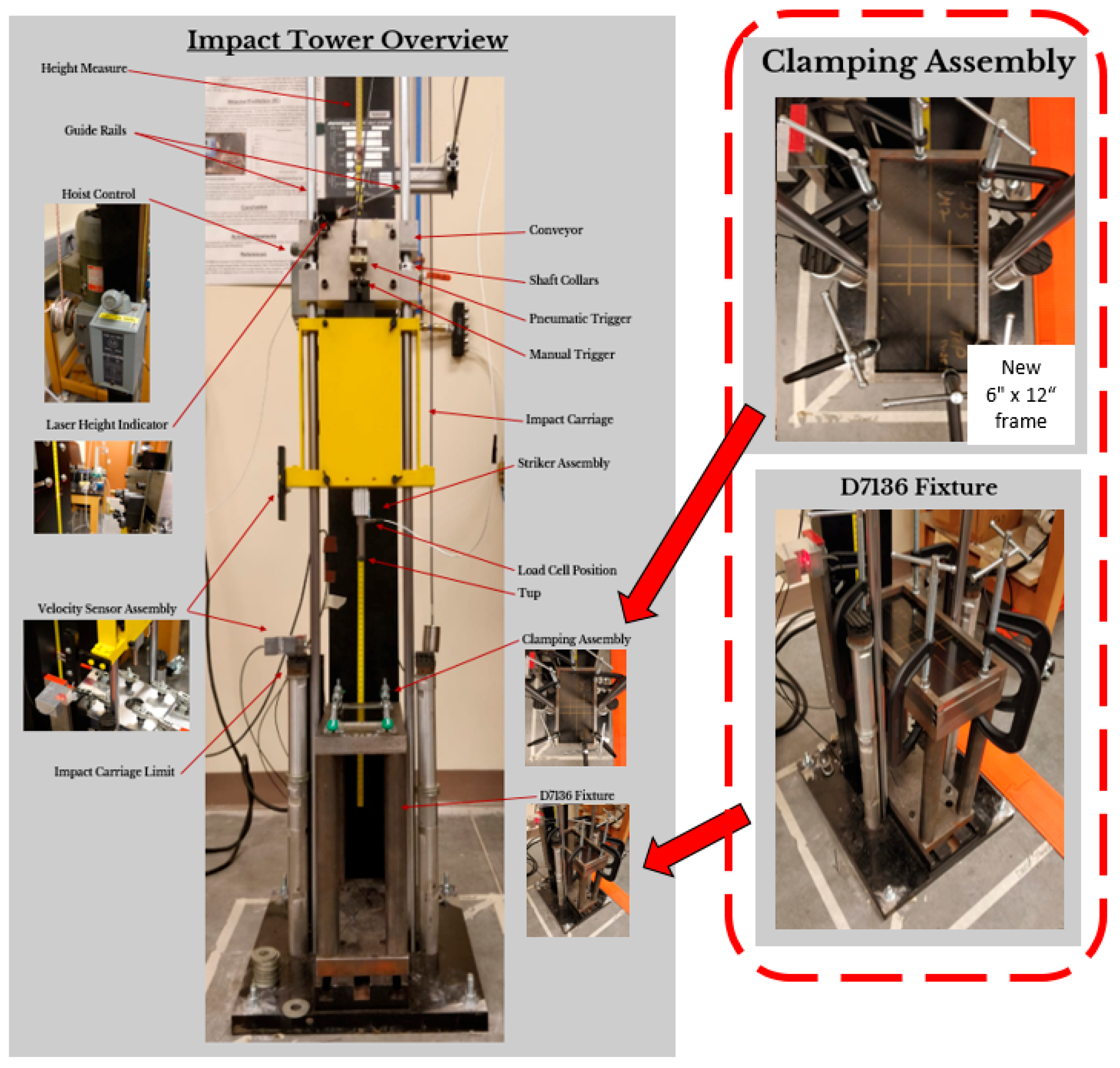





| Mass (kg) | Drop Height (cm) | Velocity (m/s) | Energy (J) | % of Absorbed Energy |
|---|---|---|---|---|
| 3.06 | 55.61 | 3.3 | 16.69 | 65 |
| E11 | E22 | E33 | ν12 | ν13 | ν23 | G12 | G13 | G23 | ρ |
|---|---|---|---|---|---|---|---|---|---|
| 153 GPa | 8.7 GPa | 8.7 GPa | 0.37 | 0.37 | 0.5 | 6.9 GPa | 6.9 GPa | 2.9 GPa | 1625 kg/m3 |
| Mass (kg) | Drop Height (cm) | Velocity (m/s) | Energy (J) | % of Absorbed Energy |
|---|---|---|---|---|
| 3.06 | 58.54 | 3.07 | 17.56 | 63 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mei, H.; James, R.; Haider, M.F.; Giurgiutiu, V. Multimode Guided Wave Detection for Various Composite Damage Types. Appl. Sci. 2020, 10, 484. https://doi.org/10.3390/app10020484
Mei H, James R, Haider MF, Giurgiutiu V. Multimode Guided Wave Detection for Various Composite Damage Types. Applied Sciences. 2020; 10(2):484. https://doi.org/10.3390/app10020484
Chicago/Turabian StyleMei, Hanfei, Robin James, Mohammad Faisal Haider, and Victor Giurgiutiu. 2020. "Multimode Guided Wave Detection for Various Composite Damage Types" Applied Sciences 10, no. 2: 484. https://doi.org/10.3390/app10020484
APA StyleMei, H., James, R., Haider, M. F., & Giurgiutiu, V. (2020). Multimode Guided Wave Detection for Various Composite Damage Types. Applied Sciences, 10(2), 484. https://doi.org/10.3390/app10020484








