Analytical Determination of Geometric Parameters of the Rotary Kiln by Novel Approach of TLS Point Cloud Segmentation
Abstract
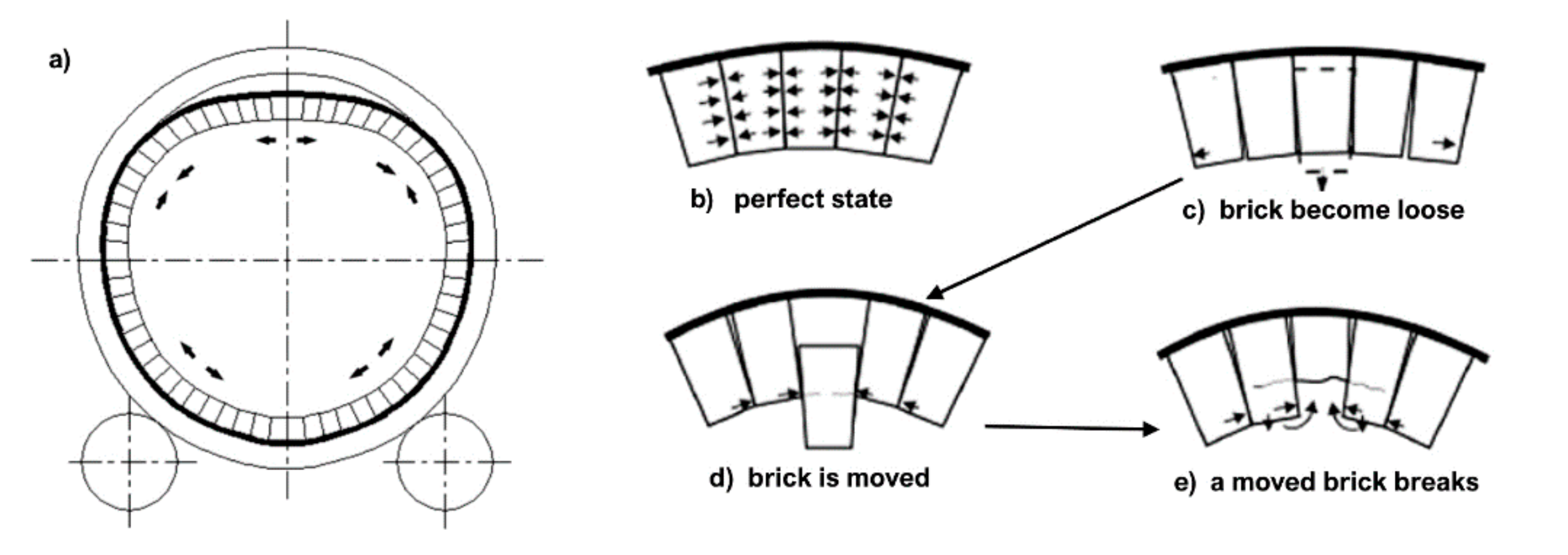
:1. Introduction
2. Materials and Methods
2.1. Study Area—The Object of Research
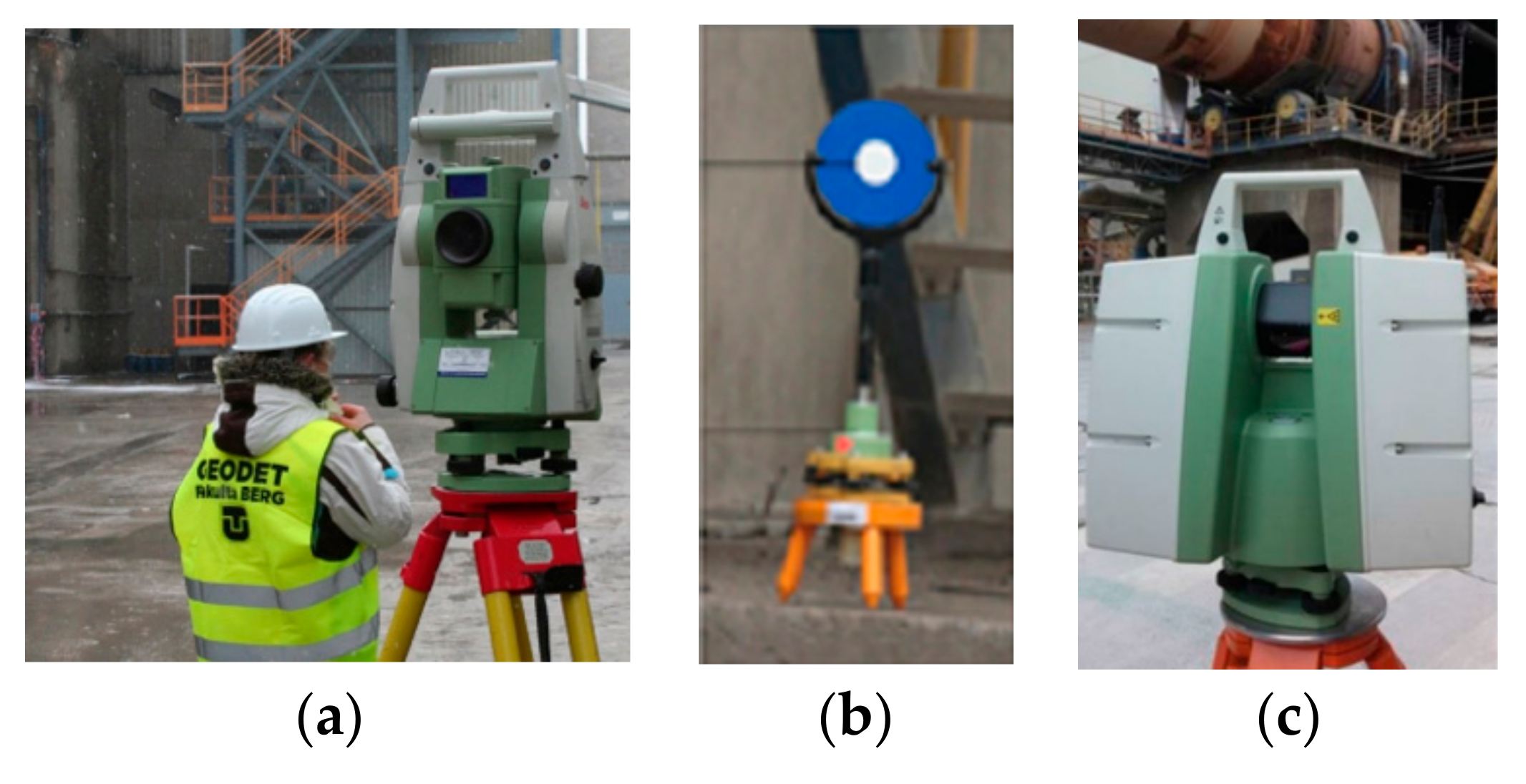
2.2. Surveying Equipment and Data Acquisition
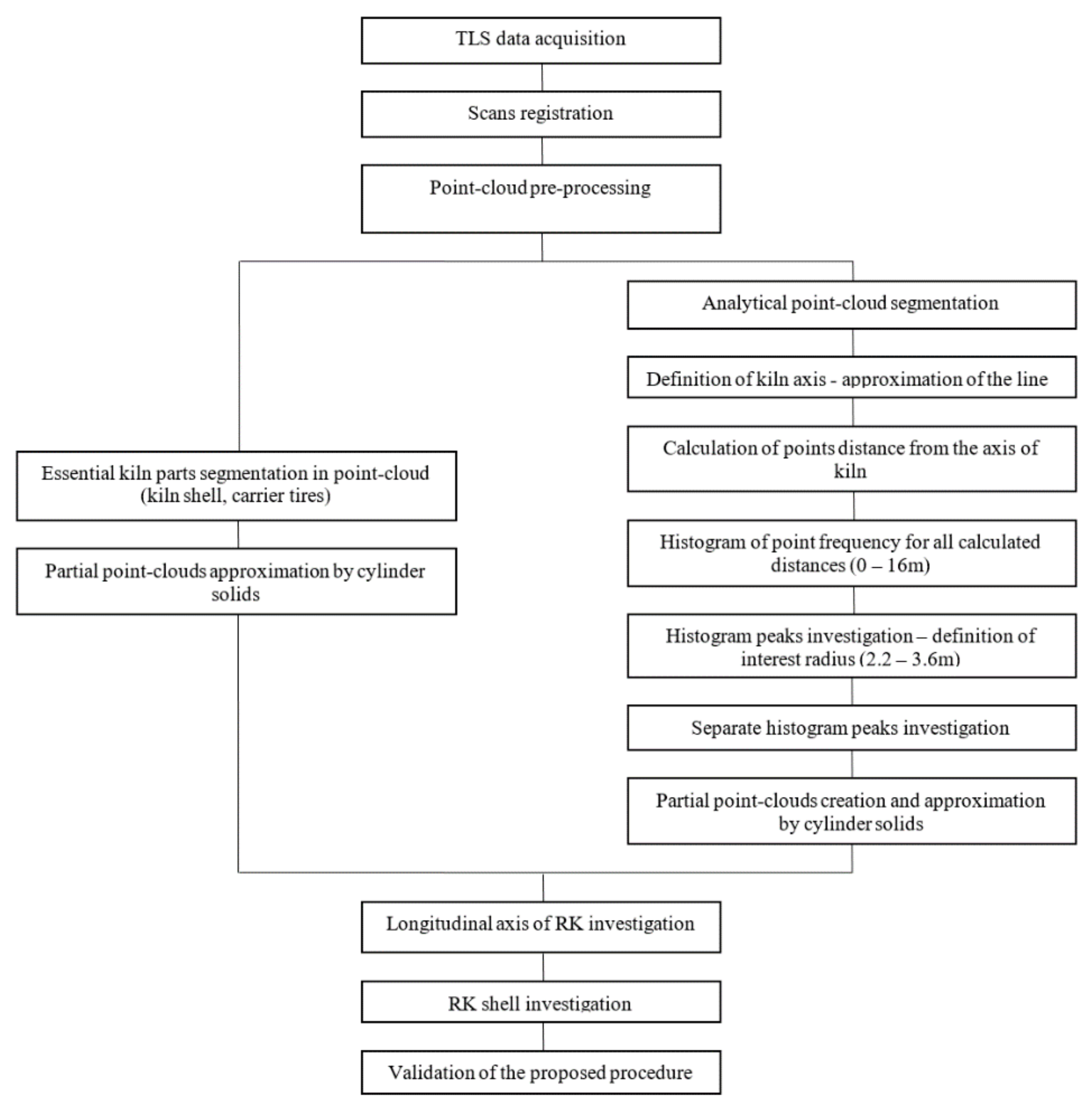
3. Data Processing
3.1. Preliminary Longitudinal Axis Investigation
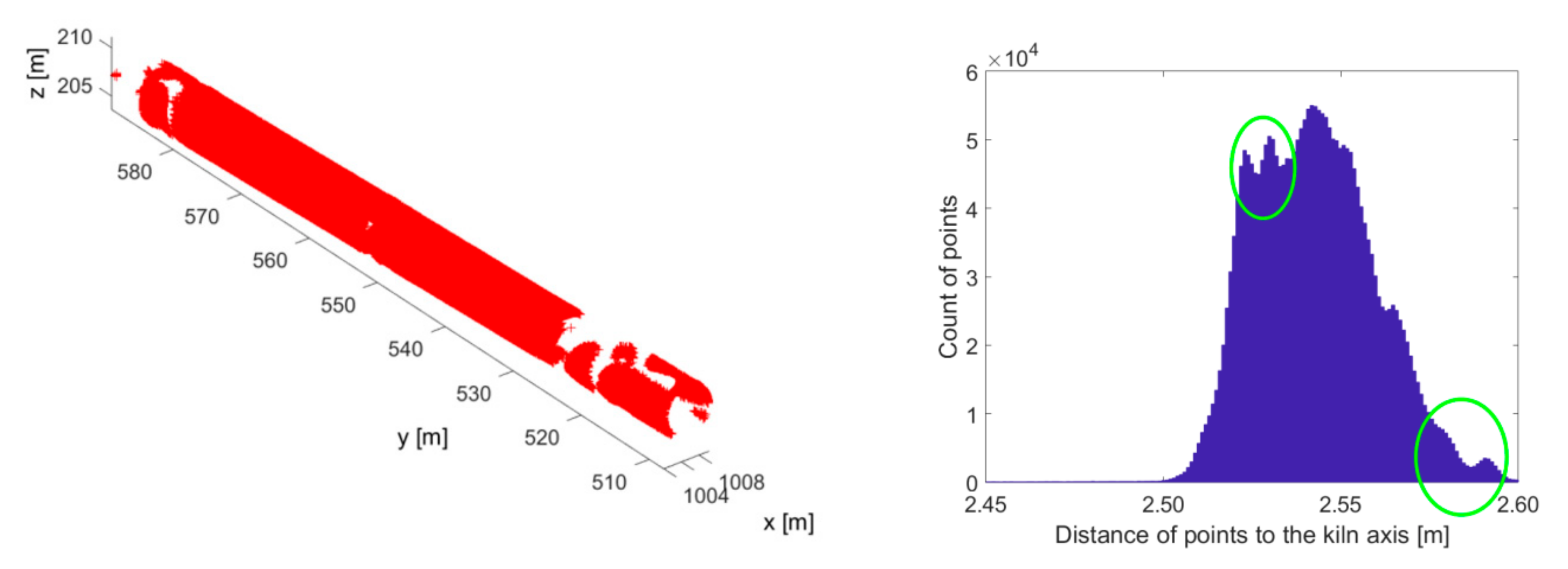
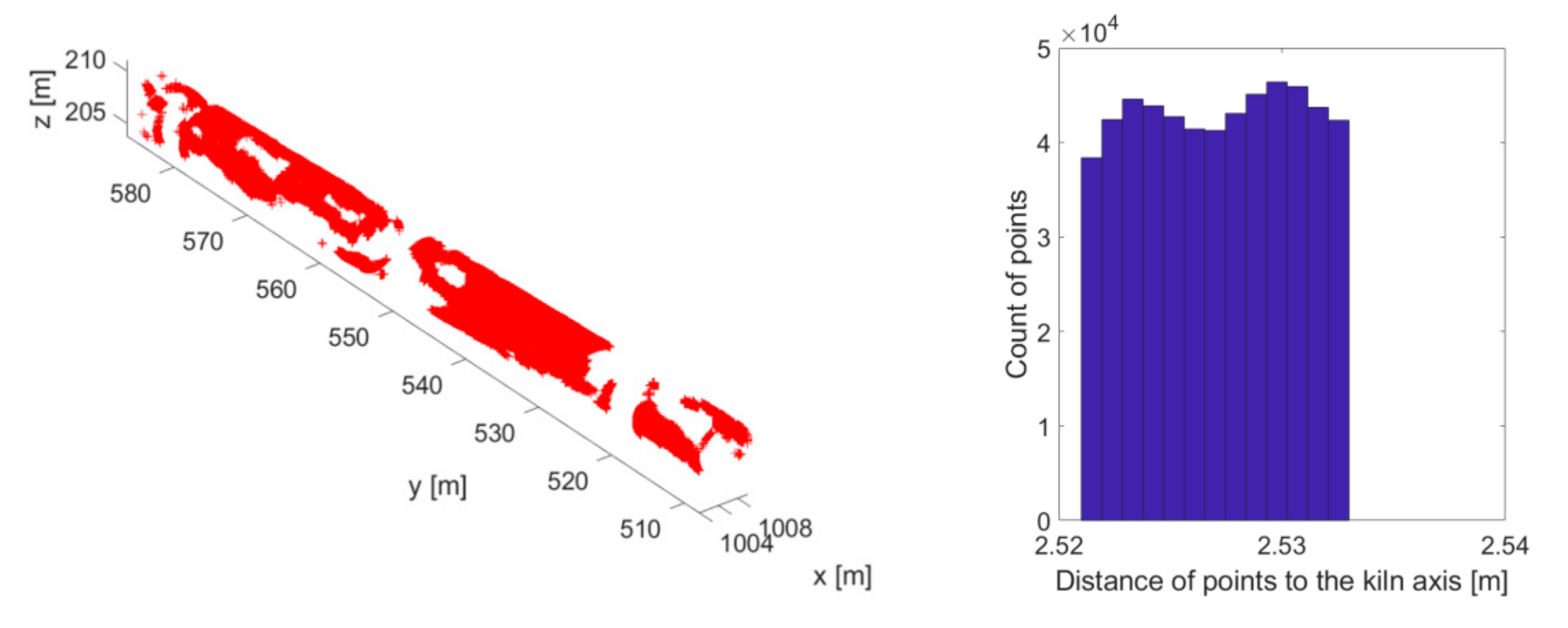
3.2. Analytical Point Cloud Segmentation
4. Results
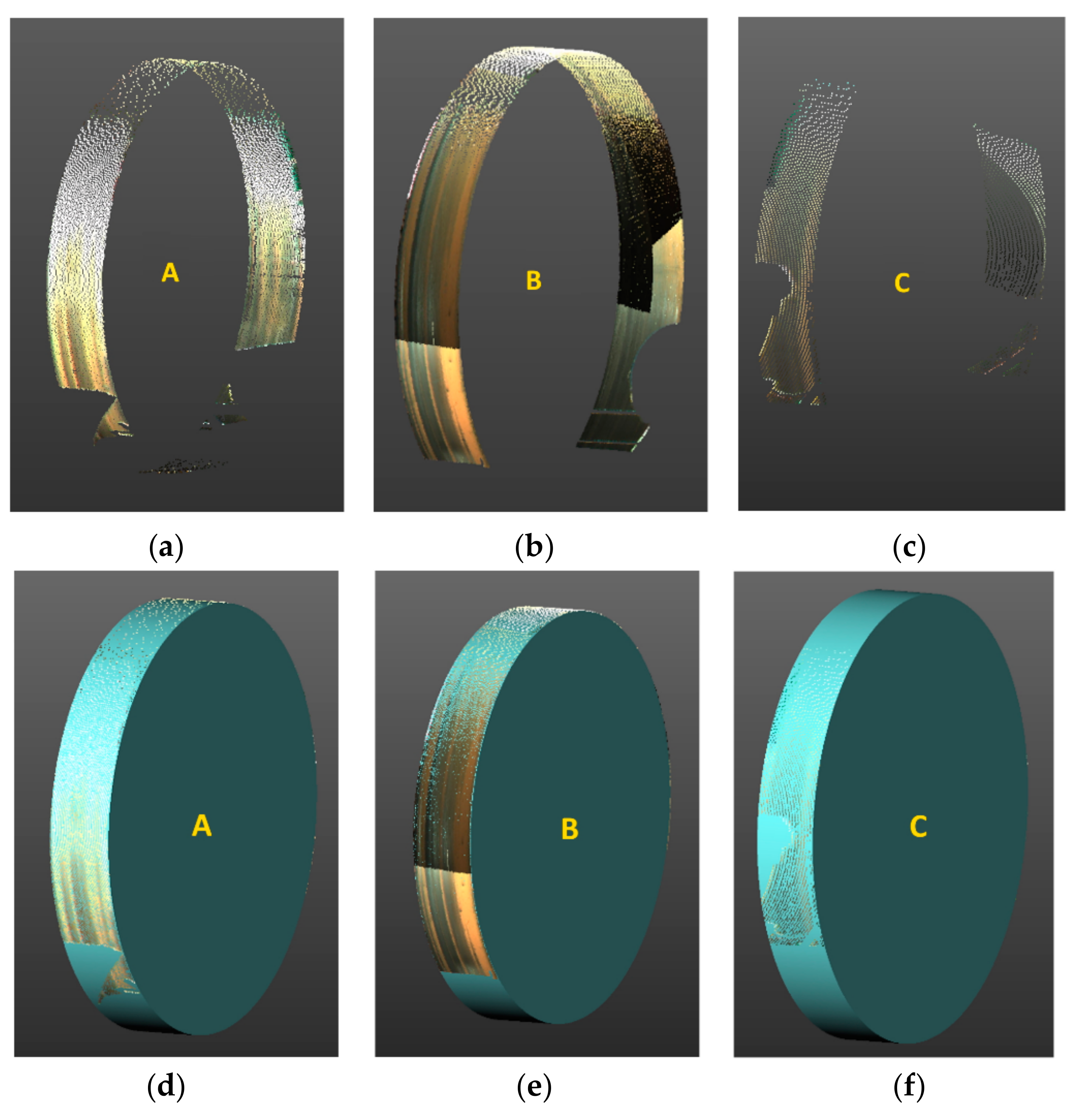
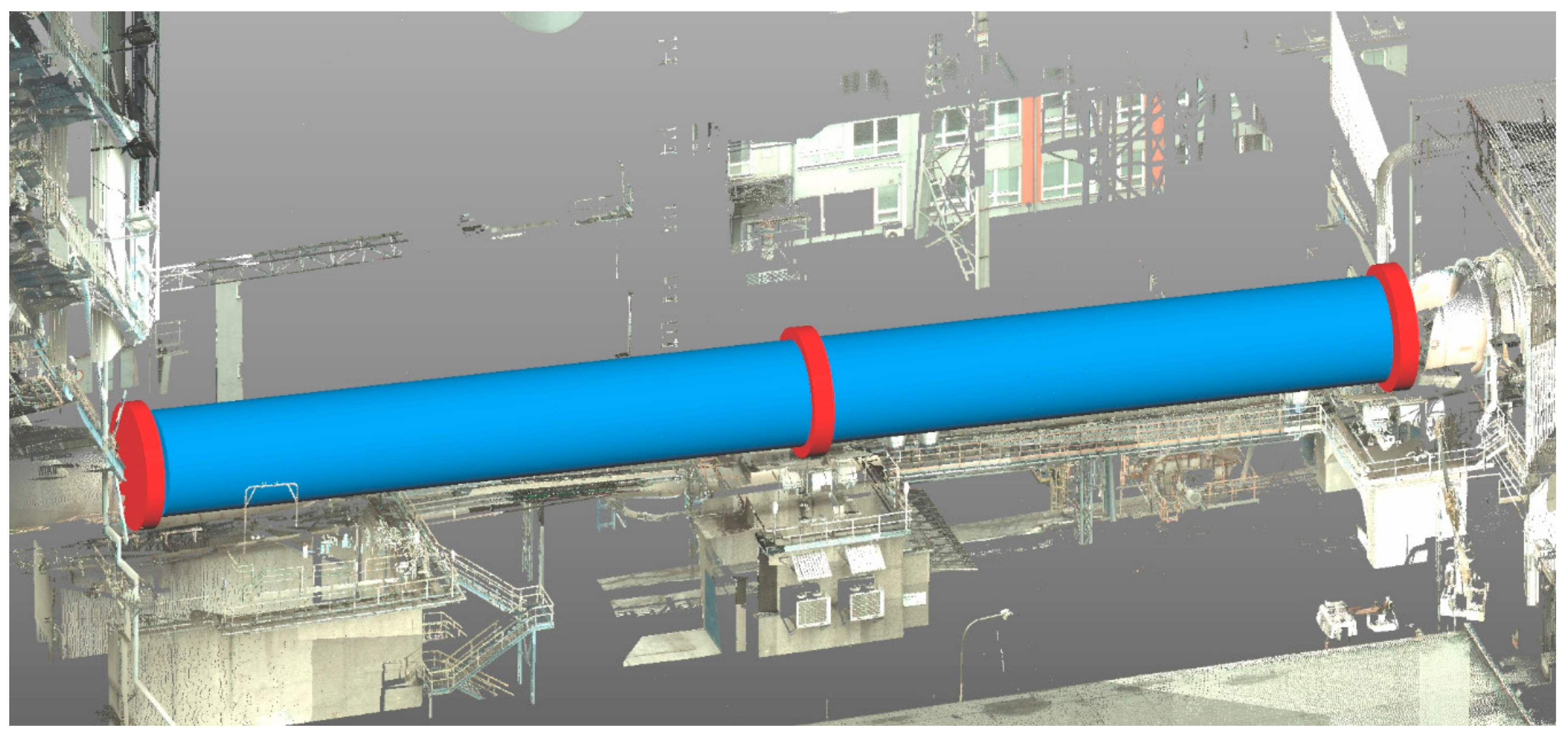
4.1. Segmentation of the Point Cloud on Carrier Tires
4.2. Effect of Cloud Point Density on Center Coordinates and Diameter of the Tires
4.3. Segmentation of the Point Cloud on the Shell of Rotary Kiln
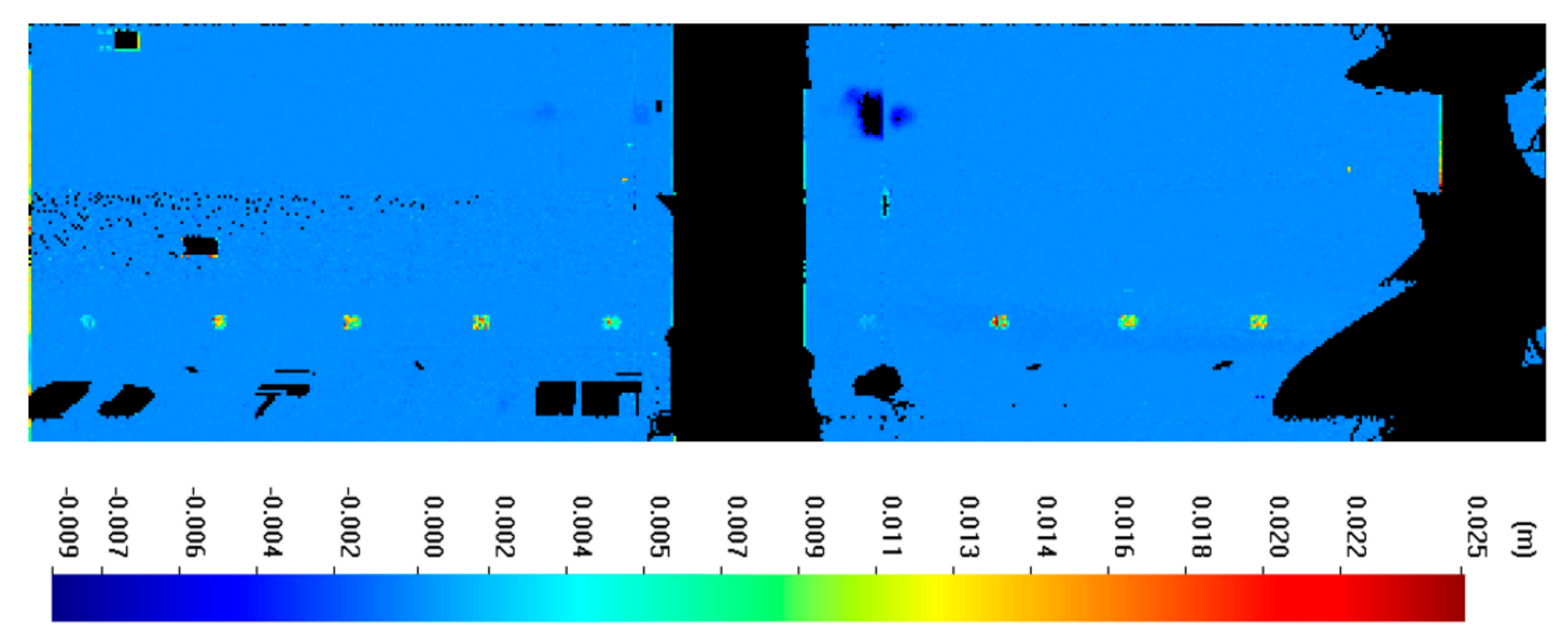
4.4. Rotary Kiln Shell Deformation Analysis
4.5. Analysis of the Coaxiality in the Cross-Sections and Ovality Ratio of the Rotary Kiln Shell
5. Discussion
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Świtalski, M. The measurement of shell’s elastic ovality as essential element of diagnostic of rotary drum’s technical state. Diagnostyka 2010, 53, 37–47. [Google Scholar]
- Krystowczyk, Z. Geometry measurements of kiln shell in dynamic conditions. Cem. Build. Mater. Rev. 2004, 16, 34–37. [Google Scholar]
- Xiao, Y.; Li, X.; Chen, X. General solution to kiln support reactions and multi-objective fuzzy optimization of kiln axis alignment. Struct. Multidiscip. Optim. 2008, 319–327. [Google Scholar] [CrossRef]
- Shi, Y.; Xiao, Y.; Li, X. Monitoring and optimum alignment of kiln axis. Innov. Comput. Inf. Control. 2007, 511. [Google Scholar] [CrossRef]
- Ethrog, U.; Shmutther, B. Determining deformations of a rotary kiln. The international archives of the photogrammetry, remote sensing and spatial information sciences (ISPRS Archives), Vol. XXVI, Part 5, Commission V. In Proceedings of the Symposium Real-Time Photogrammetry—A New Challenge, Ottawa, ON, Canada, 16–19 June 1986. [Google Scholar]
- Shelltester. Available online: https://www.zmp.com.pl/shelltester (accessed on 6 October 2020).
- Li, X.; Jiang, L.; Liu, D. Research on supporting load distribution of large-scale rotary kiln with multi-support and variable-stiffness. Chin. J. Comput. Mech. 2005, 22, 207–213. [Google Scholar]
- Mukupa, W.; Roberts, G.W.; Hancock, C.M.; Al-Manasir, K. A review of the use of terrestrial laser scanning application for change detection and deformation monitoring of structures. Surv. Rev. 2016, 1–18. [Google Scholar] [CrossRef]
- Teskey, W.F.; Radovanovic, R.S. Dynamic parallel roll alignment. J. Surv. Eng. 2002, 128, 136–143. [Google Scholar] [CrossRef]
- Teskey, W.F.; Bayly, D.A.; Martell, H.E. Use of laser targeting in precise machinery surveys. J. Surv. Eng. 1993, 119, 59–70. [Google Scholar] [CrossRef]
- Malowany, K.; Magda, K.; Rutkiewicz, J.; Malesa, M.; Kantor, J.; Michoński, J.; Kujawińska, M. Measurements of geometry of a boiler drum by time-of-flight laser scanning. Measurement 2015, 72, 88–95. [Google Scholar] [CrossRef]
- Mogilny, S.; Sholomitskii, A. Precision analysis of geometric parameters for rotating machines during cold alignment. Procedia Eng. 2017, 206, 1709–1715. [Google Scholar] [CrossRef]
- Bartoš, K.; Pukanská, K.; Repáň, P.; Kseňak, Ľ.; Sabová, J. Modelling the surface of racing vessel’s hull by laser scanning and digital photogrammetry. Remote. Sens. 2019, 11, 1526. [Google Scholar] [CrossRef] [Green Version]
- Stroner, M.; Kremen, T.; Braun, J.; Urban, R.; Blistan, P.; Kovanič, Ľ. Comparison of 2.5D volume calculation methods and software solutions using point clouds scanned before and after mining. Acta Montan. Slovaca 2020, 24, 296–306. [Google Scholar]
- Blišťan, P.; Jacko, S.; Kovanič, Ľ.; Kondela, J.; Pukanská, K.; Bartoš, K. TLS and SfM approach for bulk density determination of excavated heterogeneous raw materials. Minerals 2020, 10, 174. [Google Scholar] [CrossRef] [Green Version]
- Nuttens, T.; De Wulf, A.; Deruyter, G.; Stal, C. Deformation monitoring with terrestrial laser scanning: Measurement and processing optimization through experience. Int. Multidiscip. Sci. GeoConf.-SGEM 2012, 707–714. [Google Scholar] [CrossRef]
- Nuttens, T.; Stal, C.; De Backer, H.; DeRuyter, G.; Schotte, K.; Van Bogaert, P.; De Wulf, A.A.; De Wulf, A. Laser scanning for precise ovalization measurements: Standard deviations and smoothing levels. J. Surv. Eng. 2016, 142, 05016001. [Google Scholar] [CrossRef]
- Nuttens, T.; Stal, C.; De Backer, H.; Schotte, K.; Van Bogaert, P.; De Wulf, A. Methodology for the ovalization monitoring of newly built circular train tunnels based on laser scanning: Liefkenshoek Rail Link (Belgium). Autom. Constr. 2014, 43, 1–9. [Google Scholar] [CrossRef]
- Wang, W.; Zhao, W.; Huang, L.; Vimarlund, V.; Wang, Z. Applications of terrestrial laser scanning for tunnels: A review. J. Traffic Transp. Eng. (English Ed.) 2014, 1, 325–337. [Google Scholar] [CrossRef] [Green Version]
- Xue, B.; Yang, X.; Zhu, J. Architectural stability analysis of the rotary-laser scanning technique. Opt. Lasers Eng. 2016, 78, 26–34. [Google Scholar] [CrossRef]
- Turkan, Y.; Laflamme, S.; Tan, L. Terrestrial laser scanning-based bridge structural condition assessment. Tech Transf. Summ. 2016, 109. [Google Scholar]
- Riveiro, B.; González-Jorge, H.; Varela, M.; Jauregui, D. Validation of terrestrial laser scanning and photogrammetry techniques for the measurement of vertical underclearance and beam geometry in structural inspection of bridges. Measurement 2013, 46, 784–794. [Google Scholar] [CrossRef]
- Kim, M.-K.; Sohn, H.; Chang, C.-C. Localization and quantification of concrete spalling defects using terrestrial laser scanning. J. Comput. Civ. Eng. 2015, 29, 04014086. [Google Scholar] [CrossRef] [Green Version]
- Cao, Z.; Chen, D.; Shi, Y.; Zhang, W.; Jin, F.; Yun, T.; Xu, S.; Kang, Z.; Zhang, L. A flexible architecture for extracting metro tunnel cross sections from terrestrial laser scanning point clouds. Remote. Sens. 2019, 11, 297. [Google Scholar] [CrossRef] [Green Version]
- Pukanská, K.; Bartoš, K.; Bella, P.; Gašinec, J.; Blistan, P.; Kovanič, Ľ. Surveying and high-resolution topography of the ochtiná aragonite cave based on tls and digital photogrammetry. Appl. Sci. 2020, 10, 4633. [Google Scholar] [CrossRef]
- Zhang, Z.; Yin, T.; Huang, X.; Zhang, F.; Zhu, Y.; Liu, W. Identification and visualization of the full-ring deformation characteristics of a large stormwater sewage and storage tunnel using terrestrial laser scanning technology. Energies 2019, 12, 1304. [Google Scholar] [CrossRef] [Green Version]
- Jo, Y.H.; Hong, S. Three-Dimensional digital documentation of cultural heritage site based on the convergence of terrestrial laser scanning and unmanned aerial vehicle photogrammetry. ISPRS Int. J. Geo-Inf. 2019, 8, 53. [Google Scholar] [CrossRef] [Green Version]
- Slob, S.; Hack, R. 3D Terrestrial Laser Scanning as a New Field Measurement and Monitoring Technique. In Engineering Geology for Infrastructure Planning in Europe; Springer: Berlin/Heidelberg, Germany, 2004; Volume 104, pp. 179–189. ISBN 3-540-21075-X. [Google Scholar] [CrossRef]
- Pu, S.; Vosselman, G. Knowledge based reconstruction of building models from terrestrial laser scanning data. ISPRS J. Photogramm. Remote Sens. 2009, 64, 575–584. [Google Scholar] [CrossRef]
- Petrov, V.V.; Tyurin, S.V. Technique for control of rotary kilns geometric characteristics. Cellul. Pap. Cardboard 2005, 7, 66–70. [Google Scholar]
- Geoservex. Available online: http://www.geoservex.com.pl/ (accessed on 6 October 2020).
- TomTom-ToolsGmbH. Available online: http://tomtomtools.com/wp-content/uploads/2015/08/Measurement-Tools-For-The-Cement-Industry-03.pdf (accessed on 6 October 2020).
- Industrial Kiln & Dryer Group. Available online: http://www.industrialkiln.com (accessed on 6 October 2020).
- Ozek Makina. Available online: http://www.rotarykiln.net (accessed on 6 October 2020).
- NAK Kiln Services. Available online: https://nak-kiln.com (accessed on 6 October 2020).
- Site of Open Corporation Industrial Geodesy. Available online: http://promgeo.com/services/kiln (accessed on 6 October 2020).
- Rachakonda, P.; Muralikrishnan, B.; Sawyer, D. Metrological evaluation of contrast target center algorithm for terrestrial laser scanners. Measurement 2019, 134, 15–24. [Google Scholar] [CrossRef]
- Fan, L.; Smethurst, J.A.; Atkinson, P.; Powrie, W. Error in target-based georeferencing and registration in terrestrial laser scanning. Comput. Geosci. 2015, 83, 54–64. [Google Scholar] [CrossRef] [Green Version]
- Janßen, J.; Medić, T.; Kuhlmann, H.; Holst, C. Decreasing the uncertainty of the target center estimation at terrestrial laser scanning by choosing the best algorithm and by improving the target design. Remote. Sens. 2019, 11, 845. [Google Scholar] [CrossRef] [Green Version]
- Schmitz, B.; Holst, C.; Medic, T.; Lichti, D.D.; Kuhlmann, H. How to efficiently determine the range precision of 3d terrestrial laser scanners. Sensors 2019, 19, 1466. [Google Scholar] [CrossRef] [Green Version]
- Tan, K.; Zhang, W.; Shen, F.; Cheng, X. Investigation of TLS intensity data and distance measurement errors from target specular reflections. Remote. Sens. 2018, 10, 1077. [Google Scholar] [CrossRef] [Green Version]
- Yang, R.; Meng, X.; Yao, Y.; Chen, B.Y.; You, Y.; Xiang, Z. An analytical approach to evaluate point cloud registration error utilizing targets. ISPRS J. Photogramm. Remote. Sens. 2018, 143, 48–56. [Google Scholar] [CrossRef]
- Maalek, R.; Lichti, D.D.; Ruwanpura, J.Y. Robust Segmentation of Planar and Linear Features of Terrestrial Laser Scanner Point Clouds Acquired from Construction Sites. Sensors 2018, 18, 819. [Google Scholar] [CrossRef]
- Li, Q.; Cheng, X. Comparison of different feature sets for tls point cloud classification. Sensors 2018, 18, 4206. [Google Scholar] [CrossRef] [Green Version]
- Zivec, T.; Anzur, A.; Verbovsek, T. Determination of rock type and moisture content in flysch using TLS intensity in the Elerji quarry (south-west Slovenia). Bull. Eng. Geol. Environ. 2019, 78, 1631–1643. [Google Scholar] [CrossRef]
- Elkhrachy, I. Feature extraction of laser scan data based on geometric properties. J. Indian Soc. Remote. Sens. 2016, 45, 1–10. [Google Scholar] [CrossRef]
- Malambo, L.; Popescu, S.; Horne, D.; Pugh, N.; Rooney, W. Automated detection and measurement of individual sorghum panicles using density-based clustering of terrestrial lidar data. ISPRS J. Photogramm. Remote. Sens. 2019, 149, 1–13. [Google Scholar] [CrossRef]
- Yi, X.; Zhang, R.; Li, H.; Chen, Y. An MFF-SLIC hybrid superpixel segmentation method with multi-source rs data for rock surface extraction. Appl. Sci. 2019, 9, 906. [Google Scholar] [CrossRef] [Green Version]
- Valero, E.; Bosche, F.; Forster, A. Automatic segmentation of 3D point-clouds of rubble masonry walls, and its application to building surveying, repair and maintenance. Autom. Constr. 2018, 96, 29–39. [Google Scholar] [CrossRef]
- Koma, Z.; Rutzinger, M.; Bremer, M. Automated segmentation of leaves from deciduous trees in terrestrial laser scanning point clouds. IEEE Geosci. Remote. Sens. Lett. 2018, 15, 1456–1460. [Google Scholar] [CrossRef]
- Che, E.; Olsen, M.J. Multi-scan segmentation of terrestrial laser scanning data based on normal variation analysis. ISPRS J. Photogramm. Remote. Sens. 2018, 143, 233–248. [Google Scholar] [CrossRef]
- Xi, Z.; Hopkinson, C.; Chasmer, L. Filtering stems and branches from terrestrial laser scanning point clouds using deep 3-d fully convolutional networks. Remote. Sens. 2018, 10, 1215. [Google Scholar] [CrossRef] [Green Version]
- Kovanič, L.; Blišťan, P.; Zelizňaková, V.; Palková, J.; Baulovič, J. Deformation investigation of the shell of rotary kiln using terrestrial laser scanning (TLS) measurement. Metalurgija 2019, 58, 311–314. [Google Scholar]
- Totálne Stanice Leica TPS1200+. Technické Parametre a Porovnanie. Available online: https://www.geotech.sk/downloads/Totalne-stanice/TPS1200+-technicke-parametre.pdf (accessed on 6 October 2020).
- Leica ScanStation C10. The All-in-One Laser Scanner for Any Application. Available online: https://w3.leica-geosystems.com/downloads123/hds/hds/ScanStation%20C10/brochures-datasheet/Leica_ScanStation_C10_DS_en.pdf (accessed on 6 October 2020).
- Standard, B. Execution of steel structures and aluminium structures. Tech. Require. Alum. Struct. 2008, 1090. [Google Scholar]
- STN EN. Eurocode 3: Design of Steel Structures. 1993. Available online: https://www.phd.eng.br/wp-content/uploads/2015/12/en.1993.1.1.2005.pdf (accessed on 23 October 2020).






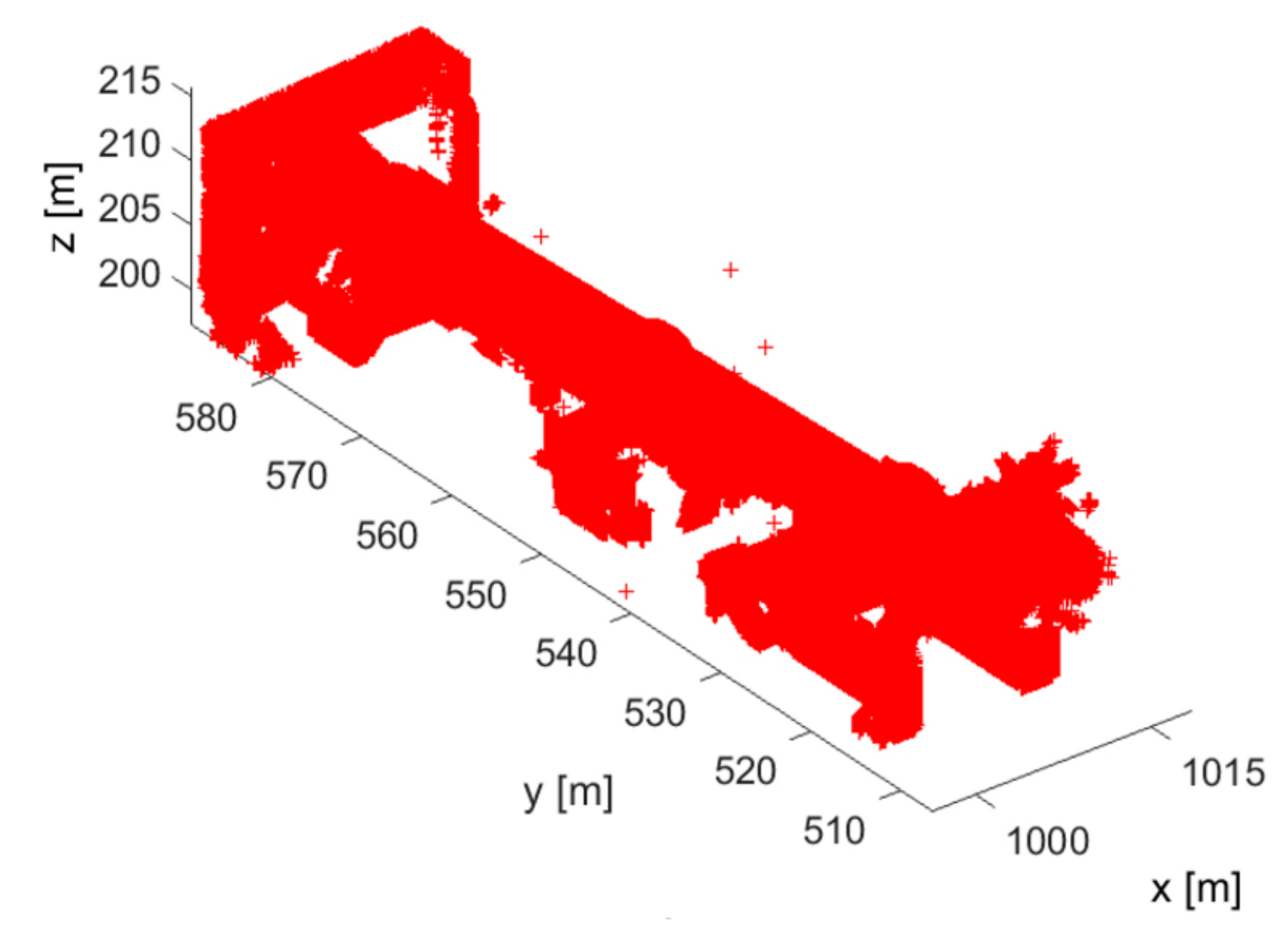
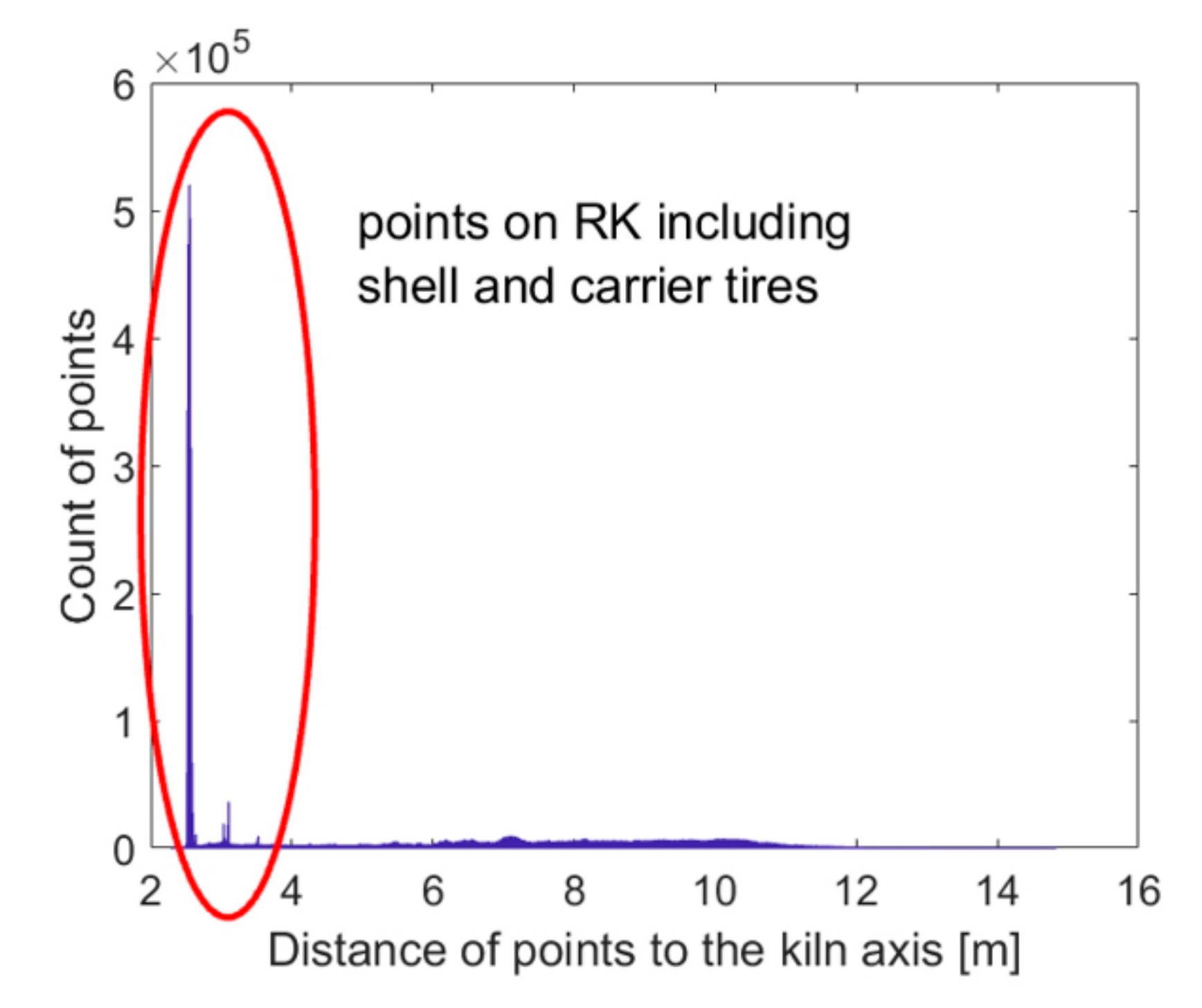
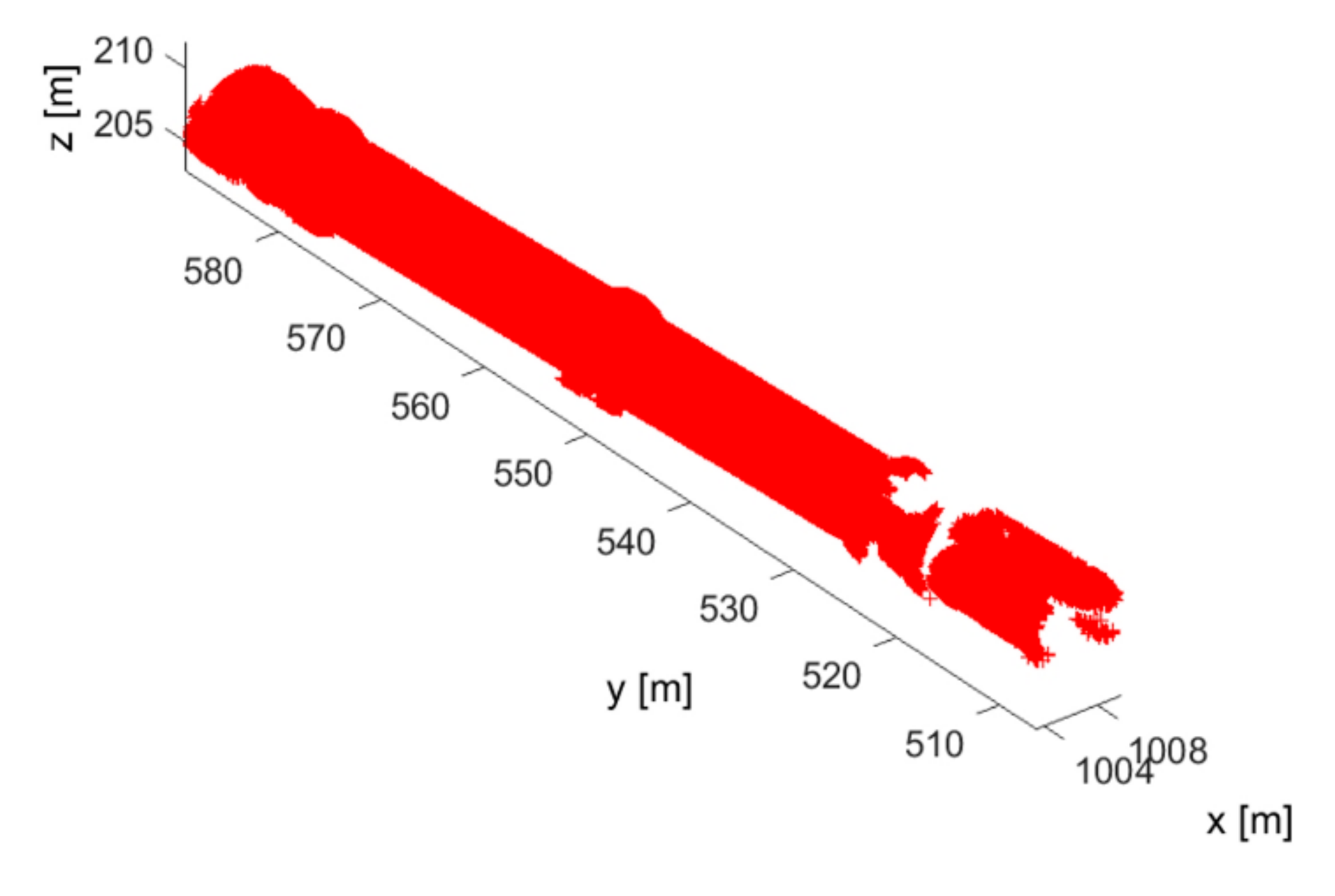

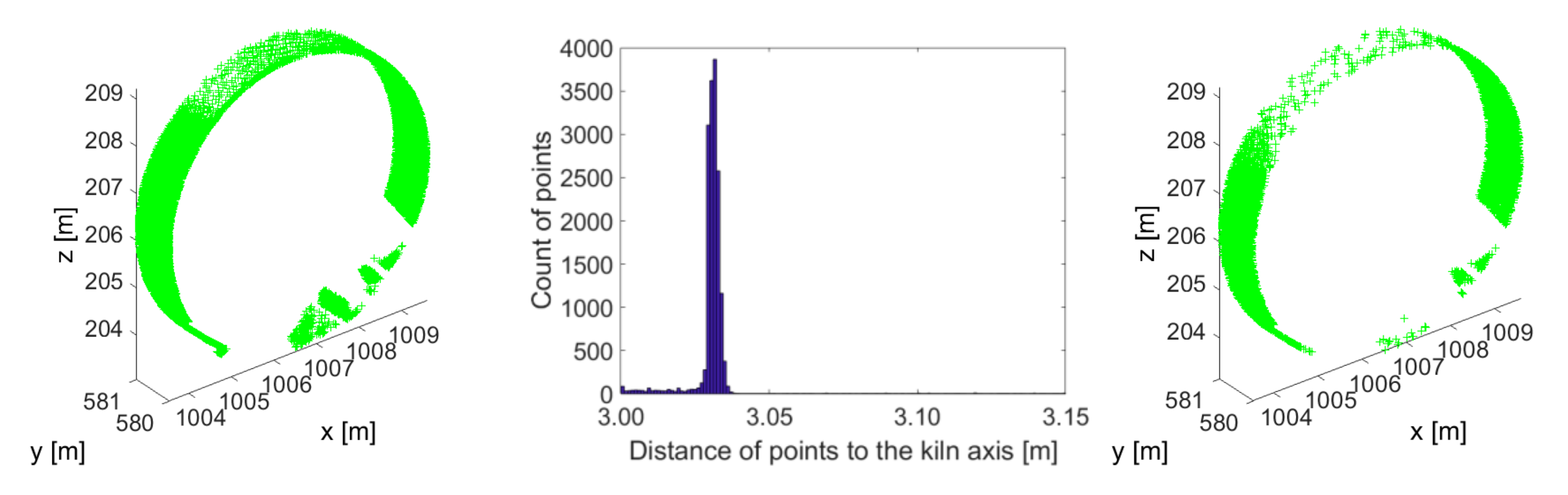
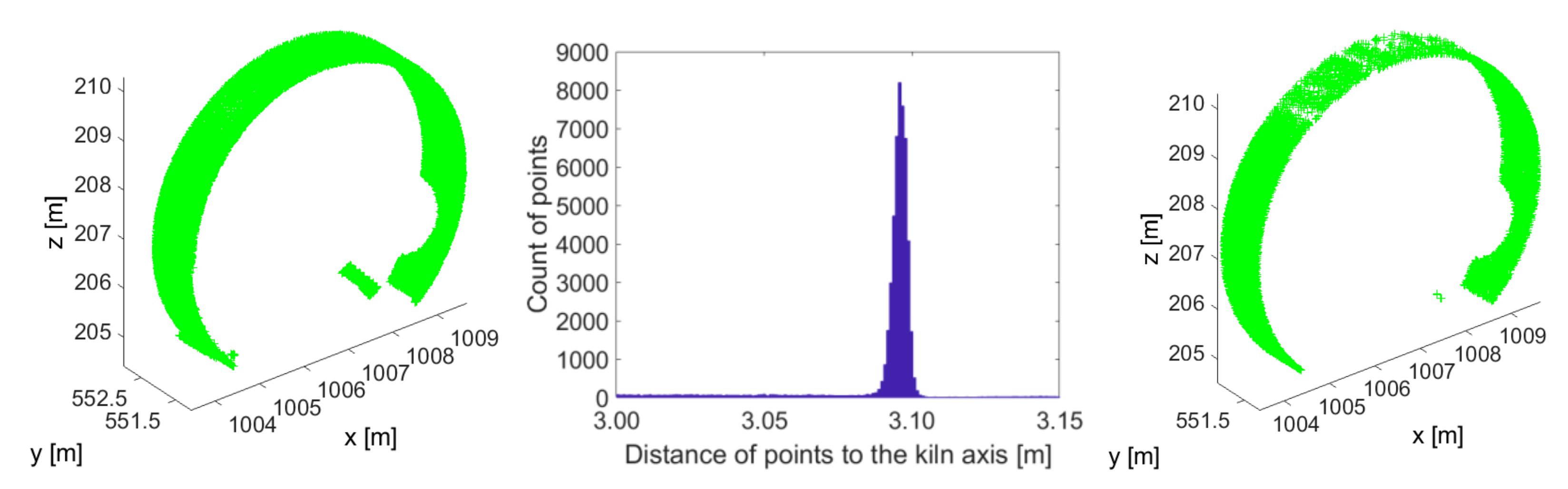
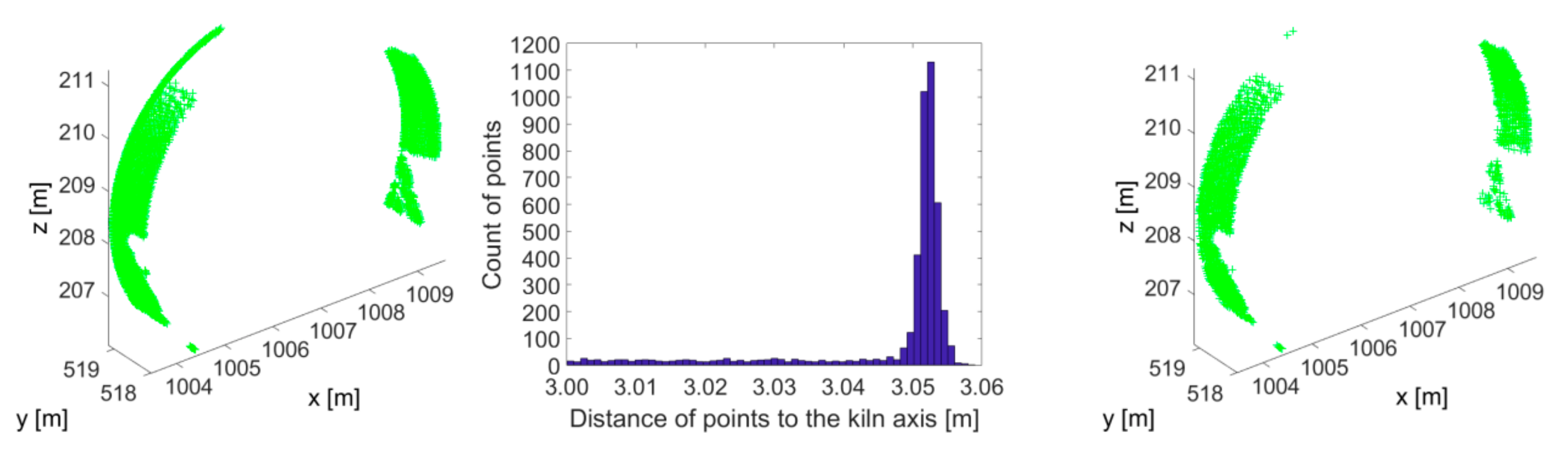
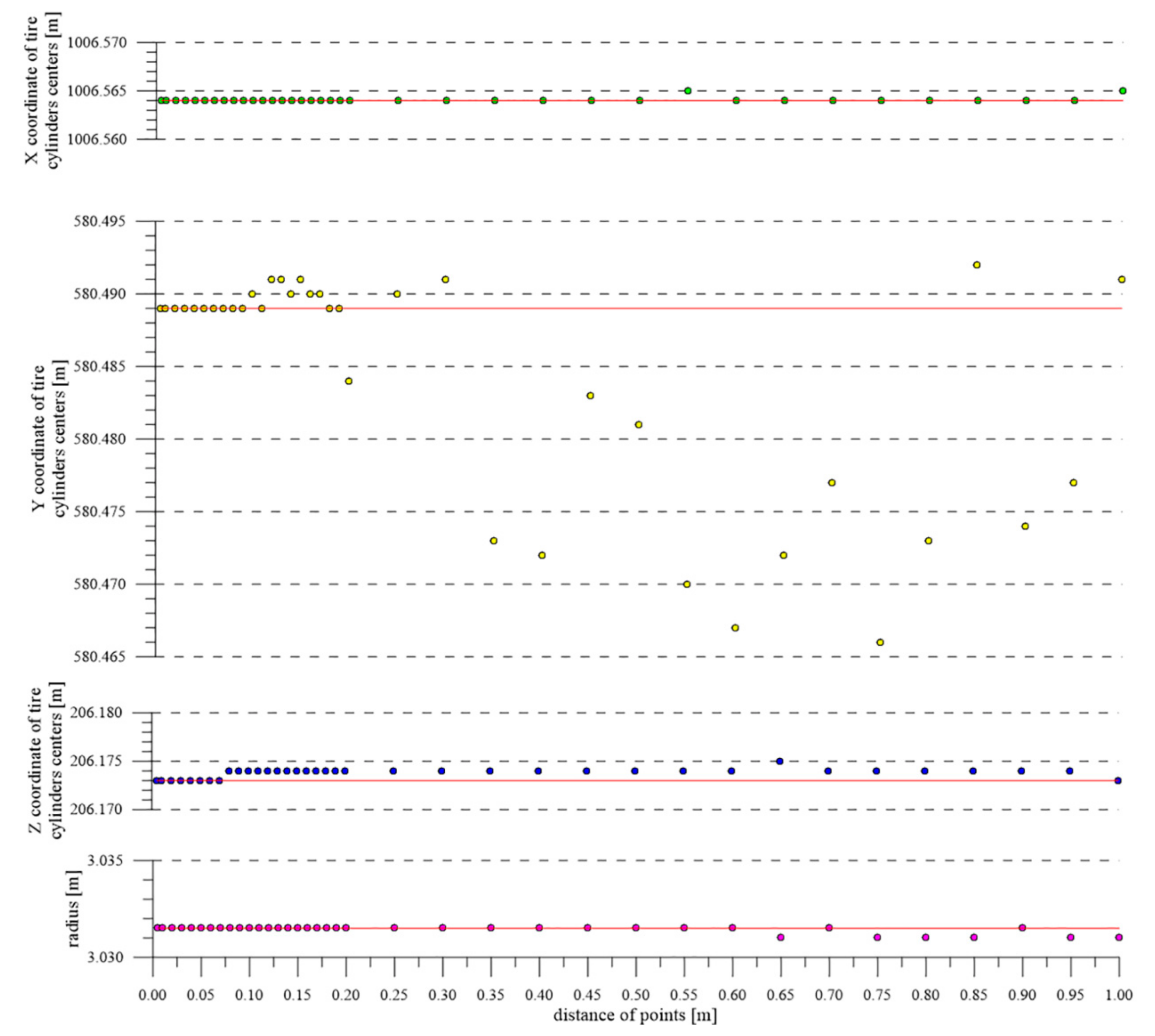

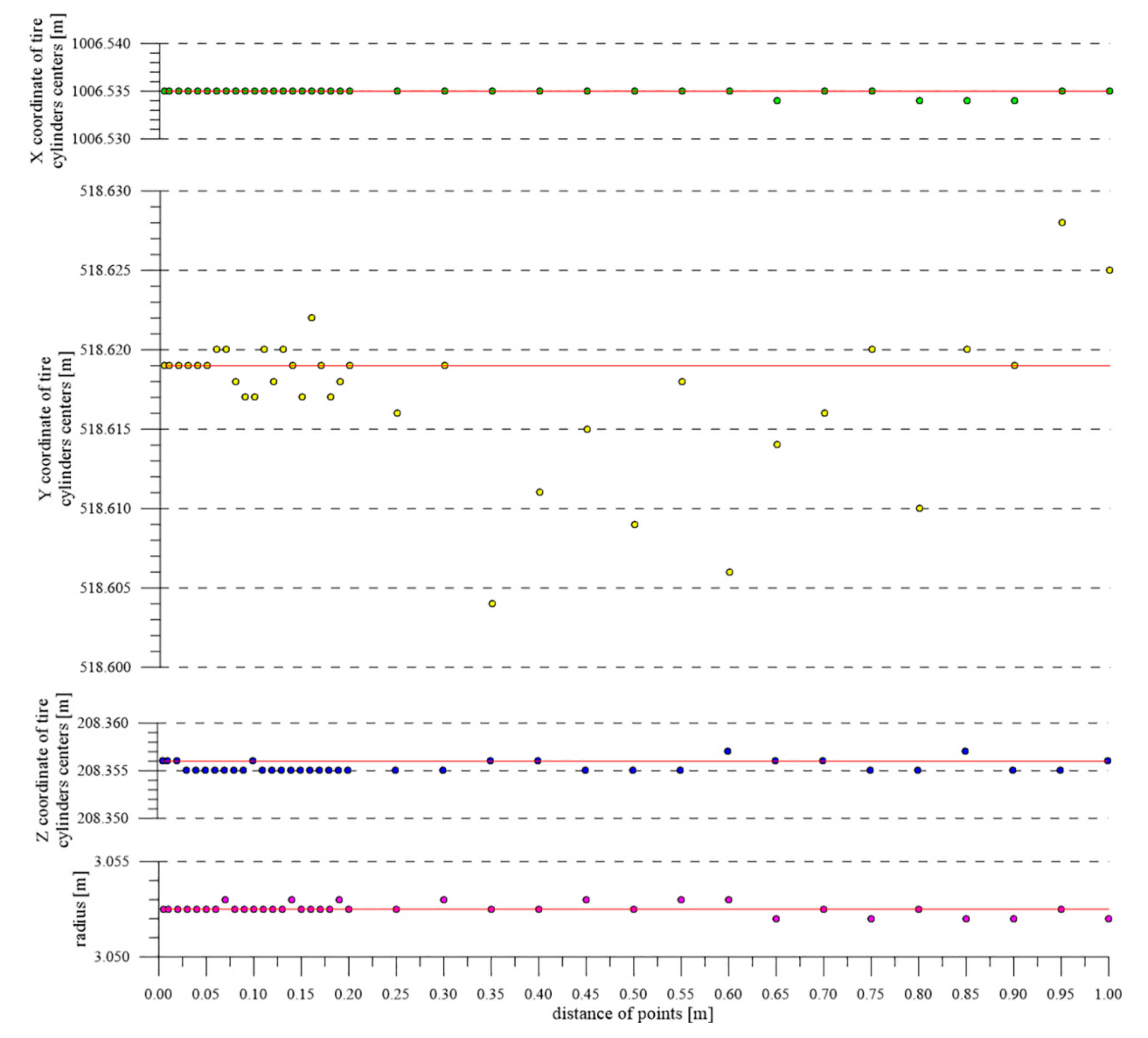



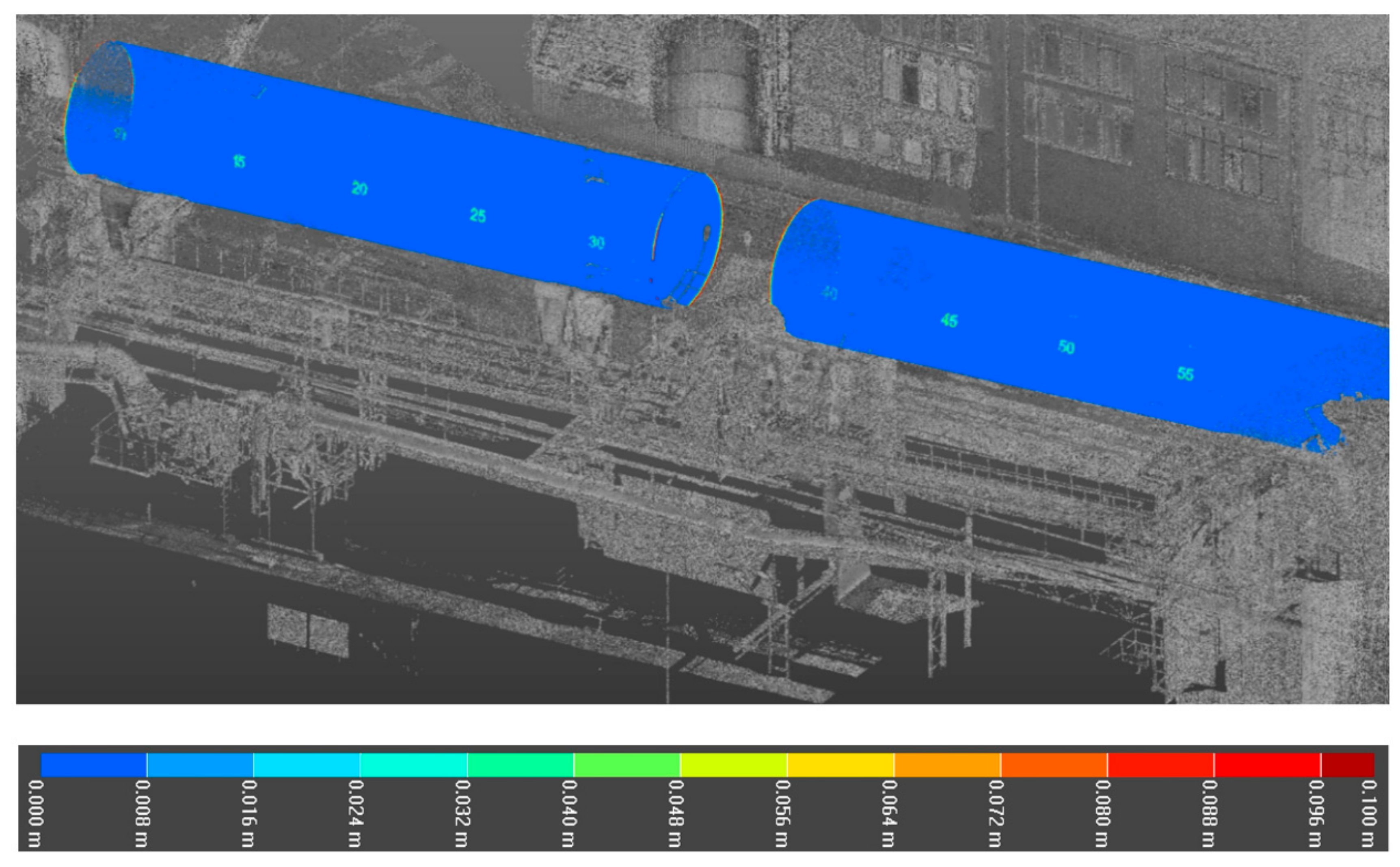
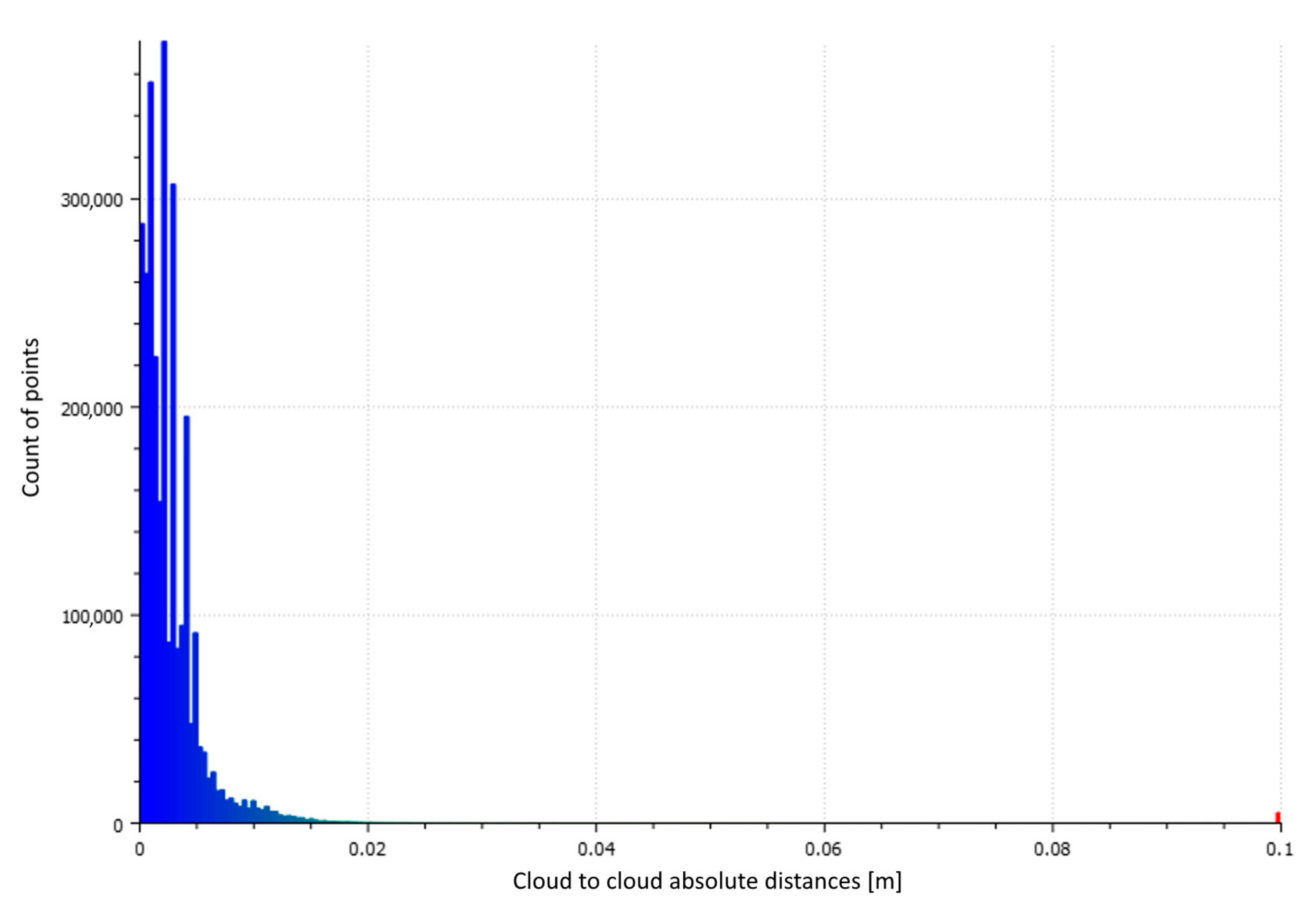


| Tire | Parameter | Manual Point Cloud Segmentation | Analytical Solution—Preselection | Analytical Solution—Fine Segmentation |
|---|---|---|---|---|
| A | Number of points | 16,084 | 16,544 | 7485 |
| Radius of RK | 3.0315 m | 3.0320 m | 3.0315 m | |
| Cylinder fit RMSD | 2 mm | 10 mm | 1 mm | |
| Direction of axis | −0.999; −0.001; 0.035 | −1.000; −0.003; 0.026 | −0.999; −0.001; 0.035 | |
| Percentage of remaining points | 0.54% | 0.54% | 0.25% | |
| B | Number of points | 76,542 | 53,447 | 15,781 |
| Radius of RK | 3.0965 m | 3.0910 m | 3.0965 m | |
| Cylinder fit RMSD | 2 mm | 19 mm | 1 mm | |
| Direction of axis | −0.999; −0.001; 0.036 | −0.999; −0.006; 0.042 | −0.999; −0.001; 0.035 | |
| Percentage of remaining points | 0.55% | 1.78% | 0.53% | |
| C | Number of points | 3573 | 4500 | 2152 |
| Radius of RK | 3.0525 | 3.0475 m | 3.0525 m | |
| Cylinder fit RMSD | 1 mm | 12 mm | 1 mm | |
| Direction of axis | −0.999; −0.000; 0.036 | −0.998; −0.005; 0.059 | −0.999; −0.000; 0.035 | |
| Percentage of remaining points | 0.12% | 0.15% | 0.07% |
| Tire | Coordinates of Tire Cylinders Centers | Manual Point Cloud Segmentation [m] | Analytical Solution—Preselection [m] | Analytical Solution—Fine Segmentation [m] | Difference Manual vs. Analytical Segmentation [mm] |
|---|---|---|---|---|---|
| A | Y | 580.489 | 580.526 | 580.485 | +4 |
| X | 1006.564 | 1006.565 | 1006.564 | 0 | |
| Z | 206.173 | 206.164 | 206.173 | 0 | |
| B | Y | 551.599 | 552.089 | 551.601 | −2 |
| X | 1006.551 | 1006.553 | 1006.551 | 0 | |
| Z | 207.192 | 207.164 | 207.190 | +2 | |
| C | Y | 518.619 | 518.617 | 518.621 | −3 |
| X | 1006.535 | 1006.534 | 1006.535 | 0 | |
| Z | 208.356 | 208.354 | 208.356 | 0 |
| Point Cloud Resolution [m] | Tire A [Points] | Tire B [Points] | Tire C [Points] |
|---|---|---|---|
| 0.005 | 15,895 | 75,821 | 3573 |
| 0.010 | 15,073 | 47,725 | 3573 |
| 0.020 | 9245 | 17,523 | 3573 |
| 0.030 | 5288 | 9134 | 2350 |
| 0.040 | 3560 | 5689 | 1835 |
| 0.050 | 2642 | 3903 | 1198 |
| 0.060 | 1981 | 2853 | 907 |
| 0.070 | 1579 | 2163 | 725 |
| 0.080 | 1282 | 1713 | 600 |
| 0.090 | 1069 | 1400 | 500 |
| 0.100 | 910 | 1151 | 426 |
| 0.110 | 777 | 958 | 361 |
| 0.120 | 676 | 820 | 314 |
| 0.130 | 589 | 715 | 275 |
| 0.140 | 522 | 630 | 242 |
| 0.150 | 468 | 549 | 216 |
| 0.160 | 420 | 498 | 196 |
| 0.170 | 383 | 442 | 173 |
| 0.180 | 351 | 403 | 157 |
| 0.190 | 307 | 371 | 149 |
| 0.200 | 286 | 334 | 132 |
| 0.250 | 197 | 216 | 92 |
| 0.300 | 147 | 163 | 65 |
| 0.350 | 112 | 119 | 54 |
| 0.400 | 93 | 96 | 42 |
| 0.450 | 76 | 83 | 34 |
| 0.500 | 61 | 67 | 30 |
| 0.550 | 53 | 54 | 25 |
| 0.600 | 47 | 45 | 23 |
| 0.650 | 43 | 41 | 22 |
| 0.700 | 40 | 35 | 21 |
| 0.750 | 38 | 35 | 19 |
| 0.800 | 36 | 34 | 17 |
| 0.850 | 31 | 30 | 14 |
| 0.900 | 28 | 28 | 13 |
| 0.950 | 27 | 25 | 11 |
| 1.000 | 23 | 24 | 11 |
| Parameter | Manual Point Cloud Segmentation | Analytical Solution—Fine Segmentation |
|---|---|---|
| Number of points | 2,963,072 | 2,192,723 |
| The radius of the fitted cylinder | 2.5395 m | 2.5390 m |
| Cylinder fit RMSD | 12 mm | 13 mm |
| Direction of axis | −0.999; −0.001; 0.035 | −0.999; −0.001; 0.035 |
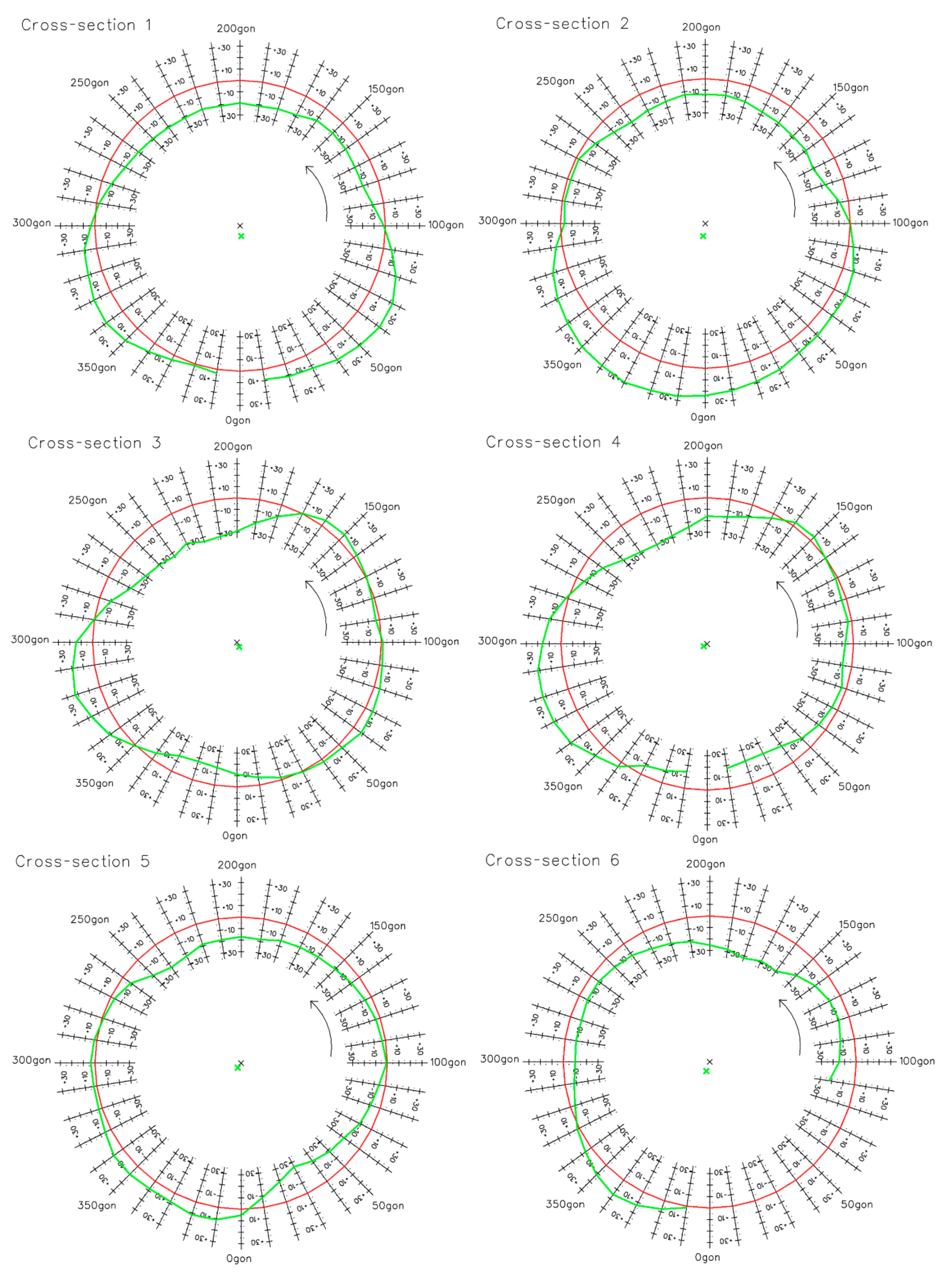
| Cross-Section | rmin [mm] | rmax [mm] | ω0 [%] | ΔX [mm] | ΔZ [mm] | R [mm] |
|---|---|---|---|---|---|---|
| 1 | 2527 | 2558 | 1.22 | 1 | −9 | 9 |
| 2 | 2526 | 2559 | 1.30 | 2 | −11 | 11 |
| 3 | 2515 | 2570 | 2.17 | 2 | −4 | 4 |
| 4 | 2510 | 2560 | 1.97 | −3 | −2 | 4 |
| 5 | 2512 | 2547 | 1.38 | −3 | −4 | 5 |
| 6 | 2515 | 2547 | 1.23 | −3 | −8 | 9 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kovanič, Ľ.; Blistan, P.; Urban, R.; Štroner, M.; Pukanská, K.; Bartoš, K.; Palková, J. Analytical Determination of Geometric Parameters of the Rotary Kiln by Novel Approach of TLS Point Cloud Segmentation. Appl. Sci. 2020, 10, 7652. https://doi.org/10.3390/app10217652
Kovanič Ľ, Blistan P, Urban R, Štroner M, Pukanská K, Bartoš K, Palková J. Analytical Determination of Geometric Parameters of the Rotary Kiln by Novel Approach of TLS Point Cloud Segmentation. Applied Sciences. 2020; 10(21):7652. https://doi.org/10.3390/app10217652
Chicago/Turabian StyleKovanič, Ľudovít, Peter Blistan, Rudolf Urban, Martin Štroner, Katarína Pukanská, Karol Bartoš, and Jana Palková. 2020. "Analytical Determination of Geometric Parameters of the Rotary Kiln by Novel Approach of TLS Point Cloud Segmentation" Applied Sciences 10, no. 21: 7652. https://doi.org/10.3390/app10217652
APA StyleKovanič, Ľ., Blistan, P., Urban, R., Štroner, M., Pukanská, K., Bartoš, K., & Palková, J. (2020). Analytical Determination of Geometric Parameters of the Rotary Kiln by Novel Approach of TLS Point Cloud Segmentation. Applied Sciences, 10(21), 7652. https://doi.org/10.3390/app10217652








