Experimental Assessment of the Effects of Low-Emissivity Paints as Interior Radiation Control Coatings
Abstract
:1. Introduction
- At the material level, to experimentally assess the emissivity properties of different paint mixtures with limited metallised effect and, therefore, higher emissivity by changing the aluminium paint concentration. For this sake, a new procedure while using Heat Flow Meter apparatus was developed.
- At the component/room level, to analyse whether some benefits (increase of wall thermal insulation and improvement of the indoor comfort) are still achievable, even by using paints with a more realistic emissivity and with limited metallised effect. In the present study, paints with different emissivity (from ~0.4 to ~0.9) were applied on one side of the partition wall of a double climatic chamber apparatus and their performance was compared.
2. Materials and Methods
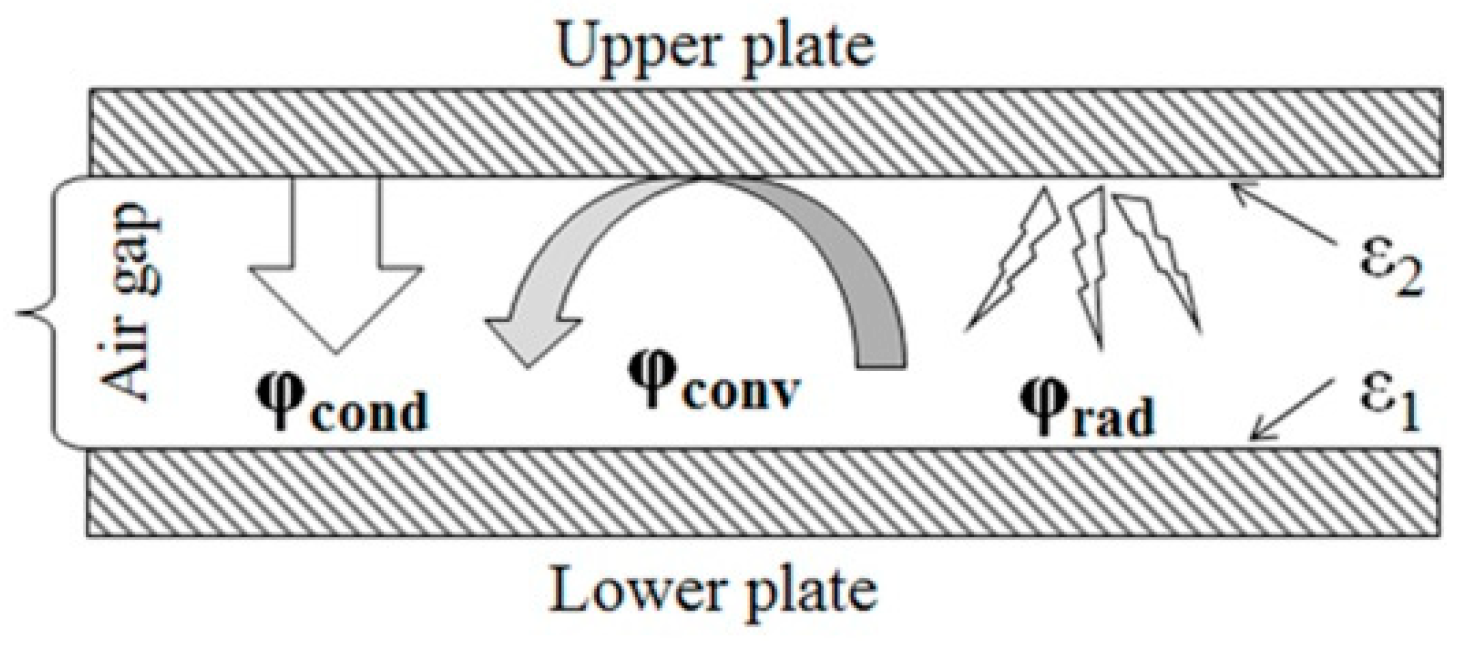
2.1. Analysis at the Material Level
- λ represents the thermal conductivity of still air calculated according to Equation (4) [21].
- φrad (W/m2) is calculated according to Equation (3) [7].
- ΔT is the temperature difference between the upper and the lower plate surfaces (K);
- σ is the Stefan–Boltzmann’s constant = 5.67 × 10−8 W/(m2 K4);
- ε1 and ε2 are the emissivities of the lower and upper surface respectively;
- Tm is the average temperature between the two surfaces.
2.1.1. Application of the HFM Measurement Method
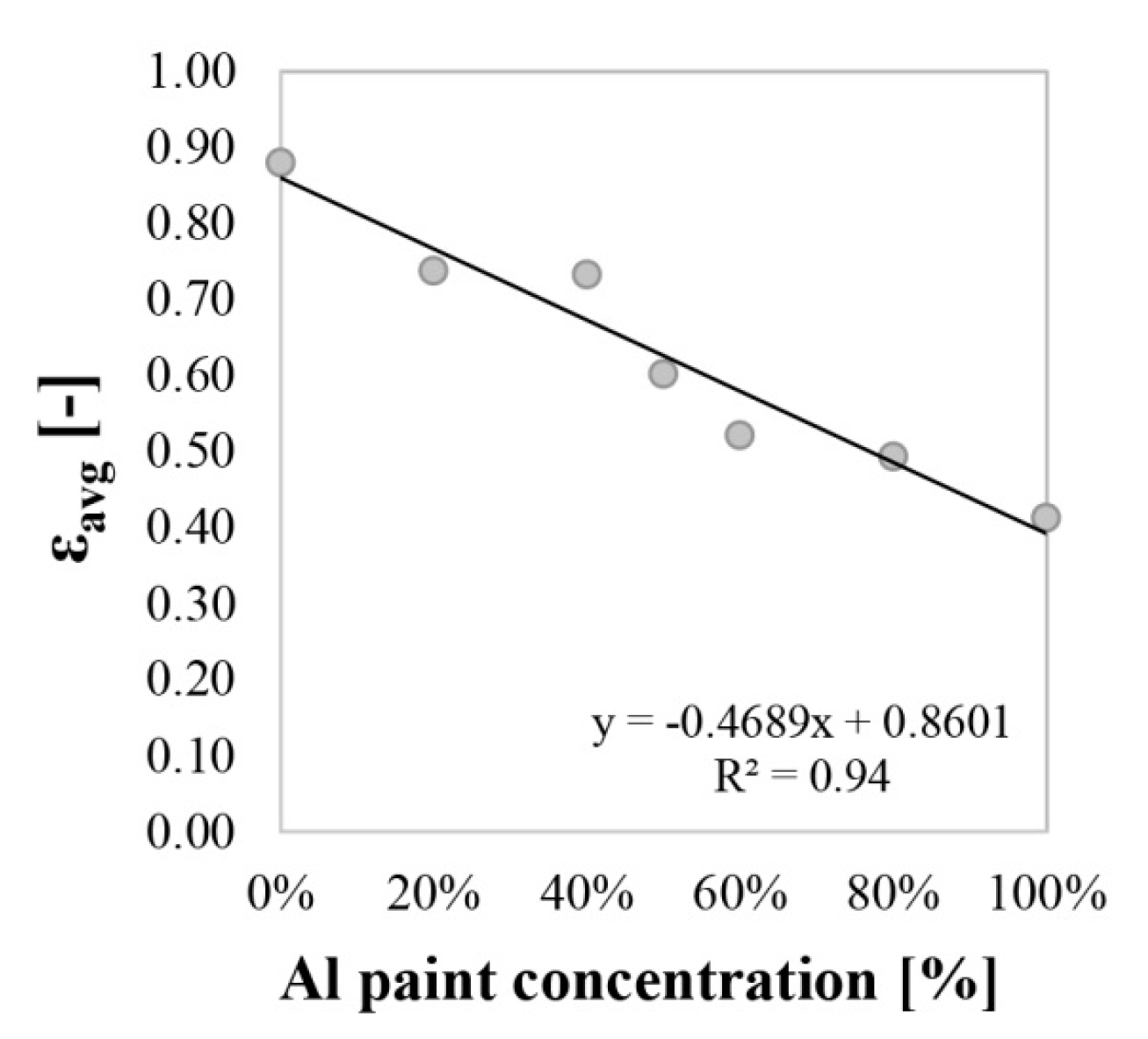
2.1.2. Measurements on Different Low-E Paint
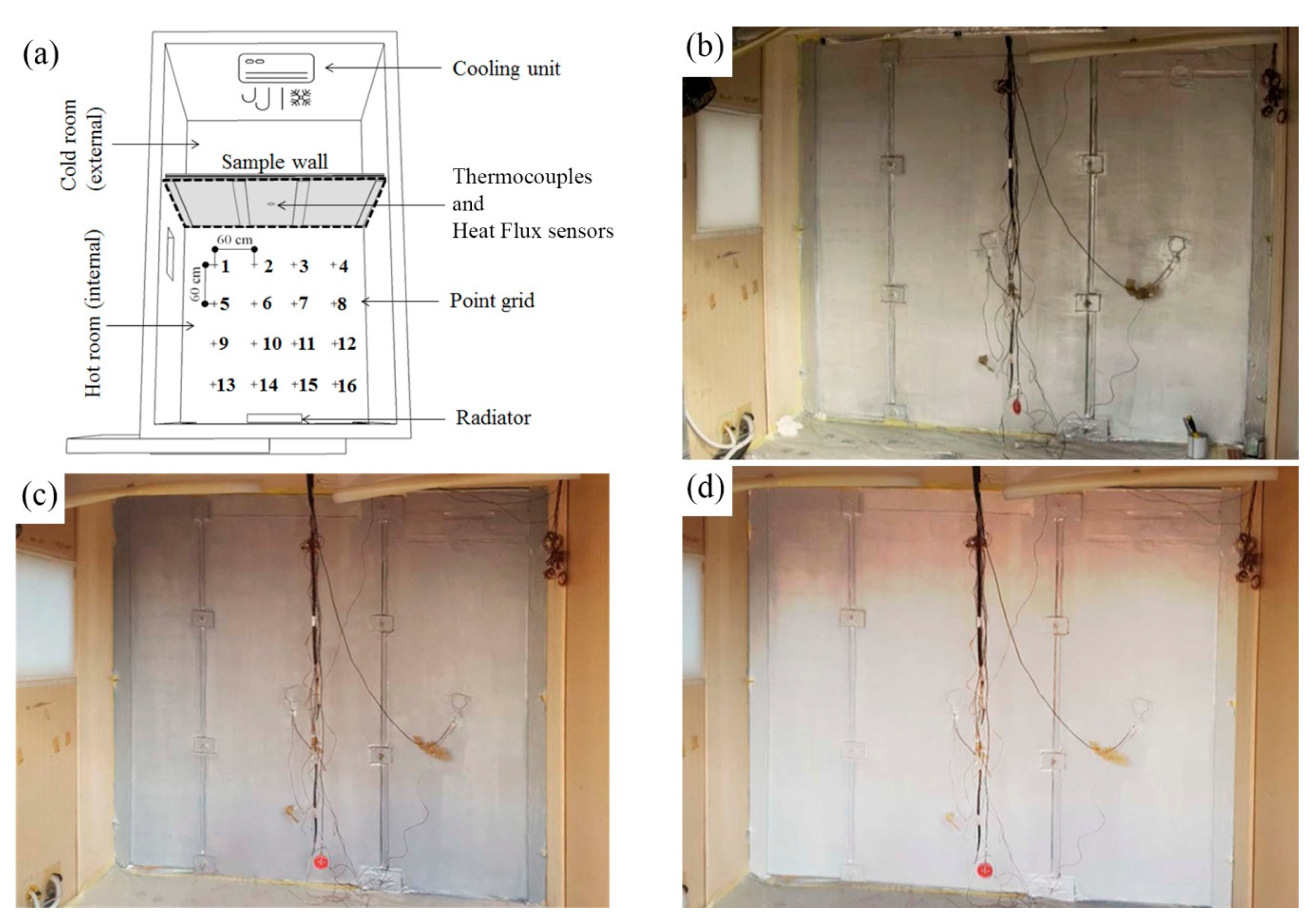
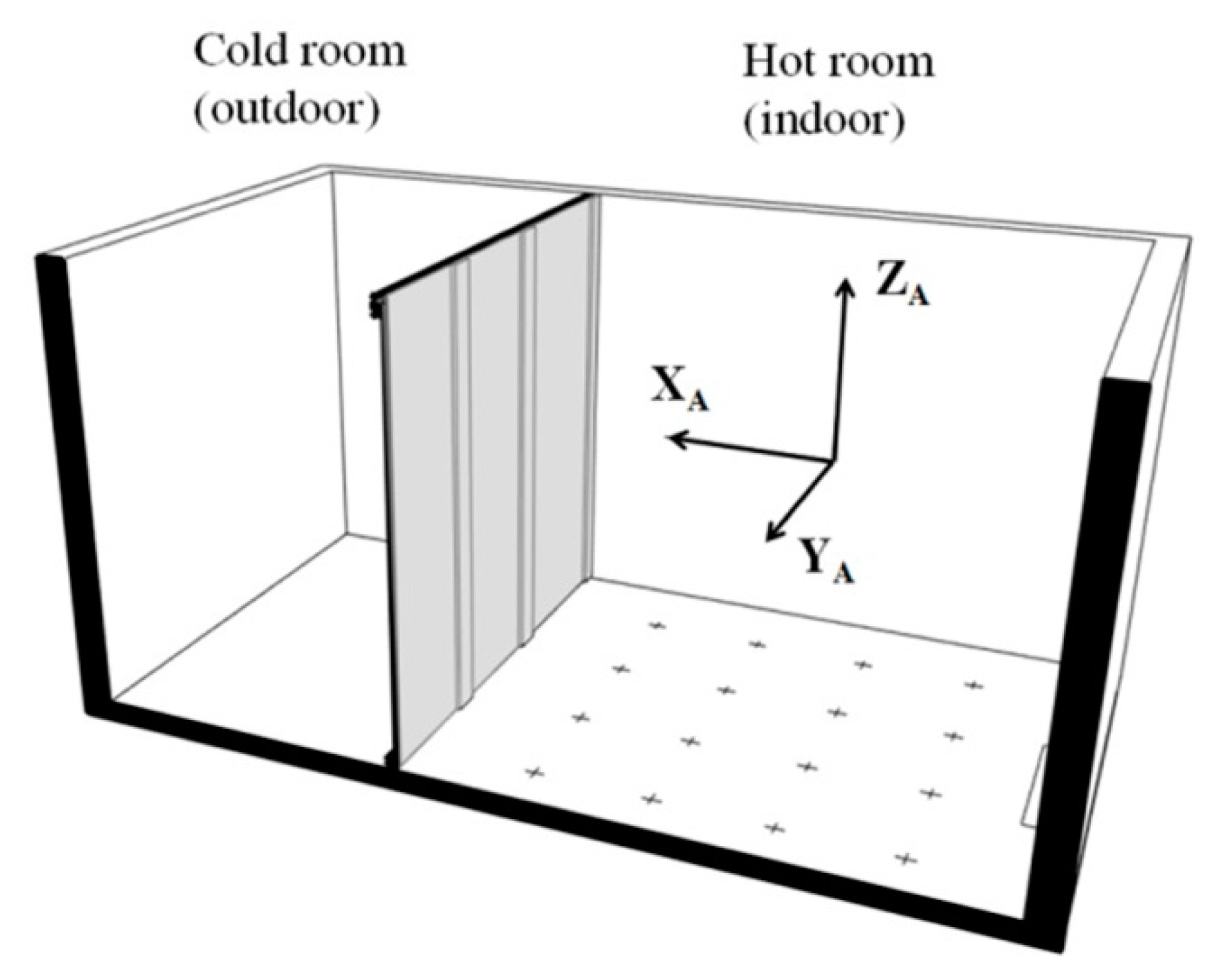
2.2. Analysis at Room Level
Thermal Comfort Measurements
3. Results
3.1. Results at the Material Level
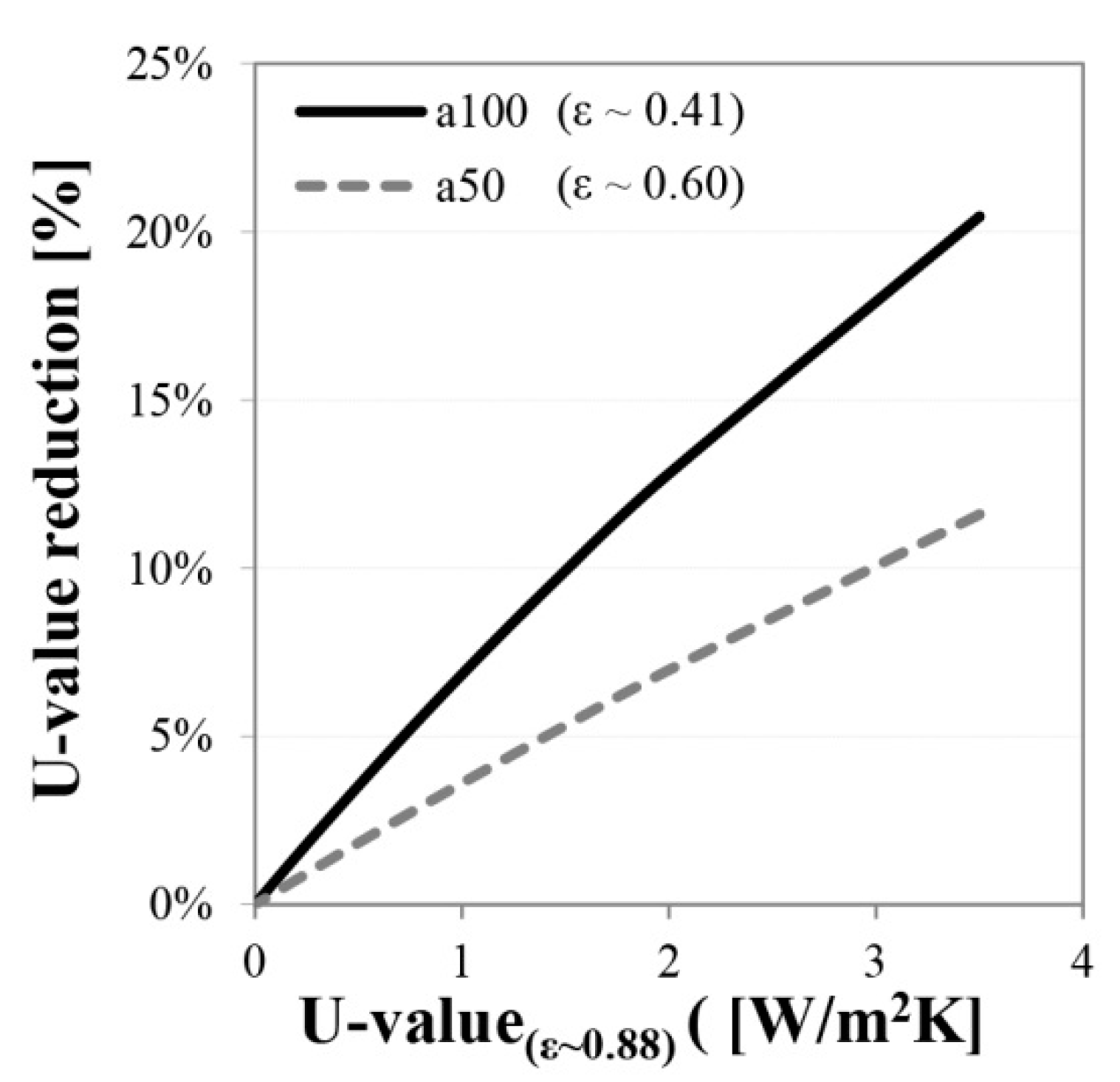
3.2. Results at Room Level
- the application of a100 paint (ε = 0.41) show a reduction of ~35% of the surface heat transfer coefficient hi both in measurements and in the results that were obtained by applying the ISO 6946:2007 (Table 5); and,
- the application of a50 paint (ε = 0.60) show a reduction of ~19% of the surface heat transfer coefficient hi, similar results with a reduction of ~21% was obtained calculating hi (predicted) according to ISO 6946:2007 (Table 5);
- an increase of the average mean radiant temperature of up to ~0.9 (ε = 0.41) and up to ~0.5 °C (ε = 0.6) respect to the high emissivity paint (ε = 0.88);
- a slight increase in the average operative temperature of the room of ~0.6 and ~0.3 °C respectively with the a100 and the a50 paint;
- linear increase of the , the and the PMV according to the decrease of the surface emissivity (Figure 13); and,
- a decrease of the average radiant planar asymmetry along the X-axis from 2.90 °C (ε = 0.88) to 1.63 °C (ε = 0.41).
4. Conclusions
- a significant reduction of the indoor surface heat transfer coefficient, respectively of ~35% with 100% aluminium paint and 19% with 50% aluminium paint that can result in the slightly but non-negligible reduction of the wall heat losses (especially in non-insulated walls);
- a decrease of the measured wall U-value above 20% (from 3.49 to 2.74 W/m2 K) with a 100% aluminium paint. However, it is worth mentioning that, for a slightly insulated wall (U-value = 0.5 W/m2 K), the U-value reduction that can be achieved is below 4%, meaning that the effect of the low emissivity coating is negligible for more insulated building components; and,
- an increase of the indoor mean radiant temperature of ~0.9 °C and ~0.5 °C and of the operative temperature of ~0.6 °C and ~0.3 °C respectively with 100% and 50% of aluminium paint.
- IRCC represents a low-cost solution that can be easily applied on the interior side of the external walls;
- IRCC can be easily coupled with other insulating technologies (i.e., aerogel rendering and coatings [17]) making it a promising solution for all the interventions, in which space-saving represents a constraint; and,
- further improvements in the paint emissivity properties can be achieved working towards optimization of the final mixture.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
| Sample | Point | Distance from Wall | Tair | RH | vair | Tpr,XB | Tpr,XA | Tpr,YB | Tpr,YA | Tpr,ZB | Tpr,ZA | Tmr | Top | PMV | PPD |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| [m] | [°C] | [%] | [m/s] | [°C] | [°C] | [°C] | [°C] | [°C] | [°C] | [°C] | [°C] | [-] | [%] | ||
| a0 | 1 | 0.6 | 22.7 | 42 | 0.00 | 21.8 | 18.2 | 21.4 | 20.0 | 20.6 | 21.1 | 20.44 | 21.57 | −0.6 | 12.5 |
| 2 | 0.6 | 22.6 | 42 | 0.01 | 21.8 | 18.3 | 21.3 | 20.2 | 20.6 | 21.1 | 20.48 | 21.54 | −0.6 | 12.5 | |
| 3 | 0.6 | 22.7 | 42 | 0.01 | 21.8 | 18.4 | 21.4 | 20.2 | 20.5 | 21.2 | 20.51 | 21.61 | −0.6 | 12.5 | |
| 4 | 0.6 | 22.6 | 42 | 0.02 | 21.8 | 18.3 | 21.5 | 20.3 | 20.6 | 21.1 | 20.52 | 21.56 | −0.6 | 12.5 | |
| 5 | 1.2 | 22.6 | 42 | 0.01 | 22.0 | 18.5 | 21.7 | 20.6 | 21.1 | 21.7 | 20.83 | 21.71 | −0.6 | 12.5 | |
| 6 | 1.2 | 22.7 | 42 | 0.02 | 21.9 | 18.5 | 21.7 | 20.7 | 21.2 | 21.6 | 20.82 | 21.76 | −0.6 | 12.5 | |
| 7 | 1.2 | 22.7 | 42 | 0.04 | 22.0 | 18.4 | 21.8 | 20.7 | 21.3 | 21.8 | 20.88 | 21.79 | −0.6 | 12.5 | |
| 8 | 1.2 | 22.6 | 42 | 0.04 | 22.0 | 18.5 | 21.8 | 20.9 | 21.3 | 21.9 | 20.94 | 21.77 | −0.6 | 12.5 | |
| 9 | 1.8 | 22.5 | 42 | 0.02 | 22.0 | 19.3 | 21.9 | 21.0 | 21.2 | 22.0 | 21.15 | 21.82 | −0.6 | 12.5 | |
| 10 | 1.8 | 22.7 | 42 | 0.02 | 21.8 | 19.4 | 21.8 | 20.9 | 21.2 | 22.1 | 21.11 | 21.90 | −0.5 | 10.2 | |
| 11 | 1.8 | 22.6 | 42 | 0.00 | 21.9 | 19.5 | 21.8 | 21.0 | 21.3 | 22.1 | 21.18 | 21.89 | −0.6 | 12.5 | |
| 12 | 1.8 | 22.5 | 42 | 0.01 | 22.0 | 19.5 | 21.8 | 21.0 | 21.4 | 22.2 | 21.22 | 21.86 | −0.5 | 10.2 | |
| 13 | 2.4 | 22.7 | 42 | 0.02 | 22.1 | 19.9 | 22.0 | 21.1 | 21.5 | 22.0 | 21.37 | 22.03 | −0.5 | 10.2 | |
| 14 | 2.4 | 22.6 | 42 | 0.02 | 21.9 | 19.8 | 21.9 | 21.2 | 21.6 | 22.2 | 21.34 | 21.97 | −0.5 | 10.2 | |
| 15 | 2.4 | 22.6 | 42 | 0.02 | 22.0 | 20.0 | 22.0 | 21.1 | 21.7 | 22.1 | 21.40 | 22.00 | −0.5 | 10.2 | |
| 16 | 2.4 | 22.5 | 42 | 0.02 | 22.1 | 20.0 | 22.0 | 21.1 | 21.6 | 22.2 | 21.43 | 21.96 | −0.5 | 10.2 | |
| a50 | 1 | 0.6 | 22.6 | 42 | 0.03 | 22.3 | 19.0 | 21.1 | 20.7 | 20.4 | 21.5 | 20.81 | 21.70 | −0.6 | 12.5 |
| 2 | 0.6 | 22.5 | 42 | 0.02 | 22.1 | 19.1 | 21.1 | 20.9 | 20.3 | 21.7 | 20.83 | 21.66 | −0.6 | 12.5 | |
| 3 | 0.6 | 22.5 | 42 | 0.03 | 22.3 | 19.0 | 21.2 | 21.0 | 20.2 | 21.5 | 20.84 | 21.67 | −0.6 | 12.5 | |
| 4 | 0.6 | 22.7 | 42 | 0.01 | 22.3 | 19.1 | 21.3 | 21.2 | 20.4 | 21.6 | 20.95 | 21.83 | −0.5 | 10.2 | |
| 5 | 1.2 | 22.7 | 42 | 0.03 | 22.3 | 19.7 | 21.5 | 21.3 | 20.7 | 22.0 | 21.22 | 21.96 | −0.5 | 10.2 | |
| 6 | 1.2 | 22.8 | 42 | 0.02 | 22.4 | 19.9 | 21.5 | 21.4 | 20.8 | 22.1 | 21.32 | 22.06 | −0.5 | 10.2 | |
| 7 | 1.2 | 22.6 | 42 | 0.02 | 22.3 | 19.9 | 21.6 | 21.6 | 20.9 | 22.3 | 21.39 | 21.99 | −0.5 | 10.2 | |
| 8 | 1.2 | 22.6 | 42 | 0.02 | 22.4 | 20.0 | 21.7 | 21.7 | 21.0 | 22.2 | 21.46 | 22.03 | −0.5 | 10.2 | |
| 9 | 1.8 | 22.7 | 42 | 0.03 | 22.3 | 20.4 | 21.9 | 21.8 | 21.2 | 22.1 | 21.58 | 22.14 | −0.4 | 8.3 | |
| 10 | 1.8 | 22.7 | 42 | 0.03 | 22.4 | 20.5 | 21.9 | 21.7 | 21.2 | 22.2 | 21.62 | 22.16 | −0.4 | 8.3 | |
| 11 | 1.8 | 22.8 | 42 | 0.02 | 22.6 | 20.7 | 22.0 | 21.8 | 21.3 | 22.2 | 21.75 | 22.28 | −0.4 | 8.3 | |
| 12 | 1.8 | 22.8 | 42 | 0.02 | 22.5 | 20.7 | 22.0 | 22.0 | 21.3 | 22.3 | 21.78 | 22.29 | −0.4 | 8.3 | |
| 13 | 2.4 | 22.7 | 42 | 0.04 | 22.6 | 20.8 | 22.1 | 22.0 | 21.4 | 22.3 | 21.85 | 22.27 | −0.4 | 8.3 | |
| 14 | 2.4 | 22.8 | 42 | 0.02 | 22.6 | 21.0 | 22.2 | 22.1 | 21.5 | 22.1 | 21.91 | 22.36 | −0.4 | 8.3 | |
| 15 | 2.4 | 22.8 | 42 | 0.03 | 22.5 | 21.0 | 22.2 | 22.0 | 21.5 | 22.3 | 21.90 | 22.35 | −0.4 | 8.3 | |
| 16 | 2.4 | 22.8 | 42 | 0.02 | 22.4 | 21.1 | 22.3 | 22.0 | 21.4 | 22.1 | 21.88 | 22.34 | −0.4 | 8.3 | |
| a100 | 1 | 0.6 | 22.7 | 42 | 0.03 | 22.1 | 19.8 | 21.3 | 20.9 | 20.6 | 21.2 | 20.98 | 21.84 | −0.5 | 12.5 |
| 2 | 0.6 | 22.7 | 42 | 0.01 | 22.5 | 20.1 | 21.2 | 21.1 | 20.8 | 21.4 | 21.20 | 21.95 | −0.5 | 12.5 | |
| 3 | 0.6 | 22.8 | 42 | 0.02 | 22.4 | 20.0 | 21.4 | 21.1 | 20.8 | 21.5 | 21.20 | 22.00 | −0.5 | 12.5 | |
| 4 | 0.6 | 22.9 | 42 | 0.03 | 22.4 | 20.2 | 21.6 | 21.5 | 21.0 | 21.6 | 21.38 | 22.14 | −0.5 | 12.5 | |
| 5 | 1.2 | 22.8 | 42 | 0.01 | 22.7 | 20.6 | 21.9 | 21.8 | 21.3 | 22.0 | 21.71 | 22.26 | −0.4 | 8.3 | |
| 6 | 1.2 | 23.0 | 42 | 0.02 | 22.7 | 20.6 | 21.8 | 21.6 | 21.5 | 21.9 | 21.68 | 22.34 | −0.4 | 8.3 | |
| 7 | 1.2 | 22.9 | 42 | 0.03 | 22.6 | 20.6 | 22.0 | 21.6 | 21.5 | 22.0 | 21.70 | 22.30 | −0.4 | 8.3 | |
| 8 | 1.2 | 22.9 | 42 | 0.01 | 22.7 | 20.8 | 22.0 | 22.0 | 21.5 | 22.1 | 21.84 | 22.37 | −0.4 | 8.3 | |
| 9 | 1.8 | 23.0 | 42 | 0.03 | 22.6 | 21.3 | 22.3 | 22.2 | 21.9 | 22.3 | 22.08 | 22.54 | −0.4 | 8.3 | |
| 10 | 1.8 | 23.0 | 42 | 0.02 | 22.8 | 21.3 | 22.4 | 22.1 | 22.1 | 22.3 | 22.15 | 22.58 | −0.3 | 6.9 | |
| 11 | 1.8 | 22.9 | 42 | 0.03 | 22.7 | 21.5 | 22.3 | 22.0 | 22.0 | 22.2 | 22.12 | 22.51 | −0.4 | 8.3 | |
| 12 | 1.8 | 22.7 | 42 | 0.03 | 22.6 | 21.5 | 22.3 | 22.2 | 21.9 | 22.2 | 22.11 | 22.41 | −0.4 | 8.3 | |
| 13 | 2.4 | 22.8 | 42 | 0.03 | 22.5 | 21.7 | 22.5 | 22.4 | 22.2 | 22.5 | 22.27 | 22.54 | −0.4 | 8.3 | |
| 14 | 2.4 | 23.0 | 42 | 0.02 | 22.8 | 21.7 | 22.2 | 22.7 | 22.2 | 22.5 | 22.34 | 22.67 | −0.3 | 6.9 | |
| 15 | 2.4 | 23.0 | 42 | 0.03 | 22.7 | 21.7 | 22.5 | 22.1 | 22.6 | 22.4 | 22.31 | 22.65 | −0.3 | 6.9 | |
| 16 | 2.4 | 22.9 | 42 | 0.02 | 22.6 | 21.9 | 22.5 | 22.3 | 22.6 | 22.3 | 22.35 | 22.62 | −0.3 | 6.9 |
References
- Lee, S.W.; Lim, C.H.; Salleh, E.I.B. Reflective thermal insulation systems in building: A review on radiant barrier and reflective insulation. Renew. Sustain. Energy Rev. 2016, 65, 643–662. [Google Scholar] [CrossRef]
- Krnst, S. Heat Insulation. U.S. patent 1890418 A, 6 December 1932. [Google Scholar]
- Eduard, D. Heat Insulation for Air Spaces. U.S. patent 1934174 A, 7 November 1933. [Google Scholar]
- Hens, H. Building Physics: Heat, Air and Moisture. Fundamentals and Engineering Methods with Examples and Exercises, 2nd ed.; Ernst & Sohn GmbH & Co. KG: Berlin, Germany, 2012. [Google Scholar]
- Incropera, F.P.; DeWitt, D.P. Fundamentals of Heat and Mass Transfer, 3rd ed.; Wiley: Hoboken, NJ, USA, 1990. [Google Scholar]
- Reflective Insulation Manufacturers Association International (RIMA-I). Understanding and Using Reflective Insulation, Radiant Barriers and Radiation Control Coatings. 2014. Available online: http://www.insulationstop.com/radiant-barrier-blog/updated-handbook-understanding-using-reflective-insulation-radiant-barrier-irccs/ (accessed on 24 December 2019).
- Jelle, B.P.; Kalnæs, S.E.; Gao, T. Low-emissivity materials for building applications: A state-of-the-art review and future research perspectives. Energy Build. 2015, 96, 329–356. [Google Scholar] [CrossRef] [Green Version]
- Fantucci, S.; Serra, V. Low-E paints enhanced building components: Performance, limits and research perspectives. Energy Procedia 2017, 126, 274–281. [Google Scholar] [CrossRef] [Green Version]
- Fantucci, S.; Serra, V.; Martinelly, A. Thermal effectiveness of low emissivity coatings in hollow bricks: A numerical analysis for different cavity concentration. In Proceedings of the 9th IMC “International Masonry Conference”, Guimarães, Portugal, 7–9 July 2014. [Google Scholar]
- Principi, P.; Fioretti, R. Thermal analysis of the application of pcm and low emissivity coating in hollow bricks. Energy Build. 2012, 51, 131–142. [Google Scholar] [CrossRef]
- Principi, P.; Fioretti, R. Thermal Performance of Hollow Clay Brick with Low Emissivity Treatment in Surface Enclosures. Coatings 2014, 4, 715–731. [Google Scholar] [CrossRef] [Green Version]
- Sedlbauer, K.; Krus, M.; Fitz, C.; Künzel, H.M. Reducing the Risk of Microbial Growth on Insulated Walls by PCM Enhanced Renders and IR Reflecting Paints. In Proceedings of the International Conference on Durability of Building Materials and Components, XII DBMC, Porto, Portugal, 12–15 April 2011. [Google Scholar]
- Fantucci, S.; Serra, V. Investigating the performance of reflective insulation and low emissivity paints for the energy retrofit of roof attics. Energy Build. 2019, 182, 300–310. [Google Scholar] [CrossRef]
- Joudi, H.; Svedung, M.; Cehlin, M. Rönnelid. Highly reflective coatings for interior and exterior steel cladding and the energy efficiency of buildings. Appl. Energy 2011, 88, 4655–4666. [Google Scholar] [CrossRef]
- Joudi, H.; Svedung, M.; Cehlin, M. Rönnelid. Reflective coatings for interior and exterior of buildings and improving thermal performance. Appl. Energy 2013, 103, 562–570. [Google Scholar] [CrossRef]
- Buckmaster, D.J.; Abramson, A.R. The effects of interior emissivity and room layout on forced air space-conditioning power usage. Int. J. Heat Mass Transf. 2015, 89, 216–228. [Google Scholar] [CrossRef]
- Ibrahim, M.; Bianco, L.; Ibrahim, O.; Wurtz, E. Low-emissivity coating coupled with aerogel-based plaster for walls’ internal surface application in buildings: Energy saving potential based on thermal comfort assessment. J. Build. Eng. 2018, 18, 454–466. [Google Scholar] [CrossRef]
- Bagdade, S.D. ASM Ready Reference: Thermal Properties of Metals; Materials Data Series; ASM: Aimere, Netherlands, 2002. [Google Scholar]
- ASTM C1371-15. Standard Test Method for Determination of Emittance of Materials Near Room Temperature Using Portable Emissometers; ASTM: West Conshohocken, PA, USA, 2015. [Google Scholar]
- Tenpierik, M.J.; Hasselaar, E. Reflective multi-foil insulations for buildings: A review. Energy Build. 2013, 56, 233–243. [Google Scholar] [CrossRef]
- EN 1946-3. Thermal Performance of Building Products and Components—Specific Criteria for the Assessment of Laboratories Measuring Heat Transfer Properties—Part 3: Measurements by Heat Flow Meter Method; European Committee For Standardization (CEN): Brussels, BE, USA, 2004. [Google Scholar]
- ISO 9869-1. Thermal Insulation: Building Elements—In-Situ Measurement of Thermal Resistance and Thermal Transmittance. Part 1: Heat Flow Meter Method; ISO: Geneva, Switzerland, 2015. [Google Scholar]
- EN ISO 7726. Ergonomics of the Thermal Environment—Instruments for Measuring Physical Quantities; European Committee For Standardization (CEN): Brussels, BE, USA, 2001. [Google Scholar]
- Fanger, P.O. Thermal Comfort; Danish Technical Press: Copenhagen, Denmark, 1970. [Google Scholar]
- EN ISO 7730. Ergonomics of Thermal Environment—Analytical Determination and Interpretation of Thermal Comfort Using Calculation of the PMV and PPD Indices and Local Thermal Comfort Criteria; European Committee For Standardization (CEN): Brussels, BE, USA, 2006. [Google Scholar]





| Temperature operation | −15 °C to 85 °C |
| Temperature control | to 0.01 °C |
| Maximum specimen size | 610 × 610 mm |
| Measurement area | 254 × 254 mm |
| Conductivity range | 0.001 to 0.35 W/m K |
| Absolute accuracy | ±1% |
| Reproducibility | ±0.5% |
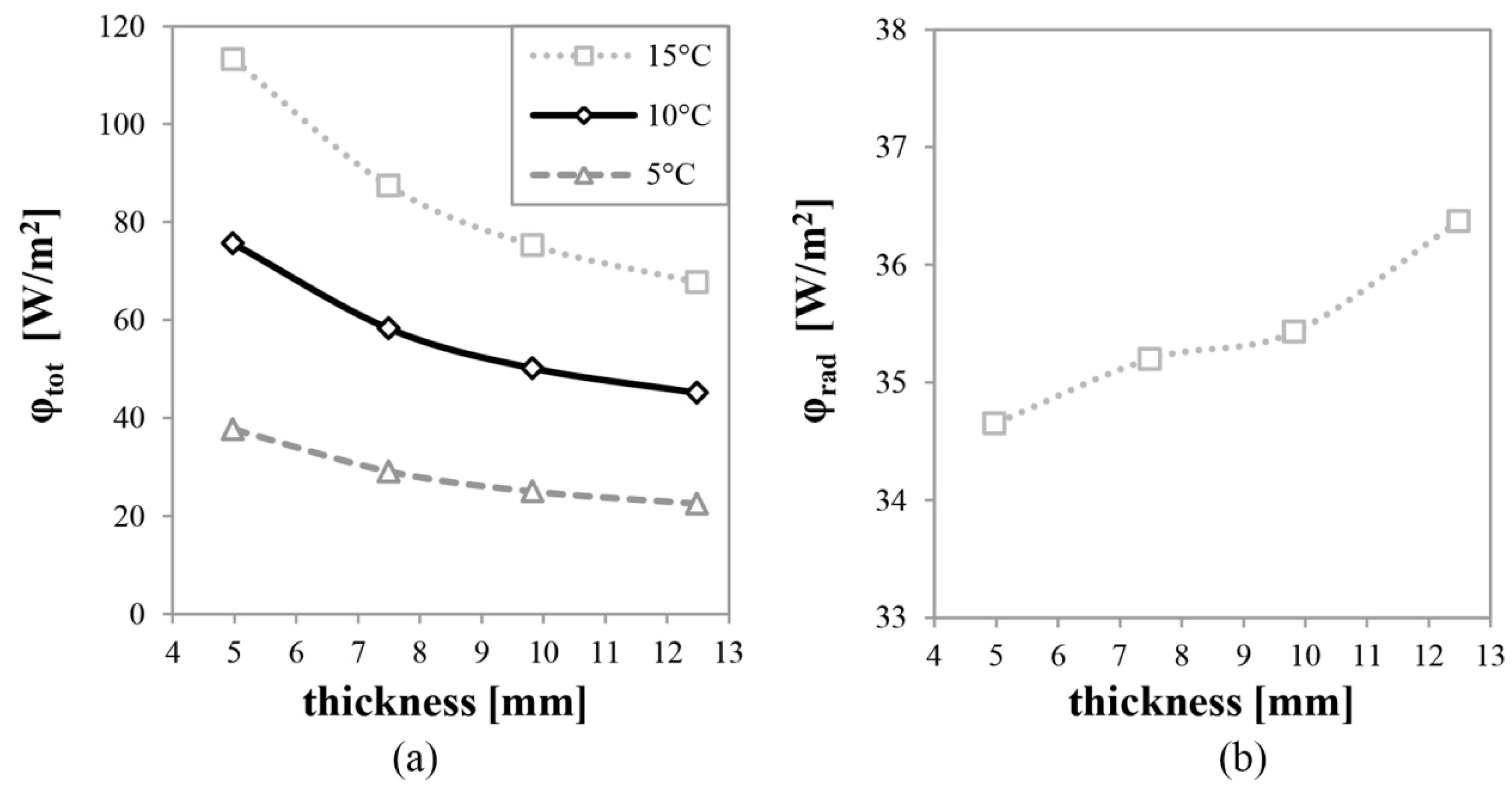
| s | ΔT | φtot | φcond | φrad | ε |
|---|---|---|---|---|---|
| [mm] | [°C] | [W/m2] | [W/m2] | [W/m2] | [-] |
| 12.48 | 15 | 67.70 | 31.33 | 36.37 | 0.432 |
| 9.82 | 15 | 75.25 | 39.82 | 35.43 | 0.420 |
| 7.49 | 15 | 87.43 | 52.23 | 35.20 | 0.417 |
| 4.98 | 15 | 113.20 | 78.55 | 34.65 | 0.410 |
| 12.48 | 10 | 45.15 | 20.88 | 24.26 | 0.433 |
| 9.82 | 10 | 50.17 | 26.54 | 23.63 | 0.421 |
| 7.49 | 10 | 58.30 | 34.79 | 23.51 | 0.418 |
| 4.98 | 10 | 75.63 | 52.42 | 23.20 | 0.412 |
| 12.48 | 5 | 22.51 | 10.44 | 12.06 | 0.430 |
| 9.82 | 5 | 25.01 | 13.27 | 11.74 | 0.418 |
| 7.49 | 5 | 29.10 | 17.40 | 11.70 | 0.416 |
| 4.98 | 5 | 37.72 | 26.18 | 11.54 | 0.410 |
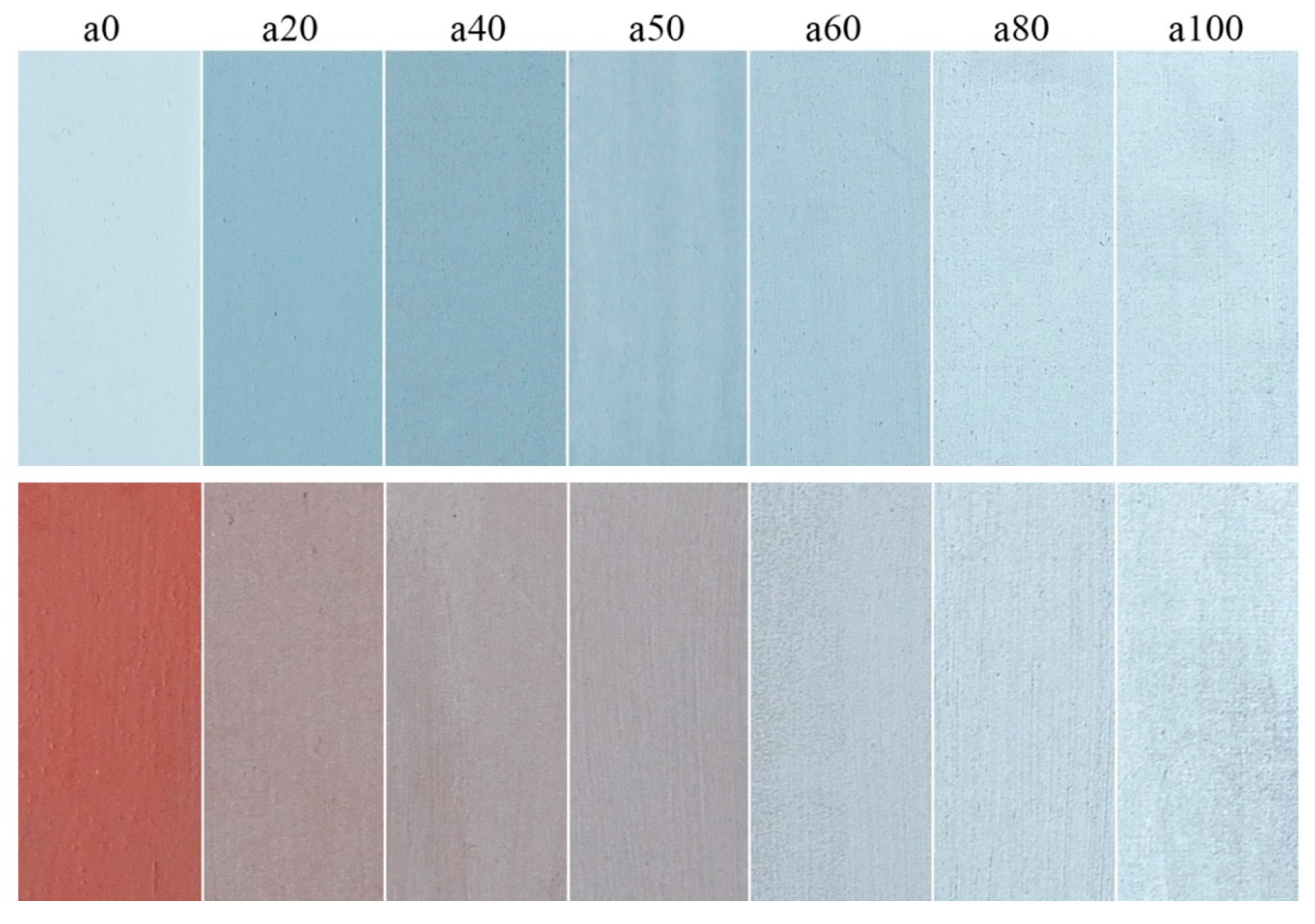
| Sample Name | Al Paint Concentration |
|---|---|
| [%] | |
| a100 | 100% |
| a80 | 80% |
| a60 | 60% |
| a50 | 50% |
| a40 | 40% |
| a20 | 20% |
| a0 | 0% |
| c (reference aluminium foil) | - |
| Sample Name | Al Paint Concentration | φ rad | ε |
|---|---|---|---|
| [%] | [W/m2] | [-] | |
| a100 | 100% | 11.54 | 0.410 |
| a80 | 80% | 13.86 | 0.500 |
| a60 | 60% | 14.42 | 0.522 |
| a50 | 50% | 16.32 | 0.597 |
| a40 | 40% | 19.60 | 0.732 |
| a20 | 20% | 19.73 | 0.737 |
| a0 | 0% | 23.04 | 0.881 |
| c (reference aluminium foil) | - | 0.040 |
| Sample | Tair,i (Heated Room) | Tair,e (Cooled Room) | Ts,i | Ts,e | φ | hi (Measured) | hi (Predicted) | U |
|---|---|---|---|---|---|---|---|---|
| [°C] | [°C] | [°C] | [°C] | [W/m2] | [W/m2 K] | [W/m2 K] | [W/m2 K] | |
| a100 | 21.6 | 9.6 | 14.3 | 12.5 | 32.8 | 4.47 | 4.73 | 2.74 |
| a50 | 21.6 | 9.7 | 15.0 | 13.0 | 37.3 | 5.64 | 5.75 | 3.14 |
| a0 | 21.6 | 9.9 | 15.8 | 13.6 | 40.4 | 6.93 | 7.25 | 3.49 |
| Config | ||||||
|---|---|---|---|---|---|---|
| [°C] | [°C] | [°C] | [°C] | [%] | ||
| a100 | 22.88 | 21.84 | 1.63 | 22.36 | −0.40 | 9.00 |
| a50 | 22.69 | 21.44 | 2.28 | 22.07 | −0.47 | 9.68 |
| a0 | 22.62 | 20.98 | 2.90 | 21.80 | −0.56 | 11.64 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fantucci, S.; Serra, V. Experimental Assessment of the Effects of Low-Emissivity Paints as Interior Radiation Control Coatings. Appl. Sci. 2020, 10, 842. https://doi.org/10.3390/app10030842
Fantucci S, Serra V. Experimental Assessment of the Effects of Low-Emissivity Paints as Interior Radiation Control Coatings. Applied Sciences. 2020; 10(3):842. https://doi.org/10.3390/app10030842
Chicago/Turabian StyleFantucci, Stefano, and Valentina Serra. 2020. "Experimental Assessment of the Effects of Low-Emissivity Paints as Interior Radiation Control Coatings" Applied Sciences 10, no. 3: 842. https://doi.org/10.3390/app10030842
APA StyleFantucci, S., & Serra, V. (2020). Experimental Assessment of the Effects of Low-Emissivity Paints as Interior Radiation Control Coatings. Applied Sciences, 10(3), 842. https://doi.org/10.3390/app10030842






