Cratering for Impact of Hypervelocity Projectiles into Granite Targets within a Velocity Range of 1.91–3.99 km/s: Experiments and Analysis
Abstract
:Featured Application
Abstract
1. Introduction
2. Experimental Methods
2.1. Projectiles
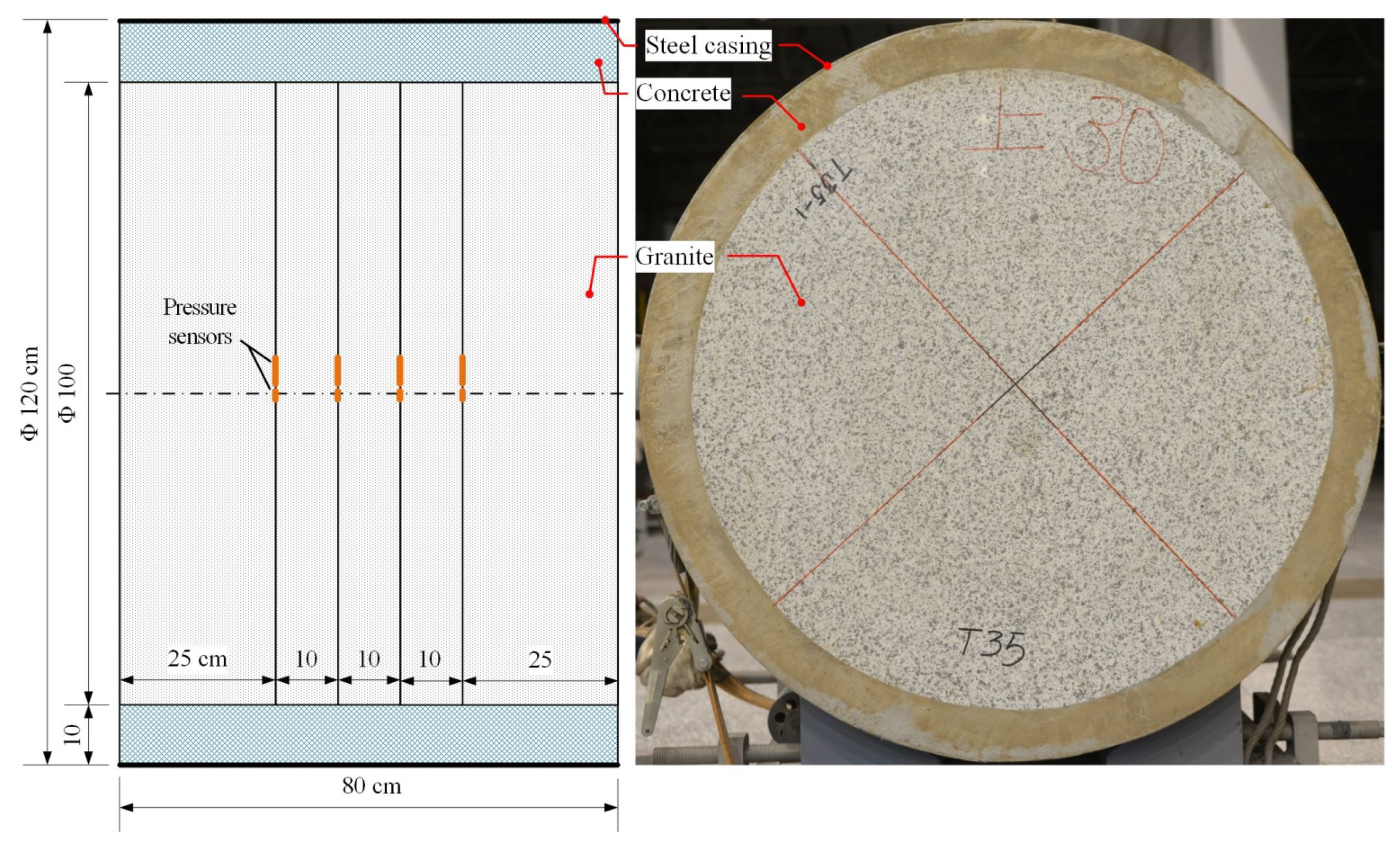
2.2. Targets
2.3. Set-Up
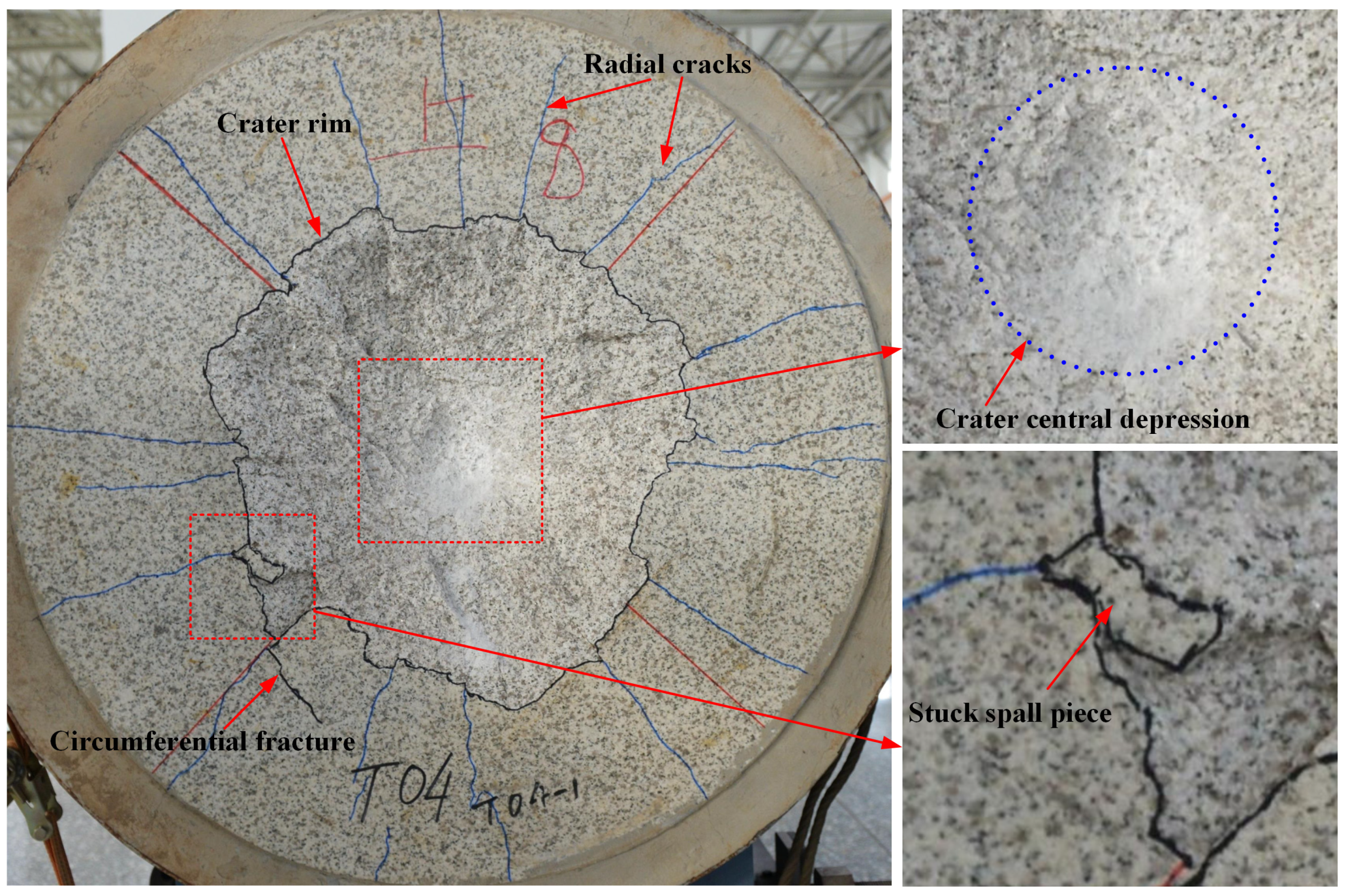
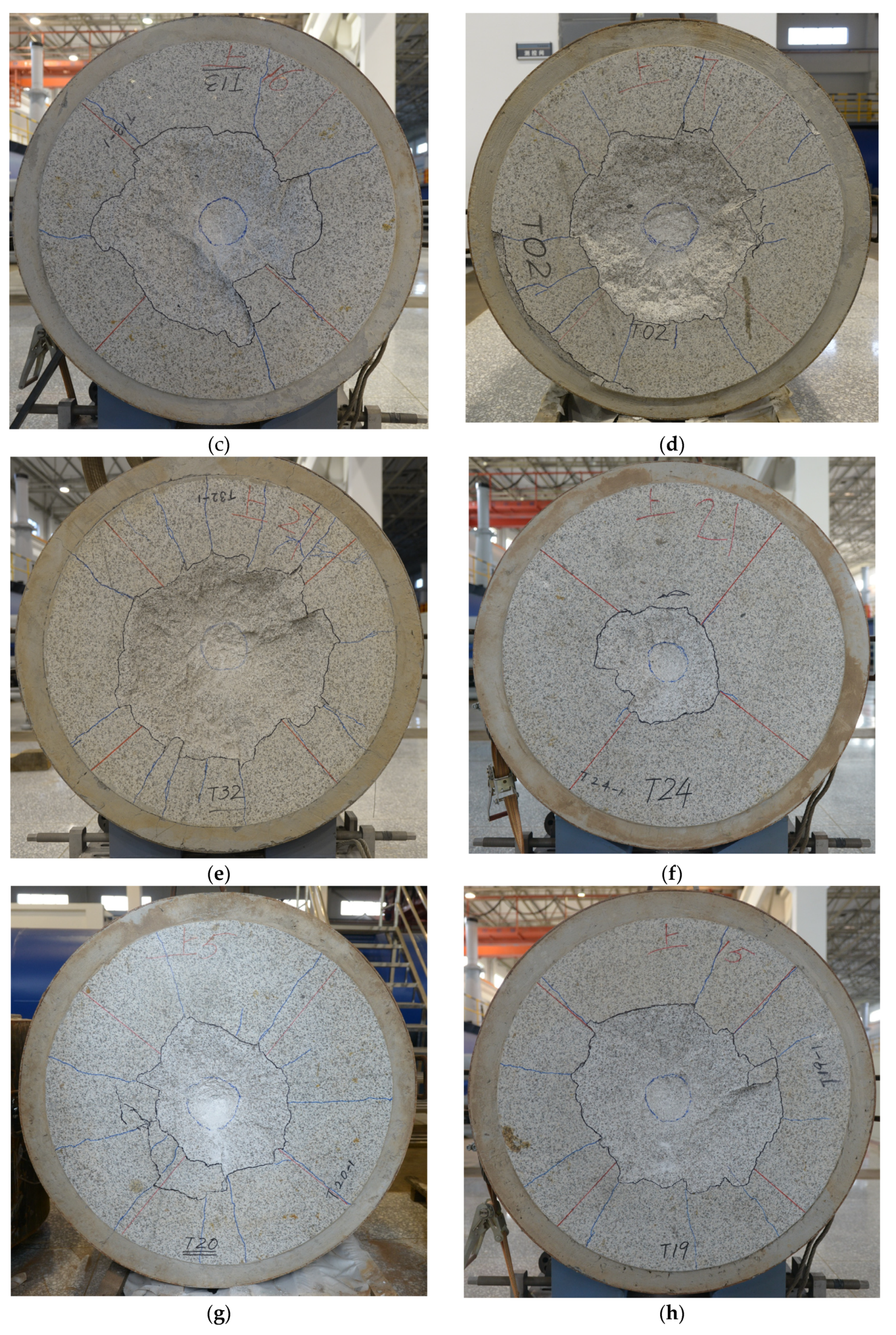
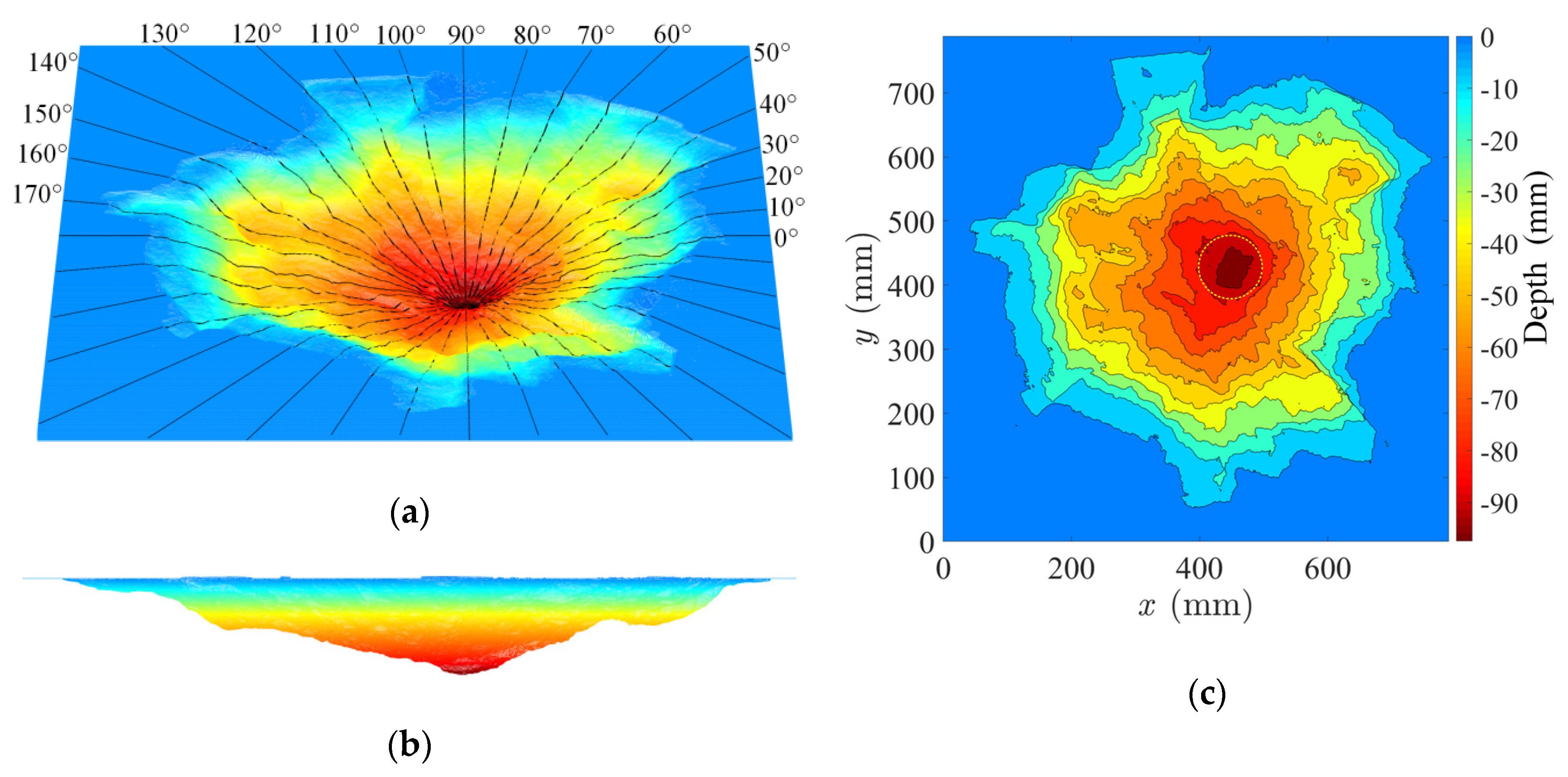
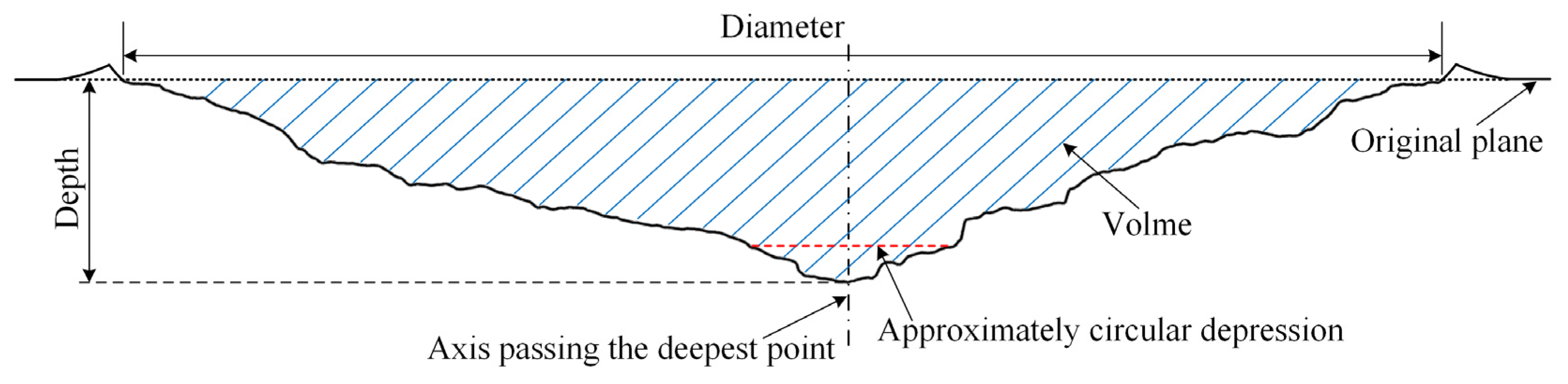
3. Experimental Results
4. Discussion
4.1. Influences of Impact Velocity
4.2. Influences of Projectile–Target Ratio
4.3. Scaling Laws of Impact Crater Parameters
5. Conclusions
- (1)
- Fifteen shots of hypervelocity impact cratering into granite targets were carried out using hemispherical-nosed cylindrical projectiles with the impact velocity range of 1.91–3.99 km/s. The mass of each projectile was 40 g, and the length–diameter ratio was 2. Projectile materials were TC4 titanium alloy, 30CrMnSiA alloy steel, and 93W tungsten alloy. The projectile–target density ratio ranged from 1.71 to 6.86.
- (2)
- The crater H/D ratio varied between 0.14 and 0.24, which shows negative correlation with the impact velocity and weak positive correlation with the projectile–target density ratio.
- (3)
- Based on the dimensional analysis, the crater parameters were expressed as power law equations of impact parameters, whose coefficients and exponents were calculated by regression analysis, and the values predicted by the regression equations agreed well with the experimental results with good reliability.
- (4)
- The crater depth is more dependent on the projectile–target density ratio than the crater diameter.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| ρp | Projectile density |
| mp | Projectile mass |
| l | Projectile length |
| d | Projectile diameter |
| l/d | Projectile length–diameter ratio |
| u | Impact velocity |
| Ek | Impact kinetic energy |
| ρt | Target density |
| Y | Uniaxial compressive strength of the target granite |
| ρp/ρt | Projectile–target density ratio |
| H | Crater depth |
| D | Crater diameter |
| V | Crater volume |
| H/D | Crater depth-diameter ratio |
| H/l | Dimensionless crater depth |
| D/d | Dimensionless crater diameter |
| V/ld | Dimensionless crater volume |
References
- Kenkmann, T.; Poelchau, M.H.; Wulf, G. Structural geology of impact craters. J. Struct. Geol. 2014, 62, 156–182. [Google Scholar] [CrossRef]
- Katz, S.; Grossman, E.; Gouzman, I.; Lempert, G.; Brill, A.; Wagner, H.D. Effect of Hypervelocity Impactor Properties on Target Materials Damage. J. Spacecr. Rockets 2012, 49, 232–273. [Google Scholar] [CrossRef]
- Kinslow, R.; Cable, A.J. High-Velocity Impact Phenomena; Academic Press: New York, NY, USA, 1970; ISBN 978-0-12-408950-1. [Google Scholar]
- Heberling, T.; Terrones, G.; Weseloh, W. Hydrocode simulations of a hypervelocity impact experiment over a range of velocities. Int. J. Impact Eng. 2018, 122, 1–9. [Google Scholar] [CrossRef]
- Chhabildas, L.C.; Davison, L.; Horie, Y. (Eds.) High-Pressure Shock Compression of Solids VIII: The Science and Technology of High-Velocity Impact; Springer: Heidelberg, Berlin, Germany, 2005; ISBN 978-3-540-27168-0. [Google Scholar]
- Lexow, B.; Wickert, M.; Thoma, K.; Schaefer, F.; Poelchau, M.H.; Kenkmann, T. The extra-large light-gas gun of the Fraunhofer EMI: Applications for impact cratering research. Meteorit. Planet. Sci. 2013, 48, 3–7. [Google Scholar] [CrossRef]
- Putzar, R.; Schaefer, F. Concept for a new light-gas gun type hypervelocity accelerator. Int. J. Impact Eng. 2016, 88, 118–124. [Google Scholar] [CrossRef]
- Charters, A.C.; Locke, G.S., Jr. A Preliminary Investigation of High-speed Impact: The Penetration of Small Spheres into Thick Copper Targets; NACA: Moffett Field, CA, USA, 1958. [Google Scholar]
- Murr, L.E.; Quinones, S.A.; Ferreyra, E.; Ayala, A.; Valerio, O.L.; Horz, F.; Bernhard, R.P. The low-velocity-to-hypervelocity penetration transition for impact craters in metal targets. Mater. Sci. Eng. Struct. Mater. Prop. Microstruct. Process. 1998, 256, 166–182. [Google Scholar] [CrossRef]
- Orphal, D.L.; Franzen, R.R. Penetration of confined silicon carbide targets by tungsten long rods at impact velocities from 1.5 to 4.6 km/s. Int. J. Impact Eng. 1997, 19, 1–13. [Google Scholar] [CrossRef]
- Barnouin-Jha, O.S.; Yamamoto, S.; Toriumi, T.; Sugita, S.; Matsui, T. Non-intrusive measurements of crater growth. Icarus 2007, 188, 506–521. [Google Scholar] [CrossRef]
- Crawford, G.; Hill, D.; Rose, F.E.; Zee, R.; Best, S.; Crumpler, M. Hypervelocity Impact Study: The Effect of Impact Angle on Crater Morphology; NASA: Washington, DC, USA, 1993.
- Burchell, M.J.; Mackay, N.G. Crater ellipticity in hypervelocity impacts on metals. J. Geophys. Res. 1998, 103, 22761–22774. [Google Scholar] [CrossRef]
- Burchell, M.J.; Grey, I.D.S. Oblique hypervelocity impacts on thick glass targets. Mater. Sci. Eng. Struct. Mater. Prop. Microstruct. Process. 2001, 303, 134–141. [Google Scholar] [CrossRef]
- Burchell, M.J.; Whitehorn, L. Oblique incidence hypervelocity impacts on rock. Mon. Not. R. Astron. Soc. 2003, 341, 192–198. [Google Scholar] [CrossRef] [Green Version]
- Michikami, T.; Hagermann, A.; Morota, T.; Haruyama, J.; Hasegawa, S. Oblique impact cratering experiments in brittle targets: Implications for elliptical craters on the Moon. Planet. Space Sci. 2017, 135, 27–36. [Google Scholar] [CrossRef]
- Kinard, W.H.; Collins, R.D. An Investigation of High-Velocity Impact Cratering into Nonmetallic Targets and Correlation of Penetration Data for Metallic and Nonmetallic Targets; NASA: Washington, DC, USA, 1961.
- Cannon, E.T.; Turner, G.H. Cratering in Low-Density Targets; NASA: Washington, DC, USA, 1967.
- Clough, N.; McMillan, A.R.; Lieblein, S. Crater Characteristics of 11 Metal Alloys under Hyper-Velocity Impact Including Effects of Projectile Density and Target Temperature; NASA: Washington, DC, USA, 1969.
- Hörz, F.; Cintala, M.J.; Bernhard, R.P.; See, T.H. Cratering and penetration experiments in teflon targets at velocities from 1 to 7 km/s. Int. J. Impact Eng. 1995, 17, 419–430. [Google Scholar] [CrossRef] [Green Version]
- Silvestrov, V.V.; Plastinin, A.V.; Pai, V.V.; Yakovlev, I.V. Hypervelocity impact on isotropic composites with metal or ceramic inclusions. Int. J. Impact Eng. 1997, 20, 733–742. [Google Scholar] [CrossRef]
- Nechitailo, N. Hypervelocity Penetration into Soil. Procedia Eng. 2015, 103, 427–435. [Google Scholar] [CrossRef] [Green Version]
- Prieur, N.C.; Rolf, T.; Luther, R.; Wuennemann, K.; Xiao, Z.; Werner, S.C. The effect of target properties on transient crater scaling for simple craters. J. Geophys. Res.-Planets 2017, 122, 1704–1726. [Google Scholar] [CrossRef]
- Denardo, B.P.; Nysmith, C.R.; Summers, J.L. Projectile Shape Effects on Hypervelocity Impact Craters in Aluminum; NASA: Washington, DC, USA, 1969.
- Hohler, V.; Stilp, A.J. Hypervelocity impact of rod projectiles with L/D from 1 to 32. Int. J. Impact Eng. 1987, 5, 323–331. [Google Scholar] [CrossRef]
- Kadono, T.; Suzuki, A.I.; Araki, S.; Asada, T.; Suetsugu, R.; Hasegawa, S. Investigation of impact craters on flat surface of cylindrical targets based on experiments and numerical simulations. Planet. Space Sci. 2018, 163, 77–82. [Google Scholar] [CrossRef]
- Itagaki, Y.; Tamura, H.; Watanabe, Y.; Taniyama, K.; Takashima, A. Effects of head shape of projectiles on hypervelocity impact cratering on aluminum 5052 alloy targets at 7 km/s. Int. J. Impact Eng. 2019, 123, 38–47. [Google Scholar] [CrossRef]
- Holsapple, K.A.; Schmidt, R.M. Point source solutions and coupling parameters in cratering mechanics. J. Geophys. Res. 1987, 92, 6350–6376. [Google Scholar] [CrossRef]
- Westine, P.S.; Mullin, S.A. Scale modeling of hypervelocity impact. Int. J. Impact Eng. 1987, 5, 693–701. [Google Scholar] [CrossRef]
- Holsapple, K.A. The Scaling of Impact Processes in Planetary Sciences. Annu. Rev. Earth Planet. Sci. 1993, 21, 333–373. [Google Scholar] [CrossRef]
- Watts, A.J.; Atkinson, D. Dimensional scaling for impact cratering and perforation. Int. J. Impact Eng. 1995, 17, 925–935. [Google Scholar] [CrossRef] [Green Version]
- Kenkmann, T.; Wuennemann, K.; Deutsch, A.; Poelchau, M.H.; Schaefer, F.; Thoma, K. Impact cratering in sandstone: The MEMIN pilot study on the effect of pore water. Meteorit. Planet. Sci. 2011, 46, 890–902. [Google Scholar] [CrossRef]
- Smrekar, S.; Cintala, M.J.; Hörz, F. Small-scale impacts into rock: An evaluation of the effects of target temperature on experimental results. Geophys. Res. Lett. 1986, 13, 745–748. [Google Scholar] [CrossRef]
- Poelchau, M.H.; Kenkmann, T.; Thoma, K.; Hoerth, T.; Dufresne, A.; Schaefer, F. The MEMIN research unit: Scaling impact cratering experiments in porous sandstones. Meteorit. Planet. Sci. 2013, 48, 8–22. [Google Scholar] [CrossRef]
- Burchell, M.J.; Johnson, E. Impact craters on small icy bodies such as icy satellites and comet nuclei. Mon. Not. R. Astron. Soc. 2005, 360, 769–781. [Google Scholar] [CrossRef] [Green Version]
- Melosh, H.J. Impact ejection, spallation, and the origin of meteorites. Icarus 1984, 59, 234–260. [Google Scholar] [CrossRef]
- Melosh, H.J. Impact Cratering: A Geologic Process; Oxford University Press: Oxford, UK, 1989. [Google Scholar]
- Ernstson, K.; Rampino, M.R.; Hiltl, M. Cratered cobbles in Triassic Buntsandstein conglomerates in northeastern Spain: An indicator of shock deformation in the vicinity of large impacts. Geology 2002, 30, 91. [Google Scholar] [CrossRef]









| Shot No. | Projectile Type | ρp (g/cm3) | mp (g) | l (cm) | d (cm) | l/d | u (km/s) | Ek (kJ) | Yaw Angle (°) | Target No. |
|---|---|---|---|---|---|---|---|---|---|---|
| 1 | TC4 | 4.44 | 39.33 | 3.666 | 1.833 | 2.00 | 1.95 | 74.78 | 4.3 | T26 |
| 2 | TC4 | 4.44 | 39.30 | 3.662 | 1.831 | 2.00 | 2.33 | 106.76 | 4.5 | T01 |
| 3 | TC4 | 4.44 | 39.37 | 3.664 | 1.830 | 2.00 | 3.15 | 195.13 | 6.7 | T13 |
| 4 | TC4 | 4.44 | 39.35 | 3.664 | 1.833 | 2.00 | 3.55 | 247.83 | 8.2 | T02 |
| 5 | TC4 | 4.44 | 39.30 | 3.664 | 1.831 | 2.00 | 3.99 | 313.07 | 0.8 | T32 |
| 6 | 30CrMnSiA | 7.81 | 39.58 | 3.053 | 1.521 | 2.01 | 1.94 | 74.43 | 24.5 | T24 |
| 7 | 30CrMnSiA | 7.81 | 39.76 | 3.047 | 1.521 | 2.00 | 2.43 | 116.77 | 3.8 | T20 |
| 8 | 30CrMnSiA | 7.81 | 39.78 | 3.047 | 1.521 | 2.00 | 3.06 | 185.17 | 0.1 | T19 |
| 9 | 30CrMnSiA | 7.81 | 39.49 | 3.049 | 1.522 | 2.00 | 3.48 | 239.48 | 0.0 | T04 |
| 10 | 30CrMnSiA | 7.81 | 39.78 | 3.047 | 1.522 | 2.00 | 3.91 | 302.32 | 13.8 | T30 |
| 11 | 93 W | 17.78 | 40.48 | 2.338 | 1.162 | 2.01 | 1.91 | 73.42 | 32.8 | T22 |
| 12 | 93 W | 17.78 | 40.46 | 2.327 | 1.161 | 2.00 | 2.51 | 126.79 | 11.9 | T35 |
| 13 | 93 W | 17.78 | 40.34 | 2.326 | 1.161 | 2.00 | 3.09 | 192.16 | 6.6 | T17 |
| 14 | 93 W | 17.78 | 40.24 | 2.323 | 1.161 | 2.00 | 3.55 | 253.63 | 3.3 | T07 |
| 15 | 93 W | 17.78 | 40.30 | 2.335 | 1.161 | 2.01 | 3.93 | 310.83 | 2.6 | T29 |
| Shot No. | ρp/ρt | H (cm) | D (cm) | V (cm3) | H/D | H/l | D/d | V/ld2 | Target No. |
|---|---|---|---|---|---|---|---|---|---|
| 1 | 1.71 | 5.89 | 26.85 | 1133 | 0.22 | 1.61 | 14.65 | 91.98 | T26 |
| 2 | 1.71 | 6.90 | 34.80 | 2288 | 0.20 | 1.88 | 19.01 | 186.36 | T01 |
| 3 | 1.71 | 7.25 | 50.51 | 5195 | 0.14 | 1.98 | 27.60 | 423.38 | T13 |
| 4 | 1.71 | 8.03 | 48.94 | 5838 | 0.16 | 2.19 | 26.70 | 474.22 | T02 |
| 5 | 1.71 | 9.33 | 54.50 | 9033 | 0.17 | 2.55 | 29.77 | 735.36 | T32 |
| 6 | 3.02 | 6.13 | 31.12 | 1510 | 0.20 | 2.01 | 20.46 | 213.79 | T24 |
| 7 | 3.02 | 8.41 | 37.12 | 2694 | 0.23 | 2.76 | 24.40 | 382.18 | T20 |
| 8 | 3.02 | 8.76 | 49.49 | 5530 | 0.18 | 2.87 | 32.54 | 784.50 | T19 |
| 9 | 3.02 | 9.76 | 49.42 | 6576 | 0.20 | 3.20 | 32.47 | 931.05 | T04 |
| 10 | 3.02 | 9.74 | 61.07 | 10,130 | 0.16 | 3.20 | 40.12 | 1435.18 | T30 |
| 11 | 6.86 | 7.77 | 31.90 | 1738 | 0.24 | 3.32 | 27.45 | 550.55 | T22 |
| 12 | 6.86 | 9.94 | 51.43 | 6012 | 0.19 | 4.27 | 44.30 | 1916.72 | T35 |
| 13 | 6.86 | 10.24 | 52.76 | 7337 | 0.19 | 4.40 | 45.44 | 2340.15 | T17 |
| 14 | 6.86 | 10.38 | 51.93 | 7399 | 0.20 | 4.47 | 44.73 | 2362.98 | T07 |
| 15 | 6.86 | 11.89 | 61.83 | 12,958 | 0.19 | 5.09 | 53.26 | 4117.05 | T29 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, X.; Liu, J.; Wu, B.; Kong, D.; Huang, J.; Xu, X.; Bao, X. Cratering for Impact of Hypervelocity Projectiles into Granite Targets within a Velocity Range of 1.91–3.99 km/s: Experiments and Analysis. Appl. Sci. 2020, 10, 1393. https://doi.org/10.3390/app10041393
Wang X, Liu J, Wu B, Kong D, Huang J, Xu X, Bao X. Cratering for Impact of Hypervelocity Projectiles into Granite Targets within a Velocity Range of 1.91–3.99 km/s: Experiments and Analysis. Applied Sciences. 2020; 10(4):1393. https://doi.org/10.3390/app10041393
Chicago/Turabian StyleWang, Xiaofeng, Jingbo Liu, Biao Wu, Defeng Kong, Jiarong Huang, Xiangyun Xu, and Xin Bao. 2020. "Cratering for Impact of Hypervelocity Projectiles into Granite Targets within a Velocity Range of 1.91–3.99 km/s: Experiments and Analysis" Applied Sciences 10, no. 4: 1393. https://doi.org/10.3390/app10041393
APA StyleWang, X., Liu, J., Wu, B., Kong, D., Huang, J., Xu, X., & Bao, X. (2020). Cratering for Impact of Hypervelocity Projectiles into Granite Targets within a Velocity Range of 1.91–3.99 km/s: Experiments and Analysis. Applied Sciences, 10(4), 1393. https://doi.org/10.3390/app10041393





