A New Computational Model of Step Gauge Calibration Based on the Synthesis Technology of Multi-Path Laser Interferometers
Abstract
Featured Application
Abstract
1. Introduction
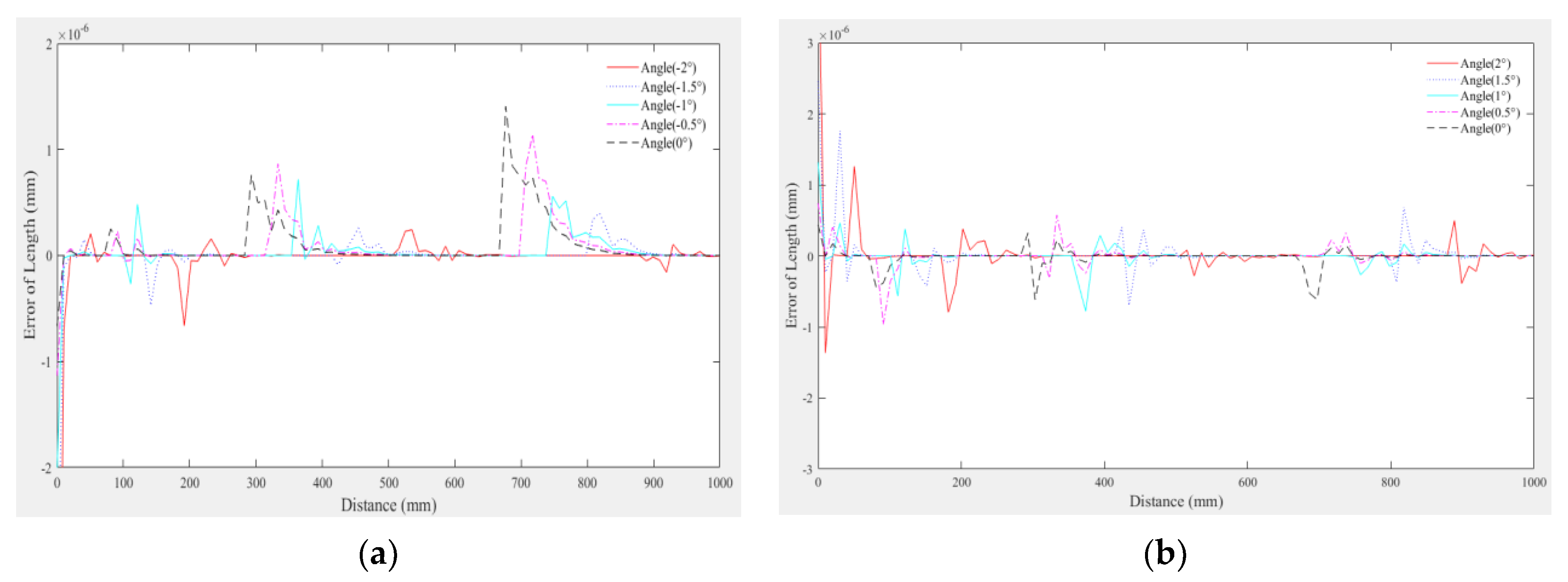
2. The Mathematical Modeling and Simulation
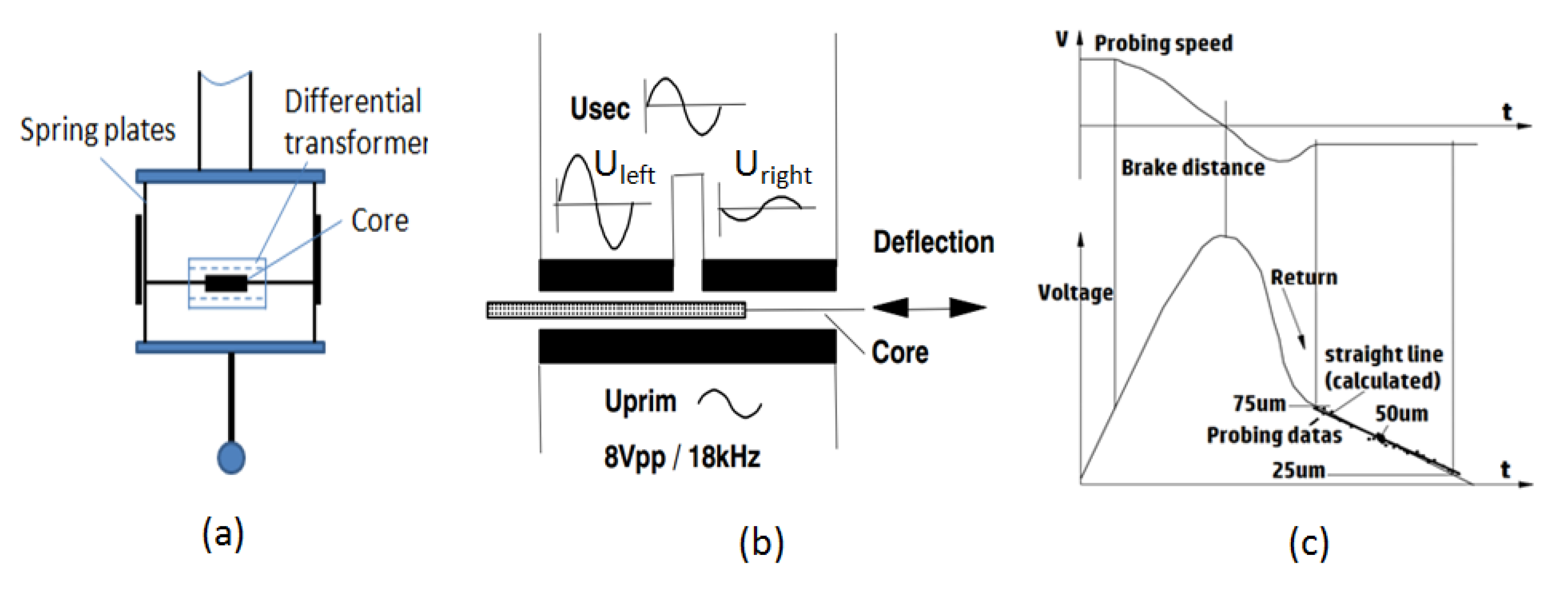
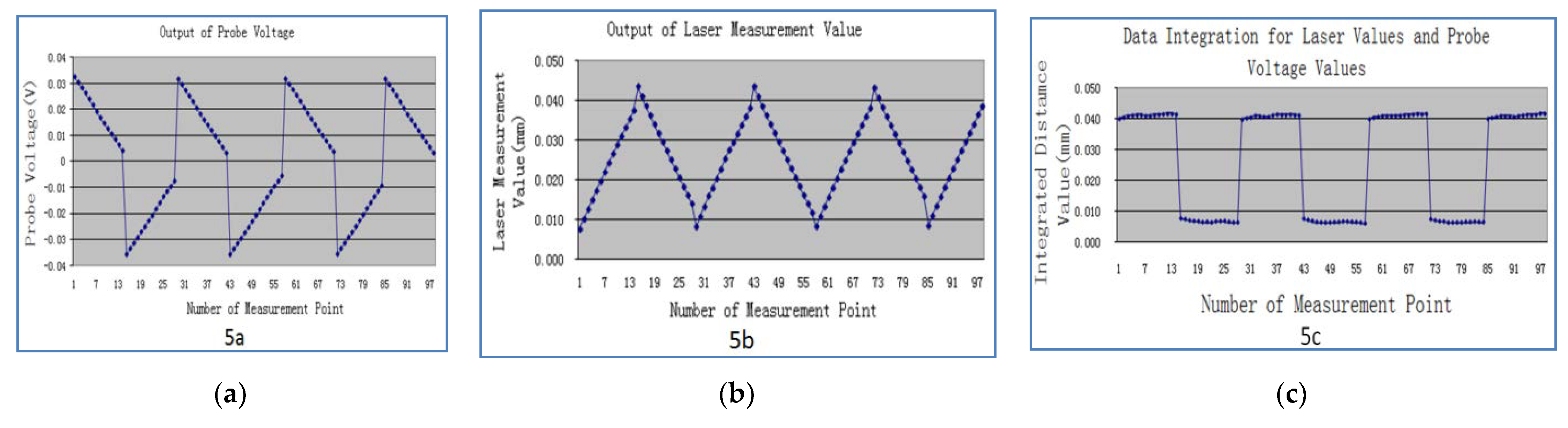
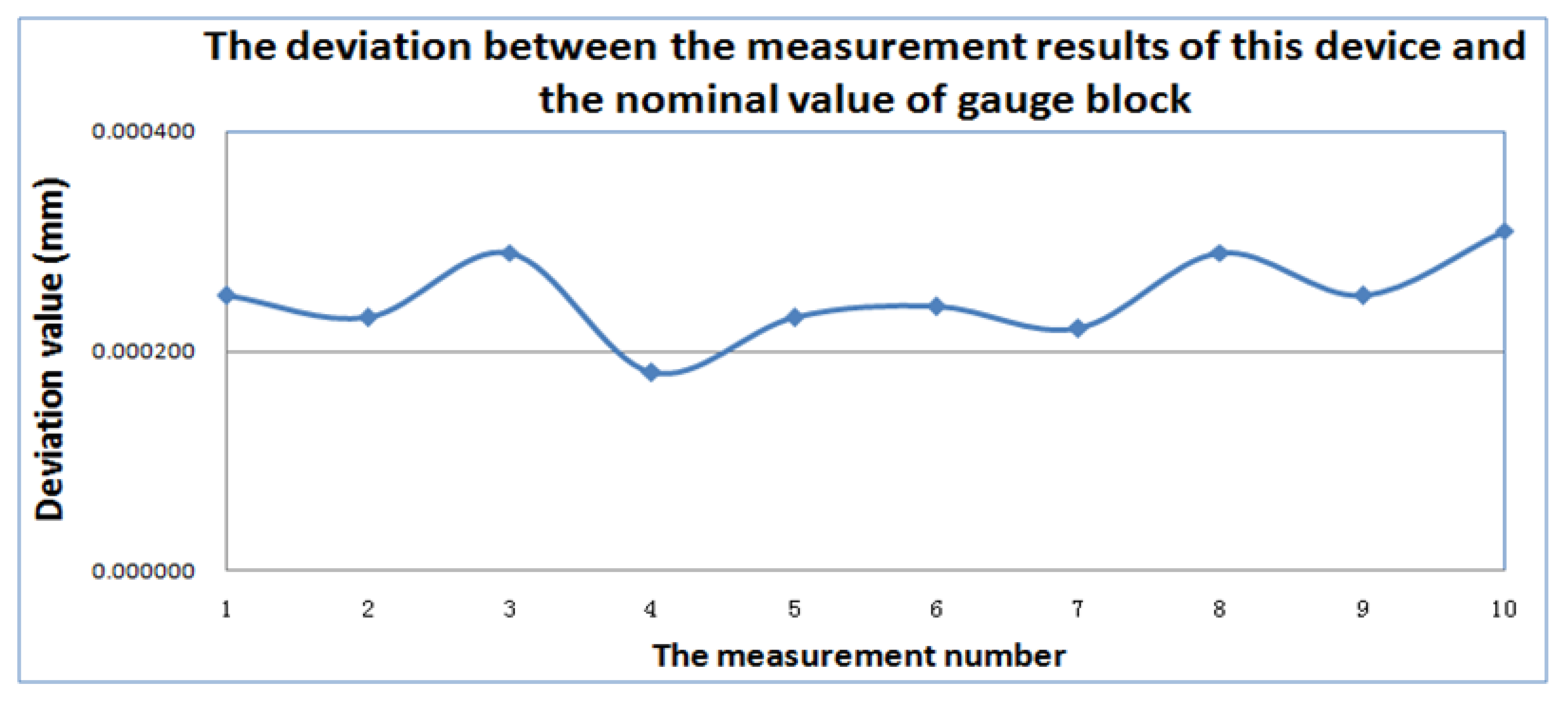
3. Device and Experiment
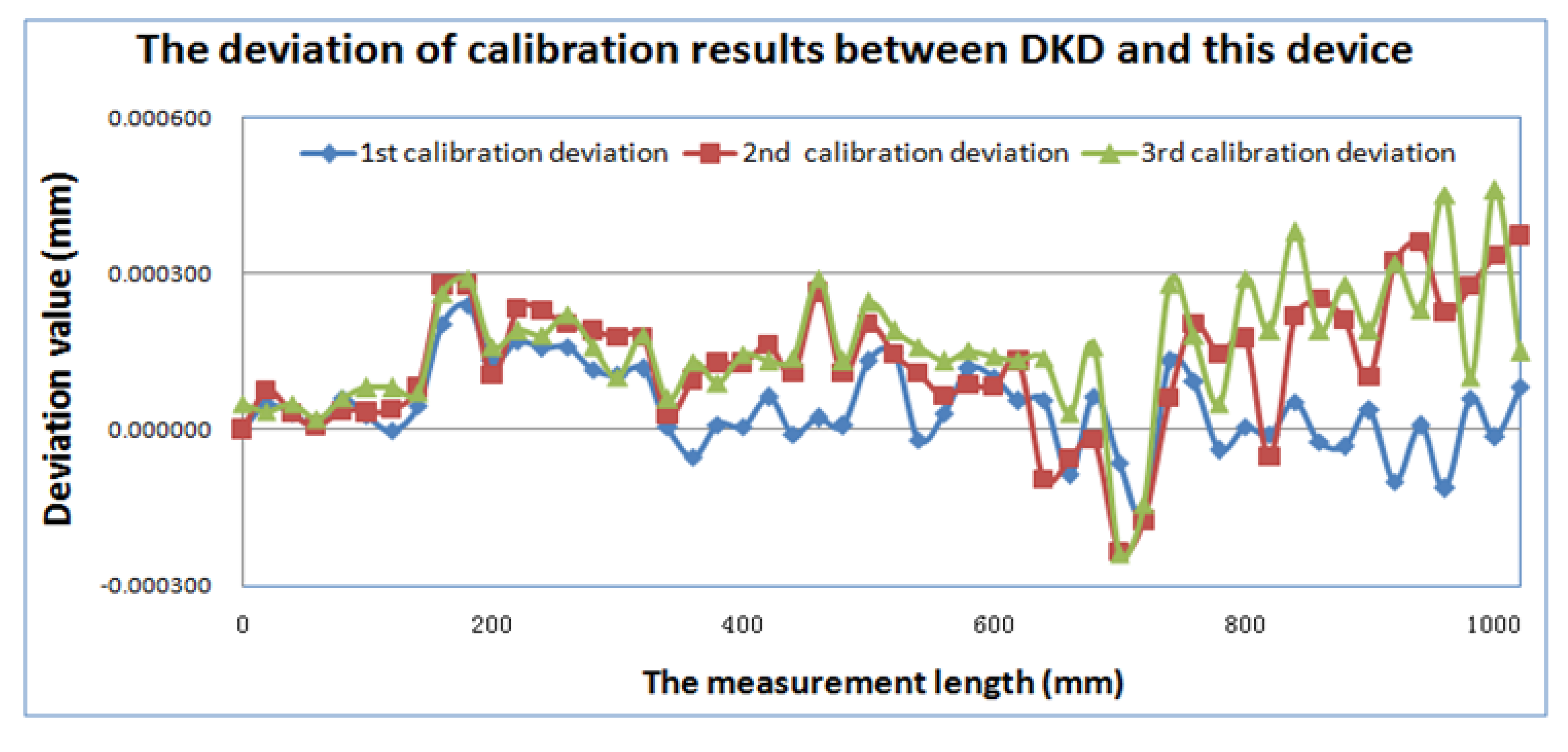
4. Measurement Uncertainty
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Jusko, O.; Brown, N.; Thalmann, R.; Reyes, E.A.; Phillips, S.; Eom, C.I.; Shaoxi, S.; Takatsuji, T.; Prieto, E. CCL-K5: CMM 1D: Step gauge and ball bars: Final report. Metrologia 2006, 43, 04006. [Google Scholar] [CrossRef]
- Eom, T.; Sitian, G.; Wong, S.Y.; Chaudhary, K.P.; Drijarkara, A.P.; Howick, E.; Tonmeanwai, A.; Pan, S.-P.; Takatsuji, T.; Cox, P.; et al. Final report on APMP Regional Comparison APMP.L-K5: Calibration of step gauge. Metrologia 2012, 49, 04007. [Google Scholar] [CrossRef]
- Prieto, E.; Brown, N.; Lassila, A.; Lewis, A.; Matus, M.; Vailleau, G.; De Chiffre, L.; Kotte, G.W.J.L.; Frennberg, M.; McQuoid, H.; et al. Final report on inter-RMO Key Comparison EUROMET.L-K5.2004: Calibration of a step gauge. Metrologia 2012, 49, 04008. [Google Scholar] [CrossRef]
- Abe, M.; Drijarkara, A.P.; Babu, V. Bi-lateral comparison APMP.L-K5.2006.1—calibration of step gauge. Metrologia 2017, 54, 4006. [Google Scholar] [CrossRef]
- Hemming, B.; Esala, V.-P.; Laukkanen, P.; Rantanen, A.; Viitala, R.; Widmaier, T.; Kuosmanen, P.; Lassila, A. Interferometric step gauge for CMM verification. Meas. Sci. Technol. 2018, 29, 074012. [Google Scholar] [CrossRef]
- A Kruger, O. High-accuracy interferometric measurements of flatness and parallelism of a step gauge. Metrologia 2001, 38, 237–240. [Google Scholar] [CrossRef][Green Version]
- Osawa, S.; Takatsuji, T.; Noguchi, H.; Kurosawa, T. Development of a ball step-gauge and an interferometric stepper used for ball-plate calibration. Precis. Eng. 2002, 26, 214–221. [Google Scholar] [CrossRef]
- Osawa, S.; Takatsuji, T.; Kurosawa, T. STEP-gauge calibration using an interferometric coordinate measuring machine and the uncertainty. In Proceedings of the XVII imeko World Congress, Dubrovnik, Croatia, 22–27 June 2003. [Google Scholar]
- Stoup, J.; Faust, B. Measuring Step Gauges Using the NIST M48 CMM. NCSLI Meas. 2011, 6, 66–73. [Google Scholar] [CrossRef]
- Byman, V.; Jaakkola, T.; Palosuo, I.; Lassila, A. High accuracy step gauge interferometer. Meas. Sci. Technol. 2018, 29, 054003. [Google Scholar] [CrossRef]
- Weichert, C.; Bütefisch, S.; Köning, R.; Flügge, J. Integration of a step gauge measurement capability at the PTB Nanometer Comparator—Concept and preliminary tests. In Proceedings of the Conference “MacroScale 2017—Recent Developments in Traceable Dimensional Measurements”, VTT MIKES Espoo, Finnland, 17–19 October 2017. [Google Scholar] [CrossRef]
- Ren, G.; Qu, X.; Ding, S. A Real-Time Measurement Method of Air Refractive Index Based on Special Material Etalon. Appl. Sci. 2018, 8, 2325. [Google Scholar] [CrossRef]
- Guo-Ying, R.; Wei-Nong, W.; Zi-Jun, W. Uncertainty Analysis for Measurement of Step Gauges Based on Coordinate Measuring Machine. Acta Metrol. Sin. 2008, 29 (Suppl. S1), 151–153. [Google Scholar]
- Bönsch, G.; Potulski, E. Measurement of the refractive index of air and comparison with modified Edlen’s formula. Metrologia 1998, 35, 133–139. [Google Scholar] [CrossRef]
- Garcia-Murillo, M.A.; Sanchez-Alonso, R.E.; Gallardo-Alvarado, J. Kinematics and Dynamics of a Translational Parallel Robot Based on Planar Mechanisms. Machines 2016, 4, 22. [Google Scholar] [CrossRef]
- Zhang, X.; Dong, Y.; Zhang, L. kinematics modeling and simulation of a five-bar Cabot by using closed loop vector method. Control Theory Appl. 2006, 25. [Google Scholar] [CrossRef]
- Buschhaus, A.; Blank, A.; Franke, J. Vector based closed-loop control methodology for industrial robots. In Proceedings of the 2015 International Conference on Advanced Robotics (ICAR), Istanbul, Turkey, 27–31 July 2015; pp. 452–458. [Google Scholar]
- BIPM; IEC; IFCC; ISO; IUPAC; IUPAP; OIML. Evaluation of measurement data — Guide to the expression of uncertainty in measurement. Int. Organ. Stand. Geneva ISBN 2008, 50, 134. [Google Scholar]


| Measurement Number | The Measured Laser Value(mm) | |
|---|---|---|
| left side | right side | |
| 1 | 713.81023 | 678.82778 |
| 2 | 713.81020 | 678.82771 |
| 3 | 713.81024 | 678.82777 |
| 4 | 713.81029 | 678.82778 |
| 5 | 713.81028 | 678.82775 |
| 6 | 713.81026 | 678.82778 |
| 7 | 713.81031 | 678.82779 |
| 8 | 713.81024 | 678.82773 |
| 9 | 713.81023 | 678.82773 |
| 10 | 713.81030 | 678.82777 |
| Std. Deviation | 0.000034 | 0.000026 |
| Average Value | 713.81026 | 678.82776 |
| No. | Sources of Nncertainty | Magnitude | Type | Uncertainty |
|---|---|---|---|---|
| 1 | Probing repeatability | 0.034 μm | A | 0.034 μm |
| 2 | Calibration of probe | 0.030 μm | B | 0.030 μm |
| 3 | Uncertainty of standard sphere | 0.030 μm | B | 0.030 μm |
| 4 | Temperature measurement | 5 mK | B | 0.143 Lμm |
| 5 | Temperature distribution | 10 mK | A | 0.286 Lμm |
| 6 | Wavelength (frequency) | 1.5 MHz | B | 0.002 Lμm |
| 7 | Wavelength (temperature) | 95 mK | A | 0.053 Lμm |
| 8 | Wavelength (air pressure) | 53 Pa | A | 0.081 Lμm |
| 9 | Wavelength (humidity) | 0.64 % | A | 0.008 Lμm |
| 10 | Wavelength (CO2) | 50 ppm | B | 0.004 Lμm |
| 11 | Thermal expansion coefficient | 1.0 × 10−6 /K | B | 0.365 Lμm |
| 12 | Error of cosine (step gauge) | 0.1 mm/ 1020 mm | B | 0.005 Lμm |
| 13 | Error of cosine (laser) | 0.1 mm/1020 mm | B | 0.005 Lμm |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ren, G.; Qu, X.; Chen, X. A New Computational Model of Step Gauge Calibration Based on the Synthesis Technology of Multi-Path Laser Interferometers. Appl. Sci. 2020, 10, 2089. https://doi.org/10.3390/app10062089
Ren G, Qu X, Chen X. A New Computational Model of Step Gauge Calibration Based on the Synthesis Technology of Multi-Path Laser Interferometers. Applied Sciences. 2020; 10(6):2089. https://doi.org/10.3390/app10062089
Chicago/Turabian StyleRen, Guoying, Xinghua Qu, and Xiangjun Chen. 2020. "A New Computational Model of Step Gauge Calibration Based on the Synthesis Technology of Multi-Path Laser Interferometers" Applied Sciences 10, no. 6: 2089. https://doi.org/10.3390/app10062089
APA StyleRen, G., Qu, X., & Chen, X. (2020). A New Computational Model of Step Gauge Calibration Based on the Synthesis Technology of Multi-Path Laser Interferometers. Applied Sciences, 10(6), 2089. https://doi.org/10.3390/app10062089





