Mapping Based Quality Metrics for Mesh Deformation Algorithms Using Radial Basis Functions
Abstract
:1. Introduction
2. RBFs Method Based on Generalized TPS
3. Mapping-Based Metrics
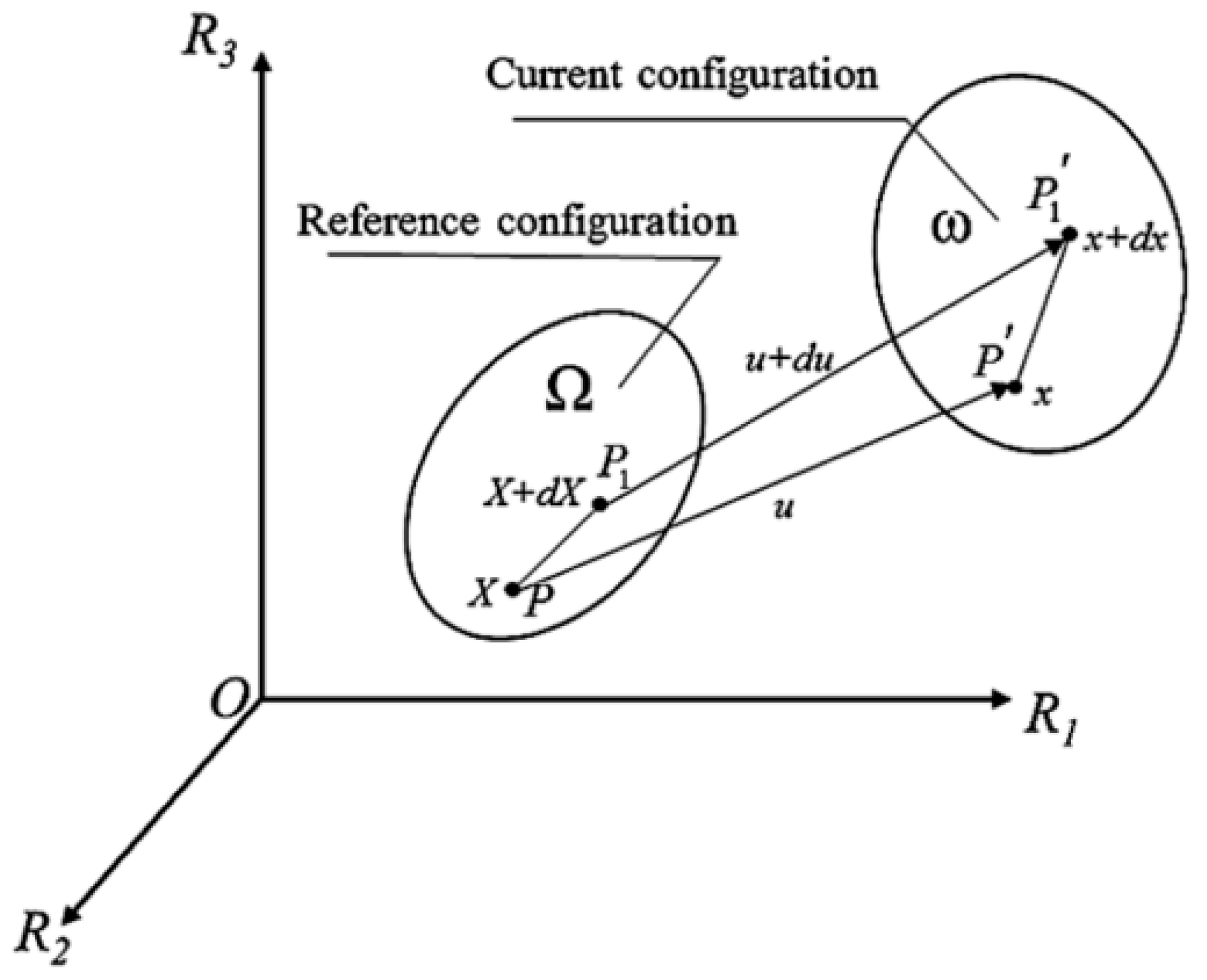
3.1. Mapping Relationships
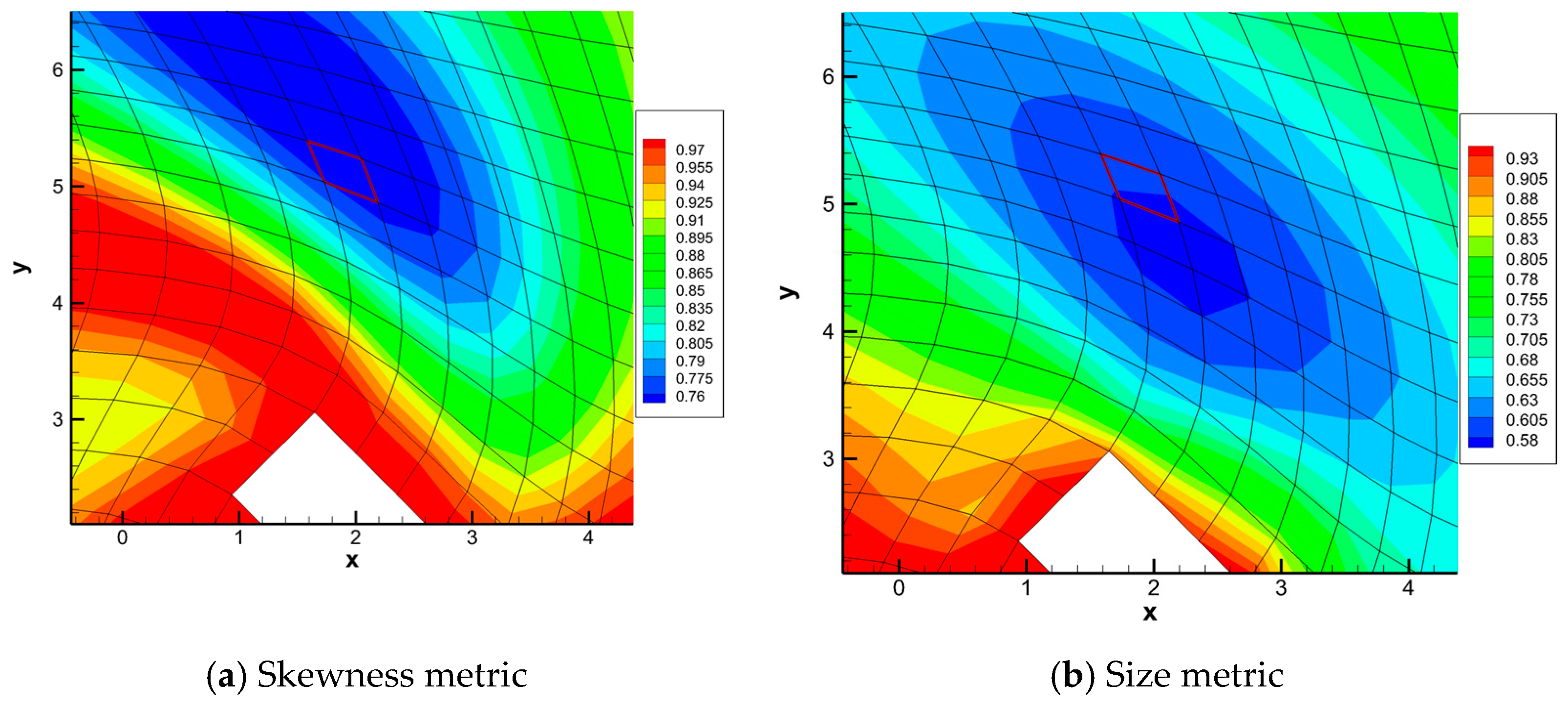
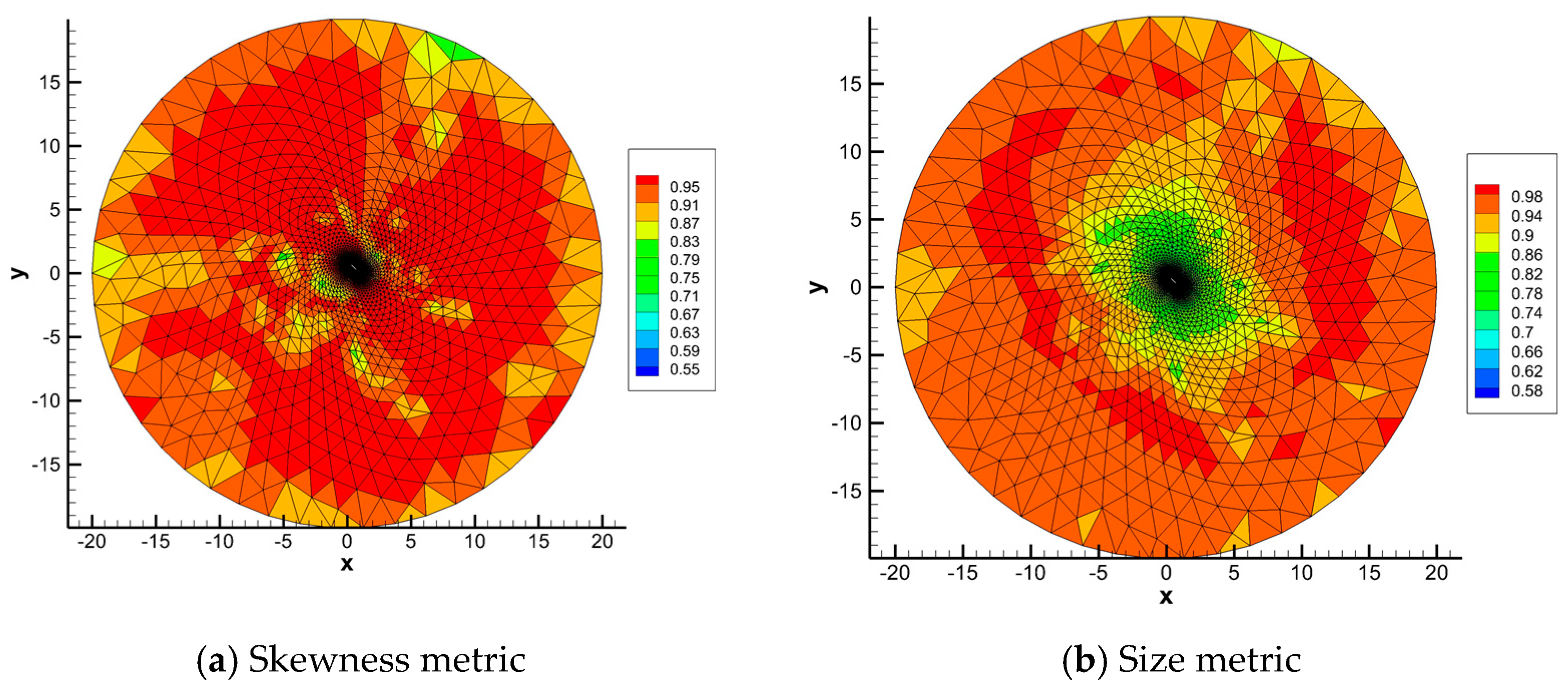
3.2. Size Metrics
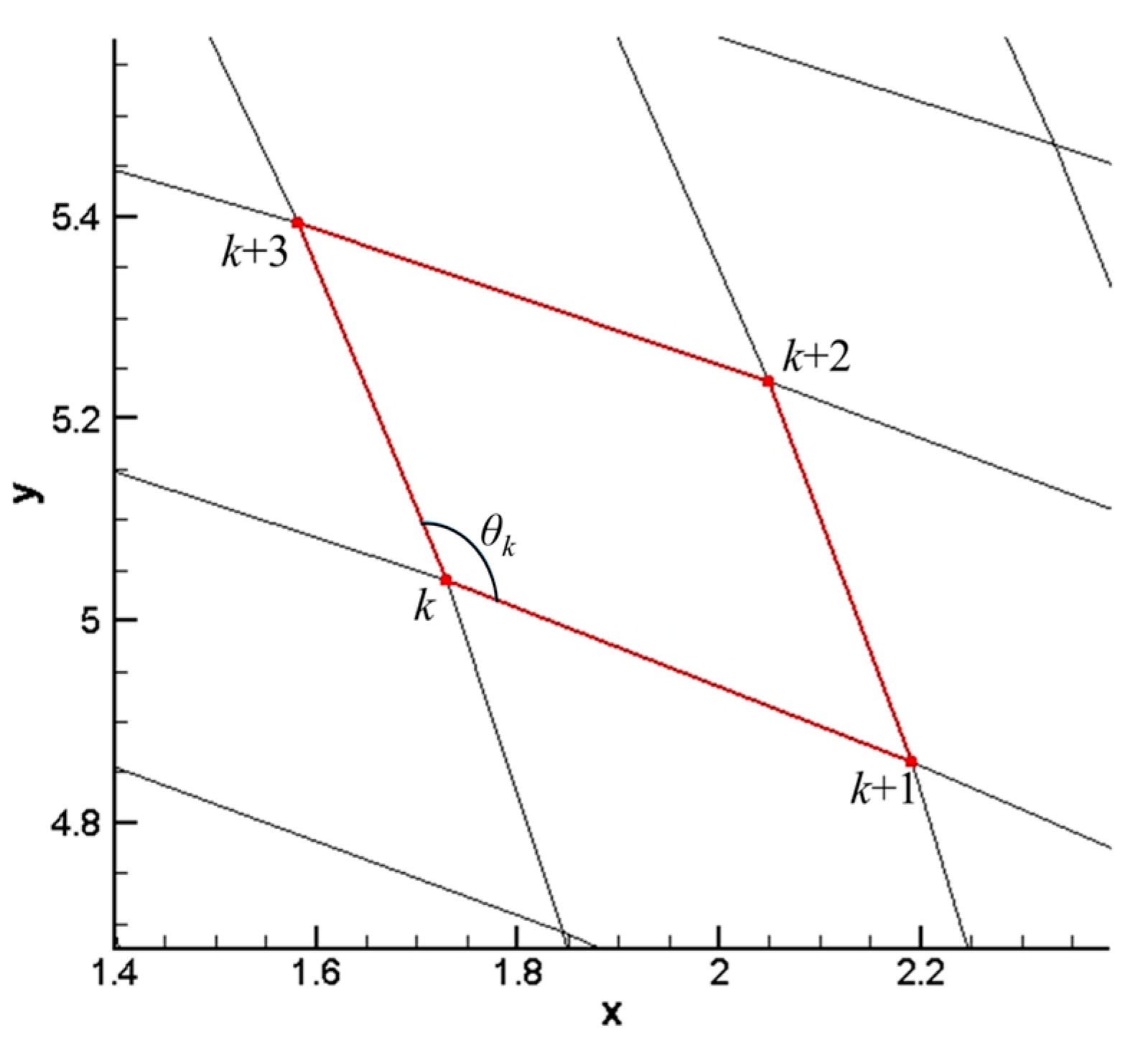
3.3. Skewness Metrics
4. Results and Discussions
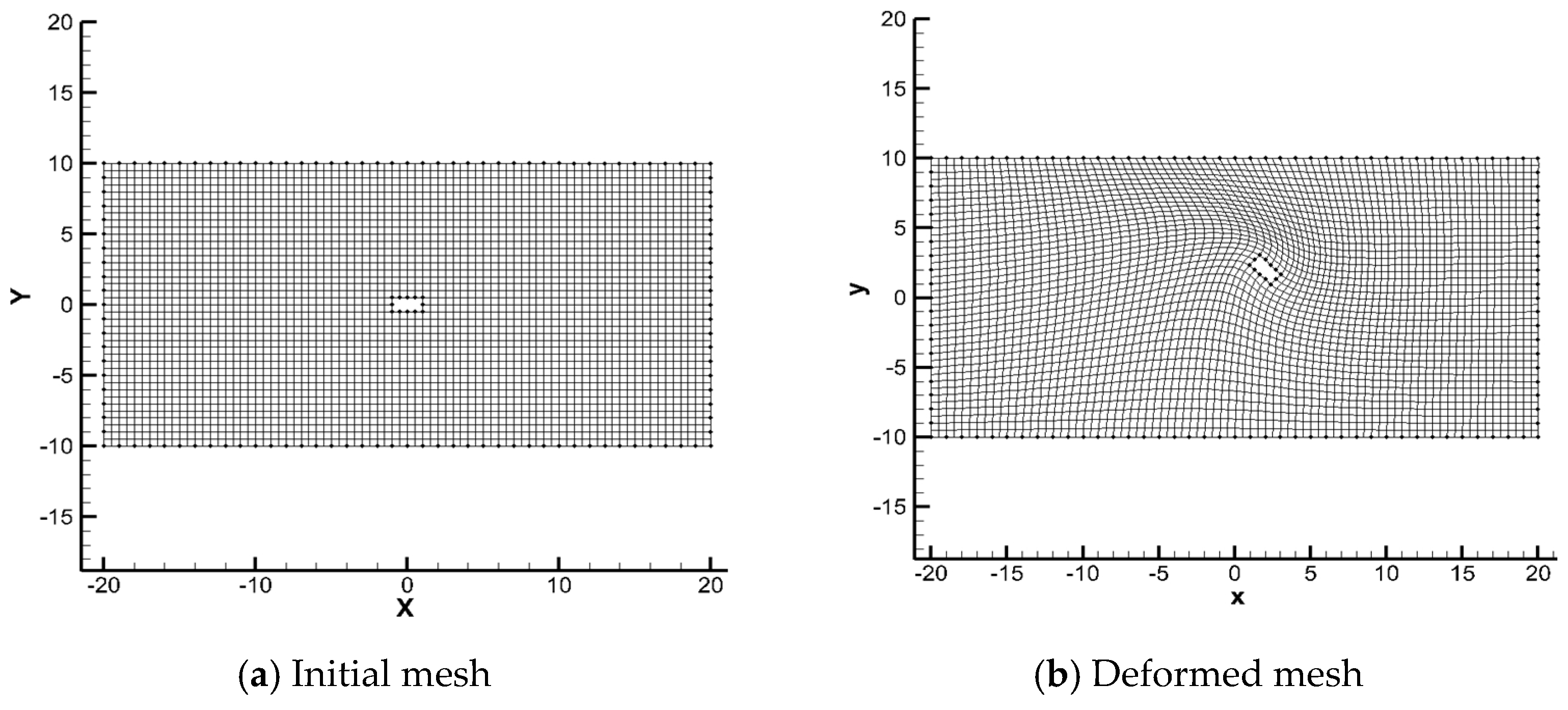
4.1. Test Case 1: Translation and Rotation of Structured Square Mesh
4.2. Test Case 2: Large Rotation of NACA0012 Unstructured Mesh
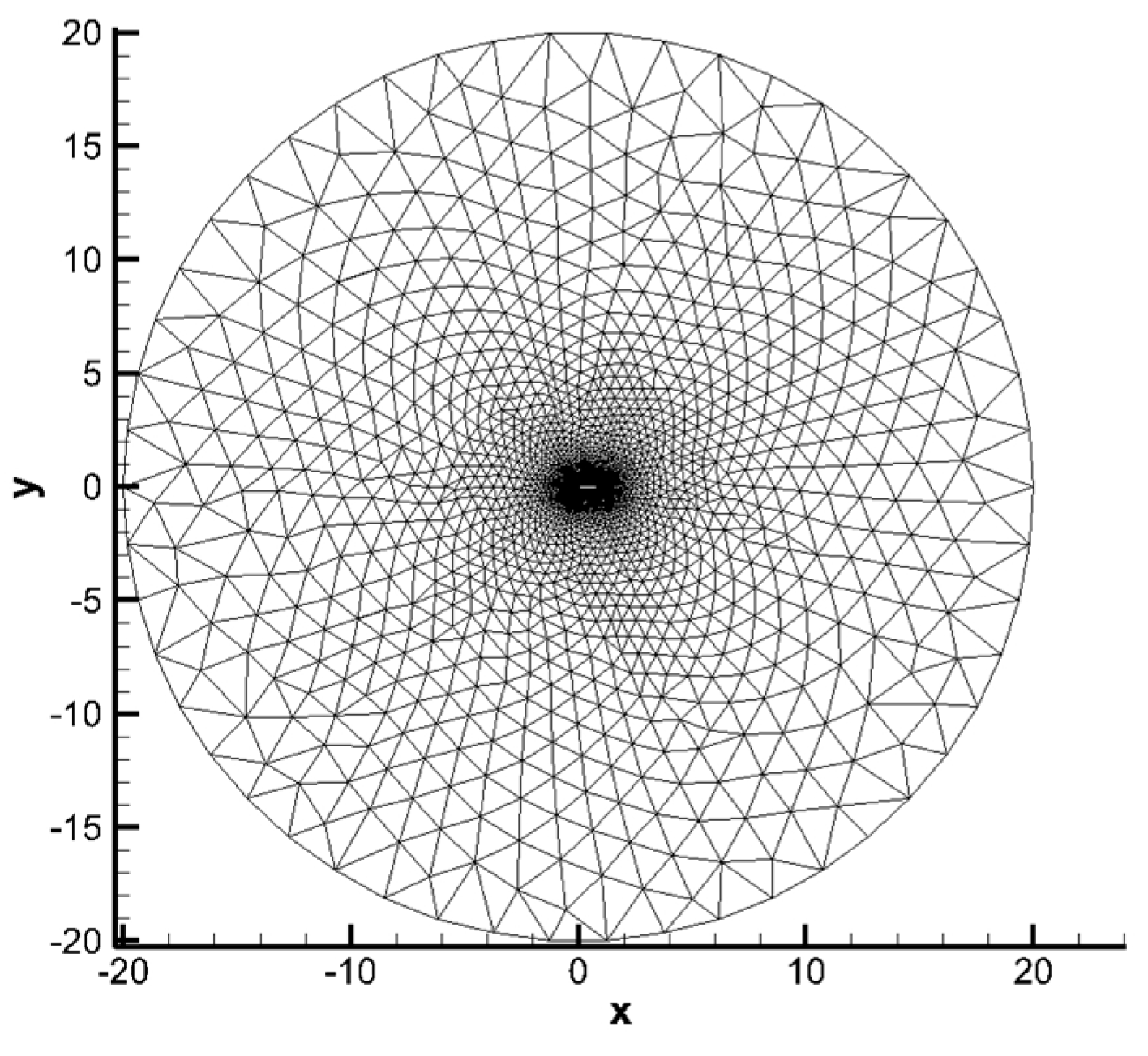
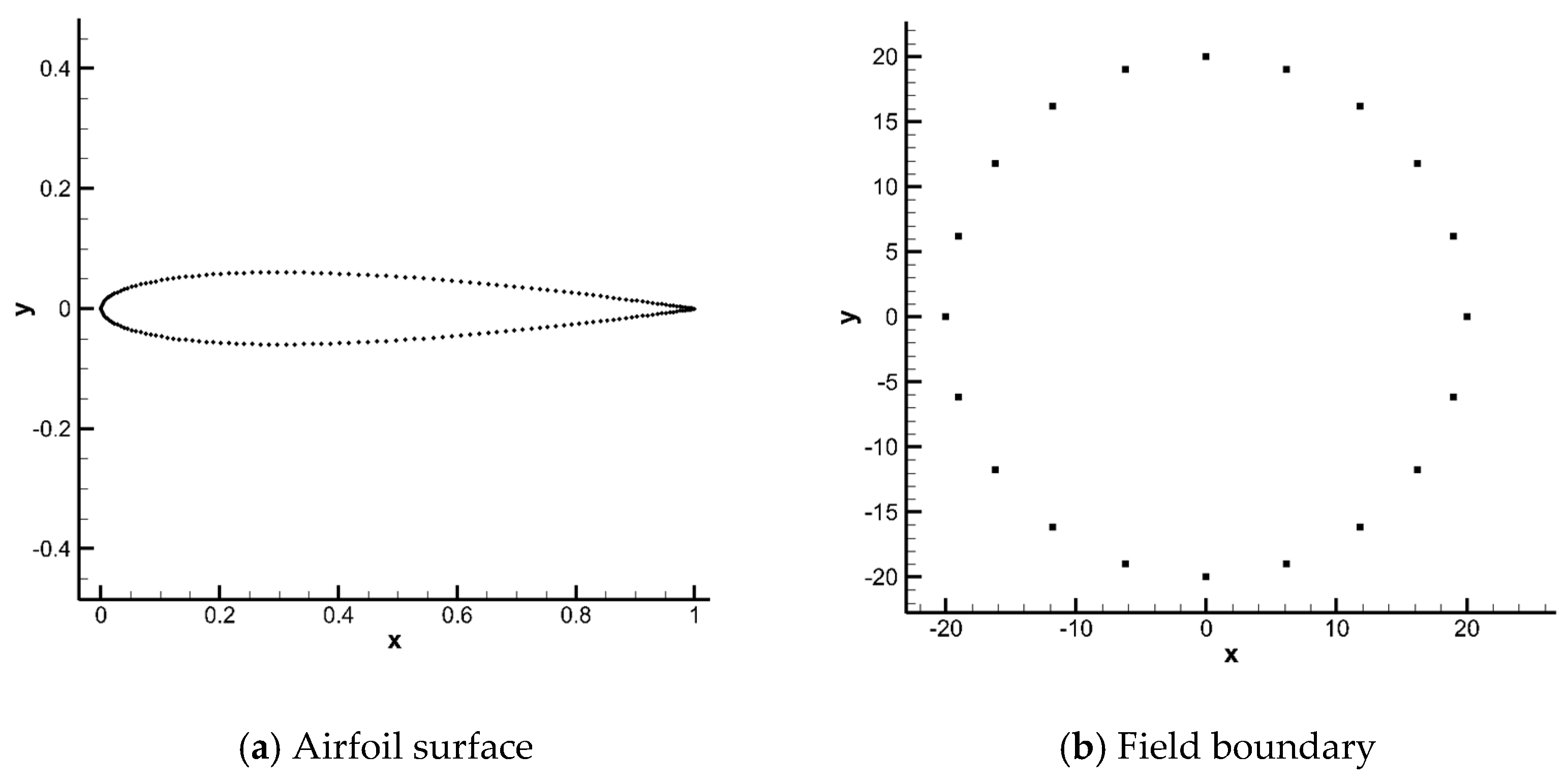
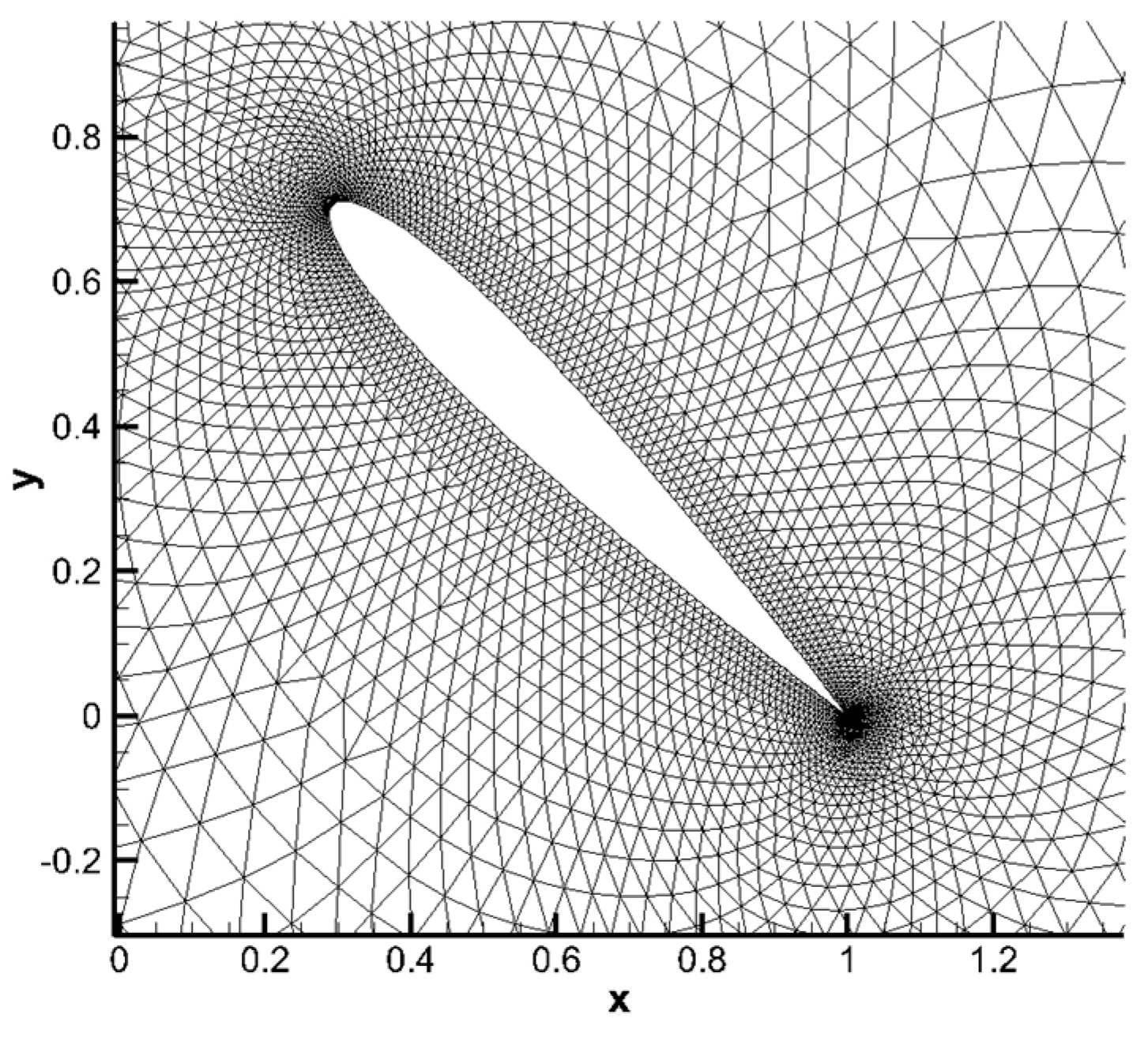
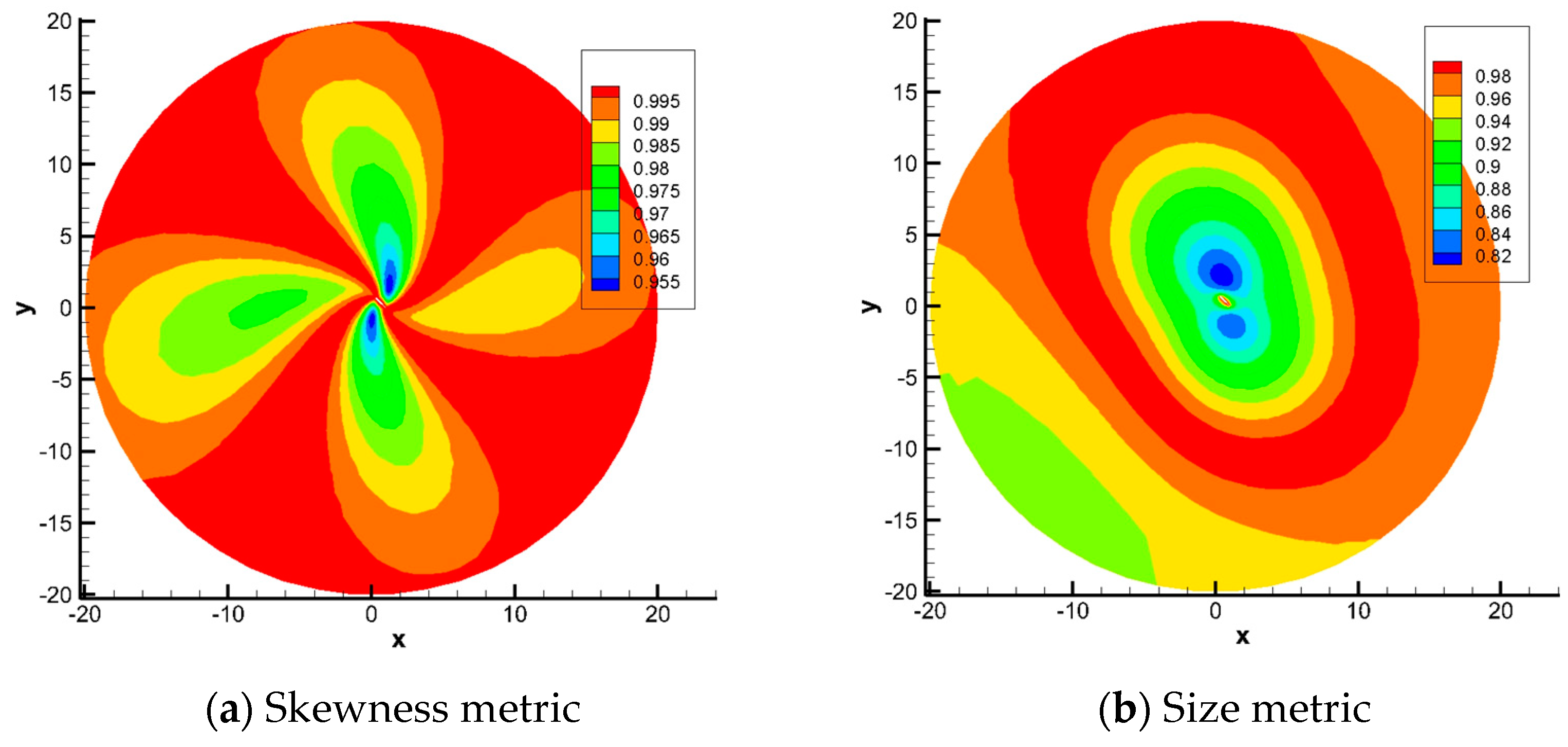
4.3. Test Case 3: Transform of Airfoil with a Viscous Layer
5. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Jakobsson, S.; Amoignon, O. Mesh deformation using radial basis functions for gradient-based aerodynamic shape optimization. Comput. Fluids 2007, 36, 1119–1136. [Google Scholar] [CrossRef]
- Bano, T.; Hegner, F.; Heinrich, M.; Schwarze, R. Investigation of fluid-structure interaction induced bending for elastic flaps in a cross flow. Appl. Sci. 2020, 10, 6177. [Google Scholar] [CrossRef]
- An, C.; Yang, C.; Xie, C.C.; Yang, L. Flutter and Gust response analysis of a wing model including geometric nonlinearities based on a modified structural ROM. Chin. J. Aeronaut. 2020, 33, 48–63. [Google Scholar] [CrossRef]
- Borazjani, I.; Sotiropoulos, F. Numerical investigation of the hydrodynamics of anguilliform swimming in the transitional and inertial flow regimes. J. Exp. Biol. 2009, 212, 576–592. [Google Scholar] [CrossRef] [Green Version]
- Rinaudo, A.; Raffa, G.M.; Scardulla, F.; Pilato, M.; Scardulla, C.; Pasta, S. Biomechanical implications of excessive endograft protrusion into the aortic arch after thoracic endovascular repair. Comput. Biol. Med. 2015, 66, 235–241. [Google Scholar] [CrossRef] [PubMed]
- Zhao, R.; Li, C.; Guo, X.W.; Fan, S.J.; Wang, Y.; Yang, C.Q. A block iteration with parallelization method for the greedy selection in radial basis functions based mesh deformation. Appl. Sci. 2019, 9, 1141. [Google Scholar] [CrossRef] [Green Version]
- Cella, U.; Biancolini, M.E. Aeroelastic analysis of aircraft wind-tunnel model coupling structural and fluid dynamic codes. J. Aircr. 2012, 49, 407–414. [Google Scholar] [CrossRef]
- Pan, J.Y.; Liu, F. Wing flutter prediction by a small-disturbance Euler method on body-fitted curvilinear grids. AIAA J. 2019, 57, 4873–4884. [Google Scholar] [CrossRef]
- Li, C.; Xu, X.H.; Wang, J.W.; Xu, L.Y.; Ye, S.; Yang, X.J. A parallel multiselection greedy method for the radial basis function-based mesh deformation. Int. J. Numer. Methods Eng. 2018, 113, 1561–1588. [Google Scholar] [CrossRef]
- Banita, J.T. Unsteady Euler airfoil solutions using unstructured dynamic meshes. AIAA J. 1990, 28, 1381–1388. [Google Scholar]
- Banita, J.T. Unsteady Euler algorithm with unstructured dynamic mesh for complex-aircraft aerodynamic analysis. AIAA J. 1991, 29, 327–333. [Google Scholar]
- Fang, H.; Hu, Y.K.; Yu, C.H.; Tie, M.; Liu, J.; Gong, C.Y. An efficient radial basis functions mesh deformation with greedy algorithm based on recurrence choleskey decomposition and parallel computing. J. Comput. Phys. 2019, 377, 183–199. [Google Scholar]
- Pan, Y.; Yuan, Q.; Huang, G.G.; Gu, J.W.; Li, P.; Zhu, G.Y. Numerical investigations on the blade tip clearance excitation forces in an unshrouded turbine. Appl. Sci. 2020, 10, 1532. [Google Scholar] [CrossRef] [Green Version]
- Bottasso, C.L.; Detomi, D.; Serra, R. The ball-vortex method: A new simple spring analogy method for unstructured dynamic meshes. Finite Elem. Anal. Des. 2005, 41, 1118–1139. [Google Scholar]
- Farhat, C.; Degand, C.; Koobus, B. Torsional springs for two-dimensional dynamic unstructured fluid meshes. Comput. Methods Appl. Mech. Eng. 1998, 163, 231–245. [Google Scholar] [CrossRef]
- Blom, F.J. Considerations on the spring analogy. Int. J. Numer. Method Fluids 2000, 32, 647–668. [Google Scholar] [CrossRef]
- Zeng, D.H.; Either, C.R. A semi-torsional spring analogy model for updating unstructured meshes in 3D moving domain. Finite Elem. Anal. Des. 2005, 41, 1118–1139. [Google Scholar] [CrossRef]
- Witteveen, J. Explicit and robust inverse distance weighting mesh deformation for CFD. In Proceedings of the 48th AIAA Aerospace Science Meeting including the New Horizons Forum and Aerospace Exposition, Orlando, FL, USA, 4–7 January 2010. [Google Scholar]
- Gaitonde, A.L.; Fiddes, S.P.A. A moving mesh system for the calculation of unsteady flows. In Proceedings of the 31st Aerospace Science Meeting & Exhibit, Reno, NV, USA, 11–14 January 1993. [Google Scholar]
- Liu, X.; Qin, N.; Xia, H. Fast dynamic grid deformation based on Delaunay graph mapping. J. Comput. Phys. 2006, 211, 405–423. [Google Scholar] [CrossRef]
- Selim, M.M.; Koomullil, R.P.; Shehata, A.S. Incremental approach for radial basis functions mesh deformation using data reduction. J. Comput. Phys. 2016, 321, 997–1025. [Google Scholar]
- De Boer, A.; van der Schoot, M.S.; Bijl, H. Mesh deformation based on radial basis function interpolation. Comput. Struct. 2007, 85, 784–795. [Google Scholar] [CrossRef]
- Beckert, A.; Wendland, H. Multivariate interpolation for fluid-structure-interaction problems using radial basis functions. Aerosp. Sci. Technol. 2001, 5, 125–134. [Google Scholar] [CrossRef]
- Sheng, C.; Allen, C.B. Efficient mesh deformation using redial basis functions on unstructured meshes. AIAA J. 2012, 51, 707–720. [Google Scholar] [CrossRef]
- Bos, F.M.; van Oudheusden, B.W. Radial basis function based mesh deformation applied to simulation of flow around flapping wings. Comput. Fluids 2013, 79, 167–177. [Google Scholar] [CrossRef]
- Rendall, T.; Allen, C. Efficient mesh motion using radial basis functions with data reduction algorithm. J. Comput. Phys. 2009, 228, 6231–6249. [Google Scholar] [CrossRef]
- Wang, G.; Mian, H.H.; Ye, Z.Y.; Lee, J.D. Improved point selection method for hybrid-unstructured mesh deformation using radial basis function. AIAA J. 2015, 53, 1016–1025. [Google Scholar] [CrossRef]
- Michler, A.K. Aircraft control surface deflection using RBF-based mesh deformation. Int. J. Numer. Method Eng. 2011, 88, 986–1007. [Google Scholar] [CrossRef]
- Fang, H.; Gong, C.; Yu, C.; Min, C.; Zhang, X.; Liu, J.; Xiao, L. Efficient mesh deformation based on Cartesian background mesh. Comput. Math. Appl. 2017, 73, 71–86. [Google Scholar] [CrossRef]
- Xie, L.; Liu, H. Efficient mesh motion using radial basis functions with volume grid points reduction algorithm. J. Comput. Phys. 2017, 228, 6231–6249. [Google Scholar] [CrossRef]
- Knupp, P.M. Algebraic mesh quality metrics for unstructured initial meshes. Finite Elem. Anal. Des. 2003, 39, 217–241. [Google Scholar] [CrossRef]
- Goldberg, U. Pointwise turbulence modeling for engineering applications. In Proceedings of the International CFD Workshop on Supersonic Transport Design, Tokyo, Japan, 16–17 March 1998. [Google Scholar]
- Harder, R.L.; Desmarais, R.N. Interpolation using surface splines. J. Aircr. 1972, 9, 189–191. [Google Scholar] [CrossRef]
- Liu, I.S. Continuum Mechanics; Springer: Berlin/Heidelberg, Germany, 2002. [Google Scholar]
- Zou, S.; Yuan, X.F.; Yang, X.; Yi, W.; Xu, X. An integrated lattice boltzmann and finite volume method for the simulation of viscoelastic fluid flows. J. Non-Newton. Fluid Mech. 2014, 211, 99–113. [Google Scholar] [CrossRef]








| No. | Name | |
|---|---|---|
| 1 | CP | |
| 2 | CP | |
| 3 | CP | |
| 4 | CP | |
| 5 | CTPS |
| No. | Name | |
|---|---|---|
| 1 | Thin plate spline (TPS) | |
| 2 | Multi-quadric bi-harmonics (MQB) | |
| 3 | Inverse multi-quadric bi-harmonics (IMQB) | |
| 4 | Quadric bi-harmonics (QB) | |
| 5 | Gaussian |
| Node | Coordinate X | Coordinate Y | Angle by Metric/Deg | Angle by Geometry/Deg | Error |
|---|---|---|---|---|---|
| 1.73 | 5.04 | 133.05 | 133.67 | 0.46% | |
| 2.19 | 4.86 | 48.73 | 48.14 | 1.23% | |
| 2.05 | 5.24 | 130.04 | 129.33 | 0.55% | |
| 1.58 | 5.39 | 48.33 | 48.87 | 1.10% |
| Mapping-based metrics | 0.97603 | 0.74225 | 0.88579 | 0.57495 |
| Mesh-based metrics | 0.97583 | 0.74057 | 0.88567 | 0.57412 |
| Error | 0.02% | 0.22% | 0.01% | 0.14% |
| Node | Coordinate X | Coordinate Y | Angle by Metric/Deg | Angle by Geometry/Deg | Error |
|---|---|---|---|---|---|
| 1.30 | 1.49 | 67.96 | 68.01 | 0.07% | |
| 1.15 | 1.57 | 45.56 | 46.00 | 0.09% | |
| 1.19 | 1.40 | 66.60 | 65.89 | 1.08% |
| Mapping based metrics | 0.996253 | 0.90933 | 0.978593 | 0.84943 |
| Mesh based metrics | 0.996248 | 0.90612 | 0.978594 | 0.84857 |
| Error | 0.0005% | 0.35% | 0.0001% | 0.1006% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xie, C.; Jia, S.; Li, Y.; An, C.; Yang, C. Mapping Based Quality Metrics for Mesh Deformation Algorithms Using Radial Basis Functions. Appl. Sci. 2021, 11, 59. https://doi.org/10.3390/app11010059
Xie C, Jia S, Li Y, An C, Yang C. Mapping Based Quality Metrics for Mesh Deformation Algorithms Using Radial Basis Functions. Applied Sciences. 2021; 11(1):59. https://doi.org/10.3390/app11010059
Chicago/Turabian StyleXie, Changchuan, Sijia Jia, Yingjie Li, Chao An, and Chao Yang. 2021. "Mapping Based Quality Metrics for Mesh Deformation Algorithms Using Radial Basis Functions" Applied Sciences 11, no. 1: 59. https://doi.org/10.3390/app11010059
APA StyleXie, C., Jia, S., Li, Y., An, C., & Yang, C. (2021). Mapping Based Quality Metrics for Mesh Deformation Algorithms Using Radial Basis Functions. Applied Sciences, 11(1), 59. https://doi.org/10.3390/app11010059






