Three-Dimensional Thematic Map Imaging of the Yacht Port on the Example of the Polish National Sailing Centre Marina in Gdańsk
Abstract
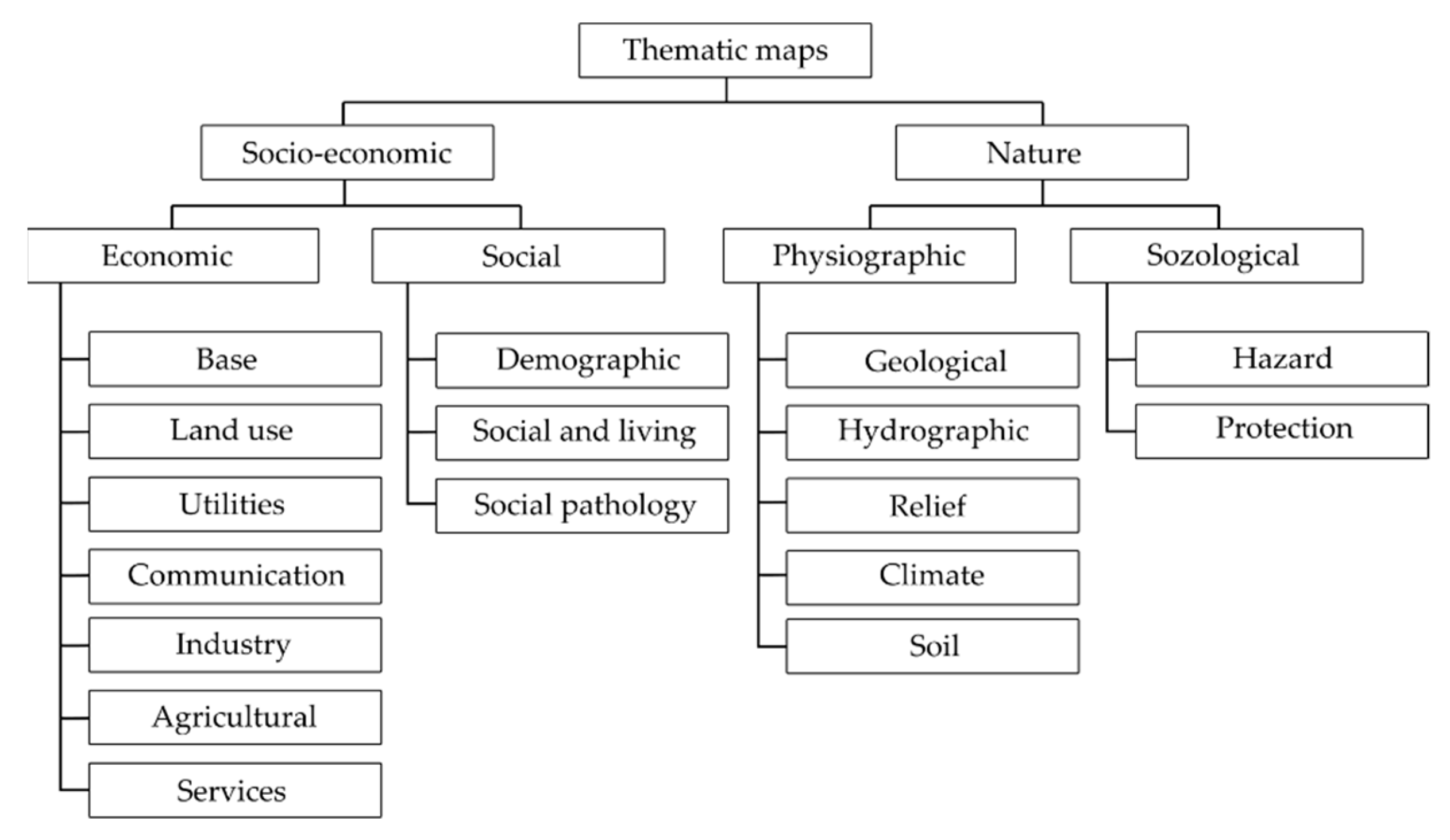
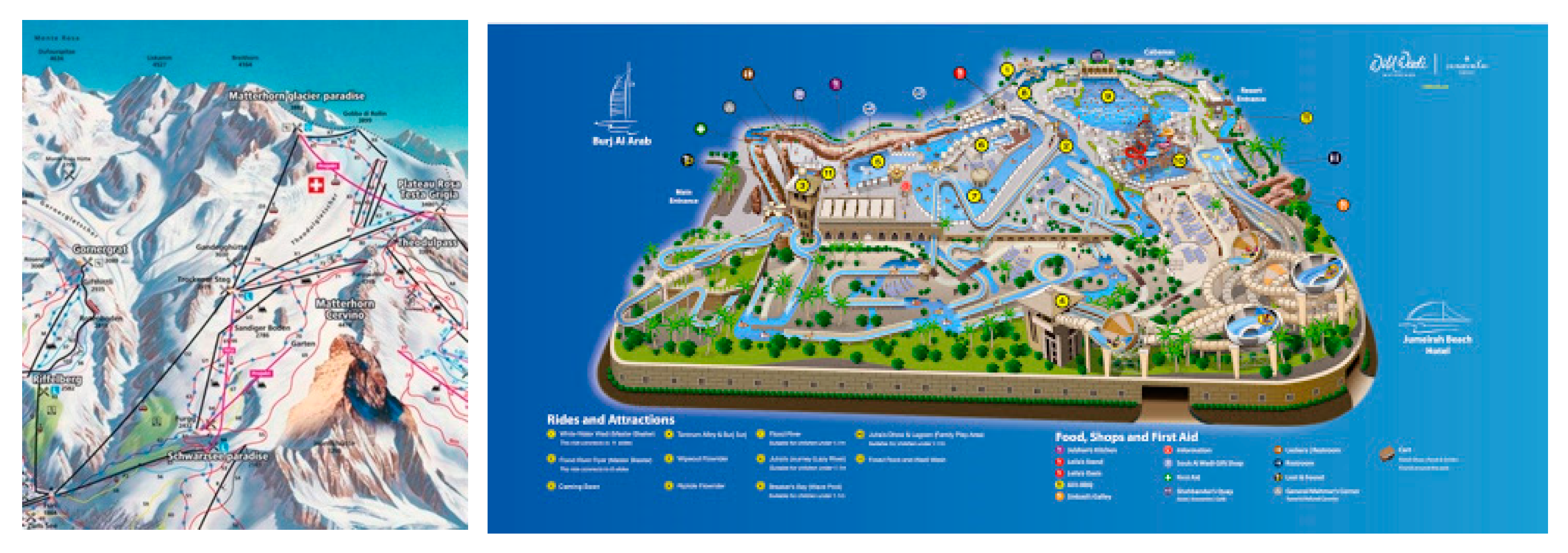
:1. Introduction
2. Materials and Methods
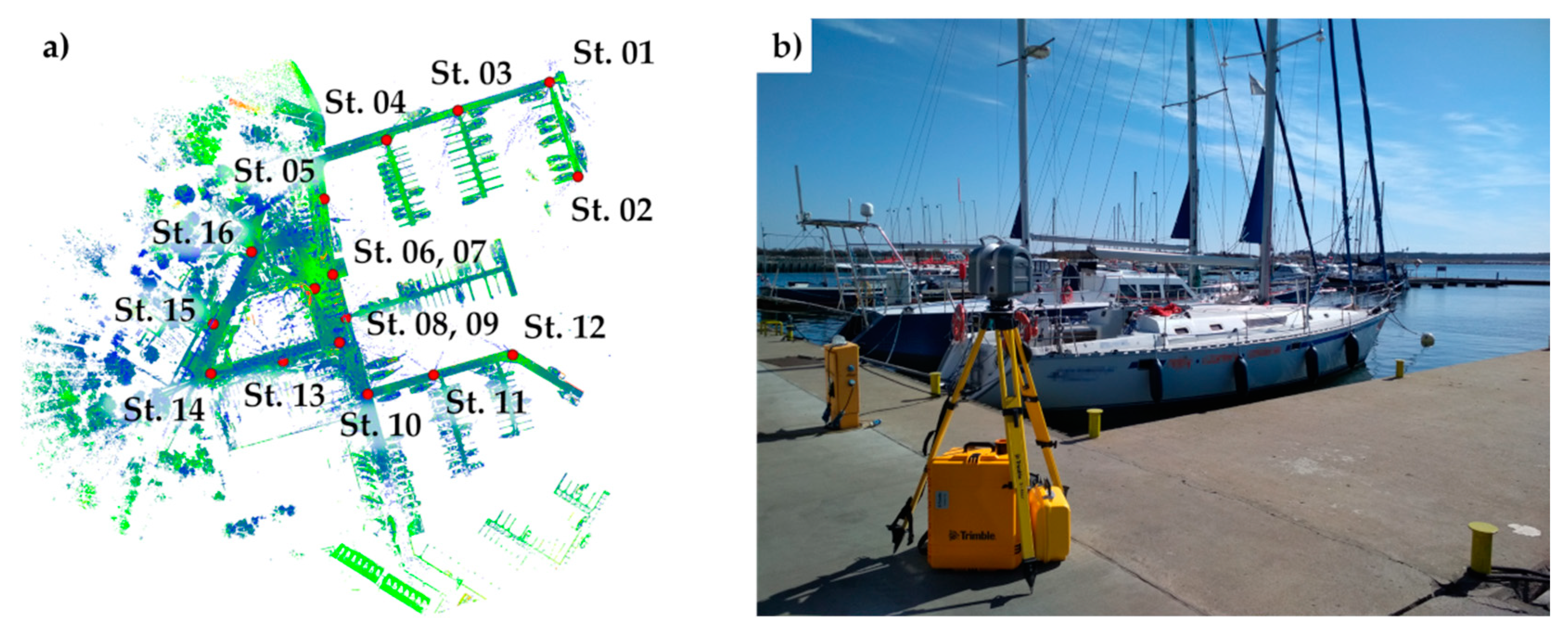
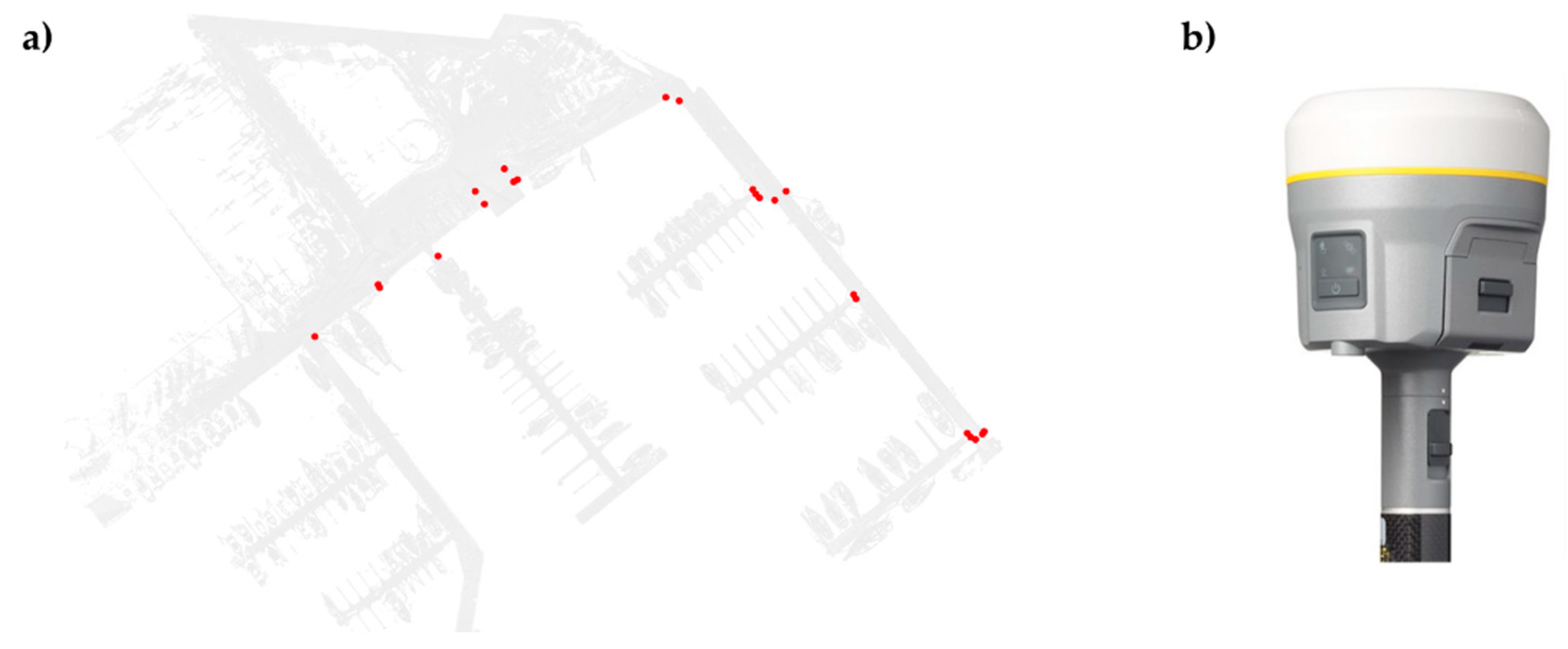
2.1. Measurement Data
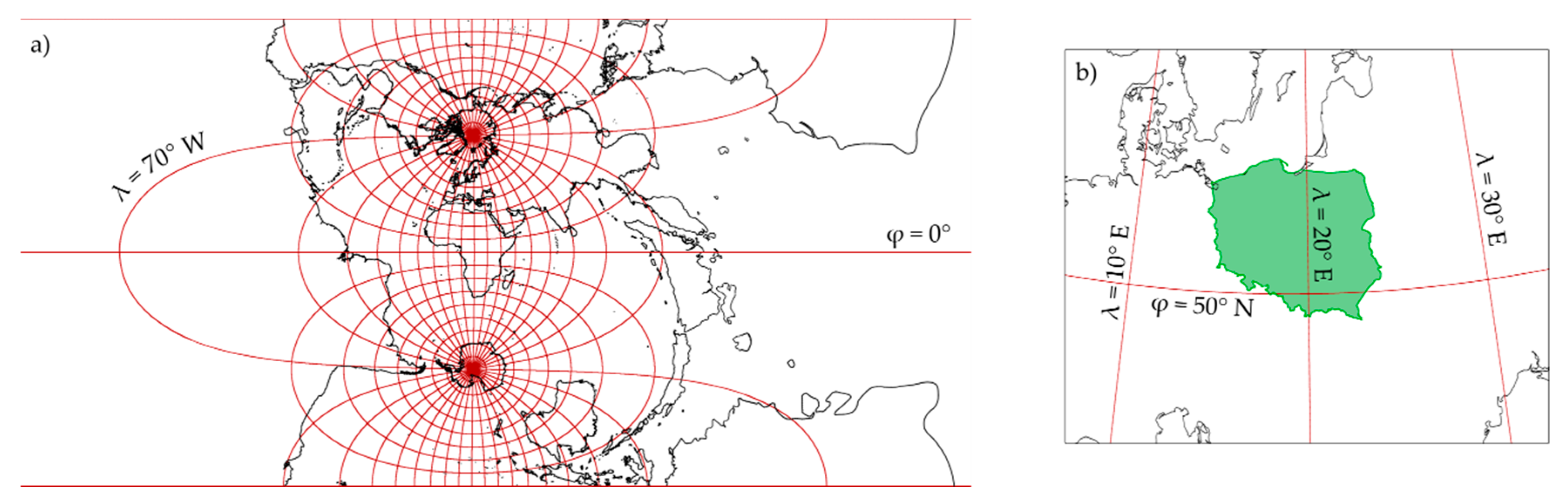
2.2. Data Processing
- —point coordinates vectors before and after transformation, respectively,
- —rotation matrix by the angle θ around the vertical axis OZ of the coordinate system,
- —translation vector.
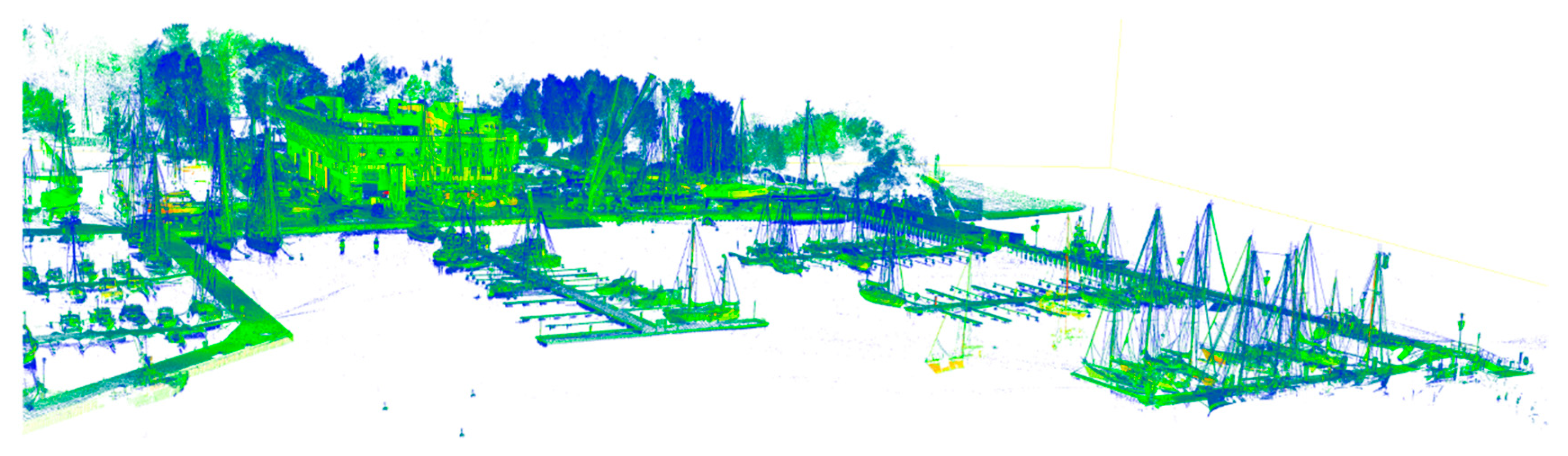
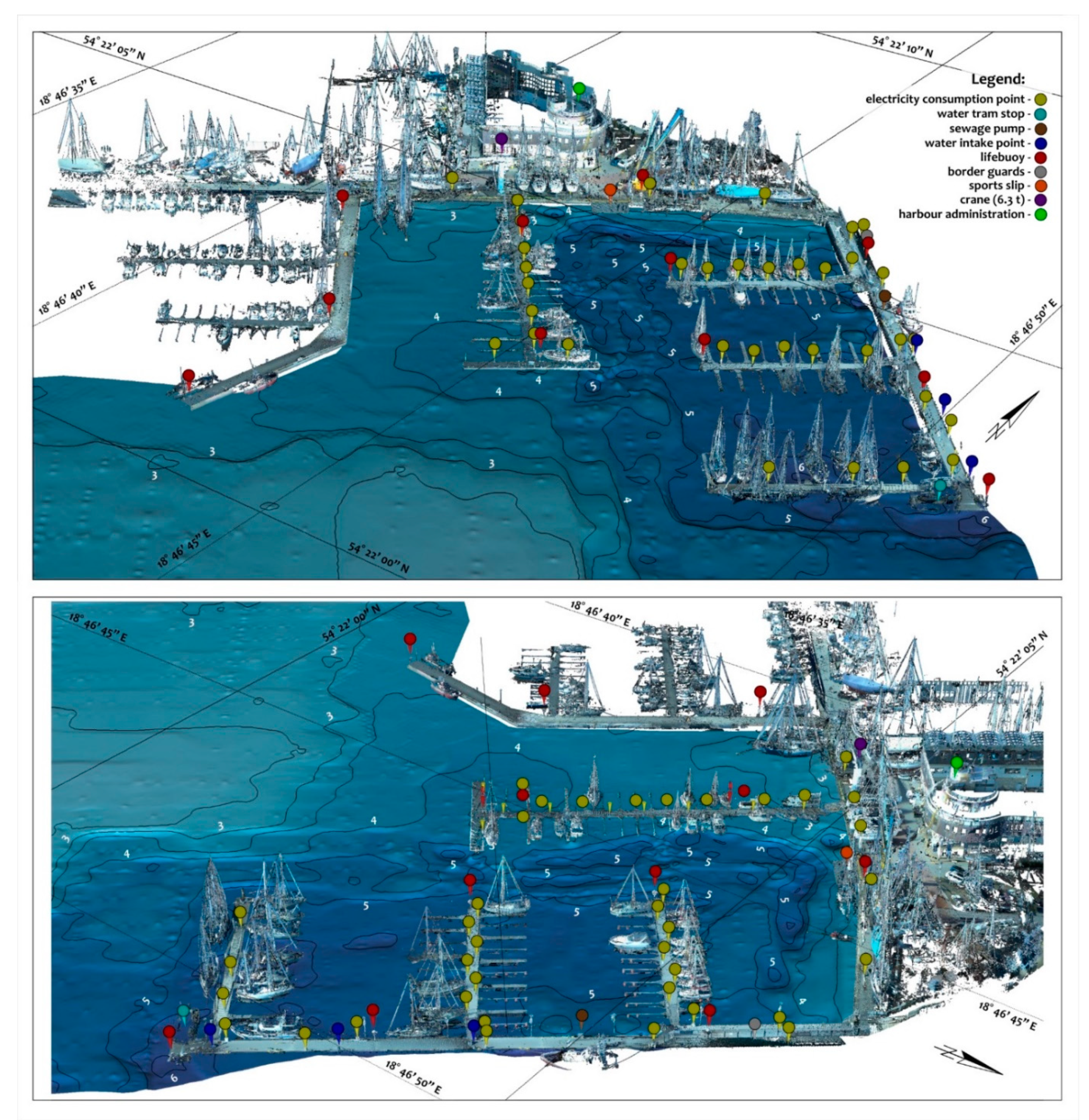
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Bagrow, L. History of Cartography; Routledge: New York, NY, USA, 2017. [Google Scholar]
- Bugayevskiy, L.M.; Snyder, J. Map Projections: A Reference Manual; CRC Press: Boca Raton, FL, USA, 1995. [Google Scholar]
- Robinson, A.H. Elements of Cartography; John and Wiley and Sons: Hoboken, NJ, USA, 1995. [Google Scholar]
- Maling, D.H. Coordinate Systems and Map Projections; Elsevier: Amsterdam, The Netherlands, 2013. [Google Scholar]
- Hooijberg, M. Practical Geodesy: Using Computers; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Peterson, G.N. GIS Cartography: A Guide to Effective Map Design; CRC Press: Boca Raton, FL, USA, 2014. [Google Scholar]
- Tyner, J.A. Principles of Map Design; Guilford Publications: New York, NY, USA, 2014. [Google Scholar]
- Monmonier, M. Rhumb Lines and Map Wars: A Social History of the Mercator Projection; University of Chicago Press: Chicago, IL, USA, 2010. [Google Scholar]
- Morgaś, W.; Kopacz, Z. Conversion of geodetic coordinates into flat (2-dimensional) coordinates PL-UTM for the purposes of navigation. Sci. J. Pol. Nav. Acad. 2017, 208, 45–60. [Google Scholar]
- Deakin, R.E.; Hunter, M.N.; Karney, C.F.F. The Gauss-Krüger projection. In Proceedings of the 23rd Victorian Regional Survey Conference, Warrnambool, Australia, 10–12 September 2010; pp. 1–20. [Google Scholar]
- Dent, B.D.; Torguson, J.S.; Holder, T.W. Cartography: Thematic Map Design; McGraw Hill Higher Education: Boston, MA, USA, 2009. [Google Scholar]
- Slocum, T.A.; MacMaster, R.B.; Kessler, F.C.; Howard, H.H. Thematic Cartography and Geovisualization, 3rd ed.; Pearson Prentice Hall: Hoboken, NJ, USA, 2009. [Google Scholar]
- Żyszkowska, W. Levels and properties of map perception. Pol. Cartogr. Rev. 2017, 49, 17–26. [Google Scholar] [CrossRef] [Green Version]
- Kraak, M.J.; Ormeling, F. Cartography: Visualization of Spatial Data; Guilford Press: New York, NY, USA, 2011. [Google Scholar]
- DiBiase, D. Visualization in the earth sciences. Earth Miner. Sci. 1990, 59, 13–18. [Google Scholar]
- Dabrowski, P.S.; Specht, C. Spatial expansion of the symmetrical objects point clouds to the lateral surface of the cylinder—Mathematical model. Measurement 2019, 134, 40–47. [Google Scholar] [CrossRef]
- Specht, M.; Specht, C.; Mindykowski, J.; Dąbrowski, P.; Maśnicki, R.; Makar, A. Geospatial Modeling of the Tombolo Phenomenon in Sopot using Integrated Geodetic and Hydrographic Measurement Methods. Remote Sens. 2020, 12, 737. [Google Scholar] [CrossRef] [Green Version]
- Dąbrowski, P.S.; Specht, C.; Felski, A.; Koc, W.; Wilk, A.; Czaplewski, K.; Karwowski, K.; Jaskólski, K.; Specht, M.; Chrostowski, P.; et al. The Accuracy of a Marine Satellite Compass under Terrestrial Urban Conditions. J. Mar. Sci. Eng. 2020, 8, 18. [Google Scholar] [CrossRef] [Green Version]
- Specht, C.; Lewicka, O.; Specht, M.; Dąbrowski, P.; Burdziakowski, P. Methodology for Carrying Out Measurements of the Tombolo Geomorphic Landform Using Unmanned Aerial and Surface Vehicles near Sopot Pier, Poland. J. Mar. Sci. Eng. 2020, 8, 384. [Google Scholar] [CrossRef]
- Specht, C.; Dąbrowski, P.; Dumalski, A.; Hejbudzka, K. Modeling 3D Objects for Navigation Purposes Using Laser Scanning. TransNav: Int. J. Mar. Navig. Saf. Sea Transp. 2016, 10. [Google Scholar] [CrossRef]
- Vasilev, S. Who is Who’ on the Map? In Proceedings of the 1st International Trade Fair of Geodesy, Cartography, Navigation and Geoinformatics GEOS 2006, Prague, Czech Republic, 1–3 March 2007. [Google Scholar]
- Friendly, M. 1995. Milestones in the History of Thematic Cartography, Statistical Graphics, and Data Visualization. In Proceedings of the 13th International Conference on Database and Expert Systmes Applications (DEXA 2002), Aix-en-Provence, France, 2–6 September 2002; pp. 59–66. [Google Scholar]
- Tennekes, M. Tmap: Thematic Maps in R. J. Stat. Softw. 2018, 84, 1–39. [Google Scholar] [CrossRef] [Green Version]
- Góralski, R. Three-Dimensional Interactive Maps Theory and Practice, A Submission Presented in Partial Fulfilment of the Requirements of the University of Glamorgan/Prifysgol Morgannwg for the Degree of Doctor of Philosophy. 2009. Available online: https://core.ac.uk/reader/9653382 (accessed on 4 May 2020).
- Van Driel, N.J. Three dimensional display of geologic data. In Three Dimensional Applications in Geographical Information Systems; Raper, J., Ed.; CRC Press: Boca Raton, FL, USA, 1989. [Google Scholar]
- Musliman, I.A.; Abdul-Rahman, A.; Coors, V. 3D Navigation for 3D-GIS—Initial Requirements. In Innovations in 3D Geo Information Systems; Abdul-Rahman, A., Zlatanova, S., Coors, V., Eds.; Springer: Berlin/Heidelberg, Germany, 2006. [Google Scholar]
- Schilling, A.; Coors, V.; Giersich, M.; Aasgaard, R. Introducing 3D GIS for the Mobile Community—Technical Aspects in the Case of TellMaris; IMC Workshop on Assistance, Mobility, Applications: Stuttgart, Germany, 2003. [Google Scholar]
- Kraak, M.J. Visual exploration of virtual environments. In Virtual Reality in Geography; Unwin, D., Fisher, P., Eds.; Taylor & Francis: Abingdon, UK, 2002. [Google Scholar]
- Makar, A.; Specht, C.; Specht, M.; Dąbrowski, P.; Szafran, M. Integrated Geodetic and Hydrographic Measurements of the Yacht Port for Nautical Charts and Dynamic Spatial Presentation. Geosciences 2020, 10, 203. [Google Scholar] [CrossRef]
- Mitchell, W.J.; McCullough, M. Digital Design Media; John Wiley & Sons: Hoboken, NJ, USA, 1995. [Google Scholar]
- Carlbom, I.; Paciorek, J. Planar geometric projections and viewing transformations. ACM Comput. Surv. (CSUR) 1978, 10, 465–502. [Google Scholar] [CrossRef] [Green Version]
- Naus, K. Electronic navigational chart as an equivalent to image produced by hypercatadioptric camera system. Pol. Marit. Res. 2015, 22, 3–9. [Google Scholar] [CrossRef] [Green Version]
- Snyder, J.P. Map Projections—A Working Manual; US Government Printing Office: Washington, DC, USA, 1987; Volume 1395.
- Pavlis, N.K.; Holmes, S.A.; Kenyon, S.C.; Factor, J.K. An earth gravitational model to degree 2160: EGM2008. EGU Gen. Assem. 2008, 10, 13–18. [Google Scholar]
- Pomerleau, F.; Colas, F.; Siegwart, R.; Magnenat, S. Comparing ICP variants on real-world data sets. Auton. Robot. 2013, 34, 133–148. [Google Scholar] [CrossRef]
- Kalman, D. A singularly valuable decomposition: The SVD of a matrix. Coll. Math. J. 1996, 27, 2–23. [Google Scholar] [CrossRef]
- Olivier, R.; Hanqiang, C. Nearest neighbor value interpolation. Int. J. Adv. Comput. Sci. Appl. 2012, 3, 25–30. [Google Scholar] [CrossRef] [Green Version]
- Wolf, P.R.; Dewitt, B.A.; Wilkinson, B.E. Elements of Photogrammetry with Applications in GIS; McGraw-Hill Education: New York, NY, USA, 2014. [Google Scholar]
- Papachristos, N.M.; Vrellis, I.; Mikropoulos, T.A. A comparison between oculus rift and a low-cost smartphone VR headset: Immersive user experience and learning. In Proceedings of the 2017 IEEE 17th International Conference on Advanced Learning Technologies (ICALT), Timisoara, Romania, 3–7 July 2017; IEEE: New York, NY, USA, 2017; pp. 477–481. [Google Scholar]
- Froehlich, M.A.; Azhar, S. Investigating virtual reality headset applications in construction. In Proceedings of the 52nd Associated Schools of Construction Annual International Conference, Provo, UT, USA, 13–16 April 2016; Volume 52, pp. 13–16. [Google Scholar]
- Walmsley, A.P.; Kersten, T.P. The IMPERIAL Cathedral in Königslutter (Germany) as an immersive experience in virtual reality with integrated 360 panoramic photography. Appl. Sci. 2020, 10, 1517. [Google Scholar] [CrossRef] [Green Version]
- Edler, D.; Keil, J.; Wiedenlübbert, T.; Sossna, M.; Kühne, O.; Dickmann, F. Immersive VR experience of redeveloped post-industrial sites: The example of “Zeche Holland” in Bochum-Wattenscheid. KN-J. Cartogr. Geogr. Inf. 2019, 69, 267–284. [Google Scholar] [CrossRef] [Green Version]
- Hruby, F. The sound of being there: Audiovisual cartography with immersive virtual environments. KN-J. Cartogr. Geogr. Inf. 2019, 69, 19–28. [Google Scholar] [CrossRef] [Green Version]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dabrowski, P.S.; Specht, C.; Specht, M.; Makar, A. Three-Dimensional Thematic Map Imaging of the Yacht Port on the Example of the Polish National Sailing Centre Marina in Gdańsk. Appl. Sci. 2021, 11, 7016. https://doi.org/10.3390/app11157016
Dabrowski PS, Specht C, Specht M, Makar A. Three-Dimensional Thematic Map Imaging of the Yacht Port on the Example of the Polish National Sailing Centre Marina in Gdańsk. Applied Sciences. 2021; 11(15):7016. https://doi.org/10.3390/app11157016
Chicago/Turabian StyleDabrowski, Pawel S., Cezary Specht, Mariusz Specht, and Artur Makar. 2021. "Three-Dimensional Thematic Map Imaging of the Yacht Port on the Example of the Polish National Sailing Centre Marina in Gdańsk" Applied Sciences 11, no. 15: 7016. https://doi.org/10.3390/app11157016
APA StyleDabrowski, P. S., Specht, C., Specht, M., & Makar, A. (2021). Three-Dimensional Thematic Map Imaging of the Yacht Port on the Example of the Polish National Sailing Centre Marina in Gdańsk. Applied Sciences, 11(15), 7016. https://doi.org/10.3390/app11157016







