Spin Dependent Transport through Driven Magnetic System with Aubry-Andre-Harper Modulation
Abstract
:1. Introduction
2. Magnetic Junction, TB Hamiltonian and Theoretical Formulation
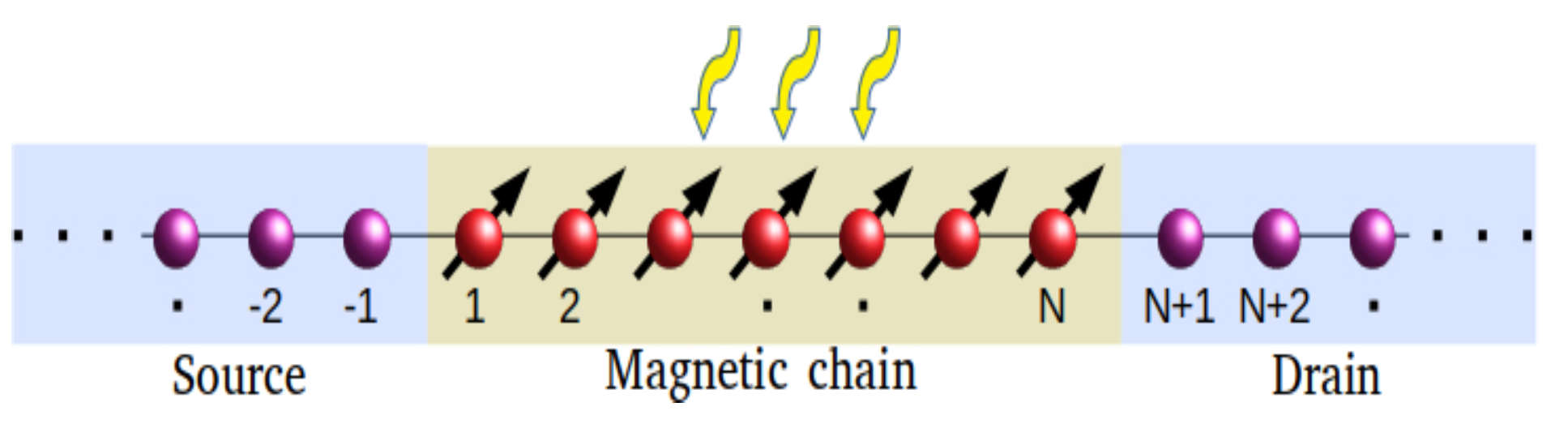
2.1. Junction Setup and the Hamiltonian
2.2. Theoretical Formulation
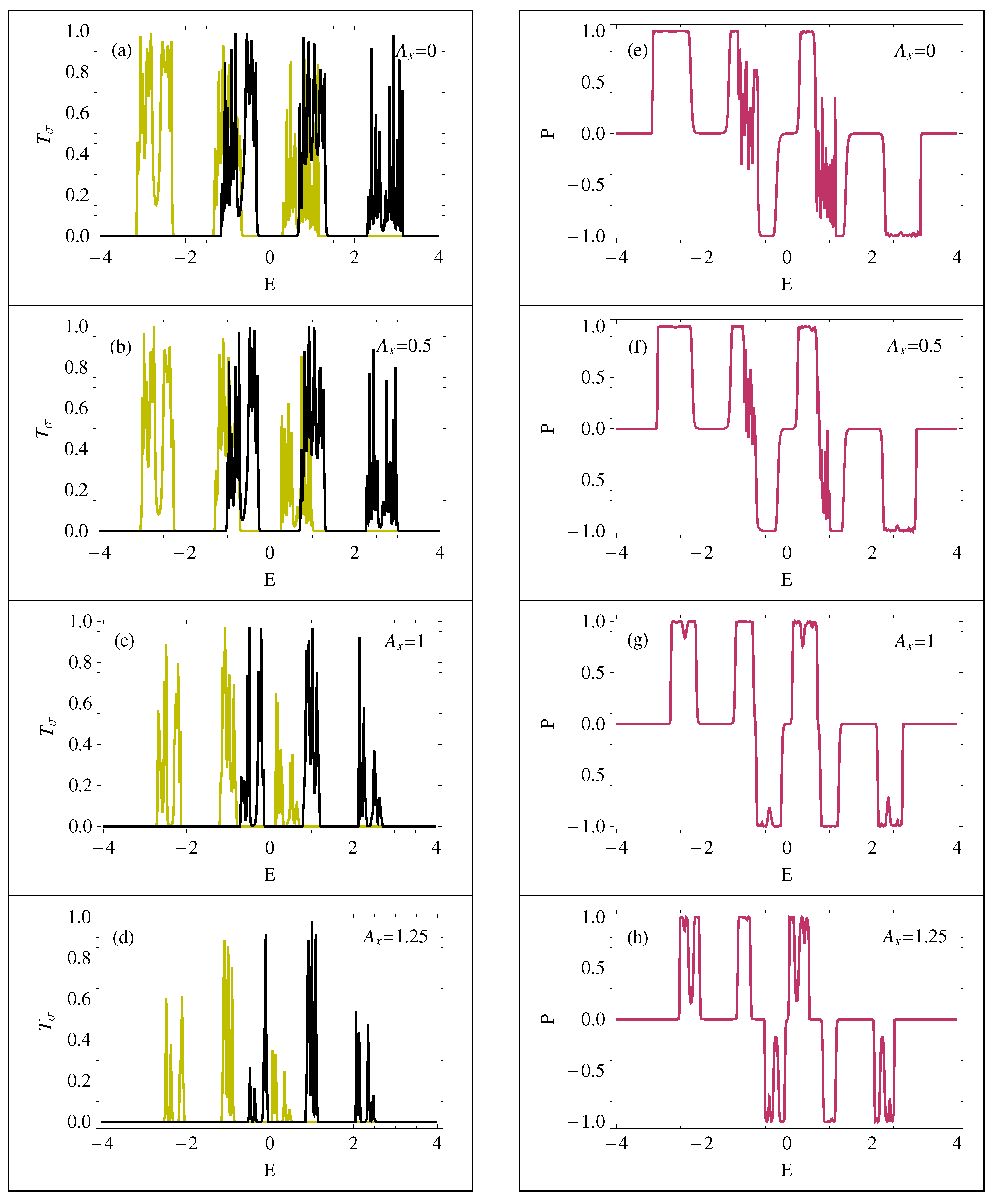
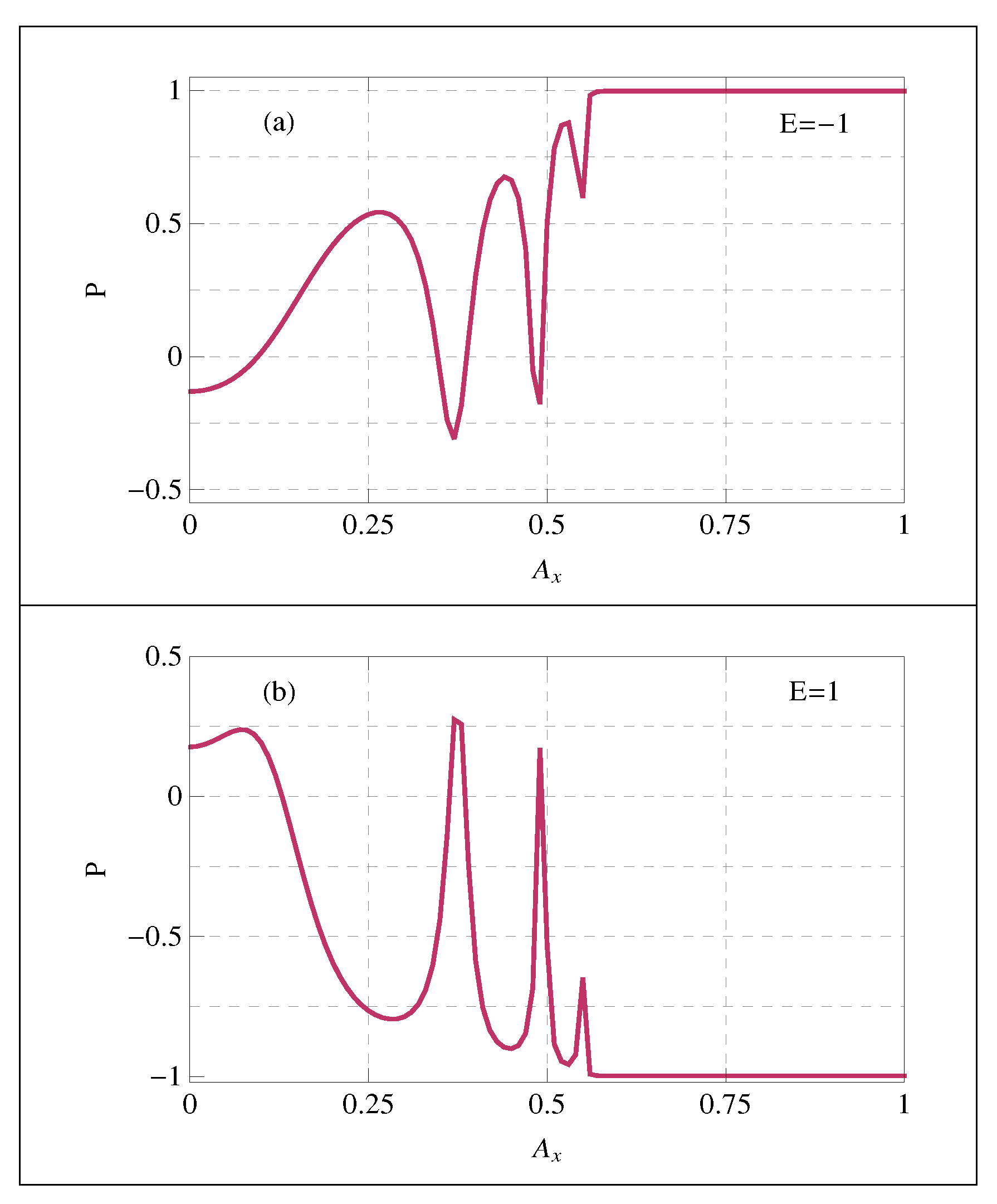
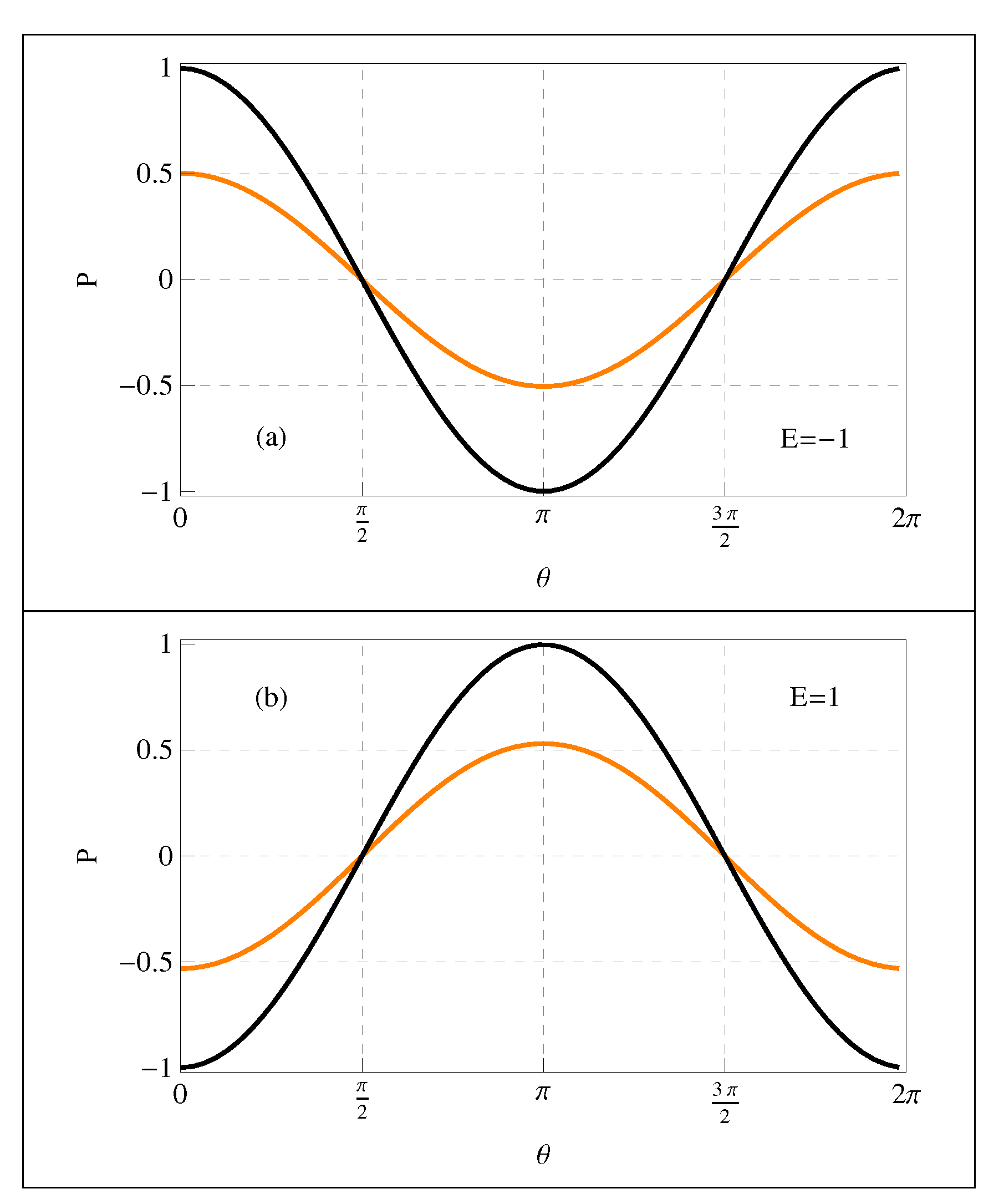
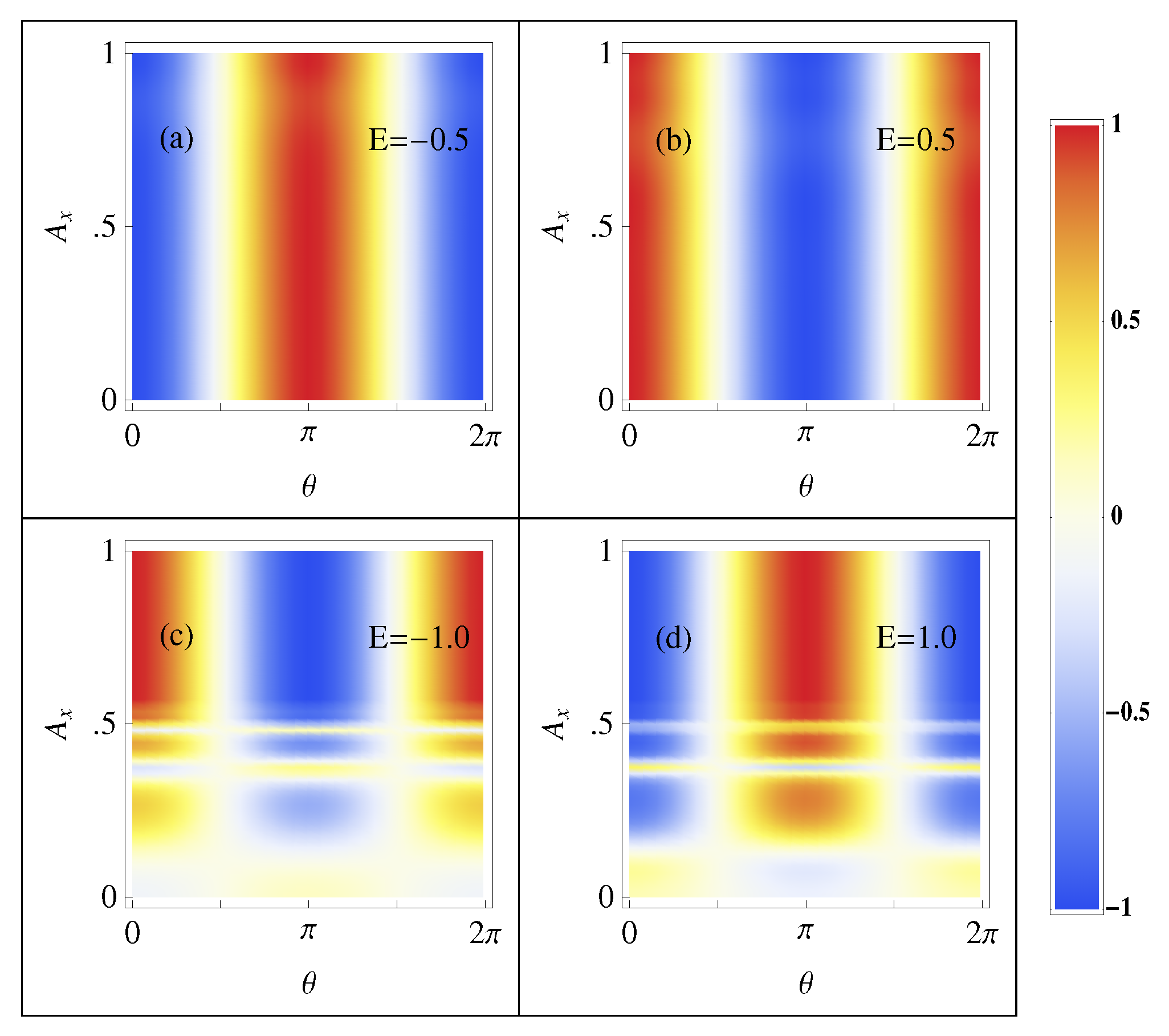
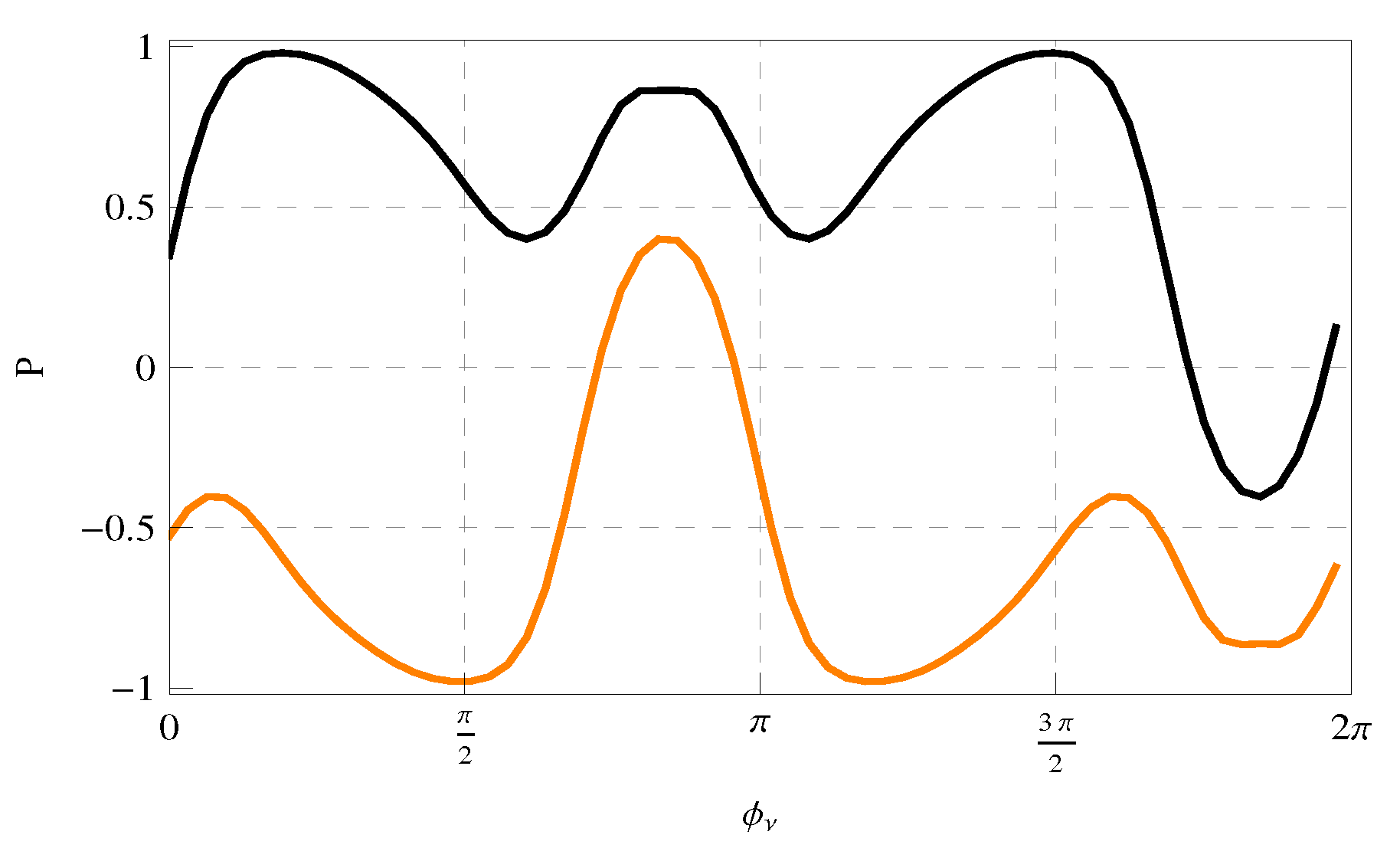
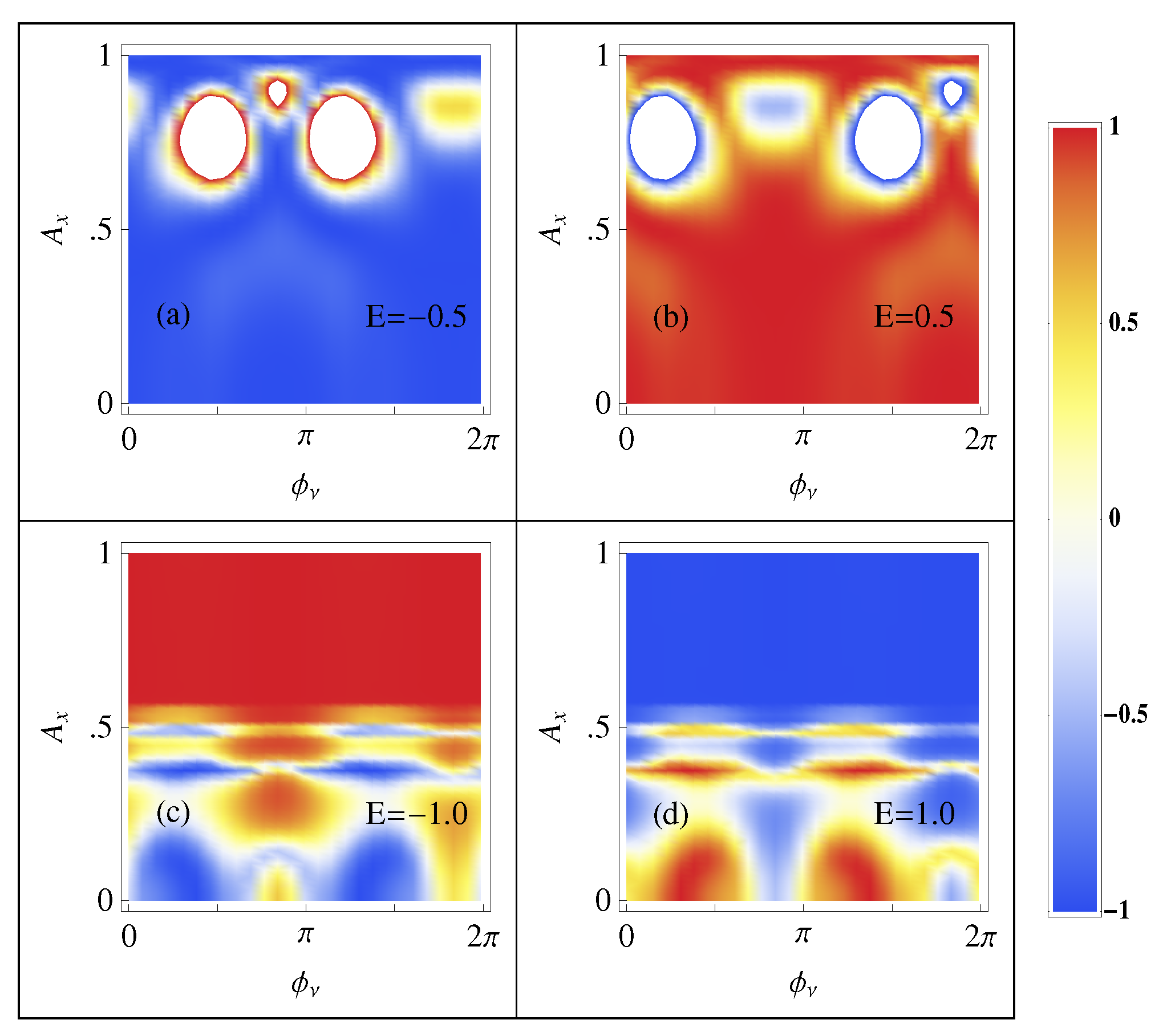
3. Numerical Results and Discussion
4. Closing Remarks
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wolf, S.A.; Awschalom, D.D.; Buhrman, R.A.; Daughton, J.M.; von Molnár, S.; Roukes, M.L.; Chtchelkanova, A.Y.; Trege, D.M. Spintronics: A spin-based electronics vision for the future. Science 2001, 294, 1488. [Google Scholar] [CrossRef] [Green Version]
- Žutić, I.; Fabian, J.; Sarma, S.D. Spintronics: Fundamentals and applications. Rev. Mod. Phys. 2004, 76, 323. [Google Scholar]
- Sahoo, S.; Kontos, T.; Furer, J.; Hoffmann, C.; Gräber, M.; Cottet, A.; Schönenberger, C. Electric field control of spin transport. Nat. Phys. 2005, 1, 99. [Google Scholar] [CrossRef] [Green Version]
- Datta, S.; Das, B. Electronic analog of the electro-optic modulator. Appl. Phys. Lett. 1990, 56, 665. [Google Scholar] [CrossRef]
- Tsukagoshi, K.; Alphenaar, B.W.; Ago, H. Coherent transport of electron spin in a ferromagnetically contacted carbon nanotube. Nature 1999, 401, 572. [Google Scholar] [CrossRef]
- Kobayashi, T.; Nakata, Y.; Yaji, K.; Shishidou, T.; Agterberg, D.; Yoshizawa, S.; Komori, F.; Shin, S.; Weinert, M.; Uchihashi, T.; et al. Orbital Angular Momentum Induced Spin Polarization of 2D Metallic Bands. Phys. Rev. Lett. 2020, 125, 176401. [Google Scholar] [CrossRef]
- Yuan, L.-D.; Wang, Z.; Luo, J.-W.; Rashba, E.I.; Zunger, A. Giant momentum-dependent spin splitting in centrosymmetric low-Z antiferromagnets. Phys. Rev. B 2020, 102, 014422. [Google Scholar] [CrossRef]
- Manchon, A.; Zelezny, J.; Miron, I.M.; Jungwirth, T.; Sinova, J.; Thiaville, A.; Garello, K.; Gambardella, P. Current-induced spin-orbit torques in ferromagnetic and antiferromagnetic systems. Rev. Mod. Phys. 2019, 91, 035004. [Google Scholar] [CrossRef] [Green Version]
- Jiao, Y.; Ma, F.; Zhang, C.; Bell, J.; Sanvito, S.; Du, A. First-Principles Prediction of Spin-Polarized Multiple Dirac Rings in Manganese Fluoride. Phys. Rev. Lett. 2017, 119, 016403. [Google Scholar] [CrossRef]
- Han, W.; Maekawa, S.; Xie, X.-C. Spin current as a probe of quantum materials. Nat. Mat. 2020, 19, 139. [Google Scholar] [CrossRef] [PubMed]
- Prinz, G.A. Magnetoelectronics. Science 1998, 282, 1660. [Google Scholar] [CrossRef] [PubMed]
- Schmidt, G.; Ferrand, D.; Molenkamp, L.W.; Filip, A.T.; van Wees, B.J. Fundamental obstacle for electrical spin injection from a ferromagnetic metal into a diffusive semiconductor. Phys. Rev. B 2000, 62, R4790. [Google Scholar] [CrossRef] [Green Version]
- Wu, M.W.; Zhou, J.; Shi, Q.W. Spin-dependent quantum transport in periodic magnetic modulations: Aharonov–Bohm ring structure as a spin filter. Appl. Phys. Lett. 2004, 85, 1012. [Google Scholar] [CrossRef] [Green Version]
- Kiselev, A.A.; Kim, K.W. T-shaped ballistic spin filter. Appl. Phys. Lett. 2001, 78, 775. [Google Scholar] [CrossRef]
- Governale, M.; Boese, D.; Zülicke, U.; Schroll, C. Filtering spin with tunnel-coupled electron wave guides. Phys. Rev. B 2002, 65, 140403. [Google Scholar] [CrossRef] [Green Version]
- Maiti, S.K. Curvature effect on spin polarization in a three-terminal geometry in presence of Rashba spin-orbit interaction. Phys. Lett. A 2015, 379, 361. [Google Scholar] [CrossRef] [Green Version]
- Khodas, M.; Shekhter, A.; Finkel’stein, A.M. Spin Polarization of Electrons by Nonmagnetic Heterostructures: The Basics of Spin Optics. Phys. Rev. Lett. 2004, 92, 086602. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Streda, P.; Seba, P. Antisymmetric Spin Filtering in One-Dimensional Electron Systems with Uniform Spin-Orbit Coupling. Phys. Rev. Lett. 2003, 90, 256601. [Google Scholar] [CrossRef]
- Dey, M.; Maiti, S.K.; Karmakar, S.N. Magnetic quantum wire as a spin filter: An exact study. Phys. Lett. A 2010, 374, 1522. [Google Scholar] [CrossRef] [Green Version]
- Dey, M.; Maiti, S.K.; Karmakar, S.N. Spin transport through a quantum network: Effects of Rashba spin-orbit interaction and Aharonov-Bohm flux. J. Appl. Phys. 2011, 109, 024304. [Google Scholar] [CrossRef] [Green Version]
- Winkler, R. Spin-Orbit Coupling Effects in Two-Dimensional Electron and Hole Systems; Springer Tracts in Modern Physics; Springer: New York, NY, USA, 2003; Volume 191. [Google Scholar]
- Pareek, T.P. Pure Spin Currents and the Associated Electrical Voltage. Phys. Rev. Lett. 2004, 92, 076601. [Google Scholar] [CrossRef] [Green Version]
- Sun, Q.F.; Xie, X.C. Bias-controllable intrinsic spin polarization in a quantum dot: Proposed scheme based on spin-orbit interaction. Phys. Rev. B 2006, 73, 235301. [Google Scholar] [CrossRef] [Green Version]
- Maiti, S.K.; Dey, M.; Sil, S.; Chakrabarti, A.; Karmakar, S.N. Magneto-transport in a mesoscopic ring with Rashba and Dresselhaus spin-orbit interactions. Europhys. Lett. 2011, 95, 57008. [Google Scholar] [CrossRef] [Green Version]
- Chi, F.; Zheng, J.; Sun, L.L. Spin-polarized current and spin accumulation in a three-terminal two quantum dots ring. Appl. Phys. Lett. 2008, 92, 172104. [Google Scholar] [CrossRef]
- Maiti, S.K. Determination of Rashba and Dresselhaus spin-orbit fields. J. Appl. Phys. 2011, 110, 064306. [Google Scholar] [CrossRef] [Green Version]
- Sil, S.; Maiti, S.K.; Chakrabarti, A. Interplay of magnetic field and geometry in magneto-transport of mesoscopic loops with Rashba and Dresselhaus spin-orbit interactions. J. Appl. Phys. 2012, 112, 024321. [Google Scholar] [CrossRef] [Green Version]
- Maiti, S.K.; Sil, S.; Chakrabarti, A. A proposal for the measurement of Rashba and Dresselhaus spin-orbit interaction strengths in a single sample. Phys. Lett. A 2012, 376, 2147. [Google Scholar] [CrossRef] [Green Version]
- Yin, H.-T.; Lu, T.-Q.; Liu, X.-J.; Xue, H.-J. Voltage-controllable spin-polarized transport through parallel-coupled double quantum dots with Rashba spin-orbit interaction. Phys. Lett. A 2009, 373, 285. [Google Scholar] [CrossRef]
- Ganguly, S.; Basu, S.; Maiti, S.K. Unconventional charge and spin dependent transport properties of a graphene nanoribbon with line-disorder. Europhys. Lett. 2018, 124, 57003. [Google Scholar] [CrossRef]
- Patra, M.; Maiti, S.K. Unconventional low-field magnetic response of a diffusive ring with spin-orbit coupling. Phys. Lett. A 2017, 381, 221. [Google Scholar] [CrossRef] [Green Version]
- Bychkov, Y.A.; Rashba, E.I. Properties of a 2D electron gas with lifted spectral degeneracy. Sov. Phys. JETP Lett. 1984, 39, 78. [Google Scholar]
- Dresselhaus, G. Spin-Orbit Coupling Effects in Zinc Blende Structures. Phys. Rev. 1955, 100, 580. [Google Scholar] [CrossRef]
- Földi, O.; Kálmán, P.; Benedict, M.G.; Peeters, F.M. Quantum rings as electron spin beam splitters. Phys. Rev. B 2006, 73, 155325. [Google Scholar] [CrossRef] [Green Version]
- Hatano, N.; Shirasaki, R.; Nakamura, H. Non-Abelian gauge field theory of the spin-orbit interaction and a perfect spin filter. Phys. Rev. A 2007, 75, 032107. [Google Scholar] [CrossRef] [Green Version]
- Dey, M.; Maiti, S.K.; Karmakar, S.N. Spin Hall effect in a Kagome lattice driven by Rashba spin-orbit interaction. J. Appl. Phys. 2012, 112, 024322. [Google Scholar] [CrossRef] [Green Version]
- Földi, O.; Kálmán, P.; Benedict, M.G.; Peeters, F.M. Networks of Quantum Nanorings: Programmable Spintronic Devices. Nano Lett. 2008, 8, 2556. [Google Scholar] [CrossRef] [Green Version]
- Dey, M.; Maiti, S.K.; Sil, S.; Karmakar, S.N. Spin-orbit interaction induced spin selective transmission through a multi-terminal mesoscopic ring. J. Appl. Phys. 2013, 114, 164318. [Google Scholar] [CrossRef] [Green Version]
- Cohen, G.; Hod, O.; Rabani, E. Constructing spin interference devices from nanometric rings. Phys. Rev. B 2007, 76, 235120. [Google Scholar] [CrossRef] [Green Version]
- Maiti, S.K. Externally controlled selective spin transfer through a two-terminal bridge setup. Eur. Phys. J. B 2015, 88, 172. [Google Scholar] [CrossRef] [Green Version]
- Földi, O.; Kálmán, P.; Peeters, F.M. Stability of spintronic devices based on quantum ring networks. Phys. Rev. B 2009, 80, 125324. [Google Scholar] [CrossRef] [Green Version]
- Patra, M.; Maiti, S.K. Externally controlled high degree of spin polarization and spin inversion in a conducting junction: Two new approaches. Sci. Rep. 2017, 7, 14313. [Google Scholar] [CrossRef]
- Göhler, B.; Hamelbeck, V.; Markus, T.Z.; Kettner, M.; Hanne, G.F.; Vager, Z.; Naaman, R.; Zacharias, H. Spin Selectivity in Electron Transmission Through Self-Assembled Monolayers of Double-Stranded DNA. Science 2011, 331, 894. [Google Scholar] [CrossRef] [PubMed]
- Ganguly, S.; Basu, S.; Maiti, S.K. Interface sensitivity on spin transport through a three-terminal graphene nanoribbon. Superlattices Microstruct. 2018, 120, 650. [Google Scholar] [CrossRef] [Green Version]
- Guo, A.-M.; Sun, Q.-F. Spin-Selective Transport of Electrons in DNA Double Helix. Phys. Rev. Lett. 2012, 108, 218102. [Google Scholar] [CrossRef] [Green Version]
- Ganguly, S.; Basu, S.; Maiti, S.K. Controlled engineering of spin polarized transport properties in a zigzag graphene nanojunction. Europhys. Lett. 2018, 124, 17005. [Google Scholar] [CrossRef] [Green Version]
- Guo, A.-M.; Sun, Q.-F. Spin-dependent electron transport in protein-like single-helical molecules. Proc. Natl. Acad. Sci. USA 2014, 111, 11658. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kuemmeth, F.; Ilani, S.; Ralph, D.C.; McEuen, P.L. Coupling of spin and orbital motion of electrons in carbon nanotubes. Nature 2008, 452, 448. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Long, W.; Sun, Q.F.; Guo, H.; Wang, J. Gate-controllable spin battery. Appl. Phys. Lett. 2003, 83, 1397. [Google Scholar] [CrossRef] [Green Version]
- Zhang, P.; Xue, Q.K.; Xie, X.C. Spin Current through a Quantum Dot in the Presence of an Oscillating Magnetic Field. Phys. Rev. Lett. 2003, 91, 196602. [Google Scholar] [CrossRef] [Green Version]
- Adachi, H.; Ino, H. A ferromagnet having no net magnetic moment. Nature 1999, 401, 148. [Google Scholar] [CrossRef]
- Xu, Y.; Wang, S.; Xia, K. Spin-Transfer Torques in Antiferromagnetic Metals from First Principles. Phys. Rev. Lett. 2008, 100, 226602. [Google Scholar] [CrossRef] [Green Version]
- Jungwirth, T.; Sinova, J.; Matri, X.; Wunderlich, J.; Felser, C. The multiple directions of antiferromagnetic spintronics. Nat. Phys. 2018, 14, 200. [Google Scholar] [CrossRef]
- Jungfleisch, M.B.; Zhang, W.; Hoffmann, A. Perspectives of antiferromagnetic spintronics. Phys. Lett. A 2018, 13, 382. [Google Scholar] [CrossRef]
- Duine, R.A.; Lee, K.; Parkin, S.P.; Stiles, M.D. Synthetic antiferromagnetic spintronics. Nat. Phys. 2018, 14, 217. [Google Scholar] [CrossRef] [PubMed]
- Gupta, D.D.; Maiti, S.K. Can a sample having zero net magnetization produce polarized spin current? J. Phys. Condens. Matter 2020, 32, 505803. [Google Scholar] [CrossRef]
- Grifoni, M.; Hänggi, P. Driven quantum tunneling. Phys. Rep. 1998, 304, 229. [Google Scholar] [CrossRef]
- Kohler, S.; Lehmann, J.; Hänggi, P. Driven quantum transport on the nanoscale. Phys. Rep. 2005, 406, 379. [Google Scholar] [CrossRef] [Green Version]
- Delplace, P.; Gómez-Lexoxn, A.; Platero, G. Merging of Dirac points and Floquet topological transitions in ac-driven graphene. Phys. Rev. B 2013, 88, 245422. [Google Scholar] [CrossRef] [Green Version]
- Gómez-Lexoxn, A.; Platero, G. Floquet-Bloch Theory and Topology in Periodically Driven Lattices. Phys. Rev. Lett. 2013, 110, 200403. [Google Scholar] [CrossRef] [PubMed]
- Eckardt, A.; Anisimovas, E. High-frequency approximation for periodically driven quantum systems from a Floquet-space perspective. New. J. Phys. 2015, 17, 093039. [Google Scholar] [CrossRef]
- Goldman, N.; Dalibard, J.; Aidelsburger, M.; Cooper, N.R. Periodically driven quantum matter: The case of resonant modulations. Phys. Rev. A 2015, 91, 033632. [Google Scholar] [CrossRef] [Green Version]
- Sarkar, M.; Dey, M.; Maiti, S.K.; Sil, S. Engineering spin polarization in a driven multistranded magnetic quantum network. Phys. Rev. B 2020, 102, 195435. [Google Scholar] [CrossRef]
- Su, Y.-H.; Chen, S.-H.; Hu, C.D.; Chang, C.-R. Competition between spin-orbit interaction and exchange coupling within a honeycomb lattice ribbon. J. Phys. D Appl. Phys. 2016, 49, 015305. [Google Scholar] [CrossRef]
- Sarkar, S.; Maiti, S.K. Spin-selective transmission through a single-stranded magnetic helix. Phys. Rev. B 2019, 100, 205402. [Google Scholar] [CrossRef] [Green Version]
- Shokri, A.A.; Mardaani, M.; Esfarjani, K. Spin filtering and spin diode devices in quantum wire systems. Physica E 2005, 27, 325. [Google Scholar] [CrossRef]
- Shokri, A.A.; Mardaani, M. Spin-flip effect on electrical transport in magnetic quantum wire systems. Solid State Commun. 2006, 137, 53. [Google Scholar] [CrossRef]
- Peters, R.; Kawakami, N. Ferromagnetic state in the one-dimensional Kondo lattice model. Phys. Rev. B 2012, 86, 165107. [Google Scholar] [CrossRef] [Green Version]
- Peters, R.; Kawakami, N.; Pruschke, T. Spin-Selective Kondo Insulator: Cooperation of Ferromagnetism and the Kondo Effect. Phys. Rev. Lett. 2012, 108, 086402. [Google Scholar] [CrossRef] [Green Version]
- Aubry, S.; André, G. Analyticity breaking and Anderson localization in incommensurate lattices. Ann. Isr. Phys. Soc. 1980, 3, 133. [Google Scholar]
- Kraus, Y.E.; Lahini, Y.; Ringel, Z.; Verbin, M.; Zilberberg, O. Topological States and Adiabatic Pumping in Quasicrystals. Phys. Rev. Lett. 2012, 109, 106402. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Rossignolo, M.; Dell’Anna, L. Localization transitions and mobility edges in coupled Aubry-André chains. Phys. Rev. B 2019, 99, 054211. [Google Scholar] [CrossRef] [Green Version]
- Sil, S.; Maiti, S.K.; Chakrabarti, A. Metal-insulator transition in an aperiodic ladder network: An exact result. Phys. Rev. Lett. 2008, 101, 076803. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Roy, S.; Maiti, S.K. Tight-binding quantum network with cosine modulations: Electronic localization and delocalization. Eur. Phys. J. B 2019, 92, 267. [Google Scholar] [CrossRef]
- Saha, S.; Maiti, S.K.; Karmakar, S.N. Multiple mobility edges in a 1D Aubry chain with Hubbard interaction in presence of electric field: Controlled electron transport. Physica E 2016, 83, 358. [Google Scholar] [CrossRef] [Green Version]
- Maiti, S.K.; Sil, S.; Chakrabarti, A. Phase controlled metal-insulator transition in multi-leg quasiperiodic optical lattices. Ann. Phys. (N. Y.) 2017, 382, 150. [Google Scholar] [CrossRef] [Green Version]
- Patra, M.; Maiti, S.K. Controlled charge and spin current rectifications in a spin polarized device. J. Magn. Magn. Mater. 2019, 484, 408. [Google Scholar] [CrossRef] [Green Version]
- Datta, S. Electronic Transport in Mesoscopic Systems; Cambridge University Press: Cambridge, UK, 1997. [Google Scholar]
- Datta, S. Quantum Transport: Atom to Transistor; Cambridge University Press: Cambridge, UK, 2005. [Google Scholar]
- Fisher, D.S.; Lee, P.A. Relation between conductivity and transmission matrix. Phys. Rev. B 1981, 23, 6851. [Google Scholar] [CrossRef] [Green Version]
- Ventra, M.D. Electrical Transport in Nanoscale Systems; Cambridge University Press: Cambridge, UK, 2008. [Google Scholar]
- Nikolić, B.K.; Allen, P.B. Quantum transport in ballistic conductors: Evolution from conductance quantization to resonant tunnelling. J. Phys. Condens. Matter 2000, 12, 9629. [Google Scholar] [CrossRef] [Green Version]
- Prinz, G.A. Spin-Polarized Transport. Phys. Today 1995, 48, 58. [Google Scholar] [CrossRef]
- Jedema, F.F.; Filip, A.T.; van Wees, B.J. Electrical spin injection and accumulation at room temperature in an all-metal mesoscopic spin valve. Nature 2001, 410, 345. [Google Scholar] [CrossRef]
- Maekawa, S.; Shinjo, T. (Eds.) Spin Dependent Transport in Magnetic Nanostructures; Taylor & Francis: London, UK, 2002. [Google Scholar]
- Nikolić, B.K.; Souma, S. Decoherence of transported spin in multichannel spin-orbit-coupled spintronic devices: Scattering approach to spin-density matrix from the ballistic to the localized regime. Phys. Rev. B 2005, 71, 195328. [Google Scholar] [CrossRef] [Green Version]
- Farokhnezhad, M.; Esmaeilzadeh, M.; Ahmadi, S.; Pournaghavi, N. Controllable spin polarization and spin filtering in a zigzag silicene nanoribbon. J. Appl. Phys. 2015, 117, 173913. [Google Scholar] [CrossRef]
- Sun, Q.-F.; Guo, A.-M. Enhanced spin-polarized transport through DNA double helix by gate voltage. Phys. Rev. B 2012, 86, 035424. [Google Scholar]
- Martinez, D.F.; Molina, R.A.; Hu, B. Length-dependent oscillations in the dc conductance of laser-driven quantum wires. Phys. Rev. B 2008, 78, 045428. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Koley, A.; Maiti, S.K.; Ojeda Silva, J.H.; Laroze, D. Spin Dependent Transport through Driven Magnetic System with Aubry-Andre-Harper Modulation. Appl. Sci. 2021, 11, 2309. https://doi.org/10.3390/app11052309
Koley A, Maiti SK, Ojeda Silva JH, Laroze D. Spin Dependent Transport through Driven Magnetic System with Aubry-Andre-Harper Modulation. Applied Sciences. 2021; 11(5):2309. https://doi.org/10.3390/app11052309
Chicago/Turabian StyleKoley, Arpita, Santanu K. Maiti, Judith Helena Ojeda Silva, and David Laroze. 2021. "Spin Dependent Transport through Driven Magnetic System with Aubry-Andre-Harper Modulation" Applied Sciences 11, no. 5: 2309. https://doi.org/10.3390/app11052309







