The Influence of Automated Machining Strategy on Geometric Deviations of Machined Surfaces

Abstract

This paper deals with various automated milling strategies and their influence on the accuracy of produced parts. Among the most important factors for surface quality is the automated milling strategy. Milling strategies were generated from two different programs, CAM system SolidCAM, with the help of workshop programming in the control system Heidenhain TNC 426. In the first step, simulations of different toolpaths were conducted. Using geometric tolerance is becoming increasingly important in robotized production, but its proper application requires a deeper understanding. This article presents the measurement of selected planes of robotized production to evaluate their flatness, parallelism and perpendicularity deviations after milling on the coordinate measuring machine Carl Zeiss Contura G2. Total average deviations, including all geometric tolerances, were 0.020 mm for SolidCAM and 0.016 mm for Heidenhain TNC 426. The result is significantly affected by the flatness of measured planes, where the overlap parameter of the tools has a significant impact on the flatness of the surface. With interchangeable cutter plate tools, it is better to use higher overlap to achieve better flatness. There is a significant difference in production time, with SolidCAM 25 min and 30 s, and Heidenhain 48 min and 19 s. In accordance with these findings, the SolidCAM system is more suitable for production.

1. Introduction

Industrial production has been accompanied by the development and innovation of manufactured products and manufacturing technologies with the primary aim of overcoming the implemented limits for the whole history. Due to this fact, innovations are an important aspect of competitiveness, which leads to the cycle of acquiring and implementing new knowledge in the practice [1]. Today’s trend is to increase productivity and reduce machining costs [2].

Although a level of automation has been achieved in the process planning of 3-axis milling, the present implementation can be improved with the incorporation of some added functionalities. Appropriate tool selection is needed to determine the optimal tool sequences and to adapt the automated feed/speed selection [3]. This technology is increasingly required in practice in automated or robotic form, where computer-assisted technology is increasingly used. These modern technologies are used in various branches of development and production [4].

The importance of the implementation of CAD CAM systems is often described in the literature. The basic sign of CAM is to minimize human intervention in the course of the production process by the exploitation of computer data processed in the main elements of activities [5].

When machining parts, we encounter several factors, the result of which is a lack of precision of parts. These are factors resulting from the CAM system that are caused by the approximation toolpaths. We can also define an error as any deviation in the actual position of the cutting edge of the cutter from the position that was theoretically programmed for the production of the part within the required tolerance [6].

At present, there is an increasing demand for higher dimensional and geometric accuracy and surface treatment of manufactured parts in various areas of the industry. Among the industries that require tighter tolerances to be achieved, typical examples constitute the automotive, aerospace and medical devices industries, including manufacturing trends such as automatic assembly and micro manufacturing. In this regard, realization of the desired part accuracy imposes criteria in the selection of manufacturing systems, e.g., machine tools. Part accuracy is closely related to machining system capability, which in turn is determined by the interaction between machine tool and cutting processes. These are mainly interfaces between the tool and the workpiece, where positional, static, thermal and dynamic accuracy has a significant effect [7]. The manufacturing of high-accuracy parts sets the basic requirement of a robust machining system able to manufacture within the specified tolerances. Although a controlled and repeatable process is important for maintaining quality, machining system accuracy and precision have a determinant role in part accuracy.

Among the challenges that machine and device parts producers face nowadays is the necessity to guarantee a high quality of machined surfaces. Obtaining a high-quality product is an extremely difficult and complex task that entails fulfilling several crucial requirements [8]. The machining parameters are an important part of process plan performance. Equally important in machining is the confidence in the measuring tools, from which part quality characteristics are ascertained [9]. Due to the importance of the finishing stage in the manufacturing processes, the face milling process is the solution that can be used to achieve good surface quality and high accuracy in a short period of time. To achieve the high quality of the desired parts, studying the tool path strategies is inevitable too.

Surface properties constitute a significant measure of the finished component quality, because they influence features such as dimensional accuracy, friction coefficient, wear, post processing requirements, appearance and cost. The quality of the surface produced by milling depends on various technological parameters, such as the cutting conditions as well as the cutting tool and the workpiece specification [10].

Lopez de Lacalle et al. [11] describe the stages of preparation of the milling process and factors impacting the optimization of cutting strategies.

To develop an effective milling process, the programmer must choose the right strategies. Appropriate selection of the tool path is important because it defines the axial depth of cut, which influences the maximum cutting force during machining. Its correct definition controls the overall productivity of the machining process and can usually be influenced by computer production systems. Likewise, the method of milling up or down together with the direction of rotation of the spindle also controls the direction of chip removal [12,13]. When choosing a milling strategy, various factors must be considered, for example, surface roughness or form deviation. The geometry of the tool-related parameters (feed and cutting speed) is equally essential to achieve quality after machining [14].

Among the most used strategies in the production of parts by milling is strategy zigzag, and contour, which we can define using a computer-aided manufacturing system [15]. The zigzag strategy, known as the raster strategy of milling, is a strategy in which the milling cutter moves back and forth along the workpiece in the X-Y plane. In the machining process, we may encounter another problem, such as the generation of toolpaths, which affects not only the performance of the production process but also the quality of machined surfaces [8]. The machining strategy is the methodology used to calculate the operation, where the purpose is to obtain the desired geometric entity in its final form [16]. The efficiency of using a particular toolpath is related to the time required to complete the machining process. The lower limit of the total cutting time for a 2D area is the area divided by the cutting width and the cutting feed rate [17]. Part of the production process is a postprocessor, which provides information about the position of the tool and process parameters [18].

An inseparable part of each manufacturing process is its control. The assessment of manufacturing correctness allows us to define the compatibility between the manufactured product and design requirements. It also allows us to verify the correctness of operation of stages of the manufacturing process [19,20]. Depending on expectations, one can choose the measuring devices which can give metrologically correct results for required time limits. These requirements apply to all control processes connected with the measurement of mass, time or geometrical quantities [21].

In industry, dimensional and geometric accuracy is checked after the end of the production process. A widely used machines for manufacturing process control is the coordinate measuring machine (CMM) [21]. The increasing importance of geometric tolerances points out many questions related to the mathematical method of evaluation, the point sampling strategies in CMM and the manufacturing possibilities and constraints.

Among the most used basic elements/features of a mechanical part are planar elements. The relations between features are defined by dimensions and geometric tolerances, such as flatness, parallelism, perpendicularity and straightness. During the production of the part, dimensional and form deviations from the prescribed value are present [22].

The most used form of deviation for controlling the milling process and checking the machine tools is the flatness [8]. A key issue in flatness measurements is the definition of an appropriate reference plane [16]. Ideally, all points of the measured plane are in one plane [23].

The different types of tolerances show the allowed errors. Considering the errors, the most often used tolerances are the dimensional tolerance, the surface roughness, the shape, position and orientation tolerances (geometric tolerances).

Mikó et al. [24] investigate the dependency between roughness and flatness measured by 12 methods of point sampling strategies. The results show that the evaluated parameters are dependent.

Hazarika et al. [25] studied the influence of surface roughness on parallelism and perpendicularity in milling. The results from the experiment show that surface roughness affects the parallelism and perpendicularity errors, thus confirming the results of Mikó [24].

Mikó and Farkas [26] state the impact of milling machines (milling machine and CNC machining center) on flatness and surface roughness. The results are represented with values of parameters and with the topographical distribution of deviations.

Gapinski et al. [27] evaluate dimensions and geometric tolerances on different types of measuring machines—optical, roundscan, contour measuring machine, CMM and computed tomography. The results clearly show the strengths and weaknesses of measuring devices. The most versatile device is the coordinate measuring machine.

Modern CNC machines or new cutting tool designs provide many possibilities for improving the accuracy of the workpiece surface. In practice, we often encounter the optimization of the machining process, in which, for example, by changing the depth of cut, changing the feed rate, or analyzing the orientation of the workpiece, it is possible to achieve a change in the flatness of the part [28].

Dobrzynski et al. [29] present in their research the impact of machining direction on surface flatness. The result show that length of the face milled section affects the values of flatness deviations; the flatness deviation grows with machining length.

Various contributions examining the flatness of machined surfaces after milling have proven their justification in choosing the right machining strategy for surface quality [30].

Sheth and George [31] investigate the effect of machining parameters on surface roughness and flatness. For the prediction of machining parameters, the results were analyzed by ANOVA. The error of predicted surface roughness was up to 11% and flatness 7%.

Gapinski et al. [32] evaluate the suitability of a coordinate measuring machine to measure the circle radius, position of the center and roundness. For element creation, different fitting methods and numbers of measuring points were used. The authors state that the uncertainty of CMM is the limiting factor.

There are always certain differences between the designed and realized properties that occur during the production process. They are caused by changes in various parameters, such as the machine tool, material, human factors, measuring devices or process parameters. The number of factors affecting the deviation can be reduced by quality control and by the planning of the production process. The acceptable level of deviations is defined as the tolerance of dimensions and geometry. During the measurement with a coordinate measuring machine or any other suitable measuring device, the measuring strategy defines the number of recorded points and the created element [33].

Mikó and Drégelyi-Kiss [34], in three types of milling machines, investigated flatness, perpendicularity and parallelism. The results show that the cutting tool and machine rigidity affect the values of form and shape errors, and the flatness of vertical and horizontal planes is affected by the depth of cut. Additionally, the machine tool, the direction of milling and the position of the surface also affect geometric errors.

Lakota and Görög [35], in their research, analyze the measurement of flatness with seven single- and multi-point measuring methods. The results show that the value of flatness is also affected by the orientation of the profile lines with respect to the machining path and data filtration.

Kawalec and Magdziak [36] investigate the influence of the number of measurement points on the accuracy of the CNC machine tool. The numerical investigation uses from 4 to 200 points, and the results show that the minimum number of points to achieve the minimum deviation is 50.

Mikó [37] presents the factors affecting the measurement of flatness (machining method, strategy, milling parameters, etc.) and the influence of the number of measuring points. The results show that the flatness values are in the range from 0.124–0.0572 mm.

Nowakowski et al. [38] present, in their research, the impact of the depth of cut on surface flatness. Results show the flatness deviation was 6.7 µm between the cutting strategies. The maximum deviation was reported at the beginning and end of the cutting process.

The milling strategy, known as raster, and the use of offset paths, as claimed by Sarma [17], have their advantages and disadvantages. When milling with raster, a shorter toolpath is created, but it is not possible to completely remove traces known as scallop height. By contrast, using a strategy known as offset, it is possible to remove the remaining scallop height to obtain a smooth surface. Toh [39] also described a comprehensive overview of the most common strategies used in the machining process, offset and zig-zag, in his publications.

The aim of the research, conducted by the authors of this work, was to provide the result of a comparison of milling strategies in two different programs and evaluated geometric characteristics of the produced test specimens. The article presents the results of the evaluation of the deviation of flatness, perpendicularity and parallelism. In two different programs, two parts were produced with the same cutting conditions. The main difference was only in the overlap of the tool. The question is the following: What is the relationship among the influence of the program, the control system of the accuracy of part production (dimensional accuracy) and tool overlap in selected geometric deviations? As part of our results, the flatness was determined based on 1945 points by least square (LS). The aim of the article was to verify the effect of the combination of the tools for finishing operations and their overlap.

2. Plan of Experiment

2.1. Materials

In this work, two identical workpieces were produced. The 1st design of the test sample was created in the CAD system SolidWorks and the 2nd in the control system Heidenhain TNC 426. Stock dimensions were programmed in the control system Heidenhain TNC 426 at the beginning of the program in line with stock marked as command BLK FORM.

Test samples were made of aluminum alloy (AlCu4Mg); the dimensions are shown in Figure 1.

Table 1. Chemical composition of AlCu4Mg alloy [17].

Chemical Composition	Content [%]
Cu	4.30
Mg	0.79
Fe	0.26
Si	0.24
Mn	0.3
Ti	0.04
Zn	0.04
Al	balance

lists the chemical composition of the aluminum alloy. The selected mechanical properties of AlCu4Mg are the following: tensile strength = 105.8 MPa; yield strength = 92.4 MPa; hardness = 73.5 BHN.

2.2. Machining Device, Cutting Tools and Cutting Parameters

The machining of aluminum parts was carried out on the 3-axis milling machine EMCO Concept Mill 155 (EMCO MAIER Ges.m.b.H., Hallein/AUSTRIA) with control system Heidenhain TNC 426 (JOHANNES HEIDENHAIN GmbH, Germany). CAM software today offers several possibilities of strategies for distributing the tool path in the domain of the designed part.

Table 2. Cutting tools and cutting parameters for milling of AlCu4Mg alloy.

Tool Diameter [mm]	Cutting Speed [m·min−1]	Feed per Tooth [mm]	Spindle Frequency [RPM]	Tool Producer	Tool Code
End Mill D 18	270	0.125	4800	Korloy	AMS2018S
End Mill D 14	299	0.021	4800	ZPS-FN	120517
End Mill D 10	232	0.03	4800	ZPS-FN	270618
End Mill D 6	259	0.03	4600	ZPS-FN	273618

shows a list of cutting tools and cutting parameters for milling of the AlCu4Mg alloy. The methodology for selecting cutting parameters was chosen based on the recommendations of the tool manufacturer and concerning the parameters of the CNC machine. The maximum RPM of the machine EMCO MILL 155 was 5000. The tool of Ø18 mm in diameter was produced by Korloy (designation AMS 2018S) with two interchangeable cutters plates marked APXT11T3PDR-MA. The other tools were produced by ZPS-FN.

The manufacturing process of the part was performed by the face milling, roughing and finishing strategies (Figure 2). For both produced test samples, the same roughing and finishing strategies were used. The coordinate system defined for the milling process is shown in Figure 1 (blue cross). The production of samples is described in the following steps:

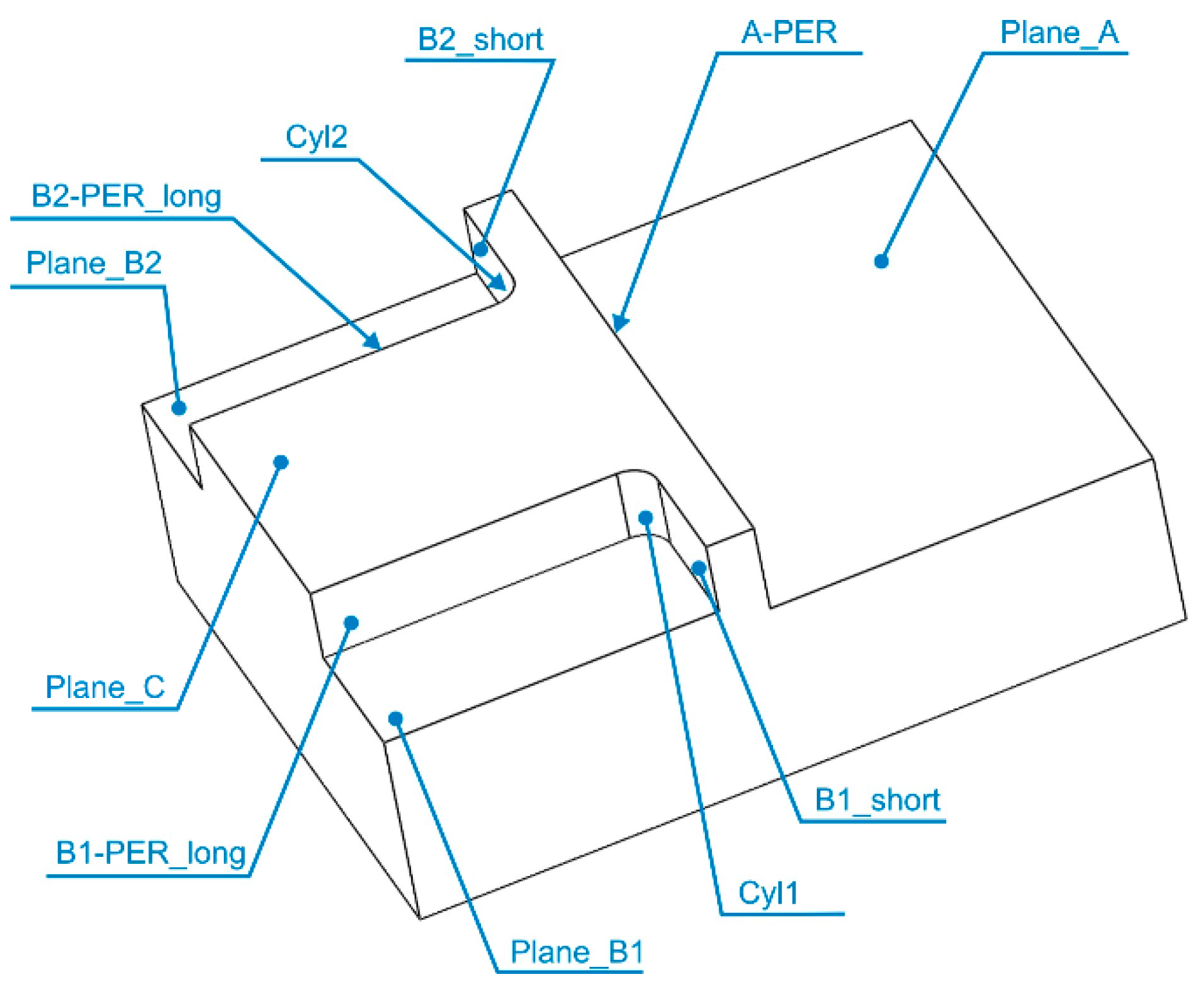
• Face milling of the top surface marked Plane C, in Figure 3, where the strategy zig-zag was used.
• Milling (roughing) a flat side surface marked Plane A, A-PER, where strategy contour with offset was used. After this operation, an allowance of 0.3 mm was left, which was removed by a tool with a smaller diameter.
• Milling (roughing) a two-sided surface marked Plane B1, B1-PER_long, Cyl1, B1_short, Plane B2, B2-PER_long, Cyl2, B2_short. After this operation, the allowance of 0.3 mm was left too and was removed by a tool with a smaller diameter.

For production two different programs were used. The first was the CAM system Solidcam and the second was the control system Heidenhain TNC 426. The tool path of the tool for the milling of this part was programmed manually in the control system Heidenhain TNC 426. For the produced test samples, both programs used the same strategies and the same cutting parameters. The shape of parts was obtained in two stages: roughing and finishing. After each roughing operation, an allowance of 0.3 mm and a depth of cut for each operation of ap = 1.5 mm were implemented on the surface.

We compared two different programs, where the strategies were programmed, and their effect on the quality of the surface of test samples was evaluated. Surface quality is influenced by several process parameters, such as tool diameter, cutting parameters, work piece material properties, clamping of the part and milling strategy. Among the most important factors is the milling strategy, which influences produced part accuracy.

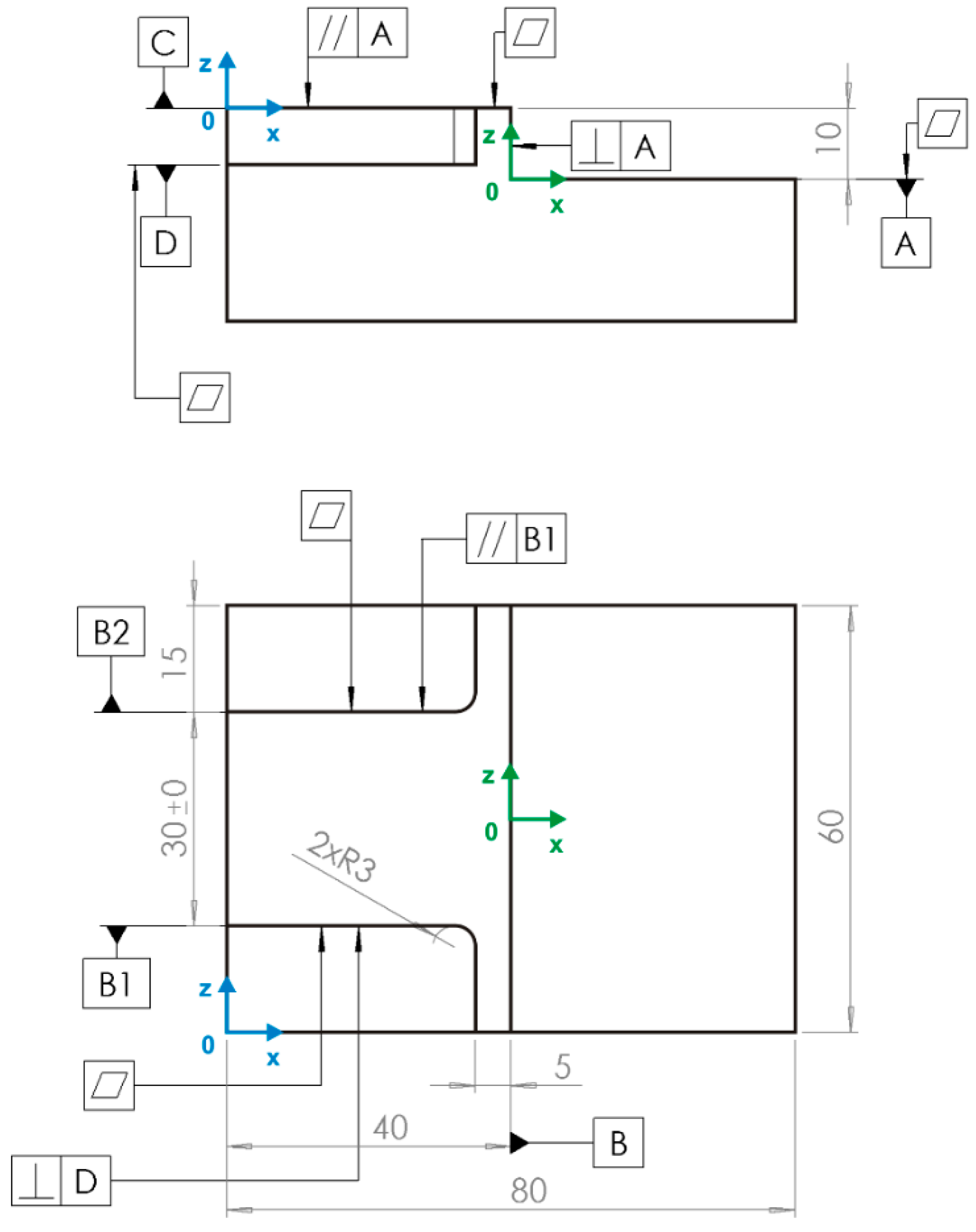
2.3. Programming NC Program

HEIDENHAIN TNC uses programmable controls that allow the user to define paths for creating contours and pockets for drilling operations, etc. It is possible to program these conventional operations directly on the machine by defining cycles. It is also possible to change the angular position of the spindle, which also corresponds to the definition of certain cycles. In this case, it is a simple creation of programs, where the graphics illustrate all the steps of the machining process of the selected part.

System SolidCAM, on the other hand, is an additional CAD model of the Solidworks system. This computer aided manufacture system offers solutions for all CNC applications. In our case, the solution used was known as 2.5D Milling.

3. Measurement of Geometrical Characteristics

The most popular method of geometrical dimension control is the coordinate measuring technique. This measurement strategy plays an important role in the accuracy of obtained results [23,24]. The number of points collected during the measurement, the calculation of geometric element, filtering and outlier eliminations are the most influencing factors. The setting of these parameters is mostly left to the operator, and significantly affects the value of the flatness and the associated uncertainty [13]. The measurement of surface quality obtained from the CAM system SolidCAM and control system Heidenhain TNC 426 was conducted. After that, geometric characteristics of the produced part were measured with the use of the coordinate measuring machine Carl Zeiss Contura G2 with an RDS articulating probe holder and VAST XXT scanning sensor. The maximum permissible error (MPE_E) of machine was calculated according to ISO 10360-2, where L is the measured length dimension in mm.

$$MP{E}_E=\left(1.8+\frac{L}{300}\right)\left(\mathsf{\mu }\mathrm{m}\right)$$

(1)

The elements used in the measurement are defined in Figure 3.

Dimension and geometric tolerance evaluation was performed with a coordinate base system consisting of PlaneA (Spatial rotation, Z origin), the PER-A plane (X origin) and the symmetry of the B1-PER_long and B2-PER_long planes (Planar rotation, Y origin). The origin is shown in Figure 1 (green color). In the analysis of production, one length dimension in four ways and 20 geometric tolerances were evaluated. The measured surfaces (Figure 3) describe their identification, surface types and deviations. Two styli were used for the measurement of test samples.

The 1st stylus was used for the measurement of all dimensions and geometrical tolerances except cylindricity. The probe diameter = 1 mm, and the length of stylus = 20 mm. For the measurement, a 50 mm extension was used, because the minimum recommended stylus length is 40 mm. The 2nd stylus with a diameter of 3 mm and length of 50 mm was used for measurement of cylindricity.

4. Evaluations of Geometrical Characteristics

The deviation results obtained by CMM for individual test samples are shown in

Table 3. Coordinate measuring machine (CMM) results.

Name	Deviation SolidCam [mm]	Deviation Heidenhain [mm]
FLT_Plane_A	0.0079	0.0085
FLT_Plane_C	0.0406	0.0139
PAR_C_pd_A	0.0389	0.0379
FLT_Plane_B1	0.0210	0.0093
FLT_Plane_B2	0.0233	0.0106
FLT_A-PER	0.0056	0.0107
PER_A_per	0.0092	0.0159
Distance_LSQ_1X_Y	−0.2299	−0.2056
Distance_LSQ_4X_Y	−0.2179	−0.2486
Distance_LSQ_1Z_Y	−0.2400	−0.2471
Distance_LSQ_10Z_Y	−0.2455	−0.2477
FLT_B1-PER_long	0.0315	0.0156
FLT_B2-PER_long	0.0167	0.0256
PAR_B1_per-long_1	0.0063	0.0045
PAR_B1_per-long_2	0.0081	0.0141
PAR_B1_per-long_3	0.0152	0.0127
PAR_B1_per-long_4	0.0054	0.0126
PER_Plane_B1_PER_long_pd_PER	0.0355	0.0266
PER_B1_short_pd_B1	0.0362	0.0431
PER_B2_short_pd_B2	0.0395	0.0463
PER_Cyl1_Par_to_Plane_A_PER	0.0167	0.0054
PER_Cyl1_Per_to_Plane_A_PER	0.0131	0.0146
PER_Cyl2_Par_to_Plane_A_PER	0.0116	0.0154
PER_Cyl2_Per_to_Plane_A_PER	0.0012	0.0035

. All data are given as deviations from the nominal value. The nominal values for dimension and geometrical tolerances are shown in Figure 1.

According to the same cutting parameters for the parts produced in the CAM system and in the control system Heidenhain TNC 426, the evaluation was divided into six groups:

• Flatness—horizontal planes;
• Flatness—vertical planes;
• Perpendicularity—planes;
• Perpendicularity—cylinders;
• Parallelism;
• Distance.

For the comparison of production, graphs were created in accordance with separate groups, which include the comparison characteristics for both systems. The green color represents SolidCAM, and blue represents the control system Heidenhain TNC 426. The results of flatness measured on the horizontal planes are shown in Figure 4.

Results of the deviations in three characteristics are higher for SolidCAM than for Heidenhain TNC 426. The smaller deviation is only by 0.6 µm of Plane_A. The surface flatness is marked as Plane_C for both systems, SolidCAM and Heidenhain TNC 426 (Figure 5).

The flatness was measured at a speed of 8 mm/s along a defined path (polyline) without data filtration and outlier elimination. In both cases, the scale was over 1000: 1. The maximum and minimum values of the flatness deviation are marked with red marks. In the case of the Heidenhain system, the process of deviations had no significant differences compared to the SolidCAM system. In both cases, the trajectory of the cutting tool was clearly visible (decrease in the values of deviations in one line).

When comparing the two systems, the difference in the deviations of the flatness can be observed. In both systems, the trajectory of the cutting tool was visible (see Figure 5). In the CAM system, SolidCAM, where the tool overlap was 50%, the trajectory of the cutting tool was clearly visible, marked with lines, but in the Heidenhain control system, where a tool overlap of 70% was used, the height difference was slight. The Heidenhain control system showed a more even distribution of flatness deviations, which can be explained by the overlapping of the tool during the milling process. The denser overlap of the toolpaths during machining increased the number of passes to machine the surface, which increased the milling time, but the surface showed better results in terms of flatness. The comparison between flatness and vertical planes is shown in Figure 6. Flatness measurement tolerances were measured at a speed of 8 mm/s along a defined path (polyline), and a spline filter with wavelength Lc = 2.5 mm was used (roughness Ra = 0.4–3.2 μm).

In two cases, flatness was better for the CAM system SolidCAM and in one case for Heidenhain TNC 426. After all flatness deviations were compared, globally smaller deviations for Heidenhain TNC 426 were found in four cases and for system SolidCAM in three cases. In the case of the evaluation of perpendicularity, it was necessary to define the reference plane to which the given tolerance would be evaluated.

Table 4. Evaluated geometric tolerances and reference planes for the perpendicularity of the planes.

	Evaluated Plane	Reference Plane
PER_A_per	A-PER	Plane_A
PER_Plane_B1_PER_long_pd_PER	B1-PER_long	Plane_A
PER_B1_short_pd_B1	B1_short	Plane_B1
PER_B1_short_pd_B2	B2_short	Plane_B2

describes the evaluated geometric tolerances and reference planes for the perpendicularity of the planes. All perpendicularity tolerances were measured at a speed of 8 mm/s along a defined path (polyline) and a spline filter with wavelength Lc = 2.5 mm.

The evaluation of the perpendicularity of the planes showed that the plane PER_A_per had the lowest perpendicular deviation, and planes B1_short a B2_short had the highest. Three deviations of four SolidCAM systems had a smaller deviation. The difference between systems SolidCAM and Heidenhain TNC 426 was less than 0.01 mm (Figure 7).

The perpendicularity of cylinders was evaluated in two methods: as the perpendicularity of the cylinder to the primary reference plane Plane_A in parallel direction to the secondary reference plane A-PER and as the perpendicularity of the cylinder to the primary reference plane Plane_A in the perpendicular direction to the secondary reference plane A-PER. In three cases, the deviation (Figure 8) was smaller for the SolidCAM system, although only minimally (maximum difference was 0.0038 mm).

The parameters of plane parallelism evaluations are shown in

Table 5. Parameters of planes parallelism evaluations.

	Evaluated Plane	Reference Plane
PAR_C_pd_A	Plane_C	Plane_A
PAR_B1_per-long_1	B2-PER_long	B1-PER_long
PAR_B1_per-long_2	B2-PER_long	B1-PER_long
PAR_B1_per-long_3	B2-PER_long	B1-PER_long
PAR_B1_per-long_4	B2-PER_long	B1-PER_long

. The parallelism was measured at a speed of 8 mm/s along a defined path (polyline), and a spline filter with wavelength Lc = 2.5 mm (roughness Ra = 0.4–3.2 μm) was used.

The parallelism deviation of the two horizontal planes (PAR_C_pd_A) showed a significantly higher value than area B2_long to area B1_long. The plane PAR_C_pd_A was measured by the polyline path. The main difference was in the form of a deviation of parallelism. The SolidCAM system showed larger differences than the Heidenhain system.

The parallelism of PAR_B1_per-long was measured using 4 lines. The parallelism of each line from plane B2_long to plane B1_long was evaluated. In two cases, the SolidCAM system had a smaller deviation (Figure 9), and in two cases, it was Heidenhain TNC 426. The maximum difference was less than 0.0072 mm. When comparing the two systems on the PAR_C_pd_A plane, no significant difference in parallel deviations or in their distribution was visible (Figure 10). With the CAM system SOLIDCAM, where the tool overlap was 50%, a more pronounced tool path on the surface could be seen than in the Heidenhain system, where the tool overlap was 70%.

When comparing the two systems, the difference in deviations of the parallelism could be seen. In the CAM system SolidCAM, where the tool overlap was 50%, a greater surface waviness was observed than in the case of the Heidenhain system, where the tool overlap was 70%. The Heidenhain control system showed a lower surface waviness of the measured deviations of parallelism compared to the CAM system SolidCAM, where the surface was machined earlier, but the deviations of parallelism were larger in this case. This is demonstrated by the greater surface waviness of the machined surface.

One dimension on the part was evaluated, namely, the distance between planes B1_long and B2_long. The LSQ Featured method was used for measurement. When measuring Distance_LSQ_1X_Y and Distance_LSQ_4X_Y, 4 horizontal lines were created on the given surfaces. The distance between them in the y-axis was evaluated. When measuring Distance_LSQ_1Z_Y and Distance_LSQ_10Z_Y, 10 vertical lines were created on the given surfaces. The results are shown in Figure 11.

The difference between results for measuring horizontal lines was minimal, and for perpendicular lines, the difference was close to zero.

5. Results

Based on the measurement of the defined elements performed on a coordinate measuring machine, values were obtained for the prescribed dimensions and geometric tolerances of the designed test part. The obtained results led to the following conclusions:

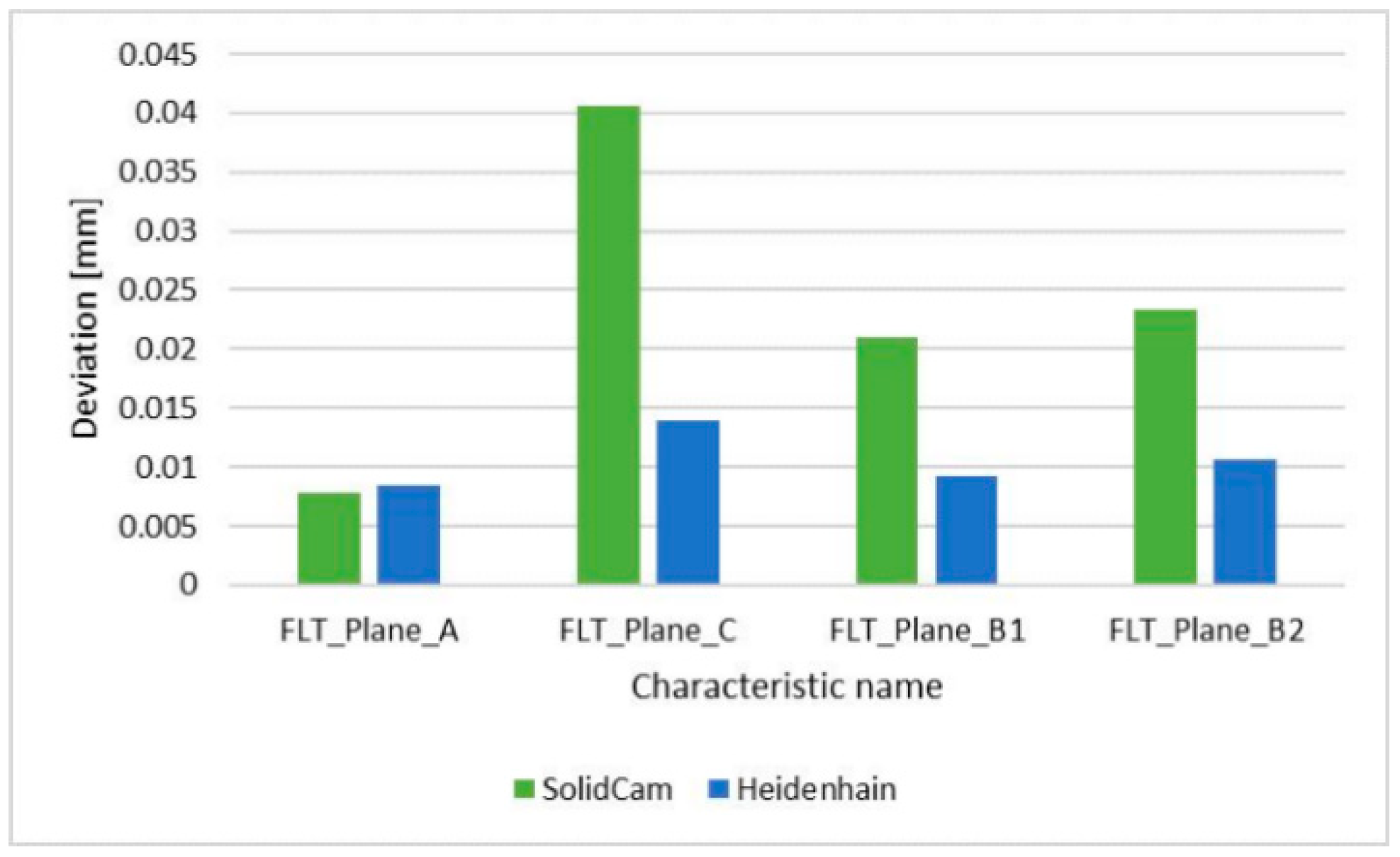
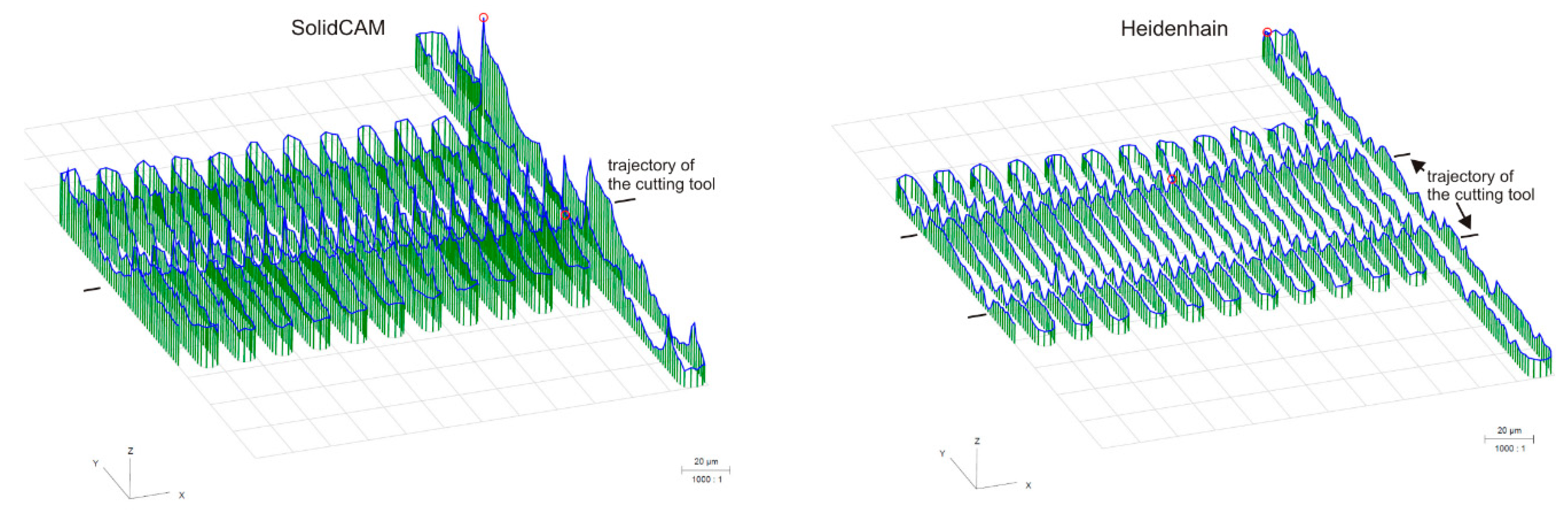
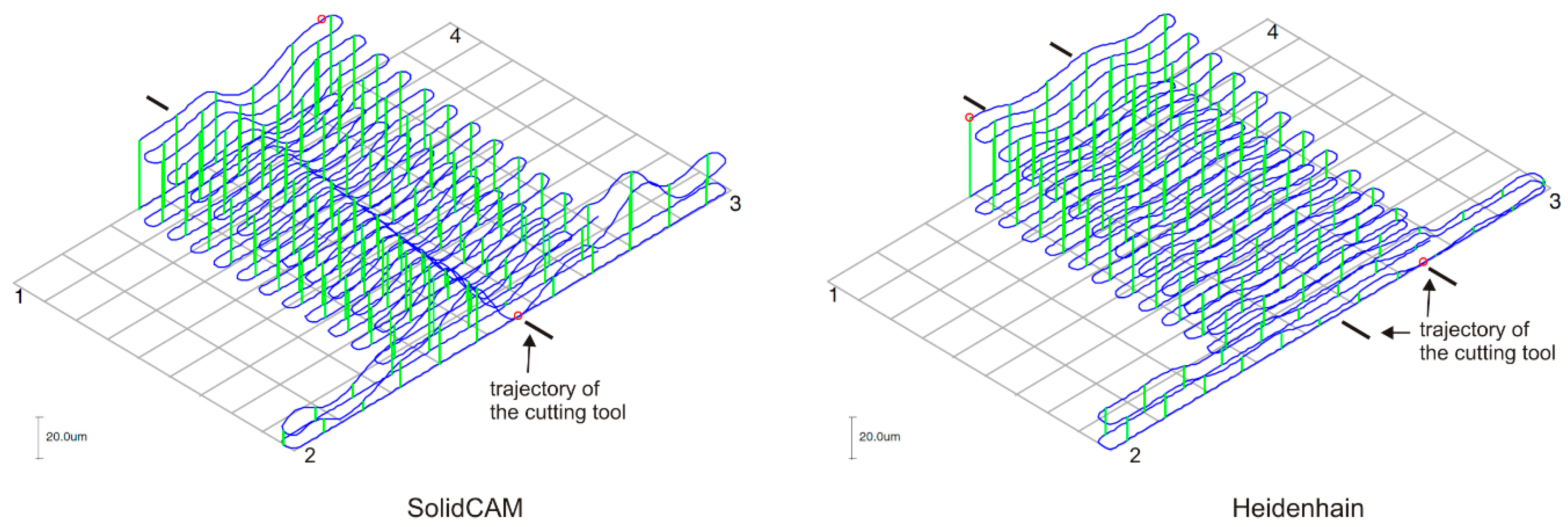
• The flatness comparison of the surfaces showed that the average flatness value for all surfaces was 0.023 mm for SolidCAM and 0.011 mm for Heidenhain. Average deviations in CAM system were significantly influenced by the flatness of plane Plane_C, which was higher than in system Heidenhain TNC 426. The comparison of the two systems in Plane_C showed the difference in the height deviations of flatness. In the CAM system, SolidCAM, where the tool overlap was 50%, the trajectory of the cutting tool was clearly visible. In the control system Heidenhain, where a tool overlap of 70% was used, the trajectory of tool was still visible, but the deviations from ideal plane were smaller. The Heidenhain control system showed more even height differences, which can be explained by the overlapping of the tool only during the milling process. The denser overlap of the toolpaths during machining increased the number of passes to machine the surface, which increased the milling time, but the surface showed better results in terms of flatness.
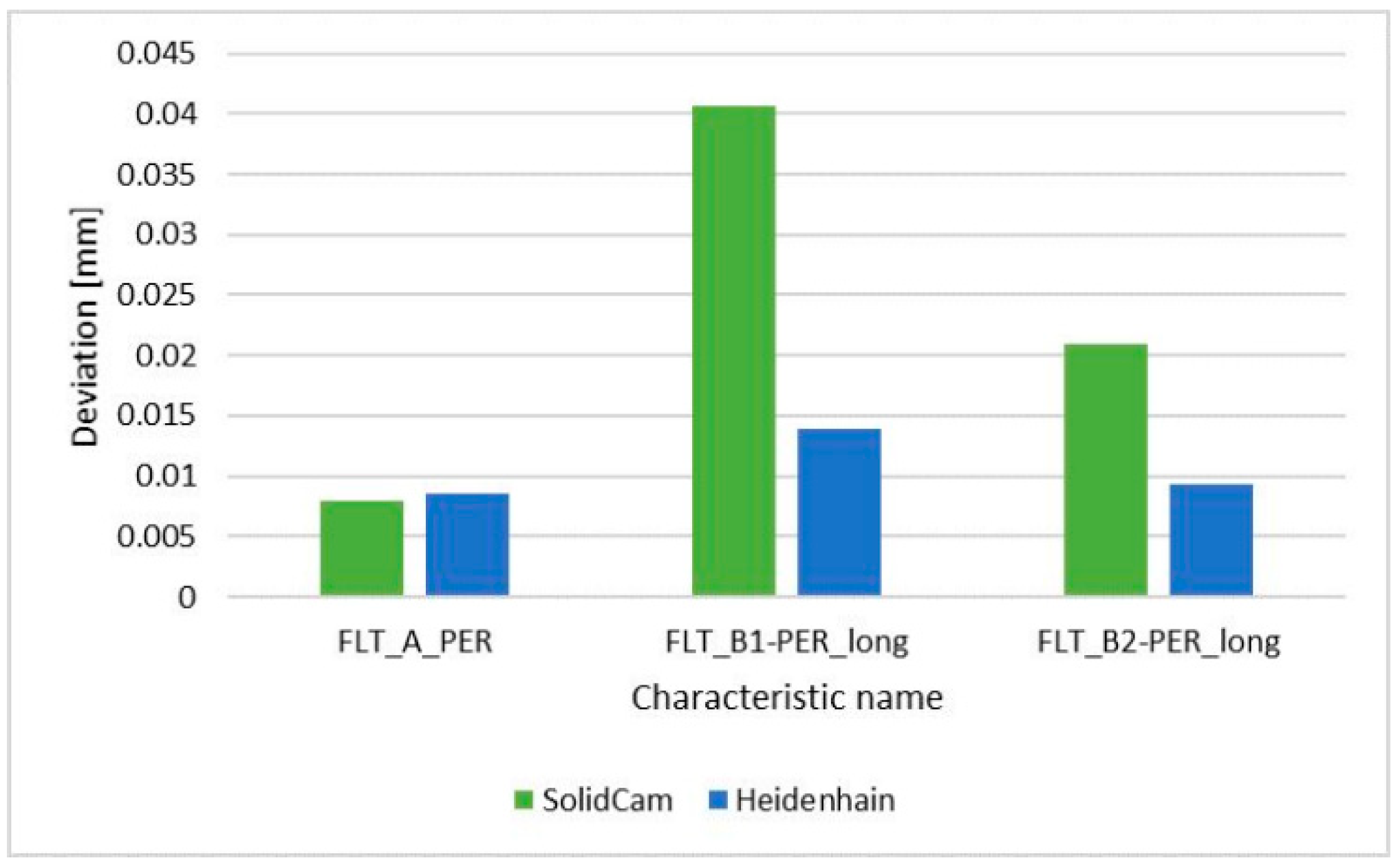
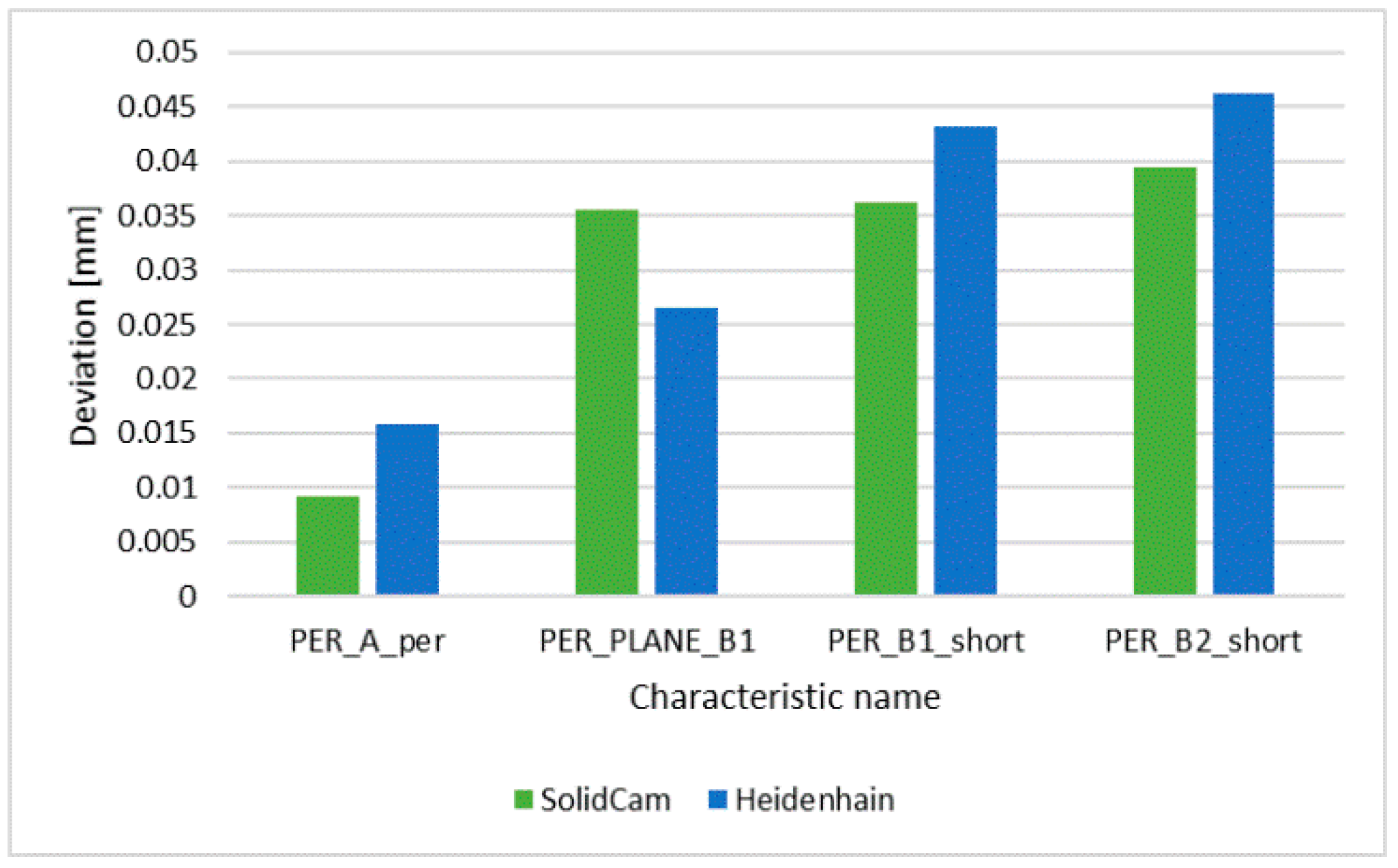
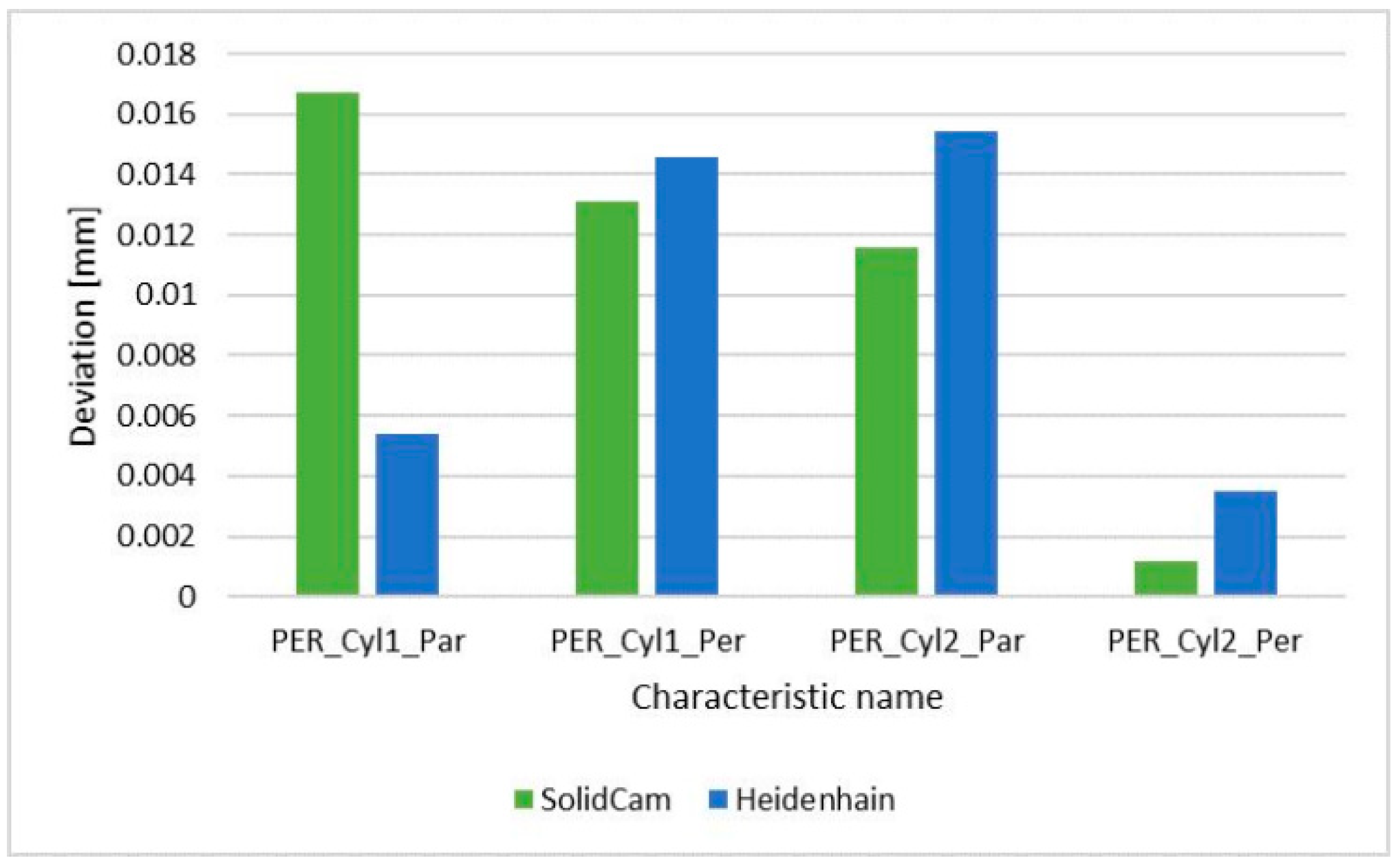
• The comparison of the geometric tolerances of perpendicularity showed that the average value for the SolidCAM system was 0.020 mm and for the Heidenhain TNC 426 system 0.020 mm, so there were no significant differences between them.
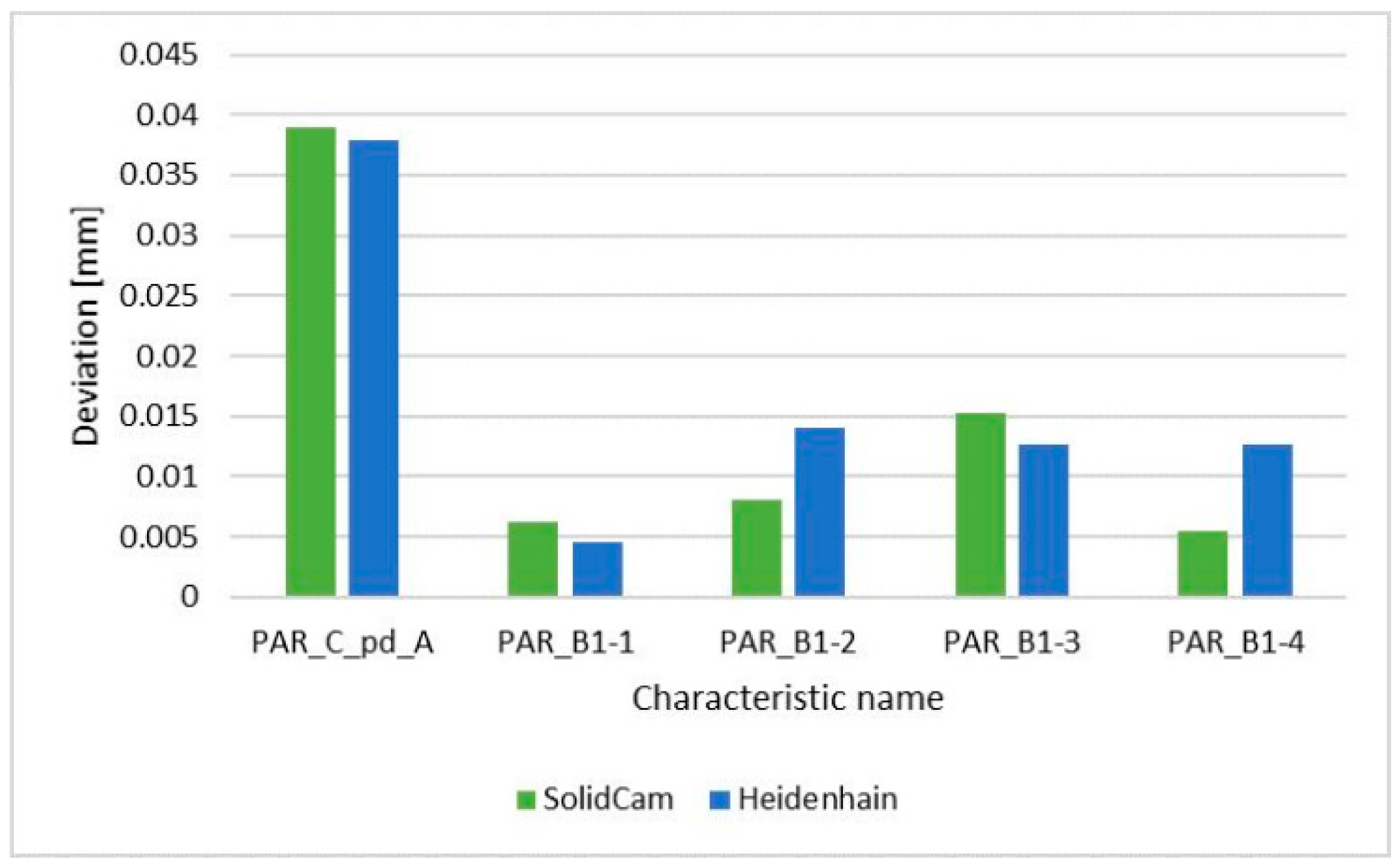
• The parallelism comparison in a global view also did not show a significant difference between the systems. For SolidCAM, the average deviation was 0.015 mm, and for Heidenhain, 0.016 mm. The comparison of the two systems in PAR_C_pd_A showed the difference in the distribution of deviations of parallelism. In the CAM system, SolidCAM, where the tool overlap was 50%, a greater surface waviness was observed than in the case of the Heidenhain system, where the tool overlap was 70%. The Heidenhain control system showed a lower surface waviness of the measured deviations of parallelism compared to the CAM system, SolidCAM, where the surface was machined earlier, but the deviations of parallelism were larger in this case. This was demonstrated by the greater surface waviness of the machined surface.
• The total average deviation, including all geometric tolerances, was 0.020 mm for SolidCAM and 0.016 mm for Heidenhain TNC 426. The result was significantly affected by flatness, where the SolidCAM system showed significantly higher values in all other comparisons than Heidenhain.
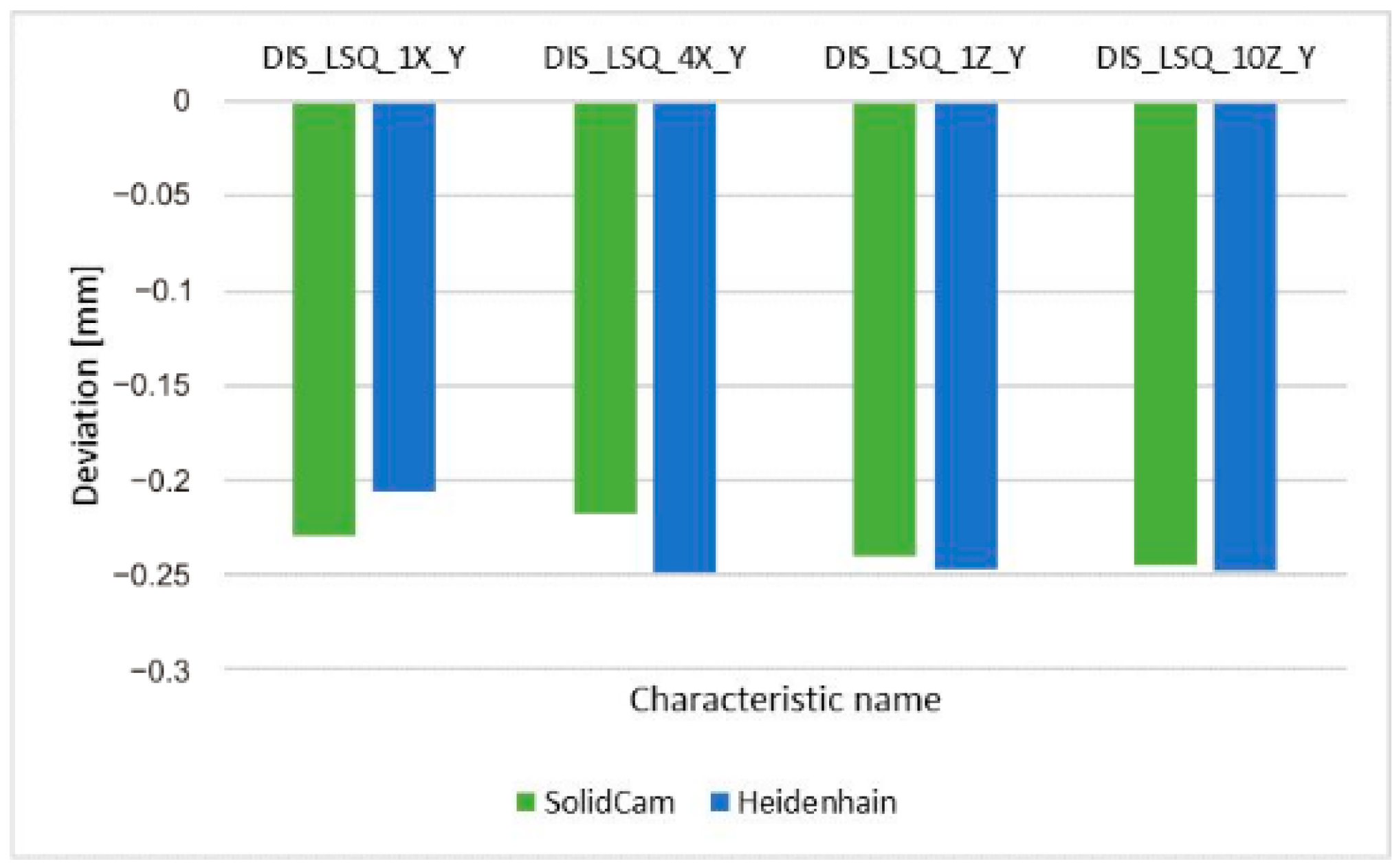
• For dimensional control, measurements between parallel surfaces B1_per-long and B2_per-long were realized. The average deviation for SolidCam was −0.233 mm, and for Heidenhain, −0.237 mm, so this was an inappreciable difference.
• There was a significant difference in production time, with SolidCAM 25 min and 30 s, and Heidenhain 48 min and 19 s. In accordance with these findings, the SolidCAM system is more suitable for production.

6. Conclusions

The paper deals with the production accuracy comparison of two different CNC programming systems. Results showed that the highest impact on accuracy is defined by tool overlap in the reference plane.

In the system comparison, the total sample production time was longer in the SolidCAM system than in Heidenhain TNC 426. The reason for this is that SolidCAM generated the tool path of the tool with the whole model (geometry, shape of the model, accuracy tolerance, etc.) in accordance with Heidenhain TNC 426, where to define the strategy, the system used concrete types of the cycles, which are included in this system.

According to the obtained results, the future plan is to provide further measurements of larger numbers of samples; select another material; and extend the measurements by other types of deviations, such as angularity, position and profile of a surface.

Therefore, it would also be interesting to monitor the impact of milling strategies on the roughness supported by measuring cutting forces.