Test Loading of Structures with a Suspect Resistance
Abstract
1. Introduction
2. Target Reliability for the Test Loading Calculations
3. Instantaneous Loading Versus the Service-Time Loading
4. Harmful Effects
- The structure must not give any noteworthy indication of cracking, such as a rupturing sound during the loading.
- The structure must stay mainly in the elastic state during the loading; i.e., the deflection must return to its unloaded state after the loading, and the load-deflection behavior must be linear.
- The test load should be adjusted to be as low as possible to satisfy the reliability demand. For example, it is feasible to utilize test loading for structures that are unlikely to carry the full load during the service time. In this case, test loading can be conducted for a lower load. If the actual load exceeds the test load, special measures will be taken. The authors have used this method to test load defective long-span roof girders. In this case, the critical load is a layer of snow. If the actual snow load exceeds the critical load, action can be taken to remove the snow in order to reduce the load. As the snow is removed directly when the critical limit is exceeded or close to that limit, the load-duration effect can be omitted.
- Only a part of the structure is test loaded. This method can be applied, for example, in a quality-assurance program.
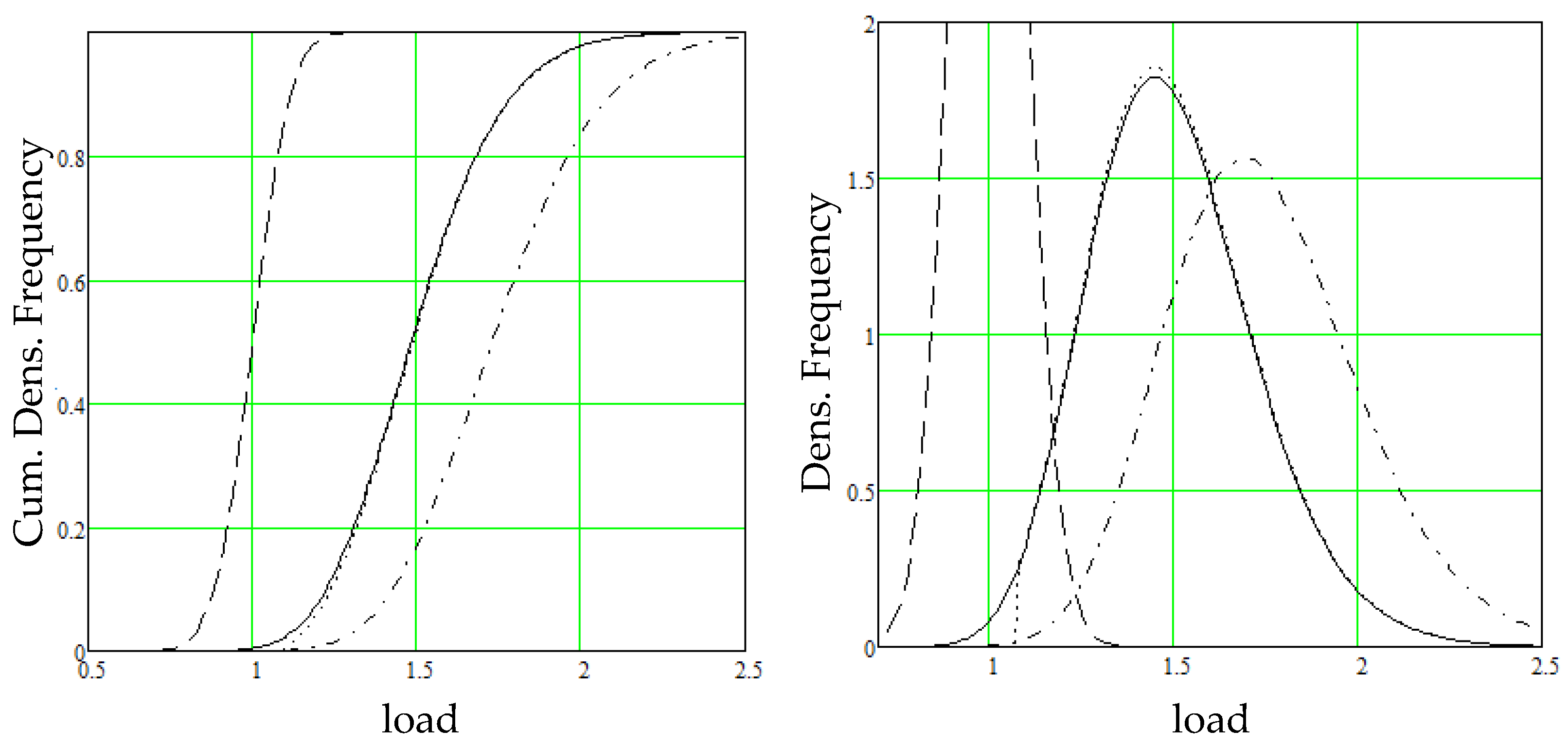
5. Choosing the Test Load
5.1. Approximate Method
5.2. Probability Method
5.2.1. Partial Factors vs. Design Values
5.2.2. Equations for Safety-Factor Calculation
5.2.3. Is the Test Loading Needed?
5.2.4. Equations for Test Loading Calculations
5.2.5. Test Loading with Known Resistance Distribution
5.2.6. Checking
6. Limitations
- Comprehensive instructions for test loading are lacking in the current codes.
- Test loading is an excess cost in comparison with the normal construction.
- Test loading constitutes an excess procedure requiring special apparatus and knowledge.
- The structure might fail in the test loading, which might cause a significant economic loss. On the other hand, if the structure fails in the actual use, the economic loss is normally much higher.
- Test loading is normally feasible in structures that have only one critical load case. Virtually all horizontal structures have one critical load case; namely, all simultaneous vertical loads, and these structures are normally suitable for test loading.
- Test loading may cause harmful effects in the structure. For example, in a concrete structure, cracks may occur even at a low overloading. On the other hand, most structures, such as timber and metal structures, resist minor overloads well.
7. Discussion
8. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- CEN. Draft prEN 1990:2020 Eurocode—Basis of Structural Design; CEN: Brussels, Belgium, 2020. [Google Scholar]
- CEN. Eurocode 7—Geotechnical Design. Part 1: General Rules; CEN: Brussels, Belgium, 2014. [Google Scholar]
- CEN. EN 1537 Execution of Special Geotechnical Works—Ground Anchors; CEN: Brussels, Belgium, 2013. [Google Scholar]
- James Jones & Sons Ltd has Launched World Leading I-Joist Production Line. Available online: https://vimeo.com/391954916 (accessed on 26 March 2021).
- Open Joist Triforce Built by Barrette. Available online: https://www.youtube.com/watch?v=K5WlA2sqF1U (accessed on 26 March 2021).
- CEN. EN ISO 22477-5 Geotechnical Investigation and Testing-Testing of Geotechnical Structures—Part 5: Testing of Grouted Anchors; CEN: Brussels, Belgium, 2018. [Google Scholar]
- Ferry Borges, J.; Castanheta, M. Structural Safety; Laboratorio Nacional de Engenharia Civil: Lisbon, Portugal, 1971. [Google Scholar]
- International Organization for Standardization. ISO 8930 General Principles of Reliability of Structures; International Organization for Standardization: Geneva, Switzerland, 1987. [Google Scholar]
- International Organization for Standardization. ISO 2394, General Principles of Reliability of Structures, List of Equivalent Terms; International Organization for Standardization: Geneva, Switzerland, 2015. [Google Scholar]
- Joint Committee on Structural Safety, Reliability Based Code Calibration. Available online: http://www.jcss.byg.dtu.dk/codecal (accessed on 26 March 2021).
- Diamantidis, D. Probabilistic Assessment of Existing Structures; RILEM Publications: Cachan, France, 2001. [Google Scholar]
- Gulvanessian, H.; Calgaro, J.-A.; Holicky, M. Designer’s Guide to EN 1990, EUROCODE: Basis of Structural Design; Thomas Telford Publishing: London, UK, 2002. [Google Scholar]
- Gulvanessian, H.; Holicky, M. Eurocodes: Using reliability analysis to combine action effects. Proc. Inst. Civ. Eng. Struct. Build. 2005, 158, 243–252. [Google Scholar] [CrossRef]
- JRC. Implementation of Eurocodes, Handbook 2, Reliability Backgrounds; JRC: Prague, Czech Republic, 2005; Available online: https://eurocodes.jrc.ec.europa.eu/showpublication.php?id=63 (accessed on 26 March 2021).
- Sykora, M.; Holicky, M.; Markova, J. Verification of existing reinforcement concrete bridges using the semi-probabilistic approach. Eng. Struct. 2013, 56, 1419–1426. [Google Scholar] [CrossRef]
- Luechinger, P.; Fischer, J. New European Technical Rules for the Assessment and Retrofitting of Existing Structures. Eur. Comm. 2015. [Google Scholar] [CrossRef]
- Ranta-Maunus, A.; Fonselius, M.; Kurkela, J.; Toratti, T. Reliability Analysis of Timber Structures, VTT Research Notes 2109, Espoo. 2001. Available online: https://www.vtt.fi/inf/pdf/tiedotteet/2001/T2109.pdf (accessed on 26 March 2021).
- Poutanen, T. Calculation of partial safety factors. In Applications of Statistics and Probability in Civil Engineering; Faber, M.H., Köhler, J., Nishijima, K., Eds.; Taylor & Francis Group: London, UK, 2011. [Google Scholar]
- Poutanen, T.; Länsivaara, T.; Pursiainen, S.; Mäkinen, J.; Asp, O. Calculation of Safety Factors of the Eurocodes. Appl. Sci. 2021, 11, 208. Available online: https://www.mdpi.com/2076-3417/11/1/208 (accessed on 19 February 2020). [CrossRef]
- Poutanen, T.; Pursiainen, S.; Mäkinen, J.; Länsivaara, T. Combination of Permanent and Variable Loads, Rakenteiden Mekaniikka. J. Struct. Mech. 2018, 51, 1–9. Available online: https://rakenteidenmekaniikka.journal.fi/issue/view/4901 (accessed on 26 March 2021).
- Fruhwald, E.; Serrano, E.; Toratti, T.; Emilsson, A.; Thelandersson, S. Design of Safe Timber Structures—How Can we Learn from Structural Failure in Concrete, Steel and Timber? Division of Structural Engineering, Lund University: Lund, Sweden, 2007. [Google Scholar]
- CEN. EN 1995-1-1:2004, Eurocode 5. Design of Timber Structures; CEN: Brussels, Belgium, 2004. [Google Scholar]







| Load-Duration Class | Examples of Loading | Duration | |
|---|---|---|---|
| Permanent | self-weight | >10 years | 0.6 |
| Long-term | storage | 6–10 months | 0.7 |
| Medium-term | imposed load, snow | 1 week–6 months | 0.8 |
| Short-term | snow, wind | <week | 0.9 |
| Instantaneous | wind, accidental load | 1.1 |
| VM | μM | σM |
|---|---|---|
| 0.10 | 1.184 | 0.184 |
| 0.15 | 1.292 | 0.194 |
| 0.20 | 1.412 | 0.282 |
| 0.10 | 1.031 | 1.281 | 1.509 | 1.073 | 1.231 |
| 0.15 | 1.114 | 1.268 | 1.512 | 1.080 | 1.253 |
| 0.20 | 1.218 | 1.285 | 1.554 | 1.116 | 1.311 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Poutanen, T.; Pursiainen, S.; Mäkinen, J. Test Loading of Structures with a Suspect Resistance. Appl. Sci. 2021, 11, 3424. https://doi.org/10.3390/app11083424
Poutanen T, Pursiainen S, Mäkinen J. Test Loading of Structures with a Suspect Resistance. Applied Sciences. 2021; 11(8):3424. https://doi.org/10.3390/app11083424
Chicago/Turabian StylePoutanen, Tuomo, Sampsa Pursiainen, and Jari Mäkinen. 2021. "Test Loading of Structures with a Suspect Resistance" Applied Sciences 11, no. 8: 3424. https://doi.org/10.3390/app11083424
APA StylePoutanen, T., Pursiainen, S., & Mäkinen, J. (2021). Test Loading of Structures with a Suspect Resistance. Applied Sciences, 11(8), 3424. https://doi.org/10.3390/app11083424






