Experimental Determination of Lamb-Wave Attenuation Coefficients

Abstract

This work determined the attenuation coefficients of Lamb waves of ten engineering materials and compared the results with calculated Lamb-wave attenuation coefficients, α–S and α–A. The Disperse program and a parametric method based on Disperse results were used for calculations. Bulk-wave attenuation coefficients, α_L and α_T, were required as input parameters to the Disperse calculations. The calculated α–S and α–A values were found to be dominated by the α_T contribution. Often α–A_o coincided with α_T. The values of α_L and α_T were previously obtained or newly measured. Attenuation measurement relied on Lamb-wave generation by pulsed excitation of ultrasonic transducers and on surface-displacement detection with point contact receivers. The frequency used ranged from 10 kHz to 1 MHz. A total of 14 sheet and plate samples were evaluated. Sample materials ranged from steel, Al, and silicate glass with low attenuation to polymers and a fiber composite with much higher attenuation. Experimentally obtained Lamb-wave attenuation coefficients, α–S and α–A, for symmetric and asymmetric modes, were mostly for the zeroth mode. Plots of α–S_o and α–A_o values against frequency were found to coincide reasonably well to theoretically calculated curves. This study confirmed that the Disperse program predicts Lamb-wave attenuation coefficients for elastically isotropic materials within the limitation of the contact ultrasonic techniques used. Further refinements in experimental methods are needed, as large deviations often occurred, especially at low and high frequencies. Methods of refinement are suggested. Displacement measurements were quantified using Rayleigh wave calibration. For signals below 300 kHz, 1-mV receiver output corresponded to 1-pm displacement. Peak displacements after 200-mm propagation were found to range from 10 pm to 1.5 nm. With the use of signal averaging, the point-contact sensor was capable of detecting 1-pm displacement with 40 dB signal-to-noise ratio and had equivalent noise of 4.3 fm/√Hz. Approximate expressions for α–S_o and α–A_o were obtained, and an empirical correlation was found between bulk-wave attenuation coefficients, i.e., α_T = 2.79 α_L, for over 150 materials.

1. Introduction

For more than half a century, ultrasonic testing (UT) and acoustic emission (AE) have played key roles in the field of nondestructive evaluation (NDE) [1,2], and more recently contributed substantially to the successful development of structural health monitoring (SHM) [3]. Among the methods that are based on elastic wave propagation, those using guided waves (GW), e.g., Lamb and Rayleigh waves, have made significant advances in recent decades, benefitting from improved computational mechanics, signal generation and sensing methods, and signal processing techniques [4,5,6]. Two reviews provided detailed mathematical developments on guided waves from Lord Rayleigh’s works to the present, covering more than a century [7,8].

The first successful NDE application of guided waves occurred at Aerojet for the AE inspection of Polaris missile chambers in the early 1960s [9], followed by many more AE tests of rocket motorcases. Soon after, AE inspection was expanded to various pressurized installations, including pipelines, tanks, vessels, etc., of both metals and fiber-reinforced composites [2]. In 1967, buried pipelines were inspected using 300-m sensor spacing and detected corrosion pits at 100-mm location accuracy [2] (p. 166). By the 1980s, many industrial tanks and vessels in the USA were regularly inspected under the ASME Boiler and Pressure Vessels Codes and ASTM standards [10]. These AE tests were, however, conducted without benefiting from the theories of GW propagation, since field measurements of wave propagation velocities were successfully incorporated into the test routines. Up to 1990, only three AE papers were found to use the Lamb wave dispersion curves. Two were for detection frequency selection by Hutton [11] and Graham and Alers [12]. The third was on long distance pipeline AE inspection by Pollock [13]. The GW attenuation was measured on long pipelines of up to 300 m [2,13], and the Pollock results at 30 kHz were analyzed in [14], separating signal reduction into geometrical spreading and steel absorption components. The GW attenuation was very small, or 0.17 dB/m, demonstrating the benefit of GW utilization on pipelines. In 1991, Gorman introduced mode-dependent velocities of GW, specifically Lamb waves, to properly conduct AE source location in thin structures [15]. In the 1990s, numerous computational studies started to make GW properties more accessible, even for anisotropic materials. A series of studies by Hamstad and coworkers [16] used three-dimensional, dynamic finite element modeling and correlated source motion to displacements at sensor positions, accounting for GW propagation effects through a plate. These studies have been extended to fiber-reinforced composites [6]. GW-based AE studies (or GWAE) also profited from wavelet analysis as mode separation with time and frequency was visualized. Takemoto and coworkers [17] developed wavelet analysis software based on Gabor wavelets and made available the source code written by Hayashi, Y. This code was implemented by Vallen, J. in 2003 into an easy-to-use free software, AGU-Vallen Wavelet [18]. With continual support of Vallen Systeme (Wolfratshausen, Germany), this wavelet software has assisted GW studies and NDE engineers everywhere.

Viktorov gathered main results of early studies of Lamb and Rayleigh waves [19]. In addition to velocity dispersion curves, parametric equations of attenuation of Lamb and Rayleigh waves were given. Around 1970, the GW dispersion curves of only a few materials (including Al, steel, and polymethyl methacrylate (PMMA)) were commonly accessible [20,21]. In the following 50 years, GW mechanics and NDE based on GW ultrasonic testing (or GWUT) have been refined, passing many milestones. Long-range inspection of pipelines has been practiced for two decades [22,23,24]. GW dispersion analysis software packages became available for GW velocities and attenuation [25,26,27]. GW analysis methods for anisotropic materials and structures were developed [4,28,29,30,31,32,33,34,35,36], and GW in non-linear solids started to provide a new NDE approach, especially for detecting fatigue damage [37,38,39]. The uses of laser devices enabled, for example, detailed evaluation of composite damages and GW fields generated by fatigue cracks [40,41]. A recent review is a source for many other articles related to GW [42], and Imperial College London’s web site [43] is a treasure trove of many doctoral theses on GW and NDE subjects.

Although many advances in GW science have materialized, it is still difficult to find data on GW attenuation for most engineering materials. A recent review gathered available bulk-wave and GW attenuation studies [14], which illuminate the scarcity of attenuation data, especially for the transverse waves and Lamb waves. Some of the studies cited above are exceptions and compared calculated and measured attenuation for carbon-fiber reinforced composite (CFRP) sheets [31,34,35]. When the attenuation coefficients of bulk waves of the same (or similar) material are available, GW attenuation coefficients can be calculated using Viktorov’s parametric equations [19]. For Lamb waves, Viktorov gave needed parameters for the zeroth and first symmetric and asymmetric modes in graphic form. Here, material damping was incorporated into complex wave numbers. Another method is to use Disperse software [25,26], which used the global matrix method. This allows a choice of hysteretic or Kelvin–Voigt damping models, but, again, bulk-wave attenuation coefficients must be supplied. Until recently, bulk-wave attenuation coefficients were of limited availability, and comparison between theoretical calculations and experimental results has been scarce. Two reports provided attenuation coefficients of longitudinal and transverse waves for over 200 materials [44,45]. This gives an impetus for experimentally verifying the theoretical prediction of Lamb-wave attenuation [19,25,26]. In turn, when such verification materializes, the experimental methods utilized in the bulk-wave attenuation measurements are validated. This is an added incentive of the present study. Note also that the two attenuation studies [44,45] showed all but a few possessing the linear frequency dependence indicative of hysteretic damping. That is, the Kelvin–Voigt damping model was inapplicable.

The objective of this work is to determine the attenuation coefficients of Lamb waves of ten engineering materials and to compare the results with calculated Lamb-wave attenuation coefficients, which utilized Disperse [25,26]. It is intended to ascertain the degree of agreement between theory and experiment. General agreements were found, but modest to large deviations were also observed. Results will be discussed in regard to possible sources of discrepancies.

2. Materials

Materials used for this work are sheets and plates of polystyrene (PS), three aluminum alloys (Al-1100, Al-6061, and Al-7475), 410 stainless steel, soda-lime glass, PMMA, polyvinyl chloride (PVC), high-density polyethylene (HDPE), and a cross-ply glass-fiber reinforced plastic (XP-GFRP): 14 sheet and plate samples in all. Their properties and sizes are tabulated in

Table 1. Tested materials and their properties.

Test	Material	Density	VL	VT	αL	αT	Thickness	Size	Hardness	Notes *
No.		Mg/m3	mm/µs		dB/m/MHz		mm	mm × mm	VHN
G1a	PS	1.06	2.34	1.16	39	113	1.38	554 × 433		αL and αT measured. *
G1b	PS	1.05	2.3	1.17	39	113	1.38	602 × 397		αL and αT of G1a used.
G2a	Al-1100-H18	2.7	6.18	3.08	15.7	26.5	2.06	1133 × 523	41	αL and αT of G2b used.
G2b	Al-1100-H18	2.7	6.18	3.08	15.7	26.5	6.38	907 × 306	40	αL and αT measured.
G3a	Al-6061-T6	2.7	6.38	3.23	4.9	10.3	12.7	1220 × 1200	109	αL and αT of G3b used.
G3b	Al-6061-T6	2.7	6.4	3.17	4.9	10.3	25.3	63.5 × 47.3	122	Only for damping tests.
G4	Al-7475-H18	2.81	6.5	2.99	5.7	12.2	2.72	610 × 367	123	αL and αT of N19 used. +
G5a	Stainless steel 410	7.74	5.74	3.11	0.97	2.6	3.16	1015 × 306	240	αL and αT of G5b used.
G5b	Stainless steel 410	7.7	5.97	3.32	0.97	2.6	104.9	(38.5 dia.)	211	Only for damping tests.
G6a	Soda-lime glass	2.55	5.73	3.43	8.7	16.2	1.98	609 × 578		αL and αT of C43 used. +
G6b	Soda-lime glass	2.49	5.9	3.3	8.7	16.2	4.72	820 × 450		αL and αT of C43 used. +
G7a	PMMA #	1.18	2.74	1.4	131	420	1.76	430 × 338		αL and αT measured. +
G7b	PMMA #	1.18	2.74	1.4	106	216	6.3	610 × 610		αL and αT measured.
G8	PVC	1.4	2.33	1.11	239	909	4.3	812 × 812		αL and αT of O2c used. +
G9	HDPE	0.95	2.18	1.13	222	1010	7.09	585 × 509		αL and αT of O10 used. +
G10	XP-GFRP §	1.81	3.84	1.99	117	445	2.3	555 × 277		αL and αT measured. *

* Four sheets were glued for attenuation tests. ⁺ Test Numbers used in Notes for G4, G6a,b, G8 and G9 refer to those from [44,45]. ^# Values of V_L and density of PMMA were incorrectly listed previously in [44,45]. ^§ Isotropy assumed for V and α values listed above. Complete V and α values are listed below.

. The bulk-wave attenuation coefficients, α_L and α_T, for longitudinal and transverse waves, were taken from [44,45] when available and measured for PS, PMMA, Al-1100-H18, Al-6061-T6, and 410 stainless steel (410 SS). However, the attenuation of thinner metallic alloy and glass samples of less than 5-mm thickness could not be measured. For thin polymer samples (PS and PMMA), four sheets were glued together for attenuation tests. Attenuation measurements were also conducted for 6.3-mm-thick plate of PMMA, as the previous values were taken on PMMA plates of 18 to 47 mm thickness. For the Al-6061 plate, a 25.3-mm thickness sample of comparable T6 condition was used for attenuation tests. For the 410 SS sheet, a round bar of the equivalent material (G5b in Table 1) was used for attenuation tests. For the GFRP, selected values of velocity and attenuation were used in Table 1, and the complete set of these values are also given. The velocity values and density can be converted to orthotropic elastic stiffness coefficients [46].

V11	α11	V22	α22	V33	α33	V13	α13	V31	α31	V32	α32	unit
2.78		3.76		3.84		1.91		1.77		1.99		mm/µs
	486		202		117		1300		861		445	dB/m

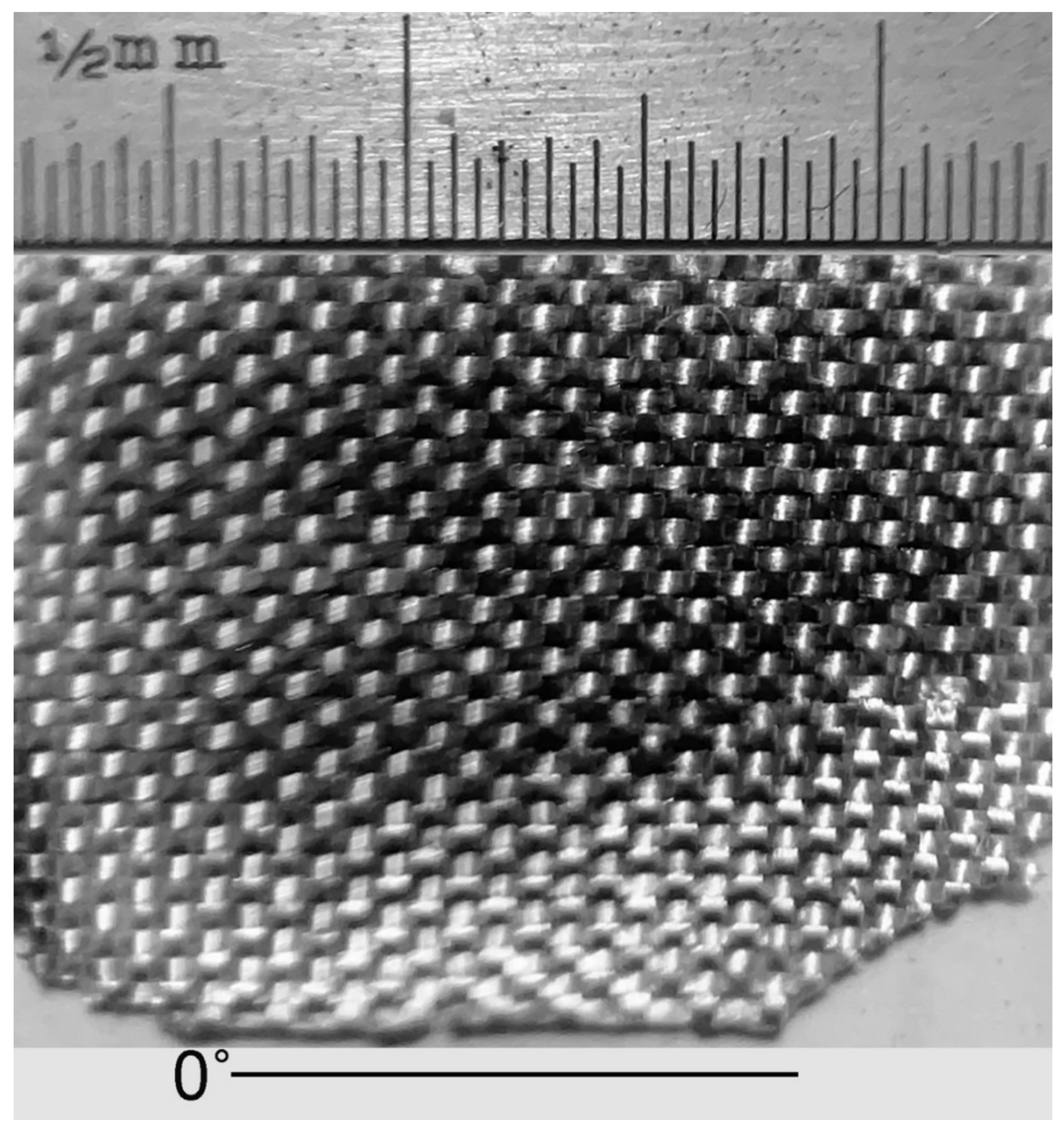
The GFRP sheet used in this study was reinforced by a plain weave of fiber rovings. It had 0.38 fiber fraction, and the weave pitches were 1.3 mm and 0.8 mm along 0° and 90° (3- and 2-directions) [47]. Figure 1 shows a macrophotograph of this glass fiber fabric. More flexible warp fibers bundles are along the 3-direction, whereas rigid weft fiber rovings are oriented in the 2-direction. Differences in fiber densities produced 2% faster velocity in the 3-direction, which corresponded to the direction of Lamb-wave propagation. The wavelengths of Lamb waves examined in this work were always more than twice the weave pitches. At 500 kHz or below, the cross-weave structure can be considered as a stacking of uniform sheets. At frequencies above 1 to 1.5 MHz, all the bulk-wave attenuation coefficients increased sharply, reflecting the effects of millimeter-sized cross-weave patterns. In the thickness direction, this GFRP sheet had eight layers of cross-weaved fiber rovings, at 0.25-mm thickness per layer. Thus, this sheet can be treated as uniform below 500 kHz.

These sheet and plate samples will be collectively called plates, but both terms will be used for a specific case. (Note that the term sheet is commonly used for thickness less than 3 mm for polymers and less than 5 or 6 mm for metallic alloys in various industrial standards.)

3. Experimental Procedures

Lamb-wave attenuation tests followed procedures similar to those described previously [48]. For generating symmetric mode waves, an ultrasonic transducer (Olympus V101, 0.5 MHz, 25 mm diameter, Olympus NDT, Waltham, MA, USA; NDT Systems C-16, 2.25 MHz, 13 mm diameter, NDT Systems, Huntington Beach, CA, USA; FC500, 2.25 MHz, 19 mm diameter, AC375L, 12.7 mm diameter, Acoustic Emission Technology Corp., Sacramento, CA, USA) was mounted to an edge of a sample plate. For asymmetric mode waves, two types were used. One was an Olympus V103 (1 MHz, 12.7 mm diameter, Olympus NDT), and the other was an NDT Systems C16. Olympus V221, 10 MHz, 6.4 mm diameter, was also used for a comparison. The ultrasonic transducer was mounted to an edge of a sample plate using a 6.4-mm thick aluminum hex rod, providing an incident angle of 60°. This incident angle was selected for obtaining repeatable and robust transducer attachment, as the sample plate needs to be turned over for two-sided detection. This process affects the sensor position accuracy, which is estimated to be better than ±1 mm. The transducer was attached to a sample plate using a mechanical jig or with a cyanoacrylate adhesive. This experimental set-up is shown in Figure 2a. The V103 transmitter was attached to a PVC plate edge with two U-shaped jigs (see insert photograph for an enlarged view). The KRN receiver was positioned vertically using a large U-shaped holder, which was held with brass plates. The total force on the sensor tip was 10.5 N. Another method for asymmetric wave generation used a point-contact transmitter (KRNBB-PCP transducer with 1-mm diameter active area, KRS Services, Richland, WA, USA), mounted vertically on a plate. Contact location was either in the middle of a large plate or within 5 mm from an edge. This was also used with edge mounting. These transmitters were usually driven by high-voltage pulses (280–320 V) or by low-voltage (±20 V) pulses from a waveform generator with an amplifier. Single sinewave and Gaussian pulse shapes were used depending on the degree of attenuation. These low-voltage pulses were tried when a certain frequency range was targeted, but some tests resulted in unsatisfactory outcomes due to weak signals. Longer sinewave excitation was used on a large Al plate, as will be subsequently described. The transmitting sensitivities of V101 and NDT C16 are shown in Figure 2b [49]. The unit is in dB in reference to 1 nm/V. The high-voltage pulse spectrum is also plotted. The sum of the sensor and pulse spectra yielded the displacement output, which reached a peak of 10 to 20 nm with a risetime of less than 0.2 µs and decay time of about 1 µs. These spectra showed that V101 was suitable for frequencies below 500 kHz, whereas NDT C16 produced high-frequency output. When a transmitter was attached to a plate through a hex rod for asymmetric wave generation, the hex rod changed its output. The use of the hex rod provided an efficient reflection path of the initial pulse with 2-µs time delay, enhancing the signal output below 400 kHz. Relative frequency spectra of V103 and NDT C16 outputs with the hex rod are shown in Figure 2c. The two spectra are similar to each other, with a dip at 271 or 276 kHz, suggesting strong effects of the hex rod. These dips were absent without the hex rod. As a transmitter, these can be considered equivalent.

Lamb-wave vibration developed following wave propagation of at least 100 mm, but usually a travel distance of 125 or 150 mm was needed for fuller development of Lamb modes. This is reflected in the increase of peak amplitude. In most of the tests, the wave propagation distance ranged from 150 to 400 mm in 50-mm steps, or 125 to 300 mm in 25-mm steps. The upper limit of the propagation distance was imposed by the arrival of reflected waves. In some tests, signal amplitude started to increase for unknown reasons at larger distances before any reflections could arrive. Such data were discarded. A point contact transducer with internal electronics (KRN-BB-PC, 1-mm diameter, KRN Services) was primarily used to detect the vertical motion of passing Lamb waves. This sensor nominally covers from 10 to 1000 kHz. A broadband PAC Pico sensor (3 mm diameter, Mistras, Princeton, NJ, USA), which has a sensing range from 30 to 800 kHz, was also used. Figure 2d gives the receiving sensitivities of these two sensors to Rayleigh waves [50]. This calibration utilized glass capillary fracture. The unit is in dB in reference to 1 V/nm = 1 mV/pm. For the KRN sensor, the average sensitivity from 10 to 300 kHz is equal to 1.00 mV/pm. That is, its output in mV can be directly converted to displacement output measured in pm. Detected signals were digitized using PicoScope 5242D (15 bit, 8 to 104 ns sampling intervals, input impedance of 1 MΩ; Pico Technology, St. Neots, UK). During signal acquisition, signal averaging was always used. Averaging counts were always more than 32. This unit also served as a waveform generator, the output of which was amplified to ±20 V using an HP-467A amplifier (Hewlett Packard, Palo Alto, CA, USA). All sensor coupling used petroleum gel as a couplant.

For all testing of Lamb-wave attenuation, vibration detection was conducted on both surfaces, as was done in [48]. This necessitated the use of the identical transmitter configuration. The set-up of Figure 2a for the asymmetric mode (and its equivalent for the symmetric mode that uses a C-clamp) can conduct the two-surface sensing by turning over the plate with the transmitter attached. Care was needed to keep the transmitter attachment undisturbed while flipping after the completion of measurements on one side. The receiver was now positioned on the back side and transmitter connection was reestablished, followed by back-side detection. This two-surface method was not used when two point-contact transducers were used as transmitter and receiver. At later stages, a different sensor attachment method allowed the use of the two-surface detection as well. Differences of the detected signals on two sides were used for further signal processing in asymmetric cases, whereas signal summation was used to enhance symmetric wave signals. Time shifting of a few tenths µs to up to 1.5 µs was needed to match the signal pair for the same or opposite phase for the symmetric and asymmetric modes, respectively. The value of 1.5 µs was only required for lower frequency signals below 100 kHz, so it is a small fraction of the wave period. Time shifting was applied at the peak location. This need resulted mainly from the lack of symmetry in the transmitter attachment. In the case of asymmetric wave generation, signal paths were different by design. Complete suppression of unwanted mode cannot be achieved, and time-based discrimination was also applied using the wavelet transform analysis method. For the symmetric wave analysis, signals were truncated when the main asymmetric wave (usually A_o mode) arrived and its amplitude grossly exceeded the expected S_o mode before applying the summation routine. When the A_o mode was well suppressed, the slow-moving S_o mode was analyzed as well, but this mode was weak and typically not analyzed. For the asymmetric wave analysis, the initial part of signals (S_o-mode segment) was truncated, although adequate suppression was usually achieved using signal differences. With the signal difference processing, faster A₁-mode signals emerged, but signal levels were low and were usually discarded in later wavelet transform analysis. These features are displayed in Figure 3 for the case of a PS sheet (Sample Number: G1b). Figure 3a shows symmetric waves with front side (blue curve), back side (green curve), and summed (halved, red curve). The propagation distance was 200 mm, and the signal arrived at 97 µs (with the velocity of 2.06 mm/µs). Although lower amplitude signals were hidden, the summed red curve represented the averaged signal. Note that the amplitude scale was converted to displacement, as the KRN sensor has 1-pm/mV sensitivity for these signals. The A_o mode arrived at 170 µs, as indicated by blue and green curves, showing peaks of 85 to 90 pm. The summed signal (red curve) suppressed strong peaks of A_o signals, and it reached only 20 pm at 176 µs. The former A_o segment of 170 to 190 µs followed the trend of the S_o signal well. In most of the tests reported, the signal summation step was utilized in attenuation determination, as signal modulation is averaged out, as shown in Figure 3a. This step may be omitted in the case of symmetric modes when A_o signals are truncated. Figure 3b is the case of asymmetric waves with the same color codes as in Figure 3a, except the red curve represents the differences of the front and back signals (and the amplitude is halved). The amplitude of the difference signal (in the pm unit), mostly A_o mode from 170 µs, follows those of the front (blue) signals (and the reverse of back (green) signals). The propagation distance was also 200 mm, and the signal arrived with the velocity of 1.18 mm/µs. The initial S_o component (to 170 µs, not shown) was substantially reduced and omitted in the subsequent wavelet transform (WT) analysis.

In order to obtain an attenuation spectrum, a set of six to eight detected signals at different propagation distances were analyzed using AGU-Vallen Wavelet software (ver. R2019.0926.2, Vallen Systeme, Wolfratshausen, Germany, 2019) [18]. A Choi–Williams transform was selected. Spectrograms of wavelet transform of signals shown in Figure 3a,b are plotted in Figure 3c,d, respectively. Corresponding velocity dispersion curves are superposed as well. For the symmetric wave WT (Figure 3c), the S_o mode was the strongest at 400 ± 20 kHz, and it followed the S_o-mode dispersion curve (drawn in red) from 100 to 600 kHz. The propagation distance was 200 mm. The superposed dispersion curves matched the initial part of the WT intensity. At 400 kHz, the S_o curve was 3.8 µs before the peak intensity. This time delay is due to the build-up of Lamb wavelet from a fast-rising excitation pulse on the transmitter. The inserted WT spectrogram is that for 125-mm propagation distance. The time delay at 400 kHz was again 3.8 µs, indicating the delay, or signal risetime, was from Lamb wavelet formation process. Other modes were absent, except for the A_o mode below 250 kHz, which was easily excluded during subsequent WT analysis. Figure 3d gives an example of the asymmetric wave WT spectrogram. A high-intensity A_o zone was located at 420 ± 60 kHz and extended down to 40 kHz and up to 700 kHz. The start time of this WT was at 169 µs and the A_o peak corresponded to 1.18 mm/µs velocity, subtracting the signal risetime of 6 µs. This matched the predicted A_o velocity of 1.18 mm/µs. On this spectrogram, a notable non-A_o-mode signal was found from 530 to 600 kHz, starting at 180 µs. This part was due to the S_o mode and was excluded from further processing.

WT analysis was conducted for each propagation distance for the set of S_o-mode and A_o-mode signals. WT intensity was obtained as a function of time and frequency. The frequency step used was typically 7.5 kHz. For a given frequency, WT intensity values were summed over a selected duration that covers the expected mode arrival times. When the range of frequency was wide, the summed values were sometimes aggregated over two or three frequency steps, doubling or tripling the frequency step to 15 or 22.5 kHz. Next, the summed WT intensity values at all propagation distances were arranged in a table, from which attenuation coefficients were determined. The WT intensity, I, obtained by AGU-Vallen Wavelet software is given as the square of amplitude multiplied by time interval. Because wave attenuation is defined in terms of wave motion, or vertical displacement, D, of Lamb waves [2] (p. 91), we have

√I = aD = D_o exp(−α′x),

(1)

where a and D_o are constants, α′ is attenuation coefficient in Np/m, and x is the wave propagation distance in m. For planer waves, geometrical spreading reduces displacement by the square-root of x [2] (p. 89). Combining these, one obtains

D = D_o exp(−α′x)/√x.

(2)

In practice, we use attenuation coefficient α using the unit of dB/m. This comes from the slope of a plot of 20 log (D√x) vs. x. This means that D√x is expressed in dB scale. This D√x term is denoted here as the WT intensity sum. An example is given in Figure 3e. This dataset produced Figure 3a,c plus six more test data for x between 125 and 275 mm. In particular, this plot is for symmetric mode waves at 496 kHz. A linear regression fit was obtained with α–S_o = 49.3 dB/m and R² = 0.993. The values of α–S_o were determined from 206 to 600 kHz and are plotted in Figure 3f.

Average R² value was 0.921, which indicates a satisfactory fit. Only one R² value was below 0.8 at 0.795 for the data in Figure 3f. Below 200 kHz, R² values decreased to below 0.7, or no attenuation was detected. Above 600 kHz, the I value decreased to below 30% of the peak, and the α–S_o value started to decline with frequency. This is caused by a slow decrease in I values at larger x, apparently due to higher contributions from background noise in the WT analysis. In most other cases, the limiting intensity was approximately 10% of the peak value. Incidentally, the α–S_o vs. frequency data in Figure 3f can be represented by a fourth order polynomial, and the fitted curve is plotted with a dotted curve. Such a curve-fitting method is useful in calculating deviation statistics, as will be discussed subsequently.

4. Calculation of Lamb-Wave Attenuation

Two methods can be used to obtain theoretical values of attenuation coefficients of Lamb-wave modes, as noted earlier [19,25,51]. Both methods utilize complex wavenumbers or elastic moduli by adding damping terms as their imaginary components. The damping terms are inserted as bulk-wave attenuation coefficients, α_L and α_T. The methods also require the plate thickness and longitudinal and transverse wave velocities, v_L and v_T. For the Viktorov method [19], Lamb-wave attenuation coefficients α–S_n and α–A_n can be calculated using A and B parameters for each mode, with the two expressions below.

α–S_n = A_Sn α_L + B_Sn α_T.

(3)

α–A_n = A_An α_L + B_An α_T.

(4)

These parameters are given graphically for the cases of n = 0 and 1 and in equation forms. These equations apparently contain typographical errors and could not be used to reproduce α–S_n and α–A_n values (private communication, Barat, V., 2021). The A and B parameters for n = 0 and 1 were digitized, and α–S_n and α–A_n were computed for an Al plate of 6.4 mm thickness. For these calculations, the bulk-wave attenuation coefficients, α_L and α_T, of the longitudinal and transverse waves were taken from Disperse, namely, α_L = 4.125 dB/m/MHz and α_T = 27.76 dB/m/MHz for Al. It is important to recognize that both Viktorov and Disperse calculations require experimentally obtained values of α_L and α_T. Results are shown by solid curves in Figure 4a,b. Using the same input values, a calculation with Disperse software [51] (ver. 2.0.20a, Imperial College, London, UK, 2013) was conducted as well, and results are shown by dotted curves together with the Viktorov calculations. Here, blue curves are for S_o and S₁ modes, and red curves are for A_o and A₁, respectively. In all cases, the trends of corresponding curves matched well, especially for the zeroth modes. Viktorov S_o showed higher attenuation than Disperse S_o, whereas the attenuation of the A_o mode was reversed. For the first mode, deviations were larger, with the Viktorov curves 5 to 20 dB/m higher. In both figures, blue and red dashed lines represent the bulk-wave attenuation coefficients, α_L and α_T, of the longitudinal and transverse waves.

In Viktorov calculations, the contributions of the longitudinal and transverse components are obtained separately, then they are summed for the final attenuation value. For the selected α_L and α_T, both α–S_n and α–A_n consisted mostly of the transverse components, which are plotted in green and purple dashed curves. These are plotted in Figure 4a, but these overlap almost completely, being barely visible only below 250 kHz. Here, the contribution of the longitudinal component is minimal. This is partly due to low α_L, which was only 15% of α_T. Even when α_L is larger with respect to α_T, however, the dominance of α_T component is expected. The A_o curve from Disperse almost overlaps the α_T line, whereas Viktorov A_o is approximately 20% below the α_T line. All Lamb-wave attenuation curves are also within a factor of three to the α_T line (excluding those near the low frequency cut-offs). These are always above the α_L line by a factor of two or more.

Because pairs of attenuation curves of the same modes from these two methods are smooth-varying, Viktorov’s A and B parameters can be modified to represent the Disperse results. Two cases were tried, and Disperse results can be expressed in the same manner as the Viktorov method. Disperse calculations were made using zero or non-zero α_T input for glass and PMMA plates with Poisson’s ratios of 0.28 and 0.34. Polynomial curve-fitting is applied sectionally to the results of the Disperse calculations. Frequency shifts need to be used in some sections to achieve better fits. By comparing Lamb-wave attenuation with zero and non-zero α_T inputs, two complete sets of A and B parameters for materials were constructed. Because Disperse provides attenuation values up to the fourth order, the tables of A and B parameters enable calculating Lamb-wave attenuation up to S₄ and A₄ in the absence of Disperse software. For the case of Poisson’s ratio of 0.34, results generally matched well to the direct Disperse calculation for HDPE, with a Poisson’s ratio of 0.36. Deviation was mainly in frequency and the maximum shift was 0.4%. Thus, the tables of A and B can cover a Poisson’s ratio of 0.25 to 0.36, with practical precision. This method may be called the Viktorov–Disperse parametric method or the Disperse-based parametric method. (See Appendix A for example calculation and parts of tables.)

Relative contributions of the longitudinal and transverse components to Disperse calculations were examined using the Disperse-based parametric method. In this case, α_L and α_T values of PMMA (Sample G7b) from Table 1 were selected, i.e., 106 and 216 dB/m/MHz. Figure 4c,d plotted S_o and A_o cases, respectively. The curves of S_o and A_o are given as solid blue and red curves, whereas the transverse components are shown by blue and red dotted curves. Reflecting a higher α_L, relative to α_T, solid and dotted curves are separated in both figures. The A_o curve from Disperse again overlaps the α_T line, except it is higher than the α_T line. This is likely due to higher α_L. The contributions of the transverse components in percent are plotted by dash-dotted curves in Figure 4c,d in blue and red, respectively. The dominant roles of transverse-wave attenuation are clearly illustrated, as 80% or higher transverse contributions were observed above 100 or 50 kHz, respectively. This is an unexpected finding, as Lamb-wave modes have always been considered to be synthesized by the interactions of reflecting longitudinal and transverse waves. In retrospect, Viktorov’s work [19] had already predicted the theoretical basis for the dominance of the transverse components on Lamb-wave attenuation. This feature is now confirmed with Disperse calculations [25,51] as well. It is difficult to suggest any plausible physical mechanisms to account for the dominance of transverse attenuation, but it is worthy of further study.

In this study, Disperse was used for eight cases, two with zero transverse attenuation. For most of the Lamb-wave tests with varied attenuation values, A–B parameter tables were used, and thickness changes were incorporated by factoring the attenuation and frequency scales. Unlike the velocity dispersion curves, which only need frequency re-scaling for a thickness change, attenuation dispersion curves must use attenuation re-scaling as well. Some published attenuation dispersion curves label abscissa as “Frequency-thickness”, while casually labeling ordinate as “Attenuation” e.g., [4,32]. The latter needs to be “Attenuation-thickness”, as given in the Disperse Manual as an example [51] (p. 148).

5. Results and Discussion

5.1. Polystyrene (PS)

PS was tested for its moderate values of bulk-wave attenuation coefficients, α_L = 39.0 dB/m/MHz and α_T = 113 dB/m/MHz. These were obtained using the methods described previously [44,45]. Two 1.38-mm thick sheets were used to obtain Lamb-wave attenuation. These two sheets were from different sources several years apart. Parts of the tests were described in Section 3 as an example. Their properties are listed in Table 1 as G1a and G1b. Figure 5a,b show α–S and α–A for PS sheets of 1.38-mm thickness in blue (for symmetric mode) and red (for asymmetric mode) symbols, respectively. In Figure 5a, two sets of α–S values are plotted in dots and + symbols from 140 to 600 kHz, and calculated values of α–S_o are plotted in a solid blue curve. Blue and red dashed lines representing the bulk-wave attenuation coefficients α_L and α_T are also shown. The Lamb-wave velocity range of these datasets ranged from 2.1 to 0.7 mm/µs. The A_o mode contribution was excluded by cutting off the low-speed portion of the WT intensity. The slow-moving S_o mode at 650 kHz had no WT intensity and was not included in the WT analysis. Other modes did not exhibit WT intensity that can be analyzed for attenuation. Both of the observed α–S data matched well with the calculated α–S_o curve to their respective high-frequency limit of 526 and 600 kHz. That is, the observed values are of the α–S_o mode.

Figure 5b gives α–A values from two tests. Calculated values of α–A_o in a solid red curve and the α_L and α_T dashed lines in blue and red are also plotted. The velocity range of these datasets was from 1.2 to 0.6 mm/µs, excluding the faster S_o mode. Differences of the two-sided signals were used to minimize the symmetric components. Both α–A attenuation data showed gradual increase straddling the calculated α–A_o (red curve), which nearly overlaps the red-dash α_T line. The observed attenuation values ranged from 140 to 700 kHz. These deviated from the predicted values by as much as 15 dB/m (or 27% at 500 kHz), but the overall trend followed the calculated attenuation curve. The agreement between theory and experiment is deemed moderately good.

In order to estimate the accuracy of the attenuation values obtained, deviation from the predicted value at the same frequency was calculated for each measurement. This is defined as the magnitude of the difference between the predicted and observed values. Percent deviation was also obtained. For this procedure, the predicted α–S_o values were fitted to a fourth order polynomial in frequency, whereas α–A_o values were represented by α_T values, as these two are close to each other. Results of the averaged deviation and percent deviation are as follows:

Wave Mode	Deviation (dB/m)	Percent Deviation (%)	Data Count
Symmetric case:	4.47	14.7	89
Asymmetric case:	4.99	10.7	61

For the Lamb-wave attenuation of PS sheets, the average deviation was approximately 5 dB/m and the average percent deviation was 11 to 15%. Because the deviation was computed using the absolute value of a difference, a measure of error is taken as twice the average deviation, or 2 × dev. This is marked in Figure 5a,b by vertical bars. Subsequent figures are similarly marked when deviation is obtained. Although there is no comparable accuracy analysis of Lamb-wave attenuation, the average deviation of α_L values of PMMA reported in reference [44] was 3.72 dB/m/MHz for nine different plate samples, where the deviation was calculated from the average value. This is similar to the above deviation of 4.47–4.99 dB/m for PS Lamb waves. However, the percent deviation of α_L values of PMMA was about three times smaller at 4.07%. Increased percent deviation for Lamb-wave attenuation is expected because this study used point-contact sensing, which introduces uncertainties to recorded displacement signals. The use of optical interferometric methods may reduce sensing errors.

Estimating the precision of Lamb-wave attenuation values requires many more repeat tests than the few series conducted in the present study. Within this limitation, two estimates of measurement precision were obtained. The first one compared observed α–S_o values at the same or similar (within a few kHz) frequencies. The other fitted α–S_o values to fourth order polynomials in frequency and determined differences at tested frequency values. Percent deviation was obtained using the average of the two α–S_o values. Results are given below.

Method	Deviation (dB/m)	Percent Deviation (%)	Data Count
1	4.98	15.7	24
2	3.94	14.7	35

Although the results can be viewed only as gross approximation of precision, the data point to: (1) The precision values are comparable to those of accuracy estimates. (2) The two precision estimation methods give comparable results. (3) The observed percent deviation values are within a qualitative estimate of ±10% precision. Because of the lack of a definitive error estimation method and limited data points at any given frequency, the average deviation is taken as an error estimate. Note that data counts and the number of symbols sometimes differ due to symbol overlapping.

Despite the above deficiencies in accuracy and precision with room for improvement, it is shown that Lamb-wave attenuation of PS sheets is found to match the theoretical prediction for both the symmetric and asymmetric modes.

5.2. Aluminum Alloys

Aluminum alloys, especially hardened structural grades like Al-2024 and Al-6061 (UNS A92024 and UNS A96061), are representative low attenuation materials, whereas commercially pure grade Al-1100 (UNS A91100), annealed Al-6061, and some Al-7000-series are slightly more attenuating [44,45]. Two Al-1100-H18 plates (G2a and G2b in Table 1), Al-6061-T6 plate (G3a), and Al-7475-H18 (UNS 97475) sheet (G4) were used. Their thicknesses were 2.06, 6.38, 12.7, and 2.72 mm, respectively. Note that H18 and T6 suffixes indicate fully cold-worked and precipitation-hardened conditions, respectively. All the Al sheets and plates were obtained many decades ago and their source information is unavailable, except for Al-7475 (Kaiser Aluminum Research Lab, Pleasanton, CA, USA).

5.2.1. Al-1100-H18

Two Al-1100 plates (G2a and G2b) have Vickers hardness numbers of 41 and 40 and are in fully cold-worked condition. The thicker plate was tested for its bulk-wave attenuation coefficients, resulting in α_L = 15.7 dB/m/MHz and α_T = 26.5 dB/m/MHz, as listed in Table 1. These values were used to calculate Lamb-wave attenuation coefficients using the Disperse-based parametric method with Poisson’s ratio of 0.34. As the Poisson’s ratio of Al-1100 was 0.33, the parametric method gives accurate values of α–S_o and α–A_o. Figure 6a,b show experimental α–S_o and α–A_o values for an Al-1100 sheet of 2.1-mm thickness in blue and red symbols, respectively. In Figure 6a, two sets of α–S values are plotted in dots and + symbols from 200 to 1000 kHz, and calculated values of α–S_o are plotted in a solid blue curve. Blue and red dashed lines represent the bulk-wave attenuation coefficients, α_L and α_T. The velocity range of these datasets was from 5.9 to 3.2 mm/µs. The A_o mode contribution was absent on WT spectrograms with the two-side summation processing and was also cut off in WT intensity summation. Both of the observed α–S_o data matched well with the calculated α–S_o curve (in solid blue). The maximum deviation was 2.4 dB/m, or 34%, of the calculated value at 427 kHz.

Figure 6b gives α–A values from four tests. Calculated values of α–A_o in a solid red curve and the α_L and α_T dashed lines in blue and red are also plotted. The velocity range of these datasets was from 3.3 to 1.1 mm/µs, excluding the faster S_o mode. Differences of the two-sided signals were used to minimize the symmetric components. The α–A_o attenuation data showed gradual increase straddling the calculated α–A_o (red curve), which is slightly above the red-dashed α_T line. The observed attenuation values ranged from 160 to 450 kHz. These deviated from the predicted values by as much as 5.2 dB/m (or 57% at 320 kHz), but the overall trend followed the calculated attenuation curve. Deviation was higher between 300 and 400 kHz. The matching between theory and experiment is deemed moderate.

In order to evaluate the accuracy of attenuation values obtained, deviation and percent deviation were calculated. For this Al sheet, the predicted α–S_o values were fitted to a second order polynomial in frequency, and α–A_o values were fitted to a fourth order polynomial. Averaged results are given below:

Wave Mode	Deviation (dB/m)	Percent Deviation (%)	Data Count
Symmetric case:	0.84	11.3	26
Asymmetric case:	1.74	19.1	36

Because the attenuation values were lower than in the PS case, deviation values were smaller, but percent deviation was 11 and 19%. The higher percent deviation for the asymmetric case resulted from large deviation at the mid-frequency range. For the 2-mm Al-1100 sheet, Lamb-wave attenuation is found to match the theoretical prediction. Agreement was slightly poorer than it was in the PS sheets in Section 5.1.

The second Al-1100 plate was thicker, with 6.4-mm thickness (G2b). Figure 7a,b show α–S and α–A for the 6.4-mm thick plate in blue and red symbols, respectively. In Figure 7a, a set of α–S values are plotted in square symbols from 200 to 460 kHz, and calculated values of α–S_o are plotted in a solid blue curve. Blue and red dashed curves represent the bulk-wave attenuation coefficients, α_L and α_T. The velocity range of the dataset was from 5.9 to 2.2 mm/µs. In this case, the slow S_o segment around the velocity minimum at 390 kHz was included in the attenuation plot. The A_o mode contribution was negligible with the two-side summation processing. The observed α–S data matched well with the calculated α–S_o curve, indicating that α–S_o was measured. The maximum deviation was 4.7 dB/m, or 79%, of the calculated value at 286 kHz.

Figure 7b gives α–A values and calculated values of α–A_o in a solid red curve plus the dashed blue and red lines for α_L and α_T. The velocity range of these datasets was from 3.3 to 1.8 mm/µs, excluding the faster S_o mode. Differences of the two-sided signals were used to minimize the symmetric components. The α–A attenuation data followed the calculated α–A_o (red curve), which almost overlaps the red-dashed α_T line. The observed attenuation values ranged from 130 to 500 kHz. These deviated from the predicted values by as much as 2.61 dB/m, or 24%, of the calculated value at 381 kHz.

In order to evaluate the accuracy of attenuation values obtained, deviation and percent deviation were calculated. For this Al plate, the predicted α–S values were fitted sectionally to third and fourth order polynomials in frequency, and α–A values were fitted to a linear equation. Averaged results are as follows:

Wave Mode	Deviation (dB/m)	Percent Deviation (%)	Data Count
Symmetric case:	2.29	21.6	42
Asymmetric case:	1.01	11.5	27

Obtained deviation values are similar to the results of the thinner Al, but larger values were found for the symmetric case. For the 6.4-mm Al-1100 plate, Lamb-wave attenuation is also found to match the theoretical prediction. Agreement was comparable to the case of the 2-mm Al sheet given above.

5.2.2. Al-6061-T6

A large 12.7-mm thick plate (G3a) of 1.22 × 1.2 m was used for this part. Because of the increased thickness, the frequency range of the low-dispersion S_o mode is limited to about 100 kHz, and its attenuation level was low, as this alloy has low bulk-wave attenuation (see Table 1). The calculated α–S_o curve is plotted in Figure 8a, indicating α–S_o values of less than 1 dB/m below 140 kHz and reaching only 3.8 dB/m at the 200–300 kHz segment, where the S_o velocity is near the minimum. Lamb-wave intensity near the velocity minimum is often low, preventing α–S_o measurements. For these reasons, short-pulse excitation methods used in all other tests failed to produce a consistent S_o-mode attenuation dataset. In order to enhance Lamb-wave intensity at lower frequencies, sinewave pulses (or tone bursts) of 100 or 200 kHz center frequency and 40 µs duration (with 12 µs rise time to the maximum, followed by 0.8 dB/µs decay) were generated using an acoustic emission simulator (AES-1, AE Associates, Laguna Beach, CA, USA). The output was set at ±10 V, which was fed to an AET FC500 transducer mounted to the plate edge. This set-up was similar to the other tests as described in Section 3. Because of the size and weight of the plate, only one-side detection was used. With this procedure, α–S_o values were determined from 90 to 280 kHz and are plotted in Figure 8a. Blue + symbols are for the data with the 100-kHz excitation, whereas blue dots used 200-kHz excitation. The observed values are two to three times the calculated α–S_o values to 170 kHz, but these become comparable to the calculated value at higher frequencies. The low frequency data can hardly be considered to agree to theory, but the values at 220 to 270 kHz can be treated as being matched to the prediction. In this case, the detected waveforms (taken from 100 to 600 mm distances in 100-mm steps using a point-contact KRN receiver) consisted of the S_o mode only. For the 100-kHz excitation, fast S_o mode (5.6 to 2.1 mm/µs) was strong (see Figure 9a), whereas slow S_o mode (2.5 to 1.0 mm/µs) was strong using 200-kHz excitation (Figure 9b). As the limit of α–S_o measurements appears to be at 1 to 2 dB/m, large deviations are unavoidable. Still, the observed α–S_o values are in the same general range as predicted, and an increasing trend with frequency is revealed.

For the α–A attenuation measurements, the usual short-pulse excitation method was used using an AET FC500 transducer through a wedge as a transmitter. Again, the one-sided detection method was used, but the S_o mode was weak and removed during the WT analysis procedures. Measured α–A attenuation data are plotted with red dots in Figure 8b, together with the calculated α–A_o attenuation (red curve). Agreement between measured and calculated values is moderately good.

In order to assess the accuracy of attenuation values obtained, deviation and percent deviation were calculated. For this Al plate, the predicted α–S_o values were fitted to third and fifth order polynomials in frequency, and α–A_o values were fitted to a linear equation. Averaged results are as follows:

Wave Mode	Deviation (dB/m)	Percent Deviation (%)	Data Count
Symmetric case:	0.81	66.6	46
Asymmetric case:	0.75	17.7	34

Obtained values are similar to the results of the thinner Al for the asymmetric case, but a larger percent deviation value was found for the symmetric case. This resulted from the limitation of the α–S_o measurements in this work because the attenuation was low. The maximum deviation of α–S_o reached 2.38 dB/m (and 260%) at 130 kHz. For the 12.7-mm Al-6061 plate, Lamb-wave attenuation is found to approximately match the theoretical prediction. Agreement for the symmetric case was poorer than for the Al-1100 cases given previously.

5.2.3. Al-7475-H18

A fully cold-worked Al-7475 alloy sheet was tested for Lamb-wave attenuation. This alloy was derived from Al 7075 by reducing Si and Fe impurities, which gives 40% higher fracture toughness. Cold-working is rarely used for structural Al alloys, but was utilized for superplasticity studies. Because of this special circumstance, no comparable material with adequate thickness for bulk-wave attenuation tests was available, even in the Al-7075 alloy. For comparison, α_L and α_T values of the 7075-T6 plate were used (cf. Table 1).

Figure 10a,b show α–S and α–A for the 2.7-mm thick sheet from two-sided tests in blue and red symbols, respectively. In Figure 10a, a set of α–S values are plotted in dot symbols from 250 to 500 kHz, and calculated values of α–S_o are plotted in a solid blue curve; also shown are dashed lines for α_L and α_T. The velocity range of the dataset was from 5.6 to 3.0 mm/µs. In this case, the slow S_o segment around the velocity minimum at 870 kHz was well beyond the attenuation test range, limited to 500 kHz. This limit was mainly imposed by the arrival of reflected signals at longer propagation distances due to the sheet size. The A_o-mode contribution was cut off in the WT analysis procedures. The observed α–S_o data matched moderately with the calculated α–S_o curve. The maximum deviation was 1.4 dB/m (41% of the calculated value) at 458 kHz. Figure 10b gives α–A_o values and calculated values of α–A_o in a solid red curve plus the blue and red dashed lines for α_L and α_T. The velocity range of these datasets was from 3.0 to 2.2 mm/µs, excluding the S_o mode. The observed α–A_o values were two to three times larger than the calculated ones. Lines for 2 α_T and 3 α_T (drawn in red dots and dash-dots) encompassed 10 out of 12 data points between them. This difference is apparently due to a lower α_T value used in the calculation. When α_T is taken to be 2.5 times, or 30.6 dB/m/MHz, agreement between observed and calculated becomes fair, giving a correct trend. This level of α_T value is similar to that of cold-worked Al-1100-H18. However, Al-1100 is three times softer in terms of Vickers hardness due to large alloying additions to Al-7475. Additionally, an increased α_T also increases α–S_o prediction. Further testing is needed to clarify the cause of the α–A_o discrepancy.

Lamb-wave attenuation tests on Al-7475 showed that the observed α–S_o and α–A_o values are in the same ranges as the calculated values. Because of large differences in α–A_o values, however, improved testing is required before these tests on Al-7475 can be used for the verification of theory. Underestimate of α_T is another likely cause. Enlargement of sheet width is one of the steps for refinement. It is also critical to obtain correct α_L and α_T values for thin sheets, although no applicable method is available at present [45].

5.3. Stainless Steel 410

A sheet of stainless steel 410 (410SS) was tested as a representative ferrous alloy. Although it was noted as an annealed sheet, its hardness was equal to that of a hardened and 650 °C-tempered condition (G5a in Table 1). Because of its low bulk-wave attenuation coefficients, a nominally annealed rod (G5b) was used for damping measurements. Tests resulted in very low values, i.e., α_L = 0.97 and α_T = 2.6 dB/m/MHz, respectively. As expected, α–S and α–A values were low, as plotted in Figure 11a,b. In Figure 11a, α–S_o values were in the same range as the calculated values, similar to the case of the Al-7475 sheet. In order to reduce data scattering effects, five wavelet frequency steps were averaged, giving a frequency step of 38.1 kHz. Above 900 kHz, observed α–S values decreased and seemed to follow α–S₁ curve, plotted in the blue dotted curve. For asymmetric modes, no attenuation data were obtained below 570 kHz, indicating that α–A_o was too low to measure using the present test method. At higher frequencies, a dozen attenuation data points were obtained. The frequency range of 570 to 1000 kHz is where A₁ mode is expected, but deviation was large below 800 kHz, making it difficult to associate the observed data to the A₁ mode at present.

From these 410SS test data and those of Al-7475 above, it is evident that the present test method requires much larger sample length and width than those listed in Table 1 when α_L and α_T are low and especially when thickness is large.

5.4. Soda Lime Glass

Two samples of soda lime glass, 2-mm (G6a) and 4.7-mm (G6b) thick, were tested for Lamb-wave attenuation. The thicker plate appears to be of tempered grade from the edge finishing, but an optical polarization test was inapplicable because of its darkened color. Direct bulk-wave attenuation tests could not be conducted due to the size and thickness. Thus, the data of a thicker soda lime glass of 5.6-mm thickness were used, namely, α_L = 8.7 dB/m/MHz and α_T = 16.2 dB/m/MHz (cf. Table 1). These are more than six times those of 410SS in Section 5.3. For the 2-mm glass sheet, observed α–S and α–A values are given in Figure 12a,b, respectively. Calculated values are given for S_o and A_o modes in each figure, as well as the bulk-wave attenuation lines, as in the previous figures. In Figure 12a, observed values were mostly below the predicated curve up to 900 kHz, although the general trend followed the calculation. This behavior was also found in Figure 12b for the α–A data. Deviations were larger in the asymmetric case than in the symmetric case. Calculation of α–S and α–A values relied on the bulk-wave attenuation of 5.6-mm thick glass plate, which was nearly three times the thickness of the sample used. Thus, the calculated values may be overestimated. When one assumes α_L = 4 dB/m/MHz and α_T = 8 dB/m/MHz, the calculated values are approximately halved (indicated by dotted curves in Figure 12a,b). The blue dotted curve in Figure 12a matched the observed data well. The red dotted curve for α–A in Figure 12b was slightly above the lower limits of the observed values, indicating that the α_T value needed to be slightly higher. Further refinements are possible by adjusting the assumed values of α_L and α_T. Presently, low levels of bulk-wave attenuation, as assumed above, cannot be measured for 2-mm thick glass. However, α–S and α–A can be obtained as shown here. In turn, these can be used to estimate bulk-wave attenuation of thin sheet materials. This approach is based on the Disperse calculation, verified using moderate to high attenuating plate samples.

Figure 13a,b show observed α–S and α–A values for 4.7-mm glass plate along with the calculated attenuation. For the symmetric case, α–S values follow the calculated α–S_o curve up to 550 kHz, with the observed values always being lower than the calculated curve. Above 650 kHz, the observed values were close to the calculated α–S₁ curve, again being lower than the prediction. In Figure 12b for the asymmetric case, the observed data followed the calculated α–A_o curve up to 1000 kHz. The data did not match the plotted A₁-mode attenuation between 400 and 800 kHz. For this glass plate, the values of α_L and α_T used in theoretical calculation were appropriate, although the α–S_o values were always below the prediction. However, increasing α_T will cause the calculated α–A_o curve to move above the observed data points. This inconsistency appeared in other cases as well, e.g., Al-7475 sheet (and PVC plate to be discussed subsequently).

Although it is immaterial to consider deviation statistics for these tests for low attenuation glasses, deviation and percent deviation were calculated. For the 2-mm glass sheet, the predicted α–S_o values were fitted to a third order polynomial in frequency, and α–A_o values were fitted to a linear equation. Averaged results are as follows:

Wave Mode	Deviation (dB/m)	Percent Deviation (%)	Data Count
Symmetric case 2 mm:	2.98	44.9	46
Asymmetric case 2 mm:	3.24	34.0	57

As expected from Figure 12a,b, deviations were larger than most values previously calculated. For the 4.7-mm glass plate, Lamb-wave attenuation data agreed with the theoretical prediction better than the test results on the 2-mm sheet. Again, a third order polynomial and a linear fitting equation were used. Averaged results are as follows:

Wave Mode	Deviation (dB/m)	Percent Deviation (%)	Data Count
Symmetric case 4.7 mm:	2.17	41.1	22 *
Asymmetric case 4.7 mm:	1.64	29.5	81
* Data below 600 kHz only.

In the 4.7-mm glass plate, deviation values were comparable to those of 6.4-mm Al plate, but percent deviation values were twice or more. Agreement between theory and experiment was poor in the case of the 2-mm glass sheet and was moderate for the 4.7-mm glass plate.

5.5. Polymethyl Methacrylate (PMMA)

Two PMMA samples of 1.8- and 6.3-mm thickness were used as listed in Table 1. Although the bulk-wave attenuation coefficients, α_L = 91 dB/m/MHz and α_T = 253 dB/m/MHz, were previously reported [44,45], these were for thick plates of 18 to 47 mm thickness, and the values of α_L and α_T were obtained for the samples used (cf. Table 1). Figure 14a,b show observed α–S_o and α–A_o values for the 1.8-mm sheet along with the calculated attenuation and dashed lines for α_L and α_T. For the symmetric case (Figure 14a), α–S_o values follow the calculated α–S_o curve (in solid blue) up to 450 kHz, with the observed values always being higher by 10–20% than the calculated curve. Here, results of three test series are plotted using different symbols. For the asymmetric case (Figure 14b), α–A_o values of three tests to 500 kHz are plotted together with the predicted α–A_o curve (in solid red). Again, the observed data points are always above the predicted curve, by approximately 25%. As the calculations of α–S_o and α–A_o used the measured values of α_L and α_T, one cannot attribute the differences to these values. However, an increase of 10–20% in α_T can bring theory and experiment into a good match, so this possibility needs further examination.

Calculated values of deviation and percent deviation were averaged and are listed below.

Wave Mode	Deviation (dB/m)	Percent Deviation (%)	Data Count
Symmetric case 1.8 mm:	7.1	13.1	67
Asymmetric case 1.8 mm:	16.5	28.3	83

Figure 15a,b show observed α–S_o and α–A_o values for the 6.3-mm sheet along with the calculated attenuation and dashed lines for α_L and α_T. One variation was added for α–A_o testing; the Olympus V221 shear transducer was used as a transmitter with the shear direction matching the plate normal, producing nearly identical attenuation data as other transmitters with wedge.

In this PMMA plate, the calculated attenuation and observed values were in better agreement than other cases, especially for the asymmetric case, as the following deviation statistics indicate.

Wave Mode	Deviation (dB/m)	Percent Deviation (%)	Data Count
Symmetric case 6.3 mm:	6.0	30.0	87
Asymmetric case 6.3 mm:	3.2	10.2	103

Here, percent deviation for the symmetrical case is larger, but this is due to low α–S_o values below 100 kHz, which are comparable to measurement error. The α_T value of this 6.3-mm plate was measured using four samples from four corners, 600 mm apart. Results were within ±5%, indicating a good uniformity. As was the case for the 1.8-mm sheet discussed above, the observed discrepancies cannot be attributed to bulk-wave attenuation, leaving the sensor–plate coupling as the main cause of the measurement error.

From the above examination of Lamb-wave attenuation of PMMA plates, a satisfactory agreement between theory and experiment is found for α–S_o and α–A_o.

5.6. Polyvinyl Chloride (PVC)

A PVC plate of 4.3-mm thickness was tested for its α–S and α–A, as it has high values of bulk wave attenuation [44,45]. Its α_L and α_T values from Table 1 were used for calculating Lamb-wave attenuation for comparison since both samples were cut from a single large plate. Figure 16a,b show α–S and α–A for the PVC plates in blue and red symbols, respectively. Two sets of test results are given. In Figure 16a, α–S values are mostly higher than the predicted values of α–S_o, plotted in the blue curve (and the α_L and α_T dashed lines in blue and red), with deviation of 3 to 25 dB/m._, The velocity range of these datasets was from 2.1 to 1.2 mm/µs, excluding the A_o mode after the summation processing. Both observed α–S data initially followed the calculated α–S_o curve up to 60 kHz, but α–S increased along a straight line at higher frequencies. This behavior was also observed in the 1.8-mm thick PMMA in the preceding section. Figure 16b gives α–A values from two tests. The red curve is calculated values of α–A_o (plus the α_L and α_T dashed lines in blue and red). The velocity range of these datasets was from 1.2 to 0.6 mm/µs, excluding the S_o mode, calculated through the use of two-sided signal differences. Any residual S_o contribution was discarded in the WT analysis. The α–A attenuation data showed gradual increase, straddling the calculated α–A_o (solid red curve) and nearly overlapping the red-dash α_T line from 40 to 100 kHz. Deviations from the calculated curve were less than those in the symmetrical mode, but the maximum still reached 17 dB/m.

The deviation statistics for this PVC are given below.

Wave Mode	Deviation (dB/m)	Percent Deviation (%)	Data Count
Symmetric case:	10.4	37.1	36
Asymmetric case:	8.5	14.9	41

These values were slightly higher than corresponding ones for the 6.3-mm thick PMMA, but these test series can be deemed comparable.

5.7. High-Density Polyethylene (HDPE)

An HDPE plate of 7.1-mm thickness was tested for its α–S and α–A, as it also has high values of bulk-wave attenuation [44,45]. The α_L and α_T values, listed in Table 1, were used for calculating Lamb-wave attenuation for comparison. Figure 17a,c show observed α–S and α–A values for the HDPE plate in blue and red symbols, respectively, together with the theoretical results. Identical test set-ups were used, but some discrepancies were found between the two sets. Because of high attenuation, special procedures had to be used in Disperse calculations for α–S and α–A, which were kindly conducted by Lowe, M. In Figure 17a, two sets of α–S_o values are given. The velocity range of these datasets was from 2.1 to 1.0 mm/µs. These mostly followed the calculated curve for α–S_o, but one set showed larger deviations over 50–90 kHz. As previously observed for PVC, this region followed a straight line from 40 to 100 kHz. The origin of this behavior is unknown, but here another dataset did not show the same behavior, suggesting the straight-line increase is likely to be an erratic behavior from sensor coupling.

Figure 17b shows α–S values vs. frequency to 500 kHz. At frequencies above 120 kHz, only attenuation of higher modes was detected. For one test (shown in dots), test data followed the predicted α–S₁ and α–S₂ curves (shown in dotted and dashed curves) to 370 kHz. The other test showed slightly lower attenuation above 300 kHz, but joined the other curve above 370 kHz. The observed data followed theoretical prediction for α–S₁ and α–S₂ well. In the higher frequency ranges for S₁ to S₃ modes, however, spectrograms from WT analyses are always diffuse. Thus, the observed good agreement between theory and experiment is surprising and requires more extensive studies before a definitive conclusion can be drawn.

Results for α–A_o are plotted in Figure 17c. Observed data points followed the calculated α–A_o curve to 110 kHz. Three datasets were plotted, two of which used pulse excitation (+ and triangles), and the third used 50-kHz sine pulse (dots). The velocity range of these datasets was from 1.1 to 0.6 mm/µs. For these asymmetric tests, data fitted better to the calculated curve than for the symmetric case above (Figure 17a), and the deviation was comparable to that of PS.

The deviation statistics for this HDPE plate are given below.

Wave Mode	Deviation (dB/m)	Percent Deviation (%)	Data Count
Symmetric case:	8.0	24.7	28
Asymmetric case:	4.6	13.0	53

This HDPE plate showed the same general trend in Lamb-wave attenuation behavior as those found in PS, PMMA, and PVC, even though these four materials have different bulk-wave attenuation coefficients. In terms of general trend in Lamb-wave attenuation, Al-1100 and glass also matched with theory. However, the data on Al-6061, Al-7475, and stainless steel 410 were limited, and the only conclusion that can be drawn is that the data on these three alloys were generally consistent with theoretical prediction.

5.8. Glass-Fiber Reinforced Plastic (GFRP)

A 2.3-mm thick GFRP sheet with cross-ply reinforcement was tested for Lamb-wave attenuation. As this is anisotropic, theoretical predictions for α–S_o and α–A_o values are not available, even though its bulk-wave attenuation coefficients were measured using laminated test samples made from the same sheet as listed in Table 1. Figure 18a,b show observed α–S_o and α–A_o values for the GFRP sheet in blue and red symbols, respectively. Lamb waves propagated along the 0°-fiber direction. In both figures, the experimental α–S_o and α–A_o values were near the lines representingits bulk-wave attenuation coefficients, α_L and α_T, plotted in dashed blue and red lines. These were given by α₃₃ and α₃₂. Here, the surface normal, 90°, and 0° fiber directions are designated as the 1, 2, and 3 axes, respectively, following Prosser [46]. In Figure 18a, a dotted blue line is added, which represents α_L = α₂₂. Initially, 0° and 90° directions were assumed to be equivalent, but subsequent measurements revealed clear differences reaching nearly twice in both longitudinal and shear attenuation. This is reflected in dashed and dotted blue lines of α_L. In order to make approximate comparisons with experiment, the Disperse-based parametric method was also used for the calculation of α–S_o and α–A_o values (solid blue and red curves), assuming isotropy.

Experimentally obtained α–S_o values in Figure 18a showed an increasing trend with frequency above 100 kHz (as they were too low to measure at frequencies below 100 kHz). Results of two test series are given, and both followed the same trend. At 250 kHz, α–S_o reached the α_L line and continued to increase at a similar rate to 430 kHz, where the value was about 50% higher than the α_L value. Figure 18b gives α–A_o plots of three test series. Data points followed the predicted α–A_o curve, which almost overlapped the α_T = α₃₂ line. Most of the observed α–A_o values exceeded the α–A_o prediction, with more than half by 10 to 20 dB, but remained below another α_T = α₃₁ line (red dotted), except one point. Despite these differences, the overall trend is recognized as a modest match between approximate theory and experiment. Although the significance of this theory–experiment matching is obscure because theory for isotropic medium was used, this finding nonetheless coincides with all other tests conducted in this study for isotropic plate samples. Because theory to deal with Lamb-wave attenuation in anisotropic solids has been published e.g., [4], exact predictions for α–S_o and α–A_o values are expected to become accessible, as was the case of Disperse, making an improved comparison of theory and experiment possible.

Note that the α_T value used in the calculation was that for α₃₂ = 445 dB/m/MHz (transverse wave traveling along the fiber direction, with the polarization along the crossing in-plane fiber direction). See Table 1 for complete listings. With the polarization along the surface normal, α₃₁ is 72% higher at 861 dB/m/MHz. Thus, the experimental α–A_o values fall between these two α_T lines. This may point to a possible resolution through the anisotropic calculation, which incorporates contributions to attenuation from different vibration modes, e.g., α₃₂ and α₃₁. In view of the dominance of transverse attenuation, this aspect is an important key to understanding the Lamb-wave behavior in anisotropic plates.

Lamb-wave attenuation tests of the XP-GFRP sheet indicated that observed α–S_o and α–A_o values were approximately equal to its bulk-wave attenuation coefficients. However, the selected α_L and α_T values were the lowest of the longitudinal and transverse attenuation coefficients, and the observed attenuation exceeded them. Because multiple vibration modes define Lamb waves, the results presented here are insufficient to suggest physical insight with respect to wave attenuation mechanisms. Complicating this task is the commanding roles of transverse-wave attenuation. Further exploration is necessary both from theory and experiment.

5.9. Summary of Lamb-Wave Attenuation

Lamb-wave attenuation data obtained in this study are summarized in

Table 2. Summary of Lamb wave attenuation values at approximate center frequencies.

Test	Material	αL	αT	Thickness	Symmetric			Asymmetric			Notes
No.		dB/m/MHz		mm	Freq. kHz	α–So (dB/m)		Freq. kHz	α–Ao (dB/m)
					Theory	Exp.			Theory	Exp.
G1	PS	39	113	1.38	300	19.7	16.4	350	37.4	34.3	Average of two.
G2a	Al-1100-H18	15.7	26.5	2.06	350	5.77	5.6	250	7.44	7
G2b	Al-1100-H18	15.7	26.5	6.38	300	6.71	7.9	250	6.71	6.6
G3	Al-6061-T6	2.7	6.38	12.7	150	1.27	1.9	450	4.84	4.3
G6a	Soda-lime glass	8.7	16.2	1.98	525	5.15	3.7	450	7.42	6.8
G6b	Soda-lime glass	8.7	16.2	4.72	300	3.15	1.5	500	8.12	7.8
G7a	PMMA	131	420	1.76	200	44.6	47.8	250	98.2	117
G7b	PMMA	106	216	6.3	200	67.5	68.7	250	55.5	56.6
G8	PVC	239	909	4.3	70	33.5	43.9	70	58.5	71.7
G9	HDPE	222	1010	7.09	60	33.1	43.8	60	55.1	54.1
G10	XP-GFRP	117	445	2.3	300	35.3 *	43.6	80	36.7	40.5	* αL value used.

. Data for Al-7475 and 410SS were omitted as their reliability is low. Theoretically predicted (Theory) and experimentally observed (Exp.) α–S_o and α–A_o values at near the middle of the frequency range of measurement for 11 sheets and plates are listed with bulk-wave attenuation coefficients and thickness. The observed attenuation values were averaged over ±5 kHz of the indicated frequency. In all cases, a comparison of theory and experiment at the mid-frequency produced a closer pairing than the previously listed deviation statistics. This is mainly due to large attenuation value differences at low and high ends of the frequency range. Still, the maximum percent deviation reached 20% (PMMA and HDPE), showing the need of additional study.

Two experimental approaches can improve Lamb-wave attenuation data to remove the uncertainty of contact sensing. One is to raise the pulse driving voltage in combination with an arbitrary waveform generator. Power amplifiers to 1 MHz are available commercially, producing ±300 V output into an ultrasonic transducer. For generating sub-MHz Lamb waves, ultrasonic transducers are better suited than a high-power laser. The other approach is to increase the length and width of samples of multiple thicknesses in low attenuation materials. It is important to have similar dimensions in the length and width because side reflections present serious interference in rectangular shapes. As for thicknesses, a thin sheet under 3-mm thickness expands the frequency range of the zeroth order modes, whereas a plate of a few times the thickness of the thin sample covers higher order modes at frequencies below 1 MHz. However, it is currently impossible to determine the bulk-wave attenuation coefficients on a thin plate with desired accuracy when the α_L and α_T values are under approximately 10 dB/m/MHz.

Next, the peak amplitude of received signals is summarized. Results are given in

Table 3. Peak displacement of detected Lamb-wave signals using a point contact sensor (KRN-BB-PC).

Test	Material	Thickness	Symmetric		Asymmetric
No.		mm	Transmitter	Peak Amplitude	Transmitter	Peak Amplitude
				nm		nm
G1	PS	1.38	NDT C16	0.08	NDT C16	0.117
G2a	Al-1100-H18	2.06	PAC Pico	1.31	NDT C16	0.39
G2b	Al-1100-H18	6.38	Oly. V101	1.46	NDT C16	1
G3	Al-6061-T6	12.7	PAC Pico	0.125	PAC Pico	0.114
G4	Al-7475	2.72	Oly. V101	0.62	KRN-BB-PCP	0.63
G5	410SS	3.16	Oly. V101	0.36	NDT C16	0.51
G6a	Soda-lime glass	1.98	Oly. V103	1.31	NDT C16	0.073
G6b	Soda-lime glass	4.72	Oly. V101	0.43	NDT C16	1.24
G7a	PMMA	1.76	Oly. V101	0.01	NDT C16	0.037
G7b	PMMA	6.3	Oly. V101	0.047	Oly. V103	0.034
G8	PVC	4.3	NDT C16	0.018	NDT C16	0.014
G9	HDPE	7.09	Oly. V101	0.015	NDT C16	0.017
G10	XP-GFRP	2.3	Oly. V101	0.26	Oly. V103	0.036

. Sample designation was the same as before, and the transmitter used and peak displacement in nanometers are listed for both wave modes, symmetric and asymmetric. Because of the use of calibrated point contact sensors (KRN-BB-PC), a quantitative comparison is possible in peak displacement, which ranged from 10 pm to 1.5 nm. The signals used in this comparison were chosen at the propagation distance of 200 mm, using the same receiving sensor. The calibration was against Rayleigh-wave motion, which is similar to Lamb-wave motion and generated vertical displacement that excited the KRN sensor [50]. The receiving sensitivity to Rayleigh waves was approximately 10 dB lower than that for normally incident waves. Lower peak-displacement values were found for tests with highly attenuating media, such as PMMA, PVC, and HDPE. Generally, Al, steel, and glass had higher values, but the peak levels varied. A part of this variation must be from the coupling of the transmitter to a plate. For attenuation measurements, maximizing Lamb-wave intensity is not critical as long as stable and adequate coupling is established. Note that sufficient signal qualities were obtained, allowing WT spectral analyses even with the peak displacements of 10–15 pm.

In order to examine the noise level of the KRN sensor, it was set on a GFRP sheet (G10) and produced 1.0 mV peak-to-peak output of a 10-cycle, 200-kHz wavelet at 300 mm from a transmitter (V101, excited by 275 mV peak-to-peak tone-burst signal). The output signal of 1.0 mV peak-to-peak has rms output voltage of 371 µV_rms, and the noise was 41.5 µV_rms, giving a signal-to-noise (SN) ratio of 19.0 dB. This corresponds to better than 100 fm resolution. When the same signal is averaged, output and noise voltages were 365 µV_rms and 2.67 µV_rms, respectively, resulting in an SN ratio of 42.7 dB and 10 fm resolution or better. Note that the resolution is conservatively estimated, but it cannot be verified at present. These results are tabulated below. As the present test set-up always uses signal averaging, the KRN sensor can detect 1 pm displacement, yielding 1 mV output with an SN ratio of better than 40 dB. Using this conversion factor of 1.0 pm/mV, the equivalent noise in displacement measurement is 4.3 fm/√Hz because the bandwidth was 400 kHz. This result shows that Lamb-wave signals with 10-pm peak can be adequately detected and recorded.

Type	rms Amplitude	rms Noise	SN Ratio	Equivalent Noise
Raw signal	371 µVrms	41.5 µVrms	19.0 dB	66 fm/√Hz
Averaged	365 µVrms	2.67 µVrms	42.7 dB	4.3 fm/√Hz

The use of laser interferometry/vibrometry allows non-contact sensing, but with high equipment cost. Some of the units have scanning capability, but a vibrometer head can be combined with a mechanical X–Y scanning system to scan a large area. With precision scanning, a positional accuracy of better than ±0.1 mm can be obtained. Non-contact and scanning features are positive attributes. In regard to the displacement sensitivity, careful technical evaluation is needed before getting a vibrometer for ultrasonic studies. PicoScale vibrometer (SmarAct, Oldenburg, Germany) has 1-pm resolution and 2.5-MHz bandwidth, while Polytec PSV-500 vibrometer (Polytec, Waldbronn, Germany) has 30-fm resolution (at 200 kHz) and 6-MHz bandwidth according to respective technical specifications. Since the resolution is the amplitude of the smallest signal above noise level, the displacement amplitude has to exceed at least 100 times the resolution in order to obtain a signal-to-noise (SN) ratio of 40 dB or higher. In the present Lamb-wave tests, signals below 100 pm were commonly observed (cf. Figure 3b and Table 3). As summarized above, peak displacements were down to 10 to 15 pm levels. Thus, a laser vibrometer must be selected with sufficient resolution to obtain the needed SN ratio. Note that 1-pm displacement detection with KRN-BB-PC sensor was achieved with 40-dB SN ratio, as described above. Thus, in order to eliminate measurement errors of a point-contact sensor emanating at the plate surface, proper selection and set-up of a laser vibrometer is required. For example, Staszewski at al. [52] reported 13.8 nm peak-to-peak displacement of A_o Lamb wave (converted from 6.5 mm/s peak-to-peak velocity at 75 kHz). This was measured at 15 mm away from a transmitter (8-mm diameter piezo wafer) on a 2-mm thick Al sheet, excited by 5 V peak-to-peak sinewave tone burst. This value is 6.2-times larger than the displacement produced by a similar piezo wafer, directly coupled to a KRN sensor, calibrated to normally incident waves. It appears that the vibrometer used (Polytec PSV-300) was not correctly operated, showing that even with a modern instrument, rigorous training is essential.

5.10. Implications for Lamb Wave Applications

In addition to the comparison between theory and experiment concerning Lamb-wave attenuation, a few more aspects deserve brief discussion. One of the observed features of Lamb-wave attenuation is the fact that the attenuation of both symmetric and asymmetric modes depends substantially on the α_T value of the propagating medium. In fact, the values of α_T and α–A_o differ less than 10% over most of the frequency range of interest, and it is possible to take α–A_o ≈ α_T. Because α–S_o values vary nonlinearly, simple approximation is not feasible. However, observed α–S_o values were often bounded by the α_L and α_T lines. Thus, approximating α–S_o with the average of α_L and α_T, or α–S_o ≈ (α_L + α_T)/2, has merit. This is valid when the S_o wave velocity is one-third higher than the A_o wave velocity. Approximate expressions are as follows:

α–S_o ≈ (α_L + α_T)/2 and α–A_o ≈ α_T.

(5)

As shown in Section 4, it is possible to obtain the α–S and α–A values up to the fourth order modes when α_L and α_T values are available by the use of the Disperse-based parametric method. That is, the access to Disperse software is not the prerequisite. Still, simple equations may be useful for the initial step.

Transverse wave attenuation had been difficult to find from the literature, but many values of α_T were recently listed in [45]. For example, two HSLA steels, SM50 and A533B, have α_T of 13.0 and 5.2 dB/m/MHz, whereas stainless steels, 304 and 17-4PH, have α_T of 17.1 and 26.3 dB/m/MHz, respectively. Using SM50 steel as a representative pipeline steel, the α–S_o approximation is valid to 120 kHz, when the thickness is assumed to be 15 mm. For this steel, α–S_o and α–A_o values were calculated and compared to Equation (5) in Figure 19. Both approximations from Equation (5) (green and red dash lines) agree well to the calculated α–S_o and α–A_o values (blue and red curves). For this steel, α–S_o = 0.25 dB/m at 30 kHz, and this value agrees well with the measured pipeline attenuation of 0.17 dB/m [13,14], which was based on AE wave propagation data for the distances from 2 to 300 m. Often, α–S_o values were undetected even with propagation distances of 10 to 100 m [14].

With low attenuation materials and thin plate shapes, it is normally not possible to measure the bulk-wave attenuation coefficients. Although it is also difficult to determine Lamb-wave attenuation coefficients, it is possible to get α–S_o and α–A_o values under certain conditions. The 2.0-mm thick glass sheet, discussed in Section 5.4, is an example. This allows one to estimate a likely α_T value. Because of weaker effects of α_L on α–S_o and α–A_o, it is expected to be difficult to get α_L, however. In this connection, a correlation between α_L and α_T values is useful. Figure 20 shows a cross-plot of α_L and α_T values from identical samples available from [14,45]. A total of 173 sets were given, 9 from [14] and the rest from [45]. Of these, 14 were excluded, as they exceeded α_L = 300 and α_T = 1200 dB/m/MHz, to avoid their oversized effects. The majority of data points had less than 80 and 200 dB/m/MHz for α_L and α_T. The linear regression analysis resulted in α_T = 2.79 α_L, with R² = 0.74. That is, on average, an α_T value is 2.79 times that of α_L. The dotted line indicates α_T = α_L. The physical significance of this empirical correlation requires further study. It is worth noting that 10% of materials showed lower α_T than α_L. The dotted line indicates α_T = α_L. This group consisted mostly of ceramics, but also included tungsten and Inconel 718 superalloy. All four Al₂O₃-based materials from single crystal sapphire to sintered almina discs have this behavior, suggesting its origin is from the chemical bonding.

6. Conclusions

Lamb-wave attenuation coefficients were determined for fourteen sheet and plate materials using ultrasonic transducer excitation and point-contact receivers. Sample materials ranged from steel, Al, and silicate glass with low attenuation to polymers and a fiber composite with much higher attenuation. Experimentally obtained Lamb-wave attenuation coefficients, α–S and α–A, for symmetric and asymmetric modes were mostly for the zeroth mode. Plots of α–S_o and α–A_o values against frequency were found to coincide reasonably well to theoretically calculated curves, which utilized the Disperse program and a parametric method based on Disperse calculations. The Disperse calculations used bulk-wave attenuation coefficients as input parameters, which were previously published or newly measured. Although further refinements are needed, this study confirmed that the Disperse program predicts Lamb-wave attenuation coefficients for elastically isotropic materials within the limitation of contact ultrasonic techniques used.

The calculated α–S and α–A values were found to be dominated by the α_T contribution. Often α–A_o coincided with a_T. This was in Viktorov calculations and in Disperse results, which were separated into the contributions from α_L and α_T by the use of the Disperse-based parametric method. The physical origin of this effect is unknown.

Displacement measurements were quantified using Rayleigh wave calibration, and peak displacements after 200-mm propagation were found to range from 10 pm to 1.5 nm. The point-contact sensor was capable of detecting 1-pm displacement with a 40 dB signal-to-noise ratio with signal averaging and had equivalent noise of 4.3 fm/√Hz.

Approximate expressions for α–S_o and α–A_o are obtained and a correlation was found between bulk-wave attenuation coefficients, i.e., α_T = 2.79 a_L, for over 150 materials.