Multi-Factor Influence Analysis on the Liquefaction Mitigation of Stone Columns Composite Foundation
Abstract
:1. Introduction
2. Numerical Model
2.1. Design Schemes of Stone Columns
2.2. Constitutive Model
2.3. Finite Element Model
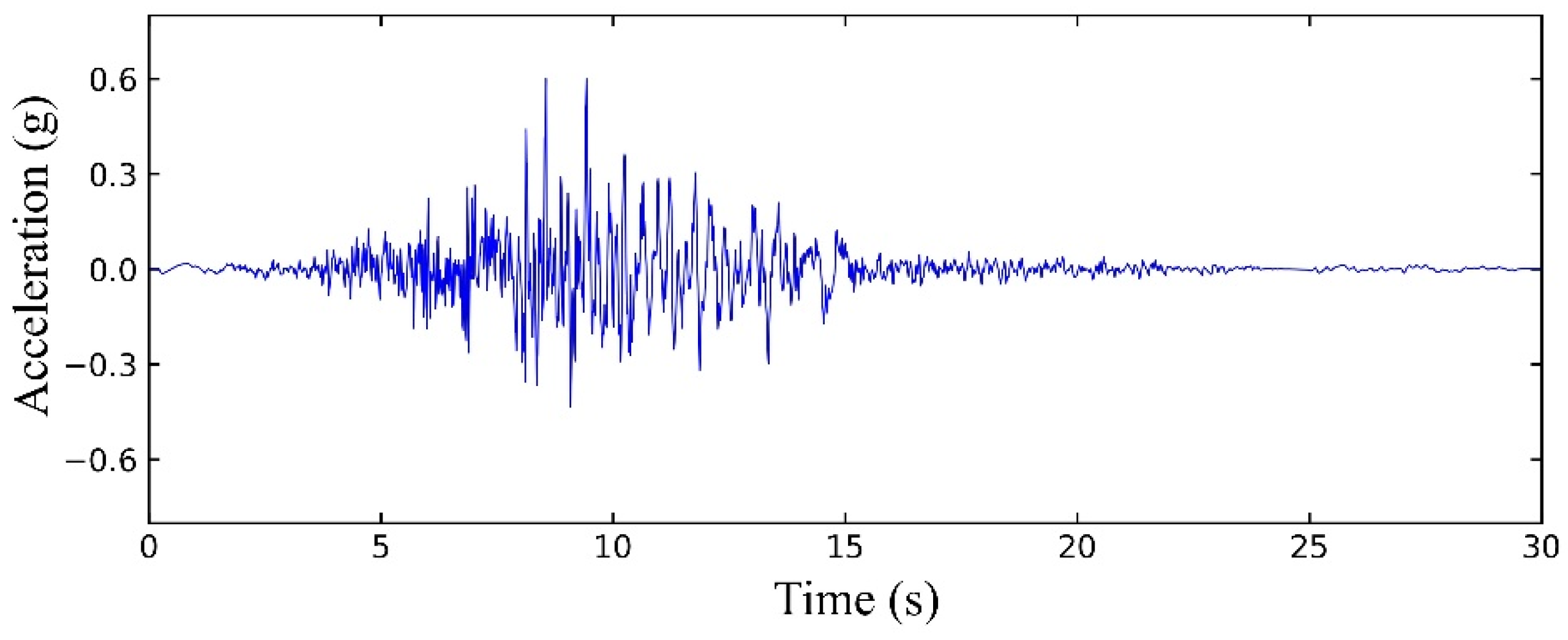
2.4. Computation Procedure
3. Simulation Results and Discussion
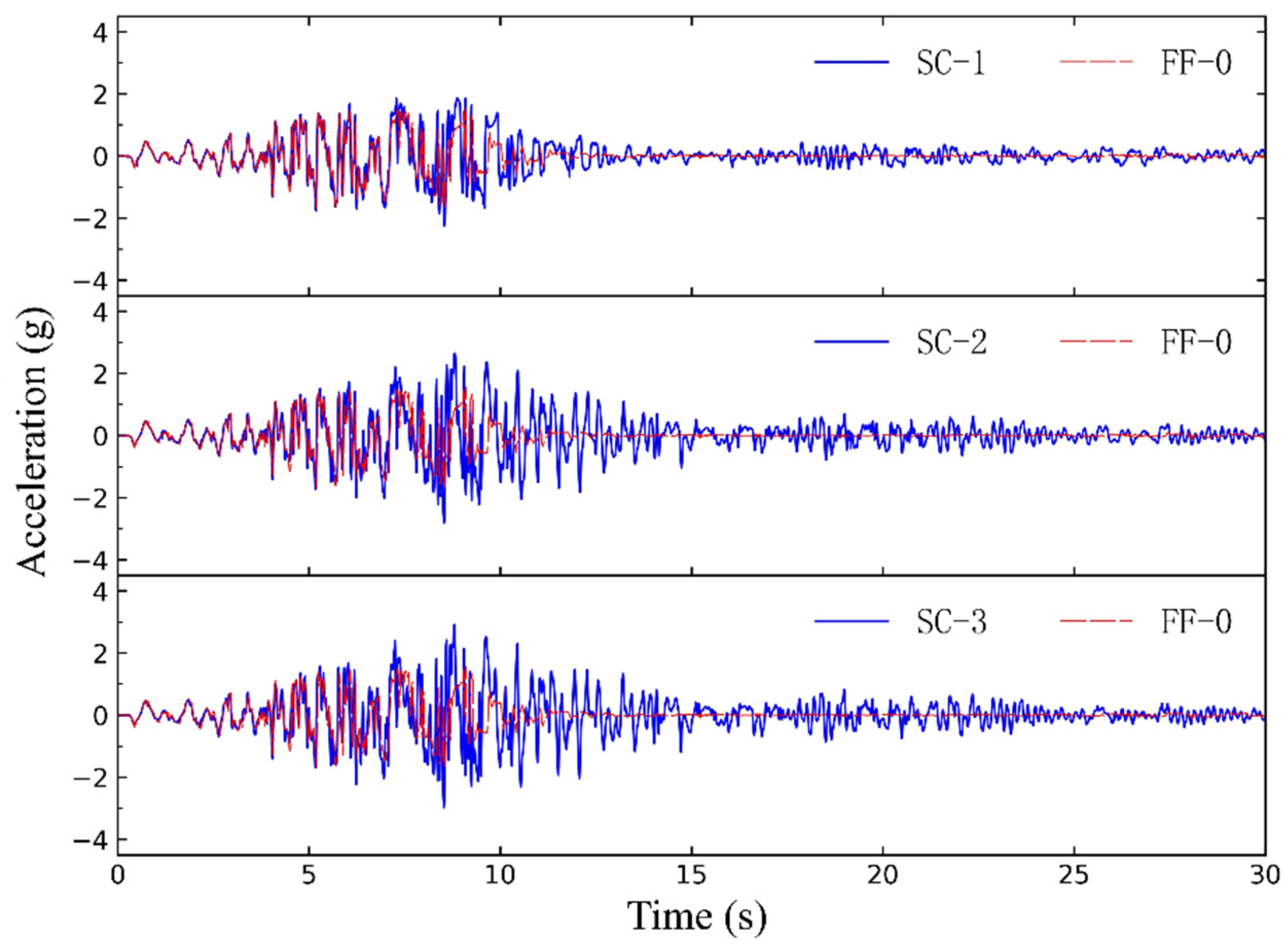
3.1. Simulation Results
3.2. Influence Analysis and Scheme Optimization
4. Recommendations for Practice
5. Conclusions
- (1)
- The stone columns can effectively reduce the peak value of the EXPPR in the surrounding soils and accelerate the pore pressure dissipation. In each simulation case, the time histories of EXPPR within the depth of stone columns present a similar development pattern and have approaching peak values.
- (2)
- The presence of stone columns will however lead to a larger PGA compared with that in free field, which can be attributed to the suppressing of vibration isolation and therefore higher transmitted ground accelerations. As a result, it could not be always beneficial to install stone columns to improve liquefiable ground which may underlie upper structures.
- (3)
- The peak values of EXPPR and the PGA are most sensitive to the permeability ratio of the stone columns among the investigated factors, which is followed by the replacement ratio, while the column diameter and shear stiffness ranging in the prescribed boundary cause negligible influence.
- (4)
- The reduction in peak values of EXPPRs and the rise in PGAs caused by the permeability ratio are rather significant when the ratio of stone columns’ permeability to surrounding soils’ reaches about 100 times. The ongoing increase of the ratio after that brings about a relatively smaller positive effect.
- (5)
- The analysis framework based on the orthogonal method and FE simulations can provide insights for design improvement in engineering practice. The framework is also referable and can be extended for other sensitivity studies on multiple factors.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Avilés-Campoverde, D.; Chunga, K.; Ortiz-Hernández, E.; Vivas-Espinoza, E.; Toulkeridis, T.; Morales-Delgado, A.; Delgado-Toala, D. Seismically Induced Soil Liquefaction and Geological Conditions in the City of Jama due to the M7. 8 Pedernales Earthquake in 2016, NW Ecuador. Geosciences 2020, 11, 20. [Google Scholar] [CrossRef]
- Potter, S.H.; Becker, J.S.; Johnston, D.M.; Rossiter, K.P. An overview of the impacts of the 2010–2011 Canterbury earthquakes. Int. J. Disaster Risk Reduct. 2015, 14, 6–14. [Google Scholar] [CrossRef] [Green Version]
- Chiaro, G.; Koseki, J.; Kiyota, T. An Investigation on the Liquefaction-Induced Sloped Ground Failure during the 1964 Niigata Earthquake. In Geotechnical Hazards from Large Earthquakes and Heavy Rainfalls; Springer: Tokyo, Japan, 2017; pp. 133–143. [Google Scholar]
- Sassa, S.; Takagawa, T. Liquefied gravity flow-induced tsunami: First evidence and comparison from the 2018 Indonesia Sulawesi earthquake and tsunami disasters. Landslides 2019, 16, 195–200. [Google Scholar] [CrossRef] [Green Version]
- Xie, D.Y. Soil Dynamics; Higher Education Press: Beijing, China, 2011; pp. 248–251. (In Chinese) [Google Scholar]
- Seed, H.B.; Booker, J.R. Stabilization of potentially liquefiable sand deposits using gravel drains. J. Geotech. Eng. Div. 1977, 103, 757–768. [Google Scholar] [CrossRef]
- Adalier, K.; Elgamal, A. Mitigation of liquefaction and associated ground deformations by stone columns. Eng. Geol. 2004, 72, 275–291. [Google Scholar] [CrossRef]
- Hausler, E.A. Influence of Ground Improvement on Settlement and Liquefaction: A Study Based on Field Case History Evidence and Dynamic Geotechnical Centrifuge Tests. Ph.D. Thesis, University of California, Berkeley, CA, USA, 2002. [Google Scholar]
- Ashford, S.A.; Rollins, K.M.; Case Bradford, V.S.; Weaver, T.J.; Baez, J.I. Liquefaction mitigation using stone columns around deep foundations: Full-scale test results. Transp. Res. Rec. 2000, 1736, 110–118. [Google Scholar] [CrossRef]
- Adalier, K.; Elgamal, A.; Meneses, J.; Baez, J.I. Stone columns as liquefaction countermeasure in non-plastic silty soils. Soil Dyn. Earthq. Eng. 2003, 23, 571–584. [Google Scholar] [CrossRef]
- Badanagki, M.; Dashti, S.; Paramasivam, B.; Tiznado, J.C. How do granular columns affect the seismic performance of non-uniform liquefiable sites and their overlying structures. Soil Dyn. Earthq. Eng. 2019, 125, 105715. [Google Scholar] [CrossRef]
- Zhou, Y.G.; Liu, K.; Sun, Z.B.; Chen, Y.M. Liquefaction mitigation mechanisms of stone column-improved ground by dynamic centrifuge model tests. Soil Dyn. Earthq. Eng. 2021, 150, 106946. [Google Scholar] [CrossRef]
- Gingery, J.R. Modeling reinforcing effects of ground improvement in mitigating seismic settlement. In Earthquake Geotechnical Engineering for Protection and Development of Environment and Constructions; CRC Press: Boca Raton, FL, USA, 2019; pp. 2651–2658. [Google Scholar]
- Wang, G.; Zhang, J.M. Advances in earthquake-induced liquefaction. Adv. Mech. 2007, 37, 575–589. (In Chinese) [Google Scholar]
- Elgamal, A.; Lu, J.; Forcellini, D. Mitigation of liquefaction-induced lateral deformation in a sloping stratum: Three-dimensional numerical simulation. J. Geotech. Geoenviron. Eng. 2009, 135, 1672–1682. [Google Scholar] [CrossRef]
- Asgari, A.; Oliaei, M.; Bagheri, M. Numerical simulation of improvement of a liquefiable soil layer using stone column and pile-pinning techniques. Soil Dyn. Earthq. Eng. 2013, 51, 77–96. [Google Scholar] [CrossRef]
- Rayamajhi, D.; Ashford, S.A.; Boulanger, R.W.; Elgamal, A. Dense granular columns in liquefiable ground. I: Shear reinforcement and cyclic stress ratio reduction. J. Geotech. Geoenviron. Eng. 2016, 142, 04016023. [Google Scholar] [CrossRef] [Green Version]
- Rayamajhi, D.; Boulanger, R.W.; Ashford, S.A.; Elgamal, A. Dense granular columns in liquefiable ground. II: Effects on deformations. J. Geotech. Geoenviron. Eng. 2016, 142, 04016024. [Google Scholar] [CrossRef]
- Demir, S.; Özener, P.T. Parametric investigation of effectiveness of high modulus columns in liquefaction mitigation. Soil Dyn. Earthq. Eng. 2020, 139, 106337. [Google Scholar] [CrossRef]
- Chan, A.H.C. A Unified Finite Element Solution to Static and Dynamic Problems in Geomechanics. Ph.D. Thesis, University College of Swansea, Swansea, UK, 1988. [Google Scholar]
- Zienkiewicz, O.C.; Chan, A.H.C.; Pastor, M.; Paul, D.K.; Shiomi, T. Static and dynamic behavior of soils: A rational approach to quantitative solutions: I. Fully saturated problems. Proc. R. Soc. Lond. A Math. Phys. Sci. 1990, 429, 285–309. [Google Scholar]
- Tang, L.; Zhang, X.; Ling, X. Numerical simulation of centrifuge experiments on liquefaction mitigation of silty soils using stone columns. KSCE J. Civ. Eng. 2016, 20, 631–638. [Google Scholar] [CrossRef]
- Sarımurat, S.; Işık, N.S.; Taşan, H.E.; Fırat, S. Numerical investigation of stone columns in liquefiable soils. Arab. J. Geosci. 2022, 15, 553. [Google Scholar] [CrossRef]
- Kumar, A.; Kumari, S.; Sawant, V.A. Numerical investigation of stone column improved ground for mitigation of liquefaction. Int. J. Geomech. 2020, 20, 04020144. [Google Scholar] [CrossRef]
- Zou, Y.X.; Wang, R.; Zhang, J.M. Analysis on the seismic response of stone columns composite foundation in liquefiable soils. Rock Soil Mech. 2019, 40, 2443–2455. (In Chinese) [Google Scholar]
- Li, P.; Dashti, S.; Badanagki, M.; Kirkwood, P. Evaluating 2D numerical simulations of granular columns in level and gently sloping liquefiable sites using centrifuge experiments. Soil Dyn. Earthq. Eng. 2018, 110, 232–243. [Google Scholar] [CrossRef]
- Qiu, Z.; Elgamal, A. Numerical simulations of LEAP dynamic centrifuge model tests for response of liquefiable sloping ground. In Model Tests and Numerical Simulations of Liquefaction and Lateral Spreading; Kutter, B.L., Manzari, M.T., Zeghal, M., Eds.; Springer: Cham, Denmark, 2020; pp. 521–544. [Google Scholar]
- Wu, Y.; Li, Y.L.; Hu, Q.J. Applied Mathematical Statistics; National University of Defense Technology Press: Beijing, China, 1995. (In Chinese) [Google Scholar]
- Yang, Z. Numerical Modeling of Earthquake Site Response including Dilation and Liquefaction. Ph.D. Thesis, Columbia University, New York, NY, USA, 2000. [Google Scholar]
- Yang, Z.; Elgamal, A.; Parra, E. Computational model for cyclic mobility and associated shear deformation. J. Geotech. Geoenviron. Eng. 2003, 129, 1119–1127. [Google Scholar] [CrossRef]
- Prevost, J.H. A simple plasticity theory for frictional cohesionless soils. Int. J. Soil Dyn. Earthq. Eng. 1985, 4, 9–17. [Google Scholar] [CrossRef]
- Mroz, Z. On the description of anisotropic work hardening. J. Mech. Phys. Solids 1967, 15, 163–175. [Google Scholar] [CrossRef]
- Parra-Colmenares, E.J. Numerical Modeling of Liquefaction and Lateral Ground Deformation including Cyclic Mobility and Dilation Response in Soil Systems. Ph.D. Thesis, Rensselaer Polytechnic Institute, New York, NY, USA, 1996. [Google Scholar]
- Yang, Z.; Lu, J.; Elgamal, A. OpenSees Soil Models and Solid-Fluid Fully Coupled Elements: User’s Manual. Available online: http://www.soilquake.net/opensees/OSManual_UCSD_soil_models_2008.pdf (accessed on 1 July 2021).
- Gingery, J.R.; Elgamal, A.; Bray, J.D. Liquefaction model calibration: Element-level versus 1-D site response. In Proceedings of the 6th International Conference on Earthquake Geotechnical Engineering (ICEGE), Christchurch, New Zealand, 1–4 November 2015. [Google Scholar]
- Law, H.K.; Lam, I.P. Application of periodic boundary for large pile group. J. Geotech. Geoenviron. Eng. 2001, 127, 889–892. [Google Scholar] [CrossRef]
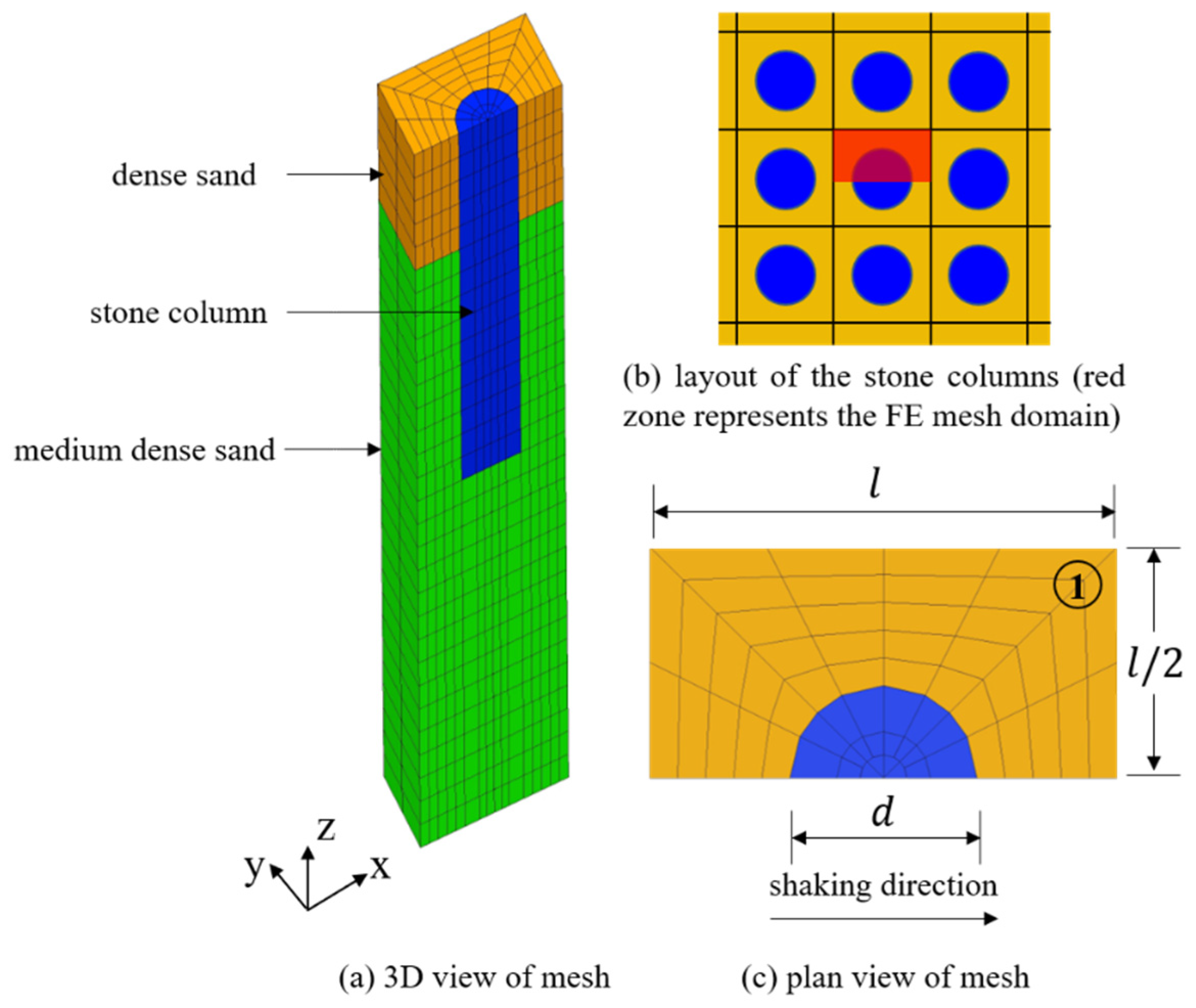

| Level | Diameter/m | Replacement Ratio/(%) | Shear Stiffness/kPa | |
|---|---|---|---|---|
| 1 | 0.8 | 10.1 | 2.20 × 105 | 1 |
| 2 | 0.9 | 12.9 | 2.75 × 105 | 5 |
| 3 | 1 | 15.7 | 3.30 × 105 | 10 |
| No. | Diameter | Replacement Ratio | Shear Stiffness | Permeability Ratio |
|---|---|---|---|---|
| FF-0 | - | - | - | - |
| SC-1 | 1 * | 1 | 1 | 1 |
| SC-2 | 1 | 2 | 2 | 2 |
| SC-3 | 1 | 3 | 3 | 3 |
| SC-4 | 2 | 1 | 2 | 3 |
| SC-5 | 2 | 2 | 3 | 1 |
| SC-6 | 2 | 3 | 1 | 2 |
| SC-7 | 3 | 1 | 3 | 2 |
| SC-8 | 3 | 2 | 1 | 3 |
| SC-9 | 3 | 3 | 2 | 1 |
| Parameters | Medium Dense Sand Dr = 50% | Dense Sand Dr = 80% | Stone |
|---|---|---|---|
| 1.9 | 2.1 | 2.14 | |
| Void ratio | 0.7 | 0.55 | 0.45 |
| Shear modulus/kPa | 1.00 × 105 | 1.30 × 105 | - |
| Bulk modulus/kPa | 2.33 × 105 | 2.60 × 105 | - |
| 33.5 | 36.5 | 48 | |
| 25.5 | 26 | 30 | |
| Peak shear strain | 0.1 | 0.1 | 0.1 |
| Reference confining pressure/kPa | 101 | 101 | 101 |
| Pressure dependent coefficient | 0.5 | 0.5 | 0.5 |
| Contraction parameter | 0.045/5.0/0.15 | 0.013/5.0/0.0 | 0.005/0.5/0.0 |
| Dilation parameter | 0.06/3.0/0.15 | 0.3/3.0/0.0 | 0.40/3.0/0.0 |
| Liquefaction parameter | 1.0/0 | 1.0/0 | 1.0/0 |
| Model | EXPPR | |||
|---|---|---|---|---|
| 2 m | 7 m | 12 m | ||
| FF-0 | 1.55 | 0.91 | 0.96 | 1.00 |
| SC-1 | 1.86 | 0.88 | 0.90 | 0.84 |
| SC-2 | 2.64 | 0.32 | 0.34 | 0.32 |
| SC-3 | 2.91 | 0.17 | 0.19 | 0.17 |
| SC-4 | 2.80 | 0.23 | 0.26 | 0.24 |
| SC-5 | 1.96 | 0.83 | 0.85 | 0.78 |
| SC-6 | 2.75 | 0.27 | 0.30 | 0.28 |
| SC-7 | 2.53 | 0.39 | 0.42 | 0.39 |
| SC-8 | 2.84 | 0.19 | 0.22 | 0.20 |
| SC-9 | 2.07 | 0.78 | 0.80 | 0.73 |
| Level | Diameter | Replacement Ratio | Shear Stiffness | Permeability Ratio |
|---|---|---|---|---|
| 1 | 2.47 | 2.40 | 2.48 | 1.96 |
| 2 | 2.50 | 2.48 | 2.50 | 2.64 |
| 3 | 2.48 | 2.58 | 2.47 | 2.85 |
| MD | 0.03 | 0.18 | 0.03 | 0.89 |
| sensitivity | 3 | 2 | 3 | 1 |
| Level | Diameter | Replacement Ratio | Shear Stiffness | Permeability Ratio |
|---|---|---|---|---|
| 1 | 0.48 | 0.53 | 0.47 | 0.85 |
| 2 | 0.47 | 0.47 | 0.47 | 0.35 |
| 3 | 0.48 | 0.43 | 0.49 | 0.22 |
| MD | 0.01 | 0.10 | 0.02 | 0.63 |
| sensitivity | 4 | 2 | 3 | 1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, P.; Lyu, W.; Liang, X.; Deng, J.; Li, C.; Yuan, Y. Multi-Factor Influence Analysis on the Liquefaction Mitigation of Stone Columns Composite Foundation. Appl. Sci. 2022, 12, 7308. https://doi.org/10.3390/app12147308
Chen P, Lyu W, Liang X, Deng J, Li C, Yuan Y. Multi-Factor Influence Analysis on the Liquefaction Mitigation of Stone Columns Composite Foundation. Applied Sciences. 2022; 12(14):7308. https://doi.org/10.3390/app12147308
Chicago/Turabian StyleChen, Pingshan, Weiqing Lyu, Xiaocong Liang, Jiangxu Deng, Chong Li, and Yong Yuan. 2022. "Multi-Factor Influence Analysis on the Liquefaction Mitigation of Stone Columns Composite Foundation" Applied Sciences 12, no. 14: 7308. https://doi.org/10.3390/app12147308
APA StyleChen, P., Lyu, W., Liang, X., Deng, J., Li, C., & Yuan, Y. (2022). Multi-Factor Influence Analysis on the Liquefaction Mitigation of Stone Columns Composite Foundation. Applied Sciences, 12(14), 7308. https://doi.org/10.3390/app12147308







