Combined Shear-Tension Loading of Composite Dowels in Cracked Concrete—Experimental Investigations and Design
Abstract
:1. Introduction
2. Experimental Investigations
2.1. Test Program
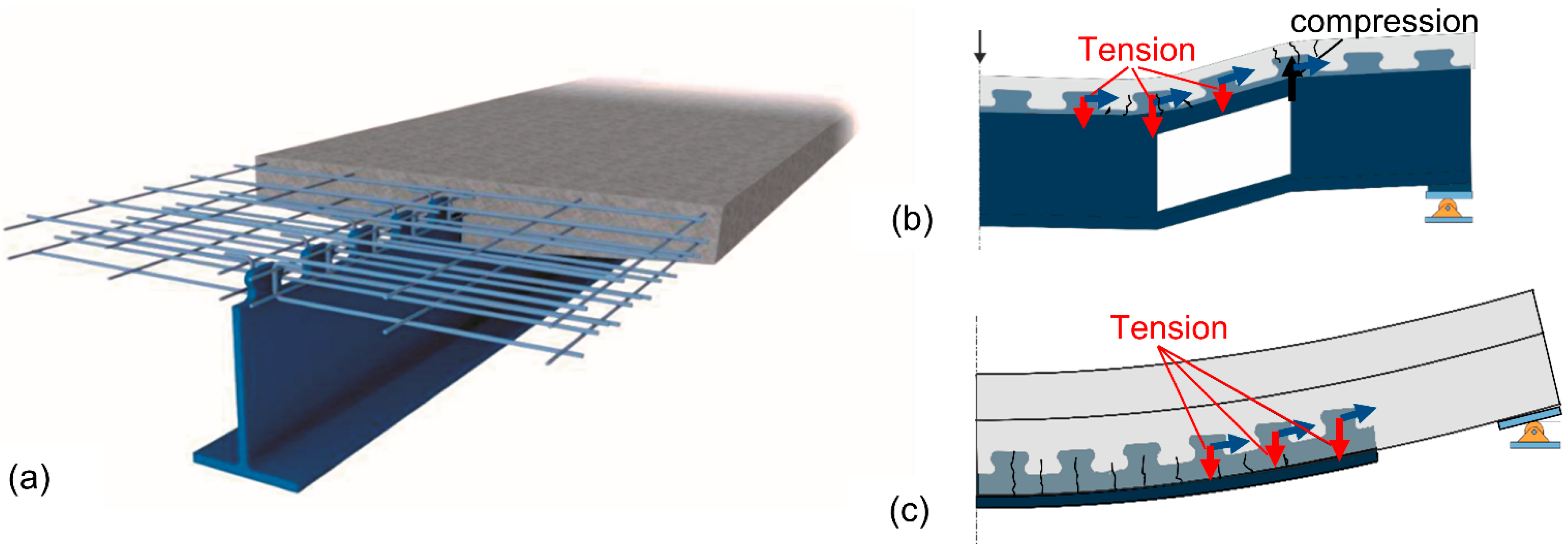
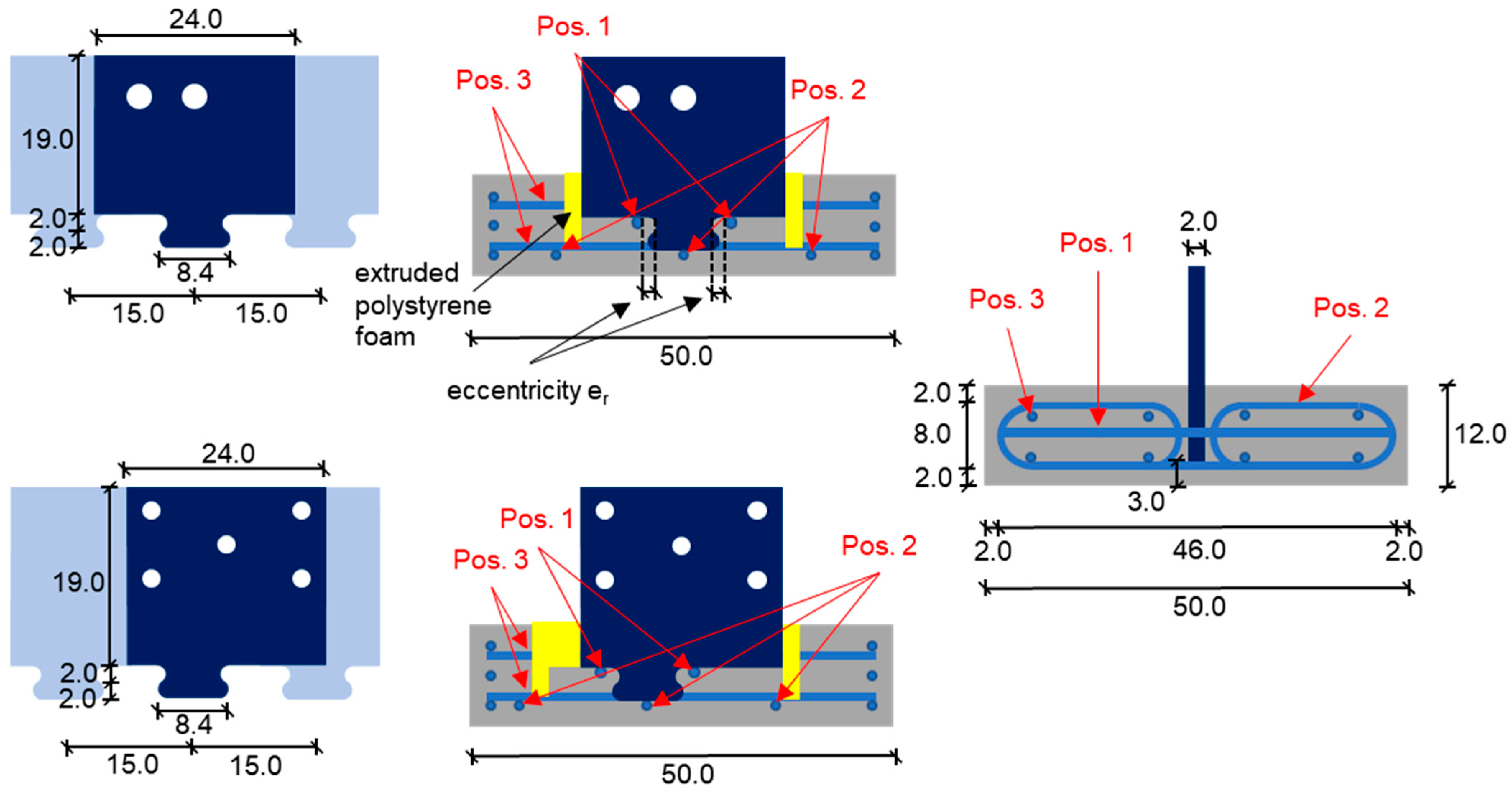
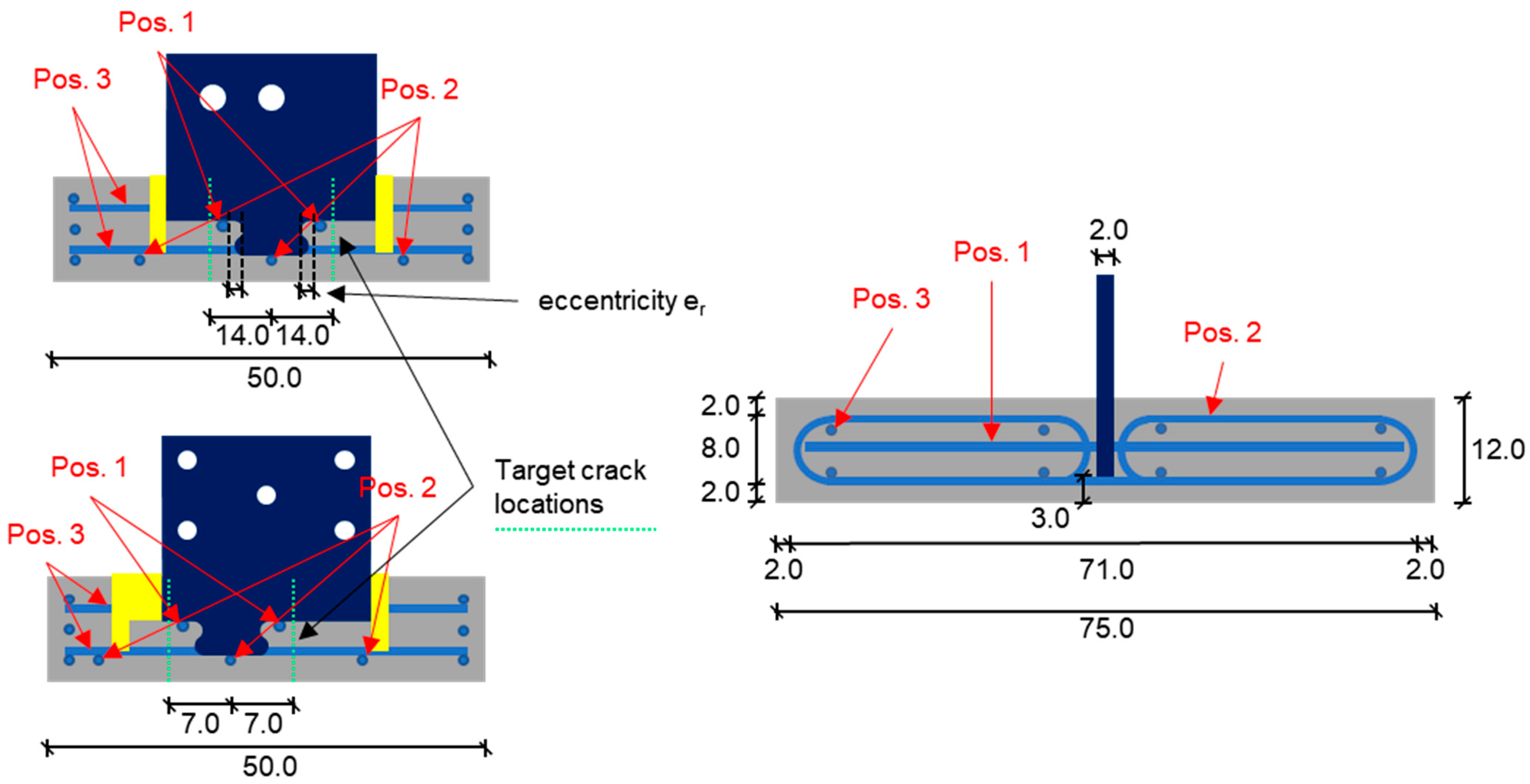
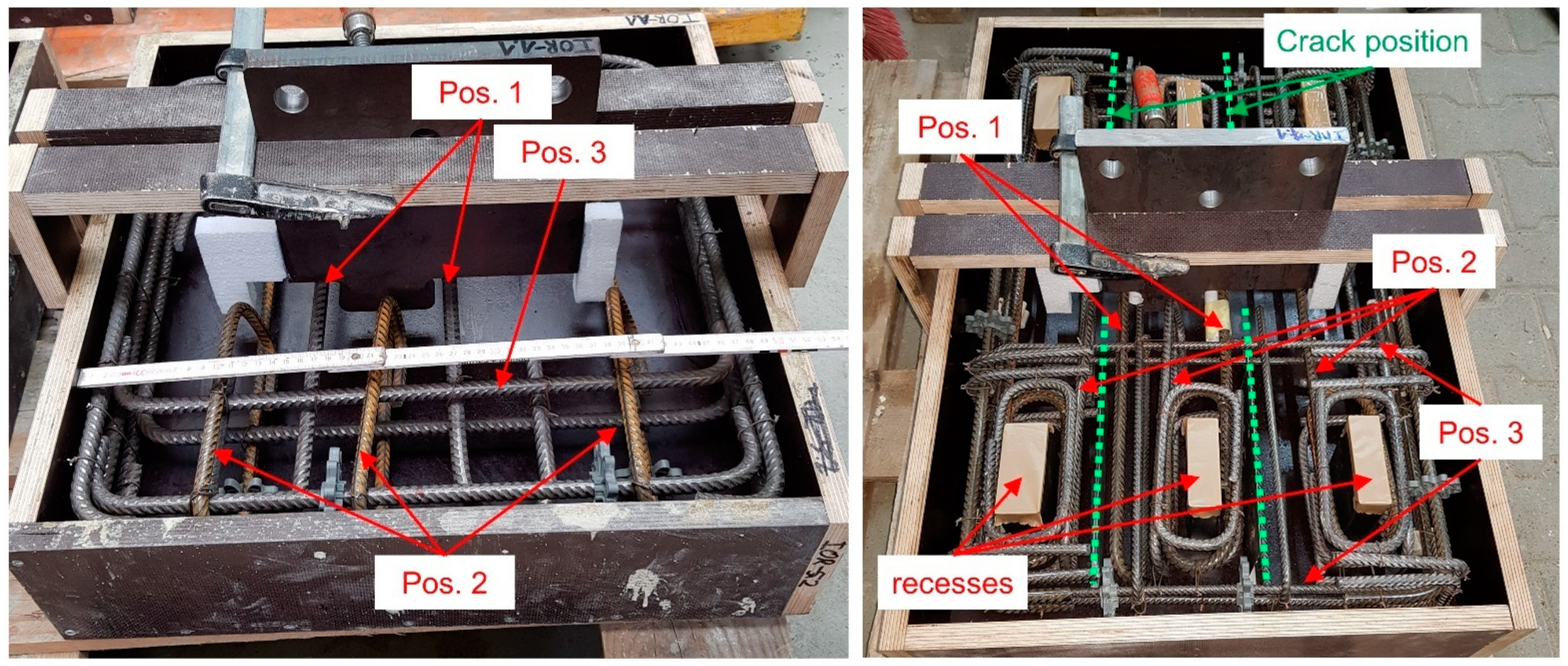
2.2. Test Specimen
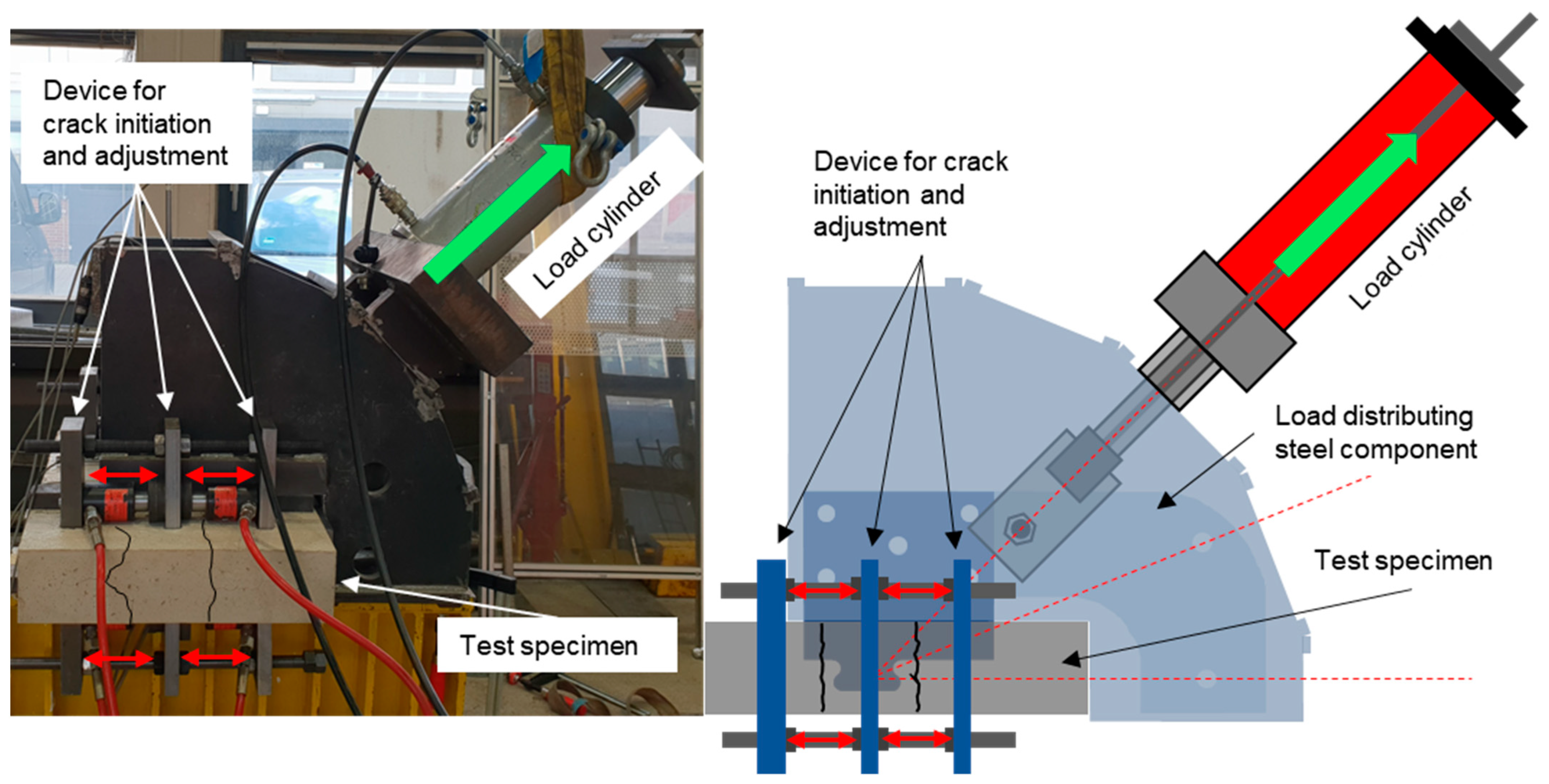
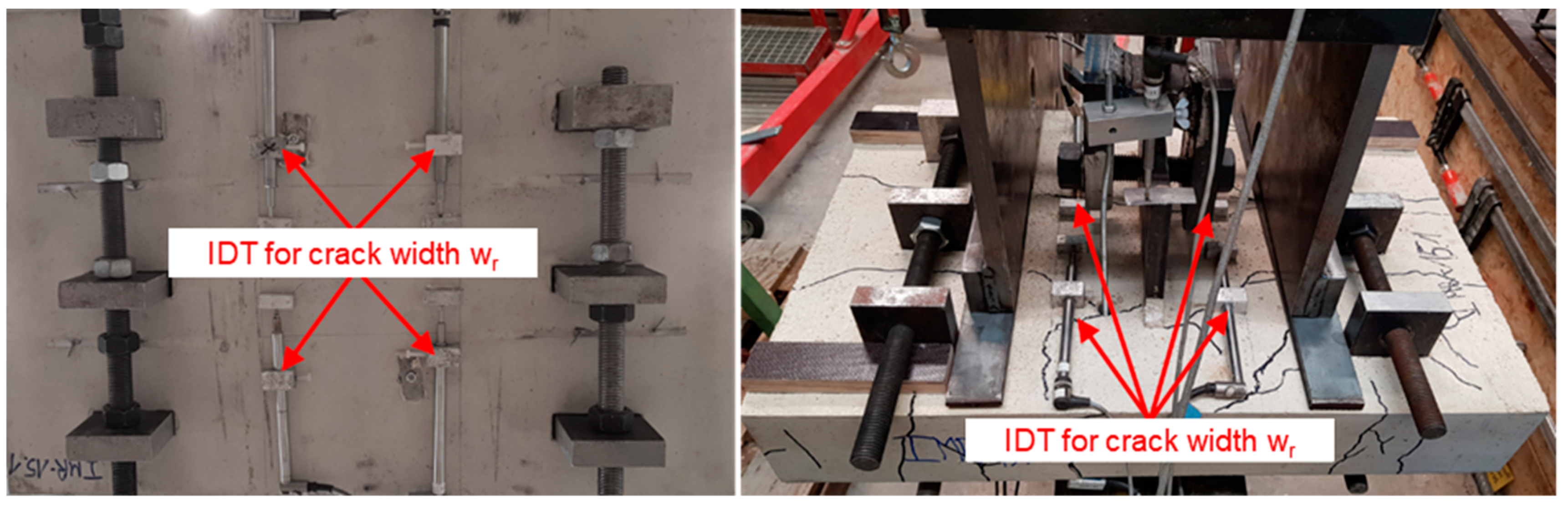
2.3. Test Rig, Procedure, and Measurements
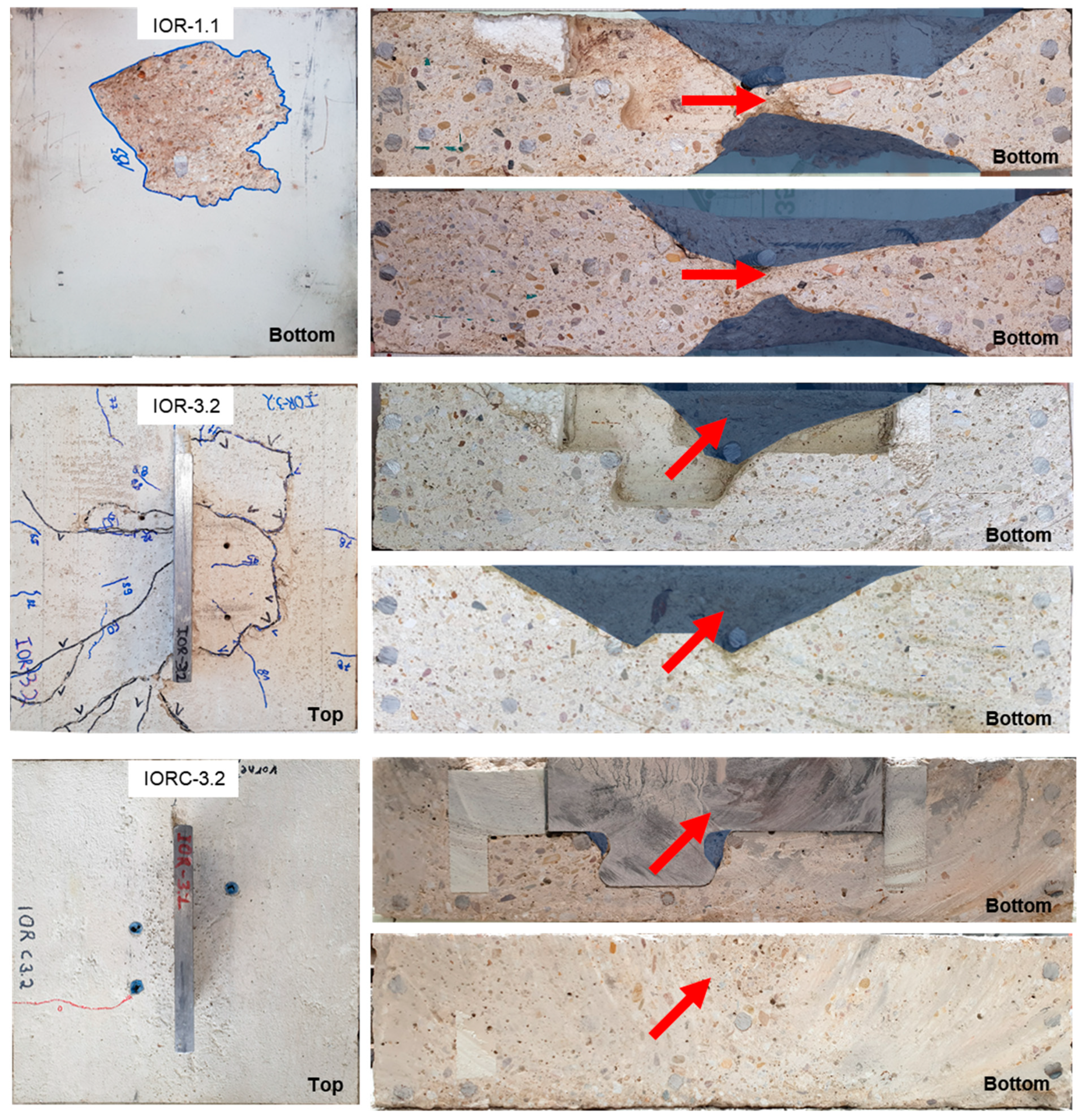
2.4. Test Results
3. Conclusions
- The load-bearing behaviour of single puzzle-shaped composite dowels was investigated for several load directions in undamaged and damaged (cracked) concrete by means of 32 tests.
- Concrete damage in terms of transverse cracks is proven to reduce the dowels’ load-bearing capacity.
- Pry-out failure is significantly impacted by wide cracks in the concrete regardless of the load’s direction.
- In the case of significant tensile loading, pry-out failure was not achieved within the presented test program since the dowels were prematurely pulled-out of the concrete. Nevertheless, the cracks and the crack-inducing stresses in the concrete impacted negatively the load-bearing capacity of the dowels, even in the case of pull-out failure.
- The unfavourable pull-out failure can be avoided by placing the dowel reinforcement as close as possible to the steel dowels, preferably within the steel dowels’ recesses.
- Securing the reinforcement bars at the steel dowels’ surface, for example, by tying the two bars in the front and back of each steel dowel together, leads to the best possible load-bearing behaviour, since the reinforcement can then contribute to the anchoring of the steel dowels and to the load distribution within the concrete.
- Depending on the position of the dowel reinforcement bars, two design modes were defined regarding shear-tension interaction.
- A quadratic interaction between pure shear and pure tensile load-bearing capacity shall be considered in the case of the dowel reinforcement being secured at the steel dowels’ surface.
- A less favourable design, namely, a linear interaction, may be considered if the dowel reinforcement is positioned within the steel dowels’ recesses without being secured at the steel dowels’ surface.
- In the case of cracked concrete, the defined shear-tension interaction curves (quadratic and linear) may be used by considering the reduced load-bearing capacity of the dowels in cracked concrete under pure shear and pure tensile loading.
4. Outlook
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Feldmann, M.; Wolters, K.; Hegger, J.; Claßen, M.; Christou, G.; Kurz, W.; Broschart, Y. Konsistentes Bemessungsmodell für Fertigungsoptimierte Verbunddübelleisten: Abschlussbericht P 1208; Forschungsvereinigung Stahlanwendung e. V. (FOSTA): Dusseldorf, Germany, 2021. [Google Scholar]
- Broschart, Y.; Kurz, W.; Wolters, K.; Christou, G.; Feldmann, M.; Hegger, J.; Claßen, M. Developement of a consistent design concept for composite dowels. In Proceedings of the 9th International Conference of Composite Construction in Steel and Concrete, Stromberg, Germany, 26–30 July 2021. [Google Scholar]
- Christou, G.; Wolters, K.; Broschart, Y.; Hegger, J.; Feldmann, M.; Kurz, W.; Claßen, M. Entwicklung eines konsistenten Bemessungsmodells für Verbunddübelleisten/Development of a consistent design concept for composite dowels. Bauingenieur 2021, 96, 212–223. [Google Scholar] [CrossRef]
- Kopp, M.; Christou, G.; Stark, A.; Hegger, J.; Feldmann, M. Integriertes Deckensystem für den Stahl- und Verbundbau. Stahlbau 2018, 87, 136–148. [Google Scholar] [CrossRef]
- Broschart, Y.; Kurz, W.; Wolters, K.; Christou, G.; Claßen, M. Influencing Parameters on the Load-bearing Capacity of Composite Dowels positioned close to the free Surface of Concrete Slabs. In Nordic Steel 2019, Proceedings of the Fourteenth Nordic Steel Construction Conference 2019, Copenhagen, Denmark, 18–20 September 2019; Jönsson, J., Ed.; Ernst & Sohn: Berlin, Germany, 2019; pp. 295–300. [Google Scholar]
- Hegger, J.; Claßen, M.; Schaumann, P.; Sothmann, J.; Feldmann, M.; Döring, B. Entwicklung einer integrierten Verbunddecke für nachhaltige Stahlbauten. Stahlbau 2013, 82, 11–17. [Google Scholar] [CrossRef]
- Berthellemy, J.; Lorenc, W.; Mensinger, M.; Ndogmo, J.; Seidl, G. Zum Tragverhalten von Verbunddübeln—Teil 2: Ermüdungsverhalten. Stahlbau 2011, 80, 256–267. [Google Scholar] [CrossRef]
- Harnatkiewicz, P.; Kopczyński, A.; Kożuch, M.; Lorenc, W.; Rowiński, S. Research on fatigue cracks in composite dowel shear connection. Eng. Fail. Anal. 2011, 18, 1279–1294. [Google Scholar] [CrossRef]
- Dudzinski, W.; Pekalski, G.; Harnatkiewicz, P.; Kopczynski, A.; Lorenc, W.; Kożuch, M.; Rowiński, S. Study on fatigue cracks in steel-concrete shear connection with composite dowels. Arch. Civ. Mech. Eng. 2011, 11, 839–858. [Google Scholar] [CrossRef]
- Seidl, G.; Stambuk, M.; Lorenc, W.; Kołakowski, T.; Petzek, E. Wirtschaftliche Verbundbauweisen im Brückenbau—Bauweisen mit Verbunddübelleisten. Stahlbau 2013, 82, 510–521. [Google Scholar] [CrossRef]
- Christou, G.; Hegger, J.; Classen, M. Fatigue of clothoid shaped rib shear connectors. J. Constr. Steel Res. 2020, 171, 106–133. [Google Scholar] [CrossRef]
- Christou, G.; Ungermann, J.; Wolters, K.; Hegger, J.; Claßen, M. Ermüdung von Verbunddübelleisten—Experimentelle Untersuchungen. Stahlbau 2020, 89, 388–398. [Google Scholar] [CrossRef]
- Christou, G.; Ungermann, J.; Wolters, K.; Hegger, J.; Claßen, M. Ermüdung von Verbunddübelleisten. Beton-Und. Stahlbetonbau 2020, 115, 355–363. [Google Scholar] [CrossRef]
- Wolters, K.; Christou, G.; Feldmann, M. Untersuchungen zum Risswachstum in Verbunddübelleisten. Bauingenieur 2019, 94, 216–227. [Google Scholar] [CrossRef]
- Christou, G.; Schmidt, C.; Hegger, J.; Classen, M. The effect of concrete fatigue on the cyclic behaviour of composite dowels—Derivation of a design approach based on experimental findings. Eng. Struct. 2022, 253, 113743. [Google Scholar] [CrossRef]
- Gallwoszus, J.; Claßen, M.; Hartje, J. Ermüdung von Verbundkonstruktionen mit Verbunddübelleisten (lokales Tragverhalten). BuSt 2015, 110, 382–393. [Google Scholar] [CrossRef]
- Deutsches Institut für Bautechnik. Stahlverbundträger mit Verbunddübelleisten in Klothoiden-und Puzzleform: Allgemeine Bauartgenehmigung Z-26.4-56; Forschungsvereinigung Stahlanwendung e. V. (FOSTA): Berlin, Germany, 2018. [Google Scholar]
- Claßen, M.; Adam, V.; Kueres, D.; Hegger, J. Zug-Schub-Interaktion von puzzleförmigen Verbunddübelleisten. BuSt 2017, 112, 334–345. [Google Scholar] [CrossRef]
- Classen, M.; Herbrand, M.; Adam, V.; Kueres, D.; Sarac, M. Puzzle-shaped rib shear connectors subjected to combined shear and tension. J. Constr. Steel Res. 2018, 145, 232–243. [Google Scholar] [CrossRef]
- Claßen, M.; Hegger, J. Verankerungsverhalten von Verbunddübelleisten in schlanken Betongurten. Bautechnik 2014, 91, 869–883. [Google Scholar] [CrossRef]
- Claßen, M.; Hegger, J. Ausstanzmodell mit Rissreibung für Verbunddübelleisten im gerissenen Beton. BuSt 2017, 112, 155–166. [Google Scholar] [CrossRef]
- Classen, M.; Hegger, J. Shear Tests on Composite Dowel Rib Connectors in Cracked Concrete. ACI SJ 2018, 115, 661–671. [Google Scholar] [CrossRef]
- Claßen, M.; Hegger, J. Assessing the pry-out resistance of open rib shear connectors in cracked concrete—Engineering model with aggregate interlock. Eng. Struct. 2017, 148, 254–262. [Google Scholar] [CrossRef]
- Classen, M.; Dressen, T. Experimental Investigations on Prestressed Concrete Beams with Openings. ACI SJ 2015, 112, 221–232. [Google Scholar] [CrossRef]
- Christou, G.; Wolters, K.; Broschart, Y. Verbunddübelleisten unter kombinierter Schub-Zug-Beanspruchung im gerissenen Beton. Bauingenieur 2019, 94, 228–236. [Google Scholar] [CrossRef]
- McMackin, P.J.; Slutter, R.G.; Fisher, J.W. Headed Steel Anchor under Combined Loading. AISC Eng. J. 1973, 10, 43–52. [Google Scholar]
- Lin, Z.F.; Liu, Y.Q. Model Experiment on Shear-Tension Interaction Relationship of Headed Stud. China J. Highw. Transp. 2015, 28, 80–86. [Google Scholar]
- Lin, Z.; Liu, Y.; He, J. Behavior of stud connectors under combined shear and tension loads. Eng. Struct. 2014, 81, 362–376. [Google Scholar] [CrossRef]
- Eligehausen, R.; Fuchs, W.; Sippel, T.M. Anchorage to concrete. Struct. Eng. Mater. 1998, 1, 392–403. [Google Scholar] [CrossRef]
- Eligehausen, R.; Mallée, R. Befestigungstechnik im Beton-Und Mauerwerkbau; Ernst & Sohn: Berlin, Germany, 2000; ISBN 3433011346. [Google Scholar]
- Yan, J.B.; Liew, J.R.; Zhang, M.H. Shear-Tension Interaction Strength of J-Hook Connectors in Steel-Concrete-Steel Sandwich Structure. Adv. Steel Constr. 2015, 11, 72–93. [Google Scholar]
- 91.010.30; 91.080.10; 91.080.40 (DIN EN 1994-1-1:2010); Eurocode 4: Bemessung und Konstruktion von Verbundtragwerken aus Stahl und Beton: Teil 1-1: Allgemeine Bemessungsregeln und Anwendungsregeln für den Hochbau; Beuth: Berlin, Germany, 2010.
- Claßen, M.; Gallwoszus, J.; Hegger, J. Einfluss von Querrissen auf das Schubtragverhalten von Verbunddübelleisten in schlanken Betongurten. Beton-Und Stahlbetonbau 2014, 109, 882–894. [Google Scholar] [CrossRef]


| Test | IOR-1 | IMR-8 | IMR-10 | IORC-2 | IOR-2 | IMR-20 | IOR-3 | IORC-3 | IMR-13 | IMR-21 | IOR-4 | IORC-4 | IMR-22 | IOR-5 | IORC-5 | IMR-17 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Angle [°] | 0 | 22.5 | 45 | 67.5 | 90 | |||||||||||
| crack width [mm] | 0 | 0.5 | 1.0 | 0 | 0 | 0.5 | 0 | 0 | 0.5 | 0.8 | 0 | 0 | 0.5 | 0 | 0 | 0.5 |
| Eccen-tricity er [mm] | 16 | 16 | 16 | 0 | 16 | 16 | 0 | 16 | 16 | 16 | 0 | 16 | 16 | 0 | 16 | 16 |
| Name | Parameters | Material Properties | Slip | Load-Bearing Capacity | Failure Mode ** | ||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Angle [°] | Crack Width wr [mm] | Cube Strength fcm,cube [N/mm²] | Elasticity Modulus Ecm * [N/mm²] | Tensile Strength fct,m [N/mm²] | δmax [mm] | δu [mm] | Pmax [kN] | PH,max [kN] | PV,max [kN] | ||
| IOR 1.1 | 0 | 0.00 | 42.3 | 24,400 | 2.9 | 6.1 | 7.6 | 186 | 186 | 0 | p-o-t&b |
| IOR 1.2 | 0.00 | 42.3 | 24,400 | 2.9 | 4.2 | 6.4 | 164 | 164 | 0 | p-o-t&b | |
| IMR 8.1 | 0.50 | 47.1 | 27,100 | 2.9 | 4,2 | 13,8 | 114 | 114 | 0 | p-o-b | |
| IMR 8.2 | 0.50 | 47.1 | 27,100 | 2.9 | 10,2 | 19,3 | 143 | 143 | 0 | p-o-b | |
| IMR 10.1 | 1.00 | 47.1 | 27,100 | 2.9 | 2.7 | 8.2 | 118 | 118 | 0 | p-o-b | |
| IMR 10.2 | 1.00 | 47.1 | 27,100 | 2.9 | 2.7 | 14.1 | 104 | 104 | 0 | p-o-b | |
| IORC 2.1 | 22.5 | 0.00 | 44.6 | 21,900 | 2.8 | 3.3 | 3.4 | 132 | 122 | 51 | p-o-t |
| IORC 2.2 | 0.00 | 44.6 | 21,900 | 2.8 | 2.2 | 2.5 | 136 | 125 | 52 | p-o-t | |
| IOR 2.1 | 0.00 | 42.3 | 24,400 | 2.9 | 3.9 | 4.8 | 129 | 119 | 49 | p-o-t | |
| IOR 2.2 | 0.00 | 42.3 | 24,400 | 2.9 | 5.4 | 8.6 | 122 | 113 | 47 | p-o-t | |
| IMR 20.1 | 0.50 | 44.3 | 22,700 | 2.8 | 6.2 | 10.9 | 94 | 87 | 36 | pull-o | |
| IMR 20.2 | 0.50 | 44.3 | 22,700 | 2.8 | 2.9 | 4.5 | 115 | 106 | 44 | pull-o | |
| IOR 3.1 | 45 | 0.00 | 42.3 | 24,400 | 2.9 | 2.8 | 4.3 | 90 | 64 | 64 | p-o-t |
| IOR 3.2 | 0.00 | 42.3 | 24,400 | 2.9 | 2.8 | 3.9 | 95 | 67 | 67 | p-o-t | |
| IORC 3.1 | 0.00 | 44.6 | 21,900 | 2.8 | 2.6 | 3.7 | 68 | 48 | 48 | pull-o | |
| IORC 3.2 | 0.00 | 44.6 | 21,900 | 2.8 | 1.3 | 3.3 | 58 | 41 | 41 | pull-o | |
| IMR 13.1 | 0.50 | 46.1 | 24,100 | 2.5 | 1.4 | 2.2 | 54 | 38 | 38 | pull-o | |
| IMR 13.2 | 0.50 | 46.1 | 24,100 | 2.5 | 2.1 | 3.1 | 50 | 35 | 35 | pull-o | |
| IMR 21.1 | 0.80 | 44.3 | 22,700 | 2.8 | 3.2 | 4.3 | 51 | 36 | 36 | pull-o | |
| IMR 21.2 | 0.80 | 44.3 | 22,700 | 2.8 | 2.7 | 3.9 | 56 | 40 | 40 | pull-o | |
| IOR 4.1 | 67.5 | 0.00 | 42.3 | 24,400 | 2.9 | 3.9 | 4.8 | 75 | 29 | 69 | p-o-t |
| IOR 4.2 | 0.00 | 42.3 | 24,400 | 2.9 | 3.6 | 5.5 | 73 | 28 | 67 | p-o-t | |
| IORC 4.1 | 0.00 | 44.6 | 21,200 | 2.8 | 1.6 | 2.8 | 51 | 19 | 47 | pull-o | |
| IORC 4.2 | 0.00 | 44.6 | 21,200 | 2.8 | 3.0 | 3.9 | 56 | 22 | 52 | pull-o | |
| IMR 22.1 | 0.50 | 44.3 | 22,700 | 2.8 | 3.7 | 4.9 | 38 | 15 | 35 | pull-o | |
| IMR 22.2 | 0.50 | 44.3 | 22,700 | 2.8 | 4.1 | 6.8 | 54 | 21 | 50 | p-o-t | |
| IOR 5.1 | 90 | 0.00 | 42.3 | 24,400 | 2.9 | 3.2 | 4.6 | 65 | 0 | 65 | p-o-t |
| IOR 5.2 | 0.00 | 42.3 | 24,400 | 2.9 | 2.2 | 3.6 | 66 | 0 | 66 | p-o-t | |
| IORC 5.1 | 0.00 | 44.6 | 21,200 | 2.8 | 0.8 | 1.1 | 48 | 0 | 48 | pull-o | |
| IORC 5.2 | 0.00 | 44.6 | 21,200 | 2.8 | 0.6 | 1.2 | 40 | 0 | 40 | pull-o | |
| IMR 17.1 | 0.50 | 46.1 | 24,100 | 2.5 | 1.4 | 2.2 | 38 | 0 | 38 | pull-o | |
| IMR 17.2 | 0.50 | 46.1 | 24,100 | 2.5 | 1.4 | 4.1 | 38 | 0 | 38 | pull-o | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Christou, G.; Wolters, K.; Ungermann, J.; Classen, M.; Hegger, J. Combined Shear-Tension Loading of Composite Dowels in Cracked Concrete—Experimental Investigations and Design. Appl. Sci. 2022, 12, 1449. https://doi.org/10.3390/app12031449
Christou G, Wolters K, Ungermann J, Classen M, Hegger J. Combined Shear-Tension Loading of Composite Dowels in Cracked Concrete—Experimental Investigations and Design. Applied Sciences. 2022; 12(3):1449. https://doi.org/10.3390/app12031449
Chicago/Turabian StyleChristou, Georgios, Kevin Wolters, Jan Ungermann, Martin Classen, and Josef Hegger. 2022. "Combined Shear-Tension Loading of Composite Dowels in Cracked Concrete—Experimental Investigations and Design" Applied Sciences 12, no. 3: 1449. https://doi.org/10.3390/app12031449
APA StyleChristou, G., Wolters, K., Ungermann, J., Classen, M., & Hegger, J. (2022). Combined Shear-Tension Loading of Composite Dowels in Cracked Concrete—Experimental Investigations and Design. Applied Sciences, 12(3), 1449. https://doi.org/10.3390/app12031449







