Design and Analysis on Decoupling Techniques for MIMO Wireless Systems in 5G Applications
Abstract
:1. Introduction
2. Mutual Coupling Issue of MIMO Systems
2.1. Causes of Mutual Coupling
- Surface-wave coupling. This coupling often occurs in microstrip antennas, due to sharing the same ground plane [15]. During the feeding process of the antenna element at the feed point, a distributed current is generated on the ground plane. This current will flow to nearby antenna elements, dispersing the energy and creating interference;
- Free-space coupling. This is because in the process of radiating electromagnetic (EM) waves outward from the excitation element, part of the energy will be coupled on the adjacent antennas and excite the adjacent antennas to generate new currents. In general, the contribution to the mutual coupling of the surface wave is smaller than the space wave due to the fields of surface wave decay as , which is slower than dependence of space wave coupling [14,16].
2.2. Negative Effects of Mutual Coupling
3. Evaluation Parameters of Mutual Coupling
3.1. S-Parameters
3.2. Envelope Correlation Coefficient (ECC)
4. The Methods of Decoupling
4.1. External Decoupling
4.1.1. NL Technique
4.1.2. PDE Technique
4.1.3. DGS Technique
4.1.4. Grounding Branch Technique
4.1.5. Metamaterials Technique
4.2. Internal Decoupling
4.2.1. Orthogonal Polarization Technique
4.2.2. Characteristic Modes Analysis
5. Additional Effects of Decoupling Techniques
5.1. Analysis of External Decoupling
- The NLs and grounding branches maintain the compact MIMO size without destroying the completeness of the antenna substrate. The additional decoupling structures can be co-formed with antenna elements to demand the insufficient matching impedance and improve the effective length of the antenna. The height of the MIMO system is not increased by those metal lines with a low profile. However, it is displayed from simulation results that the bandwidth will become narrow due to the introduction of decoupling lines. This can be explained by the fact that decoupling lines can be equal to capacitance and inductance, which lead to a rise in resonant Q-value;
- To resist mutual coupling, the design of the PDE shape is innovative and versatile. In the role of added metal patches, the impedance mismatch of antenna arrays can be solved and the impedance bandwidth can be extended. Moreover, the antenna elements loaded parasitic patches typically exhibit good gain, which is attributed to the effective suppression of the currents flow by the decoupling structure. To obtain a strong decoupling effect, the ADS composed of parasitic patches is indirectly placed above the substrate, which will lead to an increase in the height of the antenna systems;
- The DGS can be etched with simple crafts and a low profile. Due to expanding the length of the current path by added slots, it can be seen the improvement of efficient bandwidth. The introduced slots with inductance will act as the resonator circuits at the operating frequency, so another resonance frequency will appear. Additionally, the matching impedance had almost no influence by the DGS of the background. Moreover, it has heat dissipation and is friendly to high power MIMO systems such as mm-wave arrays. However, the radiation patterns display a negative effect for the DGS that it will create undesired back radiation due to its defected shape. Additionally, the phenomenon of DGS simulation that the central frequency will shift to the lower point under the impact of the bandgap effect should be noticed;
- As for metamaterials, they can be found with more significant coupling reduction effects than other techniques due to the effective control of EM wave transmission by those human-made left-hand materials. The metamaterial array is also beneficial for gain enhancement. Some 3D structures are often adopted in metamaterials construction, which is complex and takes up more space for realizing the desirable refractive indices, such as the common mushroom shape of EBG. Moreover, a challenge in metamaterials design is overcoming the state of narrow bandwidth and high transmission power, so they and slots [70] or other structures are often combined for antenna performance improvement in practical applications.
5.2. Analysis of Internal Decoupling
- Due to polarization diversity, the internal decoupling can further increase the isolation level within a common radiation element. In addition to the significant decoupling result, multipath effects and fading can be effectively mitigated by polarization diversity. Thus, these self-decoupling MIMO systems exhibit strong multi-directional robustness compared to single-polarized systems in some scattering propagation environments [71], demonstrating it has great potential to deploy in massive MIMO for large scale wireless communication devices;
- Due to the absence of extra decoupling structures, on the one hand, the MIMO systems using internal decoupling can be allowed for smaller volume, which makes the integration with the communication devices frame become easy to realize, and space utilization is enhanced again. On the other hand, this approach that generating uncorrelated polarization modes by elaborately arranging antenna elements to ensure low isolation will not change the excepted radiation characteristics of the MIMO antenna array;
- It can be noticed that the popular polarization selection is to produce linear polarization (LP) waves in the exciting literature on internal decoupling. Although LP waves have better diversity characteristics, which benefit the directional propagation of the signal, the direction of the LP wave is easily deflected when reflections are encountered. Compared to the LP antenna, the received signals using circular polarization (CP) waves are more stable regardless of the antenna orientation [72]. The MIMO arrays with orthogonal circular polarization can be considered when the signal transmission environment is complicated, such as [73].
- The antenna element with different polarized radiation often works as a compact block. Thus, the potential of building multiple antenna arrays is exhibited. It is well known that the increase in the number of antenna array elements leads to the expansion of the ergodic channel capacity. By briefly copying antenna blocks having polarization diversity, the multi-antenna arrays are convenient to be obtained, which facilitates modular design during the construction of MIMO systems. Although the polarization diversity is sufficient for isolation enhancement, the surface current cannot be suppressed. Therefore, only selecting internal decoupling is not enough for MIMO systems.
6. Conclusions and Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ezhilarasan, E.; Dinakaran, M. A Review on Mobile Technologies: 3G, 4G and 5G. In Proceedings of the 2017 Second International Conference on Recent Trends and Challenges in Computational Models, Tindivanam, India, 3–4 February 2017. [Google Scholar] [CrossRef]
- Beverage, H.H.; Peterson, H.O. Diversity Receiving System of R.C.A. Communications, Inc., for Radiotelegraphy. Proc. Inst. Radio Eng. 1931, 19, 529–561. [Google Scholar] [CrossRef]
- Peterson, H.O.; Beverage, H.H.; Moore, J.B. Diversity Telephone Receiving System of R.C.A. Communications, Inc. Proc. Inst. Radio Eng. 1931, 19, 562–584. [Google Scholar] [CrossRef]
- Jensen, M.A. A history of MIMO wireless communications. In Proceedings of the 2016 IEEE International Symposium on Antennas and Propagation (APSURSI), Fajardo, PR, USA, 26 June–1 July 2016. [Google Scholar] [CrossRef]
- Sengar, K.; Rani, N.; Singhal, A.; Sharma, D.; Verma, S.; Singh, T. Study and Capacity Evaluation of SISO, MISO and MIMO RF Wireless Communication Systems. Int. J. Eng. Trends Technol. 2014, 9, 436–440. [Google Scholar] [CrossRef] [Green Version]
- Moghe, S.; Upadhyay, R. Comparison of SISO and MIMO techniques in 802. In11n wireless local area network. In Proceedings of the 2009 International Conference on Emerging Trends in Electronic and Photonic Devices & Systems, Varanasi, India, 22–24 December 2009. [Google Scholar] [CrossRef]
- Foschini, G.J.; Gans, M.J. On Limits of Wireless Communications in a Fading Environment when Using Multiple Antennas. Wirel. Pers. Commun. 1998, 6, 311–335. [Google Scholar] [CrossRef]
- Li, J.; Zhang, X.; Wang, Z.; Chen, X.; Chen, J.; Li, Y.; Zhang, A. Dual-Band Eight-Antenna Array Design for MIMO Applications in 5G Mobile Terminals. IEEE Access 2019, 7, 71636–71644. [Google Scholar] [CrossRef]
- Qin, Z.; Geyi, W.; Zhang, M.; Wang, J. Printed eight-element MIMO system for compact and thin 5G mobile handest. Electron. Lett. 2016, 52, 416–418. [Google Scholar] [CrossRef]
- Li, Y.; Sim, C.; Luo, Y.; Yang, G. 12-Port 5G Massive MIMO Antenna Array in Sub-6GHz Mobile Handset for LTE Bands 42/43/46 Applications. IEEE Access 2018, 6, 344–354. [Google Scholar] [CrossRef]
- Wallace, J.W.; Jensen, M.A. Mutual coupling in MIMO wireless systems: A rigorous network theory analysis. IEEE Trans. Wirel. Commun. 2004, 3, 1317–1325. [Google Scholar] [CrossRef] [Green Version]
- Balanis, C.A. Antenna theory: A review. Proc. IEEE 1992, 80, 7–23. [Google Scholar] [CrossRef]
- Nikolic, M.M.; Djordjevic, A.R.; Nehorai, A. Microstrip antennas with suppressed radiation in horizontal directions and reduced coupling. IEEE Trans. Antennas Propag. 2005, 53, 3469–3476. [Google Scholar] [CrossRef]
- Wang, C.; Li, E.; Sievenpiper, D.F. Surface-Wave Coupling and Antenna Properties in Two Dimensions. IEEE Trans. Antennas Propag. 2017, 65, 5052–5060. [Google Scholar] [CrossRef] [Green Version]
- Chen, X.; Zhang, S.; Li, Q. A Review of Mutual Coupling in MIMO Systems. IEEE Access 2018, 6, 24706–24719. [Google Scholar] [CrossRef]
- Khayat, M.A.; Williams, J.T.; Jackson, D.R.; Long, S.A. Mutual coupling between reduced surface-wave microstrip antennas. IEEE Trans. Antennas Propag. 2000, 48, 1581–1593. [Google Scholar] [CrossRef]
- Lu, S.; Hui, H.T.; Bialkowski, M. Performance analysis of multiple-input multiple-output orthogonal frequency division multiplexing systems under the influence of antenna mutual coupling effect. IET Microw. Antennas Propag. 2009, 3, 288–295. [Google Scholar] [CrossRef] [Green Version]
- Lee, S.; Song, H. Accurate Statistical Model of Radiation Patterns in Analog Beamforming Including Random Error, Quantization Error, and Mutual Coupling. IEEE Trans. Antennas Propag. 2021, 69, 3886–3898. [Google Scholar] [CrossRef]
- Fletcher, P.N.; Dean, M.; Nix, A.R. Mutual coupling in multi-element array antennas and its influence on MIMO channel capacity. Electron. Lett. 2003, 39, 342–344. [Google Scholar] [CrossRef]
- He, Q.; Wang, B.; Shao, W. Radiation Pattern Calculation for Arbitrary Conformal Arrays that Include mutual-coupling effects. IEEE Antennas Propag. Mag. 2010, 52, 57–63. [Google Scholar] [CrossRef]
- Weiss, A.J.; Friedlander, B. Direction Finding in The Presence of Mutual Coupling. In Proceedings of the Twenty-Second Asilomar Conference on Signals, Systems and Computers, Pacific Grove, CA, USA, 31 October–2 November 1988. [Google Scholar] [CrossRef]
- Lui, H.; Hui, H.T. Direction-of-Arrival Estimation: Measurement using compact antenna arrays under the influence of mutual coupling. IEEE Antennas Propag. Mag. 2015, 57, 62–68. [Google Scholar] [CrossRef]
- Ye, Z.; Dai, J.; Xu, X.; Wu, X. DOA Estimation for Uniform Linear Array with Mutual Coupling. IEEE Trans. Aerospace Electron. Syst. 2009, 45, 280–288. [Google Scholar] [CrossRef]
- Janaswamy, R. Effect of element mutual coupling on the capacity of fixed length linear arrays. IEEE Antennas Wirel. Propag. Lett. 2002, 1, 157–160. [Google Scholar] [CrossRef]
- Abouda, A.A.; Hã Ggman, S.G. Effect of Mutual Coupling on Capacity of MIMO Wireless Channels in High SNR Scenario. Prog. Electromagn. Res. 2006, 65, 27–40. [Google Scholar] [CrossRef] [Green Version]
- Krusevac, S.M.; Kennedy, R.A.; Rapajic, P.B. Effect of Signal and Noise Mutual Coupling on MIMO Channel Capacity. Wirel. Pers. Commun. 2007, 40, 317–328. [Google Scholar] [CrossRef]
- Boris, S.B.; Robert, J.R.; Sanahuja, I.C. Exact representation of antenna system diversity performance from input parameter description. Electron. Lett. 2003, 39, 705–707. [Google Scholar] [CrossRef] [Green Version]
- Thaysen, J.; Jakobsen, K.B. Envelope correlation in (N,N) MIMO antenna array from scattering parameters. Microw. Opt. Technol. Lett. 2006, 48, 832–834. [Google Scholar] [CrossRef]
- Dama, Y.A.S.; Abd-Alhameed, R.A.; Zhou, D.; Jones, S.M.R.; Child, M.B.; Excell, P.S. Calculation of the spatial envelope correlation between two antennas in terms of the system scattering parameters including conducting losses. In Proceedings of the 2010 Loughborough Antennas & Propagation Conference, Loughborough, UK, 13 December 2010. [Google Scholar] [CrossRef]
- Sharawi, M.S.; Hassan, A.T.; Khan, M.U. Correlation coefficient calculations for MIMO antenna systems: A comparative study. Int. J. Microw. Wirel. Technol. 2017, 9, 1991–2004. [Google Scholar] [CrossRef]
- Diallo, A.; Luxey, C.; Le Thuc, P.; Staraj, R.; Kossiavas, G. Study and Reduction of the Mutual Coupling between Two Mobile Phone PIFAs Operating in the DCS1800 and UMTS Bands. IEEE Trans. Antennas Propag. 2006, 54, 3063–3074. [Google Scholar] [CrossRef]
- Du, C.; Zhao, Z.; Wang, X.; Yang, F. A Compact Cpw-Fed Triple-Band Mimo Antenna with Neutralization Line Decoupling for Wlan/Wimax/5g Applications. Prog. Electromagn. Res. M 2021, 103, 129–140. [Google Scholar] [CrossRef]
- Liu, R.; An, X.; Zheng, H.; Wang, M.; Gao, Z.; Li, E. Neutralization Line Decoupling Tri-Band Multiple-Input Multiple-Output Antenna Design. IEEE Access 2020, 8, 27018–27026. [Google Scholar] [CrossRef]
- Guo, J.; Cui, L.; Li, C.; Sun, B. Side-Edge Frame Printed Eight-Port Dual-Band Antenna Array for 5G Smartphone Applications. IEEE Trans. Antennas Propag. 2018, 66, 7412–7417. [Google Scholar] [CrossRef]
- Chen, S.; Wu, M.; Chiang, C.; Lin, M. 3.5-GHz four-element MIMO antenna system for 5G laptops with a large screen-to-body ratio. J. Electromagnet. Waves Appl. 2020, 34, 1195–1209. [Google Scholar] [CrossRef]
- Yang, M.; Zhou, J. A compact pattern diversity MIMO antenna with enhanced bandwidth and high-isolation characteristics for WLAN/5G/WiFi applications. Microw. Opt. Technol. Lett. 2020, 62, 2353–2364. [Google Scholar] [CrossRef]
- Yon, H.; Rahman, N.H.A.; Aris, M.A.; Jamaluddin, M.H.; Kong Cheh Lin, I.; Jumaat, H.; Mohd Redzwan, F.N.; Yamada, Y. Development of C-Shaped Parasitic MIMO Antennas for Mutual Coupling Reduction. Electronics 2021, 10, 2431. [Google Scholar] [CrossRef]
- Amin, F.; Saleem, R.; Shabbir, T.; Rehman, S.U.; Bilal, M.; Shafique, M.F. A Compact Quad-Element UWB-MIMO Antenna System with Parasitic Decoupling Mechanism. Appl. Sci. 2019, 9, 2371. [Google Scholar] [CrossRef] [Green Version]
- Li, M.; Cheung, S. A Novel Calculation-Based Parasitic Decoupling Technique for Increasing Isolation in Multiple-Element MIMO Antenna Arrays. IEEE Trans. Veh. Technol. 2021, 70, 446–458. [Google Scholar] [CrossRef]
- Wei, C.; Zhang, Z.; Wu, K. Phase Compensation for Decoupling of Large-Scale Staggered Dual-Polarized Dipole Array Antennas. IEEE Trans. Antennas Propag. 2020, 68, 2822–2831. [Google Scholar] [CrossRef]
- Deng, S.; Wang, Y.; Ding, J.; Chen, W.; Chen, C. Wideband Isolation Enhancement of Dual-Antenna Array Using Hybrid Decoupling Structures. In Proceedings of the 2020 International Symposium on Antennas and Propagation (ISAP), Osaka, Japan, 25–28 January 2021. [Google Scholar] [CrossRef]
- Venkateswara Rao, M.; Madhav, B.T.P.; Krishna, J.; Usha Devi, Y.; Anilkumar, T.; Prudhvi Nadh, B. CSRR-loaded T-shaped MIMO antenna for 5G cellular networks and vehicular communications. Int. J. RF Microw. Comput. Aided Eng. 2019, 29, e21799. [Google Scholar] [CrossRef]
- Chen, L.; Wang, D.; Zhang, S.; Xia, L.; Jiang, S.; Lan, S. A MIMO Antenna Array for 5G Mobile Terminals. In Proceedings of the 2019 IEEE International Symposium on Antennas and Propagation and USNC-URSI Radio Science Meeting, Atlanta, GA, USA, 7–12 July 2019. [Google Scholar] [CrossRef]
- Liu, Y.; Yang, X.; Jia, Y.; Guo, Y.J. A Low Correlation and Mutual Coupling MIMO Antenna. IEEE Access 2019, 7, 127384–127392. [Google Scholar] [CrossRef]
- Xing, H.; Wang, X.; Gao, Z.; An, X.; Zheng, H.; Wang, M.; Li, E. Efficient Isolation of an MIMO Antenna Using Defected Ground Structure. Electronics 2020, 9, 1265. [Google Scholar] [CrossRef]
- Khalid, M.; Naqvi, S.I.; Hussain, N.; Rahman, M.; Fawad; Mirjavadi, S.S.; Khan, M.J.; Amin, Y. 4-Port MIMO Antenna with Defected Ground Structure for 5G Millimeter Wave Applications. Electronics 2020, 9, 71. [Google Scholar] [CrossRef] [Green Version]
- Ren, Z.; Zhao, A.; Wu, S. MIMO Antenna with Compact Decoupled Antenna Pairs for 5G Mobile Terminals. IEEE Antennas Wirel. Propag. Lett. 2019, 18, 1367–1371. [Google Scholar] [CrossRef]
- Ren, Z.; Zhao, A. Dual-Band MIMO Antenna with Compact Self-Decoupled Antenna Pairs for 5G Mobile Applications. IEEE Access 2019, 7, 82288–82296. [Google Scholar] [CrossRef]
- Mathur, R.; Dwari, S. Compact planar reconfigurable UWB-MIMO antenna with on-demand worldwide interoperability for microwave access/wireless local area network rejection. IET Microw. Antennas Propag. 2019, 13, 1684–1689. [Google Scholar] [CrossRef]
- Yu, Z.; Wang, M.; Xie, Y. An improved loop ultra-wideband mimo antenna system for 5G mobile terminals. Prog. Electromagn. Res. Lett. 2020, 93, 99–106. [Google Scholar] [CrossRef]
- Hu, W.; Liu, X.; Gao, S.; Wen, L.; Qian, L.; Feng, T.; Xu, R.; Fei, P.; Liu, Y. Dual-Band Ten-Element MIMO Array Based on Dual-Mode IFAs for 5G Terminal Applications. IEEE Access 2019, 7, 178476–178485. [Google Scholar] [CrossRef]
- Smith, D.R.; Vier, D.C.; Koschny, T.; Soukoulis, C.M. Electromagnetic parameter retrieval from inhomogeneous metamaterials. Phys. Rev. E Stat. Nonlinear Soft Matter Phys. 2005, 71, 36617. [Google Scholar] [CrossRef] [Green Version]
- Dabas, T.; Gangwar, D.; Kanaujia, B.K.; Gautam, A.K. Mutual coupling reduction between elements of UWB MIMO antenna using small size uniplanar EBG exhibiting multiple stop bands. Int. J. Electron. Commun. 2018, 93, 32–38. [Google Scholar] [CrossRef]
- Ambika, A.; Tharini, C. Semicircle CSRR with Circular Slot Array Structures for High Level Mutual Coupling Reduction in MIMO Antenna. Prog. Electromagn. Res. M 2019, 87, 23–32. [Google Scholar] [CrossRef] [Green Version]
- Ishteyaq, I.; Masoodi, I.S.; Muzaffar, K. Eight-port double band printed Mimo antenna investigated for mutual-coupling and sar effects for sub-6 Ghz 5G mobile applications. Prog. Electromagn. Res. C 2021, 113, 111–122. [Google Scholar] [CrossRef]
- Li, M.; Zhong, B.G.; Cheung, S.W. Isolation Enhancement for MIMO Patch Antennas Using Near-Field Resonators as Coupling-Mode Transducers. IEEE Trans. Antennas Propag. 2019, 67, 755–764. [Google Scholar] [CrossRef]
- Rajeshkumar, V.; Rajkumar, R. SRR loaded compact tri-band MIMO Antenna for wlan/wimax applications. Prog. Electromagn. Res. Lett. 2021, 95, 43–53. [Google Scholar] [CrossRef]
- Chang, L.; Yu, Y.; Wei, K.; Wang, H. Polarization-Orthogonal Co-frequency Dual Antenna Pair Suitable for 5G MIMO Smartphone With Metallic Bezels. IEEE Trans. Antennas Propag. 2019, 67, 5212–5220. [Google Scholar] [CrossRef]
- Chang, L.; Yu, Y.; Wei, K.; Wang, H. Orthogonally Polarized Dual Antenna Pair with High Isolation and Balanced High Performance for 5G MIMO Smartphone. IEEE Trans. Antennas Propag. 2020, 68, 3487–3495. [Google Scholar] [CrossRef]
- Sun, L.; Li, Y.; Zhang, Z.; Feng, Z. Wideband 5G MIMO Antenna with Integrated Orthogonal-Mode Dual-Antenna Pairs for Metal-Rimmed Smartphones. IEEE Trans. Antennas Propag. 2020, 68, 2494–2503. [Google Scholar] [CrossRef]
- Li, M.; Xu, Z.; Ban, Y.; Sim, C.; Yu, Z. Eight-port orthogonally dual-polarised MIMO antennas using loop structures for 5G smartphone. IET Microw. Antennas Propag. 2017, 11, 1810–1816. [Google Scholar] [CrossRef]
- Li, M.; Ban, Y.; Xu, Z.; Guo, J.; Yu, Z. Tri-Polarized 12-Antenna MIMO Array for Future 5G Smartphone Applications. IEEE Access 2018, 6, 6160–6170. [Google Scholar] [CrossRef]
- Feng, B.; Chen, J.; Yin, S.; Sim, C.; Zhao, Z. A Tri-Polarized Antenna with Diverse Radiation Characteristics for 5G and V2X Communications. IEEE Trans. Veh. Technol. 2020, 69, 10115–10126. [Google Scholar] [CrossRef]
- Garbacz, R.J. Modal expansions for resonance scattering phenomena. Proc. IEEE 1965, 53, 856–864. [Google Scholar] [CrossRef]
- Zhao, X.; Yeo, S.P.; Ong, L.C. Planar UWB MIMO Antenna with Pattern Diversity and Isolation Improvement for Mobile Platform Based on the Theory of Characteristic Modes. IEEE Trans. Antennas Propag. 2018, 66, 420–425. [Google Scholar] [CrossRef]
- Kumar, N.; Khanna, R. A compact multi-band multi-input multi-output antenna for 4G/5G and IoT devices using theory of characteristic modes. Int. J. RF Microw. Comput. Aided Eng. 2019, 30, e22012. [Google Scholar] [CrossRef]
- Ganie, J.A.; Singh, C.; Jha, K.R.; Sharma, S.K. A LTE Band Integrated 5G Antenna Design using Characteristic Mode Analysis. In Proceedings of the 2019 IEEE International Symposium on Antennas and Propagation and USNC-URSI Radio Science Meeting, Atlanta, GA, USA, 7–12 July 2019. [Google Scholar] [CrossRef]
- Dong, J.; Wang, S.; Mo, J. Design of a Twelve-Port MIMO Antenna System for Multi-Mode 4G/5G Smartphone Applications Based on Characteristic Mode Analysis. IEEE Access 2020, 8, 90751–90759. [Google Scholar] [CrossRef]
- Hei, Y.; He, J.; Li, W. Wideband Decoupled 8-Element MIMO Antenna for 5G Mobile Terminal Applications. IEEE Antennas Wirel. Propag. Lett. 2021, 20, 1448–1452. [Google Scholar] [CrossRef]
- Ali, T.; Aw, M.S.; Biradar, R.C. A A Compact Bandwidth Enhanced Antenna Loaded with SRR For WLAN/WiMAX/Satellite Applications. Adv. Electromagn. 2018, 7, 78–84. [Google Scholar] [CrossRef]
- Sousa De Sena, A.; Benevides Da Costa, D.; Ding, Z.; Nardelli, P.H.J. Massive MIMO-NOMA Networks with Multi-Polarized Antennas. IEEE Trans. Wirel. Commun. 2019, 18, 5630–5642. [Google Scholar] [CrossRef]
- Dicandia, F.A.; Genovesi, S.; Monorchio, A. Analysis of the Performance Enhancement of MIMO Systems Employing Circular Polarization. IEEE Trans. Antennas Propag. 2017, 65, 4824–4835. [Google Scholar] [CrossRef]
- Chakraborty, S.; Rahman, M.A.; Hossain, M.A.; Mobashsher, A.T.; Nishiyama, E.; Toyoda, I. A 4-element MIMO antenna with orthogonal circular polarization for sub-6 GHz 5G cellular applications. SN Appl. Sci. 2020, 2, 1180. [Google Scholar] [CrossRef]
- Ozdemir, M.K.; Arvas, E.; Arslan, H. Dynamics of spatial correlation and implications on MIMO systems. IEEE Commun. Mag. 2004, 42, S14–S19. [Google Scholar] [CrossRef]
- Recioui, A.; Bentarzi, H. Genetic Algorithm Based MIMO Capacity Enhancement in Spatially Correlated Channels Including Mutual Coupling. Wirel. Pers. Commun. 2012, 63, 689–701. [Google Scholar] [CrossRef]
- Chouhan, S.; Panda, D.K.; Kushwah, V.S. Modified circular common element four-port multiple-input-multiple-output antenna using diagonal parasitic element. Int. J. RF Microw. Comput. Aided Eng. 2019, 29, e21527. [Google Scholar] [CrossRef]
- Mahmoud, K.R.; Montaser, A.M. Performance of Tri-Band Multi-Polarized Array Antenna for 5G Mobile Base Station Adopting Polarization and Directivity Control. IEEE Access 2018, 6, 8682–8694. [Google Scholar] [CrossRef]
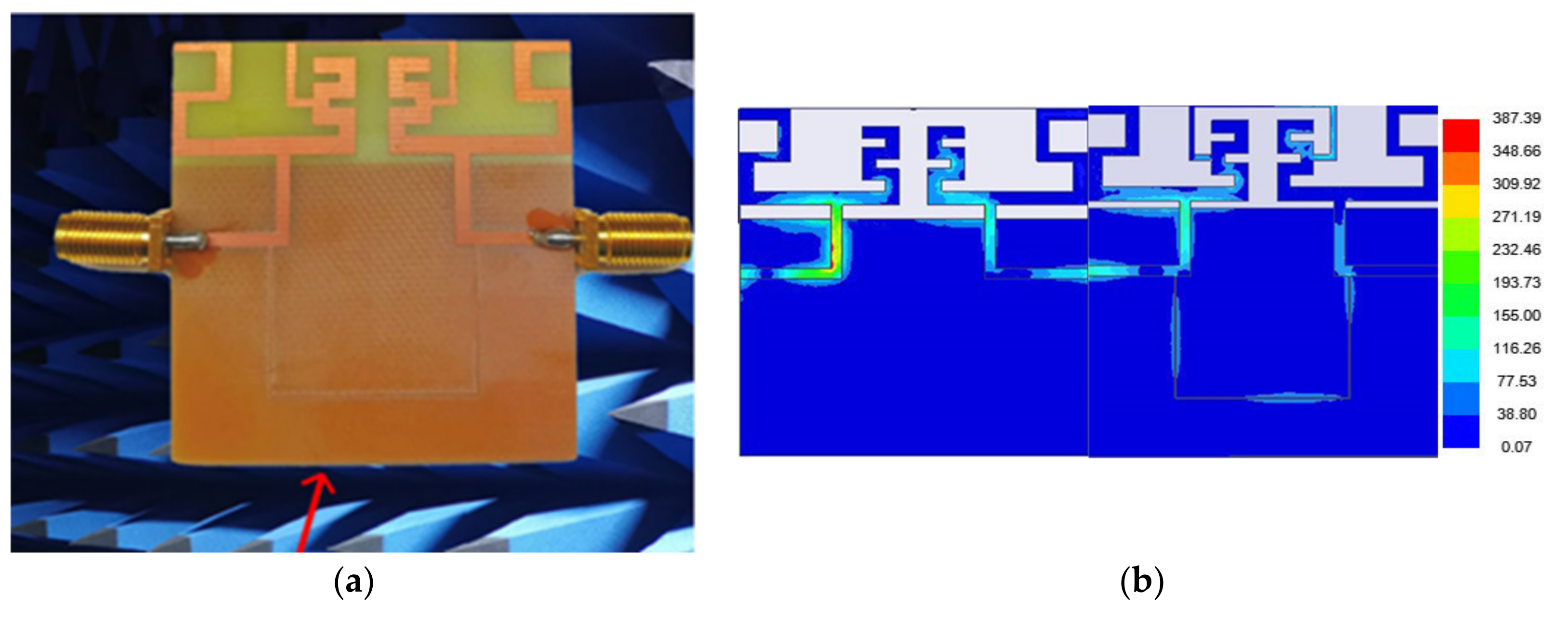
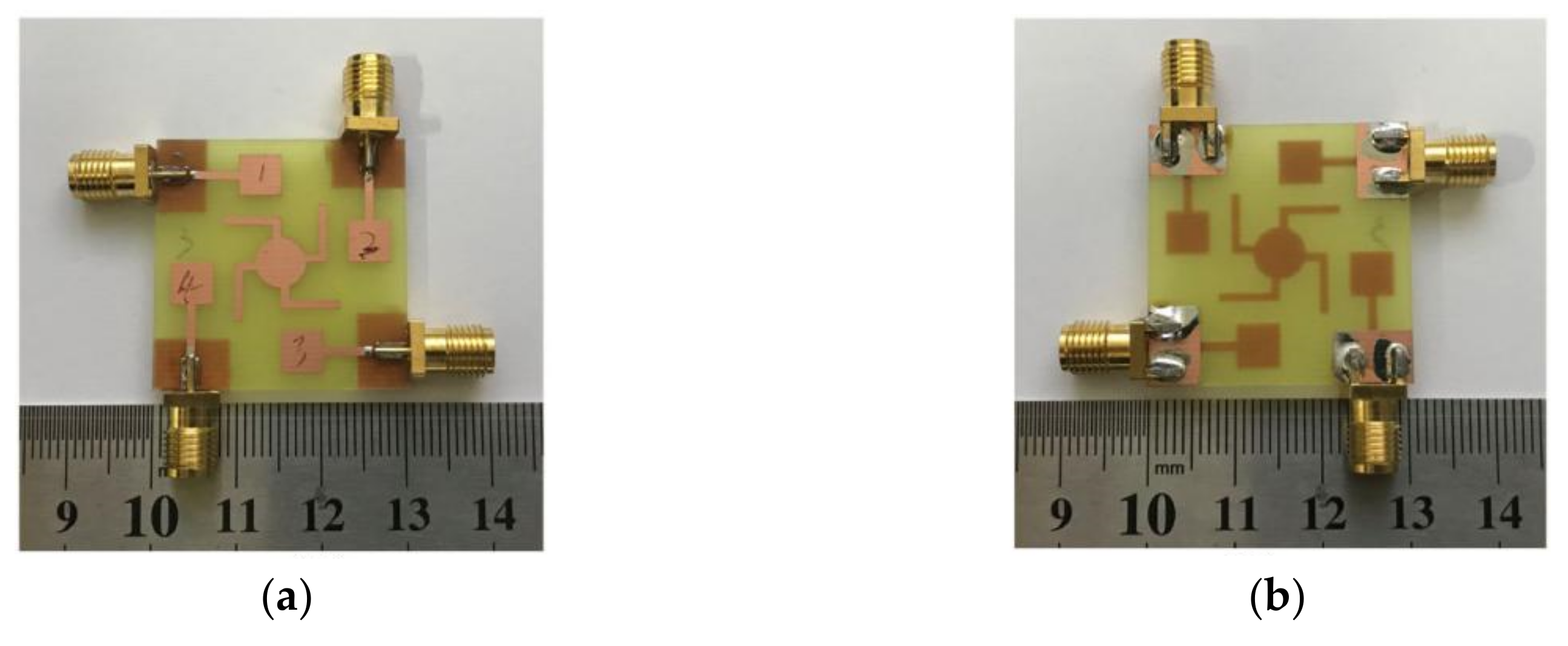
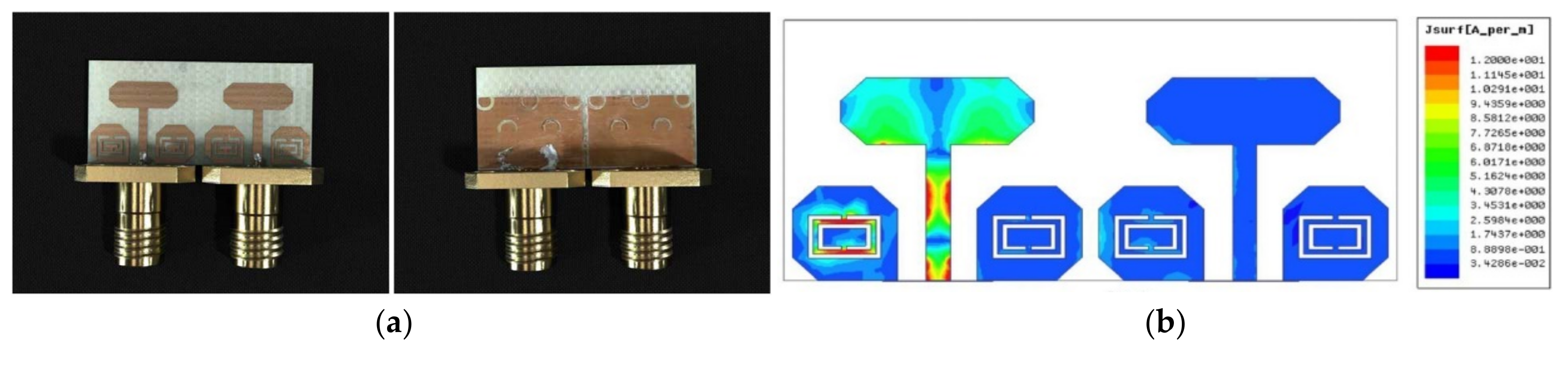

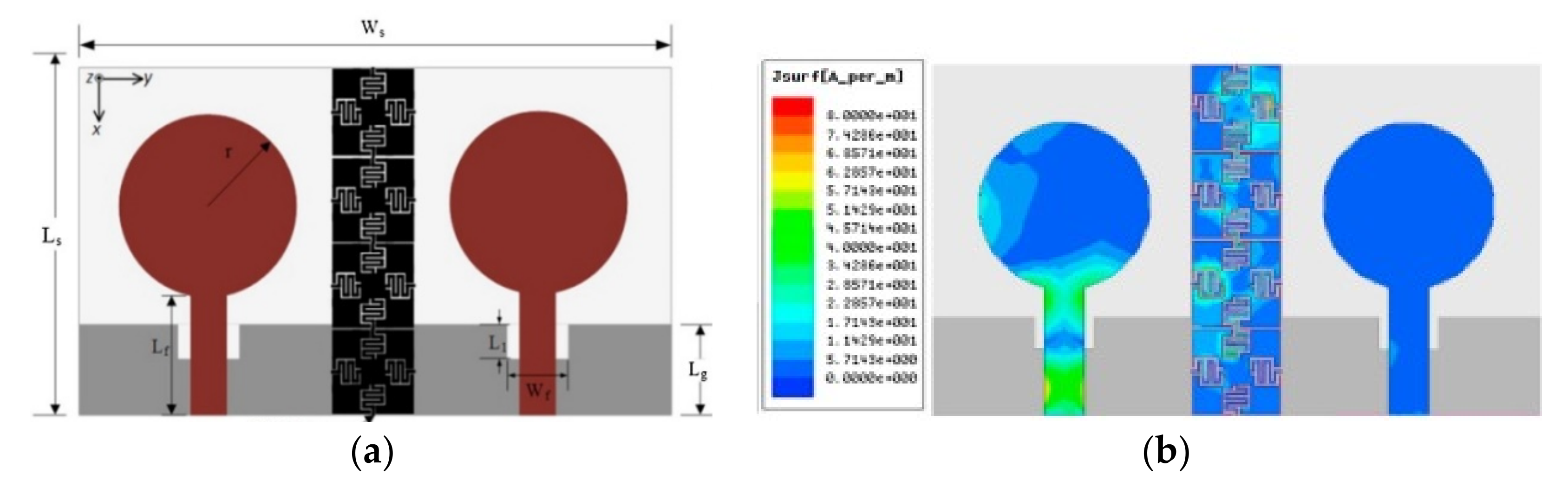

| Type of Antenna Element | System Aize (mm²) | Element Distance (mm) | Isolation (dB) | ECC * | MIMO Order |
|---|---|---|---|---|---|
| I-shaped slot antenna [8] | 140 × 70 | 15.8/21.3 | >11 | <0.1 | 8 |
| Inverted F-shaped antenna [9] | 136 × 68.8 | 14/29 | >10 | - | 8 |
| Inverted π-shaped antenna [8] | 150 × 80 | 10/13.5/17.5/18 | >12 | <0.15/<0.1 | 12 |
| Decoupling Techniques | Decoupling Shape | Frequency (GHZ) | Isolation (dB) | ECC | MIMO Order |
|---|---|---|---|---|---|
| Neutralization lines | meandering NL [32] | 2.3–2.5/3.2–3.6/5.0–6.7 | >16 | <0.005 | 2 |
| Two U-shaped NL and symmetrical distribution [33] | 2.2–2.4/3.3–4.5/5.6–5.7 | >18/>32/>24 | <0.02 | 2 | |
| Strip NL [34] | 3.4–3.6/4.8–5.1 | >11 | <0.08/<0.05 | 8 | |
| meandering NL [35] | 3.4–3.6 | >12 | <0.15 | 4 | |
| Parasitic decoupling elements | PDE [36] | 4.58–6.12 | >15.4 | <0.15 | 4 |
| PDE [37] | 15.5–17 | >30 | <0.14 | 2 | |
| PDE [38] | 3.1–10.6 | >20 | - | 4 | |
| ADS and DGS [41] | 5.1–6.2 | >20 | - | 2 | |
| Defective ground structures | Circular DGS and CSRR [42] | 26.5–38.2 | >15 | <0.005 | 2 |
| Rectangular DGS [43] | 3.3–3.6/4.8–5 | >10.7 | - | 10 | |
| DGS and hybrid EBG [44] | 4.5–8 | >20 | <0.002 | 2 | |
| Two zigzag–shaped DGS [45] | 5.8 | 28.8 | - | 2 | |
| Rectangular, circular and zigzag shaped DGS and polarization diversity [46] | 25.5–29.6 | >17 | <0.01 | 4 | |
| Grounding branch structures | Rectangular grounding branch [47] | 3.4–3.6 | >17 | - | 4 |
| Rectangular grounding branch [47] | 3.4–3.6/4.8–5.0 | >17.5/>20 | - | 4 | |
| Multi-branch T-shaped grounding branch [49] | 3–11 | >15 | <0.3 | 2 | |
| T-shaped grounding branch [50] | 3.3–5.6 | >15 | <0.03 | 8 | |
| meandering grounding branch and NLs [51] | 3.4–3.6/4.8–5.0 | >12 | <0.15 | 10 | |
| Metamaterials | Four-EBG array [53] | 3.6–17.6 | >18 | <0.015 | 2 |
| Ten-CSRR array [54] | 2.51 | 58.36 | 0.005 | 2 | |
| SRR [55] | 2.73–3.12/4.33–4.68 | >20 | <0.04 | 8 | |
| NFR consists of five split rings [56] | 2.38–2.49 | >20 | - | 8 | |
| SRR and slot [57] | 2.4/3.5/5.3 | >14 | - | 4 |
| Decoupling Techniques | Decoupling Scheme | Frequency (GHz) | Isolation (dB) | ECC | MIMO Order |
|---|---|---|---|---|---|
| Orthogonal polarization technique | Dual orthogonal polarization [58] | 3.4–3.6 | >12.7 | <0.13 | 8 |
| Dual orthogonal polarization [59] | 2.2–2.4 | >20 | 0.04 | 8 | |
| Dual orthogonal polarization [60] | 3.5–5.0 | >12 | <0.11 | 8 × 8 | |
| Dual orthogonal polarization [61] | 2.55–2.65 | >11.4 | <0.1 | 8 | |
| Triple orthogonal polarization and NLs [62] | 3.4–3.6 | >12.5 | <0.2 | 12 | |
| Triple orthogonal polarization [63] | 2.8–2.9/4.5–5.4 | >48 | - | 3 | |
| Characteristic modes analysis | CMA optimization and dual orthogonal polarization [65] | 2–9.5 | >20 | <0.03 | 2 |
| CMA optimization [68] | 3.4–3.6/4.8–5.0 | >12.5 | <0.5 | 12 | |
| CMA optimization and DGS [69] | 3.3–5.95 | >15 | <0.11 | 8 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Du, K.; Wang, Y.; Hu, Y. Design and Analysis on Decoupling Techniques for MIMO Wireless Systems in 5G Applications. Appl. Sci. 2022, 12, 3816. https://doi.org/10.3390/app12083816
Du K, Wang Y, Hu Y. Design and Analysis on Decoupling Techniques for MIMO Wireless Systems in 5G Applications. Applied Sciences. 2022; 12(8):3816. https://doi.org/10.3390/app12083816
Chicago/Turabian StyleDu, Kaiwen, Yongshun Wang, and Yao Hu. 2022. "Design and Analysis on Decoupling Techniques for MIMO Wireless Systems in 5G Applications" Applied Sciences 12, no. 8: 3816. https://doi.org/10.3390/app12083816
APA StyleDu, K., Wang, Y., & Hu, Y. (2022). Design and Analysis on Decoupling Techniques for MIMO Wireless Systems in 5G Applications. Applied Sciences, 12(8), 3816. https://doi.org/10.3390/app12083816





