Effect of Wing Membrane Material on the Aerodynamic Performance of Flexible Flapping Wing
Abstract
:1. Introduction
2. Materials and Methods
2.1. Flapping Mechanism
2.2. Wing Design
3. Data Acquisition and Deformation Measurement
3.1. Lift and Power Measurement
3.2. Deformation Measurement
4. Results and Discussion
4.1. Lift and Power
4.2. Deformation Results
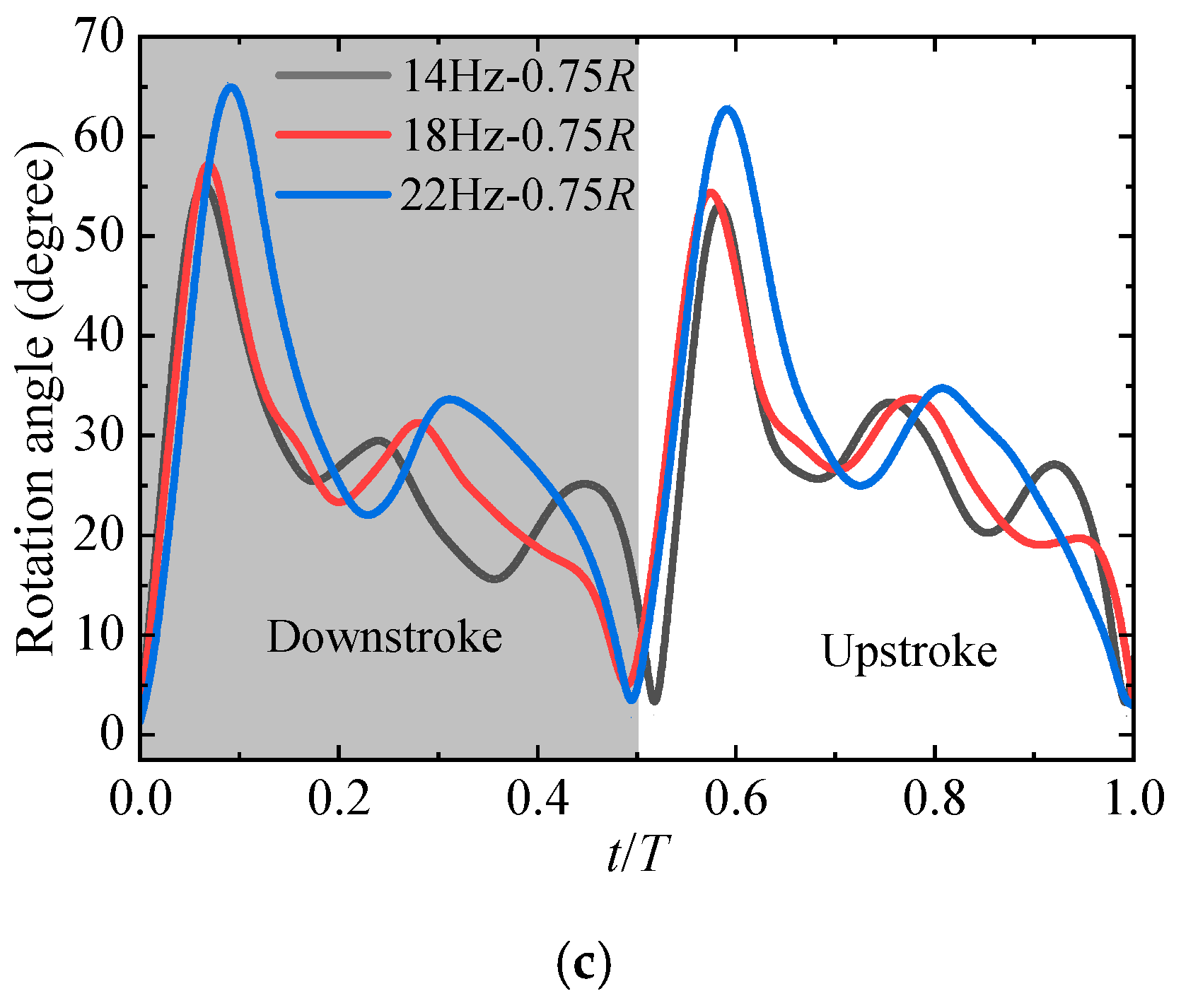
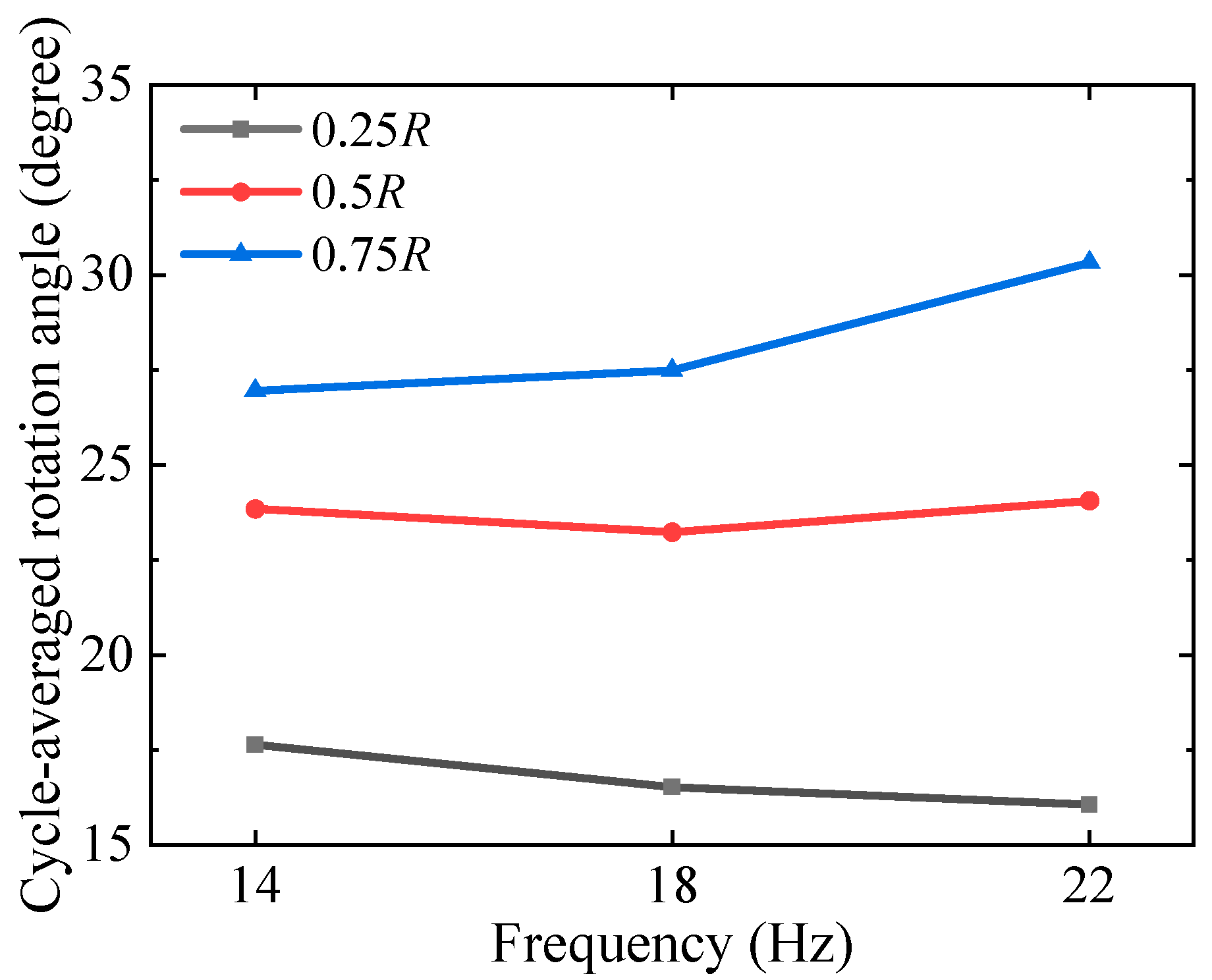
4.2.1. Effect of the Flapping Frequency
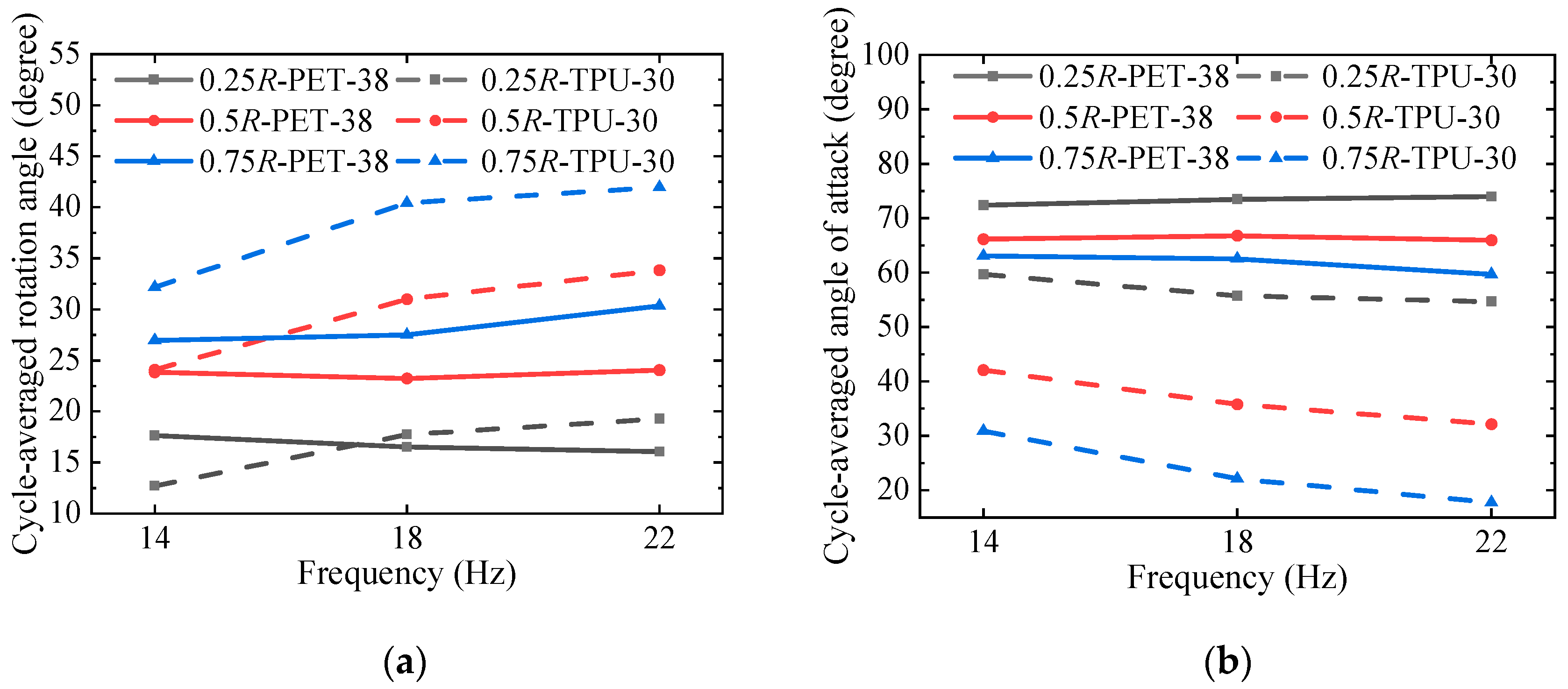
4.2.2. Effect of Wing’s Flexibility
5. Conclusions
- (1)
- Flexibility presents a significant influence on lift generation and power consumption. Wings with a higher elastic modulus membrane, which means a more rigid wing, could generate higher lift but at the cost of more power.
- (2)
- Wings with a lower elastic modulus membrane present greater twisting deformation along the spanwise direction, especially at a high flapping frequency. Meanwhile, the twisting deformation is more obvious with the increase of flapping frequency.
- (3)
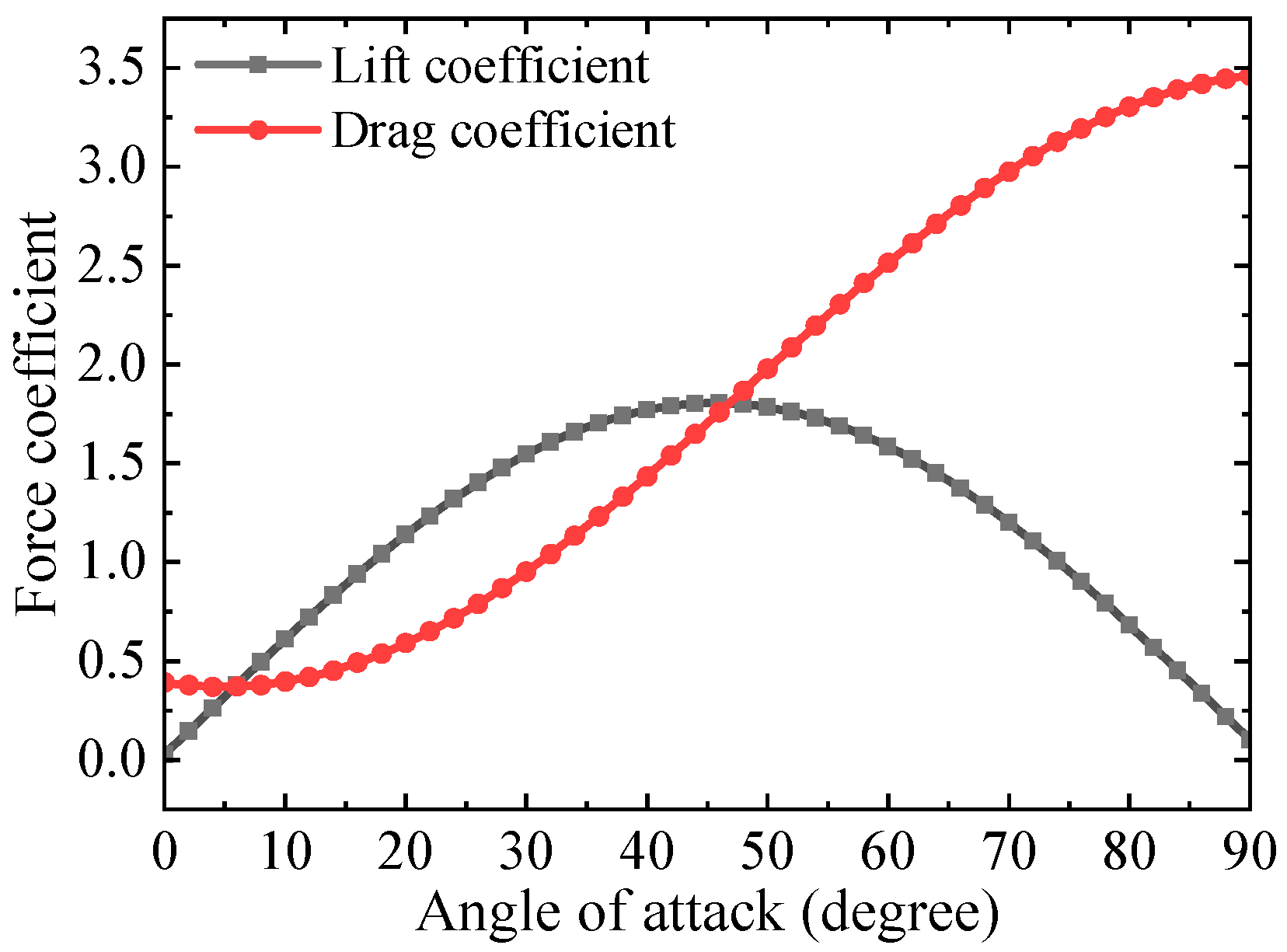
- A more flexible wing shows an advantage in translational force for a smaller angle of attack, close to the optimal value. However, the potentially superior rotational force related to higher rotation angular velocity and angular acceleration may be responsible for the higher lift of a more rigid wing, yet a larger angle of attack also forms a larger drag force and greater power consumption.
- (4)
- Rotation angle fluctuation occurs after the stroke reversal. Flexibility shows advantages in attenuating the rotation angle fluctuation, which in turn enhances the aerodynamic efficiency.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Dickinson, M.H.; Lehmann, F.O.; Sane, S.P. Wing rotation and the aerodynamic basis of insect flight. Science 1999, 284, 1954–1960. [Google Scholar] [CrossRef] [PubMed]
- Jones, S.K.; Laurenza, R.; Hedrick, T.L.; Griffith, B.E.; Miller, L.A. Lift vs. drag based mechanisms for vertical force production in the smallest flying insects. J. Theor. Biol. 2015, 384, 105–120. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Koehler, C.; Liang, Z.; Gaston, Z.; Wan, H.; Dong, H. 3D reconstruction and analysis of wing deformation in free-flying dragonflies. J. Exp. Biol. 2012, 215, 3018–3027. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Young, J.; Walker, S.M.; Bomphrey, R.J.; Taylor, G.K.; Thomas, A.L. Details of insect wing design and deformation enhance aerodynamic function and flight efficiency. Science 2009, 325, 1549–1552. [Google Scholar] [CrossRef] [PubMed]
- Reid, H.E.; Schwab, R.K.; Maxcer, M.; Peterson, R.K.; Johnson, E.L.; Jankauski, M. Wing flexibility reduces the energetic requirements of insect flight. Bioinspir. Biomim. 2019, 14, 056007. [Google Scholar] [CrossRef]
- Wootton, R. The geometry and mechanics of insect wing deformations in flight: A modelling approach. Insects 2020, 11, 446. [Google Scholar] [CrossRef]
- Combes, S.A.; Daniel, T.L. Flexural stiffness in insect wings II. Spatial distribution and dynamic wing bending. J. Exp. Biol. 2003, 206, 2989–2997. [Google Scholar] [CrossRef] [Green Version]
- Shumway, N.; Gabryszuk, M.; Laurence, S. The impact of dragonfly wing deformations on aerodynamic performance during forward flight. Bioinspir. Biomim. 2020, 15, 026005. [Google Scholar] [CrossRef]
- Addo-Akoto, R.; Han, J.S.; Han, J.H. Roles of wing flexibility and kinematics in flapping wing aerodynamics. J. Fluids Struct. 2021, 104, 103317. [Google Scholar] [CrossRef]
- Lee, N.; Lee, S.; Cho, H.; Shin, S. Effect of flexibility on flapping wing characteristics in hover and forward flight. Comput. Fluids 2018, 173, 111–117. [Google Scholar] [CrossRef]
- Fu, J.; Liu, X.; Shyy, W.; Qiu, H. Effects of flexibility and aspect ratio on the aerodynamic performance of flapping wings. Bioinspir. Biomim. 2018, 13, 036001. [Google Scholar] [CrossRef] [PubMed]
- Thiria, B.; Godoy-Diana, R. How wing compliance drives the efficiency of self-propelled flapping flyers. Phys. Rev. E 2010, 82, 015303. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zhang, Y.; Wang, X.; Wang, S.; Huang, W.; Weng, Q. Kinematic and Aerodynamic Investigation of the Butterfly in Forward Free Flight for the Butterfly-Inspired Flapping Wing Air Vehicle. Appl. Sci. 2021, 11, 2620. [Google Scholar] [CrossRef]
- Keennon, M.; Klingebiel, K.; Won, H. Development of the nano hummingbird: A tailless flapping wing micro air vehicle. In Proceedings of the 50th AIAA Aerospace Sciences Meeting Including the New Horizons Forum and Aerospace Exposition, Nashville, TN, USA, 9–12 January 2012. [Google Scholar]
- Roshanbin, A.; Abad, F.; Preumont, A. Kinematic and aerodynamic enhancement of a robotic hummingbird. AIAA J. 2019, 57, 4599–4607. [Google Scholar] [CrossRef]
- Truong, T.V.; Nguyen, Q.V.; Lee, H.P. Bio-inspired flexible flapping wings with elastic deformation. Aerospace 2017, 4, 37. [Google Scholar] [CrossRef]
- Nan, Y.; Karásek, M.; Lalami, M.E.; Preumont, A. Experimental optimization of wing shape for a hummingbird-like flapping wing micro air vehicle. Bioinspir. Biomim. 2017, 12, 026010. [Google Scholar] [CrossRef]
- Phan, H.V.; Park, H.C. Design and evaluation of a deformable wing configuration for economical hovering flight of an insect-like tailless flying robot. Bioinspir. Biomim. 2018, 13, 036009. [Google Scholar] [CrossRef]
- Au, L.T.K.; Phan, H.V.; Park, S.H.; Park, H.C. Effect of corrugation on the aerodynamic performance of three-dimensional flapping wings. Aerosp. Sci. Technol. 2020, 105, 106041. [Google Scholar] [CrossRef]
- Nguyen, K.; Au, L.T.K.; Phan, H.V.; Park, S.H.; Park, H.C. Effects of wing kinematics, corrugation, and clap-and-fling on aerodynamic efficiency of a hovering insect-inspired flapping-wing micro air vehicle. Aerosp. Sci. Technol. 2021, 118, 106990. [Google Scholar] [CrossRef]
- Lee, J.; Yoon, S.H.; Kim, C. Experimental surrogate-based design optimization of wing geometry and structure for flapping wing micro air vehicles. Aerosp. Sci. Technol. 2022, 123, 107451. [Google Scholar] [CrossRef]
- Lee, Y.J.; Lua, K.B.; Lim, T.T.; Yeo, K.S. A quasi-steady aerodynamic model for flapping flight with improved adaptability. Bioinspir. Biomim. 2016, 11, 036005. [Google Scholar] [CrossRef] [PubMed]
- Ellington, C.P. The aerodynamics of hovering insect flight. II. Morphological parameters. Philos. Trans. R. Soc. Lond. B Biol. Sci. 1984, 305, 17–40. [Google Scholar]
- Yoon, S.H.; Cho, H.; Lee, J.; Kim, C.; Shin, S.J. Effects of camber angle on aerodynamic performance of flapping-wing micro air vehicle. J. Fluids Struct. 2020, 97, 103101. [Google Scholar] [CrossRef]
- Tay, W.B.; Jadhav, S.; Wang, J.L. Application and improvements of the wing deformation capture with simulation for flapping micro aerial vehicle. J. Bionic Eng. 2020, 17, 1096–1108. [Google Scholar] [CrossRef]
- Hedrick, T.L. Software techniques for two-and three-dimensional kinematic measurements of biological and biomimetic systems. Bioinspir. Biomim. 2008, 3, 034001. [Google Scholar] [CrossRef]
- Nguyen, T.A.; Phan, H.V.; Au, T.K.L.; Park, H.C. Experimental study on thrust and power of flapping-wing system based on rack-pinion mechanism. Bioinspir. Biomim. 2016, 11, 046001. [Google Scholar] [CrossRef]
- Gertsbakh, I. Measurement Theory for Engineers; Springer: Berlin/Heidelberg, Germany, 2003; pp. 87–94. [Google Scholar]
- Heathcote, S.; Wang, Z.; Gursul, I. Effect of spanwise flexibility on flapping wing propulsion. J. Fluids Struct. 2008, 24, 183–199. [Google Scholar] [CrossRef]
- Addo-Akoto, R.; Han, J.S.; Han, J.H. Aerodynamic performance of flexible flapping wings deformed by slack angle. Bioinspir. Biomim. 2020, 15, 066005. [Google Scholar] [CrossRef]
- Phan, H.V.; Truong, Q.T.; Au, T.K.L.; Park, H.C. Effect of wing kinematics modulation on aerodynamic force generation in hovering insect-mimicking flapping-wing micro air vehicle. J. Bionic Eng. 2015, 12, 539–554. [Google Scholar] [CrossRef]
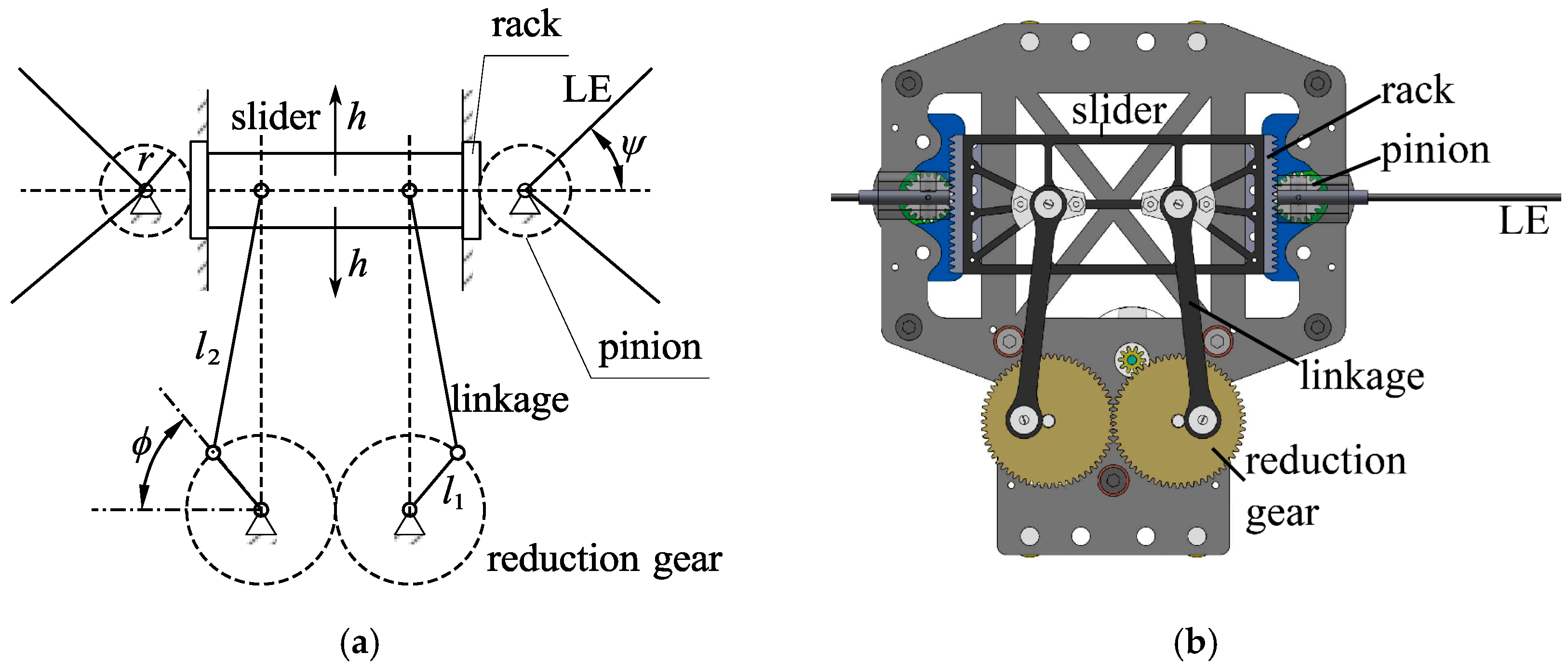
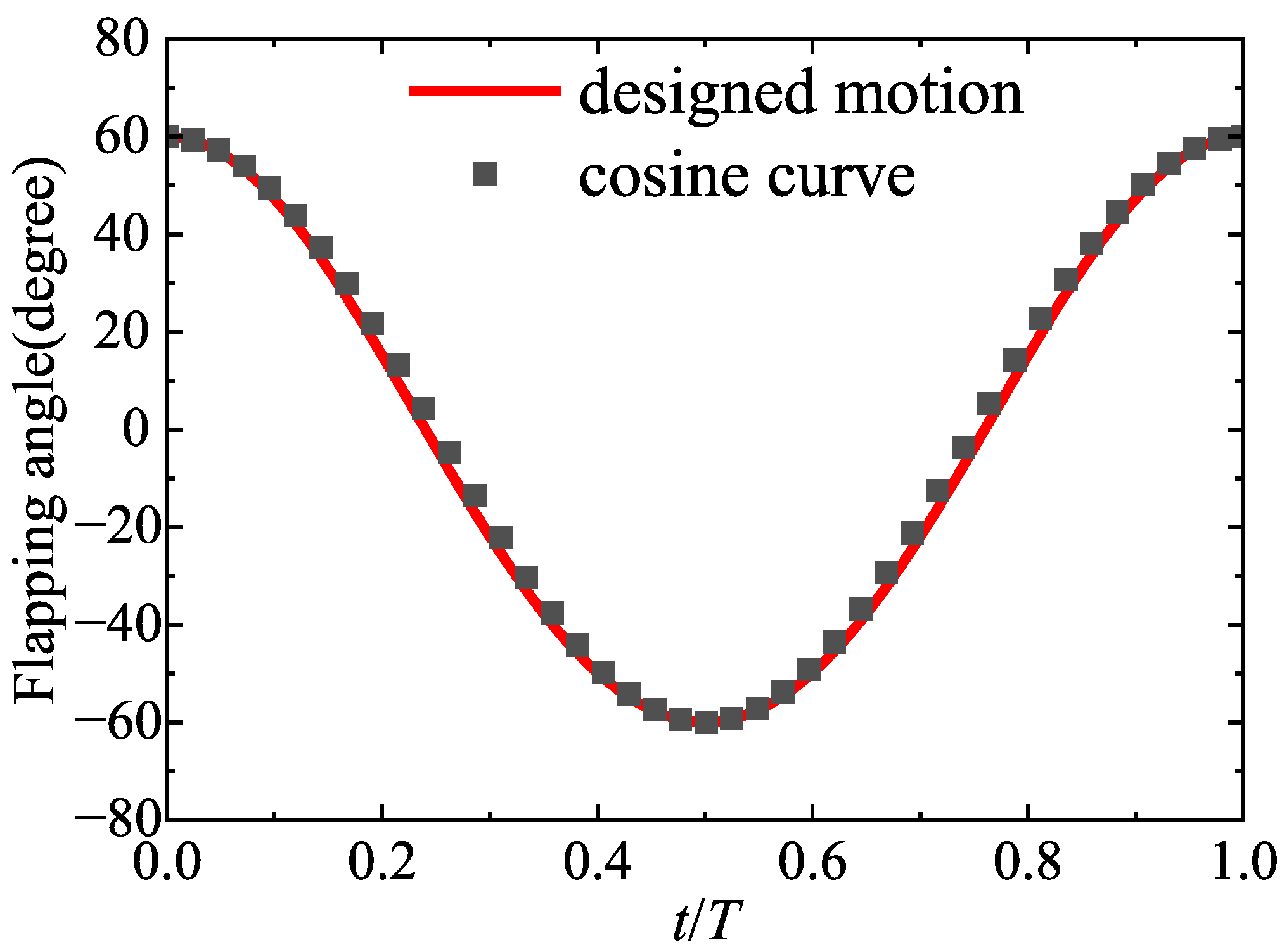

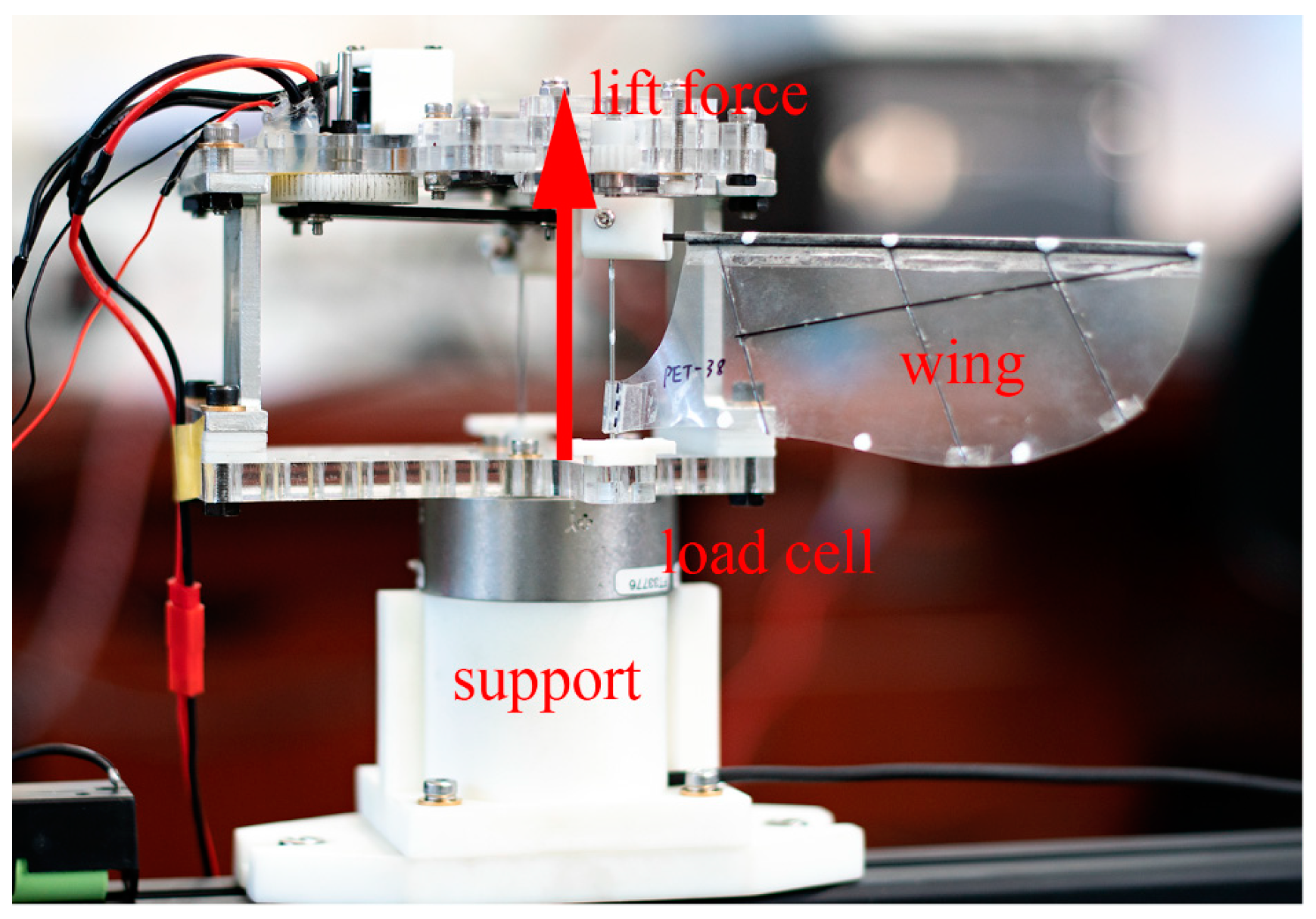


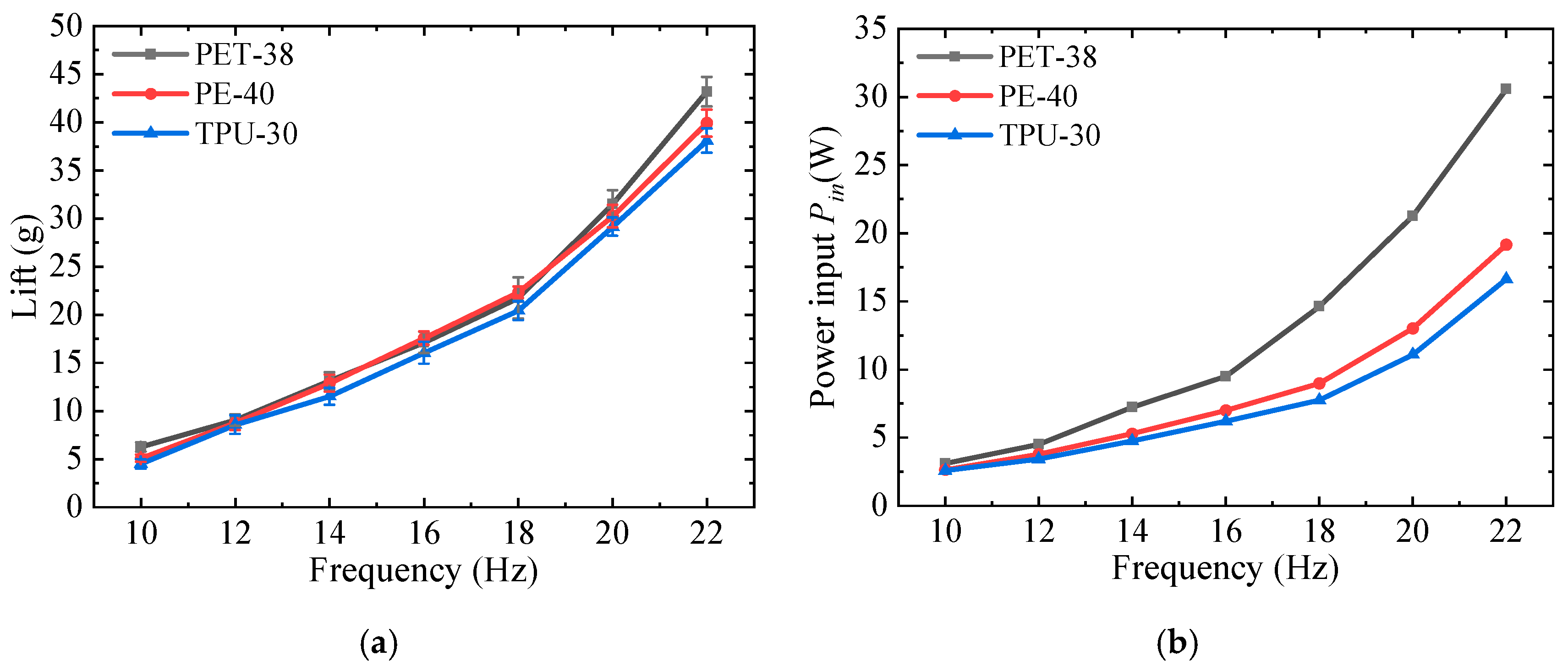





| Wing Material | Thickness (μm) | Elastic Modulus (GPa) | Density (g/cm3) |
|---|---|---|---|
| Polyethylene terephthalate (PET) | 38 | 3.30 | 1.38 |
| Polyethylene (PE) | 40 | 1.72 | 0.92 |
| Thermoplastic urethanes (TPU) | 30 | 1.08 | 1.10 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lang, X.; Song, B.; Yang, W.; Yang, X. Effect of Wing Membrane Material on the Aerodynamic Performance of Flexible Flapping Wing. Appl. Sci. 2022, 12, 4501. https://doi.org/10.3390/app12094501
Lang X, Song B, Yang W, Yang X. Effect of Wing Membrane Material on the Aerodynamic Performance of Flexible Flapping Wing. Applied Sciences. 2022; 12(9):4501. https://doi.org/10.3390/app12094501
Chicago/Turabian StyleLang, Xinyu, Bifeng Song, Wenqing Yang, and Xiaojun Yang. 2022. "Effect of Wing Membrane Material on the Aerodynamic Performance of Flexible Flapping Wing" Applied Sciences 12, no. 9: 4501. https://doi.org/10.3390/app12094501
APA StyleLang, X., Song, B., Yang, W., & Yang, X. (2022). Effect of Wing Membrane Material on the Aerodynamic Performance of Flexible Flapping Wing. Applied Sciences, 12(9), 4501. https://doi.org/10.3390/app12094501








