A Designed Calibration Approach for the Measurement-While-Drilling Instrument
Abstract
1. Introduction
- (1)
- How to align the coordinate frames of all the sensor triplets with the coordinate frame of a carrier platform in the MWD instrument?
- (2)
- Can the new calibration approach be effectively used to eliminate the measuring errors without the use of prior knowledge about the vector field?
2. Materials and Methods
2.1. Sensor Model and Error Model
2.2. Basic Calibration Algorithm
2.2.1. Ellipsoidal Fitting Method (EF)
2.2.2. Plane Fitting Method (PF)
2.2.3. Inner Product Invariance Method (IPI)
2.3. Improvement of the Calibration Methods
2.3.1. Recursive Least Square Method (RLS)
- (1)
- Initialize the coefficient vector β0 and the covariance matrix P0.
- (2)
- Gather the raw data of the nth measuring point, namely = (, , )T.
- (3)
- Calculate the gn and Pn according to Equation (20).
- (4)
- Calculate the new coefficient vector βn according to Equation (21).
- (5)
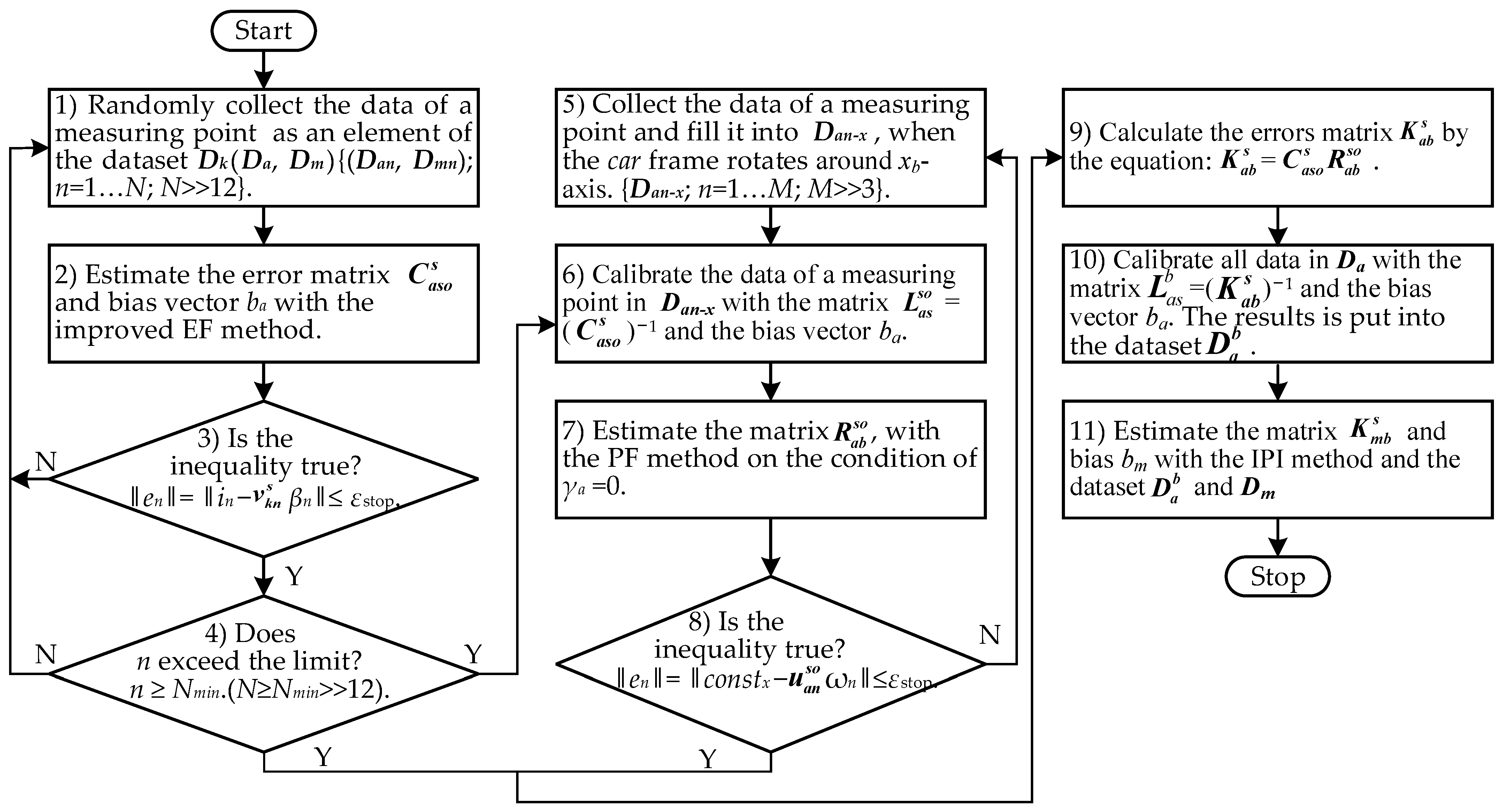
- Judge the posteriori error ‖en‖, until it meets the inequality ‖en‖ = ‖in − βn‖≤ εstop, then stop the iteration; otherwise, return to step (2). Here, the restriction value of εstop is an empirical value. The method flow chart is shown in Figure 2.
2.3.2. Optimization of Plane Fitting Method
- (1)
- The solving equation of the pitch angle θ is derived with the vector = (,,)T of the gravity vector G in the mirror coordinate frame.
- (2)
- The calculation equation of the azimuthal angle ψ can be rewritten as Equation (23).
2.4. Design of the Calibration Approach
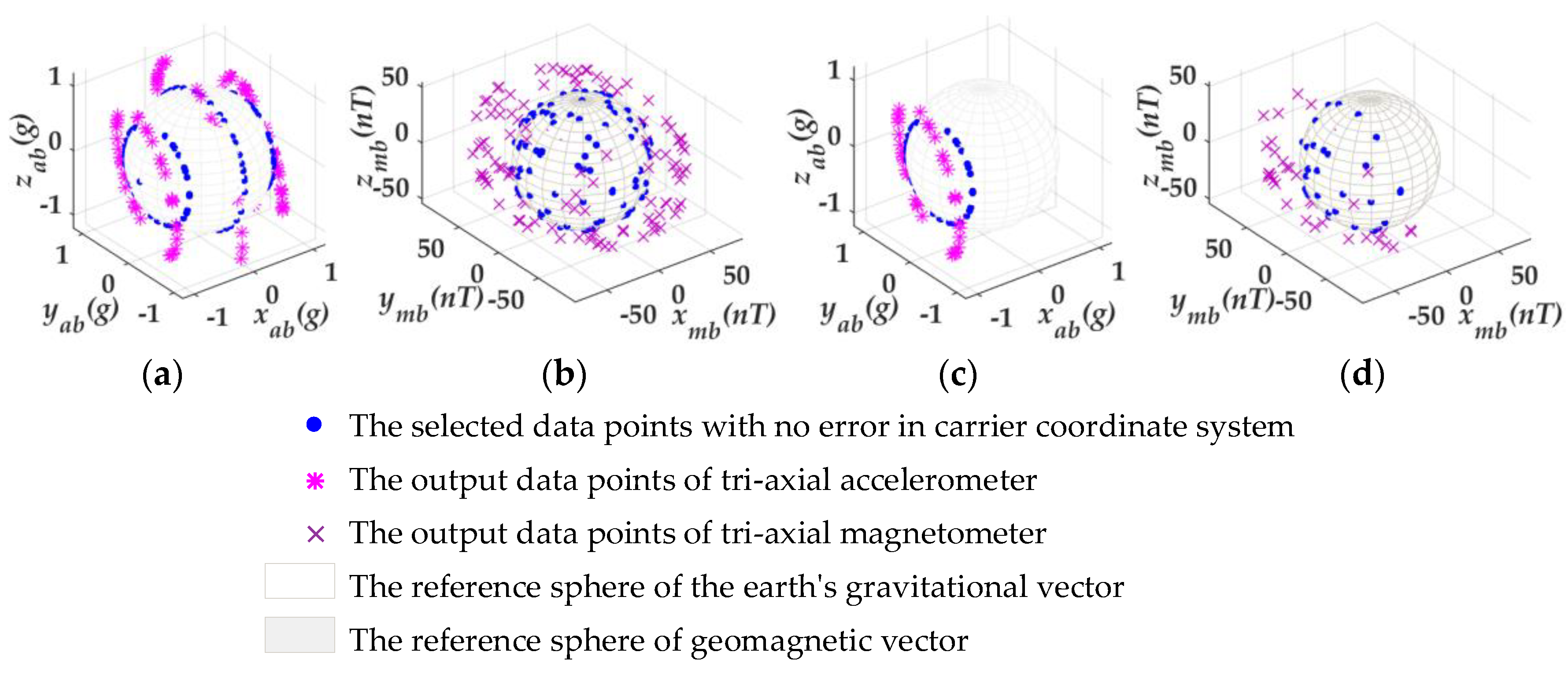
2.5. The Collecting Method for the Sample Data
- Artificially fix a pitch angle sequence [−90°, 90°, −45°, 45°, 0°] in the range of [−90°, 90°].
- Pick out a value in turn from the angle sequence in step 1 as a selected pitch angle θi.
- Calculate an azimuthal angel sequence based on the value of the selected pitch angle θi, according to Equation (24), where nψi is the total number of angles in the azimuth sequence.
- Pick out a value in turn from the azimuthal angel sequence in step 3 as a selected azimuthal angel ψij.
- Five values are randomly given as a tool-face angle sequence in the range of [0°, 360°].
- Pick out a value in turn from the tool-face angle sequence in step 5, as a selected tool-face angel φk. Then, the selected attitude angles (ψij, θi, φk) are determined.
- Solve the tri-axial components of the two constant vectors (G and M) in the car frame by the direction cosine matrix which has been built with the selected attitudes angle above. These tri-axial components are exactly the datasets of error-free data points.
3. Analysis and Discussion of the Calibration Approach
4. The Experimental Verification
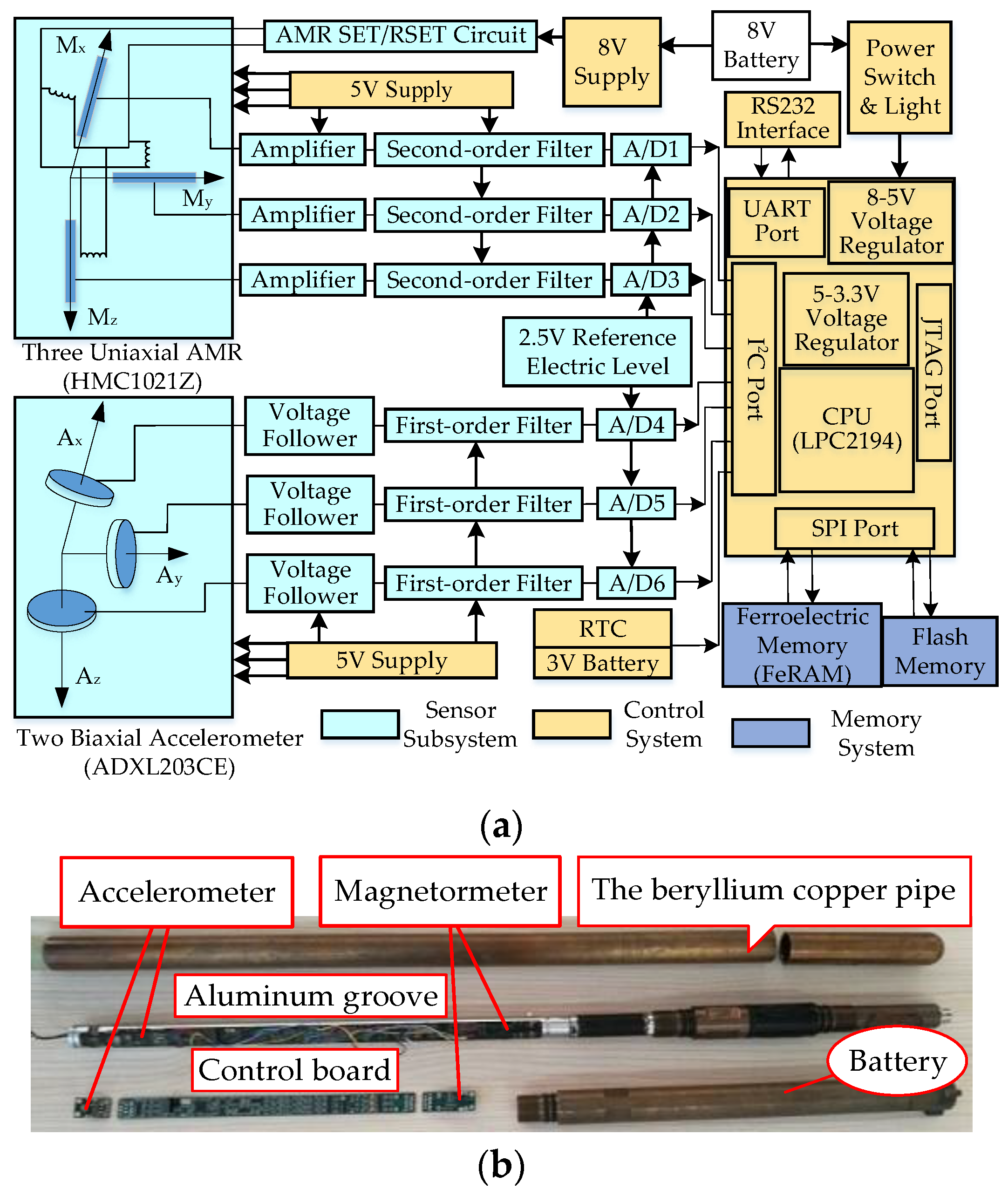
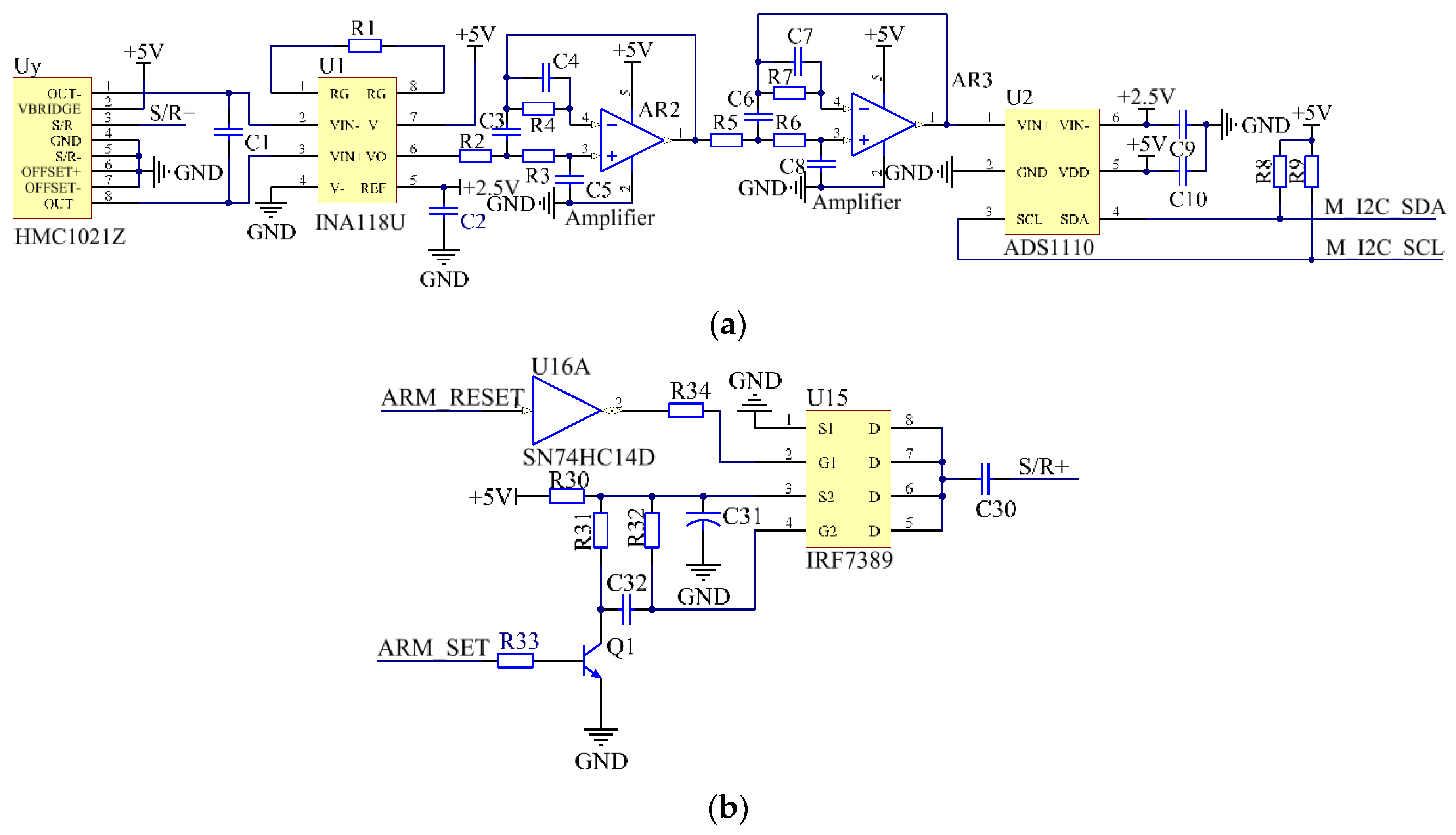
4.1. The Hardware Design for MWD Instrument
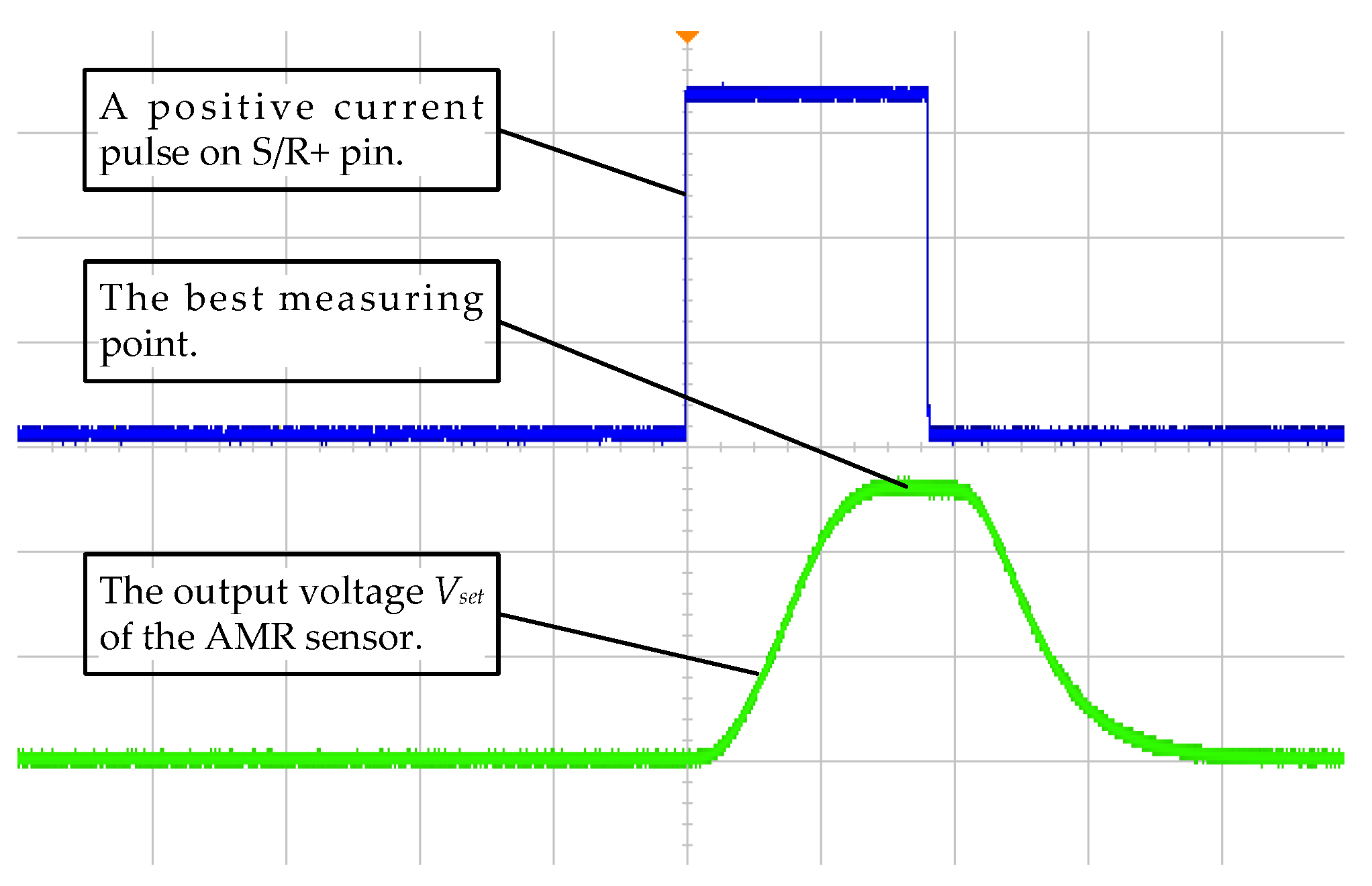
- (1)
- Apply a positive current pulse to S/R+ pin of the AMR sensors with the SET/RESET circuit, and then read the measured value Vset from the corresponding A/D converter.
- (2)
- Apply a negative current pulse and read the measured value Vreset in the same way.
- (3)
- Calculate the actual value Vout, according to the equation Vout = (Vset − Vreset)/2.
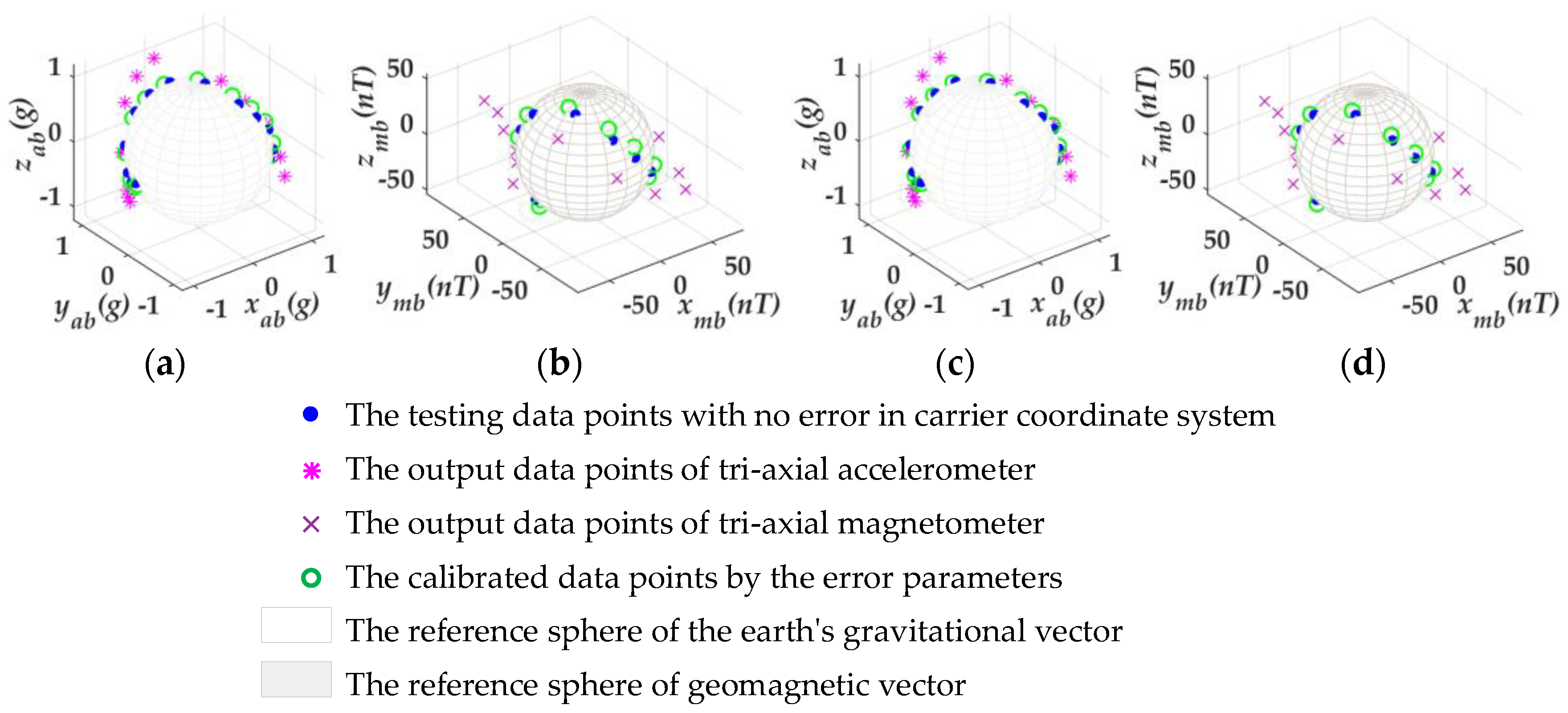
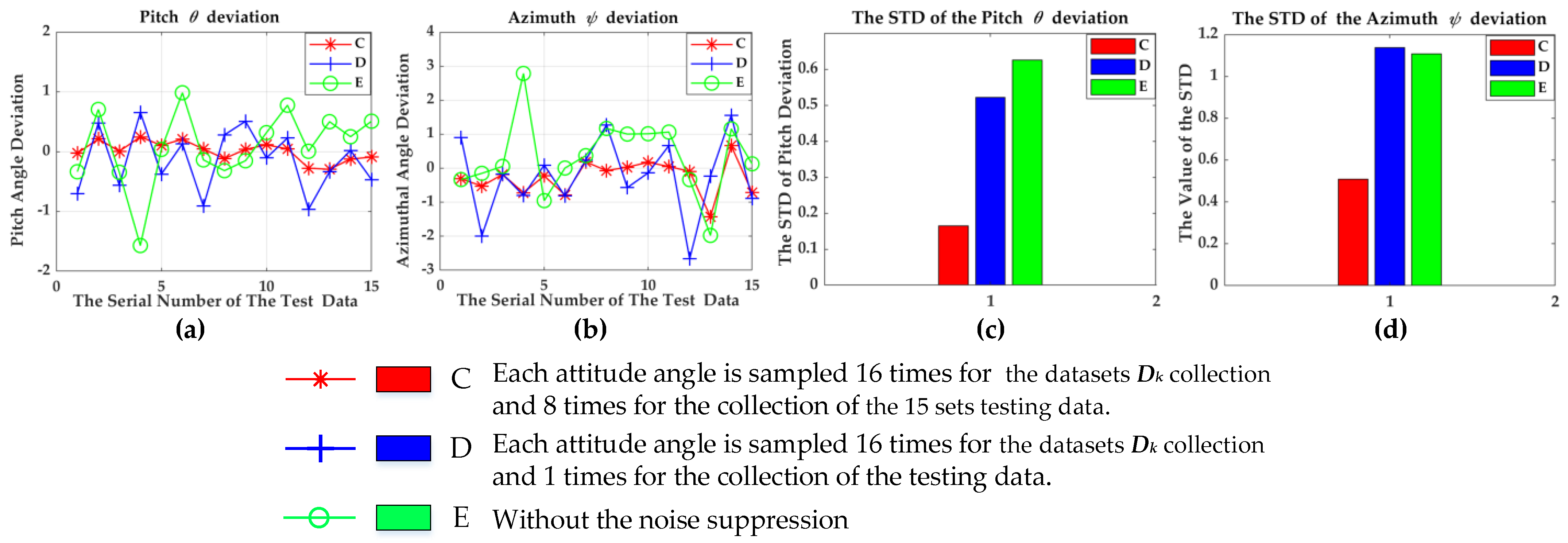
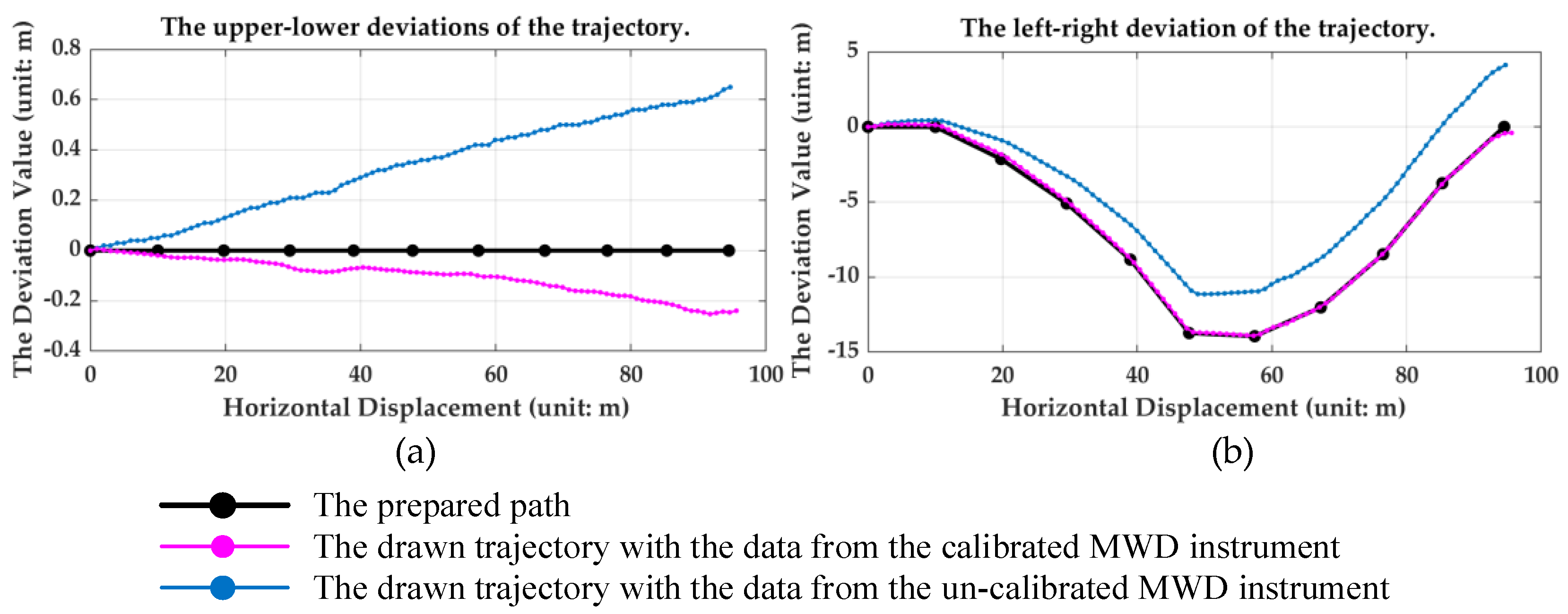
4.2. Experimental Process and Result Analysis
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Shi, Z.J.; Dong, S.N.; Yao, N.P.; Tian, D.Z. The Underground Directional Drilling Technology and Equipment for Kilometer Deep Borehole with MWD in Coalmine. Procedia Earth Planet. Sci. 2011, 3, 17–22. [Google Scholar] [CrossRef]
- Li, C. Automatic Implementation Algorithm of Pressure Relief Drilling Depth Based on an Innovative Monitoring-While-Drilling Method. Sensors 2022, 22, 3234. [Google Scholar]
- Zhang, W.; Li, C.; Jin, J.; Qu, X.; Fan, S. A new monitoring-while-drilling method of large diameter drilling in underground coal mine and their application. Measurement 2020, 173, 108840. [Google Scholar] [CrossRef]
- Rai, P.; Schunnesson, H.; Lindqvist, P.A.; Kumar, U. Measurement-while-drilling technique and its scope in design and prediction of rock blasting. Int. J. Min. Sci. Technol. 2016, 26, 697–705. [Google Scholar] [CrossRef]
- Zhang, W.; Li, C.; Ren, J.; Wu, Z. Measurement and application of vibration signals during pressure relief whole construction using micro seismic system. Measurement 2020, 158, 107696. [Google Scholar] [CrossRef]
- Rai, P.; Schunesson, H.; Lindqvist, P.-A.; Kumar, U. An Overview on Measurement-While-Drilling Technique and its Scope in Excavation Industry (Review). J. Inst. Eng. 2015, 1, 57–66. [Google Scholar]
- Zhang, Y.; Wang, S.; Fang, J. Measurement-While-Drilling Instrument Based on Predigested Inertial Measurement Unit. IEEE Trans. Instrum. Meas. 2012, 61, 3295–3302. [Google Scholar]
- Jurkov, A.S.; Mintchev, M.P. Experimental feasibility of the in drilling alignment method for inertial navigation in measurement-while-drilling. IEEE Trans. Instrum. Meas. 2005, 54, 1997–2006. [Google Scholar] [CrossRef]
- Ju, L.L.; Wang, X.F.; Ma, S.; Wei, C.M. The Algorithm Study of Sensor Compensation in MWD Instrument Based on Genetic Elman Neural Network. In Proceedings of the Third International Symposium on Intelligent Information Technology and Security Informatics, Jinggangshan, China, 2–4 April 2010. [Google Scholar]
- Du, L.; Luo, W.; Lv, H.; Xu, T. Research of measurement while drilling and azimuth correction for horizontal directional drilling. Chin. J. Sci. Instrum. 2007, 2, 236–240. [Google Scholar]
- Gong, X.; Yang, J.; Wang, W. Design of High Accuracy Orientation sensor of MWD. In Proceedings of the International Conference on Electric Information and Control Engineering (ICEICE), Wuhan, China, 15–17 April 2011. [Google Scholar]
- Yu, H.; Ye, L.; Guo, Y.; Suan, S. Effective In-Field Calibration Method for Triaxial Magnetometers based on Local Magnetic Inclination. IEEE Trans. Instrum. Meas 2020, 7, 1–9. [Google Scholar] [CrossRef]
- Frosio, I.; Pedersini, F.; Borghese, N.A. Autocalibration of MEMS Accelerometers. IEEE Trans. Instrum. Meas 2009, 6, 2034–2041. [Google Scholar] [CrossRef]
- Valérie, R.; Muhammad, H.; Gérard, L. Complete Tri-axis Magnetometer Calibration in the Magnetic Domain. J. Sens. 2010, 1, 23–59. [Google Scholar]
- Pan, D.; Li, J.; Jin, C.; Liu, T.; Lin, S.; Li, L. A New Calibration Method for Tri-axial Fluxgate Magnetometer Based on Magnetic Shielding Room. IEEE Trans. Ind. Electron 2020, 5, 67. [Google Scholar]
- Valérie, R.; Muhammad, H.; Gérard, L. New Method for Magnetometers Based Orientation Estimation. In Proceedings of the Position Location and Navigation Symposium (PLANS), Indian Wells, CA, USA, 4–6 June 2010. [Google Scholar]
- Song, Z.G.; Zhang, J.S.; Zhang, X.H.; Xi, X.L. A Calibration Method of Three-Axis Magnetometer with Noise Suppression. IEEE Trans. Magn. 2014, 11, 5011–5015. [Google Scholar]
- Long, D.; Zhang, X.; Wei, X.; Luo, Z.; Cao, J. A Fast Calibration and Compensation Method for Magnetometers in Strap-Down Spinning Projectiles. Sensors 2018, 18, 4157. [Google Scholar] [CrossRef] [PubMed]
- Yang, Z.; Li, B.; Chen, L. Calibration of tri-axis magnetometers using an improved truncated singular value decomposition method. Meas. Sci. Technol. 2018, 29, 125101. [Google Scholar]
- Liu, Y.X.; Li, X.S.; Zhang, X.J.; Feng, Y.B. Novel Calibration Algorithm for a Three-Axis Strap down Magnetometer. Sensors 2014, 14, 8485–8504. [Google Scholar] [CrossRef]
- Kiani, M.; Pourtakdoust, S.H.; Sheikhy, A.A. Consistent calibration of magnetometers for nonlinear attitude determination. Measurement 2015, 73, 180–190. [Google Scholar] [CrossRef]
- Li, T.; Zhang, J.S.; Wang, S.C.; Li, D.Y.; Lv, Z.F.; Jiang, J.J. Calibration of three-axis magnetometers with alternative iteration looping optimization method. Sens. Rev. 2018, 38, 509–516. [Google Scholar] [CrossRef]
- Wu, Y.; Shi, W. On Calibration of Three-axis Magnetometer. IEEE Sens. J. 2015, 15, 6424–6431. [Google Scholar] [CrossRef]
- Won, S.H.P.; Golnaraghi, F. A Triaxial Accelerometer Calibration Method Using a Mathematical Model. IEEE Trans. Instrum. Meas. 2010, 59, 2144–2153. [Google Scholar] [CrossRef]
- Zhang, X.; Chen, G.; Li, J.; Li, J. Calibration of tri-axial MEMS vector field measurement system. IET Sci. Meas. Technol. 2014, 8, 601–609. [Google Scholar]
- Fang, J.; Sun, H.; Cao, J.; Zhang, X.; Tao, Y. A novel calibration method of magnetic compass based on ellipsoid fitting. IEEE Trans. Instrum. Meas. 2011, 60, 2053–2061. [Google Scholar] [CrossRef]
- Hanak, F.C.; Wilson, H.; Gjertsen, M. Assessment of the Validity of MWD Survey Accuracy Following Multistation Analysis. In Proceedings of the SPE/IADC Drilling Conference & Exhibition, London, UK, 17–19 March 2015. [Google Scholar]
- Yang, W.; Fang, B.; Tang, Y.; Qian, J.; Qin, X.; Yao, W. A Robust Inclinometer System with Accurate Calibration of Tilt and Azimuth Angles. IEEE Sensors J. 2013, 13, 2313–2321. [Google Scholar] [CrossRef]
- Kok, M.; Hol, J.; Schön, T.B.; Gustafsson, F.; Luinge, H. Calibration of a magnetometer in combination with inertial sensors. In Proceedings of the 15th International Conference on Information Fusion (FUSION), Singapore, 9–12 July 2012. [Google Scholar]
- Li, W.; Du, Q.; Peng, M. A MEMS Inertial Sensor and AMR Magnetic Sensor Calibration Method. In Proceedings of the 2011 8th International Conference on Information, Communications & Signal Processing (ICICS 2011), Singapore, 13–16 December 2011. [Google Scholar]
- Li, X.; Li, Z. A new calibration method for tri-axial field sensors in strap-down navigation systems. Meas. Sci. Technol. 2012, 23, 1–6. [Google Scholar] [CrossRef]
- Salehi, S.; Mostofi, N.; Bleser, G. A practical in-field magnetometer calibration method for IMUs. In Proceedings of the IROS Workshop on Cognitive Assistive Systems: Closing the Action-Perception Loop, Vila Moura, Algarve, Portugal, 7–12 October 2012. [Google Scholar]
- Xu, T.; Luo, W.; Lu, H.; Qin, L. Design of underground sonde of a directional drilling locator system. Sens. Actuators A 2005, 119, 427–432. [Google Scholar]
- Ortega, J.M.; Rheinboldt, W.C. Iterative Solution of Nonlinear Equations in Several Variables; Academic Press: New York, NY, USA, 1970; Volume 25, pp. 347–380. [Google Scholar]
- Včelák, J.; Ripka, P.; Platil, A.; Kubík, J.; Kašpar, P. Errors of AMR compass and methods of their compensation. Sens. Actuators A 2006, 129, 53–57. [Google Scholar] [CrossRef]
- Včelák, J.; Ripka, P.; Kubik, J.; Platil, A.; Kašpar, P. AMR navigation systems and methods of their calibration. Sens. Actuators A 2005, 123, 122–128. [Google Scholar] [CrossRef]
- Liu, Z.; Song, J. A Low-Cost Calibration Strategy for Measurement-While-Drilling System. IEEE Trans. Ind. Electron. 2018, 65, 3559–3567. [Google Scholar]
- Available online: https://www.ngdc.noaa.gov/geomag/calculators/magcalc.shtml#igrfwmm (accessed on 20 July 2022).
- Hauser, H.; Fulmek, P.L.; Haumer, P.; Vopalensky, M.; Ripka, P. Flipping field and stability in anisotropic magneto resistive sensors. Sens. Actuators A Phys. 2003, 15, 121–125. [Google Scholar] [CrossRef]
- AN213 SET/RESET Function for Magnetic Sensors. Honeywell Application Note. Available online: https://aerospace.honeywell.com.cn/content/dam/aerobt/en/documents/learn/products/sensors/application-notes/AN213_Set_Reset_Function_of_Magnetic_Sensors.pdf (accessed on 20 July 2022).



| Sensor Type | Parameters Type | Value |
|---|---|---|
| Accelerometer | Error matrix | |
| Calibration matrix = ()−1 | ||
| Bias ba | ||
| Magnetometer | Error matrix | |
| Calibration matrix = ()−1 | ||
| Bias bm |
| Condition | The Error Matrixes and Biases | |||
|---|---|---|---|---|
| Pitch θ = −45° | ba | bm | ||
| γa = 0° | ||||
| γa = 5° | ||||
| Given Attitude (Unit: °) | The Raw Data Output by the Two Sensor Triplet. (Unit: °) | Calculating Attitude with the Error Parameters in Table 2 (Unit: °) | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| (γa = 0°) | (γa = 5°) | |||||||||||||
| ψ | θ | φ | xm | ym | zm | xa | ya | za | ψ | θ | φ | ψ | θ | φ |
| 30 | −80 | −150 | 82.933 | 41.3127 | 13.4254 | 1.262 | −0.1243 | −0.442 | 30 | −80 | −140.575 | 30 | −80 | −145.6115 |
| 75 | −54 | −90 | 61.0612 | −40.3344 | −21.4997 | 0.9285 | −0.5982 | −0.1536 | 75 | −54 | −80.5751 | 75 | −54 | −85.6115 |
| 240 | 18 | 45 | −27.6695 | 93.0142 | 13.2316 | −0.2186 | 1.0809 | 0.7251 | 240 | 18 | 54.4249 | 240 | 18 | 49.3885 |
| 330 | 54 | 120 | −16.8204 | 66.5614 | −23.4914 | −0.703 | 0.6639 | −0.6725 | 330 | 54 | 129.4249 | 330 | 54 | 124.3885 |
| 350 | 75 | 150 | −45.071 | 33.2847 | −27.7686 | −0.9773 | 0.2939 | −0.5187 | 350 | 75 | 159.4249 | 350 | 75 | 154.3885 |
| Condition | The Error Matrixes and Biases on the Condition of γa = 0° | |||
|---|---|---|---|---|
| Random Noise | ba | bm | ||
| Without suppression | ||||
| With suppression | ||||
| Given Attitude (Unit: °) | The Mean of the Output by the Two Sensor Triplet. (Unit: °) | Calculating Attitude with the Calibration Parameters in Table 4 (Unit: °) | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Without Suppression | With Suppression | |||||||||||||
| ψ | θ | φ | xm | ym | zm | xa | ya | za | ψ | θ | φ | ψ | θ | φ |
| 30 | −80 | −150 | 82.9511 | 41.3042 | 13.4293 | 1.2669 | −0.126 | −0.4423 | 29.667 | −80.336 | −138.882 | 29.689 | −80.028 | −140.286 |
| 75 | −54 | −90 | 61.0363 | −40.3228 | −21.4836 | 0.9248 | −0.6022 | −0.1461 | 77.786 | −55.57 | −80.506 | 74.279 | −53.753 | −80.107 |
| 240 | 18 | 45 | −27.6766 | 93.0266 | 13.2195 | −0.2212 | 1.0808 | 0.7277 | 241.013 | 18.317 | 54.259 | 240.175 | 18.114 | 54.33 |
| 330 | 54 | 120 | −16.8173 | 66.5538 | −23.4891 | −0.6962 | 0.67 | −0.6636 | 328.024 | 54.501 | 128.13 | 328.569 | 53.705 | 128.726 |
| 350 | 75 | 150 | −45.0679 | 33.2827 | −27.7877 | −0.9718 | 0.295 | −0.5164 | 350.129 | 75.509 | 159.499 | 349.289 | 74.909 | 159.004 |
| Resolved by the Calibration Approach | ||
|---|---|---|
| Sensor Type | The Calibration Matrix | Bias bk |
| Accelerometer ( and ba) | ||
| Magnetometer ( and bm) | ||
| Known Attitude Angle (Unit: °) | The Raw Reading from Sensors | Solved from Raw Reading (Unit: °) | After Calibration (Unit: °) | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Azimuth | Pitch | Magnetometer | Accelerometer | Azimuth | Pitch | Azimuth | Pitch | ||||
| ψ | θ | ψ | θ | ψ | θ | ||||||
| 1.5 | 0 | 26.98 | 6.055 | 40.3675 | −0.0311 | 0.091 | 0.9961 | 355.2305 | 1.7788 | 1.7260 | −0.1345 |
| 60 | 0 | 14.0425 | −16.935 | 43.0425 | −0.0316 | 0.0928 | 0.9956 | 53.6498 | 1.8115 | 60.2163 | −0.1011 |
| 120 | 0 | −12.285 | −17.525 | 43.6325 | −0.0319 | 0.0944 | 0.9957 | 116.9278 | 1.8256 | 119.9135 | −0.0863 |
| 178 | 0 | −25.735 | 5.2 | 41.55 | −0.03119 | 0.0937 | 0.9954 | 183.0086 | 1.7866 | 177.5677 | −0.1256 |
| 302 | 0 | 13.8625 | 28.645 | 38.37 | −0.0308 | 0.0926 | 0.9955 | 301.1897 | 1.7617 | 302.1324 | −0.1510 |
| The maximum error (Max{|AttitudeOutput − AttitudeKnown|}) | 6.35 | 1.8256 | 0.4323 | 0.1510 | |||||||
| The mean of attitude deviation | −2.2987 | 1.7928 | 0.0112 | −0.1197 | |||||||
| The Standard deviation of attitude deviation | 4.7009 | 0.0257 | 0.2780 | 0.0259 | |||||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Z.; Lei, K.; Song, J.; Li, L.; Li, T. A Designed Calibration Approach for the Measurement-While-Drilling Instrument. Appl. Sci. 2023, 13, 61. https://doi.org/10.3390/app13010061
Liu Z, Lei K, Song J, Li L, Li T. A Designed Calibration Approach for the Measurement-While-Drilling Instrument. Applied Sciences. 2023; 13(1):61. https://doi.org/10.3390/app13010061
Chicago/Turabian StyleLiu, Zongwei, Kan Lei, Jiancheng Song, Linbo Li, and Tao Li. 2023. "A Designed Calibration Approach for the Measurement-While-Drilling Instrument" Applied Sciences 13, no. 1: 61. https://doi.org/10.3390/app13010061
APA StyleLiu, Z., Lei, K., Song, J., Li, L., & Li, T. (2023). A Designed Calibration Approach for the Measurement-While-Drilling Instrument. Applied Sciences, 13(1), 61. https://doi.org/10.3390/app13010061






