Numerical Analysis of New Stainless-Steel Corrugated-Plate Reinforcement of Shield-Tunnel Segmental Joints Based on Virtual-Tracking-Element Technology
Abstract
:1. Introduction
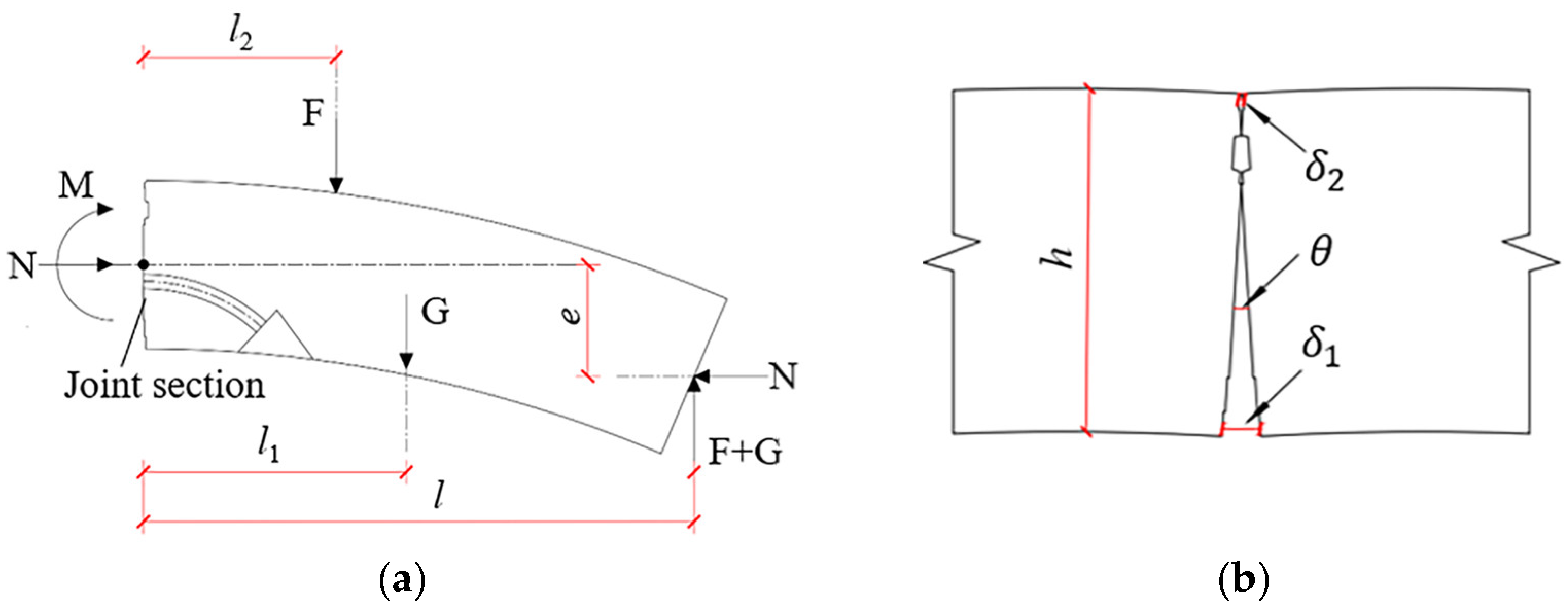
2. Data from the Full-Scale Test
3. Numerical Modeling
3.1. Geometry and Element Mesh
3.2. Constitutive Models of Materials
3.3. Contact Relationships
3.4. Load and Boundary Conditions
4. Secondary-Stress Simulation
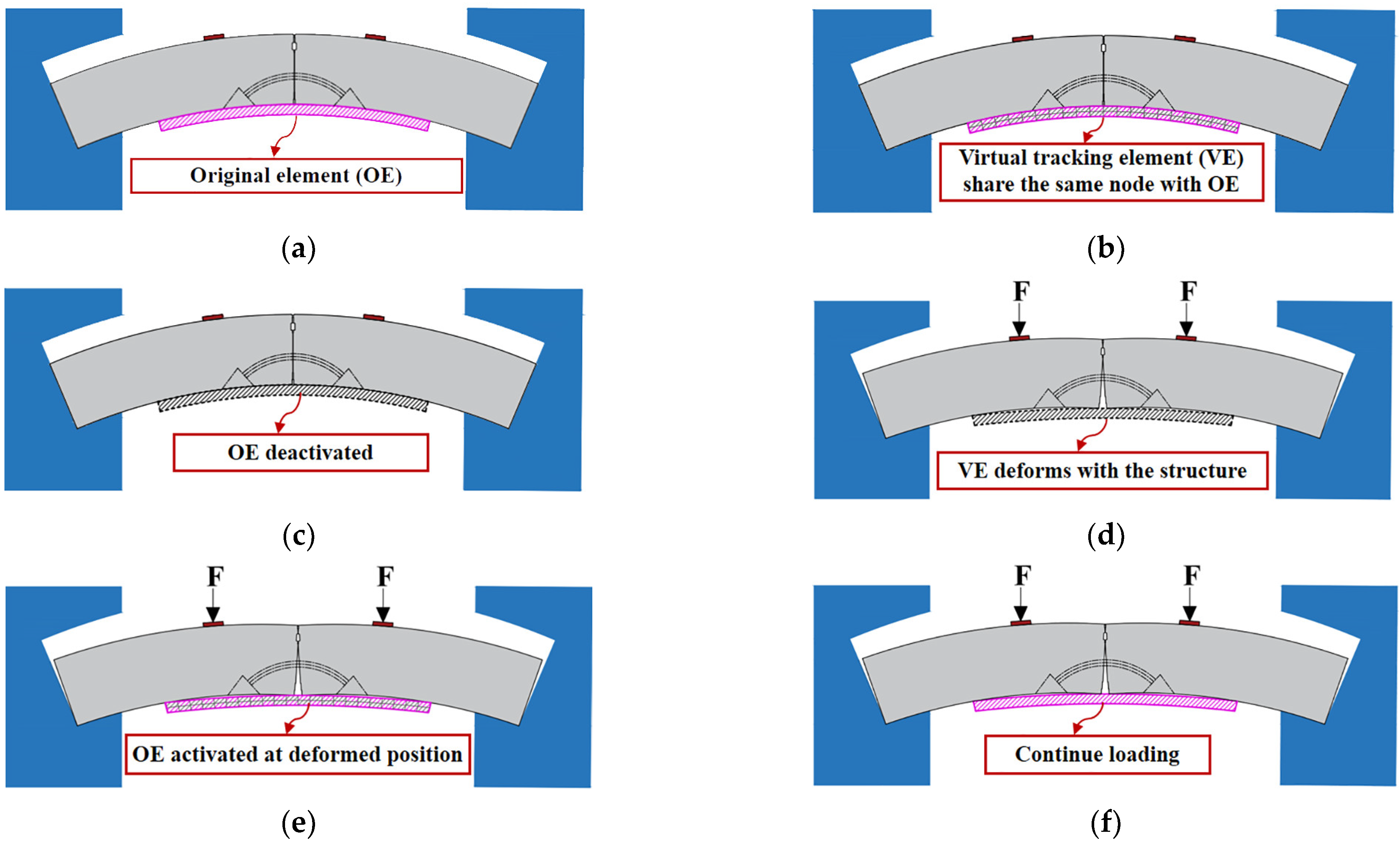
4.1. Principle of Virtual-Tracking-Element Technology
- i.
- The VE should have the same shape and contact relationship as OE and share all nodes, but have a different element number;
- ii.
- The stiffness of VE should be extremely low, so that its influence on the stress of the original structure can be ignored;
- iii.
- The mass of VE should be especially small to prevent potential displacement caused by the weight.
4.2. Simulation Methodology
- i.
- ii.
- iii.
5. Results and Analysis
5.1. Model Validation
5.2. Component Analysis
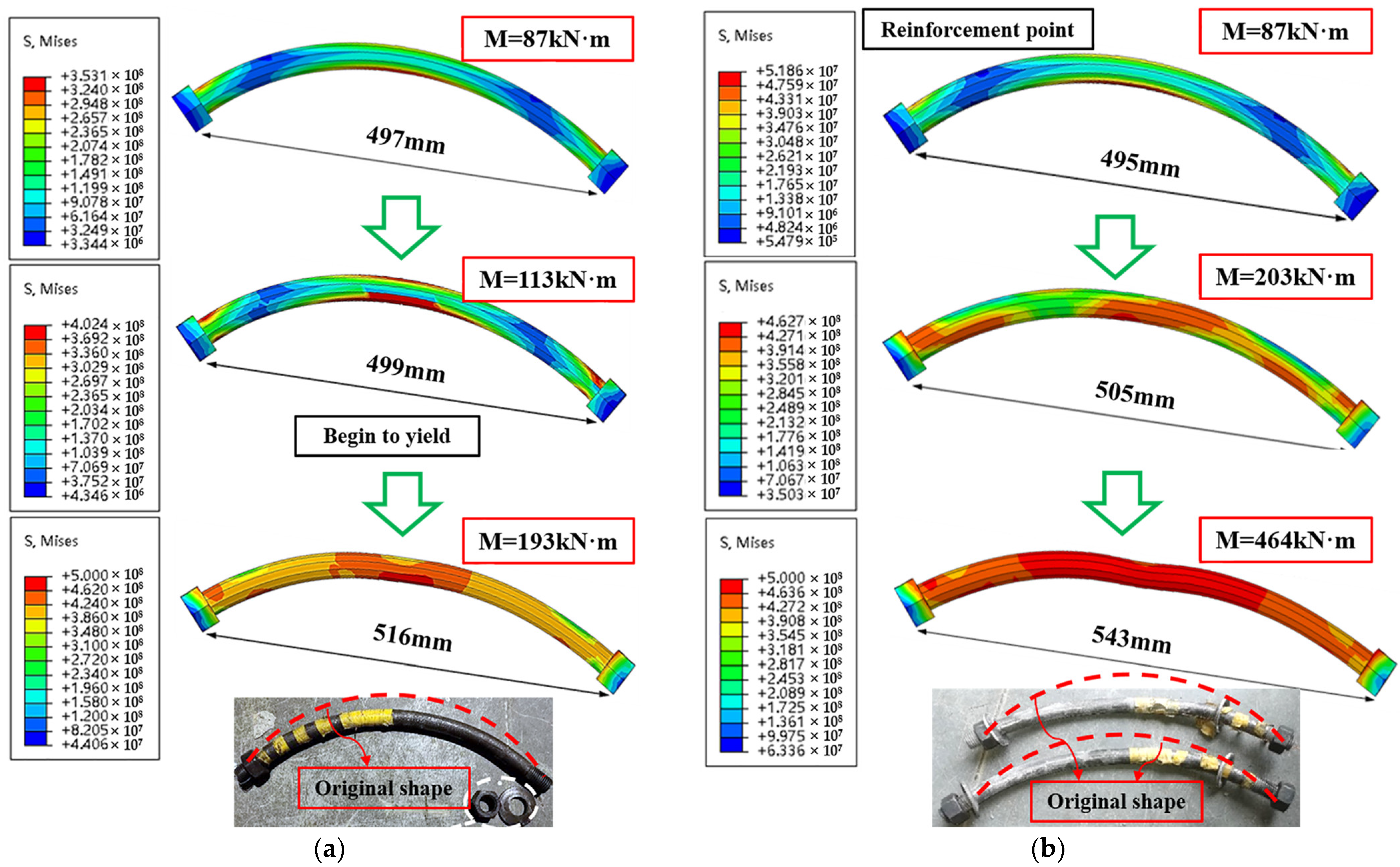
5.2.1. Bent Bolt
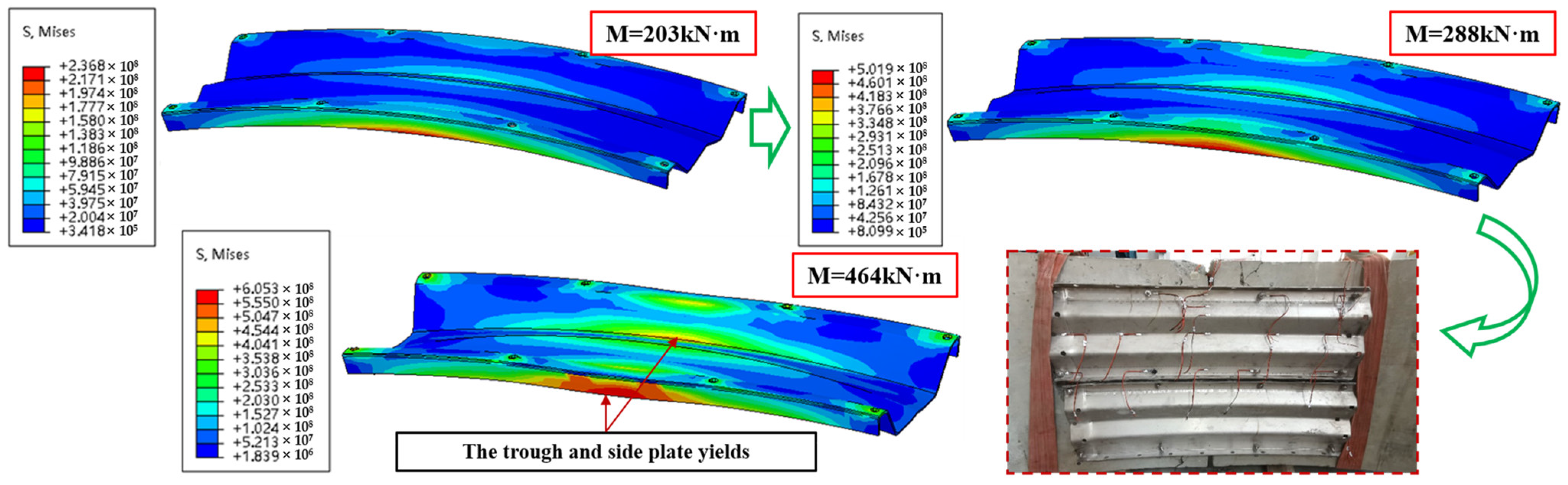
5.2.2. Stainless-Steel Corrugated Plate (SSCP)
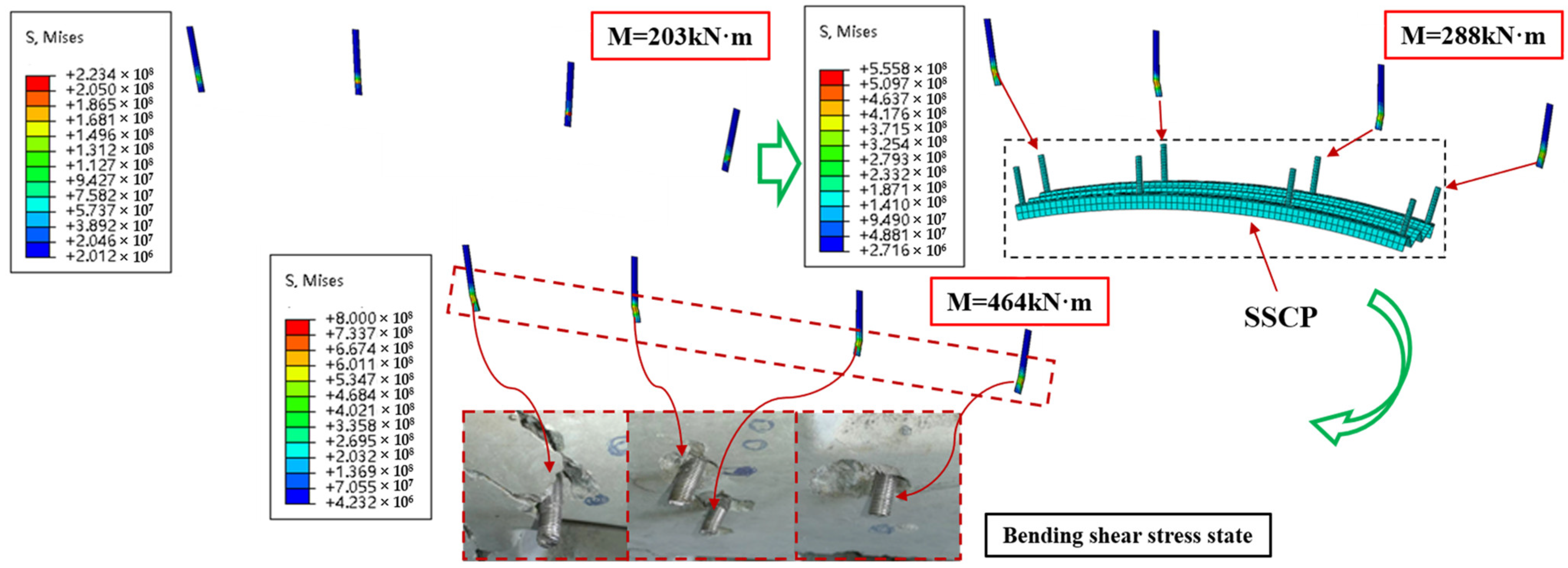
5.2.3. Chemical Anchor
5.3. Parametric Study
5.3.1. Axial Force
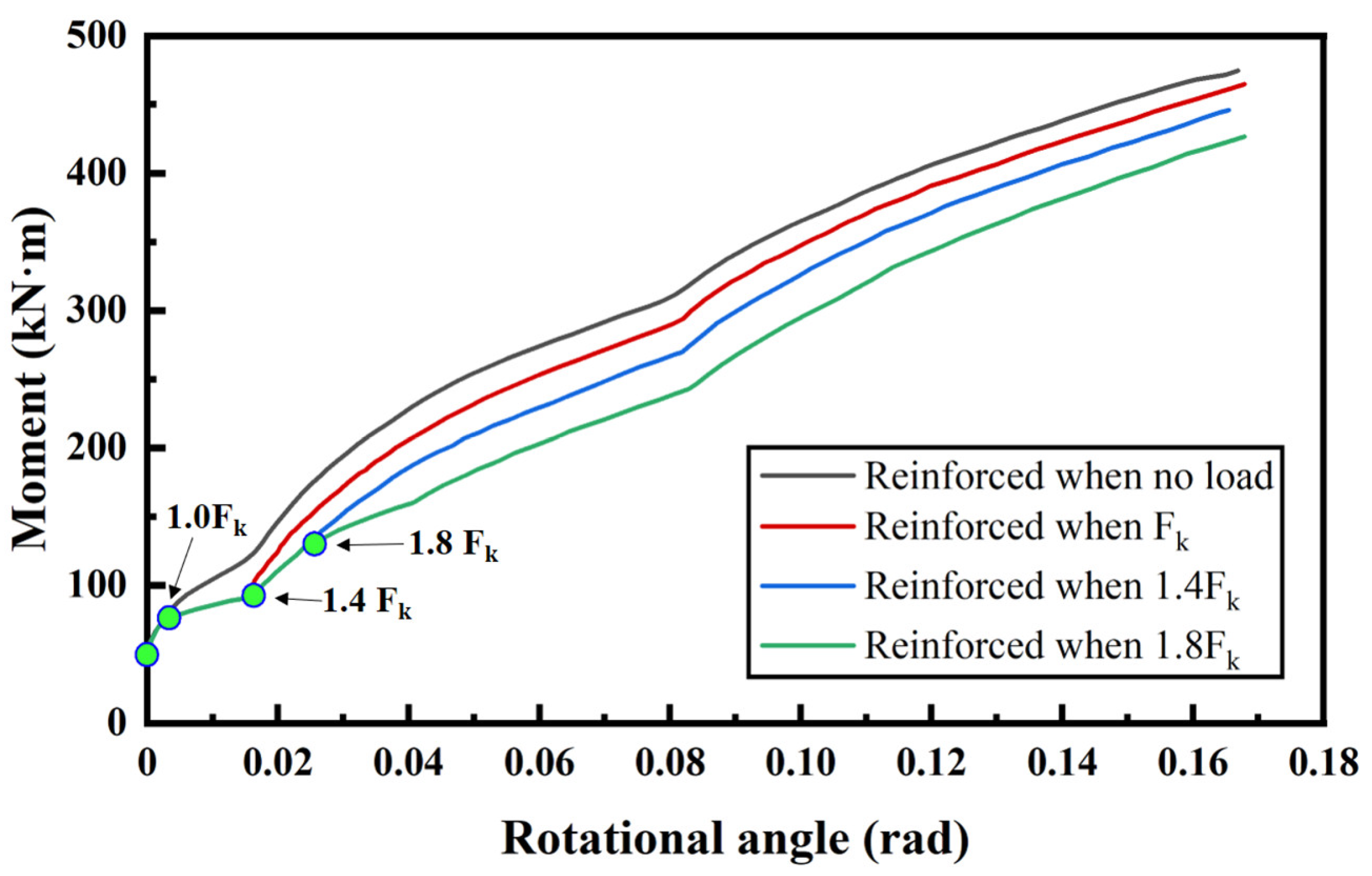
5.3.2. Reinforcement Timing
6. Conclusions
- The use of the virtual-tracking-element technology is feasible for simulating the secondary-stress state of segmental joint reinforcement. The virtual tracking element is similar to a backup of the original element, and it has no influence on the stress or the strain as the structure deforms. By removing and activating the corresponding elements in different steps, the current deformation and secondary-stress characteristics of the segmental joint can be taken into account.
- The reinforcement capacity of SSCP is not fully utilized when segmental concrete is of the C50 grade. Hence, the utilization of SSCP is greater when reinforcing segmental joint of high-grade concrete. On the other hand, the relatively low level of utilization indicates that SSCP reinforcement has a sufficient safety margin for potential excessive loads.
- The SSCP can sustain its reinforcement capability under different axial forces. Thus, SSCP is applicable to tunnel reinforcement regardless of the burial depth. In addition, reinforcement in advance is recommended, since the increase in stiffness is more obvious when the tunnel is in the elastic or elastoplastic stage.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ding, W.; Chen, X.; Jin, Y.; Qiao, Y. Flexural behavior of segmental joint containing double rows of bolts: Experiment and simulation. Tunn. Undergr. Space Technol. 2021, 112, 103940. [Google Scholar] [CrossRef]
- Jin, H.; Yuan, D.; Zhou, S.; Zhao, D. Short-Term and Long-Term Displacement of Surface and Shield Tunnel in Soft Soil: Field Observations and Numerical Modeling. Appl. Sci. 2022, 12, 3564. [Google Scholar] [CrossRef]
- Lou, P.; Li, Y.; Xiao, H.; Zhang, Z.; Lu, S. Influence of small radius curve shield tunneling on settlement of ground surface and mechanical properties of surrounding rock and segment. Appl. Sci. 2022, 12, 9119. [Google Scholar] [CrossRef]
- Zhang, J.-L.; Liu, X.; Ren, T.-Y.; Yuan, Y.; Mang, H.A. Structural behavior of reinforced concrete segments of tunnel linings strengthened by a steel-concrete composite. Compos. Part B 2019, 178, 107444. [Google Scholar] [CrossRef]
- Huang, G.; Gong, L.; Sun, X.; Liang, Z.-N.; Wang, X.-Y. Numerical investigation on flexural performance of retrofitted tunnel lining with short bolts and steel-plate. Tunn. Undergr. Space Technol. 2020, 95, 103152. [Google Scholar] [CrossRef]
- Liu, X.; Jiang, Z.; Yuan, Y.; Mang, H.A. Numerical investigation of the mechanical behavior of deformed segmental tunnel linings, strengthened by epoxy-bonded filament wound profiles. Tunn. Undergr. Space Technol. 2018, 78, 231–244. [Google Scholar] [CrossRef]
- Xu, S.; Mu, F.; Wang, J.; Li, W. Experimental study on the interfacial bonding behaviors between sprayed uhtcc and concrete substrate. Constr. Build. Mater. 2019, 195, 638–649. [Google Scholar] [CrossRef]
- Li, S.; Ding, W.; Zhang, Q.; Xiao, X.; Zhou, Q. Experimental study of the mechanical properties of a new duplex stainless steel exposed to elevated temperatures. Case Stud. Constr. Mater. 2022, 17, e01683. [Google Scholar] [CrossRef]
- Simpson, B.; Moore, I.D.; Hoult, N.A. Experimental investigation of rehabilitated steel culvert performance under static surface loading. J. Geotech. Geoenviron. Eng. 2016, 142, 04015076. [Google Scholar] [CrossRef]
- Vaslestad, J.; Madaj, A.; Janusz, L. Field measurements of long-span corrugated steel culvert replacing corroded concrete bridge. Transp. Res. Rec. 2002, 1814, 164–170. [Google Scholar] [CrossRef]
- Che, H.; Tong, L.; Liu, S.; Yang, Q. Field investigation on the mechanical performance of corrugated steel utility tunnel (csut). J. Constr. Steel Res. 2021, 183, 106693. [Google Scholar] [CrossRef]
- Kang, J.S.; Davidson, J.S. Structural effects of concrete lining for concrete-lined corrugated steel pipes. Struct. Infrastruct. Eng. 2010, 9, 130–140. [Google Scholar] [CrossRef]
- Xu, P.; Wei, Y.; Yang, Y.; Zhou, X. Application of fabricated corrugated steel plate in subway tunnel supporting structure. Case Stud. Constr. Mater. 2022, 17, e01323. [Google Scholar] [CrossRef]
- Ren, T.; Liu, S.; Liu, X. Experimental study of bending capacity of shield tunnel lining segment strengthened by corrugated steel. Tunn. Constr. 2019, 2, 317–323. [Google Scholar]
- Luo, Y. Experiment and Analysis of Shield Tunnel Strengthened with Concrete Filled Steel Tubes; South China University of Technology: Guangzhou, China, 2019. [Google Scholar]
- Zhao, H.; Liu, X.; Bao, Y.; Yuan, Y.; Bai, Y. Simplified nonlinear simulation of shield tunnel lining reinforced by epoxy bonded steel plates. Tunn. Undergr. Space Technol. 2016, 51, 362–371. [Google Scholar] [CrossRef]
- Jiang, Y.; Wang, X.; Li, B.; Higashi, Y.; Taniguchi, K.; Ishida, K. Estimation of reinforcing effects of FRP-PCM method on degraded tunnel linings. Soils Found. 2017, 57, 327–340. [Google Scholar] [CrossRef]
- Wang, Y. Study on Parameters of Plate-Short Anchor Assembly Structure of Repairing Cracking Lining; Southwest Jiaotong University: Chengdu, China, 2019. [Google Scholar]
- Saenz, L. Discussion of “equation for the stress-strain curve of concrete” by Desayi and Krishnan. J. Am. Concr. Inst. 1964, 61, 1229–1235. [Google Scholar]
- Zhang, H.; Zhang, Z.; Wang, J. 3-D FEM analysis on prefabricated segment joints of shield tunnel. J. Shanghai Jiaotong Univ. Sci. 2003, 4, 566–569. [Google Scholar] [CrossRef]
- Lin, P. Study on the Deformation Mechanism of Metro Shield Tunnel Lining Strengthened by Steel Plates with Steel Corbel; South China University of Technology: Guangzhou, China, 2018. [Google Scholar]
- GB 50010—2010; Code for Design of Concrete Structures. China Building Industry Press: Beijing, China, 2010.
- GB 50017—2017; Code for Design of Steel Structures. China Building Industry Press: Beijing, China, 2017.
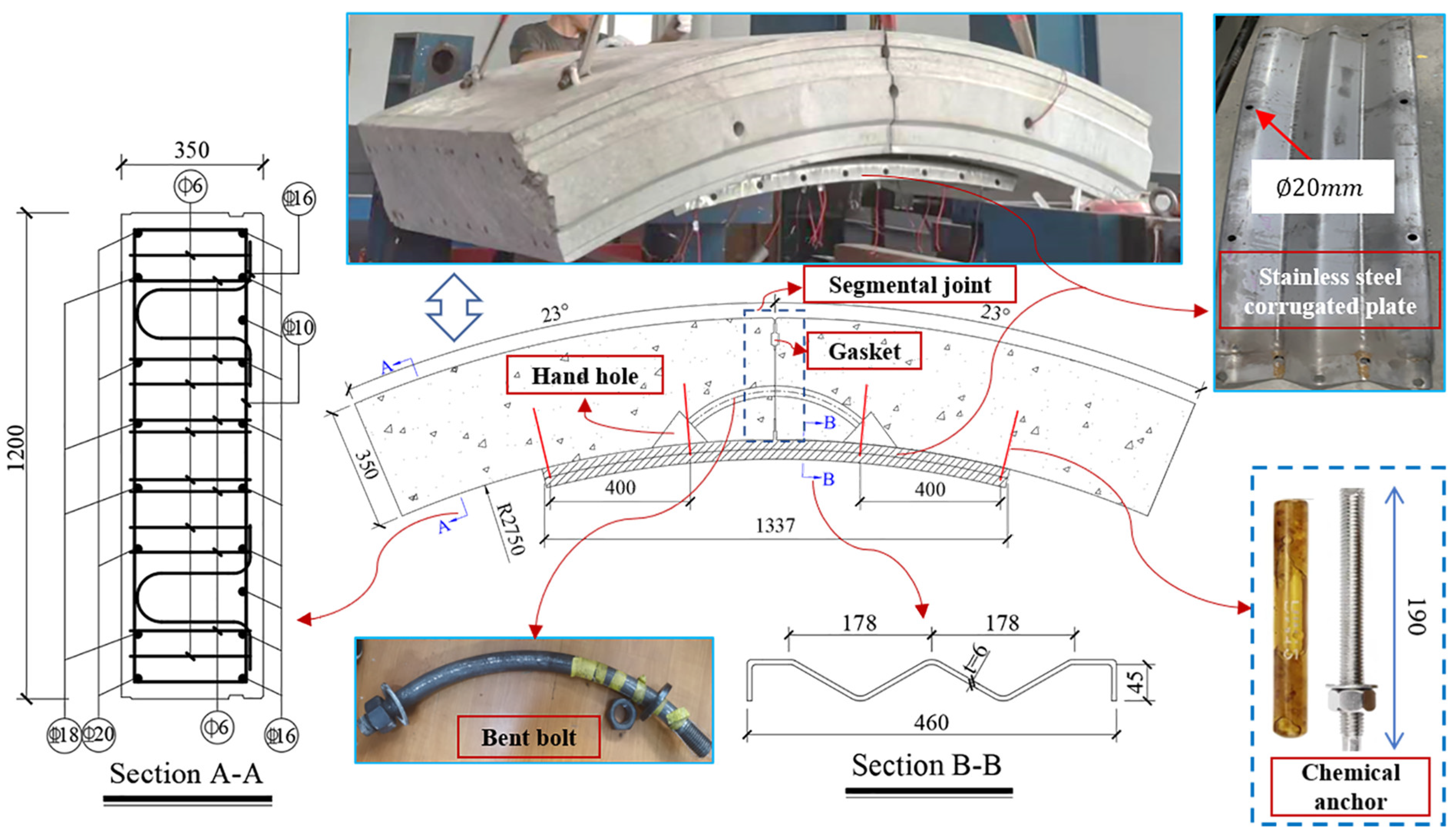

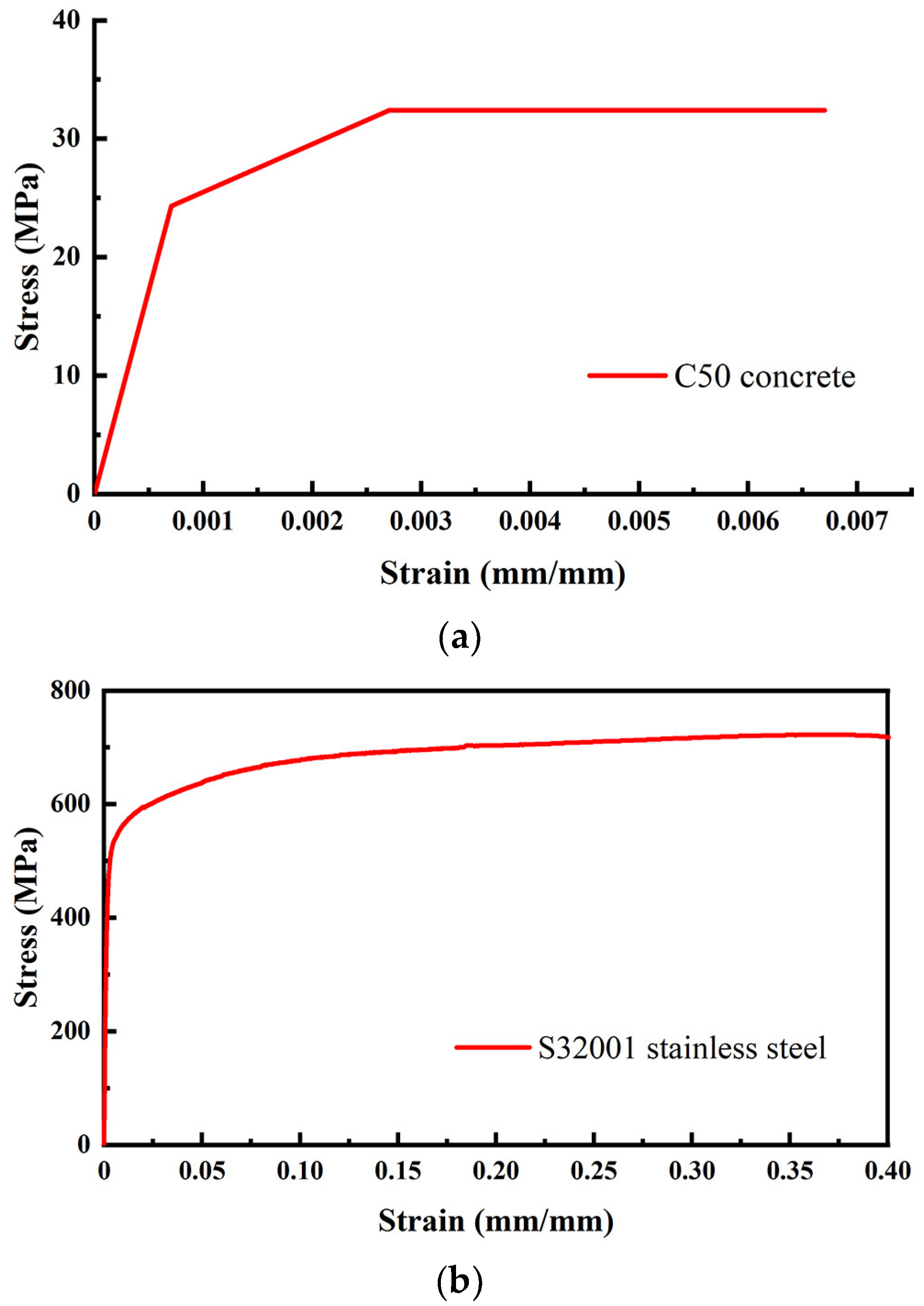

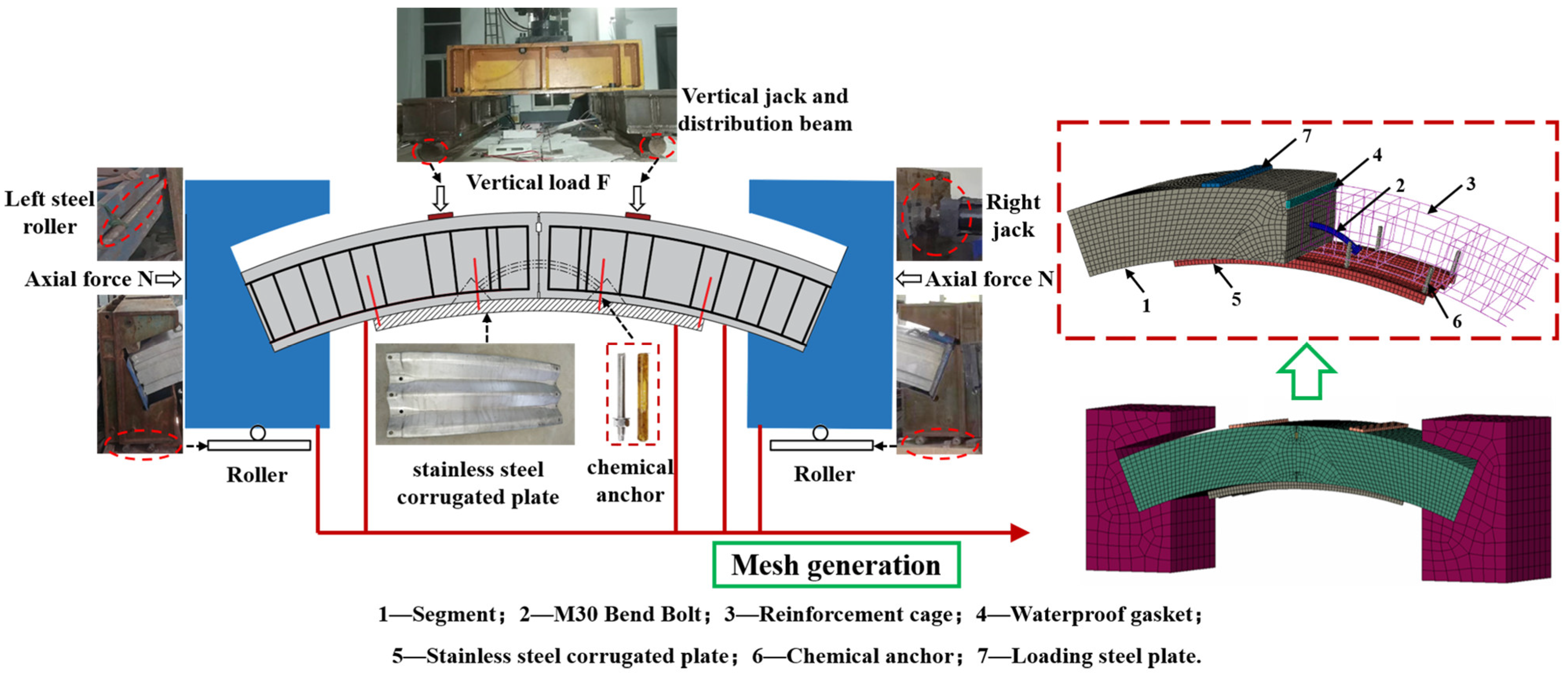



| Component | Material | E (GPa) | fy (MPa) | εy | fu (MPa) | ν |
|---|---|---|---|---|---|---|
| Concrete | C50 | 34.5 | 24.3 | 0.0007 | 32.4 | 0.2 |
| SSCP | S32001 stainless steel | 206 | 490 | 0.0024 | 720 | 0.3 |
| Bent bolt | carbon steel (5.8-grade) | 206 | 400 | 0.0019 | 500 | 0.3 |
| Chemical anchor | 2205 stainless steel (8.8-grade) | 206 | 640 | 0.0031 | 800 | 0.3 |
| Reinforcement cage | HRB400 steel | 206 | 400 | 0.0019 | 540 | 0.3 |
| Backing plate | Q235 steel | 206 | - | - | - | 0.3 |
| Support | Q235 steel | 206 | - | - | - | 0.3 |
| Number | Contact Pairs | Type |
|---|---|---|
| 1 | Segment and segment | Hard contact |
| 2 | Segment and reinforcement cage | Embedded region |
| 3 | Segment bent-bolt hole wall and bent bolts | Hard contact |
| 4 | Segment and backing plate | Tie |
| 5 | Segment and support | Tie |
| 6 | Segment and gasket | Tie |
| 7 | Segment-hand-hole face and bent-bolt nut | Tie |
| 8 | Segment and chemical anchor | Embedded region |
| 9 | Segment and SSCP | Hard contact |
| 10 | SSCP and chemical anchor | Tie |
| 11 | Gasket and gasket | Hard contact |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ding, W.; Ma, C.; Guo, Y.; Li, X.; Li, S. Numerical Analysis of New Stainless-Steel Corrugated-Plate Reinforcement of Shield-Tunnel Segmental Joints Based on Virtual-Tracking-Element Technology. Appl. Sci. 2023, 13, 5904. https://doi.org/10.3390/app13105904
Ding W, Ma C, Guo Y, Li X, Li S. Numerical Analysis of New Stainless-Steel Corrugated-Plate Reinforcement of Shield-Tunnel Segmental Joints Based on Virtual-Tracking-Element Technology. Applied Sciences. 2023; 13(10):5904. https://doi.org/10.3390/app13105904
Chicago/Turabian StyleDing, Wenqi, Chang Ma, Yingjie Guo, Xiaoran Li, and Shuobiao Li. 2023. "Numerical Analysis of New Stainless-Steel Corrugated-Plate Reinforcement of Shield-Tunnel Segmental Joints Based on Virtual-Tracking-Element Technology" Applied Sciences 13, no. 10: 5904. https://doi.org/10.3390/app13105904
APA StyleDing, W., Ma, C., Guo, Y., Li, X., & Li, S. (2023). Numerical Analysis of New Stainless-Steel Corrugated-Plate Reinforcement of Shield-Tunnel Segmental Joints Based on Virtual-Tracking-Element Technology. Applied Sciences, 13(10), 5904. https://doi.org/10.3390/app13105904







